Measurement of Stark Halfwidths of Spectral Lines of Ionized Oxygen and Silicon Emitted from T-tube Plasma
Abstract
1. Introduction
2. Details of the Experiment
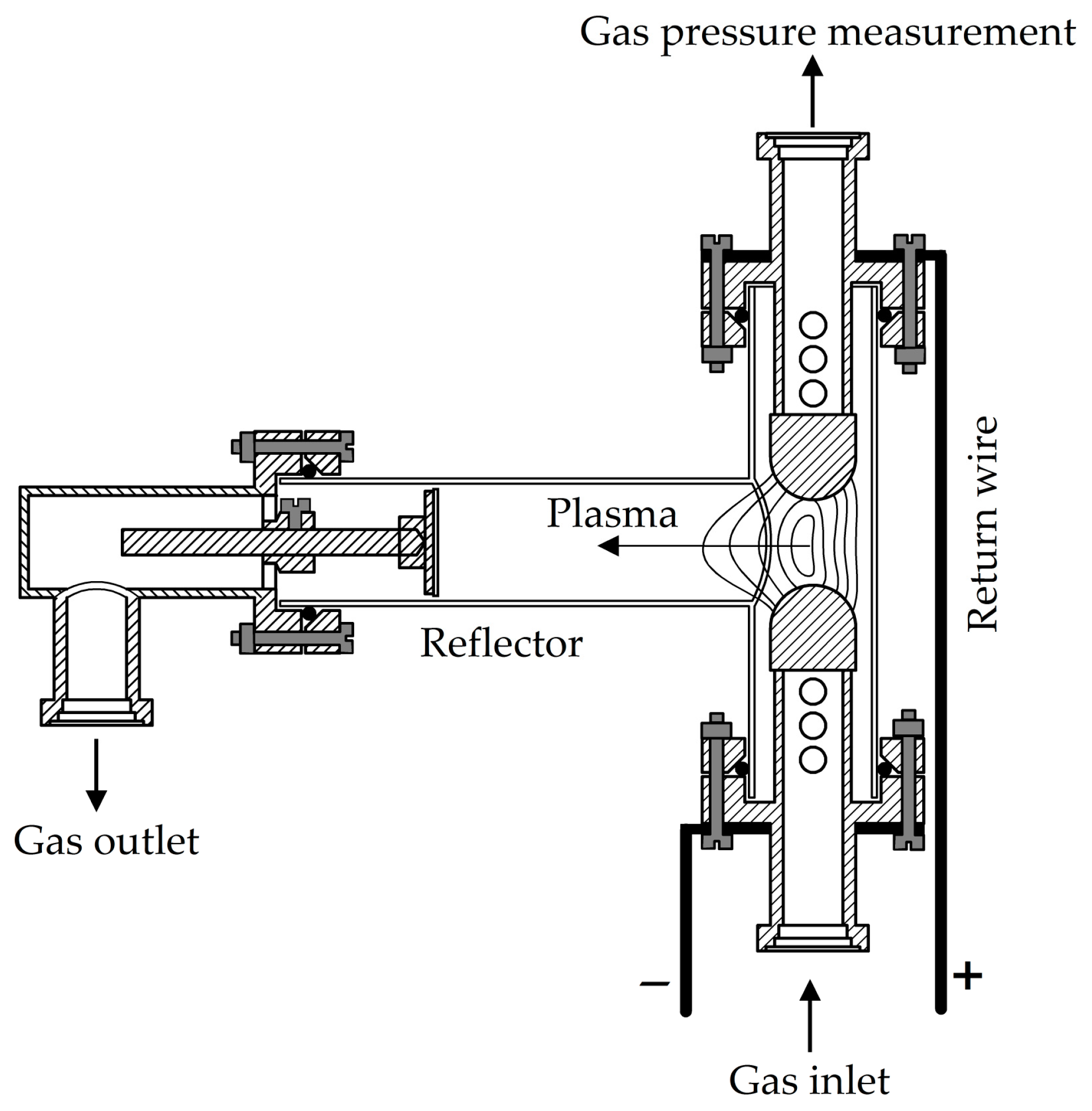
2.1. Plasma Source
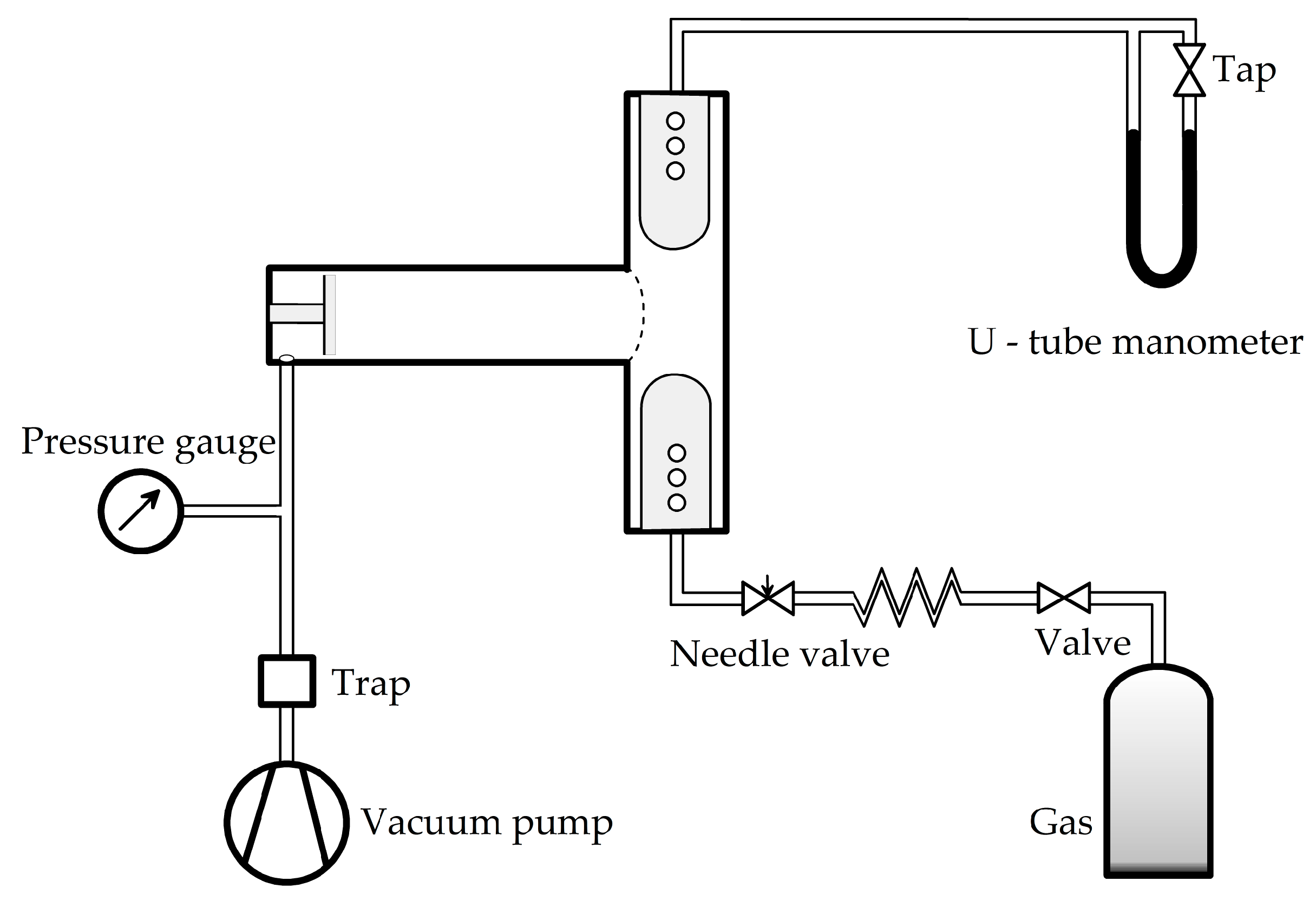
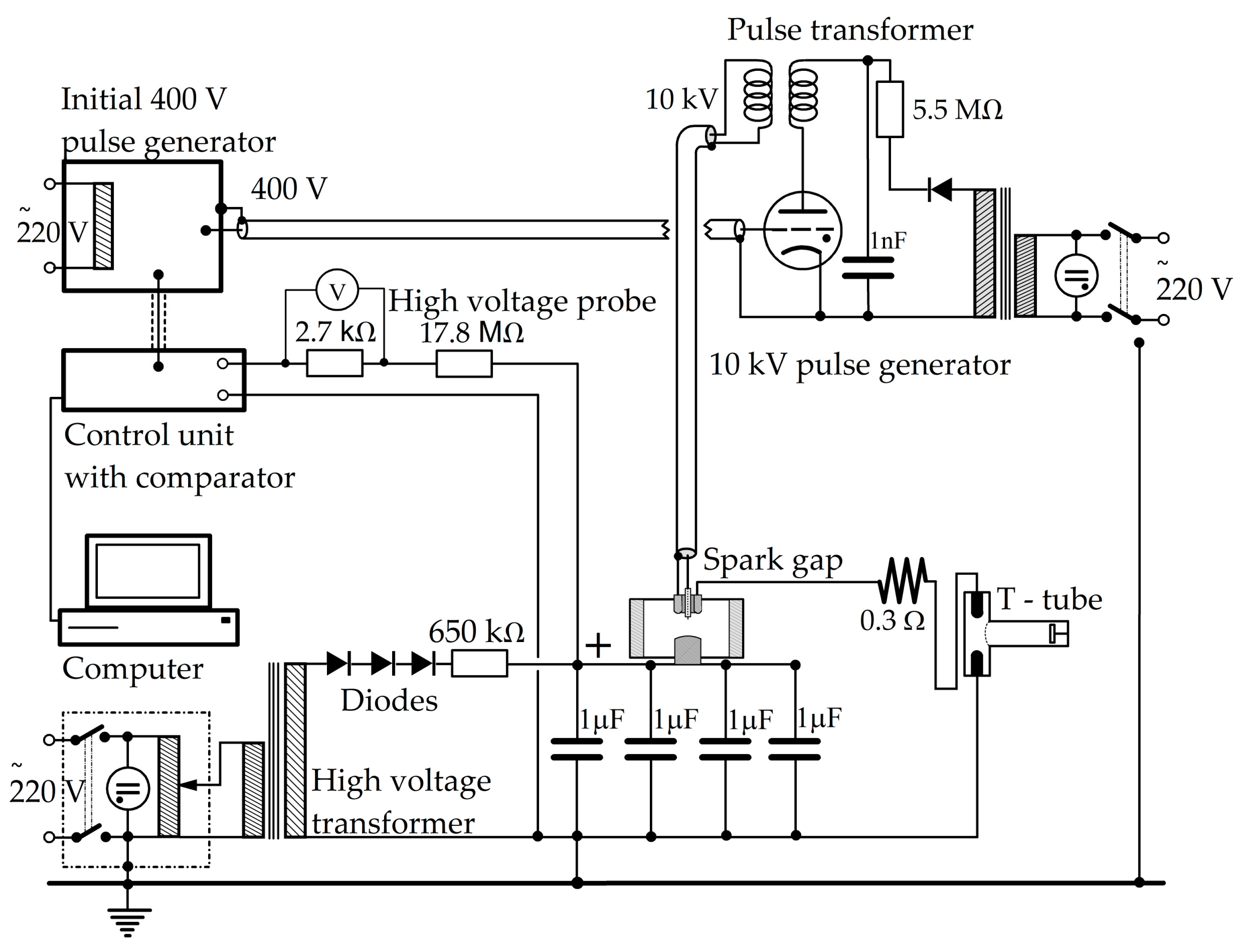
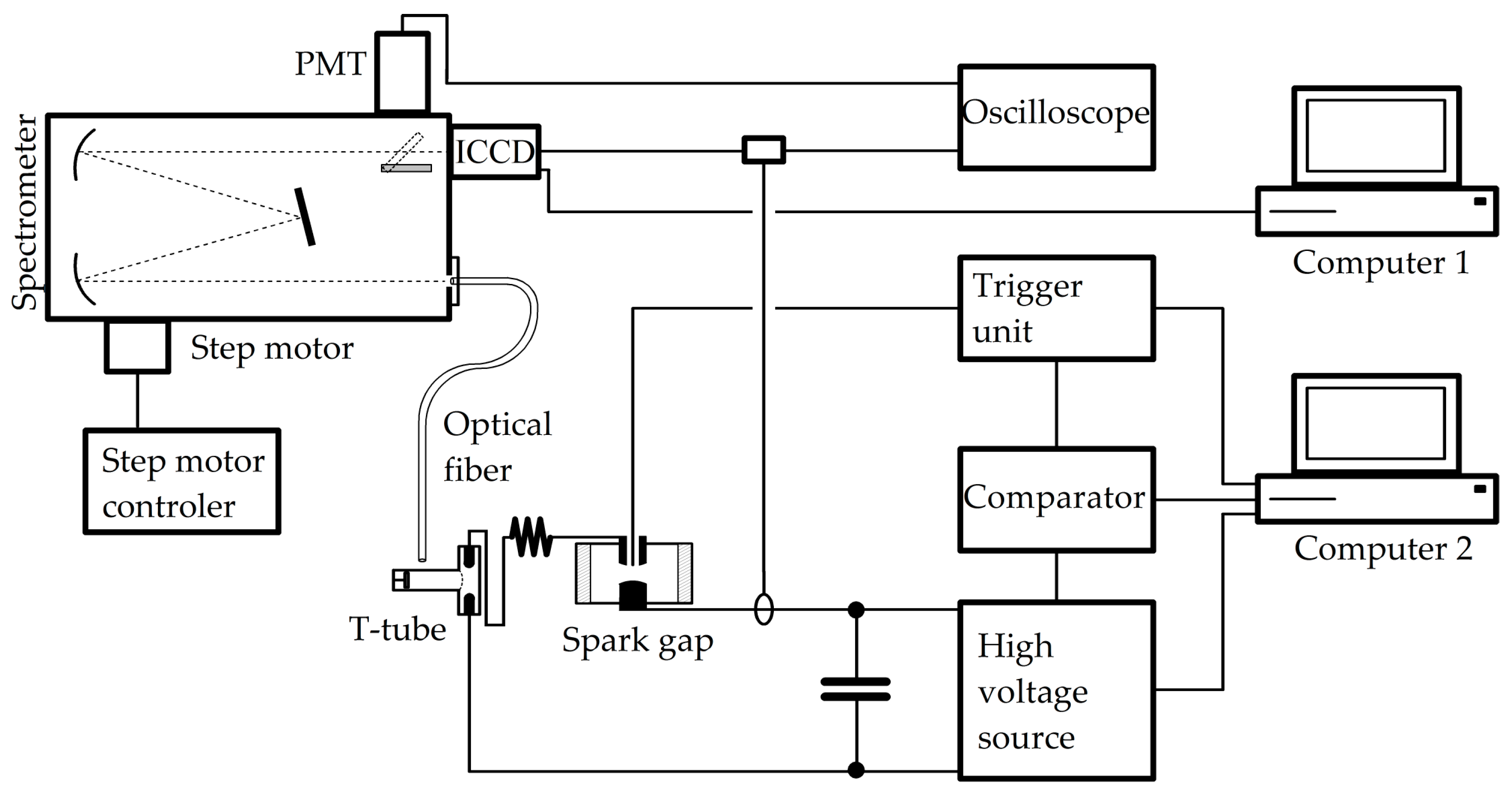
2.2. The Experimental Setup with the Spectral Recording System
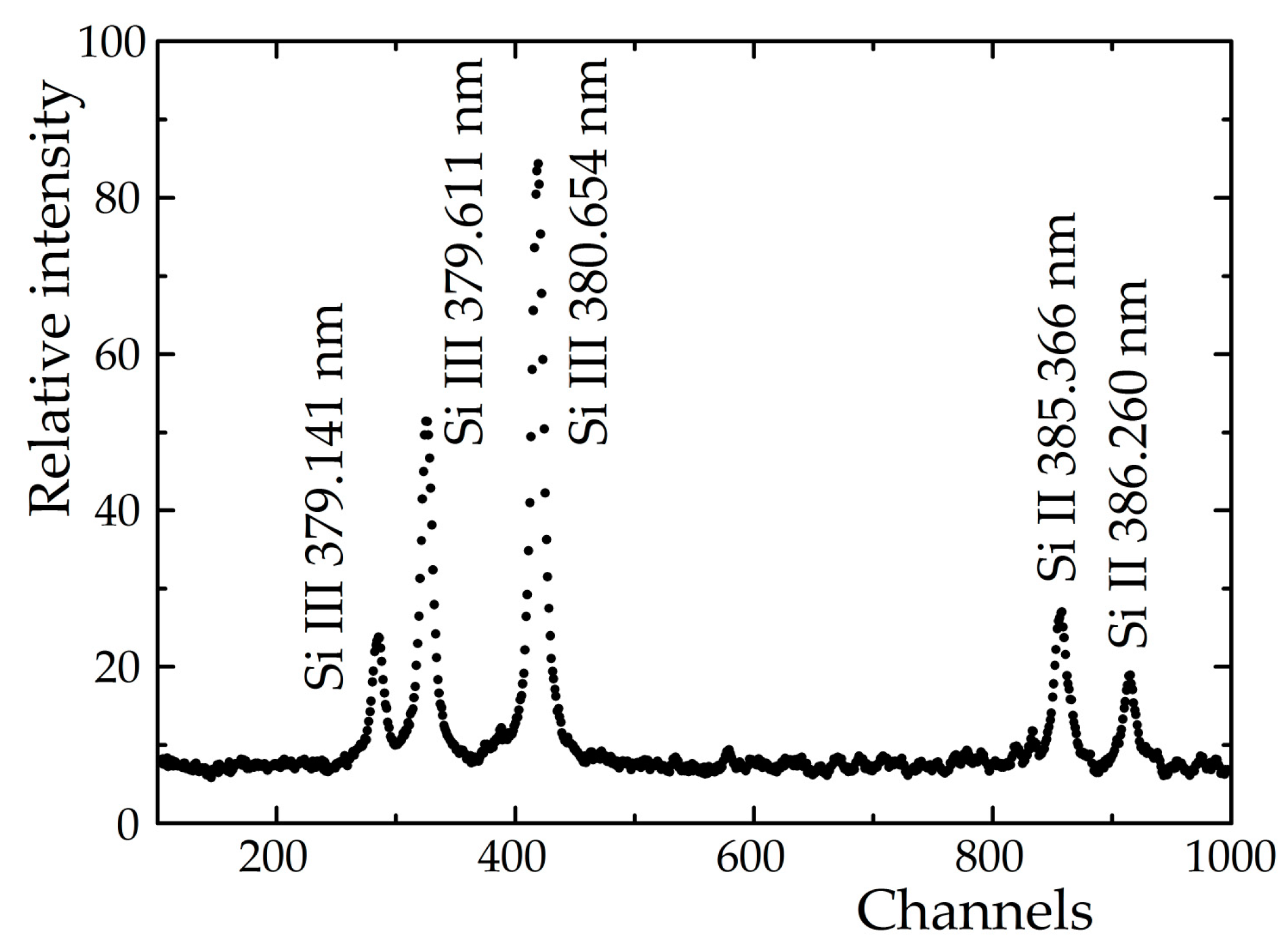
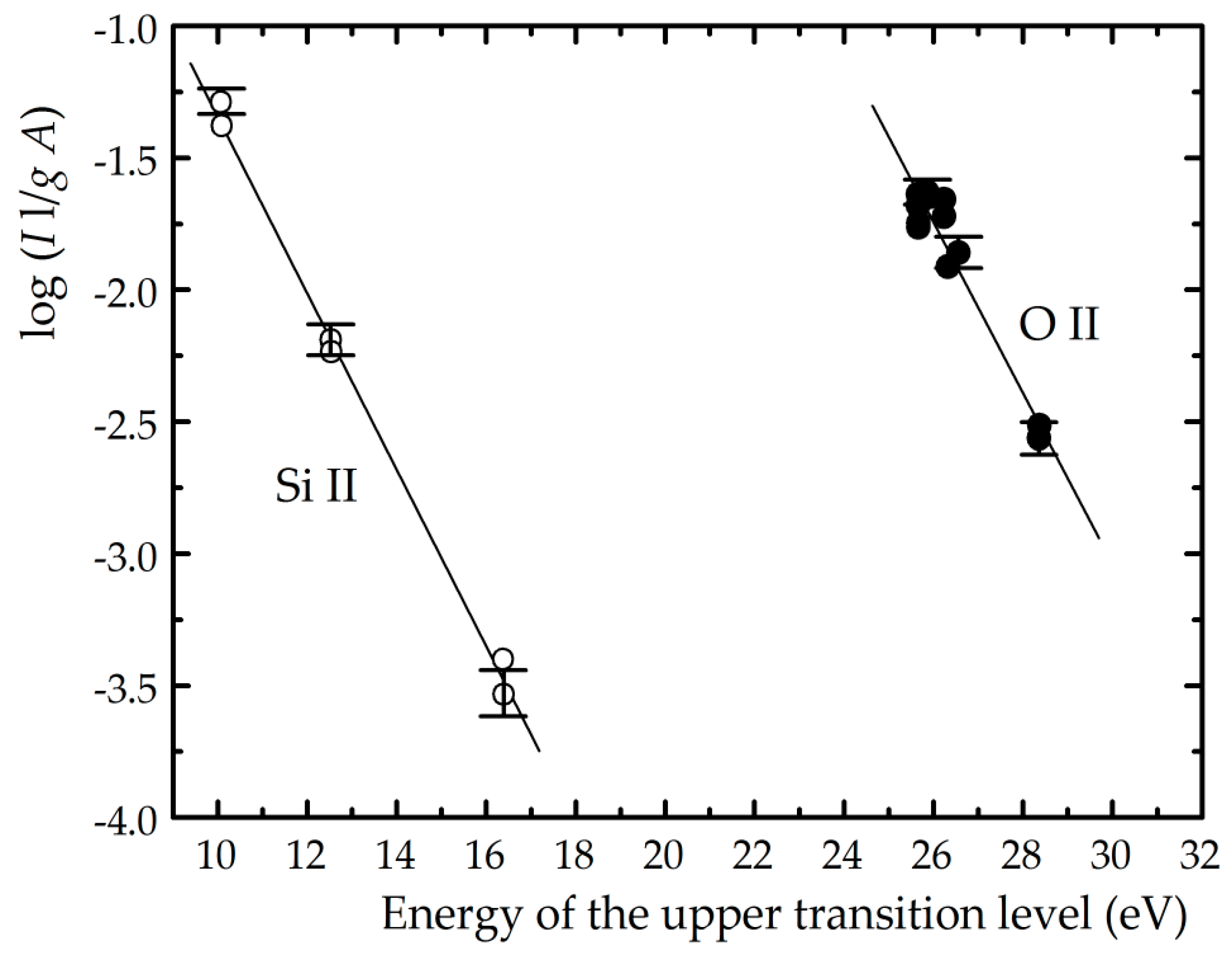
2.3. Plasma Diagnostics
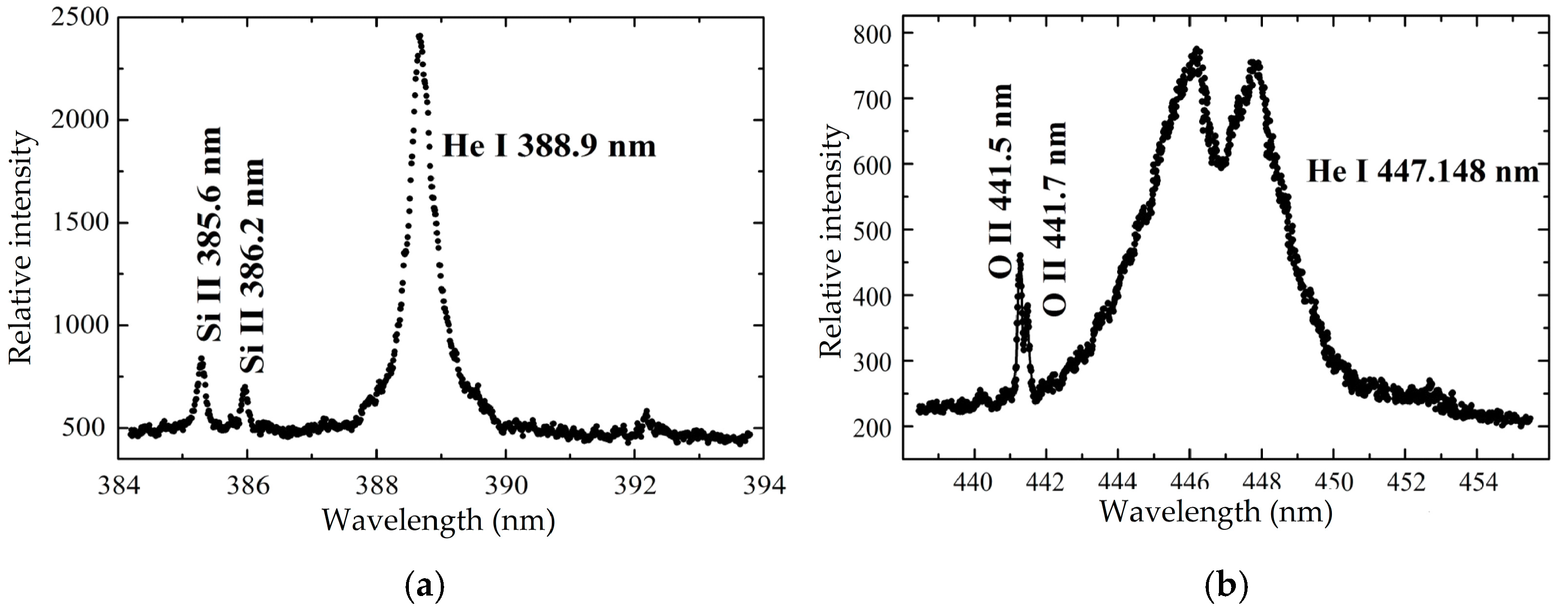
3. Spectral Line Broadening
4. Results of Stark Halfwidth Measurements for O II, Si II and Si III Spectral Lines
4.1. Analysis of Stark Halfwidths of O II Spectral Lines
4.2. Analysis of Stark Halfwidths of Si II Spectral Lines
4.3. Analysis of Stark Halfwidths of Si III Spectral Lines
5. Conclusions
Funding
Conflicts of Interest
References
- Platiša, M.; Popović, M.; Konjević, N. Stark broadening of O II and O III Lines. Astron. Astrophys. 1975, 45, 325–327. [Google Scholar]
- Purić, J.; Djeniže, S.; Srećković, A.; Platiša, M.; Labat, J. Stark-broadening regularities of prominent multiply-ionized-oxygen spectral lines in plasma. Phys. Rev. A 1998, 37, 498–503. [Google Scholar] [CrossRef]
- Djeniže, S.; Srećković, A.; Labat, J.; Platiša, M. Stark broadening and shift of OII spectral lines in higher multiplets. Z. Phys. D 1991, 21, 295–297. [Google Scholar] [CrossRef]
- Djeniže, S.; Milosavljević, V.; Srećković, A. Measured Stark widths and shifts of singly ionized oxygen spectral lines in lower multiplets. J. Quant. Spectrosc. Radiat. Transf. 1998, 59, 71–75. [Google Scholar] [CrossRef]
- Blagojević, B.; Popović, M.V.; Konjević, N. Stark Broadening of Spectral Lines of Singly Ionized C, N, O, F and Ne. Phys. Scr. 1999, 59, 374–378. [Google Scholar] [CrossRef]
- Del Val, J.A.; Aparicio, J.A.; González, V.; Mar, S. Measurement of Stark widths in single ionized oxygen. Astron. Astrophys. Suppl. Ser. 1999, 140, 171–176. [Google Scholar] [CrossRef]
- Srećković, A.; Drinčić, V.; Bukvić, S.; Djeniže, S. Stark Broadening Parameters and Transition Probabilities in the O II Spectrum. Phys. Scr. 2001, 63, 306–312. [Google Scholar] [CrossRef]
- Wollschläger, F.; Mitsching, J.; Meiners, D.; Depiesse, M.; Richou, J.; Lesage, A. Measurements of the ionised silicon multiplet (1) Stark parameters by two different methods. J. Quant. Spectrosc. Radiat. Transf. 1997, 58, 135–140. [Google Scholar] [CrossRef]
- Chiang, W.T.; Griem, H.R. Measurements of the Stark broadening of ionized silicon lines from a plasma. Phys. Rev. A 1978, 18, 1169–1175. [Google Scholar] [CrossRef]
- Pérez, C.; de la Rosa, I.; Frutos, A.M.; González, V.R.; Mar, S. Stark broadening of several SiII lines. Ann. Phys. 1990, 15, 115–116. [Google Scholar]
- Lesage, A.; Redon, R. Stark widths of faint Si II lines. Astron. Astrophys. 2004, 418, 765–769. [Google Scholar] [CrossRef]
- Pérez, C.; de la Rosa, I.; Frutos, A.M.; Mar, S. Temperature dependence of Stark broadening for several Si II lines. Phys. Rev. E 1993, 47, 756–759. [Google Scholar] [CrossRef]
- Konjević, N.; Purić, J.; Ćirković, L.; Labat, J. Measurements of the Stark broadening parameters of several Si II lines. J. Phys. B 1970, 3, 999–1003. [Google Scholar] [CrossRef]
- Purić, J.; Djeniže, S.; Labat, J.; Ćirković, L. Stark shift of neutral and ionized silicon spectral lines. Phys. Lett. A 1973, 45, 97–98. [Google Scholar] [CrossRef]
- Lesage, A.; Rathore, B.A.; Lakićević, I.S.; Purić, J. Stark widths and shifts of singly ionized silicon spectral lines. Phys. Rev. A 1983, 28, 2264–2268. [Google Scholar] [CrossRef]
- Lesage, A.; Miller, M.H. Détermination expérimentale de l’élargissment du déplacement Stark de raies d’atomes de silicium ionizes. C. R. Acad. Sci. Paris Ser. B 1975, 280, 645–647. [Google Scholar]
- González, V.R.; Aparicio, J.A.; del Val, J.A.; Mar, S. Stark broadening and shift measurements of visible Si II lines. J. Phys. B 2002, 35, 3557–3573. [Google Scholar]
- Bukvić, S.; Djeniže, S.; Srećković, A. Line broadening in the Si I, Si II, Si III, and Si IV spectra in the helium plasma. Astron. Astrophys. 2009, 508, 491–500. [Google Scholar] [CrossRef]
- Lesage, A.; Sahal-Brechot, S.; Miller, M.H. Stark broadening of singly ionized-silicon. Phys. Rev. A 1977, 16, 1617–1624. [Google Scholar] [CrossRef]
- Kusch, H.J.; Schröder, K. Experimental Stark Broadening Data of Si II and Si III Lines. Astron. Astrophys. 1982, 116, 255–259. [Google Scholar]
- Miller, M.H. Technical Note BN-550; University of Maryland: College Park, MD, USA, 1968. [Google Scholar]
- Chapelle, J.; Czernichowski, A. Stark Broadening of the Spectral Lines 6371.4 and 6347.1 A for Si II. Acta Phys. Pol. A 1972, 41, 753. [Google Scholar]
- González, V.R.; Aparicio, J.A.; del Val, J.A.; Mar, S. Stark width and shift measurements of visible SI III lines. Astron. Astrophys. 2000, 363, 1177–1185. [Google Scholar]
- Djeniže, S.; Srećković, A.; Labat, J.; Purić, J.; Platiša, M. Measured Stark widths of doubly and triply ionized silicon spectral lines. J. Phys. B 1992, 25, 785–790. [Google Scholar] [CrossRef]
- Platiša, M.; Dimitrijević, M.S.; Popović, M.; Konjević, N. Stark broadening of Si III and Si IV lines. J. Phys. B 1977, 10, 2997–3004. [Google Scholar] [CrossRef]
- Purić, J.; Djeniže, S.; Labat, J.; Ćirković, L. Stark broadening parameters of Si I, Si II and Si III lines. Z. Phys. 1974, 267, 71–75. [Google Scholar] [CrossRef]
- Sollerman, J.; Fynbo, J.P.U.; Gorosabel, J.; Halpern, J.P.; Hjorth, J.; Jakobsson, P.; Mirabal, N.; Watson, D.; Xu, D.; Castro-Tirado, A.J.; et al. The nature of the X-ray flash of 24 August 2005—Photometric evidence for an on-axis z = 0.83 burst with continuous energy injection and an associated supernova. Astron. Astrophys. 2007, 466, 839–846. [Google Scholar] [CrossRef]
- Muzzin, A.; Wilson, G.; Yee, H.K.C.; Gilbank, D.; Hoekstra, H.; Demarco, R.; Balogh, M.; van Dokkum, P.; Franx, M.; Ellingson, E.; et al. The Gemini Cluster Astrophysics Spectroscopic Survey (GCLASS): The Role of Environment and Self-regulation in Galaxy Evolution at z ~ 1. Astrophys. J. 2012, 746, 188. [Google Scholar] [CrossRef]
- Lorenzo, J.; Negueruela, I.; Castro, N.; Norton, A.J.; Vilardell, F.; Herrero, A. Astrophysical parameters of the peculiar X-ray transient IGR J11215−5952. Astron. Astrophys. 2014, 562, A18. [Google Scholar] [CrossRef]
- Berdyugina, S.V.; Ilyin, I.; Tuominen, I. The long-period RS CanumVenaticorum binary IM Pegasi I. Orbital and stellar parameters. Astron. Astrophys. 1999, 347, 932–936. [Google Scholar]
- Asplund, M. Line formation in solar granulation III. The photospheric Si and meteoritic Fe abundances. Astron. Astrophys. 2000, 359, 755–758. [Google Scholar]
- Briquet, M.; Aerts, C.; Lüftinger, T.; De Cat, P.; Piskunov, N.E.; Scuflaire, R. He and Si surface inhomogeneities of four Bp variable stars. Astron. Astrophys. 2004, 413, 273–283. [Google Scholar] [CrossRef]
- Khalack, V.; Landstreet, J.D. Partial Paschen–Back splitting of Si II and Si III lines in magnetic chemically peculiar stars. Mon. Not. R. Astron. Soc. 2012, 427, 569–580. [Google Scholar] [CrossRef]
- Gigosos, M.A.; Djurović, S.; Savić, I.; González-Herrero, D.; Mijatović, Z.; Kobilarov, R. Stark broadening of lines from transition between states n = 3 to n = 2 in neutral helium. Astron. Astrophys. 2014, 561, A135–A147. [Google Scholar] [CrossRef]
- Gavanski, L.; Belmonte, M.T.; Savić, I.; Djurović, S. Experimental Stark halfwidths of the ionized oxygen and silicon spectral lines. Mon. Not. R. Astron. Soc. 2016, 457, 4038–4050. [Google Scholar] [CrossRef]
- Djurović, S.; Mijatović, Z.; Vujičić, B.; Kobilarov, R.; Savić, I.; Gavanski, L. Measurement of the shock front velocity produced in a T-tube. Phys. Plasmas 2015, 22, 013505. [Google Scholar] [CrossRef]
- Kolb, A.C. Production of High-Energy Plasmas by Magnetically Driven Shock Waves. Phys. Rev. 1957, 107, 345–350. [Google Scholar] [CrossRef]
- Ivković, M.; González, M.Á.; Jovićević, S.; Gigosos, M.A.; Konjević, N. A simple line shape technique for electron number density diagnostics of helium and helium-seeded plasmas. Spectrochim. Acta B 2010, 65, 234–240. [Google Scholar] [CrossRef]
- Griem, H.R. Validity of Local Thermal Equilibrium in Plasma Spectroscopy. Phys. Rev. 1963, 131, 1170–1176. [Google Scholar] [CrossRef]
- NIST Atomic Spectra Database. Available online: https://www.nist.gov/pml/atomic-spectra-database (accessed on 13 November 2018).
- Griem, H.R. Plasma Spectroscopy; McGraw-Hill Book Company: New York, NY, USA, 1964. [Google Scholar]
- Mitchner, M.; Kruger, C.H. Partially Ionized Gases; John Wiley & Sons: New York, NY, USA, 1973. [Google Scholar]
- Konjević, N.; Roberts, J.R. A critical review of the Stark widths and shifts of spectral lines from non-hydrogenic atoms. J. Phys. Chem. Ref. Data 1976, 5, 209–257. [Google Scholar] [CrossRef]
- Kelleher, D.E. Stark broadening of visible neutral helium lines in a plasma. J. Quant. Spectrosc. Radiat. Transf. 1981, 25, 191–220. [Google Scholar] [CrossRef]
- Ali, A.W.; Griem, H.R. Theory of Resonance Broadening of Spectral Lines by Atom-Atom Impacts. Phys. Rev. 1965, 140, A1044–A1049. [Google Scholar] [CrossRef]
- Ali, A.W.; Griem, H.R. Errata: Theory of Resonance Broadening of Spectral Lines by Atom-Atom Impacts. Phys. Rev. 1966, 144, 366. [Google Scholar] [CrossRef]
- Davies, J.T.; Vaughan, J.M. A New Tabulation of the Voigt Profile. Astrophys. J. 1963, 137, 1302–1305. [Google Scholar] [CrossRef]
- Djurović, S.; Kobilarov, R.; Vujičić, B. Experimental difficulties in determination of the spectral line shapes emitted from plasma. Bull. Astron. Belgrade 1996, 153, 41. [Google Scholar]
- Konjević, N. Plasma broadening and shifting of non-hydrogenic spectral lines: Present status and applications. Phys. Rep. 1999, 316, 339–401. [Google Scholar] [CrossRef]
- Konjević, N.; Wiese, W.L. Experimental Stark widths and shifts for non-hydrogenic spectral lines of ionized atoms. J. Phys. Chem. Ref. Data 1976, 5, 259–308. [Google Scholar] [CrossRef]
- Konjević, N.; Dimitrijević, M.S.; Wiese, W.L. Experimental Stark Widths and Shifts for Spectral Lines of Neutral Atoms (A Critical Review of Selected Data for the Period 1976 to 1982). J. Phys. Chem. Ref. Data 1984, 13, 619–647. [Google Scholar] [CrossRef]
- Konjević, N.; Dimitrijević, M.S.; Wiese, W.L. Experimental Stark Widths and Shifts for Spectral Lines of Positive Ions (A Critical Review and Tabulation of Selected Data for the Period 1976 to 1982). J. Phys. Chem. Ref. Data 1984, 13, 649–686. [Google Scholar] [CrossRef]
- Konjević, N.; Wiese, W.L. Experimental Stark Widths and Shifts for Spectral Lines of Neutral and Ionized Atoms. J. Phys. Chem. Ref. Data 1990, 19, 1307–1385. [Google Scholar] [CrossRef]
- Konjević, N.; Lesage, A.; Fuhr, J.R.; Wiese, W.L. Experimental Stark Widths and Shifts for Spectral Lines of Neutral and Ionized Atoms (A Critical Review of Selected Data for the Period 1989 Through 2000). J. Phys. Chem. Ref. Data 2002, 31, 819–927. [Google Scholar] [CrossRef]
- Lesage, A. Experimental Stark widths and shifts for spectral lines of neutral and ionized atoms A critical review of selected data for the period 2001–2007. New Astron. Rev. 2009, 52, 471–535. [Google Scholar] [CrossRef]
- Wiese, W.L.; Konjević, N. Regularities and similarities in plasma broadened spectral line widths. J. Quant. Spectrosc. Radiat. Transf. 1982, 28, 185–198. [Google Scholar] [CrossRef]
- Dimitrijević, M.S.; Konjević, N. Stark widths of doubly- and triply-ionized atom lines. J. Quant. Spectrosc. Radiat. Transf. 1980, 24, 451–459. [Google Scholar] [CrossRef]
- Dimitrijević, M.S.; Konjević, N. Simple estimates for Stark broadening of ion lines in stellar plasmas. Astron. Astrophys. 1987, 172, 345–349. [Google Scholar]
- Griem, H.R. Spectral Line Broadening by Plasmas; Academic Press: New York, NY, USA, 1974. [Google Scholar]

 [57],
[57],  [58] and
[58] and  [59].
[59].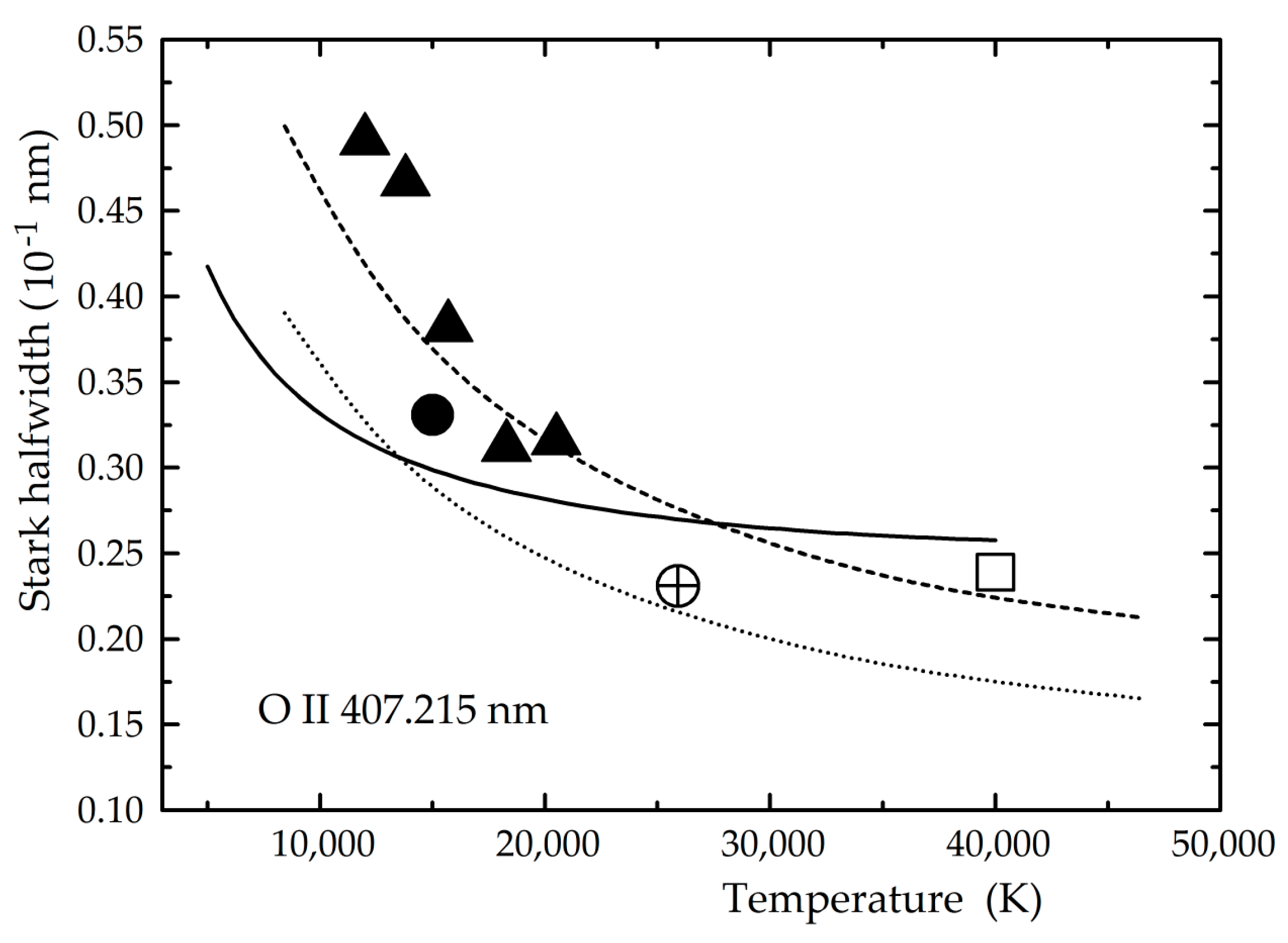
 [12], ★ [15], ◇ [16], ⬠ [17], ☆ [19], ◓ [20], ⬤ [35],
[12], ★ [15], ◇ [16], ⬠ [17], ☆ [19], ◓ [20], ⬤ [35],  [57],
[57],  [58] and
[58] and  [59].
[59].
 [25], ⛝ [26], ⬤ [35],
[25], ⛝ [26], ⬤ [35],  [57] and
[57] and  [58].
[58].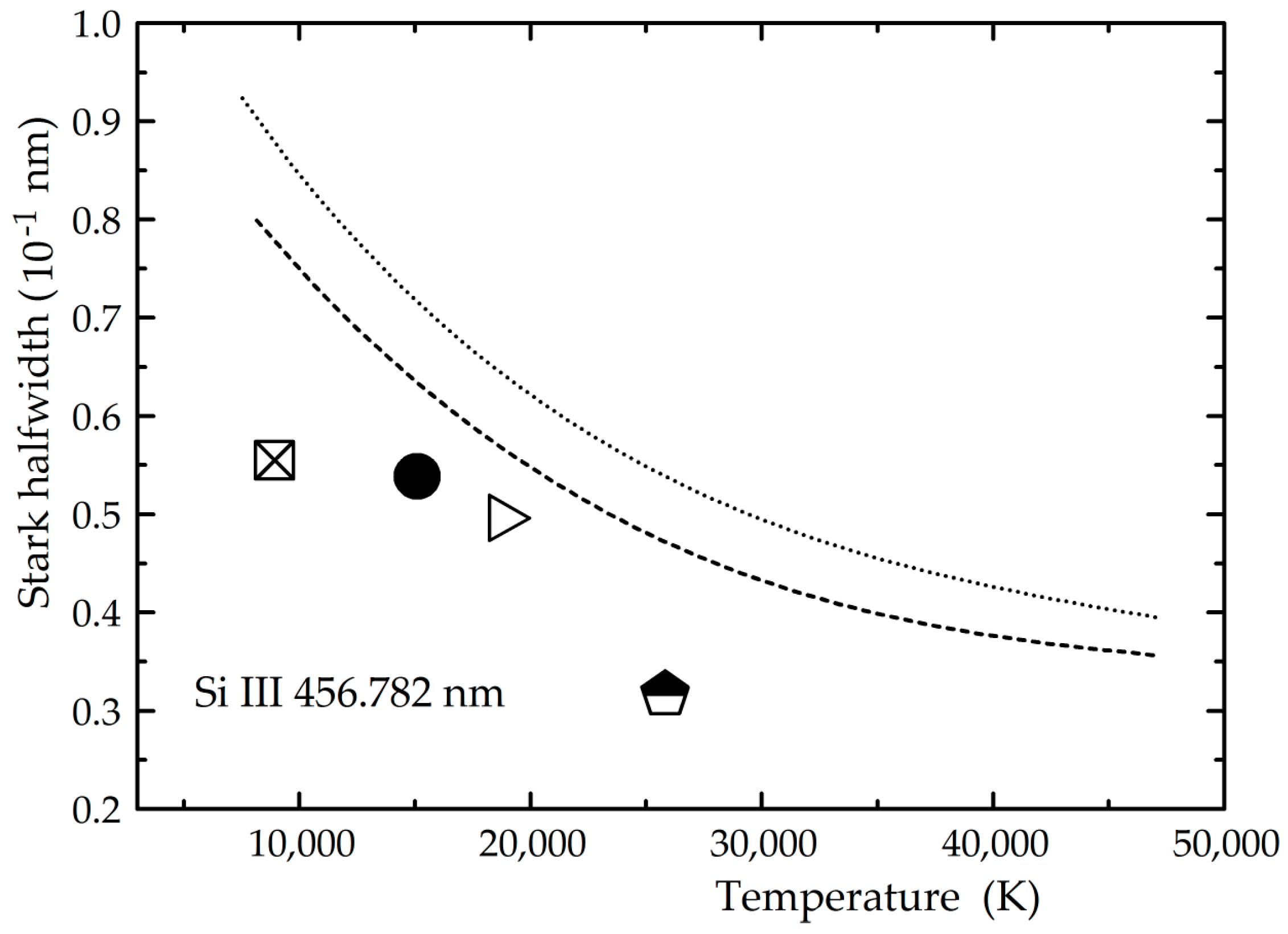
| Reference | Transition | Multiplet | No. of Measured Lines in Multiplet | Proposed Multiplet Criterion |
|---|---|---|---|---|
| [35] | (3P)3s-(3P)3p | 4P-4Do | 7 | Satisfied |
| (3P)3s-(3P)3p | 4P-4Po | 3 | Satisfied | |
| (3P)3s-(3P)3p | 4P-4So | 3 | Satisfied | |
| (3P)3s-(3P)3p | 2P-2Do | 2 | Satisfied | |
| (3P)3s-(3P)3p | 2P-2Po | 4 | Satisfied | |
| (3P)3p-(3P)3d | 4Do-4F | 2 | Satisfied | |
| (1D)3s-(1D)3p | 2D-2Fo | 2 | Satisfied | |
| (3P)3p-(3P)3d | 4Po-4P | 2 | Satisfied | |
| (3P)3p-(3P)3d | 4So-4P | 2 | Satisfied | |
| (1D)3p-(1D)3d | 2Fo-2G | 2 | Satisfied | |
| [1] | (3P)3s-(3P)3p | 4P-4Do | 2 | Satisfied |
| (3P)3s-(3P)3p | 4P-4So | 3 | Satisfied | |
| (1D)3s-(1D)3p | 2D-2Fo | 2 | Satisfied | |
| [4] | (3P)3s-(3P)3p | 4P-4Do | 2 | Satisfied |
| [5] | (3P)3s-(3P)3p | 2P-2Do | 2 | Satisfied |
| [6] | (3P)3s-(3P)3p | 4P-4Do | 4 | Satisfied |
| (3P)3s-(3P)3p | 4P-4Po | 3 | Satisfied | |
| (3P)3p-(3P)3d | 4Do-4F | 2 | Satisfied | |
| (1D)3s-(1D)3p | 2D-2Fo | 2 | Satisfied | |
| [7] | (3P)3s-(3P)3p | 2P-2Po | 2 | Not satisfied, 32.1% |
| (3P)3p-(3P)3d | 4Do-4F | 2 | Not satisfied, 26.7% | |
| (1D)3p-(1D)3d | 2Fo-2G | 2 | Not satisfied, 19.9% |
| Reference | Transition | Multiplet | No. of Measured Lines in Multiplet | Proposed Multiplet Criterion |
|---|---|---|---|---|
| [35] | 3s3p2-3s24p | 2D-2Po | 3 | Satisfied |
| 3s24s-3s24p | 2S-2Po | 2 | Satisfied | |
| 3s23d-3s24f | 2D-2Fo | 2 | Satisfied | |
| [8] | 3s3p2-3s24p | 2D-2Po | 3 | Not satisfied, 14% |
| [9] | 3s3p2-3s24p | 2D-2Po | 2 | Satisfied |
| 3s24s-3s24p | 2S-2Po | 2 | Satisfied | |
| [10] | 3s24s-3s24p | 2S-2Po | 2 | Satisfied |
| [12] | 3s24s-3s24p | 2S-2Po | 2 | Satisfied |
| 3s23d-3s24f | 2D-2Fo | 2 | Not satisfied, 25% | |
| [13] | 3s3p2-3s24p | 2D-2Po | 2 | Satisfied |
| 3s24s-3s24p | 2S-2Po | 2 | Satisfied | |
| [14] | 3s3p2-3s24p | 2D-2Po | 2 | Not satisfied, 28% |
| [15] | 3s3p2-3s24p | 2D-2Po | 2 | Not satisfied, 11.7% |
| 3s24s-3s24p | 2S-2Po | 2 | Not satisfied, 18% | |
| 3s23d-3s24f | 2D-2Fo | 2 | Not satisfied, 11.5% | |
| [17] | 3s3p2-3s24p | 2D-2Po | 3 | Satisfied |
| 3s24s-3s24p | 2S-2Po | 2 | Satisfied | |
| 3s23d-3s24f | 2D-2Fo | 2 | Satisfied | |
| [18] | 3s3p2-3s24p | 2D-2Po | 3 | Not satisfied, 11.3% |
| 3s24s-3s24p | 2S-2Po | 2 | Satisfied | |
| 3s23d-3s24f | 2D-2Fo | 2 | Satisfied | |
| [19] | 3s3p2-3s24p | 2D-2Po | 2 | Satisfied |
| 3s24s-3s24p | 2S-2Po | 2 | Satisfied | |
| 3s23d-3s24f | 2D-2Fo | 2 | Satisfied |
| Reference | Transition | Multiplet | No. of Measured Lines in Multiplet | Proposed Multiplet Criterion |
|---|---|---|---|---|
| [35] | 3s3d-3s4p | 3D-3Po | 3 | Satisfied |
| 3s4s-3s4p | 3S-3Po | 3 | Satisfied | |
| 3s4p-3s4d | 3Po-3D | 3 | Satisfied | |
| [20] | 3s4p-3s4d | 3Po-3D | 3 | Not satisfied, 16% |
| [18] | 3s3d-3s4p | 3D-3Po | 3 | Satisfied |
| 3s4s-3s4p | 3S-3Po | 3 | Satisfied | |
| 3s4p-3s4d | 3Po-3D | 3 | Satisfied | |
| [23] | 3s4s-3s4p | 3S-3Po | 3 | Satisfied |
| 3s4p-3s4d | 3Po-3D | 3 | Satisfied | |
| [25] | 3s4s-3s4p | 3S-3Po | 3 | Satisfied |
| [26] | 3s4s-3s4p | 3S-3Po | 2 | Not satisfied, 11.5% |
© 2019 by the author. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Gavanski, L. Measurement of Stark Halfwidths of Spectral Lines of Ionized Oxygen and Silicon Emitted from T-tube Plasma. Atoms 2019, 7, 8. https://doi.org/10.3390/atoms7010008
Gavanski L. Measurement of Stark Halfwidths of Spectral Lines of Ionized Oxygen and Silicon Emitted from T-tube Plasma. Atoms. 2019; 7(1):8. https://doi.org/10.3390/atoms7010008
Chicago/Turabian StyleGavanski, Lazar. 2019. "Measurement of Stark Halfwidths of Spectral Lines of Ionized Oxygen and Silicon Emitted from T-tube Plasma" Atoms 7, no. 1: 8. https://doi.org/10.3390/atoms7010008
APA StyleGavanski, L. (2019). Measurement of Stark Halfwidths of Spectral Lines of Ionized Oxygen and Silicon Emitted from T-tube Plasma. Atoms, 7(1), 8. https://doi.org/10.3390/atoms7010008




