Progress in Finding New Energy Levels Using Laser Spectroscopy
Abstract
:1. Introduction
- -
- wide wavelength range;
- -
- very good resolution; and
- -
- very large dynamical range.
- -
- Doppler-limited resolution or even higher (Doppler-free or Doppler-reduced methods); and
- -
- much more sensitivity due to the high spectral energy density.
2. Laser-Spectroscopic Experiment
3. Finding of New Energy Levels by Laser Spectroscopy
3.1. Finding of Excitation Wavelengths
3.2. Setting Excitation Wavelength, e.g., to 5683.40 Å
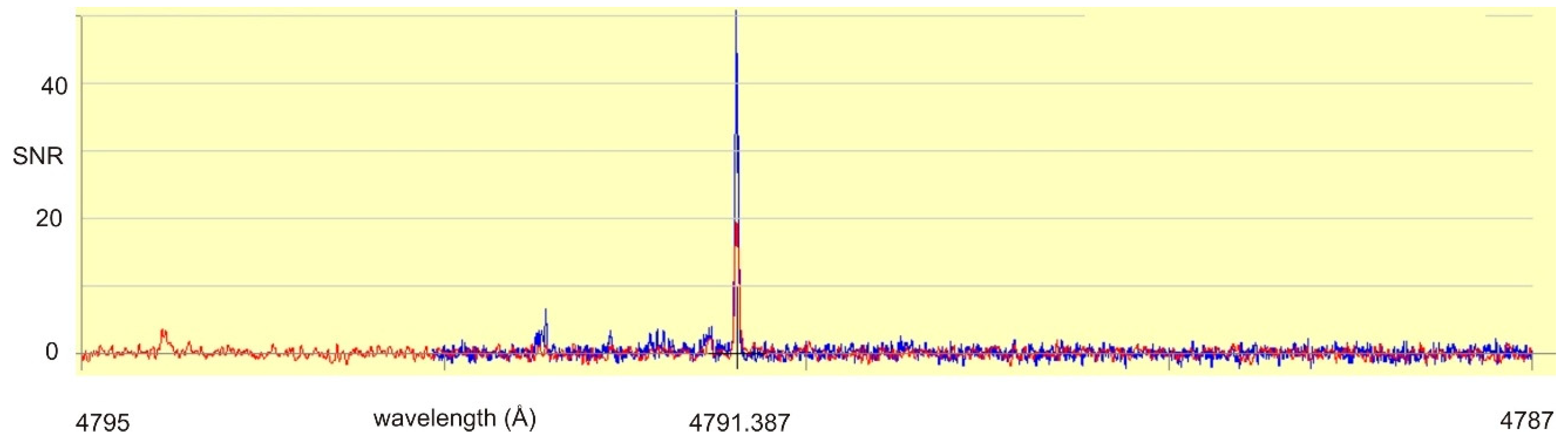
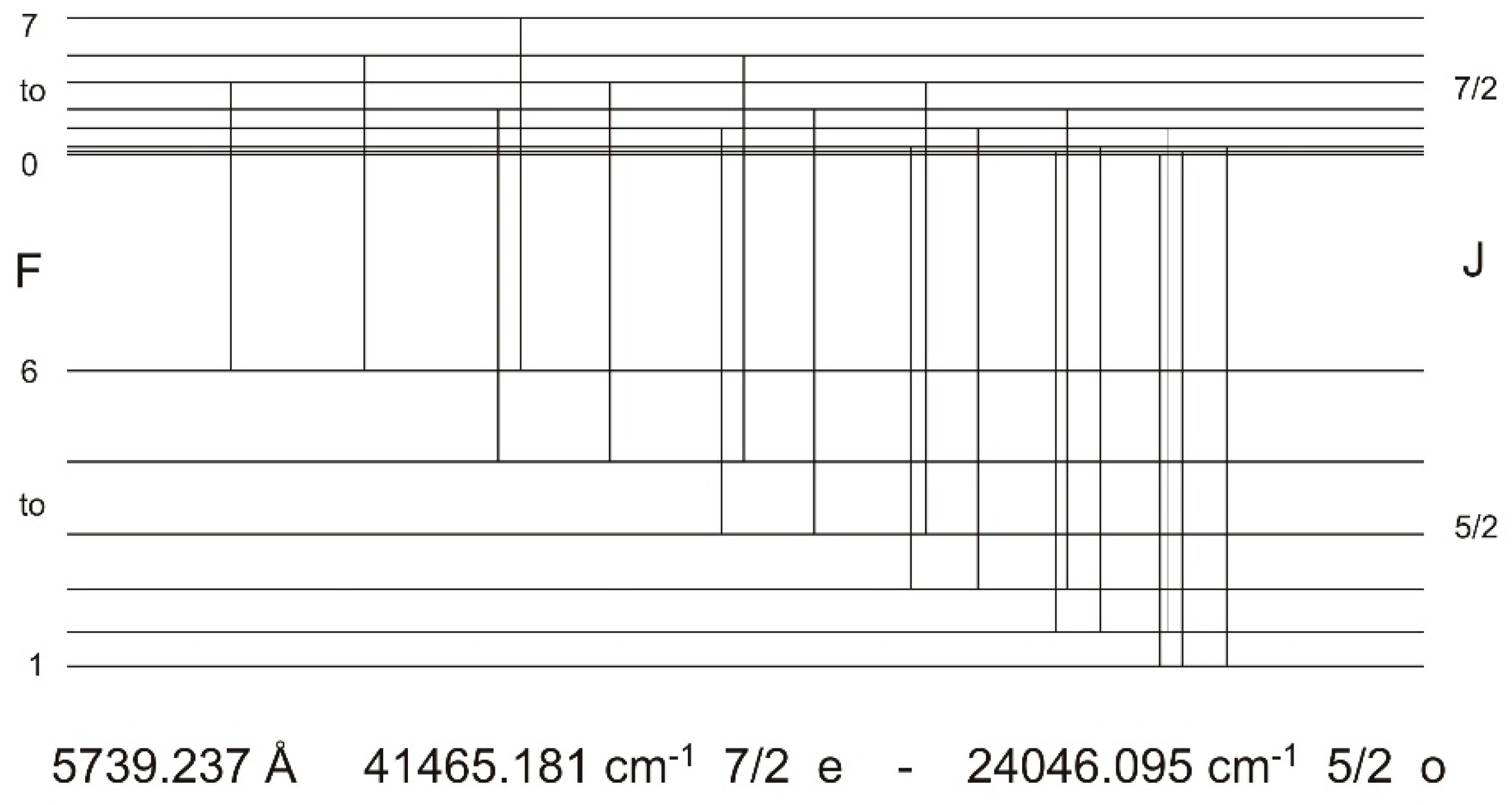
3.3. Observation of LIF Signals, Location of a New Energy Level
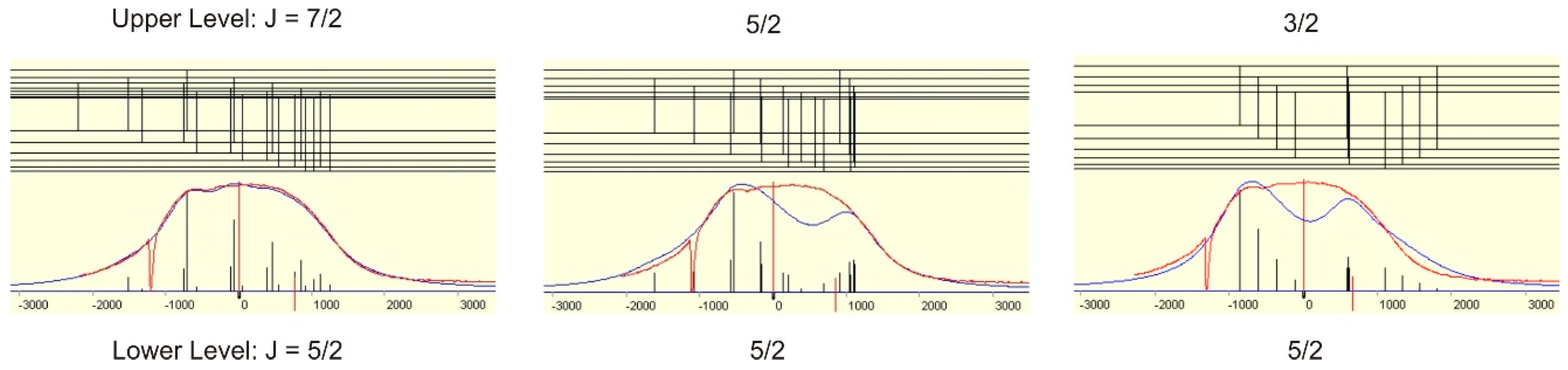
3.4. Determination of Correct J-Value
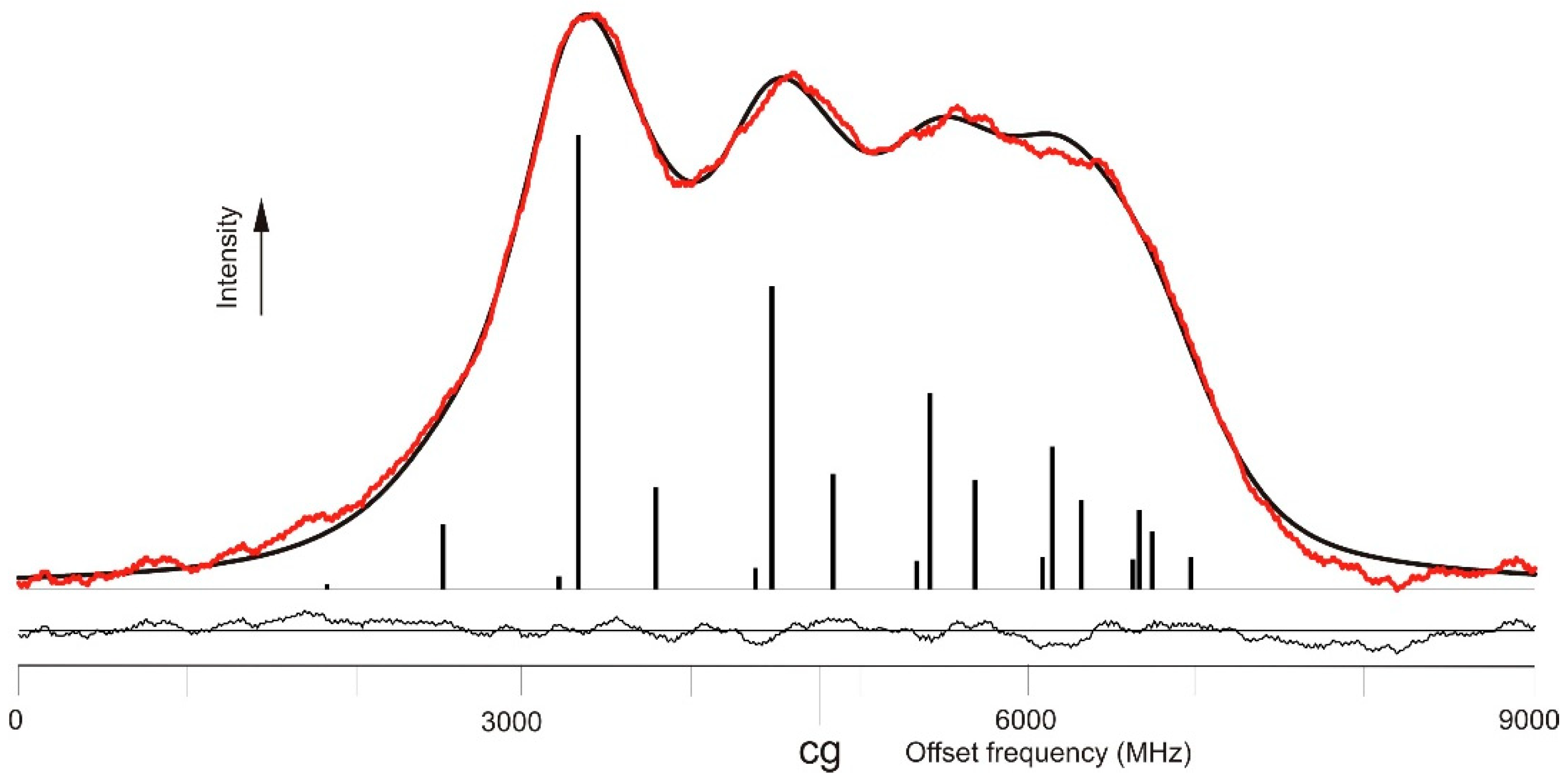
3.5. Confirmation of the Existence of the New Level
4. Conclusions
- -
- list of all observed wavelengths in an extended spectral range;
- -
- exactly known excitation wavelengths (within the Doppler width), needed uncertainty ca. 0.01 Å;
- -
- from experiment: recording of hf pattern;
- -
- from experiment: finding of LIF lines and their relative phase;
- -
- reliable classification of LIF lines;
- -
- calculation of the energy of the new level;
- -
- simulation of the hf pattern to find J and A of the unknown level involved in the observed line;
- -
- calculation of a transition list from the new level to other known levels;
- -
- prediction of excitation wavelength, hf pattern, and LIF lines; and
- -
- from experiment: confirmation by laser excitation of calculated transition wavelengths.
Funding
Acknowledgments
Conflicts of Interest
References
- Moore, C.E. Atomic Energy Levels; Circular of the National Bureau of Standards 467; U.S. Government Printing Office: Washington, DC, USA, 1958; Volume III.
- Kramida, A.; Ralchenko, Y.; Reader, J.; NIST ASD Team. NIST Atomic Spectra Database (Version 5.3); National Institute of Standards and Technology: Gaithersburg, MD, USA, 2015. Available online: http://physics.nist.gov/asd (accessed on 30 June 2018).
- Windholz, L.; Gamper, B.; Binder, T. Variation of the observed widths of La I lines with the energy of the upper excited levels, demonstrated on previously unknown energy levels. Spectr. Anal. Rev. 2016, 4, 23–40. [Google Scholar] [CrossRef]
- Windholz, L.; Gamper, B.; Glowacki, P.; Dembczynski, J. The puzzle of the La I lines 6520.644 Å and 6519.869 Å. Spectr. Anal. Rev. 2014, 2, 10. [Google Scholar] [CrossRef]
- Mu, X.L.; Deng, L.H.; Huo, X.; Ye, J.; Windholz, L.; Wang, H.L. Hyperfine structure investigations in atomic iodine. J. Quant. Spectrosc. Radiat. Transf. 2018, 217, 229–234. [Google Scholar] [CrossRef]
- Martin, W.C.; Zalubas, R.; Hagan, L. Atomic Energy Levels—The Rare-Earth Elements; National Bureau of Standards NSRDS-NBS 60; US GPO: Washington, DC, USA, 1978.
- Harrison, G.R. Wavelength Tables; Massachusetts Institute of Technology, The MIT Press: Cambridge, MA, USA, 1969. [Google Scholar]
- Güzelçimen, F.; Başar, G.Ö.; Tamanis, M.; Kruzins, A.; Ferber, R.; Windholz, L.; Kröger, S. High-resolution Fourier transform spectroscopy of lanthanum in Ar discharge in the near infrared. Astrophys. Suppl. Ser. 2013, 218, 18. [Google Scholar] [CrossRef]
- Başar, G.Ö.; (Istanbul University). Personal communication, 2017. Level not yet published.
- Furmann, B.; Stefańska, D.; Dembczyński, J. Experimental investigations of the hyperfine structure in neutral La: II. Even parity levels. J. Phys. B 2010, 43, 015001. [Google Scholar] [CrossRef]
- Gamper, B. Hyperfine Structure Analysis of Praseodymium and Lanthanum. Ph.D. Thesis, Graz University of Technology, Graz, Austria, 2013. Unpublished. [Google Scholar]
- Furmann, B.; Stefańska, D.; Dembczyński, J. Hyperfine structure analysis odd configurations levels in neutral lanthanum: I. Experimental. Phys. Scr. 2007, 76, 264. [Google Scholar] [CrossRef]
- Guthöhrlein, G.H. Program Package “Fitter”; Helmut-Schmidt-Universität, Universität der Bundeswehr Hamburg: Hamburg, Germany, 1998; Unpublished. [Google Scholar]
- Windholz, L.; Guthöhrlein, G.H. Classification of Spectral Lines by Means of their Hyperfine Structure. Application to Ta I and Ta II Levels. Phys. Scr. 2003, T105, 55. [Google Scholar] [CrossRef]
- Windholz, L. Finding of previously unknown energy levels using Fourier-transform and laser spectroscopy. Phys. Scr. 2016, 91, 114003. [Google Scholar] [CrossRef]
- Guthöhrlein, G.H.; Mocnik, H.; Windholz, L. A new energy level of the neutral tantalum atom. Z. Phys. D 1995, 35, 177–178. [Google Scholar] [CrossRef]


| Line | Upper Level | Lower Level | Comment | |||||||
|---|---|---|---|---|---|---|---|---|---|---|
| no. | Wave-Length (Å) | Energy (cm−1) | J | P | Energy (cm−1) | J | P | |||
| 1 | 5683.94 | 40,392.82 * | 3/2 | e | [9] | 22,804.250 | 5/2 | o | [3] | * preliminary, not published |
| 2 | 5683.73 | 123,826.7500 | 2 | e | [2] | 106,237.5518 | 2 | o | [2] | Ar I |
| 3 | 5643.40 | 41,465.181 | 7/2 | e | this work | 23,874.946 | 5/2 | o | [2] | nLIF 4792 |
| 4 | 5682.72 | 40,813.42 | 7/2 | e | [10] | 23,221.097 | 7/2 | o | [3] | |
| 5 | 5682.507 | 31,688.660 | 3/2 | e | [3] | 14,095.677 | 1/2 | o | [3] | |
| 6 | 5682.51 | 41,681.502 | 5/2 | e | this work | 24,088.541 | 7/2 | o | [3] | nLIF 4337Blend with line 5 |
| 7 | 5682.196 | 37,612.922 | 3/2 | e | [3] | 20,018.977 | 3/2 | o | [3] | |
| 8 | 5682.08 | 38,566.480 | 7/2 | e | [10] | 20,972.166 | 5/2 | o | [3] | |
| 9 | 5681.896 | 123,832.4190 | 3 | e | [2] | 106,237.5518 | 2 | o | [2] | Ar I |
| 10 | 5681.67 | 41,300.404 | 1/2 | e | [11] | 23,704.816 | 3/2 | o | [3] | |
| 11 | 5681.50 | 35,393.395 | 5/2 | e | [3] | 17,797.301 | 3/2 | o | [3] | |
| 12 | 5681.22 | not classified | ||||||||
| 13 | 5681.11 | not classified | ||||||||
| 14 | 5680.80 | not classified | ||||||||
© 2018 by the author. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Windholz, L. Progress in Finding New Energy Levels Using Laser Spectroscopy. Atoms 2018, 6, 54. https://doi.org/10.3390/atoms6040054
Windholz L. Progress in Finding New Energy Levels Using Laser Spectroscopy. Atoms. 2018; 6(4):54. https://doi.org/10.3390/atoms6040054
Chicago/Turabian StyleWindholz, Laurentius. 2018. "Progress in Finding New Energy Levels Using Laser Spectroscopy" Atoms 6, no. 4: 54. https://doi.org/10.3390/atoms6040054
APA StyleWindholz, L. (2018). Progress in Finding New Energy Levels Using Laser Spectroscopy. Atoms, 6(4), 54. https://doi.org/10.3390/atoms6040054




