Experimental and Theoretical Electron Collision Broadening Parameters for Several Ti II Spectral Lines of Industrial and Astrophysical Interest
Abstract
1. Introduction
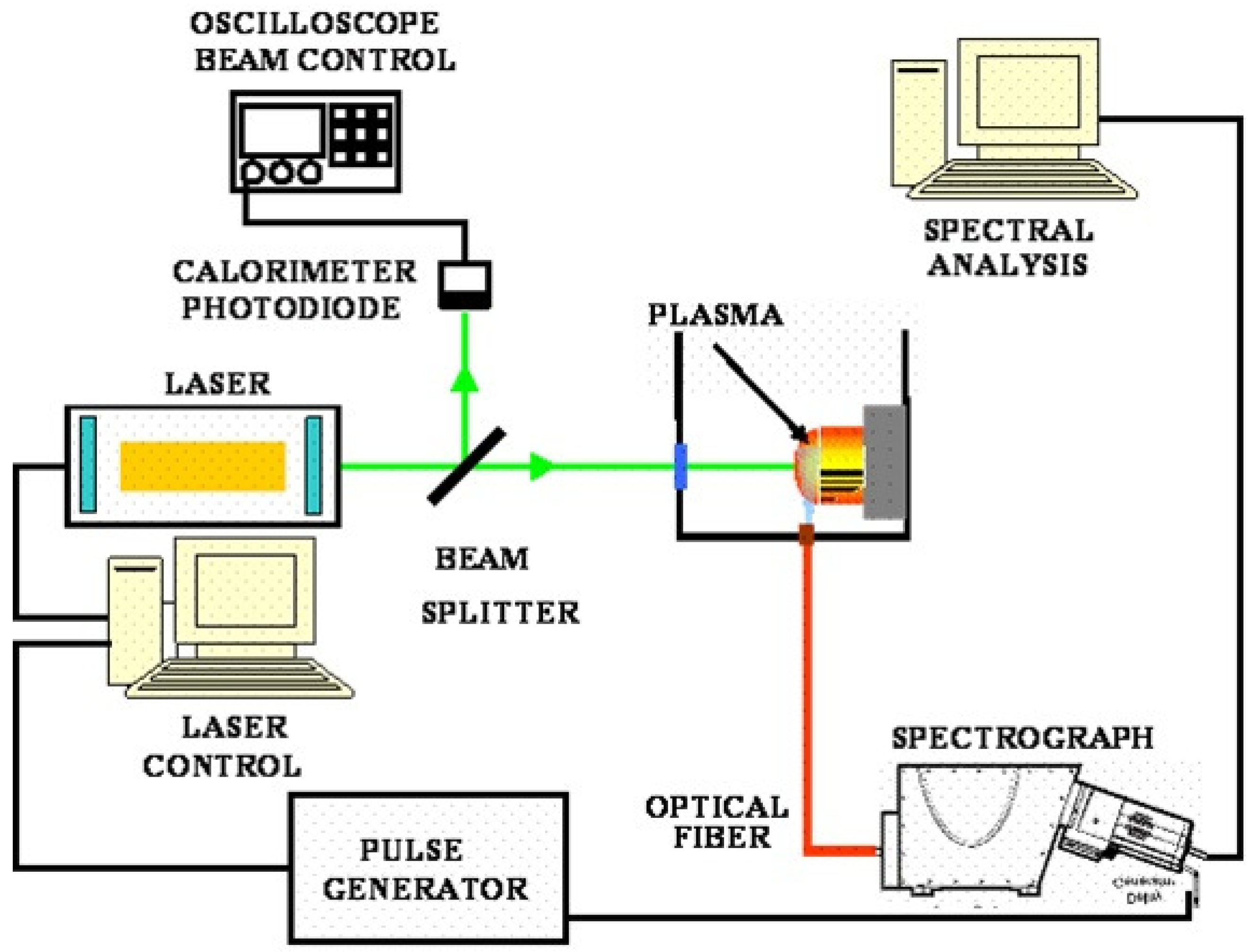
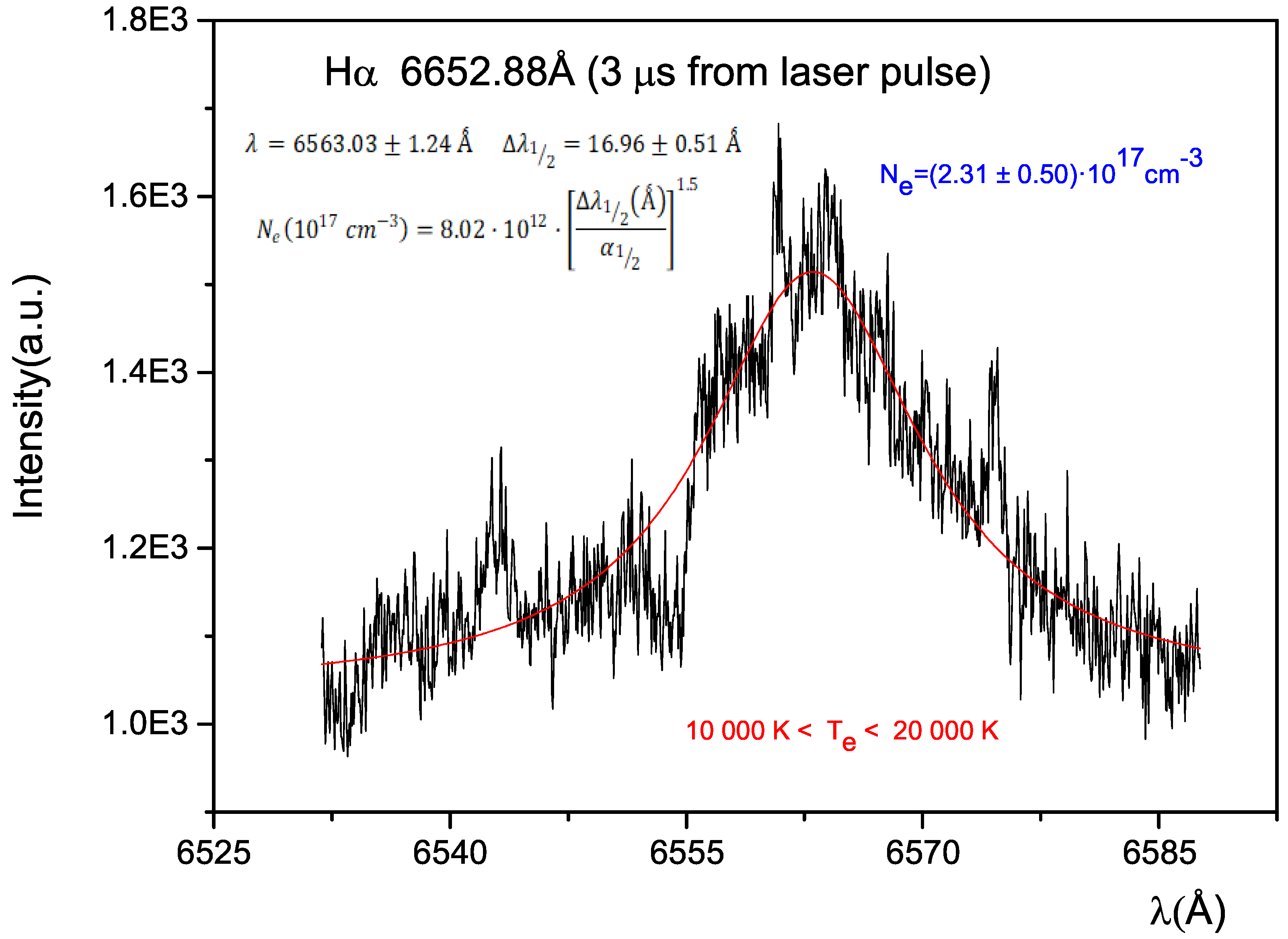
2. Experimental Setup
3. Theoretical Calculations
4. Discussion
5. Conclusions
Author Contributions
Funding
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Woosley, S.E.; Weaver, T.A. The Evolution and Explosion of Massive Stars. II. Explosive Hydrodynamics and Nucleosynthesis. Astrophys. J. Suppl. Ser. 1995, 101, 181. [Google Scholar] [CrossRef]
- Wood, M.P.; Lawler, J.E.; Sneden, C.; Cowan, J.J. Improved Ti II log(gf) values and abundance determinations in the photospheres of the Sun and metal-poor star HD 84937. Astrophys. J. Suppl. Ser. 2013, 208, 27. [Google Scholar] [CrossRef]
- Kramida, A.; Ralchenco, Y.; Reader, J. NIST ASD Team (2013) NIST Atomic Spectra Database (v.5.3). 2013. Available online: http://physics.nist.gov/asd (accessed on 1 January 2024).
- Yadav, M.; Misra, A.; Malhotra, A.; Kumar, N. Design and analysis of a high-pressure turbine blade in a jet engine using advanced materials. Mater. Today Proc. 2020, 25, 639–645. [Google Scholar] [CrossRef]
- Moayedee, Y.; Nikzad, L.; Fakhraei, O.; Paykar, Z.; Zekavat, E. Improvement of mechanical, biological, and electrochemical properties of Ti6Al4V alloy modified with Nb and Ag for biomedical applications. J. Alloys Compd. 2024, 972, 172736. [Google Scholar] [CrossRef]
- Warzanskyj, W.; Angulo, I.; Cordovilla, F.; Díaz, M.; Porro, J.; García-Beltrán, A.; Cabeza, S.; Ocaña, J. Analysis of the thermal stability of residual stresses induced in Ti-6Al-4 V by high density LSP treatments. J. Alloys Compd. 2023, 931, 167530. [Google Scholar] [CrossRef]
- Lundberg, H.; Hartman, H.; Engström, L.; Nilsson, H.; Persson, A.; Palmeri, P.; Quinet, P.; Fivet, V.; Malcheva, G.; Blagoev, K. Oscillator strengths for high-excitation Ti ii from laboratory measurements and calculations. Mon. Not. R. Astron. Soc. 2016, 460, 356–362. [Google Scholar] [CrossRef]
- Pickering, J.C.; Thorne, A.P.; Perez, R. Oscillator Strengths of Transitions in Ti II in the Visible and Ultraviolet regions. Astrophys. J. Suppl. Ser. 2001, 132, 403. [Google Scholar] [CrossRef]
- Alkallas, F.H.; Abu El Maati, L.; Ben Nessib, N.; Dimitrijević, M.S. Energy levels of the singly ionized titanium: Ti II ion. Astron. Nachrichten 2022, 343, e210059. [Google Scholar] [CrossRef]
- Hermann, J.; Thomann, A.L.; Boulmer-Leborgne, C.; Dubreuil, B.; De Giorgi, M.L.; Perrone, A.; Luches, A.; Mihailescu, I.N. Plasma diagnostics in pulsed laser TiN layer deposition. J. Appl. Phys. 1995, 77, 2928–2936. [Google Scholar] [CrossRef]
- Tankosić, D.; Popovic, L.C.; Dimitrijevic, M.S. Electron-impact Stark broadening parameters for Ti II and Ti III spectral lines. At. Data Nucl. Data Tables 2001, 77, 277–310. [Google Scholar] [CrossRef]
- Sahal-Bréchot, S.; Dimitrijević, M.S.; Moreau, N.; Nessib, N.B. The STARK-B database VAMDC node: A repository for spectral line broadening and shifts due to collisions with charged particles. Phys. Scr. 2015, 90, 054008. [Google Scholar] [CrossRef]
- Manrique, J.; Aguilera, J.A.; Aragón, C. Experimental Stark widths and shifts of Ti ii spectral lines. Mon. Not. R. Astron. Soc. 2016, 462, 1501–1507. [Google Scholar] [CrossRef]
- Sahal-Brechot, S.; Dimitrijevic, M.; Nessib, N.B.; Moreau, N. STARK-B. 2020. Available online: http://stark-b.obspm.fr/index.php/contact (accessed on 1 January 2024).
- Griem, H.T. Semiempirical formulas for the Electron-Impact widths and shifts of isolated ion lines in plasmas. Phys. Rev. 1968, 165, 258–266. [Google Scholar] [CrossRef]
- de Andrés-García, I.; Isidoro-García, L.; Fernández, F.; Porro, J.; Colón, C. Influence of Gaunt factors on the calculation of the Stark broadening parameters for 56 spectral lines of Vii with high industrial and astrophysical interest. Mon. Not. R. Astron. Soc. 2023, 523, 2146–2157. [Google Scholar] [CrossRef]
- Sampson, D.H.; Zhang, H.L. Semiempirical Formulae for Electron Impact Excitation and Ionization of Hydrogenic Ions. Astrophys. J. 1988, 335, 516. [Google Scholar] [CrossRef]
- Moreno-Díaz, C.; Alonso-Medina, A.; Colón, C.; Porro, J.; Ocaña, J. Measurement of plasma electron density generated in an experiment of Laser Shock Processing, utilizing the Halpha-line. J. Mater. Process. Technol. 2016, 232, 9–18. [Google Scholar] [CrossRef]
- Gigosos, M.; Cardeñoso-Payo, V. New plasma diagnosis tables of hydrogen Stark broadening including ion dynamics. J. Phys. B At. Mol. Opt. Phys. 1999, 29, 4795. [Google Scholar] [CrossRef]
- Ashkenazy, J.; Kipper, R.; Caner, M. Spectroscopic measurements of electron density of capillary plasma based on Stark broadening of hydrogen lines. Phys. Rev. A 1991, 43, 5568–5574. [Google Scholar] [CrossRef]
- Kepple, P.; Griem, H.R. Improved Stark Profile Calculations for the Hydrogen Lines Hα, Hβ, Hγ, and Hδ. Phys. Rev. 1968, 173, 317–325. [Google Scholar] [CrossRef]
- El Sherbini, A.; El Sherbini, T.; Hegazy, H.; Cristoforetti, G.; Legnaioli, S.; Palleschi, V.; Pardini, L.; Salvetti, A.; Tognoni, E. Evaluation of self-absorption coefficients of aluminum emission lines in laser-induced breakdown spectroscopy measurements. Spectrochim. Acta Part B At. Spectrosc. 2005, 60, 1573–1579. [Google Scholar] [CrossRef]
- Alonso-Medina, A. Experimental determination of the Stark widths of Pb I spectral lines in a laser-induced plasma. Spectrochim. Acta Part B At. Spectrosc. 2008, 63, 598–602. [Google Scholar] [CrossRef]
- Baranger, M. General Impact Theory of Pressure Broadening. Phys. Rev. 1958, 112, 855–865. [Google Scholar] [CrossRef]
- Seaton, M.J. The Theory of Excitation and Ionization by Electron Impact. In Proceedings of the Atomic and Molecular Processes; Seaton, M.J., Bates, D.R., Eds.; Academic Press Inc.: New York, NY, USA, 1962; pp. 374–420. [Google Scholar]
- van Regemorter, H. Rate of Collisional Excitation in Stellar Atmospheres. Astrophys. J. 1962, 136, 906. [Google Scholar] [CrossRef]
- Cowan, R. The Theory of Atomic Struture and Spectra; University of California Press: Berkeley, CA, USA, 1981; p. 57. [Google Scholar] [CrossRef]

| Wavelength | Transition Levels | This Work | Bibliography | |||||
|---|---|---|---|---|---|---|---|---|
| d | Ref | Ref | ||||||
| (Å) | Upper | Lower | d | d | ||||
| 3461.49 | 3d4p | 3d | 13.03 | −3.97 | 10.6 ± 1.6 | 3.6 ± 0.5 | 6 ± 1 | −2 ± 1 |
| 3465.54 | 3d4p | 3d4s | 4.96 | −1.89 | 10.5 ± 1.6 | 0.5 ± 0.1 | ||
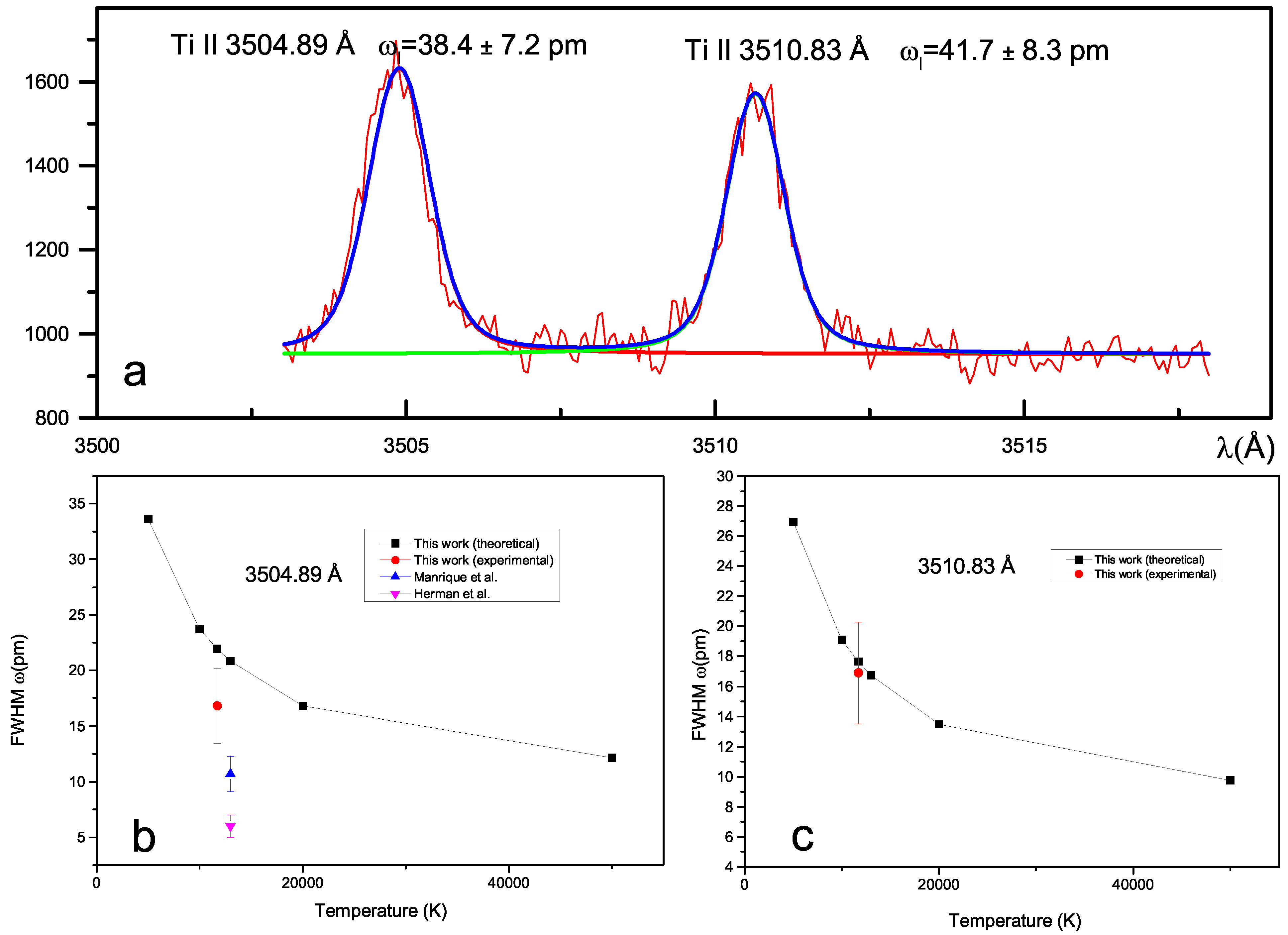
| 3504.89 | 3d4p | 3d4s | 20.83 16.8 * ± 3.6 | −7.45 | 10.7 ± 1.6 | 0.8 ± 0.1 | 6 ± 1 | 0.4 ± 0.3 |
| 3510.83 ** | 3d4p | 3d4s | 16.73 16.9 * ± 3.8 | −5.99 | ||||
| Wavelength | T (103K) | (pm) | ||||
|---|---|---|---|---|---|---|
| This Work | Bibliography | |||||
| (Å) | Reg | Samp | Ref c | Ref d | ||
| 1 | 3075.22 | 5 | 31.6 | 6.48 | ||
| Upper | 3d4p | 10 | 22.35 | 4.58 | ||
| Lower | 3d4s | 13 | 19.60 | 4.02 | 7.27 | 10.35 |
| 20 | 15.8 | 3.24 | ||||
| 50 | 10.01 | 2.3 | ||||
| 2 | 3202.53 | 5 | 71.97 | 14.75 | ||
| 3d4p | 10 | 50.89 | 10.43 | |||
| 3d4s | 20 | 35.99 | 7.38 | |||
| 50 | 22.7 | 5.41 | ||||
| 3 | 3218.26 | 5 | 81.43 | 16.69 | ||
| 3d4p | 10 | 57.58 | 11.8 | |||
| 3d | 13 | 50.50 | 10.35 | 9.18 | ||
| 20 | 40.72 | 8.34 | ||||
| 50 | 25.89 | 6.96 | ||||
| 4 | 3241.98 | 5 | 55.95 | 11.47 | ||
| 3d4p | 10 | 39.56 | 8.11 | |||
| 3d4s | 13 | 34.7 | 7.11 | 7.74 | 9.95 | |
| 20 | 27.97 | 5.73 | ||||
| 50 | 17.69 | 3.63 | ||||
| 5 | 3276.772 | 5 | 42.19 | 8.65 | ||
| 3d4p | 10 | 29.83 | 6.12 | |||
| 3d | 20 | 21.09 | 4.32 | |||
| 50 | 13.38 | 3.27 | ||||
| 6 | 3276.99 | 5 | 70.48 | 0.1445 | ||
| 3d4p | 10 | 49.83 | 0.1022 | |||
| 3d | 20 | 35.31 | 0.0744 | |||
| 50 | 22 | 0.0777 | ||||
| 7 | 3278.288 | 5 | 56.16 | 0.1151 | ||
| 3d4p | 10 | 39.71 | 0.0814 | |||
| 3d4s | 20 | 28.08 | 0.0576 | |||
| 50 | 17.75 | 0.0375 | ||||
| 8 | 3278.92 | 5 | 66.53 | 13.64 | ||
| 3d4p | 10 | 47.04 | 9.64 | |||
| 3d4s | 20 | 33.27 | 6.82 | |||
| 50 | 21.05 | 4.77 | ||||
| 9 | 3318.023 | 5 | 72.3 | 14.82 | ||
| 3d4p | 10 | 51.13 | 10.48 | |||
| 3d | 13 | 44.84 | 9.19 | 9.21 | ||
| 20 | 36.15 | 7.41 | ||||
| 50 | 22.87 | 4.69 | ||||
| 10 | 3409.808 | 5 | 54.78 | 11.23 | ||
| 3d4p | 10 | 38.74 | 7.94 | 12.0 | ||
| 3d4s | 20 | 27.39 | 5.62 | |||
| 50 | 17.32 | 3.57 | ||||
| 11 | 3452.465 | 5 | 30.2 | 6.19 | ||
| 3d4p | 10 | 21.35 | 4.38 | |||
| 3d4s | 20 | 15.1 | 3.1 | |||
| 50 | 9.68 | 2.67 | ||||
| 12 | 3533.854 | 5 | 64.56 | 13.23 | ||
| 3d4p | 10 | 45.65 | 9.36 | |||
| 3d4s | 20 | 32.28 | 6.62 | |||
| 50 | 20.48 | 6.23 | ||||
| 13 | 3535.407 | 5 | 88.12 | 18.06 | ||
| 3d4p | 10 | 62.31 | 12.77 | |||
| 3d4s | 13 | 54.65 | 11.2 | 12.3 | ||
| 20 | 44.06 | 9.03 | ||||
| 50 | 27.96 | 8.66 | ||||
| 14 | 3561.576 | 5 | 97.22 | 19.93 | ||
| 3d4p | 10 | 68.75 | 14.09 | |||
| 3d4s | 20 | 48.64 | 10.07 | |||
| 50 | 30.43 | 9.26 | ||||
| 15 | 3565.96 | 5 | 26.43 | 5.42 | ||
| 3d4p | 10 | 18.69 | 3.83 | |||
| 3d4p | 20 | 13.22 | 2.71 | |||
| 50 | 8.41 | 2.47 | ||||
| 16 | 3706.21 | 5 | 51.93 | 10.65 | ||
| 3d4p | 10 | 36.72 | 7.53 | |||
| 3d | 13 | 32.21 | 6.6 | 12.9 | ||
| 20 | 25.97 | 5.32 | ||||
| 50 | 16.41 | 4.23 | ||||
| 17 | 3741.638 | 5 | 79.79 | 16.36 | ||
| 3d4p | 10 | 56.42 | 11.57 | |||
| 3d | 20 | 39.9 | 8.18 | |||
| 50 | 25.22 | 6.49 | ||||
| 18 | 4307.866 | 5 | 71.71 | 14.7 | ||
| 3d4p | 10 | 50.71 | 10.4 | |||
| 3d | 13 | 44.47 | 9.12 | 14.9 | ||
| 20 | 35.86 | 7.35 | ||||
| 50 | 22.76 | 5.79 | ||||
| 19 | 4316.794 | 5 | 44.46 | 9.12 | ||
| 3d4p | 10 | 31.44 | 6.45 | |||
| 3d4s | 20 | 22.23 | 4.56 | |||
| 50 | 14.1 | 3.3 | ||||
| 20 | 4320.95 | 5 | 41.27 | 8.46 | ||
| 3d4p | 10 | 29.18 | 5.98 | |||
| 3d | 20 | 20.64 | 4.23 | |||
| 50 | 13.1 | 3.51 | ||||
| 21 | 4330.238 | 5 | 73.43 | 15.05 | ||
| 3d4p | 10 | 51.92 | 10.64 | |||
| 3d4s | 20 | 36.72 | 7.53 | |||
| 50 | 23.09 | 5.74 | ||||
| 22 | 4337.91 | 5 | 35.39 | 19.95 | ||
| 3d4p | 10 | 25.03 | 14.11 | |||
| 3d4s | 20 | 17.7 | 10.16 | |||
| 50 | 11.18 | 7.73 | ||||
| 23 | 4421.938 | 5 | 65.3 | 13.39 | ||
| 3d4p | 10 | 46.17 | 9.47 | |||
| 3d4s | 20 | 32.65 | 6.69 | |||
| 50 | 20.7 | 4.45 | ||||
| 24 | 4443.801 | 5 | 134.04 | 27.48 | ||
| 3d4p | 10 | 94.78 | 19.43 | |||
| 3d4s | 13 | 83.1 | 17.19 | 16.3 | ||
| 20 | 67.09 | 13.98 | ||||
| 50 | 41.92 | 13.51 | ||||
| 25 | 4444.554 | 5 | 146.89 | 30.12 | ||
| 3d4p | 10 | 103.87 | 21.29 | |||
| 3d | 20 | 73.56 | 15.46 | |||
| 50 | 45.84 | 16.54 | ||||
| 26 | 4450.482 | 5 | 153.61 | 31.49 | ||
| 3d4p | 10 | 108.62 | 22.27 | |||
| 3d4s | 20 | 76.88 | 15.99 | |||
| 50 | 48.1 | 14.98 | ||||
| 27 | 4468.492 | 5 | 131.05 | 26.86 | ||
| 3d4p | 10 | 92.67 | 19 | |||
| 3d | 20 | 65.64 | 13.84 | |||
| 50 | 40.9 | 14.45 | ||||
| 28 | 4469.151 | 5 | 188.07 | 38.55 | ||
| 3d4p | 10 | 132.98 | 27.26 | |||
| 3d4s | 20 | 94.03 | 19.28 | |||
| 50 | 59.44 | 12.83 | ||||
| 29 | 4488.324 | 5 | 216.77 | 44.44 | ||
| 3d4p | 10 | 153.28 | 31.42 | |||
| 3d4s | 20 | 108.59 | 22.87 | |||
| 50 | 69.46 | 18.08 | ||||
| 30 | 4501.26 | 5 | 117.09 | 24 | ||
| 3d4p | 10 | 82.8 | 16.97 | |||
| 3d | 13 | 72.6 | 15.04 | 18.6 | ||
| 20 | 58.62 | 12.25 | ||||
| 50 | 36.5 | 13.25 | ||||
| 31 | 4518.332 | 5 | 105.42 | 21.61 | ||
| 3d4p | 10 | 74.54 | 15.28 | |||
| 3d4s | 20 | 52.71 | 10.81 | |||
| 50 | 33.32 | 7.29 | ||||
| 32 | 4533.96 | 5 | 113.23 | 23.21 | ||
| 3d4p | 10 | 80.07 | 16.41 | |||
| 3d | 20 | 56.65 | 11.76 | |||
| 50 | 35.74 | 10.44 | ||||
| 33 | 4545.133 | 5 | 158.28 | 32.45 | ||
| 3d4p | 10 | 111.92 | 22.94 | |||
| 3d | 20 | 79.14 | 16.23 | |||
| 50 | 49.96 | 11.77 | ||||
| 34 | 4549.22 | 5 | 214.83 | 44.04 | ||
| 3d4p | 10 | 151.91 | 31.14 | |||
| 3d | 13 | 133.23 | 27.31 | 19.6 | ||
| 20 | 107.41 | 22.02 | ||||
| 50 | 68.5 | 18.93 | ||||
| 35 | 4563.76 | 5 | 74.22 | 15.21 | ||
| 3d4p | 10 | 52.48 | 10.76 | |||
| 3d | 13 | 46.03 | 9.44 | 16.5 | ||
| 20 | 37.15 | 7.81 | ||||
| 50 | 23.76 | 6.29 | ||||
| 36 | 4571.97 | 5 | 174.49 | 35.77 | ||
| 3d4pGo | 10 | 123.38 | 25.3 | |||
| 3d | 13 | 108.21 | 22.18 | 18.7 | ||
| 20 | 87.24 | 17.88 | ||||
| 50 | 55.67 | 15.42 | ||||
| 37 | 4583.409 | 5 | 114.06 | 23.38 | ||
| 3d4pFo | 10 | 80.65 | 16.53 | |||
| 3d | 20 | 57.1 | 11.95 | |||
| 50 | 35.63 | 12.79 | ||||
| 38 | 4589.94 | 5 | 80.37 | 16.47 | ||
| 3d4pDo | 10 | 56.83 | 11.65 | |||
| 3d | 20 | 40.22 | 8.45 | |||
| 50 | 25.73 | 7.07 | ||||
| 39 | 4779.97 | 5 | 51.88 | 10.64 | ||
| 3d4pSo | 10 | 36.68 | 7.52 | |||
| 3d4s | 20 | 25.94 | 5.32 | |||
| 50 | 16.44 | 4.27 | ||||
| 40 | 4805.09 | 5 | 68.9 | 14.12 | ||
| 3d4pSo | 10 | 48.72 | 9.99 | |||
| 3d4s | 20 | 34.45 | 7.06 | |||
| 50 | 21.84 | 5.68 | ||||
| 41 | 4865.61 | 5 | 132.2 | 27.1 | ||
| 3d4pGo | 10 | 93.48 | 19.16 | |||
| 3d | 20 | 66.1 | 13.55 | |||
| 50 | 41.73 | 9.96 | ||||
| 42 | 4874.009 | 5 | 108.34 | 22.21 | ||
| 3d4pPo | 10 | 76.61 | 15.7 | |||
| 3d4s | 20 | 54.31 | 11.55 | |||
| 50 | 34.98 | 10.03 | ||||
| 43 | 4911.194 | 5 | 186.64 | 38.26 | ||
| 3d4pPo | 10 | 131.98 | 27.06 | |||
| 3d4s | 20 | 93.57 | 19.92 | |||
| 50 | 60.41 | 17.52 | ||||
| 44 | 5005.167 | 5 | 98.23 | 20.14 | ||
| 3d4p | 10 | 69.46 | 14.24 | |||
| 3dDo | 20 | 49.11 | 10.07 | |||
| 50 | 31.27 | 7.68 | ||||
| 45 | 5069.09 | 5 | 201.31 | 41.27 | ||
| 3d4pDo | 10 | 142.35 | 29.18 | |||
| 3d4s | 20 | 100.92 | 21.48 | |||
| 50 | 64.68 | 19.91 | ||||
| 46 | 5072.286 | 5 | 249.94 | 51.24 | ||
| 3d4pDo | 10 | 176.74 | 36.23 | |||
| 3d4s | 20 | 125.24 | 26.46 | |||
| 50 | 80.11 | 24.93 | ||||
| 47 | 5154.06 | 5 | 147.29 | 30.2 | ||
| 3d4pDo | 10 | 104.15 | 21.35 | |||
| 3d | 20 | 73.71 | 15.33 | |||
| 50 | 47.11 | 12.01 | ||||
| 48 | 5185.902 | 5 | 300.11 | 61.52 | ||
| 3d4p | 10 | 212.21 | 43.5 | |||
| 3d4s | 20 | 150.06 | 30.77 | |||
| 50 | 95.23 | 23.05 | ||||
| 49 | 5188.68 | 5 | 156.63 | 32.11 | ||
| 3d4p | 10 | 110.75 | 22.7 | |||
| 3d | 20 | 78.38 | 16.29 | |||
| 50 | 50.14 | 12.94 | ||||
| 50 | 5226.54 | 5 | 105.75 | 21.68 | ||
| 3d4p | 10 | 74.77 | 15.33 | |||
| 3d | 20 | 52.93 | 11.11 | |||
| 50 | 33.96 | 9.01 | ||||
| 51 | 5336.786 | 5 | 211.2 | 43.3 | ||
| 3d4pFo | 10 | 149.34 | 30.62 | |||
| 3d | 20 | 105.77 | 22.22 | |||
| 50 | 66.26 | 23.2 | ||||
| 52 | 5381.022 | 5 | 158.84 | 32.56 | ||
| 3d4pFo | 10 | 112.32 | 23.03 | |||
| 3d | 20 | 79.53 | 16.64 | |||
| 50 | 49.75 | 17.46 | ||||
| 53 | 5418.768 | 5 | 169.1 | 34.66 | ||
| 3d4pFo | 10 | 119.57 | 24.51 | |||
| 3d | 20 | 84.65 | 17.69 | |||
| 50 | 53.06 | 18.55 | ||||
| 54 | 9432.151 | 5 | 667.96 | 136.94 | ||
| 3d4pFo | 10 | 472.32 | 96.83 | |||
| 3d | 20 | 334.46 | 70.59 | |||
| 50 | 209.25 | 80 | ||||
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Isidoro-García, L.; de Andrés-García, I.; Porro, J.; Fernández, F.; Colón, C. Experimental and Theoretical Electron Collision Broadening Parameters for Several Ti II Spectral Lines of Industrial and Astrophysical Interest. Atoms 2024, 12, 35. https://doi.org/10.3390/atoms12070035
Isidoro-García L, de Andrés-García I, Porro J, Fernández F, Colón C. Experimental and Theoretical Electron Collision Broadening Parameters for Several Ti II Spectral Lines of Industrial and Astrophysical Interest. Atoms. 2024; 12(7):35. https://doi.org/10.3390/atoms12070035
Chicago/Turabian StyleIsidoro-García, Lucía, Isabel de Andrés-García, Juan Porro, Francisco Fernández, and Cristóbal Colón. 2024. "Experimental and Theoretical Electron Collision Broadening Parameters for Several Ti II Spectral Lines of Industrial and Astrophysical Interest" Atoms 12, no. 7: 35. https://doi.org/10.3390/atoms12070035
APA StyleIsidoro-García, L., de Andrés-García, I., Porro, J., Fernández, F., & Colón, C. (2024). Experimental and Theoretical Electron Collision Broadening Parameters for Several Ti II Spectral Lines of Industrial and Astrophysical Interest. Atoms, 12(7), 35. https://doi.org/10.3390/atoms12070035







