Laser and Astrophysical Plasmas and Analogy between Similar Instabilities
Abstract
1. Introduction
2. Short Outlines of Plasmas Generated by Intense and Ultraintense Lasers
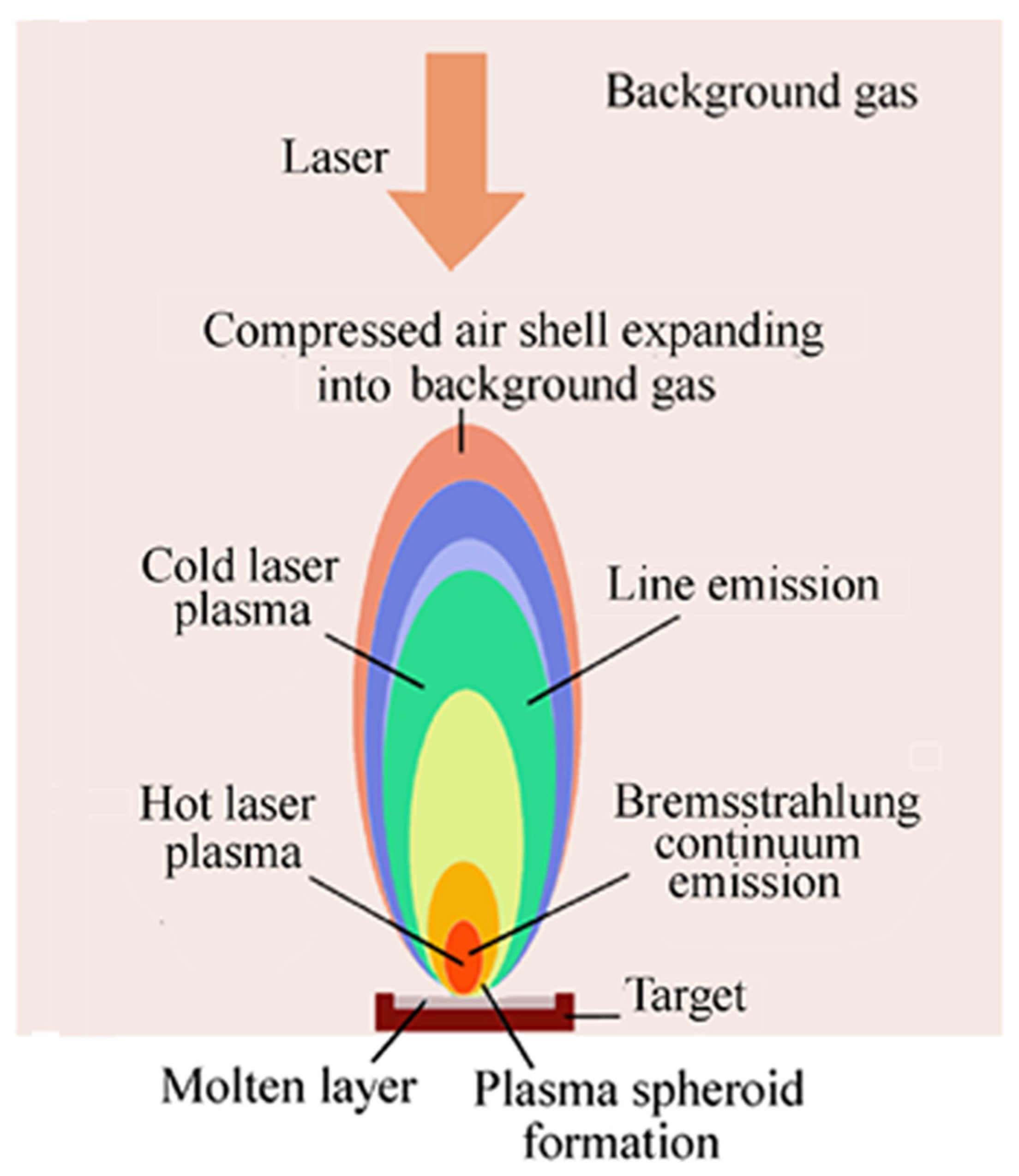
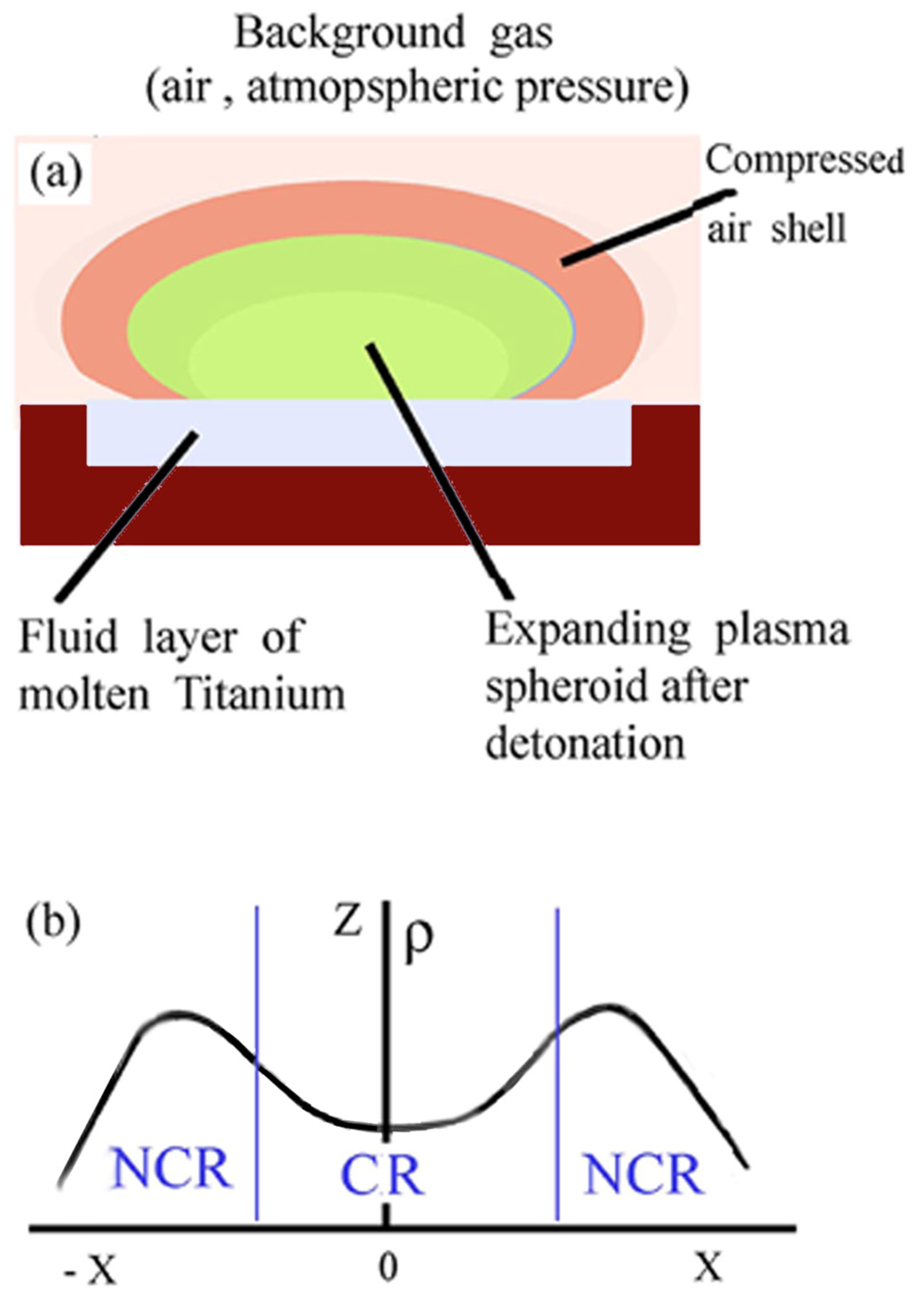
2.1. Plasmas Created by Intense Lasers
2.2. Plasmas Created by Ultraintense Power Lasers
3. Short Outlines of the Experiments
4. Results and Discussion
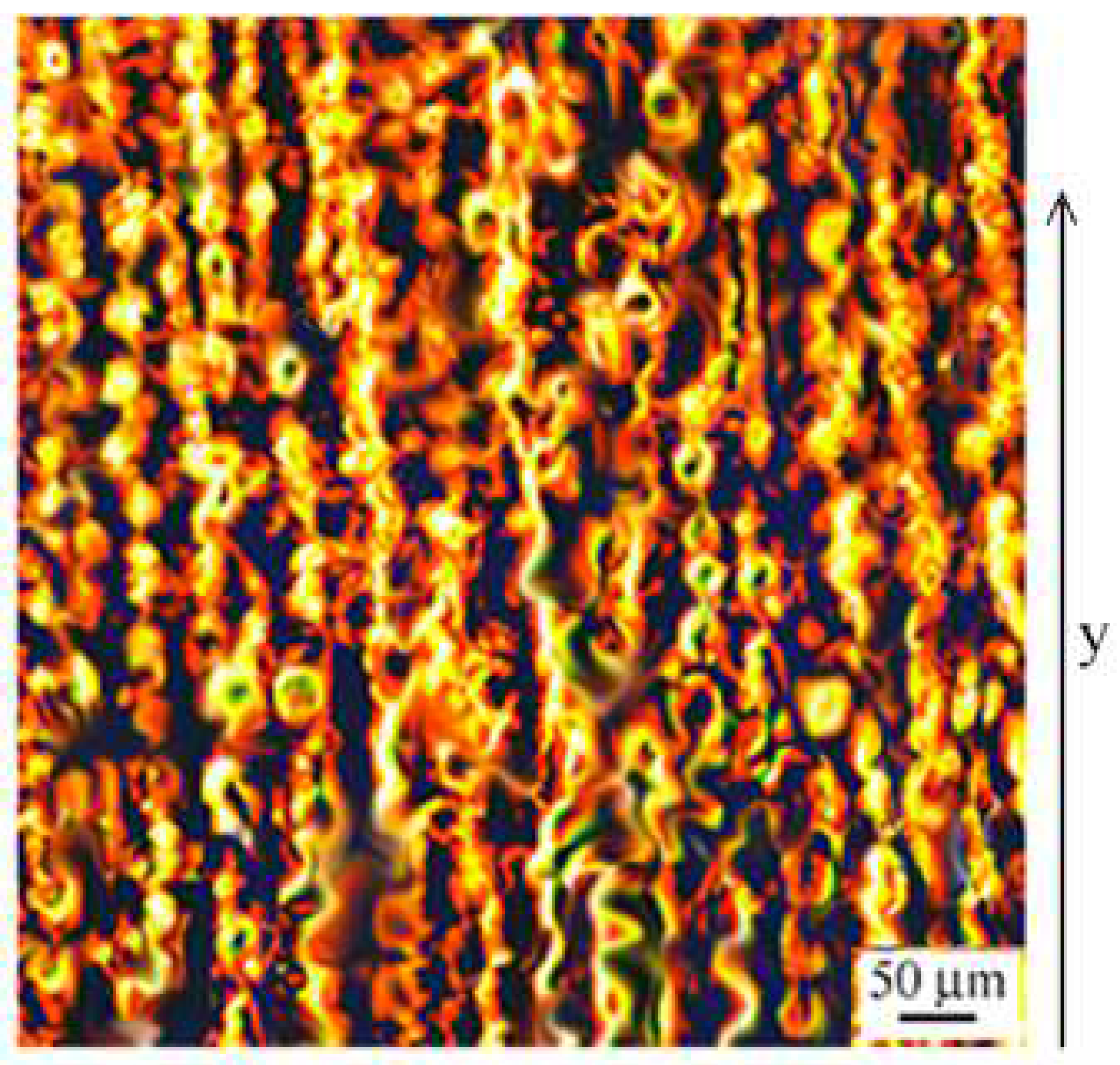
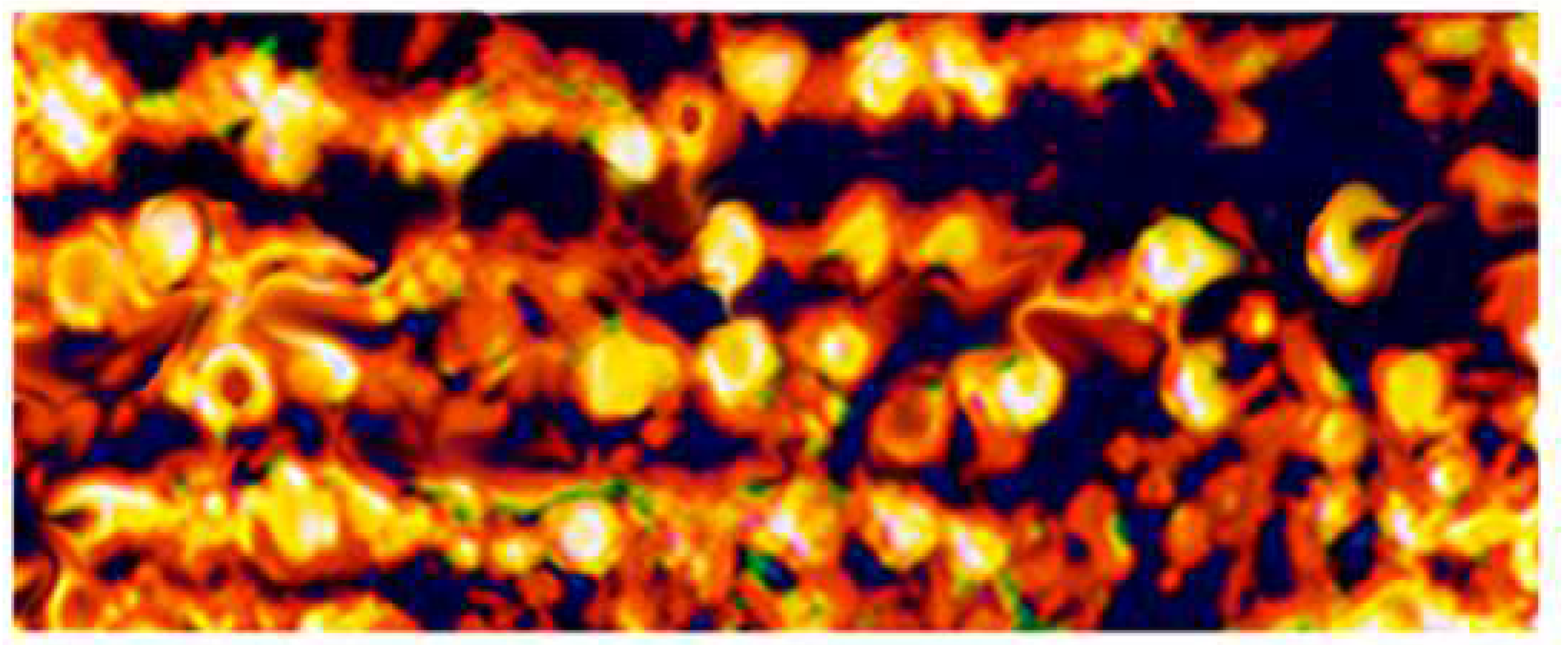
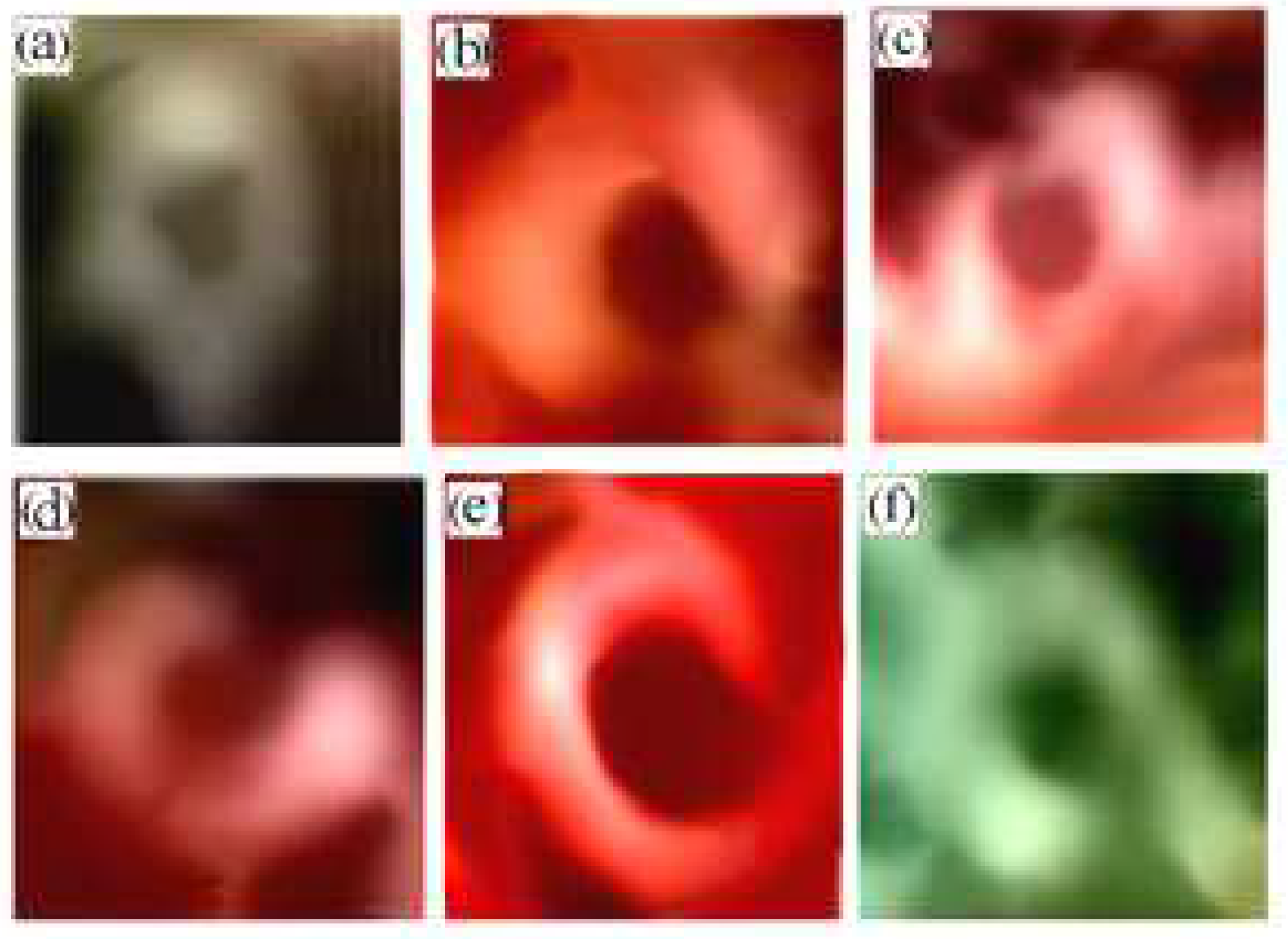
4.1. Vortex Filament Formation in Laser Plasma
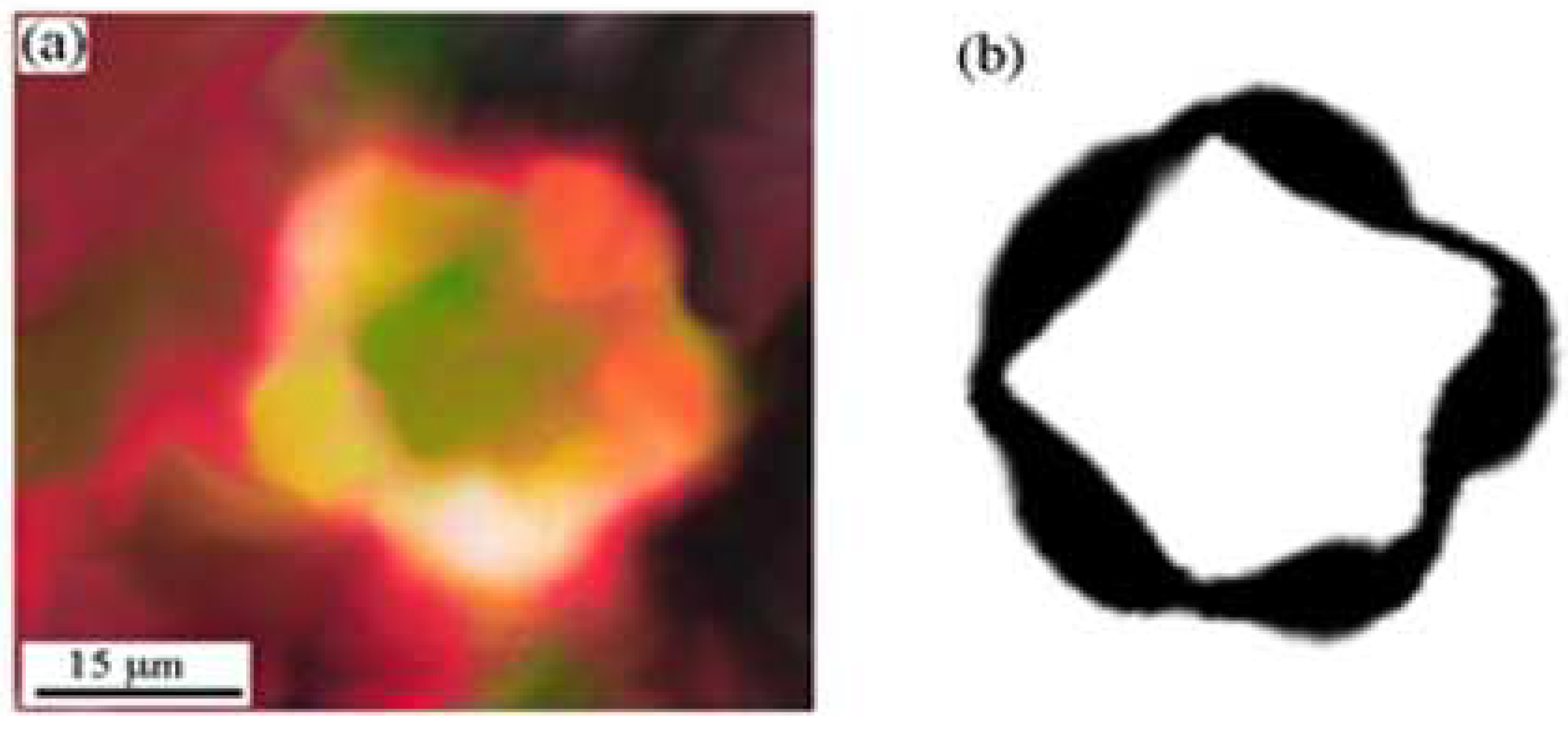
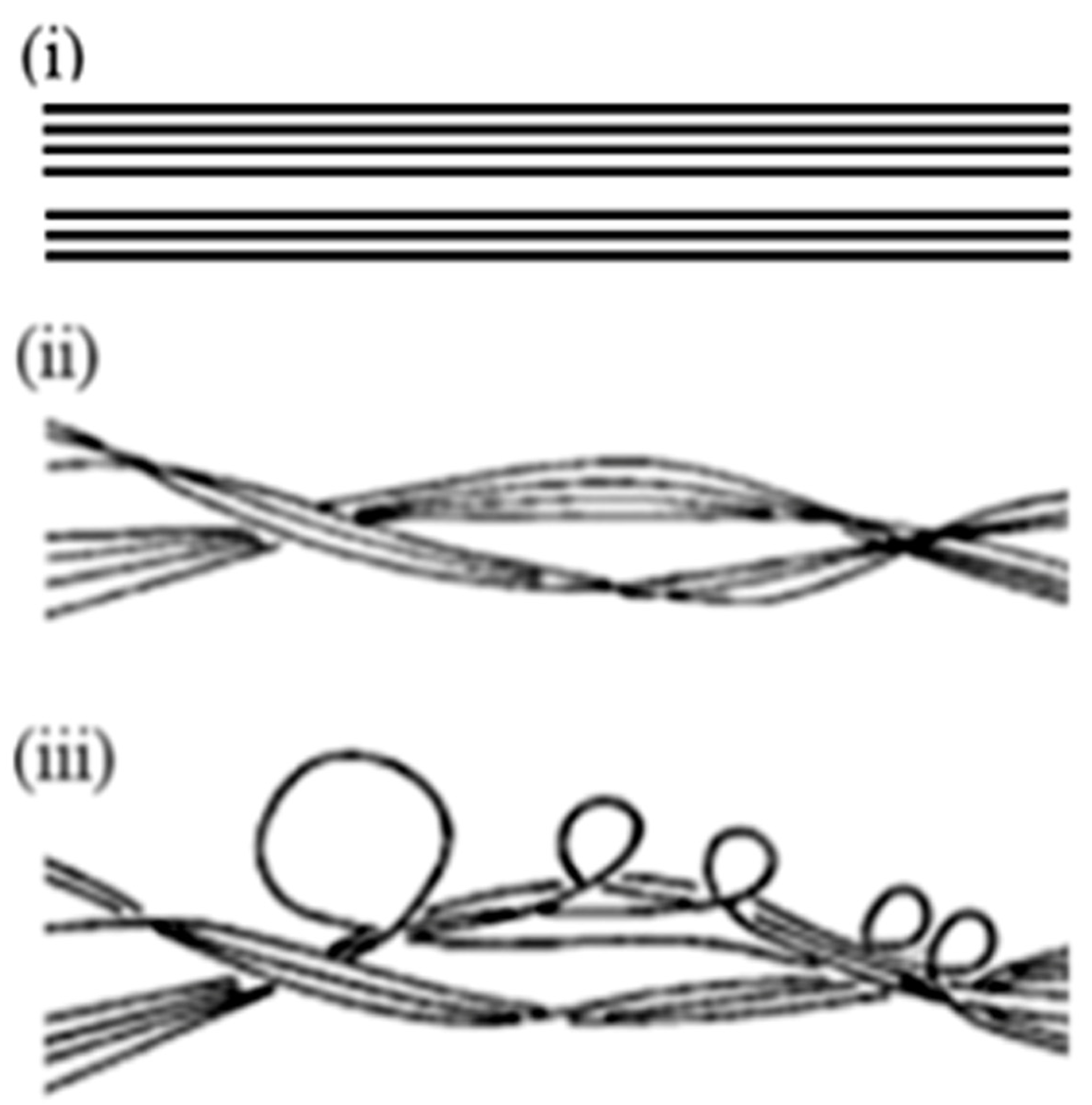
4.1.1. Individual Dynamics of Vortex Filaments
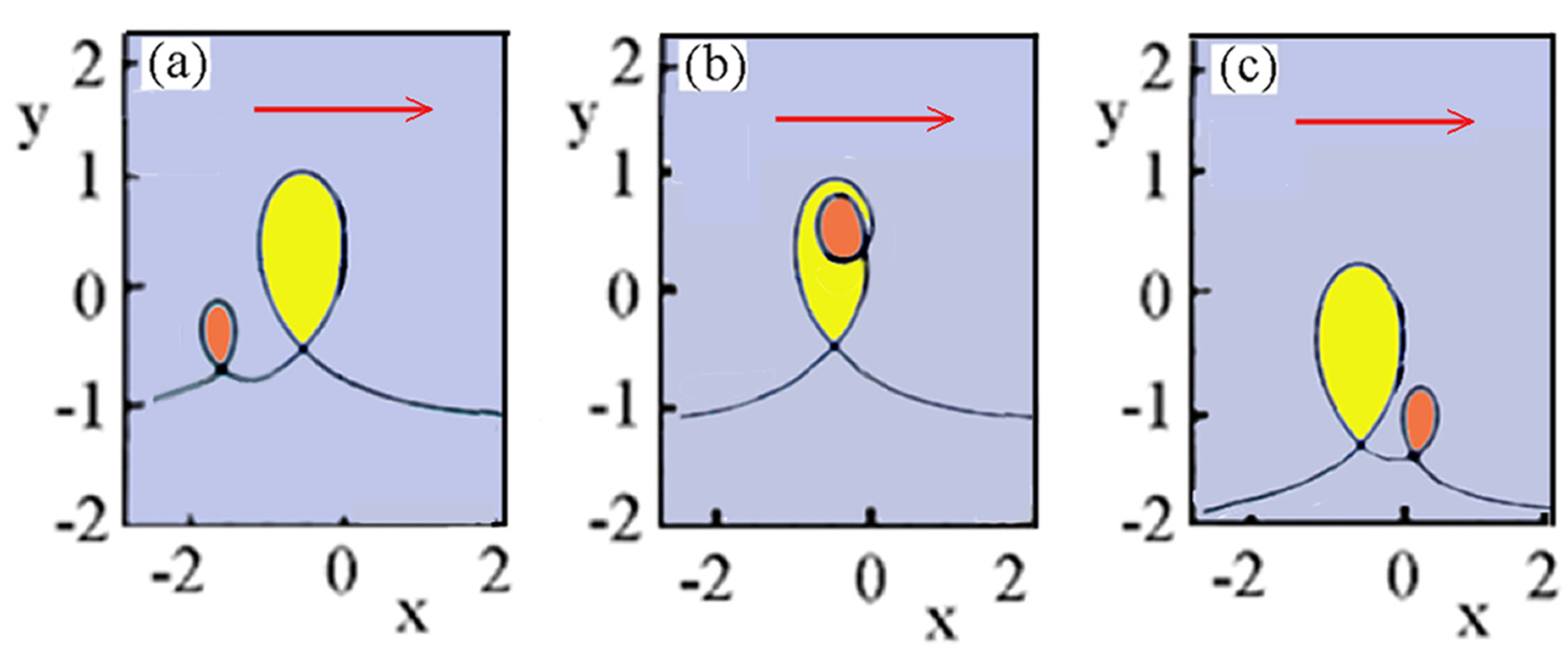
4.1.2. Generation of Loop Solitons on Vortex Filaments
4.1.3. Loop Soliton Chain: Multisolitons
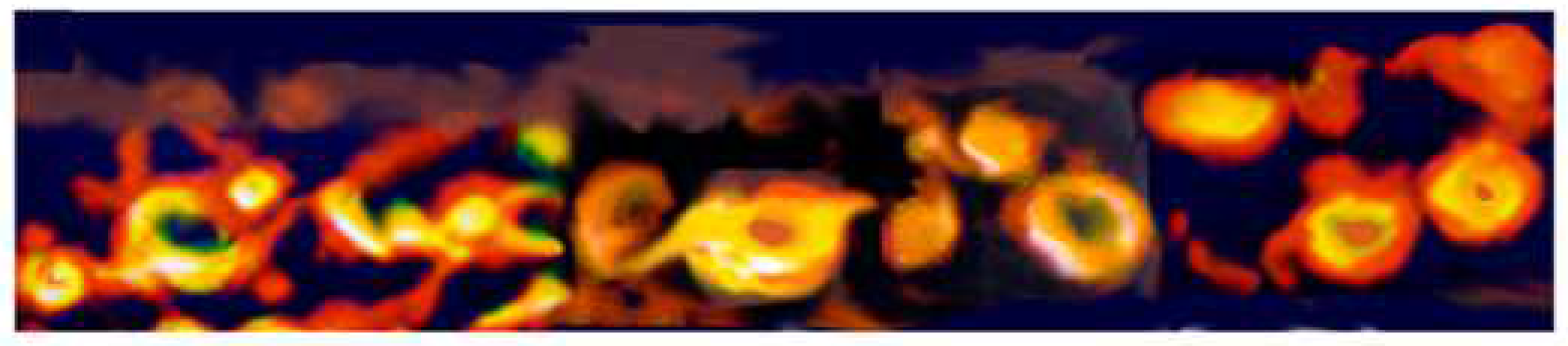
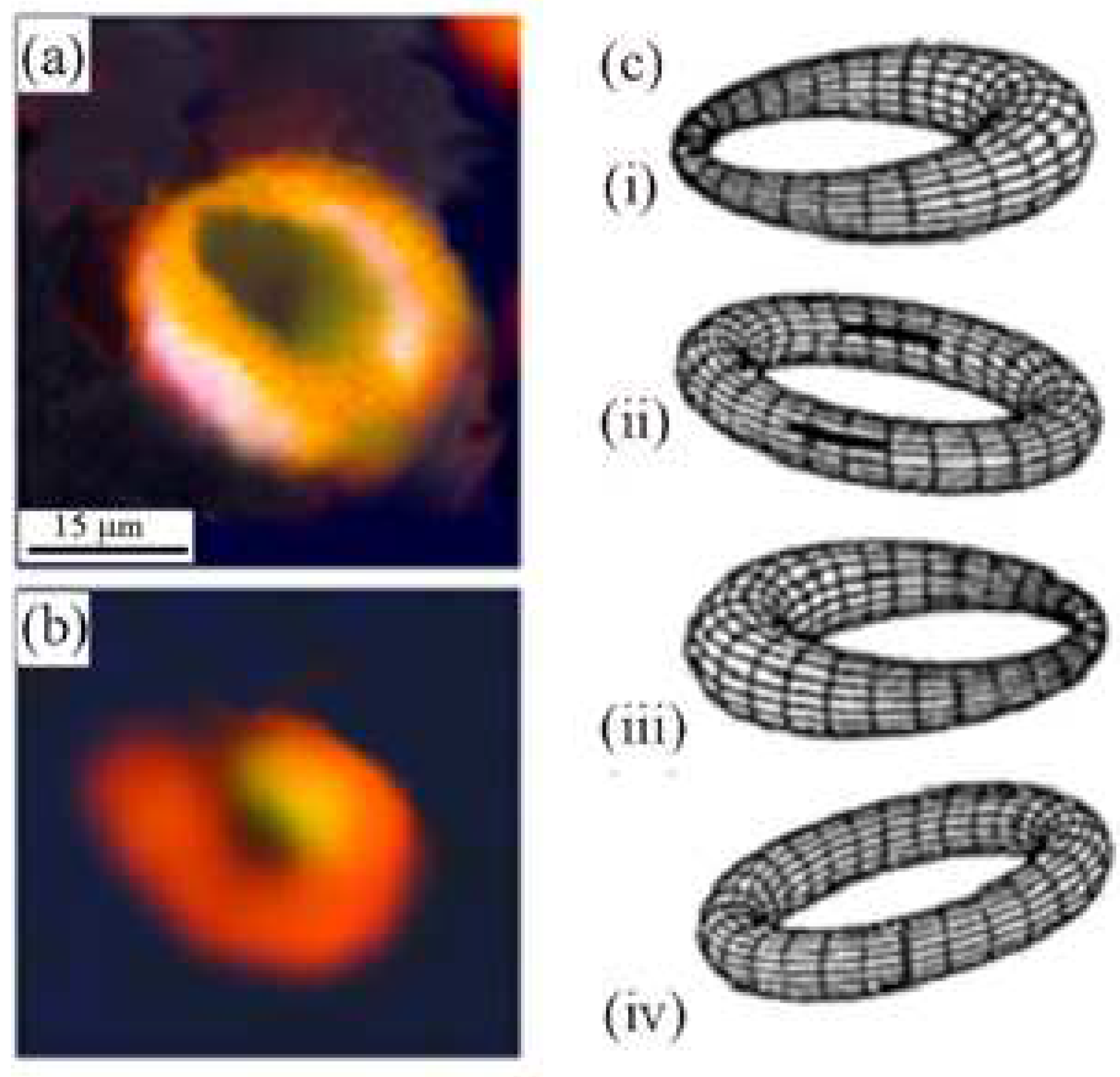
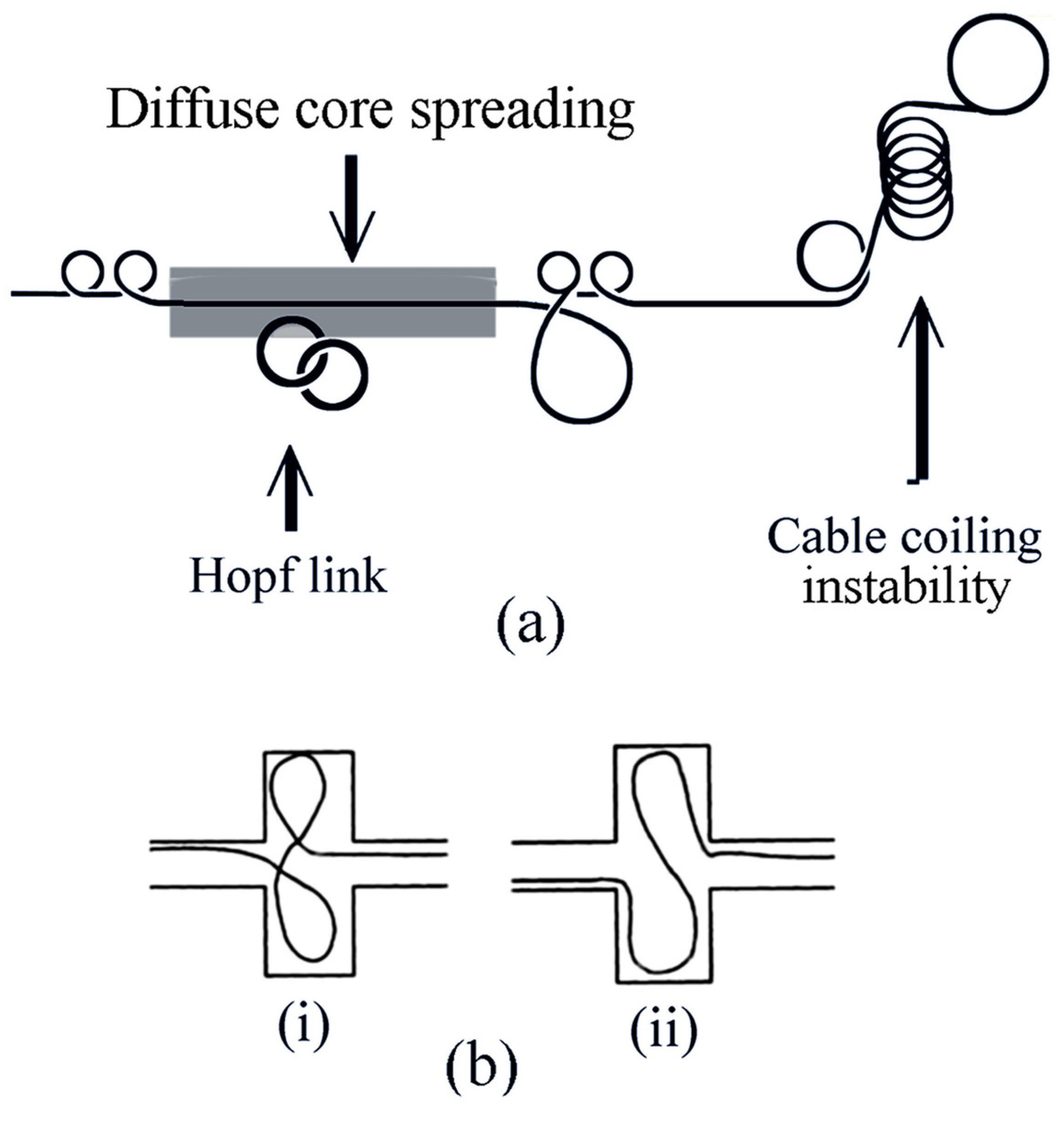
4.1.4. Breakdown of the Loop Soliton Chain: Generation of Vortex Rings and Vortex Ring Instability
4.1.5. Vortex Filament “Complexes”
4.2. Formation of Vortex Filament “Complexes”
5. Basic Aspects of Astrophysical Plasma Systems: Nebulas
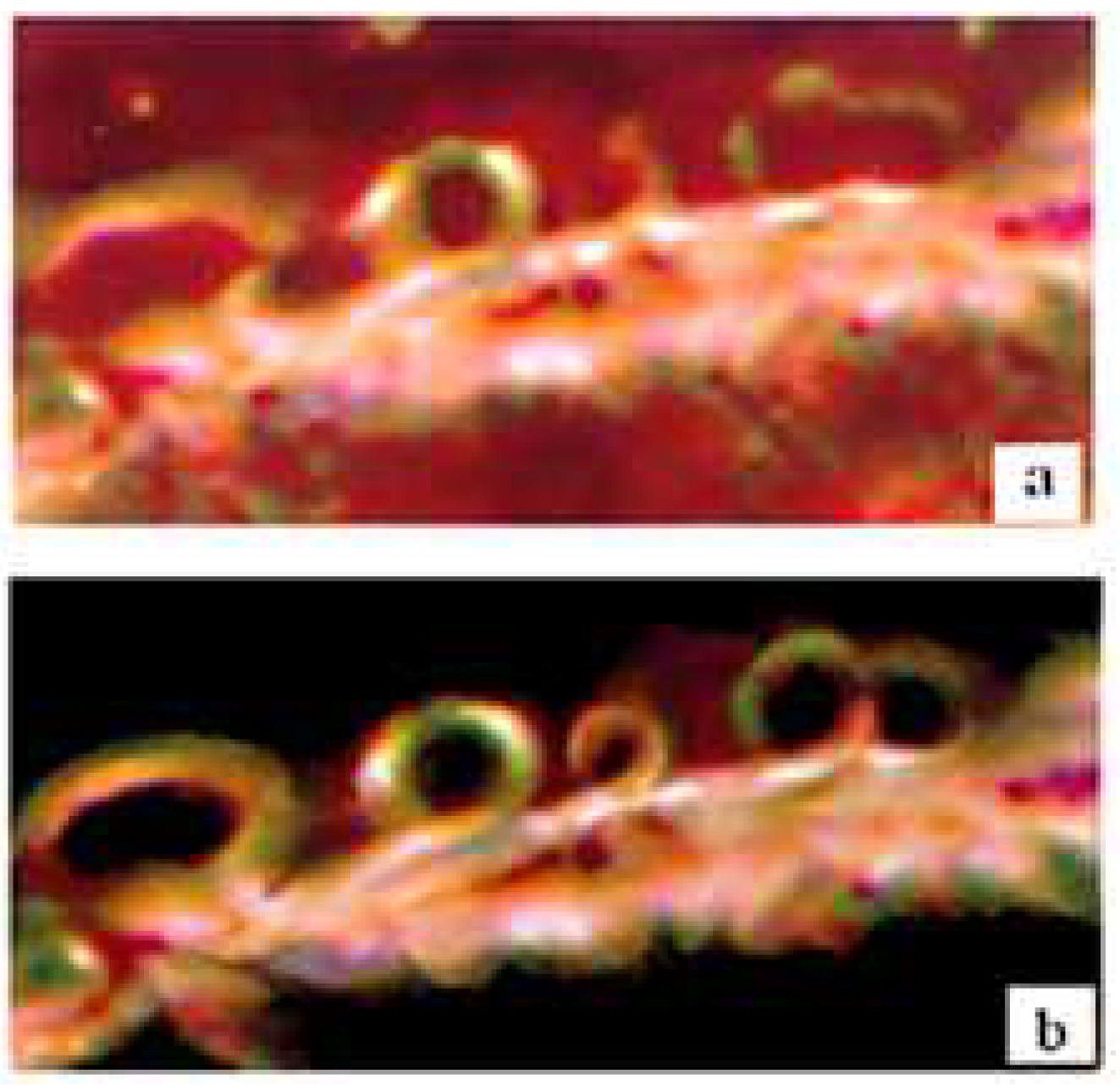
6. Crab Nebula: Filament “Complexes” in the near Periphery Region
6.1. Magnetized Vortex Filaments and Loop Solitons in the Peripheral Region of the Crab Nebula: Weak Magnetic Field
6.2. Vortex Ring Instabilities in the Near Peripheral Region of the Crab Nebula
6.3. Evolution of Rayleigh–Taylor Instability at the PWN/SNR Boundary of the Crab Nebula
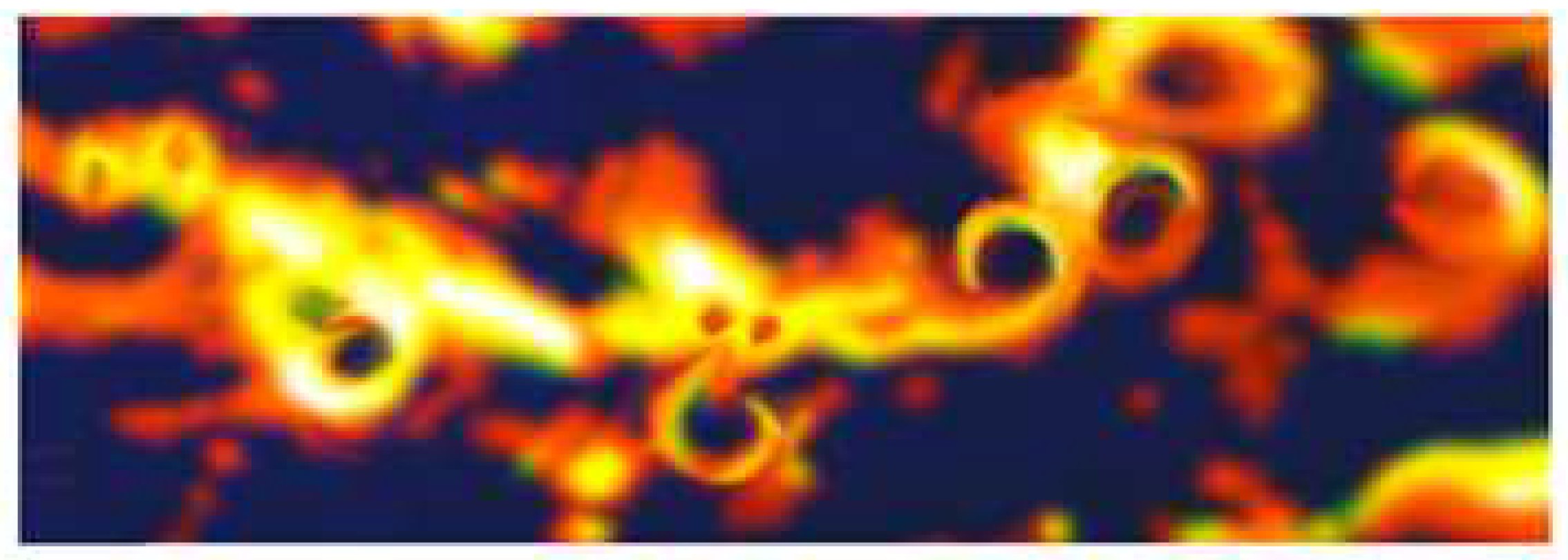
7. The Crab Nebula near Central Region: Formation of Filament “Complexes”
7.1. Bundles of Filaments Helically Paired: Very Strong Magnetic Field
7.2. Vortex Ring Instabilities in the Near Central Region of the Crab Nebula
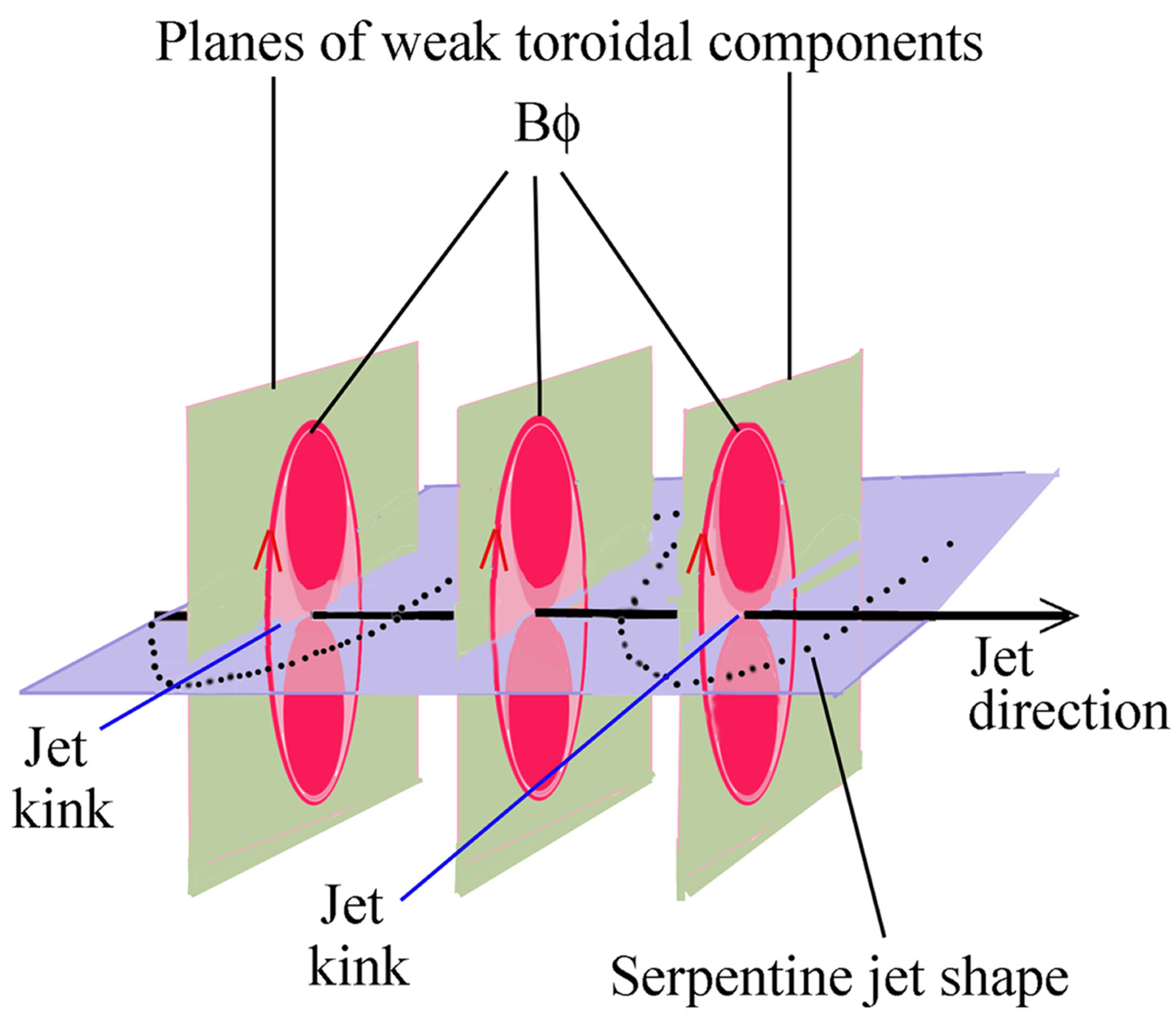
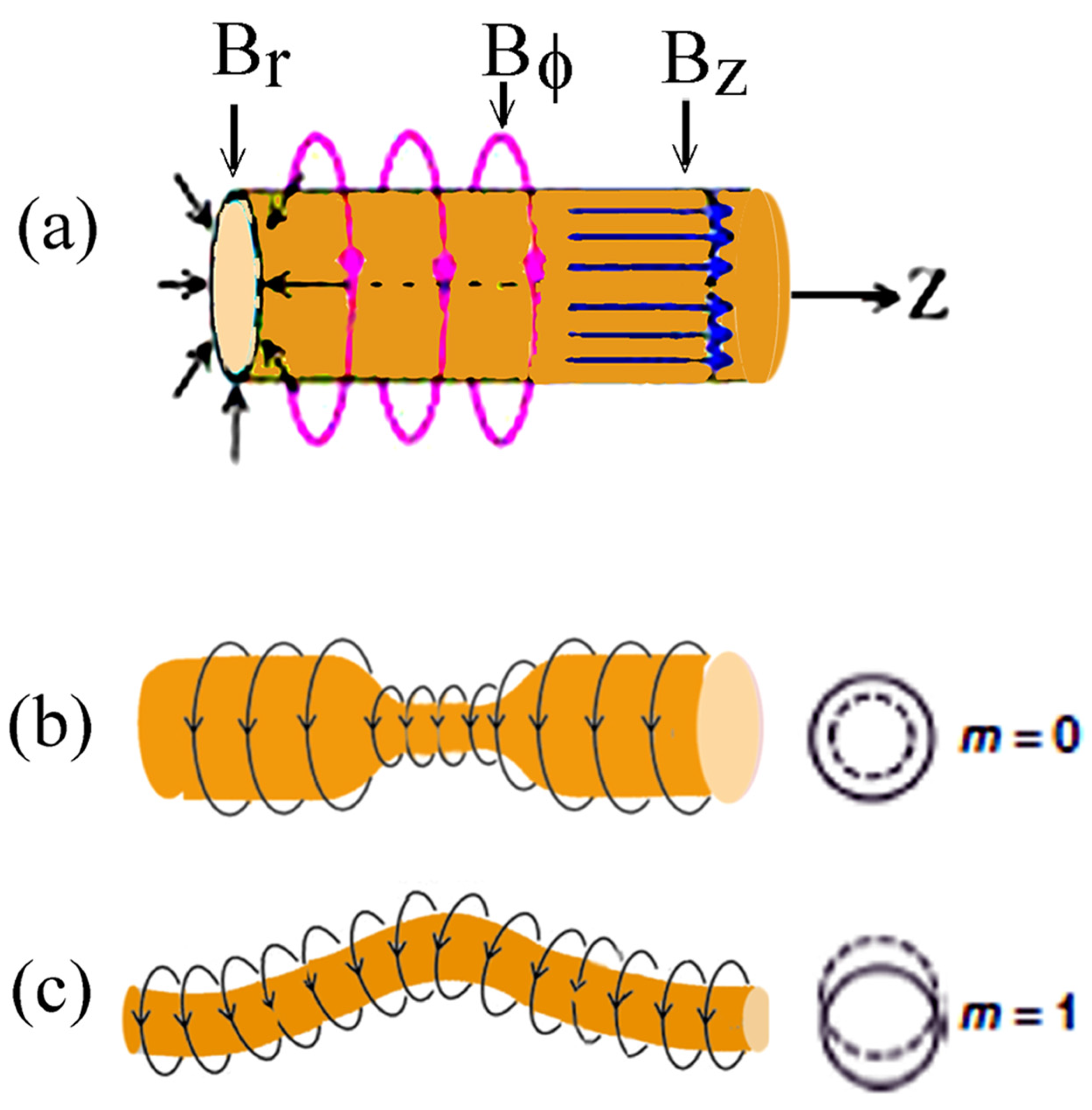
7.3. Simulation of the Kink Instability in Magnetized Jet of the Crab Nebula: Plasma Created by Ultraintense Lasers
8. Double-Helix Nebula: Formation of Filament “Complex”
9. Formation of Complex Filamentary Structures in LMI
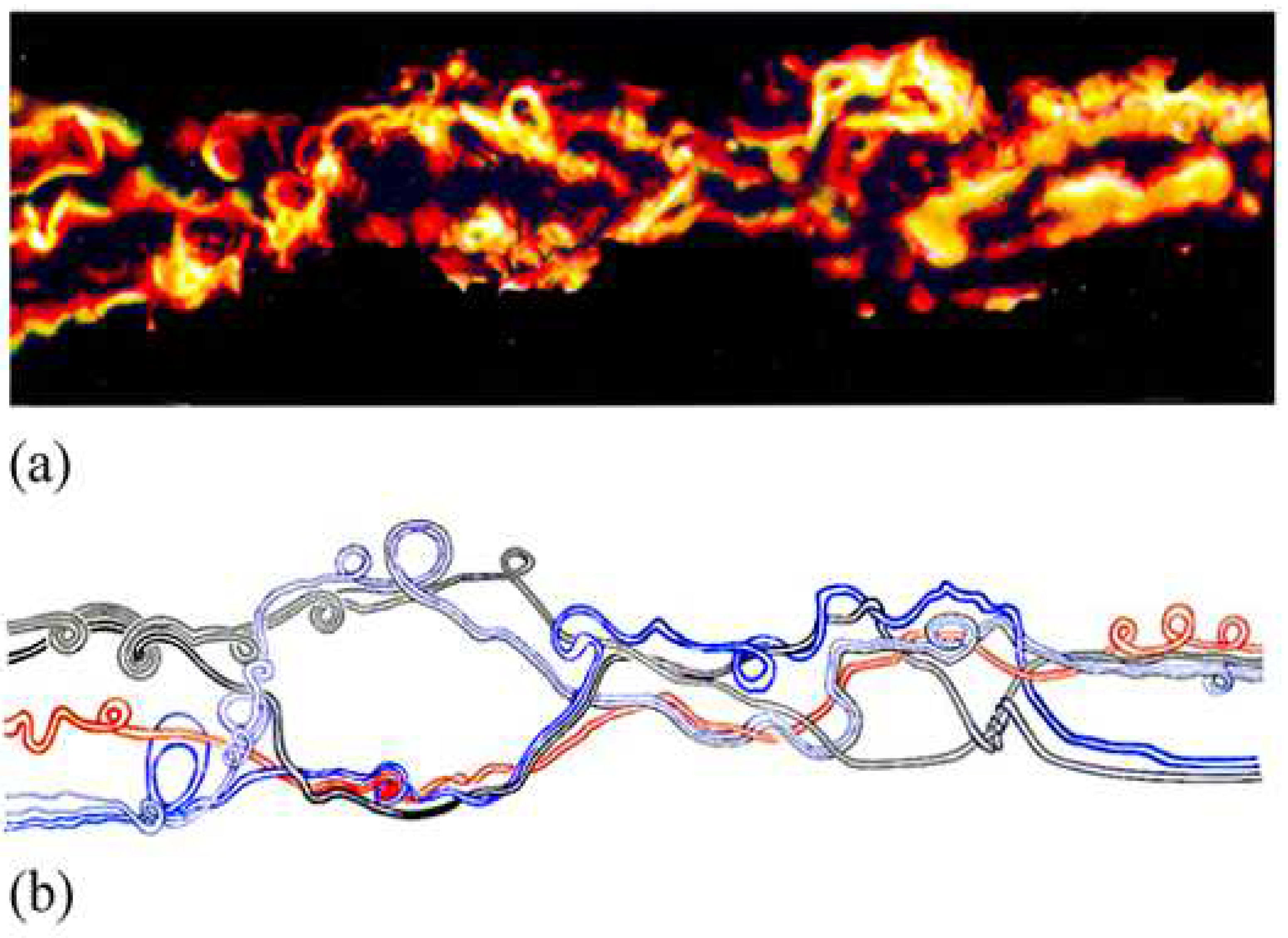
9.1. Helically Paired, Braided, and Tangled Structures of Vortex Filaments in LMI
9.2. Topological Transformations of Braided Vortex Filaments
10. Conclusions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Anisimov, S.I.; Drake, R.P.; Gauthier, S.; Meshkov, E.E.; Abarzhi, S.I. What is certain and what is not so certain in our knowledge of Rayleigh–Taylor mixing? Philos. Trans. R. Soc. A 2013, 371, 20130266. [Google Scholar] [CrossRef]
- Zhou, Y. Rayleigh–Taylor and Richtmyer–Meshkov instability induced flow, turbulence, and mixing. I. Phys. Rep. 2017, 720–722, 1–136. [Google Scholar] [CrossRef]
- Zhou, Y. Rayleigh–Taylor and Richtmyer–Meshkov instability induced flow, turbulence, and mixing. II. Phys. Rep. 2017, 723–725, 1–160. [Google Scholar] [CrossRef]
- Abarzhi, S.I.; Bhomwick, A.K.; Naveh, A.; Pandian, A.; Swisher, N.C.; Stellingwerf, R.F.; Arnett, W.D. Supernova, nuclear synthesis, fluid instabilities, and interfacial mixing. Proc. Natl. Acad. Sci. USA 2017, 116, 18184–18192. [Google Scholar] [CrossRef]
- Li, C.K.; Tzeferacos, P.; Lamb, P.; Gregori, D.; Norreys, G.; Rosenberg, P.; Follett, M.; Froula, R.; Koenig, D.; Seguin, M.; et al. Scaled laboratory experiments explain the kink behavior of the Crab Nebula jet. Nat. Commun. 2016, 7, 13081. [Google Scholar] [CrossRef]
- Gonoskov, A. Ultraintense Laser-Plasma Interaction for Applied and Fundamental Physics. Ph.D. Thesis, UMEA University, Umea, Sweeden, 2014. [Google Scholar]
- Kabashin, A.V.; Delaporte, P.; Grojo, D.; Torres, R.; Sarnet, T.; Sentis, M. A Review on Metal Nanostructures: Preparation Methods and Their Potential Applications. Nanoscale Res. Lett. 2010, 5, 454–463. [Google Scholar] [CrossRef]
- Lorazo, P.; Lewis, L.J.; Meunier, M. Short-pulse laser ablation of solids: From phase explosion to fragmentation. Phys. Rev. Lett. 2003, 91, 225502. [Google Scholar] [CrossRef]
- Rai, V.N.; Thakur, S.N. Ch.4—Physics and dynamics of plasma in laser-induced breakdown spectroscopy. In Laser Induced Breakdown Spectroscopy, 2nd ed.; Elsevier: Amsterdam, The Netherlands, 2020; pp. 71–106. [Google Scholar] [CrossRef]
- Anabitarte, F.; Cobo, A.; Lopez-Higuera, J.M. Laser-Induced Breakdown Spectroscopy: Fundamentals, Applications, and Challenges. Int. Sch. Res. Not. 2012, 2012, 285240. [Google Scholar] [CrossRef]
- Lugomer, S. Laser-Matter Interactions: Surface Self-organization; Profil International, Ed.; Profil International: Zagreb, Croatia, 2001. [Google Scholar]
- Zabusky, N.J.; Lugomer, S.; Zhang, S. Micro-fluid dynamics via laser metal surface interactions: Wave-vortex interpretation of emerging multiscale coherent structures. Fluid Dyn. Res. 2005, 36, 291–299. [Google Scholar] [CrossRef]
- Harillal, S.; Bindhu, C.V.; Tillack, M.S.; Najmabadi, F.; Gaeris, A.C. Internal structure and expansion dynamics of laser ablation plumes into ambient gases. J. Appl. Phys. 2003, 93, 2380–2387. [Google Scholar] [CrossRef]
- Dzhibladze, M.I.; Melikishvili, Z.G.; Berdzenishvili, L.E. The Interaction of Photon Clusters with Matter. Laser Phys. 2000, 10, 727–729. [Google Scholar]
- Ma, Q.I.; Motto-Ros, V.; Boueri, M.; Bai, X.S.; Zheng, L.J.; Zheng, H.P.; Yu, J. Temporal and spatial dynamics of laser-induced Al plasma in Ar background at atmospheric pressure: Interplay with the ambient gas. Spectrocim. Acta Part B 2010, 65, 896–907. [Google Scholar] [CrossRef]
- Matsumoto, J.; Masada, Y. Two-dimensional numerical study for Rayleigh-Taylor and Richtmyer-Meshkov instabilities in relativistic jets. Astrophys. J. 2013, 772, L1. [Google Scholar] [CrossRef]
- Lugomer, S. Laser generated Richtmyer-Meshkov instability and nonlinear wave paradigm in turbulent mixing: I. Central region of Gaussian spot. Laser Part. Beams 2016, 34, 687–704. [Google Scholar] [CrossRef]
- Lugomer, S. Laser generated Richtmyer-Meshkov instability and nonlinear wave paradigm in turbulent mixing: II. Near-Central region of Gaussian spot. Laser Part. Beams 2017, 35, 210–225. [Google Scholar] [CrossRef]
- Lugomer, S. Laser generated Richtmyer-Meshkov and Rayleigh-Taylor instabilities: III. Near/peripheral region of Gaussian spot. Laser Part. Beams 2017, 35, 597–609. [Google Scholar] [CrossRef]
- Boustie, M.; Berthie, L.; de Resseguier, T.; Arrigoni, M. Laser Shock Waves: Fundamentals and Applications. In Proceedings of the 1st International Symposium on Laser Ultrasonics: Science, Technology and Applications, Montreal, QC, Canada, 16–18 July 2008. [Google Scholar]
- Sundarapandian, S. Strong Blast Wave Interaction with Multiphase Media; Technical Report; Royal Institute of Tchnology, Department of Mechanics: Stockholm, Sweden, 2018; Available online: https://www.diva-portal.org/smash/get/diva2:1207069/FULLTEXT02.pdf (accessed on 26 December 2023).
- Devaux, D.; Fabbro, R.; Virmont, J. Generation of shock waves by laser-matter interaction in confined geometries. J. Phys. IV Proc. 1991, 1, C7-179–C7-182. [Google Scholar] [CrossRef][Green Version]
- Petzolt, S.; Reif, J.; Matthias, E. Laser plasma threshold of metals. Appl. Surf. Sci. 1996, 96–98, 199–204. [Google Scholar] [CrossRef]
- Fabro, R.; Fournier, R.; Ballard, P.; Devaux, D.; Virmont, J. Physical study of laser-produced plasma in confined geometry. J. Appl. Phys. 1990, 68, 775–784. [Google Scholar] [CrossRef]
- Raizer, Y.P. Subsonic propagation of alight spark and threshold conditions for the maintenance of plasma by radiation. Sov. Phys. JETP 1970, 31, 1148–1154. [Google Scholar]
- Benselama, A.; Mame, W.-I.; Monnoyer, F. A 1D–3D mixed method for the numerical simulation of blast waves in confined geometries. J. Comput. Phys. 2009, 228, 6796–6810. [Google Scholar] [CrossRef]
- Carter, J.; Pathikonda, G.; Jiang, N.; Felver, J.J.; Ranjan, D. Time-resolved measurements of turbulent mixing in shock driven variable density flows. Nat. Sci. Rep. 2019, 9, 20315. [Google Scholar] [CrossRef] [PubMed]
- Lombardini, M.; Hill, D.J.; Pullin, D.I.; Meiron, D.I. Atwood ratio dependence of Richtmyer–Meshkov flows under reshock conditions using large-eddy simulations. J. Fluid Mech. 2001, 670, 439–480. [Google Scholar] [CrossRef]
- Zhou, Y.; Cabot, W.H. Time-dependent study of anisotropy in Rayleigh-Taylor instability induced turbulent flows with a variety of density ratios. Phys. Fluids 2019, 31, 084106. [Google Scholar] [CrossRef]
- Zabusky, N.J. Vortex paradigm for accelerated inhomogenous flows: Visiometrics foer the Rayleigh-Taylor and Richtmyer-Meshkov instabilities. Annu. Rev. Fluid Mech. 1999, 32, 495–536. [Google Scholar] [CrossRef]
- Schiling, O.; Latini, M.; Don, W.S. Physics of reshock and mixing in single-mode Richtmyer-Meshkov instability. Phys. Rev. E 2007, 76, 026319. [Google Scholar] [CrossRef] [PubMed]
- Schilling, O.; Jacobs, J.F. Richtmyer-Meshkow instability and re-accelerated inhomogenous flows. Scholarpedia 2008, 3, 6090. [Google Scholar] [CrossRef]
- Zhou, Y.; Cabot, W.H.; Thornber, B. Asimptotic behavior of the mixed mass in Rayleigh-Taylor and Richtmyer-Meshkow instabity induced flows. Phys. Plasmas 2016, 23, 052712. [Google Scholar] [CrossRef]
- Banerjee, A. Rayleigh-Taylor instability: A status review of experimental designs and measurement diagnostics. J. Fluids Eng. 2020, 142, 120801. [Google Scholar] [CrossRef]
- Roberts, M.S.; Jacobs, J.W. The effects of forced small-wavelength, finite-bandwidth initial perturbations and miscibility on the turbulent Rayleigh–Taylor instability. J. Fluid Mech. 2015, 787, 50–83. [Google Scholar] [CrossRef]
- Miles, A.R.; Blue, B.; Edwards, M.J.; Greenough, J.A.; Hansen, F.; Robey, H.; Drake, R.P.; Kuranz, C.; Leibrandt, R. Transition to turbulence and effect of initial conditions on 3D compressible mixing in planar blast-wave-driven systems. Phys. Plasmas 2005, 12, 056317. [Google Scholar] [CrossRef]
- Hill, D.L.; Abarzhi, S.I. On the RT unstable dynamics of 3D interfacial coherent structures with time-dependent acceleration. AIP Adv. 2019, 9, 075012. [Google Scholar] [CrossRef]
- Hill, D.L.; Abarzhi, S.I. Richtmyer-Meshkow dynamics with variable acceleration by group theory approach. Appl. Math. Lett. 2020, 105, 106338. [Google Scholar] [CrossRef]
- Bhowmick, K.; Hill, D.L.; Matthews, M.; Abarzhi, S.I. On the fundamentals of Richtmyer Meshkov dynamics with variable acceleration. arXiv 2019, arXiv:1902.01246. [Google Scholar]
- Abarzhi, S.I. Special class od self-similar dynamics for Rayleigh-Taylor mixing with variable acceleration. arXiv 2019, arXiv:1901.04563. [Google Scholar]
- Bhomwick, A.K.; Abarzhi, S.I. Richtmyer-Meshkov unstable dynamics influenced by pressure fluctuation. Phys. Plasmas 2016, 23, 112702. [Google Scholar] [CrossRef]
- Abarzhi, S.I. Coherent structures and pattern formation in Rayleigh-Taylor turbulent mixing. Phys. Scr. 2008, 78, 015401. [Google Scholar] [CrossRef]
- Pandian, A.; Stellingwerf, R.F.; Abarzhi, S.I. Effect of elative phase of waves constituting the initial perturbation and the wave interference in the dynamics of strong-shock-driven Richtmyer-Meshkov flows. Phys. Rev. Fluids 2017, 2, 073903. [Google Scholar] [CrossRef]
- Hoefer, M.; Ablowitz, M. Dispersive shock waves. Scholarpedia 2009, 4, 5562. [Google Scholar] [CrossRef]
- Koepke, M.E. Interrelationship between Lab, Space, Astrophysical, Magnetic Fusion, and Inertial Fusion Plasma Experiments. Atoms 2019, 7, 35. [Google Scholar] [CrossRef]
- Morace, A.; Abe, Y.; Honrubia, J.J.; Iwata, N.; Arikawa, Y.; Nakata, Y.; Johzaki, T.; Yogo, A.; Sentoku, Y.; Mima, K.; et al. Super-strong magnetic field-dominated ion beam dynamics in focusing plasma devices. Sci. Rep. 2022, 12, 6876. [Google Scholar] [CrossRef] [PubMed]
- Theobald, W.; Wũlker, C.; Jasny, J.; Bakos, J.S.; Jethwa, J.; Schaffer, F.P. High-density lithium plasma columns generated by intense subpicosecond KrF laser pulses. Opt. Commun. 1988, 149, 289–295. [Google Scholar] [CrossRef]
- Levescue, J.M. Magnetized, Laser-Driven, Plasma Experiments at Astrophysically Relevant Conditions, and Proton Imaging of Magnetic Fields. Ph.D. Thesis, The University of Michigan, Ann Arbor, MI, USA, 2020. [Google Scholar]
- Stamper, J.A. Review on spontaneous magnetic filelds in laser-produced plasmas: Phenomenaand measurements. Laser Part. Beams 1991, 9, 841. [Google Scholar] [CrossRef]
- Wilson, T.C.; Sheng, Z.-M.; Eliasson, B.; McKenna, P. Magnetic field magnification by high power lasers in underdanse plasma. Plasma Phys. Control. Fusion 2021, 63, 084001. [Google Scholar] [CrossRef]
- Gao, L.; Ji, H.; Fiksel, G.; Fox, W.; Evans, M.; Alfonso, N. Ultrafast proton radiography of the magnetic fields generated by a laser-driven coil current. Phys. Plasmas 2016, 23, 043106. [Google Scholar] [CrossRef]
- Goyon, C.; Pollock, B.B.; Turnbull, D.P.; Hazi, A.; Divol, L.; Farmer, W.A.; Haberberger, D.; Javedani, J.; Johnson, A.J.; Kemp, A.; et al. Ultrafast probng of magnetic field growth inside a laser-diven solenoid. Phys. Rev. E 2017, 95, 033208. [Google Scholar] [CrossRef] [PubMed]
- Santos, J.J.; Bailly-Grandvaux, M.; Giuffrida, L.; Forestier-Colleoni, P.; Fujioka, S.; Zhang, Z.; Korneev, P.; Bouillaud, R.; Dorard, S.; Batani, D.; et al. On the Nature of the Crab Nebula’s Optical Emission. Dokl. Akad. Nauk. SSSR 1953, 90, 983–986. [Google Scholar] [CrossRef]
- Law, K.F.F.; Bailly-Grandvaux, M.; Morace, A.; Sakata, S.; Matsuo, K.; Kojima, S.; Lee, S.; Vaisseau, X.; Arikawa, Y.; Yogo, A.; et al. Direct measurement of kilo-tesla magnetic filed gennerated wia laser-driven capacitor-coil target by proton deflectometry. Appl. Phys. Lett. 2016, 108, 091104. [Google Scholar] [CrossRef]
- Wanger, U.; Wagner, U.; Tatarakis, M.; Gopal, A.; Beg, F.N.; Clark, E.L.; Dangor, A.E.; Evans, R.G.; Haines, M.G.; Mangles, S.P.D.; et al. Laboratory measurements of 0.7 GG magnetic fields generated during high-intensity laser interactions with dense plasmas. Phys. Rev. E 2004, 70, 026401. [Google Scholar] [CrossRef]
- Longman, A.; Fedosejevs, R. Kilo-Tesla axial magnetic field generation with high intensity spin and orbital angular momentum beams. Phys. Rev. Res. 2021, 3, 043180. [Google Scholar] [CrossRef]
- Fukumoto, Y.; Lugomr, S. Instability of Vortex Filaments and Stabilization of Finite Core. Available online: https://repository.kulib.kyoto-u.ac.jp/dspace/bitstream/2433/43208/1/1326_03.pdf (accessed on 26 December 2023).
- Lugomer, S.; Fukumoto, Y. Hierarchical instability of vortex ring array in multipulse laser-matter interactins. Fluid Dyn. Res. 2005, 36, 277–290. [Google Scholar] [CrossRef]
- Fukumoto, Y.; Lugomer, S. Instability od vortex filaments in laser-matter interactions. Phys. Lett. 2003, 308, 375–380. [Google Scholar] [CrossRef]
- Lugomer, S.; Fukumoto, Y. Generation of ribbons, helicoids, and complex Scherk surfaces in laser-matter interactions. Phys. Rev. E 2010, 81, 036311. [Google Scholar] [CrossRef] [PubMed]
- Lugomer, S.; Fukumoto, Y. Supercomplex network of bistable coiled vortex filaments and ribbons in laser-matter interactions. J. Mod. Appl. Phys 2018, 2, 1–9. [Google Scholar]
- Lugomer, S. Micro-fluid dynamics via laser–matter interaction: Vortex filament structures, helical instability, reconnection, merging, and undulation. Phys. Lett. A 2007, 361, 87–97. [Google Scholar] [CrossRef]
- Collis, S.S.; Lele, S.K.; Moser, R.D.; Rogers, N.M. The evolution of a plane mixing layer with spanwise nonuniform forcing. Phys. Fluids 1994, 6, 381–396. [Google Scholar] [CrossRef]
- Wu, J.-Z.; Ma, H.Y.; Zhou, M.-D. Vorticity and Vortex Dynamics; Springer: Berlin/Heidleberg, Germany, 2006. [Google Scholar]
- Nakayama, K.; Segur, H.; Wadati, M. Integrability and the motion of curves. Phys. Rev. Lett. 1992, 69, 2603–2606. [Google Scholar] [CrossRef] [PubMed]
- Maksimovic, A.; Lugomer, S.; Michieli, I. Multisolitons on vortex filaments: The origin of axial tangling. J. Fluids Struct. 2003, 17, 317–330. [Google Scholar] [CrossRef]
- Hereman, W.; Zhuang, W. Symbolic computation of solitons with Macsyma. In Proceedings of the 13th IMACS World Congress Dublin, Dublin, Ireland, 22–26 July 1991; Ames, W.F., van der Houwen, P.J., Eds.; pp. 287–296. [Google Scholar]
- Fukumoto, Y.; Moffatt, H.K. Motion and expansion of a viscous vortex ring. Part 1. A higher-order asymptotic formula for the velocity. J. Fluid Mech. 2000, 417, 1–45. [Google Scholar] [CrossRef]
- Fukumoto, Y.; Hattori, Y. Curvature instability of a vortex ring. J. Fluid Mech. 2005, 526, 77–115. [Google Scholar] [CrossRef]
- Fukumoto, Y. The three-dimensional instability of a strained vortex tube revisited. J. Fluid Mech. 2003, 493, 287–318. [Google Scholar] [CrossRef]
- Fukumoto, Y.; Hattori, Y. Linear stability of a vortex ring revisited. In Proceedings of the IUTAM Symposium on Tubes, Sheets and Singularities in Fluid Dynamics, Zakopane, Poland, 2–7 September 2001; Moffatt, H.K., Bajer, K., Eds.; Kluwer Academic Publishers: Dordrecht, The Netherlands, 2002; pp. 37–48. [Google Scholar]
- Hattori, Y.; Fukumoto, Y. Short-wavelength stability analysis of thin vortex rings. Phys. Fluids 2003, 15, 3151–3163. [Google Scholar] [CrossRef]
- Widnall, S.E.; Bliss, D.B.; Tsai, C.-Y. The instability of short waves on a vortex ring. J. Fluid Mech. 1974, 66, 35–47. [Google Scholar] [CrossRef]
- Widnal, S.E.; Tsai, C.Y. The instability of the thin vortex ring of constant vorticity. Philos. Trans. R. Soc. Lond. A 1977, 287, 273–305. [Google Scholar]
- Kop’ev, V.F.; Chernyshev, S.A. Vortex ring oscillations, the development of turbulence in vortex rings and generation of sound. Phys. Uspekhi 2000, 43, 663–690. [Google Scholar] [CrossRef]
- Abarzhi, S.I.; Goddard, W.A., III. Interfaces and mixing: Nonequilibrium transport across the scales. Proc. Natl. Acad. Sci. USA 2019, 116, 18171–18174. [Google Scholar] [CrossRef] [PubMed]
- Abarzhi, S.I.; Hill, D.L.; Williams, K.C.; Li, J.T.; Remington, B.A.; Arnett, W.D. Fluid Dynamic Mathematical Aspects of Supernova Remnants. Phys. Fluids 2023, 35, 034106. [Google Scholar] [CrossRef]
- Hester, J.J.; Loll, A. The Crab Nebula, Hubble’s Messier Catalog, Messier 1, NASA. 2017. Available online: https://science.nasa.gov/mission/hubble/science/explore-the-night-sky/hubble-messier-catalog/messier-1/ (accessed on 26 December 2023).
- Shklovskii, I. On the Nature of the Crab Nebula’s Optical Emission. Dokl. Akad. Nauk. SSSR 1953, 90, 983. [Google Scholar]
- Burn, B.J. A synchrotron model for the continuum spectrum of the Crab Nebula. Mon. Not. R. Astron. Soc. 1973, 165, 421–429. [Google Scholar] [CrossRef][Green Version]
- Hester, J.J. The Crab Nebula: An Astrophysical Chimera. Annu. Rev. Astron. Astrophys. 2008, 46, 127–155. [Google Scholar] [CrossRef]
- Fesen, R.A.; Kirshner, R.P. The Crab Nebula. I—Strophotometry of the filaments. Astrophys. J. 1982, 258, 1–10. [Google Scholar] [CrossRef]
- Dubner, G.; Castelletti, G.; Kargaltsev, O.; Pavlov, G.G.; Bietenholz, M.; Talavera, A. Morphological Properties of the Crab Nebula: A Detailed Multiwavelength Study Based on New VLA, HST, Chandra, and XMM-Newton Images. Astrophys. J. 2017, 840, 82. [Google Scholar] [CrossRef]
- Mignone, A.; Striani, E.; Tavani, M.; Ferrari, A. Modelling the kink jet of the Crab Nebula. Mon. Not. R. Astron. Soc. 2013, 436, 1102–1115. [Google Scholar] [CrossRef]
- Lawrence, S.S.; MacAlpine, G.M.; Uomoto, A.; Woodgate, B.; Brown, L.W.; Oliversen, R.J.; Lowenthal, J.D.; Liu, C. Three-dimensional Fabry-Perot Imaging Spectroscopy of the Crab Nebula, Cassiopeia A, and Nova GK Persei. Astron. J. 1995, 109, 2635. [Google Scholar] [CrossRef]
- Kuranz, C.C.; Drake, R.P.; Grosskopf, M.J.; Drake, R.P.; Grosskopf, M.J.; Drake, R.P.; Grosskopf, M.J.; Fryxell, B.; Budde, A.; Hansen, J.F.; et al. Spike morphology in blast-wave-driven instability experiments. Phys. Plasmas 2020, 17, 052709. [Google Scholar] [CrossRef]
- Kuranz, C.C.; Park, H.-S.; Huntington, C.M.; Miles, A.R.; Remington, B.A.; Plewa, T.; Trantham, M.R.; Robey, H.F.; Shvarts, D.; Shimony, A.; et al. How high energy fluxes may affect Rayleigh–Taylor instability growth in young supernova remnants. Nat. Commun. 2018, 9, 1564. [Google Scholar] [CrossRef] [PubMed]
- Bietenholz, M.F.; Kronbberg, P.P. The Magnetic field of the Crab nebula and the nature of its “jet”. Astrophys. J. 1990, 357, L13–L16. [Google Scholar] [CrossRef]
- Tandberg-Hanssen, E. The Physics of Solar Flares; Cambridge University Press: Cambridge UK, 2009; Digitally Printed Version. [Google Scholar]
- Gaensler, B.M.; Slane, P.O. The evolution and structure of pulsar wind nebulae. Ann. Rev. Astron. Astrophys. ARA&A 2006, 44, 17–47. [Google Scholar] [CrossRef]
- Reynolds, S.P.; Gaensler, B.M.; Bocchino, F. Magnetic fields in supernova remnants and pulsar—Wind nebulae. Space Sci. Rev. 2012, 166, 231–261. [Google Scholar] [CrossRef]
- Mukherjee, D.; Bult, P.; van der Klis, M.; Bhattacharya, D. The magnetic-field strengths of accreting millisecond pulsars. Mon. Not. R. Astron. Soc. 2015, 452, 3994–4012. [Google Scholar] [CrossRef]
- Flowers, E.; Ruderman, M.A. Evolution of pulsar magnetic fields. Astrophys. J. 1977, 215, 302–310. [Google Scholar] [CrossRef]
- Hester, J.J.; Scowen, P.A.; Sankrit, R.; Lauer, T.R.; Ajhar, E.A.; Baum, W.A.; Code, A.; Currie, D.G.; Danielson, G.E.; Ewald, S.P.; et al. Hubble space telescope WFPC2 imaging of M16: Photoevaporation and emerging of young stellar objects. Astron. J. 1996, 111, 2349. [Google Scholar] [CrossRef]
- Lapenta, G.; Kronberg, P.P. Simultion of astrophysical jets: Collimation and expansion into radio lobes. Astron. J. 2005, 625, 37–50. [Google Scholar] [CrossRef]
- Andrade, L.C.G. Torsion effects on vortex filaments and Hasimoto soliton transformation in magnetar. arXiv 2005, arXiv:astro-ph/0509847. [Google Scholar]
- Ricca, R. Inflexional disequilibrium of magnetic flux-tubes. Fluid Dyn. Res. 2005, 36, 319. [Google Scholar] [CrossRef]
- Ricca, R. The effect of torsion on the motion pf a helical vortex filament. J. Fluid Mech. 1994, 237, 241. [Google Scholar] [CrossRef]
- Gull, T.R.; Fesen, R.A. Deep optical imagery of the Crab Nebula’s jet. Astrophys. J. 1982, 260, L75. [Google Scholar] [CrossRef]
- Sankrit, R.; Hester, J.J. The shock and extended remnant around the Crab Nebula. Astrophys. J. 1997, 491, 796–807. [Google Scholar] [CrossRef]
- Chevalier, R.A.; Gull, T.R. The outer structure of the Crab nebula. Astrophys. J. 1975, 200, 399–401. [Google Scholar] [CrossRef]
- Chevalier, R.A.; Fransson, C. Pulsar Nebulae in Supernovae. Astrophys. J. 1992, 395, 540–552. [Google Scholar] [CrossRef]
- Porth, O.; Komissarov, S.S.; Keppens, R. Rayleigh–Taylor instability in magnetohydrodynamic simulations of the Crab nebula. Mon. Not. R. Astron. Soc. 2014, 443, 547–558. [Google Scholar] [CrossRef]
- Clark, D.H.; Murdin, P.; Wood, R.; Gilmozzi, R.; Danziger, J.; Furr, A.W. Three-dimensional structure of the Crab Nebula. Mon. Not. R. Astron. Soc. 1983, 204, 415. [Google Scholar] [CrossRef]
- Becker, L.E.; Shelly, M.J. Instability of Elastic Filaments in Shear Flow Yields First-Normal-Stress Differences. Phys. Rev. Lett. 2001, 87, 198301. [Google Scholar] [CrossRef]
- Morris, M.; Uchida, K.; Do, T. A magnetic torsional wave near the Galactic Centre traced by a ‘double helix nebula’. Nature 2006, 440, 308–310. [Google Scholar] [CrossRef] [PubMed]
- Hildebrand, R.H.; Davidson, J.A.; Dotson, J.; Figer, D.F.; Novak, G.; Platt, S.R.; Tao, L. Polarization of the thermal emission from the dust ring at the center of the Galaxy. Astrophys. J. 1993, 417, 565–571. [Google Scholar] [CrossRef]
- Wardle, M.; Königl, A. A model for the magnetic field in the molecular disk at the Galactic center. Astrophys. J. 1990, 362, 120–134. [Google Scholar] [CrossRef]
- Novak, G.; Dotson, J.L.; Dowell, C.D.; Hildebrand, R.H.; Renbarger, T.; Schleuning, D.A. Submillimeter polarimetric observations of the galactic center. Astrophys. J. 2000, 529, 241–250. [Google Scholar] [CrossRef]
- Tufillaro, N.B.; Abott, T.; Reilly, J. An Experimental Approach to Nonlinear Dynamics and Chaos; Adison-Wesley Rub. Co.: New York, NY, USA; Amsterdam, The Netherlands, 1992. [Google Scholar]
- Lugomer, S. Braids of vortex filaments generated by laser on metal surface. Phys. Lett. A 1999, 259, 479–487. [Google Scholar] [CrossRef]
- Ricca, R.L.; Berger, M.A. Topological ideas and fluid mechanics. Phys. Today 1996, 49, 28–34. [Google Scholar] [CrossRef]
















Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the author. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Lugomer, S. Laser and Astrophysical Plasmas and Analogy between Similar Instabilities. Atoms 2024, 12, 23. https://doi.org/10.3390/atoms12040023
Lugomer S. Laser and Astrophysical Plasmas and Analogy between Similar Instabilities. Atoms. 2024; 12(4):23. https://doi.org/10.3390/atoms12040023
Chicago/Turabian StyleLugomer, Stjepan. 2024. "Laser and Astrophysical Plasmas and Analogy between Similar Instabilities" Atoms 12, no. 4: 23. https://doi.org/10.3390/atoms12040023
APA StyleLugomer, S. (2024). Laser and Astrophysical Plasmas and Analogy between Similar Instabilities. Atoms, 12(4), 23. https://doi.org/10.3390/atoms12040023





