Commissioning of the HITRAP Cooling Trap with Offline Ions
Abstract
1. Introduction
2. Materials and Methods
3. Results
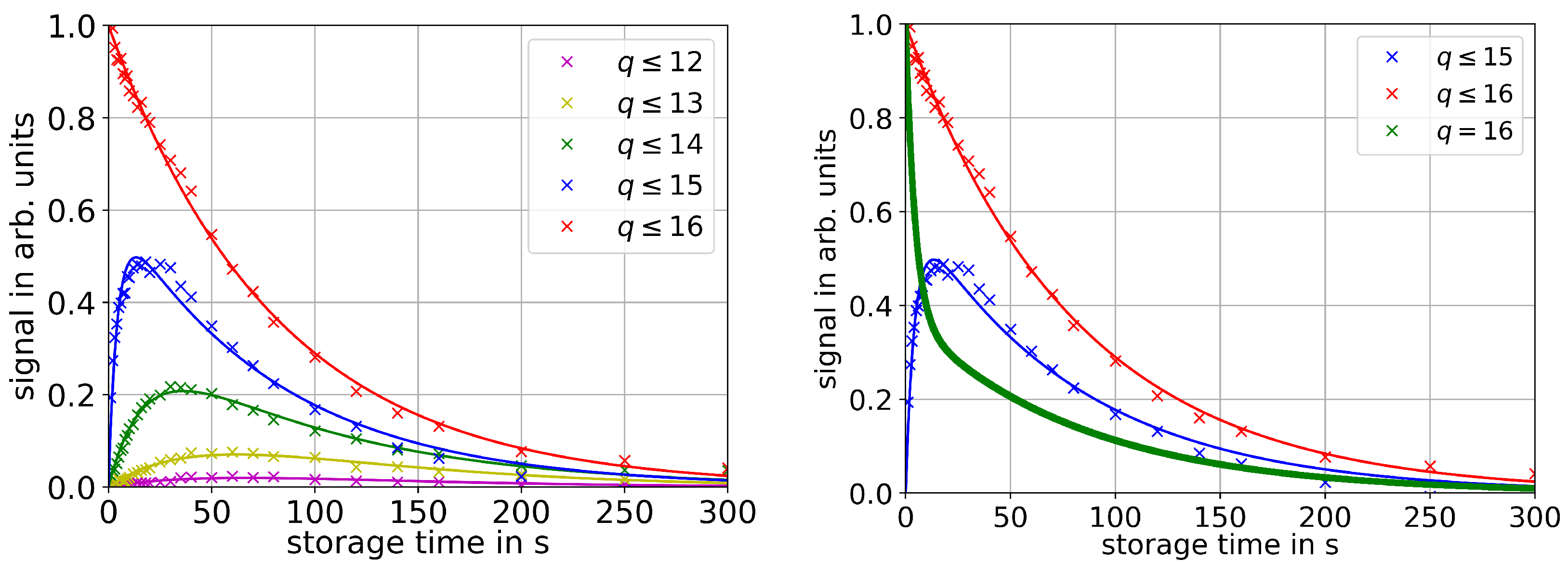
3.1. Charge Exchange
3.2. Magnetron Motion
4. Discussion
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
Abbreviations
| GSI | GSI Helmholtzzentrum für Schwerionenforschung GmbH |
| HCI | highly-charged ions |
| ESR | experimental storage ring |
| QED | quantum electrodynamics |
| RFQ | radiofrequency quadrupole |
| EBIT | electron beam ion trap |
| MCP | microchannel plate |
| FWHM | full width at half maximum |
References
- Repp, J.; Böhm, C.; Crespo López-Urrutia, J.R.; Dörr, A.; Eliseev, S.; George, S.; Goncharov, M.; Novikov, Y.N.; Roux, C.; Sturm, S.; et al. PENTATRAP: A novel cryogenic multi-Penning-trap experiment for high-precision mass measurements on highly charged ions. Appl. Phys. B 2012, 107, 983–996. [Google Scholar] [CrossRef]
- Murböck, T.; Albrecht, S.; Andelkovic, Z.; Cazan, R.; Hannen, V.; Jöhren, R.; Vollbrecht, J.; Schmidt, S.; Segal, D.; Thompson, R.; et al. SpecTrap: Precision spectroscopy of highly charged ions-status and prospects. Phys. Scr. 2013, 2013, 014096. [Google Scholar] [CrossRef]
- Micke, P.; Leopold, T.; King, S.A.; Benkler, E.; Spieß, L.J.; Schmoeger, L.; Schwarz, M.; Crespo López-Urrutia, J.R.; Schmidt, P.O. Coherent laser spectroscopy of highly charged ions using quantum logic. Nature 2020, 578, 60–65. [Google Scholar] [CrossRef] [PubMed]
- Sturm, S.; Werth, G.; Blaum, K. Electron g-factor determinations in Penning traps. Ann. der Phys. 2013, 525, 620–635. [Google Scholar] [CrossRef]
- Arapoglou, I.; Egl, A.; Höcker, M.; Sailer, T.; Tu, B.; Weigel, A.; Wolf, R.; Cakir, H.; Yerokhin, V.A.; Oreshkina, N.S.; et al. g Factor of Boronlike Argon 40Ar13+. Phys. Rev. Lett. 2019, 122, 253001. [Google Scholar] [CrossRef] [PubMed]
- Versolato, O.O.; Schwarz, M.; Windberger, A.; Ullrich, J.; Schmidt, P.O.; Drewsen, M.; Crespo López-Urrutia, J.R. Cold highly charged ions in a cryogenic Paul trap. Hyperfine Interact. 2013, 214, 189–194. [Google Scholar] [CrossRef]
- Herfurth, F.; Andelkovic, Z.; Barth, W.; Chen, W.; Dahl, L.A.; Fedotova, S.; Gerhard, P.; Kaiser, M.; Kester, O.K.; Kluge, H.J.; et al. The HITRAP facility for slow highly charged ions. Phys. Scr. 9 2015, T166, 014065. [Google Scholar] [CrossRef]
- Maero, G. Cooling of Highly Charged Ions in a Penning Trap for HITRAP. Ph.D. Thesis, Rupertus-Carola University of Heidelberg, Heidelberg, Germany, 2008. [Google Scholar] [CrossRef]
- Fedotova, S. Experimental Characterization of the Hitrap Cooler Trap with Highly Charged Ions. Ph.D. Thesis, Rupertus-Carola University of Heidelberg, Heidelberg, Germany, 2013. [Google Scholar] [CrossRef]
- Andelkovic, Z.; Fischer, J.; Herfurth, F.; König, K.; Neidherr, D.; Nörtershäuser, W.; Shroff, M.; Trotsenko, S.; Vorobjev, G. Development of the HITRAP cooling trap and the EBIT offline ion source. Hyperfine Interact. 2019, 240, 29. [Google Scholar] [CrossRef]
- Geyer, S.; Sokolov, A.; Thorn, A.; Vorobyev, G.; Stöhlker, T.; Kester, O. Characterization of the SPARC-EBIT at GSI. J. Instrum. 2010, 5, C10003. [Google Scholar] [CrossRef]
- Andelkovic, Z.; Herfurth, F.; Kotovskiy, N.; König, K.; Maaß, B.; Murböck, T.; Neidherr, D.; Schmidt, S.; Steinmann, J.; Vogel, M.; et al. Beamline for low-energy transport of highly charged ions at HITRAP. Nucl. Instruments Methods Phys. Res. Sect. A Accel. Spectrometers 2015, 795, 109–114. [Google Scholar] [CrossRef]
- Chill, F. Vermessung der Pumpeigenschafen einer Kryogenen Oberfäche. Ph.D. Thesis, Goethe University Frankfurt am Main, Frankfurt am Main, Germany, 2015. [Google Scholar]
- Müller, A.; Salzborn, E. Scaling of cross sections for multiple electron transfer to highly charged ions colliding with atoms and molecules. Phys. Lett. A 1977, 62, 391–394. [Google Scholar] [CrossRef]
- Liu, J.; Salumbides, E.J.; Hollenstein, U.; Koelemeij, J.C.; Eikema, K.S.; Ubachs, W.; Merkt, F. Determination of the ionization and dissociation energies of the hydrogen molecule. J. Chem. Phys. 2009, 130, 174306. [Google Scholar] [CrossRef] [PubMed]
- Vogel, M. Particle Confinement in Penning Traps; Springer: Heidelberg, Germany, 2018; Volume 100, pp. 48–51. [Google Scholar]

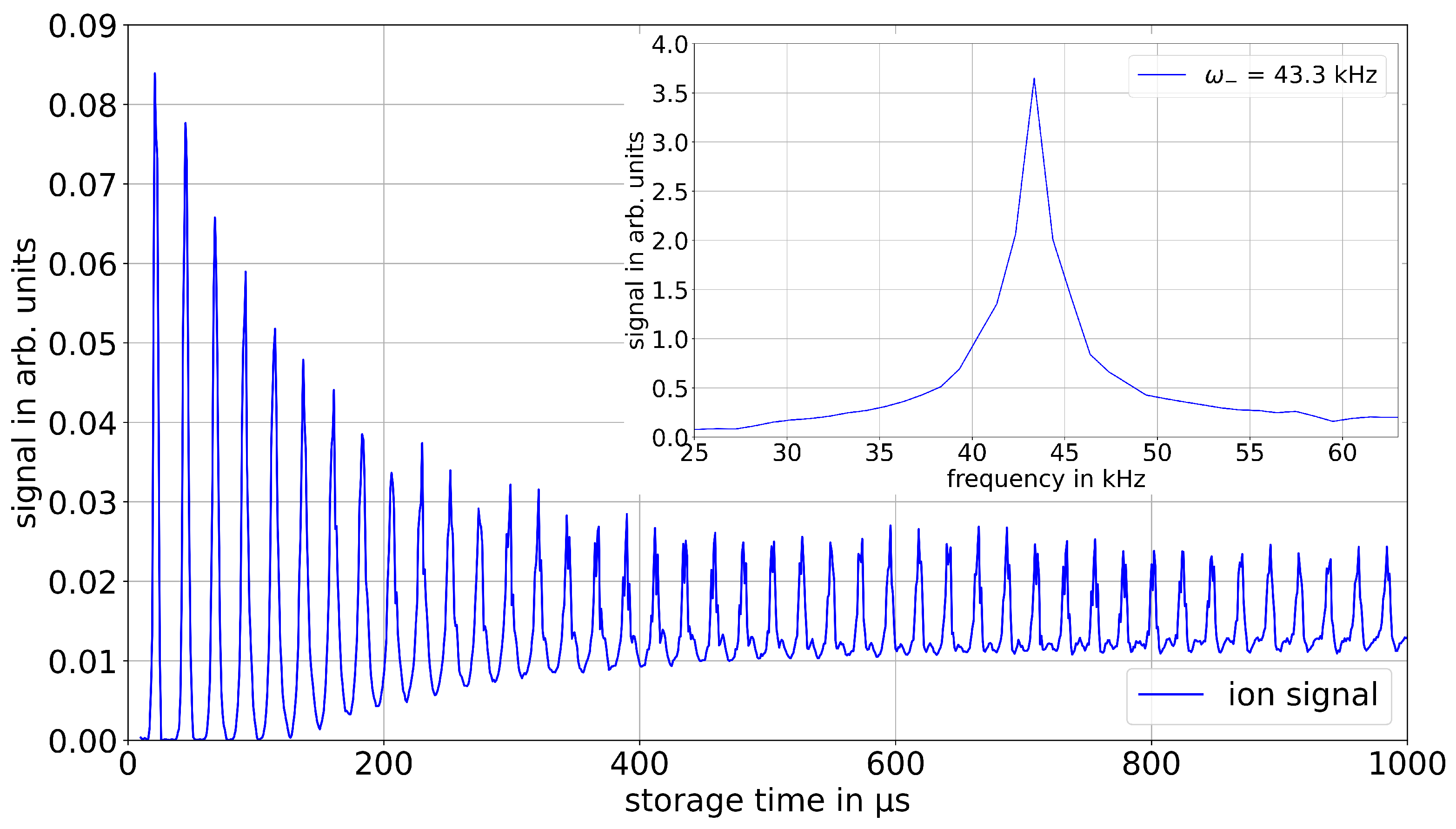
| B-Field | Capture Potential | Simulated | Measured |
|---|---|---|---|
| in T | in kV | in kHz | in kHz |
| 3 | 6 | 62 | |
| 3 | 7 | 77 | |
| 3 | 7.5 | 83 | |
| 3 | 8 | 83 | |
| 4 | 6 | 45 | |
| 4 | 8 | 64 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Rausch, S.; Horst, M.; Andelkovic, Z.; Fedotova, S.; Geithner, W.; Herfurth, F.; Neidherr, D.; Nörtershäuser, W.; Stallkamp, N.; Trotsenko, S.; et al. Commissioning of the HITRAP Cooling Trap with Offline Ions. Atoms 2022, 10, 142. https://doi.org/10.3390/atoms10040142
Rausch S, Horst M, Andelkovic Z, Fedotova S, Geithner W, Herfurth F, Neidherr D, Nörtershäuser W, Stallkamp N, Trotsenko S, et al. Commissioning of the HITRAP Cooling Trap with Offline Ions. Atoms. 2022; 10(4):142. https://doi.org/10.3390/atoms10040142
Chicago/Turabian StyleRausch, Simon, Max Horst, Zoran Andelkovic, Svetlana Fedotova, Wolfgang Geithner, Frank Herfurth, Dennis Neidherr, Wilfried Nörtershäuser, Nils Stallkamp, Sergiy Trotsenko, and et al. 2022. "Commissioning of the HITRAP Cooling Trap with Offline Ions" Atoms 10, no. 4: 142. https://doi.org/10.3390/atoms10040142
APA StyleRausch, S., Horst, M., Andelkovic, Z., Fedotova, S., Geithner, W., Herfurth, F., Neidherr, D., Nörtershäuser, W., Stallkamp, N., Trotsenko, S., & Vorobyev, G. (2022). Commissioning of the HITRAP Cooling Trap with Offline Ions. Atoms, 10(4), 142. https://doi.org/10.3390/atoms10040142





