Analytical Formulas for Approximating Cross Sections of Electron Collisions with Hydrogen, Noble Gases, Alkali and Other Atoms
Abstract
:1. Introduction
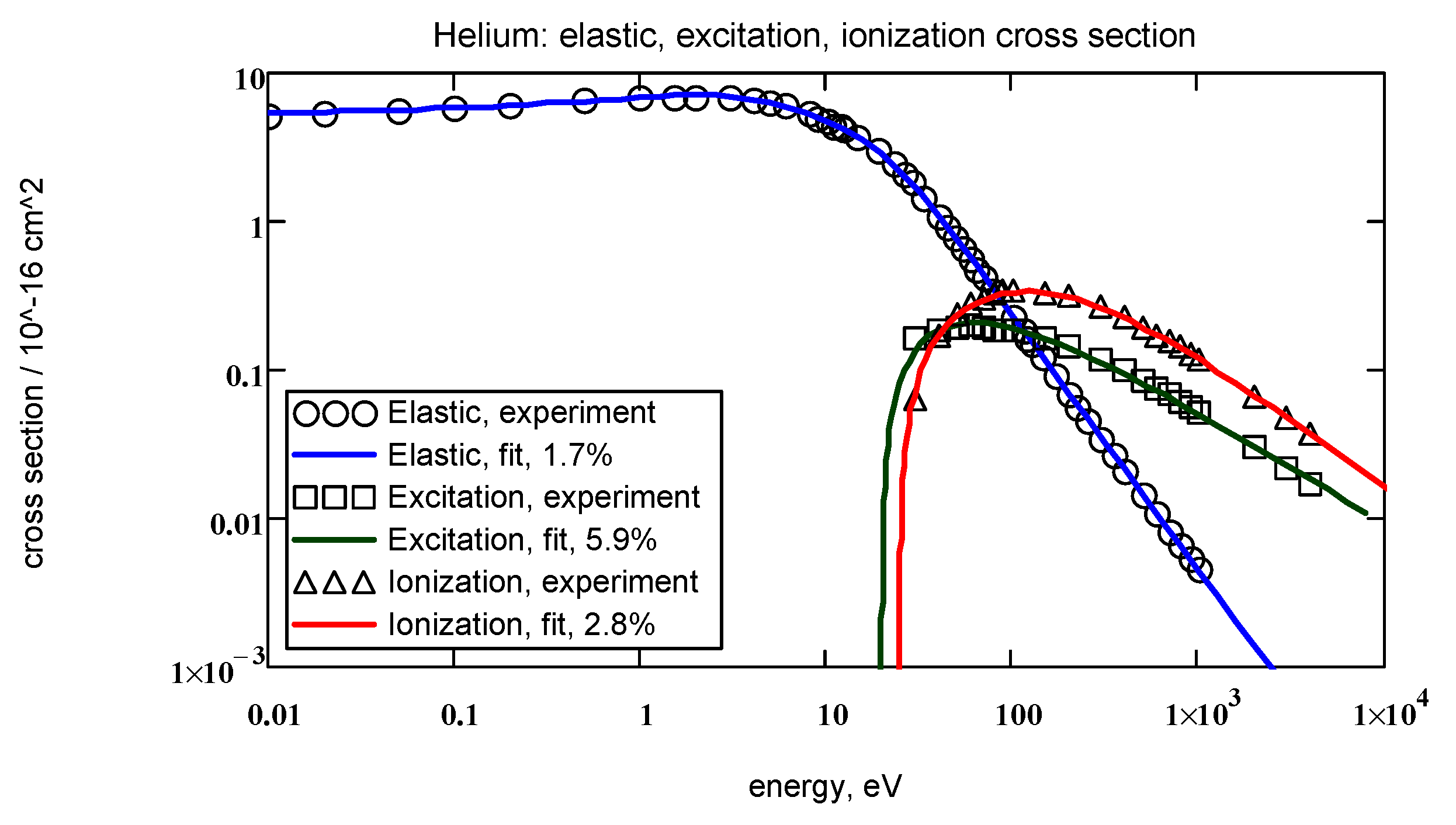
2. Analytical Expression for the Transport Cross Sections in Elastic Collisions
3. Approximation of the Excitation Cross Sections for Nobles Gases Atoms
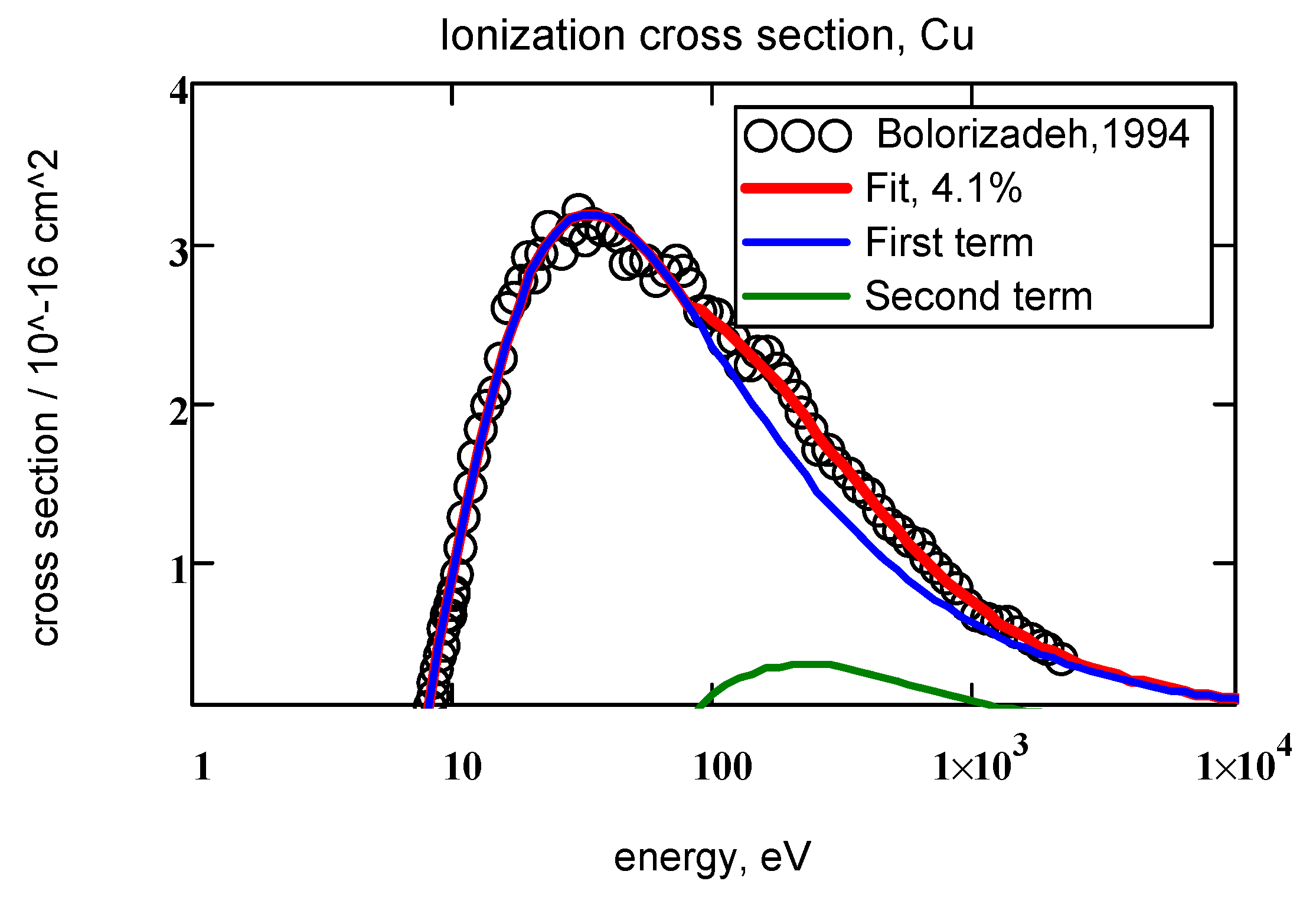
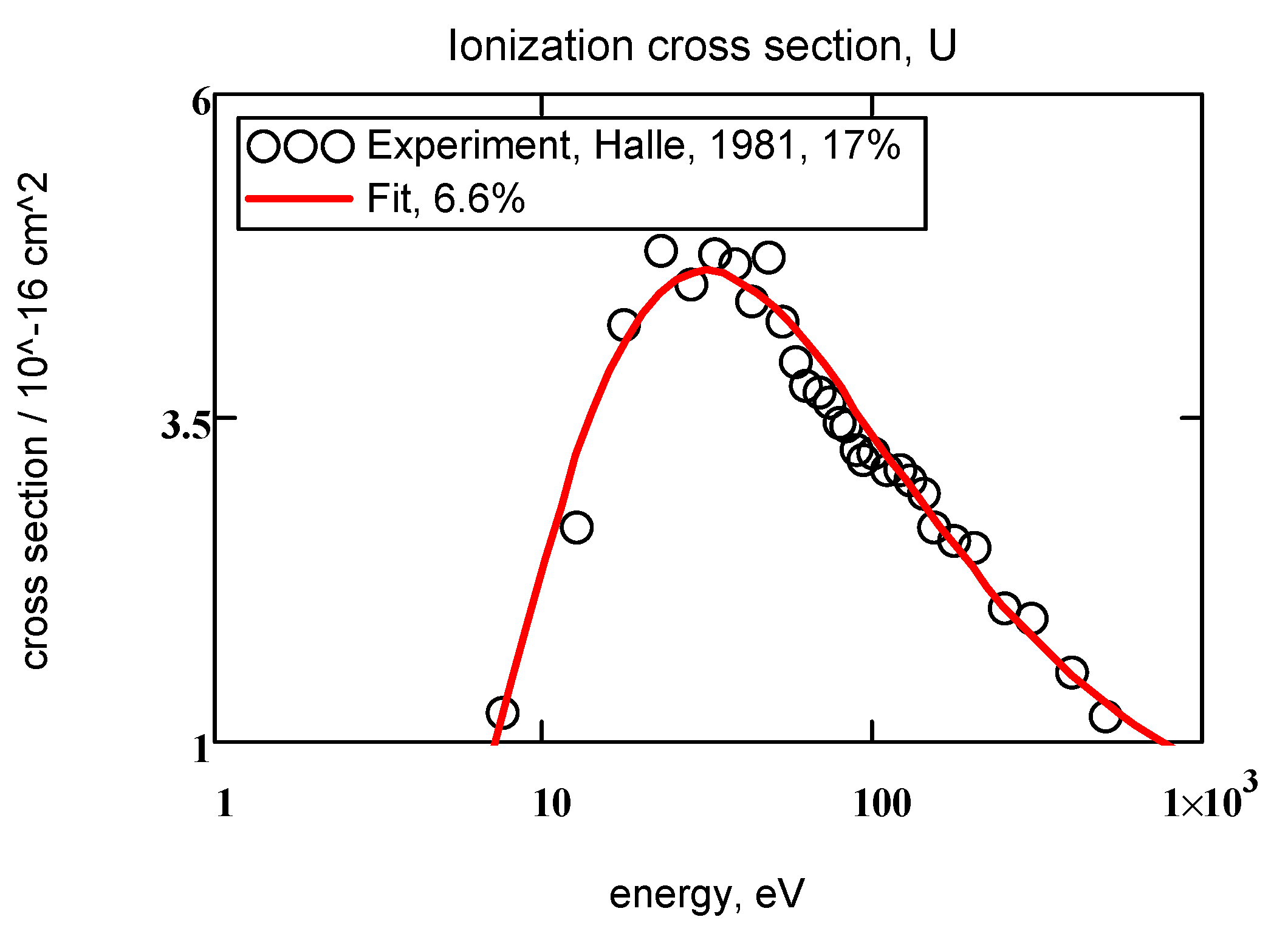
4. Approximation of the Ionization Cross Sections by One-Term Formula
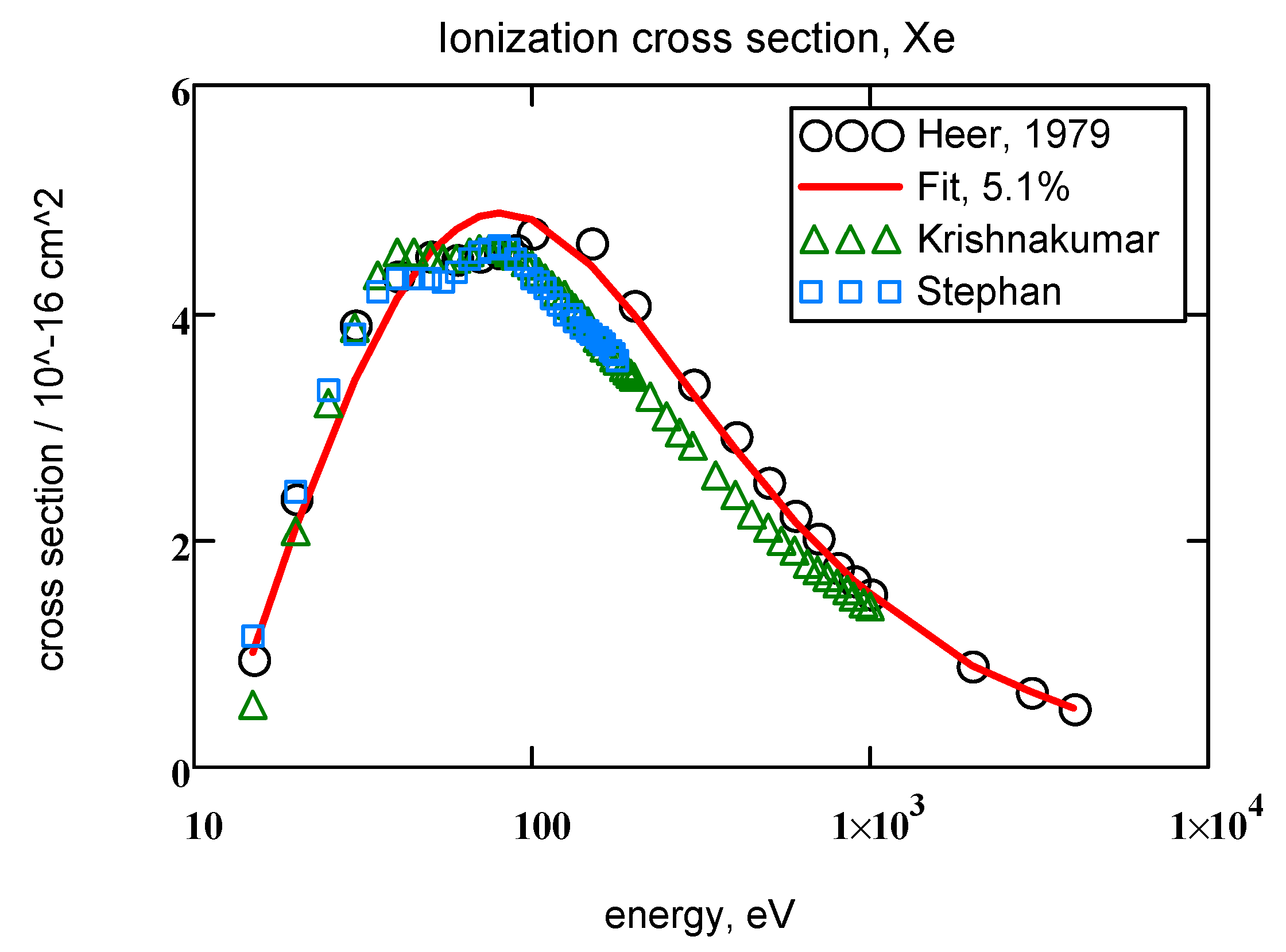
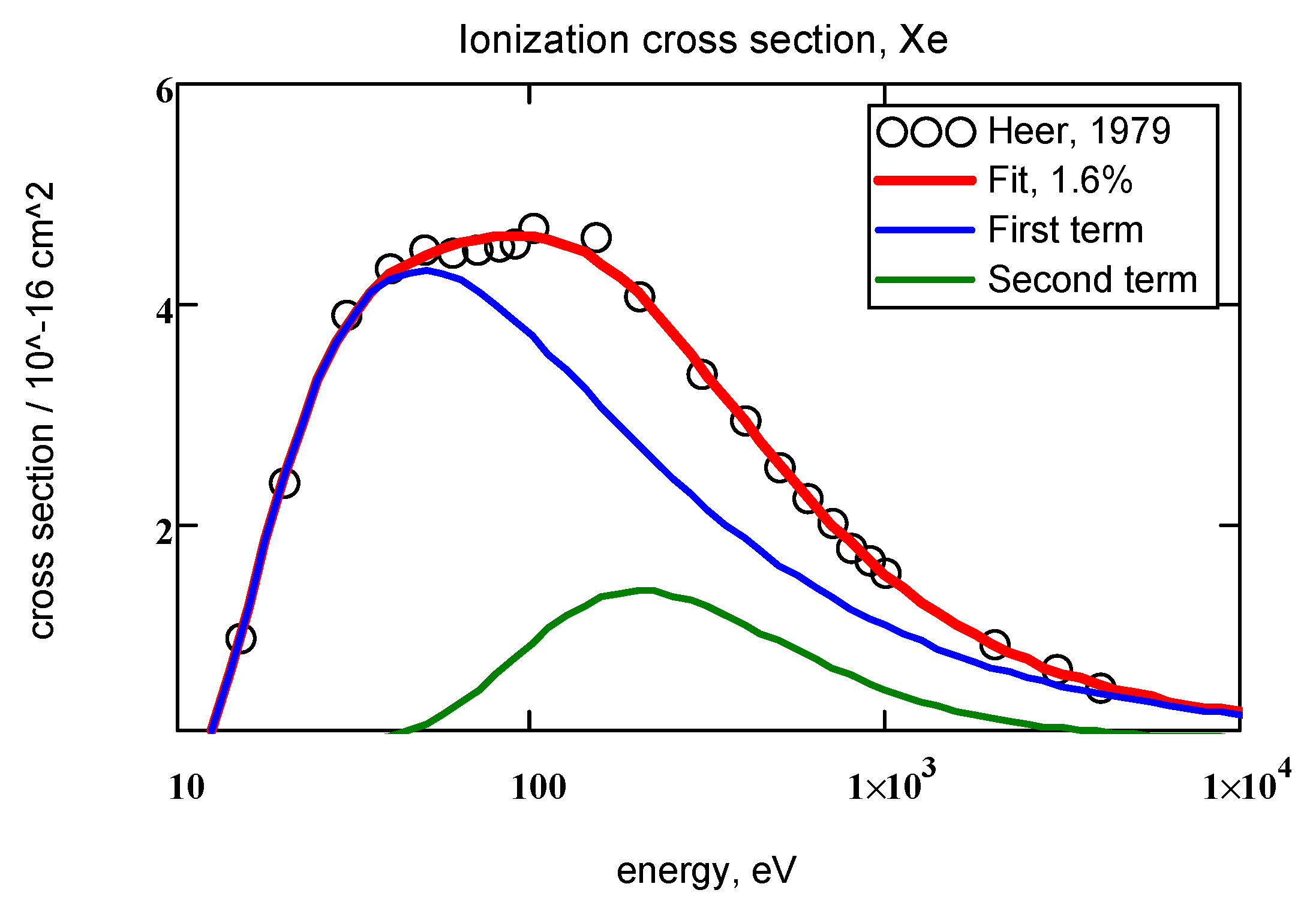
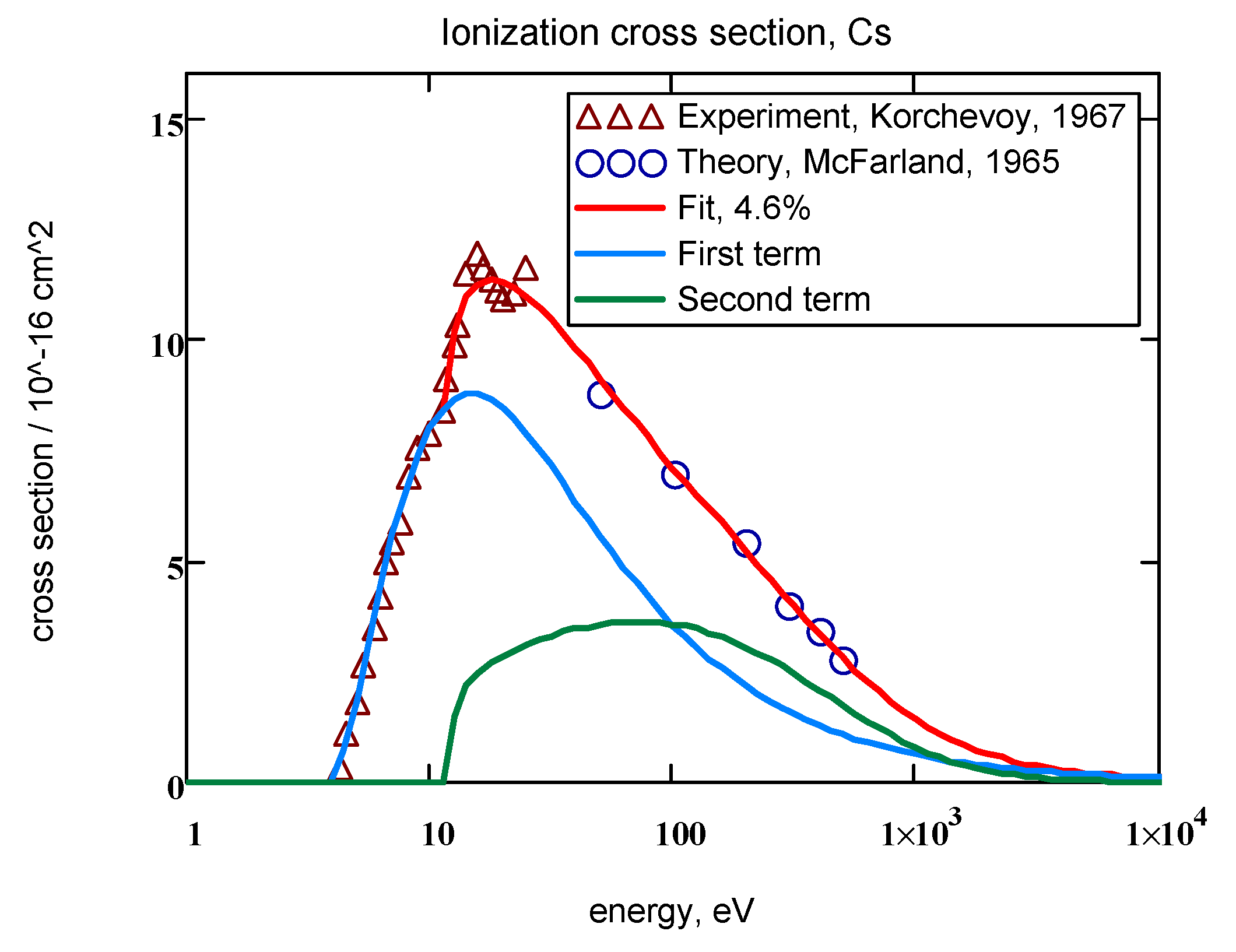
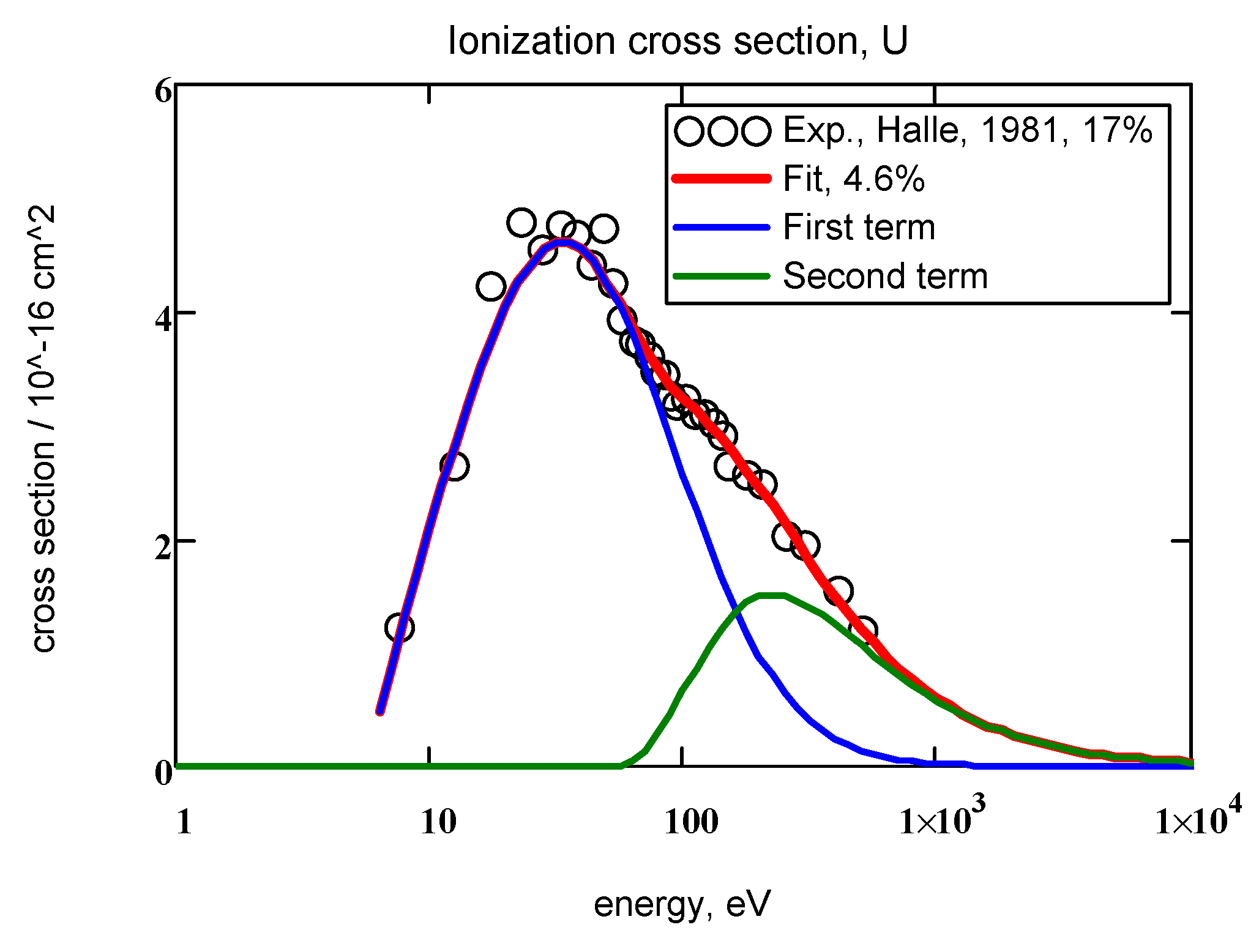
5. Approximation of the Ionization Cross Sections by Two-Term Formulas
6. Discussion and Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Conflicts of Interest
References
- Huxley, G.H.; Crompton, R.W. The Diffusion and Drift of Electrons in Gases; John Wiley & Sons: New York, NY, USA, 1974. [Google Scholar]
- Petrović, Z.; Dujko, S.; Marić, D.; Malović, G.; Nikitović, Ž.; Šašić, O.; Jovanović, J.; Stojanović, V.; Radmilović-Radenović, M.J. Measurement and interpretation of swarm parameters and their application in plasma modelling. Phys. D Appl. Phys. 2009, 42, 194002. [Google Scholar] [CrossRef]
- Carbone, E.; Graef, W.; Hagelaar, G.; Boer, D.; Hopkins, M.; Stephens, J.; Yee, B.; Pancheshnyi, S.; van Dijk, J.; Pitchford, L.; et al. Data Needs for Modeling Low-Temperature Non-Equilibrium Plasmas: The LXCat Project, History, Perspectives and a Tutorial. Atoms 2021, 9, 16. [Google Scholar] [CrossRef]
- Dutton, J. A survey of electron swarm data. J. Phys. Chem. Ref. Data 1975, 4, 577–856. [Google Scholar] [CrossRef]
- Zecca, A.; Karwasz, G.P.; Brusa, R.S. One century of experiments on electron-atom and molecule scattering: A aacritical review of integral cross-sections. Riv. Nuovo 1996, 19, 1–146. [Google Scholar] [CrossRef]
- Pancheshnyi, S.; Biagi, S.; Bordage, M.C.; Hagelaar, G.J.M.; Morgan, W.L.; Phelps, A.V.; Pitchford, L.C. The LXCat project: Electron scattering cross sections and swarm parameters for low temperature plasma modeling. Chem. Phys. 2012, 398, 148–153. [Google Scholar] [CrossRef]
- Brusa, R.S.; Karwasz, G.P.; Zecca, A. Analytical partitioning of total cross sections for scattering on noble gases. Eur. Phys. J. D 1996, 38, 279–287. [Google Scholar] [CrossRef]
- Kaur, J.; Gupta, D.; Naghma, R.; Ghoshal, D.; Antony, B. Electron impact ionization cross sections of atoms. Can. J. Phys. 2015, 93, 617–625. [Google Scholar] [CrossRef]
- McGuire, E.J. Electron ionization cross sections in the Born approximation. Phys. Rev. A 1977, 16, 62–72. [Google Scholar] [CrossRef]
- Freund, R.S.; Wetzel, R.C.; Shul, R.J.; Hayes, T.R. Cross-section measurements for electron-impact ionization of atoms. Phys. Rev. A 1990, 41, 3575–3595. [Google Scholar] [CrossRef]
- McFarland, R.H.; Kinney, J.D. Absolute cross sections of lithium and other alkali metal atoms for ionization by electrons. Phys. Rev. 1965, 137, 1058–1061. [Google Scholar] [CrossRef]
- Goswami, B.; Saikia, U.; Naghma, R.; Antony, B. Electron impact total ionization cross sections for plasma wall coating elements. Chin. J. Phys. 2013, 51, 1172–1183. [Google Scholar]
- Alves, L.L.; Bartschat, K.; Biagi, S.F.; Bordage, M.C.; Pitchford, L.C.; Ferreira, C.M.; Hagelaar, G.J.M.; Morgan, W.L.; Pancheshnyi, S.; Phelps, A.V.; et al. Comparisons of sets of electron–neutral scattering cross sections and swarm parameters in noble gases: II. Helium and neon. J. Phys. D Appl. Phys. 2013, 46, 334002. [Google Scholar] [CrossRef]
- Alves, L.L.; Bartschat, K.; Biagi, S.F.; Bordage, M.C.; Pitchford, L.C.; Ferreira, C.M.; Hagelaar, G.J.M.; Morgan, W.L.; Pancheshnyi, S.; Phelps, A.V.; et al. Comparisons of sets of electron–neutral scattering cross sections and swarm parameters in noble gases: I. Argon. J. Phys. D Appl. Phys. 2013, 46, 334001. [Google Scholar] [CrossRef]
- Bordage, M.C.; Biagi, S.F.; Alves, L.L.; Bartschat, K.; Chowdhury, S.; Pitchford, L.C.; Hagelaar, G.J.M.; Morgan, W.L.; Puech, V.; Zatsarinny, O. Comparisons of sets of electron–neutral scattering cross sections and swarm parameters in noble gases: III. Krypton and xenon. J. Phys. D Appl. Phys. 2013, 46, 334003. [Google Scholar] [CrossRef]
- Rejoub, R.; Lindsay, B.G.; Stebbings, R.F. Determination of the absolute partial and total cross sections for electron-impact ionization of the rare gases. Phys. Rev. A 2002, 65, 042713. [Google Scholar] [CrossRef]
- Heer, F.J.; Jansen, R.H.J. Total cross sections for electron scattering by He. J. Phys. B Atom. Mol. Phys. 1977, 10, 3741–3758. [Google Scholar] [CrossRef]
- Heer, F.J.; Jansen, R.H.J.; van der Kaay, W. Total cross sections for electron scattering by Ne, Ar, Kr and Xe. J. Phys. B At. Mol. Phys. 1979, 12, 979–1002. [Google Scholar] [CrossRef]
- Robertson, A.G. The momentum transfer cross section for low energy electrons in neon. J. Phys. B At. Mol. Phys. 1972, 5, 648–664. [Google Scholar] [CrossRef]
- Hasted, J.B. Physics of Atomic Collisions; Butterworths: Seattle, WA, USA, 1964; p. 536. [Google Scholar]
- Golyatina, R.I.; Maiorov, S.A. Analytical approximation of cross sections of collisions of electrons with atoms of inert gases. Phys. Sci. Technol. 2021, 8, 4–13. [Google Scholar] [CrossRef]
- Golyatina, R.I.; Maiorov, S.A. Analytical Approximation of Cross Sections of Collisions of Electrons with Inert Gas Atoms. Plasma Phys. Rep. 2022, 48, 193–199. [Google Scholar] [CrossRef]
- Golyatina, R.I.; Maiorov, S.A. Analytical Cross Section Approximation for Electron Impact Ionization of Alkali and Other Metals, Inert Gases and Hydrogen Atoms. Atoms 2021, 9, 90. [Google Scholar] [CrossRef]
- Thomson, J.J. XLII. Ionization by moving electrified particles. Lond. Edinb. Dublin. Philos. Mag. J. Sci. 1912, 23, 449–457. [Google Scholar] [CrossRef]
- Lotz, W.Z. Electron Binding Energies in Free Atoms. J. Opt. Soc. Am. 1970, 60, 206–210. [Google Scholar] [CrossRef]
- Krishnakumar, E.; Srivastava, S.K. Ionisation cross sections of rare-gas atoms by electron impact. J. Phys. B At. Mol. Opt. Phys. 1988, 21, 1055–1082. [Google Scholar] [CrossRef]
- Stephan, K.; Märk, T.D. Absolute partial electron impact ionization cross sections of Xe from threshold up to 180 eV. J. Chem. Phys. 1984, 81, 3116–3117. [Google Scholar] [CrossRef]
- Korchevoi, Y.P.; Przhonskii, A.M. Effective Electron Impact Excitation and Ionization Cross Sections for Cesium, Rubidium, and Potassium Atoms in the Pre-threshold Region. Sov. Phys. JETP 1967, 24, 1089. Available online: http://www.jetp.ras.ru/cgi-bin/dn/e_024_06_1089.pdf (accessed on 1 August 2020).
- Bolorizadeh, M.A.; Patton, C.J.; Shah, M.B.; Gilbody, H.B. Multiple ionization of copper by electron impact. J. Phys. B At. Mol. Opt. Phys. 1994, 27, 175–183. [Google Scholar] [CrossRef]
- Halle, J.C.; Lo, H.H.; Fite, W.L. Ionization of uranium atoms by electron impact. Phys. Rev. A 1981, 23, 1708–1716. [Google Scholar] [CrossRef]
- Mayorov, S.A. Calculation of characterisctics of electron drift in neon under a dc electric field. Bull. Lebedev Phys. Inst. 2009, 36, 299–304. [Google Scholar] [CrossRef]
- Kodanova, S.; Bastykova, N.; Ramazanov, T.; Maiorov, S. Drift of electrons in gas in spatially inhomogeneous periodic electric field. Ukr. J. Phys. 2014, 59. [Google Scholar] [CrossRef]
- Mayorov, S.A. Electron transport coefficients in a helium–xenon mixture. Bull. Lebedev Phys. Inst. 2014, 41, 285–291. [Google Scholar] [CrossRef]
- Golyatina, R.I.; Maiorov, S.A. Characteristics of electron drift in an Ar–Hg Mixture. Plasma Phys. Rep. 2018, 44, 453–457. [Google Scholar] [CrossRef]
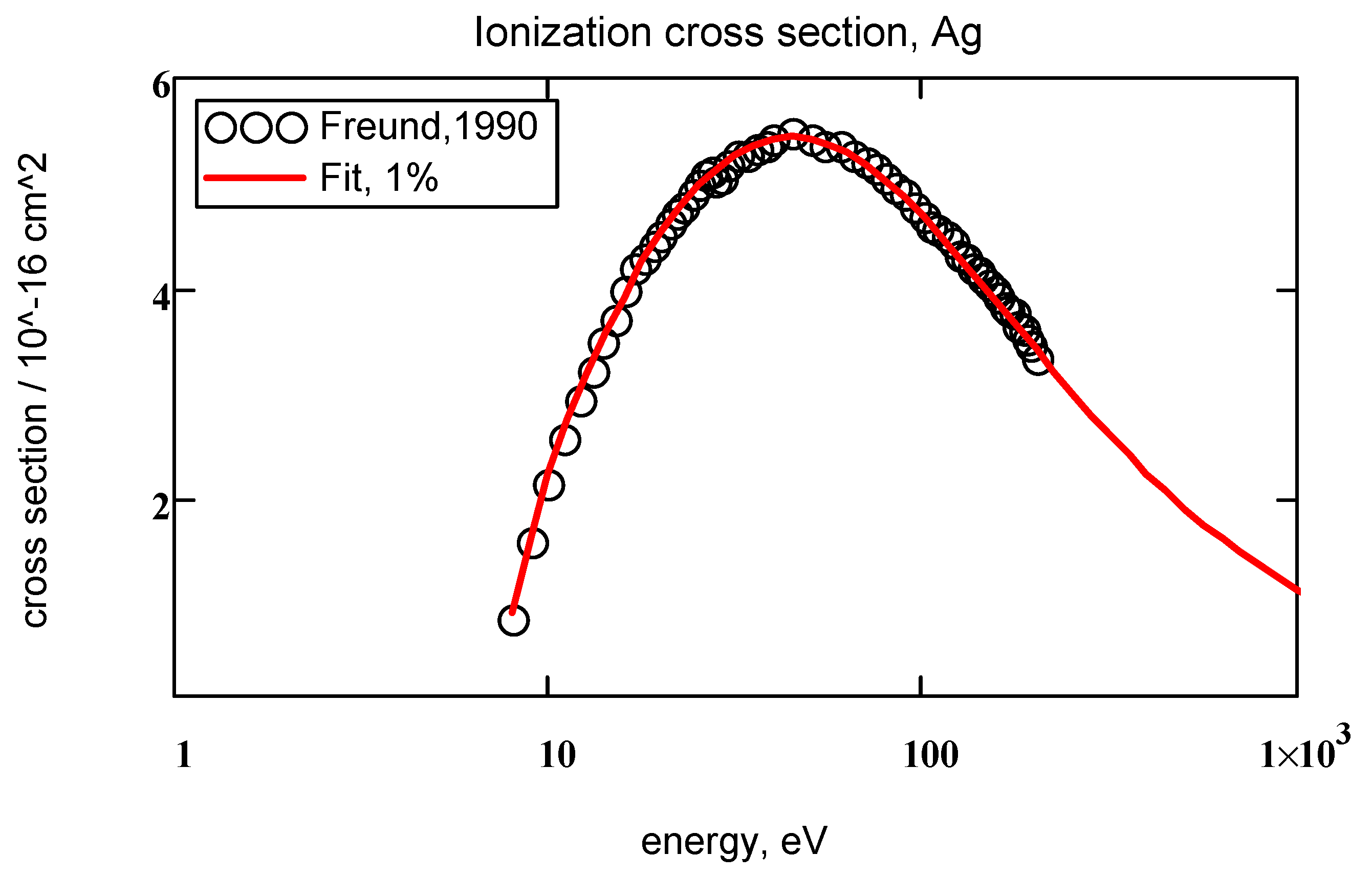



| No, Symbol, I, eV | σ1 Å2 | α1 Å2 | β1 | γ1 | δ1 | σ2 Å2 | α2 Å2 | β2 | γ2 | δ2 | Δ, % |
|---|---|---|---|---|---|---|---|---|---|---|---|
| He, 24.584 | 0 | 7.19 | 3.67 | 2.79 | 1 | 5.16 | 6.09 | 15.0 | 1.91 | 0.41 | 1.7% |
| Ne, 21.564 | 0 | 38.7 | 267. | 1.64 | 1 | 0.31 | 2.99 | 0.20 | 1.93 | 0.50 | 1.7% |
| Ar, 15.759 | 0.02 | 24.0 | 1.03 | 2.83 | 1 | 7.64 | −65.4 | 1961 | 1.37 | 0.455 | 8.0% |
| Kr, 13.996 | 0.17 | 115. | 9.52 | 3.58 | 1.82 | 40.5 | −101. | 1275 | 1.40 | 0.28 | 6.1% |
| Xe, 12.127 | −3.1 | 182 | 10.1 | 2.8 | 1.53 | 136 | −143. | 1453 | 1.37 | 0.169 | 9.0% |
| No, Symbol | E1, eV | α, Å2 | β | γ | Δ, % | eV | Å2 |
|---|---|---|---|---|---|---|---|
| He | 19.8 | 0.42 | 0.61 | 1.75 | 5.9% | 63 | 0.21 |
| Ne | 16.619 | 0.20 | 0.34 | 1.85 | 1.9% | 75 | 0.17 |
| Ar | 11.50 | 0.98 | 0.35 | 1.81 | 3.8% | 52 | 0.80 |
| Kr | 9.915 | 1.15 | 0.33 | 1.82 | 2.8% | 47 | 1.01 |
| Xe | 8.315 | 1.14 | 0.25 | 1.87 | 3.8% | 47 | 1.28 |
| No, Symbol | I1, E2, eV | α, Å2 | β | γ | δ | Δ, % | eV | Å2 |
|---|---|---|---|---|---|---|---|---|
| 2, He | 24.587 | 0.407 | 0.333 | 2.01 | 1.11 | 0.97% | 116 | 0.348 |
| 10, Ne | 21.564, 48.47 | 0.454 | 0.229 | 2.22 | 1.33 | 1.2% | 161 | 0.716 |
| 18, Ar | 15.759, 29.24 | 3.18 | 0.327 | 1.92 | 1.08 | 2.5% | 78 | 2.86 |
| 36, Kr | 13.996, 27.51 | 3.74 | 0.302 | 1.86 | 1.07 | 2.7% | 77 | 3.81 |
| 54, Xe | 12.127, 23.40 | 3.64 | 0.179 | 1.66 | 0.836 | 5.1% | 81 | 4.86 |
| No, Symbol | I1, E2, eV | α, Å2 | β | γ | δ | Δ, % | eV | Å2 |
|---|---|---|---|---|---|---|---|---|
| 1, H | 13.595 | 0.872 | 0.376 | 1.93 | 1.03 | 1.6% | 55 | 0.63 |
| 3, Li | 5.392, 58 | 71.9 | 1.28 | 3.65 | 2.84 | 5.6% | 20 | 5.2 |
| 11, Na | 5.139, 34 | 28.1 | 1.23 | 1.85 | 1.26 | 1.5% | 14 | 6.83 |
| 19, K | 4.339, 18.7 | 3.49 | 0.022 | 1.25 | 0.31 | 4.7% | 69 | 5.65 |
| 37, Rb | 4.176, 15.3 | 8.18 | 0.07 | 1.04 | 0.105 | 2.3% | 11 | 7.7 |
| 55, Cs | 3.893, 12.3 | 19.2 | 0.64 | 2.19 | 1.61 | 6.6% | 11 | 4.86 |
| No, Symbol | I1, E2, eV | α, Å2 | β | γ | δ | Δ, % | eV | Å2 |
|---|---|---|---|---|---|---|---|---|
| 4, Be | 9.322, 115 | 3.82 | 0.439 | 2.16 | 1.09 | 6.9% | 31 | 2.11 |
| 12, Mg | 7.646, 54 | 13.6 | 0.713 | 1.87 | 1.0 | 3.1% | 20 | 5.3 |
| 13, Al | 5.986, 10.62 | 48.7 | 1.11 | 2.54 | 1.95 | 2.9% | 24 | 10.1 |
| 14, Si | 8.157, 13.46 | 16.75 | 0.893 | 1.77 | 1.28 | 2.5% | 32 | 6.8 |
| 15, P | 10.486, 16.15 | 18.9 | 0.911 | 4.05 | 3.31 | 4.6% | 62 | 3.75 |
| 16, S | 9.75, 20.20 | 66.9 | 1.19 | 5.32 | 4.6 | 3.0% | 63 | 3.74 |
| 21, Sc | 6.56, 33 | 66.5 | 1.28 | 3.76 | 3.19 | 7% | 35 | 6.12 |
| 22, Ti | 6.83, 38 | 13.4 | 0.456 | 1.58 | 0.71 | 3.1% | 19 | 7.89 |
| 24, Cr | 6.764, 48 | 674 | 1.94 | 4.75 | 4.17 | 4.9% | 31 | 7.29 |
| 25, Mn | 7.432, 53 | 219 | 1.75 | 3.75 | 3.19 | 3.8% | 31 | 7.46 |
| 26, Fe | 7.90, 59 | 5.34 | 0.129 | 1.29 | 0.35 | 4.6% | 31 | 5.15 |
| 27, Co | 7.86, 66 | 337 | 1.79 | 4.71 | 4.15 | 4.3% | 41 | 5.43 |
| 28, Ni | 7.663, 73 | 9.97 | 0.632 | 2.18 | 1.35 | 4.6% | 27 | 4.36 |
| 29, Cu | 7.724, 80 | 4.45 | 0.469 | 1.78 | 1.13 | 6.4% | 37 | 3.29 |
| 30, Zn | 9.391, 91 | 300 | 1.85 | 4.90 | 4.38 | 3.5% | 52 | 3.86 |
| 31, Ga | 6.00, 11 | 163 | 1.5 | 4.53 | 3.98 | 4.1% | 35 | 6.15 |
| 32, Ge | 7.88, 14.3 | 6.37 | 0.529 | 2.17 | 1.58 | 6.0% | 48 | 4.91 |
| 41, Nb | 6.88, 38 | 422 | 1.81 | 4.14 | 3.56 | 3.9% | 30 | 9.53 |
| 45, Rh | 7.46, 53 | 10.5 | 0.745 | 2.46 | 1.96 | 3.4% | 47 | 5.43 |
| 46, Pd | 8.33, 57 | 33.2 | 1.23 | 3.91 | 3.46 | 2.0% | 60 | 3.98 |
| 47, Ag | 7.574, 63 | 4.52 | 0.141 | 1.434 | 0.59 | 1.0% | 45 | 5.44 |
| 48, Cd | 8.991, 71 | 588 | 2.08 | 4.60 | 4.09 | 3.7% | 43 | 5.82 |
| 74, W | 7.98, 41 | 27.6 | 1.00 | 3.86 | 3.31 | 2.1% | 62 | 5.66 |
| 79, Au | 9.223, 61 | 214 | 1.12 | 3.99 | 3.17 | 9.1% | 41 | 20 |
| 80, Hg | 10.434, 68 | 1.04 | 0.211 | 1.75 | 1.1 | 11.4 | 94 | 1.82 |
| 82, Pb | 7.415, 25 | 7.63 | 0.174 | 1.51 | 0.63 | 3.3% | 38 | 8.23 |
| 92, U | 5.65, 48 | 4.71 | 0.328 | 1.76 | 1.05 | 6.6% | 30 | 4.49 |
| No, Symbol | I1, E2, eV | Term Index | αi, Å2 | βi | γi | δi | Δ, % | Fit Terms |
|---|---|---|---|---|---|---|---|---|
| 54, Xe | 12.127, 23.40 | i = 1 | 3.64 | 0.179 | 1.66 | 0.836 | 5.1% | 1 TA |
| i = 1 | 9.18 | 0.685 | 2.03 | 1.37 | 1.6% | 2 TA | ||
| i = 2 | 0.586 | 0.371 | 5.29 | 3.91 | ||||
| 55, Cs | 3.893, 12.3 | i = 1 | 19.2 | 0.64 | 2.19 | 1.61 | 6.6% | 1 TA |
| i = 1 | 30.3 | 8.22 | 2.59 | 1.8 | 4.6% | 2 TA | ||
| i = 2 | 3.24 | 0.016 | 2.73 | 0.203 | ||||
| 29, Cu | 7.724, 80. | i = 1 | 4.45 | 0.469 | 1.78 | 1.13 | 6.4% | 1 TA |
| i = 1 | 5.12 | 0.537 | 1.87 | 1.19 | 4.1% | 2 TA | ||
| i = 2 | 0.565 | 0.220 | 2.74 | 0.784 | ||||
| 92, U | 5.65, 48 | i = 1 | 4.71 | 0.328 | 1.76 | 1.05 | 6.6% | 1 TA |
| i = 1 | 3.1 | 0.06 | 3.76 | 0.86 | 4.6% | 2 TA | ||
| i = 2 | 8.74 | 0.75 | 4.8 | 3.53 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Maiorov, S.A.; Golyatina, R.I. Analytical Formulas for Approximating Cross Sections of Electron Collisions with Hydrogen, Noble Gases, Alkali and Other Atoms. Atoms 2022, 10, 93. https://doi.org/10.3390/atoms10030093
Maiorov SA, Golyatina RI. Analytical Formulas for Approximating Cross Sections of Electron Collisions with Hydrogen, Noble Gases, Alkali and Other Atoms. Atoms. 2022; 10(3):93. https://doi.org/10.3390/atoms10030093
Chicago/Turabian StyleMaiorov, Sergey A., and Rusudan I. Golyatina. 2022. "Analytical Formulas for Approximating Cross Sections of Electron Collisions with Hydrogen, Noble Gases, Alkali and Other Atoms" Atoms 10, no. 3: 93. https://doi.org/10.3390/atoms10030093
APA StyleMaiorov, S. A., & Golyatina, R. I. (2022). Analytical Formulas for Approximating Cross Sections of Electron Collisions with Hydrogen, Noble Gases, Alkali and Other Atoms. Atoms, 10(3), 93. https://doi.org/10.3390/atoms10030093




