Electron-Impact Ionization of the Tungsten Ions: W38+ − W45+
Abstract
:1. Introduction
2. Methods
3. Results
3.1. W
3.2. W
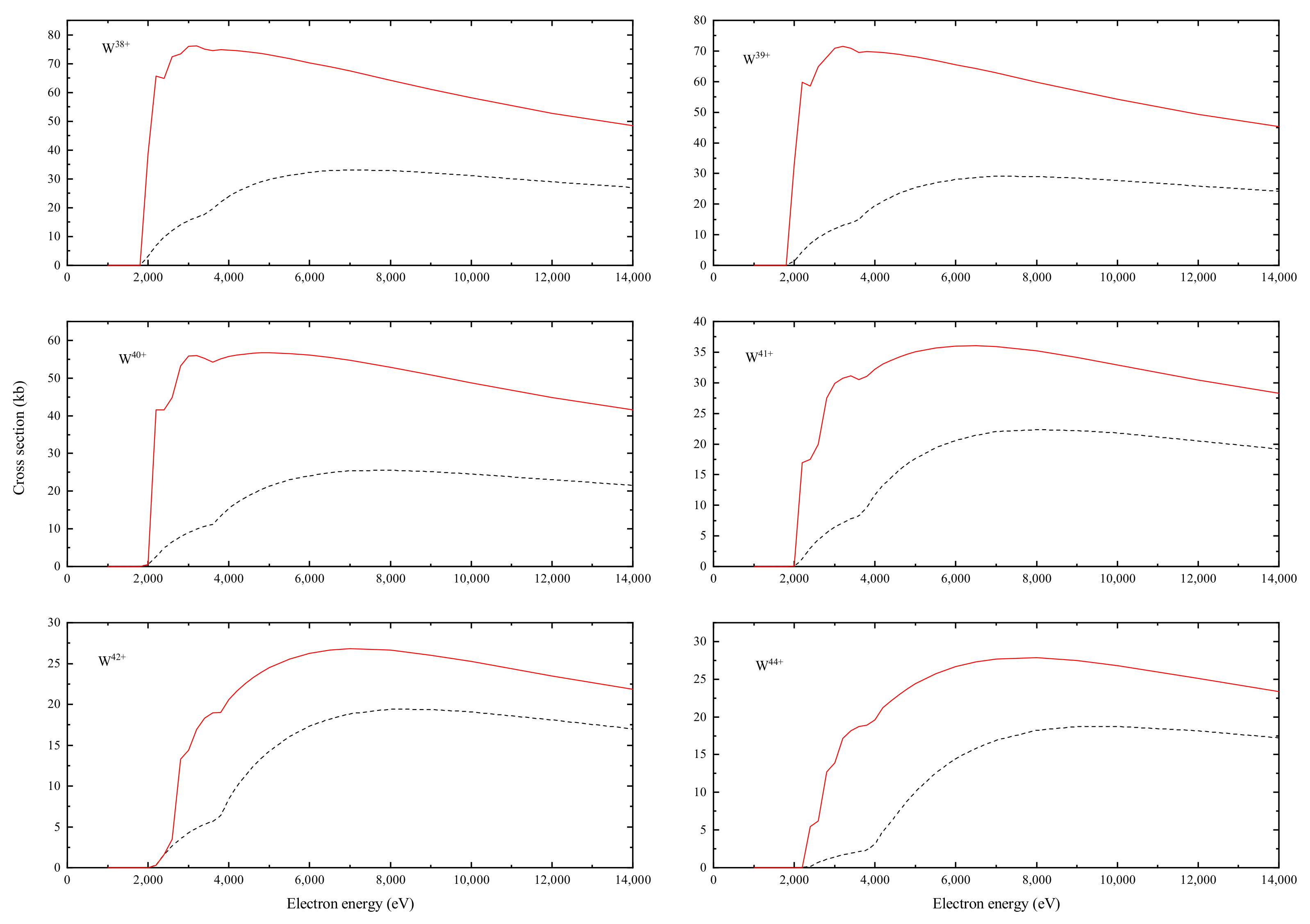
3.3. W, W−W
3.4. Fitting Coefficients
4. Summary
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Federici, G. Plasma wall interactions in ITER. Phys. Scr. 2006, T124, 1. [Google Scholar] [CrossRef]
- Groth, M.; Brezinsek, S.; Belo, P.; Beurskens, M.N.; Brix, M.; Clever, M.; Coenen, J.W.; Corrigan, C.; Eich, T.; Flanagan, J.; et al. Impact of carbon and tungsten as divertor materials on the scrape-off layer conditions in JET. Nucl. Fusion 2013, 53, 093016. [Google Scholar] [CrossRef]
- Pütterich, T.; Neu, R.; Dux, R.; Whiteford, A.; O’Mullane, M.; Summers, H. and the ASDEX Upgrade Team. Calculation and experimental test of the cooling factor of tungsten. Nucl. Fusion 2010, 50, 025012. [Google Scholar] [CrossRef]
- Pütterich, T.; Fable, E.; Dux, R.; O’Mullane, M.; Neu, R.; Siccinio, M. Determination of the tolerable impurity concentrations in a fusion reactor using a consistent set of cooling factors. Nucl. Fusion 2019, 55, 056013. [Google Scholar] [CrossRef]
- Müller, A. Fusion-Related Ionization and Recombination Data for Tungsten Ions in Low to Moderately High Charge States. Atoms 2015, 3, 120. [Google Scholar] [CrossRef]
- Pütterich, T.; Neu, R.; Dux, R.; Whiteford, A.D.; O’Mullane, M.G.; ASDEX Upgrade Team. Modelling of measured tungsten spectra from ASDEX Upgrade and predictions for ITER. Plasma Phys. Control. Fusion 2008, 50, 085016. [Google Scholar] [CrossRef]
- Stenke, M.; Aichele, K.; Harthiramani, D.; Hofmann, G.; Steidl, M.; Volpel, R.; Salzborn, E. Electron-impact single-ionization of singly and multiply charged tungsten ions. J. Phys. B At. Mol. Opt. Phys. 1995, 28, 2711. [Google Scholar] [CrossRef]
- Schury, D.; Borovik, A., Jr.; Ebinger, B.; Jin, F.; Spruck, K.; Müller, A.; Schippers, S. Electron-impact single ionisation of Wq+ ions: Experiment and theory for 11 ≤ q ≤ 18. J. Phys. B At. Mol. Opt. Phys. 2020, 53, 015201. [Google Scholar] [CrossRef]
- Rausch, J.; Becker, A.; Spruck, K.; Hellhund, J.; Borovik, A., Jr.; Huber, K.; Schippers, S.; Müller, A. Electron-impact single and double ionization of W17+. J. Phys. B At. Mol. Opt. Phys. 2011, 44, 165202. [Google Scholar] [CrossRef]
- Borovik, B.A., Jr.; Ebinger; Schury, D.; Schippers, S.; Müller, A. Electron-impact single ionization of W19+ ions. Phys. Rev. A 2016, 93, 012708. [Google Scholar] [CrossRef]
- Loch, S.D.; Ludlow, J.A.; Pindzola, M.S.; Whiteford, A.D.; Griffin, D.C. Electron-impact ionization of atomic ions in the W isonuclear sequence. Phys. Rev. A 2005, 72, 052716. [Google Scholar] [CrossRef]
- Jin, F.; Borovik, A., Jr.; Ebinger, B.; Schippers, S. Electron-impact single ionisation of W14+ ions: Subconfiguration-average and level-to-level distorted wave calculations. J. Phys. B At. Mol. Opt. Phys. 2020, 53, 075201. [Google Scholar] [CrossRef]
- Jin, F.; Borovik, A., Jr.; Ebinger, B.; Schippers, S. Hybrid subconfiguration-average and level-to-level distorted-wave treatment of electron-impact single ionisation of W15+ and W16+. J. Phys. B At. Mol. Opt. Phys. 2020, 53, 175201. [Google Scholar] [CrossRef]
- Zhang, D.H.; Kwon, D.H. Theoretical electron-impact ionization of W17+ forming W18+. J. Phys. B At. Mol. Opt. Phys. 2014, 47, 075202. [Google Scholar] [CrossRef]
- Zhang, D.H.; Xie, L.Y.; Jiang, J.; Wu, Z.W.; Dong, C.Z.; Shi, Y.L.; Qu, Y.Z. Electron-impact single ionizaiton for W4+ and W5+. Chin. Phys. B 2018, 27, 053402. [Google Scholar] [CrossRef]
- Pindzola, M.S.; Griffin, D.C. Electron-impact ionization of tungsten ions in the configuration-average distorted-wave approximation. Phys. Rev. A 1997, 56, 1654. [Google Scholar] [CrossRef]
- Jonauskas, V.; Kynienė, A.; Kučas, S.; Pakalka, S.; Masys, Š.; Prancikevičius, A.; Borovik, A., Jr.; Gharaibeh, M.F.; Schippers, S.; Müller, A. Electron-impact ionization of W5+. Phys. Rev. A 2019, 100, 062701. [Google Scholar] [CrossRef]
- Kynienė, A.; Pakalka, S.; Masys, Š.; Jonauskas, V. Electron-impact ionization of W25+. J. Phys. B At. Mol. Opt. Phys. 2016, 49, 185001. [Google Scholar] [CrossRef]
- Kynienė, A.; Merkelis, G.; Šukys, A.; Masys, Š.; Pakalka, S.; Kisielius, R.; Jonauskas, V. Maxwellian rate coefficients for electron-impact ionization of W26+. J. Phys. B At. Mol. Opt. Phys. 2018, 51, 155202. [Google Scholar] [CrossRef]
- Purohit, G.; Kato, D.; Murakami, I. Electron Impact Ionization Cross Sections of Tungsten Atoms and Tungsten Ions. Plasma Fusion Res. 2018, 13, 3401026. [Google Scholar] [CrossRef]
- Chen, L.; Li, B.W.; Chen, X.M. Contribution of the metastable states to electron-impact single ionization for W7+. J. Quant. Spectrosc. Radiat. Transf. 2022, 285, 108179. [Google Scholar] [CrossRef]
- Technical Meeting on the Collisional-Radiative Properties of Tungsten and Hydrogen in Edge Plasma of Fusion Devices, 29 March–1 April 2021, Vienna, Austria. Available online: https://amdis.iaea.org/meetings/tm-tungsten-hydrogen/ (accessed on 22 August 2022).
- Badnell, N.R.; Pindzola, M.S. Resonance contributions to the electron-impact ionization of few-electron highly charged ions. Phys. Rev. A 1993, 47, 2937. [Google Scholar] [CrossRef] [PubMed]
- Gu, M.F. The flexible atomic code. Can. J. Phys. 2008, 86, 675. [Google Scholar] [CrossRef]
- Kramida, A.; Ralchenko, Y.; Reader, J.; NIST ASD Team. NIST Atomic Spectra Database (Version 5.9); National Institute of Standards and Technology: Gaithersburg, MD, USA, 2021. Available online: https://physics.nist.gov/asd (accessed on 22 August 2022).
- Dipti; Das, T.; Bartschat, K.; Bray, I.; Fursa, D.V.; Zatsarinny, O.; Ballance, C.; Chung, H.K.; Ralchenko, Y. Recommended electron-impact excitation and ionization cross sections for Be I. At. Data Nucl. Data Tables 2019, 127–128, 1–21. [Google Scholar] [CrossRef] [PubMed]
- Yang, J.C.; Xiao, G.Q.; Xu, H.S.; Zhao, X.H.; Ma, X.W.; He, Y.; Ma, L.Z.; Gao, D.Q.; Meng, J.; Xu, Z.; et al. High Intensity heavy ion Accelerator Facility (HIAF) in China. Nucl. Instrum. Methods Phys. Res. B 2013, 317, 263–265. [Google Scholar] [CrossRef]
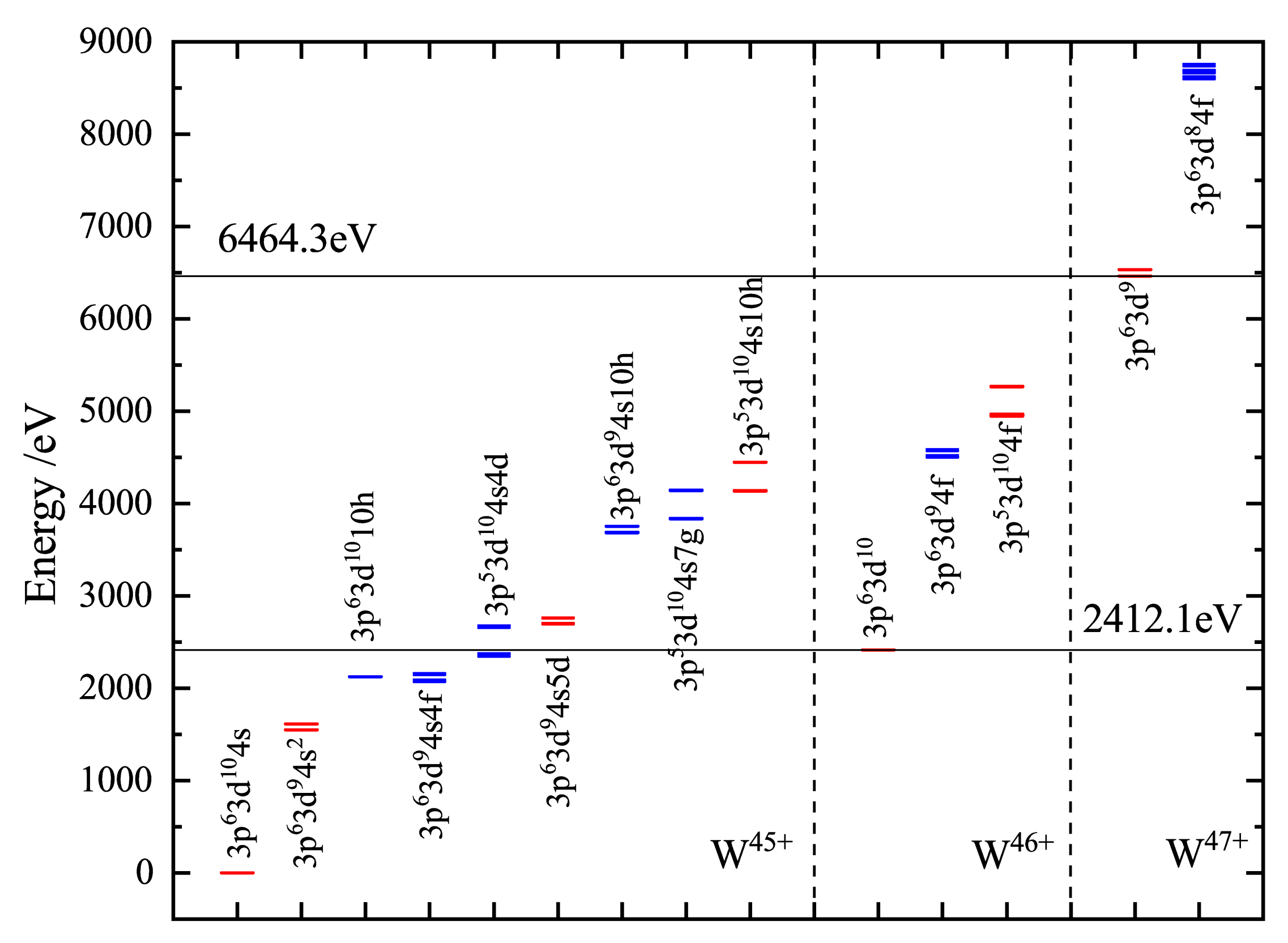
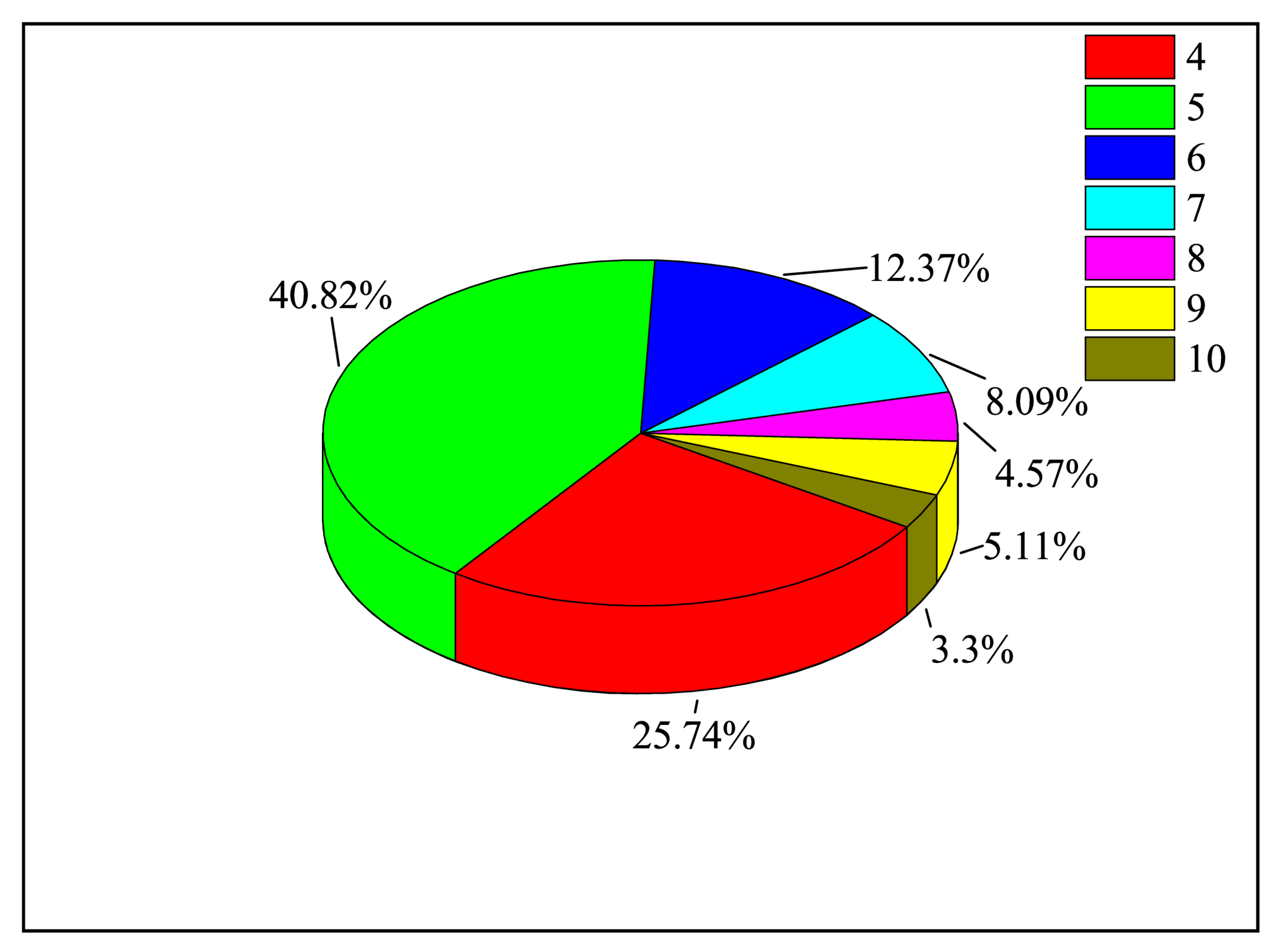

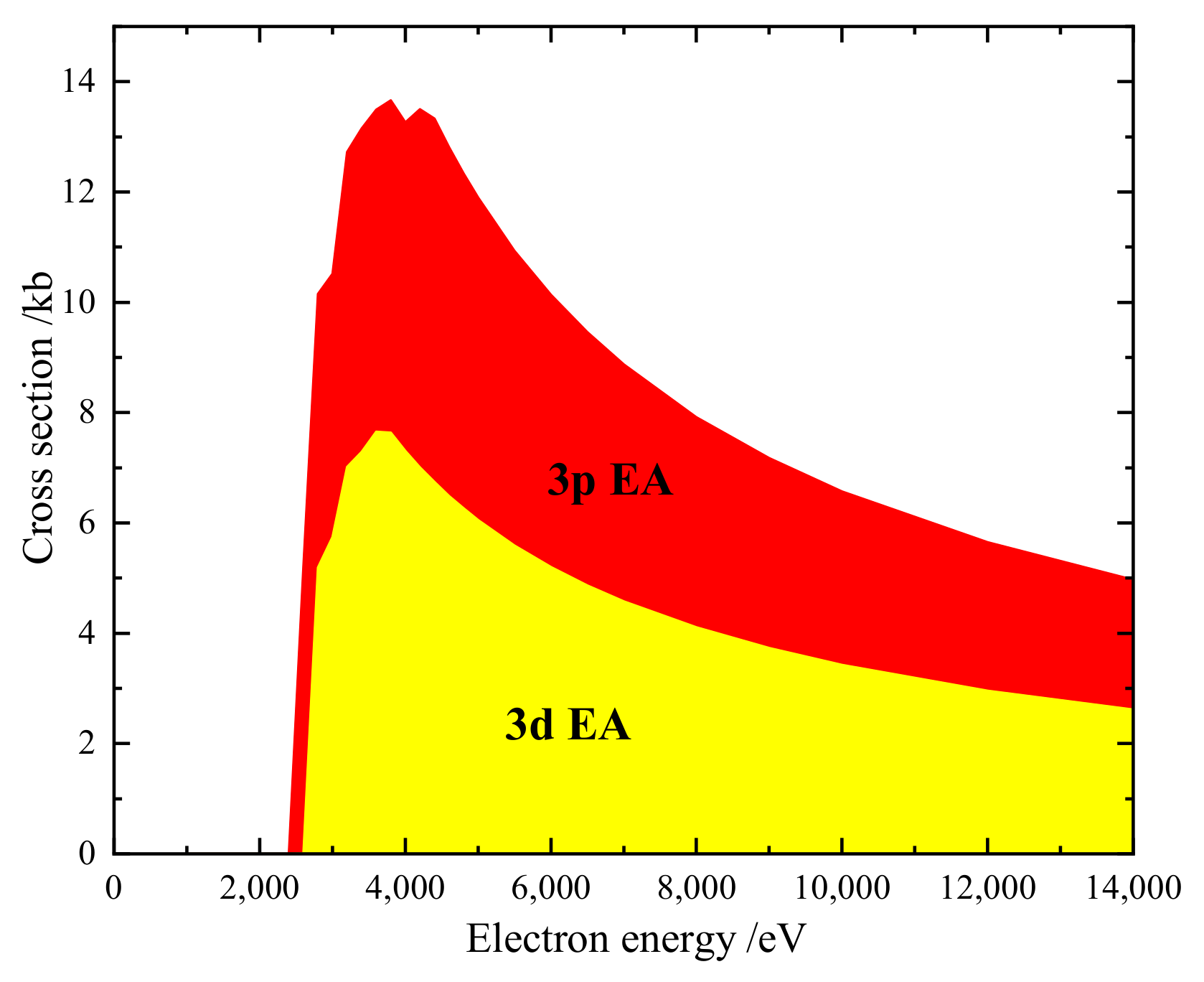
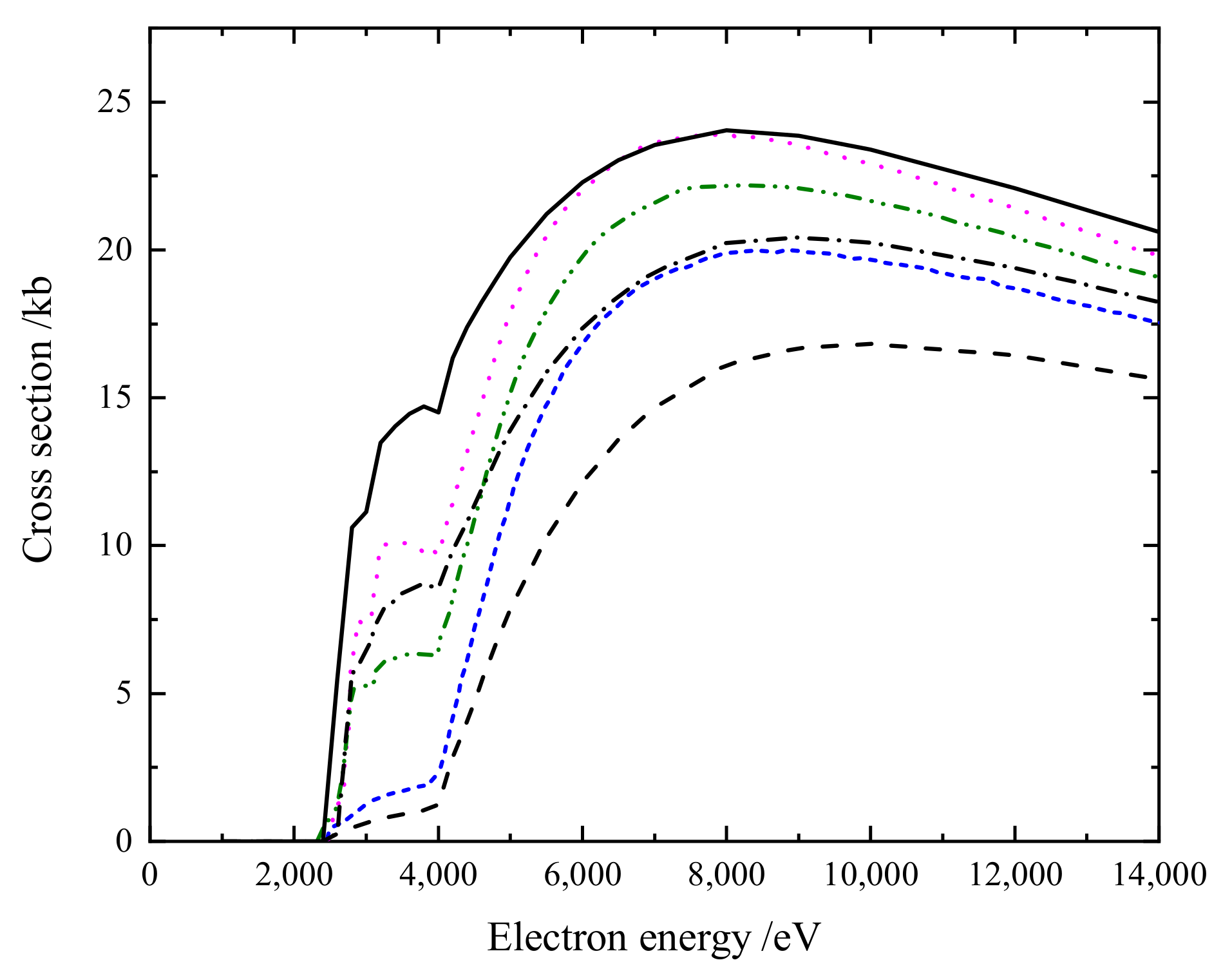
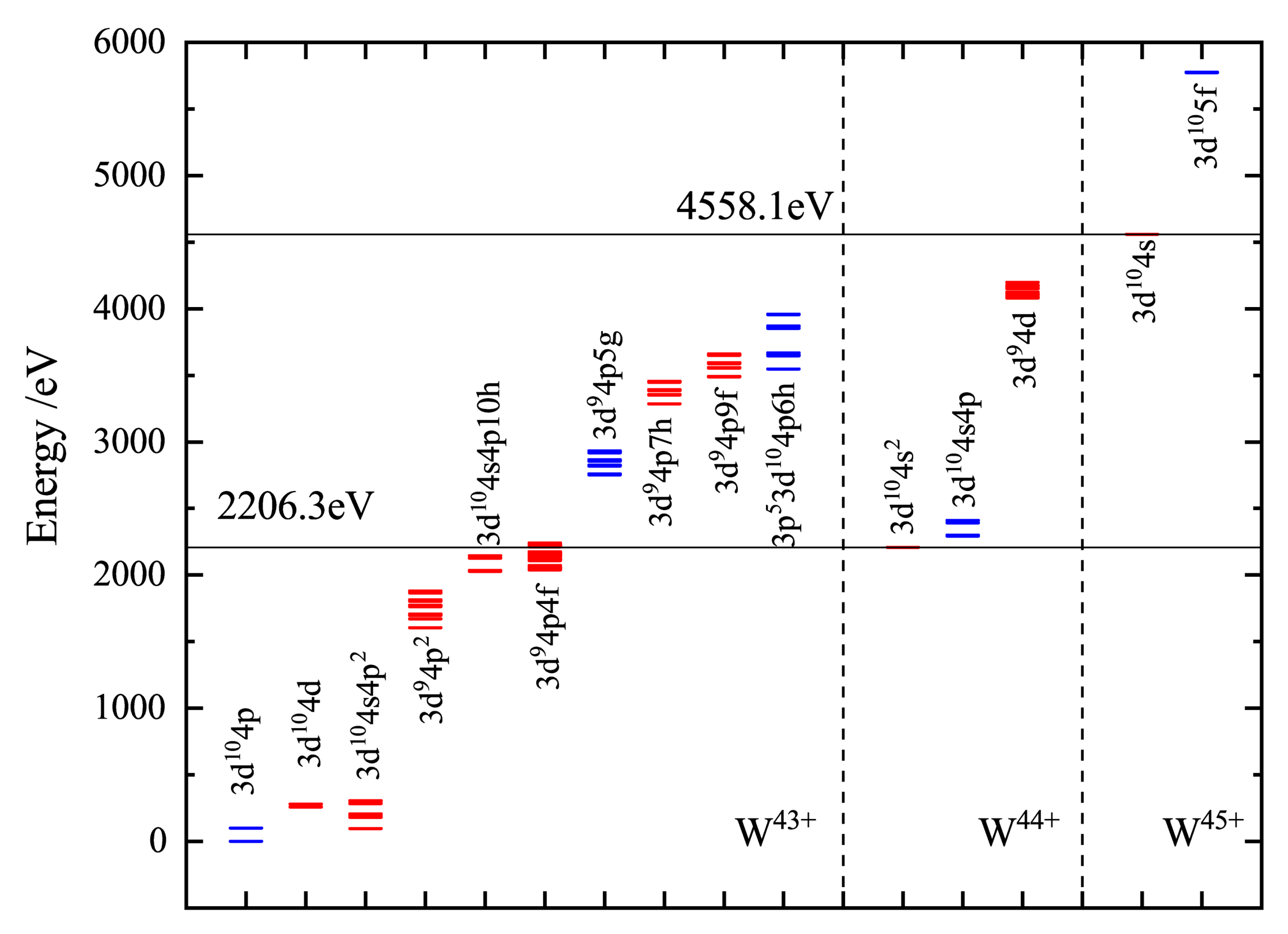
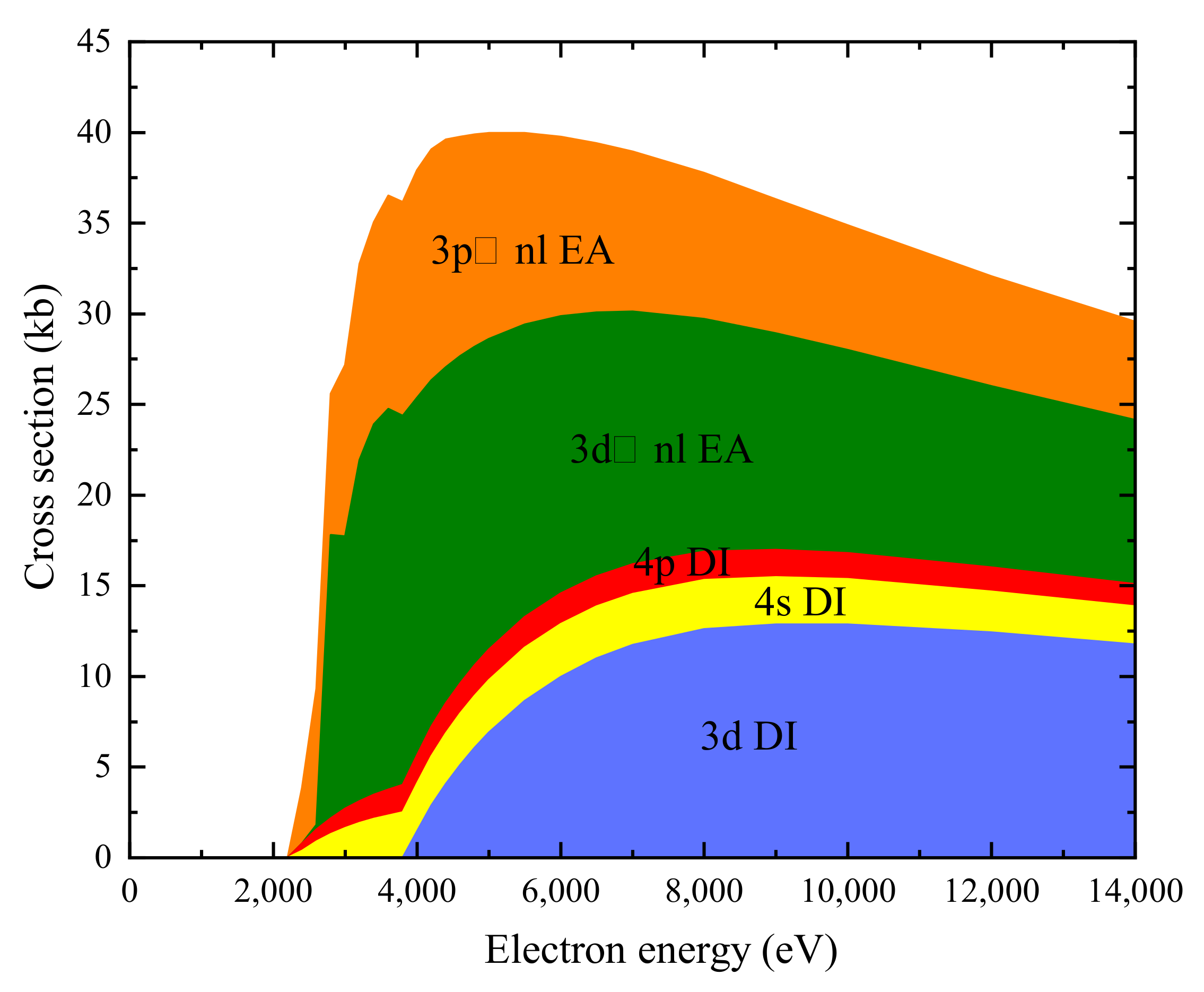
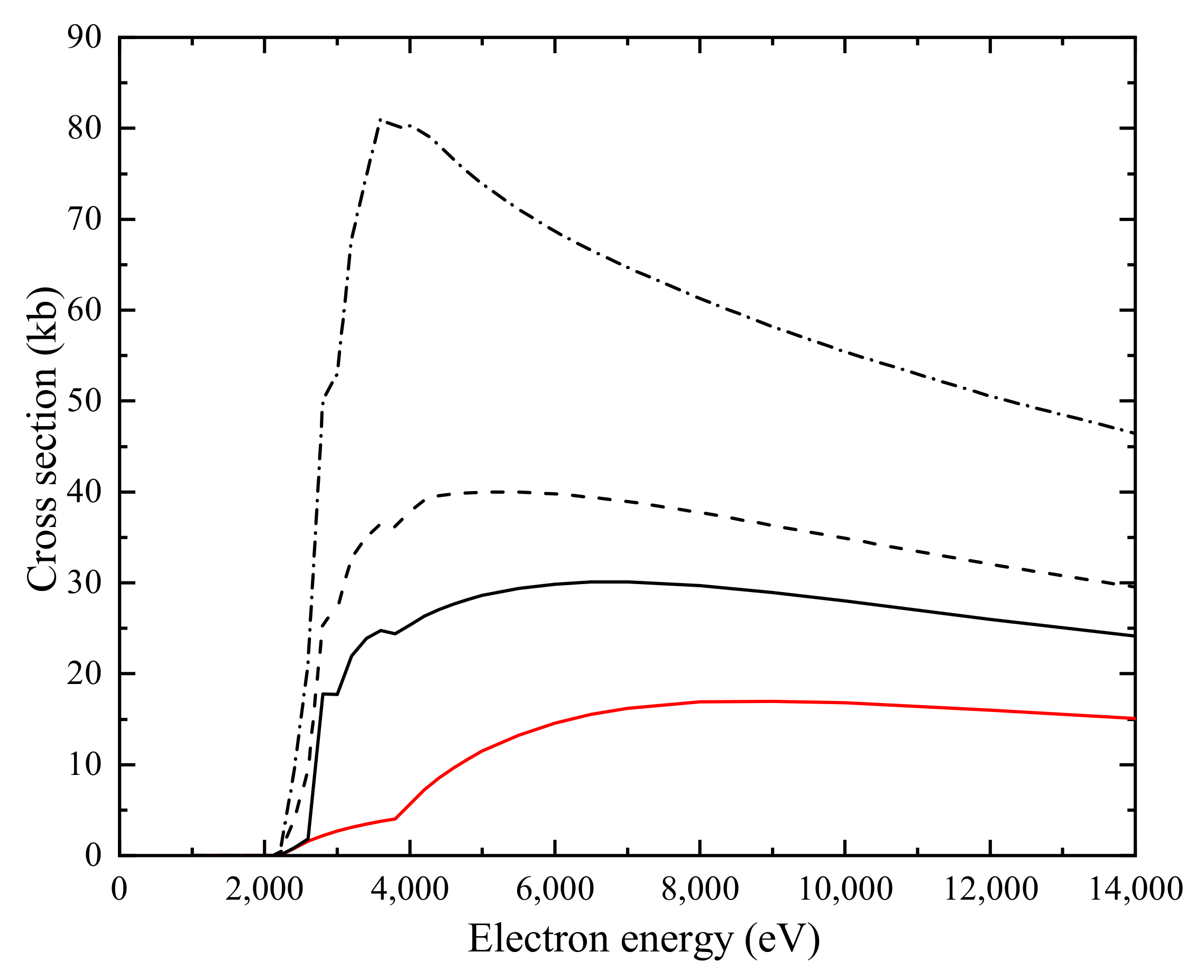


| Ions | Configuration | Direct Subshell Contributions | Excitation Subshell Contributions |
|---|---|---|---|
| W | [Ar]3d4s4p | 4p,4s,3d | 3d |
| W | [Ar]3d4s4p | 4p,4s,3d | 3d,4s |
| W | [Ar]3d4s4p | 4p,4s,3d | 3d,4s |
| W | [Ar]3d4s4p | 4p,4s,3d | 3d,4s |
| W | [Ar]3d4s4p | 4p,4s,3d | 3d,4s |
| W | [Ar]3d4s4p | 4p,4s,3d | 3d,3p |
| W | [Ar]3d4s | 4s,3d,3p | 3d,3p |
| W | [Ar]3d4s | 4s,3d,3p | 3d,3p |
| Ions | Configuration | FAC | NIST [25] | Relative Difference |
|---|---|---|---|---|
| W | [Ar]3d4s4p | 1.8289 × | 1.8298 × | 0.0492% |
| W | [Ar]3d4s4p | 1.8818 × | 1.8829 × | 0.0584% |
| W | [Ar]3d4s4p | 1.9387 × | 1.9406 × | 0.0979% |
| W | [Ar]3d4s4p | 1.9944 × | 1.9948 × | 0.0201% |
| W | [Ar]3d4s4p | 2.1452 × | 2.1491 × | 0.1815% |
| W | [Ar]3d4s4p | 2.2063 × | 2.2100 × | 0.1674% |
| W | [Ar]3d4s | 2.3518 × | 2.3545 × | 0.1147% |
| W | [Ar]3d4s | 2.4134 × | 2.4141 × | 0.0290% |
| Ions | Initial Configuration | Final Configuration | Ionization Potentials (keV) |
|---|---|---|---|
| W | 3d4s4p | 3d4s4p | 1.829 |
| 3d4s4p | 2.036 | ||
| 3d4s4p | 3.471 | ||
| 3d4s4p | 3.711 | ||
| W | 3d4s4p | 3d4s4p | 1.882 |
| 3d4s4p | 2.079 | ||
| 3d4s4p | 3.538 | ||
| 3d4s4p | 3.821 | ||
| W | 3d4s4p | 3d4s4p | 1.939 |
| 3d4s4p | 2.127 | ||
| 3d4s4p | 3.607 | ||
| 3d4s4p | 3.933 | ||
| W | 3d4s4p | 3d4s4p | 1.994 |
| 3d4s4p | 2.182 | ||
| 3d4s4p | 3.677 | ||
| 3d4s4p | 4.140 | ||
| W | 3d4s4p | 3d4s4p | 2.145 |
| 3d4s4p | 2.241 | ||
| 3d4s4p | 3.750 | ||
| 3d4s | 4.352 | ||
| W | 3p3d4s4p | 3p3d4s | 2.206 |
| 3p3d4s4p | 2.291 | ||
| 3p3d4s4p | 3.825 | ||
| 3p3d4s4p | 4.280 | ||
| 3p3d4s | 4.558 | ||
| W | 3p3d4s | 3p3d4s | 2.352 |
| 3p3d4s | 3.901 | ||
| 3p3d4s | 4.355 | ||
| 3p3d | 4.765 | ||
| W | 3p3d4s | 3p3d | 2.413 |
| 3p3d4s | 3.975 | ||
| 3p3d4s | 4.427 | ||
| 3p3d | 6.645 |
| Ions | Transition | Cross Section (Mb) |
|---|---|---|
| W | 3d → 4l (3.6 keV) | 4.035 × |
| 3d → 5l | 8.980 × | |
| 3d → 10l | 3.062 × | |
| W | 3d → 4l (3.4 keV) | 3.854 × |
| 3d → 5l | 1.028 × | |
| 3d → 10l | 3.200 × | |
| 4s → 9l (2.0 keV) | 1.691 × | |
| 4s → 10l | 1.334 × | |
| W | 3d → 4l (3.6 keV) | 2.888 × |
| 3d → 5l | 9.690 × | |
| 3d → 10l | 5.889 × | |
| 4s → 9l (2.0 keV) | 1.846 × | |
| 4s → 10l | 8.797 × | |
| W | 3d → 4l (3.6 keV) | 1.025 × |
| 3d → 5l | 7.560 × | |
| 3d → 10l | 1.162 × | |
| 4s → 9l (2.2 keV) | 9.182 × | |
| 4s → 10l | 1.269 × | |
| W | 3d → 5l (3.6 keV) | 7.880 × |
| 3d → 6l | 2.650 × | |
| 3d → 10l | 2.868 × | |
| 4s → 10l (2.2 keV) | 8.790 × | |
| W | 3d → 4l (3.8 keV) | 1.636 × |
| 3d → 6l | 4.240 × | |
| 3d → 10l | 3.700 × | |
| 3p → 4l (4.0 keV) | 1.263 × | |
| 3p → 6l | 3.280 × | |
| 3p → 10l | 1.039 × | |
| W | 3d → 5l (3.8 keV) | 6.070 × |
| 3d → 6l | 2.000 × | |
| 3d → 10l | 1.874 × | |
| 3p → 4l (4.0 keV) | 3.890 × | |
| 3p → 5l | 1.650 × | |
| 3p → 10l | 2.896 × | |
| W | 3d → 5l (3.8 keV) | 4.690 × |
| 3d → 6l | 1.540 × | |
| 3d → 10l | 1.330 × | |
| 3p → 4l (4.0 keV) | 3.730 × | |
| 3p → 5l | 1.400 × | |
| 3p → 6l | 3.125 × | |
| 3p → 10l | 3.671 × |
| Ions | I | Coefficients | 0 | 1 | 2 | 3 | 4 | 5 | 6 | 7 | 8 |
|---|---|---|---|---|---|---|---|---|---|---|---|
| W | 1828.9 | −1.193 × | 1.245 × | 4.011 × | −3.426 × | 1.588 × | −3.938 × | 5.518 × | −4.090 × | 1.260 × | |
| W | 1881.8 | −2.644 × | 2.820 × | 3.374 × | −3.088 × | 1.748 × | −4.859 × | 7.416 × | −5.878 × | 1.921 × | |
| W | 1938.7 | 2.020 × | −1.916 × | −3.114 × | −7.557 × | 2.241 × | −2.957 × | −4.633 × | 4.137 × | −2.687 × | |
| W | 1994.4 | −2.949 × | 3.167 × | −8.707 × | 1.109 × | −4.990 × | −7.115 × | 2.113 × | −2.335 × | 9.584 × | |
| W | 2145.2 | −2.166 × | 2.216 × | 2.207 × | 4.400 × | 7.520 × | −7.466 × | 1.830 × | −1.904 × | 7.572 × | |
| W | 2206.3 | 5.257 × | −5.091 × | −7.169 × | 4.875 × | −2.555 × | 6.852 × | −1.035 × | 8.233 × | −2.737 × | |
| W | 2351.8 | −4.332 × | 4.383 × | 2.388 × | −2.984 × | 3.324 × | −1.169 × | 2.312 × | −2.290 × | 9.932 × | |
| W | 2413.4 | −1.218 × | 1.243 × | 1.223 × | −4.974 × | 2.190 × | −5.046 × | 7.507 × | −6.382 × | 2.423 × |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Bao, R.; Wei, J.; Li, B.; Chen, X. Electron-Impact Ionization of the Tungsten Ions: W38+ − W45+. Atoms 2022, 10, 92. https://doi.org/10.3390/atoms10030092
Bao R, Wei J, Li B, Chen X. Electron-Impact Ionization of the Tungsten Ions: W38+ − W45+. Atoms. 2022; 10(3):92. https://doi.org/10.3390/atoms10030092
Chicago/Turabian StyleBao, Runjia, Junkui Wei, Bowen Li, and Ximeng Chen. 2022. "Electron-Impact Ionization of the Tungsten Ions: W38+ − W45+" Atoms 10, no. 3: 92. https://doi.org/10.3390/atoms10030092
APA StyleBao, R., Wei, J., Li, B., & Chen, X. (2022). Electron-Impact Ionization of the Tungsten Ions: W38+ − W45+. Atoms, 10(3), 92. https://doi.org/10.3390/atoms10030092






