Abstract
Research on superheavy elements enables probing the limits of nuclear existence and provides a fertile ground to advance our understanding of the atom’s structure. However, experimental access to these atomic species is very challenging and often requires the development of new technologies and experimental techniques optimized for the study of a single atomic species. The Laser Resonance Chromatography (LRC) technique was recently conceived to enable atomic structure investigations in the region of the superheavy elements. Here, we give an update on the experimental progress and simulation results.
1. Introduction
In the last two decades, there have been outstanding and exceptional efforts in the discovery and study of the superheavy elements []. One of the highlights is the completion of the seventh row in the periodic table with the addition of four new synthetic elements in 2016, including oganesson (Og, element number ), the last and heaviest element to date. The development of new selective and efficient techniques has had an impact on the discovery of these elements and their detailed study. Some of these elements are predicted to not behave chemically like their lighter homologs, with relativistic effects being the dominant cause of this peculiarity [,].
The challenges to study them are manifold. Superheavy elements are produced in nuclear fusion-evaporation reactions using powerful accelerators at extremely low rates in the presence of a huge background from primary-beam particles. In addition, they usually exist only for a few seconds after their production, which explains why their basic chemical and atomic properties are often not known []. Efficient gas chromatography has been used to elucidate the adsorption enthalpies. The heaviest element studied with this technique is flerovium (Fl, ), with a half-life ranging between one and two seconds [,,]. A few years ago, experiments using surface-ionization techniques were successfully applied to lawrencium (Lr, ), aiming at establishing the element’s ionization potential [].
Deeper insights into the atomic properties and structure can be gained from optical spectroscopy. At present, in-gas-cell laser resonance ionization spectroscopy [,,] is the most advanced method for atomic structure studies on the heaviest elements. A recent breakthrough in this research field was achieved with the spectroscopy of nobelium (No, ) [] using the RAdiation-Detected-Resonance-Ionization-Spectroscopy (RADRIS) technique.
Our alternative way of optical spectroscopy, namely, Laser Resonance Chromatography, has already been proposed for optical spectroscopy of lawrencium ions and is explained in detail in Ref. []. Briefly, this technique combines resonant laser excitation with electronic-state chromatography [,,,] and is conducted directly on the ion in-situ, without the need for a neutralization step. Given the fusion products are stopped and extracted from a gas catcher in a +1 charge state, a laser of a proper wavelength optically pumps the ions into a metastable state from the ionic ground state. After this step, the ions are injected into a drift tube filled with diluted helium (He) gas, where they undergo a constant drift under the influence of an external electric field. Different interactions of the ions in the different states with helium result in state-specific ion mobilities, which enable modern electronic-state chromatography, i.e., separating the ions in the ground state from the metastable ions by drift time [,]. In other words, the changes in the arrival time distributions caused by laser excitations give the resonance signal. Although only applicable to ions and dependent on the presence of metastable states, the method of electronic state chromatography is well established for many elemental cations of the first-, second- and third-row transition metals [,,,,,].
To this end, a first-generation drift tube chamber has been designed for LRC applications. This design is different from traditional ion-mobility-experiment applications since suppressing deactivation of metastable states is mandatory and is pursued by reducing the length of the tube and operating at relatively low pressures.
In the next two sections, we give a brief report on the experimental progress of the laser resonance chromatography project by presenting the experimental apparatus including the laser system, the cryogenic drift tube, and the corresponding ion trajectory simulations. Due to the scarcity of data and since scandium (Sc, ) can be deemed as a homolog of lutetium (Lu, ), the later simulations were conducted for singly charged scandium in its ground and metastable states of known ion mobilities. In the last section, we give prospects of LRC experiments on Sc, Lu and its heavier iso-electronic system, Lr. A summary of the important properties of these elements is compiled in Table 1.

Table 1.
Relevant electronic states in Sc, Lu, and Lr ions. The experimental ion mobilities are given for a helium temperature of K. Predictions are marked by ★.
2. Experimental Approach
Laser resonance chromatography couples laser spectroscopy with ion mobility spectrometry. It is based on a population transfer between metastable ionic states in a resonant laser excitation process. A laser excites the ion, e.g., from the ground state to an intermediate level (to be optically probed) in an allowed optical transition. The intermediate level depopulates partly to lower-lying metastable states that do not easily quench to the ground state. In a simplified picture, the ion changes its size during this process, which can then be exploited for purposes of diagnostics. The resonant process is identified by a change in the characteristic arrival-time distributions of the ions on a particle detector after passing a drift tube filled with helium gas at pressures mbar. Since the mobility is function of the gas temperature and could be distinct for the different states, the operation of the drift tube at cryogenic temperatures usually provides an additional degree of freedom to optimize time resolution and state separation [,].
The method is generally applicable for transition-metal ions including Lu and Lr. Promising optical pumping schemes for singly charged rutherfordium, the next-heavier element within the fourth row transition metals, have already been proposed []. Compared to many existing spectroscopy techniques, the LRC approach has a number of key advantages, some of which are:
- No neutralization of the thermalized fusion products is required. The ions can be manipulated and guided with high efficiency by electric fields;
- No further ionization of the ions is required. Only one laser is needed for spectroscopy;
- No radiation detection is required as in fluorescence spectroscopy. The sensitivity does not depend on the solid angle coverage of the detectors;
- A mass filter is a useful option at low ion production rates, but is not mandatory to suppress molecular sidebands or even isobars of different electronic configurations, as the drift itself provides the required ion discrimination.
2.1. The LRC Apparatus
The LRC setup is shown in Figure 1. It consists of five different pressure sections (PS) for stopping, extraction, separation, mass selection and detection of the sample ions. The ionized residual nuclei produced during the fusion-evaporation process lose most of their kinetic energy by passing through a metallic window of a few m thickness before they are thermalized by collisions with the He buffer gas inside of the stopping cell (PS1) at a pressure of about 60 mbar. The thermalized ions are ejected through a convergent–divergent nozzle of mm throat diameter towards a radio frequency quadrupole (RFQ) in PS2 that serves to extract and further cool the ions and to guide them towards the buncher in the next pumping section (PS3). The first-generation stopping cell of the SHIPTRAP setup together with its extraction RFQ i is used for this purpose [].
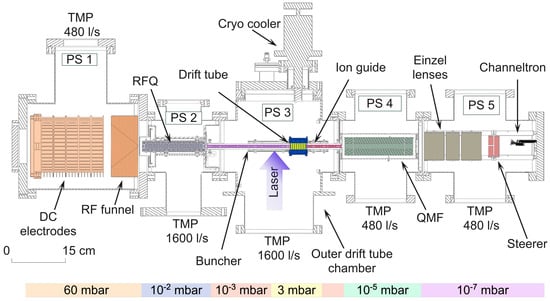
Figure 1.
Schematic overview of the LRC apparatus. See text for more information.
The subsequent buncher installation enables a spatial confinement of the ions for laser spectroscopy and a precise referencing of their arrival time distributions. It consists of four stainless steel rods with diameters of mm, each divided into 25 segments. The distance between opposite rods is mm. PS3 also incorporates a cryogenic drift tube and an ion guide. The drift tube is used for electronic-state chromatography and is explained in more detail in Section 2.3. The ion guide comprises 10 segments of similar geometry as the buncher segments and is used to transport and focus the ions into the quadrupole mass filter (Extrel QMS) in the pumping section PS4, where the ions are selected based on their mass-to-charge ratio. Next, the ions are focused by einzel lenses and a X and Y steerer towards the detection system, which contains a channeltron detector (Dr Sjuts K15) installed in the last pumping section PS5.
2.2. The Laser System
One of the characteristics and a potential advantage of the LRC technique compared with conventional resonance ionization spectroscopy is the use of only one laser beam to search for optical resonances by optical pumping of metastable states. The laser system is shown in Figure 2. It consists of a 10-kHz Nd:YAG laser (Edgewave, 90 W at 532 nm) that pumps a dye laser (Sirah Credo), providing laser pulse energies between 10 and J in the ultraviolet (UV) range from 220 up to nm after frequency doubling or tripling, depending on the dye. Both lasers are installed next to the LRC apparatus and the laser beam path and optics are arranged as shown in Figure 2. The fundamental wavelength is monitored using a wavelength meter (HighFinesse WS7 UVU) featuring autocalibration via an integrated calibration source. For initial experiments and offline studies, a Nd:YAG laser (Continuum Minilite II) operated at Hz repetition rate was used in addition to produce ions via ablation from primed samples inserted inside of the stopping cell (PS1).
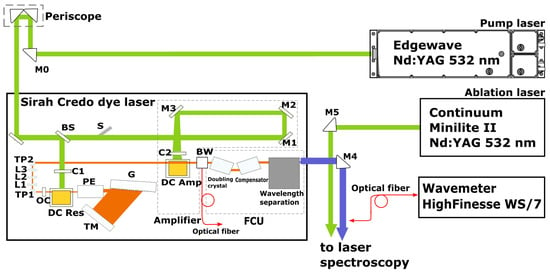
Figure 2.
LRC laser system. Edgewave Nd:YAG laser pumps a Sirah Credo dye laser. Laser ablation is carried out using a Continuum Minilite II Nd:YAG laser. The wavemeter is used for wavelength monitoring. Abbreviations: M, mirror; L, lens; TP, telescopic lens; C, cylindrical lens; BS, beam splitter; BW, Brewster window; G, grating; PE, prism expander; DC Res, dye cell resonator; DC Amp, dye cell amplifier; OC, output coupler; FCU, frequency conversion unit.
2.3. The Drift Tube Outer Chamber
The main components of the drift tube section are shown in Figure 1 (PS3). The section incorporates two stainless steel chambers: The outer vacuum chamber and the cryogenic drift tube are connected to the buncher on the left side and to the ion guide on its exit on the right side. The outer chamber has a cuboid shape with edge lengths of (L × W × H) = (mm × mm × mm). A 1600 l/s turbomolecular pump (TMP, Edwards STP 1603C) is connected to this chamber via a DN-200 ConFlat flange to pump it down to pressures <mbar in standby mode or <mbar in operation mode. The chamber provides vacuum and thermal shielding for the cryogenic drift tube and features exhibits high voltage and RF feedthroughs, a gas inlet, pressure gauges, electrical feedthroughs for heaters and temperature sensors, view ports for the laser beam and a DN-63 ConFlat flange to connect a free piston Stirling cryocooler (CryoTel-CT). The latter has a cooling capacity of about W at K and is connected to the drift tube via four copper strands with a cross sectional area of mm.
2.4. The Drift Tube Inner Chamber
The cryogenic drift tube sits at the heart of the LRC apparatus. A schematic overview of this is shown in Figure 3. The tube has a hexagonal shape with an inner diameter of mm and a length of mm. It is fixed to the outer chamber via 12 titanium spokes (M2, DIN 975/DIN 976 Titanium Grade 2). The drift tube chamber is plated on the outside with a thin copper layer of 50–100 m thickness to enable better heat conductance and a homogeneous distribution of the temperature over the whole drift tube during cooling and warming phases. It includes exhibits a gas inlet and outlet, a connection for a pressure gauge (Pfeiffer Vacuum PKR 360) and several tapered holes to fix heaters (high power resistors TCP100U) and temperature sensors (Lake Shore Germanium-CD). The tube has octagonal flanges at both ends that also serve to attach it to the spokes on the outer chamber.
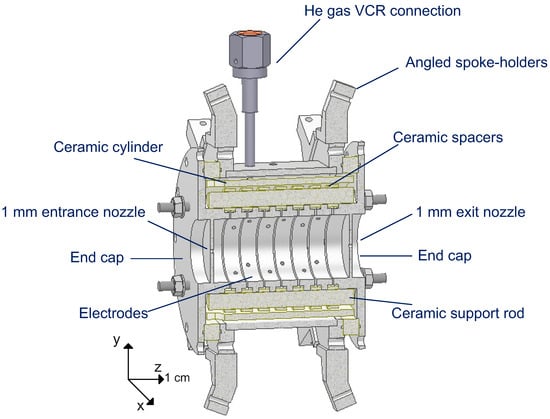
Figure 3.
3D cross-sectional view of the LRC drift tube and its components.
In its interior, the drift tube chamber incorporates eight stainless steel electrodes of mm inner diameter, mm outer diameter and of a width of mm. Six of the electrodes are enclosed by two identical end caps designed to have an electrode in one side and a diaphragm of mm on the other side, serving either as injection or exit nozzle. The caps also serve as a support for the stainless steel fixation of the buncher and the ion guide; cf. Figure 3. All inner electrodes are electrically connected to each other by seven 1-M resistors in series to build built up a resistance of M between the end caps. The electrodes are supported via Vitronit ceramic rods of mm diameter and mm length and separated by mm from each other with Vitronit ceramic cylindrical spacers of mm length and mm inner diameter. All inner electrodes plus the end caps are surrounded by a ceramic cylinder of mm inner diameter, mm outer diameter and length of mm to isolate the electrodes from the grounded tube housing.
3. Ion Drift Simulations
Simulations were performed for the drift tube using the SIMION software package [] in order to estimate Sc drift times at a given He pressure and temperature and to extract suitable voltage configurations to be used in future LRC experiments. In the simulations, we considered both Statistical Diffusion (SDS) and Viscous Damping (VD) models []. Hard sphere model simulations could not be performed thus far due to the lack of reference data for the collision cross sections.
The reduced mobility for Sc in the ground state and Sc in the metastable state for the SDS and VD models were taken from []; cf. Table 1. We tested two configurations, one with a roughly constant electric field (unfocused beam) and a second with a gradually increasing electric field (focused beam). Ions were generated at the entrance of the drift tube and their drift times were recorded when they exited through a mm or mm diameter nozzle to explore the feasibility of enhancing the transmission while keeping time resolution unchanged. The two voltage configurations we used are shown in Table 2. We simulated different voltage values of U in a way that the resulting average ratio of electric-field strength to gas number density, E/n, spanned a range between 1 and Td, with 1 Td = 10. For each value of U, ions were generated in a 3D Gaussian distribution with a standard deviation of mm. The ion mobilities were calculated from the reduced mobilities by considering a pressure of mbar of helium gas at a temperature of K. Figure 4a shows ion trajectories projected on the symmetry plane obtained for the two different electric field configurations at an average reduced field of Td. Using the VD model, the electric fields acting on each ion during its drift were recorded for each voltage configuration and allowed us to extract the mean electric field; cf. Figure 4b.

Table 2.
Voltage configurations for unfocused (*) and focused beam (**). n is the electrode number. In the case of the unfocused beam, the voltages applied to the different electrodes were scaled by n, whereby values of U between 0.1–10 V were applied in steps of 0.1 V to span a range of E/n between 1–30 Td. For the focused beam, we added different offsets to the unfocused beam configurations as given in the table, where V and U was varied between 0.1–2.7 V to span in total an E/n range between 1–30 Td.
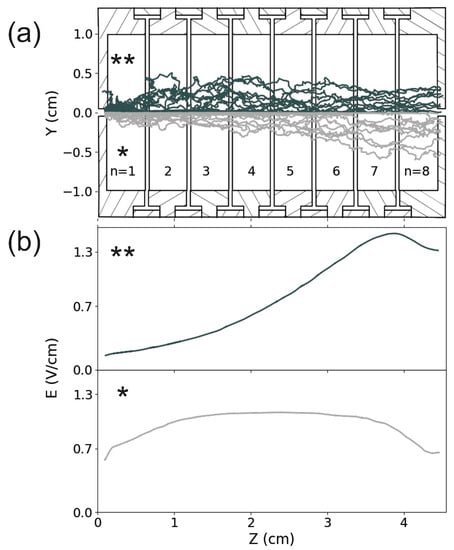
Figure 4.
(a) Trajectories for E/n = 15 Td for unfocused (*) and focused beams (**). (b) Electric fields along the electrodes for focused (top) and unfocused (bottom) beams.
Results
We made a comparison of the transmission efficiency between focused and unfocused beam configurations and between 1 mm and 2 mm exit nozzles for Sc ions. To this end, we defined this efficiency as the fraction of the number of ions arriving at the exit nozzle within a radius of mm (mm) from the center axis respective to the initial number of ions for mm (mm) nozzle diameters. Figure 5 shows this transmission efficiency for the different states as function of the reduced field. For the unfocused beam using the mm diameter exit nozzle, it grows quickly as the reduced field increases to reach a maximum of % at an E/n value of about Td and decreases with increasing fields to stagnate at about 2%. When using the mm diameter exit nozzle, it grows even quicker as the reduced field increases to reach a maximum of % at an E/n value of about Td and stays stable up to around Td to then increase again. According to the simulations, there is nearly no difference for the unfocused beam in terms of transmission efficiency between the ground and the metastable state for both mm and mm exit diameters below 20 Td. In the case of a focused beam using the mm exit nozzle, the efficiency follows the unfocused beam up to Td, then gradually increases with increasing fields, but stays below that achieved for the unfocused beam till reaching Td. In the case of the mm nozzle, the focused beam and the unfocused beam have similar transmission up to Td. From these values onward for both nozzles, mm and mm, the deviation in the transmission of the two states becomes apparent. A maximum transmission efficiency of for mm and 20% for mm nozzles in the focused beam configuration is achieved for the ground state ions at Td, while the metastable metatstable state transmission stagnates at about 5% and 15% for mm and mm, respectively. Since the ions in the ground state exhibit a higher mobility, they can drift faster compared with the ions in the metastable states and thus are less prone to transversal diffusion losses. Theoretically, even higher efficiencies can be expected for these latter scenarios if the reduced field is increased beyond Td, but only at the cost of deactivating states due to gas collisions that would degrade the metastable signal [,,]. In addition, increased electric fields carry the risk of gas discharges with only a few hundred volts for 2 mbar of He gas and lead to shorter drift times due to higher velocity, which can in turn lead to both neutralization and a lower resolving power, respectively.
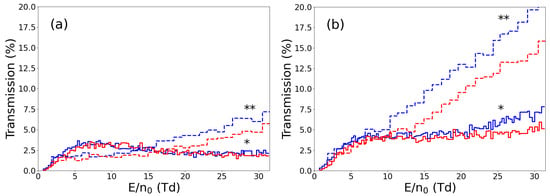
Figure 5.
Ion transmission for unfocused (*) and focused (**) beams for both ground (blue) and metastable states (red) and for (a) mm and (b) mm exit diameter nozzles. Dash-line: focused beam; solid line: unfocused beam.
To better understand the behavior of the different electric field configurations in terms of resolution, we analyzed the drift time differences between the ground state and metastable state. Figure 6 shows the drift time for the two Sc states in the different beam configurations in the case of the mm nozzle; similar behavior was observed for the mm exit diameter nozzle. It becomes apparent that, irrespective of the electronic states, the gradual increase of the electric field (corresponding to the beam focusing scenario) cause the ions to drift at small velocities the majority of the time and to lag behind in comparison when they are exposed to an average but rather homogeneous electric field (unfocused beam). The relative drift time differences exhibit a maximum at reduced field values between Td and Td in both configurations, indicating the best time resolution. However, a deeper insight is obtained by including peak broadening effects in the analysis by comparing the time histograms of the transmitted ions from the simulations. The different ionic states can be disentangled better from each other at larger reduced fields, which means at smaller absolute drift times and thus at the cost of relative drift time differences. If we compare the two different configurations, it becomes clear that, here as well, the unfocused beam provides better working conditions because it provides better time resolution over a larger range of E/n values. In the case of a focused beam, the time peaks can be partly disentangled only at fields higher than ≈10 Td. The mm configuration shows a similar trend with respect to the resolution. However, even though the larger nozzle provides higher efficiency, this latter is not so remarkably higher as to trade off the vacuum conditions inside of the PS3 section. We can consider this option for future tests.
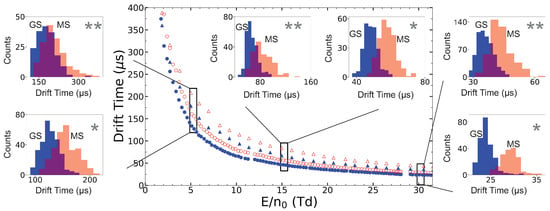
Figure 6.
Absolute drift time comparison between ground and metastable states for both focused and unfocused beams. Unfocused beam: ● = ground state; ○ = metastable state. Focused beam: ▲ = ground state; △ = metastable state. Insets: selected histograms for E/n = 5, 15, and 30 Td to demonstrate resolution behavior. * = unfocused beam; ** = focused beam; blue = ground state; red = metastable state.
4. Current Status and Outlook
In the summer of 2022, the LRC setup is nearly complete and the commissioning phase has already begun with testing of the vacuum and functionality of key components such as the buffer gas stopping cell, the quadrupole mass filter and the laser systems. The cryogenic drift tube together with the miniature ion guide and buncher are being assembled and are ready for integration into the setup. Different ion sources are available, including a laser ablation source and a Ra recoil ion source, with the latter being best suited for optimizing and quantifying the transmission efficiency through the whole apparatus.
SIMION simulations were performed for the LRC drift tube using two electric field configurations: unfocused and focused beams; and two geometry configurations: mm and mm exit nozzle diameters. From these simulations, we inferred that a rather homogeneous electric field enables a comparably higher ion transmission while maintaining a good resolution at relatively low E/n values using a mm exit diameter nozzle. In addition, it can be expected that working at lower fields minimizes the risk of gas discharges and deactivation of states. This can therefore also be very beneficial for a successful application of the LRC method. Higher transmission can be achieved when using a 2 mm exit nozzle and eventually focusing the beam into the nozzle.
Our first proof-of-principle experiments will target Sc, cf. Table 1. These offline measurements are currently being prepared and we expect them to last for up to one year. The relative mobility difference between the ground and the metastable state is about 20% at K in He [], which should be sufficient to enable LRC measurements. The laser probing occurs inside the buncher, i.e. before the ion drift, via laser resonant excitation of the D state at 27,917.78 cm to optically pump the ion into the metastable state F at 4802.87 cm. Since the metastable state is energetically relatively close to the ground state, deactivation of states will likely occur during the ion-atom collisions []. If such collisional de-excitations dominate and entirely prevent the chromatography of Sc, we will pursue LRC experiments on Lu, the lighter chemical homologue of Lr. In the Lu experiments, we will probe the P state at 28,503.16 cm that feeds the D metastable state at 11,796.24 cm. Since this latter state is energetically high enough above the ground state, level crossings in the corresponding diabatic potential curves become unlikely. Thus, for short drift paths, as in the LRC experiments, we expect deactivation of states to be suppressed in Lu-He collisions, particularly at moderate kinetic energies. Here, one should note that in Ref. [], the signal of the metastable state could still be observed even for Sc drifting inside a drift tube of about m length. However, since the drift tube of the LRC apparatus is only mm long, the chromatography will require detailed analysis of the arrival-time distributions due to expected moderate time resolution; cf. Figure 6.
Applying the LRC technique to stable Lu ions can give us a better understanding of the trade-off we should make to achieve maximum count rates without losing the chromatography information. Once experimentally optimized for low yields, LRC can then be applied to search for atomic levels in the heavier iso-electronic system Lr in on-line experiments.
Author Contributions
Conceptualization, M.L.; Setup design, E.R.R and M.L.; Simulations, E.R.R.; Simulations Methodology, E.R.R. and M.L.; Formal analysis, E.R.R.; Funding acquisition, M.L.; Investigation, E.R.R. and M.L.; Project administration, M.L.; Resources, B.J., E.K., H.R., E.R. and J.S.; Software, E.R.R.; Supervision, M.B. and M.L.; Validation, E.R.R, M.B., S.R. and M.L.; Visualization, E.R.R.; Writing—original draft, E.R.R; Writing—review & editing, E.R.R., M.B., M.L., S.N., S.R. and P.S. All authors have read and agreed to the published version of the manuscript.
Funding
This project has received funding from the European Research Council (ERC) under the European Union’s Horizon 2020 Research and Innovation Programme (Grant Agreement No. 819957).
Institutional Review Board Statement
Not applicable.
Informed Consent Statement
Not applicable.
Data Availability Statement
Not applicable.
Acknowledgments
We would like to thank the workshops of the Chemistry, Nuclear Chemistry, Physics and Nuclear Physics departments of the JGU Mainz.
Conflicts of Interest
The authors declare no conflict of interest.
Abbreviations
The following abbreviations are used in this manuscript:
| LRC | Laser Resonance Chromatography |
| SHE | Super Heavy Elements |
| PS | Pressure Section |
| SDS | Statistical Diffusion Simulation |
| VD | Viscous Damping |
References
- Giuliani, S.A.; Matheson, Z.; Nazarewicz, W.; Olsen, E.; Reinhard, P.G.; Sadhukhan, J.; Schuetrumpf, B.; Schunck, N.; Schwerdtfeger, P. Colloquium: Superheavy elements: Oganesson and beyond. Rev. Mod. Phys. 2019, 91, 011001. [Google Scholar] [CrossRef]
- Nazarewicz, W. The limits of nuclear mass and charge. Nat. Phys. 2018, 14, 537–541. [Google Scholar] [CrossRef]
- Düllmann, C.E. Studying chemical properties of the heaviest elements: One atom at a time. Nucl. Phys. News 2017, 27, 14–20. [Google Scholar] [CrossRef]
- Yakushev, A.; Gates, J.M.; Türler, A.; Schädel, M.; Düllmann, C.E.; Ackermann, D.; Andersson, L.L.; Block, M.; Brüchle, W.; Dvorak, J.; et al. Superheavy element flerovium (element 114) is a volatile metal. Inorg. Chem. 2014, 53, 1624–1629. [Google Scholar] [CrossRef]
- Eichler, R.; Aksenov, N.; Albin, Y.V.; Belozerov, A.; Bozhikov, G.; Chepigin, V.; Dmitriev, S.; Dressler, R.; Gäggeler, H.; Gorshkov, V.; et al. Indication for a volatile element 114. Rca-Radiochim. Acta 2010, 98, 133–139. [Google Scholar] [CrossRef]
- Yakushev, A.; Lens, L.; Düllmann, C.E.; Block, M.; Brand, H.; Calverley, T.; Dasgupta, M.; Di Nitto, A.; Götz, M.; Götz, S.; et al. First study on nihonium (Nh, element 113) chemistry at TASCA. Front. Chem. 2021, 9, 753738. [Google Scholar] [CrossRef]
- Sato, T.K.; Sato, N.; Asai, M.; Tsukada, K.; Toyoshima, A.; Ooe, K.; Miyashita, S.; Schädel, M.; Kaneya, Y.; Nagame, Y.; et al. First successful ionization of Lr (Z = 103) by a surface-ionization technique. Rev. Sci. Instrum. 2013, 84, 023304. [Google Scholar] [CrossRef]
- Block, M.; Giacoppo, F.; Heßberger, F.P.; Raeder, S. Recent progress in experiments on the heaviest nuclides at SHIP. Riv. Nuovo C 2022, 45, 279–323. [Google Scholar] [CrossRef]
- Ferrer, R.; Barzakh, A.; Bastin, B.; Beerwerth, R.; Block, M.; Creemers, P.; Grawe, H.; de Groote, R.; Delahaye, P.; Fléchard, X.; et al. Towards high-resolution laser ionization spectroscopy of the heaviest elements in supersonic gas jet expansion. Nat. Commun. 2017, 8, 14520. [Google Scholar] [CrossRef]
- Laatiaoui, M.; Lauth, W.; Backe, H.; Block, M.; Ackermann, D.; Cheal, B.; Chhetri, P.; Düllmann, C.E.; Van Duppen, P.; Even, J.; et al. Atom-at-a-time laser resonance ionization spectroscopy of nobelium. Nature 2016, 538, 495–498. [Google Scholar] [CrossRef]
- Laatiaoui, M.; Buchachenko, A.A.; Viehland, L.A. Laser Resonance Chromatography of Superheavy Elements. Phys. Rev. Lett. 2020, 125, 023002. [Google Scholar] [CrossRef]
- Backe, H.; Lauth, W.; Block, M.; Laatiaoui, M. Prospects for laser spectroscopy, ion chemistry and mobility measurements of superheavy elements in buffer-gas traps. Nucl. Phys. A 2015, 944, 492–517. [Google Scholar] [CrossRef]
- Laatiaoui, M.; Backe, H.; Habs, D.; Kunz, P.; Lauth, W.; Sewtz, M. Low-field mobilities of rare-earth metals. Eur. Phys. J. D 2012, 66, 232. [Google Scholar] [CrossRef]
- Baumbach, J. Ion mobility spectrometry in scientific literature and in the International Journal for Ion Mobility Spectrometry (1998–2007). Int. J. Ion Mobil. Spectrom. 2008, 11, 3–11. [Google Scholar] [CrossRef]
- Mason, E.A.; McDaniel, E.W. Transport Properties of Ions in Gases; Wiley Online Library: Hoboken, NJ, USA, 1988; Volume 26. [Google Scholar]
- Kemper, P.R.; Bowers, M.T. State-selected mobilities of atomic cobalt ions. J. Am. Chem. Soc. 1990, 112, 3231. [Google Scholar] [CrossRef]
- Kemper, P.R.; Bowers, M.T. Electronic-state chromatography: Application to first-row transition-metal ions. J. Phys. Chem. 1991, 95, 5134–5146. [Google Scholar] [CrossRef]
- Iceman, C.; Rue, C.; Moision, R.M.; Chatterjee, B.K.; Armentrout, P.B. Ion mobility studies of electronically excited states of atomic transition metal cations: Development of an ion mobility source for guided ion beam experiments. J. Am. Soc. Mass Spectrom. 2007, 18, 1196. [Google Scholar] [CrossRef]
- Ibrahim, Y.; Alsharaeh, E.; Mabrouki, R.; Momoh, P.; Xie, E.; El-Shall, M.S. Ion mobility of ground and excited states of laser-generated transition metal cations. J. Phys. Chem. A 2008, 112, 1112–1124. [Google Scholar] [CrossRef]
- Manard, M.J.; Kemper, P.R. Ion Mobility Mass Spectrometry: The design of a new high-resolution mobility instrument with applications toward electronic-state characterization of first-row transition metal cations. Int. J. Mass Spectrom. 2016, 402, 1–11. [Google Scholar] [CrossRef]
- Manard, M.J.; Kemper, P.R. Characterizing the electronic states of the second-row transitionmetal cations using high-resolution ion mobility mass spectrometry. Int. J. Mass Spectrom. 2016, 407, 69–76. [Google Scholar] [CrossRef] [Green Version]
- Manard, M.J.; Kemper, P.R. An experimental investigation into the reduced mobilities of lanthanide cations using high-resolution ion mobility mass spectrometry. Int. J. Mass Spectrom. 2017, 423, 54–58. [Google Scholar] [CrossRef]
- Lawler, J.E.; Dakin, J.T. Absolute transition probabilities in Sc I and Sc II. J. Opt. Soc. Am. B 1989, 6, 1457. [Google Scholar] [CrossRef]
- Kramida, A.; Ralchenko, Y.; Reader, J. NIST Atomic Spectra Database (Ver. 5.7.1); National Institute of Standards and Technology: Gaithersburg, MD, USA, 2020. [CrossRef]
- Manard, M.J.; Kemper, P.R. Reduced mobilities of lanthanide cations measured using high-resolution ion mobility mass spectrometry with comparisons between experiment and theory. Int. J. Mass Spectrom. 2017, 412, 14. [Google Scholar] [CrossRef]
- Quinet, P.; Palmeri, P.; Biémont, E.; McCurdy, M.M.; Rieger, G.; Pinnington, E.H.; Wickliffe, M.E.; Lawler, J.E. Experimental and theoretical radiative lifetimes, branching fractions and oscillator strengths in Lu II. Mon. Not. Royal Astron. Soc. 1999, 307, 934. [Google Scholar] [CrossRef]
- Arifin. Lutetium Ion Spectroscopy. Bachelor’s Thesis, Department of Physics, National University of Singapore, Singapore, 2014.
- Visentin, G.; Laatiaoui, M.; Viehland, L.A.; Buchachenko, A.A. Mobility of the Singly-Charged Lanthanide and Actinide Cations: Trends and Perspectives. Front. Chem. 2020, 8, 438. [Google Scholar] [CrossRef] [PubMed]
- Kahl, E.V.; Berengut, J.C.; Laatiaoui, M.; Eliav, E.; Borschevsky, A. High-precision ab initio calculations of the spectrum of Lr+. Phys. Rev. A 2019, 100, 062505. [Google Scholar] [CrossRef]
- Ramanantoanina, H.; Borschevsky, A.; Block, M.; Laatiaoui, M. Electronic Structure of Lr+ (Z = 103) from Ab Initio Calculations. Atoms 2022, 10, 48. [Google Scholar] [CrossRef]
- Ramanantoanina, H.; Borschevsky, A.; Block, M.; Laatiaoui, M. State specific mobility of Lr+ ion in He. Phys. Rev. A 2022. Manuscript in preparation. [Google Scholar]
- Buchachenko, A.A.; Visentin, G.; Viehland, L.A. Gaseous transport properties of the ground and excited Cr, Co and Ni cations in He: Ab initio study of electronic state chromatography. J. Chem. Phys. 2022, in press. [CrossRef]
- Ramanantoanina, H.; Borschevsky, A.; Block, M.; Laatiaoui, M. Electronic structure of Rf+(Z=104) from ab initio calculations. Phys. Rev. A 2021, 104, 022813. [Google Scholar] [CrossRef]
- Neumayr, J.B.; Beck, L.; Habs, D.; Heinz, S.; Szerypo, J.; Thirolf, P.G.; Varentsov, V.; Voit, F.; Ackermann, D.; Beck, D.; et al. The ion-catcher device for SHIPTRAP. Nucl. Instrum. Methods Phys. Res. B 2006, 244, 489. [Google Scholar] [CrossRef]
- Scientific-Instrument-Services. SIMION 8.0. Available online: https://simion.com/ (accessed on 9 July 2022).
- Appelhans, A.D.; Dahl, D.A. SIMION ion optics simulations at atmospheric pressure. Int. J. Mass Spectrom. 2005, 244, 1–14. [Google Scholar] [CrossRef]
- Wilkins, C.L.; Trimpin, S. Ion Mobility Spectrometry-Mass Spectrometry: Theory and Applications; CRC Press: Boca Raton, FL, USA, 2010. [Google Scholar]
- Loh, S.; Fisher, E.; Lian, L.; Schulz, R.; Armentrout, P. State-specific reactions of Fe+ (6D, 4F) with O2 and c-C2H4O: D∘ 0 (Fe+-O) and effects of collisional relaxation. J. Phys. Chem. 1989, 93, 3159–3167. [Google Scholar] [CrossRef]
- Chhetri, P.; Ackermann, D.; Backe, H.; Block, M.; Cheal, B.; Düllmann, C.E.; Even, J.; Ferrer, R.; Giacoppo, F.; Götz, S.; et al. Impact of buffer gas quenching on the 1S0→1P1 ground-state atomic transition in nobelium. Eur. Phys. J. D 2017, 71, 195. [Google Scholar] [CrossRef]
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).