Cosmic-Ray Acceleration and Magnetic Fields in Galaxy Clusters and Beyond: Insights from Radio Observations
Abstract
1. Introduction
2. Large-Scale Motions in the ICM
3. Measuring Magnetic Fields and Cosmic Rays
4. The Evolution of Magnetic Fields
5. Radio Halos—Turbulent Re-Acceleration in the ICM
5.1. Pure Hadronic Model of Radio Halos
5.2. Turbulent Re-Acceleration
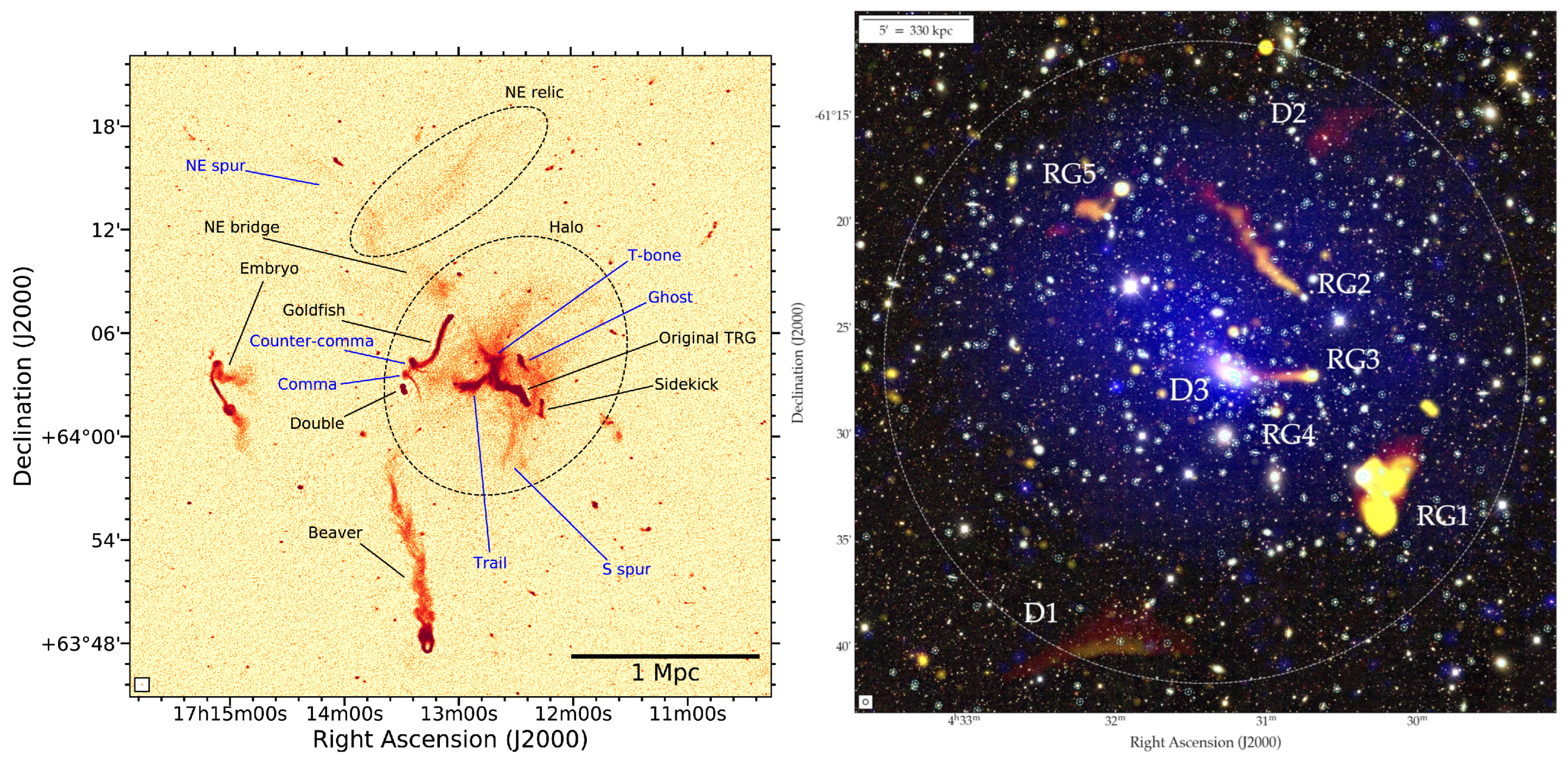
5.3. Mega Radio Halos
5.4. Polarisation
5.5. High Redshifts
5.6. Basics of Mini-Halos
6. Radio Relics—Shock Acceleration in the ICM
6.1. Basics of Shock Acceleration
6.2. Pre-Acceleration of Cosmic Rays
6.3. The Shocks’ Mach Numbers
6.4. Polarisation
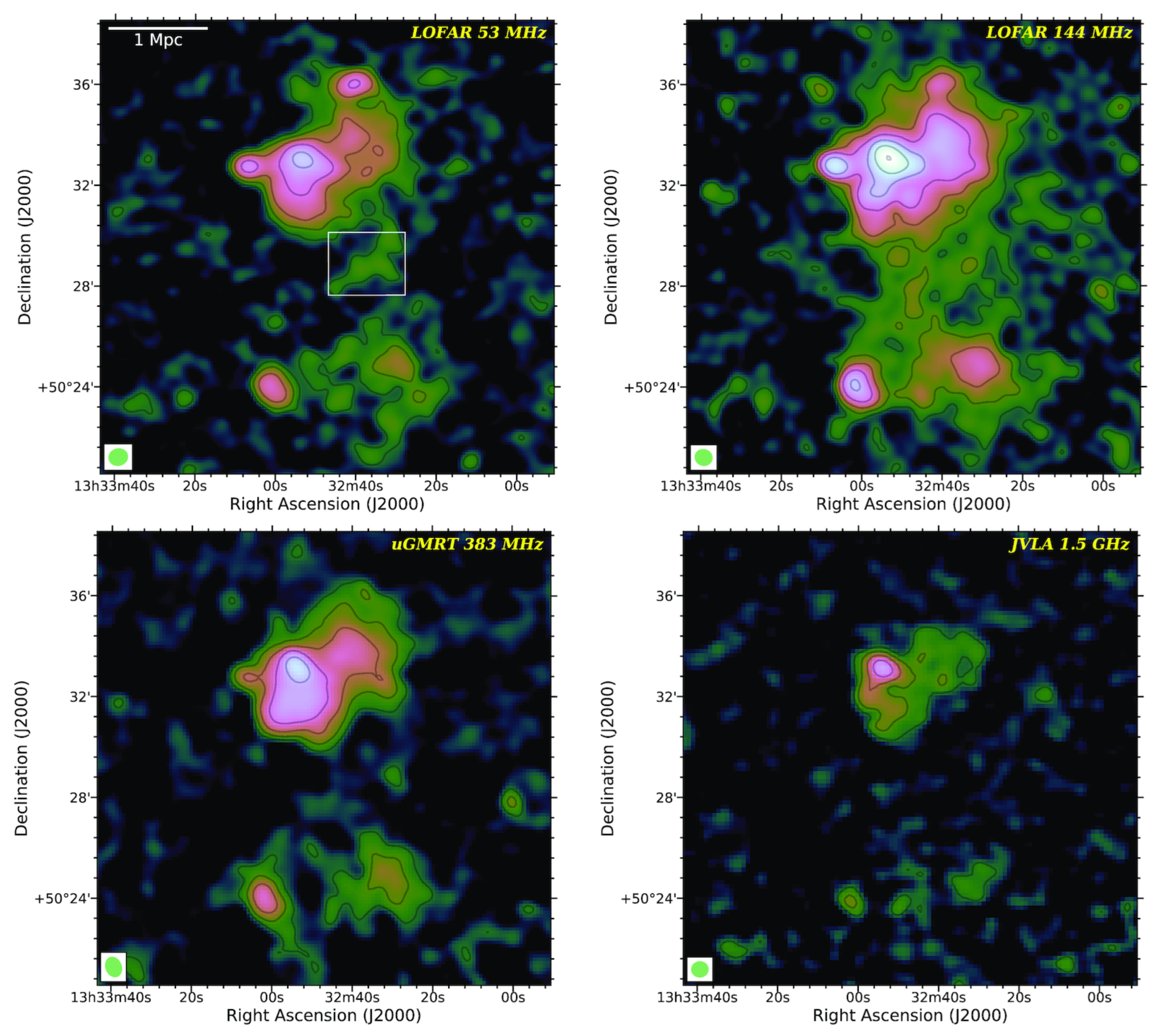
6.5. Lowest Radio Frequencies
6.6. Shock Acceleration of Cosmic-Ray Protons
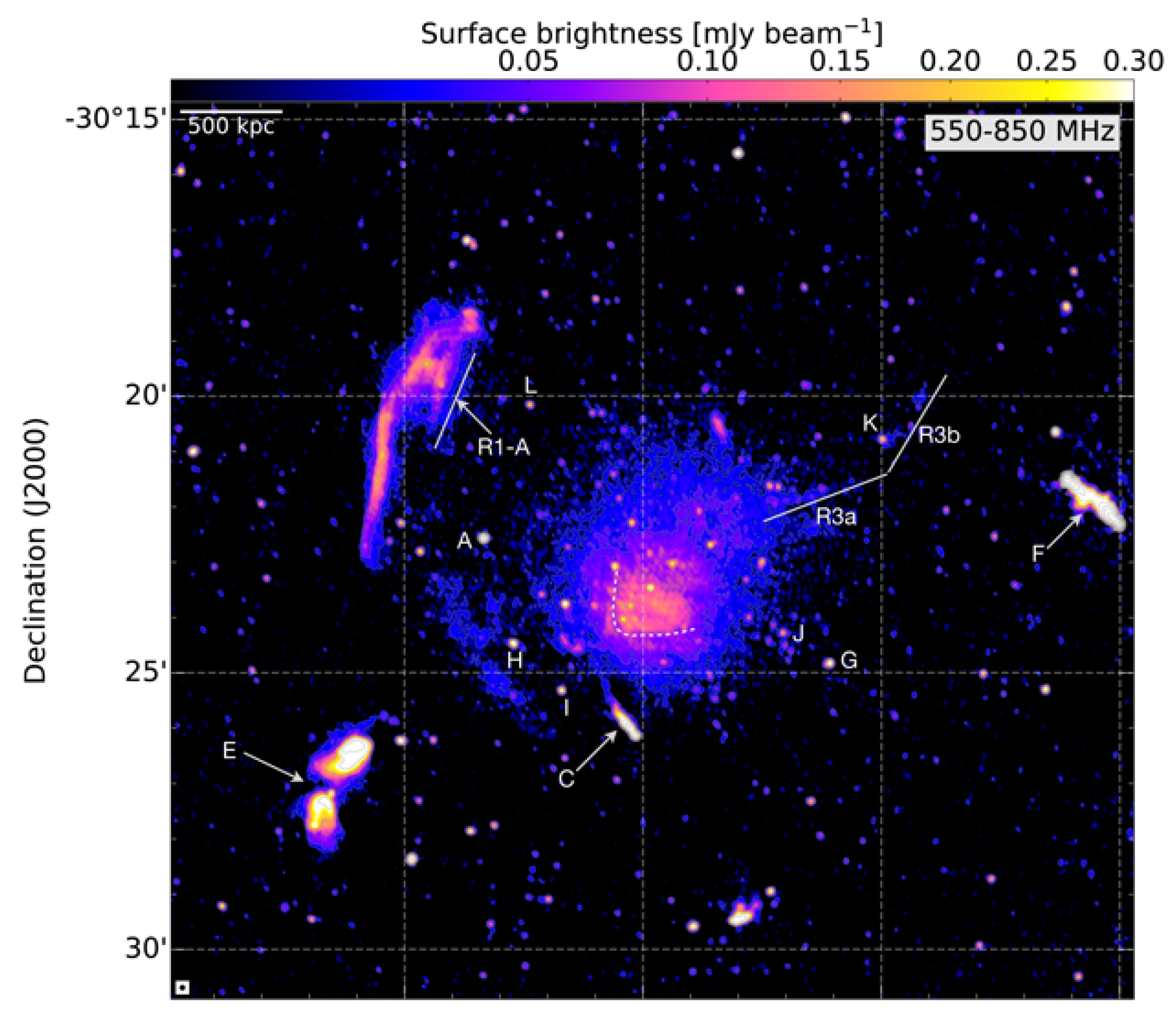
7. Beyond the Classical Radio Sources
7.1. Gently Re-Energising Tails
7.2. Remnant Sources
7.3. Odd Radio Circles
8. Beyond Clusters: Cosmic Filaments and Voids
8.1. Intercluster Bridges
8.2. Cosmic Filaments and Voids
9. Summary
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
| 1 | In principle, also cosmic-ray protons could be present in the Universe. However, they have never been unambiguously observed. Throughout this paper, we use cosmic-ray electrons and cosmic rays interchangeably. We state explicitly when we are talking about cosmic-ray protons. |
| 2 | To put this fast evolution of the field into numbers, according to the ADS abstract service, in the period 2000 to 2009, 47 papers were published that contained the word “radio relic” in the abstract. Between 2010 and 2019, this number increased to 212, and, between 2020 and 2022, already 69 such papers have been published. Similar numbers are found when searching for “radio halo”. Here, the number of papers increased from 157 (period 2000–2009) to 282 (period 2010–2019). Between 2020 and 2022, 83 such papers have been published. |
References
- Klein, U.; Fletcher, A. Galactic and Intergalactic Magnetic Fields; Springer: Berlin/Heidelberg, Germany, 2015. [Google Scholar]
- Sarazin, C.L. The Physics of Cluster Mergers. In Merging Processes in Galaxy Clusters; Feretti, L., Gioia, I.M., Giovannini, G., Eds.; Astrophysics and Space Science Library; Springer: Berlin/Heidelberg, Germany, 2002; Volume 272, pp. 1–38. [Google Scholar] [CrossRef]
- Schneider, P. Extragalactic Astronomy and Cosmology; Springer: Berlin/Heidelberg, Germany, 2006. [Google Scholar]
- Planelles, S.; Schleicher, D.R.G.; Bykov, A.M. Large-Scale Structure Formation: From the First Non-linear Objects to Massive Galaxy Clusters. Space Sci. Rev. 2015, 188, 93–139. [Google Scholar] [CrossRef]
- Subramanian, K. The origin, evolution and signatures of primordial magnetic fields. Rep. Prog. Phys. 2016, 79, 076901. [Google Scholar] [CrossRef] [PubMed]
- Donnert, J.; Vazza, F.; Brüggen, M.; ZuHone, J. Magnetic Field Amplification in Galaxy Clusters and Its Simulation. Space Sci. Rev. 2018, 214, 122. [Google Scholar] [CrossRef]
- Vazza, F.; Locatelli, N.; Rajpurohit, K.; Banfi, S.; Domínguez-Fernández, P.; Wittor, D.; Angelinelli, M.; Inchingolo, G.; Brienza, M.; Hackstein, S.; et al. Magnetogenesis and the Cosmic Web: A Joint Challenge for Radio Observations and Numerical Simulations. Galaxies 2021, 9, 109. [Google Scholar] [CrossRef]
- Govoni, F.; Orrù, E.; Bonafede, A.; Iacobelli, M.; Paladino, R.; Vazza, F.; Murgia, M.; Vacca, V.; Giovannini, G.; Feretti, L.; et al. A radio ridge connecting two galaxy clusters in a filament of the cosmic web. Science 2019, 364, 981–984. [Google Scholar] [CrossRef]
- Cho, J. Origin of Magnetic Field in the Intracluster Medium: Primordial or Astrophysical? Astrophys. J. 2014, 797, 133. [Google Scholar] [CrossRef]
- Brentjens, M.A.; de Bruyn, A.G. Faraday rotation measure synthesis. Astron. Astrophys. 2005, 441, 1217–1228. [Google Scholar] [CrossRef]
- Ferrari, C.; Govoni, F.; Schindler, S.; Bykov, A.M.; Rephaeli, Y. Observations of Extended Radio Emission in Clusters. Space Sci. Rev. 2008, 134, 93–118. [Google Scholar] [CrossRef]
- Bell, M.R.; Enßlin, T.A. Faraday synthesis. The synergy of aperture and rotation measure synthesis. Astron. Astrophys. 2012, 540, A80. [Google Scholar] [CrossRef]
- Feretti, L.; Giovannini, G.; Govoni, F.; Murgia, M. Clusters of galaxies: Observational properties of the diffuse radio emission. Astron. Astrophys. Rev. 2012, 20, 54. [Google Scholar] [CrossRef]
- Govoni, F.; Johnston-Hollitt, M.; Agudo, I.; Akahori, T.; Beck, R.; Bonafede, A.; Carozzi, T.D.; Colafrancesco, S.; Feretti, L.; Ferriere, K.; et al. Cosmic Magnetism Science in the SKA1 Era. Square Kilometre Array Organisation Science Working Group Assessment Workshop Summary, No. 6, Cosmic Magnetism, 26 Pages. Published Online by the SKA Organisation, March 2014. 2014. Available online: https://indico.skatelescope.org/event/274/attachments/1958/2463/Magnetism_SAW_Memo_8Feb2014.pdf (accessed on 26 June 2023).
- Rudnick, L.; Katz, D.; Sebokolodi, L. Polarization Tomography with Stokes Parameters. Galaxies 2021, 9, 92. [Google Scholar] [CrossRef]
- Vachaspati, T. Progress on cosmological magnetic fields. Rep. Prog. Phys. 2021, 84, 074901. [Google Scholar] [CrossRef] [PubMed]
- Locatelli, N.; Vazza, F.; Bonafede, A.; Banfi, S.; Bernardi, G.; Gheller, C.; Botteon, A.; Shimwell, T. New constraints on the magnetic field in cosmic web filaments. Astron. Astrophys. 2021, 652, A80. [Google Scholar] [CrossRef]
- Van Weeren, R.J.; de Gasperin, F.; Akamatsu, H.; Brüggen, M.; Feretti, L.; Kang, H.; Stroe, A.; Zandanel, F. Diffuse Radio Emission from Galaxy Clusters. Space Sci. Rev. 2019, 215, 16. [Google Scholar] [CrossRef]
- Rajpurohit, K.; Vazza, F.; van Weeren, R.J.; Hoeft, M.; Brienza, M.; Bonnassieux, E.; Riseley, C.J.; Brunetti, G.; Bonafede, A.; Brüggen, M.; et al. Dissecting nonthermal emission in the complex multiple-merger galaxy cluster Abell 2744: Radio and X-ray analysis. Astron. Astrophys. 2021, 654, A41. [Google Scholar] [CrossRef]
- Jaffe, W.J. Origin and transport of electrons in the halo radio source in the Coma cluster. Astrophys. J. 1977, 212, 1–7. [Google Scholar] [CrossRef]
- Ensslin, T.A.; Biermann, P.L.; Klein, U.; Kohle, S. Cluster radio relics as a tracer of shock waves of the large-scale structure formation. Astron. Astrophys. 1998, 332, 395–409. [Google Scholar]
- Brunetti, G.; Setti, G.; Feretti, L.; Giovannini, G. Particle reacceleration in the Coma cluster: Radio properties and hard X-ray emission. Mon. Not. R. Astron. Soc. 2001, 320, 365–378. [Google Scholar] [CrossRef]
- Petrosian, V. On the Nonthermal Emission and Acceleration of Electrons in Coma and Other Clusters of Galaxies. Astrophys. J. 2001, 557, 560–572. [Google Scholar] [CrossRef]
- Gitti, M.; Brunetti, G.; Setti, G. Modeling the interaction between ICM and relativistic plasma in cooling flows: The case of the Perseus cluster. Astron. Astrophys. 2002, 386, 456–463. [Google Scholar] [CrossRef]
- Vernstrom, T.; Gaensler, B.M.; Rudnick, L.; Andernach, H. Differences in Faraday Rotation between Adjacent Extragalactic Radio Sources as a Probe of Cosmic Magnetic Fields. Astrophys. J. 2019, 878, 92. [Google Scholar] [CrossRef]
- O’Sullivan, S.P.; Brüggen, M.; Vazza, F.; Carretti, E.; Locatelli, N.T.; Stuardi, C.; Vacca, V.; Vernstrom, T.; Heald, G.; Horellou, C.; et al. New constraints on the magnetization of the cosmic web using LOFAR Faraday rotation observations. Mon. Not. R. Astron. Soc. 2020, 495, 2607–2619. [Google Scholar] [CrossRef]
- Xu, J.; Han, J.L. Evidence for Strong Intracluster Magnetic Fields in the Early Universe. Astrophys. J. 2022, 926, 65. [Google Scholar] [CrossRef]
- Carretti, E.; O’Sullivan, S.P.; Vacca, V.; Vazza, F.; Gheller, C.; Vernstrom, T.; Bonafede, A. Magnetic field evolution in cosmic filaments with LOFAR data. Mon. Not. R. Astron. Soc. 2023, 518, 2273–2286. [Google Scholar] [CrossRef]
- Vernstrom, T.; Heald, G.; Vazza, F.; Galvin, T.J.; West, J.L.; Locatelli, N.; Fornengo, N.; Pinetti, E. Discovery of magnetic fields along stacked cosmic filaments as revealed by radio and X-ray emission. Mon. Not. R. Astron. Soc. 2021, 505, 4178–4196. [Google Scholar] [CrossRef]
- Hodgson, T.; Vazza, F.; Johnston-Hollitt, M.; McKinley, B. Stacking the synchrotron cosmic web with FIGARO. Publ. Astron. Soc. Aust. 2022, 39, e033. [Google Scholar] [CrossRef]
- Subramanian, K.; Shukurov, A.; Haugen, N.E.L. Evolving turbulence and magnetic fields in galaxy clusters. Mon. Not. R. Astron. Soc. 2006, 366, 1437–1454. [Google Scholar] [CrossRef]
- Brüggen, M.; Bykov, A.; Ryu, D.; Röttgering, H. Magnetic Fields, Relativistic Particles, and Shock Waves in Cluster Outskirts. Space Sci. Rev. 2012, 166, 187–213. [Google Scholar] [CrossRef]
- Brunetti, G.; Jones, T.W. Cosmic Rays in Galaxy Clusters and Their Nonthermal Emission. Int. J. Mod. Phys. D 2014, 23, 1430007–1430098. [Google Scholar] [CrossRef]
- Bykov, A.M.; Vazza, F.; Kropotina, J.A.; Levenfish, K.P.; Paerels, F.B.S. Shocks and Non-thermal Particles in Clusters of Galaxies. Space Sci. Rev. 2019, 215, 14. [Google Scholar] [CrossRef]
- Simionescu, A.; ZuHone, J.; Zhuravleva, I.; Churazov, E.; Gaspari, M.; Nagai, D.; Werner, N.; Roediger, E.; Canning, R.; Eckert, D.; et al. Constraining Gas Motions in the Intra-Cluster Medium. Space Sci. Rev. 2019, 215, 24. [Google Scholar] [CrossRef]
- Brunetti, G.; Lazarian, A. Compressible turbulence in galaxy clusters: Physics and stochastic particle re-acceleration. Mon. Not. R. Astron. Soc. 2007, 378, 245–275. [Google Scholar] [CrossRef]
- Markevitch, M.; Vikhlinin, A. Shocks and cold fronts in galaxy clusters. Phys. Rep. 2007, 443, 1–53. [Google Scholar] [CrossRef]
- Vazza, F.; Brunetti, G.; Kritsuk, A.; Wagner, R.; Gheller, C.; Norman, M. Turbulent motions and shocks waves in galaxy clusters simulated with adaptive mesh refinement. Astron. Astrophys. 2009, 504, 33–43. [Google Scholar] [CrossRef]
- Miniati, F. The Matryoshka Run: A Eulerian Refinement Strategy to Study the Statistics of Turbulence in Virialized Cosmic Structures. Astrophys. J. 2014, 782, 21. [Google Scholar] [CrossRef]
- Miniati, F. The Matryoshka Run. II. Time-dependent Turbulence Statistics, Stochastic Particle Acceleration, and Microphysics Impact in a Massive Galaxy Cluster. Astrophys. J. 2015, 800, 60. [Google Scholar] [CrossRef]
- Beresnyak, A.; Miniati, F. Turbulent Amplification and Structure of the Intracluster Magnetic Field. Astrophys. J. 2016, 817, 127. [Google Scholar] [CrossRef]
- Vazza, F.; Jones, T.W.; Brüggen, M.; Brunetti, G.; Gheller, C.; Porter, D.; Ryu, D. Turbulence and vorticity in Galaxy clusters generated by structure formation. Mon. Not. R. Astron. Soc. 2017, 464, 210–230. [Google Scholar] [CrossRef]
- Wittor, D.; Jones, T.; Vazza, F.; Brüggen, M. Evolution of vorticity and enstrophy in the intracluster medium. Mon. Not. R. Astron. Soc. 2017, 471, 3212–3225. [Google Scholar] [CrossRef]
- Vazza, F.; Brunetti, G.; Gheller, C.; Brunino, R.; Brüggen, M. Massive and refined. II. The statistical properties of turbulent motions in massive galaxy clusters with high spatial resolution. Astron. Astrophys. 2011, 529, A17. [Google Scholar] [CrossRef]
- Cassano, R.; Brunetti, G. Cluster mergers and non-thermal phenomena: A statistical magneto-turbulent model. Mon. Not. R. Astron. Soc. 2005, 357, 1313–1329. [Google Scholar] [CrossRef]
- Liu, W.; Sun, M.; Nulsen, P.; Clarke, T.; Sarazin, C.; Forman, W.; Gaspari, M.; Giacintucci, S.; Lal, D.V.; Edge, T. AGN feedback in galaxy group 3C 88: Cavities, shock, and jet reorientation. Mon. Not. R. Astron. Soc. 2019, 484, 3376–3392. [Google Scholar] [CrossRef]
- Gaspari, M.; Tombesi, F.; Cappi, M. Linking macro-, meso- and microscales in multiphase AGN feeding and feedback. Nat. Astron. 2020, 4, 10–13. [Google Scholar] [CrossRef]
- Wittor, D.; Gaspari, M. Dissecting the turbulent weather driven by mechanical AGN feedback. Mon. Not. R. Astron. Soc. 2020, 498, 4983–5002. [Google Scholar] [CrossRef]
- Kolmogorov, A. The Local Structure of Turbulence in Incompressible Viscous Fluid for Very Large Reynolds’ Numbers. Akad. Nauk. SSSR Dokl. 1941, 30, 301–305. [Google Scholar]
- Hitomi Collaboration. The quiescent intracluster medium in the core of the Perseus cluster. Nature 2016, 535, 117–121. [Google Scholar] [CrossRef]
- Ha, J.H.; Ryu, D.; Kang, H. Properties of Merger Shocks in Merging Galaxy Clusters. Astrophys. J. 2018, 857, 26. [Google Scholar] [CrossRef]
- Gu, L.; Akamatsu, H.; Shimwell, T.W.; Intema, H.T.; van Weeren, R.J.; de Gasperin, F.; Mernier, F.; Mao, J.; Urdampilleta, I.; de Plaa, J.; et al. Observations of a pre-merger shock in colliding clusters of galaxies. Nat. Astron. 2019, 3, 838–843. [Google Scholar] [CrossRef]
- Zhang, C.; Churazov, E.; Forman, W.R.; Lyskova, N. Runaway merger shocks in galaxy cluster outskirts and radio relics. Mon. Not. R. Astron. Soc. 2019, 488, 5259–5266. [Google Scholar] [CrossRef]
- Zhang, C.; Churazov, E.; Dolag, K.; Forman, W.R.; Zhuravleva, I. Collision of merger and accretion shocks: Formation of Mpc-scale contact discontinuity in the Perseus cluster. Mon. Not. R. Astron. Soc. 2020, 498, L130–L134. [Google Scholar] [CrossRef]
- Zhang, C.; Churazov, E.; Zhuravleva, I. Pairs of giant shock waves (N-waves) in merging galaxy clusters. Mon. Not. R. Astron. Soc. 2021, 501, 1038–1045. [Google Scholar] [CrossRef]
- Ryu, D.; Kang, H.; Hallman, E.; Jones, T.W. Cosmological Shock Waves and Their Role in the Large-Scale Structure of the Universe. Astrophys. J. 2003, 593, 599–610. [Google Scholar] [CrossRef]
- Ghizzardi, S.; Rossetti, M.; Molendi, S. Cold fronts in galaxy clusters. Astron. Astrophys. 2010, 516, A32. [Google Scholar] [CrossRef]
- Zuhone, J.A.; Roediger, E. Cold fronts: Probes of plasma astrophysics in galaxy clusters. J. Plasma Phys. 2016, 82, 535820301. [Google Scholar] [CrossRef]
- Zinger, E.; Dekel, A.; Birnboim, Y.; Nagai, D.; Lau, E.; Kravtsov, A.V. Cold fronts and shocks formed by gas streams in galaxy clusters. Mon. Not. R. Astron. Soc. 2018, 476, 56–70. [Google Scholar] [CrossRef]
- Rybicki, G.B.; Lightman, A.P. Radiative Processes in Astrophysics; John Wiley & Sons: Hoboken, NJ, USA, 1986. [Google Scholar]
- Beck, R.; Krause, M. Revised equipartition and minimum energy formula for magnetic field strength estimates from radio synchrotron observations. Astron. Nachrichten 2005, 326, 414–427. [Google Scholar] [CrossRef]
- Rephaeli, Y.; Nevalainen, J.; Ohashi, T.; Bykov, A.M. Nonthermal Phenomena in Clusters of Galaxies. Space Sci. Rev. 2008, 134, 71–92. [Google Scholar] [CrossRef]
- Mirakhor, M.S.; Walker, S.A.; Runge, J.; Diwanji, P. Possible non-thermal origin of the hard X-ray emission in the merging galaxy cluster SPT-CL J2031-4037. Mon. Not. R. Astron. Soc. 2022, 516, 1855–1864. [Google Scholar] [CrossRef]
- Brunetti, G.; Zimmer, S.; Zandanel, F. Relativistic protons in the Coma galaxy cluster: First gamma-ray constraints ever on turbulent reacceleration. Mon. Not. R. Astron. Soc. 2017, 472, 1506–1525. [Google Scholar] [CrossRef]
- Ackermann, M.; Ajello, M.; Albert, A.; Allafort, A.; Atwood, W.B.; Baldini, L.; Ballet, J.; Barbiellini, G.; Bastieri, D.; Bechtol, K.; et al. Search for Cosmic-Ray-induced Gamma-Ray Emission in Galaxy Clusters. Astrophys. J. 2014, 787, 18. [Google Scholar] [CrossRef]
- Ackermann, M.; Ajello, M.; Albert, A.; Atwood, W.B.; Baldini, L.; Barbiellini, G.; Bastieri, D.; Bechtol, K.; Bellazzini, R.; Bissaldi, E.; et al. Search for Extended Gamma-Ray Emission from the Virgo Galaxy Cluster with FERMI-LAT. Astrophys. J. 2015, 812, 159. [Google Scholar] [CrossRef]
- Ackermann, M.; Ajello, M.; Albert, A.; Atwood, W.B.; Baldini, L.; Ballet, J.; Barbiellini, G.; Bastieri, D.; Bechtol, K.; Bellazzini, R.; et al. Search for Gamma-Ray Emission from the Coma Cluster with Six Years of Fermi-LAT Data. Astrophys. J. 2016, 819, 149. [Google Scholar] [CrossRef]
- Xi, S.Q.; Wang, X.Y.; Liang, Y.F.; Peng, F.K.; Yang, R.Z.; Liu, R.Y. Detection of gamma-ray emission from the Coma cluster with Fermi Large Area Telescope and tentative evidence for an extended spatial structure. Phys. Rev. D 2018, 98, 063006. [Google Scholar] [CrossRef]
- Adam, R.; Goksu, H.; Brown, S.; Rudnick, L.; Ferrari, C. γ-ray detection toward the Coma cluster with Fermi-LAT: Implications for the cosmic ray content in the hadronic scenario. Astron. Astrophys. 2021, 648, A60. [Google Scholar] [CrossRef]
- Baghmanyan, V.; Zargaryan, D.; Aharonian, F.; Yang, R.; Casanova, S.; Mackey, J. Detailed study of extended γ-ray morphology in the vicinity of the Coma cluster with Fermi Large Area Telescope. Mon. Not. R. Astron. Soc. 2022, 516, 562–571. [Google Scholar] [CrossRef]
- Aleksić, J.; Alvarez, E.A.; Antonelli, L.A.; Antoranz, P.; Asensio, M.; Backes, M.; Barres de Almeida, U.; Barrio, J.A.; Bastieri, D.; Becerra González, J.; et al. Constraining cosmic rays and magnetic fields in the Perseus galaxy cluster with TeV observations by the MAGIC telescopes. Astron. Astrophys. 2012, 541, A99. [Google Scholar] [CrossRef]
- Bonafede, A.; Feretti, L.; Murgia, M.; Govoni, F.; Giovannini, G.; Dallacasa, D.; Dolag, K.; Taylor, G.B. The Coma cluster magnetic field from Faraday rotation measures. Astron. Astrophys. 2010, 513, A30. [Google Scholar] [CrossRef]
- Bonafede, A.; Vazza, F.; Brüggen, M.; Murgia, M.; Govoni, F.; Feretti, L.; Giovannini, G.; Ogrean, G. Measurements and simulation of Faraday rotation across the Coma radio relic. Mon. Not. R. Astron. Soc. 2013, 433, 3208–3226. [Google Scholar] [CrossRef]
- Stuardi, C.; Bonafede, A.; Lovisari, L.; Domínguez-Fernández, P.; Vazza, F.; Brüggen, M.; van Weeren, R.J.; de Gasperin, F. The intracluster magnetic field in the double relic galaxy cluster Abell 2345. Mon. Not. R. Astron. Soc. 2021, 502, 2518–2535. [Google Scholar] [CrossRef]
- Ferrière, K.; West, J.L.; Jaffe, T.R. The correct sense of Faraday rotation. Mon. Not. R. Astron. Soc. 2021, 507, 4968–4982. [Google Scholar] [CrossRef]
- Lazarian, A.; Yuen, K.H. Gradients of Synchrotron Polarization: Tracing 3D Distribution of Magnetic Fields. Astrophys. J. 2018, 865, 59. [Google Scholar] [CrossRef]
- Hurley-Walker, N.; Callingham, J.R.; Hancock, P.J.; Franzen, T.M.O.; Hindson, L.; Kapińska, A.D.; Morgan, J.; Offringa, A.R.; Wayth, R.B.; Wu, C.; et al. GaLactic and Extragalactic All-sky Murchison Widefield Array (GLEAM) survey—I. A low-frequency extragalactic catalogue. Mon. Not. R. Astron. Soc. 2017, 464, 1146–1167. [Google Scholar] [CrossRef]
- Herrera Ruiz, N.; O’Sullivan, S.P.; Vacca, V.; Jelić, V.; Nikiel-Wroczyński, B.; Bourke, S.; Sabater, J.; Dettmar, R.J.; Heald, G.; Horellou, C.; et al. LOFAR Deep Fields: Probing a broader population of polarized radio galaxies in ELAIS-N1. Astron. Astrophys. 2021, 648, A12. [Google Scholar] [CrossRef]
- Samui, S.; Subramanian, K.; Srianand, R. Efficient cold outflows driven by cosmic rays in high-redshift galaxies and their global effects on the IGM. Mon. Not. R. Astron. Soc. 2018, 476, 1680–1695. [Google Scholar] [CrossRef]
- Federrath, C.; Klessen, R.S.; Iapichino, L.; Beattie, J.R. The sonic scale of interstellar turbulence. Nat. Astron. 2021, 5, 365–371. [Google Scholar] [CrossRef]
- Schekochihin, A.; Cowley, S.; Maron, J.; Malyshkin, L. Structure of small-scale magnetic fields in the kinematic dynamo theory. Phys. Rev. E 2001, 65, 016305. [Google Scholar] [CrossRef]
- Schekochihin, A.A.; Cowley, S.C.; Taylor, S.F.; Maron, J.L.; McWilliams, J.C. Simulations of the Small-Scale Turbulent Dynamo. Astrophys. J. 2004, 612, 276–307. [Google Scholar] [CrossRef]
- Kulsrud, R.M.; Ostriker, E.C. Plasma Physics for Astrophysics. Phys. Today 2006, 59, 58. [Google Scholar] [CrossRef]
- Dolag, K.; Grasso, D.; Springel, V.; Tkachev, I. Constrained simulations of the magnetic field in the local Universe and the propagation of ultrahigh energy cosmic rays. J. Cosmol. Astropart. Phys. 2005, 2005, 009. [Google Scholar] [CrossRef]
- Dolag, K.; Borgani, S.; Schindler, S.; Diaferio, A.; Bykov, A.M. Simulation Techniques for Cosmological Simulations. Space Sci. Rev. 2008, 134, 229–268. [Google Scholar] [CrossRef]
- Iapichino, L.; Brüggen, M. Magnetic field amplification by shocks in galaxy clusters: Application to radio relics. Mon. Not. R. Astron. Soc. 2012, 423, 2781–2788. [Google Scholar] [CrossRef]
- Binney, J. Galaxy formation without primordial turbulence: Mechanisms for generating cosmic vorticity. Mon. Not. R. Astron. Soc. 1974, 168, 73–92. [Google Scholar] [CrossRef]
- Lyutikov, M. Magnetic draping of merging cores and radio bubbles in clusters of galaxies. Mon. Not. R. Astron. Soc. 2006, 373, 73–78. [Google Scholar] [CrossRef]
- Dennison, B. Formation of radio halos in clusters of galaxies from cosmic-ray protons. Astrophys. J. 1980, 239, L93–L96. [Google Scholar] [CrossRef]
- Pfrommer, C.; Enßlin, T.A.; Springel, V. Simulating cosmic rays in clusters of galaxies—II. A unified scheme for radio haloes and relics with predictions of the γ-ray emission. Mon. Not. R. Astron. Soc. 2008, 385, 1211–1241. [Google Scholar] [CrossRef]
- Enßlin, T.; Pfrommer, C.; Miniati, F.; Subramanian, K. Cosmic ray transport in galaxy clusters: Implications for radio halos, gamma-ray signatures, and cool core heating. Astron. Astrophys. 2011, 527, A99. [Google Scholar] [CrossRef]
- Sreekumar, P.; Bertsch, D.L.; Dingus, B.L.; Esposito, J.A.; Fichtel, C.E.; Fierro, J.; Hartman, R.C.; Hunter, S.D.; Kanbach, G.; Kniffen, D.A.; et al. EGRET Observations of the North Galactic Pole Region. Astrophys. J. 1996, 464, 628. [Google Scholar] [CrossRef]
- Reimer, O.; Pohl, M.; Sreekumar, P.; Mattox, J.R. EGRET Upper Limits on the High-Energy Gamma-Ray Emission of Galaxy Clusters. Astrophys. J. 2003, 588, 155–164. [Google Scholar] [CrossRef]
- Huber, B.; Tchernin, C.; Eckert, D.; Farnier, C.; Manalaysay, A.; Straumann, U.; Walter, R. Probing the cosmic-ray content of galaxy clusters by stacking Fermi-LAT count maps. Astron. Astrophys. 2013, 560, A64. [Google Scholar] [CrossRef]
- Zandanel, F.; Ando, S. Constraints on diffuse gamma-ray emission from structure formation processes in the Coma cluster. Mon. Not. R. Astron. Soc. 2014, 440, 663–671. [Google Scholar] [CrossRef]
- Aharonian, F.; Akhperjanian, A.G.; Anton, G.; Barres de Almeida, U.; Bazer-Bachi, A.R.; Becherini, Y.; Behera, B.; Bernlöhr, K.; Boisson, C.; Bochow, A.; et al. Very high energy gamma-ray observations of the galaxy clusters Abell 496 and Abell 85 with HESS. Astron. Astrophys. 2009, 495, 27–35. [Google Scholar] [CrossRef]
- Aleksić, J.; Antonelli, L.A.; Antoranz, P.; Backes, M.; Baixeras, C.; Balestra, S.; Barrio, J.A.; Bastieri, D.; Becerra González, J.; Bednarek, W.; et al. MAGIC Gamma-ray Telescope Observation of the Perseus Cluster of Galaxies: Implications for Cosmic Rays, Dark Matter, and NGC 1275. Astrophys. J. 2010, 710, 634–647. [Google Scholar] [CrossRef]
- Arlen, T.; Aune, T.; Beilicke, M.; Benbow, W.; Bouvier, A.; Buckley, J.H.; Bugaev, V.; Byrum, K.; Cannon, A.; Cesarini, A.; et al. Constraints on Cosmic Rays, Magnetic Fields, and Dark Matter from Gamma-Ray Observations of the Coma Cluster of Galaxies with VERITAS and Fermi. Astrophys. J. 2012, 757, 123. [Google Scholar] [CrossRef]
- Ahnen, M.L.; Ansoldi, S.; Antonelli, L.A.; Antoranz, P.; Babic, A.; Banerjee, B.; Bangale, P.; Barres de Almeida, U.; Barrio, J.A.; Becerra González, J.; et al. Deep observation of the NGC 1275 region with MAGIC: Search of diffuse γ-ray emission from cosmic rays in the Perseus cluster. Astron. Astrophys. 2016, 589, A33. [Google Scholar] [CrossRef]
- Brunetti, G.; Blasi, P.; Reimer, O.; Rudnick, L.; Bonafede, A.; Brown, S. Probing the origin of giant radio haloes through radio and γ-ray data: The case of the Coma cluster. Mon. Not. R. Astron. Soc. 2012, 426, 956–968. [Google Scholar] [CrossRef]
- Bruno, L.; Rajpurohit, K.; Brunetti, G.; Gastaldello, F.; Botteon, A.; Ignesti, A.; Bonafede, A.; Dallacasa, D.; Cassano, R.; van Weeren, R.J.; et al. The LOFAR and JVLA view of the distant steep spectrum radio halo in MACS J1149.5+2223. Astron. Astrophys. 2021, 650, A44. [Google Scholar] [CrossRef]
- Fisk, L.A. The acceleration of energetic particles in the interplanetary medium by transit time damping. J. Geophys. Res. 1976, 81, 4633. [Google Scholar] [CrossRef]
- Schlickeiser, R.; Miller, J.A. Quasi-linear Theory of Cosmic-Ray Transport and Acceleration: The Role of Oblique Magnetohydrodynamic Waves and Transit-Time Damping. Astrophys. J. 1998, 492, 352–378. [Google Scholar] [CrossRef]
- Pilipp, W.; Völk, H.J. Analysis of electromagnetic instabilities parallel to the magnetic field. J. Plasma Phys. 1971, 6, 1–17. [Google Scholar] [CrossRef]
- Yan, H.; Lazarian, A. Cosmic-Ray Scattering and Streaming in Compressible Magnetohydrodynamic Turbulence. Astrophys. J. 2004, 614, 757–769. [Google Scholar] [CrossRef]
- Lazarian, A.; Beresnyak, A. Cosmic ray scattering in compressible turbulence. Mon. Not. R. Astron. Soc. 2006, 373, 1195–1202. [Google Scholar] [CrossRef]
- Eilek, J.A. Particle reacceleration in radio galaxies. Astrophys. J. 1979, 230, 373–385. [Google Scholar] [CrossRef]
- Porter, D.H.; Jones, T.W.; Ryu, D. Vorticity, Shocks, and Magnetic Fields in Subsonic, ICM-like Turbulence. Astrophys. J. 2015, 810, 93. [Google Scholar] [CrossRef]
- Brunetti, G.; Lazarian, A. Stochastic reacceleration of relativistic electrons by turbulent reconnection: A mechanism for cluster-scale radio emission? Mon. Not. R. Astron. Soc. 2016, 458, 2584–2595. [Google Scholar] [CrossRef]
- Cassano, R.; Ettori, S.; Giacintucci, S.; Brunetti, G.; Markevitch, M.; Venturi, T.; Gitti, M. On the Connection Between Giant Radio Halos and Cluster Mergers. Astrophys. J. 2010, 721, L82–L85. [Google Scholar] [CrossRef]
- Cuciti, V.; Cassano, R.; Brunetti, G.; Dallacasa, D.; de Gasperin, F.; Ettori, S.; Giacintucci, S.; Kale, R.; Pratt, G.W.; van Weeren, R.J.; et al. Radio halos in a mass-selected sample of 75 galaxy clusters. II. Statistical analysis. Astron. Astrophys. 2021, 647, A51. [Google Scholar] [CrossRef]
- Cuciti, V.; Cassano, R.; Brunetti, G.; Dallacasa, D.; van Weeren, R.J.; Giacintucci, S.; Bonafede, A.; de Gasperin, F.; Ettori, S.; Kale, R.; et al. Radio halos in a mass-selected sample of 75 galaxy clusters. I. Sample selection and data analysis. Astron. Astrophys. 2021, 647, A50. [Google Scholar] [CrossRef]
- Wilber, A.; Brüggen, M.; Bonafede, A.; Savini, F.; Shimwell, T.; van Weeren, R.J.; Rafferty, D.; Mechev, A.P.; Intema, H.; Andrade-Santos, F.; et al. LOFAR discovery of an ultra-steep radio halo and giant head-tail radio galaxy in Abell 1132. Mon. Not. R. Astron. Soc. 2018, 473, 3536–3546. [Google Scholar] [CrossRef]
- Rajpurohit, K.; Brunetti, G.; Bonafede, A.; van Weeren, R.J.; Botteon, A.; Vazza, F.; Hoeft, M.; Riseley, C.J.; Bonnassieux, E.; Brienza, M.; et al. Physical insights from the spectrum of the radio halo in MACS J0717.5+3745. Astron. Astrophys. 2021, 646, A135. [Google Scholar] [CrossRef]
- Vacca, V.; Govoni, F.; Perley, R.A.; Murgia, M.; Carretti, E.; Loi, F.; Feretti, L.; Giovannini, G. Spectral Index of the Filaments in the Abell 523 Radio Halo. Galaxies 2021, 9, 112. [Google Scholar] [CrossRef]
- Di Gennaro, G.; van Weeren, R.J.; Cassano, R.; Brunetti, G.; Brüggen, M.; Hoeft, M.; Osinga, E.; Botteon, A.; Cuciti, V.; de Gasperin, F.; et al. A LOFAR-uGMRT spectral index study of distant radio halos. Astron. Astrophys. 2021, 654, A166. [Google Scholar] [CrossRef]
- Duchesne, S.W.; Johnston-Hollitt, M.; Wilber, A.G. MWA and ASKAP observations of atypical radio-halo-hosting galaxy clusters: Abell 141 and Abell 3404. Publ. Astron. Soc. Aust. 2021, 38, e031. [Google Scholar] [CrossRef]
- Duchesne, S.W.; Johnston-Hollitt, M.; Bartalucci, I. Low-frequency integrated radio spectra of diffuse, steep-spectrum sources in galaxy clusters: Palaeontology with the MWA and ASKAP. Publ. Astron. Soc. Aust. 2021, 38, e053. [Google Scholar] [CrossRef]
- Botteon, A.; Brunetti, G.; van Weeren, R.J.; Shimwell, T.W.; Pizzo, R.F.; Cassano, R.; Iacobelli, M.; Gastaldello, F.; Bîrzan, L.; Bonafede, A.; et al. The Beautiful Mess in Abell 2255. Astrophys. J. 2020, 897, 93. [Google Scholar] [CrossRef]
- Bonafede, A.; Brunetti, G.; Rudnick, L.; Vazza, F.; Bourdin, H.; Giovannini, G.; Shimwell, T.W.; Zhang, X.; Mazzotta, P.; Simionescu, A.; et al. The Coma Cluster at LOFAR Frequencies. II. The Halo, Relic, and a New Accretion Relic. Astrophys. J. 2022, 933, 218. [Google Scholar] [CrossRef]
- Cassano, R.; Ettori, S.; Brunetti, G.; Giacintucci, S.; Pratt, G.W.; Venturi, T.; Kale, R.; Dolag, K.; Markevitch, M. Revisiting Scaling Relations for Giant Radio Halos in Galaxy Clusters. Astrophys. J. 2013, 777, 141. [Google Scholar] [CrossRef]
- Cassano, R.; Brunetti, G.; Setti, G. Statistics of giant radio haloes from electron reacceleration models. Mon. Not. R. Astron. Soc. 2006, 369, 1577–1595. [Google Scholar] [CrossRef]
- Angelinelli, M.; Vazza, F.; Giocoli, C.; Ettori, S.; Jones, T.W.; Brunetti, G.; Brüggen, M.; Eckert, D. Turbulent pressure support and hydrostatic mass bias in the intracluster medium. Mon. Not. R. Astron. Soc. 2020, 495, 864–885. [Google Scholar] [CrossRef]
- Simonte, M.; Vazza, F.; Brighenti, F.; Brüggen, M.; Jones, T.W.; Angelinelli, M. Exploring the relation between turbulent velocity and density fluctuations in the stratified intracluster medium. Astron. Astrophys. 2022, 658, A149. [Google Scholar] [CrossRef]
- Sanders, J.S.; Biffi, V.; Brüggen, M.; Bulbul, E.; Dennerl, K.; Dolag, K.; Erben, T.; Freyberg, M.; Gatuzz, E.; Ghirardini, V.; et al. Studying the merging cluster Abell 3266 with eROSITA. Astron. Astrophys. 2022, 661, A36. [Google Scholar] [CrossRef]
- De Vries, M.; Mantz, A.B.; Allen, S.W.; Morris, R.G.; Zhuravleva, I.; Canning, R.E.A.; Ehlert, S.R.; Ogorzałek, A.; Simionescu, A.; Werner, N. Chandra measurements of gas homogeneity and turbulence at intermediate radii in the Perseus Cluster. Mon. Not. R. Astron. Soc. 2023, 518, 2954–2970. [Google Scholar] [CrossRef]
- Dupourqué, S.; Clerc, N.; Pointecouteau, E.; Eckert, D.; Ettori, S.; Vazza, F. Investigating the turbulent hot gas in X-COP galaxy clusters. arXiv 2023, arXiv:2303.15102. [Google Scholar] [CrossRef]
- Zhuravleva, I.; Chen, M.C.; Churazov, E.; Schekochihin, A.A.; Zhang, C.; Nagai, D. Indirect measurements of gas velocities in galaxy clusters: Effects of ellipticity and cluster dynamic state. Mon. Not. R. Astron. Soc. 2023, 520, 5157–5172. [Google Scholar] [CrossRef]
- Riseley, C.J.; Bonnassieux, E.; Vernstrom, T.; Galvin, T.J.; Chokshi, A.; Botteon, A.; Rajpurohit, K.; Duchesne, S.W.; Bonafede, A.; Rudnick, L.; et al. Radio fossils, relics, and haloes in Abell 3266: Cluster archaeology with ASKAP-EMU and the ATCA. Mon. Not. R. Astron. Soc. 2022, 515, 1871–1896. [Google Scholar] [CrossRef]
- Hoang, D.N.; Zhang, X.; Stuardi, C.; Shimwell, T.W.; Bonafede, A.; Brüggen, M.; Brunetti, G.; Botteon, A.; Cassano, R.; de Gasperin, F.; et al. A 3.5 Mpc long radio relic in the galaxy cluster ClG 0217+70. Astron. Astrophys. 2021, 656, A154. [Google Scholar] [CrossRef]
- Dolag, K.; Enßlin, T.A. Radio halos of galaxy clusters from hadronic secondary electron injection in realistic magnetic field configurations. Astron. Astrophys. 2000, 362, 151–157. [Google Scholar] [CrossRef]
- Govoni, F.; Feretti, L.; Giovannini, G.; Böhringer, H.; Reiprich, T.H.; Murgia, M. Radio and X-ray diffuse emission in six clusters of galaxies. Astron. Astrophys. 2001, 376, 803–819. [Google Scholar] [CrossRef]
- Storm, E.; Jeltema, T.E.; Rudnick, L. A radio and X-ray study of the merging cluster A2319. Mon. Not. R. Astron. Soc. 2015, 448, 2495–2503. [Google Scholar] [CrossRef]
- Rajpurohit, K.; Osinga, E.; Brienza, M.; Botteon, A.; Brunetti, G.; Forman, W.R.; Riseley, C.J.; Vazza, F.; Bonafede, A.; van Weeren, R.J.; et al. Deep low-frequency radio observations of Abell 2256. II. The ultra-steep spectrum radio halo. Astron. Astrophys. 2023, 669, A1. [Google Scholar] [CrossRef]
- Cuciti, V.; de Gasperin, F.; Brüggen, M.; Vazza, F.; Brunetti, G.; Shimwell, T.W.; Edler, H.W.; van Weeren, R.J.; Botteon, A.; Cassano, R.; et al. Galaxy clusters enveloped by vast volumes of relativistic electrons. Nature 2022, 609, 911–914. [Google Scholar] [CrossRef]
- Botteon, A.; van Weeren, R.J.; Brunetti, G.; Vazza, F.; Shimwell, T.W.; Brüggen, M.; Röttgering, H.J.A.; de Gasperin, F.; Akamatsu, H.; Bonafede, A.; et al. Magnetic fields and relativistic electrons fill entire galaxy cluster. Sci. Adv. 2022, 8, eabq7623. [Google Scholar] [CrossRef] [PubMed]
- Govoni, F.; Murgia, M.; Xu, H.; Li, H.; Norman, M.L.; Feretti, L.; Giovannini, G.; Vacca, V. Polarization of cluster radio halos with upcoming radio interferometers. Astron. Astrophys. 2013, 554, A102. [Google Scholar] [CrossRef]
- Loi, F.; Murgia, M.; Govoni, F.; Vacca, V.; Prandoni, I.; Li, H.; Feretti, L.; Giovannini, G. Simulations of the Polarized Sky for the SKA: How to Constrain Intracluster Magnetic Fields. Galaxies 2018, 6, 133. [Google Scholar] [CrossRef]
- Loi, F.; Murgia, M.; Govoni, F.; Vacca, V.; Bonafede, A.; Ferrari, C.; Prandoni, I.; Feretti, L.; Giovannini, G.; Li, H. Rotation measure synthesis applied to synthetic SKA images of galaxy clusters. Mon. Not. R. Astron. Soc. 2019, 490, 4841–4857. [Google Scholar] [CrossRef]
- Basu, A.; Sur, S. Properties of Polarized Synchrotron Emission from Fluctuation Dynamo Action—II. Effects of Turbulence Driving in the ICM and Beam Smoothing. Galaxies 2021, 9, 62. [Google Scholar] [CrossRef]
- Govoni, F.; Murgia, M.; Feretti, L.; Giovannini, G.; Dallacasa, D.; Taylor, G.B. A2255: The first detection of filamentary polarized emission in a radio halo. Astron. Astrophys. 2005, 430, L5–L8. [Google Scholar] [CrossRef]
- Bonafede, A.; Feretti, L.; Giovannini, G.; Govoni, F.; Murgia, M.; Taylor, G.B.; Ebeling, H.; Allen, S.; Gentile, G.; Pihlström, Y. Revealing the magnetic field in a distant galaxy cluster: Discovery of the complex radio emission from MACS J0717.5 +3745. Astron. Astrophys. 2009, 503, 707–720. [Google Scholar] [CrossRef]
- Girardi, M.; Boschin, W.; Gastaldello, F.; Giovannini, G.; Govoni, F.; Murgia, M.; Barrena, R.; Ettori, S.; Trasatti, M.; Vacca, V. A multiwavelength view of the galaxy cluster Abell 523 and its peculiar diffuse radio source. Mon. Not. R. Astron. Soc. 2016, 456, 2829–2847. [Google Scholar] [CrossRef]
- Rajpurohit, K.; Hoeft, M.; Wittor, D.; van Weeren, R.J.; Vazza, F.; Rudnick, L.; Rajpurohit, S.; Forman, W.R.; Riseley, C.J.; Brienza, M.; et al. Turbulent magnetic fields in the merging galaxy cluster MACS J0717.5+3745. Astron. Astrophys. 2022, 657, A2. [Google Scholar] [CrossRef]
- Sur, S.; Basu, A.; Subramanian, K. Properties of polarized synchrotron emission from fluctuation-dynamo action—I. Application to galaxy clusters. Mon. Not. R. Astron. Soc. 2021, 501, 3332–3349. [Google Scholar] [CrossRef]
- Di Gennaro, G.; van Weeren, R.J.; Brunetti, G.; Cassano, R.; Brüggen, M.; Hoeft, M.; Shimwell, T.W.; Röttgering, H.J.A.; Bonafede, A.; Botteon, A.; et al. Fast magnetic field amplification in distant galaxy clusters. Nat. Astron. 2021, 5, 268–275. [Google Scholar] [CrossRef]
- Giacintucci, S.; Markevitch, M.; Venturi, T.; Clarke, T.E.; Cassano, R.; Mazzotta, P. New Detections of Radio Minihalos in Cool Cores of Galaxy Clusters. Astrophys. J. 2014, 781, 9. [Google Scholar] [CrossRef]
- Giacintucci, S.; Markevitch, M.; Brunetti, G.; ZuHone, J.A.; Venturi, T.; Mazzotta, P.; Bourdin, H. Mapping the Particle Acceleration in the Cool Core of the Galaxy Cluster RX J1720.1+2638. Astrophys. J. 2014, 795, 73. [Google Scholar] [CrossRef]
- Giacintucci, S.; Markevitch, M.; Cassano, R.; Venturi, T.; Clarke, T.E.; Brunetti, G. Occurrence of Radio Minihalos in a Mass-limited Sample of Galaxy Clusters. Astrophys. J. 2017, 841, 71. [Google Scholar] [CrossRef]
- ZuHone, J.A.; Brunetti, G.; Giacintucci, S.; Markevitch, M. Testing Secondary Models for the Origin of Radio Mini-Halos in Galaxy Clusters. Astrophys. J. 2015, 801, 146. [Google Scholar] [CrossRef]
- Riseley, C.J.; Rajpurohit, K.; Loi, F.; Botteon, A.; Timmerman, R.; Biava, N.; Bonafede, A.; Bonnassieux, E.; Brunetti, G.; Enßlin, T.; et al. A MeerKAT-meets-LOFAR study of MS 1455.0 + 2232: A 590 kiloparsec ’mini’-halo in a sloshing cool-core cluster. Mon. Not. R. Astron. Soc. 2022, 512, 4210–4230. [Google Scholar] [CrossRef]
- Mazzotta, P.; Markevitch, M.; Vikhlinin, A.; Forman, W.R.; David, L.P.; van Speybroeck, L. Chandra Observation of RX J1720.1+2638: A Nearly Relaxed Cluster with a Fast-moving Core? Astrophys. J. 2001, 555, 205–214. [Google Scholar] [CrossRef]
- Mazzotta, P.; Giacintucci, S. Do Radio Core-Halos and Cold Fronts in Non-Major-Merging Clusters Originate from the Same Gas Sloshing? Astrophys. J. 2008, 675, L9. [Google Scholar] [CrossRef]
- Rossetti, M.; Eckert, D.; De Grandi, S.; Gastaldello, F.; Ghizzardi, S.; Roediger, E.; Molendi, S. Abell 2142 at large scales: An extreme case for sloshing? Astron. Astrophys. 2013, 556, A44. [Google Scholar] [CrossRef]
- Savini, F.; Bonafede, A.; Brüggen, M.; van Weeren, R.; Brunetti, G.; Intema, H.; Botteon, A.; Shimwell, T.; Wilber, A.; Rafferty, D.; et al. First evidence of diffuse ultra-steep-spectrum radio emission surrounding the cool core of a cluster. Mon. Not. R. Astron. Soc. 2018, 478, 2234–2242. [Google Scholar] [CrossRef]
- Fujita, Y.; Kohri, K.; Yamazaki, R.; Kino, M. Nonthermal Emission Associated with Strong AGN Outbursts at the Centers of Galaxy Clusters. Astrophys. J. 2007, 663, L61–L64. [Google Scholar] [CrossRef]
- Bravi, L.; Gitti, M.; Brunetti, G. Do radio mini-halos and gas heating in cool-core clusters have a common origin? Mon. Not. R. Astron. Soc. 2016, 455, L41–L45. [Google Scholar] [CrossRef]
- Richard-Laferrière, A.; Hlavacek-Larrondo, J.; Nemmen, R.S.; Rhea, C.L.; Taylor, G.B.; Prasow-Émond, M.; Gendron-Marsolais, M.; Latulippe, M.; Edge, A.C.; Fabian, A.C.; et al. On the relation between mini-halos and AGN feedback in clusters of galaxies. Mon. Not. R. Astron. Soc. 2020, 499, 2934–2958. [Google Scholar] [CrossRef]
- Ignesti, A.; Brunetti, G.; Gitti, M.; Giacintucci, S. Radio and X-ray connection in radio mini-halos: Implications for hadronic models. Astron. Astrophys. 2020, 640, A37. [Google Scholar] [CrossRef]
- Skillman, S.W.; Hallman, E.J.; O’Shea, B.W.; Burns, J.O. Cosmological Shockwaves as Plasma Physics Laboratories: Radio Relics and Electron Acceleration. In Bulletin of the American Astronomical Society; American Astronomical Society: Washington, DC, USA, 2010; Volume 42, p. 389. [Google Scholar]
- Kang, H.; Ryu, D.; Jones, T.W. Diffusive Shock Acceleration Simulations of Radio Relics. Astrophys. J. 2012, 756, 97. [Google Scholar] [CrossRef]
- Skillman, S.W.; Xu, H.; Hallman, E.J.; O’Shea, B.W.; Burns, J.O.; Li, H.; Collins, D.C.; Norman, M.L. Cosmological Magnetohydrodynamic Simulations of Galaxy Cluster Radio Relics: Insights and Warnings for Observations. Astrophys. J. 2013, 765, 21. [Google Scholar] [CrossRef]
- Vazza, F.; Brüggen, M. Do radio relics challenge diffusive shock acceleration? Mon. Not. R. Astron. Soc. 2014, 437, 2291–2296. [Google Scholar] [CrossRef]
- Trasatti, M.; Akamatsu, H.; Lovisari, L.; Klein, U.; Bonafede, A.; Brüggen, M.; Dallacasa, D.; Clarke, T. The radio relic in Abell 2256: Overall spectrum and implications for electron acceleration. Astron. Astrophys. 2015, 575, A45. [Google Scholar] [CrossRef]
- Locatelli, N.T.; Rajpurohit, K.; Vazza, F.; Gastaldello, F.; Dallacasa, D.; Bonafede, A.; Rossetti, M.; Stuardi, C.; Bonassieux, E.; Brunetti, G.; et al. Discovering the most elusive radio relic in the sky: Diffuse shock acceleration caught in the act? Mon. Not. R. Astron. Soc. 2020, 496, L48–L53. [Google Scholar] [CrossRef]
- Brüggen, M.; Vazza, F. Analytical model for cluster radio relics. Mon. Not. R. Astron. Soc. 2020, 493, 2306–2317. [Google Scholar] [CrossRef]
- Fermi, E. On the Origin of the Cosmic Radiation. Phys. Rev. 1949, 75, 1169–1174. [Google Scholar] [CrossRef]
- Blandford, R.D.; Ostriker, J.P. Particle acceleration by astrophysical shocks. Astrophys. J. 1978, 221, L29–L32. [Google Scholar] [CrossRef]
- Drury, L.O. An introduction to the theory of diffusive shock acceleration of energetic particles in tenuous plasmas. Rep. Prog. Phys. 1983, 46, 973–1027. [Google Scholar] [CrossRef]
- Loi, F.; Murgia, M.; Vacca, V.; Govoni, F.; Melis, A.; Wittor, D.; Kierdorf, M.; Bonafede, A.; Boschin, W.; Brienza, M.; et al. Spectropolarimetric observations of the CIZA J2242.8+5301 northern radio relic: No evidence of high-frequency steepening. Mon. Not. R. Astron. Soc. 2020, 498, 1628–1637. [Google Scholar] [CrossRef]
- Rajpurohit, K.; Wittor, D.; van Weeren, R.J.; Vazza, F.; Hoeft, M.; Rudnick, L.; Locatelli, N.; Eilek, J.; Forman, W.R.; Bonafede, A.; et al. Understanding the radio relic emission in the galaxy cluster MACS J0717.5+3745: Spectral analysis. Astron. Astrophys. 2021, 646, A56. [Google Scholar] [CrossRef]
- Rajpurohit, K.; Hoeft, M.; Vazza, F.; Rudnick, L.; van Weeren, R.J.; Wittor, D.; Drabent, A.; Brienza, M.; Bonnassieux, E.; Locatelli, N.; et al. New mysteries and challenges from the Toothbrush relic: Wideband observations from 550 MHz to 8 GHz. Astron. Astrophys. 2020, 636, A30. [Google Scholar] [CrossRef]
- Rajpurohit, K.; Vazza, F.; Hoeft, M.; Loi, F.; Beck, R.; Vacca, V.; Kierdorf, M.; van Weeren, R.J.; Wittor, D.; Govoni, F.; et al. A perfect power-law spectrum even at the highest frequencies: The Toothbrush relic. Astron. Astrophys. 2020, 642, L13. [Google Scholar] [CrossRef]
- Schellenberger, G.; Giacintucci, S.; Lovisari, L.; O’Sullivan, E.; Vrtilek, J.; David, L.P.; Melin, J.B.; Lal, D.V.; Ettori, S.; Kolokythas, K.; et al. The Unusually Weak and Exceptionally Steep Radio Relic in A2108. Astrophys. J. 2022, 925, 91. [Google Scholar] [CrossRef]
- Stuardi, C.; Bonafede, A.; Wittor, D.; Vazza, F.; Botteon, A.; Locatelli, N.; Dallacasa, D.; Golovich, N.; Hoeft, M.; van Weeren, R.J.; et al. Particle re-acceleration and Faraday-complex structures in the RXC J1314.4-2515 galaxy cluster. Mon. Not. R. Astron. Soc. 2019, 489, 3905–3926. [Google Scholar] [CrossRef]
- Di Gennaro, G.; van Weeren, R.J.; Hoeft, M.; Kang, H.; Ryu, D.; Rudnick, L.; Forman, W.; Röttgering, H.J.A.; Brüggen, M.; Dawson, W.A.; et al. Deep Very Large Array Observations of the Merging Cluster CIZA J2242.8+5301: Continuum and Spectral Imaging. Astrophys. J. 2018, 865, 24. [Google Scholar] [CrossRef]
- Jones, A.; de Gasperin, F.; Cuciti, V.; Hoang, D.N.; Botteon, A.; Brüggen, M.; Brunetti, G.; Finner, K.; Forman, W.R.; Jones, C.; et al. Radio relics in PSZ2 G096.88+24.18: A connection with pre-existing plasma. Mon. Not. R. Astron. Soc. 2021, 505, 4762–4774. [Google Scholar] [CrossRef]
- Kang, H.; Ryu, D. Diffusive Shock Acceleration at Cosmological Shock Waves. Astrophys. J. 2013, 764, 95. [Google Scholar] [CrossRef]
- Kang, H. Shock Acceleration Model with Postshock Turbulence for Giant Radio Relics. J. Korean Astron. Soc. 2017, 50, 93–103. [Google Scholar] [CrossRef]
- Kang, H.; Ryu, D. Effects of Alfvénic Drift on Diffusive Shock Acceleration at Weak Cluster Shocks. Astrophys. J. 2018, 856, 33. [Google Scholar] [CrossRef]
- Kang, H. Semi-Analytic Models for Electron Acceleration in Weak ICM Shocks. J. Korean Astron. Soc. 2020, 53, 59–67. [Google Scholar]
- Caprioli, D.; Spitkovsky, A. Simulations of Ion Acceleration at Non-relativistic Shocks. II. Magnetic Field Amplification. Astrophys. J. 2014, 794, 46. [Google Scholar] [CrossRef]
- Caprioli, D.; Zhang, H.; Spitkovsky, A. Diffusive shock re-acceleration. J. Plasma Phys. 2018, 84, 715840301. [Google Scholar] [CrossRef]
- Caprioli, D.; Haggerty, C.C.; Blasi, P. Kinetic Simulations of Cosmic-Ray-modified Shocks. II. Particle Spectra. Astrophys. J. 2020, 905, 2. [Google Scholar] [CrossRef]
- Guo, X.; Sironi, L.; Narayan, R. Non-thermal Electron Acceleration in Low Mach Number Collisionless Shocks. I. Particle Energy Spectra and Acceleration Mechanism. Astrophys. J. 2014, 794, 153. [Google Scholar] [CrossRef]
- Guo, X.; Sironi, L.; Narayan, R. Non-thermal Electron Acceleration in Low Mach Number Collisionless Shocks. II. Firehose-mediated Fermi Acceleration and its Dependence on Pre-shock Conditions. Astrophys. J. 2014, 797, 47. [Google Scholar] [CrossRef]
- Pais, M.; Pfrommer, C.; Ehlert, K.; Pakmor, R. The effect of cosmic ray acceleration on supernova blast wave dynamics. Mon. Not. R. Astron. Soc. 2018, 478, 5278–5295. [Google Scholar] [CrossRef]
- Dubois, Y.; Commerçon, B.; Marcowith, A.; Brahimi, L. Shock-accelerated cosmic rays and streaming instability in the adaptive mesh refinement code Ramses. Astron. Astrophys. 2019, 631, A121. [Google Scholar] [CrossRef]
- Botteon, A.; Brunetti, G.; Ryu, D.; Roh, S. Shock acceleration efficiency in radio relics. Astron. Astrophys. 2020, 634, A64. [Google Scholar] [CrossRef]
- Bonafede, A.; Intema, H.T.; Brüggen, M.; Girardi, M.; Nonino, M.; Kantharia, N.; van Weeren, R.J.; Röttgering, H.J.A. Evidence for Particle Re-acceleration in the Radio Relic in the Galaxy Cluster PLCKG287.0+32.9. Astrophys. J. 2014, 785, 1. [Google Scholar] [CrossRef]
- Van Weeren, R.J.; Andrade-Santos, F.; Dawson, W.A.; Golovich, N.; Lal, D.V.; Kang, H.; Ryu, D.; Brìggen, M.; Ogrean, G.A.; Forman, W.R.; et al. The case for electron re-acceleration at galaxy cluster shocks. Nat. Astron. 2017, 1, 0005. [Google Scholar] [CrossRef]
- Inchingolo, G.; Wittor, D.; Rajpurohit, K.; Vazza, F. Radio relics radio emission from multishock scenario. Mon. Not. R. Astron. Soc. 2022, 509, 1160–1174. [Google Scholar] [CrossRef]
- ZuHone, J.A.; Markevitch, M.; Weinberger, R.; Nulsen, P.; Ehlert, K. How Merger-driven Gas Motions in Galaxy Clusters Can Turn AGN Bubbles into Radio Relics. Astrophys. J. 2021, 914, 73. [Google Scholar] [CrossRef]
- ZuHone, J.; Ehlert, K.; Weinberger, R.; Pfrommer, C. Turning AGN Bubbles into Radio Relics with Sloshing: Modeling CR Transport with Realistic Physics. Galaxies 2021, 9, 91. [Google Scholar] [CrossRef]
- Lee, W.; James Jee, M.; Finner, K.; HyeongHan, K.; Kale, R.; Yoon, H.; Forman, W.; Kraft, R.; Jones, C.; Chung, A. Discovery of a Double Radio Relic in ZwCl1447.2+2619: A Rare Testbed for Shock-acceleration Models with a Peculiar Surface-brightness Ratio. Astrophys. J. 2022, 924, 18. [Google Scholar] [CrossRef]
- Matsukiyo, S.; Ohira, Y.; Yamazaki, R.; Umeda, T. Relativistic Electron Shock Drift Acceleration in Low Mach Number Galaxy Cluster Shocks. Astrophys. J. 2011, 742, 47. [Google Scholar] [CrossRef]
- Akamatsu, H.; Kawahara, H. Systematic X-ray Analysis of Radio Relic Clusters with Suzaku. Publ. Astron. Soc. Jpn. 2013, 65, 16. [Google Scholar] [CrossRef]
- Eckert, D.; Jauzac, M.; Vazza, F.; Owers, M.S.; Kneib, J.P.; Tchernin, C.; Intema, H.; Knowles, K. A shock front at the radio relic of Abell 2744. Mon. Not. R. Astron. Soc. 2016, 461, 1302–1307. [Google Scholar] [CrossRef]
- Van Weeren, R.J.; Brunetti, G.; Brüggen, M.; Andrade-Santos, F.; Ogrean, G.A.; Williams, W.L.; Röttgering, H.J.A.; Dawson, W.A.; Forman, W.R.; de Gasperin, F.; et al. LOFAR, VLA, and Chandra Observations of the Toothbrush Galaxy Cluster. Astrophys. J. 2016, 818, 204. [Google Scholar] [CrossRef]
- Hoang, D.N.; Shimwell, T.W.; van Weeren, R.J.; Intema, H.T.; Röttgering, H.J.A.; Andrade-Santos, F.; Akamatsu, H.; Bonafede, A.; Brunetti, G.; Dawson, W.A.; et al. Radio observations of the double-relic galaxy cluster Abell 1240. Mon. Not. R. Astron. Soc. 2018, 478, 2218–2233. [Google Scholar] [CrossRef]
- Markevitch, M.; Govoni, F.; Brunetti, G.; Jerius, D. Bow Shock and Radio Halo in the Merging Cluster A520. Astrophys. J. 2005, 627, 733–738. [Google Scholar] [CrossRef]
- Hong, S.E.; Kang, H.; Ryu, D. Radio and X-Ray Shocks in Clusters of Galaxies. Astrophys. J. 2015, 812, 49. [Google Scholar] [CrossRef]
- Kang, H.; Ryu, D. Curved Radio Spectra of Weak Cluster Shocks. Astrophys. J. 2015, 809, 186. [Google Scholar] [CrossRef]
- Stroe, A.; Shimwell, T.; Rumsey, C.; van Weeren, R.; Kierdorf, M.; Donnert, J.; Jones, T.W.; Röttgering, H.J.A.; Hoeft, M.; Rodríguez-Gonzálvez, C.; et al. The widest frequency radio relic spectra: Observations from 150 MHz to 30 GHz. Mon. Not. R. Astron. Soc. 2016, 455, 2402–2416. [Google Scholar] [CrossRef]
- Akamatsu, H.; Mizuno, M.; Ota, N.; Zhang, Y.Y.; van Weeren, R.J.; Kawahara, H.; Fukazawa, Y.; Kaastra, J.S.; Kawaharada, M.; Nakazawa, K.; et al. Suzaku observations of the merging galaxy cluster Abell 2255: The northeast radio relic. Astron. Astrophys. 2017, 600, A100. [Google Scholar] [CrossRef]
- Hoang, D.N.; Shimwell, T.W.; Stroe, A.; Akamatsu, H.; Brunetti, G.; Donnert, J.M.F.; Intema, H.T.; Mulcahy, D.D.; Röttgering, H.J.A.; van Weeren, R.J.; et al. Deep LOFAR observations of the merging galaxy cluster CIZA J2242.8+5301. Mon. Not. R. Astron. Soc. 2017, 471, 1107–1125. [Google Scholar] [CrossRef]
- Kang, H.; Petrosian, V.; Ryu, D.; Jones, T.W. Injection of κ-like Suprathermal Particles into Diffusive Shock Acceleration. Astrophys. J. 2014, 788, 142. [Google Scholar] [CrossRef]
- Zimbardo, G.; Perri, S. Understanding the radio spectral indices of galaxy cluster relics by superdiffusive shock acceleration. Mon. Not. R. Astron. Soc. 2018, 478, 4922–4930. [Google Scholar] [CrossRef]
- Ge, C.; Liu, R.Y.; Sun, M.; Yu, H.; Rudnick, L.; Eilek, J.; Owen, F.; Dasadia, S.; Rossetti, M.; Markevitch, M.; et al. Chandra and XMM-Newton observations of A2256: Cold fronts, merger shocks, and constraint on the IC emission. Mon. Not. R. Astron. Soc. 2020, 497, 4704–4717. [Google Scholar] [CrossRef]
- Churazov, E.; Khabibullin, I.; Bykov, A.M.; Lyskova, N.; Sunyaev, R. Tempestuous life beyond R500: X-ray view on the Coma cluster with SRG/eROSITA. II. Shock & Relic. arXiv 2022, arXiv:2205.07511. [Google Scholar]
- Wittor, D.; Ettori, S.; Vazza, F.; Rajpurohit, K.; Hoeft, M.; Domínguez-Fernández, P. Exploring the spectral properties of radio relics—I: Integrated spectral index and Mach number. Mon. Not. R. Astron. Soc. 2021, 506, 396–414. [Google Scholar] [CrossRef]
- Hoeft, M.; Nuza, S.E.; Gottlöber, S.; van Weeren, R.J.; Röttgering, H.J.A.; Brüggen, M. Radio Relics in Cosmological Simulations. J. Astrophys. Astron. 2011, 32, 509–517. [Google Scholar] [CrossRef]
- Wittor, D.; Hoeft, M.; Vazza, F.; Brüggen, M.; Domínguez-Fernández, P. Polarization of radio relics in galaxy clusters. Mon. Not. R. Astron. Soc. 2019, 490, 3987–4006. [Google Scholar] [CrossRef]
- Roh, S.; Ryu, D.; Kang, H.; Ha, S.; Jang, H. Turbulence Dynamo in the Stratified Medium of Galaxy Clusters. Astrophys. J. 2019, 883, 138. [Google Scholar] [CrossRef]
- Russell, H.R.; Nulsen, P.E.J.; Caprioli, D.; Chadayammuri, U.; Fabian, A.C.; Kunz, M.W.; McNamara, B.R.; Sanders, J.S.; Richard-Laferrière, A.; Beleznay, M.; et al. The structure of cluster merger shocks: Turbulent width and the electron heating time-scale. Mon. Not. R. Astron. Soc. 2022, 514, 1477–1493. [Google Scholar] [CrossRef]
- Domínguez-Fernández, P.; Brüggen, M.; Vazza, F.; Banda-Barragán, W.E.; Rajpurohit, K.; Mignone, A.; Mukherjee, D.; Vaidya, B. Morphology of radio relics—I. What causes the substructure of synchrotron emission? Mon. Not. R. Astron. Soc. 2020, 500, 795–816. [Google Scholar] [CrossRef]
- Bonafede, A.; Giovannini, G.; Feretti, L.; Govoni, F.; Murgia, M. Double relics in Abell 2345 and Abell 1240. Spectral index and polarization analysis. Astron. Astrophys. 2009, 494, 429–442. [Google Scholar] [CrossRef]
- Kale, R.; Dwarakanath, K.S.; Bagchi, J.; Paul, S. Spectral and polarization study of the double relics in Abell 3376 using the Giant Metrewave Radio Telescope and the Very Large Array. Mon. Not. R. Astron. Soc. 2012, 426, 1204–1211. [Google Scholar] [CrossRef]
- Stuardi, C.; Bonafede, A.; Rajpurohit, K.; Brüggen, M.; de Gasperin, F.; Hoang, D.; van Weeren, R.J.; Vazza, F. Using the polarization properties of double radio relics to probe the turbulent compression scenario. Astron. Astrophys. 2022, 666, A8. [Google Scholar] [CrossRef]
- Laing, R.A. A model for the magnetic-field structure in extended radio sources. Mon. Not. R. Astron. Soc. 1980, 193, 439–449. [Google Scholar] [CrossRef]
- Di Gennaro, G.; van Weeren, R.J.; Rudnick, L.; Hoeft, M.; Brüggen, M.; Ryu, D.; Röttgering, H.J.A.; Forman, W.; Stroe, A.; Shimwell, T.W.; et al. Downstream Depolarization in the Sausage Relic: A 1-4 GHz Very Large Array Study. Astrophys. J. 2021, 911, 3. [Google Scholar] [CrossRef]
- Domínguez-Fernández, P.; Brüggen, M.; Vazza, F.; Hoeft, M.; Banda-Barragán, W.E.; Rajpurohit, K.; Wittor, D.; Mignone, A.; Mukherjee, D.; Vaidya, B. Morphology of radio relics—II. Properties of polarized emission. Mon. Not. R. Astron. Soc. 2021, 507, 2714–2734. [Google Scholar] [CrossRef]
- Hoeft, M.; Rajpurohit, K.; Wittor, D.; di Gennaro, G.; Domínguez-Fernández, P. On the Polarisation of Radio Relics. Galaxies 2022, 10, 10. [Google Scholar] [CrossRef]
- De Gasperin, F.; Brunetti, G.; Brüggen, M.; van Weeren, R.; Williams, W.L.; Botteon, A.; Cuciti, V.; Dijkema, T.J.; Edler, H.; Iacobelli, M.; et al. Reaching thermal noise at ultra-low radio frequencies. Toothbrush radio relic downstream of the shock front. Astron. Astrophys. 2020, 642, A85. [Google Scholar] [CrossRef]
- Wittor, D. On the Challenges of Cosmic-Ray Proton Shock Acceleration in the Intracluster Medium. New Astron. 2021, 85, 101550. [Google Scholar] [CrossRef]
- Blasi, P.; Colafrancesco, S. Cosmic rays, radio halos and nonthermal X-ray emission in clusters of galaxies. Astropart. Phys. 1999, 12, 169–183. [Google Scholar] [CrossRef]
- Wiener, J.; Oh, S.P.; Guo, F. Cosmic ray streaming in clusters of galaxies. Mon. Not. R. Astron. Soc. 2013, 434, 2209–2228. [Google Scholar] [CrossRef]
- Pinzke, A.; Oh, S.P.; Pfrommer, C. Turbulence and particle acceleration in giant radio haloes: The origin of seed electrons. Mon. Not. R. Astron. Soc. 2017, 465, 4800–4816. [Google Scholar] [CrossRef]
- Ha, J.H.; Ryu, D.; Kang, H.; van Marle, A.J. Proton Acceleration in Weak Quasi-parallel Intracluster Shocks: Injection and Early Acceleration. Astrophys. J. 2018, 864, 105. [Google Scholar] [CrossRef]
- Ha, J.H.; Ryu, D.; Kang, H. Gamma-ray and Neutrino Emissions due to Cosmic-Ray Protons Accelerated at Intracluster Shocks in Galaxy Clusters. arXiv 2019, arXiv:1910.02429. [Google Scholar] [CrossRef]
- Ryu, D.; Kang, H.; Ha, J.H. A Diffusive Shock Acceleration Model for Protons in Weak Quasi-parallel Intracluster Shocks. Astrophys. J. 2019, 883, 60. [Google Scholar] [CrossRef]
- Wittor, D.; Vazza, F.; Ryu, D.; Kang, H. Limiting the shock acceleration of cosmic ray protons in the ICM. Mon. Not. R. Astron. Soc. 2020, 495, L112–L117. [Google Scholar] [CrossRef]
- Wittor, D.; Vazza, F.; Brüggen, M. Studying the Effect of Shock Obliquity on the γ-ray and Diffuse Radio Emission in Galaxy Clusters. Galaxies 2016, 4, 71. [Google Scholar] [CrossRef]
- Wittor, D.; Vazza, F.; Brüggen, M. Testing cosmic ray acceleration with radio relics: A high-resolution study using MHD and tracers. Mon. Not. R. Astron. Soc. 2017, 464, 4448–4462. [Google Scholar] [CrossRef]
- Banfi, S.; Vazza, F.; Wittor, D. Shock waves in the magnetized cosmic web: The role of obliquity and cosmic ray acceleration. Mon. Not. R. Astron. Soc. 2020, 496, 3648–3667. [Google Scholar] [CrossRef]
- Giacintucci, S.; Markevitch, M.; Johnston-Hollitt, M.; Wik, D.R.; Wang, Q.H.S.; Clarke, T.E. Discovery of a Giant Radio Fossil in the Ophiuchus Galaxy Cluster. Astrophys. J. 2020, 891, 1. [Google Scholar] [CrossRef]
- Gupta, N.; Huynh, M.; Norris, R.P.; Wang, X.R.; Hopkins, A.M.; Andernach, H.; Koribalski, B.S.; Galvin, T.J. Discovery of peculiar radio morphologies with ASKAP using unsupervised machine learning. Publ. Astron. Soc. Aust. 2022, 39, e051. [Google Scholar] [CrossRef]
- Rahaman, M.; Raja, R.; Datta, A. On the detection of multiple shock fronts in A1914 using deep Chandra X-ray observations. Mon. Not. R. Astron. Soc. 2022, 509, 5821–5835. [Google Scholar] [CrossRef]
- Mandal, S.; Intema, H.T.; van Weeren, R.J.; Shimwell, T.W.; Botteon, A.; Brunetti, G.; de Gasperin, F.; Brüggen, M.; Di Gennaro, G.; Kraft, R.; et al. Revived fossil plasma sources in galaxy clusters. Astron. Astrophys. 2020, 634, A4. [Google Scholar] [CrossRef]
- de Gasperin, F.; Intema, H.T.; Shimwell, T.W.; Brunetti, G.; Brüggen, M.; Enßlin, T.A.; van Weeren, R.J.; Bonafede, A.; Röttgering, H.J.A. Gentle reenergization of electrons in merging galaxy clusters. Sci. Adv. 2017, 3, e1701634. [Google Scholar] [CrossRef]
- Botteon, A.; Giacintucci, S.; Gastaldello, F.; Venturi, T.; Brunetti, G.; van Weeren, R.J.; Shimwell, T.W.; Rossetti, M.; Akamatsu, H.; Brüggen, M.; et al. Nonthermal phenomena in the center of Abell 1775. An 800 kpc head-tail, revived fossil plasma and slingshot radio halo. Astron. Astrophys. 2021, 649, A37. [Google Scholar] [CrossRef]
- Pasini, T.; Edler, H.W.; Brüggen, M.; de Gasperin, F.; Botteon, A.; Rajpurohit, K.; van Weeren, R.J.; Gastaldello, F.; Gaspari, M.; Brunetti, G.; et al. Particle re-acceleration and diffuse radio sources in the galaxy cluster Abell 1550. Astron. Astrophys. 2022, 663, A105. [Google Scholar] [CrossRef]
- Giacintucci, S.; Venturi, T.; Markevitch, M.; Bourdin, H.; Mazzotta, P.; Merluzzi, P.; Dallacasa, D.; Bardelli, S.; Sikhosana, S.P.; Smirnov, O.; et al. A Candle in the Wind: A Radio Filament in the Core of the A3562 Galaxy Cluster. Astrophys. J. 2022, 934, 49. [Google Scholar] [CrossRef]
- Hodgson, T.; Bartalucci, I.; Johnston-Hollitt, M.; McKinley, B.; Vazza, F.; Wittor, D. Ultra-steep-spectrum Radio “Jellyfish” Uncovered in A2877. Astrophys. J. 2021, 909, 198. [Google Scholar] [CrossRef]
- Brienza, M.; Shimwell, T.W.; de Gasperin, F.; Bikmaev, I.; Bonafede, A.; Botteon, A.; Brüggen, M.; Brunetti, G.; Burenin, R.; Capetti, A.; et al. A snapshot of the oldest active galactic nuclei feedback phases. Nat. Astron. 2021, 5, 1261–1267. [Google Scholar] [CrossRef]
- Brienza, M.; Lovisari, L.; Rajpurohit, K.; Bonafede, A.; Gastaldello, F.; Murgia, M.; Vazza, F.; Bonnassieux, E.; Botteon, A.; Brunetti, G.; et al. The galaxy group NGC 507: Newly detected AGN remnant plasma transported by sloshing. Astron. Astrophys. 2022, 661, A92. [Google Scholar] [CrossRef]
- Norris, R.P.; Crawford, E.; Macgregor, P. Odd Radio Circles and Their Environment. Galaxies 2021, 9, 83. [Google Scholar] [CrossRef]
- Norris, R.P.; Collier, J.D.; Crocker, R.M.; Heywood, I.; Macgregor, P.; Rudnick, L.; Shabala, S.; Andernach, H.; da Cunha, E.; English, J.; et al. MeerKAT uncovers the physics of an odd radio circle. Mon. Not. R. Astron. Soc. 2022, 513, 1300–1316. [Google Scholar] [CrossRef]
- Koribalski, B.S.; Norris, R.P.; Andernach, H.; Rudnick, L.; Shabala, S.; Filipović, M.; Lenc, E. Discovery of a new extragalactic circular radio source with ASKAP: ORC J0102-2450. Mon. Not. R. Astron. Soc. 2021, 505, L11–L15. [Google Scholar] [CrossRef]
- Koribalski, B.S.; Veronica, A.; Brüggen, M.; Reiprich, T.H.; Dolag, K.; Heywood, I.; Andernach, H.; Dettmar, R.J.; Hoeft, M.; Zhang, X.; et al. MeerKAT discovery of a double radio relic and odd radio circle. arXiv 2023, arXiv:2304.11784. [Google Scholar] [CrossRef]
- Omar, A. Odd radio circles as supernovae remnants in the intragroup medium. Mon. Not. R. Astron. Soc. 2022, 513, 101–105. [Google Scholar] [CrossRef]
- Filipović, M.D.; Payne, J.L.; Alsaberi, R.Z.E.; Norris, R.P.; Macgregor, P.J.; Rudnick, L.; Koribalski, B.S.; Leahy, D.; Ducci, L.; Kothes, R.; et al. Mysterious odd radio circle near the large magellanic cloud—An intergalactic supernova remnant? Mon. Not. R. Astron. Soc. 2022, 512, 265–284. [Google Scholar] [CrossRef]
- Sarbadhicary, S.K.; Thompson, T.A.; Lopez, L.A.; Mathur, S. On Odd Radio Circles as Supernova Remnants. arXiv 2022, arXiv:2209.10554. [Google Scholar]
- Kirillov, A.A.; Savelova, E.P. Possible formation of ring galaxies by torus-shaped magnetic wormholes. Eur. Phys. J. C 2020, 80, 810. [Google Scholar] [CrossRef]
- Kirillov, A.A.; Savelova, E.P.; Vladykina, P.O. Possible Effects of the Fractal Distribution of Relic Wormholes. Universe 2021, 7, 178. [Google Scholar] [CrossRef]
- Beck, A.M.; Dolag, K.; Lesch, H.; Kronberg, P.P. Strong magnetic fields and large rotation measures in protogalaxies from supernova seeding. Mon. Not. R. Astron. Soc. 2013, 435, 3575–3586. [Google Scholar] [CrossRef]
- Botteon, A.; van Weeren, R.J.; Brunetti, G.; de Gasperin, F.; Intema, H.T.; Osinga, E.; Di Gennaro, G.; Shimwell, T.W.; Bonafede, A.; Brüggen, M.; et al. A giant radio bridge connecting two galaxy clusters in Abell 1758. Mon. Not. R. Astron. Soc. 2020, 499, L11–L15. [Google Scholar] [CrossRef]
- Bonafede, A.; Brunetti, G.; Vazza, F.; Simionescu, A.; Giovannini, G.; Bonnassieux, E.; Shimwell, T.W.; Brüggen, M.; van Weeren, R.J.; Botteon, A.; et al. The Coma Cluster at Low Frequency ARray Frequencies. I. Insights into Particle Acceleration Mechanisms in the Radio Bridge. Astrophys. J. 2021, 907, 32. [Google Scholar] [CrossRef]
- Brüggen, M.; Reiprich, T.H.; Bulbul, E.; Koribalski, B.S.; Andernach, H.; Rudnick, L.; Hoang, D.N.; Wilber, A.G.; Duchesne, S.W.; Veronica, A.; et al. Radio observations of the merging galaxy cluster system Abell 3391-Abell 3395. Astron. Astrophys. 2021, 647, A3. [Google Scholar] [CrossRef]
- Brunetti, G.; Vazza, F. Second-order Fermi Reacceleration Mechanisms and Large-Scale Synchrotron Radio Emission in Intracluster Bridges. Phys. Rev. Lett. 2020, 124, 051101. [Google Scholar] [CrossRef] [PubMed]
- Jedamzik, K.; Pogosian, L. Relieving the Hubble Tension with Primordial Magnetic Fields. Phys. Rev. Lett. 2020, 125, 181302. [Google Scholar] [CrossRef]
- Vacca, V.; Murgia, M.; Govoni, F.; Enßlin, T.; Oppermann, N.; Feretti, L.; Giovannini, G.; Loi, F. Magnetic Fields in Galaxy Clusters and in the Large-Scale Structure of the Universe. Galaxies 2018, 6, 142. [Google Scholar] [CrossRef]
- Vazza, F.; Ferrari, C.; Bonafede, A.; Brüggen, M.; Gheller, C.; Braun, R.; Brown, S. Filaments of the radio cosmic web: Opportunities and challenges for SKA. In Proceedings of the Advancing Astrophysics with the Square Kilometre Array (AASKA14), Giardini Naxos, Italy, 9–13 June 2014; p. 97. [Google Scholar]
- Vernstrom, T.; West, J.; Vazza, F.; Wittor, D.; Riseley, C.J.; Heald, G. Polarized accretion shocks from the cosmic web. Sci. Adv. 2023, 9, eade7233. [Google Scholar] [CrossRef]
- Neronov, A.; Vovk, I. Evidence for Strong Extragalactic Magnetic Fields from Fermi Observations of TeV Blazars. Science 2010, 328, 73. [Google Scholar] [CrossRef] [PubMed]
- Braun, R.; Bourke, T.; Green, J.A.; Keane, E.; Wagg, J. Advancing Astrophysics with the Square Kilometre Array. In Proceedings of the Advancing Astrophysics with the Square Kilometre Array (AASKA14), Giardini Naxos, Italy, 9–13 June 2014; p. 174. [Google Scholar] [CrossRef]
- Merloni, A.; Predehl, P.; Becker, W.; Böhringer, H.; Boller, T.; Brunner, H.; Brusa, M.; Dennerl, K.; Freyberg, M.; Friedrich, P.; et al. eROSITA Science Book: Mapping the Structure of the Energetic Universe. arXiv 2012, arXiv:1209.3114. [Google Scholar]
- ZuHone, J.A.; Markevitch, M.; Ruszkowski, M.; Lee, D. Cold Fronts and Gas Sloshing in Galaxy Clusters with Anisotropic Thermal Conduction. Astrophys. J. 2013, 762, 69. [Google Scholar] [CrossRef]
- Lazarian, A.; Xu, S. Diffusion of Cosmic Rays in MHD Turbulence with Magnetic Mirrors. Astrophys. J. 2021, 923, 53. [Google Scholar] [CrossRef]
- Montanino, D.; Vazza, F.; Mirizzi, A.; Viel, M. Enhancing the Spectral Hardening of Cosmic TeV Photons by Mixing with Axionlike Particles in the Magnetized Cosmic Web. Phys. Rev. Lett. 2017, 119, 101101. [Google Scholar] [CrossRef]
- Basu, A.; Goswami, J.; Schwarz, D.J.; Urakawa, Y. Searching for Axionlike Particles under Strong Gravitational Lenses. Phys. Rev. Lett. 2021, 126, 191102. [Google Scholar] [CrossRef]
- Berezinsky, V.S.; Blasi, P.; Ptuskin, V.S. Clusters of Galaxies as Storage Room for Cosmic Rays. Astrophys. J. 1997, 487, 529–535. [Google Scholar] [CrossRef]
- Hackstein, S.; Vazza, F.; Brüggen, M.; Sorce, J.G.; Gottlöber, S. Simulations of ultra-high energy cosmic rays in the local Universe and the origin of cosmic magnetic fields. Mon. Not. R. Astron. Soc. 2018, 475, 2519–2529. [Google Scholar] [CrossRef]
- Dolag, K.; Bartelmann, M.; Lesch, H. SPH simulations of magnetic fields in galaxy clusters. Astron. Astrophys. 1999, 348, 351–363. [Google Scholar]
- Shimwell, T.W.; Röttgering, H.J.A.; Best, P.N.; Williams, W.L.; Dijkema, T.J.; de Gasperin, F.; Hardcastle, M.J.; Heald, G.H.; Hoang, D.N.; Horneffer, A.; et al. The LOFAR Two-metre Sky Survey. I. Survey description and preliminary data release. Astron. Astrophys. 2017, 598, A104. [Google Scholar] [CrossRef]
- Shimwell, T.W.; Hardcastle, M.J.; Tasse, C.; Best, P.N.; Röttgering, H.J.A.; Williams, W.L.; Botteon, A.; Drabent, A.; Mechev, A.; Shulevski, A.; et al. The LOFAR Two-metre Sky Survey. V. Second data release. Astron. Astrophys. 2022, 659, A1. [Google Scholar] [CrossRef]
- McConnell, D.; Hale, C.L.; Lenc, E.; Banfield, J.K.; Heald, G.; Hotan, A.W.; Leung, J.K.; Moss, V.A.; Murphy, T.; O’Brien, A.; et al. The Rapid ASKAP Continuum Survey I: Design and first results. Publ. Astron. Soc. Aust. 2020, 37, e048. [Google Scholar] [CrossRef]
- Hale, C.L.; McConnell, D.; Thomson, A.J.M.; Lenc, E.; Heald, G.H.; Hotan, A.W.; Leung, J.K.; Moss, V.A.; Murphy, T.; Pritchard, J.; et al. The Rapid ASKAP Continuum Survey Paper II: First Stokes I Source Catalogue Data Release. Publ. Astron. Soc. Aust. 2021, 38, e058. [Google Scholar] [CrossRef]
- Knowles, K.; Cotton, W.D.; Rudnick, L.; Camilo, F.; Goedhart, S.; Deane, R.; Ramatsoku, M.; Bietenholz, M.F.; Brüggen, M.; Button, C.; et al. The MeerKAT Galaxy Cluster Legacy Survey. I. Survey Overview and Highlights. Astron. Astrophys. 2022, 657, A56. [Google Scholar] [CrossRef]
- Gupta, Y.; Ajithkumar, B.; Kale, H.S.; Nayak, S.; Sabhapathy, S.; Sureshkumar, S.; Swami, R.V.; Chengalur, J.N.; Ghosh, S.K.; Ishwara-Chandra, C.H.; et al. The upgraded GMRT: Opening new windows on the radio Universe. Curr. Sci. 2017, 113, 707–714. [Google Scholar] [CrossRef]
- Lal, D.V. Upgraded GMRT Observations of the Coma Cluster of Galaxies: The Observations. Astrophys. J. Suppl. Ser. 2020, 250, 22. [Google Scholar] [CrossRef]
- Perley, R.A. The VLA Upgrade. In American Astronomical Society Meeting Abstracts; American Astronomical Society: Madison, WI, USA, 1996; Volume 188, p. 895. [Google Scholar]



Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the author. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Wittor, D. Cosmic-Ray Acceleration and Magnetic Fields in Galaxy Clusters and Beyond: Insights from Radio Observations. Universe 2023, 9, 319. https://doi.org/10.3390/universe9070319
Wittor D. Cosmic-Ray Acceleration and Magnetic Fields in Galaxy Clusters and Beyond: Insights from Radio Observations. Universe. 2023; 9(7):319. https://doi.org/10.3390/universe9070319
Chicago/Turabian StyleWittor, Denis. 2023. "Cosmic-Ray Acceleration and Magnetic Fields in Galaxy Clusters and Beyond: Insights from Radio Observations" Universe 9, no. 7: 319. https://doi.org/10.3390/universe9070319
APA StyleWittor, D. (2023). Cosmic-Ray Acceleration and Magnetic Fields in Galaxy Clusters and Beyond: Insights from Radio Observations. Universe, 9(7), 319. https://doi.org/10.3390/universe9070319




