The Lense–Thirring Effect on the Galilean Moons of Jupiter
Abstract
1. Introduction
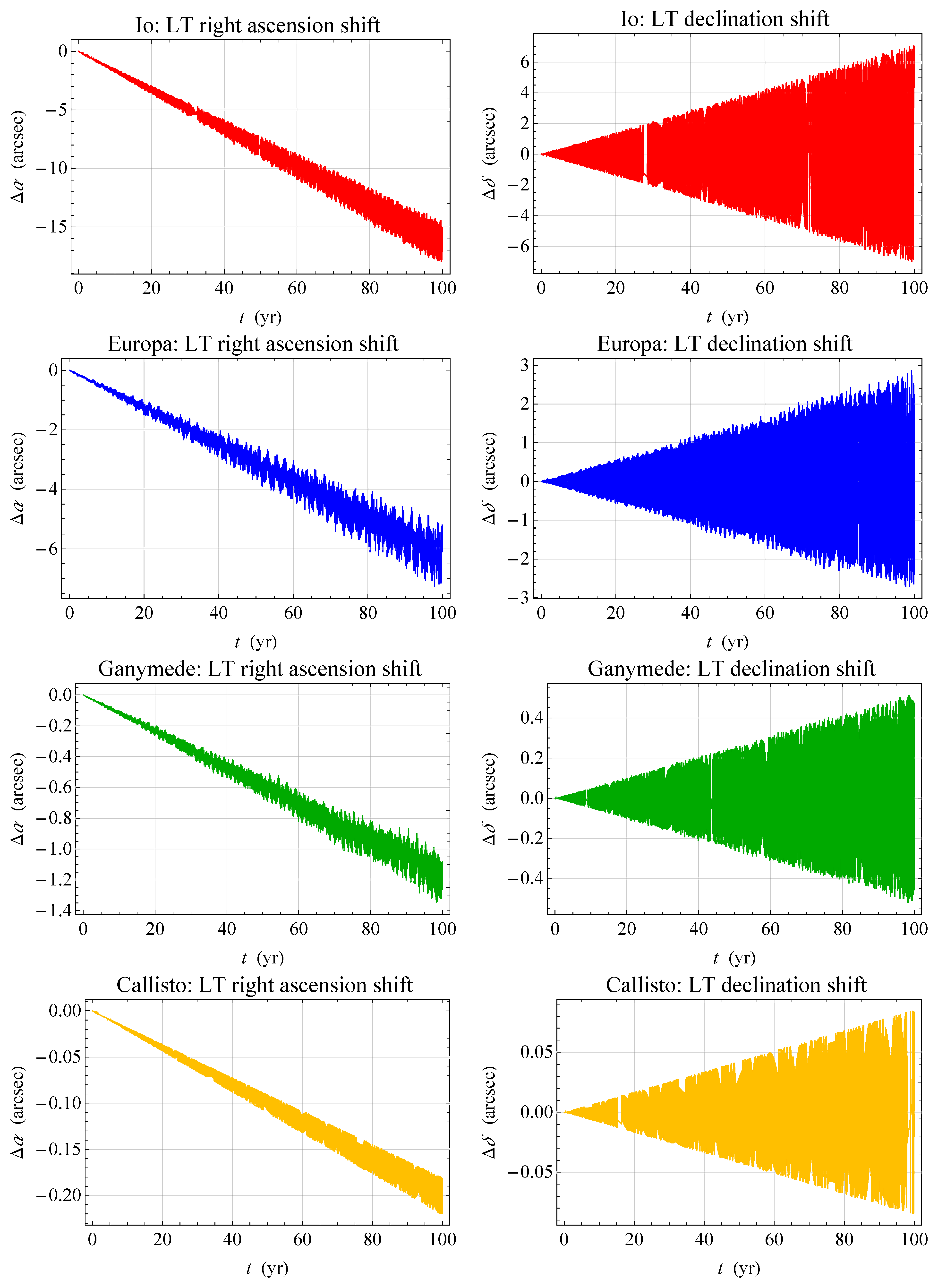
2. The Lense–Thirring Signatures
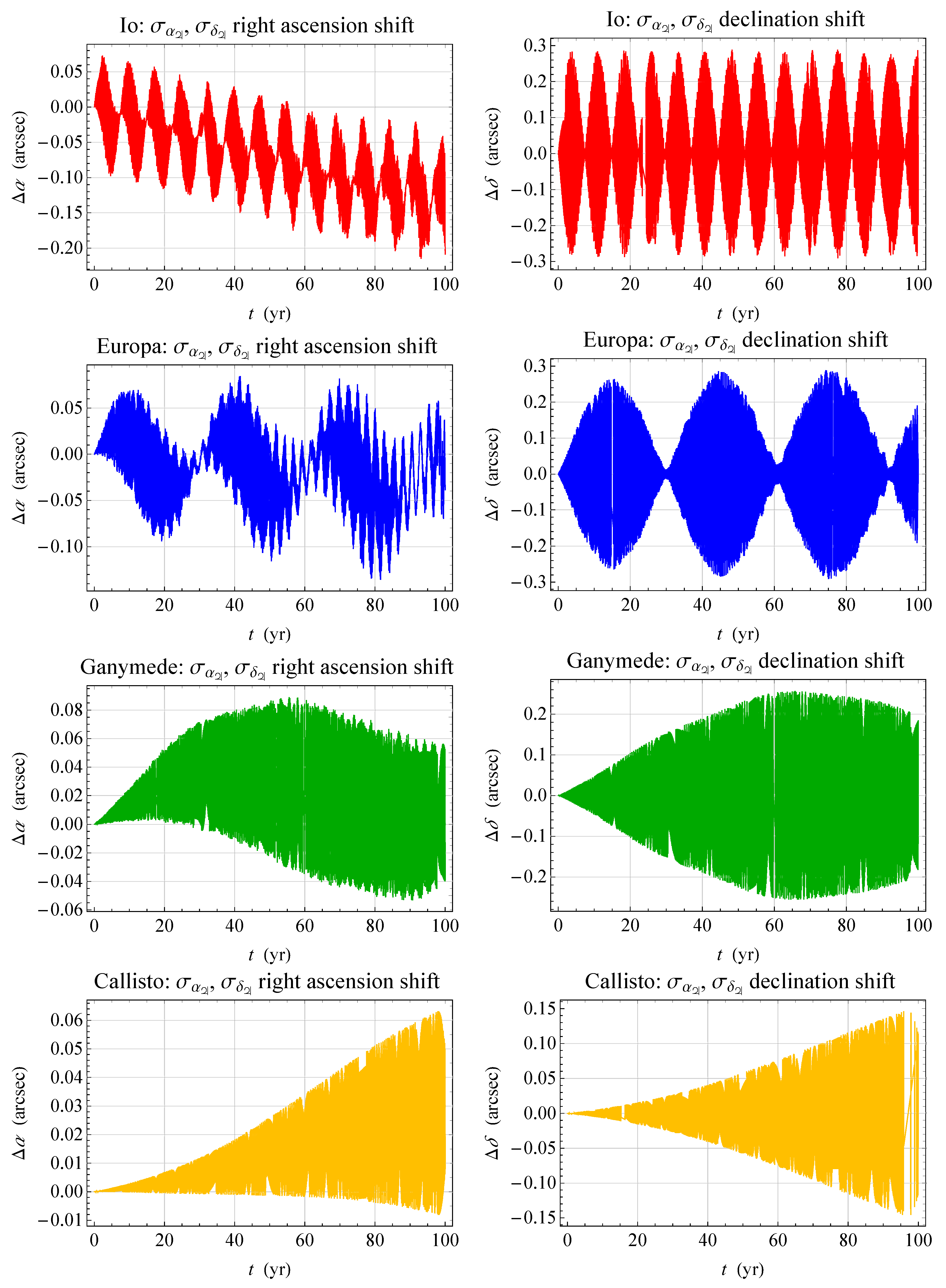
3. The Impact of the Zonal Harmonics of the Jovian Multipolar Gravity Field
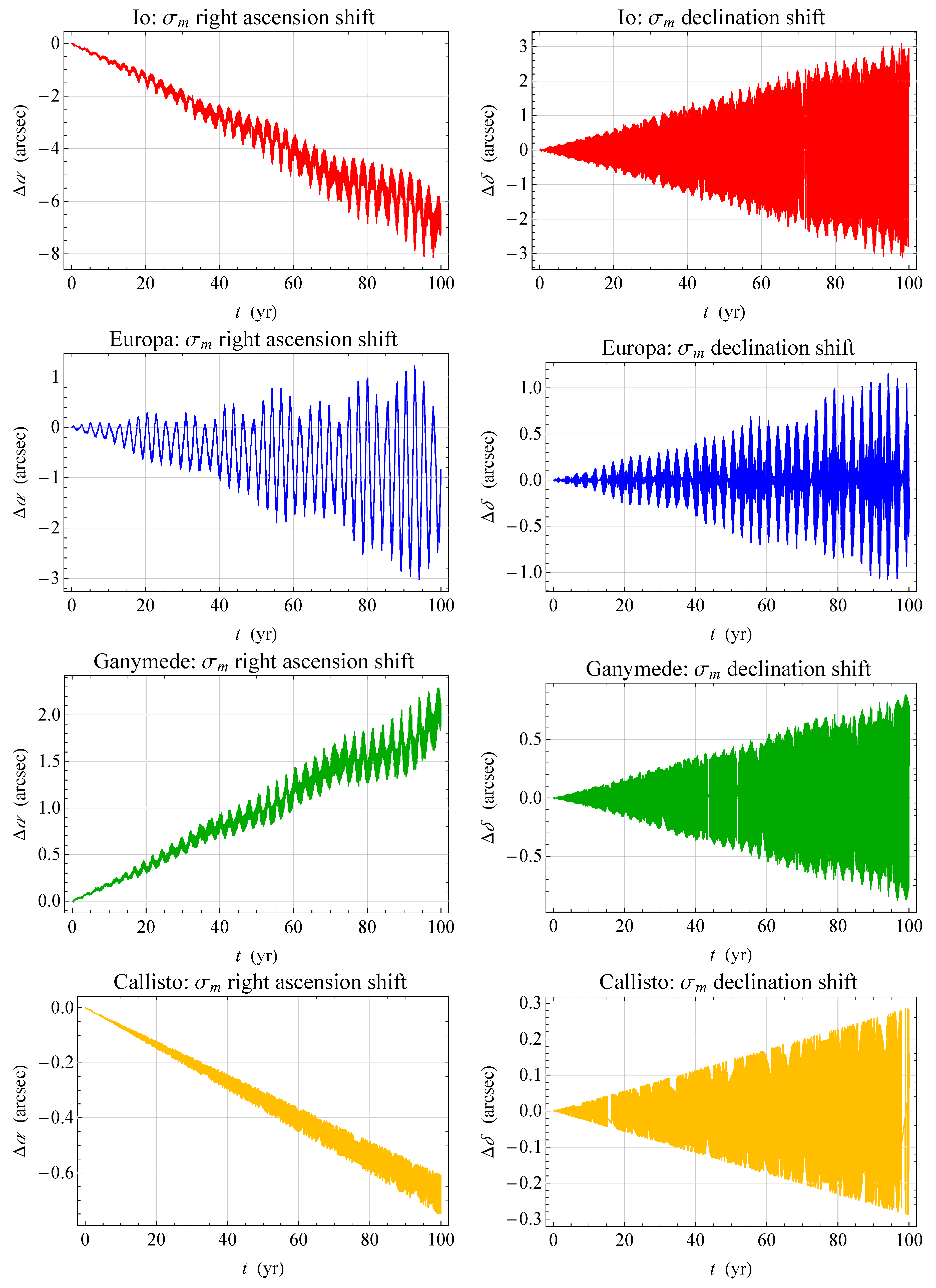
4. The Impact of the Newtonian -Body Mutual Perturbations
5. Discussion and Conclusions
Funding
Data Availability Statement
Conflicts of Interest
| 1 | It was launched on 14 April 2023. See the mission’s webpage https://www.esa.int/Science_Exploration/Space_Science/Juice (accessed on 20 June 2023). |
| 2 | Its launch is scheduled for October 2024. See the mission’s website https://www.jpl.nasa.gov/missions/europa-clipper (accessed on 20 June 2023). |
| 3 | R. A. Jacobson, personal communication to L. Iorio, March 2023. |
| 4 | R. A. Jacobson, private communication to L. Iorio, March 2023. |
| 5 | In Equation (1), G is the Newtonian constant of gravitation, c is the speed of light in vacuum, and and are the position and velocity vectors of the test particle with respect to the spinning central body, respectively. |
References
- Misner, C.W.; Thorne, K.S.; Wheeler, J.A. Gravitation; Princeton University Press: Oxford, UK, 2017. [Google Scholar]
- Cattaneo, C. General relativity: Relative standard mass, momentum, energy and gravitational field in a general system of reference. Nuovo Cim. 1958, 10, 318–337. [Google Scholar] [CrossRef]
- Thorne, K.S.; MacDonald, D.A.; Price, R.H. Black Holes: The Membrane Paradigm; Yale University Press: London, UK, 1986. [Google Scholar]
- Thorne, K.S. Black Holes: The Membrane Viewpoint. In Highlights of Modern Astrophysics: Concepts and Controversies; Shapiro, S.L., Teukolsky, S.A., Salpeter, E.E., Eds.; Wiley: New York, NY, USA, 1986; pp. 103–161. [Google Scholar]
- Thorne, K.S. Gravitomagnetism, jets in quasars, and the Stanford Gyroscope Experiment. In Near Zero: New Frontiers of Physics; Fairbank, J.D., Deaver, B.S.J., Everitt, C.W.F., Michelson, P.F., Eds.; Freeman: New York, NY, USA, 1988; pp. 573–586. [Google Scholar]
- Harris, E.G. Analogy between general relativity and electromagnetism for slowly moving particles in weak gravitational fields. Am. J. Phys. 1991, 59, 421–425. [Google Scholar] [CrossRef]
- Jantzen, R.T.; Carini, P.; Bini, D. The many faces of gravitoelectromagnetism. Ann. Phys. 1992, 215, 1–50. [Google Scholar] [CrossRef]
- Mashhoon, B. Gravitoelectromagnetism. In Reference Frames and Gravitomagnetism; Pascual-Sánchez, J.F., Floría, L., San Miguel, A., Vicente, F., Eds.; World Scientific: Singapore, 2001; pp. 121–132. [Google Scholar] [CrossRef]
- Rindler, W. Relativity: Special, General, and Cosmological; Oxford University Press: New York, NY, USA, 2001. [Google Scholar]
- Mashhoon, B. Gravitoelectromagnetism: A Brief Review. In The Measurement of Gravitomagnetism: A Challenging Enterprise; Iorio, L., Ed.; Nova Science: New York, NY, USA, 2007; pp. 29–39. [Google Scholar]
- Costa, L.F.O.; Herdeiro, C.A.R. Gravitoelectromagnetic analogy based on tidal tensors. PRD 2008, 78, 024021. [Google Scholar] [CrossRef]
- Costa, L.F.O.; Natário, J. Gravito-electromagnetic analogies. Gen. Relativ. Gravit. 2014, 46, 1792. [Google Scholar] [CrossRef]
- Costa, L.F.O.; Natário, J. Frame-Dragging: Meaning, Myths, and Misconceptions. Universe 2021, 7, 388. [Google Scholar] [CrossRef]
- Ruggiero, M.L. A Note on the Gravitoelectromagnetic Analogy. Universe 2021, 7, 451. [Google Scholar] [CrossRef]
- Braginsky, V.B.; Caves, C.M.; Thorne, K.S. Laboratory experiments to test relativistic gravity. PRD 1977, 15, 2047–2068. [Google Scholar] [CrossRef]
- Dymnikova, I.G. Reviews of Topical Problems: Motion of particles and photons in the gravitational field of a rotating body (In memory of Vladimir Afanas’evich Ruban). Sov. Phys. Usp. 1986, 29, 215–237. [Google Scholar] [CrossRef]
- Tartaglia, A. Angular-momentum effects in weak gravitational fields. Europhys. Lett. 2002, 60, 167–173. [Google Scholar] [CrossRef]
- Ruggiero, M.L.; Tartaglia, A. Gravitomagnetic effects. Nuovo Cim. B 2002, 117, 743. [Google Scholar]
- Schäfer, G. Gravitomagnetic Effects. Gen. Relativ. Gravit. 2004, 36, 2223–2235. [Google Scholar] [CrossRef]
- Schäfer, G. Gravitomagnetism in Physics and Astrophysics. Space Sci. Rev. 2009, 148, 37–52. [Google Scholar] [CrossRef]
- Stella, L.; Possenti, A. Lense-Thirring Precession in the Astrophysical Context. Space Sci. Rev. 2009, 148, 105–121. [Google Scholar] [CrossRef]
- Petit, G.; Luzum, B. IERS Conventions (2010); IERS Technical Note; Verlag des Bundesamts für Kartographie und Geodäsie: Frankfurt, Germany, 2010; Volume 36. [Google Scholar]
- Soffel, M.H. Relativity in Astrometry, Celestial Mechanics and Geodesy; Springer: Heidelberg, Germany, 1989. [Google Scholar]
- Brumberg, V.A. Essential Relativistic Celestial Mechanics; Adam Hilger: Bristol, UK, 1991. [Google Scholar]
- Soffel, M.H.; Han, W.B. Applied General Relativity; Astronomy and Astrophysics Library; Springer: Cham, Switzerland, 2019. [Google Scholar]
- Lense, J.; Thirring, H. Über den Einfluß der Eigenrotation der Zentralkörper auf die Bewegung der Planeten und Monde nach der Einsteinschen Gravitationstheorie. Phys. Z 1918, 19, 156–163. [Google Scholar]
- Mashhoon, B.; Hehl, F.W.; Theiss, D.S. On the gravitational effects of rotating masses: The Thirring-Lense papers. Gen. Relativ. Gravit. 1984, 16, 711–750. [Google Scholar] [CrossRef]
- Pfister, H. On the history of the so-called Lense-Thirring effect. Gen. Relativ. Gravit. 2007, 39, 1735–1748. [Google Scholar] [CrossRef]
- Pfister, H. The History of the So-Called Lense-Thirring Effect. In The Eleventh Marcel Grossmann Meeting: On Recent Developments in Theoretical and Experimental General Relativity, Gravitation and Relativistic Field Theories; Kleinert, H., Jantzen, R.T., Ruffini, R., Eds.; World Scientific: Singapore, 2008; pp. 2456–2458. [Google Scholar] [CrossRef]
- Pfister, H. Editorial note to: Hans Thirring, On the formal analogy between the basic electromagnetic equations and Einstein’s gravity equations in first approximation. Gen. Relativ. Gravit. 2012, 44, 3217–3224. [Google Scholar] [CrossRef]
- Pfister, H. Gravitomagnetism: From Einstein’s 1912 Paper to the Satellites LAGEOS and Gravity Probe B. In Relativity and Gravitation; Bičák, J., Ledvinka, T., Eds.; Springer: Berlin/Heidelberg, Germany, 2014; Volume 157, pp. 191–197. [Google Scholar] [CrossRef]
- Bardeen, J.M.; Petterson, J.A. The Lense-Thirring Effect and Accretion Disks around Kerr Black Holes. Astrophys. J. Lett. 1975, 195, L65. [Google Scholar] [CrossRef]
- Rees, M.J. Relativistic jets and beams in radio galaxies. Nature 1978, 275, 516–517. [Google Scholar] [CrossRef]
- MacDonald, D.; Thorne, K.S. Black-hole electrodynamics—An absolute-space/universal-time formulation. Mon. Not. Roy. Astron. Soc. 1982, 198, 345–382. [Google Scholar] [CrossRef]
- Rees, M.J. Black Hole Models for Active Galactic Nuclei. Annu. Rev. Astron. Astr. 1984, 22, 471–506. [Google Scholar] [CrossRef]
- Armitage, P.J.; Natarajan, P. Lense-Thirring Precession of Accretion Disks around Compact Objects. Astrophys. J 1999, 525, 909–914. [Google Scholar] [CrossRef]
- Ingram, A.; Done, C.; Fragile, P.C. Low-frequency quasi-periodic oscillations spectra and Lense-Thirring precession. Mon. Not. Roy. Astron. Soc. 2009, 397, L101–L105. [Google Scholar] [CrossRef]
- Veledina, A.; Poutanen, J.; Ingram, A. A Unified Lense-Thirring Precession Model for Optical and X-Ray Quasi-periodic Oscillations in Black Hole Binaries. Astrophys. J 2013, 778, 165. [Google Scholar] [CrossRef]
- Franchini, A.; Lodato, G.; Facchini, S. Lense-Thirring precession around supermassive black holes during tidal disruption events. Mon. Not. Roy. Astron. Soc. 2016, 455, 1946–1956. [Google Scholar] [CrossRef]
- Penrose, R. “Golden Oldie”: Gravitational Collapse: The Role of General Relativity. Gen. Relativ. Gravit. 2002, 7, 1141–1165. [Google Scholar] [CrossRef]
- Penrose, R.; Floyd, R.M. Extraction of Rotational Energy from a Black Hole. Nat. Phys. Sci. 1971, 229, 177–179. [Google Scholar] [CrossRef]
- Stuchlík, Z.; Kološ, M.; Tursunov, A. Penrose Process: Its Variants and Astrophysical Applications. Universe 2021, 7, 416. [Google Scholar] [CrossRef]
- Blandford, R.D.; Znajek, R.L. Electromagnetic extraction of energy from Kerr black holes. Mon. Not. Roy. Astron. Soc. 1977, 179, 433–456. [Google Scholar] [CrossRef]
- Zel’Dovich, Y.B. Generation of Waves by a Rotating Body. J. Exp. Theor. Phys. 1971, 14, 180. [Google Scholar]
- Teukolsky, S.A. The Kerr metric. Class. Quantum Gravity 2015, 32, 124006. [Google Scholar] [CrossRef]
- Everitt, C.W.F. The Gyroscope experiment-I: General description and analysis of gyroscope performance. In Proceedings of the International School of Physics “Enrico Fermi”; Course LVI. Experimental Gravitation; Bertotti, B., Ed.; Academic Press: New York, NY, USA; London, UK, 1974; pp. 331–360. [Google Scholar]
- Pugh, G.E. Proposal for a Satellite Test of the Coriolis Prediction of General Relativity; Research Memorandum 11; Weapons Systems Evaluation Group, The Pentagon: Washington, DC, USA, 1959. [Google Scholar]
- Schiff, L. Possible new experimental test of general relativity theory. Phys. Rev. Lett. 1960, 4, 215–217. [Google Scholar] [CrossRef]
- Everitt, C.W.F.; Debra, D.B.; Parkinson, B.W.; Turneaure, J.P.; Conklin, J.W.; Heifetz, M.I.; Keiser, G.M.; Silbergleit, A.S.; Holmes, T.; Kolodziejczak, J.; et al. Gravity Probe B: Final Results of a Space Experiment to Test General Relativity. Phys. Rev. Lett. 2011, 106, 221101. [Google Scholar] [CrossRef]
- Everitt, C.W.F.; Buchman, S.; Debra, D.B.; Keiser, G.M.; Lockhart, J.M.; Muhlfelder, B.; Parkinson, B.W.; Turneaure, J.P. Gravity Probe B: Countdown to Launch. In Gyros, Clocks, Interferometers …: Testing Relativistic Gravity in Space; Lecture Notes in Physics; Lämmerzahl, C., Everitt, C.W.F., Hehl, F.W., Eds.; Springer: Berlin, Germany, 2001; Volume 562, p. 52. [Google Scholar] [CrossRef]
- Pearlman, M.; Arnold, D.; Davis, M.; Barlier, F.; Biancale, R.; Vasiliev, V.; Ciufolini, I.; Paolozzi, A.; Pavlis, E.C.; Sośnica, K.; et al. Laser geodetic satellites: A high-accuracy scientific tool. J. Geod. 2019, 93, 2181–2194. [Google Scholar] [CrossRef]
- Coulot, D.; Deleflie, F.; Bonnefond, P.; Exertier, P.; Laurain, O.; de Saint-Jean, B. Satellite Laser Ranging. In Encyclopedia of Solid Earth Geophysics; Gupta, H.K., Ed.; Encyclopedia of Earth Sciences Series; Springer: Dordrecht, The Netherlands, 2011; pp. 1049–1055. [Google Scholar] [CrossRef]
- Ciufolini, I.; Paolozzi, A.; Koenig, R.; Pavlis, E.C.; Ries, J.; Matzner, R.; Gurzadyan, V.; Penrose, R.; Sindoni, G.; Paris, C. Fundamental Physics and General Relativity with the LARES and LAGEOS satellites. Nucl. Phys. B Proc. Suppl. 2013, 243, 180–193. [Google Scholar] [CrossRef]
- Renzetti, G. History of the attempts to measure orbital frame—Dragging with artificial satellites. Centr. Eur. J. Phys. 2013, 11, 531–544. [Google Scholar] [CrossRef]
- Iorio, L.; Ruggiero, M.L.; Corda, C. Novel considerations about the error budget of the LAGEOS-based tests of frame-dragging with GRACE geopotential models. Acta Astronaut. 2013, 91, 141–148. [Google Scholar] [CrossRef]
- Iorio, L.; Lichtenegger, H.I.M.; Ruggiero, M.L.; Corda, C. Phenomenology of the Lense-Thirring effect in the solar system. Astrophys. Space Sci. 2011, 331, 351–395. [Google Scholar] [CrossRef]
- Venkatraman Krishnan, V.; Bailes, M.; van Straten, W.; Wex, N.; Freire, P.C.C.; Keane, E.F.; Tauris, T.M.; Rosado, P.A.; Bhat, N.D.R.; Flynn, C.; et al. Lense-Thirring frame dragging induced by a fast-rotating white dwarf in a binary pulsar system. Science 2020, 367, 577–580. [Google Scholar] [CrossRef]
- Iorio, L. A comment on ’Lense-Thirring frame dragging induced by a fast-rotating white dwarf in a binary pulsar system’ by V. Venkatraman Krishnan et al. Mon. Not. Roy. Astron. Soc. 2020, 495, 2777–2785. [Google Scholar] [CrossRef]
- Burgay, M.; D’Amico, N.; Possenti, A.; Manchester, R.N.; Lyne, A.G.; Joshi, B.C.; McLaughlin, M.A.; Kramer, M.; Sarkissian, J.M.; Camilo, F.; et al. An increased estimate of the merger rate of double neutron stars from observations of a highly relativistic system. Nature 2003, 426, 531–533. [Google Scholar] [CrossRef] [PubMed]
- Lyne, A.G.; Burgay, M.; Kramer, M.; Possenti, A.; Manchester, R.N.; Camilo, F.; McLaughlin, M.A.; Lorimer, D.R.; D’Amico, N.; Joshi, B.C.; et al. A Double-Pulsar System: A Rare Laboratory for Relativistic Gravity and Plasma Physics. Science 2004, 303, 1153–1157. [Google Scholar] [CrossRef] [PubMed]
- Kehl, M.S.; Wex, N.; Kramer, M.; Liu, K. Future measurements of the Lense-Thirring effect in the Double Pulsar. In The Fourteenth Marcel Grossmann Meeting, Proceedings of the MG14 Meeting on General Relativity, Rome, Italy, 12–18 July 2015; Bianchi, M., Jantzen, R., Ruffini, R., Eds.; World Scientific: Singapore, 2017; pp. 1860–1865. [Google Scholar] [CrossRef]
- Hu, H.; Kramer, M.; Wex, N.; Champion, D.J.; Kehl, M.S. Constraining the dense matter equation-of-state with radio pulsars. Mon. Not. R. Astron. Soc. 2020, 497, 3118–3130. [Google Scholar] [CrossRef]
- Arlot, J.E. Four centuries of observations of the Galilean satellites of Jupiter: Increasing the astrometric accuracy. J. Astron. Hist. Herit. 2019, 22, 78–92. [Google Scholar]
- Schlosser, W.; Schmidt-Kaler, T.; Milone, E.F. The Galilean Moons of Jupiter. In Challenges of Astronomy: Hands-on Experiments for the Sky and Laboratory; Springer: New York, NY, USA, 1991; pp. 54–58. [Google Scholar] [CrossRef]
- Grasset, O.; Dougherty, M.K.; Coustenis, A.; Bunce, E.J.; Erd, C.; Titov, D.; Blanc, M.; Coates, A.; Drossart, P.; Fletcher, L.N.; et al. JUpiter ICy moons Explorer (JUICE): An ESA mission to orbit Ganymede and to characterise the Jupiter system. Planet. Space Sci. 2013, 78, 1–21. [Google Scholar] [CrossRef]
- Korth, H.; Pappalardo, R.; Craft, K.; Daubar, I.; Hay, H.; Howell, S.; Klima, R.; Leonard, E.; Matiella Novak, A.; Persaud, D.; et al. Europa Clipper Mission Update. In Proceedings of the EGU General Assembly Conference Abstracts, Vienna, Austria, 23–27 May 2022. [Google Scholar] [CrossRef]
- Cappuccio, P.; Hickey, A.; Durante, D.; Di Benedetto, M.; Iess, L.; De Marchi, F.; Plainaki, C.; Milillo, A.; Mura, A. Ganymede’s gravity, tides and rotational state from JUICE’s 3GM experiment simulation. Planet. Space Sci. 2020, 187, 104902. [Google Scholar] [CrossRef]
- Magnanini, A. Estimation of the Ephemerides and Gravity Fields of the Galilean Moons Through Orbit Determination of the JUICE Mission. Aerotec. Missili Spaz. 2021, 100, 195–206. [Google Scholar] [CrossRef]
- Cappuccio, P.; Di Benedetto, M.; Durante, D.; Iess, L. Callisto and Europa Gravity Measurements from JUICE 3GM Experiment Simulation. Planet. Sci. J. 2022, 3, 199. [Google Scholar] [CrossRef]
- Fayolle, M.; Dirkx, D.; Lainey, V.; Gurvits, L.I.; Visser, P.N.A.M. Decoupled and coupled moons’ ephemerides estimation strategies application to the JUICE mission. Planet. Space Sci. 2022, 219, 105531. [Google Scholar] [CrossRef]
- Fienga, A.; Minazzoli, O. Testing GR and alternative theories with planetary ephemerides. arXiv 2023, arXiv:2303.01821. [Google Scholar]
- Soffel, M.; Klioner, S.A.; Petit, G.; Wolf, P.; Kopeikin, S.M.; Bretagnon, P.; Brumberg, V.A.; Capitaine, N.; Damour, T.; Fukushima, T.; et al. The IAU 2000 Resolutions for Astrometry, Celestial Mechanics, and Metrology in the Relativistic Framework: Explanatory Supplement. Astron J. 2003, 126, 2687–2706. [Google Scholar] [CrossRef]
- Durante, D.; Parisi, M.; Serra, D.; Zannoni, M.; Notaro, V.; Racioppa, P.; Buccino, D.R.; Lari, G.; Gomez Casajus, L.; Iess, L.; et al. Jupiter’s Gravity Field Halfway Through the Juno Mission. Geophys. Res. Lett. 2020, 47, e86572. [Google Scholar] [CrossRef]
- Bolton, S. The Juno Mission; Springer: Dordrecht, The Netherlands, 2018. [Google Scholar]
- Jacobson, R.A. The Orbits of the Regular Jovian Satellites and the Orientation of the Pole of Jupiter. Personal Communication to Horizons/NAIF, 2021. [Google Scholar]

Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the author. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Iorio, L. The Lense–Thirring Effect on the Galilean Moons of Jupiter. Universe 2023, 9, 304. https://doi.org/10.3390/universe9070304
Iorio L. The Lense–Thirring Effect on the Galilean Moons of Jupiter. Universe. 2023; 9(7):304. https://doi.org/10.3390/universe9070304
Chicago/Turabian StyleIorio, Lorenzo. 2023. "The Lense–Thirring Effect on the Galilean Moons of Jupiter" Universe 9, no. 7: 304. https://doi.org/10.3390/universe9070304
APA StyleIorio, L. (2023). The Lense–Thirring Effect on the Galilean Moons of Jupiter. Universe, 9(7), 304. https://doi.org/10.3390/universe9070304





