On the Possibility of Observing Negative Shapiro-like Delay Using Michelson–Morley-Type Experiments
Abstract
1. Introduction
2. Gravitational Time Advancement
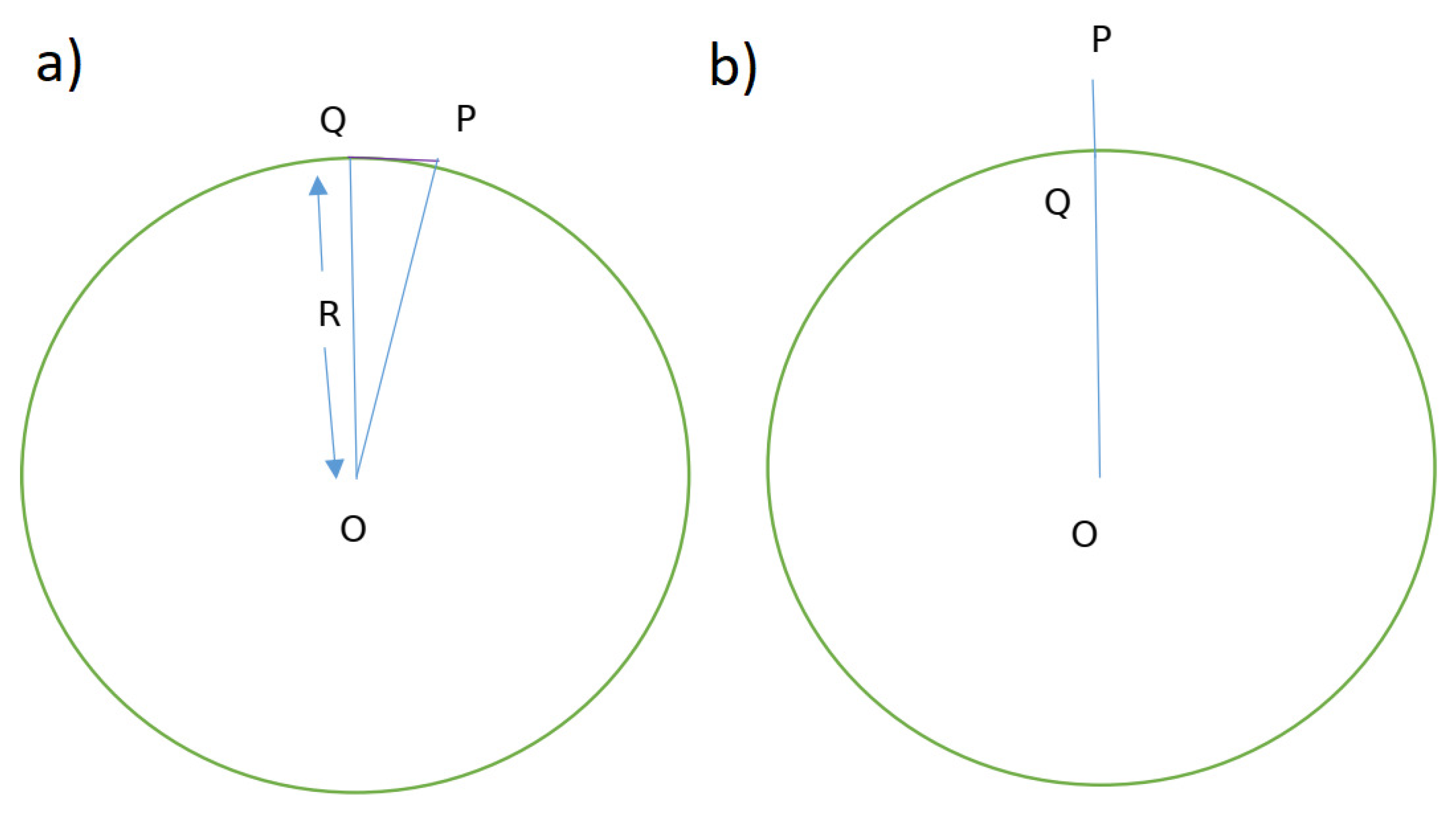
3. Gravitational Negative Time Delay for Small Distance Travel from the Earth’s Surface
4. Possibility of Experimental Detection of the Negative Time Delay
5. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Michelson, A.A. The relative motion of the Earth and the Luminiferous ether. Am. J. Sci. 1881, 22, 120–129. [Google Scholar] [CrossRef]
- Michelson, A.A.; Morley, E.W. On the Relative Motion of the Earth and the Luminiferous Ether. Am. J. Sci. 1887, 34, 333–345. [Google Scholar] [CrossRef]
- Michelson, A.A.; Morley, E.W. On the relative motion of the earth and the luminiferous aether. Philos. Mag. 1887, 5, 449–463. [Google Scholar] [CrossRef]
- Lipa, J.A.; Nissen, J.A.; Wang, S.; Stricker, D.A.; Avaloff, D. New Limit on Signals of Lorentz Violation in Electrodynamics. Phys. Rev. Lett. 2003, 90, 060403. [Google Scholar] [CrossRef]
- Müller, H.; Herrmann, S.; Braxmaier, C.; Schiller, S.; Peters, A. Modern Michelson-Morley Experiment using Cryogenic Optical Resonators. Phys. Rev. Lett. 2003, 91, 020401. [Google Scholar] [CrossRef] [PubMed]
- Wolf, P.; Bize, S.; Clairon, A.; Santarelli, G.; Tobar, M.E.; Luiten, A.N. Improved test of Lorentz invariance in electrodynamics. Phys. Rev. D 2004, 70, 051902. [Google Scholar] [CrossRef]
- Stanwix, P.L.; Tobar, M.E.; Wolf, P.; Susli, M.; Locke, C.R.; Ivanov, E.N.; Winterflood, J.; van Kann, F. Test of Lorentz invariance in electrodynamics using rotating cryogenic sapphire microwave oscillators. Phys. Rev. Lett. 2005, 94, 040404. [Google Scholar] [CrossRef]
- Stanwix, P.L.; Tobar, M.E.; Wolf, P.; Locke, C.R.; Ivanov, E.N. Improved test of Lorentz invariance in electrodynamics using rotating cryogenic sapphire oscillators. Phys. Rev. D 2006, 74, 081101. [Google Scholar] [CrossRef]
- Herrmann, S.; Senger, A.; Kovalchuk, E.; Müller, H.; Peters, A. Test of the Isotropy of the Speed of Light Using a Continuously Rotating Optical Resonator. Phys. Rev. Lett. 2005, 95, 150401. [Google Scholar] [CrossRef]
- Antonini, P.; Okhapkin, M.; Göklü, E.; Schiller, S. Test of constancy of speed of light with rotating cryogenic optical resonators. Phys. Rev. A 2005, 71, 050101. [Google Scholar] [CrossRef]
- Müller, H.; Stanwix, P.L.; Tobar, M.E.; Ivanov, E.N.; Wolf, P.; Herrmann, S.; Senger, A.; Kovalchuk, E.; Peters, A. Tests of Relativity by Complementary Rotating Michelson-Morley Experiments. Phys. Rev. Lett. 2007, 99, 050401. [Google Scholar] [CrossRef] [PubMed]
- Kostelecky, V.A.; Mewes, M. Signals for Lorentz violation in electrodynamics. Phys. Rev. D 2002, 66, 056005. [Google Scholar] [CrossRef]
- Kostelecky, V.A.; Mewes, M. Sensitive Polarimetric Search for Relativity Violations in Gamma-Ray Bursts. Phys. Rev. Lett. 2006, 97, 140401. [Google Scholar] [CrossRef]
- Müller, H.; Stanwix, P.L.; Herrmann, S.; Peters, A.; Lümmerzahl, C. Electromagnetic cavities and Lorentz invariance violation. Phys. Rev. D 2003, 67, 056006. [Google Scholar] [CrossRef]
- Müller, H. Testing Lorentz invariance by the use of vacuum and matter filled cavity resonators. Phys. Rev. D 2005, 71, 045004. [Google Scholar] [CrossRef]
- Eisele, C.; Nevsky, A.Y.; Schiller, S. Laboratory Test of the Isotropy of Light Propagation at the 10−17 Level. Phys. Rev. Lett. 2009, 103, 090401. [Google Scholar] [CrossRef]
- Shapiro, I.I. Fourth Test of General Relativity. Phys. Rev. Lett. 1964, 13, 789. [Google Scholar] [CrossRef]
- Bhadra, A.; Nandi, K.K. Gravitational time advancement and its possible detection. Gen. Relativ. Gravit. 2010, 42, 293–302. [Google Scholar] [CrossRef]
- Shapiro, I.I.; Pettengill, G.H.; Ash, M.E.; Stone, M.L.; Smith, W.B.; Ingalls, R.P.; Brockelman, R.A. Fourth Test of General Relativity: Preliminary Results. Phys. Rev. Lett. 1968, 20, 1265. [Google Scholar] [CrossRef]
- Desai, S.; Kahya, E.O. The Galactic One-Way Shapiro Delay to PSR B1937+21. Mod. Phys. Lett. 2016, 31, 1650083. [Google Scholar] [CrossRef]
- Desai, S.; Kahya, E.O.; Woodard, R.P. Reduced time delay for gravitational waves with dark matter emulators. Phys. Rev. D 2008, 77, 124041. [Google Scholar] [CrossRef]
- Cromartie, H.T.; Fonseca, E.; Ransom, S.M.; Demorest, P.B.; Arzoumanian, Z.; Blumer, H.; Brook, P.R.; DeCesar, M.E.; Dolch, T.; Ellis, J.A.; et al. Relativistic Shapiro delay measurements of an extremely massive millisecond pulsar. Nat. Astron. 2020, 4, 72. [Google Scholar]
- Ghosh, S.; Bhadra, A. Influences of dark energy and dark matter on gravitational time advancement. Eur. Phys. J. C 2015, 75, 494. [Google Scholar] [CrossRef]
- Bertotti, B.; Iess, L.; Tortora, P. A test of general relativity using radio links with the Cassini spacecraft. Nature 2003, 425, 374–376. [Google Scholar] [CrossRef]
- Will, C.M. Theory and Experiment in Gravitational Physics; Revised ed.; Cambridge University Press: Cambridge, UK, 1993. [Google Scholar]
- Ghosh, S.; Bhadra, A.; Mukhopadhyay, A. Probing dark matter and dark energy through gravitational time advancement. Gen. Rel. Grav. 2019, 51, 54. [Google Scholar] [CrossRef]
- Deng, X.-M.; Xie, Y. Gravitational time advancement under gravity’s rainbow. Phys. Lett. B 2017, 772, 152. [Google Scholar] [CrossRef]
- Tuleganova, G.Y.; Karimov, R.K.; Izmailov, R.N.; Potapov, A.A.; Bhadra, A.; Nandi, K.K. Gravitational time advancement effect in Bumblebee gravity for Earth bound systems. Eur. Phys. J. Plus 2023, 138, 94. [Google Scholar] [CrossRef]
- Tartaglia, A.; Ruggiero, M.L. Angular Momentum Effects in Michelson–Morley Type Experiments. Gen. Rel. Grav. 2002, 34, 1371–1382. [Google Scholar]
- Misner, C.W.; Thorne, K.S.; Wheeler, J.A. Gravitation; Freeman: San Francisco, CA, USA, 1973. [Google Scholar]
- Richter, G.W.; Matzner, R.A. Second-order contributions to relativistic time delay in the parametrized post-Newtonian formalism. Phys. Rev. D 1983, 28, 3007. [Google Scholar] [CrossRef]
- Zhang, Y.Z. On the Theory of the Michelson-Morley Experiment in the Earth’s Gravitational Field. Europhys. Lett. 1987, 3, 1251. [Google Scholar] [CrossRef]
- Lipa, J.A.; Buchman, S.; Saraf, S.; Zhou, J.; Alfauwaz, A.; Conklin, J.; Cutler, G.D.; Byer, R.L. Prospects for an advanced Kennedy-Thorndike experiment in low Earth orbit. arXiv 2012, arXiv:1203.3914. [Google Scholar]
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Bhadra, A.; Izmailov, R.N.; Nandi, K.K. On the Possibility of Observing Negative Shapiro-like Delay Using Michelson–Morley-Type Experiments. Universe 2023, 9, 263. https://doi.org/10.3390/universe9060263
Bhadra A, Izmailov RN, Nandi KK. On the Possibility of Observing Negative Shapiro-like Delay Using Michelson–Morley-Type Experiments. Universe. 2023; 9(6):263. https://doi.org/10.3390/universe9060263
Chicago/Turabian StyleBhadra, Arunava, Ramil N. Izmailov, and Kamal K. Nandi. 2023. "On the Possibility of Observing Negative Shapiro-like Delay Using Michelson–Morley-Type Experiments" Universe 9, no. 6: 263. https://doi.org/10.3390/universe9060263
APA StyleBhadra, A., Izmailov, R. N., & Nandi, K. K. (2023). On the Possibility of Observing Negative Shapiro-like Delay Using Michelson–Morley-Type Experiments. Universe, 9(6), 263. https://doi.org/10.3390/universe9060263







