Proton Electric Charge Radius from Lepton Scattering
Abstract
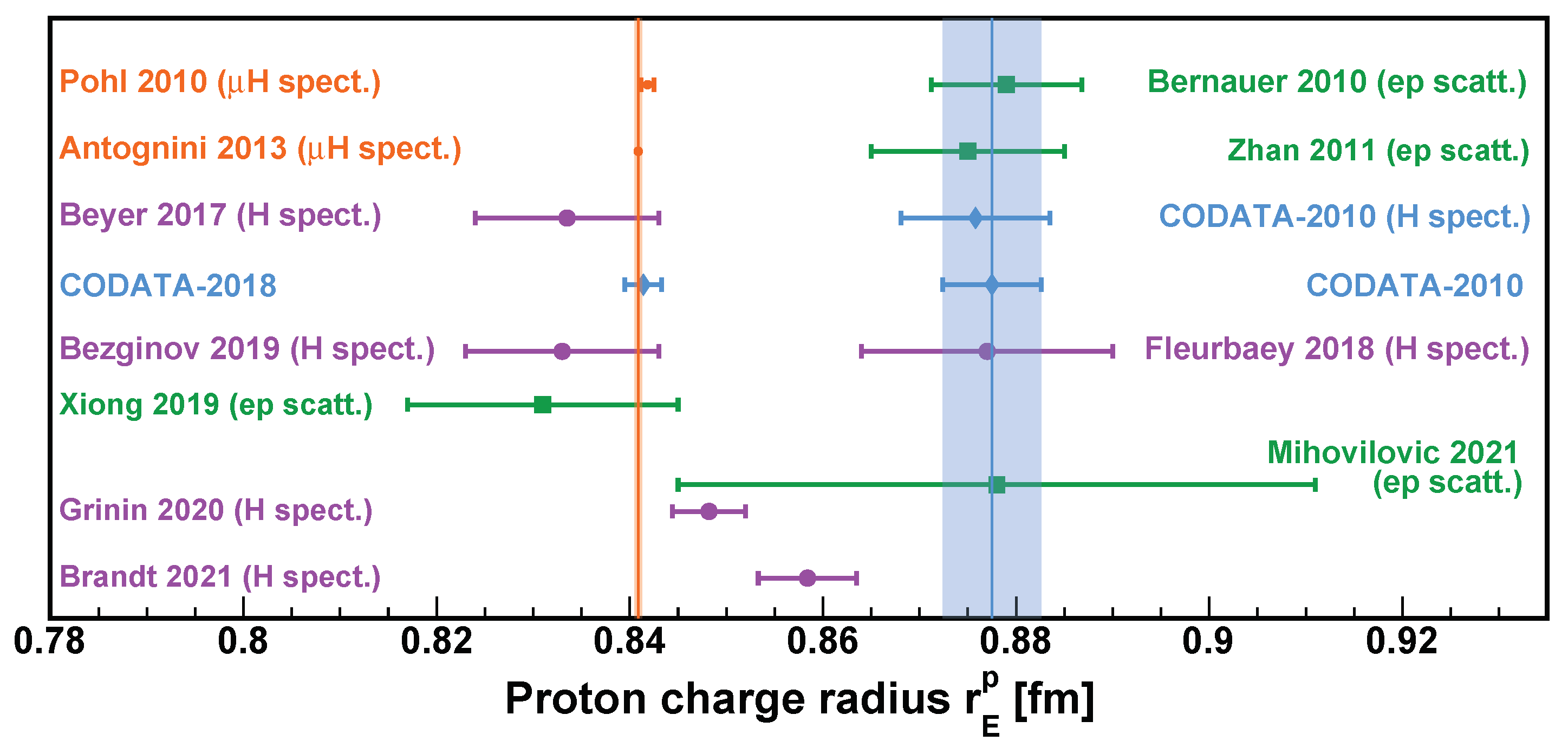
1. Introduction
2. Radius Extraction from Unpolarized Lepton-Proton Scattering Experiments
2.1. Empirical Fits of Electromagnetic Form Factors
2.2. Moments of Transverse Charge Density
3. Recent Progress from Electron Scattering Experiments
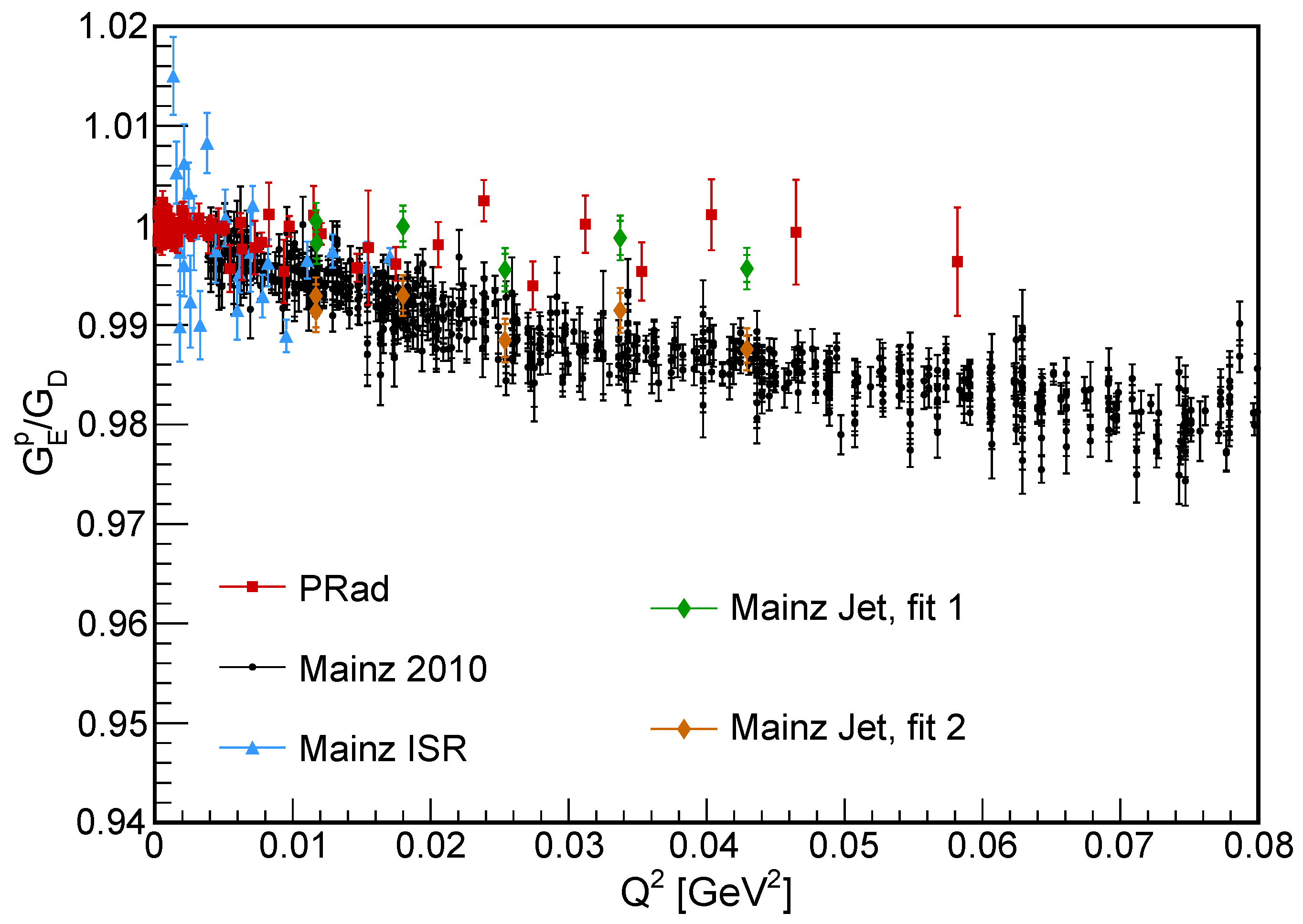
3.1. Initial-State Radiation Experiment at Mainz
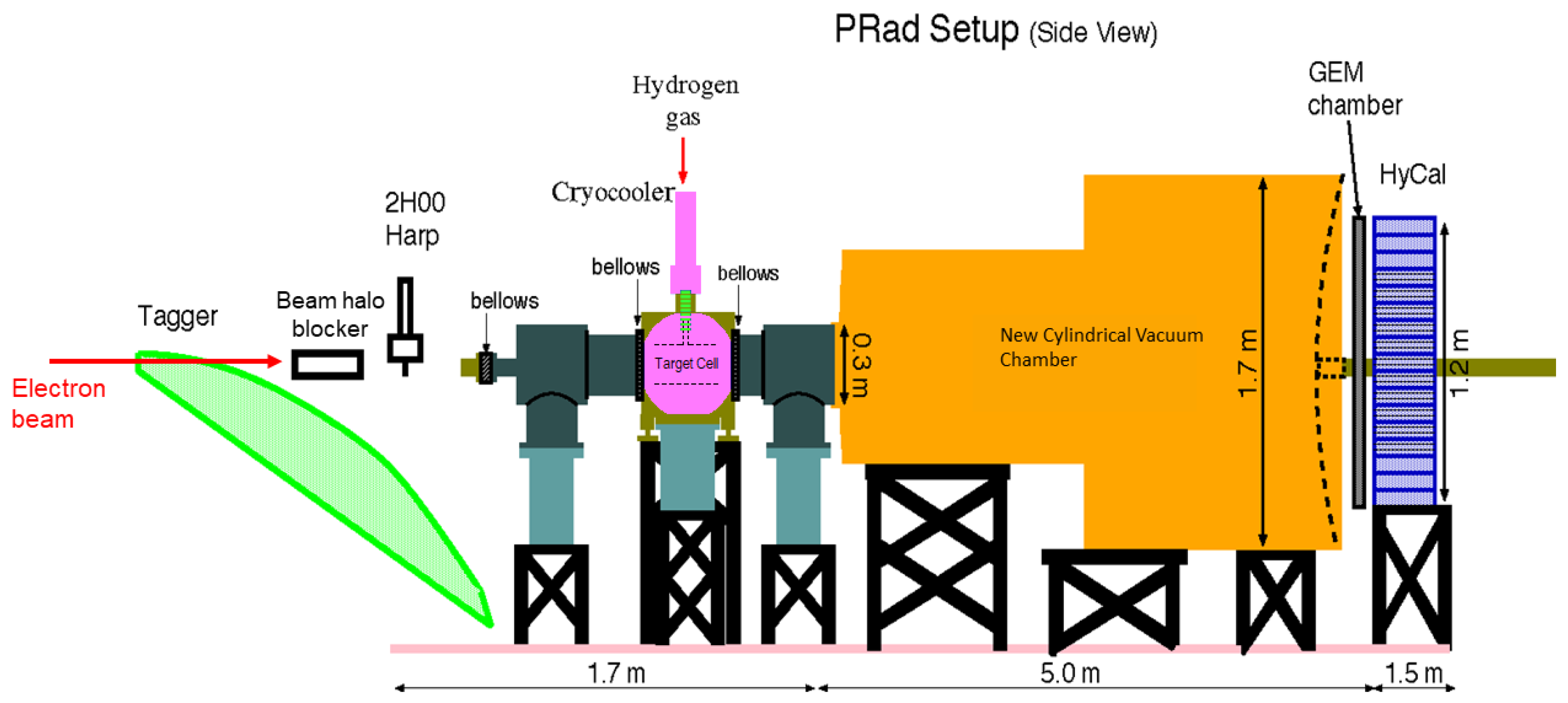
3.2. Proton Charge Radius Experiment at JLab
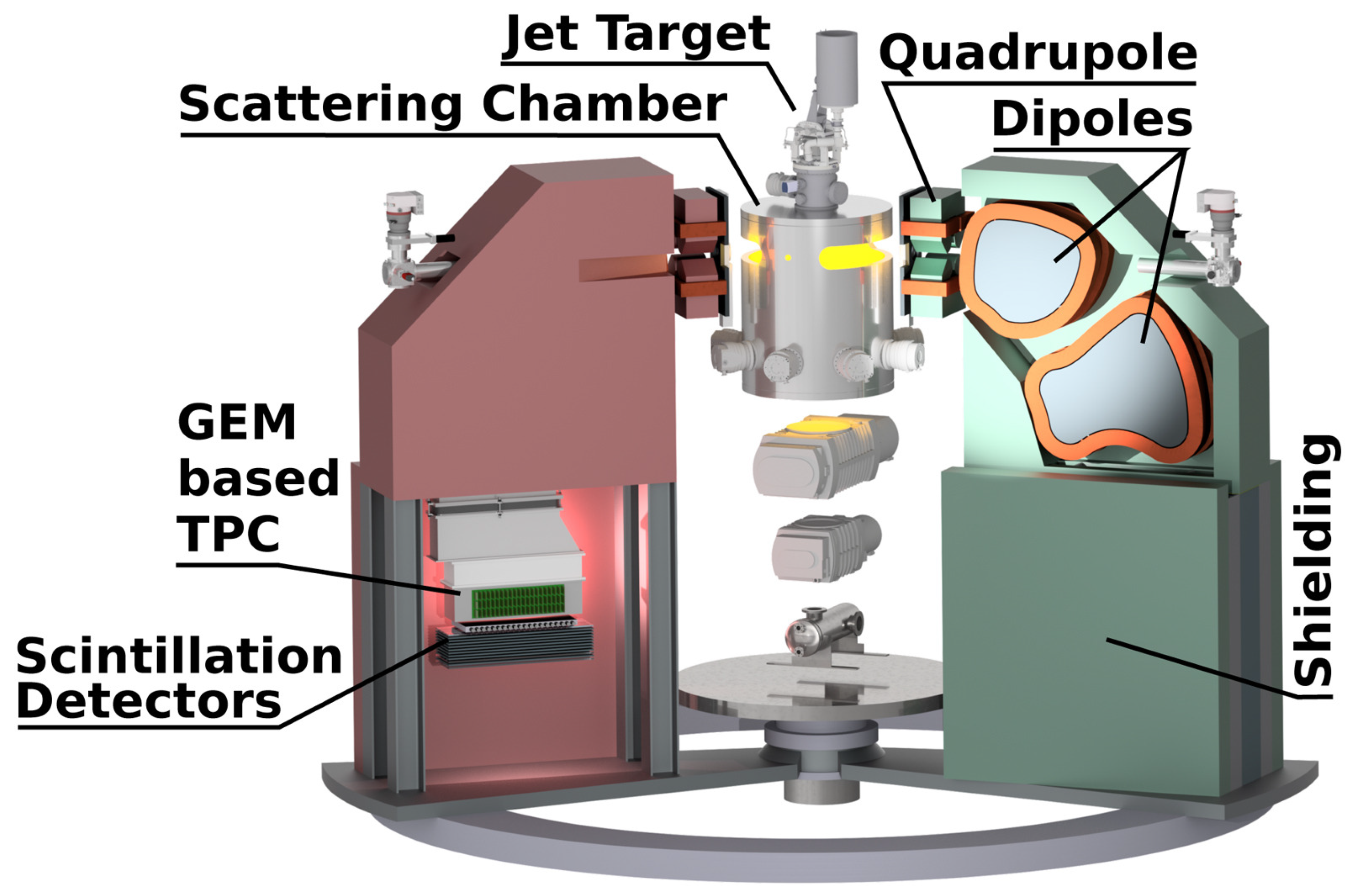
3.3. Jet-Target Experiment at Mainz
4. Recent Re-Analyses and Lattice QCD Calculations
4.1. Re-Analysis of Form Factor Data
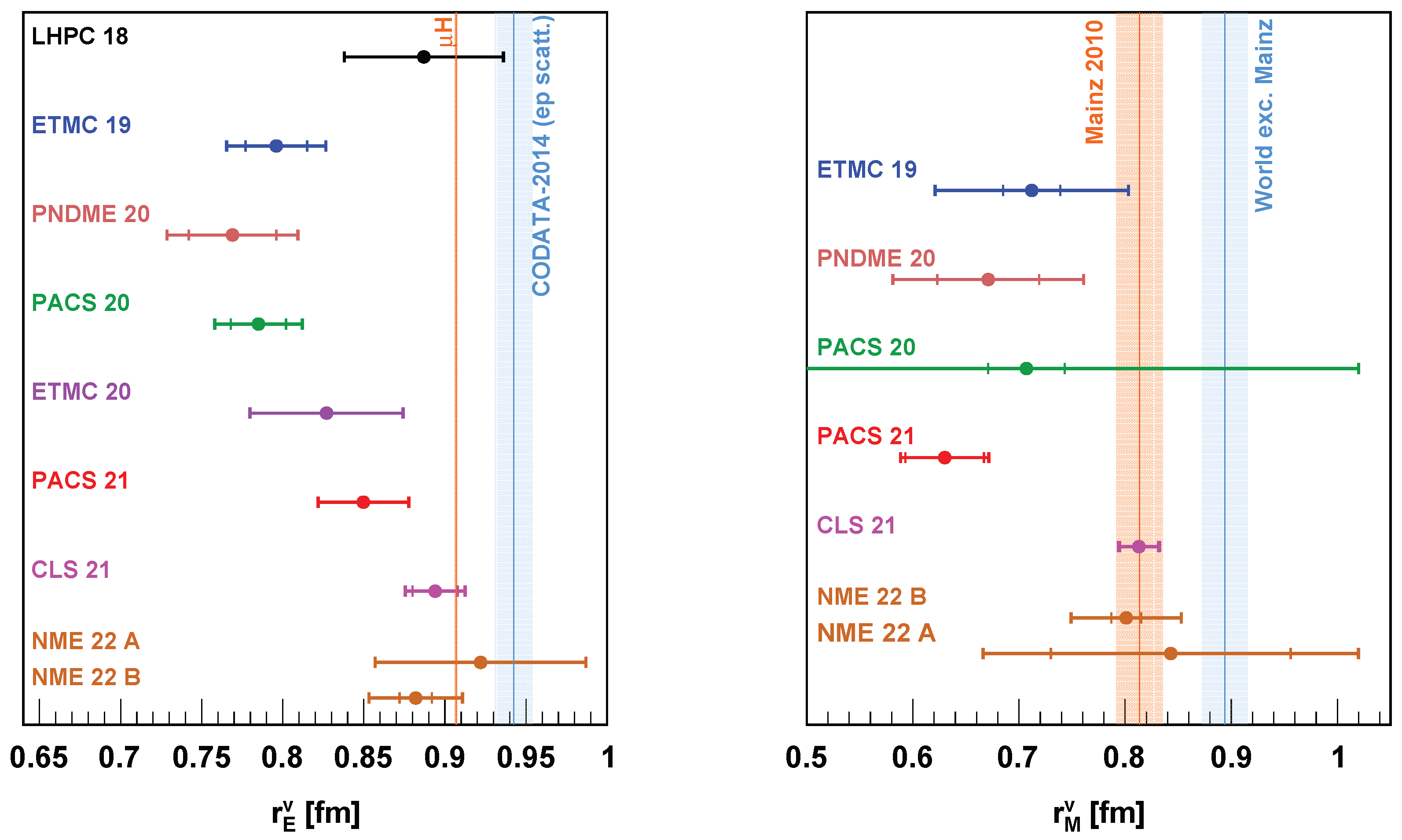
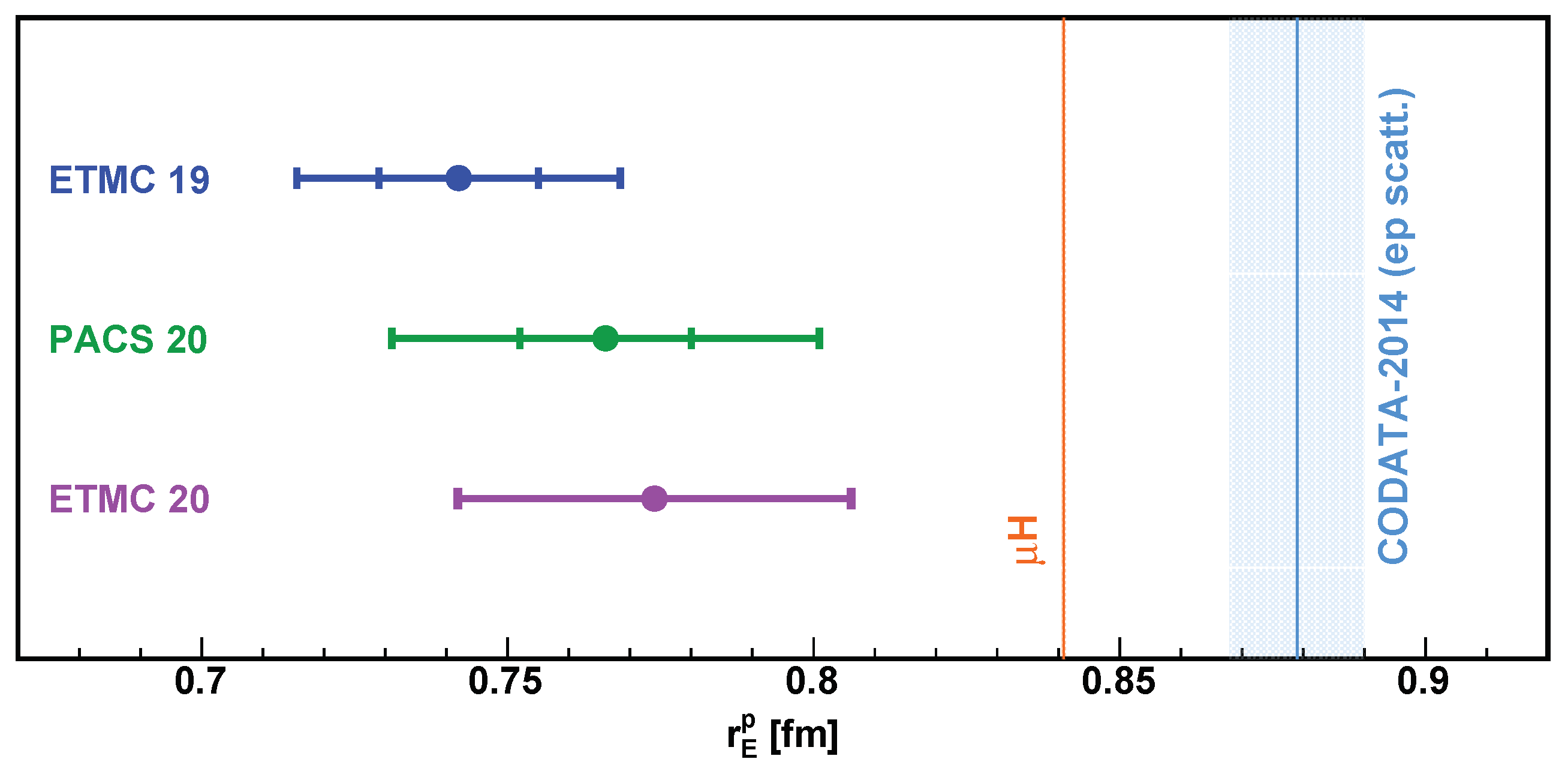
4.2. Progress from Lattice QCD
5. Remaining Issues in Lepton Scattering Experiments and Possible Explanation
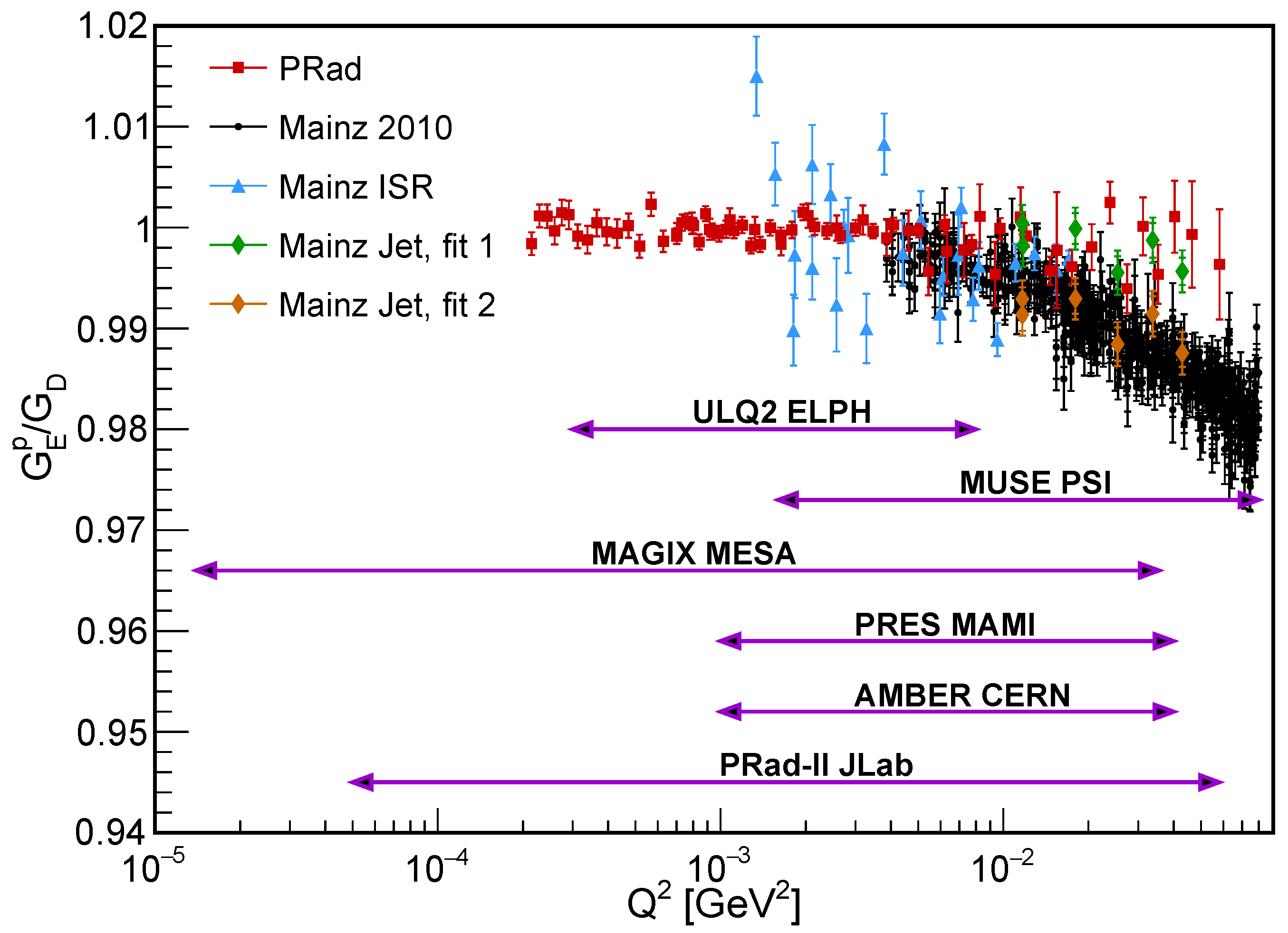
6. Future Lepton–Proton Scattering Experiments
6.1. MUSE Experiment
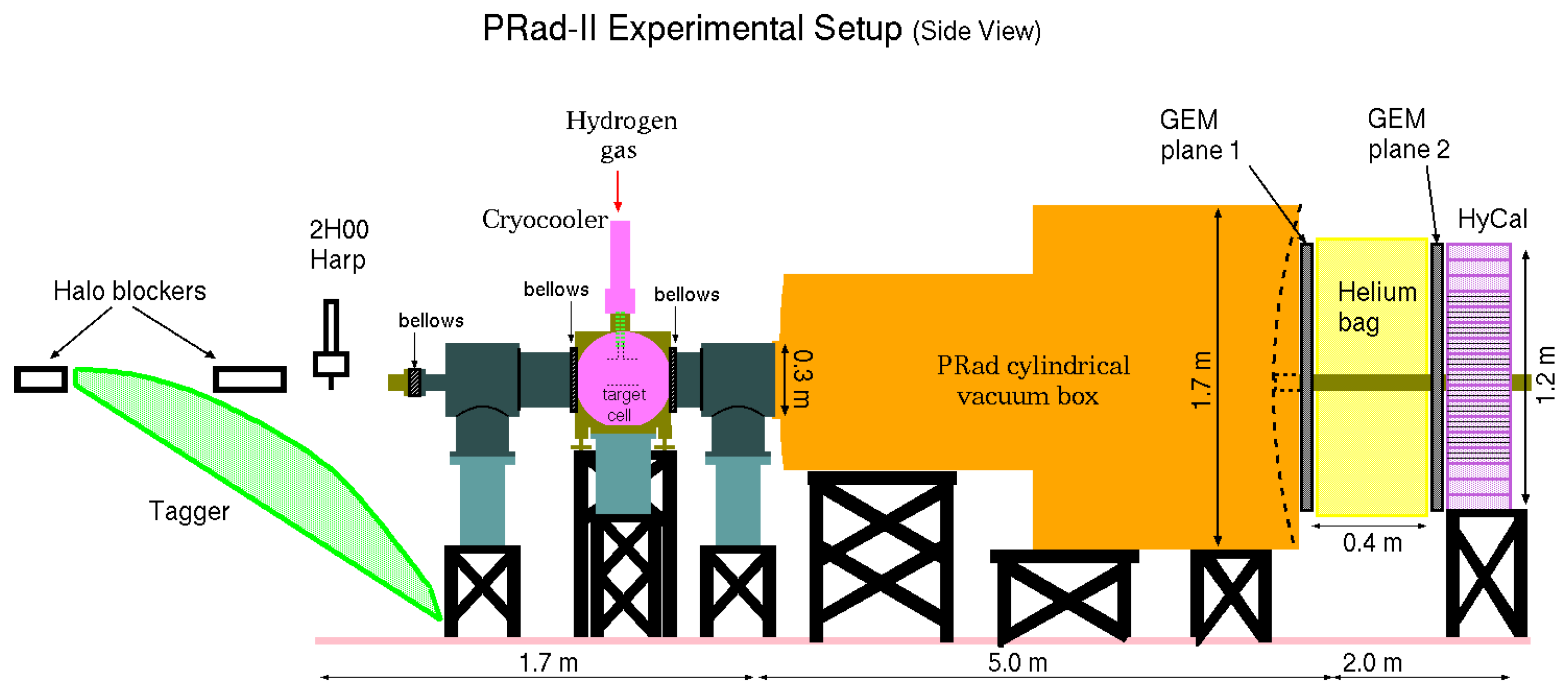
6.2. PRad-II Experiment
6.3. Compass++/AMBER Experiment
6.4. The PRES Experiment at Mainz
6.5. Mainz MAGIX Experiment
6.6. ULQ2 Experiment
7. Conclusions and Outlook
Author Contributions
Funding
Institutional Review Board Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
| 1 | For simplicity, the name “proton charge radius” is used for this quantity for the rest of the paper. |
| 2 | This figure is contained in the article that was published by B. S. Schlimme et al. [A1 and MAGIX], “Operation and characterization of a windowless gas jet target in high-intensity electron beams,” Nucl. Instrum. Meth. A 1013, 165668 (2021), Copyright Elsevier 2023. |
References
- Gao, H.; Vanderhaeghen, M. The proton charge radius. Rev. Mod. Phys. 2022, 94, 015002. [Google Scholar] [CrossRef]
- Bernauer, J.C.; Achenbach, P.; Gayoso, C.A.; Böhm, R.; Bosnar, D.; Debenjak, L.; Distler, M.O.; Doria, L.; Esser, A.; Fonvieille, H.; et al. High-precision determination of the electric and magnetic form factors of the proton. Phys. Rev. Lett. 2010, 105, 242001. [Google Scholar] [CrossRef] [PubMed]
- Xiong, W.; Gasparian, A.; Gao, H.; Dutta, D.; Khandaker, M.; Liyanage, N.; Pasyuk, E.; Peng, C.; Bai, X.; Ye, L.; et al. A small proton charge radius from an electron–proton scattering experiment. Nature 2019, 575, 147–150. [Google Scholar] [CrossRef] [PubMed]
- Mihovilovič, M.; Achenbach, P.; Beranek, T.; Beričič, J.; Bernauer, J.C.; Böhm, R.; Bosnar, D.; Cardinali, M.; Correa, L.; Debenjak, L.; et al. The proton charge radius extracted from the initial-state radiation experiment at MAMI. Eur. Phys. J. A 2021, 57, 107. [Google Scholar] [CrossRef]
- Puckett, A.J.R.; Brash, E.; Jones, M.; Luo, W.; Meziane, M.; Pentchev, L.; Perdrisat, C.; Punjabi, V.; Wesselmann, F.; Ahmidouch, A.; et al. Recoil Polarization Measurements of the Proton Electromagnetic Form Factor Ratio to Q2 = 8.5 GeV2. Phys. Rev. Lett. 2010, 104, 242301. [Google Scholar] [CrossRef]
- Zhan, X.; Allada, K.; Armstrong, D.S.; Arrington, J.; Bertozzi, W.; Boeglin, W.; Chen, J.P.; Chirapatpimol, K.; Choi, S.; Chudakov, E.; et al. High-Precision Measurement of the Proton Elastic Form Factor Ratio μpGE/GM at low Q2. Phys. Lett. B 2011, 705, 59–64. [Google Scholar] [CrossRef]
- Paolone, M. [E03-104 Collaboration] Polarization Transfer in the 4He(,e′)3H Reaction at Q2 = 0.8 and 1.3 (GeV/c)2. Phys. Rev. Lett. 2010, 105, 072001. [Google Scholar] [CrossRef]
- Crawford, C.B.; Sindile, A.; Akdogan, T.; Alarcon, R.; Bertozzi, W.; Booth, E.; Botto, T.; Calarco, J.; Clasie, B.; DeGrush, A.; et al. Measurement of the proton electric to magnetic form factor ratio from vector H-1(vector e, e’ p). Phys. Rev. Lett. 2007, 98, 052301. [Google Scholar] [CrossRef]
- Punjabi, V. [Jefferson Lab Hall A Collaboration] Proton elastic form-factor ratios to Q**2 = 3.5-GeV**2 by polarization transfer. Phys. Rev. C 2005, 71, 055202. [Google Scholar] [CrossRef]
- Lin, Y.H.; Hammer, H.W.; Meißner, U.G. New Insights into the Nucleon’s Electromagnetic Structure. Phys. Rev. Lett. 2022, 128, 052002. [Google Scholar] [CrossRef]
- Ablikim, M. [BESIII Collaboration] Measurement of proton electromagnetic form factors in the time-like region using initial state radiation at BESIII. Phys. Lett. B 2021, 817, 136328. [Google Scholar] [CrossRef]
- Beyer, A.; Maisenbacher, L.; Matveev, A.; Pohl, R.; Khabarova, K.; Grinin, A.; Lamour, T.; Yost, D.C.; Hänsch, T.W.; Kolachevsky, N.; et al. The Rydberg constant and proton size from atomic hydrogen. Science 2017, 358, 79–85. [Google Scholar] [CrossRef] [PubMed]
- Fleurbaey, H.; Galtier, S.; Thomas, S.; Bonnaud, M.; Julien, L.; Biraben, F.; Nez, F.; Abgrall, M.; Guéna, J. New Measurement of the 1S–3S Transition Frequency of Hydrogen: Contribution to the Proton Charge Radius Puzzle. Phys. Rev. Lett. 2018, 120, 183001. [Google Scholar] [CrossRef] [PubMed]
- Bezginov, N.; Valdez, T.; Horbatsch, M.; Marsman, A.; Vutha, A.C.; Hessels, E.A. A measurement of the atomic hydrogen Lamb shift and the proton charge radius. Science 2019, 365, 1007–1012. [Google Scholar] [CrossRef]
- Grinin, A.; Matveev, A.; Yost, D.C.; Maisenbacher, L.; Wirthl, V.; Pohl, R.; Hänsch, T.W.; Udem, T. Two-photon frequency comb spectroscopy of atomic hydrogen. Science 2020, 370, 1061–1066. [Google Scholar] [CrossRef]
- Brandt, A.D.; Cooper, S.F.; Rasor, C.; Burkley, Z.; Yost, D.C.; Matveev, A. Measurement of the 2S1/2-8D5/2 Transition in Hydrogen. Phys. Rev. Lett. 2022, 128, 023001. [Google Scholar] [CrossRef]
- Karr, J.P.; Marchand, D.; Voutier, E. The proton size. Nat. Rev. Phys. 2020, 2, 601–614. [Google Scholar] [CrossRef]
- Mohr, P.J.; Taylor, B.N.; Newell, D.B. CODATA Recommended Values of the Fundamental Physical Constants: 2010. Rev. Mod. Phys. 2012, 84, 1527–1605. [Google Scholar] [CrossRef]
- Pohl, R.; Antognini, A.; Nez, F.; Amaro, F.D.; Biraben, F.; Cardoso, J.M.R.; Covita, D.S.; Dax, A.; Dhawan, S.; Fernandes, L.M.P.; et al. The size of the proton. Nature 2010, 466, 213–216. [Google Scholar] [CrossRef]
- Antognini, A.; Nez, F.; Schuhmann, K.; Amaro, F.D.; Biraben, F.; Cardoso, J.M.R.; Covita, D.S.; Dax, A.; Dhawan, S.; Diepold, M.; et al. Proton Structure from the Measurement of 2S–2P Transition Frequencies of Muonic Hydrogen. Science 2013, 339, 417–420. [Google Scholar] [CrossRef]
- Carlson, C.E.; Vanderhaeghen, M. Higher order proton structure corrections to the Lamb shift in muonic hydrogen. Phys. Rev. A 2011, 84, 020102. [Google Scholar] [CrossRef]
- Gorchtein, M.; Llanes-Estrada, F.J.; Szczepaniak, A.P. Muonic-hydrogen Lamb shift: Dispersing the nucleon-excitation uncertainty with a finite-energy sum rule. Phys. Rev. A 2013, 87, 052501. [Google Scholar] [CrossRef]
- Tomalak, O. Two-Photon Exchange Correction to the Lamb Shift and Hyperfine Splitting of S Levels. Eur. Phys. J. A 2019, 55, 64. [Google Scholar] [CrossRef]
- Birse, M.C.; McGovern, J.A. Proton polarisability contribution to the Lamb shift in muonic hydrogen at fourth order in chiral perturbation theory. Eur. Phys. J. A 2012, 48, 120. [Google Scholar] [CrossRef]
- Peset, C.; Pineda, A. The Lamb shift in muonic hydrogen and the proton radius from effective field theories. Eur. Phys. J. A 2015, 51, 156. [Google Scholar] [CrossRef]
- Hill, R.J.; Paz, G. Nucleon spin-averaged forward virtual Compton tensor at large Q2. Phys. Rev. D 2017, 95, 094017. [Google Scholar] [CrossRef]
- Miller, G.A. Proton Polarizability Contribution: Muonic Hydrogen Lamb Shift and Elastic Scattering. Phys. Lett. B 2013, 718, 1078–1082. [Google Scholar] [CrossRef]
- Fu, Y.; Feng, X.; Jin, L.C.; Lu, C.F. Lattice QCD Calculation of the Two-Photon Exchange Contribution to the Muonic-Hydrogen Lamb Shift. Phys. Rev. Lett. 2022, 128, 172002. [Google Scholar] [CrossRef]
- Carlson, C.E. The Proton Radius Puzzle. Prog. Part. Nucl. Phys. 2015, 82, 59–77. [Google Scholar] [CrossRef]
- Liu, Y.S.; Miller, G.A. Validity of the Weizsäcker-Williams approximation and the analysis of beam dump experiments: Production of an axion, a dark photon, or a new axial-vector boson. Phys. Rev. D 2017, 96, 016004. [Google Scholar] [CrossRef]
- Liu, Y.S.; Cloët, I.C.; Miller, G.A. Eta Decay and Muonic Puzzles. Nucl. Phys. B 2019, 944, 114638. [Google Scholar] [CrossRef]
- Bordes, J.; Chan, H.M.; Tsou, S.T. Accommodating three low-scale anomalies (g-2, Lamb shift, and Atomki) in the framed standard model. Int. J. Mod. Phys. A 2019, 34, 1950140. [Google Scholar] [CrossRef]
- Pauk, V.; Vanderhaeghen, M. Lepton universality test in the photoproduction of e−e+ versus μ−μ+ pairs on a proton target. Phys. Rev. Lett. 2015, 115, 221804. [Google Scholar] [CrossRef] [PubMed]
- Carlson, C.E.; Pauk, V.; Vanderhaeghen, M. Dilepton photoproduction on a deuteron target. Phys. Lett. B 2019, 797, 134872. [Google Scholar] [CrossRef]
- Heller, M.; Tomalak, O.; Vanderhaeghen, M. Soft-photon corrections to the Bethe-Heitler process in the γp→l+l−p reaction. Phys. Rev. D 2018, 97, 076012. [Google Scholar] [CrossRef]
- Heller, M.; Tomalak, O.; Vanderhaeghen, M.; Wu, S. Leading Order Corrections to the Bethe-Heitler Process in the γp→l+l−p Reaction. Phys. Rev. D 2019, 100, 076013. [Google Scholar] [CrossRef]
- Peset, C.; Pineda, A.; Tomalak, O. The proton radius (puzzle?) and its relatives. Prog. Part. Nucl. Phys. 2021, 121, 103901. [Google Scholar] [CrossRef]
- Lee, G.; Arrington, J.R.; Hill, R.J. Extraction of the proton radius from electron-proton scattering data. Phys. Rev. D 2015, 92, 013013. [Google Scholar] [CrossRef]
- Higinbotham, D.W.; Kabir, A.A.; Lin, V.; Meekins, D.; Norum, B.; Sawatzky, B. Proton radius from electron scattering data. Phys. Rev. C 2016, 93, 055207. [Google Scholar] [CrossRef]
- Griffioen, K.; Carlson, C.; Maddox, S. Consistency of electron scattering data with a small proton radius. Phys. Rev. C 2016, 93, 065207. [Google Scholar] [CrossRef]
- Bernauer, J.C.; Distler, M.O. Avoiding common pitfalls and misconceptions in extractions of the proton radius. arXiv 2016, arXiv:1606.02159. [Google Scholar]
- Yan, X.; Higinbotham, D.W.; Dutta, D.; Gao, H.; Gasparian, A.; Khandaker, M.A.; Liyanage, N.; Pasyuk, E.; Peng, C.; Xiong, W. Robust extraction of the proton charge radius from electron-proton scattering data. Phys. Rev. C 2018, 98, 025204. [Google Scholar] [CrossRef]
- Kraus, E.; Mesick, K.E.; White, A.; Gilman, R.; Strauch, S. Polynomial fits and the proton radius puzzle. Phys. Rev. C 2014, 90, 045206. [Google Scholar] [CrossRef]
- Alarcón, J.M.; Higinbotham, D.W.; Weiss, C. Precise determination of the proton magnetic radius from electron scattering data. Phys. Rev. C 2020, 102, 035203. [Google Scholar] [CrossRef]
- Cui, Z.F.; Binosi, D.; Roberts, C.D.; Schmidt, S.M. Fresh Extraction of the Proton Charge Radius from Electron Scattering. Phys. Rev. Lett. 2021, 127, 092001. [Google Scholar] [CrossRef]
- Hasan, N.; Green, J.; Meinel, S.; Engelhardt, M.; Krieg, S.; Negele, J.; Pochinsky, A.; Syritsyn, S. Computing the nucleon charge and axial radii directly at Q2=0 in lattice QCD. Phys. Rev. D 2018, 97, 034504. [Google Scholar] [CrossRef]
- Alexandrou, C.; Bacchio, S.; Constantinou, M.; Finkenrath, J.; Hadjiyiannakou, K.; Jansen, K.; Koutsou, G.; Aviles-Casco, A.V. Proton and neutron electromagnetic form factors from lattice QCD. Phys. Rev. D 2019, 100, 014509. [Google Scholar] [CrossRef]
- Jang, Y.C.; Gupta, R.; Lin, H.W.; Yoon, B.; Bhattacharya, T. Nucleon electromagnetic form factors in the continuum limit from (2 + 1 + 1)-flavor lattice QCD. Phys. Rev. D 2020, 101, 014507. [Google Scholar] [CrossRef]
- Shintani, E.; Ishikawa, K.I.; Kuramashi, Y.; Sasaki, S.; Yamazaki, T. Nucleon form factors and root-mean-square radii on a (10.8 fm)4 lattice at the physical point. Phys. Rev. D 2019, 99, 014510. [Google Scholar] [CrossRef]
- Alexandrou, C.; Hadjiyiannakou, K.; Koutsou, G.; Ottnad, K.; Petschlies, M. Model-independent determination of the nucleon charge radius from lattice QCD. Phys. Rev. D 2020, 101, 114504. [Google Scholar] [CrossRef]
- Ishikawa, K.-I.; Kuramashi, Y.; Sasaki, S.; Shintani, E.; Yamazaki, T. Calculation of the derivative of nucleon form factors in Nf=2+1 lattice QCD at Mπ=138 MeV on a (5.5 fm)3 volume. Phys. Rev. D 2021, 104, 074514. [Google Scholar] [CrossRef]
- Park, S.; Gupta, R.; Yoon, B.; Mondal, S.; Bhattacharya, T.; Jang, Y.-C.; Joó, B.; Winter, F. Precision nucleon charges and form factors using (2+1)-flavor lattice QCD. Phys. Rev. D 2022, 105, 054505. [Google Scholar] [CrossRef]
- Djukanovic, D.; Harris, T.; von Hippel, G.; Junnarkar, P.M.; Meyer, H.B.; Mohler, D.; Ottnad, K.; Schulz, T.; Wilhelm, J.; Wittig, H. Isovector electromagnetic form factors of the nucleon from lattice QCD and the proton radius puzzle. Phys. Rev. D 2021, 103, 094522. [Google Scholar] [CrossRef]
- Antognini, A.; Hagelstein, F.; Pascalutsa, V. The proton structure in and out of muonic hydrogen. Ann. Rev. Nucl. Part. Sci. 2022, 72, 389. [Google Scholar] [CrossRef]
- Pachucki, K.; Lensky, V.; Hagelstein, F.; Muli, S.S.L.; Bacca, S.; Pohl, R. Comprehensive theory of the Lamb shift in μH, μD, μ3He+, and μ4He+. arXiv 2022, arXiv:2212.13782. [Google Scholar]
- Wang, Y. [A1 and MAGIX Collaborations] Low-Q2 elastic electron-proton scattering using a gas jet target. Phys. Rev. C 2022, 106, 044610. [Google Scholar] [CrossRef]
- Tiesinga, E.; Mohr, P.J.; Newell, D.B.; Taylor, B.N. CODATA recommended values of the fundamental physical constants: 2018. Rev. Mod. Phys. 2021, 93, 025010. [Google Scholar] [CrossRef]
- Gramolin, A.V.; Fadin, V.S.; Feldman, A.L.; Gerasimov, R.E.; Nikolenko, D.M.; Rachek, I.A.; Toporkov, D.K. A new event generator for the elastic scattering of charged leptons on protons. J. Phys. G 2014, 41, 115001. [Google Scholar] [CrossRef]
- Tomalak, O.; Vanderhaeghen, M. Two-photon exchange corrections in elastic muon-proton scattering. Phys. Rev. D 2014, 90, 013006. [Google Scholar] [CrossRef]
- Tomalak, O.; Vanderhaeghen, M. Dispersion relation formalism for the two-photon exchange correction to elastic muon–proton scattering: Elastic intermediate state. Eur. Phys. J. C 2018, 78, 514. [Google Scholar] [CrossRef]
- Ernst, F.J.; Sachs, R.G.; Wali, K.C. Electromagnetic form factors of the nucleon. Phys. Rev. 1960, 119, 1105–1114. [Google Scholar] [CrossRef]
- Sachs, R.G. High-Energy Behavior of Nucleon Electromagnetic Form Factors. Phys. Rev. 1962, 126, 2256–2260. [Google Scholar] [CrossRef]
- Miller, G.A. Defining the proton radius: A unified treatment. Phys. Rev. C 2019, 99, 035202. [Google Scholar] [CrossRef]
- Jaffe, R.L. Ambiguities in the definition of local spatial densities in light hadrons. Phys. Rev. D 2021, 103, 016017. [Google Scholar] [CrossRef]
- Rinehimer, J.A.; Miller, G.A. Connecting the Breit Frame to the Infinite Momentum Light Front Frame: How G(E) turns into F(1). Phys. Rev. C 2009, 80, 015201. [Google Scholar] [CrossRef]
- Atac, H.; Constantinou, M.; Meziani, Z.E.; Paolone, M.; Sparveris, N. Charge radii of the nucleon from its flavor dependent Dirac form factors. Eur. Phys. J. A 2021, 57, 65. [Google Scholar] [CrossRef]
- Gramolin, A.V.; Russell, R.L. Transverse charge density and the radius of the proton. Phys. Rev. D 2022, 105, 054004. [Google Scholar] [CrossRef]
- Freese, A.; Miller, G.A. Light front synchronization and rest frame densities of the proton: I. Electromagnetic densities. arXiv 2023, arXiv:2302.09171. [Google Scholar]
- Lorcé, C. Charge Distributions of Moving Nucleons. Phys. Rev. Lett. 2020, 125, 232002. [Google Scholar] [CrossRef]
- Chen, Y.; Lorcé, C. Pion and nucleon relativistic electromagnetic four-current distributions. Phys. Rev. D 2022, 106, 116024. [Google Scholar] [CrossRef]
- Chen, Y.; Lorcé, C. Nucleon relativistic polarization and magnetization distributions. arXiv 2023, arXiv:2302.04672. [Google Scholar]
- Epelbaum, E.; Gegelia, J.; Lange, N.; Meißner, U.G.; Polyakov, M.V. Definition of Local Spatial Densities in Hadrons. Phys. Rev. Lett. 2022, 129, 012001. [Google Scholar] [CrossRef] [PubMed]
- Li, Y.; Dong, W.; Yin, Y.; Wang, Q.; Vary, J.P. Minkowski’s lost legacy and hadron electromagnetism. Phys. Lett. B 2013, 838, 137676. [Google Scholar] [CrossRef]
- Mo, L.W.; Tsai, Y.S. Radiative Corrections to Elastic and Inelastic e p and mu p Scattering. Rev. Mod. Phys. 1969, 41, 205–235. [Google Scholar] [CrossRef]
- Pierce, J.; Brock, J.; Carlin, C.; Keith, C.; Maxwell, J.; Meekins, D.; Bai, X.; Deur, A.; Dutta, D.; Gao, H.; et al. The PRad Windowless Gas Flow Target. Nucl. Instrum. Meth. A 2021, 1003, 165300. [Google Scholar] [CrossRef]
- Bai, X. Jefferson Lab High Precision Proton Radius Measurement Experiment: PRad. Ph.D. Thesis, University of Virginia, Charlottesville, VA, USA, 2020. [Google Scholar] [CrossRef]
- Agostinelli, S.; Allison, J.; Amako, K.; Apostolakis, J.; Araujo, H.; Arce, P.; Asai, M.; Axen, D.; Banerjee, S.; Barrand, G.; et al. GEANT4–a simulation toolkit. Nucl. Instrum. Meth. A 2003, 506, 250–303. [Google Scholar] [CrossRef]
- Akushevich, I.; Gao, H.; Ilyichev, A.; Meziane, M. Radiative corrections beyond the ultra relativistic limit in unpolarized ep elastic and Møller scatterings for the PRad Experiment at Jefferson Laboratory. Eur. Phys. J. A 2015, 51, 1. [Google Scholar] [CrossRef]
- Kelly, J.J. Simple parametrization of nucleon form factors. Phys. Rev. C 2004, 70, 068202. [Google Scholar] [CrossRef]
- Schlimme, B.; Aulenbacher, S.; Brand, P.; Littich, M.; Wang, Y.; Achenbach, P.; Ball, M.; Bernauer, J.; Biroth, M.; Bonaventura, D.; et al. Operation and characterization of a windowless gas jet target in high-intensity electron beams. Nucl. Instrum. Meth. A 2021, 1013, 165668. [Google Scholar] [CrossRef]
- Schmidt, A. Measuring the lepton sign asymmetry in elastic electron-proton scattering with OLYMPUS. arXiv 2017, arXiv:1711.09894. [Google Scholar]
- Meißner, U.G. The proton radius and its relatives—Much ado about nothing? arXiv 2022, arXiv:2211.05419. [Google Scholar]
- Hayward, T.B.; Griffioen, K.A. Evaluation of low-Q2 fits to ep and ed elastic scattering data. Nucl. Phys. A 2020, 999, 121767. [Google Scholar] [CrossRef]
- Borah, K.; Hill, R.J.; Lee, G.; Tomalak, O. Parametrization and applications of the low-Q2 nucleon vector form factors. Phys. Rev. D 2020, 102, 074012. [Google Scholar] [CrossRef]
- Paz, G. Model-independent extraction of the proton charge radius from PRad data. Mod. Phys. Lett. A 2021, 36, 2150143. [Google Scholar] [CrossRef]
- Lin, Y.H.; Hammer, H.W.; Meißner, U.G. Dispersion-theoretical analysis of the electromagnetic form factors of the nucleon: Past, present and future. Eur. Phys. J. A 2021, 57, 255. [Google Scholar] [CrossRef]
- Boone, B.; Chen, M.; Sturm, K.; Yoo, J.; Higinbotham, D. Comment on Transverse Charge Density and the Radius of the Proton. arXiv 2023, arXiv:2302.07356. [Google Scholar]
- Bernauer, J.C.; Distler, M.O.; Friedrich, J.; Walcher, T.; Achenbach, P.; Gayoso, C.A.; Böhm, R.; Bosnar, D.; Debenjak, L.; Doria, L.; et al. Electric and magnetic form factors of the proton. Phys. Rev. C 2014, 90, 015206. [Google Scholar] [CrossRef]
- Alarcón, J.M.; Higinbotham, D.W.; Weiss, C.; Ye, Z. Proton charge radius extraction from electron scattering data using dispersively improved chiral effective field theory. Phys. Rev. C 2019, 99, 044303. [Google Scholar] [CrossRef]
- Alarcón, J.M.; Weiss, C. Accurate nucleon electromagnetic form factors from dispersively improved chiral effective field theory. Phys. Lett. B 2018, 784, 373–377. [Google Scholar] [CrossRef]
- Alarcón, J.M.; Weiss, C. Nucleon form factors in dispersively improved Chiral Effective Field Theory II: Electromagnetic form factors. Phys. Rev. C 2018, 97, 055203. [Google Scholar] [CrossRef]
- Horbatsch, M. Properties of the Sachs electric form factor of the proton on the basis of recent e−p scattering experiments and hydrogen spectroscopy. Phys. Lett. B 2020, 804, 135373. [Google Scholar] [CrossRef]
- Lin, Y.H.; Hammer, H.W.; Meißner, U.G. High-precision determination of the electric and magnetic radius of the proton. Phys. Lett. B 2021, 816, 136254. [Google Scholar] [CrossRef]
- Hohler, G.; Pietarinen, E.; Stefanescu, I.S.; Borkowski, F.; Simon, G.G.; Walther, V.H.; Wendling, R.D. Analysis of Electromagnetic Nucleon Form-Factors. Nucl. Phys. B 1976, 114, 505–534. [Google Scholar] [CrossRef]
- Mergell, P.; Meissner, U.G.; Drechsel, D. Dispersion theoretical analysis of the nucleon electromagnetic form-factors. Nucl. Phys. A 1996, 596, 367–396. [Google Scholar] [CrossRef]
- Hammer, H.W.; Meissner, U.G. Updated dispersion theoretical analysis of the nucleon electromagnetic form-factors. Eur. Phys. J. A 2004, 20, 469–473. [Google Scholar] [CrossRef]
- Belushkin, M.A.; Hammer, H.W.; Meissner, U.G. Dispersion analysis of the nucleon form-factors including meson continua. Phys. Rev. C 2007, 75, 035202. [Google Scholar] [CrossRef]
- Lorenz, I.T.; Hammer, H.W.; Meissner, U.G. The size of the proton - closing in on the radius puzzle. Eur. Phys. J. A 2012, 48, 151. [Google Scholar] [CrossRef]
- Lorenz, I.T.; Meißner, U.G.; Hammer, H.W.; Dong, Y.B. Theoretical Constraints and Systematic Effects in the Determination of the Proton Form Factors. Phys. Rev. D 2015, 91, 014023. [Google Scholar] [CrossRef]
- Pacetti, S.; Ferroli, R.B.; Tomasi-Gustafsson, E. Proton electromagnetic form factors: Basic notions, present achievements and future perspectives. Phys. Rep. 2015, 550–551, 1–103. [Google Scholar] [CrossRef]
- Barcus, S.K.; Higinbotham, D.W.; McClellan, R.E. How Analytic Choices Can Affect the Extraction of Electromagnetic Form Factors from Elastic Electron Scattering Cross Section Data. Phys. Rev. C 2020, 102, 015205. [Google Scholar] [CrossRef]
- Zhou, J.; Khachatryan, V.; Gao, H.; Gorbaty, S.; Higinbotham, D.W. Understanding the systematic differences in extractions of the proton electric form factors at low Q2. Phys. Rev. C 2022, 106, 065505. [Google Scholar] [CrossRef]
- Cui, Z.F.; Binosi, D.; Roberts, C.D.; Schmidt, S.M. Hadron and light nucleus radii from electron scattering. Chin. Phys. C 2022, 46, 122001. [Google Scholar] [CrossRef]
- Cui, Z.F.; Binosi, D.; Roberts, C.D.; Schmidt, S.M. Pauli Radius of the Proton. Chin. Phys. Lett. 2021, 38, 121401. [Google Scholar] [CrossRef]
- Ma, Y.Q.; Qiu, J.W. Extracting Parton Distribution Functions from Lattice QCD Calculations. Phys. Rev. D 2018, 98, 074021. [Google Scholar] [CrossRef]
- Musch, B.U.; Hagler, P.; Negele, J.W.; Schafer, A. Exploring quark transverse momentum distributions with lattice QCD. Phys. Rev. D 2011, 83, 094507. [Google Scholar] [CrossRef]
- Bhattacharya, T.; Cirigliano, V.; Cohen, S.; Gupta, R.; Lin, H.W.; Yoon, B. Axial, Scalar and Tensor Charges of the Nucleon from 2+1+1-flavor Lattice QCD. Phys. Rev. D 2016, 94, 054508. [Google Scholar] [CrossRef]
- Djukanovic, D. Recent progress on nucleon form factors. PoS 2022, 396, 9. [Google Scholar] [CrossRef]
- Mohr, P.J.; Newell, D.B.; Taylor, B.N. CODATA Recommended Values of the Fundamental Physical Constants: 2014. Rev. Mod. Phys. 2016, 88, 035009. [Google Scholar] [CrossRef]
- Zyla, P.A.; Barnett, R.M.; Beringer, J.; Dahl, O.; Dwyer, D.A.; Groom, D.E.; Lin, C.J.; Lugovsky, K.S.; Pianori, E.; Robinson, D.J.; et al. Review of Particle Physics. Prog. Theor. Exp. Phys. 2020, 2020, 083C01. [Google Scholar] [CrossRef]
- Workman, R.L.; Burkert, V.D.; Crede, V.; Klempt, E.; Thoma, U.; Tiator, L.; Agashe, K.; Aielli, G.; Allanach, B.C.; Amsler, C.; et al. Review of Particle Physics. Prog. Theor. Exp. Phys. 2022, 2022, 083C01. [Google Scholar] [CrossRef]
- Maximon, L.C.; Tjon, J.A. Radiative corrections to electron proton scattering. Phys. Rev. C 2000, 62, 054320. [Google Scholar] [CrossRef]
- Vanderhaeghen, M.; Friedrich, J.M.; Lhuillier, D.; Marchand, D.; Van Hoorebeke, L.; Van de Wiele, J. QED radiative corrections to virtual Compton scattering. Phys. Rev. C 2000, 62, 025501. [Google Scholar] [CrossRef]
- Xiong, W. A High Precision Measurement of the Proton Charge Radius at JLab. JLAB-PHY-20-3266. Ph.D. Dissertation, Duke University, Durham, NC, USA, 2020. [Google Scholar]
- Afanasev, A.; Ahmed, J.; Akushevich, I.; Bernauer, J.C.; Blunden, P.G.; Bressan, A.; Byer, D.; Cline, E.; Diefenthaler, M.; Friedrich, J.M.; et al. CFNS Ad-Hoc meeting on Radiative Corrections Whitepaper. arXiv 2020, arXiv:2012.09970. [Google Scholar]
- Tomalak, O. Two-photon exchange correction in elastic lepton-proton scattering. Few Body Syst. 2018, 59, 87. [Google Scholar] [CrossRef]
- Tomalak, O.; Vanderhaeghen, M. Two-photon exchange correction in elastic unpolarized electron-proton scattering at small momentum transfer. Phys. Rev. D 2016, 93, 013023. [Google Scholar] [CrossRef]
- Tomalak, O.; Vanderhaeghen, M. Subtracted dispersion relation formalism for the two-photon exchange correction to elastic electron-proton scattering: Comparison with data. Eur. Phys. J. A 2015, 51, 24. [Google Scholar] [CrossRef]
- Tomalak, O.; Pasquini, B.; Vanderhaeghen, M. Two-photon exchange contribution to elastic e−-proton scattering: Full dispersive treatment of πN states and comparison with data. Phys. Rev. D 2017, 96, 096001. [Google Scholar] [CrossRef]
- Kohl, M. Lepton Universality Test with MUSE at PSI. J. Phys. Conf. Ser. 2022, 2391, 012015. [Google Scholar] [CrossRef]
- Cline, E.; Bernauer, J.; Downie, E.J.; Gilman, R. MUSE: The MUon Scattering Experiment. SciPost Phys. Proc. 2021, 5, 23. [Google Scholar] [CrossRef]
- Gilman, R.; Downie, E.J.; Ron, G.; Strauch, S.; Afanasev, A.; Akmal, A.; Arrington, J.; Atac, H.; Ayerbe-Gayoso, C.; Benmokhtar, F.; et al. Technical Design Report for the Paul Scherrer Institute Experiment R-12-01.1: Studying the Proton ”Radius” Puzzle with μp Elastic Scattering. arXiv 2017, arXiv:1709.09753. [Google Scholar]
- Gasparian, A.; Gao, H.; Dutta, D.; Liyanage, N.; Pasyuk, E.; Higinbotham, D.W.; Peng, C.; Gnanvo, K.; Xiong, W.; Bai, X. PRad-II: A New Upgraded High Precision Measurement of the Proton Charge Radius. arXiv 2020, arXiv:2009.10510. [Google Scholar]
- Quintans, C. The New AMBER Experiment at the CERN SPS. Few Body Syst. 2022, 63, 72. [Google Scholar] [CrossRef]
- Dreisbach, C.; Friedrich, J.; Hoffmann, M.; Inglessi, A.; Kabuß, E.; Ketzer, B.; Kiselev, O.; Maev, E.; Paul, S.; Petrov, G.; et al. Measuring the Proton Radius in High-Energy Muon-Proton Scattering. PoS 2019, DIS2019, 222. [Google Scholar] [CrossRef]
- Belostotski, S.; Sagidova, N.; Vorobyev, A. Proton radius reconstruction from simulated electron-proton elastic scattering cross sections at low transfer momenta. arXiv 2019, arXiv:1903.04975. [Google Scholar]
- Vorobyev, A. Precision measurement of the proton charge radius in electron proton scattering. Phys. Part. Nucl. Lett. 2019, 16, 524–529. [Google Scholar] [CrossRef]
- Suda, T. Low-energy electron scattering facilities in Japan. J. Phys. Conf. Ser. 2022, 2391, 012004. [Google Scholar] [CrossRef]
- Suda, T.; Aoyagi, T.; Honda, Y.; Maeda, Y.; Miura, S.; Muto, T.; Namba, K.; Nambu, K.; Takahashi, K.; Tamae, T.; et al. Measurement of Proton Charge Radius by Low-Energy Electron Scattering. J. Part. Accel. Soc. Jpn. 2018, 15, 52–59. [Google Scholar]
- Ahmed, J.; Blunden, P.G.; Melnitchouk, W. Two-photon exchange from intermediate state resonances in elastic electron-proton scattering. Phys. Rev. C 2020, 102, 045205. [Google Scholar] [CrossRef]
- Tomalak, O. Forward two-photon exchange in elastic lepton–proton scattering and hyperfine-splitting correction. Eur. Phys. J. C 2017, 77, 517. [Google Scholar] [CrossRef]
- Tomalak, O.; Pasquini, B.; Vanderhaeghen, M. Two-photon exchange corrections to elastic e−-proton scattering: Full dispersive treatment of πN states at low momentum transfers. Phys. Rev. D 2017, 95, 096001. [Google Scholar] [CrossRef]
- Tomalak, O.; Vanderhaeghen, M. Two-photon exchange correction to muon–proton elastic scattering at low momentum transfer. Eur. Phys. J. C 2016, 76, 125. [Google Scholar] [CrossRef]
- Cline, E.; Lin, W.; Roy, P.; Reimer, P.E.; Mesick, K.E.; Akmal, A.; Alie, A.; Atac, H.; Atencio, A.; Gayoso, C.A.; et al. Characterization of muon and electron beams in the Paul Scherrer Institute PiM1 channel for the MUSE experiment. Phys. Rev. C 2022, 105, 055201. [Google Scholar] [CrossRef]
- Vorobyev, A.; Sagidova, N. Method for precision measurement of the Range—Energy relation for protons in pure hydrogen gas. arXiv 2019, arXiv:1912.06065. [Google Scholar]
- Hug, F.; Aulenbacher, K.; Friederich, S.; Heil, P.; Heine, R.; Kempf, R.; Matejcek, C.; Simon, D. Status of the MESA ERL Project. In Proceedings of the 63rd ICFA Advanced Beam Dynamics Workshop on Energy Recovery Linacs, Berlin, Germany, 15–20 September 2019. [Google Scholar] [CrossRef]
- Caiazza, S.S.; Achenbach, P.; Aulenbacher, S.; Biroth, M.; Christmann, M.; Denig, A.; Doria, L.; Geimer, J.; Gülker, P.; Littich, M.; et al. The MAGIX focal plane time projection chamber. J. Phys. Conf. Ser. 2020, 1498, 012022. [Google Scholar] [CrossRef]
- Bernauer, J.C. The proton radius puzzle—9 years later. EPJ Web Conf. 2020, 234, 01001. [Google Scholar] [CrossRef]




Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Xiong, W.; Peng, C. Proton Electric Charge Radius from Lepton Scattering. Universe 2023, 9, 182. https://doi.org/10.3390/universe9040182
Xiong W, Peng C. Proton Electric Charge Radius from Lepton Scattering. Universe. 2023; 9(4):182. https://doi.org/10.3390/universe9040182
Chicago/Turabian StyleXiong, Weizhi, and Chao Peng. 2023. "Proton Electric Charge Radius from Lepton Scattering" Universe 9, no. 4: 182. https://doi.org/10.3390/universe9040182
APA StyleXiong, W., & Peng, C. (2023). Proton Electric Charge Radius from Lepton Scattering. Universe, 9(4), 182. https://doi.org/10.3390/universe9040182




