Mission Target: Exotic Multiquark Hadrons—Sharpened Blades
Abstract
1. Significance of Fundamental Diverseness of Ordinary Hadrons and Multiquark States
- Conventional (ordinary) hadrons include all mesons that consist of only a pair of quark and antiquark, as well as all baryons that consist of three quarks or of three antiquarks.
- Exotic hadrons are characterized by non-conventional quark and/or gluon compositions comprising multiquark states (tetraquarks, pentaquarks, hexaquarks, heptaquarks, etc.), “hybrid” quark–gluon bound states, or pure-gluon bound states (nick) named glueballs.
2. Tetraquark Mesons—The Example of Multiquark Exotic Hadron States Par Excellence
3. Correlation Functions of Hadron Interpolating Operators: Application to Multiquarks
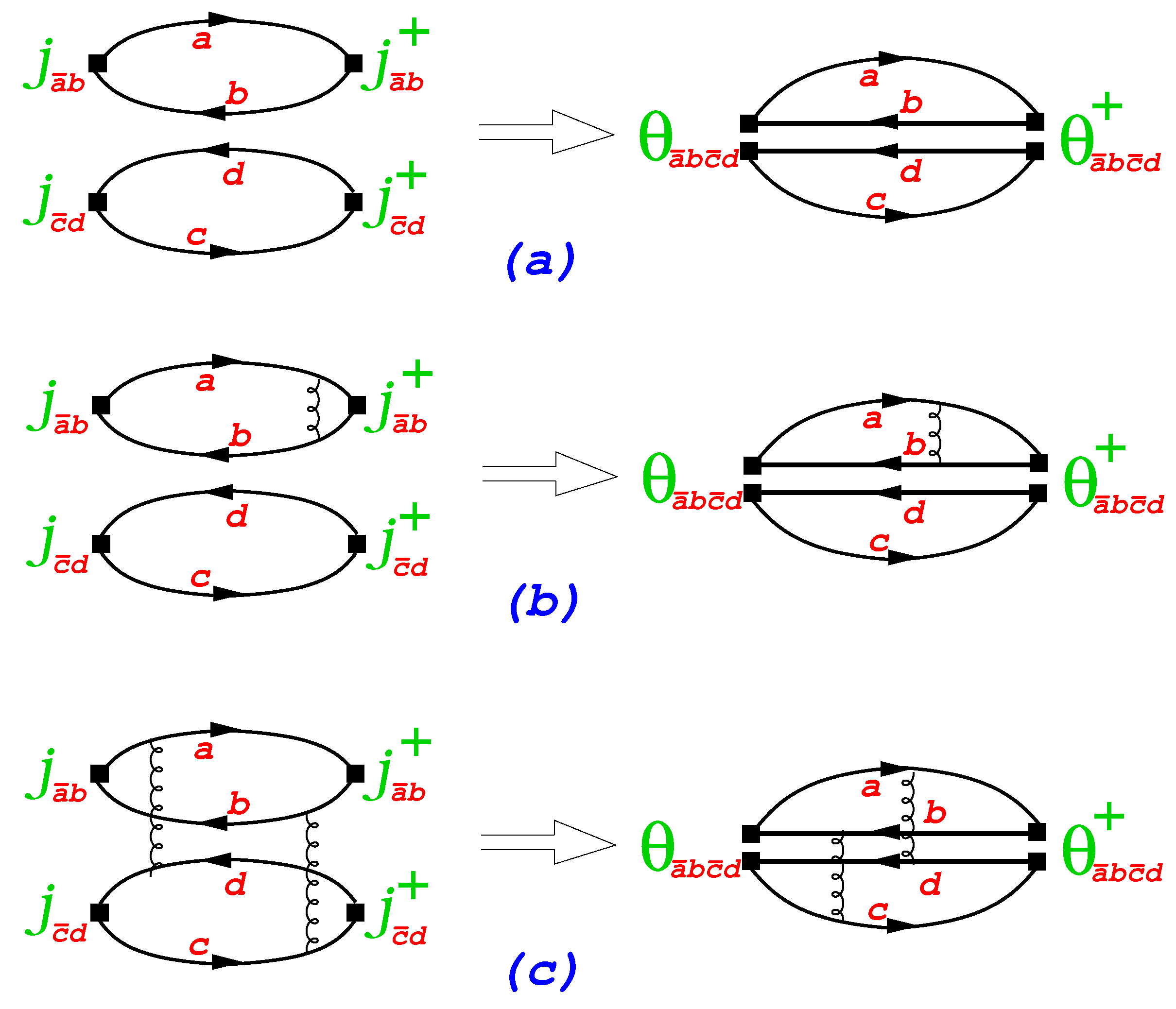
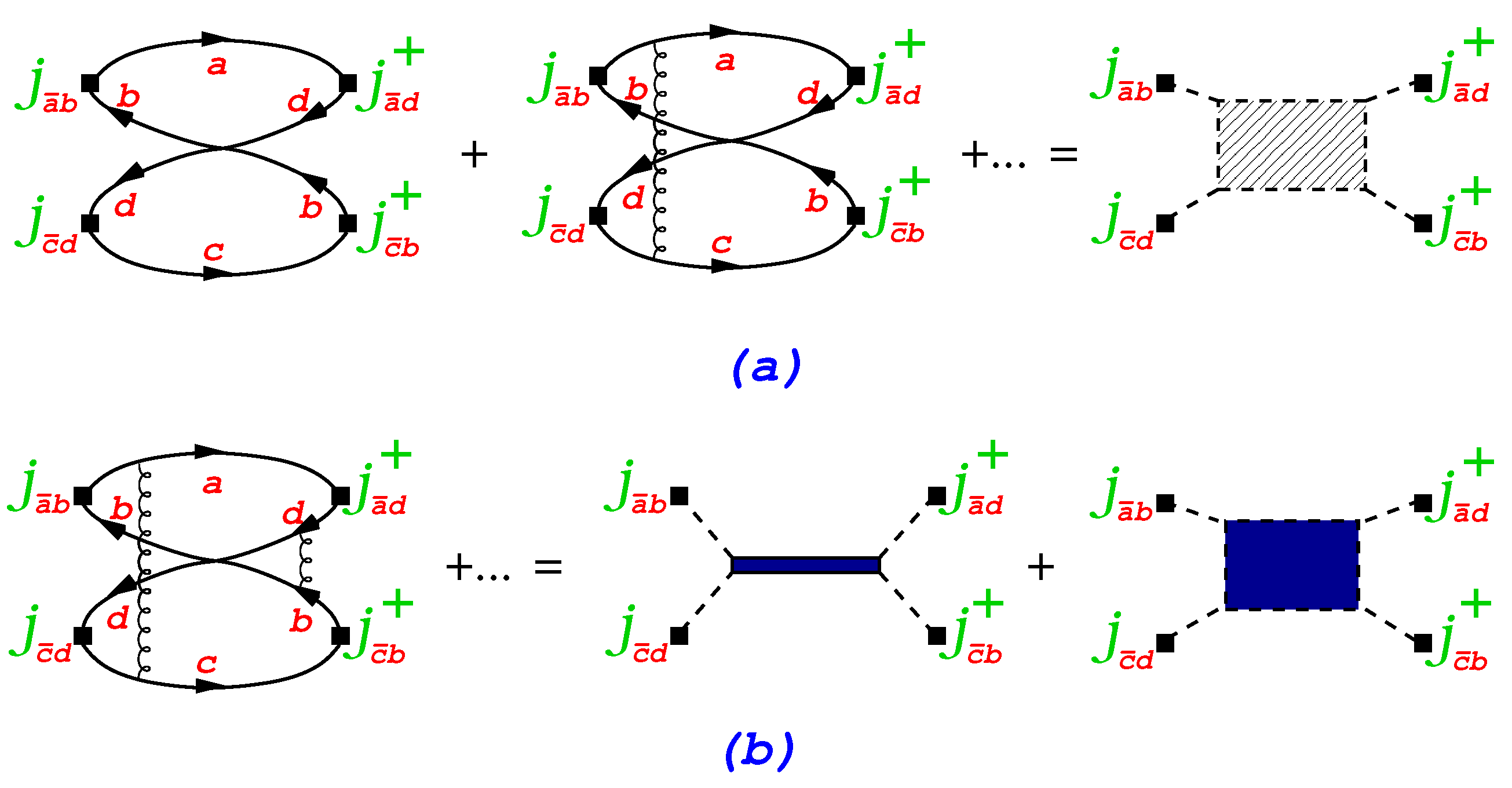
- For flavor-preserving correlation functions, the line of argument proves to be, more or less, evident. All the contributions of the type of Figure 1a or of the type of Figure 1b, involving at most one gluon exchange, are doubtlessly disconnected. The contributions that involve a single gluon exchange between their two (otherwise disconnected) quark loops vanish identically, due to the vanishing of the sum over color degrees of freedom of each of the two quark loops. Phrased slightly more technically, this can be traced back to the tracelessness of all generators of a special unitary group, governing the couplings of quarks and gluons. Consequently, exclusively contributions that involve, at least, two gluon exchanges of an appropriate topology may be viewed as tetraquark-phile. These insights are, of course, corroborated by identifying these tetraquark-phile contributions according to Proposition 1 by explicit inspection [16] by way of their Landau equations. Replacing any double contraction (8) in Figure 1 by a single contraction (9) confirms the tetraquark-phile nature of contributions of the type of Figure 2 or related higher orders.
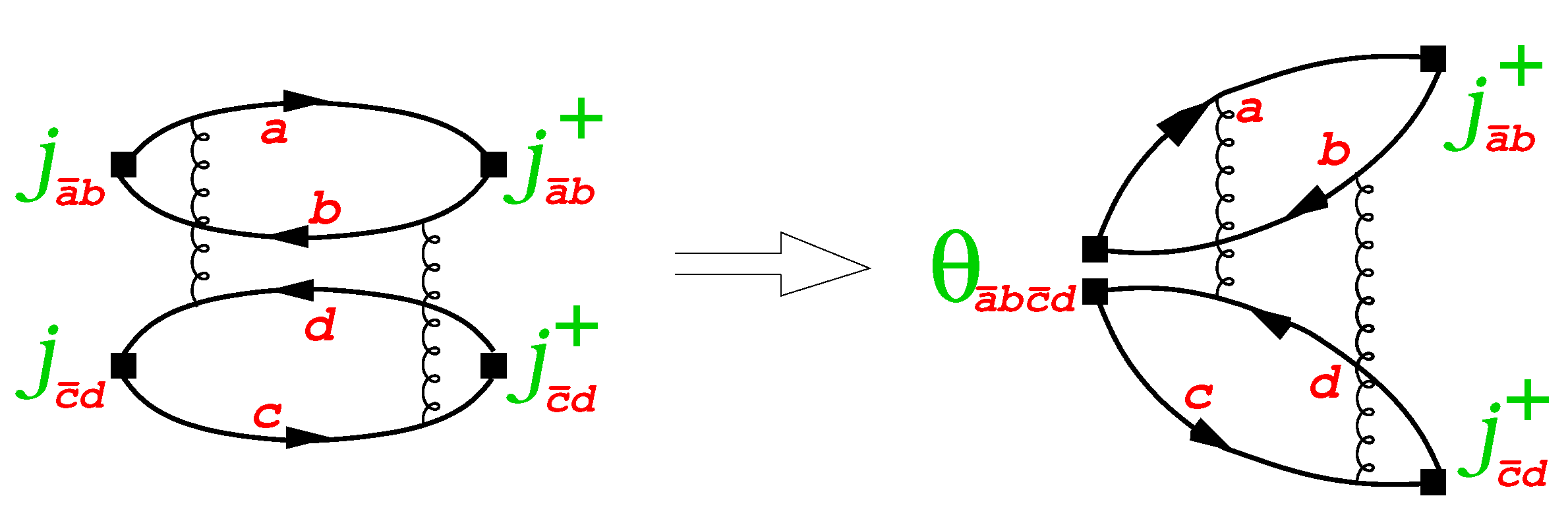
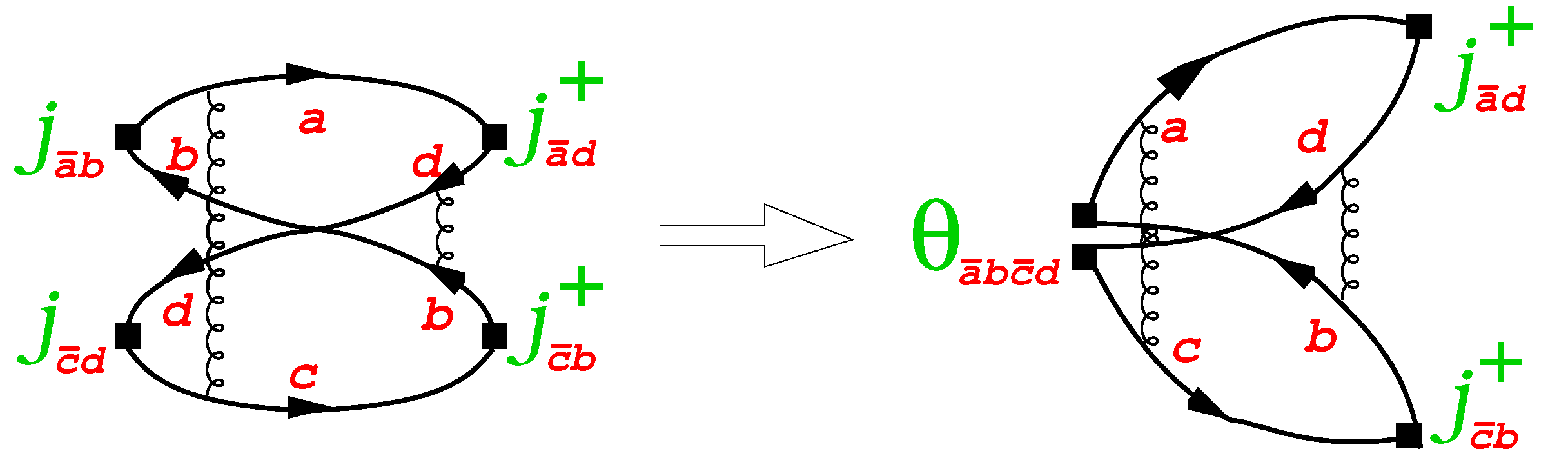
- For flavor-rearranging correlation functions, simple optical guidance in this analysis is, beyond doubt, hardly imaginable: already the lowest-order contributions turn out to be connected. Rather, one has to gladly accept any assistance offered by the tool called Landau equations. For the three lowest-order contributions exemplified in Figure 3, the usage of this formalism is demonstrated, in full detail, in Appendix A of Reference [18], in the Appendix of Reference [31], as well as in Section 4 of Reference [24]. For this kind of analysis, it might prove advantageous to recast the encountered plots into a box shape, by “unfolding” all these plots [14,18,24,31]. These efforts’ outcome is that contributions of the type of Figure 3a or of the type of Figure 3b, being characterized by no or only one internal gluon exchange, do not incorporate the requested four-quark singularities. The involvement of this feature starts not before the level of two gluon exchanges of suitable positioning, which then holds, of course, likewise for the single contractions (9) in Figure 4.
4. Number of Color Degrees of Freedom, Unfixed: Large- Limit and Expansion
- Generalize QCD to the gauge theories invariant under a non-Abelian Lie group SU(). The dynamical degrees of freedom of each of the latter quantum field theories hence are its gauge bosons, still retaining their designation as gluons and transforming according to the -dimensional, adjoint representation of SU(), and its fermionic quarks that transform according to the -dimensional, fundamental representation of SU().
- Allow the number of color degrees of freedom, , to increase from to infinity:
- For the strong coupling strength , demand the related decrease, with rising , to zero:Clearly, for the strong fine-structure coupling this requirement implies the behaviorTherefore, in the large- limit, the product approaches a meaningful finite value.
- the number of closed loops of the color degrees of freedom carried by quarks or gluons,
5. Multiquark-Adequate QCD Sum Rules Recognizing “Peculiarities” of Exotic Hadrons
- In the course of QCD-level evaluation, Wilson’s operator product expansion [28] (enabling conversion of a nonlocal product of operators into a series of local operators) is invoked to separate nonperturbative and (to some extent calculable) perturbative contributions.
- −
- The perturbative contributions, identical to the lowest term of this operator product expansion, can be inferred in the form of a series in powers of the strong coupling (2).
- −
- The nonperturbative contributions involve, apart from derivable prefactors, vacuum condensates, i.e., the vacuum expectation values of products of quark and/or gluon field operators, which may be interpreted as a kind of effective parameters of QCD.
- In the course of hadron-level evaluation, the insertion of a complete set of hadron states guarantees that the hadron under study shows up by way of its intermediate-state pole.
- 1.
- Subject both sides of such a relation to a Borel transformation to another variable called Borel parameter . This results in the entire removal of any subtraction term introduced and the suppression of the hadron-level contributions above the hadronic ground state. Under a Borel transformation, all vacuum condensates in the nonperturbative QCD-level contributions are multiplied by powers of . So, these terms are called power corrections.
- 2.
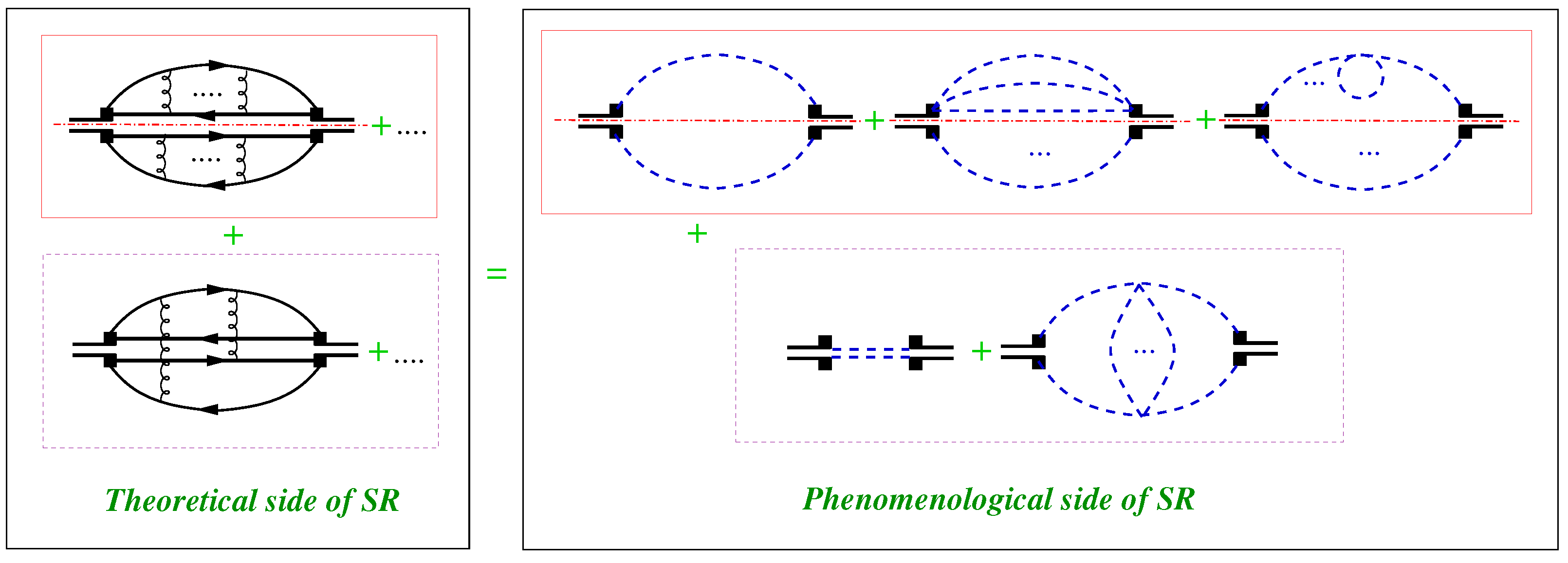
- Rely on the assumption of quark–hadron duality, which postulates a (needless to stress, approximately realized) cancellation of all perturbative QCD-level contributions above suitably defined effective thresholds, , against all higher hadron-level contributions, consisting of hadron excitations and hadron continuum. In implementing this concept, the problem of pinning down the nature of may be dealt with in two different ways:
- Without knowing better, just a guessed fixed value of the parameter is adopted:
- In contrast, slipping in limited information about a targeted hadron state opens the possibility [29] to work out the expected dependence on the Borel parameters :
- In the flavor-preserving case, one has to start from the four-point correlation functionsApplying the traditional QCD sum-rule manipulations to twofold contractions (8) of the correlation functions (25) yields as an outcome of this enterprise a relationship, depicted in Figure 5, that incorporates a (vast) multitude of QCD-level and hadron-level quantities.However, a more in-depth analysis [30] reveals that, already on diagrammatic grounds, this conglomerate decomposes, in fact, into two QCD sum rules for conventional mesons (Figure 6) and one further QCD sum rule that, potentially, supports the development of tetraquark poles and rightly deserves the label of being “tetraquark-adequate” (Figure 7). In the course of its QCD-level evaluation, this latter QCD sum rule receives, exclusively, tetraquark-phile contributions, in the sense of Proposition 1; all the perturbative among these enter in form of dispersion integrals of tetraquark-adequate spectral densities, . An analogous reflection for single contractions (9) of the correlation functions (25) leads to similar QCD sum-rule findings, all perturbative tetraquark-phile QCD contributions being encoded, in dispersive formulation, in tetraquark-adequate spectral densities .
- In the flavor-rearranging case, one has to deal with the four-point correlation functionHere, irrespective of (ultimately necessary) spatial contractions (8) and (9) of four-point correlation functions (7), the analysis is unfortunately not thus straightforward as in the flavor-preserving case: Within QCD-level evaluation, all tetraquark-phile contributions (defined by requiring them to satisfy the constraint formulated in Proposition 1) may be identified, case by case, by inspection of the solutions of the relevant Landau equations. Within hadron-level evaluation, that QCD-level characteristic of being tetraquark-phile or not is mirrored by the ability of any contributions at the hadron level to accommodate, in their s channel, two-meson intermediate states or not, in addition to a possible presence of tetraquark intermediate-state poles [31]. Hardly surprisingly, these insights translate the outcome of the QCD sum-rule formalism based on the correlation function (26) into a quark–hadron relation of (expected) two-component structure symbolically shown in Figure 8. All perturbative tetraquark-phile QCD-level contributions find their way into a tetraquark-adequate QCD sum rule arising from a precursor as in Figure 8b by spectral densities in the double-contractions case (8) and in the single-contraction case (9).
- momentum-space amplitudes and , Fourier-transformed vacuum–tetraquark matrix elements of appropriate pairs of quark bilinear currents (5),
6. Summary, Conclusion, and Outlook—Multiquark-Instigated Theoretical Adaptations
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
Abbreviations
| LHCb | Large Hadron Collider beauty |
| OPE | operator product expansion |
| QCD | quantum chromodynamics |
References
- Lucha, W.; Schöberl, F.F.; Gromes, D. Bound states of quarks. Phys. Rep. 1991, 200, 127. [Google Scholar] [CrossRef]
- Lucha, W.; Melikhov, D.; Sazdjian, H. Cluster reducibility of multiquark operators. Phys. Rev. D 2019, 100, 094017. [Google Scholar] [CrossRef]
- Particle Data Group; Workman, R.L.; Burkert, V.D.; Crede, V.; Klempt, E.; Thoma, U.; Tiator, L.; Agashe, K.; Aielli, G.; Allanach, B.C.; et al. Review of particle physics. Prog. Theor. Exp. Phys. 2022, 2022, 083C01. [Google Scholar]
- Gershon, T. et al. [LHCb Collaboration] Exotic hadron naming convention. arXiv 2022, arXiv:2206.15233. [Google Scholar]
- Aaij, R.; Abellán Beteta, C.; Ackernley, T.; Adeva, B.; Adinolfi, M.; Afsharnia, H.; Aidala, C.A.; Aiola, S.; Ajaltouni, Z.; Akar, S.; et al. Model-independent study of structure in B+→D+D−K+ decays. Phys. Rev. Lett. 2020, 125, 242001. [Google Scholar] [CrossRef]
- Aaij, R.; Abellán Beteta, C.; Ackernley, T.; Adeva, B.; Adinolfi, M.; Afsharnia, H.; Aidala, C.A.; Aiola, S.; Ajaltouni, Z.; Akar, S.; et al. Amplitude analysis of the B+→D+D−K+ decay. Phys. Rev. D 2020, 102, 112003. [Google Scholar] [CrossRef]
- Aaij, R.; Abdelmotteleb, A.S.W.; Abellán Beteta, C.; Abudinen Gallego, F.J.; Ackernley, T.; Adeva, B.; Adinolfi, M.; Afsharnia, H.; Agapopoulou, C.; Aidala, C.A.; et al. Observation of an exotic narrow doubly charmed tetraquark. Nat. Phys. 2022, 18, 751. [Google Scholar]
- Aaij, R.; Abdelmotteleb, A.S.W.; Abellán Beteta, C.; Abudinen Gallego, F.J.; Ackernley, T.; Adeva, B.; Adinolfi, M.; Afsharnia, H.; Agapopoulou, C.; Aidala, C.A.; et al. Study of the doubly charmed tetraquark Tcc+. Nat. Commun. 2022, 13, 3351. [Google Scholar]
- Aaij, R. et al. [LHCb Collaboration] First observation of a doubly charged tetraquark and its neutral partner. arXiv 2022, arXiv:2212.02716. [Google Scholar]
- Aaij, R. et al. [LHCb Collaboration] Amplitude analysis of B0→D¯0Ds+π- and B+→D-Ds+π+ decays. arXiv 2022, arXiv:2212.02717. [Google Scholar]
- ’t Hooft, G. A planar diagram theory for strong interactions. Nucl. Phys. B 1974, 72, 461. [Google Scholar] [CrossRef]
- Witten, E. Baryons in the 1/N expansion. Nucl. Phys. B 1979, 160, 57. [Google Scholar] [CrossRef]
- Knecht, M.; Peris, S. Narrow tetraquarks at large. N. Phys. Rev. D 2013, 88, 036016. [Google Scholar] [CrossRef]
- Cohen, T.D.; Lebed, R.F. Are there tetraquarks at large Nc in QCD(F)? Phys. Rev. D 2014, 90, 016001. [Google Scholar] [CrossRef]
- Maiani, L.; Polosa, A.D.; Riquer, V. Tetraquarks in the 1/N expansion and meson–meson resonances. J. High Energy Phys. 2016, 6, 160. [Google Scholar] [CrossRef]
- Lucha, W.; Melikhov, D.; Sazdjian, H. Narrow exotic tetraquark mesons in large-Nc QCD. Phys. Rev. D 2017, 96, 014022. [Google Scholar] [CrossRef]
- Lucha, W.; Melikhov, D.; Sazdjian, H. Exotic states and their properties from large-Nc QCD. PoS 2018, 340, 390. [Google Scholar]
- Lucha, W.; Melikhov, D.; Sazdjian, H. Tetraquark and two-meson states at large Nc. Eur. Phys. J. C 2017, 77, 866. [Google Scholar] [CrossRef]
- Lucha, W.; Melikhov, D.; Sazdjian, H. Constraints from the 1/Nc expansion on properties of exotic tetraquark mesons. PoS 2018, 310, 233. [Google Scholar]
- Lucha, W.; Melikhov, D.; Sazdjian, H. Exotic tetraquark mesons in large-Nc limit: An unexpected great surprise. EPJ Web Conf. 2018, 192, 00044. [Google Scholar] [CrossRef]
- Lucha, W.; Melikhov, D.; Sazdjian, H. Compact flavour-exotic tetraquarks in large-Nc QCD—To be or not to be? PoS 2019, 336, 087. [Google Scholar]
- Lucha, W.; Melikhov, D.; Sazdjian, H. Are there narrow flavour-exotic tetraquarks in large-Nc QCD? Phys. Rev. D 2018, 98, 094011. [Google Scholar] [CrossRef]
- Lucha, W.; Melikhov, D.; Sazdjian, H. OPE and quark–hadron duality for two-point functions of tetraquark currents in the 1/Nc expansion. Phys. Rev. D 2021, 103, 014012. [Google Scholar] [CrossRef]
- Lucha, W.; Melikhov, D.; Sazdjian, H. Tetraquarks in large-Nc QCD. Prog. Part Nucl. Phys. 2021, 120, 103867. [Google Scholar] [CrossRef]
- Shifman, M.A.; Vainshtein, A.I.; Zakharov, V.I. QCD and resonance physics. Theoretical foundations. Nucl. Phys. B 1979, 147, 385. [Google Scholar] [CrossRef]
- Reinders, L.J.; Rubinstein, H.; Yazaki, S. Hadron properties from QCD sum rules. Phys. Rep. 1985, 127, 1–97. [Google Scholar] [CrossRef]
- Colangelo, P.; Khodjamirian, A. QCD sum rules, a modern perspective. In At the Frontier of Particle Physics—Handbook of QCD. Boris Ioffe Festschrift; Shifman, M., Ed.; World Scientific: Singapore, 2001; Volume 3, p. 1495. [Google Scholar]
- Wilson, K.G. Non-Lagrangian models of current algebra. Phys. Rev. 1969, 179, 1499. [Google Scholar] [CrossRef]
- Lucha, W.; Melikhov, D.; Simula, S. Effective continuum threshold in dispersive sum rules. Phys. Rev. D 2009, 79, 096011. [Google Scholar] [CrossRef]
- Lucha, W.; Melikhov, D.; Sazdjian, H. Tetraquark-adequate formulation of QCD sum rules. Phys. Rev. D 2019, 100, 014010. [Google Scholar] [CrossRef]
- Lucha, W.; Melikhov, D.; Sazdjian, H. Tetraquark-adequate QCD sum rules for quark-exchange processes. Phys. Rev. D 2019, 100, 074029. [Google Scholar] [CrossRef]
- Lucha, W.; Melikhov, D.; Sazdjian, H. Multiquark-adequate QCD sum rules: The case of flavour-exotic tetraquarks. EPJ Web Conf. 2019, 222, 03016. [Google Scholar] [CrossRef]
- Jaffe, R.L. Two types of hadrons. Nucl. Phys. A 2008, 804, 25. [Google Scholar] [CrossRef]
- Fierz, M. Zur Fermischen Theorie des β-Zerfalls. Zeitschrift für Physik 1937, 104, 553. [Google Scholar] [CrossRef]
- Landau, L.D. On analytic properties of vertex parts in quantum field theory. Nucl. Phys. 1959, 13, 181. [Google Scholar] [CrossRef]
- Chen, H.-X.; Chen, W.; Zhu, S.-L. Possible interpretations of the Pc(4312), Pc(4440), and Pc(4457). Phys. Rev. D 2019, 100, 051501. [Google Scholar] [CrossRef]
- Pimikov, A.; Lee, H.-J.; Zhang, P. Hidden-charm pentaquarks with color-octet substructure in QCD sum rules. Phys. Rev. D 2020, 101, 014002. [Google Scholar] [CrossRef]
- Brambilla, N.; Eidelman, S.; Hanhart, C.; Nefediev, A.; Shen, C.-P.; Thomas, C.E.; Vairo, A.; Yuan, C.-Z. The XYZ states: Experimental and theoretical status and perspectives. Phys. Rep. 2020, 873, 1–154. [Google Scholar] [CrossRef]
- Qin, S.-X.; Roberts, C.D. Impressions of the continuum bound state problem in QCD. Chin. Phys. Lett. 2020, 37, 121201. [Google Scholar] [CrossRef]
- Li, S.-H.; Chen, Z.-S.; Jin, H.-Y.; Chen, W. Mass of 1-+ four-quark–hybrid mixed states. Phys. Rev. D 2022, 105, 054030. [Google Scholar] [CrossRef]
- Cid-Mora, B.A.; Steele, T.G. Next-to-leading order (NLO) perturbative effects in QCD sum-rule analyses of light tetraquark systems: A case study in the scalar-isoscalar channel. Nucl. Phys. A 2022, 1028, 122538. [Google Scholar] [CrossRef]
- Sundu, H.; Agaev, S.S.; Azizi, K. Axial-vector and pseudoscalar tetraquarks [ud][c¯s¯]. Eur. Phys. J. C 2023, 83, 198. [Google Scholar] [CrossRef]

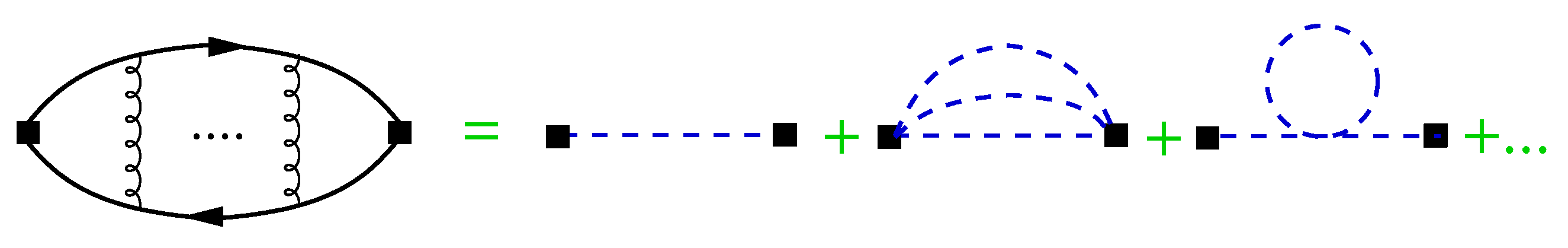
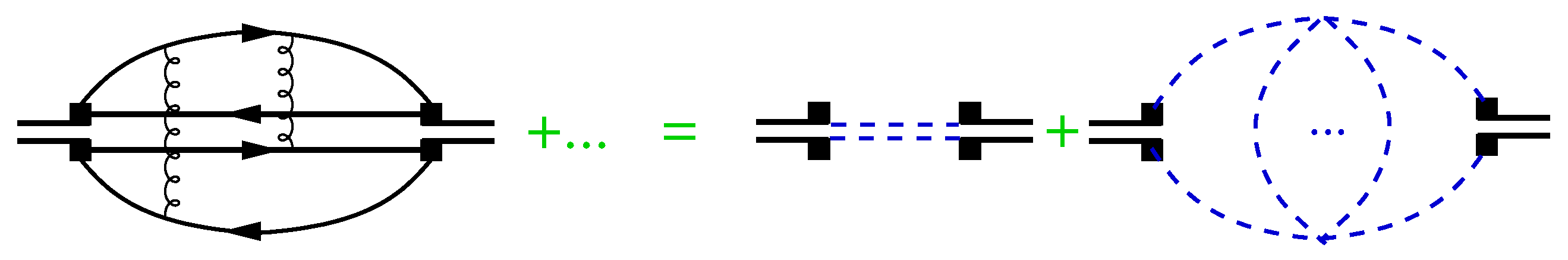
| Number of Different Quark Flavors Involved | Quark Composition | Number of Open Quark Flavors Involved |
|---|---|---|
| 4 | 4 | |
| 3 | 4 | |
| 4 | ||
| 2 | ||
| 2 | ||
| 2 | 4 | |
| 2 | ||
| 2 | ||
| 0 | ||
| 0 | ||
| 1 | 0 |
| Candidate Tetraquark Meson | (Minimal) Quark-Flavor Content | References |
|---|---|---|
| [5,6] | ||
| [5,6] | ||
| [7,8] | ||
| [9,10] | ||
| [9,10] |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the author. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Lucha, W. Mission Target: Exotic Multiquark Hadrons—Sharpened Blades. Universe 2023, 9, 171. https://doi.org/10.3390/universe9040171
Lucha W. Mission Target: Exotic Multiquark Hadrons—Sharpened Blades. Universe. 2023; 9(4):171. https://doi.org/10.3390/universe9040171
Chicago/Turabian StyleLucha, Wolfgang. 2023. "Mission Target: Exotic Multiquark Hadrons—Sharpened Blades" Universe 9, no. 4: 171. https://doi.org/10.3390/universe9040171
APA StyleLucha, W. (2023). Mission Target: Exotic Multiquark Hadrons—Sharpened Blades. Universe, 9(4), 171. https://doi.org/10.3390/universe9040171






