Integral Fluxes of Neutrinos and Gamma-Rays Emitted from Neighboring X-ray Binaries †
Abstract
1. Introduction
2. Model Calculations for the Astrophysical Outflows from X-ray Binaries
2.1. p-p Collision Mechanism Inside the Jets
2.2. Neutrino and Gamma-Ray Production in the Jet Frame
3. Photon Annihilation in the X-ray Binary Environment
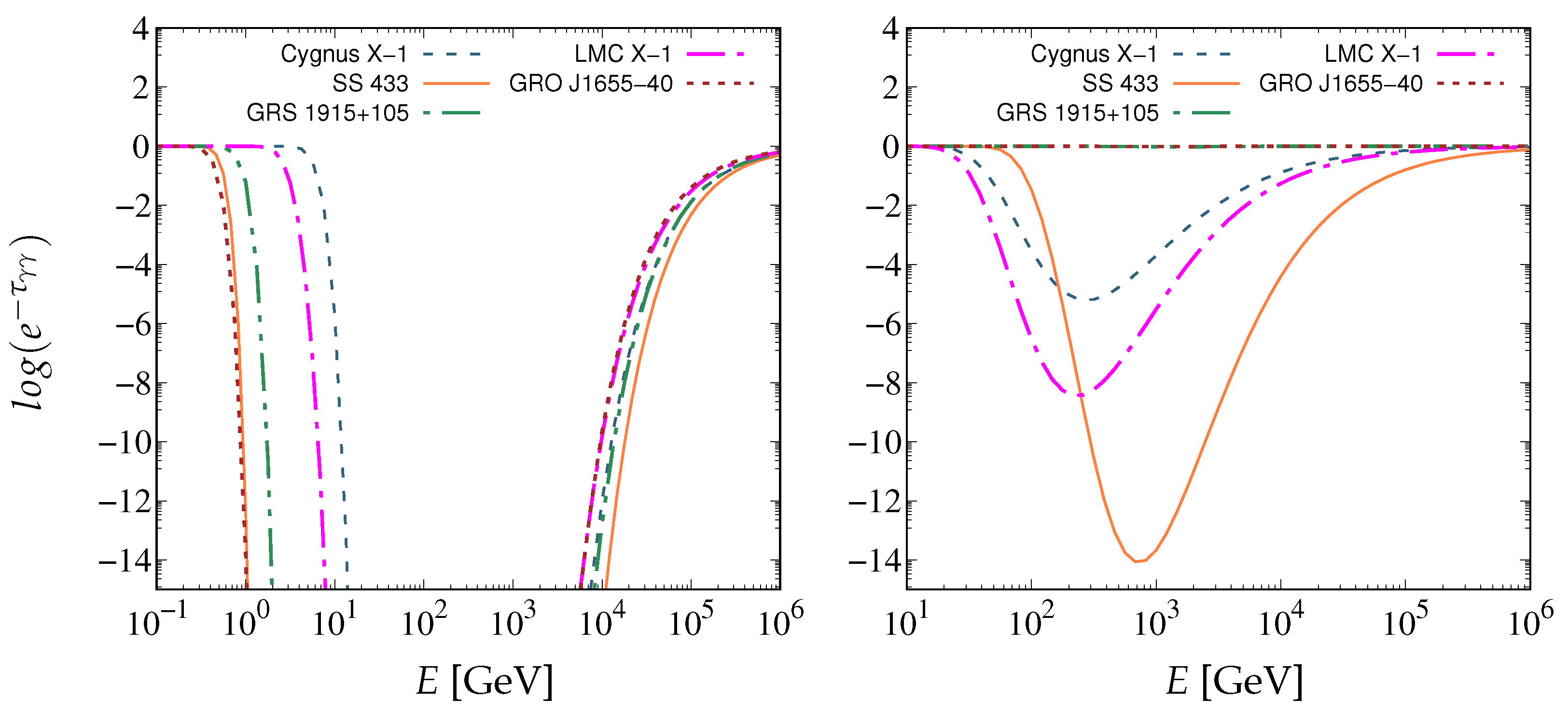
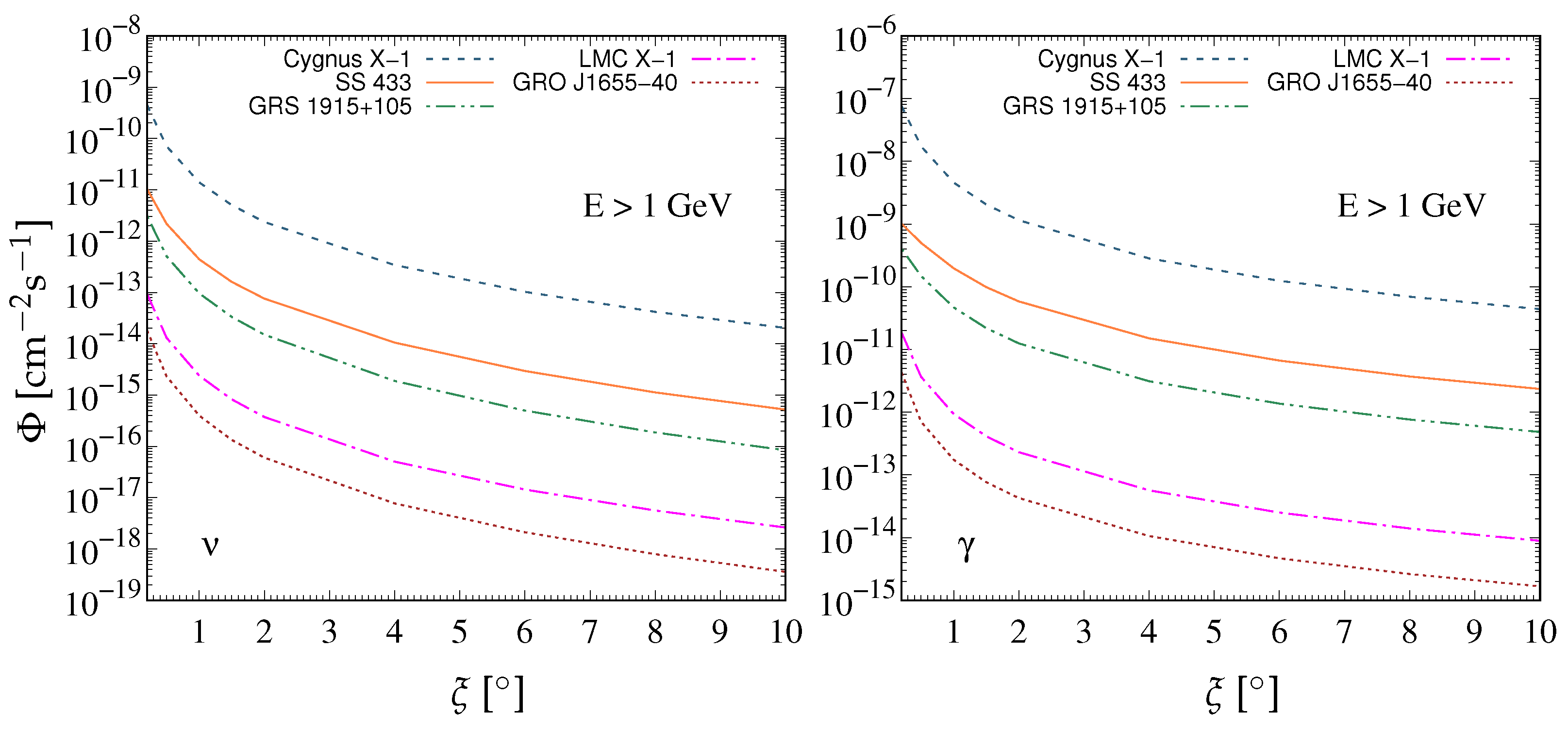
4. Results
5. Summary and Outlook
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Blandford, R.D.; Znajek, R.L. Electromagnetic extraction of energy from Kerr black holes. Mon. Not. R. Astron. Soc. 1977, 179, 433–456. [Google Scholar] [CrossRef]
- Aharonian, F.; Akhperjanian, A.G.; Aye, K.M.; Bazer-Bachi, A.R.; Beilicke, M.; Benbow, W.; Berge, D.; Berghaus, P.; Bernlohr, K.; Boisson, C.; et al. Discovery of Very High Energy Gamma Rays Associated with an X-ray Binary. Science 2005. [Google Scholar] [CrossRef] [PubMed]
- Aharonian, F.; Anchordoqui, L.; Khangulyan, D.; Montaruli, T. Microquasar LS 5039: A TeV gamma-ray emitter and a potential TeV neutrino source. J. Phys. Conf. Ser. 2006, 39, 408. [Google Scholar] [CrossRef]
- Aartsen, M.G. et al. [The IceCube Collaboration] Searches for Sterile Neutrinos with the IceCube Detector. Phys. Rev. Lett. 2016, 117, 071801. [Google Scholar] [CrossRef] [PubMed]
- Aartsen, M.G. et al. [The IceCube Collaboration] All-sky Search for Time-integrated Neutrino Emission from Astrophysical Sources with 7 yr of IceCube Data. Astrophys. J. 2017, 835, 151. [Google Scholar] [CrossRef]
- Adrian-Martinez, S.; Ageron, M.; Aharonian, F.; Aiello, S.; Albert, A.; Ameli, F.; Anassontzis, E.; Andre, M.; Androulakis, G.; Anghinolfi, M.; et al. Letter of intent for KM3NeT 2.0. J. Phys. G Nucl. Part. Phys. 2016, 43, 084001. [Google Scholar] [CrossRef]
- Albert, A.; André, M.; Anghinolfi, M.; Anton, G.; Ardid, M.; Aubert, J.J.; Aublin, J.; Baret, B.; Basa, S.; Belhorma, B.; et al. ANTARES and IceCube Combined Search for Neutrino Point-like and Extended Sources in the Southern Sky. Astrophys. J. 2020, 892, 92. [Google Scholar] [CrossRef]
- Albert, A.; André, M.; Anghinolfi, M.; Anton, G.; Ardid, M.; Aubert, J.J.; Avgitas, T.; Baret, B.; Barrios-Martí, J.; Basa, S.; et al. First all-flavor neutrino pointlike source search with the ANTARES neutrino telescope. Phys. Rev. D 2017, 96, 082001. [Google Scholar] [CrossRef]
- Atwood, W.B.; Abdo, A.A.; Ackermann, M.; Althouse, W.; Anderson, B.; Axelsson, M.; Baldini, L.; Ballet, J.; Band, D.L.; Barbiellini, G.; et al. The large area telescope on the Fermi gamma-ray space telescope mission. Astrophys. J. 2009, 697, 1071. [Google Scholar] [CrossRef]
- Aleksić, J.; Ansoldi, S.; Antonelli, L.A.; Antoranz, P.; Babic, A.; Bangale, P.; Barceló, M.; Barrio, J.A.; González, J.B.; Bednarek, W.; et al. The major upgrade of the MAGIC telescopes, Part I: The hardware improvements and the commissioning of the system. Astropart. Phys. 2016, 72, 61–75. [Google Scholar] [CrossRef]
- Acharya B.S. et al. [Cherenkov Telescope Array Consortium] Science with the Cherenkov Telescope Array; World Scientific Publishing Co. Pte Ltd.: Singapore, 2019. [Google Scholar] [CrossRef]
- Zanin, R.; Fernández-Barral, A.; de Oña Wilhelmi, E.; Aharonian, F.; Blanch, O.; Bosch-Ramon, V.; Galindo, D. Gamma rays detected from Cygnus with likely jet origin. Astron. Astrophys. 2016, 596, A55. [Google Scholar] [CrossRef]
- Zdziarski, A.A.; Malyshev, D.; Chernyakova, M.; Pooley, G.G. High-energy gamma-rays from Cyg X-1. Mon. Not. R. Astron. Soc. 2017, 471, 3657–3667. [Google Scholar] [CrossRef]
- Ahnen, M.L.; Ansoldi, S.; Antonelli, L.A.; Arcaro, C.; Babić, A.; Banerjee, B.; Bangale, P.; de Almeida, U.B.; Barrio, J.A.; González, J.B.; et al. Search for very high-energy gamma-ray emission from the microquasar Cygnus X-1 with the MAGIC telescopes. Mon. Not. R. Astron. Soc. 2017, 472, 3474–3485. [Google Scholar] [CrossRef]
- Abeysekara, A.U.; Albert, A. Very-high-energy particle acceleration powered by the jets of the microquasar SS 433. Nature 2018, 562, 82–85. [Google Scholar] [CrossRef]
- Aharonian, F.; Akhperjanian, A.; Beilicke, M.; Bernlöhr, K.; Börst, H.G.; Bojahr, H.; Bolz, O.; Coarasa, T.; Contreras, J.; Cortina, J.; et al. TeV gamma-ray observations of SS-433 and a survey of the surrounding field with the HEGRA IACT-System. Astron. Astrophys. 2005, 439, 635–643. [Google Scholar] [CrossRef][Green Version]
- Saito, T.Y.; Zanin, R.; Bordas, P.; Bosch-Ramon, V.; Jogler, T.; Paredes, J.M.; Ribo, M.; Rissi, M.; Rico, J.; Torres, D.F. Microquasar observations with the MAGIC telescope. arXiv 2009, arXiv:0907.1017. [Google Scholar] [CrossRef]
- Ahnen, M.L. et al. [MAGIC Collaboration] Constraints on particle acceleration in SS433/W50 from MAGIC and H.E.S.S. observations. Astron. Astrophys. 2018, 612, A14. [Google Scholar] [CrossRef]
- Zhang, S.N.; Ebisawa, K.; Sunyaev, R.; Ueda, Y.; Harmon, B.A.; Sazonov, S.; Fishman, G.J.; Inoue, H.; Paciesas, W.S.; Takahash, T. Broadband High-Energy Observations of the Superluminal Jet Source GRO J1655–40 during an Outburst. Astrophys. J. 1997, 479, 381. [Google Scholar] [CrossRef]
- Kroeger, R.A.; Strickman, M.S.; Grove, J.E.; Kaaret, P.; Ford, E.; Harmon, B.A.; McConnell, M. Gamma-Ray Observations of GRO J1655-40. Astron. Astrophys. Suppl. Ser. 1996, 120, C117. [Google Scholar]
- Bodaghee, A.; Tomsick, J.A.; Pottschmidt, K.; Rodriguez, J.; Wilms, J.; Pooley, G.G. Gamma-ray observations of the microquasars cygnus X-1, CYGNUS X-3, GRS 1915+105, and GX 339-4 with the FERMI large area telescope. Astrophys. J. 2013, 775, 98. [Google Scholar] [CrossRef]
- Szostek, A.; Dubus, G.; Brun, F.; de Naurois, M. VHE gamma-ray observations of the microquasar GRS 1915+105. arXiv 2009, arXiv:0907.3034. [Google Scholar] [CrossRef]
- Schüssler, F.; Bordas, P.; Chadwick, P.M.; Dickinson, H.; Ernenwein, J.P. Simultaneous H.E.S.S. and RXTE observations of the microquasars GRS 1915+105, Circinus X-1 and V4641 Sgr. arXiv 2015, arXiv:1509.03039. [Google Scholar] [CrossRef]
- Aartsen, M.G.; Ackermann, M.; Adams, J.; Aguilar, J.A.; Ahlers, M.; Ahrens, M.; Altmann, D.; Anderson, T.; Archinger, M.; Arguelles, C.; et al. Searches for time-dependent neutrino sources with icecube data from 2008 to 2012. Astrophys. J. 2015, 807, 46. [Google Scholar] [CrossRef]
- Albert, A.; André, M.; Anton, G.; Ardid, M.; Aubert, J.J.; Avgitas, T.; Baret, B.; Barrios-Martí, J.; Basa, S.; Bertin, V.; et al. Time-dependent search for neutrino emission from X-ray binaries with the ANTARES telescope. J. Cosmol. Astropart. Phys. 2017, 2017, 019. [Google Scholar] [CrossRef]
- Aartsen, M.G. et al. [The IceCube Collaboration] Search for steady point-like sources in the astrophysical muon neutrino flux with 8 years of IceCube data. Eur. Phys. J. C 2019, 79, 234. [Google Scholar] [CrossRef]
- Reynoso, M.M.; Carulli, A.M. On the possibilities of high-energy neutrino production in the jets of microquasar SS433 in light of new observational data. Astropart. Phys. 2019, 109, 25–32. [Google Scholar] [CrossRef]
- Abbasi, R.; Ackermann, M.; Adams, J.; Aguilar, J.A.; Ahlers, M.; Ahrens, M.; Alameddine, J.M.; Alves, A.A.; Amin, N.M.; Andeen, K.; et al. Search for High-energy Neutrino Emission from Galactic X-ray Binaries with IceCube. Astrophys. J. Lett. 2022, 930, L24. [Google Scholar] [CrossRef]
- Aharonian, F.; Atoyan, A. Gamma rays from galactic sources with relativistic jets. New Astron. Rev. 1998, 42, 579–584. [Google Scholar] [CrossRef]
- Markoff, S.; Falcke, H.; Fender, R. A jet model for the broadband spectrum of XTE J1118+480 - Synchrotron emission from radio to X-rays in the Low/Hard spectral state. Astron. Astrophys. 2001, 372, L25–L28. [Google Scholar] [CrossRef]
- Bosch-Ramon, V.; Romero, G.E.; Paredes, J.M. A broadband leptonic model for gamma-ray emitting microquasars. Astron. Astrophys. 2006, 447, 263–276. [Google Scholar] [CrossRef]
- Levinson, A.; Waxman, E. Probing Microquasars with TeV Neutrinos. Phys. Rev. Lett. 2001, 87, 171101. [Google Scholar] [CrossRef] [PubMed]
- Romero, G.E.; Torres, D.F.; Kaufman Bernadó, M.M.; Mirabel, I.F. Hadronic gamma-ray emission from windy microquasars. Astron. Astrophys. 2003, 410, L1–L4. [Google Scholar] [CrossRef]
- Bednarek, W. TeV Neutrinos from Microquasars in Compact Massive Binaries. Astrophys. J. 2005, 631, 466. [Google Scholar] [CrossRef][Green Version]
- Reynoso, M.M.; Romero, G.E. Magnetic field effects on neutrino production in microquasars. Astron. Astrophys. 2009, 493, 1–11. [Google Scholar] [CrossRef]
- Reynoso, M.M.; Romero, G.E.; Christiansen, H.R. Production of gamma rays and neutrinos in the dark jets of the microquasar SS433. Mon. Not. R. Astron. Soc. 2008, 387, 1745–1754. [Google Scholar] [CrossRef][Green Version]
- Böttcher, M.; Dermer, C.D. Photon-Photon Absorption of Very High Energy Gamma Rays from Microquasars: Application to LS 5039. Astrophys. J. 2005, 634, L81–L84. [Google Scholar] [CrossRef]
- Cerutti, B.; Dubus, G.; Malzac, J.; Szostek, A.; Belmont, R.; Zdziarski, A.A.; Henri, G. Absorption of high-energy gamma rays in Cygnus X-3. Astron. Astrophys. 2011, 529, A120. [Google Scholar] [CrossRef][Green Version]
- Papavasileiou, T.V.; Kosmas, O.T.; Sinatkas, I. Prediction of gamma-ray emission from Cygnus X-1, SS 433, and GRS 1915+105 after absorption. Astron. Astrophys. 2023, in press. [Google Scholar] [CrossRef]
- Papavasileiou, T.; Kosmas, O.; Sinatkas, I. Studying the Spectral Energy Distributions Emanating from Regular Galactic XRBs. Universe 2023, 9, 312. [Google Scholar] [CrossRef]
- Lipari, P.; Lusignoli, M.; Meloni, D. Flavor composition and energy spectrum of astrophysical neutrinos. Phys. Rev. D 2007, 75, 123005. [Google Scholar] [CrossRef]
- Papadopoulos, D.A.; Kosmas, O.T.; Ganatsios, S. Modeling Particle Transport in Astrophysical Outflows and Simulations of Associated Emissions from Hadronic Microquasar Jets. Adv. High Energy Phys. 2022, 2022, 8146675. [Google Scholar] [CrossRef]
- Gallant, Y.A.; Achterberg, A. Ultra-high-energy cosmic ray acceleration by relativistic blast waves. Mon. Not. R. Astron. Soc. 1999, 305, L6–L10. [Google Scholar] [CrossRef]
- Vieyro, F.L.; Romero, G.E. Particle transport in magnetized media around black holes and associated radiation. Astron. Astrophys. 2012, 542, A7. [Google Scholar] [CrossRef]
- Kelner, S.R.; Aharonian, F.A.; Bugayov, V.V. Energy spectra of gamma rays, electrons, and neutrinos produced at proton-proton interactions in the very high energy regime. Phys. Rev. D 2006, 74, 034018. [Google Scholar] [CrossRef]
- Papavasileiou, T.; Kosmas, O.; Sinatkas, I. Simulations of Neutrino and Gamma-Ray Production from Relativistic Black-Hole Microquasar Jets. Galaxies 2021, 9, 67. [Google Scholar] [CrossRef]
- García, F.; Karpouzas, K.; Méndez, M.; Zhang, L.; Zhang, Y.; Belloni, T.; Altamirano, D. The evolving properties of the corona of GRS 1915+105: A spectral-timing perspective through variable-Comptonization modelling. Mon. Not. R. Astron. Soc. 2022, 513, 4196–4207. [Google Scholar] [CrossRef]
- Shakura, N.I.; Sunyaev, R.A. Black holes in binary systems. Observational appearance. Astron. Astrophys. 1973, 24, 337–355. [Google Scholar]
- Eggleton, P.P. Aproximations to the radii of Roche lobes. Astrophys. J. 1983, 268, 368–369. [Google Scholar] [CrossRef]
- Done, C.; Gierliński, M.; Kubota, A. Modelling the behaviour of accretion flows in X-ray binaries. Astron. Astrophys. Rev. 2007, 15, 1–66. [Google Scholar] [CrossRef]
- Maitra, D.; Markoff, S.; Brocksopp, C.; Noble, M.; Nowak, M.; Wilms, J. Constraining jet/disc geometry and radiative processes in stellar black holes XTE J1118+480 and GX 339-4. Mon. Not. R. Astron. Soc. 2009, 398, 1638–1650. [Google Scholar] [CrossRef][Green Version]
- Fabrika, S. The jets and supercritical accretion disk in SS433. Astrophys. Space Phys. Rev. 2004, 12, 1–152. [Google Scholar]
- Stuchlík, Z.; Kološ, M. Controversy of the gro J1655-40 black hole mass and spin estimates and its possible solutions. Astrophys. J. 2016, 825, 13. [Google Scholar] [CrossRef]
- Reid, M.J.; McClintock, J.E.; Steiner, J.F.; Steeghs, D.; Remillard, R.A.; Dhawan, V.; Narayan, R. A parallax distance to the microquasar GRS 1915+ 105 and a revised estimate of its black hole mass. Astrophys. J. 2014, 796, 2. [Google Scholar] [CrossRef]
- Miller-Jones, J.C.A.; Bahramian, A.; Orosz, J.A.; Mandel, I.; Gou, L.; Maccarone, T.J.; Neijssel, C.J.; Zhao, X.; Ziółkowski, J.; Reid, M.J.; et al. Cygnus X-1 contains a 21–solar mass black hole—Implications for massive star winds. Science Science 2021, 371, 1046–1049. [Google Scholar] [CrossRef] [PubMed]
- Bowler, M.G. SS 433: Two robust determinations fix the mass ratio. Astron. Astrophys. 2018, 619, L4. [Google Scholar] [CrossRef]
- Orosz, J.A.; Steeghs, D.; McClintock, J.E.; Torres, M.A.P.; Bochkov, I.; Gou, L.; Narayan, R.; Blaschak, M.; Levine, A.M.; Remillard, R.A.; et al. A new dynamical model for the black hole binary LMC X-1. Astrophys. J. 2009, 697, 573–591. [Google Scholar] [CrossRef]
- Beer, M.E.; Podsiadlowski, P. The quiescent light curve and the evolutionary state of GRO J1655-40. Mon. Not. R. Astron. Soc. 2002, 331, 351–360. [Google Scholar] [CrossRef]
- Hjellming, R.M.; Rupen, M.P. Episodic ejection of relativistic jets by the X-ray transient GRO J1655-40. Nature 1995, 375, 464–468. [Google Scholar] [CrossRef]
- Romney, J.D.; Schilizzi, R.T.; Fejes, I.; Spencer, R.E. The Inner Beams of SS 433. Astrophys. J. 1987, 321, 822. [Google Scholar] [CrossRef]
- Bailyn, C.D.; Orosz, J.A.; McClintock, J.E.; Remillard, R.A. Dynamical evidence for a black hole in the eclipsing X-ray nova GRO J1655-40. Nature 1995, 378, 157–159. [Google Scholar] [CrossRef]
- Steeghs, D.; McClintock, J.E.; Parsons, S.G.; Reid, M.J.; Littlefair, S.; Dhillon, V.S. THE NOT-SO-MASSIVE BLACK HOLE IN THE MICROQUASAR GRS1915+105. Astrophys. J. 2013, 768, 185. [Google Scholar] [CrossRef]
- Brocksopp, C.; Fender, R.P.; Larionov, V.; Lyuty, V.M.; Tarasov, A.E.; Pooley, G.G.; Paciesas, W.S.; Roche, P. Orbital, precessional and flaring variability of Cygnus X-1. Mon. Not. R. Astron. Soc. 1999, 309, 1063–1073. [Google Scholar] [CrossRef]
- Cherepashchuk, A.M.; Belinski, A.A.; Dodin, A.V.; Postnov, K.A. Discovery of orbital eccentricity and evidence for orbital period increase of SS433. Mon. Not. R. Astron. Soc. Let 2021, 507, L19–L23. [Google Scholar] [CrossRef]
- Saikia, P.; Russell, D.M.; Bramich, D.M.; Miller-Jones, J.C.A.; Baglio, M.C.; Degenaar, N. Lorentz Factors of Compact Jets in Black Hole X-ray Binaries. Astrophys. J. 2019, 887, 21. [Google Scholar] [CrossRef]
- Koljonen, K.I.I.; Hovatta, T. ALMA/NICER observations of GRS 1915+105 indicate a return to a hard state. Astron. Astrophys. 2021, 647, A173. [Google Scholar] [CrossRef]
- Zdziarski, A.A.; Egron, E. What are the Composition and Power of the Jet in Cyg X-1? Astrophys. J. Lett. 2022, 935, L4. [Google Scholar] [CrossRef]
- Punsly, B.; Rodriguez, J. A temporal analysis indicates a mildly relativistic compact jet in GRS 1915+105. Astrophys. J. 2016, 823, 54. [Google Scholar] [CrossRef]
- Tetarenko, A.J.; Casella, P.; Miller-Jones, J.C.A.; Sivakoff, G.R.; Tetarenko, B.E.; Maccarone, T.J.; Gandhi, P.; Eikenberry, S. Radio frequency timing analysis of the compact jet in the black hole X-ray binary Cygnus X-1. Mon. Not. R. Astron. Soc. 2019, 484, 2987–3003. [Google Scholar] [CrossRef]
- Miller-Jones, J.C.A.; Fender, R.P.; Nakar, E. Opening angles, Lorentz factors and confinement of X-ray binary jets. Mon. Not. R. Astron. Soc. 2006, 367, 1432–1440. [Google Scholar] [CrossRef]
- Stirling, A.; Spencer, R.; de La Force, C.; Garrett, M.; Fender, R.; Ogley, R. A relativistic jet from Cygnus X-1 in the low/hard X-ray state. Mon. Not. R. Astron. Soc. 2001, 327, 1273–1278. [Google Scholar] [CrossRef]
- Cherepashchuk, A.M.; Sunyaev, R.A.; Fabrika, S.N.; Postnov, K.A.; Molkov, S.V.; Barsukova, E.A.; Antokhina, E.A.; Irsmambetova, T.R.; Panchenko, I.E.; Seifina, E.V.; et al. INTEGRAL observations of SS433: Results of a coordinated campaign. Astron. Astrophys. 2005, 437, 561–573. [Google Scholar] [CrossRef]
- Abdalla, H.; Abramowski, A.; Aharonian, F.; Benkhali, F.A.; Akhperjanian, A.G.; Angüner, E.O.; Arrieta, M.; Aubert, P.; Backes, M.; Balzer, A.; et al. A search for very high-energy flares from the microquasars GRS 1915+105, Circinus X-1, and V4641 Sgr using contemporaneous H.E.S.S. and RXTE observations. Astron. Astrophys. 2018, 612, A10. [Google Scholar] [CrossRef]
- Romero, G.E.; Okazaki, A.T.; Orellana, M.; Owocki, S.P. Accretion vs. colliding wind models for the gamma-ray binary LS I +61 303: An assessment. Astron. Astrophys. 2007, 474, 15–22. [Google Scholar] [CrossRef]
- Kantzas, D.; Markoff, S.; Beuchert, T.; Lucchini, M.; Chhotray, A.; Ceccobello, C.; Tetarenko, A.J.; Miller-Jones, J.C.A.; Bremer, M.; Garcia, J.A.; et al. A new lepto-hadronic model applied to the first simultaneous multiwavelength data set for Cygnus X–1. Mon. Not. R. Astron. Soc. 2020, 500, 2112–2126. [Google Scholar] [CrossRef]
- Punsly, B. Models of the compact jet in GRS 1915+105. Mon. Not. R. Astron. Soc. 2011, 418, 2736–2743. [Google Scholar] [CrossRef][Green Version]

| Free Parameter | Symbol | Definition | Value | Units |
|---|---|---|---|---|
| Acceleration region start | - | cm | ||
| Acceleration zone length factor | j | 5 | - | |
| Acceleration efficiency | - | |||
| Jet portion of relativistic matter | - | |||
| Hadron-to-lepton ratio | 1 | - | ||
| Mass accretion rate | - | /yr |
| Description | Symbol | GRO | GRS | Cygnus X-1 | SS 433 | LMC X-1 | Units |
|---|---|---|---|---|---|---|---|
| J1655-40 | 1915+105 | ||||||
| Black hole mass | 5.40 [53] | 12.40 [54] | 21.20 [55] | 15.00 [56] | 10.91 [57] | ||
| Companion stellar mass | 1.45 [58] | 0.50 [54] | 40.60 [55] | 21.00 [56] | 31.79 [57] | ||
| Distance | d | 3.2 [59] | 8.6 [54] | 2.2 [55] | 5.5 [60] | 48.0 [57] | kpc |
| Orbital period | 2.62 [61] | 33.85 [62] | 5.59 [63] | 13.08 [64] | 3.91 [57] | days | |
| Stellar luminosity | 8 | ||||||
| Stellar surface temperature | 6500 | 5000 | 31,138 | 8000 | 33,200 | K | |
| Inclination | i | 70.16 [65] | 60.00 [66] | 27.51 [67] | 78.80 [64] | 36.38 [57] | |
| Jet’s bulk velocity | 0.99 [65] | 0.43 [68] | 0.92 [69] | 0.26 [52] | 0.92 | - | |
| Jet’s half-opening angle | 3.1 [59] | 5.0 [70] | 1.2 [71] | 0.6 [72] | 3.0 |
| (), | GRO | GRS | Cygnus X-1 | SS 433 | LMC X-1 |
|---|---|---|---|---|---|
| J1655-40 | 1915+105 | ||||
| - | |||||
| (), | GRO | GRS | Cygnus X-1 | SS 433 | LMC X-1 |
| J1655-40 | 1915+105 | ||||
| Before absorption | |||||
| After absorption | |||||
| (), | GRO | GRS | Cygnus X-1 | SS 433 | LMC X-1 |
| J1655-40 | 1915+105 | ||||
| Before absorption | |||||
| After absorption | |||||
| (), | GRO | GRS | Cygnus X-1 | SS 433 | LMC X-1 |
| 99% | J1655-40 | 1915+105 | |||
| - | - | ||||
| () | 562 | 200 | 300 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Kosmas, O.; Papavasileiou, T.; Kosmas, T. Integral Fluxes of Neutrinos and Gamma-Rays Emitted from Neighboring X-ray Binaries. Universe 2023, 9, 517. https://doi.org/10.3390/universe9120517
Kosmas O, Papavasileiou T, Kosmas T. Integral Fluxes of Neutrinos and Gamma-Rays Emitted from Neighboring X-ray Binaries. Universe. 2023; 9(12):517. https://doi.org/10.3390/universe9120517
Chicago/Turabian StyleKosmas, Odysseas, Theodora Papavasileiou, and Theocharis Kosmas. 2023. "Integral Fluxes of Neutrinos and Gamma-Rays Emitted from Neighboring X-ray Binaries" Universe 9, no. 12: 517. https://doi.org/10.3390/universe9120517
APA StyleKosmas, O., Papavasileiou, T., & Kosmas, T. (2023). Integral Fluxes of Neutrinos and Gamma-Rays Emitted from Neighboring X-ray Binaries. Universe, 9(12), 517. https://doi.org/10.3390/universe9120517






