Dark Coincidences: Small-Scale Solutions with Refracted Gravity and MOND
Abstract
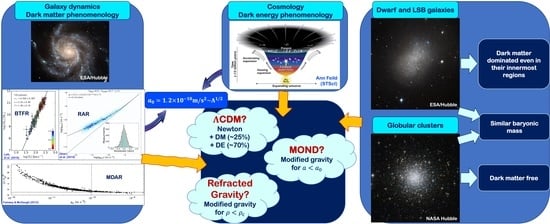
1. Introduction
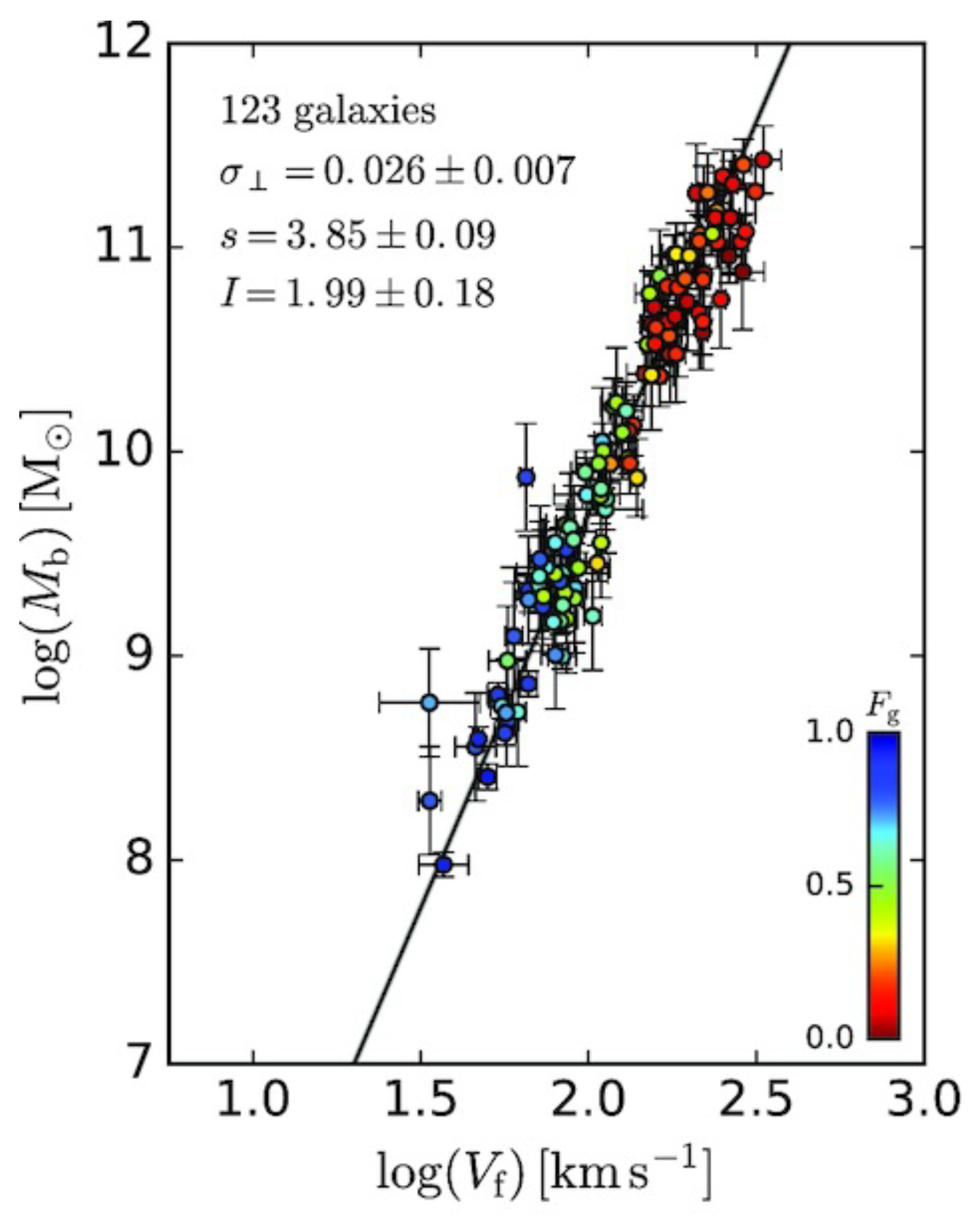
2. The Baryonic Scaling Relations
2.1. Description of the Three Relations
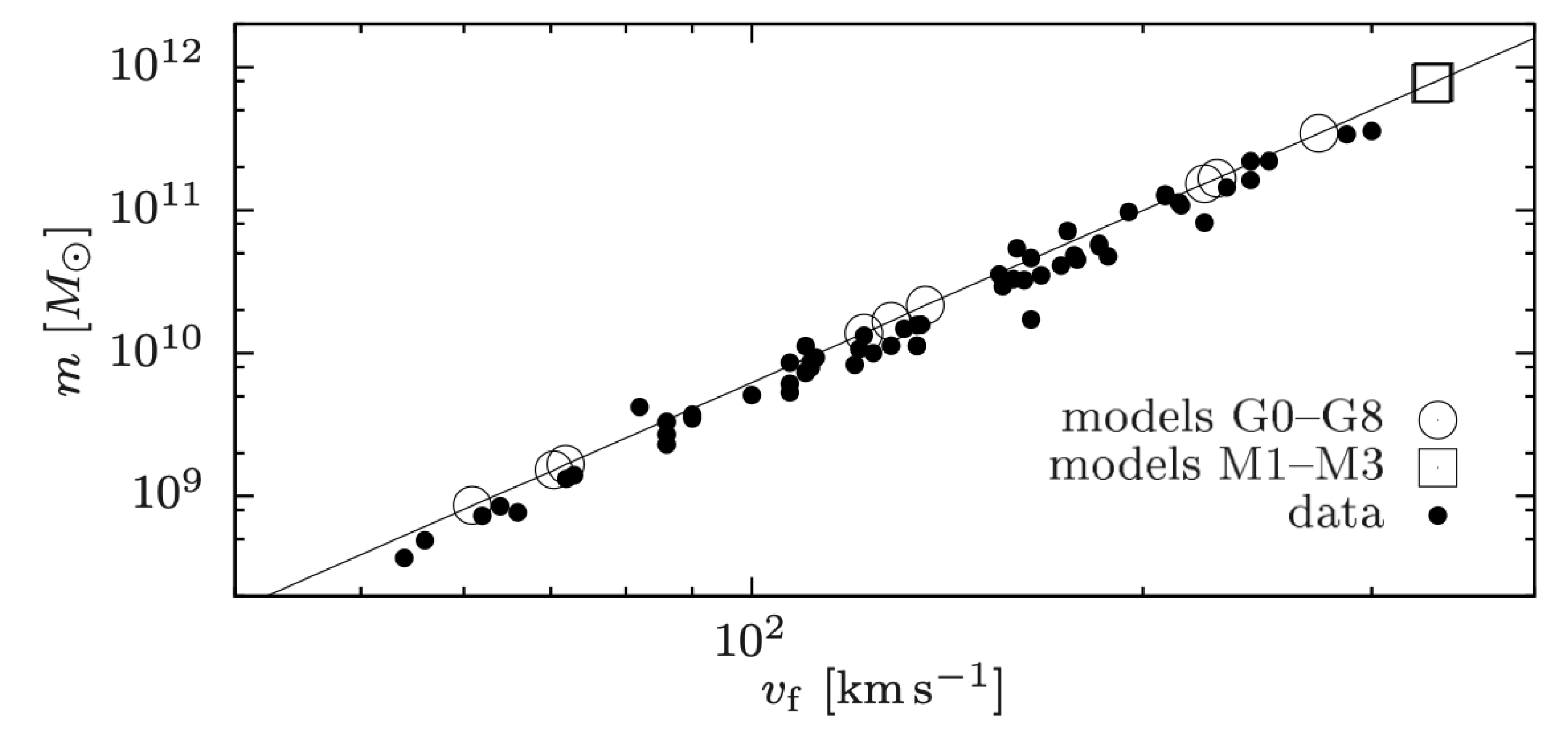
2.2. Interpretation of the Three Relations in Newtonian Gravity
3. MOND and RG
3.1. Summary of Theories Formulation
3.1.1. MOND
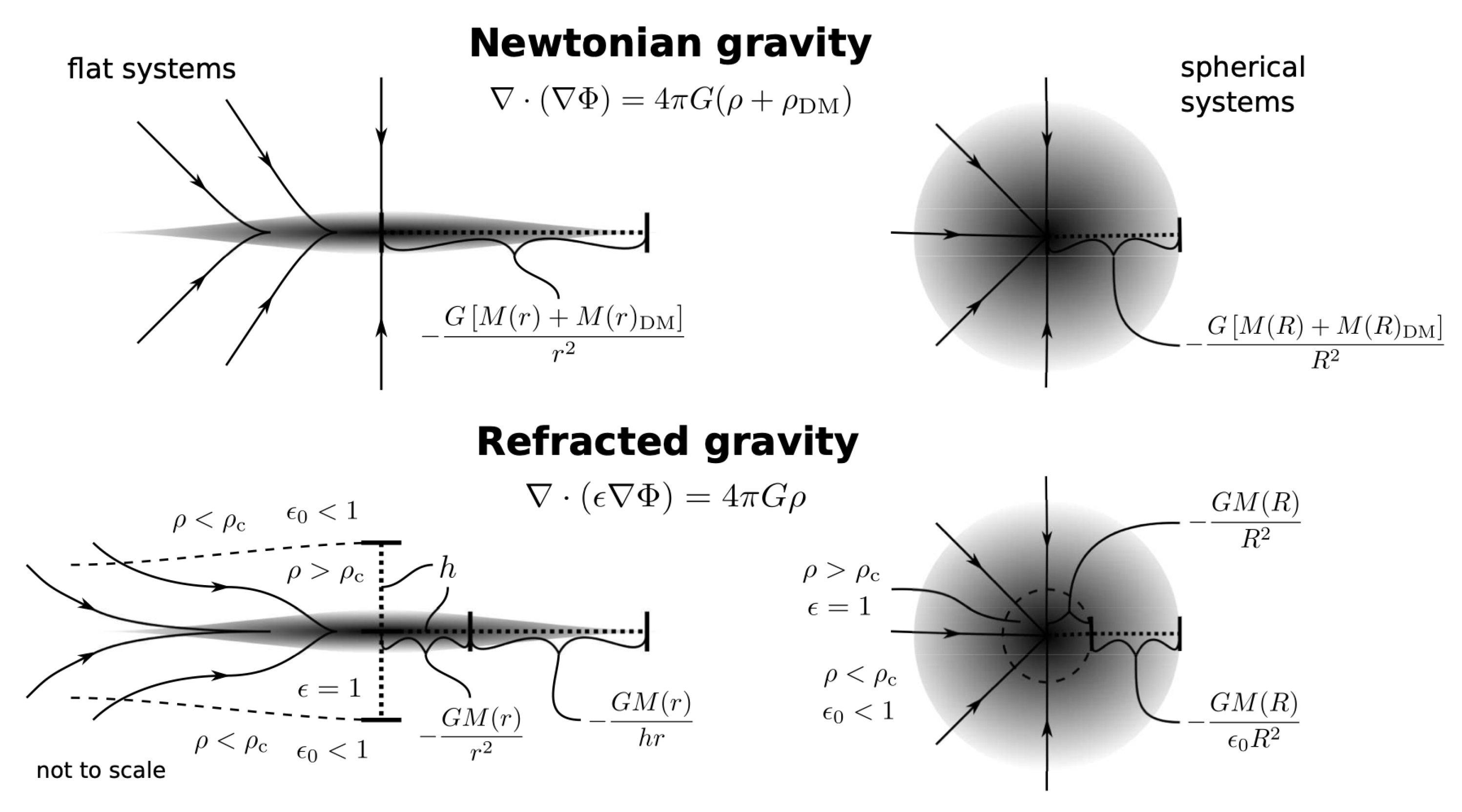
3.1.2. Refracted Gravity
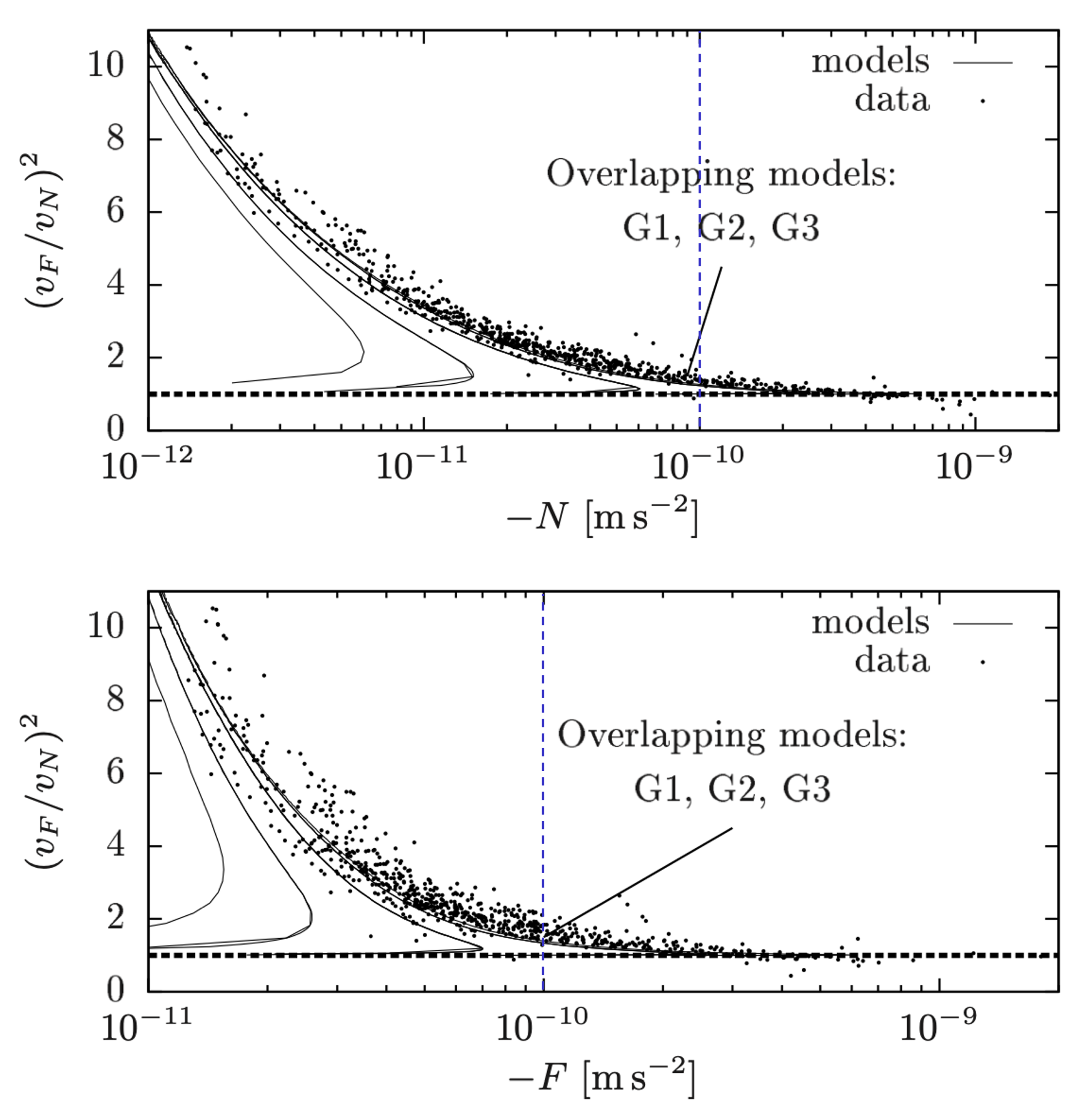
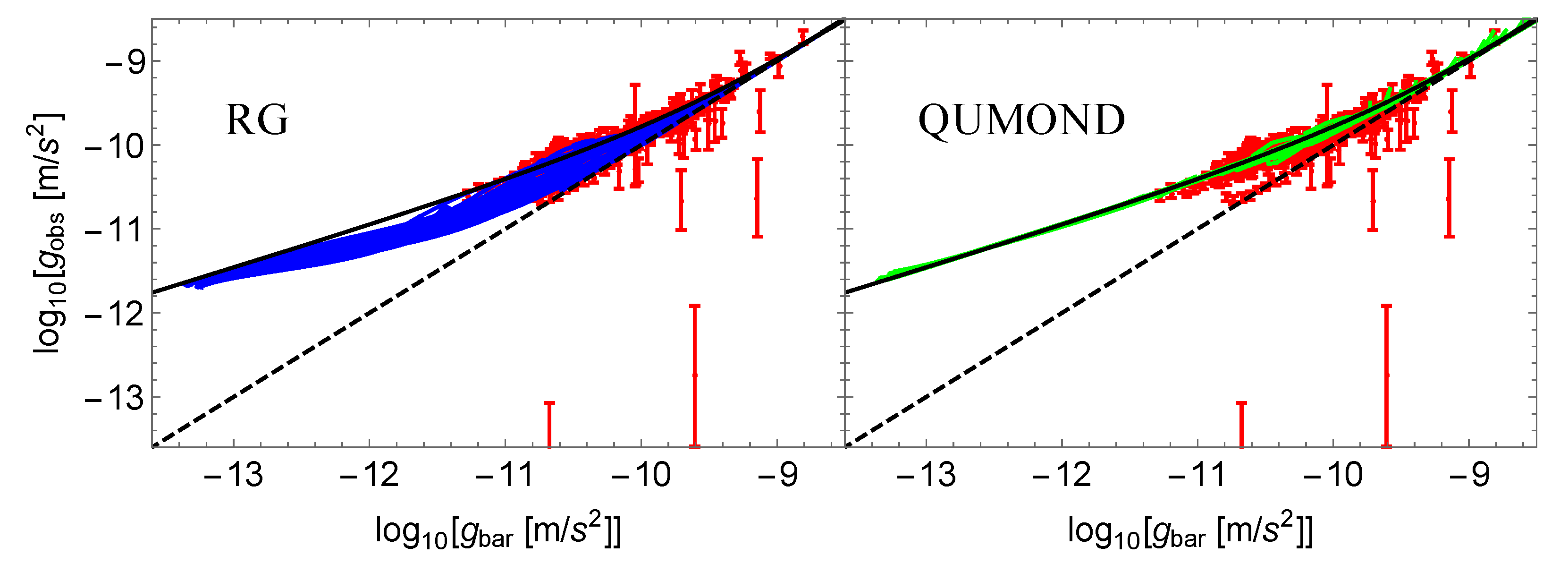
3.2. Interpretation of the Three Scaling Relations in MOND and RG
4. Possible Interpretations for an Intriguing Acceleration Scale
5. Dwarf Galaxies and Globular Clusters
6. Discussion and Conclusions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
Abbreviations
| AGN | Active Galactic Nuclei |
| BIMOND | bimetric MOND |
| BTFR | baryonic Tully–Fisher relation |
| CEG | Covariant Emergent Gravity |
| CMB | cosmic microwave background |
| CRG | covariant refracted gravity |
| DE | dark energy |
| DM | dark matter |
| DMS | DiskMass Survey |
| dSph | dwarf spheroidal |
| EH | Einstein-Hilbert |
| GCs | globular clusters |
| GR | General Relativity |
| HSB | high surface brightness |
| CDM | cold dark matter |
| LSB | low surface brightness |
| MDAR | mass discrepancy–acceleration relation |
| MOG | MOdified Gravity |
| MOND | MOdified Newtonian Dynamics |
| MW | Milky Way |
| NFW | Navarro Frenk White |
| RAR | radial acceleration relation |
| RG | Refracted Gravity |
| SPARC | Spitzer Photometry and Accurate Rotation Curves |
| SPS | stellar population synthesis |
| SRG | simplified refracted gravity |
| SVTG | Scalar-Vector-Tensor gravity |
| TeVeS | Tensor Vector Scalar gravity |
| WFL | weak field limit |
| WIMPs | weakly interacting massive particles |
| 1 | In fact, the gravitational sources depend on other scalar quantities besides the mass density, such as their total mechanical and thermodynamical energy or their entropy. Yet, these quantities depend in turn on the mass density and, thus, adopting a gravitational permittivity also dependent on these quantities would be likely to produce a phenomenology comparable to the one obtained with the simple dependence on the mass density alone [44,45]. |
References
- Aghanim, N. et al. [Planck Collaboration] Planck 2018 results. VI. Cosmological parameters. Astron. Astrophys. 2020, 641, A6. [Google Scholar] [CrossRef]
- Davis, M.; Efstathiou, G.; Frenk, C.S.; White, S.D.M. The evolution of large-scale structure in a universe dominated by cold dark matter. Astrophys. J. 1985, 292, 371–394. [Google Scholar] [CrossRef]
- Springel, V.; Frenk, C.S.; White, S.D.M. The large-scale structure of the Universe. Nature 2006, 440, 1137–1144. [Google Scholar] [CrossRef] [PubMed]
- Markevitch, M. Chandra Observation of the Most Interesting Cluster in the Universe. In The X-ray Universe 2005; Wilson, A., Ed.; ESA Special Publication: Washington, DC, USA, 2006; Volume 604, p. 723. [Google Scholar]
- Clowe, D.; Bradač, M.; Gonzalez, A.H.; Markevitch, M.; Randall, S.W.; Jones, C.; Zaritsky, D. A Direct Empirical Proof of the Existence of Dark Matter. Astrophys. J. 2006, 648, L109–L113. [Google Scholar] [CrossRef]
- Paraficz, D.; Kneib, J.P.; Richard, J.; Morandi, A.; Limousin, M.; Jullo, E.; Martinez, J. The Bullet cluster at its best: Weighing stars, gas, and dark matter. Astron. Astrophys. 2016, 594, A121. [Google Scholar] [CrossRef]
- Zwicky, F. Die Rotverschiebung von extragalaktischen Nebeln. Helv. Phys. Acta 1933, 6, 110–127. [Google Scholar]
- Rubin, V.C.; Ford, W.K., Jr. Rotation of the Andromeda Nebula from a Spectroscopic Survey of Emission Regions. Astrophys. J. 1970, 159, 379. [Google Scholar] [CrossRef]
- Bosma, A. The Distribution and Kinematics of Neutral Hydrogen in Spiral Galaxies of Various Morphological Types. Ph.D. Thesis, Rijksuniversiteit te Groningen, Groningen, The Netherlands, 1978. [Google Scholar]
- Sanders, R.H. Mass discrepancies in galaxies: Dark matter and alternatives. Astron. Astrophys. Rev. 1990, 2, 1–28. [Google Scholar] [CrossRef]
- Kirshner, R. Measuring the Universe with Supernovae. In Proceedings of the APS Meeting Abstracts, Vancouver, BC, Canada, 16–19 October 1996; p. F4.01. [Google Scholar]
- Peebles, P.J.; Ratra, B. The cosmological constant and dark energy. Rev. Mod. Phys. 2003, 75, 559–606. [Google Scholar] [CrossRef]
- Weinberg, S. The cosmological constant problem. Rev. Mod. Phys. 1989, 61, 1–23. [Google Scholar] [CrossRef]
- Luo, M.J. The cosmological constant problem and re-interpretation of time. Nucl. Phys. B 2014, 884, 344–356. [Google Scholar] [CrossRef]
- Velten, H.E.S.; vom Marttens, R.F.; Zimdahl, W. Aspects of the cosmological “coincidence problem”. Eur. Phys. J. C 2014, 74, 3160. [Google Scholar] [CrossRef]
- Fleury, P.; Dupuy, H.; Uzan, J.P. Can All Cosmological Observations Be Accurately Interpreted with a Unique Geometry? Phys. Rev. Lett. 2013, 111, 091302. [Google Scholar] [CrossRef] [PubMed]
- Douspis, M.; Salvati, L.; Aghanim, N. On the tension between Large Scale Structures and Cosmic Microwave Background. arXiv 2019, arXiv:1901.05289. [Google Scholar]
- Del Popolo, A.; Le Delliou, M. Small Scale Problems of the ΛCDM Model: A Short Review. Galaxies 2017, 5, 17. [Google Scholar] [CrossRef]
- de Martino, I.; Chakrabarty, S.S.; Cesare, V.; Gallo, A.; Ostorero, L.; Diaferio, A. Dark Matters on the Scale of Galaxies. Universe 2020, 6, 107. [Google Scholar] [CrossRef]
- McGaugh, S.S.; Schombert, J.M.; Bothun, G.D.; de Blok, W.J.G. The Baryonic Tully-Fisher Relation. Astrophys. J. 2000, 533, L99–L102. [Google Scholar] [CrossRef] [PubMed]
- McGaugh, S.S. The Mass Discrepancy-Acceleration Relation: Disk Mass and the Dark Matter Distribution. Astrophys. J. 2004, 609, 652–666. [Google Scholar] [CrossRef]
- McGaugh, S.S.; Lelli, F.; Schombert, J.M. Radial Acceleration Relation in Rotationally Supported Galaxies. Phys. Rev. Lett. 2016, 117, 201101. [Google Scholar] [CrossRef]
- Famaey, B.; McGaugh, S.S. Modified Newtonian Dynamics (MOND): Observational Phenomenology and Relativistic Extensions. Living Rev. Relativ. 2012, 15, 10. [Google Scholar] [CrossRef]
- van Albada, T.S.; Bahcall, J.N.; Begeman, K.; Sancisi, R. Distribution of dark matter in the spiral galaxy NGC 3198. Astrophys. J. 1985, 295, 305–313. [Google Scholar] [CrossRef]
- van Albada, T.S.; Sancisi, R. Dark Matter in Spiral Galaxies. Philos. Trans. R. Soc. Lond. Ser. A 1986, 320, 447–464. [Google Scholar] [CrossRef]
- Sackett, P.D. Does the Milky Way Have a Maximal Disk? Astrophys. J. 1997, 483, 103–110. [Google Scholar] [CrossRef]
- Courteau, S.; Rix, H.W. Maximal Disks and the Tully-Fisher Relation. Astrophys. J. 1999, 513, 561–571. [Google Scholar] [CrossRef]
- Bissantz, N.; Gerhard, O. Spiral arms, bar shape and bulge microlensing in the Milky Way. Mon. Not. R. Astron. Soc. 2002, 330, 591–608. [Google Scholar] [CrossRef]
- Sellwood, J.A.; Debattista, V.P. Re-interpretation of “Bar slowdown and the distribution of dark matter in barred galaxies” by Athanassoula. arXiv 2014, arXiv:1410.0834. [Google Scholar]
- McGaugh, S.S.; Schombert, J.M. Weighing Galaxy Disks With the Baryonic Tully-Fisher Relation. Astrophys. J. 2015, 802, 18. [Google Scholar] [CrossRef]
- Strigari, L.E.; Bullock, J.S.; Kaplinghat, M.; Simon, J.D.; Geha, M.; Willman, B.; Walker, M.G. A common mass scale for satellite galaxies of the Milky Way. Nature 2008, 454, 1096–1097. [Google Scholar] [CrossRef]
- Di Paolo, C.; Salucci, P.; Erkurt, A. The universal rotation curve of low surface brightness galaxies—IV. The interrelation between dark and luminous matter. Mon. Not. R. Astron. Soc. 2019, 490, 5451–5477. [Google Scholar] [CrossRef]
- Mateo, M.L. Dwarf Galaxies of the Local Group. Ann. Rev. Astron. Astrophys. 1998, 36, 435–506. [Google Scholar] [CrossRef]
- Baumgardt, H.; Grebel, E.K.; Kroupa, P. Using distant globular clusters as a test for gravitational theories. Mon. Not. R. Astron. Soc. 2005, 359, L1–L4. [Google Scholar] [CrossRef]
- Jordi, K.; Grebel, E.K.; Hilker, M.; Baumgardt, H.; Frank, M.; Kroupa, P.; Haghi, H.; Côté, P.; Djorgovski, S.G. Testing Fundamental Physics with Distant Star Clusters: Analysis of Observational Data on Palomar 14. Astron. J. 2009, 137, 4586–4596. [Google Scholar] [CrossRef]
- Baumgardt, H.; Côté, P.; Hilker, M.; Rejkuba, M.; Mieske, S.; Djorgovski, S.G.; Stetson, P. The velocity dispersion and mass-to-light ratio of the remote halo globular cluster NGC2419. Mon. Not. R. Astron. Soc. 2009, 396, 2051–2060. [Google Scholar] [CrossRef]
- Sollima, A.; Nipoti, C. Globular clusters in modified Newtonian dynamics: Velocity dispersion profiles from self-consistent models. Mon. Not. R. Astron. Soc. 2010, 401, 131–142. [Google Scholar] [CrossRef]
- Ibata, R.; Sollima, A.; Nipoti, C.; Bellazzini, M.; Chapman, S.C.; Dalessandro, E. The Globular Cluster NGC 2419: A Crucible for Theories of Gravity. Astrophys. J. 2011, 738, 186. [Google Scholar] [CrossRef]
- Ibata, R.; Sollima, A.; Nipoti, C.; Bellazzini, M.; Chapman, S.C.; Dalessandro, E. Polytropic Model Fits to the Globular Cluster NGC 2419 in Modified Newtonian Dynamics. Astrophys. J. 2011, 743, 43. [Google Scholar] [CrossRef]
- Frank, M.J.; Hilker, M.; Baumgardt, H.; Côté, P.; Grebel, E.K.; Haghi, H.; Küpper, A.H.W.; Djorgovski, S.G. The velocity dispersion and mass function of the outer halo globular cluster Palomar 4. Mon. Not. R. Astron. Soc. 2012, 423, 2917–2932. [Google Scholar] [CrossRef]
- Milgrom, M. A modification of the Newtonian dynamics as a possible alternative to the hidden mass hypothesis. Astrophys. J. 1983, 270, 365–370. [Google Scholar] [CrossRef]
- Milgrom, M. A modification of the Newtonian dynamics—Implications for galaxies. Astrophys. J. 1983, 270, 371–383. [Google Scholar] [CrossRef]
- Milgrom, M. A modification of the newtonian dynamics: Implications for galaxy systems. Astrophys. J. 1983, 270, 384–389. [Google Scholar] [CrossRef]
- Matsakos, T.; Diaferio, A. Dynamics of galaxies and clusters in refracted gravity. arXiv 2016, arXiv:1603.04943. [Google Scholar]
- Cesare, V.; Diaferio, A.; Matsakos, T.; Angus, G. Dynamics of DiskMass Survey galaxies in refracted gravity. Astron. Astrophys. 2020, 637, A70. [Google Scholar] [CrossRef]
- Cesare, V.; Diaferio, A.; Matsakos, T. The dynamics of three nearby E0 galaxies in refracted gravity. Astron. Astrophys. 2022, 657, A133. [Google Scholar] [CrossRef]
- Sanna, A.P.; Matsakos, T.; Diaferio, A. Covariant Formulation of refracted gravity. arXiv 2021, arXiv:2109.11217. [Google Scholar]
- McGaugh, S.S. The Baryonic Tully-Fisher Relation of Gas-rich Galaxies as a Test of ΛCDM and MOND. Astron. J. 2012, 143, 40. [Google Scholar] [CrossRef]
- Lelli, F.; McGaugh, S.S.; Schombert, J.M. The Small Scatter of the Baryonic Tully-Fisher Relation. Astrophys. J. 2016, 816, L14. [Google Scholar] [CrossRef]
- Desmond, H.; Wechsler, R.H. The Tully-Fisher and mass-size relations from halo abundance matching. Mon. Not. R. Astron. Soc. 2015, 454, 322–343. [Google Scholar] [CrossRef]
- Lelli, F.; McGaugh, S.S.; Schombert, J.M. SPARC: Mass Models for 175 Disk Galaxies with Spitzer Photometry and Accurate Rotation Curves. Astron. J. 2016, 152, 157. [Google Scholar] [CrossRef]
- Lelli, F.; McGaugh, S.S.; Schombert, J.M.; Desmond, H.; Katz, H. The baryonic Tully-Fisher relation for different velocity definitions and implications for galaxy angular momentum. Mon. Not. R. Astron. Soc. 2019, 484, 3267–3278. [Google Scholar] [CrossRef]
- Lelli, F.; McGaugh, S.S.; Schombert, J.M.; Pawlowski, M.S. One Law to Rule Them All: The Radial Acceleration Relation of Galaxies. Astrophys. J. 2017, 836, 152. [Google Scholar] [CrossRef]
- Mayer, A.C.; Teklu, A.F.; Dolag, K.; Remus, R.S. ΛCDM with baryons versus MOND: The time evolution of the universal acceleration scale in the Magneticum simulations. Mon. Not. R. Astron. Soc. 2023, 518, 257–269. [Google Scholar] [CrossRef]
- Milgrom, M. MOND impact on and of the recently updated mass-discrepancy-acceleration relation. arXiv 2016, arXiv:1609.06642. [Google Scholar]
- Li, P.; Lelli, F.; McGaugh, S.; Schombert, J. Fitting the radial acceleration relation to individual SPARC galaxies. Astron. Astrophys. 2018, 615, A3. [Google Scholar] [CrossRef]
- Ghari, A.; Haghi, H.; Zonoozi, A.H. The radial acceleration relation and dark baryons in MOND. Mon. Not. R. Astron. Soc. 2019, 487, 2148–2165. [Google Scholar] [CrossRef]
- Santos-Santos, I.M.E.; Navarro, J.F.; Robertson, A.; Benítez-Llambay, A.; Oman, K.A.; Lovell, M.R.; Frenk, C.S.; Ludlow, A.D.; Fattahi, A.; Ritz, A. Baryonic clues to the puzzling diversity of dwarf galaxy rotation curves. Mon. Not. R. Astron. Soc. 2020, 495, 58–77. [Google Scholar] [CrossRef]
- McGaugh, S.S.; Li, P.; Lelli, F.; Schombert, J.M. Presence of a fundamental acceleration scale in galaxies. Nat. Astron. 2018, 2, 924. [Google Scholar] [CrossRef]
- Kroupa, P.; Banik, I.; Haghi, H.; Zonoozi, A.H.; Dabringhausen, J.; Javanmardi, B.; Müller, O.; Wu, X.; Zhao, H. A common Milgromian acceleration scale in nature. Nat. Astron. 2018, 2, 925–926. [Google Scholar] [CrossRef]
- Rodrigues, D.C.; Marra, V.; del Popolo, A.; Davari, Z. Absence of a fundamental acceleration scale in galaxies. Nat. Astron. 2018, 2, 668–672. [Google Scholar] [CrossRef]
- Walter, F.; Brinks, E.; de Blok, W.J.G.; Bigiel, F.; Kennicutt, R.C., Jr.; Thornley, M.D.; Leroy, A. THINGS: The H I Nearby Galaxy Survey. Astron. J. 2008, 136, 2563–2647. [Google Scholar] [CrossRef]
- de Blok, W.J.G.; Walter, F.; Brinks, E.; Trachternach, C.; Oh, S.H.; Kennicutt, R.C.J. High-Resolution Rotation Curves and Galaxy Mass Models from THINGS. Astron. J. 2008, 136, 2648–2719. [Google Scholar] [CrossRef]
- Marra, V.; Rodrigues, D.C.; de Almeida, Á.O.F. A fundamental test for MOND. Mon. Not. R. Astron. Soc. 2020, 494, 2875–2885. [Google Scholar] [CrossRef]
- Zhou, Y.; Del Popolo, A.; Chang, Z. On the absence of a universal surface density, and a maximum Newtonian acceleration in dark matter haloes: Consequences for MOND. Phys. Dark Universe 2020, 28, 100468. [Google Scholar] [CrossRef]
- Rodrigues, D.C.; Marra, V.; Del Popolo, A.; Davari, Z. Reply to ‘Presence of a fundamental acceleration scale in galaxies’ and ‘A common Milgromian acceleration scale in nature’. Nat. Astron. 2018, 2, 927–929. [Google Scholar] [CrossRef]
- Edmonds, D.; Minic, D.; Takeuchi, T. Presence of a Fundamental Acceleration Scale in Galaxy Clusters. arXiv 2020, arXiv:2009.12915. [Google Scholar]
- Dutton, A.A. The baryonic Tully-Fisher relation and galactic outflows. Mon. Not. R. Astron. Soc. 2012, 424, 3123–3128. [Google Scholar] [CrossRef]
- Di Cintio, A.; Lelli, F. The mass discrepancy acceleration relation in a ΛCDM context. Mon. Not. R. Astron. Soc. 2016, 456, L127–L131. [Google Scholar] [CrossRef]
- Ludlow, A.D.; Benítez-Llambay, A.; Schaller, M.; Theuns, T.; Frenk, C.S.; Bower, R.; Schaye, J.; Crain, R.A.; Navarro, J.F.; Fattahi, A.; et al. Mass-Discrepancy Acceleration Relation: A Natural Outcome of Galaxy Formation in Cold Dark Matter Halos. Phys. Rev. Lett. 2017, 118, 161103. [Google Scholar] [CrossRef]
- Keller, B.W.; Wadsley, J.W. ΛCDM is Consistent with SPARC Radial Acceleration Relation. Astrophys. J. 2017, 835, L17. [Google Scholar] [CrossRef]
- Stone, C.; Courteau, S. The Intrinsic Scatter of the Radial Acceleration Relation. Astrophys. J. 2019, 882, 6. [Google Scholar] [CrossRef]
- Di Paolo, C.; Salucci, P.; Fontaine, J.P. The Radial Acceleration Relation (RAR): Crucial Cases of Dwarf Disks and Low-surface-brightness Galaxies. Astrophys. J. 2019, 873, 106. [Google Scholar] [CrossRef]
- Ferrero, I.; Navarro, J.F.; Abadi, M.G.; Sales, L.V.; Bower, R.G.; Crain, R.A.; Frenk, C.S.; Schaller, M.; Schaye, J.; Theuns, T. Size matters: Abundance matching, galaxy sizes, and the Tully-Fisher relation in EAGLE. Mon. Not. R. Astron. Soc. 2017, 464, 4736–4746. [Google Scholar] [CrossRef]
- Ferrero, I.; Abadi, M.G. Redshift evolution of Tully-Fisher relation. In Formation and Evolution of Galaxy Outskirts; Gil de Paz, A., Knapen, J.H., Lee, J.C., Eds.; Cambridge University Press: Cambridge, UK, 2017; Volume 321, p. 126. [Google Scholar] [CrossRef]
- Navarro, J.F.; Benítez-Llambay, A.; Fattahi, A.; Frenk, C.S.; Ludlow, A.D.; Oman, K.A.; Schaller, M.; Theuns, T. The origin of the mass discrepancy-acceleration relation in ΛCDM. Mon. Not. R. Astron. Soc. 2017, 471, 1841–1848. [Google Scholar] [CrossRef]
- Dutton, A.A.; Macciò, A.V.; Obreja, A.; Buck, T. NIHAO-XVIII. Origin of the MOND phenomenology of galactic rotation curves in a ΛCDM universe. Mon. Not. R. Astron. Soc. 2019, 485, 1886–1899. [Google Scholar] [CrossRef]
- Bekenstein, J.; Milgrom, M. Does the missing mass problem signal the breakdown of Newtonian gravity? Astrophys. J. 1984, 286, 7–14. [Google Scholar] [CrossRef]
- Milgrom, M. Dynamics with a Nonstandard Inertia-Acceleration Relation: An Alternative to Dark Matter in Galactic Systems. Ann. Phys. 1994, 229, 384–415. [Google Scholar] [CrossRef]
- Milgrom, M. Quasi-linear formulation of MOND. Mon. Not. R. Astron. Soc. 2010, 403, 886–895. [Google Scholar] [CrossRef]
- Bekenstein, J.D. Phase coupling gravitation: Symmetries and gauge fields. Phys. Lett. B 1988, 202, 497–500. [Google Scholar] [CrossRef]
- Sanders, R.H. Phase coupling gravity and astronomical mass discrepancies. Mon. Not. R. Astron. Soc. 1988, 235, 105–121. [Google Scholar] [CrossRef]
- Skordis, C. TOPICAL REVIEW: The tensor-vector-scalar theory and its cosmology. Class. Quantum Gravity 2009, 26, 143001. [Google Scholar] [CrossRef]
- Bekenstein, J.D. Tensor-vector-scalar-modified gravity: From small scale to cosmology. Philos. Trans. R. Soc. Lond. Ser. A 2011, 369, 5003–5017. [Google Scholar] [CrossRef]
- Brada, R.; Milgrom, M. Exact solutions and approximations of MOND fields of disc galaxies. Mon. Not. R. Astron. Soc. 1995, 276, 453–459. [Google Scholar] [CrossRef]
- Eriksen, M.H.; Frandsen, M.T.; From, M.H. A Cusp-Core like challenge for Modified Newtonian Dynamics. arXiv 2019, arXiv:1906.07823. [Google Scholar] [CrossRef]
- McGaugh, S.S. The Baryonic Tully-Fisher Relation of Galaxies with Extended Rotation Curves and the Stellar Mass of Rotating Galaxies. Astrophys. J. 2005, 632, 859–871. [Google Scholar] [CrossRef]
- Bershady, M.A.; Verheijen, M.A.W.; Swaters, R.A.; Andersen, D.R.; Westfall, K.B.; Martinsson, T. The DiskMass Survey. I. Overview. Astrophys. J. 2010, 716, 198–233. [Google Scholar] [CrossRef]
- Angus, G.W.; Gentile, G.; Swaters, R.; Famaey, B.; Diaferio, A.; McGaugh, S.S.; Heyden, K.J.v.d. Mass models of disc galaxies from the DiskMass Survey in modified Newtonian dynamics. Mon. Not. R. Astron. Soc. 2015, 451, 3551–3580. [Google Scholar] [CrossRef]
- Bershady, M.A.; Verheijen, M.A.W.; Westfall, K.B.; Andersen, D.R.; Swaters, R.A.; Martinsson, T. The DiskMass Survey. II. Error Budget. Astrophys. J. 2010, 716, 234–268. [Google Scholar] [CrossRef]
- Bekenstein, J.D.; Sagi, E. Do Newton’s G and Milgrom’s a0 vary with cosmological epoch? Phys. Rev. D 2008, 77, 103512. [Google Scholar] [CrossRef]
- Bekenstein, J.D. Relativistic gravitation theory for the modified Newtonian dynamics paradigm. Phys. Rev. D 2004, 70, 083509. [Google Scholar] [CrossRef]
- Hossenfelder, S. Covariant version of Verlinde’s emergent gravity. Phys. Rev. D 2017, 95, 124018. [Google Scholar] [CrossRef]
- Hossenfelder, S.; Mistele, T. The redshift-dependence of radial acceleration: Modified gravity versus particle dark matter. Int. J. Mod. Phys. D 2018, 27, 1847010. [Google Scholar] [CrossRef]
- Milgrom, M. The a0—Cosmology connection in MOND. arXiv 2020, arXiv:2001.09729. [Google Scholar]
- Milgrom, M. Alternatives to Dark Matter. Comments Astrophys. 1989, 13, 215. [Google Scholar]
- Milgrom, M. The modified dynamics as a vacuum effect. Phys. Lett. A 1999, 253, 273–279. [Google Scholar] [CrossRef]
- Zlosnik, T.G.; Ferreira, P.G.; Starkman, G.D. Modifying gravity with the aether: An alternative to dark matter. Phys. Rev. D 2007, 75, 044017. [Google Scholar] [CrossRef]
- Milgrom, M. Bimetric MOND gravity. Phys. Rev. D 2009, 80, 123536. [Google Scholar] [CrossRef]
- Blanchet, L.; Heisenberg, L. Dipolar dark matter as an effective field theory. Phys. Rev. D 2017, 96, 083512. [Google Scholar] [CrossRef]
- Milgrom, M. Noncovariance at low accelerations as a route to MOND. Phys. Rev. D 2019, 100, 084039. [Google Scholar] [CrossRef]
- Milgrom, M. MOND from a Brane-World Picture; World Scientific Publishing: Singapore, 2020. [Google Scholar] [CrossRef]
- Khoury, J.; Weltman, A. Chameleon cosmology. Phys. Rev. D 2004, 69, 044026. [Google Scholar] [CrossRef]
- Kunz, M.; Liddle, A.R.; Parkinson, D.; Gao, C. Constraining the dark fluid. Phys. Rev. D 2009, 80, 083533. [Google Scholar] [CrossRef]
- Kunz, M. Degeneracy between the dark components resulting from the fact that gravity only measures the total energy-momentum tensor. Phys. Rev. D 2009, 80, 123001. [Google Scholar] [CrossRef]
- Mannheim, P.D.; Kazanas, D. Exact Vacuum Solution to Conformal Weyl Gravity and Galactic Rotation Curves. Astrophys. J. 1989, 342, 635. [Google Scholar] [CrossRef]
- Nesbet, R. Conformal Gravity: Dark Matter and Dark Energy. Entropy 2013, 15, 162–176. [Google Scholar] [CrossRef]
- Campigotto, M.C.; Diaferio, A.; Fatibene, L. Conformal gravity: Light deflection revisited and the galactic rotation curve failure. Class. Quantum Gravity 2019, 36, 245014. [Google Scholar] [CrossRef]
- Makler, M.; de Oliveira, S.Q.; Waga, I. Constraints on the generalized Chaplygin gas from supernovae observations. Phys. Lett. B 2003, 555, 1–6. [Google Scholar] [CrossRef]
- Brandenberger, R.; Cuzinatto, R.R.; Fröhlich, J.; Namba, R. New scalar field quartessence. J. Cosmol. Astropart. Phys. 2019, 2019, 043. [Google Scholar] [CrossRef]
- Ferreira, E.G.M.; Franzmann, G.; Khoury, J.; Brandenberger, R. Unified superfluid dark sector. J. Cosmol. Astropart. Phys. 2019, 2019, 027. [Google Scholar] [CrossRef]
- Bento, M.C.; Bertolami, O.; Sen, A.A. Generalized Chaplygin gas, accelerated expansion, and dark-energy-matter unification. Phys. Rev. D 2002, 66, 043507. [Google Scholar] [CrossRef]
- Carturan, D.; Finelli, F. Cosmological effects of a class of fluid dark energy models. Phys. Rev. D 2003, 68, 103501. [Google Scholar] [CrossRef]
- Sandvik, H.B.; Tegmark, M.; Zaldarriaga, M.; Waga, I. The end of unified dark matter? Phys. Rev. D 2004, 69, 123524. [Google Scholar] [CrossRef]
- Scherrer, R.J. Purely Kinetic k Essence as Unified Dark Matter. Phys. Rev. Lett. 2004, 93, 011301. [Google Scholar] [CrossRef]
- Giannakis, D.; Hu, W. Challenges for the kinetic unified dark matter model. Phys. Rev. D 2005, 72, 063502. [Google Scholar] [CrossRef]
- Bruni, M.; Lazkoz, R.; Rozas-Fernández, A. Phenomenological models for unified dark matter with fast transition. Mon. Not. R. Astron. Soc. 2013, 431, 2907–2916. [Google Scholar] [CrossRef]
- Leanizbarrutia, I.; Rozas-Fernández, A.; Tereno, I. Cosmological constraints on a unified dark matter-energy scalar field model with fast transition. Phys. Rev. D 2017, 96, 023503. [Google Scholar] [CrossRef]
- Cadoni, M.; Casadio, R.; Giusti, A.; Mück, W.; Tuveri, M. Effective fluid description of the dark universe. Phys. Lett. B 2018, 776, 242–248. [Google Scholar] [CrossRef]
- Cadoni, M.; Casadio, R.; Giusti, A.; Tuveri, M. Emergence of a dark force in corpuscular gravity. Phys. Rev. D 2018, 97, 044047. [Google Scholar] [CrossRef]
- Alexander, S.; Biswas, T.; Calcagni, G. Cosmological Bardeen-Cooper-Schrieffer condensate as dark energy. Phys. Rev. D 2010, 81, 043511. [Google Scholar] [CrossRef]
- Sotiriou, T.P.; Faraoni, V. f(R) theories of gravity. Rev. Mod. Phys. 2010, 82, 451–497. [Google Scholar] [CrossRef]
- Sebastiani, L.; Vagnozzi, S.; Myrzakulov, R. Mimetic gravity: A review of recent developments and applications to cosmology and astrophysics. arXiv 2016, arXiv:1612.08661. [Google Scholar] [CrossRef]
- Berezhiani, L.; Khoury, J.; Wang, J. Universe without dark energy: Cosmic acceleration from dark matter-baryon interactions. Phys. Rev. D 2017, 95, 123530. [Google Scholar] [CrossRef]
- Arbey, A.; Coupechoux, J.F. Unifying dark matter, dark energy and inflation with a fuzzy dark fluid. J. Cosmol. Astropart. Phys. 2021, 2021, 033. [Google Scholar] [CrossRef]
- McConnachie, A.W. The Observed Properties of Dwarf Galaxies in and around the Local Group. Astron. J. 2012, 144, 4. [Google Scholar] [CrossRef]
- Javanmardi, B.; Martinez-Delgado, D.; Kroupa, P.; Henkel, C.; Crawford, K.; Teuwen, K.; Gabany, R.J.; Hanson, M.; Chonis, T.S.; Neyer, F. DGSAT: Dwarf Galaxy Survey with Amateur Telescopes. I. Discovery of low surface brightness systems around nearby spiral galaxies. Astron. Astrophys. 2016, 588, A89. [Google Scholar] [CrossRef]
- Mateo, M.; Olszewski, E.W.; Pryor, C.; Welch, D.L.; Fischer, P. The Carina Dwarf Spheroidal Galaxy: How Dark is it? Astron. J. 1993, 105, 510. [Google Scholar] [CrossRef]
- Navarro, J.F.; Frenk, C.S.; White, S.D.M. The Structure of Cold Dark Matter Halos. Astrophys. J. 1996, 462, 563. [Google Scholar] [CrossRef]
- Dubinski, J.; Carlberg, R.G. The Structure of Cold Dark Matter Halos. Astrophys. J. 1991, 378, 496. [Google Scholar] [CrossRef]
- Torrealba, G.; Belokurov, V.; Koposov, S.E.; Li, T.S.; Walker, M.G.; Sanders, J.L.; Geringer-Sameth, A.; Zucker, D.B.; Kuehn, K.; Evans, N.W.; et al. The hidden giant: Discovery of an enormous Galactic dwarf satellite in Gaia DR2. Mon. Not. R. Astron. Soc. 2019, 488, 2743–2766. [Google Scholar] [CrossRef]
- Helmi, A. et al. [Gaia Collaboration] Gaia Data Release 2. Kinematics of globular clusters and dwarf galaxies around the Milky Way. Astron. Astrophys. 2018, 616, A12. [Google Scholar] [CrossRef]
- Giersz, M. Monte Carlo simulations of star clusters—III. A million-body star cluster. Mon. Not. R. Astron. Soc. 2006, 371, 484–494. [Google Scholar] [CrossRef]
- Mashchenko, S.; Sills, A. Globular Clusters with Dark Matter Halos. I. Initial Relaxation. Astrophys. J. 2005, 619, 243–257. [Google Scholar] [CrossRef]
- Moore, B. Constraints on the Global Mass-to-Light Ratios and on the Extent of Dark Matter Halos in Globular Clusters and Dwarf Spheroidals. Astrophys. J. 1996, 461, L13. [Google Scholar] [CrossRef]
- Forbes, D.A.; Lasky, P.; Graham, A.W.; Spitler, L. Uniting old stellar systems: From globular clusters to giant ellipticals. Mon. Not. R. Astron. Soc. 2008, 389, 1924–1936. [Google Scholar] [CrossRef]
- Deur, A. A relation between the dark mass of elliptical galaxies and their shape. Mon. Not. R. Astron. Soc. 2014, 438, 1535–1551. [Google Scholar] [CrossRef]
- Deur, A. A correlation between the dark content of elliptical galaxies and their ellipticity. arXiv 2020, arXiv:2010.06692. [Google Scholar]
- Cesare, V. Dynamics of Disk and Elliptical Galaxies in Refracted Gravity. Phys. Sci. Forum 2021, 2, 9292. [Google Scholar] [CrossRef]
- Tanabashi, M.; Hagiwara, K.; Hikasa, K.; Nakamura, K.; Sumino, Y.; Takahashi, F.; Tanaka, J.; Agashe, K.; Aielli, G.; Amsler, C.; et al. Review of Particle Physics*. Phys. Rev. D 2018, 98, 030001. [Google Scholar] [CrossRef]
- Sanders, R.H. The Virial Discrepancy in Clusters of Galaxies in the Context of Modified Newtonian Dynamics. Astrophys. J. 1999, 512, L23–L26. [Google Scholar] [CrossRef]
- Sanders, R.H. Clusters of galaxies with modified Newtonian dynamics. Mon. Not. R. Astron. Soc. 2003, 342, 901–908. [Google Scholar] [CrossRef]
- Hodson, A.O.; Zhao, H. Generalizing MOND to explain the missing mass in galaxy clusters. Astron. Astrophys. 2017, 598, A127. [Google Scholar] [CrossRef]
- Hernandez, X.; Sussman, R.A.; Nasser, L. Approaching the Dark Sector through a bounding curvature criterion. Mon. Not. R. Astron. Soc. 2019, 483, 147–151. [Google Scholar] [CrossRef]
- Skordis, C.; Złośnik, T. New Relativistic Theory for Modified Newtonian Dynamics. Phys. Rev. Lett. 2021, 127, 161302. [Google Scholar] [CrossRef]
- Forbes, D.A.; Alabi, A.; Brodie, J.P.; Romanowsky, A.J.; Strader, J.; Foster, C.; Usher, C.; Spitler, L.; Bellstedt, S.; Pastorello, N.; et al. The SLUGGS Survey: A Catalog of Over 4000 Globular Cluster Radial Velocities in 27 Nearby Early-type Galaxies. Astron. J. 2017, 153, 114. [Google Scholar] [CrossRef]
- Pulsoni, C.; Gerhard, O.; Arnaboldi, M.; Coccato, L.; Longobardi, A.; Napolitano, N.R.; Moylan, E.; Narayan, C.; Gupta, V.; Burkert, A.; et al. The extended Planetary Nebula Spectrograph (ePN.S) early-type galaxy survey: The kinematic diversity of stellar halos and the relation between halo transition scale and stellar mass. Astron. Astrophys. 2018, 618, A94. [Google Scholar] [CrossRef]
- Salucci, P.; Wilkinson, M.I.; Walker, M.G.; Gilmore, G.F.; Grebel, E.K.; Koch, A.; Frigerio Martins, C.; Wyse, R.F.G. Dwarf spheroidal galaxy kinematics and spiral galaxy scaling laws. Mon. Not. R. Astron. Soc. 2012, 420, 2034–2041. [Google Scholar] [CrossRef]
- Oh, S.H.; Hunter, D.A.; Brinks, E.; Elmegreen, B.G.; Schruba, A.; Walter, F.; Rupen, M.P.; Young, L.M.; Simpson, C.E.; Johnson, M.C.; et al. High-resolution Mass Models of Dwarf Galaxies from LITTLE THINGS. Astron. J. 2015, 149, 180. [Google Scholar] [CrossRef]
- Rines, K.; Diaferio, A. CIRS: Cluster Infall Regions in the Sloan Digital Sky Survey. I. Infall Patterns and Mass Profiles. Astron. J. 2006, 132, 1275–1297. [Google Scholar] [CrossRef]
- Rines, K.; Geller, M.J.; Diaferio, A.; Kurtz, M.J. Measuring the Ultimate Halo Mass of Galaxy Clusters: Redshifts and Mass Profiles from the Hectospec Cluster Survey (HeCS). Astrophys. J. 2013, 767, 15. [Google Scholar] [CrossRef]
- Moffat, J.W. Scalar tensor vector gravity theory. J. Cosmol. Astropart. Phys. 2006, 2006, 004. [Google Scholar] [CrossRef]
- Moffat, J.W. A new nonsymmetric gravitational theory. Phys. Lett. B 1995, 355, 447–452. [Google Scholar] [CrossRef]
- Moffat, J.W. Gravitational theory, galaxy rotation curves and cosmology without dark matter. J. Cosmol. Astropart. Phys. 2005, 2005, 003. [Google Scholar] [CrossRef]
- Brownstein, J.R.; Moffat, J.W. Galaxy Rotation Curves without Nonbaryonic Dark Matter. Astrophys. J. 2006, 636, 721–741. [Google Scholar] [CrossRef]
- Moffat, J.W.; Toth, V.T. Fundamental parameter-free solutions in modified gravity. Class. Quantum Gravity 2009, 26, 085002. [Google Scholar] [CrossRef]
- Moffat, J.W.; Toth, V.T. Testing modified gravity with motion of satellites around galaxies. arXiv 2007, arXiv:0708.1264. [Google Scholar]
- Moffat, J.W.; Toth, V.T. Testing Modified Gravity with Globular Cluster Velocity Dispersions. Astrophys. J. 2008, 680, 1158–1161. [Google Scholar] [CrossRef]
- Brownstein, J.R.; Moffat, J.W. Galaxy cluster masses without non-baryonic dark matter. Mon. Not. R. Astron. Soc. 2006, 367, 527–540. [Google Scholar] [CrossRef]
- Brownstein, J.R.; Moffat, J.W. The Bullet Cluster 1E0657-558 evidence shows modified gravity in the absence of dark matter. Mon. Not. R. Astron. Soc. 2007, 382, 29–47. [Google Scholar] [CrossRef]
- Moffat, J.W. A Modified Gravity and its Consequences for the Solar System, Astrophysics and Cosmology. Int. J. Mod. Phys. D 2007, 16, 2075–2090. [Google Scholar] [CrossRef]
- Moffat, J.W.; Toth, V.T. Modified Gravity: Cosmology without dark matter or Einstein’s cosmological constant. arXiv 2007, arXiv:0710.0364. [Google Scholar]
- Moffat, J.W.; Toth, V.T. Modified Gravity and the origin of inertia. Mon. Not. R. Astron. Soc. 2009, 395, L25–L28. [Google Scholar] [CrossRef]
- Moffat, J.W. Acceleration in Modified Gravity (MOG) and the Mass-Discrepancy Baryonic Relation. arXiv 2016, arXiv:1610.06909. [Google Scholar]
- Prada, F.; Vitvitska, M.; Klypin, A.; Holtzman, J.A.; Schlegel, D.J.; Grebel, E.K.; Rix, H.W.; Brinkmann, J.; McKay, T.A.; Csabai, I. Observing the Dark Matter Density Profile of Isolated Galaxies. Astrophys. J. 2003, 598, 260–271. [Google Scholar] [CrossRef]
- Sheldon, E.S.; Johnston, D.E.; Frieman, J.A.; Scranton, R.; McKay, T.A.; Connolly, A.J.; Budavári, T.; Zehavi, I.; Bahcall, N.A.; Brinkmann, J.; et al. The Galaxy-Mass Correlation Function Measured from Weak Lensing in the Sloan Digital Sky Survey. Astron. J. 2004, 127, 2544–2564. [Google Scholar] [CrossRef]


Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the author. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Cesare, V. Dark Coincidences: Small-Scale Solutions with Refracted Gravity and MOND. Universe 2023, 9, 56. https://doi.org/10.3390/universe9010056
Cesare V. Dark Coincidences: Small-Scale Solutions with Refracted Gravity and MOND. Universe. 2023; 9(1):56. https://doi.org/10.3390/universe9010056
Chicago/Turabian StyleCesare, Valentina. 2023. "Dark Coincidences: Small-Scale Solutions with Refracted Gravity and MOND" Universe 9, no. 1: 56. https://doi.org/10.3390/universe9010056
APA StyleCesare, V. (2023). Dark Coincidences: Small-Scale Solutions with Refracted Gravity and MOND. Universe, 9(1), 56. https://doi.org/10.3390/universe9010056