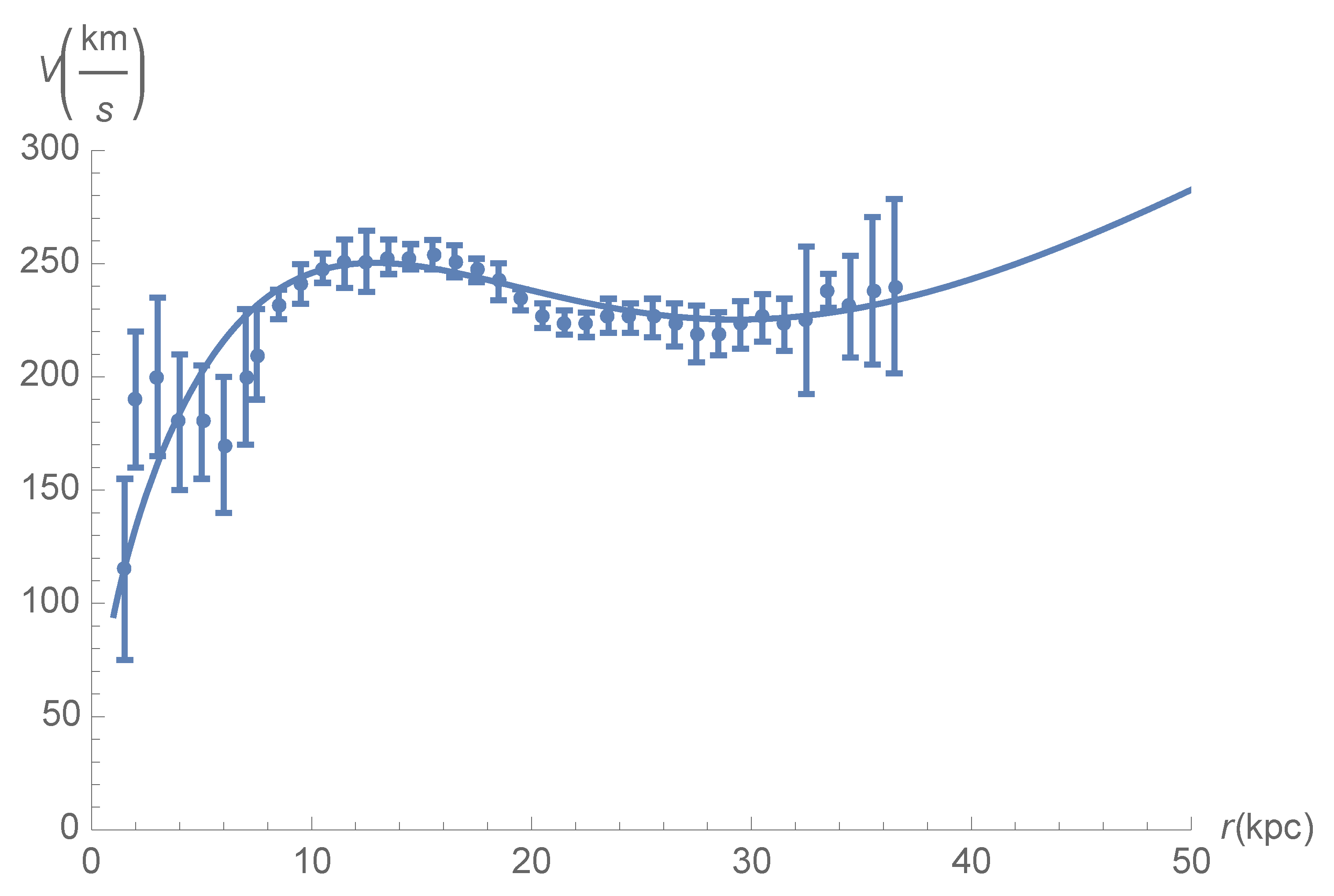
The Effect of a Spiral Density Wave on the Galaxy’s Rotation Curve, as Applied to the Andromeda Galaxy (M31)
Abstract
1. Introduction
2. Model: Non-Linear Integrable Equation and Bright Soliton Solution
2.1. Stellar Component of the Disk
- We have followed the density wave description conducted by [28];
- We have kept nonlinear terms in the calculations—multiplication of perturbed variables;
- The existence of the NLSE means that these nonlinear terms are balanced by the dispersive properties of the wave;
- The dispersive properties of the wave are due to specific relationships between wave vector and wave frequency—the top of the wave moves faster then the bottom, causing the breakdown of the wave;
- Balance of nonlinear effects and dispersive effects results in the wave of constant amplitude and constant group velocity traveling with unchanged form as long as the conditions for the existence are fulfilled;
- In this particular case, that condition is the marginal stability of the disk (marginal stability of disk galaxies has been discussed in Chapter 15.2 of [30]);
- The azimuthal direction of the group velocity is a direct consequence of the marginal stability condition due to the transformation of specific stretched coordinates;
- The curvature of the one-dimensional wave is due to rotation;
- In [28], according to linearized theory, these dispersive effects were responsible for very short wave existence;
- Accompanied by strong rotation, they had no chance to last long enough to explain the observed structure;
- NLSE as a solution has an envelope wave that contains a carrier wave—a fine structure of space with a period much smaller than the width of a soliton, explaining the process of star formation with sharp density gradients;
- Although the fluid model description cannot predict resonances, it is very useful, and the behavior of density waves at resonance can be discussed without involving kinetic theory; the obtained cubic type of nonlinearity provides the main contribution to the Lindblad resonances;
- Nonlinear theory allows that the amplitude of the wave should not be considered as very small; therefore, even facing the resonance, it should not be completely damped, but it rather broadens the resonance function (a detailed discussion on the linearized density wave dynamics at resonance is given in Chapter 17 of [30]).
2.2. Gaseous Component of the Disk
3. Methods
Derivation of Parameters
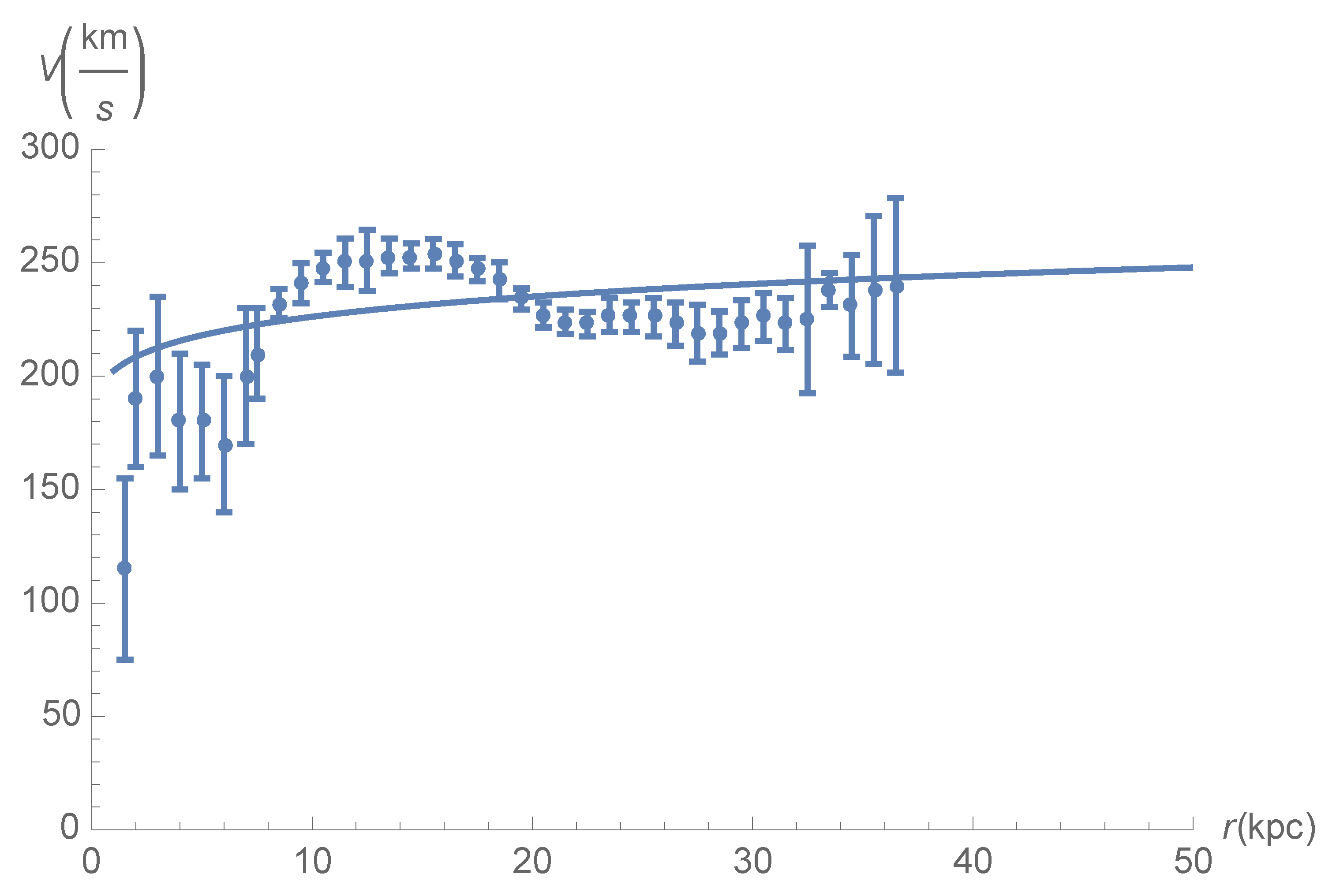
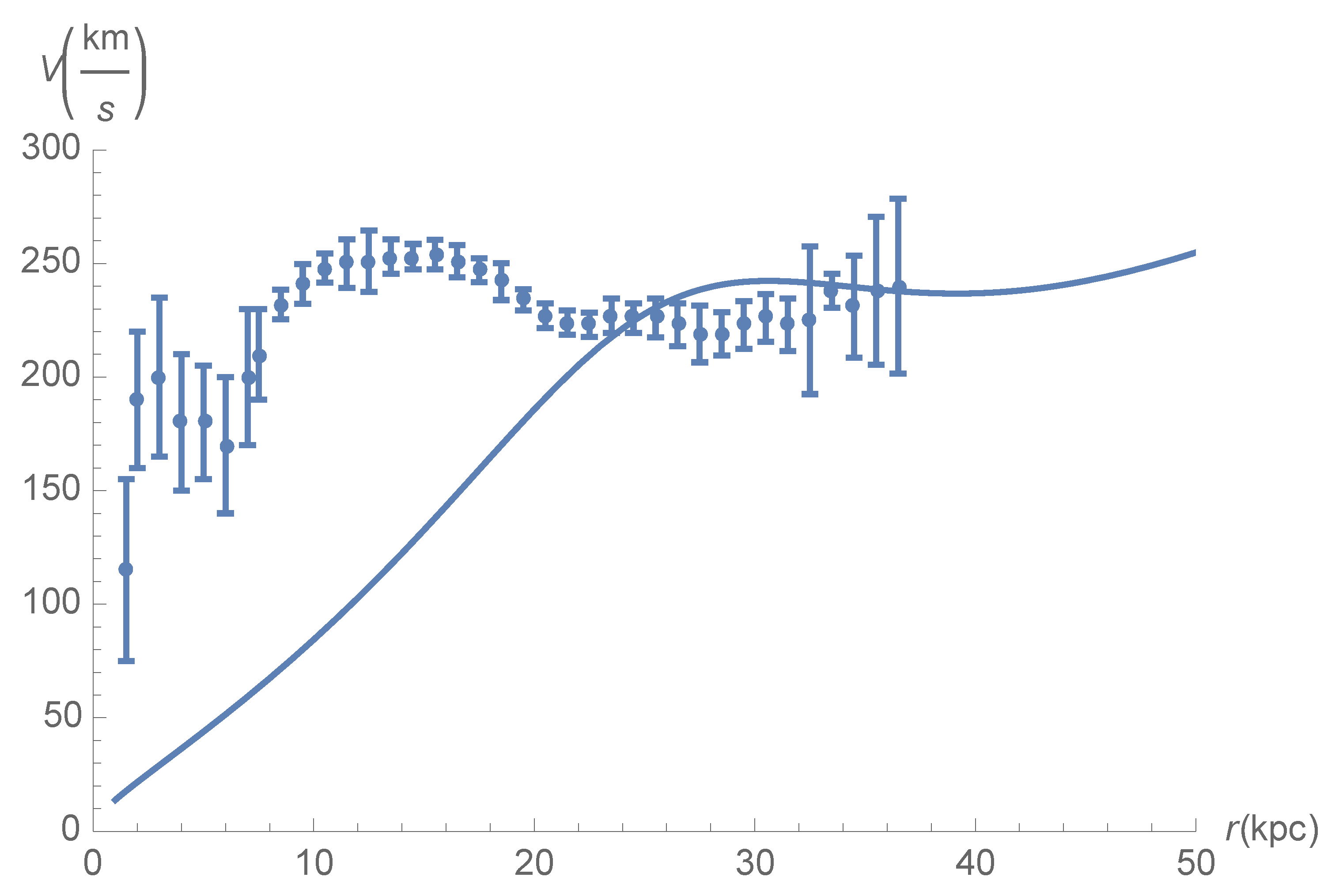
4. Results
4.1. Radial Dependence of Angular Velocity and Surface Mass Density
4.2. Rotation Curve of M31 Galaxy for Constant Parameters
4.2.1. Outer Part of the Disk
4.2.2. Rotation Period Influence on the Parameters Estimation
5. Discussion
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Zwicky, F. On the masses of nebulae and of clusters of nebulae. APJ 1937, 86, 217–246. [Google Scholar] [CrossRef]
- Casertano, S. Rotation curve of the edge-on spiral galaxy NGC 5907: Disc and halo masses. MNRAS 1983, 203, 735–747. [Google Scholar] [CrossRef]
- Sofue, Y. Rotation and mass in the Milky Way and spiral galaxies. PASJ 2017, 69, R1. [Google Scholar] [CrossRef]
- Binney, J. More dynamical models of our Galaxy. MNRAS 2012, 426, 1328–1337. [Google Scholar] [CrossRef]
- Milgrom, M. A modification of the Newtonian dynamics as a possible alternative to the hidden mass hypothesis. APJ 1983, 270, 365–370. [Google Scholar] [CrossRef]
- Moffat, J.W. Scalar tensor vector gravity theory. JCAP 2006, 3, 4–24. [Google Scholar] [CrossRef]
- McGaugh, S. Novel test of modified Newtonian Dynamics with a gas rich galaxies. PRL 2011, 106, 121303. [Google Scholar] [CrossRef]
- Carignan, C.; Frank, B.S.; Hess, K.M.; Lucero, D.M.; Randriamampandry, T.H.; Goedhart, S.; Passmoor, S.S. KAT-7 Science Verification: Using H I observations of NGC 3109 to understand its kinematics and mass distribution. AJ 2013, 146, 48–68. [Google Scholar] [CrossRef]
- Mannheim, P.D.; O’Brien, J.G. Fitting galactic rotation curves with conformal gravity and a global quadratic potential. PRD 2012, 85, 124020. [Google Scholar] [CrossRef]
- McGaugh, S. Predictions and Outcomes for the Dynamics of Rotating Galaxies. Galaxies 2020, 8, 35. [Google Scholar] [CrossRef]
- Feng, J.Q. Rotating Disk Galaxies without Dark Matter Based on Scientific Reasoning. Galaxies 2020, 8, 9. [Google Scholar] [CrossRef]
- Binney, J.; Tremaine, S. Galactic Dynamics; Princeton University Press: Princeton, NJ, USA, 1983; p. 102. [Google Scholar]
- Sanders, R.H. Anty-gravity and galaxy rotation curves. A&A 1984, 136, L21–L23. [Google Scholar]
- Sipols, A.; Pavlovich, A. Dark Matter Dogma: A Study of 214 Galaxies. Galaxies 2020, 8, 36. [Google Scholar] [CrossRef]
- Criss, R.E.; Hofmeister, A.M. Density Profiles of 51 Galaxies from Parameter-Free Inverse Models of Their Measured Rotation Curves. Galaxies 2020, 8, 19. [Google Scholar] [CrossRef]
- Vukcevic, M. The Spiral galaxies flat rotational velocity curve explained by the constant group velocity of a nonlinear density wave. AJ 2021, 161, 118–123. [Google Scholar] [CrossRef]
- Babckok, H.W. The rotation of the Andromeda Nebula. Lick Obs. Bull. 1939, 19, 41–51. [Google Scholar] [CrossRef]
- Rubin, V.; Ford, W.K., Jr. Rotation of the Andromeda Nebula from a spectroscopic survey of emission regions. APJ 1970, 159, 379–403. [Google Scholar] [CrossRef]
- Carignan, C.; Chemin, L.; Huchtmeier, W.K.; Lockman, F.J. The extended HI rotation curve and mass distribution of M31. APJ 2006, 641, L109. [Google Scholar] [CrossRef]
- Norman, C.A. A non-linear theory of spiral density waves. MNRAS 1978, 182, 457–472. [Google Scholar] [CrossRef]
- Sellwood, J.A. The global stability of our Galaxy. MNRAS 1985, 217, 127–148. [Google Scholar] [CrossRef]
- Sellwood, J.A.; Athanassoula, E. Unstable modes from galaxy simulations. MNRAS 1986, 221, 195–212. [Google Scholar] [CrossRef]
- Vukcevic, M. Non-linear density wave solutions for different models of galaxies. MNRAS 2014, 441, 565–570. [Google Scholar] [CrossRef][Green Version]
- Lelli, F.; McGaugh, S.S.; Schombert, J.M. SPARC: Mass models for 157 disk galaxies with SPITZER photometry and accurate rotation curves. AJ 2016, 152, 157–171. [Google Scholar] [CrossRef]
- de Blok, W.J.G.; Walter, F.; Brinks, E.; Trachernach, C.; Oh, S.-H.; Kennicutt, R.C., Jr. High-resolution rotation curves and galaxy mass models from THINGS. AJ 2008, 136, 2648–2719. [Google Scholar] [CrossRef]
- Vukcevic, M.; Zekovic, V.; Radeta, M. Spiral structure of the galactic disk and its influence on the rotational velocity curve. AN 2021, 343, e210108. [Google Scholar] [CrossRef]
- Vukcevic, M. Non-linear vortex solution for the inner region of a galaxy. MNRAS 2019, 484, 3410–3418. [Google Scholar]
- Lin, C.C.; Shu, F.H. On the Spiral Structure of Disk Galaxies. APJ 1964, 140, 646–655. [Google Scholar] [CrossRef]
- Jaffrey, A.; Taniuti, T. Non-Liner Wave Propagation: With Applications to Physics and Magnetohydrodynamics; Academic Press: Princeton, NJ, USA, 1964. [Google Scholar]
- Bertin, G. Dynamics of Galaxies; Princeton University Press: Princeton, NJ, USA, 2000. [Google Scholar]
- Watanabe, T. A nonlinear theory of two-stream instability. PSJ 1969, 27, 1341. [Google Scholar] [CrossRef]
- Martinez-Garcia, E.E. Testing theories in barred-spiral galaxies. ApJ 2012, 744, 92–105. [Google Scholar] [CrossRef]
- Pour-Imani, H.; Kennefick, D.; Keneffick, J.; Davis, B.L.; Shields, D.W.; Shameer, A.M. Strong evidence for the density-wave theory of spiral structure in disk galaxies. ApJL 2016, 827, L2. [Google Scholar] [CrossRef]
- Yu, S.-Y.; Ho, L.C. On the connection between spiral arm pitch angle and galaxy properties. APJ 2019, 871, 194–208. [Google Scholar] [CrossRef]
- Elmegreen, D.M.; Elmegreen, B.G.; Yau, A.; Athanassoula, E.; Bosma, A.; Buta, R.J.; Helou, G.; Ho, L.C.; Gadotti, D.A.; Knapen, J.H. Grand design and flocculent spirals in the Spitzer survey of stellar structure in galaxies (S4G). APJ 2011, 737, 32–49. [Google Scholar] [CrossRef]
- Fich, M.; Blitz, L.; Stark, A.A. The rotation curve of the Milky Way to 2R0. APJ 1989, 342, 272–284. [Google Scholar] [CrossRef]
- Bertin, G.; Amorisco, N.C. Prominent spiral arms in the gaseous outer galaxy disks. A&A 2010, 512, A17. [Google Scholar]
- Nieten, C.; Neininger, N.; Guelin, M.; Ungerechts, H.; Lucas, R.; Berkhuijsen, E.M.; Beck, R.; Wielebinski, R. Molecular gas in the Andromeda galaxy. A&A 2010, 453, 459–475. [Google Scholar]
- Freeman, K.C. On the disks of spiral and S0 galaxies. APJ 1970, 160, 811–830. [Google Scholar] [CrossRef]
- Miyamoto, M.; Nagai, R. Three-dimensional models for the distribution of mass in galaxies. PASJ 1975, 27, 533–543. [Google Scholar]
- Sofue, Y. Radial distributions of surface mass density and mass-to-luminosity ratio in spiral galaxies. PASJ 2018, 70, 1–15. [Google Scholar] [CrossRef]
- Tamm, A.; Tempel, E.; Tenjes, P.; Tihhonova, O.; Tuvikene, T. Stellar mass map and dark matter distribution in M 31. A&A 2012, 546, A4. [Google Scholar]
- Sofue, Y.; Rubin, V. Rotation curves of spiral galaxies. ARAA 2001, 39, 137–174. [Google Scholar] [CrossRef]
- Verheijen, M.A.W. The Ursa Major Cluster of Galaxies; Tully-Fisher Relations and Dark Matter, Rijksuniversiteit Groningen; Astronomical Society of the Pacific: San Francisco, CA, USA, 1997. [Google Scholar]
- Corbelli, E.; Lorenzoni, S.; Walterbos, R.; Braun, R.; Thilker, D. A wide-field H I mosaic of Messier 31 II. The disk warp, rotation, and the dark matter halo. A&A 2010, 511, A89. [Google Scholar]

Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the author. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Vukcevic, M. The Effect of a Spiral Density Wave on the Galaxy’s Rotation Curve, as Applied to the Andromeda Galaxy (M31). Universe 2022, 8, 522. https://doi.org/10.3390/universe8100522
Vukcevic M. The Effect of a Spiral Density Wave on the Galaxy’s Rotation Curve, as Applied to the Andromeda Galaxy (M31). Universe. 2022; 8(10):522. https://doi.org/10.3390/universe8100522
Chicago/Turabian StyleVukcevic, Miroslava. 2022. "The Effect of a Spiral Density Wave on the Galaxy’s Rotation Curve, as Applied to the Andromeda Galaxy (M31)" Universe 8, no. 10: 522. https://doi.org/10.3390/universe8100522
APA StyleVukcevic, M. (2022). The Effect of a Spiral Density Wave on the Galaxy’s Rotation Curve, as Applied to the Andromeda Galaxy (M31). Universe, 8(10), 522. https://doi.org/10.3390/universe8100522