Electron Temperature Anisotropy Effects on Alpha/Proton Instability in the Solar Wind
Abstract
:1. Introduction
2. Plasma Model and Wave Equation
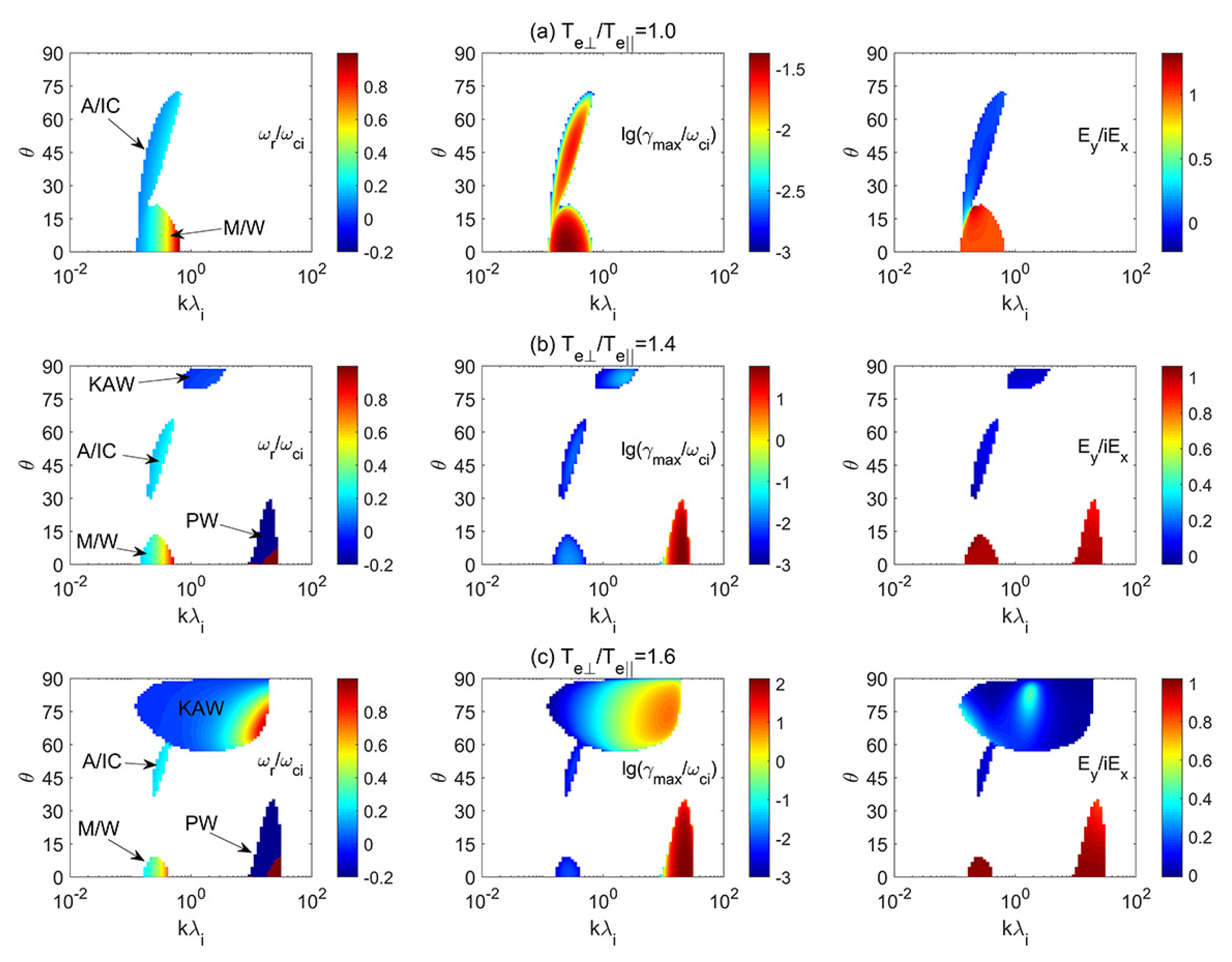
3. Alpha Beam Instability Induced by Electron Temperature Anisotropy
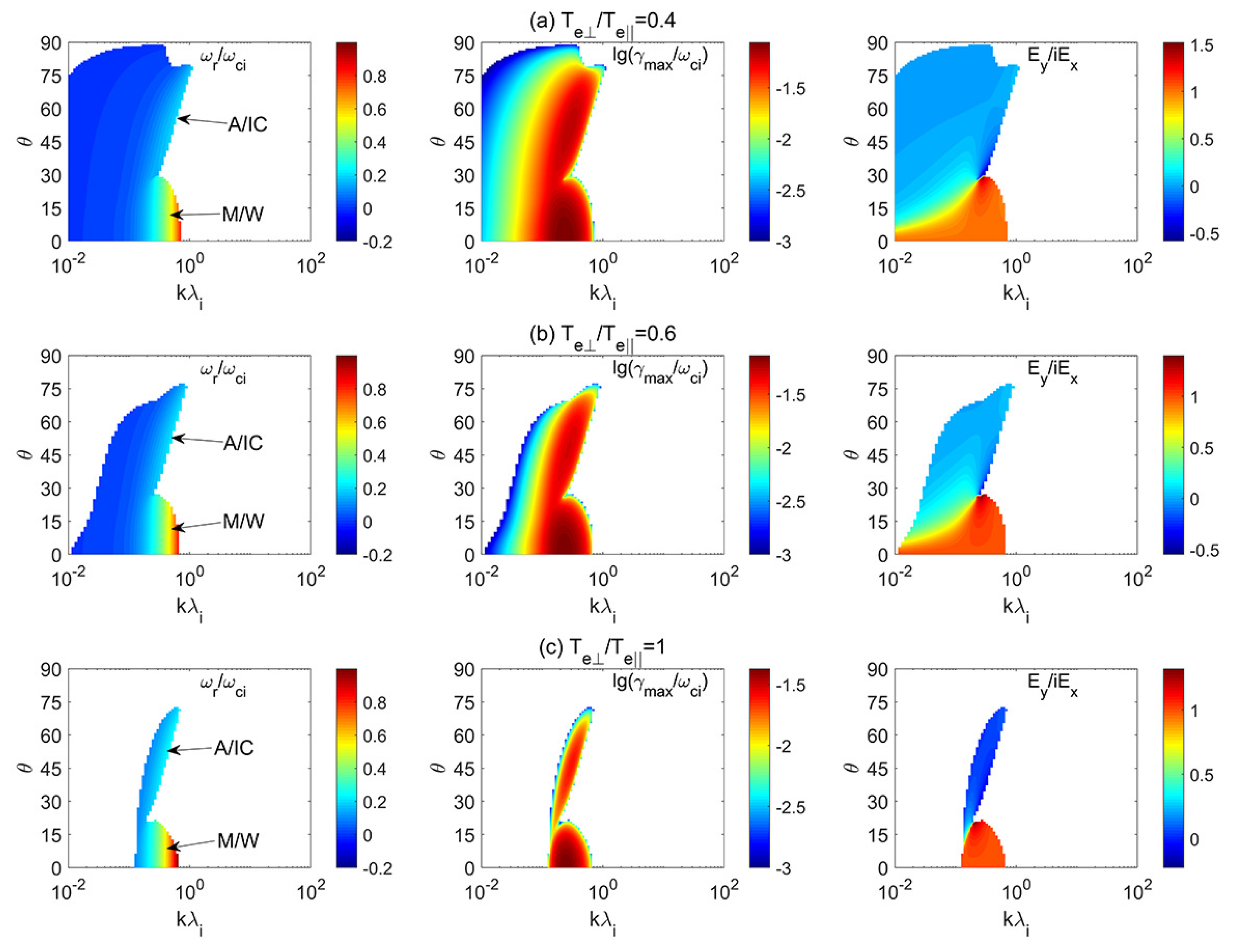
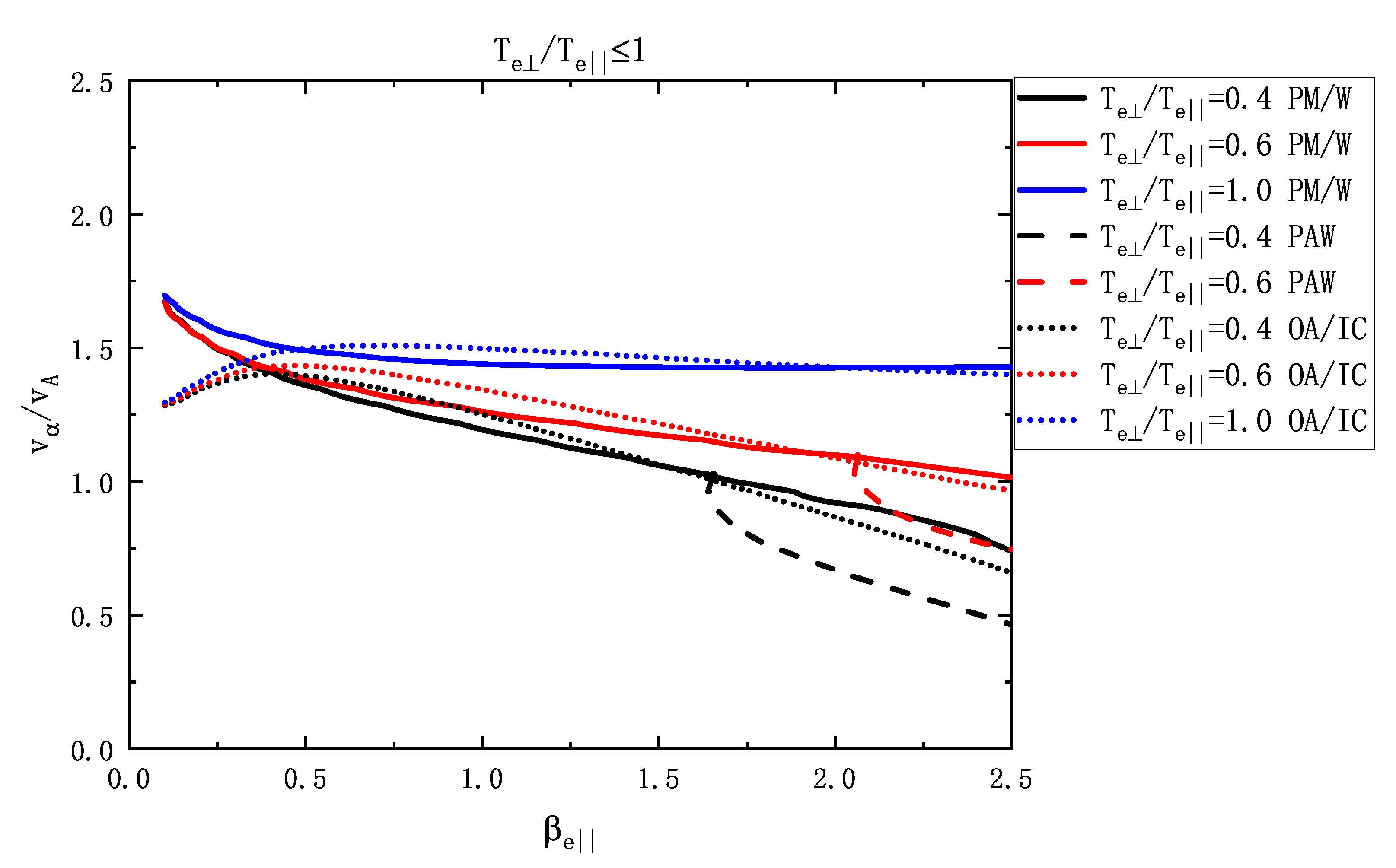
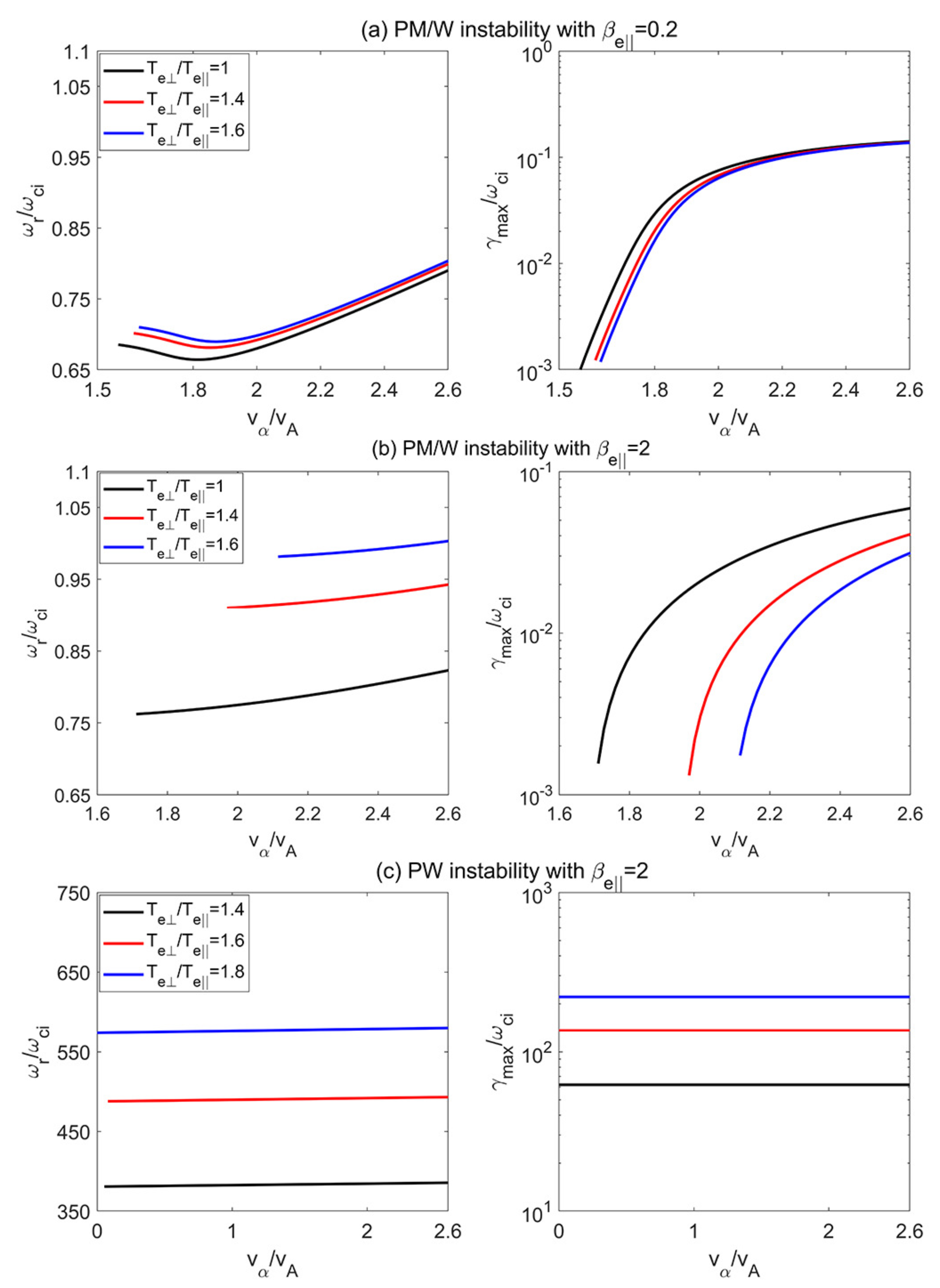
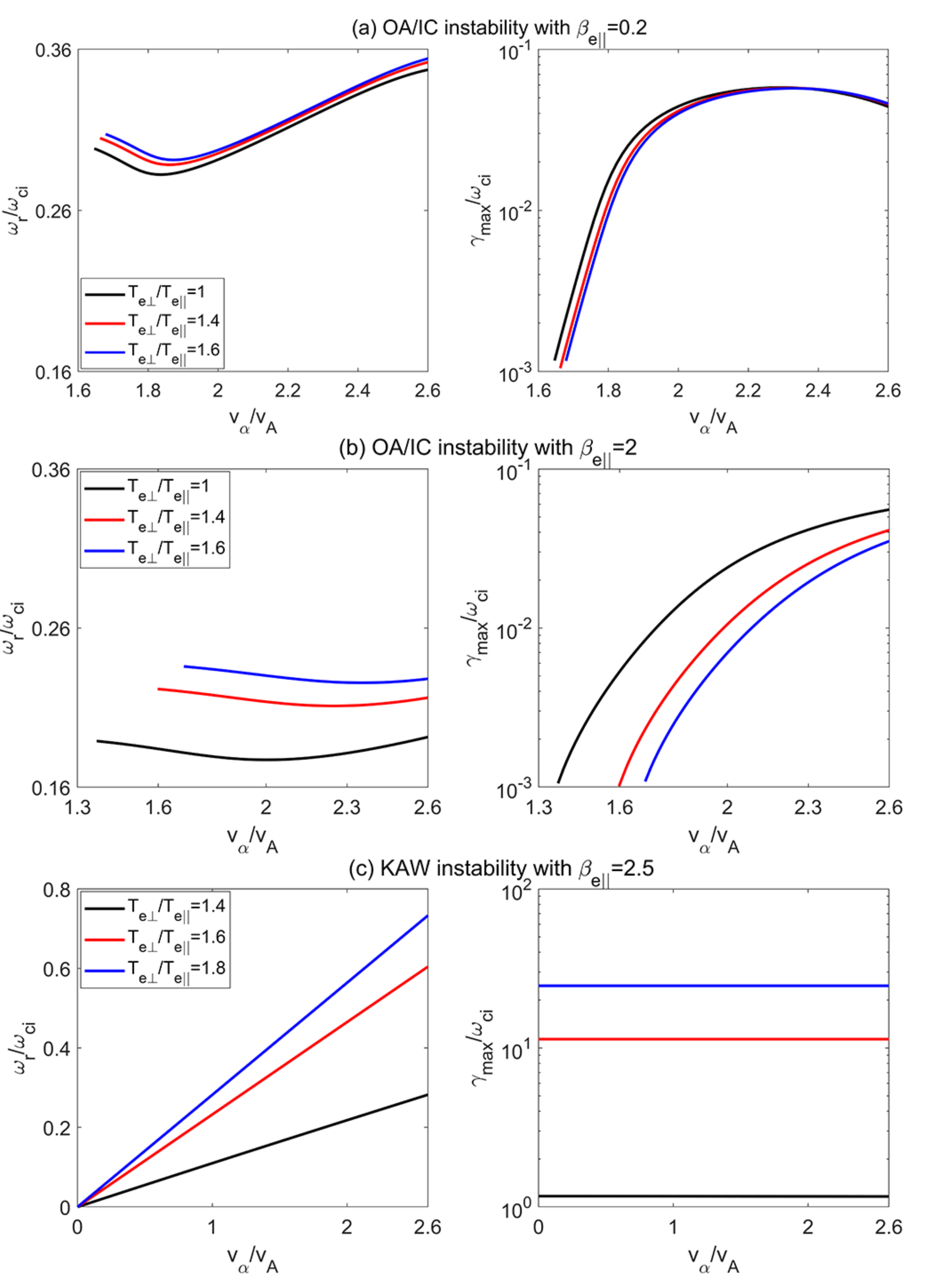
3.1. Alpha Beam Instability with Excess Parallel Electron Temperature Anisotropy
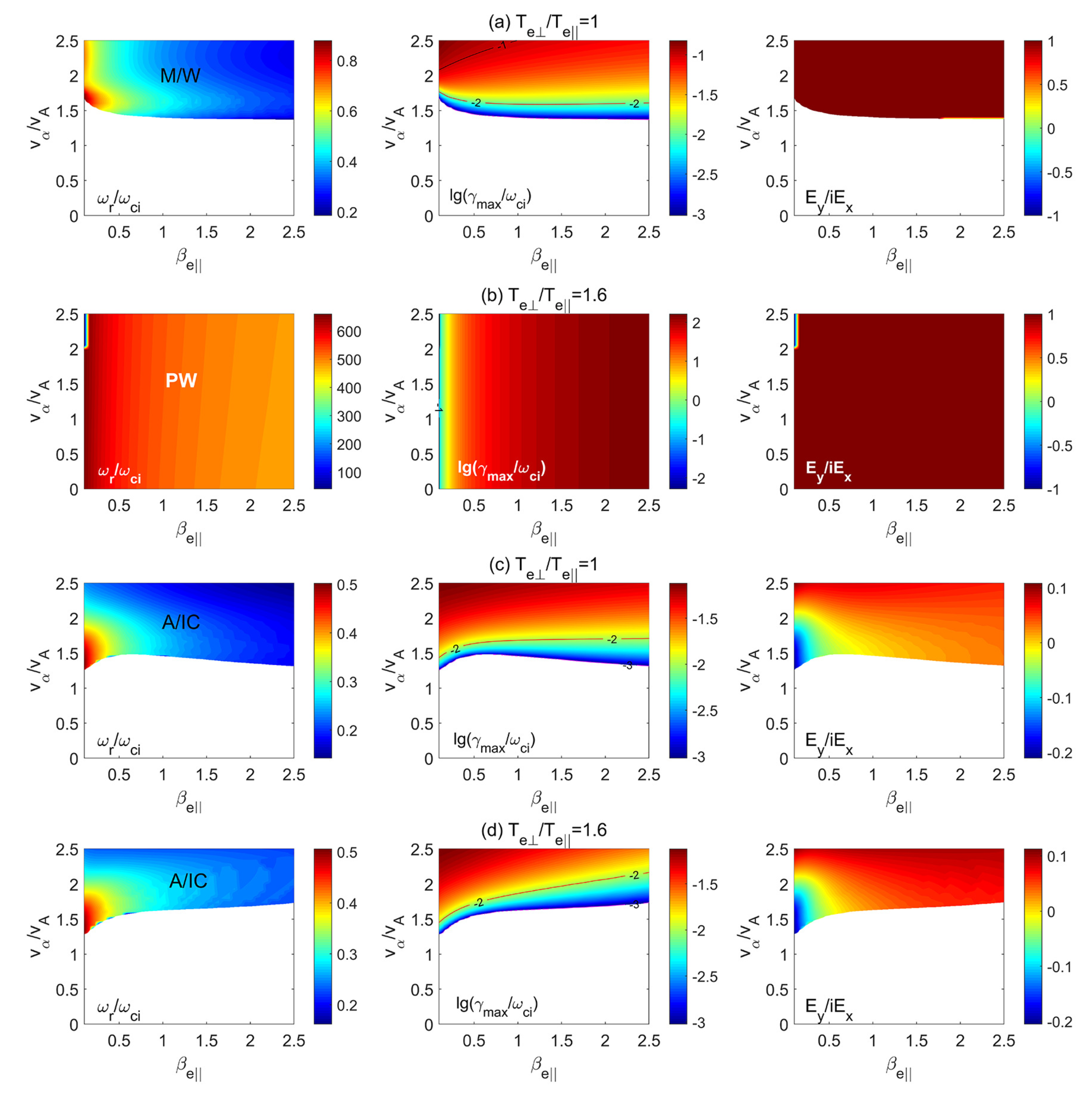
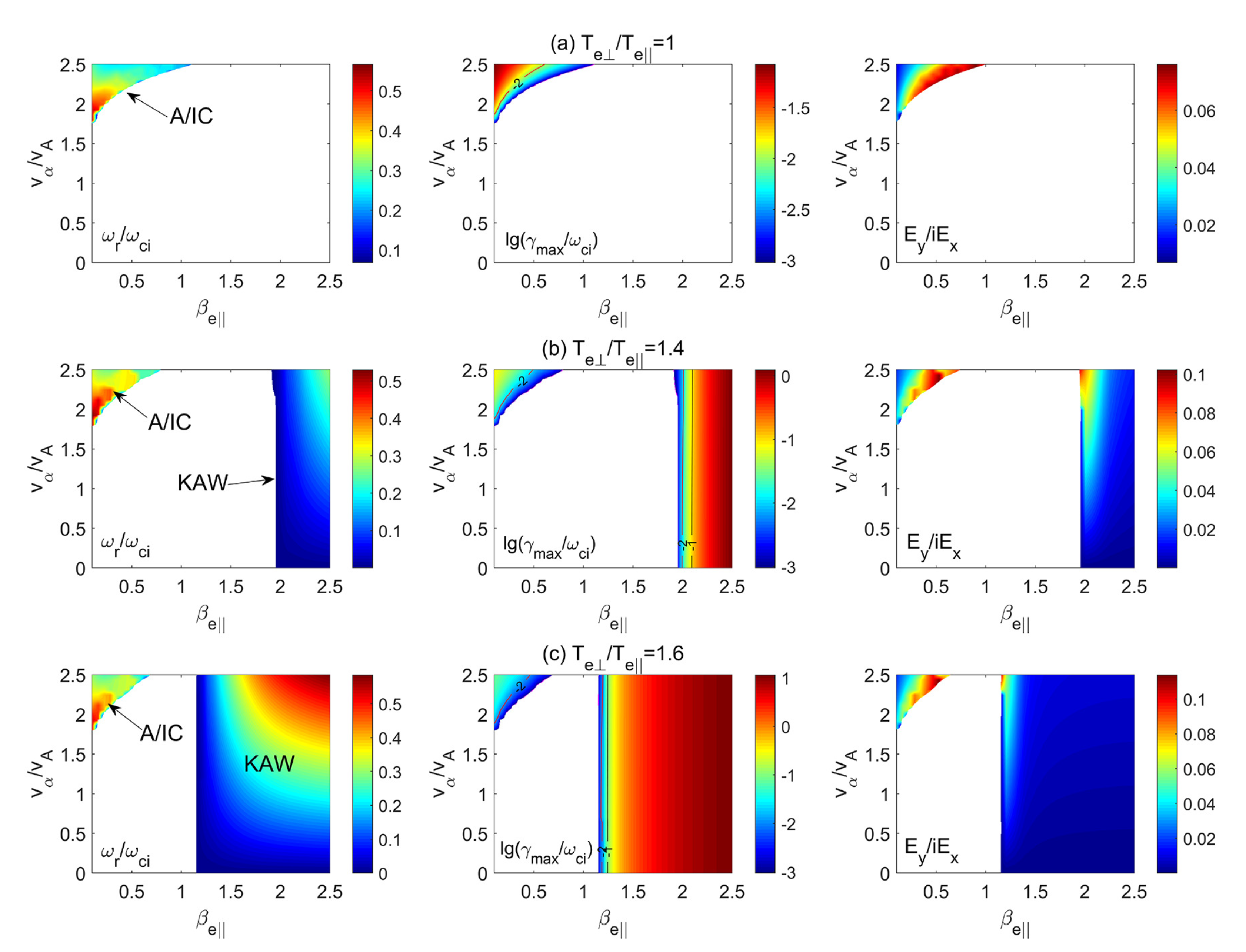
3.2. Alpha Beam Instability with Excess Perpendicular Electron Temperature Anisotropy
4. Discussion and Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Bame, S.J.; Asbridge, J.R.; Feldman, W.C.; Gosling, J.T. Evidence for a structure-free state at high solar wind speeds. J. Geophys. Res. 1977, 82, 1487–1492. [Google Scholar] [CrossRef]
- Pierrard, V.; Lazar, M.; Poedts, S.; Štverák, Š.; Maksimovic, M.; Trávníček, P.M. The Electron Temperature and Anisotropy in the Solar Wind. Comparison of the Core and Halo Populations. Sol. Phys. 2016, 291, 2165–2179. [Google Scholar] [CrossRef]
- Verscharen, D.; Bourouaine, S.; Chandran, B.D.G.; Maruca, B.A. A Parallel-propagating Alfvénic Ion-beam Instability in the High-beta Solar Wind. Astrophys. J. 2013, 773, 8. [Google Scholar] [CrossRef]
- Steinberg, J.T.; Lazarus, A.J.; Ogilvie, K.W.; Lepping, R.; Byrnes, J. Differential flow between solar wind protons and alpha particles: First WIND observations. Geophys. Res. Lett. 1996, 23, 1183–1186. [Google Scholar] [CrossRef]
- Neugebauer, M.; Goldstein, B.E.; Smith, E.J.; Feldman, W.C. Ulysses observations of differential alpha-proton streaming in the solar wind. J. Geophys. Res. 1996, 101, 17047–17055. [Google Scholar] [CrossRef]
- Borovsky, J.E. The plasma structure of coronal hole solar wind: Origins and evolution. J. Geophys. Res. Space Phys. 2016, 121, 5055–5087. [Google Scholar] [CrossRef]
- Ďurovcová, T.; Němeček, Z.; Šafránková, J. Evolution of the α-proton Differential Motion across Stream Interaction Regions. Astrophys. J. 2019, 873, 24. [Google Scholar] [CrossRef]
- Ebert, R.W.; McComas, D.J.; Elliott, H.A.; Forsyth, R.J.; Gosling, J.T. Bulk properties of the slow and fast solar wind and interplanetary coronal mass ejections measured by Ulysses: Three polar orbits of observations. J. Geophys. Res. Space Phys. 2009, 114. [Google Scholar] [CrossRef]
- Goldstein, B.E.; Neugebauer, M.; Zhang, L.D.; Gary, S.P. Observed constraint on proton-proton relative velocities in the solar wind. Geophys. Res. Lett. 2000, 27, 53–56. [Google Scholar] [CrossRef]
- Gomberoff, L.; Gnavi, G.; Gratton, F.T. Minor heavy ion electromagnetic beam-plasma interactions in the solar wind. J. Geophys. Res. 1996, 101, 13517–13522. [Google Scholar] [CrossRef]
- Marsch, E.; Livi, S. Observational evidence for marginal stability of solar wind ion beams. J. Geophys. Res. 1987, 92, 7263–7268. [Google Scholar] [CrossRef]
- Gary, S.P.; Yin, L.; Winske, D.; Reisenfeld, D.B. Electromagnetic alpha/proton instabilities in the solar wind. Geophys. Res. Lett. 2000, 27, 1355–1358. [Google Scholar] [CrossRef]
- Gary, S.P.; Jian, L.K.; Broiles, T.W.; Stevens, M.L.; Podesta, J.J.; Kasper, J.C. Ion-driven instabilities in the solar wind: Wind observations of 19 March 2005. J. Geophys. Res. 2016, 121, 30–41. [Google Scholar] [CrossRef]
- Gary, S.P.; Yin, L.; Winske, D.; Reisenfeld, D.B. Alpha/proton magnetosonic instability in the solar wind. J. Geophys. Res. 2000, 105, 20989–20996. [Google Scholar] [CrossRef]
- Revathy, P. Magnetosonic instability driven by an alpha particle beam in the solar wind. J. Geophys. Res. 1978, 83, 5750–5752. [Google Scholar] [CrossRef]
- Verscharen, D.; Chandran, B.D.G.; Bourouaine, S.; Hollweg, J.V. Deceleration of Alpha Particles in the Solar Wind by Instabilities and the Rotational Force: Implications for Heating, Azimuthal Flow, and the Parker Spiral Magnetic Field. Astrophys. J. 2015, 806, 157. [Google Scholar] [CrossRef]
- Verscharen, D.; Bourouaine, S.; Chandran, B.D.G. Instabilities Driven by the Drift and Temperature Anisotropy of Alpha Particles in the Solar Wind. Astrophys. J. 2013, 773, 163. [Google Scholar] [CrossRef]
- Li, X.; Habbal, S.R. Proton/alpha magnetosonic instability in the fast solar wind. J. Geophys. Res. 2000, 105, 7483–7489. [Google Scholar] [CrossRef]
- Xiang, L.; Wu, D.J.; Chen, L. Effect of alpha beams on low-frequency electromagnetic waves driven by proton beams. Astrophys. J. 2018, 869, 64. [Google Scholar] [CrossRef]
- Bourouaine, S.; Verscharen, D.; Chandran, B.D.G.; Maruca, B.A.; Kasper, J.C. Limits on Alpha Particle Temperature Anisotropy and Differential Flow from Kinetic Instabilities: Solar Wind Observations. Astrophys. J. 2013, 777, L3. [Google Scholar] [CrossRef] [Green Version]
- Sun, H.; Zhao, J.; Xie, H.; Wu, D. On Kinetic Instabilities Driven By Ion Temperature Anisotropy and Differential Flow in the Solar Wind. Astrophys. J. 2019, 884, 44. [Google Scholar] [CrossRef]
- Klein, K.G.; Howes, G.G. Predicted impacts of proton temperature anisotropy on solar wind turbulence. Phys. Plasmas 2015, 22, 032903. [Google Scholar] [CrossRef]
- Masood, W.; Schwartz, S.J. Observations of the development of electron temperature anisotropies in Earth’s magnetosheath. J. Geophys. Res. Space Phys. 2008, 113. [Google Scholar] [CrossRef]
- Feldman, W.C.; Asbridge, J.R.; Bame, S.J.; Montgomery, M.D.; Gary, S.P. Solar wind electrons. J. Geophys. Res. 1975, 80, 4181–4196. [Google Scholar] [CrossRef]
- Pilipp, W.G.; Miggenrieder, H.; Montgomery, M.D.; Mühlhäuser, K.-H.; Rosenbauer, H.; Schwenn, R. Characteristics of electron velocity distribution functions in the solar wind derived from the helios plasma experiment. J. Geophys. Res. 1987, 92, 1075–1092. [Google Scholar] [CrossRef]
- ŠtveráK, Š.; Trávníček, P.; Maksimovic, M.; Marsch, E.; Fazakerley, A.N.; Scime, E.E. Electron temperature anisotropy constraints in the solar wind. J. Geophys. Res. Space Phys. 2008, 113, A03103. [Google Scholar] [CrossRef]
- Sun, H.; Zhao, J.; Liu, W.; Xie, H.; Wu, D. Electron Temperature Anisotropy and Electron Beam Constraints from Electron Kinetic Instabilities in the Solar Wind. Astrophys. J. 2020, 902, 59. [Google Scholar] [CrossRef]
- Bale, S.D.; Kasper, J.C.; Howes, G.G.; Quataert, E.; Salem, C.; Sundkvist, D. Magnetic Fluctuation Power Near Proton Temperature Anisotropy Instability Thresholds in the Solar Wind. Phys. Rev. Lett. 2009, 103, 211101. [Google Scholar] [CrossRef]
- Vafin, S.; Lazar, M.; Fichtner, H.; Schlickeiser, R.; Drillisch, M. Solar wind temperature anisotropy constraints from streaming instabilities. Astron. Astrophys. 2018, 613, A23. [Google Scholar] [CrossRef]
- Hellinger, P.; Trávníček, P.; Kasper, J.C.; Lazarus, A.J. Solar wind proton temperature anisotropy: Linear theory and WIND/SWE observations. Geophys. Res. Lett. 2006, 33, L09101. [Google Scholar] [CrossRef] [Green Version]
- Maruca, B.A.; Kasper, J.C.; Bale, S.D. What Are the Relative Roles of Heating and Cooling in Generating Solar Wind Temperature Anisotropies? Phys. Rev. Lett. 2011, 107, 201101. [Google Scholar] [CrossRef] [PubMed]
- Michno, M.J.; Lazar, M.; Yoon, P.H.; Schlickeiser, R. Effects of Electrons on the Solar Wind Proton Temperature Anisotropy. Astrophys. J. 2014, 781, 49. [Google Scholar] [CrossRef]
- Ahmadi, N.; Germaschewski, K.; Raeder, J. Effects of electron temperature anisotropy on proton mirror instability evolution. J. Geophys. Res. Space Phys. 2016, 6, 5350–5365. [Google Scholar] [CrossRef]
- Shaaban, S.M.; Lazar, M.; Yoon, P.H.; Poedts, S. Beaming electromagnetic (or heat-flux) instabilities from the interplay with the electron temperature anisotropies. Phys. Plasmas 2018, 25, 082105. [Google Scholar] [CrossRef]
- Xiang, L.; Lee, K.H.; Wu, D.J.; Lee, L.C. Effects of Electron Temperature Anisotropy on Proton-beam Instability in the Solar Wind. Astrophys. J. 2020, 899, 61. [Google Scholar] [CrossRef]
- Maruca, B.A.; Kasper, J.C.; Gary, S.P. Instability-driven Limits on Helium Temperature Anisotropy in the Solar Wind: Observations and Linear Vlasov Analysis. Astrophys. J. 2012, 748, 137. [Google Scholar] [CrossRef]
- Lu, Q.M.; Xia, L.D.; Wang, S. Hybrid simulations of parallel and oblique electromagnetic alpha/proton instabilities in the solar wind. J. Geophys. Res. 2006, 111, A09101. [Google Scholar] [CrossRef]
- Gao, X.; Lu, Q.; Li, X.; Huang, C.; Wang, S. Heating of the background plasma by obliquely propagating Alfven waves excited in the electromagnetic alpha/proton instability. Phys. Plasmas 2012, 19, 032901. [Google Scholar] [CrossRef]
- Zhang, W.-L.; Xiang, L.; Li, Q.-H.; Lang, S.-Y.; Yu, H.-W. Alpha/proton Instability in the Presence of Proton and Alpha Temperature Anisotropy and its Application to the Deceleration of Alpha Particles in the Solar Wind. Res. Astron. Astrophys. 2022, 22, 015018. [Google Scholar] [CrossRef]
- Wu, D.J. Kinetic Alfvén Waves: Theory, Experience, and Application; Science Press: Beijing, China, 2012. [Google Scholar]
- Marsch, E.; Schwenn, R.; Rosenbauer, H.; Muehlhaeuser, K.-H.; Pilipp, W.; Neubauer, F.M. Solar wind protons: Three-dimensional velocity distributions and derived plasma parameters measured between 0.3 and 1 AU. J. Geophys. Res. 1982, 87, 52–72. [Google Scholar] [CrossRef]
- Marsch, E.; Rosenbauer, H.; Schwenn, R.; Muehlhaeuser, K.-H.; Neubauer, F.M. Solar wind helium ions: Obsevations of the Helios solar probes between 0.3 and 1 AU. J. Abbr. 1982, 1, 35–51. [Google Scholar] [CrossRef]
- Xiang, L.; Lee, K.H.; Wu, D.J.; Yu, H.W.; Lee, L.C. Linear and nonlinear effects of proton temperature anisotropy on proton-beam instability in the solar wind. Astrophys. J. 2021, 916, 30. [Google Scholar] [CrossRef]
- Stix, T.H. Waves in Plasmas; American Institute of Physics: New York, NY, USA, 1992. [Google Scholar]
- Li, Q.H.; Yang, L.; Xiang, L.; Wu, D.J. Spatial Distribution of Electromagnetic Waves near the Proton Cyclotron Frequency in ICME Sheath Regions Associated with Quasi-perpendicular Shocks: Wind Observations. Astrophys. J. 2020, 892, 98. [Google Scholar] [CrossRef]
- Gary, S.P.; Bandyopadhyay, R.; Qudsi, R.A.; Matthaeus, W.H.; Maruca, B.A.; Parashar, T.N.; Roytershteyn, V. Particle-in-cell Simulations of Decaying Plasma Turbulence: Linear Instabilities versus Nonlinear Processes in 3D and 2.5D Approximations. Astrophys. J. 2020, 901, 160. [Google Scholar] [CrossRef]





Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Lang, S.-Y.; Xiang, L.; Li, Q.-H.; Zhang, W.-L.; Yu, H.-W. Electron Temperature Anisotropy Effects on Alpha/Proton Instability in the Solar Wind. Universe 2022, 8, 466. https://doi.org/10.3390/universe8090466
Lang S-Y, Xiang L, Li Q-H, Zhang W-L, Yu H-W. Electron Temperature Anisotropy Effects on Alpha/Proton Instability in the Solar Wind. Universe. 2022; 8(9):466. https://doi.org/10.3390/universe8090466
Chicago/Turabian StyleLang, Si-Yi, Liang Xiang, Qiu-Huan Li, Wen-Lu Zhang, and Hong-Wei Yu. 2022. "Electron Temperature Anisotropy Effects on Alpha/Proton Instability in the Solar Wind" Universe 8, no. 9: 466. https://doi.org/10.3390/universe8090466
APA StyleLang, S.-Y., Xiang, L., Li, Q.-H., Zhang, W.-L., & Yu, H.-W. (2022). Electron Temperature Anisotropy Effects on Alpha/Proton Instability in the Solar Wind. Universe, 8(9), 466. https://doi.org/10.3390/universe8090466




