Neutrino Flavor Conversions in High-Density Astrophysical and Cosmological Environments
Abstract
1. Introduction
2. Equations of Motions
3. Flavor Conversions in the Early Universe
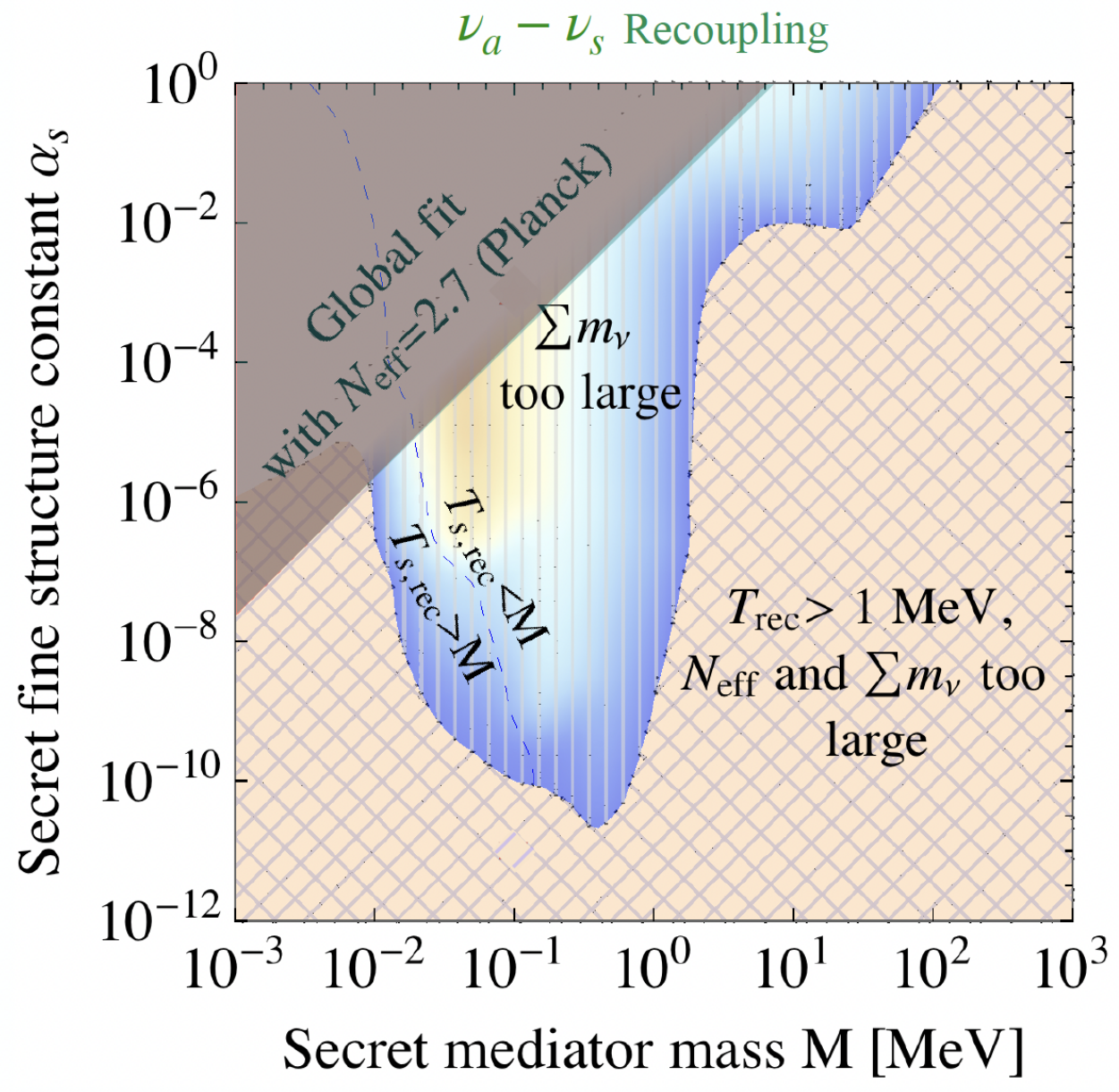
Active–Sterile Neutrino Oscillations
4. Flavor Conversions in Core-Collapse Supernovae
4.1. MSW Resonances
4.2. Collective Effects
4.2.1. Slow Flavor Conversions
- Multi angle effects [171,172,173,174]. When the flavor asymmetries are mild the phase dispersion induced by different propagation lengths of neutrinos can smear or completely remove the effects of the spectral splits, eventually leading to complete decoherence, i.e., all flavors are equilibrated up to lepton number conservation.
- Sponteneous breaking of azimuthal [181,182,183,184,185], spatial homogeneity [186,187,188,189,190,191,192], and stationarity [193,194]. It has been realized that the symmetries used as initial conditions of neutrino emission (azimuthal symmetry, spatial homogeineity, stationarity) are spontaneously broken during neutrino propagation. This leads to new instabilities that can develop in both mass orderings, but they are in general suppressed when the matter potential is dominating. Nevertheless, it has been shown [193,194] that self-interacting neutrinos can generate a pulsating solution with a frequency that effectively compensates the phase dispersion associated with the large matter term, lifting the suppression and making collective oscillations possible deep inside the supernova. However, because the matter potential changes during neutrino propagation, it is not clear whether a flavor wave with a specific pulsation can have enough time to grow and lead to significant flavor conversions. The presence of turbulent variations of the matter potential may introduce a coupling among flavor waves with different k, so making it more likely to have an instability even when neutrinos are propagating away in a supernova [195].
- Neutrino halo effect [196,197,198,199,200,201]. Neutrinos are not completely free-streaming after the neutrinosphere and even a small fraction of scattering neutrinos can produce a small “neutrino halo”. Such inward-going neutrinos can modify the outcome of conversions and the shape of spectral splits, if present, but, according to the latest simulations, the effects have been found to be relatively small.
4.2.2. Fast Flavor Conversions
- Proto-neutron star [220,221,222]. The physical origin of the crossings is a strong convective activity happening in the proto-neutron star, which can generate large amplitude modulations in the spatial distributions of and number densities. The physical implications are not very clear due to the nearly equal distributions of neutrinos and antineutrinos of all flavors.
- Neutrino decoupling region [221,223,224]. Their existence can be explained by the neutron richness of matter, which induces a later decoupling of with respect to and, thus, a more forward peaked angular distributions of . Another possibility is the presence of LESA [225,226,227,228], i.e., an asymmetric emission of lepton number, or other phenomena [228].
- Free streaming regime [229,230,231]. Crossings can be generated by neutrino backward scatterings off heavy nuclei and their size seems to get larger for smaller radial distances [229,230,231]. Such crossings are ubiquitous in the pre-shock region, but they can also occur in the post-shock region. In the former case, there is hardly an impact on astrophysical processes and on the detection at Earth [232,233], both because of the slower growth rates of the instabilities and the very small size of the amount of conversions expected. On the other hand, the instabilities developing in the post-shock region might produce an observable effect.
- Propagation of the power of the instability to small angular scales [236,237,238,239,240,241]. During its evolution, the power of a fast instability is moved from large scales to small ones in momentum space, accelerating the decoherence of the system (and the equilibration of flavors) [236,237,238,239,240,241]. Moreover, when considering a coarse-graining over space, fast conversions seem to eventually reach a steady state [239,240,241]. In particular in [240] an approximate analytical formula has been derived for calculating the amount of decoherence reached in fast conversions and, thus, the final amount of flavor conversions. This formula depends on the propagation angle of neutrinos and on the initial asymmetry between the total number of neutrinos and antineutrinos. If the asymmetry is extremely small, as expected for the crossings generated by backward scattering of neutrinos in the free streaming regime, then the amount of flavor conversions is expected to be negligible, as confirmed in [232,233]. In [240] the analytical formula is independent from the type of perturbation used to seed the flavor instabilities. However, in [241] a distinction is made between localized and random seeds. In the first case a coherent behavior in the space and momentum evolution of the flavor wave is retained for a longer time. The difference between [240] and [241] can be associated to heterogeneous numerical methods employed for calculating spatial derivatives.
- Spontaneous symmetry breaking [242]. As also happens to slow conversions, in the context of fast ones there is also a spontaneous breaking of the symmetries imposed in the initial conditions. This has been shown to happen for the azimuthal symmetry in [242] and in [243], though in the second reference it was not explicitly stated in the conclusions.
- Dependence on neutrino energy [244]. It has been proposed that the outcome of fast conversions depends on the size of the mass differences between mass eigenstates and on their ordering [244]. This claim has been criticized in [245] where only a modest dependence has been observed. However, the first simulation has been performed considering an homogeneous system, whereas the second has also taken into account the spatial evolution.
- Impact of inelastic collisions [245,246,247,248,249]. Since the conditions for fast conversions have been found even in locations where neutrinos are still partially or completely coupled to the plasma, there have been a few studies implementing collisions in numerical simulations. In this context, the authors of [247,248] reported the possibility of enhancement of flavor conversions, assuming only evolution in time. On the other hand, in [245,249] both time and space evolution have been taken into account and it was observed that collisions might cause flavor depolarization, thus suppressing conversions, but that a much larger mean free path than expected is required in order for this happen. This is in agreement to what found in [246], where the role of collisions in the generation of crossing has been also pointed out.
- Dependence on the number of neutrino species [242,243]. Considering six neutrino species the crossings and, thus, fast instabilities can occur in one (or more) of the three sectors and then propagate to other ones [243]. Moreover, even considering the distributions of to be the same, the outcome of flavor conversions is different [242] with respect to what obtained by using only the equation of motions for three species, as usually done in literature.
5. Fast Flavor Conversions in Compact Binary Mergers
6. Conclusions and Future Perspectives
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
| 1 | In order to include the correction due to the non-local nature of the Z boson propagator which mediate forward scattering on neutrinos of the same species. |
References
- Kajita, T. Nobel lecture: Discovery of atmospheric neutrino oscillations. Rev. Mod. Phys. 2016, 88, 030501. [Google Scholar] [CrossRef]
- McDonald, A.B. Nobel lecture: The sudbury neutrino observatory: Observation of flavor change for solar neutrinos. Rev. Mod. Phys. 2016, 88, 030502. [Google Scholar] [CrossRef]
- Capozzi, F.; Valentino, E.D.; Lisi, E.; Marrone, A.; Melchiorri, A.; Palazzo, A. Unfinished fabric of the three neutrino paradigm. Phys. Rev. D 2021, 104, 083031. [Google Scholar] [CrossRef]
- Gonzalez-Garcia, M.C.; Maltoni, M.; Schwetz, T. NuFIT: Three-Flavour Global Analyses of Neutrino Oscillation Experiments. Universe 2021, 7, 459. [Google Scholar] [CrossRef]
- de Salas, P.F.; Forero, D.V.; Ternes, C.A.; Tortola, M.; Valle, J.W.F. Status of neutrino oscillations 2018: 3σ hint for normal mass ordering and improved CP sensitivity. Phys. Lett. B 2018, 782, 633. [Google Scholar] [CrossRef]
- Aguilar-Arevalo, A.; LSND Collaboration. Evidence for neutrino oscillations from the observation of appearance in a beam. Phys. Rev. D 2001, 64, 112007. [Google Scholar] [CrossRef]
- Aguilar-Arevalo, A.A.; MINIBOONE Collaboration. Significant Excess ElectronLike Events MiniBooNE Short-Baseline Neutrino Experiment. Phys. Rev. Lett. 2018, 121, 221801. [Google Scholar] [CrossRef]
- Abdurashitov, J.N.; SAGE Collaboration. Measurement of the response of a Ga solar neutrino experiment to neutrinos from an Ar-37 source. Phys. Rev. C 2006, 73, 045805. [Google Scholar] [CrossRef]
- Kaether, F.; Hampel, W.; Heusser, G.; Kiko, J.; Kirsten, T. Reanalysis of the GALLEX solar neutrino flux and source experiments. Phys. Lett. B 2010, 685, 47. [Google Scholar] [CrossRef]
- Mention, G.; Fechner, M.; Lasserre, T.; Mueller, T.A.; Lhuillier, D.; Cribier, M.; Letourneau, A. The Reactor Antineutrino 605 Anomaly. Phys. Rev. D 2011, 83, 073006. [Google Scholar] [CrossRef]
- Dolgov, A.D.; Hansen, S.H.; Pastor, S.; Petcov, S.T.; Raffelt, G.G.; Semikoz, D.V. Cosmological bounds on neutrino degeneracy 607 improved by flavor oscillations. Nucl. Phys. B 2002, 632, 363. [Google Scholar] [CrossRef]
- Wolfenstein, L. Neutrino Oscillations in Matter. Phys. Rev. D 1978, 17, 2369. [Google Scholar] [CrossRef]
- Mikheyev, S.P.; Smirnov, A.Y. Resonance Amplification of Oscillations in Matter and Spectroscopy of Solar Neutrinos. Sov. J. Nucl. Phys. 1985, 42, 913. [Google Scholar]
- Stodolsky, L. On the Treatment of Neutrino Oscillations in a Thermal Environment. Phys. Rev. D 1987, 36, 2273. [Google Scholar] [CrossRef]
- Pantaleone, J.T. Neutrino flavor evolution near a supernova’s core. Phys. Lett. B 1995, 342, 250. [Google Scholar] [CrossRef]
- Sigl, G.; Raffelt, G. General kinetic description of relativistic mixed neutrinos. Nucl. Phys. B 1993, 406, 423. [Google Scholar] [CrossRef]
- Raffelt, G.; Sigl, G.; Stodolsky, L. Quantum statistics in particle mixing phenomena. Phys. Rev. D 1992, 45, 1782. [Google Scholar] [CrossRef] [PubMed]
- Raffelt, G.; Sigl, G.; Stodolsky, L. NonAbelian Boltzmann equation for mixing and decoherence. Phys. Rev. Lett. 1993, 70, 2363, Erratum in Phys. Rev. Lett. 2007, 98, 069902. [Google Scholar] [CrossRef]
- Dolgov, A.D. Neutrinos in the Early Universe. Sov. J. Nucl. Phys. 1981, 33, 700. [Google Scholar]
- Barbieri, R.; Dolgov, A. Neutrino Oscil. Early Universe. Nucl. Phys. B 1991, 349, 743. [Google Scholar] [CrossRef]
- Friedland, A.; Lunardini, C. Do many particle neutrino interactions cause a novel coherent effect? J. High Energy Phys. 2003, 10, 043. [Google Scholar] [CrossRef]
- Friedland, A.; Lunardini, C. Neutrino flavor conversion in a neutrino background: Single particle versus multiparticle description. Phys. Rev. D 2003, 68, 013007. [Google Scholar] [CrossRef]
- Balantekin, A.B.; Pehlivan, Y. Neutrino-Neutrino Interactions and Flavor Mixing in Dense Matter. J. Phys. G 2007, 34, 47. [Google Scholar] [CrossRef]
- Pehlivan, Y.; Balantekin, A.B.; Kajino, T.; Yoshida, T. Invariants of Collective Neutrino Oscillations. Phys. Rev. D 2011, 84, 065008. [Google Scholar] [CrossRef]
- Birol, S.; Pehlivan, Y.; Balantekin, A.B.; Kajino, T. Neutrino Spectral Split in the Exact Many Body Formalism. Phys. Rev. D 2018, 98, 083002. [Google Scholar] [CrossRef]
- Patwardhan, A.V.; Cervia, M.J.; Balantekin, A.B. Eigenvalues and eigenstates of the many-body collective neutrino oscillation problem. Phys. Rev. D 2019, 99, 123013. [Google Scholar] [CrossRef]
- Cervia, M.J.; Patwardhan, A.V.; Balantekin, A.B.; Coppersmith, T.S.N.; Johnson, C.W. Entanglement and collective flavor oscillations in a dense neutrino gas. Phys. Rev. D 2019, 100, 083001. [Google Scholar] [CrossRef]
- Rrapaj, E. Exact solution of multiangle quantum many-body collective neutrino-flavor oscillations. Phys. Rev. C 2020, 101, 065805. [Google Scholar] [CrossRef]
- Roggero, A. Entanglement and Many-Body effects in Collective Neutrino Oscillations. arXiv 2021, arXiv:2102.10188. [Google Scholar] [CrossRef]
- Roggero, A. Dynamical Phase Transitions in models of Collective Neutrino Oscillations. arXiv 2021, arXiv:2103.11497. [Google Scholar] [CrossRef]
- Lesgourgues, J.; Mangano, G.; Miele, G.; Pastor, S. Neutrino Cosmology; Cambridge University Press: Cambridge, UK, 2013. [Google Scholar]
- Dolgov, A.D. Neutrinos in cosmology. Phys. Rept. 2002, 370, 333. [Google Scholar] [CrossRef]
- Mangano, G.; Miele, G.; Pastor, S.; Pinto, T.; Pisanti, O.; Serpico, P.D. Relic neutrino decoupling including flavor oscillations. Nucl. Phys. B 2005, 729, 221. [Google Scholar] [CrossRef]
- Bennett, J.J.; Buldgen, G.; Salas, P.F.D.; Drewes, M.; Gariazzo, S.; Pastor, S.; Wong, Y.Y.Y. Towards a precision calculation of Neff in the Standard Model II: Neutrino decoupling in the presence of flavour oscillations and finite-temperature QED. J. Cosmol. Astropart. Phys. 2021, 4, 073. [Google Scholar] [CrossRef]
- Froustey, J.; Pitrou, C.; Volpe, M.C. Neutrino decoupling including flavour oscillations and primordial nucleosynthesis. J. Cosmol. Astropart. Phys. 2020, 12, 015. [Google Scholar] [CrossRef]
- Mangano, G.; Miele, G.; Pastor, S.; Pisanti, O.; Sarikas, S. Updated BBN bounds on the cosmological lepton asymmetry for non-zero θ13. Phys. Lett. B 2012, 708, 1. [Google Scholar] [CrossRef]
- Giunti, C.; Laveder, M. 3 + 1 and 3 + 2 Sterile Neutrino Fits. Phys. Rev. D 2011, 84, 073008. [Google Scholar] [CrossRef]
- Kopp, J.; Machado, P.A.N.; Maltoni, M.; Schwetz, T. Sterile Neutrino Oscillations: The Global Picture. J. High Energy Phys. 2013, 2013, 50. [Google Scholar] [CrossRef]
- Dentler, M.; Hernández-Cabezudo, A.; Kopp, J.; Machado, P.A.N.; Maltoni, M.; Martinez-Soler, I.; Schwetz, T. Updated Global Analysis of Neutrino Oscillations in the Presence of eV-Scale Sterile Neutrinos. J. High Energy Phys. 2018, 8, 10. [Google Scholar] [CrossRef]
- Gariazzo, S.; Giunti, C.; Laveder, M.; Li, Y.F. Updated Global 3+1 Analysis of Short-BaseLine Neutrino Oscillations. J. High Energy Phys. 2017, 6, 135. [Google Scholar] [CrossRef]
- Diaz, A.; Argüelles, C.A.; Collin, G.H.; Conrad, J.M.; Shaevitz, M.H. Where Are We Light Sterile Neutrinos? Phys. Rept. 2020, 884, 1. [Google Scholar] [CrossRef]
- Böser, S.; Buck, C.; Giunti, C.; Lesgourgues, J.; Ludhova, L.; Mertens, S.; Schukraft, A.; Wurm, M. Status of Light Sterile Neutrino Searches. Prog. Part. Nucl. Phys. 2020, 111, 103736. [Google Scholar] [CrossRef]
- Abratenko, P.; MicroBooNE Collaboration. Search for an anomalous excess of inclusive charged-current νe interactions in the MicroBooNE experiment using Wire-Cell reconstruction. arXiv 2021, arXiv:2110.13978. [Google Scholar]
- Abratenko, P.; MicroBooNE Collaboration. Search for an Excess of Electron Neutrino Interactions in MicroBooNE Using Multiple Final State Topologies. arXiv 2021, arXiv:2110.14054. [Google Scholar]
- Abratenko, P.; MicroBooNE Collaboration. Search for an anomalous excess of charged-current νe interactions without pions in the final state with the MicroBooNE experiment. arXiv 2021, arXiv:2110.14065. [Google Scholar]
- Abratenko, P.; MicroBooNE Collaboration. Search for an anomalous excess of charged-current quasi-elastic νe interactions with the MicroBooNE experiment using Deep-Learning-based reconstruction. arXiv 2021, arXiv:2110.14080. [Google Scholar]
- Argüelles, C.A.; Esteban, I.; Hostert, M.; Kelly, K.J.; Kopp, J.; Machado, P.A.N.; Martinez-Soler, I.; Perez-Gonzalez, Y.F. MicroBooNE and the νe Interpretation of the MiniBooNE Low-Energy Excess. arXiv 2021, arXiv:2111.10359. [Google Scholar]
- Aguilar-Arevalo, A.A.; MiniBooNE Collaboration. MiniBooNE and MicroBooNE Joint Fit to a 3 + 1 Sterile Neutrino Scenario. arXiv 2022, arXiv:2201.01724. [Google Scholar]
- Barinov, V.V.; BEST Collaboration. Results from the Baksan Experiment on Sterile Transitions (BEST). arXiv 2021, arXiv:2109.11482. [Google Scholar]
- Kopeikin, V.; Skorokhvatov, M.; Titov, O. Reevaluating reactor antineutrino spectra with new measurements of the ratio between U235 and Pu239 β spectra. Phys. Rev. D 2021, 104, L071301. [Google Scholar] [CrossRef]
- Giunti, C.; Li, Y.F.; Ternes, C.A.; Xin, Z. Reactor antineutrino anomaly in light of recent flux model refinements. arXiv 2021, arXiv:2110.06820. [Google Scholar]
- Berryman, J.M.; Coloma, P.; Huber, P.; Schwetz, T.; Zhou, A. Statistical significance of the sterile-neutrino hypothesis in the context of reactor and gallium data. arXiv 2021, arXiv:2111.12530. [Google Scholar]
- Hagstotz, S.; de Salas, P.F.; Gariazzo, S.; Gerbino, M.; Lattanzi, M.; Vagnozzi, S.; Freese, K.; Pastor, S. Bounds on light sterile neutrino mass and mixing from cosmology and laboratory searches. Phys. Rev. D 2021, 104, 123524. [Google Scholar] [CrossRef]
- Mangano, G.; Serpico, P.D. A robust upper limit on Neff from BBN, circa 2011. Phys. Lett. B 2011, 701, 296. [Google Scholar] [CrossRef]
- Giusarma, E.; Corsi, M.; Archidiacono, M.; de Putter, R.; Melchiorri, A.; Mena, O.; Pandolfi, S. Constraints on massive sterile neutrino species from current and future cosmological data. Phys. Rev. D 2011, 83, 115023. [Google Scholar] [CrossRef]
- Lesgourgues, J.; Pastor, S. Neutrino mass from Cosmology. Adv. High Energy Phys. 2012, 2012, 608515. [Google Scholar] [CrossRef]
- Riemer-Sorensen, S.; Parkinson, D.; Davis, T.M. What is half a neutrino? Reviewing cosmological constraints on neutrinos and dark radiation. Publ. Astron. Soc. Austral. 2013, 30, e029. [Google Scholar] [CrossRef]
- Cooke, R.J.; Pettini, M.; Steidel, C.C. One Percent Determination of the Primordial Deuterium Abundance. Astrophys. J. 2018, 855, 102. [Google Scholar] [CrossRef]
- Grohs, E.B.; Bond, J.R.; Cooke, R.J.; Fuller, G.M.; Meyers, J.; Paris, M.W. Big Bang Nucleosynthesis and Neutrino Cosmology. arXiv 2019, arXiv:1903.09187. [Google Scholar]
- Hsyu, T.; Cooke, R.J.; Prochaska, J.X.; Bolte, M. The PHLEK Survey: A New Determination of the Primordial Helium Abundance. Astrophys. J. 2020, 896, 77. [Google Scholar] [CrossRef]
- Fields, B.D.; Olive, K.A.; Yeh, T.-H.; Young, C. Big-Bang Nucleosynthesis after Planck. J. Cosmol. Astropart. Phys. 2020, 3, 10. [Google Scholar] [CrossRef]
- Aghanim, N.; Planck Collaboration. Planck 2018 results. VI. Cosmological parameters. Astron. Astrophys. 2021, 641, A6. [Google Scholar] [CrossRef]
- Mirizzi, A.; Mangano, G.; Saviano, N.; Borriello, E.; Giunti, C.; Miele, G.; Pisanti, O. The strongest bounds on active-sterile neutrino mixing after Planck data. Phys. Lett. B 2013, 726, 8. [Google Scholar] [CrossRef][Green Version]
- Gariazzo, S.; de Salas, P.F.; Pastor, S. Thermalisation of sterile neutrinos in the early Universe in the 3+1 scheme with full mixing matrix. J. Cosmol. Astropart. Phys. 2019, 7, 14. [Google Scholar] [CrossRef]
- Mirizzi, A.; Saviano, N.; Miele, G.; Serpico, P.D. Light sterile neutrino production in the early universe with dynamical neutrino asymmetries. Phys. Rev. D 2012, 86, 053009. [Google Scholar] [CrossRef]
- Saviano, N.; Mirizzi, A.; Pisanti, O.; Serpico, P.D.; Mangano, G.; Miele, G. Multi-momentum and multi-flavour active-sterile neutrino oscillations in the early universe: Role of neutrino asymmetries and effects on nucleosynthesis. Phys. Rev. D 2013, 87, 073006. [Google Scholar] [CrossRef]
- Chu, X.; Dasgupta, B.; Kopp, J. Sterile neutrinos with secret interactions—Lasting friendship with cosmology. J. Cosmol. Astropart. Phys. 2015, 10, 011. [Google Scholar] [CrossRef]
- Hannestad, S.; Hansen, R.S.; Tram, T. How Self-Interactions can Reconcile Sterile Neutrinos with Cosmology. Phys. Rev. Lett. 2014, 112, 031802. [Google Scholar] [CrossRef]
- Saviano, N.; Pisanti, O.; Mangano, G.; Mirizzi, A. Unveiling secret interactions among sterile neutrinos with big-bang nucleosynthesis. Phys. Rev. D 2014, 90, 113009. [Google Scholar] [CrossRef]
- Mirizzi, A.; Mangano, G.; Pisanti, O.; Saviano, N. Collisional production of sterile neutrinos via secret interactions and cosmological implications. Phys. Rev. D 2015, 91, 025019. [Google Scholar] [CrossRef]
- Forastieri, F.; Lattanzi, M.; Mangano, G.; Mirizzi, A.; Natoli, P.; Saviano, N. Cosmic microwave background constraints on secret interactions among sterile neutrinos. J. Cosmol. Astropart. Phys. 2017, 07, 038. [Google Scholar] [CrossRef]
- Chu, X.; Dasgupta, B.; Dentler, M.; Kopp, J.; Saviano, N. Sterile neutrinos with secret interactions—Cosmological discord? J. Cosmol. Astropart. Phys. 2018, 11, 48. [Google Scholar] [CrossRef]
- Archidiacono, M.; Hannestad, S.; Hansen, R.S.; Tram, T. Cosmology with self-interacting sterile neutrinos and dark matter—A pseudoscalar model. Phys. Rev. D 2015, 91, 065021. [Google Scholar] [CrossRef]
- Archidiacono, M.; Gariazzo, S.; Giunti, C.; Hannestad, S.; Hansen, R.; Laveder, M.; Tram, T. Pseudoscalar—Sterile neutrino interactions: Reconciling the cosmos with neutrino oscillations. J. Cosmol. Astropart. Phys. 2016, 08, 067. [Google Scholar] [CrossRef]
- Archidiacono, M.; Gariazzo, S.; Giunti, C.; Hannestad, S.; Tram, T. Sterile Neutrino Self-Interactions: H0 Tension and Short-Baseline Anomalies. J. Cosmol. Astropart. Phys. 2020, 12, 029. [Google Scholar] [CrossRef]
- Corona, M.A.; Murgia, R.; Cadeddu, M.; Archidiacono, M.; Gariazzo, S.; Giunti, C.; Hannestad, S. Pseudoscalar sterile neutrino self-interactions in light of Planck, SPT and ACT data. arXiv 2021, arXiv:2112.00037. [Google Scholar]
- Valentino, E.D.; Gariazzo, S.; Giunti, C.; Mena, O.; Pan, S.; Yang, W. Minimal dark energy: Key to sterile neutrino and Hubble constant tensions? arXiv 2021, arXiv:2110.03990. [Google Scholar]
- Adhikari, R.; Agostini, M.; Anh Ky, N.; Araki, T.; Archidiacono, M.; Bahr, M.; Baur, J.; Behrens, J.; Bezrukov, F.; Bhupal Dev, P.S.; et al. A White Paper on keV Sterile Neutrino Dark Matter. J. Cosmol. Astropart. Phys. 2017, 01, 025. [Google Scholar] [CrossRef]
- Abazajian, K.N. Sterile neutrinos in cosmology. Phys. Rept. 2017, 711–712, 1–28. [Google Scholar] [CrossRef]
- Boyarsky, A.; Drewes, M.; Lasserre, T.; Mertens, S.; Ruchayskiy, O. Sterile neutrino Dark Matter. Prog. Part. Nucl. Phys. 2019, 104, 1. [Google Scholar] [CrossRef]
- Bulbul, E.; Markevitch, M.; Foster, A.; Smith, R.K.; Loewenstein, M.; Randall, S.W. Detection of An Unidentified Emission Line in the Stacked X-ray spectrum of Galaxy Clusters. Astrophys. J. 2014, 789, 13. [Google Scholar] [CrossRef]
- Boyarsky, A.; Ruchayskiy, O.; Iakubovskyi, D.; Franse, J. Unidentified Line in X-Ray Spectra of the Andromeda Galaxy and Perseus Galaxy Cluster. Phys. Rev. Lett. 2014, 113, 251301. [Google Scholar] [CrossRef]
- Murgia, R.; Merle, A.; Viel, M.; Totzauer, M.; Schneider, A. ”Non-cold” dark matter at small scales: A general approach. J. Cosmol. Astropart. Phys. 2017, 11, 046. [Google Scholar] [CrossRef]
- Dodelson, S.; Widrow, L.M. Sterile-neutrinos as dark matter. Phys. Rev. Lett. 1994, 72, 17. [Google Scholar] [CrossRef] [PubMed]
- Tremaine, S.; Gunn, J.E. Dynamical Role of Light Neutral Leptons in Cosmology. Phys. Rev. Lett. 1979, 42, 407. [Google Scholar] [CrossRef]
- Boyarsky, A.; Ruchayskiy, O.; Iakubovskyi, D. A Lower bound on the mass of Dark Matter particles. J. Cosmol. Astropart. Phys. 2009, 03, 005. [Google Scholar] [CrossRef]
- Gorbunov, D.; Khmelnitsky, A.; Rubakov, V. Constraining sterile neutrino dark matter by phase-space density observations. J. Cosmol. Astropart. Phys. 2008, 10, 041. [Google Scholar] [CrossRef]
- Horiuchi, S.; Humphrey, P.J.; Onorbe, J.; Abazajian, K.N.; Kaplinghat, M.; Garrison-Kimmel, S. Sterile neutrino dark matter bounds from galaxies of the Local Group. Phys. Rev. D 2014, 89, 025017. [Google Scholar] [CrossRef]
- Ng, K.C.Y.; Roach, B.M.; Perez, K.; Beacom, J.F.; Horiuchi, S.; Krivonos, R.; Wik, D.R. New Constraints on Sterile Neutrino Dark Matter from NuSTAR M31 Observations. Phys. Rev. D 2019, 99, 083005. [Google Scholar] [CrossRef]
- Shi, X.-D.; Fuller, G.M. A New dark matter candidate: Nonthermal sterile neutrinos. Phys. Rev. Lett. 1999, 82, 2832. [Google Scholar] [CrossRef]
- Cherry, J.F.; Horiuchi, S. Closing in on Resonantly Produced Sterile Neutrino Dark Matter. Phys. Rev. D 2017, 95, 083015. [Google Scholar] [CrossRef]
- Wang, M.-Y.; Cherry, J.F.; Horiuchi, S.; Strigari, L.E. Bounds on Resonantly-Produced Sterile Neutrinos from Phase Space Densities of Milky Way Dwarf Galaxies. arXiv 2017, arXiv:1712.04597. [Google Scholar]
- Schneider, A. Testing the sterile neutrino dark matter paradigm with astrophysical observations. PoS NOW 2017, 2016, 093. [Google Scholar] [CrossRef][Green Version]
- Schneider, A. Astrophysical constraints on resonantly produced sterile neutrino dark matter. J. Cosmol. Astropart. Phys. 2016, 04, 059. [Google Scholar] [CrossRef][Green Version]
- Dessert, C.; Rodd, N.L.; Safdi, B.R. The dark matter interpretation of the 3.5-keV line is inconsistent with blank-sky observations. Science 2020, 367, 1465. [Google Scholar] [CrossRef] [PubMed]
- Enzi, W.; Murgia, R.; Newton, O.; Vegetti, S.; Frenk, C.; Viel, M.; Cautun, M.; Fassnacht, C.D.; Auger, M.; Despali, G.; et al. Joint Constraints Therm. Relic Dark Matter Strong Gravitational Lensing, Lyman-α For. Milky Way Satellites. Mon. Not. R. Astron. Soc. 2021, 506, 4. [Google Scholar] [CrossRef]
- Gouvêa, A.D.; Sen, M.; Tangarife, W.; Zhang, Y. Dodelson-Widrow Mechanism in the Presence of Self-Interacting Neutrinos. Phys. Rev. Lett. 2020, 124, 081802. [Google Scholar] [CrossRef]
- Kelly, K.J.; Sen, M.; Tangarife, W.; Zhang, Y. Origin of sterile neutrino dark matter via secret neutrino interactions with vector bosons. Phys. Rev. D 2020, 101, 115031. [Google Scholar] [CrossRef]
- Johns, L.; Fuller, G.M. Self-interacting sterile neutrino dark matter: The heavy-mediator case. Phys. Rev. D 2019, 100, 023533. [Google Scholar] [CrossRef]
- Asaka, T.; Blanchet, S.; Shaposhnikov, M. The nuMSM, dark matter and neutrino masses. Phys. Lett. B 2005, 631, 151. [Google Scholar] [CrossRef]
- Asaka, T.; Shaposhnikov, M. The νMSM, dark matter and baryon asymmetry of the universe. Phys. Lett. B 2005, 620, 17. [Google Scholar] [CrossRef]
- Alekhin, S.; SHiP Collaboration. A facility to Search for Hidden Particles at the CERN SPS: The SHiP physics case. Rept. Prog. Phys. 2016, 79, 124201. [Google Scholar] [CrossRef]
- Chun, E.J.; Das, A.; Mandal, S.; Mitra, M.; Sinha, N. Sensitivity of Lepton Number Violating Meson Decays in Different Experiments. Phys. Rev. D 2019, 100, 095022. [Google Scholar] [CrossRef]
- Orloff, J.; Rozanov, A.N.; Santoni, C. Limits on the mixing of tau neutrino to heavy neutrinos. Phys. Lett. B 2002, 550, 8. [Google Scholar] [CrossRef]
- Dolgov, A.D.; Hansen, S.H.; Raffelt, G.; Semikoz, D.V. Heavy sterile neutrinos: Bounds from big bang nucleosynthesis and SN1987A. Nucl. Phys. B 2000, 590, 562. [Google Scholar] [CrossRef]
- Fuller, G.M.; Kusenko, A.; Petraki, K. Heavy sterile neutrinos and supernova explosions. Phys. Lett. B 2009, 670, 281. [Google Scholar] [CrossRef]
- Mastrototaro, L.; Mirizzi, A.; Serpico, P.D.; Esmaili, A. Heavy sterile neutrino emission in core-collapse supernovae: Constraints and signatures. J. Cosmol. Astropart. Phys. 2020, 01, 010. [Google Scholar] [CrossRef]
- Mastrototaro, L.; Serpico, P.D.; Mirizzi, A.; Saviano, N. Massive sterile neutrinos in the early Universe: From thermal decoupling to cosmological constraints. Phys. Rev. D 2021, 104, 016026. [Google Scholar] [CrossRef]
- Colgate, S.A.; Johnson, M.H. Hydrodynamic Origin of Cosmic Rays. Phys. Rev. Lett. 1960, 5, 235. [Google Scholar] [CrossRef]
- Colgate, S.A.; White, R.H. The Hydrodynamic Behavior of Supernovae Explosions. Astrophys. J. 1966, 143, 626. [Google Scholar] [CrossRef]
- Bethe, H.A.; Wilson, J.R. Revival of a stalled supernova shock by neutrino heating. Astrophys. J. 1985, 295, 14. [Google Scholar] [CrossRef]
- Gamow, G.; Schoenberg, M. The Possible Role of Neutrinos in Stellar Evolution. Phys. Rev. 1940, 58, 1117. [Google Scholar] [CrossRef]
- Gamow, G.; Schoenberg, M. Neutrino Theory of Stellar Collapse. Phys. Rev. 1941, 59, 539. [Google Scholar] [CrossRef]
- Mirizzi, A.; Tamborra, I.; Janka, H.-T.; Saviano, N.; Scholberg, K.; Bollig, R.; Hudepohl, L.; Chakraborty, S. Supernova Neutrinos: Production, Oscillations and Detection. Riv. Nuovo Cim. 2016, 39, 1. [Google Scholar] [CrossRef]
- Janka, H.T. Neutrino Emission from Supernovae. arXiv 2017, arXiv:1702.08713. [Google Scholar]
- Burrows, A.; Vartanyan, D. Core-Collapse Supernova Explosion Theory. Nature 2021, 589, 29. [Google Scholar] [CrossRef]
- Mazurek, T.J. Degeneracy effects on neutrino mass ejection in supernovae. Nature 1974, 252, 287. [Google Scholar] [CrossRef]
- Freedman, D.Z. Coherent Neutrino Nucleus Scattering as a Probe of the Weak Neutral Current. Phys. Rev. D 1974, 9, 1389. [Google Scholar] [CrossRef]
- Mazurek, T.J. Chemical Potential Effects on Neutrino Diffusion in Supernovae. Astrophys. Space Sci. 1975, 35, 117. [Google Scholar] [CrossRef]
- Mazurek, T.J. Stellar collapse and transport supernovae with neutrino degeneracy. Comments Astrophys. 1977, 7, 77. [Google Scholar]
- Burbidge, M.E.; Burbidge, G.R.; Fowler, W.A.; Hoyle, F. Synthesis of the elements in stars. Rev. Mod. Phys. 1957, 29, 547. [Google Scholar] [CrossRef]
- Qian, Y.-Z.; Fuller, G.M.; Mathews, G.J.; Mayle, R.W.; Wilson, J.R.; Woosley, S.E. Connection between flavor-mixing of cosmologically significant neutrinos and heavy element nucleosynthesis in supernovae. Phys. Rev. Lett. 1993, 71, 1965. [Google Scholar] [CrossRef] [PubMed]
- Chakraborty, S.; Choubey, S.; Goswami, S.; Kar, K. Collective Flavor Oscillations Of Supernova Neutrinos and r-Process Nucleosynthesis. J. Cosmol. Astropart. Phys. 2010, 06, 007. [Google Scholar] [CrossRef]
- Duan, H.; Friedland, A.; McLaughlin, G.; Surman, R. The influence of collective neutrino oscillations on a supernova r-process. J. Phys. G 2011, 38, 035201. [Google Scholar] [CrossRef]
- Xiong, Z.; Sieverding, A.; Sen, M.; Qian, Y.-Z. Potential Impact of Fast Flavor Oscillations on Neutrino-driven Winds and Their Nucleosynthesis. Astrophys. J. 2020, 900, 144. [Google Scholar] [CrossRef]
- Fuller, G.M.; Mayle, R.; Meyer, B.S.; Wilson, J.R. Can a Closure Mass Neutrino Help Solve the Supernova Shock Reheating Problem? Astrophys. J. 1992, 389, 517. [Google Scholar] [CrossRef][Green Version]
- Dighe, A.S.; Smirnov, A.Y. Identifying the neutrino mass spectrum from the neutrino burst from a supernova. Phys. Rev. D 2000, 62, 033007. [Google Scholar] [CrossRef]
- Schirato, R.C.; Fuller, G.M. Connection between supernova shocks, flavor transformation, and the neutrino signal. arXiv 2002, arXiv:astro-ph/0205390. [Google Scholar]
- Fogli, G.L.; Lisi, E.; Montanino, D.; Mirizzi, A. Analysis of energy and time dependence of supernova shock effects on neutrino crossing probabilities. Phys. Rev. D 2003, 68, 033005. [Google Scholar] [CrossRef]
- Fogli, G.L.; Lisi, E.; Mirizzi, A.; Montanino, D. Probing supernova shock waves and neutrino flavor transitions in next-generation water-Cerenkov detectors. J. Cosmol. Astropart. Phys. 2005, 4, 002. [Google Scholar] [CrossRef]
- Tomas, R.; Kachelriess, M.; Raffelt, G.; Dighe, A.; Janka, H.T.; Scheck, L. Neutrino signatures of supernova shock and reverse shock propagation. J. Cosmol. Astropart. Phys. 2004, 9, 015. [Google Scholar] [CrossRef]
- Dasgupta, B.; Dighe, A. Phase effects in neutrino conversions during a supernova shock wave. Phys. Rev. D 2007, 75, 093002. [Google Scholar] [CrossRef]
- Choubey, S.; Harries, N.P.; Ross, G.G. Probing neutrino oscillations from supernovae shock waves via the IceCube detector. Phys. Rev. D 2006, 74, 053010. [Google Scholar] [CrossRef]
- Kneller, J.P.; McLaughlin, G.C.; Brockman, J. Oscillation Effects and Time Variation of the Supernova Neutrino Signal. Phys. Rev. D 2008, 77, 045023. [Google Scholar] [CrossRef]
- Friedland, A.; Mukhopadhyay, P. Neutrino signatures of near-critical supernova outflows. arXiv 2020, arXiv:2009.10059. [Google Scholar]
- Fogli, G.L.; Lisi, E.; Mirizzi, A.; Montanino, D. Damping of supernova neutrino transitions in stochastic shock-wave density profiles. J. Cosmol. Astropart. Phys. 2006, 06, 012. [Google Scholar] [CrossRef]
- Friedland, A.; Gruzinov, A. Neutrino signatures of supernova turbulence. arXiv 2006, arXiv:astro-ph/0607244. [Google Scholar]
- Kneller, J.P.; Volpe, C. Turbulence effects on supernova neutrinos. Phys. Rev. D 2010, 82, 123004. [Google Scholar] [CrossRef]
- Lund, T.; Kneller, J.P. Combining collective, MSW, and turbulence effects in supernova neutrino flavor evolution. Phys. Rev. D 2013, 88, 023008. [Google Scholar] [CrossRef]
- Loreti, F.N.; Qian, Y.Z.; Fuller, G.M.; Balantekin, A.B. Effects of random density fluctuations on matter enhanced neutrino flavor transitions in supernovae and implications for supernova dynamics and nucleosynthesis. Phys. Rev. D 1995, 52, 6664. [Google Scholar] [CrossRef]
- Choubey, S.; Harries, N.P.; Ross, G.G. Turbulent supernova shock waves and the sterile neutrino signature in megaton water detectors. Phys. Rev. D 2007, 76, 073013. [Google Scholar] [CrossRef]
- Benatti, F.; Floreanini, R. Dissipative neutrino oscillations in randomly fluctuating matter. Phys. Rev. D 2005, 71, 013003. [Google Scholar] [CrossRef]
- Kneller, J.P.; Mauney, A.W. Consequences of large θ13 for the turbulence signatures in supernova neutrinos. Phys. Rev. D 2013, 88, 025004. [Google Scholar] [CrossRef]
- Sawyer, R.F. The multi-angle instability in dense neutrino systems. Phys. Rev. D 2009, 79, 105003. [Google Scholar] [CrossRef]
- Banerjee, A.; Dighe, A.; Raffelt, G. Linearized flavor-stability analysis of dense neutrino streams. Phys. Rev. D 2011, 84, 053013. [Google Scholar] [CrossRef]
- Morinaga, T. Fast neutrino flavor instability and neutrino flavor lepton number crossings. arXiv 2021, arXiv:2103.15267. [Google Scholar]
- Dasgupta, B. Collective Neutrino Flavor Instability Requires Spectral Crossing. arXiv 2021, arXiv:2110.00192. [Google Scholar]
- Chakraborty, S.; Hansen, R.; Izaguirre, I.; Raffelt, G. Collective neutrino flavor conversion: Recent developments. Nucl. Phys. B 2016, 908, 366. [Google Scholar] [CrossRef]
- Tamborra, I.; Shalgar, S. New Developments in Flavor Evolution of a Dense Neutrino Gas. Ann. Rev. Nucl. Part. Sci. 2021, 71, 165. [Google Scholar] [CrossRef]
- Duan, H.; Fuller, G.M.; Qian, Y.-Z. Collective neutrino flavor transformation in supernovae. Phys. Rev. D 2006, 74, 123004. [Google Scholar] [CrossRef]
- Duan, H.; Fuller, G.M.; Carlson, J.; Qian, Y.-Z. Simulation of Coherent Non-Linear Neutrino Flavor Transformation in the Supernova Environment. 1. Correlated Neutrino Trajectories. Phys. Rev. D 2006, 74, 105014. [Google Scholar] [CrossRef]
- Duan, H.; Fuller, G.M.; Carlson, J.; Qian, Y.-Z. Coherent Development of Neutrino Flavor in the Supernova Environment. Phys. Rev. Lett. 2006, 97, 241101. [Google Scholar] [CrossRef] [PubMed]
- Hannestad, S.; Raffelt, G.G.; Sigl, G.; Wong, Y.Y.Y. Self-induced conversion in dense neutrino gases: Pendulum in flavour space. Phys. Rev. D 2007, 74, 105010. [Google Scholar] [CrossRef]
- Fogli, G.L.; Lisi, E.; Marrone, A.; Mirizzi, A. Collective neutrino flavor transitions in supernovae and the role of trajectory averaging. J. Cosmol. Astropart. Phys. 2007, 12, 010. [Google Scholar] [CrossRef]
- Duan, H.; Fuller, G.M.; Carlson, J.; Qian, Y.-Z. Analysis of Collective Neutrino Flavor Transformation in Supernovae. Phys. Rev. D 2007, 75, 125005. [Google Scholar] [CrossRef]
- Duan, H.; Fuller, G.M.; Carlson, J.; Qian, Y.-Z. Neutrino Mass Hierarchy and Stepwise Spectral Swapping of Supernova Neutrino Flavors. Phys. Rev. Lett. 2007, 99, 241802. [Google Scholar] [CrossRef]
- Raffelt, G.G.; Smirnov, A.Y. Self-induced spectral splits in supernova neutrino fluxes. Phys. Rev. D 2007, 76, 081301, Erratum in Phys. Rev. D 2008, 77, 029903. [Google Scholar] [CrossRef]
- Fogli, G.L.; Lisi, E.; Marrone, A.; Mirizzi, A.; Tamborra, I. Low-energy spectral features of supernova (anti)neutrinos in inverted hierarchy. Phys. Rev. D 2008, 78, 097301. [Google Scholar] [CrossRef]
- Raffelt, G.G.; Smirnov, A.Y. Adiabaticity and spectral splits in collective neutrino transformations. Phys. Rev. D 2007, 76, 125008. [Google Scholar] [CrossRef]
- Galais, S.; Volpe, C. The neutrino spectral split in core-collapse supernovae: A magnetic resonance phenomenon. Phys. Rev. D 2011, 84, 085005. [Google Scholar] [CrossRef]
- Esteban-Pretel, A.; Pastor, S.; Tomas, R.; Raffelt, G.G.; Sigl, G. Decoherence in supernova neutrino transformations suppressed by deleptonization. Phys. Rev. D 2007, 76, 125018. [Google Scholar] [CrossRef]
- Fogli, G.; Lisi, E.; Marrone, A.; Tamborra, I. Supernova neutrinos and antineutrinos: Ternary luminosity diagram and spectral split patterns. J. Cosmol. Astropart. Phys. 2009, 2009, 002. [Google Scholar] [CrossRef]
- Chakraborty, S.; Raffelt, G.; Janka, H.-T.; Müller, B. Supernova deleptonization asymmetry: Impact on self-induced flavor conversion. Phys. Rev. D 2015, 92, 105002. [Google Scholar] [CrossRef]
- Dasgupta, B.; Dighe, A.; Raffelt, G.G.; Smirnov, A.Y. Multiple Spectral Splits of Supernova Neutrinos. Phys. Rev. Lett. 2009, 103, 051105. [Google Scholar] [CrossRef] [PubMed]
- Chakraborty, S.; Fischer, T.; Mirizzi, A.; Saviano, N.; Tomas, R. No collective neutrino flavor conversions during the supernova accretion phase. Phys. Rev. Lett. 2011, 107, 151101. [Google Scholar] [CrossRef]
- Chakraborty, S.; Fischer, T.; Mirizzi, A.; Saviano, N.; Tomas, R. Analysis of matter suppression in collective neutrino oscillations during the supernova accretion phase. Phys. Rev. D 2011, 84, 025002. [Google Scholar] [CrossRef]
- Dasgupta, B.; O’Connor, E.P.; Ott, C.D. The Role of Collective Neutrino Flavor Oscillations in Core-Collapse Supernova Shock Revival. Phys. Rev. D 2012, 85, 065008. [Google Scholar] [CrossRef]
- Sarikas, S.; Raffelt, G.G.; Hudepohl, L.; Janka, H.-T. Suppression of Self-Induced Flavor Conversion in the Supernova Accretion Phase. Phys. Rev. Lett. 2012, 108, 061101. [Google Scholar] [CrossRef] [PubMed]
- Saviano, N.; Chakraborty, S.; Fischer, T.; Mirizzi, A. Stability analysis of collective neutrino oscillations in the supernova accretion phase with realistic energy and angle distributions. Phys. Rev. D 2012, 85, 113002. [Google Scholar] [CrossRef]
- Chakraborty, S.; Mirizzi, A.; Saviano, N.; Seixas, D.d.S. Suppression of the multi-azimuthal-angle instability in dense neutrino gas during supernova accretion phase. Phys. Rev. D 2014, 89, 093001. [Google Scholar] [CrossRef]
- Mirizzi, A.; Tomas, R. Multi-angle effects in self-induced oscillations for different supernova neutrino fluxes. Phys. Rev. D 2011, 84, 033013. [Google Scholar] [CrossRef]
- Cherry, J.F.; Fuller, G.M.; Carlson, J.; Duan, H.; Qian, Y.-Z. Multi-Angle Simulation of Flavor Evolution in the Neutrino Neutronization Burst From an O-Ne-Mg Core-Collapse Supernova. Phys. Rev. D 2010, 82, 085025. [Google Scholar] [CrossRef]
- Mirizzi, A.; Serpico, P.D. Instability in the Dense Supernova Neutrino Gas with Flavor-Dependent Angular Distributions. Phys. Rev. Lett. 2012, 108, 231102. [Google Scholar] [CrossRef]
- Mirizzi, A.; Serpico, P.D. Flavor Stability Analysis of Dense Supernova Neutrinos with Flavor-Dependent Angular Distributions. Phys. Rev. D 2012, 86, 085010. [Google Scholar] [CrossRef]
- Dasgupta, B.; Dighe, A. Collective three-flavor oscillations of supernova neutrinos. Phys. Rev. D 2008, 77, 113002. [Google Scholar] [CrossRef]
- Duan, H.; Fuller, G.M.; Qian, Y.-Z. Stepwise spectral swapping with three neutrino flavors. Phys. Rev. D 2008, 77, 085016. [Google Scholar] [CrossRef]
- Fogli, G.; Lisi, E.; Marrone, A.; Tamborra, I. Supernova neutrino three-flavor evolution with dominant collective effects. J. Cosmol. Astropart. Phys. 2009, 04, 030. [Google Scholar] [CrossRef]
- Dasgupta, B.; Raffelt, G.G.; Tamborra, I. Triggering collective oscillations by three-flavor effects. Phys. Rev. D 2010, 81, 073004. [Google Scholar] [CrossRef]
- Friedland, A. Self-refraction of supernova neutrinos: Mixed spectra and three-flavor instabilities. Phys. Rev. Lett. 2010, 104, 191102. [Google Scholar] [CrossRef]
- Dasgupta, B.; Mirizzi, A.; Tamborra, I.; Tomas, R. Neutrino mass hierarchy and three-flavor spectral splits of supernova neutrinos. Phys. Rev. D 2010, 81, 093008. [Google Scholar] [CrossRef]
- Raffelt, G.; Sarikas, S.; de Sousa Seixas, D. Axial Symmetry Break. Self-Induc. Flavor Convers. Supernova Neutrino Fluxes. Phys. Rev. Lett. 2013, 111, 091101. [Google Scholar] [CrossRef]
- Raffelt, G.; de Sousa Seixas, D. Neutrino Flavor Pendul. Both Mass Hierarchies. Phys. Rev. D 2013, 88, 045031. [Google Scholar] [CrossRef]
- Duan, H. Flavor Oscillation Modes In Dense Neutrino Media. Phys. Rev. D 2013, 88, 125008. [Google Scholar] [CrossRef]
- Mirizzi, A. Multi-azimuthal-angle effects in self-induced supernova neutrino flavor conversions without axial symmetry. Phys. Rev. D 2013, 88, 073004. [Google Scholar] [CrossRef]
- Chakraborty, S.; Mirizzi, A. Multi-azimuthal-angle instability for different supernova neutrino fluxes. Phys. Rev. D 2014, 90, 033004. [Google Scholar] [CrossRef]
- Mangano, G.; Mirizzi, A.; Saviano, N. Damping the neutrino flavor pendulum by breaking homogeneity. Phys. Rev. D 2014, 89, 073017. [Google Scholar] [CrossRef]
- Duan, H.; Shalgar, S. Flavor instabilities in the neutrino line model. Phys. Lett. B 2015, 747, 139. [Google Scholar] [CrossRef]
- Mirizzi, A.; Mangano, G.; Saviano, N. Self-induced flavor instabilities of a dense neutrino stream in a two-dimensional model. Phys. Rev. D 2015, 92, 021702. [Google Scholar] [CrossRef]
- Mirizzi, A. Breaking the symmetries in self-induced flavor conversions of neutrino beams from a ring. Phys. Rev. D 2015, 92, 105020. [Google Scholar] [CrossRef]
- Duan, H. Collective neutrino oscillations and spontaneous symmetry breaking. Int. J. Mod. Phys. E 2015, 24, 1541008. [Google Scholar] [CrossRef]
- Abbar, S.; Duan, H. Neutrino flavor instabilities in a time-dependent supernova model. Phys. Lett. B 2015, 751, 43. [Google Scholar] [CrossRef]
- Chakraborty, S.; Hansen, R.S.; Izaguirre, I.; Raffelt, G. Self-induced flavor conversion of supernova neutrinos on small scales. J. Cosmol. Astropart. Phys. 2016, 01, 028. [Google Scholar] [CrossRef]
- Dasgupta, B.; Mirizzi, A. Temporal Instability Enables Neutrino Flavor Conversions Deep Inside Supernovae. Phys. Rev. D 2015, 92, 125030. [Google Scholar] [CrossRef]
- Capozzi, F.; Dasgupta, B.; Mirizzi, A. Self-induced temporal instability from a neutrino antenna. J. Cosmol. Astropart. Phys. 2016, 04, 043. [Google Scholar] [CrossRef]
- Abbar, S. Turbulence Fingerprint on Collective Oscillations of Supernova Neutrinos. Phys. Rev. D 2021, 103, 045014. [Google Scholar] [CrossRef]
- Cherry, J.F.; Carlson, J.; Friedland, A.; Fuller, G.M.; Vlasenko, A. Neutrino scattering and flavor transformation in supernovae. Phys. Rev. Lett. 2012, 108, 261104. [Google Scholar] [CrossRef] [PubMed]
- Cherry, J.F.; Carlson, J.; Friedland, A.; Fuller, G.M.; Vlasenko, A. Halo Modification of a Supernova Neutronization Neutrino Burst. Phys. Rev. D 2013, 87, 085037. [Google Scholar] [CrossRef]
- Sarikas, S.; Tamborra, I.; Raffelt, G.; Hudepohl, L.; Janka, H.-T. Supernova neutrino halo and the suppression of self-induced flavor conversion. Phys. Rev. D 2012, 85, 113007. [Google Scholar] [CrossRef]
- Zaizen, M.; Cherry, J.F.; Takiwaki, T.; Horiuchi, S.; Kotake, K.; Umeda, H.; Yoshida, T. Neutrino halo effect on collective neutrino oscillation in iron core-collapse supernova model of a 9.6 M⊙ star. J. Cosmol. Astropart. Phys. 2020, 06, 011. [Google Scholar] [CrossRef]
- Cherry, J.F.; Fuller, G.M.; Horiuchi, S.; Kotake, K.; Takiwaki, T.; Fischer, T. Time of Flight and Supernova Progenitor Effects on the Neutrino Halo. Phys. Rev. D 2020, 102, 023022. [Google Scholar] [CrossRef]
- Zaizen, M.; Cherry, J.F.; Takiwaki, T.; Horiuchi, S.; Kotake, K.; Umeda, H.; Yoshida, T. Collective flavor conversion including the neutrino halo in core-collapse supernovae. J. Phys. Conf. Ser. 2020, 1468, 012137. [Google Scholar] [CrossRef]
- Sawyer, R.F. Speed-up of neutrino transformations in a supernova environment. Phys. Rev. D 2005, 72, 045003. [Google Scholar] [CrossRef]
- Sawyer, R.F. Neutrino cloud instabilities just above the neutrino sphere of a supernova. Phys. Rev. Lett. 2016, 116, 081101. [Google Scholar] [CrossRef]
- Izaguirre, I.; Raffelt, G.; Tamborra, I. Fast Pairwise Conversion of Supernova Neutrinos: A Dispersion-Relation Approach. Phys. Rev. Lett. 2017, 118, 021101. [Google Scholar] [CrossRef] [PubMed]
- Capozzi, F.; Dasgupta, B.; Lisi, E.; Marrone, A.; Mirizzi, A. Fast flavor conversions of supernova neutrinos: Classifying instabilities via dispersion relations. Phys. Rev. D 2017, 96, 043016. [Google Scholar] [CrossRef]
- Yi, C.; Ma, L.; Martin, J.D.; Duan, H. Dispersion relation of the fast neutrino oscillation wave. Phys. Rev. D 2019, 99, 063005. [Google Scholar] [CrossRef]
- Airen, S.; Capozzi, F.; Chakraborty, S.; Dasgupta, B.; Raffelt, G.; Stirner, T. Normal-mode Analysis for Collective Neutrino Oscillations. J. Cosmol. Astropart. Phys. 2018, 12, 019. [Google Scholar] [CrossRef]
- Capozzi, F.; Raffelt, G.; Stirner, T. Fast Neutrino Flavor Conversion: Collective Motion vs. Decoherence. J. Cosmol. Astropart. Phys. 2019, 09, 002. [Google Scholar] [CrossRef]
- Chakraborty, M.; Chakraborty, S. Three flavor neutrino conversions in supernovae: Slow & fast instabilities. J. Cosmol. Astropart. Phys. 2020, 01, 005. [Google Scholar] [CrossRef]
- Dasgupta, B.; Sen, M. Fast Neutrino Flavor Conversion as Oscillations in a Quartic Potential. Phys. Rev. D 2018, 97, 023017. [Google Scholar] [CrossRef]
- Johns, L.; Nagakura, H.; Fuller, G.M.; Burrows, A. Neutrino oscillations in supernovae: Angular moments and fast instabilities. Phys. Rev. D 2020, 101, 043009. [Google Scholar] [CrossRef]
- Padilla-Gay, I.; Tamborra, I.; Raffelt, G.G. Neutrino flavor pendulum reloaded: The case of fast pairwise conversion. arXiv 2021, arXiv:2109.14627. [Google Scholar]
- Dasgupta, B.; Mirizzi, A.; Sen, M. Simple method of diagnosing fast flavor conversions of supernova neutrinos. Phys. Rev. D 2018, 98, 103001. [Google Scholar] [CrossRef]
- Abbar, S. Searching for Fast Neutrino Flavor Conversion Modes in Core-collapse Supernova Simulations. J. Cosmol. Astropart. Phys. 2020, 05, 027. [Google Scholar] [CrossRef]
- Nagakura, H.; Johns, L. Constructing angular distributions of neutrinos in core-collapse supernovae from zeroth and first moments calibrated by full Boltzmann neutrino transport. Phys. Rev. D 2021, 103, 123025. [Google Scholar] [CrossRef]
- Nagakura, H.; Johns, L. A new method for detecting fast neutrino flavor conversions in core-collapse supernova models with two-moment neutrino transport. arXiv 2021, arXiv:2106.02650. [Google Scholar] [CrossRef]
- Nagakura, H.; Johns, L.; Burrows, A.; Fuller, G.M. Where, when and why: Occurrence of fast-pairwise collective neutrino oscillation in three-dimensional core-collapse supernova models. arXiv 2021, arXiv:2108.07281. [Google Scholar] [CrossRef]
- Tamborra, I.; Huedepohl, L.; Raffelt, G.; Janka, H.-T. Flavor-dependent neutrino angular distribution in core-collapse supernovae. Astrophys. J. 2017, 839, 132. [Google Scholar] [CrossRef]
- Azari, M.D.; Yamada, S.; Morinaga, T.; Iwakami, W.; Okawa, H.; Nagakura, H.; Sumiyoshi, K. Linear Analysis of Fast-Pairwise Collective Neutrino Oscillations in Core-Collapse Supernovae based on the Results of Boltzmann Simulations. Phys. Rev. D 2019, 99, 103011. [Google Scholar] [CrossRef]
- Azari, M.D.; Yamada, S.; Morinaga, T.; Nagakura, H.; Furusawa, S.; Harada, A.; Okawa, H.; Iwakami, W.; Sumiyoshi, K. Fast collective neutrino oscillations inside the neutrino sphere in core-collapse supernovae. Phys. Rev. D 2020, 101, 023018. [Google Scholar] [CrossRef]
- Abbar, S.; Duan, H.; Sumiyoshi, K.; Takiwaki, T.; Volpe, M.C. Fast Neutrino Flavor Conversion Modes in Multidimensional Core-collapse Supernova Models: The Role of the Asymmetric Neutrino Distributions. Phys. Rev. D 2020, 101, 043016. [Google Scholar] [CrossRef]
- Glas, R.; Janka, H.T.; Capozzi, F.; Sen, M.; Dasgupta, B.; Mirizzi, A.; Sigl, G. Fast Neutrino Flavor Instability in the Neutron-star Convection Layer of Three-dimensional Supernova Models. Phys. Rev. D 2020, 101, 063001. [Google Scholar] [CrossRef]
- Abbar, S.; Duan, H.; Sumiyoshi, K.; Takiwaki, T.; Volpe, M.C. On the occurrence of fast neutrino flavor conversions in multidimensional supernova models. Phys. Rev. D 2019, 100, 043004. [Google Scholar] [CrossRef]
- Nagakura, H.; Morinaga, T.; Kato, C.; Yamada, S. Fast-pairwise collective neutrino oscillations associated with asymmetric neutrino emissions in core-collapse supernova. arXiv 2019, arXiv:1910.04288. [Google Scholar]
- Tamborra, I.; Hanke, F.; Janka, H.-T.; Müller, B.; Raffelt, G.G.; Marek, A. Self-sustained asymmetry of lepton-number emission: A new phenomenon during the supernova shock-accretion phase in three dimensions. Astrophys. J. 2014, 792, 96. [Google Scholar] [CrossRef]
- O’Connor, E.P.; Couch, S.M. Exploring Fundamentally Three-dimensional Phenomena in High-fidelity Simulations of Core-collapse Supernovae. Astrophys. J. 2018, 865, 81. [Google Scholar] [CrossRef]
- Glas, R.; Janka, H.T.; Melson, T.; Stockinger, G.; Just, O. Effects of LESA in Three-Dimensional Supernova Simulations with Multi-Dimensional and Ray-by-Ray-plus Neutrino Transport. arXiv 2018, arXiv:1809.10150. [Google Scholar]
- Nagakura, H.; Sumiyoshi, K.; Yamada, S. Possible early linear acceleration of proto-neutron stars via asymmetric neutrino emission in core-collapse supernovae. Astrophys. J. Lett. 2019, 880, L28. [Google Scholar] [CrossRef]
- Morinaga, T.; Nagakura, H.; Kato, C.; Yamada, S. Fast neutrino-flavor conversion in the preshock region of core-collapse supernovae. Phys. Rev. Res. 2020, 2, 012046. [Google Scholar] [CrossRef]
- Capozzi, F.; Abbar, S.; Bollig, R.; Janka, H.T. Fast neutrino flavor conversions in one-dimensional core-collapse supernova models with and without muon creation. Phys. Rev. D 2021, 103, 063013. [Google Scholar] [CrossRef]
- Abbar, S.; Capozzi, F.; Glas, R.; Janka, H.T.; Tamborra, I. On the characteristics of fast neutrino flavor instabilities in three-dimensional core-collapse supernova models. Phys. Rev. D 2021, 103, 063033. [Google Scholar] [CrossRef]
- Zaizen, M.; Morinaga, T. Nonlinear evolution of fast neutrino flavor conversion in the preshock region of core-collapse supernovae. arXiv 2021, arXiv:2104.10532. [Google Scholar] [CrossRef]
- Abbar, S.; Capozzi, F. Suppression of Scattering-Induced Fast Neutrino Flavor Conversions in Core-Collapse Supernovae. arXiv 2021, arXiv:2111.14880. [Google Scholar]
- Dasgupta, B.; Mirizzi, A.; Sen, M. Fast neutrino flavor conversions near the supernova core with realistic flavor-dependent angular distributions. J. Cosmol. Astropart. Phys. 2017, 02, 019. [Google Scholar] [CrossRef]
- Shalgar, S.; Padilla-Gay, I.; Tamborra, I. Neutrino propagation hinders fast pairwise flavor conversions. J. Cosmol. Astropart. Phys. 2020, 06, 048. [Google Scholar] [CrossRef]
- Abbar, S.; Volpe, M.C. On Fast Neutrino Flavor Conversion Modes in the Nonlinear Regime. Phys. Lett. B 2019, 790, 545. [Google Scholar] [CrossRef]
- Martin, J.D.; Yi, C.; Duan, H. Dynamic Fast Flavor Oscillation Waves Dense Neutrino Gases. Phys. Lett. B 2020, 800, 135088. [Google Scholar] [CrossRef]
- Johns, L.; Nagakura, H.; Fuller, G.M.; Burrows, A. Fast oscillations, collisionless relaxation, and spurious evolution of supernova neutrino flavor. Phys. Rev. D 2020, 102, 103017. [Google Scholar] [CrossRef]
- Bhattacharyya, S.; Dasgupta, B. Late-time behavior of fast neutrino oscillations. Phys. Rev. D 2020, 102, 063018. [Google Scholar] [CrossRef]
- Bhattacharyya, S.; Dasgupta, B. Fast Flavor Depolarization of Supernova Neutrinos. Phys. Rev. Lett. 2021, 126, 061302. [Google Scholar] [CrossRef] [PubMed]
- Wu, M.-R.; George, M.; Lin, C.-Y.; Xiong, Z. Collective fast neutrino flavor conversions in a 1D box: Initial conditions and long-term evolution. Phys. Rev. D 2021, 104, 103003. [Google Scholar] [CrossRef]
- Shalgar, S.; Tamborra, I. Three flavor revolution in fast pairwise neutrino conversion. Phys. Rev. D 2021, 104, 023011. [Google Scholar] [CrossRef]
- Capozzi, F.; Chakraborty, M.; Chakraborty, S.; Sen, M. Fast flavor conversions in supernovae: The rise of mu-tau neutrinos. Phys. Rev. Lett. 2020, 125, 251801. [Google Scholar] [CrossRef] [PubMed]
- Shalgar, S.; Tamborra, I. Dispelling a myth on dense neutrino media: Fast pairwise conversions depend on energy. J. Cosmol. Astropart. Phys. 2021, 01, 014. [Google Scholar] [CrossRef]
- Martin, J.D.; Carlson, J.; Cirigliano, V.; Duan, H. Fast flavor oscillations in dense neutrino media with collisions. Phys. Rev. D 2021, 103, 063001. [Google Scholar] [CrossRef]
- Capozzi, F.; Dasgupta, B.; Mirizzi, A.; Sen, M.; Sigl, G. Collisional triggering of fast flavor conversions of supernova neutrinos. Phys. Rev. Lett. 2019, 122, 091101. [Google Scholar] [CrossRef] [PubMed]
- Shalgar, S.; Tamborra, I. A change of direction in pairwise neutrino conversion physics: The effect of collisions. Phys. Rev. D 2021, 103, 063002. [Google Scholar] [CrossRef]
- Sasaki, H.; Takiwaki, T. Dynamics of fast neutrino flavor conversions with scattering effects: A detailed analysis. arXiv 2021, arXiv:2109.14011. [Google Scholar]
- Sigl, G. Simulations of Fast Neutrino Flavor Conversions with Interactions in Inhomogeneous Media. arXiv 2021, arXiv:2109.00091. [Google Scholar]
- Bhattacharyya, S.; Dasgupta, B. Fast flavor oscillations of astrophysical neutrinos with 1, 2, …, ∞ crossings. J. Cosmol. Astropart. Phys. 2021, 07, 023. [Google Scholar] [CrossRef]
- Dighe, A.; Sen, M. Nonstandard neutrino self-interactions in a supernova and fast flavor conversions. Phys. Rev. D 2018, 97, 043011. [Google Scholar] [CrossRef]
- Ruffert, M.; Janka, H.T.; Takahashi, K.; Schaefer, G. Coalescing neutron stars: A Step towards physical models. 2. Neutrino emission, neutron tori, and gamma-ray bursts. Astron. Astrophys. 1997, 319, 122. [Google Scholar]
- Foucart, F.; O’Connor, E.; Roberts, L.; Duez, M.D.; Haas, R.; Kidder, L.E.; Ott, C.D.; Pfeiffer, H.P.; Scheel, M.A.; Szilagyi, B. Post-merger evolution of a neutron star-black hole binary with neutrino transport. Phys. Rev. D 2015, 91, 124021. [Google Scholar] [CrossRef]
- Kulkarni, S.R. Modeling supernova-like explosions associated with gamma-ray bursts with short durations. arXiv 2005, arXiv:astro-ph/0510256. [Google Scholar]
- Metzger, B.D.; Martínez-Pinedo, G.; Darbha, S.; Quataert, E.; Arcones, A.; Kasen, D.; Thomas, R.; Nugent, P.; Panov, I.V.; Zinner, N.T. Electromagnetic counterparts of compact object mergers powered by the radioactive decay of r-process nuclei. Mon. Not. R. Astron. Soc. 2010, 406, 2650. [Google Scholar] [CrossRef]
- Perego, A.; Rosswog, S.; Cabezón, R.M.; Korobkin, O.; Käppeli, R.; Arcones, A.; Liebendörfer, M. Neutrino-driven winds from neutron star merger remnants. Mon. Not. Roy. Astron. Soc. 2014, 443, 3134. [Google Scholar] [CrossRef]
- Malkus, A.; Friedland, A.; McLaughlin, G.C. Matter-Neutrino Resonance Above Merging Compact Objects. arXiv 2014, arXiv:1403.5797. [Google Scholar]
- Malkus, A.; McLaughlin, G.C.; Surman, R. Symmetric and Standard Matter-Neutrino Resonances Above Merging Compact Objects. Phys. Rev. D 2016, 93, 045021. [Google Scholar] [CrossRef]
- Wu, M.-R.; Duan, H.; Qian, Y.-Z. Physics of neutrino flavor transformation through matter–neutrino resonances. Phys. Lett. B 2016, 752, 89. [Google Scholar] [CrossRef]
- Wu, M.-R.; Tamborra, I. Fast neutrino conversions: Ubiquitous in compact binary merger remnants. Phys. Rev. D 2017, 95, 103007. [Google Scholar] [CrossRef]
- George, M.; Wu, M.-R.; Tamborra, I.; Ardevol-Pulpillo, R.; Janka, H.-T. Fast neutrino flavor conversion, ejecta properties, and nucleosynthesis in newly-formed hypermassive remnants of neutron-star mergers. Phys. Rev. D 2020, 102, 103015. [Google Scholar] [CrossRef]
- Wu, M.-R.; Tamborra, I.; Just, O.; Janka, H.-T. Imprints of neutrino-pair flavor conversions on nucleosynthesis in ejecta from neutron-star merger remnants. Phys. Rev. D 2017, 96, 123015. [Google Scholar] [CrossRef]
- Li, X.; Siegel, D.M. Neutrino Fast Flavor Conversions in Neutron-Star Postmerger Accretion Disks. Phys. Rev. Lett. 2021, 126, 251101. [Google Scholar] [CrossRef] [PubMed]
- Padilla-Gay, I.; Shalgar, S.; Tamborra, I. Multi-Dimensional Solution of Fast Neutrino Conversions in Binary Neutron Star Merger Remnants. J. Cosmol. Astropart. Phys. 2021, 01, 017. [Google Scholar] [CrossRef]
- Myers, M.; Cooper, T.; Warren, M.; Kneller, J.; McLaughlin, G.; Richers, S.; Grohs, E.; Frohlich, C. Neutrino flavor mixing with moments. arXiv 2021, arXiv:2111.13722. [Google Scholar]
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Capozzi, F.; Saviano, N. Neutrino Flavor Conversions in High-Density Astrophysical and Cosmological Environments. Universe 2022, 8, 94. https://doi.org/10.3390/universe8020094
Capozzi F, Saviano N. Neutrino Flavor Conversions in High-Density Astrophysical and Cosmological Environments. Universe. 2022; 8(2):94. https://doi.org/10.3390/universe8020094
Chicago/Turabian StyleCapozzi, Francesco, and Ninetta Saviano. 2022. "Neutrino Flavor Conversions in High-Density Astrophysical and Cosmological Environments" Universe 8, no. 2: 94. https://doi.org/10.3390/universe8020094
APA StyleCapozzi, F., & Saviano, N. (2022). Neutrino Flavor Conversions in High-Density Astrophysical and Cosmological Environments. Universe, 8(2), 94. https://doi.org/10.3390/universe8020094






