Taxonomy of Dark Energy Models
Abstract
1. Introduction
2. Basic Equations for the Background Cosmology
3. Cosmological Samples
3.1. Type Ia Supernovae
3.2. Baryon Acoustic Oscillations
3.3. Cosmic Microwave Background Radiation
3.4. Observational Hubble Parameter
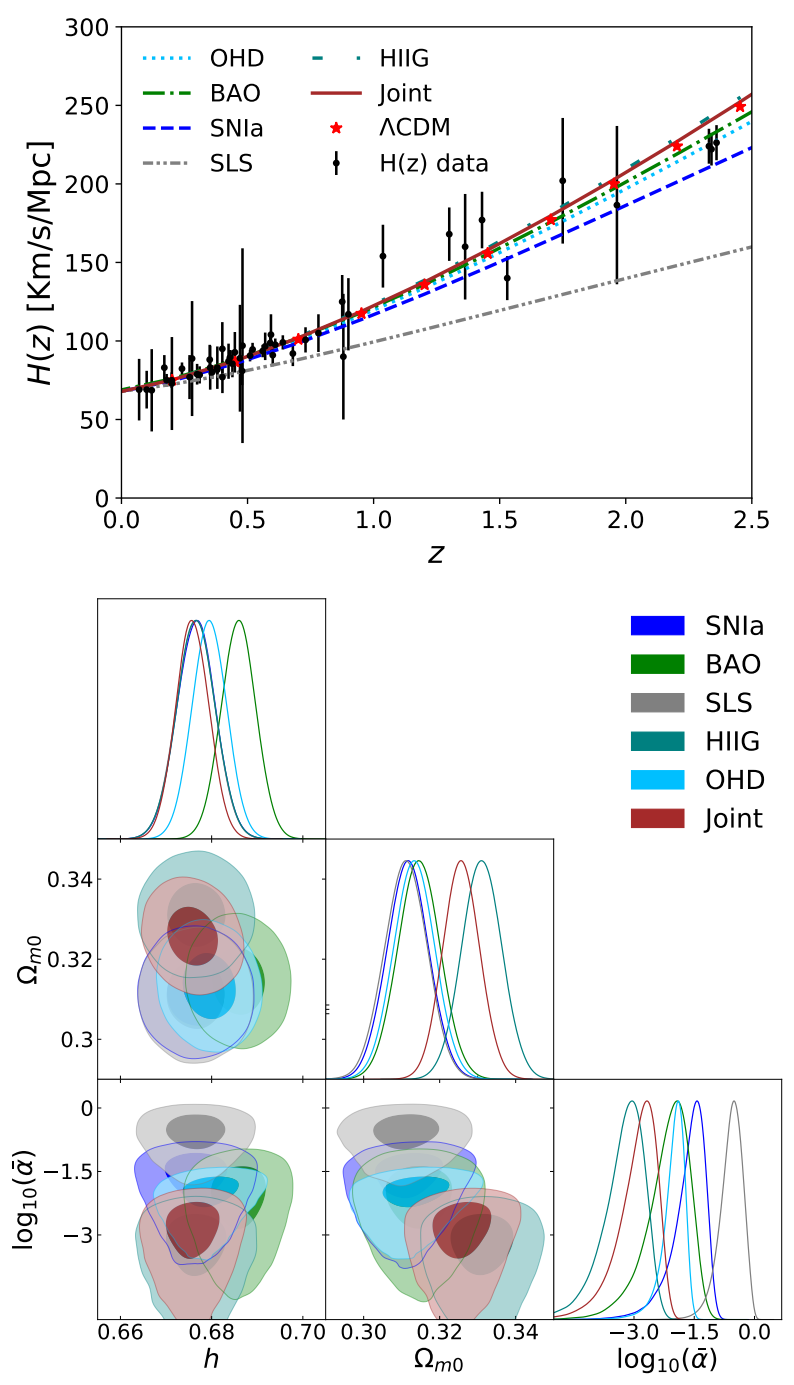
3.5. Strong Gravitational Lens Systems
3.6. Ionized Gas in Starburst Galaxies
3.7. Joint Analysis
4. Taxonomy of Dark Energy Models
4.1. Accelerating Universe Fluids
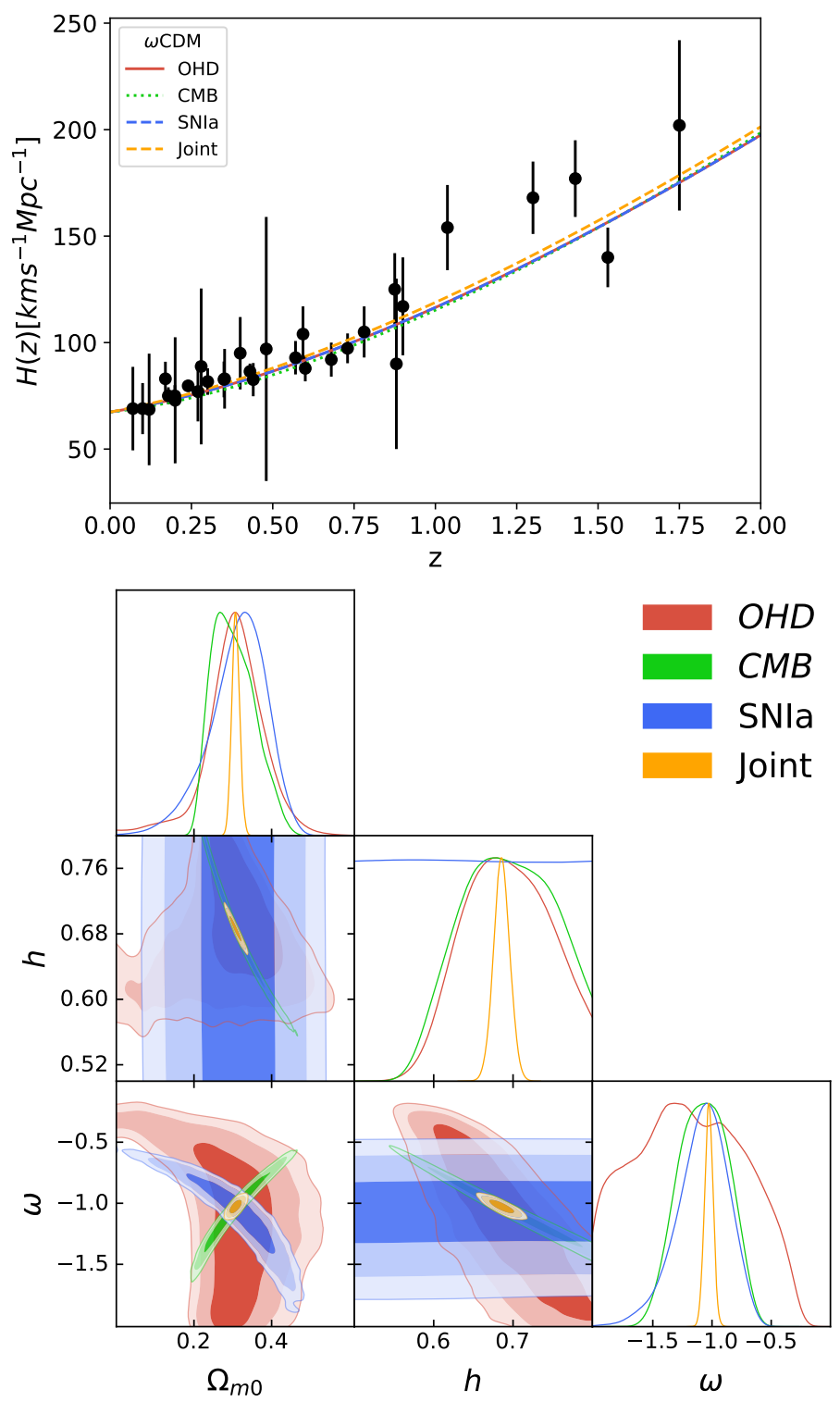
4.1.1. The CDM Model
4.1.2. The CDM Model
4.1.3. Dark Energy Parameterizations
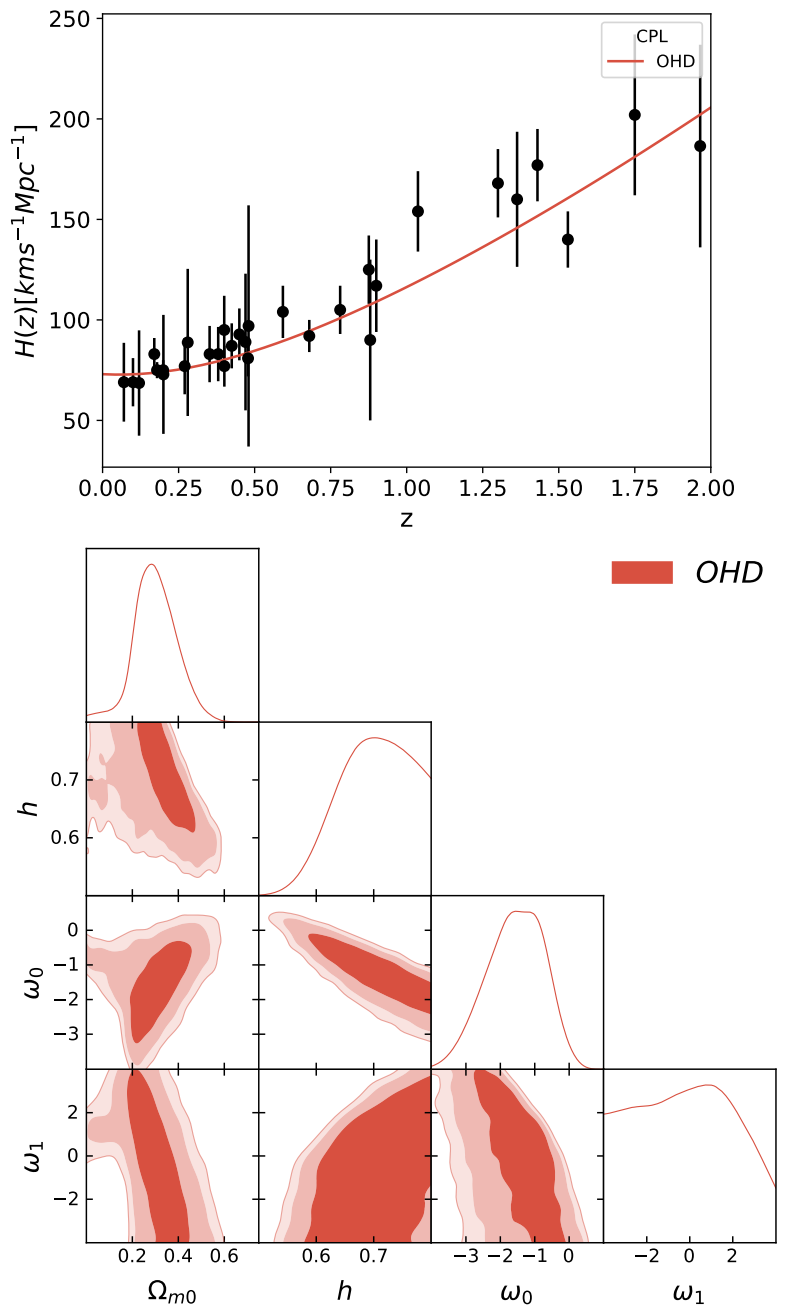
- The Chevallier–Polarski–Linder parametrization (CPL) [99,100]. An approach to study dynamical DE models is through a parametrization of its EoS. The dimensionless Hubble parameter for this Universe is given byWe compute in the same form as is given by Equation (44).The density parameter for DE is written as , and the function depends on aswhere is the energy density of DE at redshift z, and is its present value. One of the most popular parameterizations is proposed by [99,101], and reads aswhere is the EoS at redshift and . Although this function is widely used, it has a divergence problem when . The function for the CPL parametrization is
- The Jassal-Bagla-Padmanabhan (JBP) parametrization. Jassal et al. [102] proposed the following ansatz to parametrize the dark energy EoS,where is the EoS at redshift and . The function is
- The Barbosa–Alcaniz (BA) parametrization. Barboza and Alcaniz [103] considered a EoS given by:This ansatz behaves linearly at low redshifts as , and when . In addition, is well-behaved for all epochs of the Universe. For instance, the DE dynamics in the future, at , can be investigated without dealing with a divergence. Solving the integral in Equation (47) and using Equation (52) results in:
- Feng–Shen–Li–Li (FSLL, [104]) parametrizations.- The authors suggested two dark energy EoS given by:Both functions have the advantage of being divergence-free throughout the entire cosmic evolution, even at . At low redshifts, behaves as and for FSLLI and FSLLII, respectively. In addition, when , the EoS has the same value () as the present epoch for FSLLI and for FSLLII. Using Equations (54) and (55) to solve Equation (47) leads to:where and correspond to FSLLI and FSLLII, respectively.
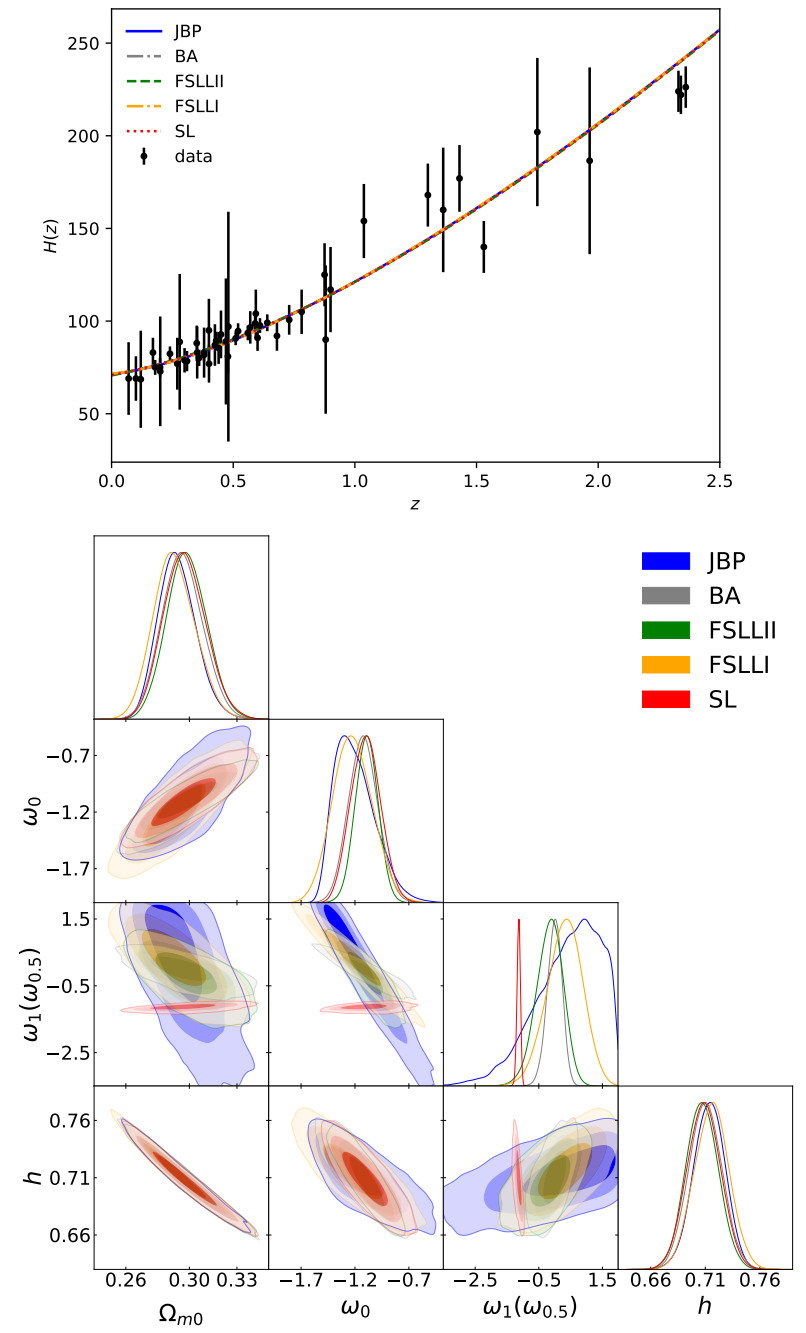
- Sendra–Lazkoz (SL, [105]) introduced new polynomial parameterizations to reduce the parameter correlation, so they can be better constrained by the observations at low redshifts. One of these parameterizations is given by:where the constants are defined as , and , and is the value of the EoS at . This function is well-behaved at higher redshifts as . The substitution of Equation (57) into Equation (47) results in the following:Notice that, although DE parameterizations are common and they could solve the coincidence problem, there is not a unique way to choose the form of the function. Furthermore, in many cases, there are not strong arguments to justify the functional form by an association with a first-principles theory of quantum fields or gravity. A different approach, which is model-independent, consists of, for example investigating the cosmographic parameters that characterize the kinematics of the cosmic expansion (e.g., [106,107,108,109,110]). Some authors have used the Hubble parameter, the deceleration parameter (), or even higher-order derivatives of the scale factor a, such as Jerk and Snap (e.g., [111,112]). By estimating these cosmographic parameters using cosmological data, it is possible to associate its features to a given DE model (see [111,113,114,115,116,117]).The cosmological constraints for the aforementioned models are obtained assuming flat priors on the DE parameters and a Gaussian prior on h. Table 2 provides the mean values for the , , and () parameters of the JBP, BA, FSLLI, FSLLII, and SL DE parameterizations using the joint of the OHD sample (34 data points from DA and BAO measurements) in the redshift range [118], distance posteriors from Planck [119], and different BAO measurements (see details in [120]). Figure 3 shows the reconstruction of for these parametrizations using the parameter mean values (top panel) and the and confidence contour of the cosmological constraints (bottom panel). Notice that the DE parameterizations are consistent for and .
4.1.4. Chaplygin-Like Fluid
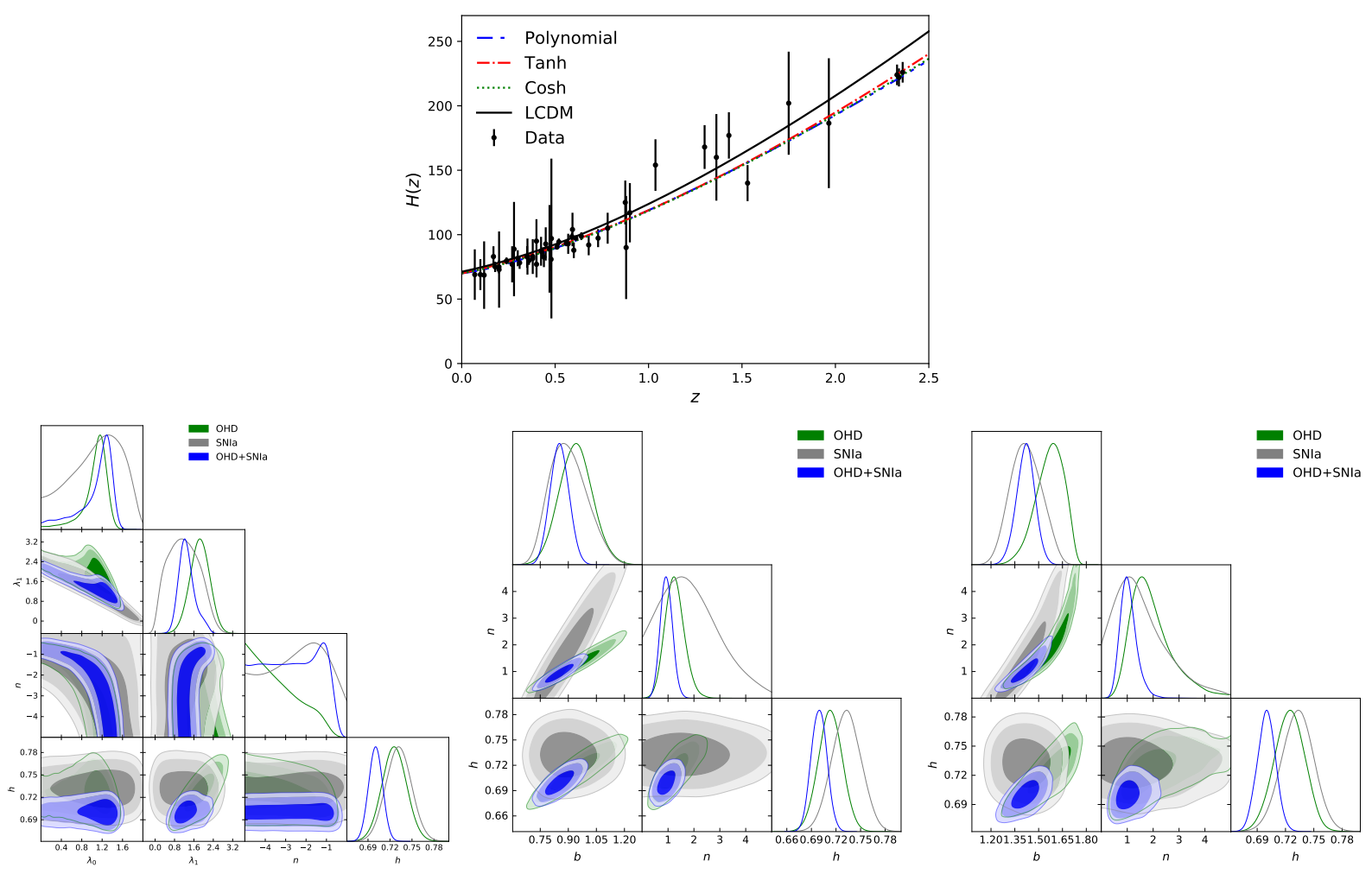
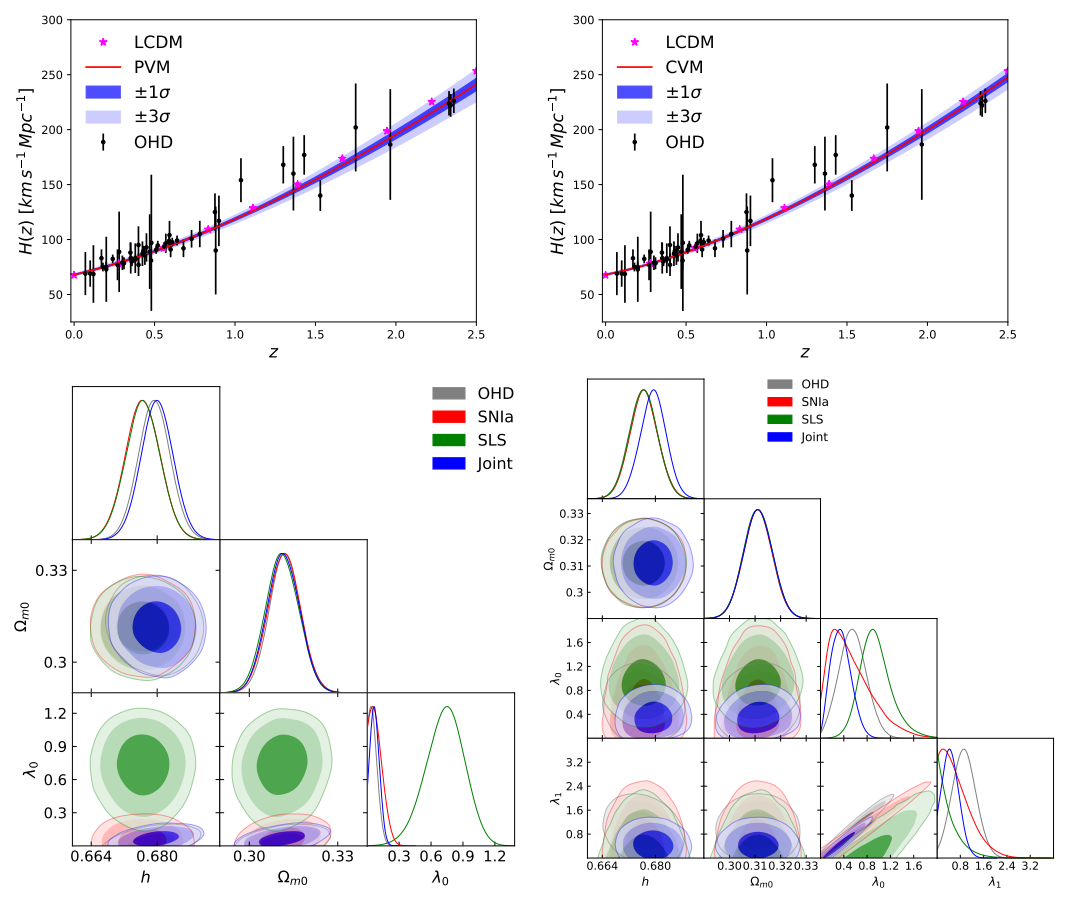
4.1.5. Viscous Model
- . This model, where is the energy density of dust matter and are constants, is probably the simplest one that successfully reproduces the late accelerated stage of the Universe. Some studies that consider a single fluid in the Universe are presented in [132] (see, for example [133], for a case in a causal theory). Additionally, there are other works that include several components such as radiation and DE [65].
- . In spite of the success of the previous model at late epochs of the Universe, it has problems in early epochs because diverges. This motivates the use of alternative viscosity models such as those proposed by [134], in particular polynomial forms of the redshift.
- and . Alternatively, more complex models are investigated in [134] by proposing the viscosity as a hyperbolic function of the dimensionless Hubble parameter E.
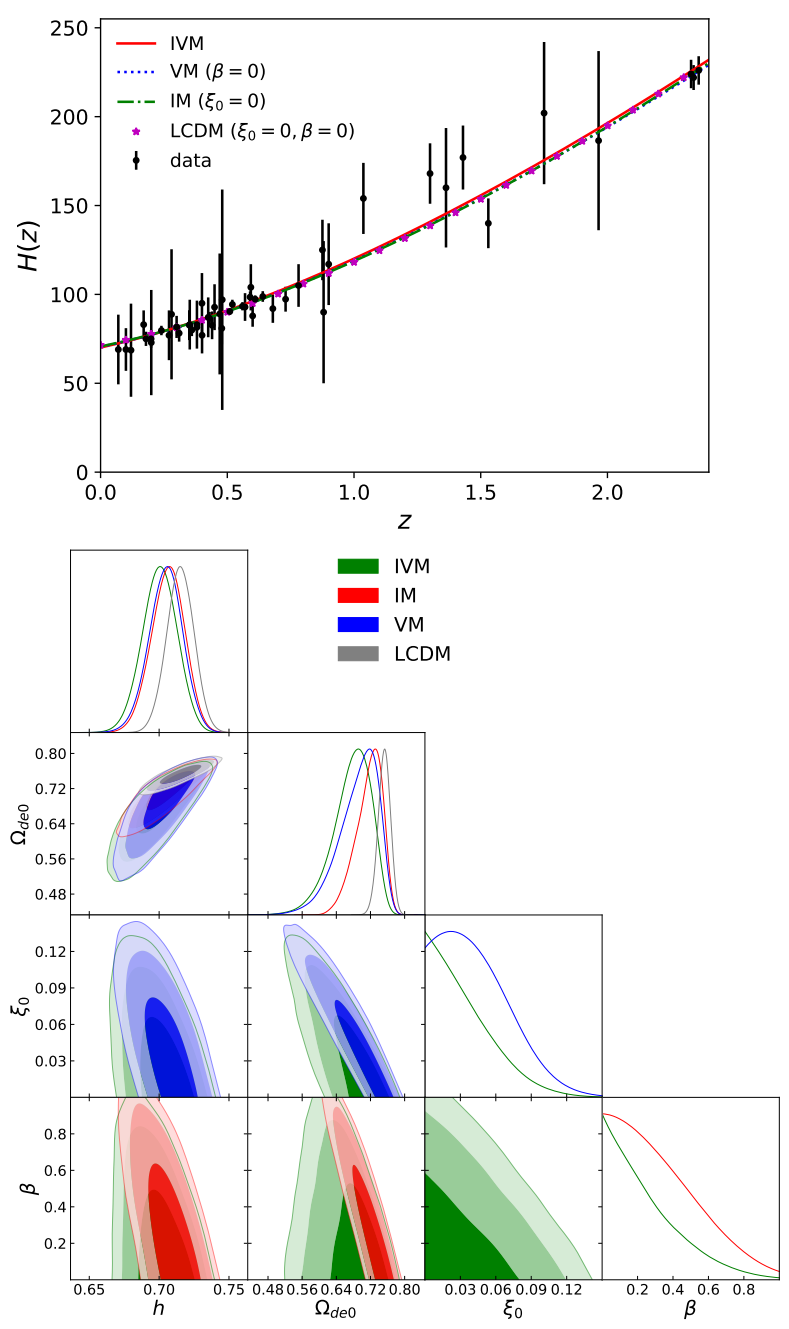
4.1.6. Interacting Viscous Models
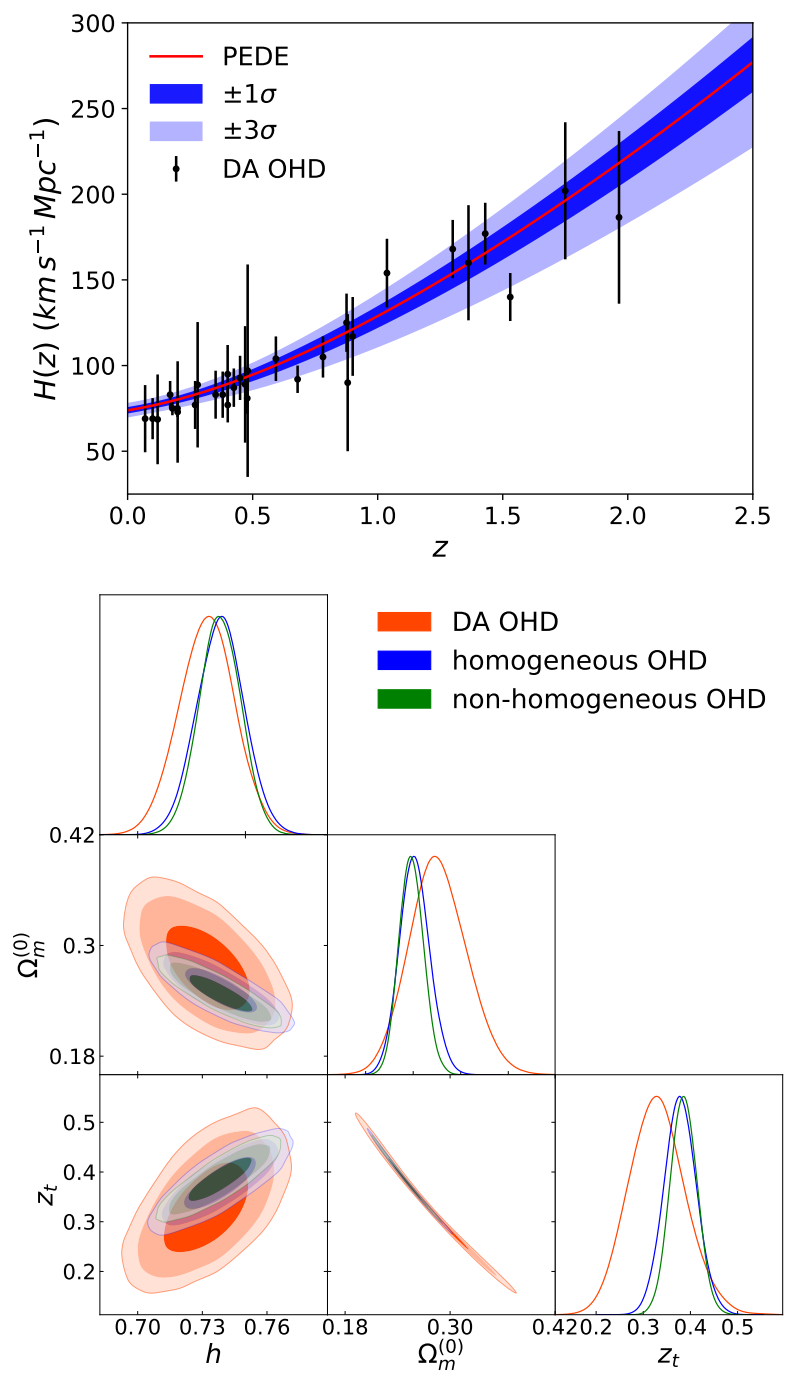
4.1.7. Phenomenological Emergent Dark Energy Model
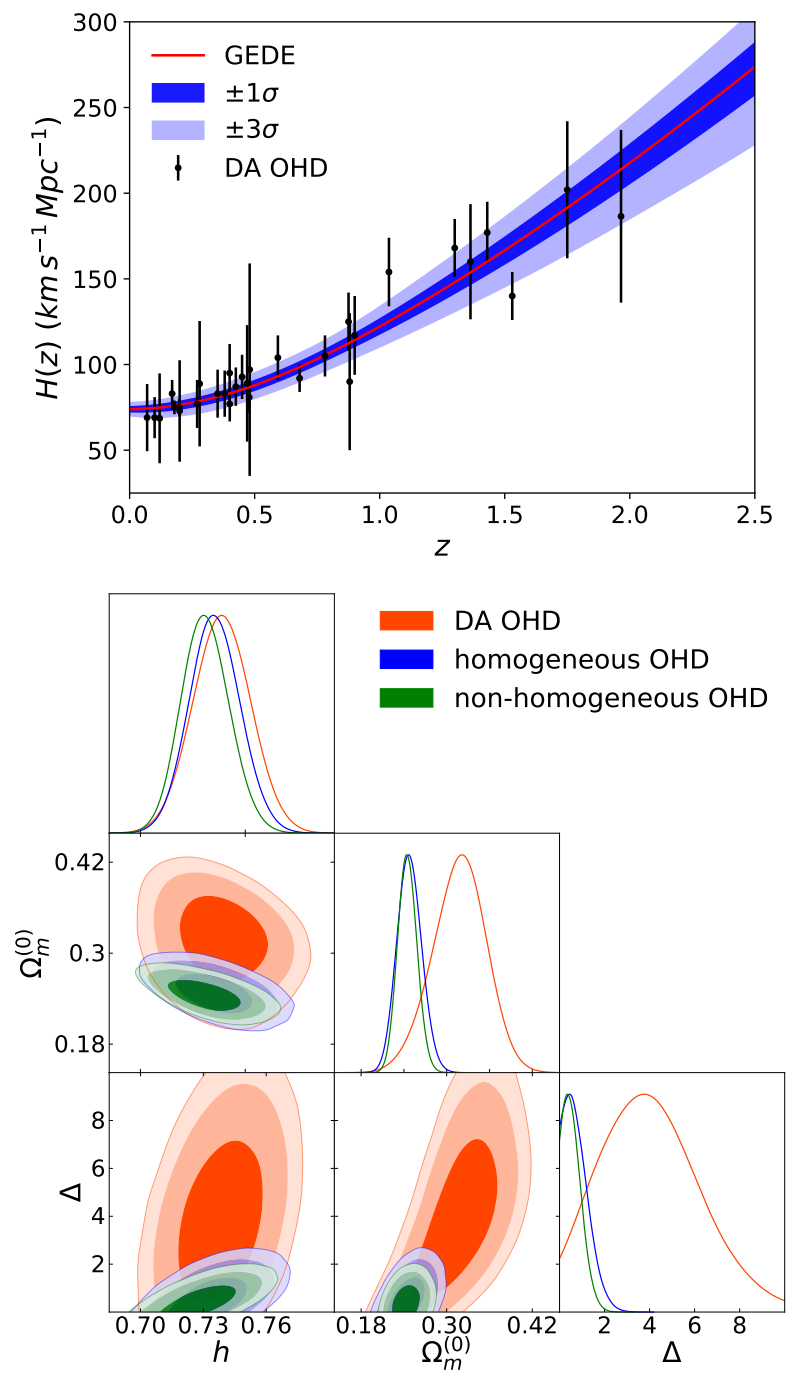
4.1.8. Generalized Emergent Dark Energy
4.2. Modifications to General Theory of Relativity
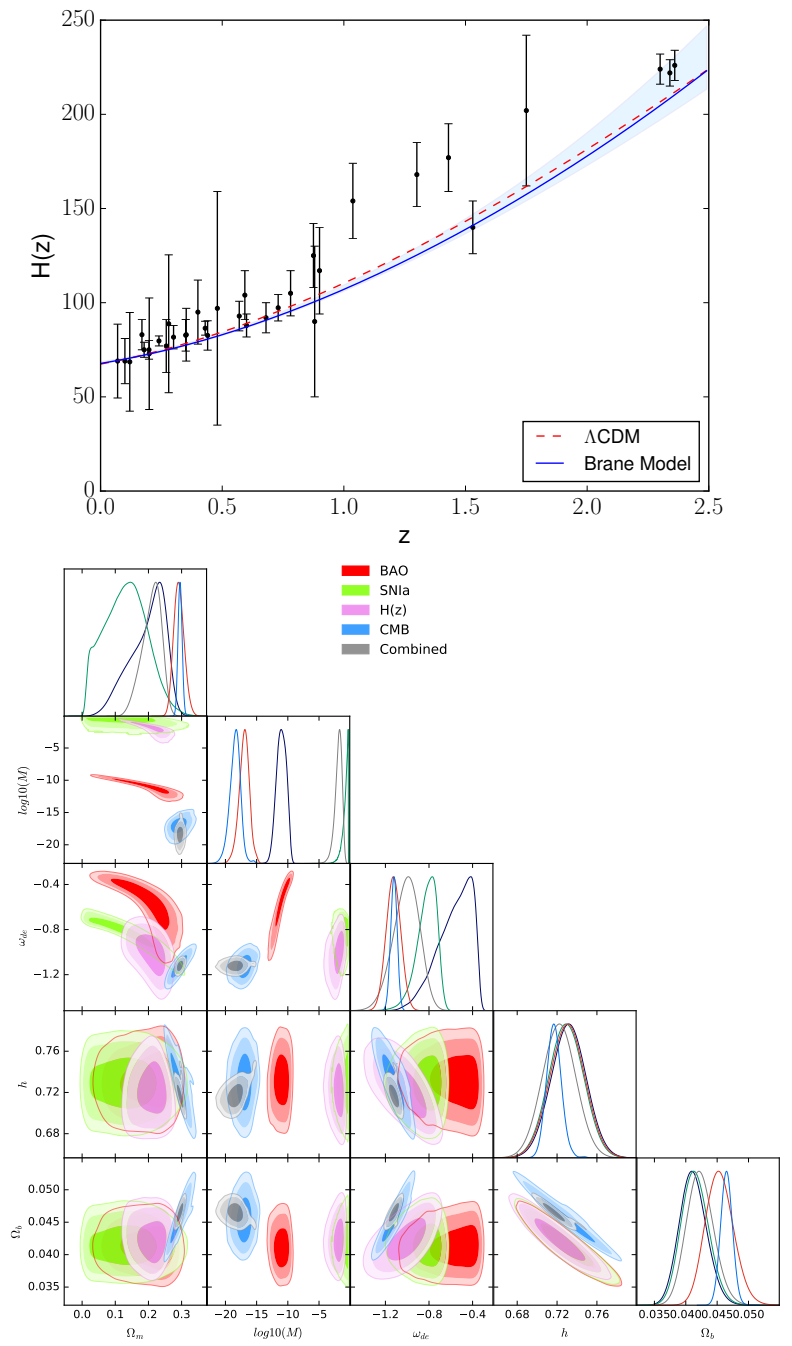
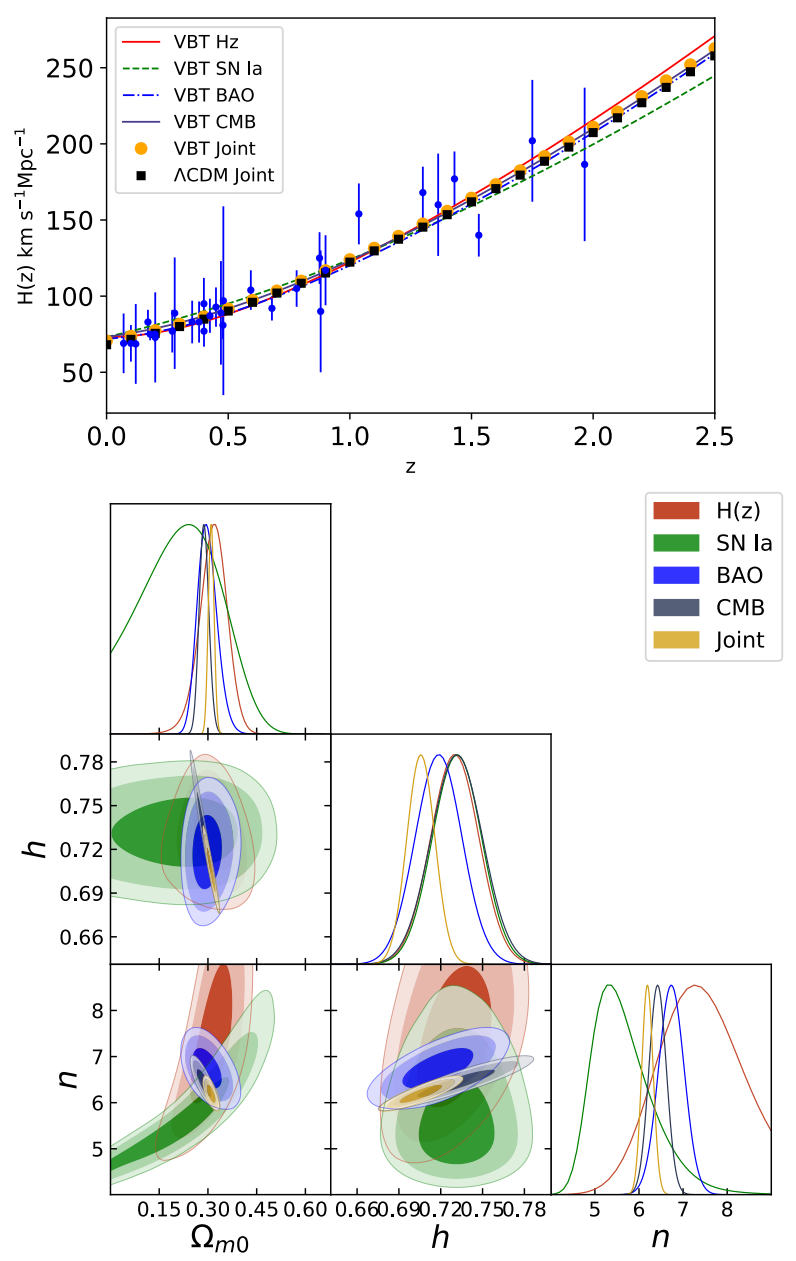
4.2.1. Constant Brane Tension
4.2.2. Variable Brane Tension
4.2.3. Unimodular Gravity
4.2.4. Einstein–Gauss–Bonet
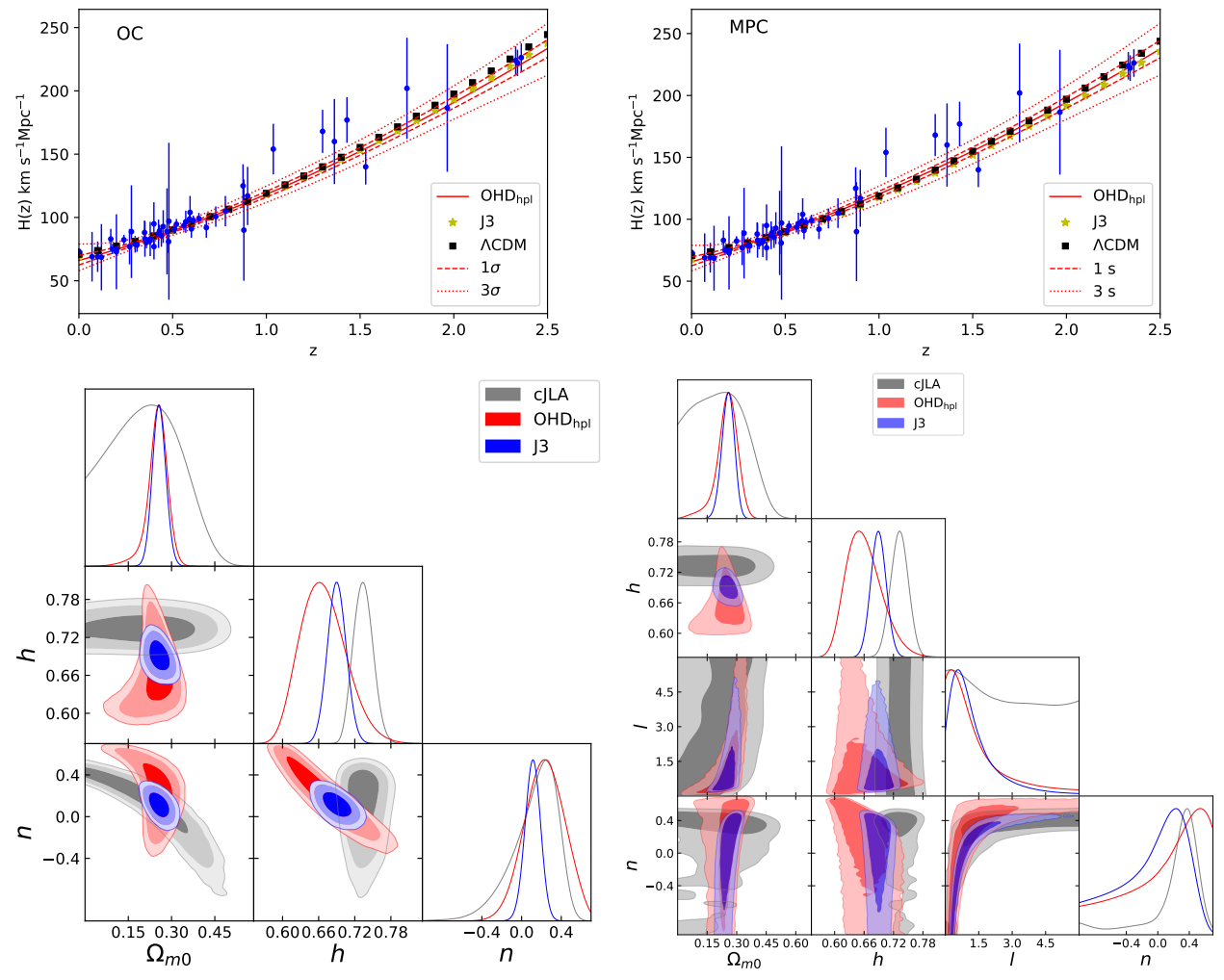
4.2.5. Cardassian Models
5. Discussion and Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Lemaître, G. Un Univers homogène de masse constante et de rayon croissant rendant compte de la vitesse radiale des nébuleuses extra-galactiques. Ann. Société Sci. Brux. 1927, 47, 49–59. [Google Scholar]
- Hubble, E. A Relation between Distance and Radial Velocity among Extra-Galactic Nebulae. Proc. Natl. Acad. Sci. USA 1929, 15, 168–173. [Google Scholar] [CrossRef] [PubMed]
- Penzias, A.A.; Wilson, R.W. A Measurement of Excess Antenna Temperature at 4080 Mc/s. Astrophys. J. 1965, 142, 419–421. [Google Scholar] [CrossRef]
- Dicke, R.H.; Peebles, P.J.E.; Roll, P.G.; Wilkinson, D.T. Cosmic Black-Body Radiation. Astrophys. J. 1965, 142, 414–419. [Google Scholar] [CrossRef]
- Riess, A.G.; Filippenko, A.V.; Challis, P.; Clocchiatti, A.; Diercks, A.; Garnavich, P.M.; Tonry, J. Observational Evidence from Supernovae for an Accelerating Universe and a Cosmological Constant. Astron. J. 1998, 116, 1009. [Google Scholar] [CrossRef]
- Perlmutter, S.; Aldering, G.; Goldhaber, G.; Knop, R.A.; Nugent, P.; Project, T.S.C. Measurements of Ω and Λ from 42 High-Redshift Supernovae. Astrophys. J. 1999, 517, 565. [Google Scholar] [CrossRef]
- De Bernardis, P.; Ade, P.A.R.; Bock, J.J.; Bond, J.R.; Borrill, J.; Boscaleri, A.; Coble, K.; Crill, B.P.; De Gasperis, G.; Farese, P.C.; et al. A flat Universe from high-resolution maps of the cosmic microwave background radiation. Nature 2000, 404, 955–959. [Google Scholar] [CrossRef]
- Spergel, D.N.; Verde, L.; Peiris, H.V.; Komatsu, E.; Nolta, M.R.; Bennett, C.L.; Halpern, M.; Hinshaw, G.; Jarosik, N.; Kogut, A.; et al. First-Year Wilkinson Microwave Anisotropy Probe (WMAP) Observations: Determination of Cosmological Parameters. Astrophys. J. Suppl. Ser. 2003, 148, 175–194. [Google Scholar] [CrossRef]
- Eisenstein, D. Dark energy and cosmic sound. Wide-Field Imaging from Space. New Astron. Rev. 2005, 49, 360–365. [Google Scholar] [CrossRef]
- Percival, W.J.; Cole, S.; Eisenstein, D.J.; Nichol, R.C.; Peacock, J.A.; Pope, A.C.; Szalay, A.S. Measuring the Baryon Acoustic Oscillation scale using the Sloan Digital Sky Survey and 2dF Galaxy Redshift Survey. Mon. Not. R. Astron. Soc. 2007, 381, 1053–1066. [Google Scholar] [CrossRef]
- Suyu, S.H.; Marshall, P.J.; Auger, M.W.; Hilbert, S.; Blandford, R.D.; Koopmans, L.V.E.; Fassnacht, C.D.; Treu, T. Dissecting the Gravitational lens B1608+656. II. Precision Measurements of the Hubble Constant, Spatial Curvature, and the Dark Energy Equation of State. Astrophys. J. 2010, 711, 201–221. [Google Scholar] [CrossRef]
- Schrabback, T.; Hartlap, J.; Joachimi, B.; Kilbinger, M.; Simon, P.; Benabed, K.; Bradač, M.; Eifler, T.; Erben, T.; Fassnacht, C.D.; et al. Evidence of the accelerated expansion of the Universe from weak lensing tomography with COSMOS. Astron. Astrophys. 2010, 516, A63. [Google Scholar] [CrossRef]
- Huterer, D.; Shafer, D.L. Dark energy two decades after: Observables, probes, consistency tests. Rep. Prog. Phys. 2018, 81, 016901. [Google Scholar] [CrossRef]
- Bennett, C.L.; Larson, D.; Weiland, J.L.; Jarosik, N.; Hinshaw, G.; Odegard, N.; Smith, K.M.; Hill, R.S.; Gold, B.; Halpern, M.; et al. Nine-year Wilkinson Microwave Anisotropy Probe (WMAP) Observations: Final Maps and Results. Astrophys. J. Suppl. Ser. 2013, 208, 20. [Google Scholar] [CrossRef]
- Planck Collaboration; Aghanim, N.; Akrami, Y.; Ashdown, M.; Aumont, J.; Baccigalupi, C.; Ballardini, M.; Banday, A.J.; Barreiro, R.B.; Bartolo, N.; et al. Planck 2018 results. VI. Cosmological parameters. Astron. Astrophys. 2020, 641, A6. [Google Scholar] [CrossRef]
- Feng, J.L. Dark Matter Candidates from Particle Physics and Methods of Detection. Ann. Rev. Astron. Astrophys. 2010, 48, 495–545. [Google Scholar] [CrossRef]
- Bertone, G.; Hooper, D. History of dark matter. Rev. Mod. Phys. 2018, 90, 045002. [Google Scholar] [CrossRef]
- De Martino, I.; Chakrabarty, S.S.; Cesare, V.; Gallo, A.; Ostorero, L.; Diaferio, A. Dark Matters on the Scale of Galaxies. Universe 2020, 6, 107. [Google Scholar] [CrossRef]
- Martin, S.P. A Supersymmetry primer. Adv. Ser. Direct. High Energy Phys. 1998, 18, 1–98. [Google Scholar] [CrossRef]
- Abazov, V.M. Search for squarks and gluinos in events with jets and missing transverse energy using 2.1 fb-1 of pp¯ collision data at = 1.96-TeV. Phys. Lett. B 2008, 660, 449–457. [Google Scholar] [CrossRef]
- Wilczek, F. Problem of Strong P and T Invariance in the Presence of Instantons. Phys. Rev. Lett. 1978, 40, 279–282. [Google Scholar] [CrossRef]
- Lee, J.W.; Koh, I.G. Galactic halos as boson stars. Phys. Rev. D 1996, 53, 2236–2239. [Google Scholar] [CrossRef] [PubMed]
- Ureña López, L.A.; Matos, T. New cosmological tracker solution for quintessence. Phys. Rev. D 2000, 62, 081302. [Google Scholar] [CrossRef]
- Zeldovich, Y.B. The cosmological constant and the theory of elementary particles. Sov. Phys. Uspekhi 1968, 11, 381. [Google Scholar] [CrossRef]
- Weinberg, S. The cosmological constant problem. Rev. Mod. Phys. 1989, 61, 1. [Google Scholar] [CrossRef]
- Carroll, S.M. The Cosmological constant. Living Rev. Rel. 2001, 4, 1. [Google Scholar] [CrossRef]
- Millon, M.; Galan, A.; Courbin, F.; Treu, T.; Suyu, S.H.; Ding, X.; Birrer, S.; Chen, G.C.F.; Shajib, A.J.; Sluse, D.; et al. TDCOSMO. I. An exploration of systematic uncertainties in the inference of H0 from time-delay cosmography. Astron. Astrophys. 2020, 639, A101. [Google Scholar] [CrossRef]
- Birrer, S.; Shajib, A.J.; Galan, A.; Millon, M.; Treu, T.; Agnello, A.; Auger, M.; Chen, G.C.F.; Christensen, L.; Collett, T.; et al. TDCOSMO. IV. Hierarchical time-delay cosmography – joint inference of the Hubble constant and galaxy density profiles. Astron. Astrophys. 2020, 643, A165. [Google Scholar] [CrossRef]
- Joudaki, S.; Blake, C.; Heymans, C.; Choi, A.; Harnois-Deraps, J.; Hildebrandt, H.; van Waerbeke, L. CFHTLenS revisited: Assessing concordance with Planck including astrophysical systematics. Mon. Not. R. Astron. Soc. 2017, 465, 2033–2052. [Google Scholar] [CrossRef]
- Hildebrandt, H.; Viola, M.; Heymans, C.; Joudaki, S.; Kuijken, K.; Blake, C.; Van Waerbeke, L. KiDS-450: Cosmological parameter constraints from tomographic weak gravitational lensing. Mon. Not. R. Astron. Soc. 2017, 465, 1454. [Google Scholar] [CrossRef]
- Riess, A.G.; Casertano, S.; Yuan, W.; Macri, L.; Anderson, J.; MacKenty, J.W.; Bowers, J.B.; Clubb, K.I.; Filippenko, A.V.; Jones, D.O.; et al. New Parallaxes of Galactic Cepheids from Spatially Scanning theHubble Space Telescope: Implications for the Hubble Constant. Astrophys. J. 2018, 855, 136. [Google Scholar] [CrossRef]
- Riess, A.G.; Casertano, S.; Yuan, W.; Macri, L.M.; Scolnic, D. Large Magellanic Cloud Cepheid Standards Provide a 1% Foundation for the Determination of the Hubble Constant and Stronger Evidence for Physics beyond ΛCDM. Astrophys. J. 2019, 876, 85. [Google Scholar] [CrossRef]
- Di Valentino, E.; Mena, O.; Pan, S.; Visinelli, L.; Yang, W.; Melchiorri, A.; Mota, D.F.; Riess, A.G.; Silk, J. In the Realm of the Hubble tension—A Review of Solutions. arXiv 2021, arXiv:2103.01183. [Google Scholar]
- Luković, V.V.; D’Agostino, R.; Vittorio, N. Is there a concordance value for H0? Astron. Astrophys. 2016, 595, A109. [Google Scholar] [CrossRef]
- Brax, P. What makes the Universe accelerate? A review on what dark energy could be and how to test it. Rep. Prog. Phys. 2017, 81, 016902. [Google Scholar] [CrossRef]
- Kabáth, P.; Jones, D.; Skarka, M. Reviews in Frontiers of Modern Astrophysics; From Space Debris to Cosmology; Springer International Publishing: New York, NY, USA, 2020. [Google Scholar] [CrossRef]
- Li, X.; Shafieloo, A. A Simple Phenomenological Emergent Dark Energy Model can Resolve the Hubble Tension. ApJL 2019, 883, L3. [Google Scholar] [CrossRef]
- Li, X.; Shafieloo, A. Generalised Emergent Dark Energy Model: Confronting Λ and PEDE. arXiv 2020, arXiv:2001.05103. [Google Scholar]
- Hernández-Almada, A.; Leon, G.; Magaña, J.; García-Aspeitia, M.A.; Motta, V. Generalized Emergent Dark Energy: Observational Hubble data constraints and stability analysis. Mon. Not. R. Astron. Soc. 2020, 497, 1590–1602. [Google Scholar] [CrossRef]
- Maartens, R. Cosmological dynamics on the brane. Phys. Rev. D 2000, 62, 084023. [Google Scholar] [CrossRef]
- García-Aspeitia, M.A.; Magaña, J.; Hernández-Almada, A.; Motta, V. Probing dark energy with braneworld cosmology in the light of recent cosmological data. Int. J. Mod. Phys. D 2017, 27, 1850006. [Google Scholar] [CrossRef]
- Garcia-Aspeitia, M.A.; Hernandez-Almada, A.; Magaña, J.; Amante, M.H.; Motta, V.; Martínez-Robles, C. Brane with variable tension as a possible solution to the problem of the late cosmic acceleration. Phys. Rev. D 2018, 97, 101301. [Google Scholar] [CrossRef]
- Wainwright, J.; Ellis, G.F.R. Dynamical Systems in Cosmology; Cambridge University Press: Cambridge, UK, 1997. [Google Scholar]
- Gao, C.; Brandenberger, R.H.; Cai, Y.; Chen, P. Cosmological Perturbations in Unimodular Gravity. JCAP 2014, 1409, 021. [Google Scholar] [CrossRef]
- García-Aspeitia, M.A.; Martínez-Robles, C.; Hernández-Almada, A.; Magaña, J.; Motta, V. Cosmic acceleration in unimodular gravity. Phys. Rev. 2019, D99, 123525. [Google Scholar] [CrossRef]
- Glavan, D.Z.; Lin, C. Einstein–Gauss–Bonnet Gravity in Four-Dimensional Spacetime. Phys. Rev. Lett. 2020, 124, 081301. [Google Scholar] [CrossRef]
- García-Aspeitia, M.A.; Hernández-Almada, A. Einstein–Gauss–Bonnet gravity: Is it compatible with modern cosmology? Phys. Dark Universe 2021, 32, 100799. [Google Scholar] [CrossRef]
- Freese, K.; Lewis, M. Cardassian expansion: A model in which the universe is flat, matter dominated, and accelerating. Phys. Lett. B 2002, 540, 1–8. [Google Scholar] [CrossRef]
- Gondolo, P.; Freese, K. Fluid interpretation of cardassian expansion. Phys. Rev. D 2003, 68, 063509. [Google Scholar] [CrossRef]
- Jaime, L.G.; Jaber, M.; Escamilla-Rivera, C. New parametrized equation of state for dark energy surveys. Phys. Rev. D 2018, 98, 083530. [Google Scholar] [CrossRef]
- Iorio, L. Editorial for the Special Issue 100 Years of Chronogeometrodynamics: The Status of the Einstein’s Theory of Gravitation in Its Centennial Year. Universe 2015, 1, 38–81. [Google Scholar] [CrossRef]
- Debono, I.; Smoot, G.F. General Relativity and Cosmology: Unsolved Questions and Future Directions. Universe 2016, 2, 23. [Google Scholar] [CrossRef]
- Vishwakarma, R.G. Einstein and Beyond: A Critical Perspective on General Relativity. Universe 2016, 2, 11. [Google Scholar] [CrossRef]
- Cao, S.; Pan, Y.; Biesiada, M.; Godlowski, W.; Zhu, Z.H. Constraints on cosmological models from strong gravitational lensing systems. JCAP 2012, 2012, 016. [Google Scholar] [CrossRef]
- Cao, S.; Biesiada, M.; Gavazzi, R.; Piórkowska, A.; Zhu, Z.H. Cosmology With Strong-lensing Systems. Astrophys. J. 2015, 806, 185. [Google Scholar] [CrossRef]
- Amante, M.H.; Magaña, J.; Motta, V.; García-Aspeitia, M.A.; Verdugo, T. Testing dark energy models with a new sample ofstrong-lensing systems. Mon. Not. R. Astron. Soc. 2019, 498, 6013–6033. [Google Scholar] [CrossRef]
- Chávez, R.; Terlevich, E.; Terlevich, R.; Plionis, M.; Bresolin, F.; Basilakos, S.; Melnick, J. Determining the Hubble constant using giant extragalactic H II regions and H II galaxies. Mon. Not. R. Astron. Soc. 2012, 425, L56–L60. [Google Scholar] [CrossRef]
- Chávez, R.; Terlevich, R.; Terlevich, E.; Bresolin, F.; Melnick, J.; Plionis, M.; Basilakos, S. The L-σ relation for massive bursts of star formation. Mon. Not. R. Astron. Soc. 2014, 442, 3565–3597. [Google Scholar] [CrossRef]
- Terlevich, R.; Terlevich, E.; Melnick, J.; Chávez, R.; Plionis, M.; Bresolin, F.; Basilakos, S. On the road to precision cosmology with high-redshift H II galaxies. Mon. Not. R. Astron. Soc. 2015, 451, 3001–3010. [Google Scholar] [CrossRef]
- Chávez, R.; Plionis, M.; Basilakos, S.; Terlevich, R.; Terlevich, E.; Melnick, J.; Bresolin, F.; González-Morán, A.L. Constraining the dark energy equation of state with H II galaxies. Mon. Not. R. Astron. Soc. 2016, 462, 2431–2439. [Google Scholar] [CrossRef]
- González-Morán, A.L.; Chávez, R.; Terlevich, R.; Terlevich, E.; Bresolin, F.; Fernández-Arenas, D.; Plionis, M.; Basilakos, S.; Melnick, J.; Telles, E. Independent cosmological constraints from high-z H II galaxies. Mon. Not. R. Astron. Soc. 2019, 487, 4669–4694. [Google Scholar] [CrossRef]
- Cao, S.; Ryan, J.; Ratra, B. Cosmological constraints from HII starburst galaxy apparent magnitude and other cosmological measurements. Mon. Not. R. Astron. Soc. 2020, 497, 3191–3203. [Google Scholar] [CrossRef]
- Jimenez, R.; Loeb, A. Constraining cosmological parameters based on relative galaxy ages. Astrophys. J. 2002, 573, 37–42. [Google Scholar] [CrossRef]
- Scolnic, D.M.; Jones, D.O.; Rest, A.; Pan, Y.C.; Chornock, R.; Foley, R.J.; Smith, K.W. The Complete Light-curve Sample of Spectroscopically Confirmed SNe Ia from Pan-STARRS1 and Cosmological Constraints from the Combined Pantheon Sample. Astrophys. J. 2018, 859, 101. [Google Scholar] [CrossRef]
- Hernández-Almada, A.; García-Aspeitia, M.A.; Magaña, J.; Motta, V. Stability analysis and constraints on interacting viscous cosmology. Phys. Rev. D 2020, 101, 063516. [Google Scholar] [CrossRef]
- Magaña, J.; Amante, M.H.; García-Aspeitia, M.A.; Motta, V. The Cardassian expansion revisited: Constraints from updated Hubble parameter measurements and type Ia supernova data. Mon. Not. R. Astron. Soc. 2018, 476, 1036–1049. [Google Scholar] [CrossRef]
- Eisenstein, D.J.; Hu, W. Baryonic features in the matter transfer function. Astrophys. J. 1998, 496, 605. [Google Scholar] [CrossRef]
- Nunes, R.C.; Yadav, S.K.; Jesus, J.F.; Bernui, A. Cosmological parameter analyses using transversal BAO data. Mon. Not. R. Astron. Soc. 2020, 497, 2133–2141. [Google Scholar] [CrossRef]
- Carvalho, G.C.; Bernui, A.; Benetti, M.; Carvalho, J.C.; Alcaniz, J.S. Baryon acoustic oscillations from the SDSS DR10 galaxies angular correlation function. Phys. Rev. D 2016, 93, 023530. [Google Scholar] [CrossRef]
- Alcaniz, J.S.; Carvalho, G.C.; Bernui, A.; Carvalho, J.C.; Benetti, M. Measuring Baryon Acoustic Oscillations with Angular Two-Point Correlation Function. In Gravity and the Quantum: Pedagogical Essays on Cosmology, Astrophysics, and Quantum Gravity; Bagla, J.S., Engineer, S., Eds.; Springer International Publishing: Cham, Switzerland, 2017; pp. 11–19. [Google Scholar] [CrossRef]
- Carvalho, G.; Bernui, A.; Benetti, M.; Carvalho, J.; de Carvalho, E.; Alcaniz, J. The transverse baryonic acoustic scale from the SDSS DR11 galaxies. Astropart. Phys. 2020, 119, 102432. [Google Scholar] [CrossRef]
- De Carvalho, E.; Bernui, A.; Carvalho, G.; Novaes, C.; Xavier, H. Angular Baryon Acoustic Oscillation measure atz=2.225 from the SDSS quasar survey. J. Cosmol. Astropart. Phys. 2018, 2018, 064. [Google Scholar] [CrossRef]
- De Carvalho, E.; Bernui, A.; Xavier, H.S.; Novaes, C.P. Baryon acoustic oscillations signature in the three-point angular correlation function from the SDSS-DR12 quasar survey. Mon. Not. R. Astron. Soc. 2020, 492, 4469–4476. [Google Scholar] [CrossRef]
- York, D.G.; Adelman, J.; John, E.; Anderson, J.; Anderson, S.F.; Annis, J.; Bahcall, N.A.; Bakken, J.A.; Barkhouser, R.; Bastian, S.; et al. The Sloan Digital Sky Survey: Technical Summary. Astron. J. 2000, 120, 1579–1587. [Google Scholar] [CrossRef]
- Giostri, R.; dos Santos, M.V.; Waga, I.; Reis, R.; Calvão, M.; Lago, B.L. From cosmic deceleration to acceleration: New constraints from SN Ia and BAO/CMB. J. Cosmol. Astropart. Phys. 2012, 2012, 027. [Google Scholar] [CrossRef]
- Percival, W.J.; Reid, B.A.; Eisenstein, D.J.; Bahcall, N.A.; Budavari, T.; Frieman, J.A.; Fukugita, M.; Gunn, J.E.; Ivezic, Z.; Knapp, G.R.; et al. Baryon acoustic oscillations in the Sloan Digital Sky Survey Data Release 7 galaxy sample. Mon. Not. R. Astron. Soc. 2010, 401, 2148–2168. [Google Scholar] [CrossRef]
- Blake, C.; Kazin, E.A.; Beutler, F.; Davis, T.M.; Parkinson, D.; Brough, S.; Colless, M.; Contreras, C.; Couch, W.; Croom, S.; et al. The WiggleZ Dark Energy Survey: Mapping the distance–redshift relation with baryon acoustic oscillations. Mon. Not. R. Astron. Soc. 2011, 418, 1707–1724. [Google Scholar] [CrossRef]
- Beutler, F.; Blake, C.; Colless, M.; Jones, D.H.; Staveley-Smith, L.; Campbell, L.; Parker, Q.; Saunders, W.; Watson, F. The 6dF Galaxy Survey: Baryon acoustic oscillations and the local Hubble constant. Mon. Not. R. Astron. Soc. 2011, 416, 3017–3032. [Google Scholar] [CrossRef]
- Eisenstein, D.J.; Zehavi, I.; Hogg, D.W.; Scoccimarro, R.; Blanton, M.R.; Nichol, R.C.; York, D.G. Detection of the baryon acoustic peak in the large-scale correlation function of SDSS luminous red galaxies. Astrophys. J. 2005, 633, 560–574. [Google Scholar] [CrossRef]
- Hinshaw, G.; Larson, D.; Komatsu, E.; Spergel, D.N.; Bennett, C.L.; Dunkley, J.; Nolta, M.R.; Halpern, M.; Hill, R.S.; Odegard, N.; et al. Nine-year Wilkinson Microwave Anisotropy Probe (WMAP) Observations: Cosmological Parameter Results. Astrophys. J. Suppl. Ser. 2013, 208, 19. [Google Scholar] [CrossRef]
- Ade, P.A.R.; Aghanim, N.; Arnaud, M.; Ashdown, M.; Aumont, J.; Baccigalupi, C.; Matarrese, S. Planck 2015 results. XIII. Cosmological parameters. Astron. Astrophys. 2015, 594, A13. [Google Scholar]
- Hu, W.; Dodelson, S. Cosmic Microwave Background Anisotropies. Ann. Rev. Astron. Astrophys. 2002, 40, 171–216. [Google Scholar] [CrossRef]
- Komatsu, E.; Smith, K.M.; Dunkley, J.; Bennett, C.L.; Gold, B.; Hinshaw, G.; Jarosik, N.; Larson, D.; Nolta, M.R.; Page, L.; et al. Seven-year wilkinson microwave anisotropy probe (WMAP) observations: Cosmological interpretation. Astrophys. J. Suppl. Ser. 2011, 192, 18. [Google Scholar] [CrossRef]
- Wang, Y.; Mukherjee, P. Robust dark energy constraints from supernovae, galaxy clustering, and three-year wilkinson microwave anisotropy probe observations. Astrophys. J. 2006, 650, 1–6. [Google Scholar] [CrossRef]
- Wang, Y.; Chuang, C.H.; Mukherjee, P. A Comparative Study of Dark Energy Constraints from Current Observational Data. Phys. Rev. D 2012, 85, 023517. [Google Scholar] [CrossRef]
- Hu, W.; Sugiyama, N. Small scale cosmological perturbations: An Analytic approach. Astrophys. J. 1996, 471, 542–570. [Google Scholar] [CrossRef]
- Bond, J.R.; Efstathiou, G.; Tegmark, M. Forecasting cosmic parameter errors from microwave background anisotropy experiments. Mon. Not. R. Astron. Soc. 1997, 291, L33–L41. [Google Scholar] [CrossRef]
- Neveu, J.; Ruhlmann-Kleider, V.; Astier, P.; Besançon, M.; Guy, J.; Möller, A.; Babichev, E. Constraining the ΛCDM and Galileon models with recent cosmological data. Astron. Astrophys. 2017, 600, A40. [Google Scholar] [CrossRef]
- Moresco, M.; Cimatti, A.; Jimenez, R.; Pozzetti, L.; Zamorani, G.; Bolzonella, M.; Welikala, N. Improved constraints on the expansion rate of the Universe up toz∼ 1.1 from the spectroscopic evolution of cosmic chronometers. J. Cosmol. Astropart. Phys. 2012, 2012, 006. [Google Scholar] [CrossRef]
- Chae, K.H.; Biggs, A.D.; Blandford, R.D.; Browne, I.W.A.; De Bruyn, A.G.; Fassnacht, C.D.; York, T. Constraints on cosmological parameters from the analysis of the Cosmic Lens All sky Survey radio - selected gravitational lens statistics. Phys. Rev. Lett. 2002, 89, 151301. [Google Scholar] [CrossRef]
- Biesiada, M.; Piórkowska, A.; Malec, B. Cosmic equation of state from strong gravitational lensing systems. Mon. Not. R. Astron. Soc. 2010, 406, 1055–1059. [Google Scholar] [CrossRef]
- Magaña, J.; Motta, V.; Cárdenas, V.H.; Verdugo, T.; Jullo, E. A magnified glance into the dark sector: Probing cosmological models with strong lensing in A1689. Astrophys. J. 2015, 813, 69. [Google Scholar] [CrossRef]
- Magaña, J.; Acebrón, A.; Motta, V.; Verdugo, T.; Jullo, E.; Limousin, M. Strong Lensing Modeling in Galaxy Clusters as a Promising Method to Test Cosmography. I. Parametric Dark Energy Models. Astrophys. J. 2018, 865, 122. [Google Scholar] [CrossRef]
- Ofek, E.O.; Rix, H.W.; Maoz, D. The redshift distribution of gravitational lenses revisited: Constraints on galaxy mass evolution. Mon. Not. R. Astron. Soc. 2003, 343, 639. [Google Scholar] [CrossRef][Green Version]
- Foreman-Mackey, D.; Hogg, D.W.; Lang, D.; Goodman, J. emcee: The MCMC Hammer. PASP 2013, 125, 306. [Google Scholar] [CrossRef]
- Gelman, A.; Rubin, D. Inference from Iterative Simulation using Multiple Sequences. Stat. Sci. 1992, 67, 457–511. [Google Scholar] [CrossRef]
- García-Aspeitia, M.A.; Hernández-Almada, A.; Magaña, J.; Motta, V. On the birth of the cosmological constant and the reionization era. arXiv 2019, arXiv:1912.07500. [Google Scholar]
- Riess, A.G.; Casertano, S.; Yuan, W.; Bowers, J.B.; Macri, L.; Zinn, J.C.; Scolnic, D. Cosmic Distances Calibrated to 1% Precision with Gaia EDR3 Parallaxes and Hubble Space Telescope Photometry of 75 Milky Way Cepheids Confirm Tension with ΛCDM. Astrophys. J. Lett. 2021, 908, L6. [Google Scholar] [CrossRef]
- Chevallier, M.; Polarski, D. Accelerating universes with scaling dark matter. Int. J. Mod. Phys. 2001, D10, 213–224. [Google Scholar] [CrossRef]
- Linder, E.V. Exploring the Expansion History of the Universe. Phys. Rev. Lett. 2003, 90, 091301. [Google Scholar] [CrossRef]
- Linder, E.V. Cosmic shear with next generation redshift surveys as a cosmological probe. Phys. Rev. 2003, D68, 083503. [Google Scholar] [CrossRef]
- Jassal, H.K.; Bagla, J.S.; Padmanabhan, T. WMAP constraints on low redshift evolution of dark energy. Mon. Not. R. Astron. Soc. 2005, 356, L11–L16. [Google Scholar] [CrossRef]
- Barboza, E.M., Jr.; Alcaniz, J.S. A parametric model for dark energy. Phys. Lett. 2008, B666, 415–419. [Google Scholar] [CrossRef]
- Feng, C.J.; Shen, X.Y.; Li, P.; Li, X.Z. A New Class of Parametrization for Dark Energy without Divergence. JCAP 2012, 1209, 023. [Google Scholar] [CrossRef]
- Sendra, I.; Lazkoz, R. Supernova and baryon acoustic oscillation constraints on (new) polynomial dark energy parametrizations: Current results and forecasts. Mon. Not. R. Astron. Soc. 2012, 422, 776–793. [Google Scholar] [CrossRef]
- Luongo, O. Cosmography with the Hubble Parameter. Mod. Phys. Lett. A 2011, 26, 1459–1466. [Google Scholar] [CrossRef]
- Aviles, A.; Gruber, C.; Luongo, O.; Quevedo, H. Cosmography and constraints on the equation of state of the Universe in various parametrizations. Phys. Rev. D 2012, 86, 123516. [Google Scholar] [CrossRef]
- Gruber, C.; Luongo, O. Cosmographic analysis of the equation of state of the universe through Padé approximations. Phys. Rev. D 2014, 89, 103506. [Google Scholar] [CrossRef]
- Demianski, M.; Piedipalumbo, E.; Rubano, C.; Scudellaro, P. High-redshift cosmography: New results and implications for dark energy. Mon. Not. R. Astron. Soc. 2012, 426, 1396–1415. [Google Scholar] [CrossRef]
- Zhang, M.J.; Li, H.; Xia, J.Q. What do we know about cosmography. Eur. Phys. J. 2017, C77, 434. [Google Scholar] [CrossRef]
- Al Mamon, A.; Das, S. A divergence-free parametrization of deceleration parameter for scalar field dark energy. Int. J. Mod. Phys. D 2016, 25, 1650032. [Google Scholar] [CrossRef]
- Lizardo, A.; Amante, M.H.; García-Aspeitia, M.A.; Magaña, J.; Motta, V. Cosmography using strong lensing systems and cosmic chronometers. arXiv 2020, arXiv:2008.10655. [Google Scholar]
- Santos, B.; Carvalho, J.C.; Alcaniz, J.S. Current constraints on the epoch of cosmic acceleration. Astropart. Phys. 2011, 35, 17–20. [Google Scholar] [CrossRef]
- Del Campo, S.; Duran, I.; Herrera, R.; Pavón, D. Three thermodynamically based parametrizations of the deceleration parameter. Phys. Rev. D 2012, 86, 083509. [Google Scholar] [CrossRef]
- Nair, R.; Jhingan, S.; Jain, D. Cosmokinetics: A joint analysis of standard candles, rulers and cosmic clocks. J. Cosmol. Astropart. Phys. 2012, 2012, 018. [Google Scholar] [CrossRef]
- Mamon, A.A.; Das, S. A parametric reconstruction of the deceleration parameter. Eur. Phys. J. C 2017, 77, 495. [Google Scholar] [CrossRef]
- Román-Garza, J.; Verdugo, T.; Magaña, J.; Motta, V. Constraints on barotropic dark energy models by a new phenomenological q(z) parameterization. Eur. Phys. J. C 2019, 79, 890. [Google Scholar] [CrossRef]
- Sharov, G.S.; Vorontsova, E.G. Parameters of cosmological models and recent astronomical observations. JCAP 2014, 10, 057. [Google Scholar] [CrossRef]
- Ade, P.A.R.; Aghanim, N.; Arnaud, M.; Ashdown, M.; Aumont, J.; Baccigalupi, C.; Munshi, D. Planck 2015 results. XIV. Dark energy and modified gravity. Astron. Astrophys. 2015, 594, A14. [Google Scholar]
- Magaña, J.; Motta, V.; Cardenas, V.H.; Foex, G. Testing cosmic acceleration for w(z) parameterizations using fgas measurements in galaxy clusters. Mon. Not. R. Astron. Soc. 2017, 469, 47–61. [Google Scholar] [CrossRef]
- Shenavar, H.; Javidan, K. A Modified Dynamical Model of Cosmology I Theory. Universe 2020, 6, 1. [Google Scholar] [CrossRef]
- Dymnikova, I.; Dobosz, A.; Sołtysek, B. Lemaître Class Dark Energy Model for Relaxing Cosmological Constant. Universe 2017, 3, 39. [Google Scholar] [CrossRef]
- Deng, X.M. A Modified Generalized Chaplygin Gas as the Unified Dark Matter–Dark Energy Revisited. Braz. J. Phys. 2011, 41, 333–348. [Google Scholar] [CrossRef]
- Bhadra, J.; Debnath, U. Dynamical system analysis of interacting variable modified Chaplygin gas model in FRW universe. Eur. Phys. J. Plus 2012, 127, 30. [Google Scholar] [CrossRef][Green Version]
- Pourhassan, B. Viscous Modified Cosmic Chaplygin Gas Cosmology. Int. J. Mod. Phys. D 2013, 22, 1350061. [Google Scholar] [CrossRef]
- Bento, M.C.; Bertolami, O.; Sen, A.A. Letter: Generalized Chaplygin Gas Model: Dark Energy—Dark Matter Unification and CMBR Constraints. Gen. Relativ. Gravit. 2003, 35, 2063–2069. [Google Scholar] [CrossRef]
- Bento, M.C.; Bertolami, O.; Sen, A.A. Revival of the unified dark energy–dark matter model? Phys. Rev. D 2004, 70, 083519. [Google Scholar] [CrossRef]
- Chaplygin, S.A. On gas jets. Sci. Mem. Mosc. Univ. Math. Phys. 1904, 21, 1–121. [Google Scholar]
- Amendola, L.; Finelli, F.; Burigana, C.; Carturan, D. WMAP and the generalized Chaplygin gas. J. Cosmol. Astropart. Phys. 2003, 2003, 005. [Google Scholar] [CrossRef]
- Hova, H.; Yang, H. A dark energy model alternative to generalized Chaplygin gas. Int. J. Mod. Phys. D 2017, 26, 1750178. [Google Scholar] [CrossRef]
- Hernandez-Almada, A.; Magana, J.; Garcia-Aspeitia, M.A.; Motta, V. Cosmological constraints on alternative model to Chaplygin fluid revisited. Eur. Phys. J. 2019, C79, 12. [Google Scholar] [CrossRef]
- Brevik, I.H.; Gorbunova, O. Dark energy and viscous cosmology. Gen. Rel. Grav. 2005, 37, 2039–2045. [Google Scholar] [CrossRef]
- Cruz, M.; Cruz, N.; Lepe, S. Accelerated and decelerated expansion in a causal dissipative cosmology. Phys. Rev. D 2017, 96, 124020. [Google Scholar] [CrossRef]
- Hernández-Almada, A. Cosmological test on viscous bulk models using Hubble parameter measurements and type Ia supernovae data. Eur. Phys. J. C 2019, 79, 751. [Google Scholar] [CrossRef]
- Herrera-Zamorano, L.; Hernández-Almada, A.; García-Aspeitia, M. Constraints and cosmography of ΛCDM in presence of viscosity. Eur. Phys. J. C 2020, 80, 637. [Google Scholar] [CrossRef]
- Zimdahl, W.; Pavón, D. Scaling Cosmology. Gen. Relativ. Gravit. 2003, 35, 413–422. [Google Scholar] [CrossRef]
- Randall, L.; Sundrum, R. A large mass hierarchy from a small extra dimension. Phys. Rev. Lett. 1999, 83, 3370–3373. [Google Scholar] [CrossRef]
- Randall, L.; Sundrum, R. An Alternative to compactification. Phys. Rev. Lett. 1999, 83, 4690–4693. [Google Scholar] [CrossRef]
- Shiromizu, T.; Maeda, K.; Sasaki, M. The Einstein equations on the 3-brane world. Phys. Rev. D 2000, 62, 024012. [Google Scholar] [CrossRef]
- Al Mamon, A.; Bamba, K. Observational constraints on the jerk parameter with the data of the Hubble parameter. Eur. Phys. J. 2018, C78, 862. [Google Scholar] [CrossRef]
- Ai, W.Y. A note on the novel 4D Einstein–Gauss–Bonnet gravity. Commun. Theor. Phys. 2020, 72, 095402. [Google Scholar] [CrossRef]
- Gürses, M.; Şişman, T.C.; Tekin, B. Is there a novel Einstein–Gauss–Bonnet theory in four dimensions? Eur. Phys. J. C 2020, 80, 647. [Google Scholar] [CrossRef]
- Lu, H.; Pang, Y. Horndeski gravity as D→4 limit of Gauss–Bonnet. Phys. Lett. B 2020, 809, 135717. [Google Scholar] [CrossRef]
- Fernandes, P.G.S.; Carrilho, P.; Clifton, T.; Mulryne, D.J. Derivation of Regularized Field Equations for the Einstein–Gauss–Bonnet Theory in Four Dimensions. Phys. Rev. D 2020, 102, 024025. [Google Scholar] [CrossRef]
- Mahapatra, S. A note on the total action of 4D Gauss–Bonnet theory. Eur. Phys. J. C 2020, 80, 992. [Google Scholar] [CrossRef]
- Clifton, T.; Carrilh, P.; Fernandes, P.G.; Mulryne, D.J. Observational Constraints on the Regularized 4D Einstein–Gauss–Bonnet Theory of Gravity. Phys. Rev. D 2020, 102, 084005. [Google Scholar] [CrossRef]
- Efstathiou, G. To H0 or not to H0? arXiv 2021, arXiv:2103.08723. [Google Scholar]


| Data | ||||
| OHD | ||||
| SNIa | ||||
| CMB | ||||
| Joint | ||||
| DE Parametrizations | |||||
|---|---|---|---|---|---|
| Model | |||||
| JBP | |||||
| BA | |||||
| FSLLI | |||||
| FSLLII | |||||
| SL | |||||
| Data Set | OHD | JLA | Joint |
|---|---|---|---|
| h | |||
| a | - | ||
| b | - | ||
| - | |||
| - |
| Data | n | h | ||||
|---|---|---|---|---|---|---|
| OHD | - | |||||
| SNIa | ||||||
| OHD+SNIa |
| Tanh Model | |||||
|---|---|---|---|---|---|
| Data | b | n | h | ||
| OHD | - | ||||
| SNIa | |||||
| OHD+SNIa | |||||
| Cosh Model | |||||
| Data | b | n | h | ||
| OHD | - | ||||
| SNIa | |||||
| OHD+SNIa | |||||
| Sample | h | |||||
|---|---|---|---|---|---|---|
| OHD | – | – | ||||
| SNIa | – | |||||
| SLS | – | – | ||||
| Joint | – | |||||
| OHD | – | |||||
| SNIa | ||||||
| SLS | – | |||||
| Joint | ||||||
| Model | h | ||||
|---|---|---|---|---|---|
| IVM | |||||
| IM | 0 | ||||
| VM | 0 | ||||
| LCDM | 0 | 0 |
| Sample | h | ||||
|---|---|---|---|---|---|
| PEDE | |||||
| homogeneous OHD | () | () | () | 0 | |
| non-homogeneous OHD | () | () | () | 0 | |
| DA OHD | () | () | () | 0 | |
| Sample | h | ||||
|---|---|---|---|---|---|
| GEDE | |||||
| homogeneous OHD | () | () | () | () | () |
| non-homogeneous OHD | () | () | () | () | () |
| DA OHD | () | () | () | () | () |
| Sample | h | ||||
|---|---|---|---|---|---|
| OHD | <−0.88 | ||||
| BAO | <−9.52 | ||||
| SNIa | <−0.31 | ||||
| CMB | <−15.0 | ||||
| Joint | <−16.2 |
| Sample | h | n | eV | ||
|---|---|---|---|---|---|
| OHD | |||||
| BAO | |||||
| SNIa | |||||
| CMB | |||||
| Joint |
| Sample | h | ||
|---|---|---|---|
| OHD | |||
| SnIa | |||
| CMB | |||
| BAO | |||
| Joint |
| Sample | h | ||||
|---|---|---|---|---|---|
| OHD | – | ||||
| BAO | – | ||||
| SNIa | |||||
| SLS | – | ||||
| HIIG | – | ||||
| Joint |
| Orignal Cardassian | |||||
|---|---|---|---|---|---|
| Data | n | l | h | ||
| OHD (DA) | |||||
| SNIa | |||||
| Joint | |||||
| Modified polytropic Cardassian | |||||
| OHD (DA) | |||||
| SNIa | |||||
| Joint | |||||
| Model | h | Sample | Prior |
|---|---|---|---|
| wCDM | OHD, SNIa, CMB | Gaussian ( | |
| CPL | OHD | Flat [0.2,1.0] | |
| JBP | OHD, CMB, BAO | Gaussian () | |
| BA | OHD, CMB, BAO | Gaussian () | |
| FSLLI | OHD, CMB, BAO | Gaussian () | |
| FSLLII | OHD, CMB, BAO | Gaussian () | |
| SL | OHD, CMB, BAO | Gaussian () | |
| Chaplygin-Like Fluid | OHD, SNIa | Gaussian () | |
| Viscous (Polynomial) | OHD, SNIa | Gaussian () | |
| Viscous (Hyperbolic tanh) | OHD, SNIa | Gaussian () | |
| Viscous (Hyperbolic cosh) | OHD, SNIa | Gaussian () | |
| Viscous ( Constant) | OHD, SNIa, SLS | Gaussian () | |
| Viscous ( Polynomial) | OHD, SNIa, SLS | Gaussian () | |
| Interacting Viscous | OHD | Gaussian () | |
| PEDE | Homogeneous OHD | Gaussian () | |
| GEDE | Homogeneous OHD | Gaussian () | |
| CBT | BAO, SNIa, OHD, CMB | Gaussian () | |
| VBT | OHD, SNIa, BAO, BAO | Gaussian () | |
| UG | OHD, SNIa, CMB, BAO | Flat [0.2,1.0] | |
| EGB | SNIa, BAO, OHD, SLS, HIIG | Gaussian () | |
| Original Cardassian | SNIa, OHD | Flat [0,1] | |
| Modified Polytropic Cardassian | SNIa, OHD | Flat [0,1] |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Motta, V.; García-Aspeitia, M.A.; Hernández-Almada, A.; Magaña, J.; Verdugo, T. Taxonomy of Dark Energy Models. Universe 2021, 7, 163. https://doi.org/10.3390/universe7060163
Motta V, García-Aspeitia MA, Hernández-Almada A, Magaña J, Verdugo T. Taxonomy of Dark Energy Models. Universe. 2021; 7(6):163. https://doi.org/10.3390/universe7060163
Chicago/Turabian StyleMotta, Verónica, Miguel A. García-Aspeitia, Alberto Hernández-Almada, Juan Magaña, and Tomás Verdugo. 2021. "Taxonomy of Dark Energy Models" Universe 7, no. 6: 163. https://doi.org/10.3390/universe7060163
APA StyleMotta, V., García-Aspeitia, M. A., Hernández-Almada, A., Magaña, J., & Verdugo, T. (2021). Taxonomy of Dark Energy Models. Universe, 7(6), 163. https://doi.org/10.3390/universe7060163






