1S0 Pairing Gaps, Chemical Potentials and Entrainment Matrix in Superfluid Neutron-Star Cores for the Brussels–Montreal Functionals
Abstract
:1. Introduction
2. Nuclear Superfluidity within the Time-Dependent Hartree–Fock–Bogoliubov Theory
2.1. General Principles
2.2. Functionals of Local Densities and Currents
- (i)
- the nucleon densities at position at time t ( distinguishing the two spin states),
- (ii)
- the kinetic-energy densities (in units of at position and time t,
- (iii)
- the momentum densities (in units of ℏ) at position and time t,
- (iv)
- the abnormal densities at position and time t
2.3. Application to Homogeneous Systems
2.4. Physical Interpretation of the Different Velocities and Momentum Densities
2.5. Landau’s Approximations
- the single-particle energies (31) are calculated at zero temperature, in the absence of currents ignoring any dependence on the pairing gaps, and expanding linearly around the Fermi surface (denoting by the approximate expression for a quantity Q)
- the quasiparticle energies (30) are similarly expanded as
- the density of single-particle states in -space integrations (36) is approximated by its value on the Fermi surface, with
3. Application to Neutron Stars
3.1. Brussels–Montreal Functionals
3.2. Numerical Implementation
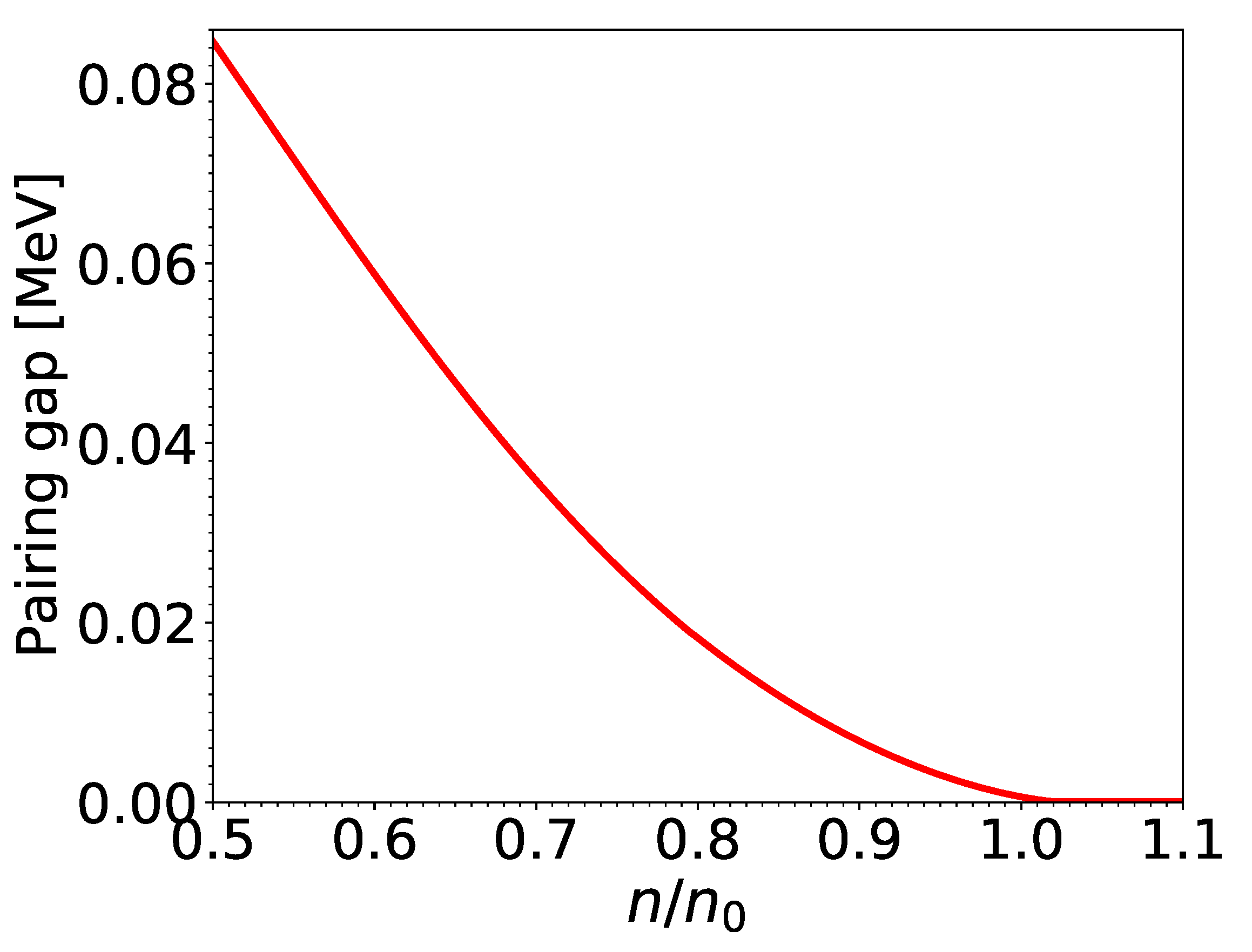
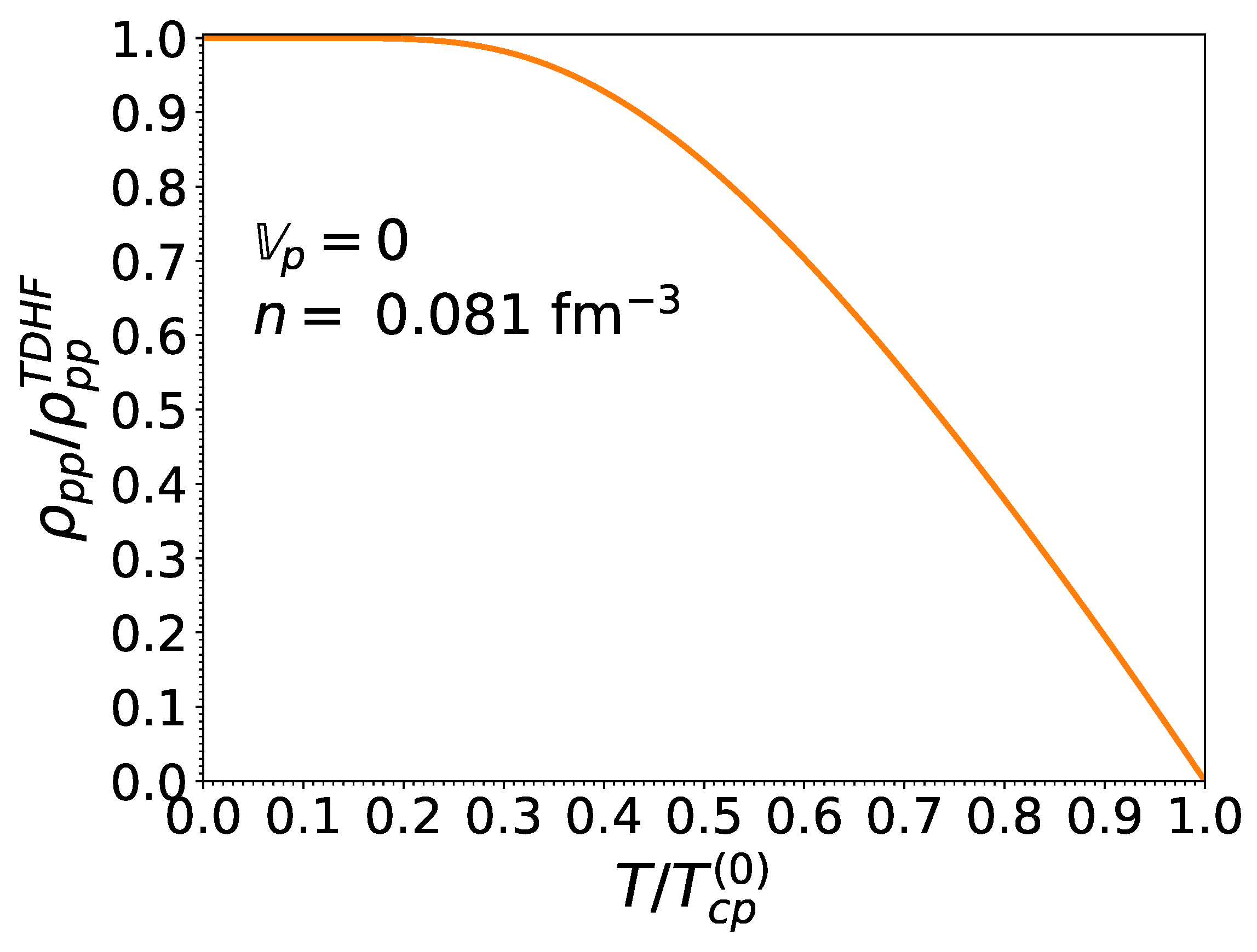
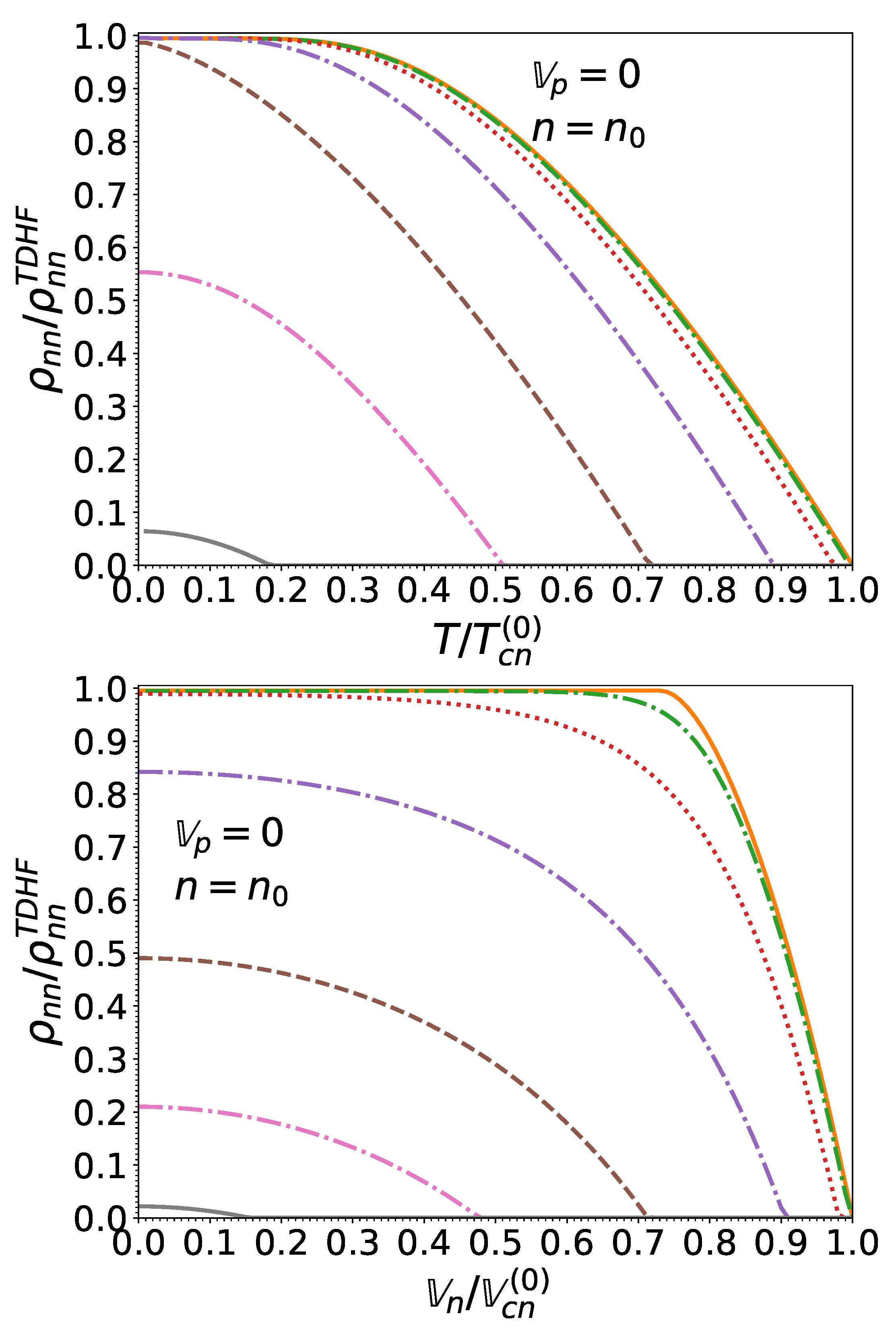
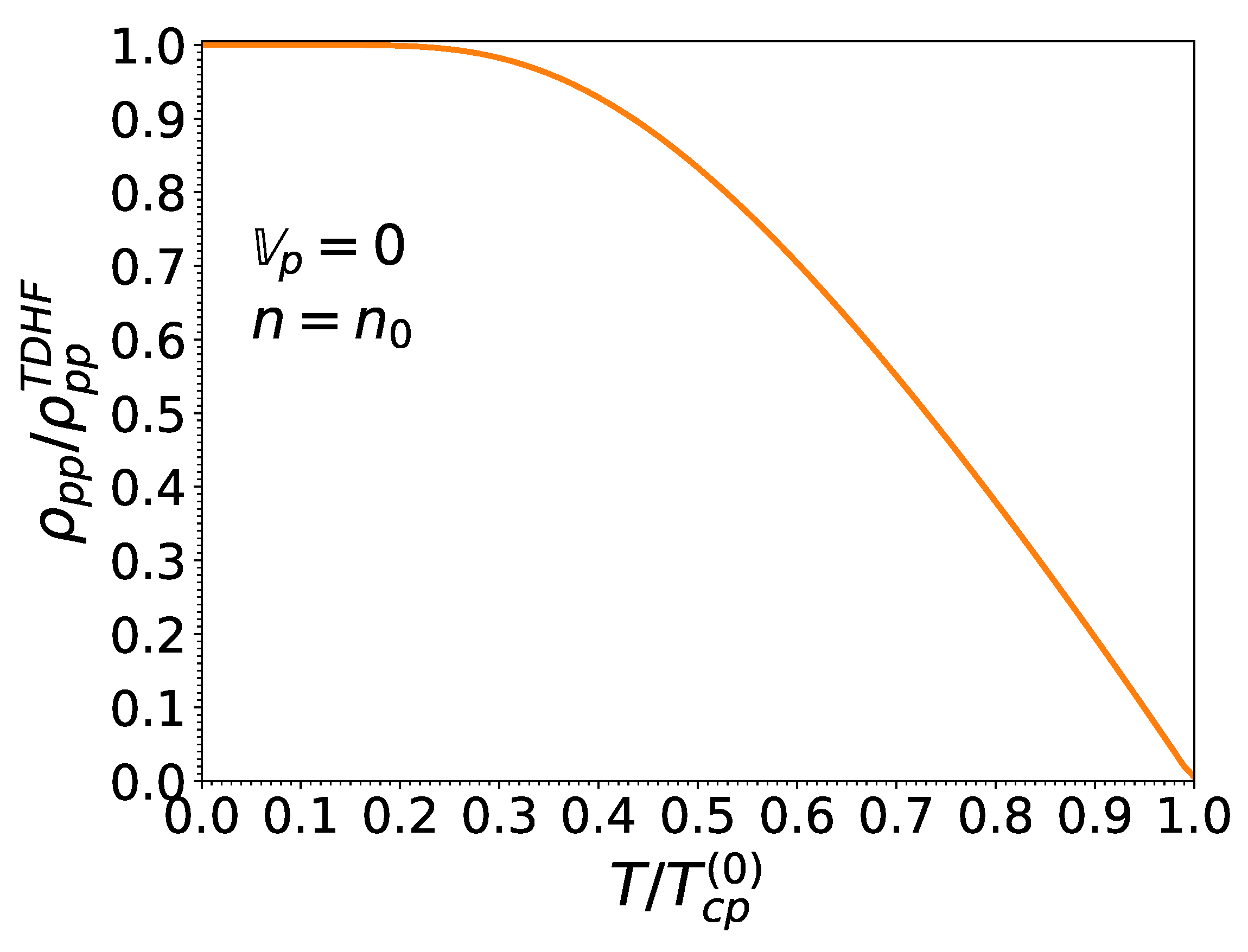
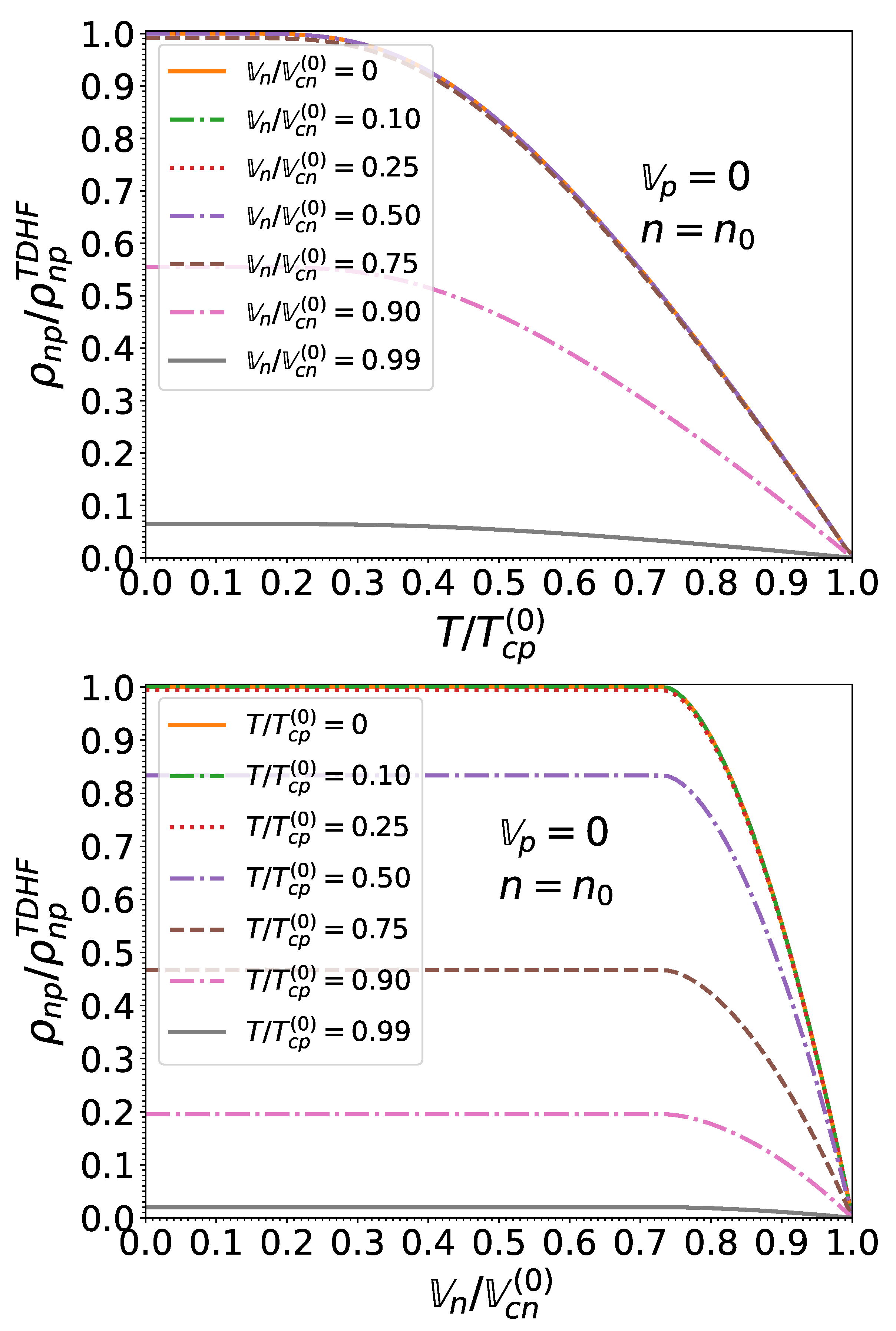
3.3. S Pairing Gaps
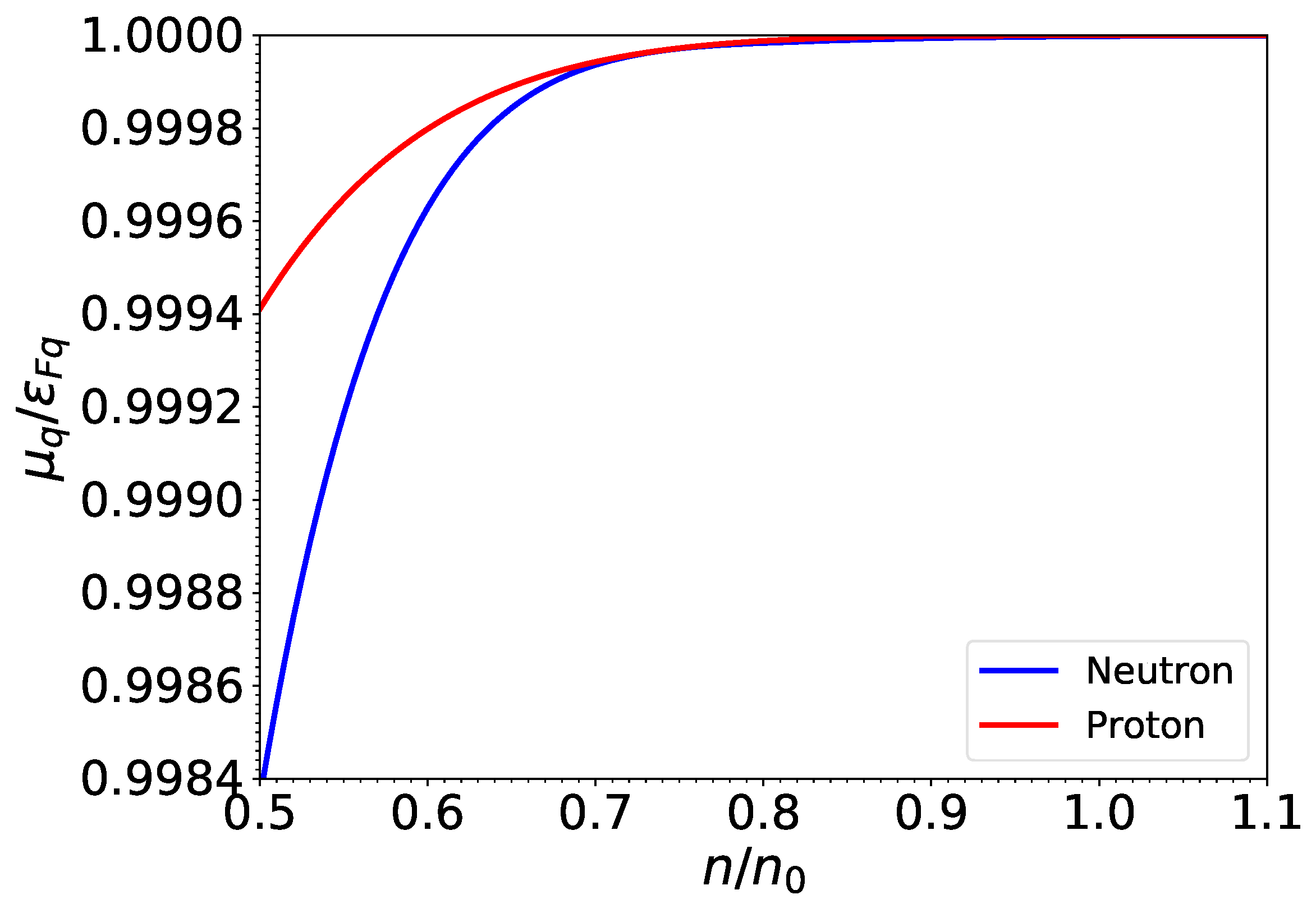
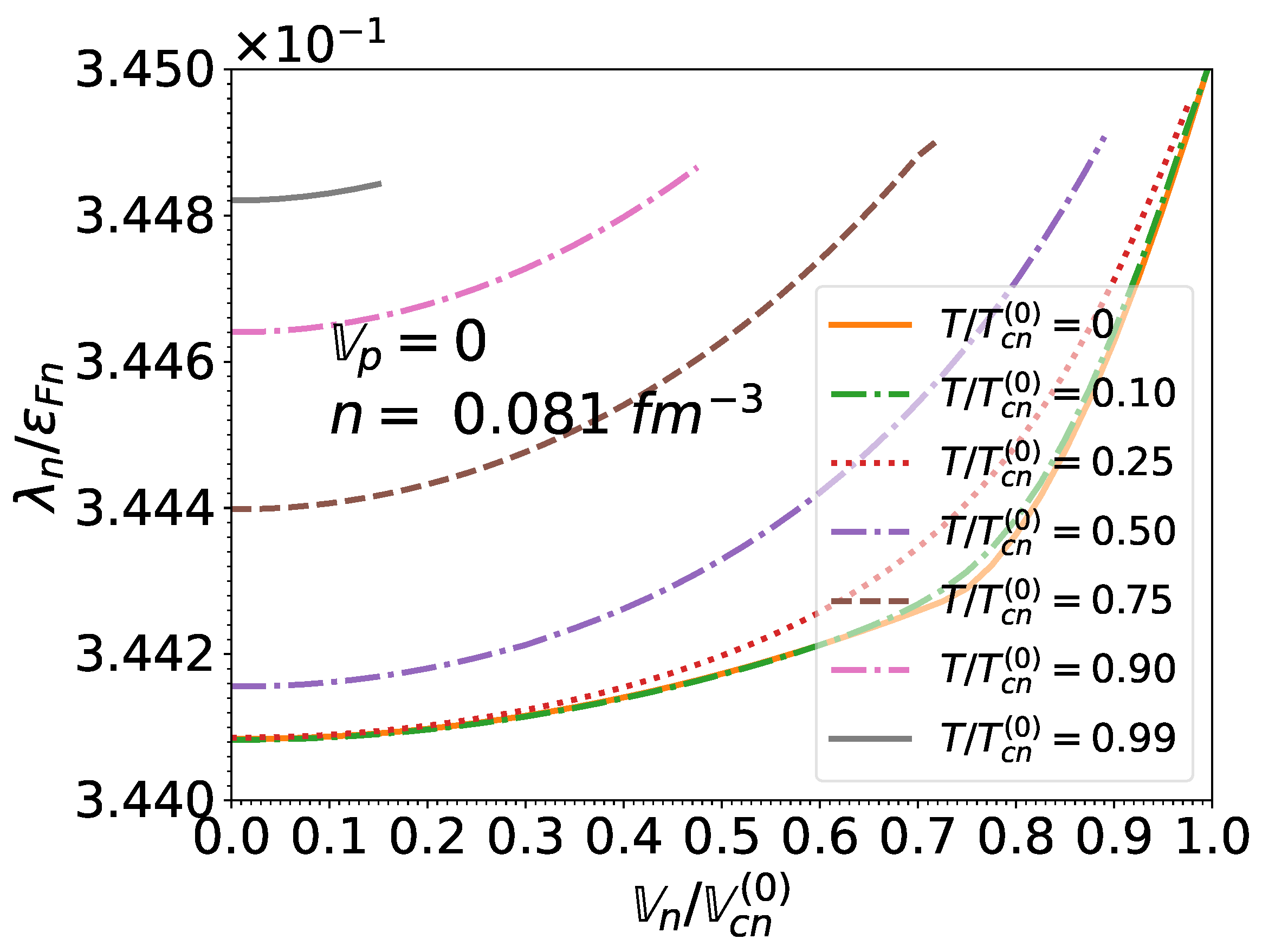
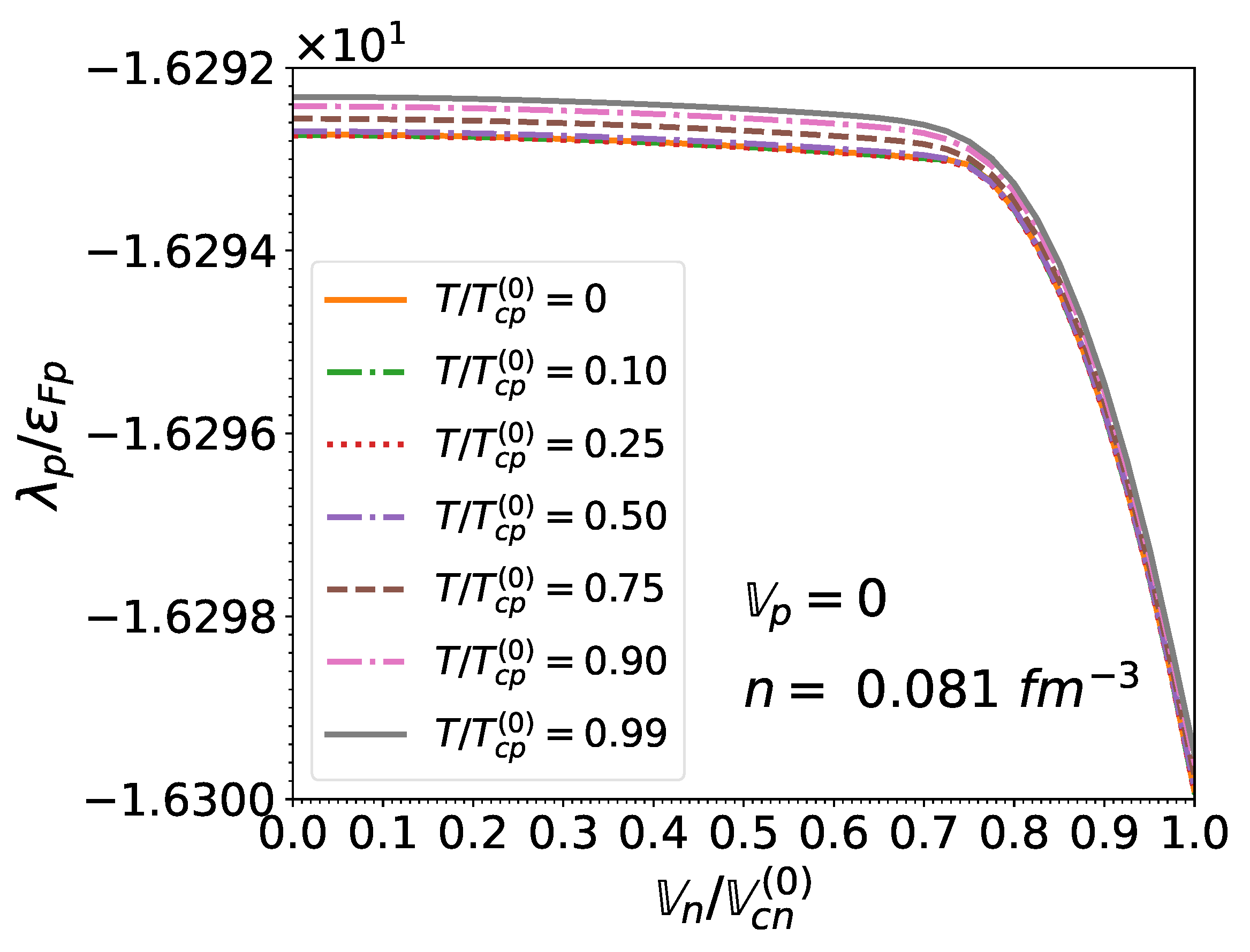
3.4. Reduced Chemical Potentials
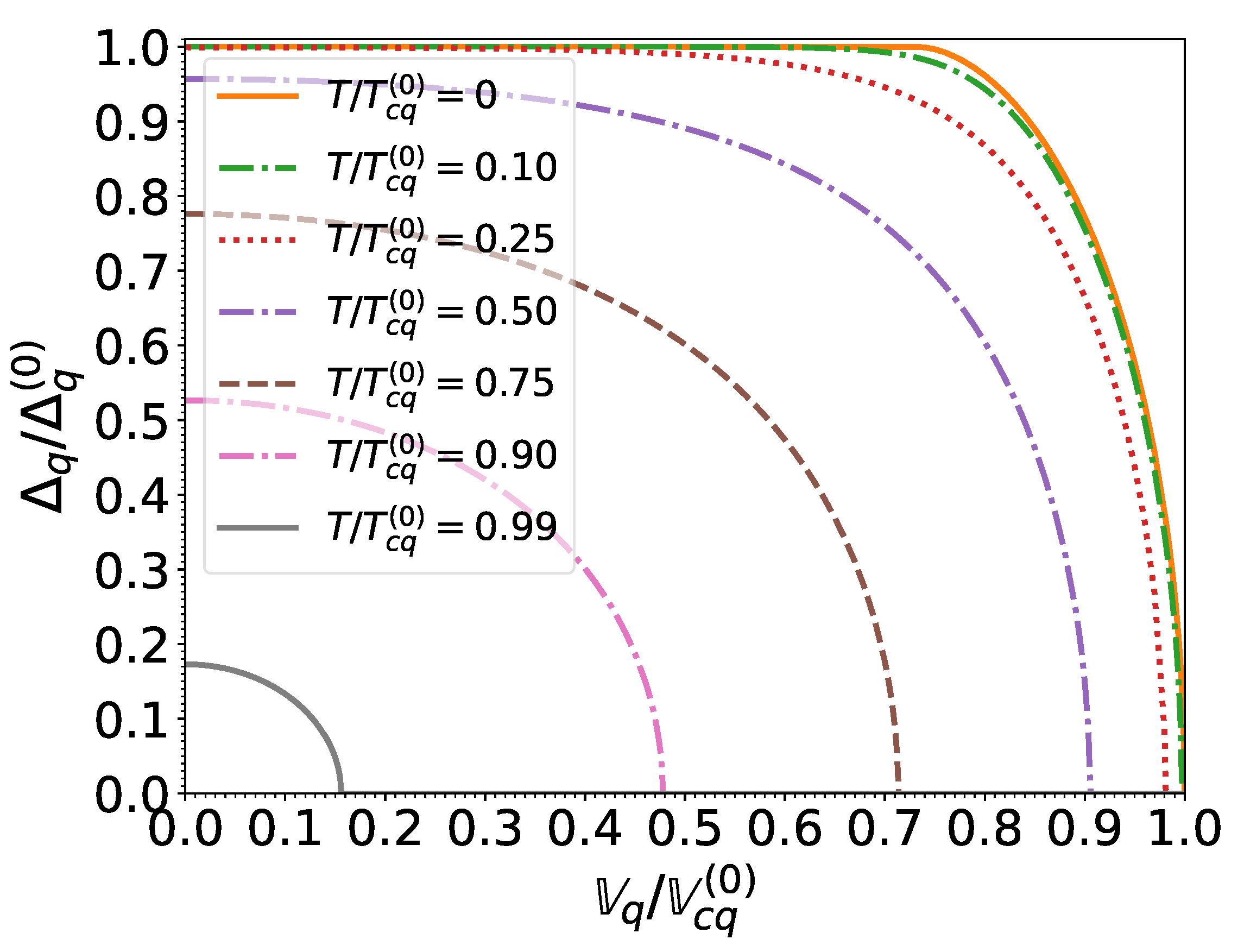
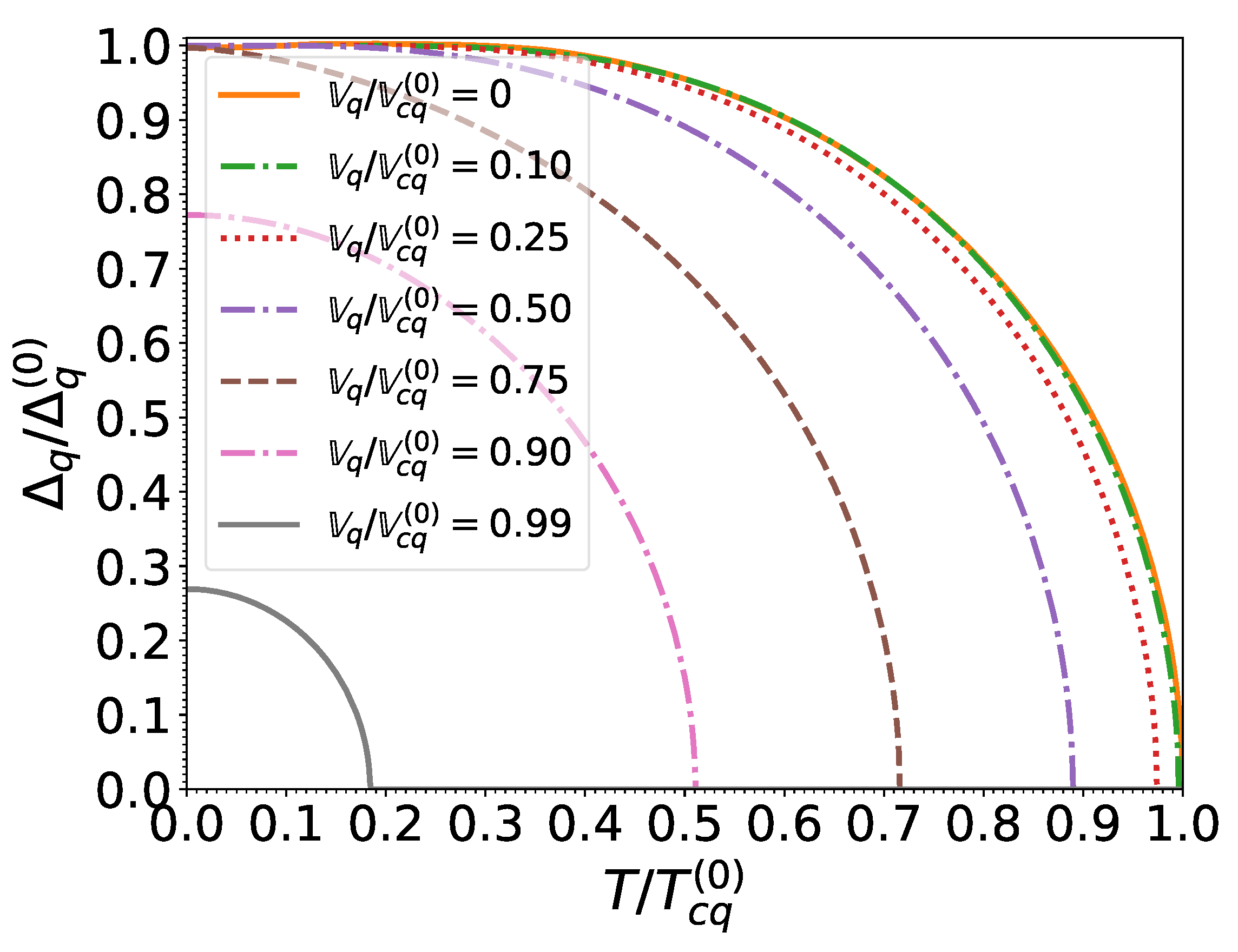
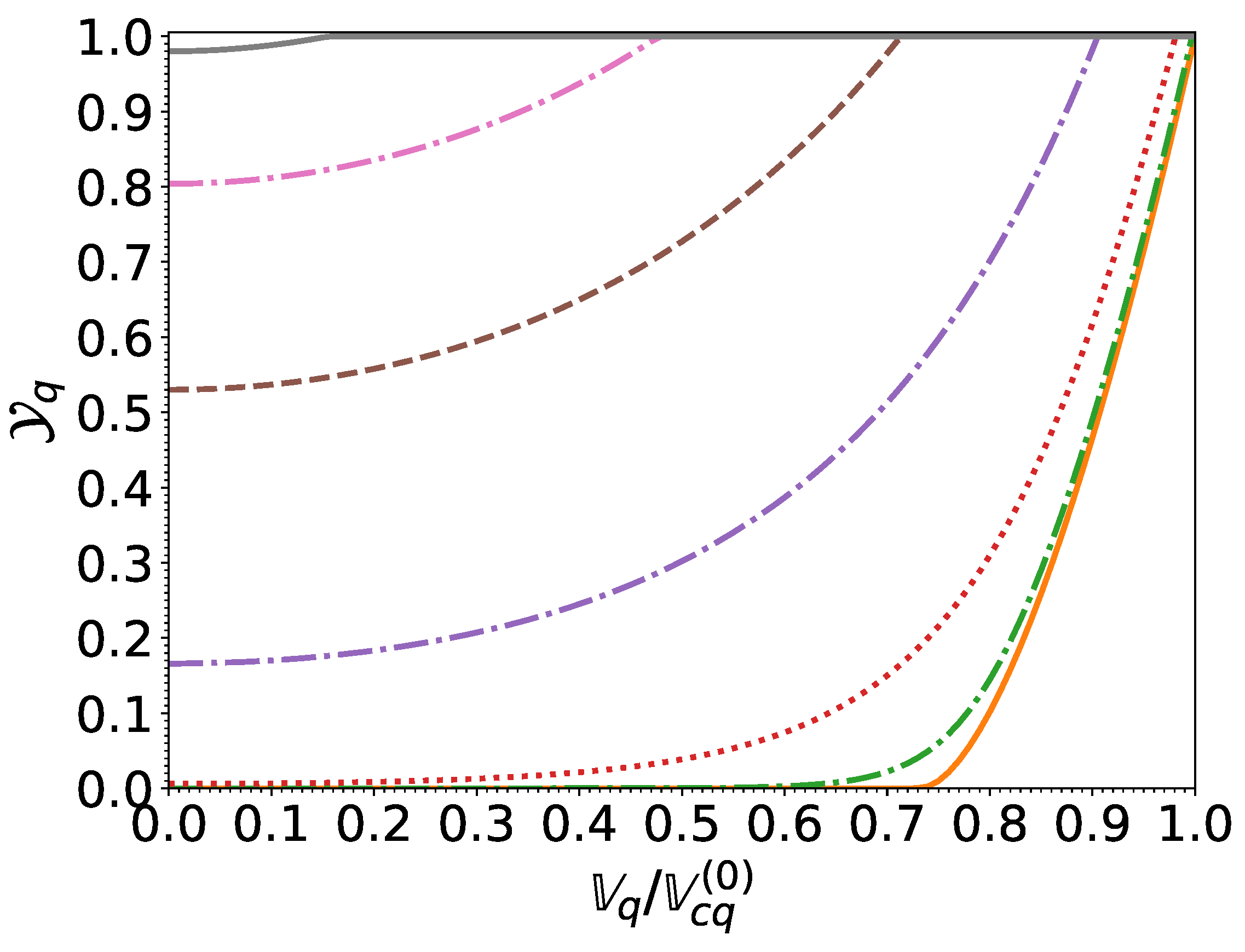
3.5. Functions
3.6. Effective versus True Superfluid Velocities
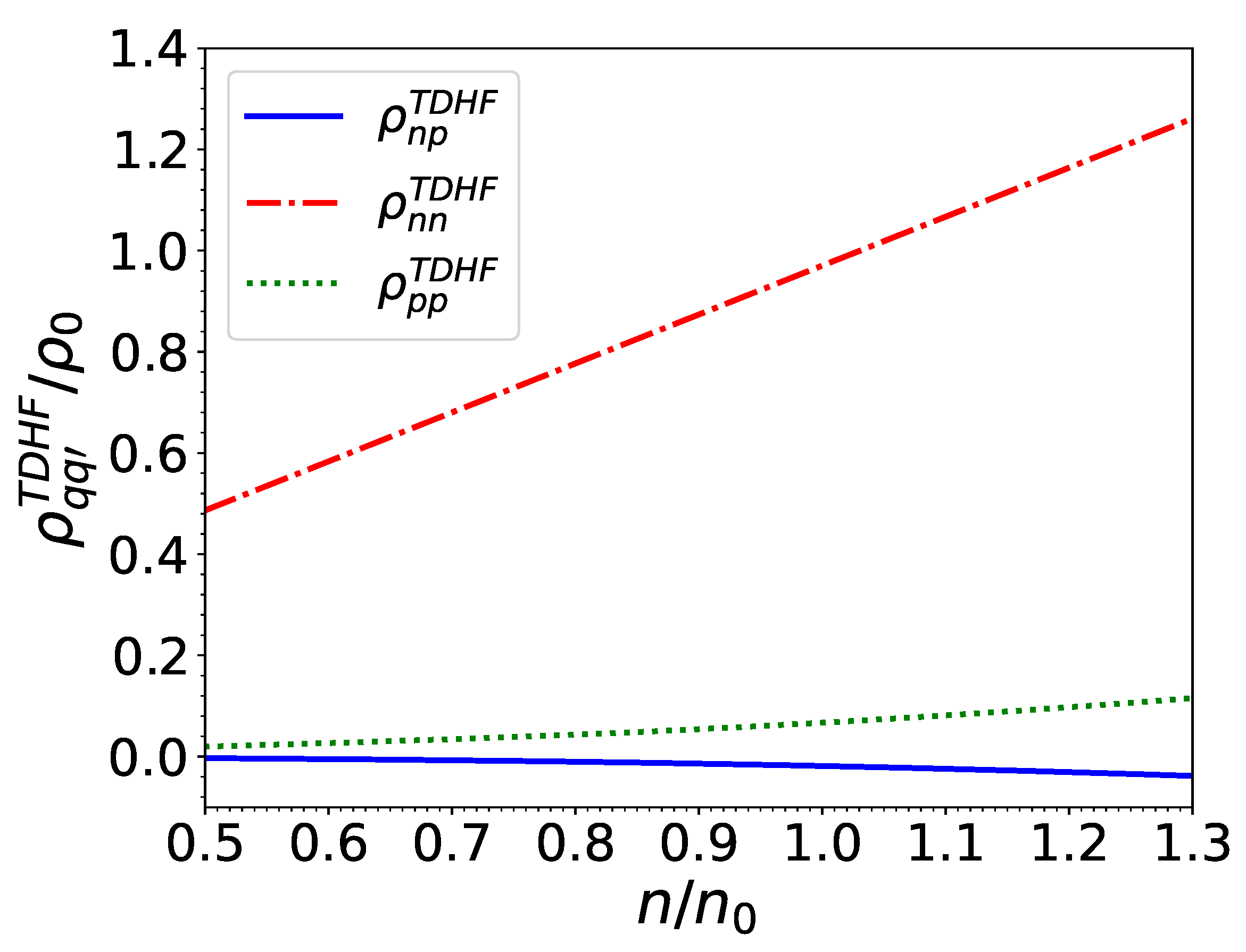
3.7. Entrainment Matrix
3.8. Chemical Potentials
4. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
Abbreviations
| TDHFB | time-dependent Hartree–Fock–Bogoliubov |
| TDHF | time-dependent Hartree–Fock |
| NM | (Pure) Neutron matter |
| SM | Symmetric matter |
Appendix A. Weak-Coupling Approximation
References
- Chamel, N. Superfluidity and Superconductivity in Neutron Stars. J. Astrophys. Astron. 2017, 38, 43. [Google Scholar] [CrossRef] [Green Version]
- Ho, W.C.G.; Andersson, N. Rotational evolution of young pulsars due to superfluid decoupling. Nat. Phys. 2012, 8, 787–789. [Google Scholar] [CrossRef] [Green Version]
- Gusakov, M.E.; Chugunov, A.I.; Kantor, E.M. Instability Windows and Evolution of Rapidly Rotating Neutron Stars. Phys. Rev. Lett. 2014, 112, 151101. [Google Scholar] [CrossRef] [Green Version]
- Ho, W.C.G.; Elshamouty, K.G.; Heinke, C.O.; Potekhin, A.Y. Tests of the nuclear equation of state and superfluid and superconducting gaps using the Cassiopeia A neutron star. Phys. Rev. C 2015, 91, 015806. [Google Scholar] [CrossRef]
- Andersson, N. A Superfluid Perspective on Neutron Star Dynamics. Universe 2021, 7, 17. [Google Scholar] [CrossRef]
- Andreev, A.F.; Bashkin, E.P. Three-velocity hdrodynamics of superfluid solutions. Sov. Phys. JETP 1975, 42, 164–167. [Google Scholar]
- Carter, B.; Khalatnikov, I.M. Canonically Covariant Formulation of Landau’s Newtonian Superfluid Dynamics. Rev. Math. Phys. 1994, 6, 277–304. [Google Scholar] [CrossRef]
- Prix, R. Variational description of multifluid hydrodynamics: Uncharged fluids. Phys. Rev. D 2004, 69, 043001. [Google Scholar] [CrossRef] [Green Version]
- Gusakov, M.E.; Kantor, E.M. Velocity-dependent energy gaps and dynamics of superfluid neutron stars. Mon. Not. R. Astron. Soc. 2013, 428, L26–L30. [Google Scholar] [CrossRef] [Green Version]
- Dommes, V.A.; Kantor, E.M.; Gusakov, M.E. Temperature-dependent oscillation modes in rotating superfluid neutron stars. Mon. Not. R. Astron. Soc. 2019, 482, 2573–2587. [Google Scholar] [CrossRef] [Green Version]
- Kantor, E.M.; Gusakov, M.E. Entrainment matrix for BSk energy-density functionals. J. Phys. Conf. Ser. 2020, 1697, 012005. [Google Scholar] [CrossRef]
- Kantor, E.M.; Gusakov, M.E.; Dommes, V.A. Resonance suppression of the r -mode instability in superfluid neutron stars: Accounting for muons and entrainment. Phys. Rev. D 2021, 103, 023013. [Google Scholar] [CrossRef]
- Gusakov, M.E.; Haensel, P. The entrainment matrix of a superfluid neutron-proton mixture at a finite temperature. Nucl. Phys. A 2005, 761, 333–348. [Google Scholar] [CrossRef] [Green Version]
- Leinson, L.B. Non-linear approach to the entrainment matrix of superfluid nucleon mixture at zero temperature. Mon. Not. R. Astron. Soc. 2017, 470, 3374–3387. [Google Scholar] [CrossRef] [Green Version]
- Leinson, L.B. The entrainment matrix of a superfluid nucleon mixture at finite temperatures. Mon. Not. R. Astron. Soc. 2018, 479, 3778–3790. [Google Scholar] [CrossRef]
- Chamel, N.; Allard, V. Entrainment effects in neutron-proton mixtures within the nuclear energy-density functional theory: Low-temperature limit. Phys. Rev. C 2019, 100, 065801. [Google Scholar] [CrossRef] [Green Version]
- Allard, V.; Chamel, N. Entrainment effects in neutron-proton mixtures within the nuclear energy-density functional theory. II. Finite temperatures and arbitrary currents. Phys. Rev. C 2021, 103, 025804. [Google Scholar] [CrossRef]
- Goriely, S.; Chamel, N.; Pearson, J.M. Further explorations of Skyrme-Hartree-Fock-Bogoliubov mass formulas. XIII. The 2012 atomic mass evaluation and the symmetry coefficient. Phys. Rev. C 2013, 88, 024308. [Google Scholar] [CrossRef]
- Pearson, J.M.; Chamel, N.; Potekhin, A.Y.; Fantina, A.F.; Ducoin, C.; Dutta, A.K.; Goriely, S. Unified equations of state for cold non-accreting neutron stars with Brussels-Montreal functionals-I. Role of symmetry energy. Mon. Not. R. Astron. Soc. 2018, 481, 2994–3026. [Google Scholar] [CrossRef]
- Shelley, M.; Pastore, A. Comparison between the Thomas-Fermi and Hartree-Fock-Bogoliubov Methods in the Inner Crust of a Neutron Star: The Role of Pairing Correlations. Universe 2020, 6, 206. [Google Scholar] [CrossRef]
- Pearson, J.M.; Chamel, N.; Potekhin, A.Y. Unified equations of state for cold nonaccreting neutron stars with Brussels-Montreal functionals. II. Pasta phases in semiclassical approximation. Phys. Rev. C 2020, 101, 015802. [Google Scholar] [CrossRef] [Green Version]
- Mutafchieva, Y.D.; Chamel, N.; Stoyanov, Z.K.; Pearson, J.M.; Mihailov, L.M. Role of Landau-Rabi quantization of electron motion on the crust of magnetars within the nuclear energy density functional theory. Phys. Rev. C 2019, 99, 055805. [Google Scholar] [CrossRef] [Green Version]
- Perot, L.; Chamel, N.; Sourie, A. Role of the symmetry energy and the neutron-matter stiffness on the tidal deformability of a neutron star with unified equations of state. Phys. Rev. C 2019, 100, 035801. [Google Scholar] [CrossRef] [Green Version]
- Perot, L.; Chamel, N. Role of dense matter in tidal deformations of inspiralling neutron stars and in gravitational waveforms with unified equations of state. Phys. Rev. C 2021, 103, 025801. [Google Scholar] [CrossRef]
- Sedrakian, A.; Clark, J.W. Superfluidity in nuclear systems and neutron stars. Eur. Phys. J. A 2019, 55, 167. [Google Scholar] [CrossRef] [Green Version]
- Yasui, S.; Inotani, D.; Nitta, M. Coexistence phase of 1S0 and 3P2 superfluids in neutron stars. Phys. Rev. C 2020, 101, 055806. [Google Scholar] [CrossRef]
- Blaizot, J.; Ribka, G. Quantum Theory of Finite Systems; MIT Press: Cambridge, MA, USA, 1986. [Google Scholar]
- Dobaczewski, J.; Flocard, H.; Treiner, J. Hartree-Fock-Bogolyubov description of nuclei near the neutron-drip line. Nucl. Phys. A 1984, 422, 103–139. [Google Scholar] [CrossRef]
- Bardeen, J.; Cooper, L.N.; Schrieffer, J.R. Theory of Superconductivity. Phys. Rev. 1957, 108, 1175–1204. [Google Scholar] [CrossRef] [Green Version]
- Alexandrov, A.S. Theory of Superconductivity: From Weak to Strong Coupling; CRC Press: Boca Raton, FL, USA, 2003. [Google Scholar]
- Chamel, N.; Pearson, J.M.; Fantina, A.F.; Ducoin, C.; Goriely, S.; Pastore, A. Brussels–Montreal Nuclear Energy Density Functionals, from Atomic Masses to Neutron Stars. Acta Phys. Pol. B 2015, 46, 349. [Google Scholar] [CrossRef]
- Goriely, S.; Chamel, N.; Pearson, J.M. Further explorations of Skyrme-Hartree-Fock-Bogoliubov mass formulas. XVI. Inclusion of self-energy effects in pairing. Phys. Rev. C 2016, 93, 034337. [Google Scholar] [CrossRef]
- Bertsch, G.F.; Esbensen, H. Pair correlations near the neutron drip line. Ann. Phys. 1991, 209, 327–363. [Google Scholar] [CrossRef]
- Dobaczewski, J.; Nazarewicz, A.A.; Werner, A.A. Closed shells at drip-line nuclei. Phys. Scr. Vol. 1995, 56, 15–22. [Google Scholar] [CrossRef] [Green Version]
- Chamel, N.; Goriely, S.; Pearson, J. Further explorations of Skyrme–Hartree–Fock–Bogoliubov mass formulas. IX: Constraint of pairing force to 1S0 neutron-matter gap. Nucl. Phys. A 2008, 812, 72–98. [Google Scholar] [CrossRef] [Green Version]
- Goriely, S.; Chamel, N.; Pearson, J.M. Skyrme-Hartree-Fock-Bogoliubov Nuclear Mass Formulas: Crossing the 0.6MeV Accuracy Threshold with Microscopically Deduced Pairing. Phys. Rev. Lett. 2009, 102, 152503. [Google Scholar] [CrossRef] [Green Version]
- Goriely, S.; Chamel, N.; Pearson, J.M. Recent breakthroughs in Skyrme-Hartree-Fock-Bogoliubov mass formulas. Eur. Phys. J. A 2009, 42, 547–552. [Google Scholar] [CrossRef]
- Chamel, N. Effective contact pairing forces from realistic calculations in infinite homogeneous nuclear matter. Phys. Rev. C 2010, 82, 014313. [Google Scholar] [CrossRef] [Green Version]
- Chamel, N.; Goriely, S.; Pearson, J.M. Further explorations of Skyrme-Hartree-Fock-Bogoliubov mass formulas. XI. Stabilizing neutron stars against a ferromagnetic collapse. Phys. Rev. C 2009, 80, 065804. [Google Scholar] [CrossRef] [Green Version]
- Goriely, S.; Chamel, N.; Pearson, J.M. Further explorations of Skyrme-Hartree-Fock-Bogoliubov mass formulas. XII. Stiffness and stability of neutron-star matter. Phys. Rev. C 2010, 82, 035804. [Google Scholar] [CrossRef] [Green Version]
- Goriely, S. Further explorations of Skyrme-Hartree-Fock-Bogoliubov mass formulas. XV: The spin-orbit coupling. Nucl. Phys. A 2015, 933, 68–81. [Google Scholar] [CrossRef]
- Cao, L.G.; Lombardo, U.; Shen, C.W.; Giai, N.V. From Brueckner approach to Skyrme-type energy density functional. Phys. Rev. C 2006, 73, 014313. [Google Scholar] [CrossRef]
- Gulminelli, F.; Fantina, A.F. The Equation of State of Neutron Stars and the Role of Nuclear Experiments. Nucl. Phys. News 2021, 31, 9–13. [Google Scholar] [CrossRef]
- Dinh Thi, H.; Carreau, T.; Fantina, A.F.; Gulminelli, F. Uncertainties in the pasta-phase properties of catalysed neutron stars. Astron. Astrophys. 2021, 654, A114. [Google Scholar] [CrossRef]
- Abbott, B.P.; LIGO Scientific Collaboration; Virgo Collaboration. GW170817: Measurements of Neutron Star Radii and Equation of State. Phys. Rev. Lett. 2018, 121, 161101. [Google Scholar] [CrossRef] [Green Version]
- Riley, T.E.; Watts, A.L.; Bogdanov, S.; Ray, P.S.; Ludlam, R.M.; Guillot, S.; Arzoumanian, Z.; Baker, C.L.; Bilous, A.V.; Chakrabarty, D.; et al. A NICER View of PSR J0030+0451: Millisecond Pulsar Parameter Estimation. Astrophys. J. Lett. 2019, 887, L21. [Google Scholar] [CrossRef] [Green Version]
- Miller, M.C.; Lamb, F.K.; Dittmann, A.J.; Bogdanov, S.; Arzoumanian, Z.; Gendreau, K.C.; Guillot, S.; Harding, A.K.; Ho, W.C.G.; Lattimer, J.M.; et al. PSR J0030+0451 Mass and Radius from NICER Data and Implications for the Properties of Neutron Star Matter. Astrophys. J. Lett. 2019, 887, L24. [Google Scholar] [CrossRef] [Green Version]
- Riley, T.E.; Watts, A.L.; Ray, P.S.; Bogdanov, S.; Guillot, S.; Morsink, S.M.; Bilous, A.V.; Arzoumanian, Z.; Choudhury, D.; Deneva, J.S.; et al. A NICER View of the Massive Pulsar PSR J0740+6620 Informed by Radio Timing and XMM-Newton Spectroscopy. Astrophys. J. Lett. 2021, 918, L27. [Google Scholar] [CrossRef]
- Miller, M.C.; Lamb, F.K.; Dittmann, A.J.; Bogdanov, S.; Arzoumanian, Z.; Gendreau, K.C.; Guillot, S.; Ho, W.C.G.; Lattimer, J.M.; Loewenstein, M.; et al. The Radius of PSR J0740+6620 from NICER and XMM-Newton Data. Astrophys. J. Lett. 2021, 918, L28. [Google Scholar] [CrossRef]
- Baldo, M.; Burgio, G.F. Properties of the nuclear medium. Rep. Prog. Phys. 2012, 75, 026301. [Google Scholar] [CrossRef]
- Lombardo, U.; Schulze, H.J.; Zuo, W. Induced Pairing Interaction in Neutron Star Matter. In Fifty Years of Nuclear BCS: Pairing in Finite Systems; World Scientific Publishing Co. Pte. Ltd.: Singapore, 2013; pp. 338–347. [Google Scholar] [CrossRef]
- Guo, W.; Dong, J.M.; Shang, X.; Zhang, H.F.; Zuo, W.; Colonna, M.; Lombardo, U. Proton-proton 1S0 pairing in neutron stars. Nucl. Phys. A 2019, 986, 18–25. [Google Scholar] [CrossRef]
- Zuo, W.; Li, Z.; Lu, G.; Li, J.; Scheid, W.; Lombardo, U.; Schulze, H.J.; Shen, C. 1S0 proton and neutron superfluidity in beta-stable neutron star matter. Phys. Lett. B 2004, 595, 44–49. [Google Scholar] [CrossRef] [Green Version]
- Zhou, X.R.; Schulze, H.J.; Zhao, E.G.; Pan, F.; Draayer, J.P. Pairing gaps in neutron stars. Phys. Rev. C 2004, 70, 048802. [Google Scholar] [CrossRef] [Green Version]
- Levenfish, K.P.; Yakovlev, D.G. Specific heat of neutron star cores with superfluid nucleons. Astron. Rep. 1994, 38, 247–251. [Google Scholar]
- Bardeen, J. Critical Fields and Currents in Superconductors. Rev. Mod. Phys. 1962, 34, 667–681. [Google Scholar] [CrossRef]
- Gnedin, O.Y.; Yakovlev, D.G. Thermal conductivity of electrons and muons in neutron star cores. Nucl. Phys. A 1995, 582, 697–716. [Google Scholar] [CrossRef]
- Mendell, G. Superfluid Hydrodynamics in Rotating Neutron Stars. I. Nondissipative Equations. Astrophys. J. 1991, 380, 515. [Google Scholar] [CrossRef]







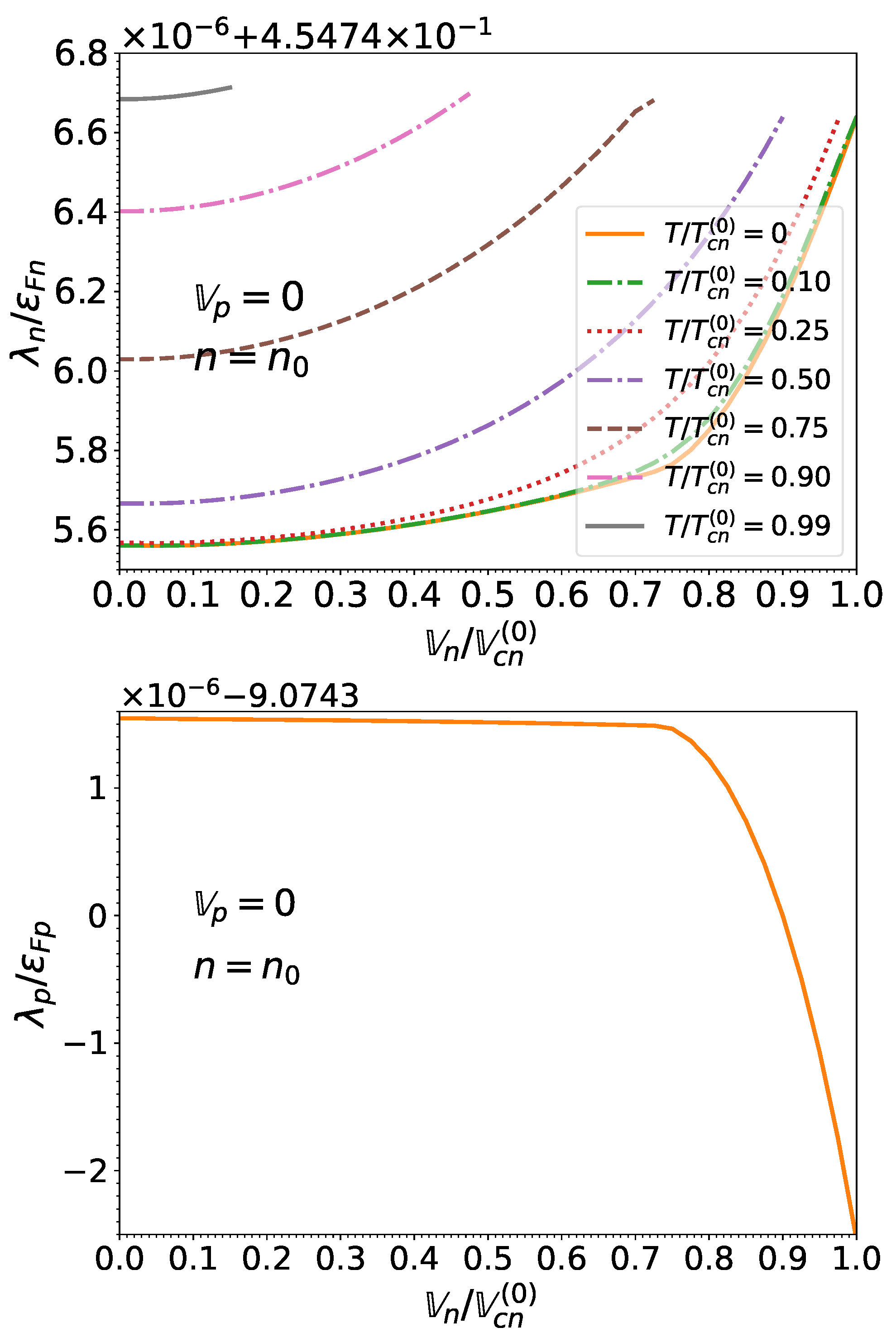
| −3970.29 | |
| 395.766 | |
| 22648.6 | |
| −100.000 | |
| −150.000 | |
| 0.894371 | |
| 0.0563535 | |
| −0.138961 × | |
| 1.05119 | |
| 2.00000 | |
| −11.0000 | |
| 1/12 | |
| 1/2 | |
| 1/12 | |
| 16.0 | |
| 1 |
| 133.779 | 0.943146 | 1.52786 | 2.11577 | 1.51 | |
| 14.9003 | 1.18847 | 1.51854 | 0.639489 | 1.52 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Allard, V.; Chamel, N. 1S0 Pairing Gaps, Chemical Potentials and Entrainment Matrix in Superfluid Neutron-Star Cores for the Brussels–Montreal Functionals. Universe 2021, 7, 470. https://doi.org/10.3390/universe7120470
Allard V, Chamel N. 1S0 Pairing Gaps, Chemical Potentials and Entrainment Matrix in Superfluid Neutron-Star Cores for the Brussels–Montreal Functionals. Universe. 2021; 7(12):470. https://doi.org/10.3390/universe7120470
Chicago/Turabian StyleAllard, Valentin, and Nicolas Chamel. 2021. "1S0 Pairing Gaps, Chemical Potentials and Entrainment Matrix in Superfluid Neutron-Star Cores for the Brussels–Montreal Functionals" Universe 7, no. 12: 470. https://doi.org/10.3390/universe7120470
APA StyleAllard, V., & Chamel, N. (2021). 1S0 Pairing Gaps, Chemical Potentials and Entrainment Matrix in Superfluid Neutron-Star Cores for the Brussels–Montreal Functionals. Universe, 7(12), 470. https://doi.org/10.3390/universe7120470







