A Superfluid Perspective on Neutron Star Dynamics
Abstract
1. Neutron Star Superfluidity
2. The Essence of the Two-Fluid Model
2.1. The Equations of Motion
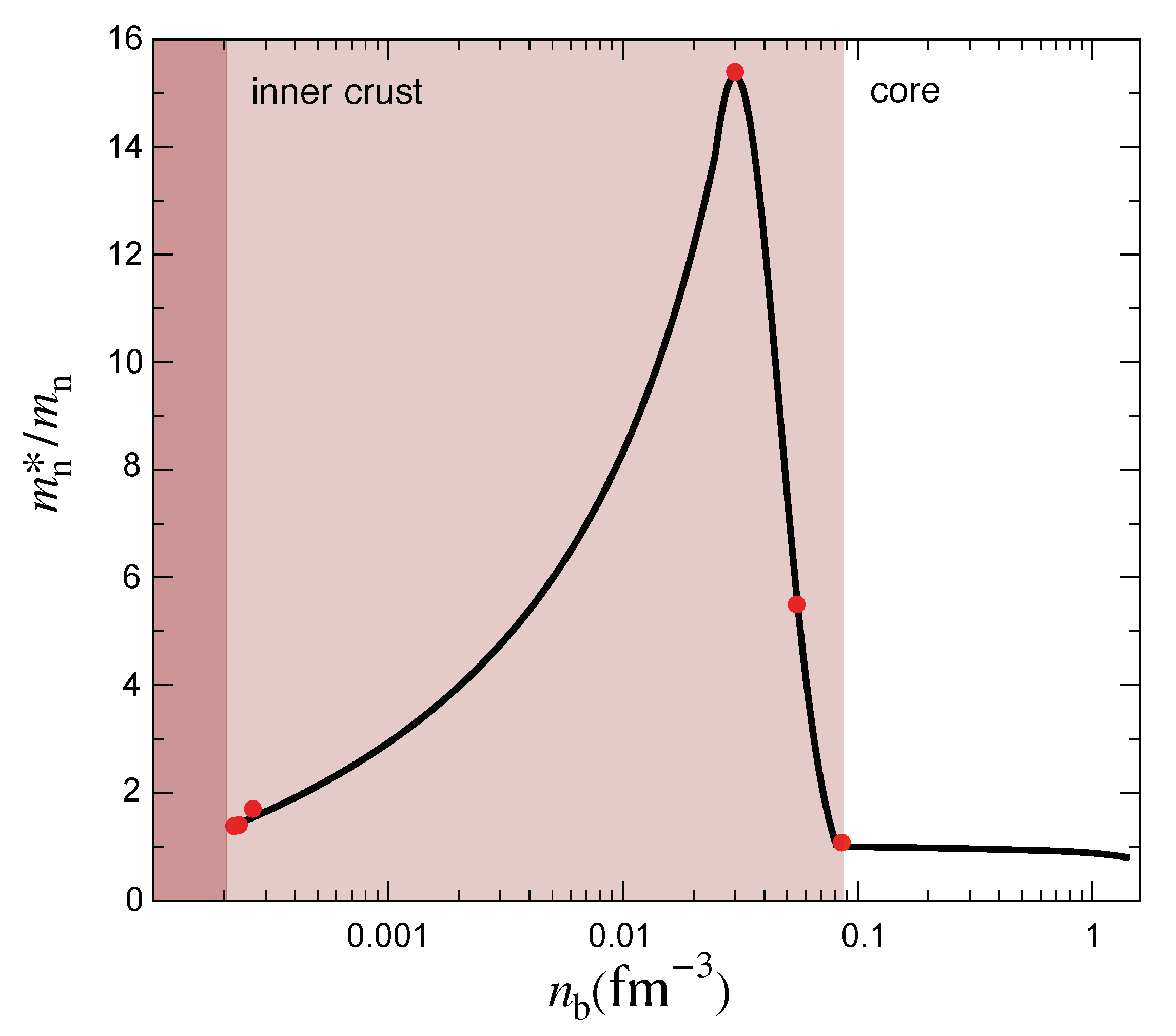
2.2. The Crust and the Chemical Gauge
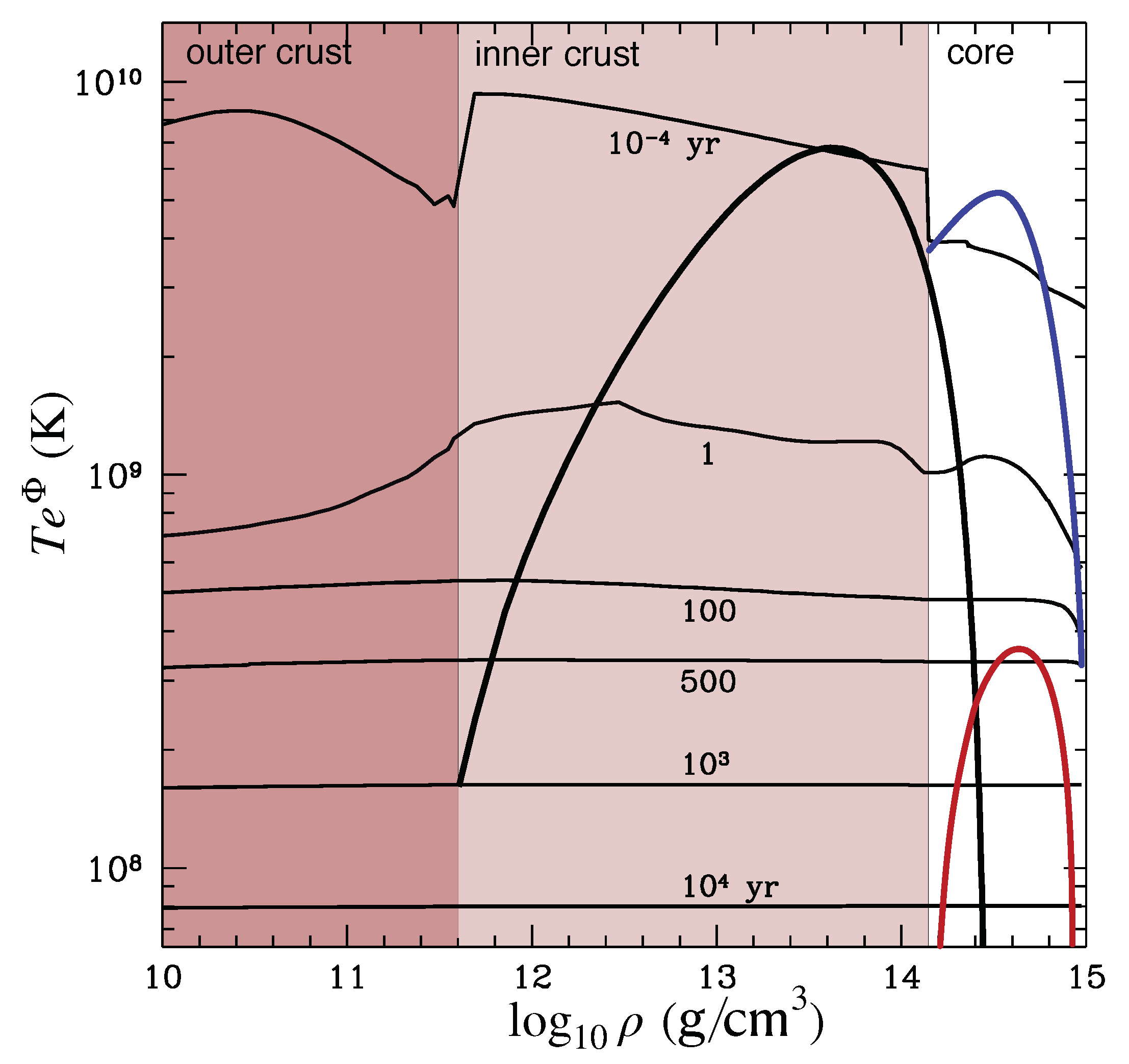
2.3. Thermal Excitations
3. Vortex Dynamics
3.1. Mutual Friction
3.2. Pulsar Glitches
3.3. Superfluid Turbulence
4. Oscillations and Instabilities
Decoupling the Degrees of Freedom
5. Final Remarks
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Burrows, A.; Lattimer, J.M. The birth of neutron stars. Ap. J. 1986, 307, 178. [Google Scholar] [CrossRef]
- Page, D.; Lattimer, J.M.; Prakash, M.; Steiner, A.W. Minimal cooling of neutron stars: A new paradigm. Ap. J. Suppl. 2004, 155, 623. [Google Scholar] [CrossRef]
- Chamel, N.; Haensel, P. Physics of neutron star crusts. Liv. Rev. Relativ. 2008, 11, 10. [Google Scholar] [CrossRef] [PubMed]
- Glendenning, N.K. Nuclear Physics, Particle Physics, and General Relativity. In Compact Stars; Springer: Berlin/Heidelberg, Germany, 1996. [Google Scholar]
- Alford, M.G.; Schmitt, A.; Rajagopal, K.; Schäfer, T. Color superconductivity in dense quark matter. Rev. Mod. Phys. 2008, 80, 1455. [Google Scholar] [CrossRef]
- Ho, W.C.G.; Glampedakis, K.; Andersson, N. Magnetars: Super (ficially) hot and super (fluid) cool. MNRAS 2012, 422, 2632. [Google Scholar] [CrossRef]
- Deibel, A.; Cumming, A.; Brown, E.F.; Reddy, S. Late-time Cooling of Neutron Star Transients and the Physics of the Inner Crust. Ap. J. 2017, 839, 95. [Google Scholar] [CrossRef]
- Page, D.; Prakash, M.; Lattimer, J.M.; Steiner, A.W. Rapid Cooling of the Neutron Star in Cassiopeia A Triggered by Neutron Superfluidity in Dense Matter. Phys. Rev. Lett. 2011, 106, 081101. [Google Scholar] [CrossRef]
- Shternin, P.S.; Yakovlev, D.G.; Heinke, C.O.; Ho, W.C.G.; Patnaude, D.J. Cooling neutron star in the Cassiopeia A supernova remnant: Evidence for superfluidity in the core. MNRAS 2011, 412, L108. [Google Scholar] [CrossRef]
- Baym, G.; Pethick, C.; Pines, D.; Ruderman, M. Spin Up in Neutron Stars: The Future of the Vela Pulsar. Nature 1969, 224, 872. [Google Scholar] [CrossRef]
- Anderson, P.W.; Itoh, N. Pulsar glitches and restlessness as a hard superfluidity phenomenon. Nature 1975, 256, 27. [Google Scholar] [CrossRef]
- Espinoza, C.M.; Lyne, A.G.; Stappers, B.W.; Kramer, M. A study of 315 glitches in the rotation of 102 pulsars. MNRAS 2011, 414, 1679. [Google Scholar] [CrossRef]
- Migdal, A.B. Superfluidity and the moments of inertia of nuclei. Nucl. Phys. 1959, 13, 655. [Google Scholar] [CrossRef]
- Lombardo, U.; Schulze, H.J. Physics of Neutron Star Interiors; Blaschke, D., Glendenning, N.K., Sedrakian, A., Eds.; Lecture Notes in Physics; Springer: Berlin/Heidelberg, Germany, 2001; Volume 578, p. 30. [Google Scholar]
- Haskell, B.; Sedrakian, A. Superfluidity and Superconductivity in Neutron Stars. Astrophys. Space Sci. Libr. 2018, 457, 401. [Google Scholar]
- Sedrakian, A.; Clark, J.W. Superfluidity in nuclear systems and neutron stars. Eur. Phys. J. A 2019, 55, 167. [Google Scholar] [CrossRef]
- Chen, J.M.C.; Clark, J.W.; Davé, R.D.; Khodel, V.V. Pairing gaps in nucleonic superfluids. Nucl. Phys. A 1993, 555, 59. [Google Scholar] [CrossRef]
- Gusakov, M.E.; Kantor, E.M. Velocity-dependent energy gaps and dynamics of superfluid neutron stars. MNRAS 2013, 428, L26. [Google Scholar] [CrossRef]
- Alpar, M.A. Pinning and Threading of Quantized Vortices in the Pulsar Crust Superfluid. Ap. J. 1977, 213, 527. [Google Scholar] [CrossRef]
- Epstein, R.E.; Baym, G. Vortex Pinning in Neutron Stars. Ap. J. 1988, 328, 680. [Google Scholar] [CrossRef]
- Donati, P.; Pizzochero, P.M. Is there nuclear pinning of vortices in superfluid pulsars? Phys. Rev. Lett. 2003, 90, 211101. [Google Scholar] [CrossRef]
- Donati, P.; Pizzochero, P.M. Realistic energies for vortex pinning in intermediate-density neutron star matter. Phys. Lett. B 2006, 640, 74. [Google Scholar] [CrossRef]
- Avogadro, P.; Barranco, F.; Broglia, R.A.; Vigezzi, E. Vortex nucleus interaction in the inner crust of neutron stars. Nucl. Phys. A 2008, 811, 378. [Google Scholar] [CrossRef]
- Barranco, F.; Broglia, R.A.; Vigezzi, E. Quantum size effects in the inner crust of neutron stars. J. Phys. G 2010, 37, 064023. [Google Scholar] [CrossRef]
- Seveso, S.; Pizzochero, P.M.; Grill, F.; Haskell, B. Mesoscopic pinning forces in neutron star crusts. MNRAS 2016, 455, 3952. [Google Scholar] [CrossRef]
- Wlazlowski, G.; Sekizawa, K.; Magierski, P.; Bulgac, A.; McNeil, M.F. Vortex pinning and dynamics in the neutron star crust. Phys. Rev. Lett. 2016, 117, 232701. [Google Scholar] [CrossRef] [PubMed]
- Andreev, A.; Bashkin, E. Three-velocity hydrodynamics of superfluid solutions. Sov. Phys. JETP 1975, 42, 164. [Google Scholar]
- Alpar, M.A.; Langer, S.A.; Sauls, J.A. Rapid postglitch spin-up of the superfluid core in pulsars. Ap. J. 1984, 282, 533. [Google Scholar] [CrossRef]
- Borumand, M.; Joynt, R.; Kluzniak, W. Superfluid densities in neutron-star matter. Phys. Rev. C 1996, 54, 2745. [Google Scholar] [CrossRef]
- Comer, G.L.; Joynt, R. Relativistic mean field model for entrainment in general relativistic superfluid neutron stars. Phys. Rev. D 2003, 68, 023002. [Google Scholar] [CrossRef]
- Chamel, N.; Allard, V. Entrainment effects in neutron-proton mixtures within the nuclear energy-density functional theory: Low-temperature limit. Phys. Rev. C 2019, 100, 065801. [Google Scholar] [CrossRef]
- Landau, L.; Lifshitz, E. Fluid Mechanics; Pergamon Press: London, UK, 1959. [Google Scholar]
- Khalatnikov, I.M. An Introduction to the Theory of Superfluidity; W. A. Benjamin: New York, NY, USA, 1965. [Google Scholar]
- Wilks, J. Liquid and Solid Helium; Clarendon Press: Oxford, UK, 1967. [Google Scholar]
- Putterman, S.J. Superfluid Hydrodynamics; North-Holland Publishing: Amsterdam, The Netherlands; Elsevier: New York, NY, USA, 1974. [Google Scholar]
- Graber, V.; Andersson, B.; Hogg, M. Neutron stars in the laboratory. Int. J. Mod. Phys. D 2017, 26, 1730015–1730347. [Google Scholar] [CrossRef]
- Andersson, N.; Comer, G.L. Relativistic fluid dynamics: Physics for many different scales. Liv. Rev. Relativ. 2007, 10, 1. [Google Scholar]
- Mendell, G. Superfluid Hydrodynamics in Rotating Neutron Stars. I. Nondissipative Equations. Ap. J. 1991, 380, 515. [Google Scholar] [CrossRef]
- Mendell, G. Superfluid Hydrodynamics in Rotating Neutron Stars. II. Dissipative Effects. Ap. J. 1991, 380, 530. [Google Scholar] [CrossRef]
- Andersson, N.; Sidery, T.; Comer, G.L. Mutual friction in superfluid neutron stars. MNRAS 2006, 368, 162. [Google Scholar]
- Lindblom, L.; Mendell, G. Does Gravitational Radiation Limit the Angular Velocities of Superfluid Neutron Stars? Ap. J. 1995, 444, 804. [Google Scholar] [CrossRef]
- Prix, R. Onset of the nonlinear regime in unified dark matter models. Phys. Rev. D 2004, 69, 043001. [Google Scholar]
- Carter, B.; Chamel, N. Covariant Analysis of Newtonian Multi-Fluid Models For Neutron Stars I: Milne–Cartan Struc-ture and Variational Formulation. Int. J. Mod. Phys. D 2004, 13, 291. [Google Scholar]
- Carter, B.; Chamel, N. Covariant Analysis of Newtonian Multi-Fluid Models For Neutron Stars II: Stress–Energy Ten-sors and Virial Theorems. Int. J. Mod. Phys. D 2005, 14, 717. [Google Scholar]
- Carter, B.; Chamel, N. Covariant analysis of Newtonian multi-fluid models for neutron stars III: Transvective, viscous, and superfluid drag dissipation. Int. J. Mod. Phys. D 2005, 14, 749. [Google Scholar]
- Kobyakov, D.N.; Pethick, C.J. Two-component superfluid hydrodynamics of neutron star cores. Ap. J. 2017, 836, 203. [Google Scholar]
- Andersson, N.; Comer, G.L. A flux-conservative formalism for convective and dissipative multi-fluid systems, with application to Newtonian superfluid neutron stars. Class. Quantum Grav. 2006, 23, 5505. [Google Scholar] [CrossRef]
- Carter, B.; Chamel, N.; Haensel, P. Entrainment coefficient and effective mass for conduction neutrons in neutron star crust: II Macroscopic treatment. Int. J. Mod. Phys. D 2006, 15, 777. [Google Scholar] [CrossRef]
- Carter, B.; Samuelsson, L. Relativistic mechanics of neutron superfluid in (magneto) elastic star crust. Class. Quantum Grav. 2006, 23, 5367. [Google Scholar] [CrossRef]
- Chamel, N. Band structure effects for dripped neutrons in neutron star crust. Nucl. Phys. A 2005, 747, 109. [Google Scholar] [CrossRef]
- Carter, B.; Chamel, N.; Haensel, P. Entrainment coefficient and effective mass for conduction neutrons in neutron star crust: Simple microscopic models. Nucl. Phys. A 2005, 748, 675. [Google Scholar] [CrossRef]
- Chamel, N. Effective mass of free neutrons in neutron star crust. Nucl. Phys. A 2006, 773, 263. [Google Scholar] [CrossRef]
- Carter, B.; Chachoua, E.; Chamel, N. Covariant Newtonian and relativistic dynamics of (magneto)-elastic solid model for neutron star crust. Gen. Rel. Grav. 2006, 38, 83. [Google Scholar] [CrossRef]
- Pethick, C.J.; Chamel, N.; Reddy, S. Superfluid dynamics in neutron star crusts. Prog. Theor. Phys. Suppl. 2010, 186, 9. [Google Scholar] [CrossRef]
- Andersson, N.; Haskell, B.; Samuelsson, L. Lagrangian perturbation theory for a superfluid immersed in an elastic neutron star crust. MNRAS 2011, 416, 118. [Google Scholar] [CrossRef]
- Kobyakov, D.; Pethick, C.J. Dynamics of the inner crust of neutron stars: Hydrodynamics, elasticity, and collective modes. Phys. Rev. 2013, 87, 055803. [Google Scholar] [CrossRef]
- Chamel, N.; Low, J. Entrainment in Superfluid Neutron-Star Crusts: Hydrodynamic Description and Microscopic Origin. Temp. Phys. 2017, 189, 328. [Google Scholar] [CrossRef]
- Andersson, N.; Haskell, B.; Comer, G.L.; Samuelsson, L. A variational approach to relativistic superfluid vortex elasticity. Class. Quantum Grav. 2019, 36, 105004. [Google Scholar] [CrossRef]
- Chamel, N. Neutron conduction in the inner crust of a neutron star in the framework of the band theory of solids. Phys. Rev. C 2012, 85, 035801. [Google Scholar] [CrossRef]
- Noël, M.; Urban, M. Superfluid hydrodynamics in the inner crust of neutron stars. Phys. Rev. C 2016, 94, 065801. [Google Scholar]
- Delsate, T.; Chamel, N.; Gürlebeck, N.; Fantina, A.F.; Pearson, J.M.; Ducoin, C. Giant pulsar glitches and the inertia of neutron star crusts. Phys. Rev. D 2016, 94, 023008. [Google Scholar] [CrossRef]
- Watanabe, G.; Pethick, C.J. Superfluid density of neutrons in the inner crust of neutron stars: New life for pulsar glitch models. Phys. Rev. Lett. 2017, 119, 062701. [Google Scholar] [CrossRef]
- Sauls, J.A.; Chamel, N.; Alpar, M.A. Superfluidity in Disordered Neutron Stars Crusts. arXiv 2020, arXiv:2001.09959. [Google Scholar]
- Andersson, N.; Comer, G.L. Entropy entrainment and dissipation in superfluid Helium. Int. J. Mod. Phys. D 2011, 20, 1215. [Google Scholar] [CrossRef]
- Gusakov, M.E.; Andersson, N. Temperature-dependent pulsations of superfluid neutron stars. MNRAS 2006, 372, 1776. [Google Scholar] [CrossRef]
- Kantor, E.M.; Gusakov, M.E. Temperature effects in pulsating superfluid neutron stars. Phys. Rev. D 2011, 83, 103008. [Google Scholar] [CrossRef]
- Chugunov, A.I.; Gusakov, M.E. Non-radial superfluid modes in oscillating neutron stars. MNRAS 2011, 418, L54. [Google Scholar] [CrossRef][Green Version]
- Gusakov, M.E.; Haensel, P. The entrainment matrix of a superfluid neutron–proton mixture at a finite temperature. Nucl. Phys. A 2005, 761, 333. [Google Scholar] [CrossRef][Green Version]
- Leinson, L.B. The entrainment matrix of a superfluid nucleon mixture at finite temperatures. MNRAS 2018, 479, 3778–3790. [Google Scholar] [CrossRef]
- Andersson, N.; Krüger, C.; Comer, G.L.; Samuelsson, L. A minimal model for finite temperature superfluid dynamics. Class. Quantum Grav. 2013, 30, 235025. [Google Scholar] [CrossRef][Green Version]
- Kantor, E.M.; Gusakov, M.E. Temperature-dependent r modes in superfluid neutron stars stratified by muons. MNRAS 2017, 469, 3928. [Google Scholar] [CrossRef][Green Version]
- Kantor, E.M.; Dommes, V.A.; Gusakov, M.E. r-modes in stratified neutron stars with entrainment. J. Phys. Conf. 2019, 1400, 022007. [Google Scholar] [CrossRef]
- Kantor, E.M.; Gusakov, M.E.; Dommes, V.A. Constraining Neutron Superfluidity with R-Mode Physics. Phys. Rev. Lett. 2020, 125, 151101. [Google Scholar] [CrossRef]
- Hall, H.E.; Vinen, W.F. The rotation of liquid helium II II. The theory of mutual friction in uniformly rotating helium II. Proc. Roy. Soc. Lond. A 1956, 238, 215. [Google Scholar]
- Jones, P.B. Rotation of the neutron-drip superfluid in pulsars: The Kelvin phonon contribution to dissipation. MNRAS 1992, 257, 501. [Google Scholar] [CrossRef]
- Epstein, R.I.; Baym, G. Vortex drag and the spin-up time scale for pulsar glitches. Ap. J. 1992, 387, 276. [Google Scholar] [CrossRef]
- Graber, V.; Cummings, A.; Andersson, N. Glitch rises as a test for rapid superfluid coupling in neutron stars. Ap. J. 2018, 865, 23. [Google Scholar] [CrossRef]
- Antonelli, M.; Haskell, B. Superfluid vortex-mediated mutual friction in non-homogeneous neutron star interiors. MNRAS 2020, 499, 3690. [Google Scholar] [CrossRef]
- Haskell, B.; Melatos, A. Models of pulsar glitches. Int. J. Mod. Phys. D 2015, 24, 1530008. [Google Scholar] [CrossRef]
- Sidery, T.; Passamonti, A.; Andersson, N. The dynamics of pulsar glitches: Contrasting phenomenology with numerical evolutions. MNRAS 2010, 405, 1061. [Google Scholar] [CrossRef]
- Warszawski, L.; Melatos, A. Gross–Pitaevskii model of pulsar glitches. MNRAS 2011, 415, 1611. [Google Scholar] [CrossRef]
- Warszawski, L.; Melatos, A.; Berloff, N.G. Unpinning triggers for superfluid vortex avalanches. Phys. Rev. B 2012, 85, 104503. [Google Scholar] [CrossRef]
- Haskell, B.; Pizzochero, P.M.; Sidery, T. Modelling pulsar glitches with realistic pinning forces: A hydrodynamical approach. MNRAS 2012, 420, 658. [Google Scholar] [CrossRef]
- Newton, W.G.; Berger, S.; Haskell, B. Observational constraints on neutron star crust–core coupling during glitches. MNRAS 2015, 454, 4400. [Google Scholar] [CrossRef]
- Khomenko, V.; Haskell, B. Core and Crust Contributions in Pulsar Glitches: Constraints from the Slow Rise of the Largest Glitch Observed in the Crab Pulsar. PASA 2018, 35, e02015. [Google Scholar]
- Haskell, B.; Khomenko, V.; Antonelli, M.; Antonopoulou, D. Crust or core? Insights from the slow rise of large glitches in the Crab pulsar. MNRAS 2018, 481, L146. [Google Scholar] [CrossRef]
- Link, B.; Epstein, R.I.; Lattimer, J.M. Pulsar Constraints on Neutron Star Structure and Equation of State. Phys. Rev. Lett. 1999, 83, 3362. [Google Scholar] [CrossRef]
- Ravenhall, D.G.; Pethick, C.J. Neutron star moments of inertia. Ap. J. 1994, 424, 846. [Google Scholar] [CrossRef]
- Andersson, N.; Glampedakis, K.; Ho, W.C.G.; Espinoza, C.M. Pulsar Glitches: The Crust is not Enough. Phys. Rev. Lett. 2012, 109, 241103. [Google Scholar] [CrossRef] [PubMed]
- Chamel, N. Crustal Entrainment and Pulsar Glitches. Phys. Rev. Lett. 2013, 110, 011101. [Google Scholar] [CrossRef] [PubMed]
- Antonopoulo, D.; Espinoza, C.M.; Kuiper, L.; Andersson, N. Pulsar spin-down: The glitch-dominated rotation of PSR J0537–6910. MNRAS 2018, 473, 1644. [Google Scholar] [CrossRef]
- Ferdman, R.D.; Archibald, R.F.; Gourgouliatos, K.N.; Kaspi, V.M. The Glitches and Rotational History of the Highly Energetic Young Pulsar PSR J0537–6910. Ap. J. 2018, 852, 123. [Google Scholar] [CrossRef]
- Piekarewicz, J.; Fattoyev, F.J.; Horowitz, C.J. Pulsar glitches: The crust may be enough. Phys. Rev. C 2014, 90, 015803. [Google Scholar] [CrossRef]
- Ho, W.C.G.; Espinoza, C.M.; Antonopoulou, D.; Andersson, N. Pinning down the superfluid and measuring masses using pulsar glitches. Sci. Adv. 2015, 1, e1500578. [Google Scholar] [CrossRef]
- Pizzochero, P.M.; Antonelli, M.; Haskell, B.; Seveso, S. Constraints on pulsar masses from the maximum observed glitch. Nat. Astron. 2017, 1, 0134. [Google Scholar] [CrossRef]
- Montoli, A.; Antonelli, M.; Pizzochero, P.M. Bayesian estimate of the superfluid moments of inertia from the 2016 glitch in the Vela pulsar. MNRAS 2020, 92, 4837. [Google Scholar] [CrossRef]
- Dodson, R.G.; McCulloch, P.M.; Lewis, D.R. High time resolution observations of the January 2000 glitch in the Vela pulsar. Ap. J. Lett. 2002, 564, L85. [Google Scholar] [CrossRef]
- Palfreyman, J.; Dickey, J.M.; Hotan, A.; Ellingsen, S.; van Straten, W. Alteration of the magnetosphere of the Vela pulsar during a glitch. Nature 2018, 556, 219. [Google Scholar] [CrossRef] [PubMed]
- Ashton, G.; Lasky, P.D.; Graber, V.; Palfreyman, J. Rotational evolution of the Vela pulsar during the 2016 glitch. Nat. Astron. 2019, 417, 1143. [Google Scholar] [CrossRef]
- Packard, R.E. Pulsar speedups related to metastability of the superfluid neutron-star core. Phys. Rev. Lett. 1972, 28, 1080. [Google Scholar] [CrossRef]
- Chevalier, E. Vortex Entanglement in Neutron Stars. Europhys. Lett. 1995, 29, 181. [Google Scholar] [CrossRef]
- Andersson, N.; Sidery, T.; Comer, G.L. Superfluid neutron star turbulence. MNRAS 2007, 381, 747. [Google Scholar] [CrossRef]
- Glaberson, W.I.; Johnson, W.W.; Ostermeier, R.M. Instability of a Vortex Array in He II. Phys. Rev. Lett. 1974, 33, 1197. [Google Scholar] [CrossRef]
- Gorter, C.J.; Mellink, J.H. On the irreversible processes in liquid helium II. Physica 1949, 15, 285. [Google Scholar] [CrossRef]
- Peralta, C.; Melatos, A.; Giacobello, M.; Ooi, A. Global three-dimensional flow of a neutron superfluid in a spherical shell in a neutron star. Ap. J. 2005, 635, 1224. [Google Scholar] [CrossRef]
- Peralta, C.; Melatos, A.; Giacobello, M.; Ooi, A. Transitions between turbulent and laminar superfluid vorticity states in the outer core of a neutron star. Ap. J. 2006, 651, 1079. [Google Scholar] [CrossRef]
- Sidery, T.L.; Andersson, N.; Comer, G.L. Waves and instabilities in dissipative rotating superfluid neutron stars. MNRAS 2008, 385, 335. [Google Scholar] [CrossRef][Green Version]
- Glampedakis, K.; Andersson, N.; Jones, D.I. Stability of precessing superfluid neutron stars. Phys. Rev. Lett. 2008, 100, 081101. [Google Scholar] [CrossRef] [PubMed]
- Glampedakis, K.; Andersson, N.; Jones, D.I. Do superfluid instabilities prevent neutron star precession? MNRAS 2009, 394, 1908. [Google Scholar] [CrossRef][Green Version]
- Glampedakis, K.; Andersson, N. Hydrodynamical trigger mechanism for pulsar glitches. Phys. Rev. Lett. 2009, 102, 141101. [Google Scholar] [CrossRef] [PubMed]
- Link, B. Instability of superfluid flow in the neutron star inner crust. MNRAS 2012, 422, 1640. [Google Scholar] [CrossRef]
- Drummond, L.V.; Melatos, A. Stability of interlinked neutron vortex and proton flux tube arrays in a neutron star: Equilibrium configurations. MNRAS 2017, 472, 4851. [Google Scholar] [CrossRef]
- van Eysden, C.A.; Link, B. Hydrodynamic Stability Analysis of the Neutron Star Core. Ap. J. 2018, 865, 60. [Google Scholar] [CrossRef]
- Haskell, B.; Antonopoulou, D.A.; Barenghi, C. Turbulent, pinned superfluids in neutron stars and pulsar glitch recoveries. MNRAS 2020, 499, 161. [Google Scholar] [CrossRef]
- Epstein, R.I. Acoustic properties of neutron stars. Ap. J. 1988, 333, 880. [Google Scholar] [CrossRef]
- Lindblom, L.; Mendell, G. The oscillations of superfluid neutron stars. Ap. J. 1994, 421, 689. [Google Scholar] [CrossRef]
- Lee, U. Nonradial oscillations of neutron stars with the superfluid core. Astron. Astrophys. 1995, 303, 586. [Google Scholar]
- Comer, G.L.; Langlois, D.; Lin, L.M. Quasinormal modes of general relativistic superfluid neutron stars. Phys. Rev. D 1999, 60, 104025. [Google Scholar] [CrossRef]
- Andersson, N.; Comer, G.L. On the dynamics of superfluid neutron star cores. MNRAS 2001, 328, 1129. [Google Scholar] [CrossRef]
- Prix, R.; Rieutord, M. Adiabatic oscillations of non-rotating superfluid neutron stars. Astron. Astrophys. 2002, 393, 949. [Google Scholar] [CrossRef]
- Andersson, N.; Comer, G.L.; Langlois, D. Oscillations of general relativistic superfluid neutron stars. Phys. Rev. D 2002, 66, 104002. [Google Scholar] [CrossRef]
- Lin, L.M.; Comer, G.L.; Andersson, N. Oscillations of general relativistic multifluid/multilayer compact stars. Phys, Rev. D 2008, 78, 083008. [Google Scholar] [CrossRef]
- Andersson, N.; Glampedakis, K.; Haskell, B. Oscillations of dissipative superfluid neutron stars. Phys. Rev. D 2009, 79, 103009. [Google Scholar] [CrossRef]
- Wong, K.S.; Lin, L.M.; Leung, P.T. Universality in oscillation modes of superfluid neutron stars? Ap. J. 2009, 699, 1809. [Google Scholar] [CrossRef]
- Passamonti, A.; Haskell, B.; Andersson, N. Oscillations of rapidly rotating superfluid stars. MNRAS 2009, 396, 951. [Google Scholar] [CrossRef][Green Version]
- Passamonti, A.; Andersson, N. Hydrodynamics of rapidly rotating superfluid neutron stars with mutual friction. MNRAS 2011, 413, 47. [Google Scholar] [CrossRef][Green Version]
- Andersson, N.; Comer, G.L. Probing neutron-star superfluidity with gravitational-wave data. Phys. Rev. Lett. 2001, 87, 241101. [Google Scholar] [CrossRef] [PubMed]
- Andersson, N. Gravitational waves from instabilities in relativistic stars. Class. Quantum Grav. 2003, 20, R105. [Google Scholar] [CrossRef]
- Lindblom, L.; Mendell, G. γ-modes in superfluid neutron stars. Phys. Rev. D 2000, 61, 104003. [Google Scholar] [CrossRef]
- Lee, U.; Yoshida, S. r-modes of neutron stars with superfluid cores. Ap. J. 2003, 586, 403. [Google Scholar] [CrossRef][Green Version]
- Yoshida, S.; Lee, U. r-modes in relativistic superfluid stars. Phys. Rev. D 2003, 67, 124019. [Google Scholar] [CrossRef]
- Kinney, J.B.; Mendell, G. r-modes in accreting neutron stars with magnetoviscous boundary layers. Phys. Rev. D 2003, 67, 024032. [Google Scholar] [CrossRef]
- Yoshida, S.; Lee, U. Non-radial oscillations of the magnetized rotating stars with purely toroidal magnetic fields. MNRAS 2003, 344, 207. [Google Scholar] [CrossRef][Green Version]
- Prix, R.; Comer, G.L.; Andersson, N. The superfluid two-stream instability. MNRAS 2004, 348, 625. [Google Scholar] [CrossRef]
- Andersson, N.; Comer, G.L.; Prix, R. Are pulsar glitches triggered by a superfluid two-stream instability? Phys. Rev. Lett. 2003, 90, 091101. [Google Scholar] [CrossRef]
- Andersson, N.; Comer, G.L.; Prix, R. The superfluid two-stream instability. MNRAS 2004, 354, 101. [Google Scholar] [CrossRef]
- Hawke, I.; Comer, G.L.; Andersson, N. The nonlinear development of the relativistic two-stream instability. Class. Quantum Grav. 2013, 30, 145007. [Google Scholar] [CrossRef][Green Version]
- Haber, A.; Schmitt, A.; Stetina, S. Instabilities in relativistic two-component (super) fluids. Phys. Rev. D 2016, 93, 025011. [Google Scholar] [CrossRef]
- Andersson, N.; Schmitt, A. Dissipation triggers dynamical two-stream instability. Particles 2019, 2, 28. [Google Scholar] [CrossRef]
- Andersson, N.; Glampedakis, K.; Hogg, M. Superfluid instability of r-modes in “differentially rotating” neutron stars. Phys. Rev. D 2013, 87, 063007. [Google Scholar] [CrossRef]
- Andersson, N.; Pnigouras, P. MNRAS The g-mode spectrum of reactive neutron star cores. MNRAS 2019, 489, 4043. [Google Scholar]
- Gusakov, M.E.; Kantor, E.M. Thermal g-modes and unexpected convection in superfluid neutron stars. Phys. Rev. D 2013, 88, 101302. [Google Scholar] [CrossRef]
- Passamonti, A.; Andersson, N.; Ho, W.C.G. Buoyancy and g-modes in young superfluid neutron stars. MNRAS 2016, 455, 1489. [Google Scholar] [CrossRef]
- Watts, A.L.; Andersson, N.; Chakrabarty, D.; Feroci, M.; Hebeler, K.; Israel, G.; Lamb, F.K.; Miller, M.C.; Morsink, S.; Özel, F.; et al. Colloquium: Measuring the neutron star equation of state using x-ray timing. Rev. Mod. Phys. 2016, 88, 021001. [Google Scholar]
- Andersson, N.; Glampedakis, K.; Samuelsson, L. Superfluid signatures in magnetar seismology. MNRAS 2009, 396, 894. [Google Scholar] [CrossRef]
- Gabler, M.; Cerdá-Durán, P.; Stergioulas, N.; Font, J.A.; Müller, E. Imprints of superfluidity on magnetoelastic quasiperiodic oscillations of soft gamma-ray repeaters. Phys. Rev. D 2011, 111, 211102. [Google Scholar] [CrossRef]
- Sotani, H.; Nakazato, K.; Iida, K.; Oyamatsu, K. Effect of superfluidity on neutron star oscillations. MNRAS 2013, 428, L21. [Google Scholar] [CrossRef]
- Passamonti, A.; Pons, J.A. Quasi-periodic oscillations in superfluid, relativistic magnetars with nuclear pasta phases. MNRAS 2016, 463, 1173. [Google Scholar] [CrossRef]
- Gabler, M.; Cerdá-Durán, P.; Stergioulas, N.; Font, J.A.; Müller, E. Coherent magneto-elastic oscillations in superfluid magnetars. MNRAS 2016, 460, 4242. [Google Scholar] [CrossRef][Green Version]
- Yu, H.; Weinberg, N.N. Resonant tidal excitation of superfluid neutron stars in coalescing binaries. MNRAS 2017, 464, 2622. [Google Scholar] [CrossRef]
- Yu, H.; Weinberg, N.N. Dynamical tides in coalescing superfluid neutron star binaries with hyperon cores and their detectability with third-generation gravitational-wave detectors. MNRAS 2017, 470, 350. [Google Scholar] [CrossRef]
- Aguilera, D.N.; Cirigliano, V.; Pons, J.A.; Reddy, S.; Sharma, R. Superfluid Heat Conduction and the Cooling of Magnetized Neutron Stars. Phys. Rev. Lett. 2009, 102, 091101. [Google Scholar] [CrossRef]
- Mendell, G. Magnetohydrodynamics in superconducting-superfluid neutron stars. MNRAS 1998, 296, 903. [Google Scholar] [CrossRef]
- Glampedakis, K.; Andersson, N.; Samuelsson, L. Magnetohydrodynamics of superfluid and superconducting neutron star cores. MNRAS 2011, 410, 805. [Google Scholar] [CrossRef]
- Gusakov, M.E.; Dommes, V.A. Relativistic dynamics of superfluid-superconducting mixtures in the presence of topological defects and an electromagnetic field with application to neutron stars. Phys. Rev. D 2016, 94, 083006. [Google Scholar] [CrossRef]


Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the author. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Andersson, N. A Superfluid Perspective on Neutron Star Dynamics. Universe 2021, 7, 17. https://doi.org/10.3390/universe7010017
Andersson N. A Superfluid Perspective on Neutron Star Dynamics. Universe. 2021; 7(1):17. https://doi.org/10.3390/universe7010017
Chicago/Turabian StyleAndersson, Nils. 2021. "A Superfluid Perspective on Neutron Star Dynamics" Universe 7, no. 1: 17. https://doi.org/10.3390/universe7010017
APA StyleAndersson, N. (2021). A Superfluid Perspective on Neutron Star Dynamics. Universe, 7(1), 17. https://doi.org/10.3390/universe7010017




