Stellar Modeling via the Tolman IV Solution: The Cases of the Massive Pulsar J0740+6620 and the HESS J1731-347 Compact Object
Abstract
1. Introduction
2. Anisotropic Relativistic Stars in GR
3. Kohler Chao and Tolman IV Solutions
4. Modeling Objects of Known Mass and Radius
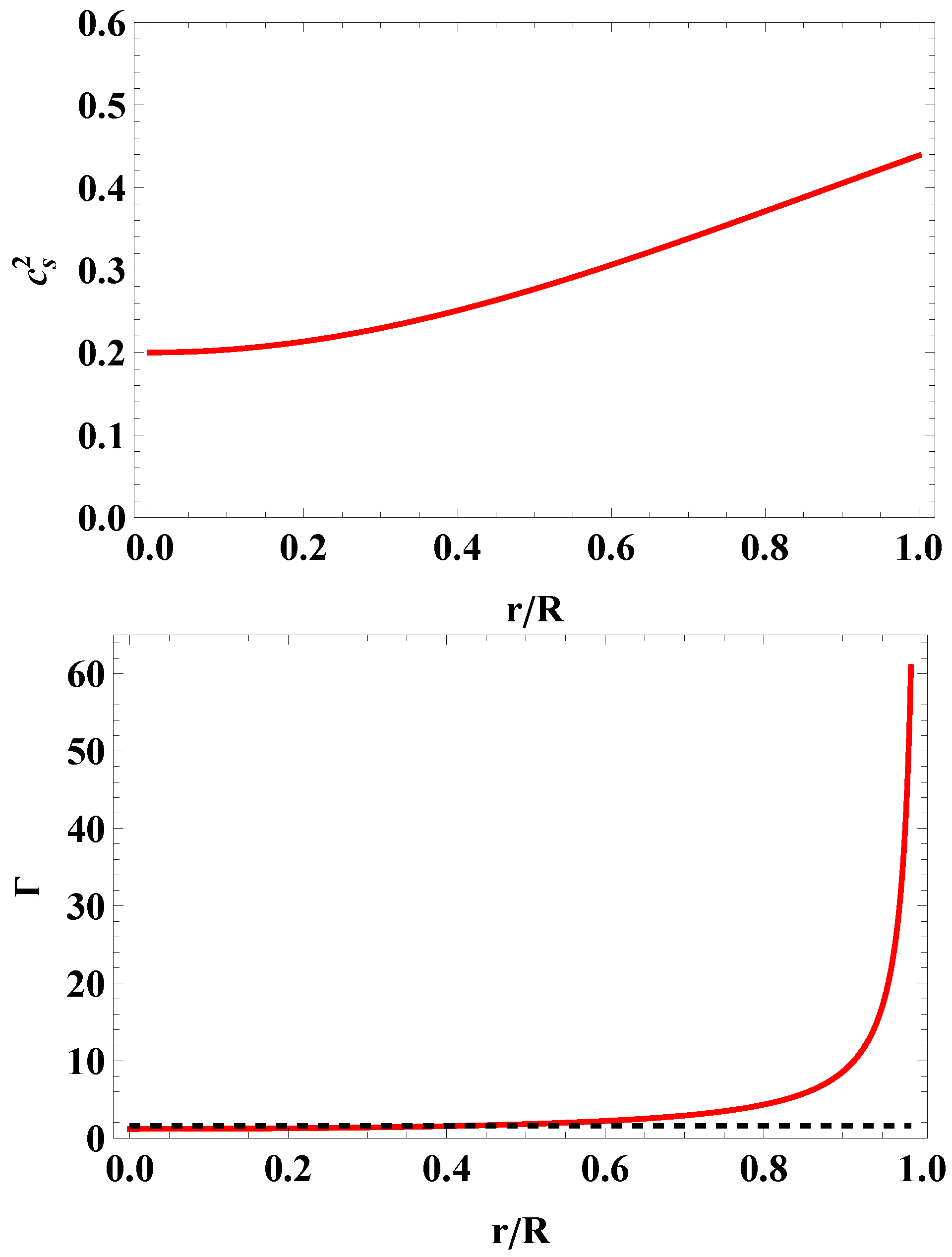
4.1. Criteria for Realistic Solutions
- Causality, that is, the sound speed cannot exceed the speed of light in vacuum
- The energy conditions are the constraints on the energy–momentum tensor of the matter content within a given theory of gravity. The standard acceptable conditions assumed for the energy–momentum tensor are: weak energy condition (WEC), dominant energy condition (DEC), null energy condition (NEC), and strong energy condition (SEC) (see, for instance, [70,71,72]). If and are arbitrary time-like and null vectors, respectively, then the conditions for the energy–momentum tensor are expressed with the following inequalities
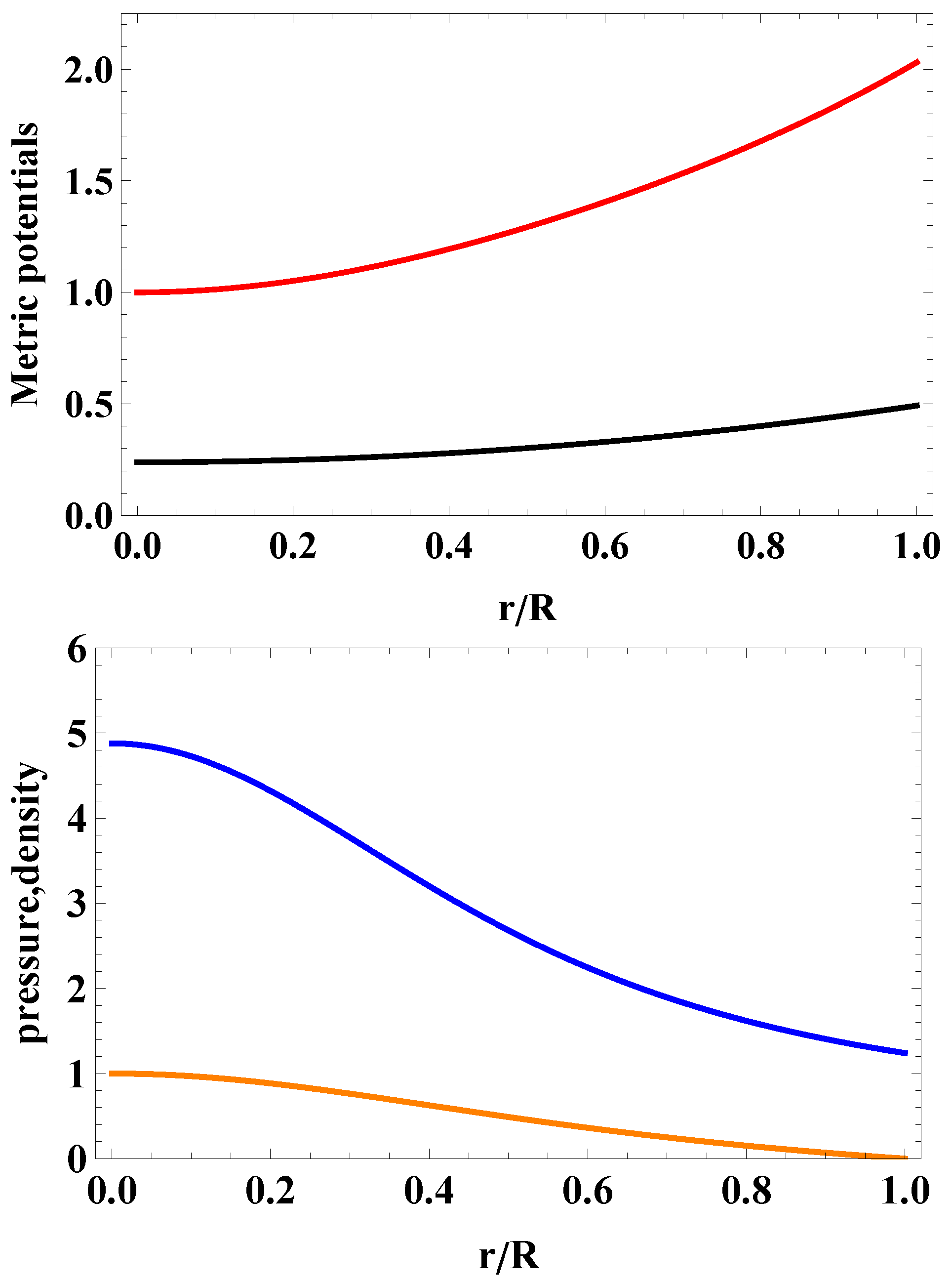
4.2. Case I: Massive Pulsar J0740+6620
4.3. Case II: Light Object HESS J1731-347
5. Conclusions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Einstein, A. The Field Equations of Gravitation; Sitzungsberichte der Preussischen Akademie der Wissenschaften: Berlin, Germany, 1915; pp. 844–847. [Google Scholar]
- Weinberg, S. Gravitation and Cosmology: Principles and Applications of the General Theory of Gravitation; John Wiley and Sons: Hoboken, NJ, USA, 1972. [Google Scholar]
- Meisner, C.W.; Thorne, K.S.; Wheeler, J.A. Gravitation; Princeton University Press: Princeton, NJ, USA, 1973. [Google Scholar]
- Abbott, B.P.; LIGO Scientific and Virgo. Observation of Gravitational Waves from a Binary Black Hole Merger. Phys. Rev. Lett. 2016, 116, 061102. [Google Scholar] [CrossRef]
- Abbott, B.P.; LIGO Scientific and Virgo. GW151226: Observation of Gravitational Waves from a 22-Solar-Mass Binary Black Hole Coalescence. Phys. Rev. Lett. 2016, 116, 241103. [Google Scholar] [CrossRef] [PubMed]
- Abbott, B.P.; LIGO Scientific and VIRGO. GW170104: Observation of a 50-Solar-Mass Binary Black Hole Coalescence at Redshift 0.2. Phys. Rev. Lett. 2017, 118, 221101, Erratum in: Phys. Rev. Lett. 2018, 121, 129901. [Google Scholar] [CrossRef] [PubMed]
- Asmodelle, E. Tests of General Relativity: A Review. arXiv 2004, arXiv:1705.04397. [Google Scholar]
- Stephani, H.; Kramer, D.; Maccallum, M.; Hoenselaers, C.; Herlt, E. Exact Solutions of Einstein’s Field Equations; Cambridge University Press: Cambridge, UK, 2003. [Google Scholar]
- Ruderman, R. Pulsars: Structure and dynamics. Ann. Rev. Astron. Astrophys. 1972, 10, 427. [Google Scholar] [CrossRef]
- Bowers, R.L.; Liang, E.P.T.; Astrophys, J. Anisotropic spheres in general relativity. Astrophys. J. 1974, 188, 657. [Google Scholar] [CrossRef]
- Sokolov, A.I. Fazovye perekhody v sverkhtekuchej nejtronno zhidkosti. J. Exp. Theor. Phys. 1980, 79, 1137. [Google Scholar]
- Sawyer, R.F. Condensed Π-Phase in Neutron-Star Matter. Phys. Rev. Lett. 1972, 29, 382. [Google Scholar] [CrossRef]
- Kippenhahn, R.; Weigert, A. Stellar Structure and Evolution; Springer: Berlin, Germany, 1990. [Google Scholar]
- Ovalle, J. Decoupling gravitational sources in general relativity: From perfect to anisotropic fluids. Phys. Rev. D 2017, 95, 104019. [Google Scholar] [CrossRef]
- Ovalle, J. Searching exact solutions for compact stars in braneworld: A Conjecture. Mod. Phys. Lett. A 2008, 23, 3247–3263. [Google Scholar] [CrossRef]
- Randall, L.; Sundrum, R. A Large mass hierarchy from a small extra dimension. Phys. Rev. Lett. 1999, 83, 3370–3373. [Google Scholar] [CrossRef]
- Randall, L.; Sundrum, R. An Alternative to compactification. Phys. Rev. Lett. 1999, 83, 4690–4693. [Google Scholar] [CrossRef]
- Estrada, M.; Tello-Ortiz, F. A new family of analytical anisotropic solutions by gravitational decoupling. Eur. Phys. J. Plus 2018, 133, 453. [Google Scholar] [CrossRef]
- Morales, E.; Tello-Ortiz, F. Compact Anisotropic Models in General Relativity by Gravitational Decoupling. Eur. Phys. J. C 2018, 78, 841. [Google Scholar] [CrossRef]
- Estrada, M.; Prado, R. The Gravitational decoupling method: The higher dimensional case to find new analytic solutions. Eur. Phys. J. Plus 2019, 134, 168. [Google Scholar] [CrossRef]
- Ovalle, J.; Casadio, R.; da Rocha, R.; Sotomayor, A.; Stuchlik, Z. Black holes by gravitational decoupling. Eur. Phys. J. C 2018, 78, 960. [Google Scholar] [CrossRef]
- Ovalle, J. Non-uniform Braneworld Stars: An Exact Solution. Int. J. Mod. Phys. D 2009, 18, 837–852. [Google Scholar] [CrossRef]
- Ovalle, J. The Schwarzschild’s Braneworld Solution. Mod. Phys. Lett. A 2010, 25, 3323–3334. [Google Scholar] [CrossRef]
- Casadio, R.; Ovalle, J. Brane-world stars and (microscopic) black holes. Phys. Lett. B 2012, 715, 251–255. [Google Scholar] [CrossRef]
- Casadio, R.; Ovalle, J. Brane-world stars from minimal geometric deformation, and black holes. Gen. Rel. Grav. 2014, 46, 1669. [Google Scholar] [CrossRef]
- Ovalle, J.; Linares, F. Tolman IV solution in the Randall-Sundrum Braneworld. Phys. Rev. D 2013, 88, 104026. [Google Scholar] [CrossRef]
- Ovalle, J.; Gergely, L.; Casadio, R. Brane-world stars with a solid crust and vacuum exterior. Class. Quant. Grav. 2015, 32, 045015. [Google Scholar] [CrossRef]
- Ovalle, J. Decoupling gravitational sources in general relativity: The extended case. Phys. Lett. B 2019, 788, 213–218. [Google Scholar] [CrossRef]
- Fernandes-Silva, A.; Ferreira-Martins, A.J.; da Rocha, R. Extended quantum portrait of MGD black holes and information entropy. Phys. Lett. B 2019, 791, 323–330. [Google Scholar] [CrossRef]
- Contreras, E. Minimal Geometric Deformation: The inverse problem. Eur. Phys. J. C 2018, 78, 678. [Google Scholar] [CrossRef]
- Contreras, E.; Bargueño, P. Minimal Geometric Deformation in asymptotically (A-)dS space-times and the isotropic sector for a polytropic black hole. Eur. Phys. J. C 2018, 78, 985. [Google Scholar] [CrossRef]
- Herrera, L. New definition of complexity for self-gravitating fluid distributions: The spherically symmetric, static case. Phys. Rev. D 2018, 97, 044010. [Google Scholar] [CrossRef]
- Abbas, G.; Nazar, H. Complexity Factor for Static Anisotropic Self-Gravitating Source in f(R) Gravity. Eur. Phys. J. C 2018, 78, 510. [Google Scholar] [CrossRef]
- Sharif, M.; Butt, I.I. Complexity Factor for Charged Spherical System. Eur. Phys. J. C 2018, 78, 688. [Google Scholar] [CrossRef]
- Abbas, G.; Nazar, H. Complexity Factor for Anisotropic Source in Non-minimal Coupling Metric f(R) Gravity. Eur. Phys. J. C 2018, 78, 957. [Google Scholar] [CrossRef]
- Nazar, H.; Abbas, G. Complexity factor for dynamical spherically symmetric fluid distributions in f(R) gravity. Int. J. Geom. Meth. Mod. Phys. 2019, 16, 1950170. [Google Scholar] [CrossRef]
- Sharif, M.; Majid, A. Complexity factor for static sphere in self-interacting Brans–Dicke gravity. Chin. J. Phys. 2019, 61, 38–46. [Google Scholar] [CrossRef]
- Sharif, M.; Majid, A.; Nasir, M.M.M. Complexity factor for self-gravitating system in modified Gauss–Bonnet gravity. Int. J. Mod. Phys. A 2019, 34, 1950210. [Google Scholar] [CrossRef]
- Khan, S.; Mardan, S.A.; Rehman, M.A. Framework for generalized polytropes with complexity factor. Eur. Phys. J. C 2019, 79, 1037. [Google Scholar] [CrossRef]
- Nazar, H.; Alkhaldi, A.H.; Abbas, G.; Shahzad, M.R. Complexity factor for anisotropic self-gravitating sphere in Rastall gravity. Int. J. Mod. Phys. A 2021, 36, 2150233. [Google Scholar] [CrossRef]
- Arias, C.; Contreras, E.; Fuenmayor, E.; Ramos, A. Anisotropic star models in the context of vanishing complexity. Ann. Phys. 2022, 436, 168671. [Google Scholar] [CrossRef]
- Rincon, A.; Panotopoulos, G.; Lopes, I. Anisotropic Quark Stars with an Interacting Quark Equation of State within the Complexity Factor Formalism. Universe 2023, 9, 72. [Google Scholar] [CrossRef]
- Rincon, A.; Panotopoulos, G.; Lopes, I. Anisotropic stars made of exotic matter within the complexity factor formalism. Eur. Phys. J. C 2023, 83, 116. [Google Scholar] [CrossRef]
- Karmarkar, K.R. Gravitational metrics of spherical symmetry and class one. Proc. Ind. Acad. Sci. A 1948, 27, 56. [Google Scholar] [CrossRef]
- Maurya, S.K.; Gupta, Y.K.; Smitha, T.T.; Rahaman, F. A new exact anisotropic solution of embedding class one. Eur. Phys. J. A 2016, 52, 191. [Google Scholar] [CrossRef]
- Singh, K.N.; Bhar, P.; Pant, N. A new solution of embedding class I representing anisotropic fluid sphere in general relativity. Int. J. Mod. Phys. D 2016, 25, 1650099. [Google Scholar] [CrossRef]
- Bhar, P.; Maurya, S.K.; Gupta, Y.K.; Manna, T. Modelling of anisotropic compact stars of embedding class one. Eur. Phys. J. A 2016, 52, 312. [Google Scholar] [CrossRef]
- Maurya, S.K.; Gupta, Y.K.; Ray, S.; Deb, D. A new model for spherically symmetric charged compact stars of embedding class 1. Eur. Phys. J. C 2017, 77, 45. [Google Scholar] [CrossRef]
- Bhar, P.; Govender, M. Anisotropic charged compact star of embedding class I. Int. J. Mod. Phys. D 2016, 26, 1750053. [Google Scholar] [CrossRef]
- Maurya, S.K.; Maharaj, S.D. Anisotropic fluid spheres of embedding class one using Karmarkar condition. Eur. Phys. J. C 2017, 77, 328. [Google Scholar] [CrossRef]
- Bhar, P.; Singh, K.N.; Manna, T. A new class of relativistic model of compact stars of embedding class I. Int. J. Mod. Phys. D 2017, 26, 1750090. [Google Scholar] [CrossRef]
- Tello-Ortiz, F.; Maurya, S.K.; Errehymy, A.; Singh, K.N.; Daoud, M. Anisotropic relativistic fluid spheres: An embedding class I approach. Eur. Phys. J. C 2019, 79, 885. [Google Scholar] [CrossRef]
- Jasim, M.K.; Maurya, S.K.; Al-Sawaii, A.S.M. A generalised embedding class one static solution describing anisotropic fluid sphere. Astrophys. Space Sci. 2020, 365, 9. [Google Scholar] [CrossRef]
- Baskey, L.; Das, S.; Rahaman, F. An analytical anisotropic compact stellar model of embedding class I. Mod. Phys. Lett. A 2021, 36, 2150028. [Google Scholar] [CrossRef]
- Zubair, M.; Waheed, S.; Javaid, H. A Generic Embedding Class-I Model via Karmarkar Condition in Gravity. Adv. Astron. 2021, 2021, 6685578. [Google Scholar] [CrossRef]
- Demorest, P.; Pennucci, T.; Ransom, S.; Roberts, M.; Hessels, J. Shapiro Delay Measurement of A Two Solar Mass Neutron Star. Nature 2010, 467, 1081–1083. [Google Scholar] [CrossRef]
- Antoniadis, J.; Freire, P.C.C.; Wex, N.; Tauris, T.M.; Lynch, R.S.; van Kerkwijk, M.H.; Kramer, M.; Bassa, C.; Dhillon, V.S.; Driebe, T.; et al. A Massive Pulsar in a Compact Relativistic Binary. Science 2013, 340, 6131. [Google Scholar] [CrossRef] [PubMed]
- Sullivan, A.G.; Romani, R.W. A Joint X-ray and Optical Study of the Massive Redback Pulsar J2215+5135. arXiv 2024, arXiv:2405.13889v. [Google Scholar] [CrossRef]
- Weber, F. Strange quark matter and compact stars. Prog. Part. Nucl. Phys. 2005, 54, 193–288. [Google Scholar] [CrossRef]
- Aziz, A.; Ray, S.; Rahaman, F.; Khlopov, M.; Guha, B.K. Constraining values of bag constant for strange star candidates. Int. J. Mod. Phys. D 2019, 28, 1941006. [Google Scholar] [CrossRef]
- Miller, M.C.; Lamb, F.K.; Dittmann, A.J.; Bogdanov, S.; Arzoumanian, Z.; Gendreau, K.C.; Guillot, S.; Ho, W.C.G.; Lattimer, J.M.; Loewenstein, M.; et al. The Radius of PSR J0740+6620 from NICER and XMM-Newton Data. Astrophys. J. Lett. 2021, 918, L28. [Google Scholar] [CrossRef]
- Riley, T.E.; Watts, A.L.; Ray, P.S.; Bogdanov, S.; Guillot, S.; Morsink, S.M.; Bilous, A.V.; Arzoumanian, Z.; Choudhury, D.; Deneva, J.S.; et al. A NICER View of the Massive Pulsar PSR J0740+6620 Informed by Radio Timing and XMM-Newton Spectroscopy. Astrophys. J. Lett. 2021, 918, L27. [Google Scholar] [CrossRef]
- Salmi, T.; Vinciguerra, S.; Choudhury, D.; Riley, T.E.; Watts, A.L.; Remillard, R.A.; Ray, P.S.; Bogdanov, S.; Guillot, S.; Arzoumanian, Z.; et al. The Radius of PSR J0740+6620 from NICER with NICER Background Estimates. Astrophys. J. 2022, 941, 150. [Google Scholar] [CrossRef]
- Doroshenko, V.; Suleimanov, V.; Pühlhofer, G.; Santangelo, A. A strangely light neutron star within a supernova remnant. Nat. Astron. 2022, 6, 1444–1451. [Google Scholar] [CrossRef]
- Tolman, R.C. Static solutions of Einstein’s field equations for spheres of fluid. Phys. Rev. 1939, 55, 364–373. [Google Scholar] [CrossRef]
- Oppenheimer, J.R.; Volkoff, G.M. On massive neutron cores. Phys. Rev. 1939, 55, 374–381. [Google Scholar] [CrossRef]
- Schwarzschild, K. On the Gravitational Field of a Mass Point According to Einstein’s Theory; Sitzungsberichte der Preussischen Akademie der Wissenschaften: Berlin, Germany, 1916; pp. 189–196. [Google Scholar]
- Kohler, M.; Chao, K.L. Zentralsymmetrische statische Schwerefelder mit Räumen der Klasse 1. Z. Naturforsch. Ser. A 1965, 20, 1537. [Google Scholar] [CrossRef]
- Moustakidis, C.C. The stability of relativistic stars and the role of the adiabatic index. Gen. Rel. Grav. 2017, 49, 68. [Google Scholar] [CrossRef]
- Hawking, S.W.; Ellis, G.F.R. The Large Scale Structure of Space-Time; Cambridge University Press: Cambridge, UK, 2023. [Google Scholar]
- Wald, R.M. General Relativity; Chicago University Press: Chicago, IL, USA, 1984. [Google Scholar]
- Frolov, V.P.; Novikov, I.D. Black Hole Physics: Basic Concepts and New Developments; Springer: Dordrecht, The Netherlands, 1998. [Google Scholar]
- Panotopoulos, G.; Vernieri, D.; Lopes, I. Quark stars with isotropic matter in Hořava gravity and Einstein–æther theory. Eur. Phys. J. C 2020, 80, 537. [Google Scholar] [CrossRef]
- Balart, L.; Panotopoulos, G.; Rincon, A. Regular Charged Black Holes, Energy Conditions, and Quasinormal Modes. Fortsch. Phys. 2023, 71, 2300075. [Google Scholar] [CrossRef]
- Pourhassan, B.; Kahya, E.O. Extended Chaplygin gas model. Results Phys. 2014, 4, 101–102. [Google Scholar] [CrossRef]
- Alford, M.G.; Schmitt, A.; Rajagopal, K.; Schäfer, T. Color superconductivity in dense quark matter. Rev. Mod. Phys. 2008, 80, 1455–1515. [Google Scholar] [CrossRef]
- Flores, C.V.; Lugones, G. Constraining color flavor locked strange stars in the gravitational wave era. Phys. Rev. C 2017, 95, 025808. [Google Scholar] [CrossRef]



Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the author. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Panotopoulos, G. Stellar Modeling via the Tolman IV Solution: The Cases of the Massive Pulsar J0740+6620 and the HESS J1731-347 Compact Object. Universe 2024, 10, 342. https://doi.org/10.3390/universe10090342
Panotopoulos G. Stellar Modeling via the Tolman IV Solution: The Cases of the Massive Pulsar J0740+6620 and the HESS J1731-347 Compact Object. Universe. 2024; 10(9):342. https://doi.org/10.3390/universe10090342
Chicago/Turabian StylePanotopoulos, Grigoris. 2024. "Stellar Modeling via the Tolman IV Solution: The Cases of the Massive Pulsar J0740+6620 and the HESS J1731-347 Compact Object" Universe 10, no. 9: 342. https://doi.org/10.3390/universe10090342
APA StylePanotopoulos, G. (2024). Stellar Modeling via the Tolman IV Solution: The Cases of the Massive Pulsar J0740+6620 and the HESS J1731-347 Compact Object. Universe, 10(9), 342. https://doi.org/10.3390/universe10090342








