A Partial Eruption of a Sigmoid Filament in the Small Dipole Active Region 12734
Abstract
1. Introduction
2. Data and Methods
3. Results
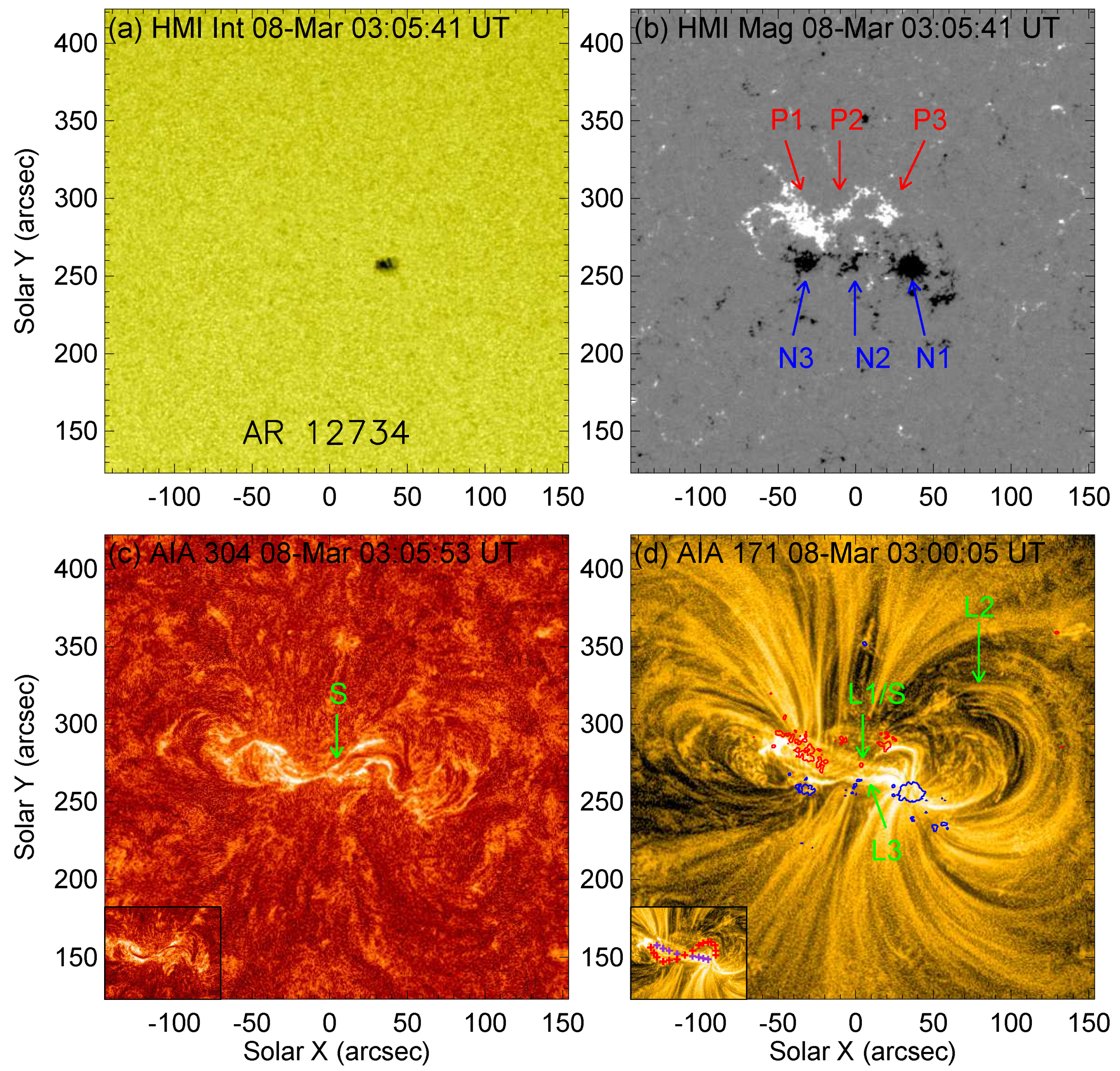
3.1. The Magnetic Topological Structure of the AR
3.1.1. The Sigmoidal Structure and the Eruptive Events
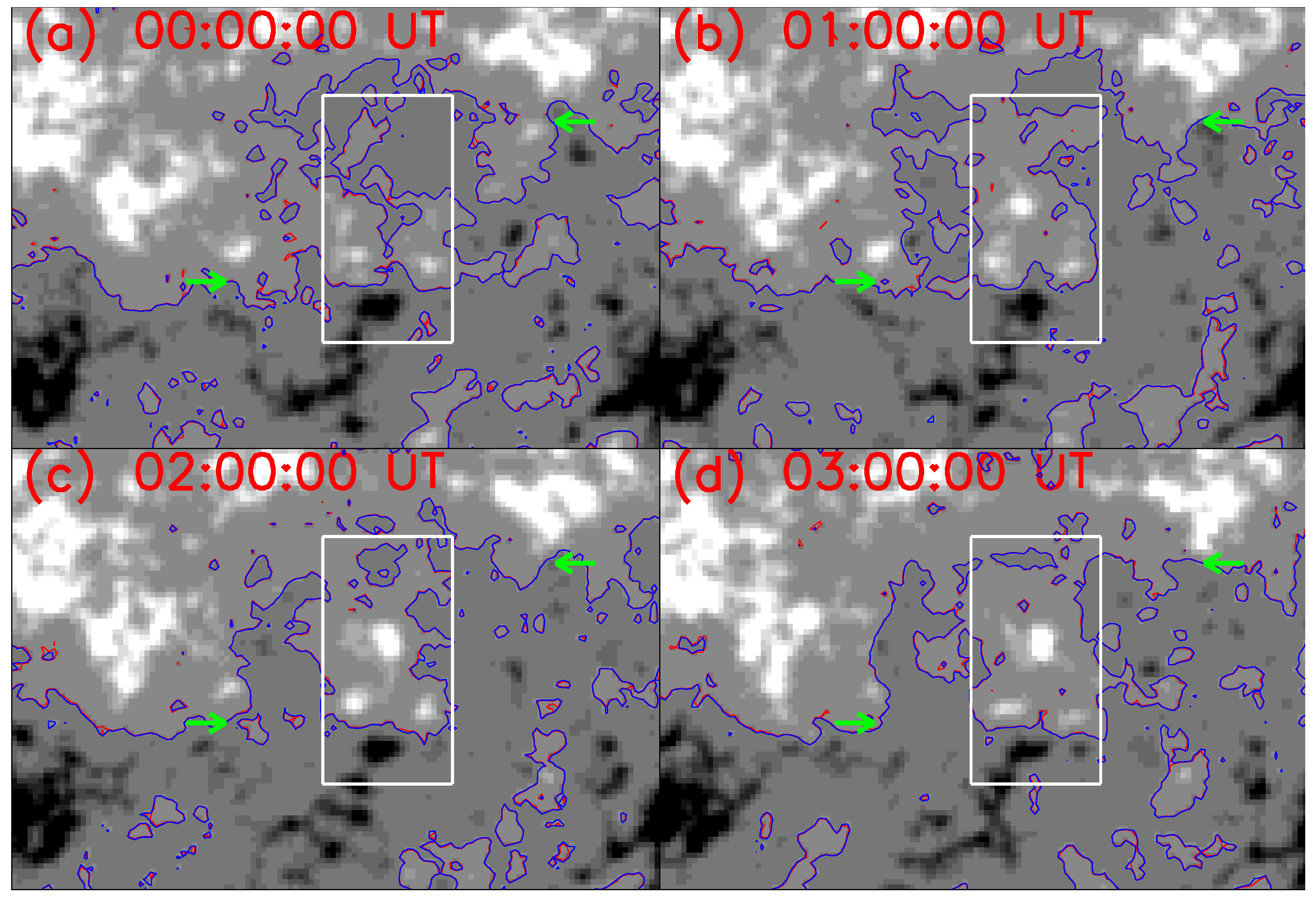
3.1.2. The ‘X’-Shaped Structure of Sunspot Pairs
3.2. The Magnetic Chirality of the AR
3.2.1. Magnetic Helicity Flux and Helicity Accumulations
3.2.2. Right-Handed Spiral: Positive Magnetic Twist and Writhe of the AR
3.2.3. The Partial Eruption and Kink Instability
4. Conclusions and Discussions
- 1.
- The AR shows a sigmoidal structure in the low corona. It disobeys the hemispherical helicity rule and has magnetic twist and writhe of the same signs. These properties make it eruptive.
- 2.
- An ‘X’-shaped structure is formed between the original ‘S’-shaped magnetic loop and the newly rising one between the main positive and negative magnetic polarities of sunspots. The intersection point of magnetic flux ropes L1 and L3 correspond well with the initial brightening of the flare. Therefore, the continuous magnetic emergence and cancellation along the PIL may cause the magnetic reconnection of the highly twisted helical flux ropes, which is probably responsible for the observed evolution of the event.
- 3.
- The calculated maximum helicity flux is about Mx h around the flare time. The ratio of helicity accumulation to the square of magnetic flux reaches for AR 12734. The AR has a right-handed spiral which is likely strong enough to cause a flare.
- 4.
- The decay index n is about 1.74 at the height of 27.2 Mm, which is more than enough to support a partial filament eruption.
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Pesnell, W.D.; Thompson, B.J.; Chamberlin, P.C. The Solar Dynamics Observatory (SDO). Sol. Phys. 2012, 275, 3–15. [Google Scholar] [CrossRef]
- Lemen, J.R.; Title, A.M.; Akin, D.J.; Boerner, P.F.; Chou, C.; Drake, J.F.; Duncan, D.W.; Edwards, C.G.; Friedlaender, F.M.; Heyman, G.F.; et al. The Atmospheric Imaging Assembly (AIA) on the Solar Dynamics Observatory (SDO). Sol. Phys. 2012, 275, 17–40. [Google Scholar] [CrossRef]
- Yang, S.H.; Zhang, J.; Song, Q.; Bi, Y.; Li, T. Two-step Evolution of a Rising Flux Rope Resulting in a Confined Solar Flare. Astrophys. J. 2019, 878, 38. [Google Scholar] [CrossRef]
- Zhang, Y.; Liu, J.; Tan, B.; Zhu, X.; Yan, Y. Dynamic Evolution of Magnetic Flux Ropes in Active Region 11429. I. EUV Observations. Astrophys. J. 2022, 940, 125. [Google Scholar] [CrossRef]
- Liu, R. Magnetic flux ropes in the solar corona: Structure and evolution toward eruption. Res. Astron. Astrophys. 2020, 20, 165. [Google Scholar] [CrossRef]
- Shibata, K.; Masuda, S.; Shimojo, M.; Hara, H.; Yokoyama, T.; Tsuneta, S.; Kosugi, T.; Ogawara, Y. Hot-Plasma Ejections Associated with Compact-Loop Solar Flares. Astrophys. J. 1995, 451, L83. [Google Scholar] [CrossRef]
- Lin, J.; Forbes, T.G. Effects of reconnection on the coronal mass ejection process. J. Geophys. Res. 2000, 105, 2375. [Google Scholar] [CrossRef]
- Zweibel, E.G.; Yamada, M. Magnetic Reconnection in Astrophysical and Laboratory Plasmas. Annu. Rev. Astron. Astrophys. 2009, 47, 291–332. [Google Scholar] [CrossRef]
- Deng, Y.Y.; Wang, J.X.; Yan, Y.H.; Zhang, J. Evolution of Magnetic Nonpotentiality in NOAA AR 9077. Sol. Phys. 2001, 204, 11–26. [Google Scholar] [CrossRef]
- Su, J.; Jing, J.; Wang, S.; Wiegelmann, T.; Wang, H. Statistical Study of Free Magnetic Energy and Flare Productivity of Solar Active Regions. Astrophys. J. 2014, 788, 150. [Google Scholar] [CrossRef][Green Version]
- Chen, J.; Su, J.; Yin, Z.; Priya, T.G.; Zhang, H.; Liu, J.; Xu, H.; Yu, S. Recurrent Solar Jets Induced By a Satellite Spot and Moving Magnetic Features. Astrophys. J. 2015, 815, 71. [Google Scholar] [CrossRef]
- Guo, J.; Wang, H.; Wang, J.; Zhu, X.; Dai, X.; Huang, X.; He, H.; Yan, Y.; Zhao, H. The Role of a Magnetic Topology Skeleton in a Solar Active Region. Astrophys. J. 2019, 874, 181. [Google Scholar] [CrossRef]
- Ruan, G.; Chen, Y.; Wang, H. Gradual Magnetic Evolution of Sunspot Structure Furthermore, Filament-Corona Dynamics Associated With the X1.8 Flare in AR11283. Astrophys. J. 2015, 812, 120–127. [Google Scholar] [CrossRef]
- Xu, H.; Su, J.; Chen, J.; Ruan, G.; Awasthi, A.K.; Zhang, H.; Zhang, M.; Ji, K.; Zhang, Y.; Liu, J. Multiwavelength Observation of a Failed Eruption from a Helical Kink-unstable Prominence. Astrophys. J. 2020, 901, 121. [Google Scholar] [CrossRef]
- Yang, S.; Zhang, J.; Xiang, Y. Magnetic Reconnection between Small-scale Loops Observed with the New Vacuum Solar Telescope. Astrophys. J. Lett. 2015, 798, L11. [Google Scholar] [CrossRef]
- Wyper, P.F.; Antiochos, S.K.; DeVore, C.R. A Universal Model For Solar Eruptions. Nature 2017, 544, 452–455. [Google Scholar] [CrossRef] [PubMed]
- Zhang, Y.; Tan, B.L.; Tan, C.M.; Huang, J.; Yan, Y.H. Multi-Wavelength Observations of a Failed Filament Eruption and Associated Hovered Coronal Mass Ejection. Universe 2021, 7, 405. [Google Scholar] [CrossRef]
- Moore, R.L.; Sterling, A.C.; Hudson, H.S.; Lemen, J.R. Onset of the Magnetic Explosion in Solar Flares and Coronal Mass Ejections. Astrophys. J. 2001, 552, 833. [Google Scholar] [CrossRef]
- Antiochos, S.K.; DeVore, C.R.; Klimchuk, J.A. A Model for Solar Coronal Mass Ejections. Astrophys. J. 1999, 510, 485. [Google Scholar] [CrossRef]
- Liu, L.J.; Wang, Y.M.; Zhou, Z.J.; Cui, J. The Source Locations of Major Flares and CMEs in Emerging Active Regions. Astrophys. J. 2021, 909, 142. [Google Scholar] [CrossRef]
- Dai, J.; Li, Z.T.; Wang, Y.; Xu, Z.; Zhang, Y.J.; Li, L.P.; Zhang, Q.M.; Su, Y.N.; Ji, H.S. A Partial Filament Eruption in Three Steps Induced by External Magnetic Reconnection. Astrophys. J. 2022, 929, 85–96. [Google Scholar] [CrossRef]
- Tian, L.R.; Alexander, D. Role of Sunspot and Sunspot-Group Rotation in Driving Sigmoidal Active Region Eruptions. Sol. Phys. 2006, 233, 29–43. [Google Scholar] [CrossRef]
- Rust, D.M.; Kumar, A. Evidence for Helically Kinked Magnetic Flux Ropes in Solar Eruptions. Astrophys. J. Lett. 1996, 464, L199. [Google Scholar] [CrossRef]
- Leka, K.D.; Fan, Y.; Barnes, G. On the Availability of Sufficient Twist in Solar Active Regions to Trigger the Kink Instability. Astrophys. J. 2005, 626, 1091. [Google Scholar] [CrossRef]
- Régnier, S.; Amari, T.; Kersalé, E. 3D Coronal magnetic field from vector magnetograms: Non-constant-α force-free configuration of the active region NOAA 8151. Astron. Astrophys. 2002, 392, 1119–1127. [Google Scholar] [CrossRef]
- Régnier, S.; Amari, T. 3D magnetic configuration of the Hα filament and X-ray sigmoid in NOAA AR 8151. Astron. Astrophys. 2004, 425, 345–352. [Google Scholar] [CrossRef]
- Ruan, G.; Jejčič, S.; Schmieder, B.; Mein, P.; Mein, N.; Heinzel, P.; Gunár, S.; Chen, Y. Diagnostics of the Prominence Plasma from Hα and Mg II Spectral Observations. Astrophys. J. 2019, 886, 134–147. [Google Scholar] [CrossRef]
- Jing, J.; Liu, C.; Lee, J.; Ji, H.; Liu, N.; Xu, Y.; Wang, H. Statistical Analysis of Torus and Kink Instabilities in Solar Eruptions. Astrophys. J. 2018, 864, 138. [Google Scholar] [CrossRef]
- Wang, D.; Liu, R.; Wang, Y.M.; Liu, K.; Chen, J.; Liu, J.J.; Zhou, Z.J.; Zhang, M. Critical Height of the Torus Instability in Two-ribbon Solar Flares. Astrophys. J. Lett. 2017, 843, L9–L14. [Google Scholar] [CrossRef]
- Pevtsov, A.A.; Canfield, R.C.; Metcalf, T.R. Latitudinal Variation of Helicity of Photospheric Magnetic Fields. Astrophys. J. Lett. 1995, 440, L109–L112. [Google Scholar] [CrossRef]
- Abramenko, V.I.; Wang, T.; Yurchishin, V.B. Analysis of Electric Current Helicity in Active Regions on the Basis of Vector Magnetograms. Sol. Phys. 1996, 168, 75–89. [Google Scholar] [CrossRef]
- Bao, S.; Zhang, H. Patterns of Current Helicity for Solar Cycle 22. Astrophys. J. Lett. 1998, 496, L43–L46. [Google Scholar] [CrossRef]
- Zhang, H.; Moss, D.; Kleeorin, N.; Kuzanyan, K.; Rogachevskii, I.; Sokoloff, D.; Gao, Y.; Xu, H. Current Helicity of Active Regions as A Tracer of Large-Scale Solar Magnetic Helicity. Astrophys. J. 2012, 751, 47. [Google Scholar] [CrossRef]
- Kutsenko, A.S.; Abramenko, V.I.; Pevtsov, A.A. Extended statistical analysis of emerging solar active regions. Mon. Not. R. Astron. Soc. 2019, 484, 4393–4400. [Google Scholar] [CrossRef]
- Shen, Y.D.; Li, B.; Chen, P.F.; Zhou, X.P.; Liu, Y. Research Progress on Coronal Extreme Ultraviolet Waves. Sci. Bull. 2020, 65, 3909–3923. (In Chinese) [Google Scholar] [CrossRef]
- Shen, Y.; Zhou, X.; Duan, Y.; Tang, Z.; Zhou, C.; Tan, S. Coronal Quasi-periodic Fast-mode Propagating Wave Trains. Sol. Phys. 2022, 297, 20. [Google Scholar] [CrossRef]
- Scherrer, P.H.; Schou, J.; Bush, R.I.; Kosovichev, A.G.; Bogart, R.S.; Hoeksema, J.T.; Liu, Y.; Duvall, T.L., Jr.; Zhao, J.; Title, A.M.; et al. The Helioseismic and Magnetic Imager (HMI) Investigation for the Solar Dynamics Observatory (SDO). Sol. Phys. 2012, 275, 207–227. [Google Scholar] [CrossRef]
- Sun, X.D. On the Coordinate System of Space-Weather HMI Active Region Patches (SHARPs): A Technical Note. arXiv 2013, arXiv:1309.2392. [Google Scholar]
- Bobra, M.G.; Sun, X.D.; Hoeksema, J.T.; Turmon, M.; Liu, Y.; Hayashi, K.; Barnes, G.; Leka, K.D. The Helioseismic and Magnetic Imager (HMI) Vector Magnetic Field Pipeline: SHARPs-Space-Weather HMI Active Region Patches. Sol. Phys. 2014, 289, 3549–3578. [Google Scholar] [CrossRef]
- Liu, Y.; Hoeksema, J.T.; Bobra, M.; Hayashi, K.; Schuck, P.W.; Sun, X. Magnetic Helicity in Emerging Solar Active Regions. Astrophys. J. 2014, 785, 13. [Google Scholar] [CrossRef]
- Liu, Y.; Zhao, J.; Schuck, P.W. Horizontal Flows in the Photosphere and Subphotosphere of Two Active Regions. Sol. Phys. 2013, 287, 279–291. [Google Scholar] [CrossRef]
- Berger, M.A.; Field, G.B. The Topological Properties of Magnetic Helicity. J. Fluid Mech. 1984, 147, 133–148. [Google Scholar] [CrossRef]
- Démoulin, P.; Berger, M.A. Magnetic Energy and Helicity Fluxes at the Photospheric Level. Sol. Phys. 2003, 215, 203–215. [Google Scholar] [CrossRef]
- Yang, S.; Büchner, J.; Skála, J.; Zhang, H. Evolution of Relative Magnetic Helicity New Boundary Conditions for the Vector Potential. Astron. Astrophys. 2018, 613, A27. [Google Scholar] [CrossRef]
- Berger, M.A.; Ruzmaikin, A. Rate of Helicity Production by Solar Rotation. Geophys. Res. 2000, 105, 10481–10489. [Google Scholar] [CrossRef]
- Gao, Y. A Quantity Characterizing Variation of Observed Magnetic Twist in Solar Active Regions. Res. Astron. Astrophys. 2018, 18, 28. [Google Scholar] [CrossRef]
- Zhang, H.; Sakurai, T.; Pevtsov, A.; Gao, Y.; Xu, H.; Sokoloff, D.; Kuzanyan, K. A New Dynamo Pattern Revealed by Solar Helical Magnetic Fields. Mon. Not. R. Astron. Soc. 2010, 402, L30–L33. [Google Scholar] [CrossRef]
- Liu, J.H.; Liu, Y.; Zhang, Y.; Huang, J.; Zhang, H.Q. Helicity observations of active regions during the exchange period of Solar Cycle 24 and 25. Mon. Not. R. Astron. Soc. 2022, 509, 5298–5304. [Google Scholar] [CrossRef]
- Liu, Y.; Hoeksema, J.T.; Sun, X. Test of The Hemispheric Rule of Magnetic Helicity in the Sun Using the Helioseismic and Furthermore, Magnetic Imager (HMI) Data. Astrophys. J. Lett. 2014, 783, L1–L6. [Google Scholar] [CrossRef]
- Miao, Y.H.; Li, D.; Yuan, D.; Jiang, C.W.; Elmhamdi, A.; Zhao, M.Y.; Anfinogentov, S. Diagnosing a Solar Flaring Core with Bidirectional Quasi-periodic Fast Propagating Magnetoacoustic Waves. Astrophys. J. Lett. 2021, 908, L37. [Google Scholar] [CrossRef]
- Hale, G.E. The Fields of Force in the Atmosphere of the Sun. Nature 1927, 119, 708. [Google Scholar] [CrossRef]
- Zhang, Y.; Liu, J.H.; Zhang, H.Q. Relationship between Rotating Sunspots and Flares. Sol. Phys. 2008, 247, 39–52. [Google Scholar] [CrossRef]
- LaBonte, B.J.; Georgoulis, M.K.; Rust, D.M. Survey of Magnetic Helicity Injection in Regions Producing X-class Flares. Astrophys. J. 2007, 671, 955. [Google Scholar] [CrossRef]
- Liu, J.H.; Zhang, H.Q. The Magnetic Field, Horizontal Motion and Helicity in a Fast Emerging Flux Region Which Even-tually Forms a Delta Spot. Sol. Phys. 2006, 234, 21–40. [Google Scholar] [CrossRef]
- Jiang, J.; Cameron, R.H.; Schüssler, M. Effects of the Scatter in Sunspot Group Tilt Angles on the Large-Scale Magnetic Field at the Solar Surface. Astrophys. J. 2014, 791, 5. [Google Scholar] [CrossRef]
- Leka, K.D.; Canfield, R.C.; McClymont, A.N.; van Driel-Gesztelyi, L. Evidence for Current-carrying Emerging Flux. Astrophys. J. 1996, 462, 547. [Google Scholar] [CrossRef]
- Wiegelmann, T. Optimization Code With Weighting Function for the Reconstruction of Coronal Magnetic Fields. Sol. Phys. 2004, 219, 87–108. [Google Scholar] [CrossRef]
- Wiegelmann, T.; Inhester, B.; Sakurai, T. Preprocessing of Vector Magnetograph Data for a Nonlinear Force-Free Magnetic Field Reconstruction. Sol. Phys. 2006, 233, 215–232. [Google Scholar] [CrossRef]
- Török, T.; Kliem, B. Confined and Ejective Eruptions of Kink-unstable Flux Ropes. Astrophys. J. 2005, 630, L97–L100. [Google Scholar] [CrossRef]
- Zhang, Y.; Tan, B.; Liu, J.; Yu, S.; Feng, S.; Chen, J.; Yan, Y. Multiwavelength Observations of the Formation and Eruption of a Complex Filament. Astrophys. J. 2021, 910, 40. [Google Scholar] [CrossRef]
- Nindos, A.; Patsourakos, S.; Wiegelmann, T. On the Role of the Background Overlying Magnetic Field in Solar Eruptions. Astrophys. J. Lett. 2012, 748, L6. [Google Scholar] [CrossRef]
- Liu, L.J.; Liu, J.J.; Chen, J.; Wang, Y.M.; Wang, G.Q.; Zhou, Z.J.; Cui, J. The configuration and failed eruption of a complex magnetic flux rope above a δ sunspot region. Astron. Astrophys. 2021, 648, A106. [Google Scholar] [CrossRef]




Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Liu, J.; Zhang, Y.; Zheng, Y.; Liu, Y.; Chen, J. A Partial Eruption of a Sigmoid Filament in the Small Dipole Active Region 12734. Universe 2024, 10, 42. https://doi.org/10.3390/universe10010042
Liu J, Zhang Y, Zheng Y, Liu Y, Chen J. A Partial Eruption of a Sigmoid Filament in the Small Dipole Active Region 12734. Universe. 2024; 10(1):42. https://doi.org/10.3390/universe10010042
Chicago/Turabian StyleLiu, Jihong, Yin Zhang, Yuhong Zheng, Yu Liu, and Jie Chen. 2024. "A Partial Eruption of a Sigmoid Filament in the Small Dipole Active Region 12734" Universe 10, no. 1: 42. https://doi.org/10.3390/universe10010042
APA StyleLiu, J., Zhang, Y., Zheng, Y., Liu, Y., & Chen, J. (2024). A Partial Eruption of a Sigmoid Filament in the Small Dipole Active Region 12734. Universe, 10(1), 42. https://doi.org/10.3390/universe10010042







