Design, Dynamics Analysis, and Real-Time Stiffness Control of a Variable Stiffness Joint
Abstract
1. Introduction
2. Mechanical Design and Mathematical Analysis of SCM-VSJ
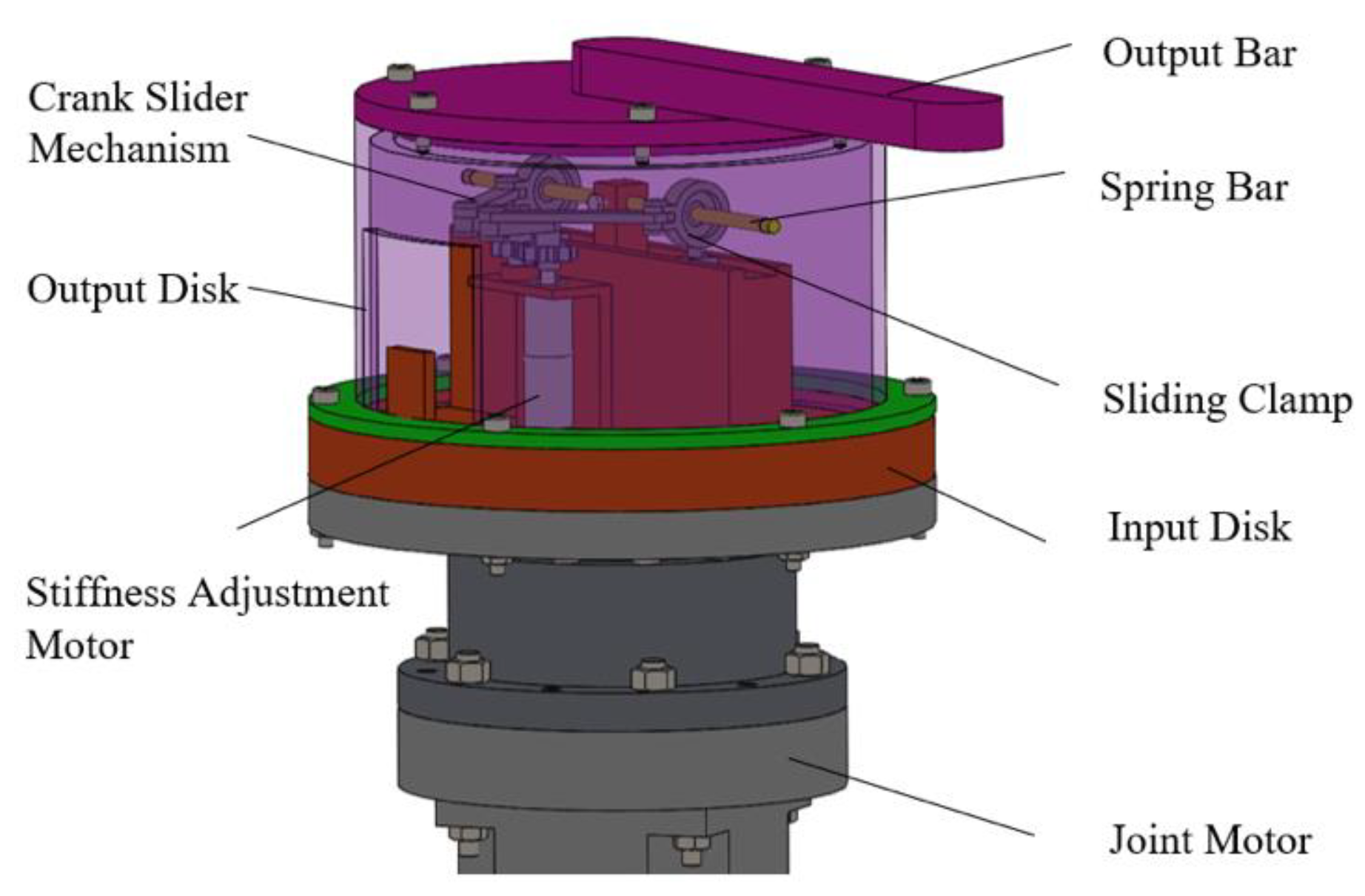
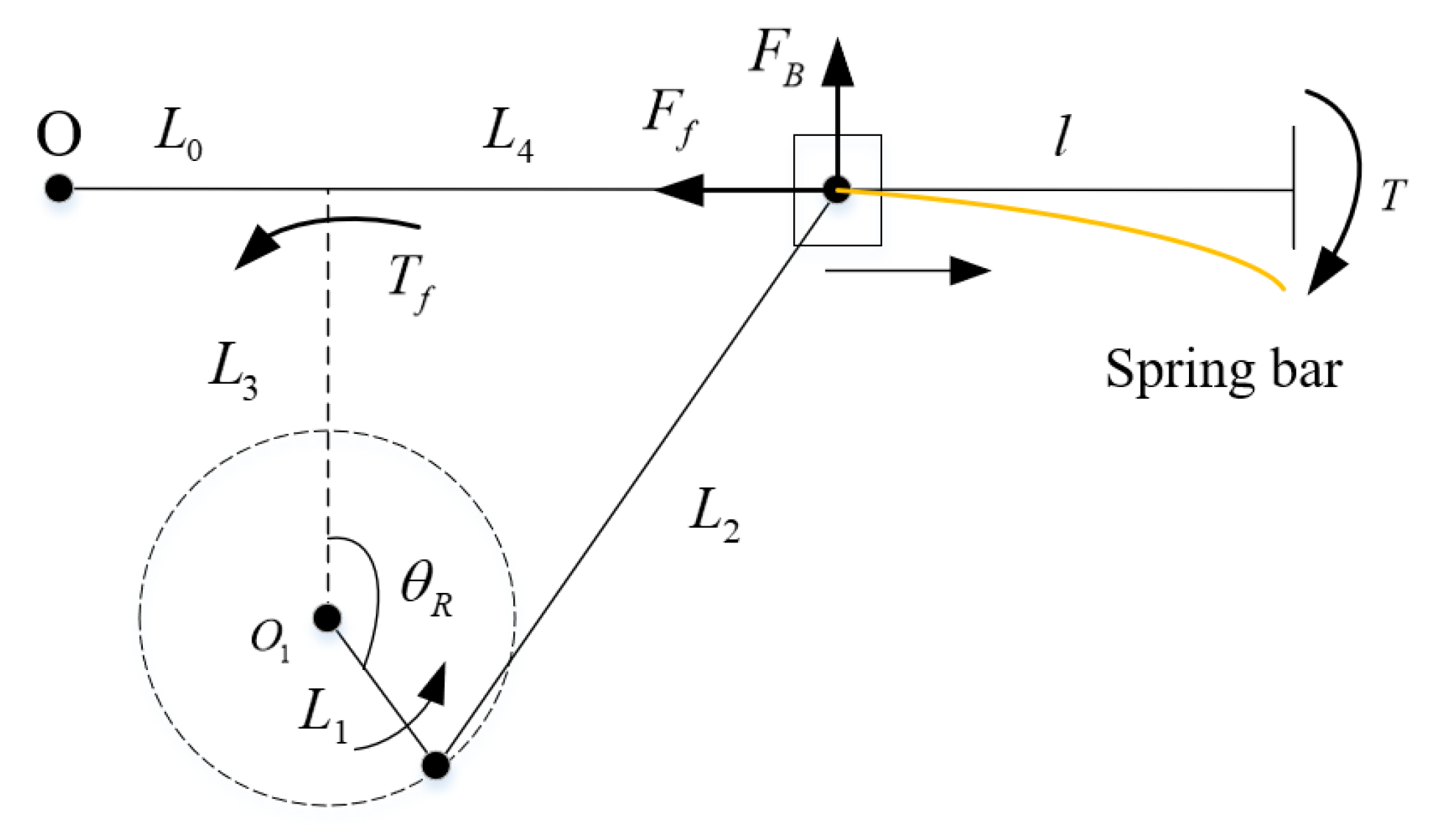
2.1. Mechanical Design
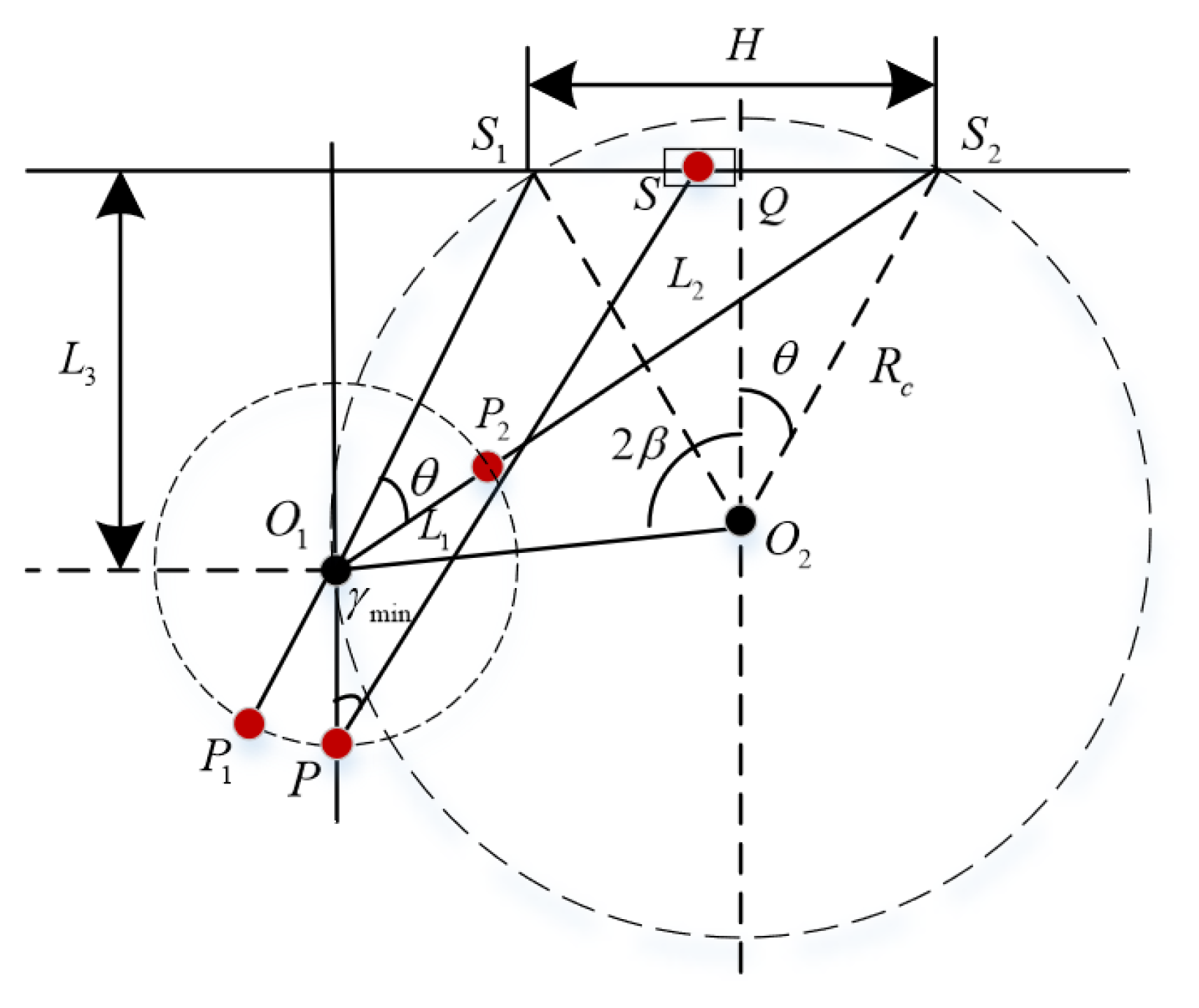
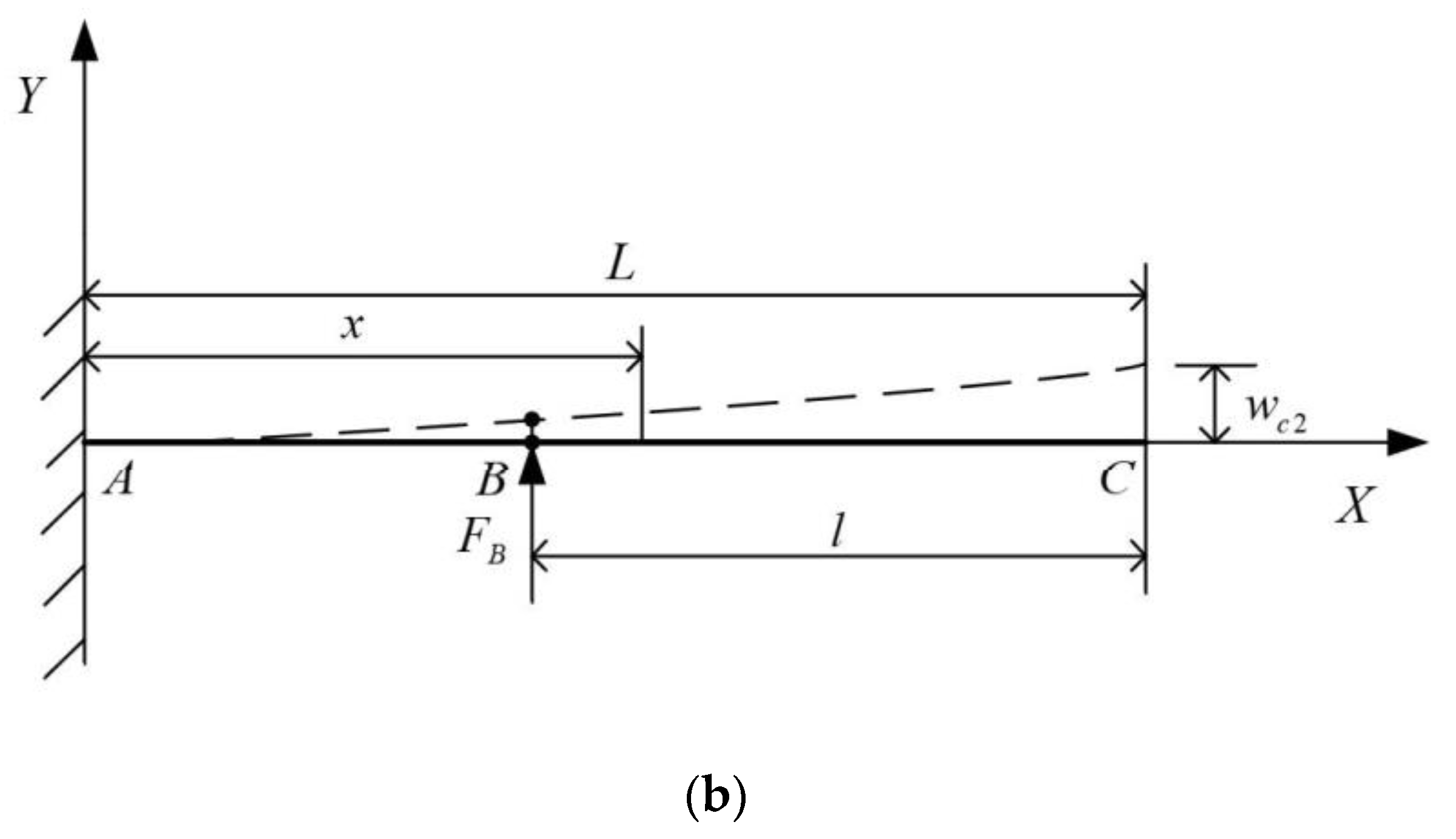
2.2. Mathematical Derivation
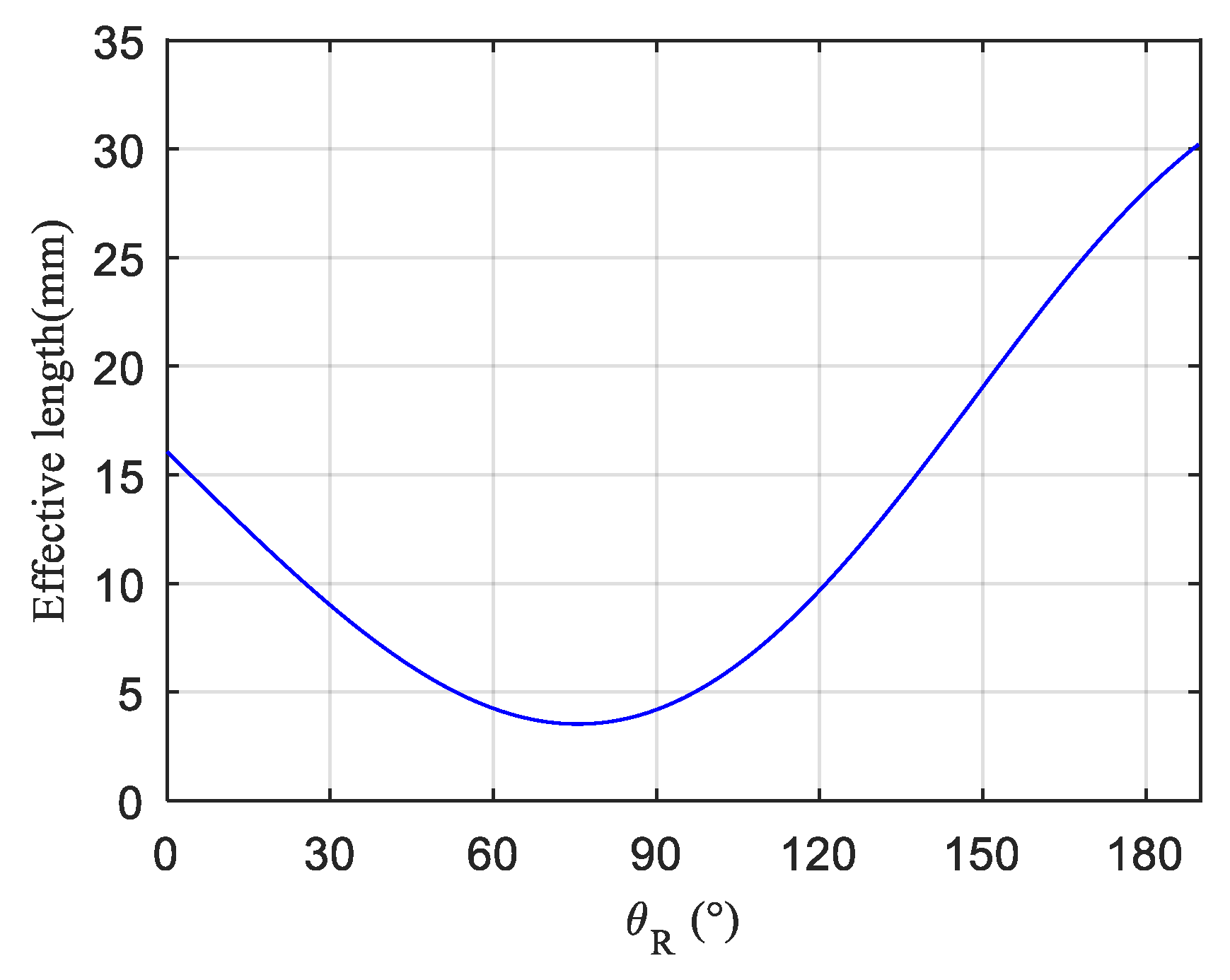
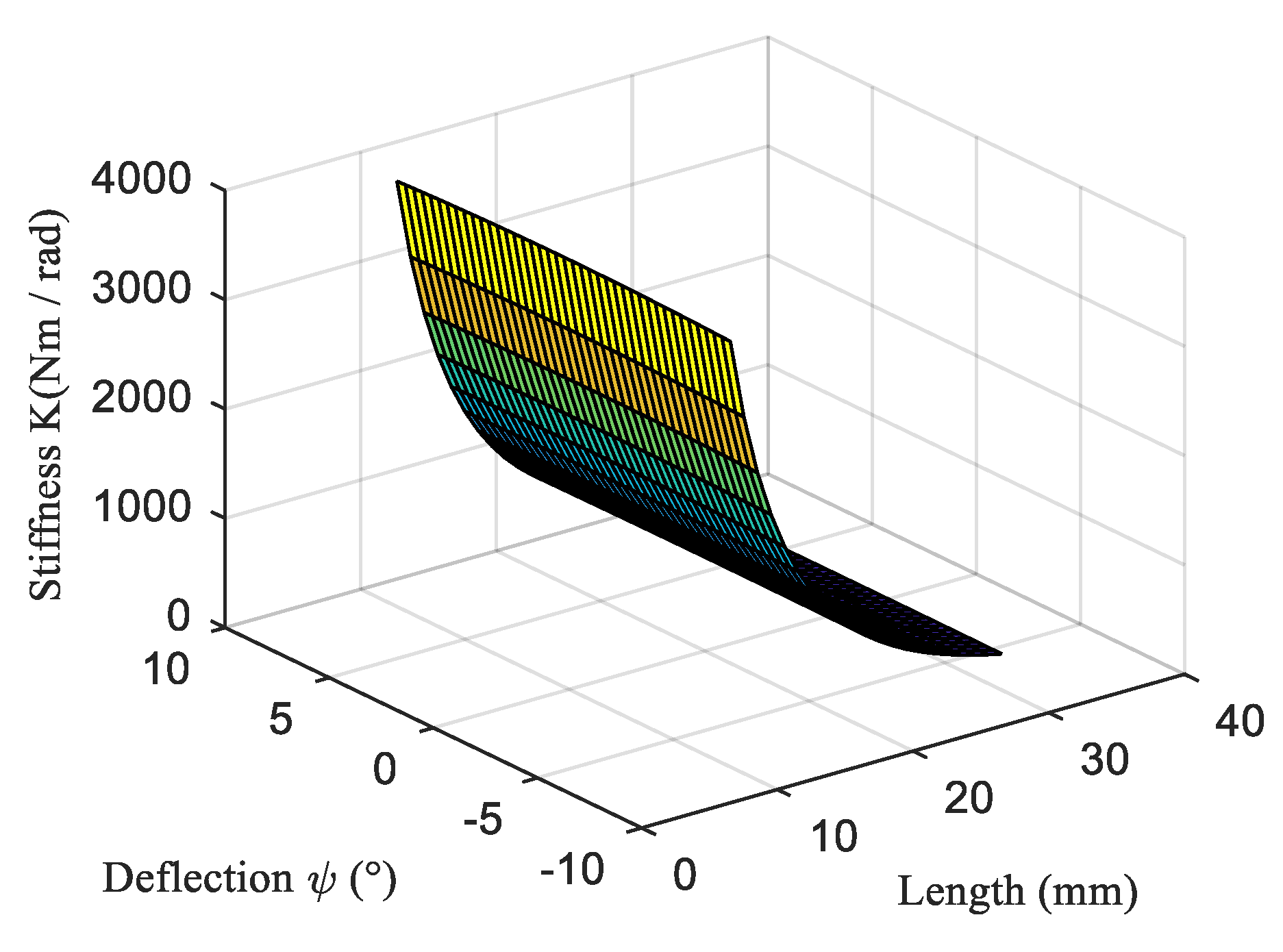
2.3. Characteristic Analysis
3. Dynamics Analysis of SCM-VSJ
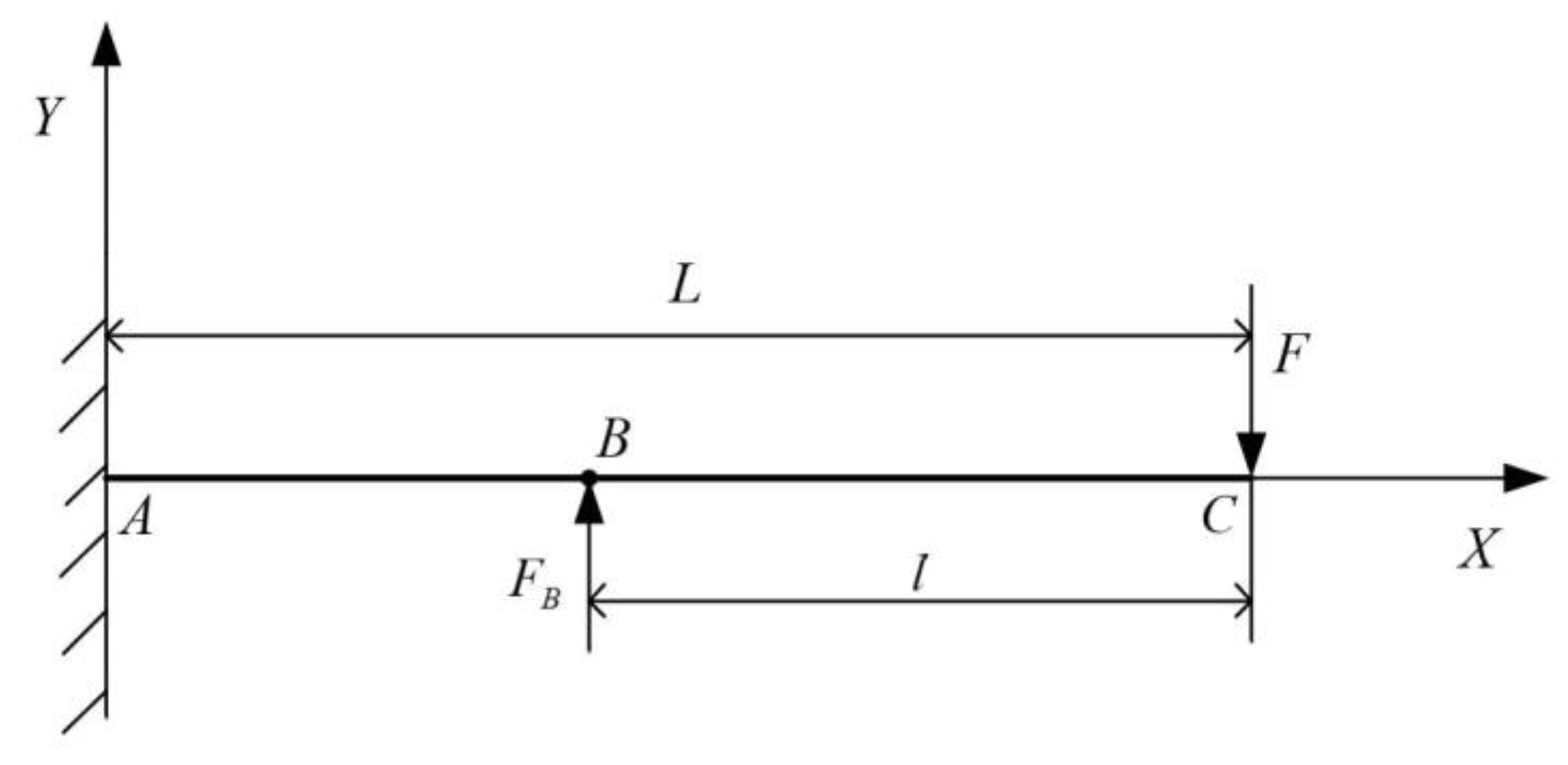
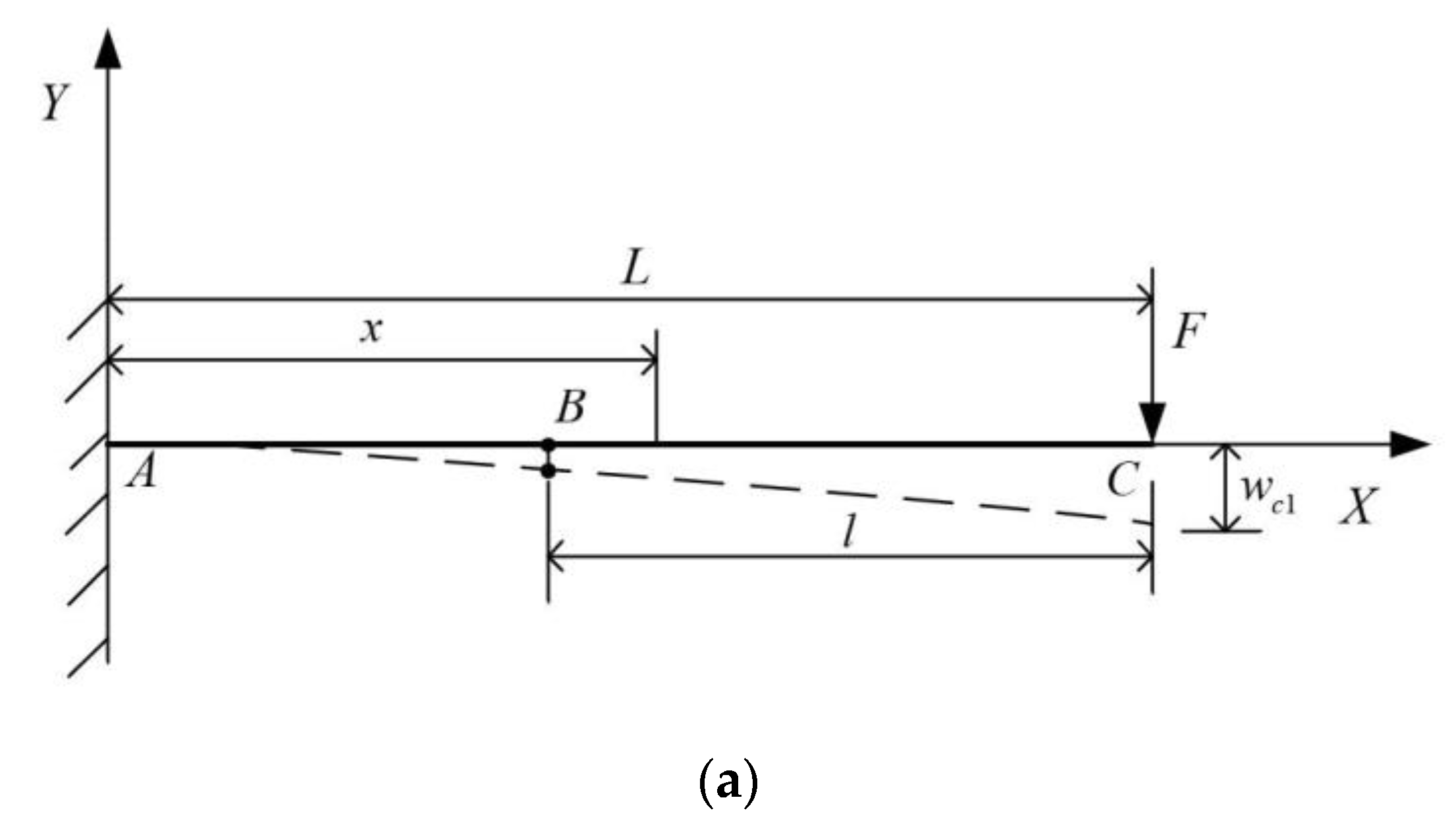
3.1. Dynamical Model
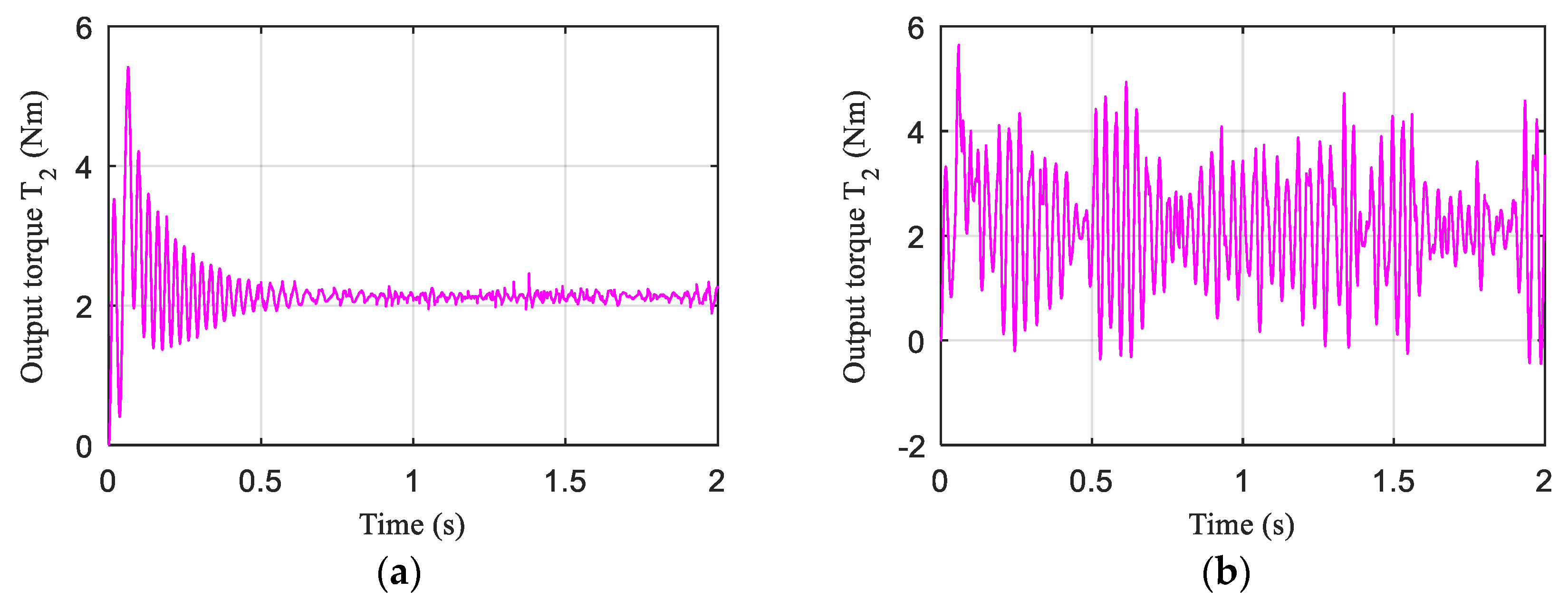
3.2. Dynamics Characteristics of SCM-VSJ
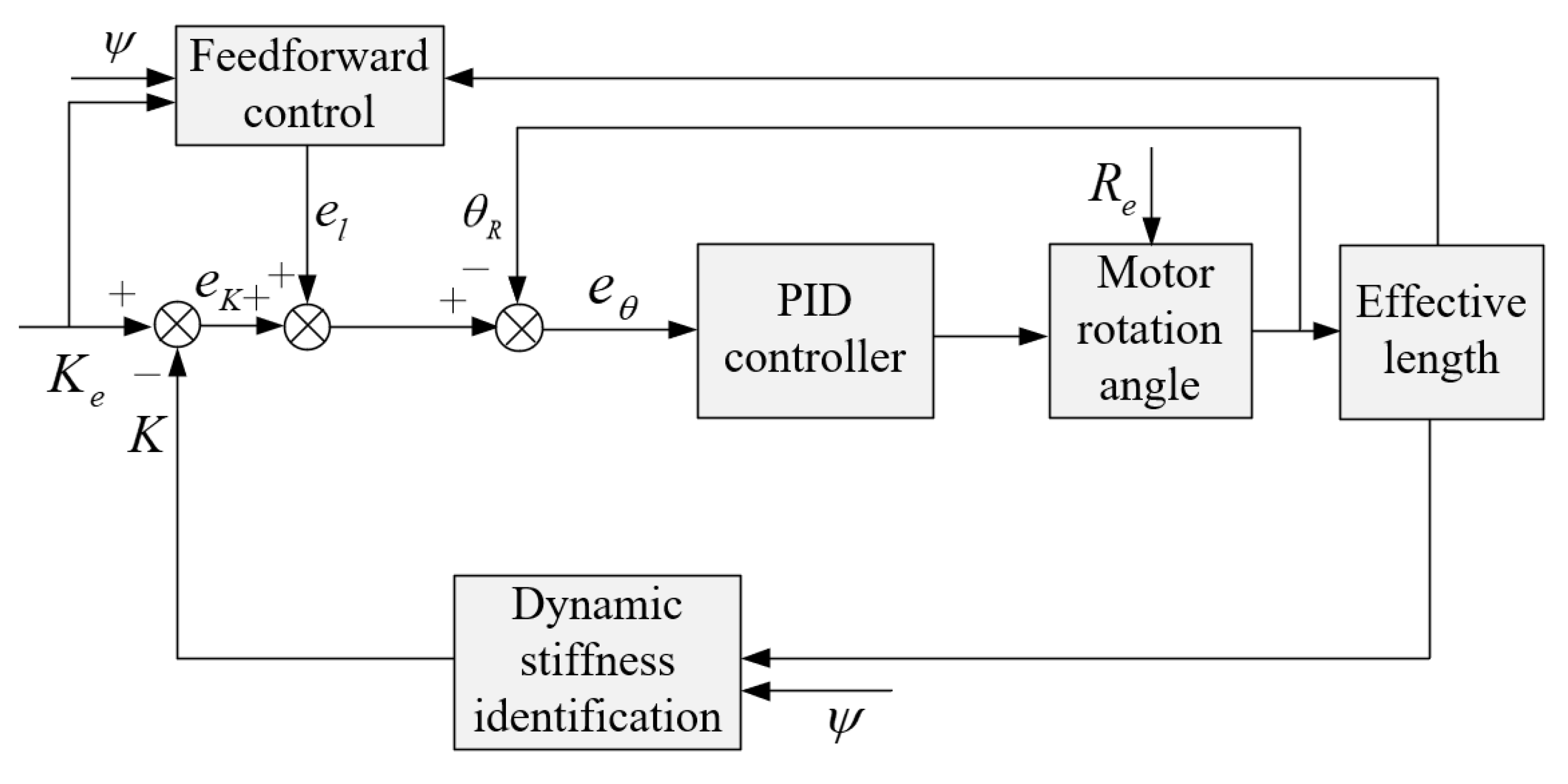
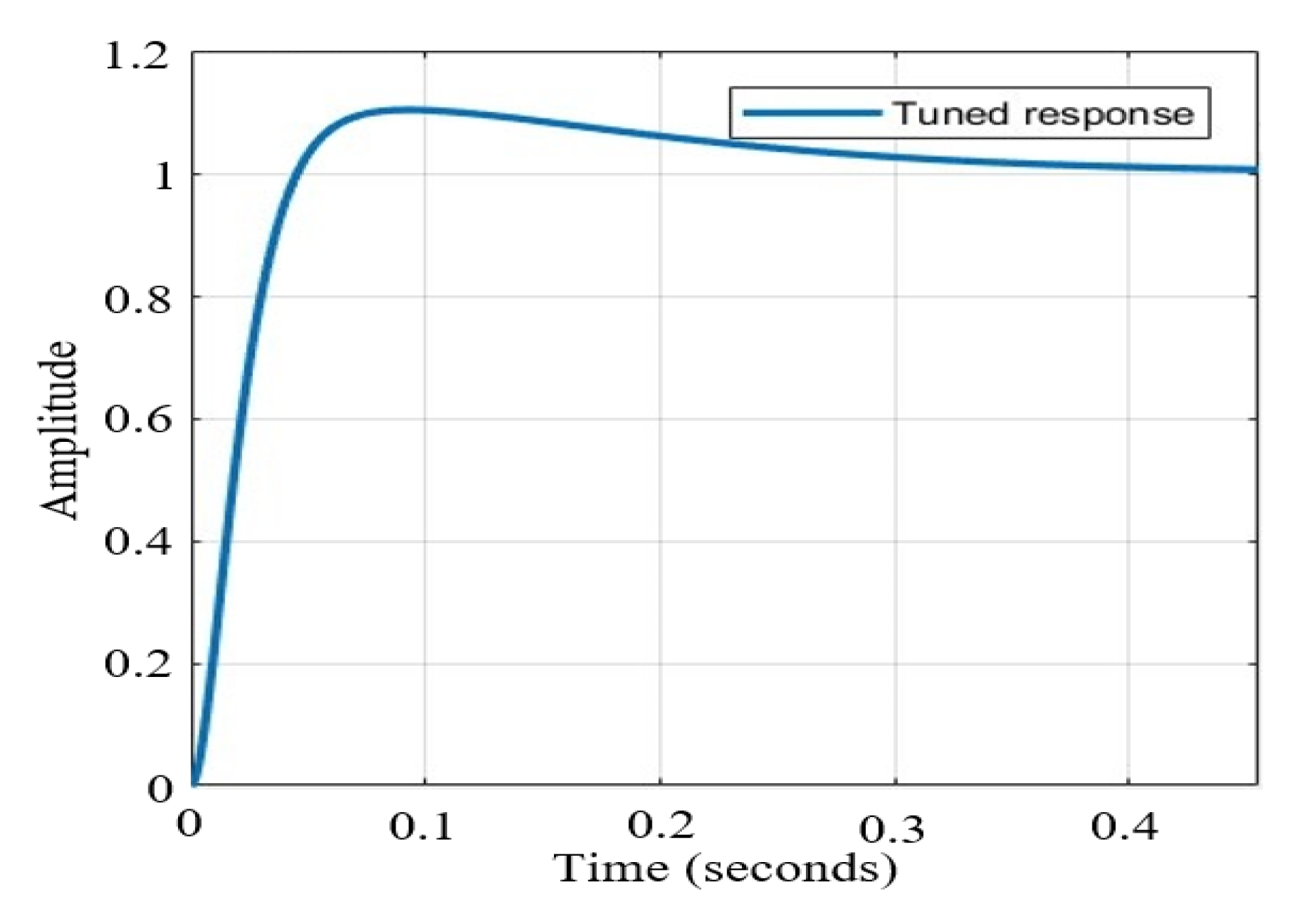
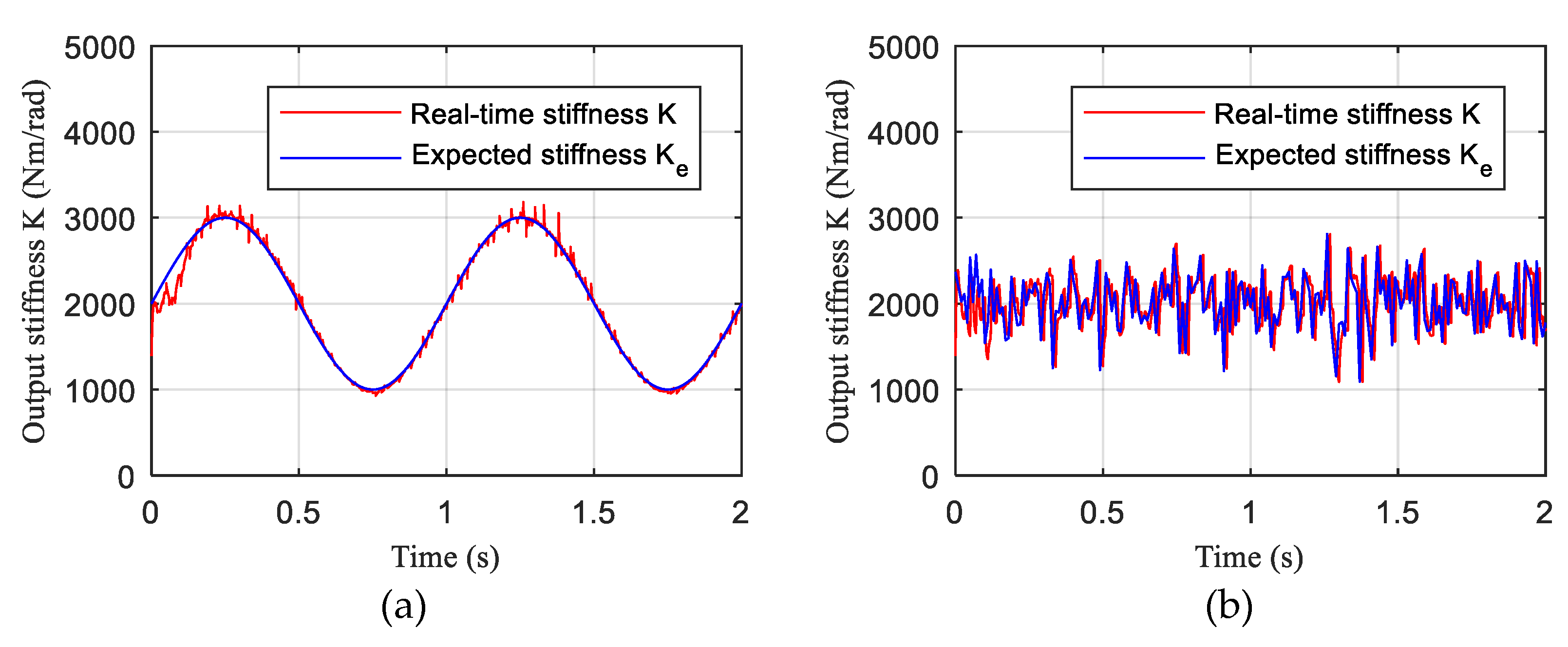
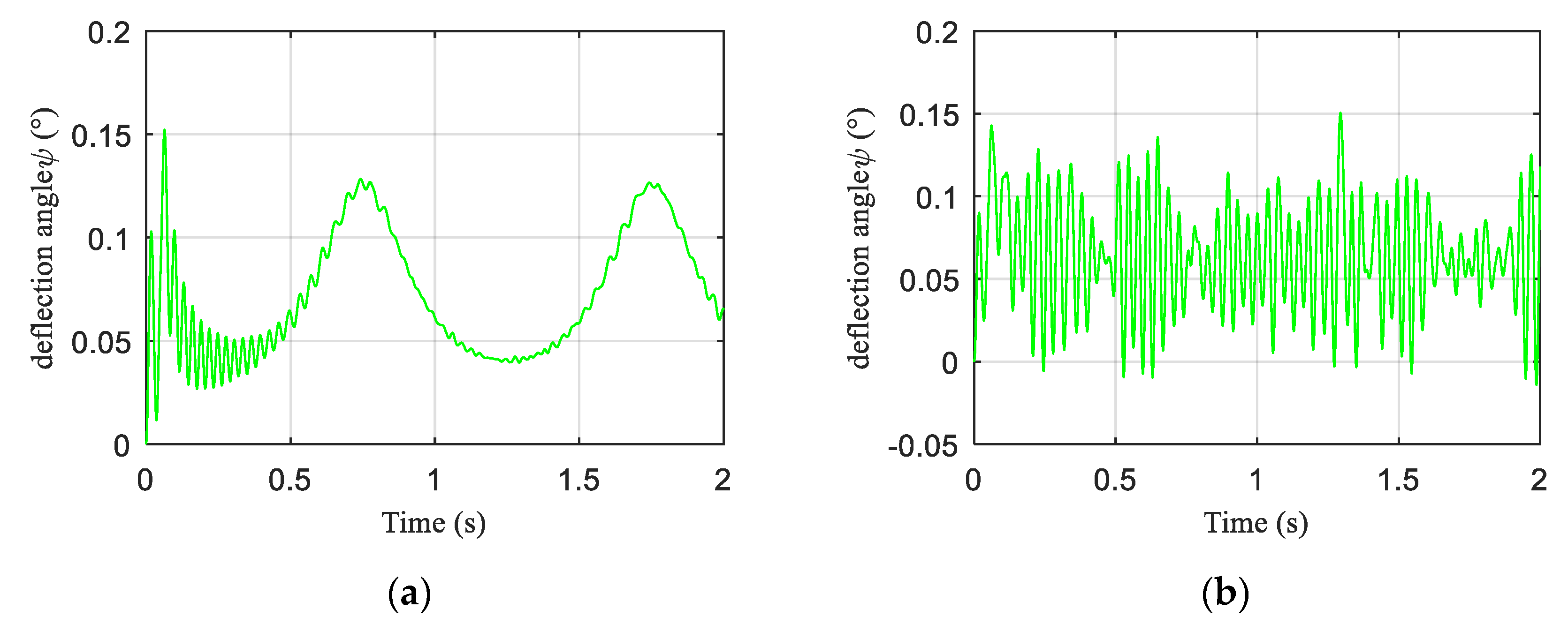
4. Real-Time Dynamics Stiffness Control of SCM-VSJ
5. Conclusions
Author Contributions
Funding
Conflicts of Interest
References
- Wolf, S.; Bahls, T.; Chalon, M.; Friedl, W.; Grebenstein, M.; Höppner, H.; Kühne, M.; Lakatos, D.; Mansfeld, N.; Özparpucu, M.C.; et al. Soft robotics with variable stiffness actuators: Tough robots for soft human robot interaction. In Soft Robotics; Verl, A., Albu-Schäffer, A., Brock, O., Raatz, A., Eds.; Springer: Berlin/Heidelberg, Germany, 2015; pp. 231–254. [Google Scholar]
- Velasco, A.; Garabini, M.; Catalano, M.G.; Bicchi, A. Soft actuation in cyclic motions: Stiffness profile optimization for energy efficiency. In Proceedings of the 2015 IEEE-RAS 15th International Conference on Humanoid Robots (Humanoids), Seoul, Korea, 3–5 November 2015; pp. 107–113. [Google Scholar]
- Chu, M.; Wu, X. Modeling and Self-learning Soft-grasp Control for Free-floating Space Manipulator during Target Capturing using Variable Stiffness Method. IEEE Access 2018, 6, 7044–7054. [Google Scholar] [CrossRef]
- Uemura, M.; Kawamura, S. Resonance-based motion control method for multi-joint robot through combining stiffness adaptation and iterative learning control. In Proceedings of the 2009 IEEE International Conference on Robotics and Automation, Kobe, Japan, 12–17 May 2009; pp. 1543–1548. [Google Scholar]
- Ye, X.; Hong, J.-C.; Dong, Z.-H. Research on Angle and Stiffness Cooperative Tracking Control of VSJ of Space Manipulator Based on LESO and NSFAR Control. Electronics 2019, 8, 893. [Google Scholar] [CrossRef]
- Pratt, G.A.; Williamson, M.M. Series elastic actuators. In Proceedings of the IEEE/RSJ International Conference on Intelligent Robots and Systems, Pittsburgh, PA, USA, 5–9 August 1995; pp. 399–406. [Google Scholar]
- van Ham, R.; Sugar, T.G.; Vanderborght, B.; Hollander, K.W.; Lefeber, D. Compliant actuator designs. IEEE Robot. Autom. Mag. 2009, 16, 81–94. [Google Scholar]
- Tagliamonte, N.L.; Sergi, F.; Accoto, D.; Carpino, G.; Guglielmelli, E. Double actuation architectures for rendering variable impedance in compliant robots: A review. Mechatronics 2012, 22, 1187–1203. [Google Scholar] [CrossRef]
- Wolf, S.; Grioli, G.; Eiberger, O.; Friedl, W.; Grebenstein, M.; Höppner, H.; Burdet, E.; Caldwell, D.G.; Carloni, R.; Catalano, M.G.; et al. Variable Stiffness Actuators: Review on Design and Components. IEEE/ASME Trans. Mechatron. 2016, 21, 2418–2430. [Google Scholar] [CrossRef]
- Tonietti, G.; Schiavi, R.; Bicchi, A. Design and control of a variable stiffness actuator for safe and fast physical human/robot interaction. In Proceedings of the IEEE Int. Conf. on Robotics and Automation, Barcelona, Spain, 18–22 April 2005; pp. 528–533. [Google Scholar]
- Hurst, J.W.; Chestnutt, J.; Rizzi, A.A. The actuator with mechanically adjustable series compliance. IEEE Trans. Robot. 2010, 26, 597–606. [Google Scholar] [CrossRef]
- Eiberger, O.; Haddadin, S.; Weis, M.; Albu-Schäffer, A.; Hirzinger, G. On joint design with intrinsic variable compliance: Derivation of the DLR QA-Joint. In Proceedings of the IEEE International Conference on Robotics and Automation (ICRA 2010), Anchorage, AK, USA, 3–7 May 2010; pp. 1687–1694. [Google Scholar]
- Wolf, S.; Hirzinger, G. A new variable stiffness design: Matching requirements of the next robot generation. In Proceedings of the IEEE International Conf. on Robotics and Automation (ICRA), Pasadena, CA, USA, 19–23 May 2008; pp. 1741–1746. [Google Scholar]
- Wolf, S.; Eiberger, O.; Hirzinger, G. The DLR FSJ: Energy based design of a variable stiffness joints. In Proceedings of the IEEE International Conference on Robotics and Automation (ICRA), Shanghai, China, 9–13 May 2011; pp. 5082–5089. [Google Scholar]
- Awad, M.I.; Gan, D.; Cempini, M.; Cortese, M.; Vitiello, N.; Dias, J.; Dario, P.; Seneviratne, L. Modeling, design and characterization of a novel passive variable stiffness joint (pVSJ). In Proceedings of the IEEE/RSJ International Conference on Intelligent Robots and Systems (IROS), Daejeon, Korea, 9–14 October 2016; pp. 323–329. [Google Scholar]
- Jafari, A.; Tsagarakis, N.; Vanderborght, B.; Caldwell, D. A novel actuator with adjustable stiffness (AwAS). In Proceedings of the IEEE/RSJ international conference on intelligent robots and systems (IROS 2010), Taipei, Taiwan, 18–22 October 2010; pp. 4201–4206. [Google Scholar]
- Jafari, A.; Tsagarakis, N.G.; Caldwell, D.G. AwAS-II: A new Actuator with Adjustable Stiffness based on the novel principle of adaptable pivot point and variable lever ratio. In Proceedings of the 2011 IEEE International Conference on Robotics and Automation, Shanghai, China, 9–13 May 2011. [Google Scholar]
- Jafari, A.; Tsagarakis, N.G.; Sardellitti, I.; Caldwell, D.G. A New Actuator with Adjustable Stiffness Based on a Variable Ratio Lever Mechanism. IEEE/ASME Trans. Mechatron. 2014, 19, 55–63. [Google Scholar] [CrossRef]
- Choi, J.; Hong, S.; Lee, W.; Kang, S.; Kim, M. A Robot Joint with Variable Stiffness Using Leaf Springs. IEEE Trans. Robot. 2011, 27, 229–238. [Google Scholar] [CrossRef]
- Groothuis, S.; Carloni, R.; Stramigioli, S. A Novel Variable Stiffness Mechanism Capable of an Infinite Stiffness Range and Unlimited Decoupled Output Motion. Actuators 2014, 3, 107–123. [Google Scholar] [CrossRef]
- Vanderborght, B.; Tsagarakis, N.G.; Van Ham, R.; Thorson, I.; Caldwell, D.G. MACCEPA 2.0: Compliant actuator used for energy efficient hopping robot Chobino1D. Auton. Robot. 2011, 31, 55–65. [Google Scholar] [CrossRef]
- Fumagalli, M.; Barrett, E.; Stramigioli, S.; Carloni, R. The mVSA-UT: A miniaturized differential mechanism for a continuous rotational variable stiffness actuator. In Proceedings of the 2012 4th IEEE RAS & EMBS International Conference on Biomedical Robotics and Biomechatronics (BioRob), Rome, Italy, 24–27 June 2012; pp. 1943–1948. [Google Scholar]
- Hussain, I.; Albalasie, A.; Awad, M.I.; Seneviratne, L.; Gan, D. Modeling, Control, and Numerical Simulations of a Novel Binary-Controlled Variable Stiffness Actuator (BcVSA). Front. Robot. AI 2018, 5, 68. [Google Scholar] [CrossRef]
- Awad, M.I.; Gan, D.; Thattamparambil, J.; Stefanini, C.; Dias, J.; Seneviratne, L. Novel passive Diskrete Variable Stiffness Joint (pDVSJ): Modeling, design, and characterization. In Proceedings of the IEEE International Conference on Robotics and Biomimetics, Qingdao, China, 3–7 December 2016; pp. 1808–1813. [Google Scholar]
- Schiavi, R.; Grioli, G.; Sen, S.; Bicchi, A. VSA-II: A novel prototype of variable stiffness actuator for safe and performing robots interacting with humans. In Proceedings of the IEEE International Conference on Robotics & Automation IEEE, Pasadena, CA, USA, 19–23 May 2008; pp. 2171–2176. [Google Scholar]
- Grioli, G.; Bicchi, A. A non-invasive, real-time method for measuring variable stiffness. In Robotics: Science and Systems Vi; Universidad De Zaragoza: Zaragoza, Spain, June 2010; Volume 6, pp. 89–96. [Google Scholar]
- Grioli, G.; Bicchi, A. A real-time parametric stiffness observer for VSA devices. In Proceedings of the 2011 IEEE International Conference on Robotics and Automation, Shanghai, China, 9–13 May 2011; pp. 5535–5540. [Google Scholar]
- Flacco, F.; de Luca, A.; Sardellitti, I.; Tsagarakis, N.G. Robust estimation of variable stiffness in flexible joints. In Proceedings of the 2011 IEEE/RSJ International Conference on Intelligent Robots and Systems, San Francisco, CA, USA, 25–30 September 2011; pp. 4026–4033. [Google Scholar]
- Zhakatayev, A.; Rubagotti, M.; Varol, H.A. Closed-Loop Control of Variable Stiffness Actuated Robots via Nonlinear Model Predictive Control. IEEE Access 2015, 3, 235–248. [Google Scholar] [CrossRef]
- Albu-Schaffer, A.; Wolf, S.; Eiberger, O.; Haddadin, S.; Petit, F.; Chalon, M. Dynamic modelling and control of variable stiffness actuators. In Proceedings of the 2010 IEEE International Conference on Robotics and Automation, Anchorage, AK, USA, 3–7 May 2010; pp. 2155–2162. [Google Scholar]
- Andrea, C.; Giovanni, F.; Massimo, R. Preconditioning Strategies for Nonlinear Conjugate Gradient Methods, Based on Quasi-Newton Updates. In Proceedings of the AIP Conference Proceedings, Pizzo Calabro, Italy, 19–25 June 2016; Sergeyev, Y., Kvasov, D., Dell’Accio, F., Mukhametzhanov, M., Eds.; Volume 1776, pp. 090007-1–090007-4. [Google Scholar]
- Al-Baali, M.; Caliciotti, A.; Fasano, G.; Roma, M. Quasi-Newton Based Preconditioning and Damped Quasi-Newton Schemes for Nonlinear Conjugate Gradient Methods. In Numerical Analysis and Optimization. NAO 2017; Al-Baali, M., Grandinetti, L., Purnama, A., Eds.; Springer Proceedings in Mathematics & Statistics; Springer: Cham, Switzerland, 2018; Volume 235. [Google Scholar]
- Groothuis, S.S.; Rusticelli, G.; Zucchelli, A.; Stramigioli, S.; Carloni, R. The Variable Stiffness Actuator vsaUT-II: Mechanical Design, Modeling, and Identification. IEEE/ASME Trans. Mechatron. 2014, 19, 589–597. [Google Scholar] [CrossRef]




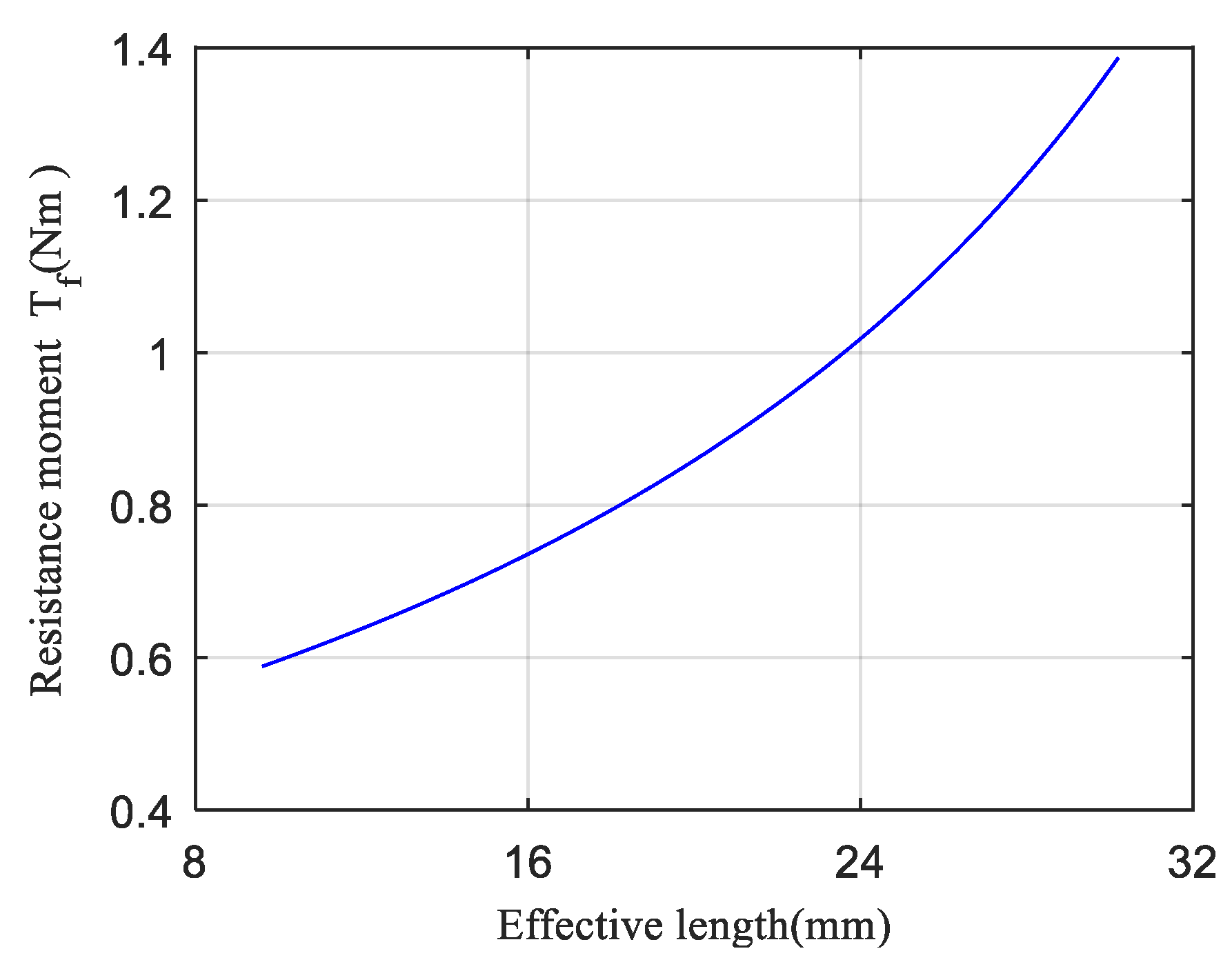
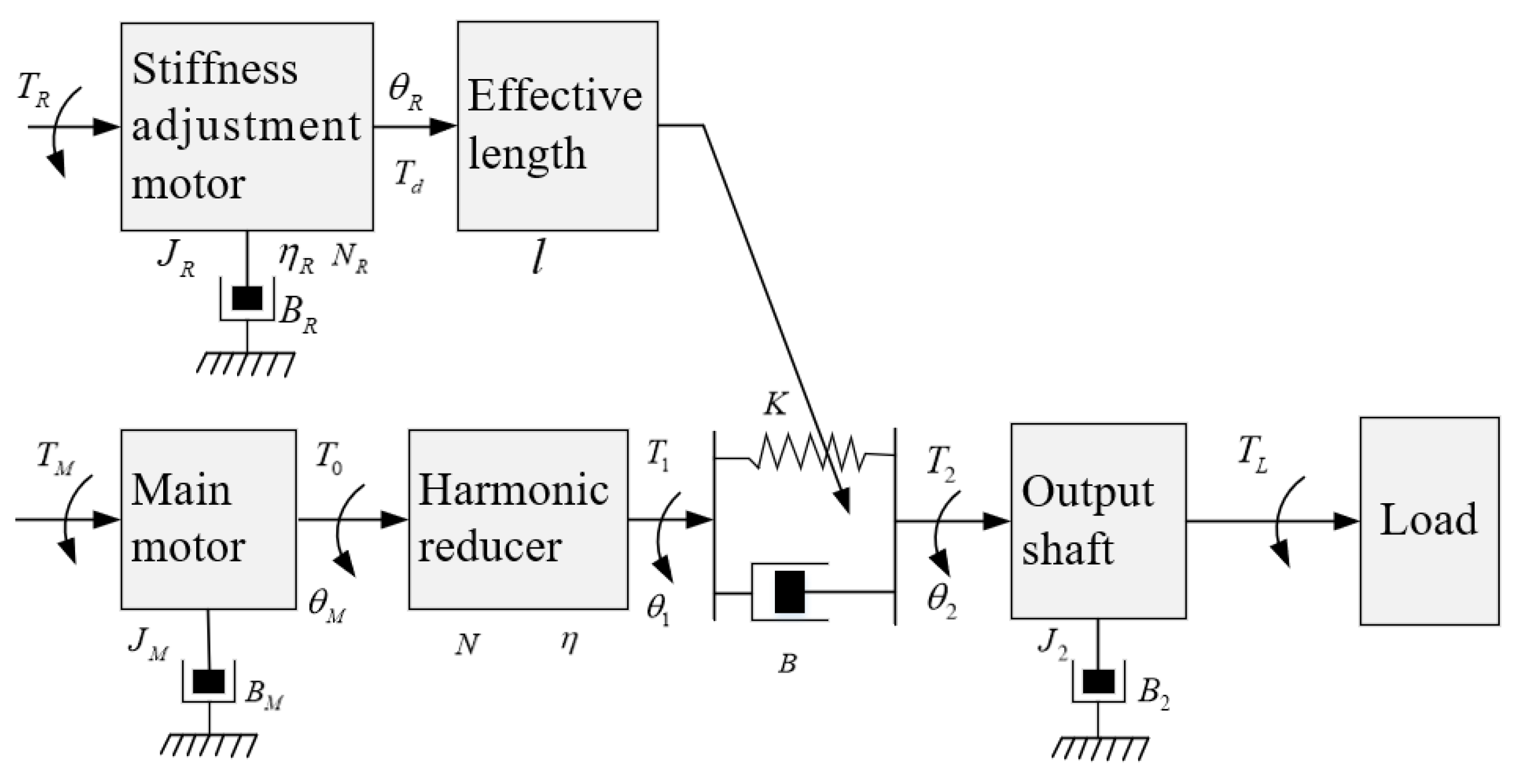
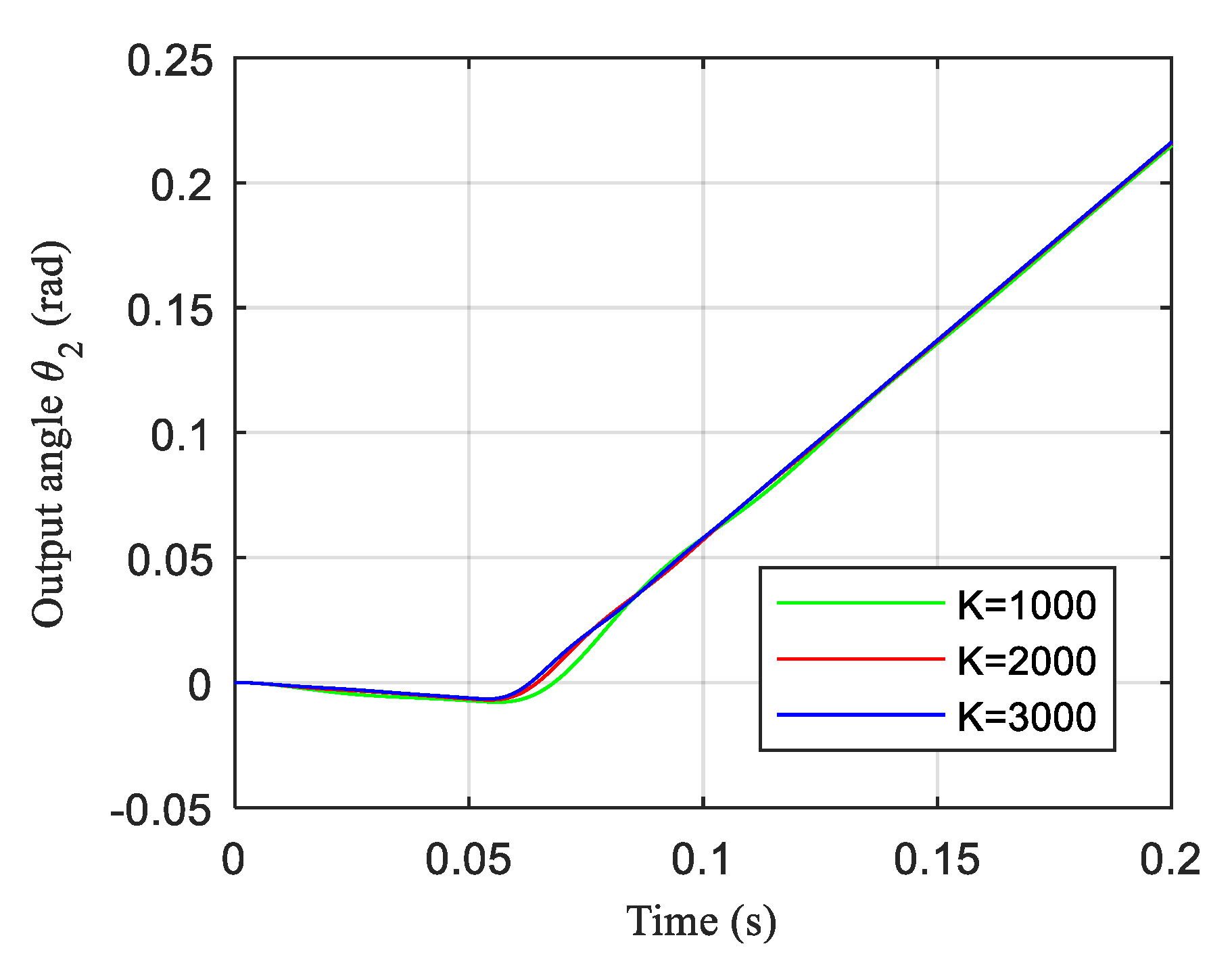

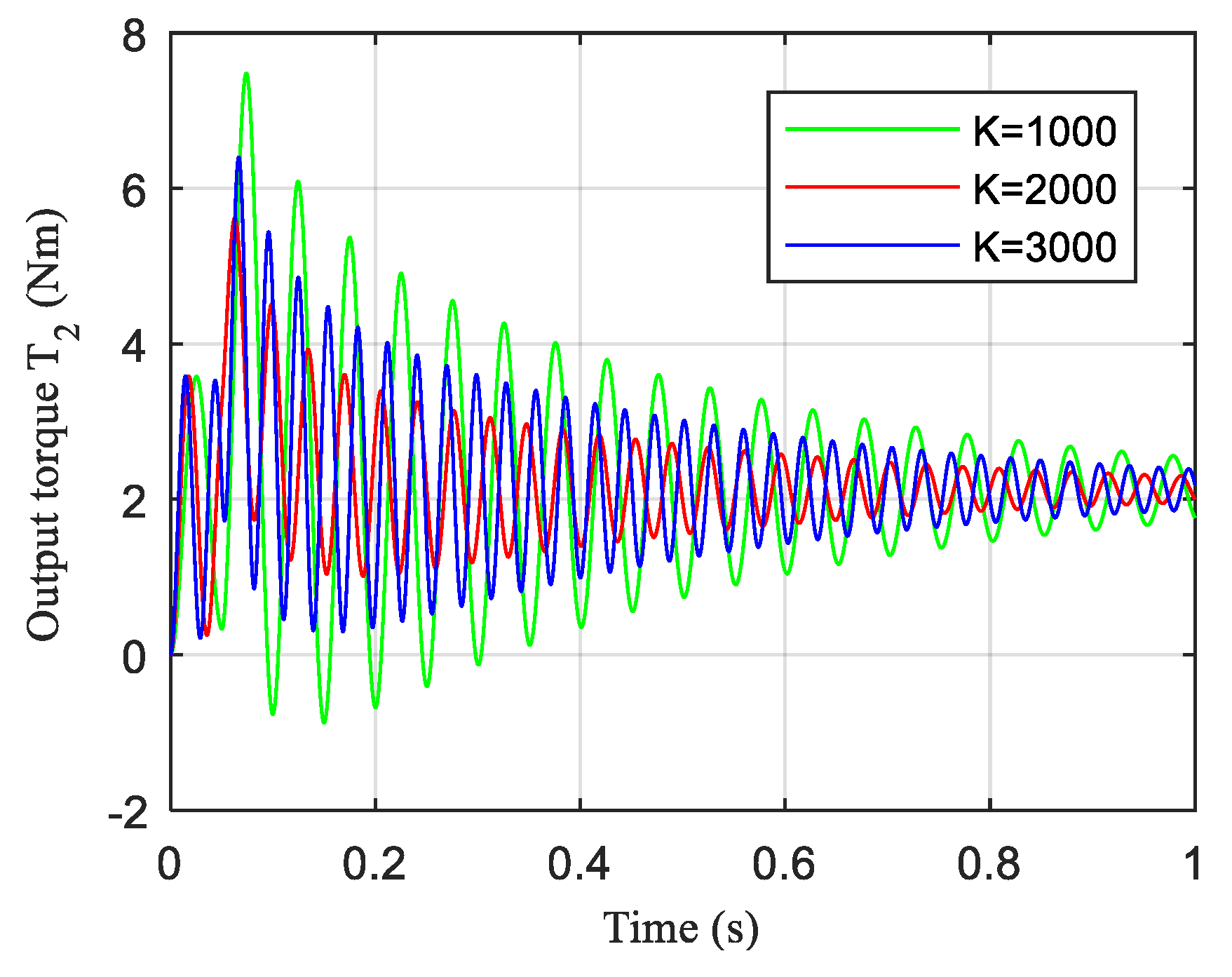

| Parameters | Value | Unit |
|---|---|---|
| Rated power of servo motor | 200 | |
| Rated torque of servo motor | 0.64 | |
| Reduction ratio of harmonic reducer | 50 | - |
| Rated torque of harmonic reducer | 25 | |
| Rated torque of DC geared motor | 0.2 | |
| Reduction ratio of DC geared motor | 19 | - |
| Speed before deceleration of DC geared motor | 395 |
| Parameters | Value | Unit |
|---|---|---|
| SCM-VSJ maximum diameter D | 130 | mm |
| Central cube side length | 8 | mm |
| SCM-VSJ passive deflection angle | [−8,8] | ° |
| Spring bar diameter | 3 | mm |
| Spring bar length | 110 | mm |
| The length from one side of the spring bar to the central cube | 51 | mm |
| Spring bar elastic modulus | 206 | |
| Horizontal distance between the joint center point O and the gear shaft | 5 | mm |
| Length of the crank | 14 | mm |
| Length of connecting rod | 34 | mm |
| The eccentricity of the crank slider mechanism | 12 | mm |
| Category | SCM-VSJ | VSJ | VsaUT-II |
|---|---|---|---|
| Size/mm | ∅130 × 260 | ∅146 × 144 | - |
| Weight/kg | 4.5 | 4.95 | 2.5 |
| Stiffness/() | [368, 3968] | [252, 3674] | [0.7, 948] |
| Deformation angle/° | [−8, 8] | [−180, 180] | [−28.6, 28.6] |
| Rated power/ | 0.2 | 0.2 | - |
| Parameters | Meaning | Unit |
|---|---|---|
| The input torque of the main motor | ||
| The electrical damping coefficient of the main motor | ||
| The input angle of the main motor | ||
| The moment of inertia of the main motor | ||
| The output torque of the main motor | ||
| The reduction ratio of the harmonic reducer | - | |
| The transmission efficiency of the harmonic reducer | - | |
| The output angle of the harmonic reducer | ||
| The output torque of the harmonic reducer | ||
| The joint stiffness | ||
| The damping coefficient of the variable stiffness module | ||
| The output torque of the flexible part | ||
| The equivalent moment of inertia of the output | ||
| The joint output angle | ||
| The damping coefficient of the output | ||
| The load torque | ||
| The input moment of the stiffness adjustment motor | ||
| The moment inertia of the stiffness adjustment motor | ||
| The damping coefficient of the stiffness adjustment motor | ||
| The angle of the stiffness adjustment motor | ||
| Transfer efficiency of stiffness adjusting motor | - | |
| Resistance moment caused by spring bar | ||
| Output torque of stiffness adjustment motor | ||
| Reduction ratio of the stiffness adjusting motor | - | |
| The joint passive deflection angle |
| Parameters | Value | Parameters | Value | Parameters | Value |
|---|---|---|---|---|---|
| 0.071 | 0.08 | ||||
| 0.1 | 0.095 | ||||
| 0.8 | 50 | ||||
| 19 | 0.8 |
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Yu, Y.; Wei, S.; Ji, Q.; Yang, Z. Design, Dynamics Analysis, and Real-Time Stiffness Control of a Variable Stiffness Joint. Electronics 2020, 9, 973. https://doi.org/10.3390/electronics9060973
Yu Y, Wei S, Ji Q, Yang Z. Design, Dynamics Analysis, and Real-Time Stiffness Control of a Variable Stiffness Joint. Electronics. 2020; 9(6):973. https://doi.org/10.3390/electronics9060973
Chicago/Turabian StyleYu, Yang, Shimin Wei, Qiunan Ji, and Zheng Yang. 2020. "Design, Dynamics Analysis, and Real-Time Stiffness Control of a Variable Stiffness Joint" Electronics 9, no. 6: 973. https://doi.org/10.3390/electronics9060973
APA StyleYu, Y., Wei, S., Ji, Q., & Yang, Z. (2020). Design, Dynamics Analysis, and Real-Time Stiffness Control of a Variable Stiffness Joint. Electronics, 9(6), 973. https://doi.org/10.3390/electronics9060973






