Thermoelectric Performance Enhancement of Naturally Occurring Bi and Chitosan Composite Films Using Energy Efficient Method
Abstract
1. Introduction
2. Materials and Methods
3. Results and Discussion
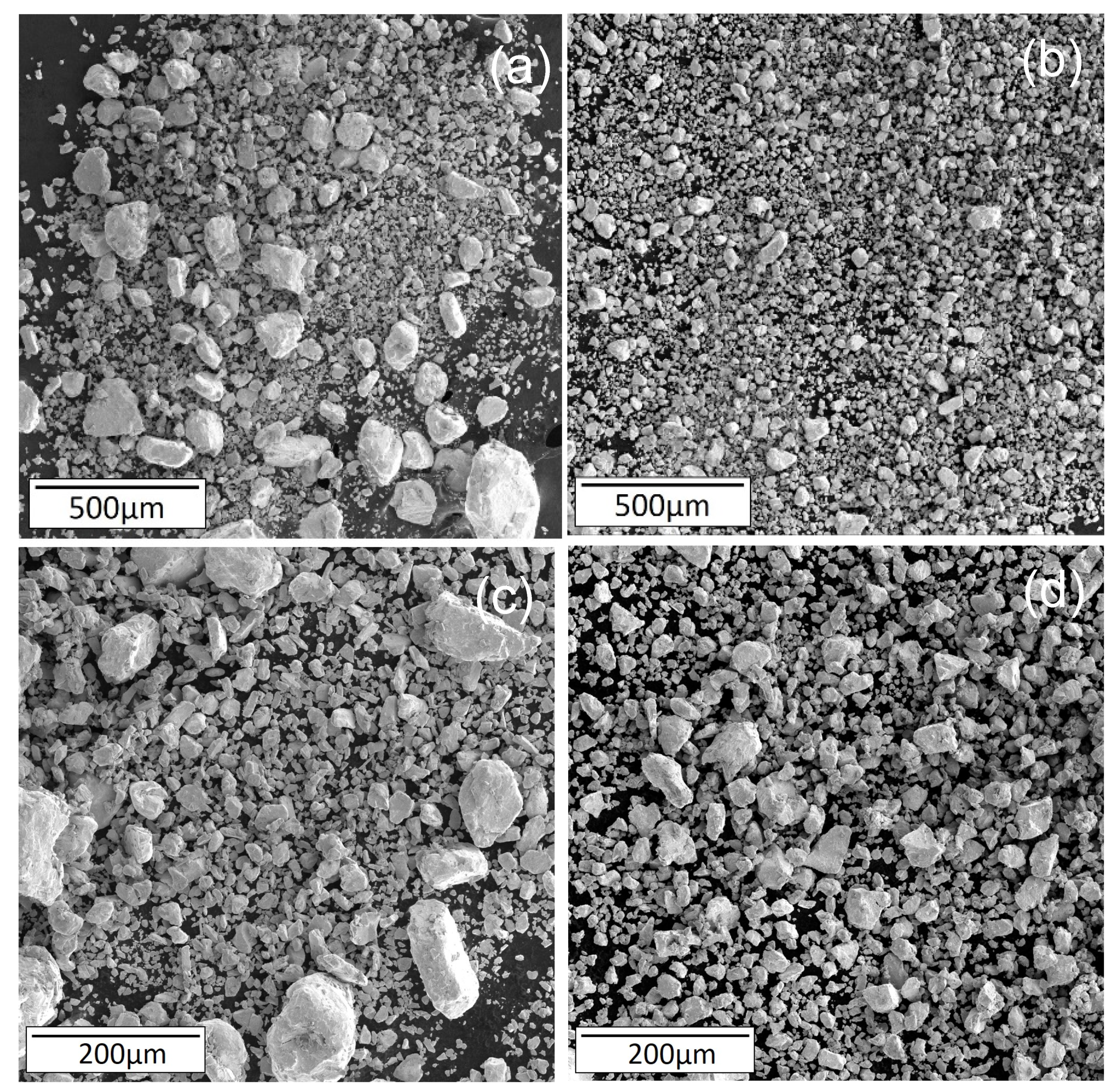
3.1. Particle Size Anaysis
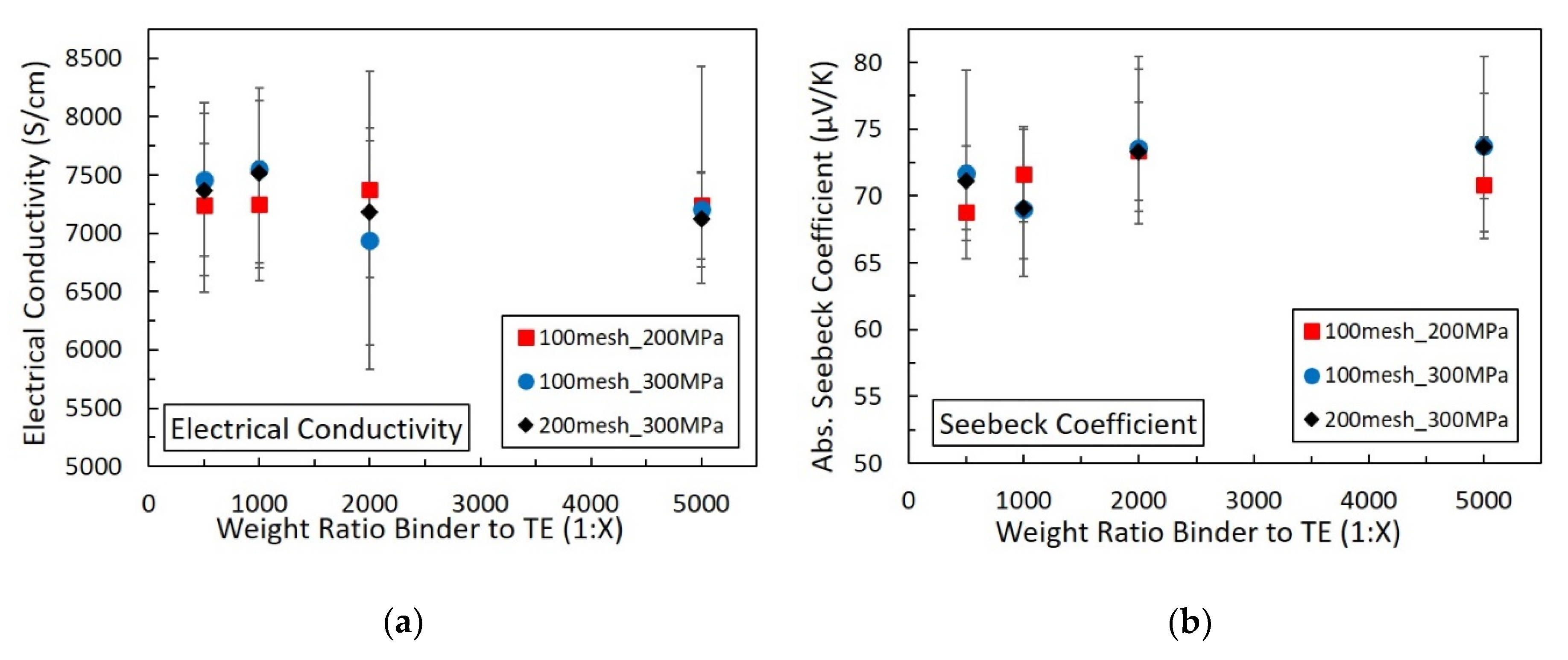
3.2. Thermoelectric Performance
3.3. Electrical Conductivity
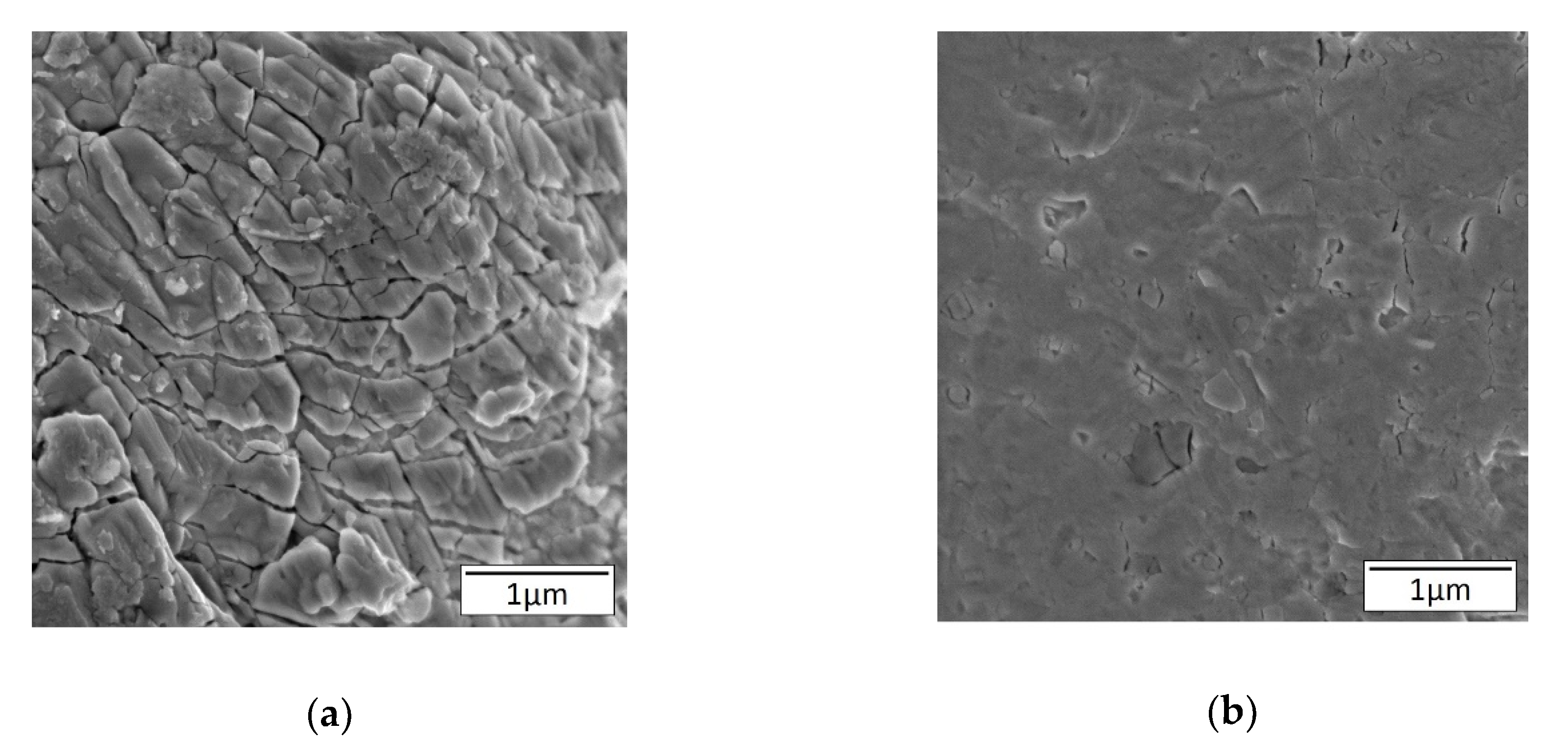
3.4. Microstructure Anaysis
3.5. Density
3.6. Seebeck Coefficient
3.7. Power Factor
3.8. Thermal Conductivity
4. Conclusions
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
References
- Kamarudin, M.A.; Sahamir, S.R.; Datta, R.S.; Long, B.D.; Mohd Sabri, M.F.; Mohd Said, S. A review on the fabrication of polymer-based thermoelectric materials and fabrication methods. Sci. World J. 2013, 2013, 713640. [Google Scholar] [CrossRef] [PubMed]
- Madavali, B.; Kim, H.; Hong, S.J. Reduction of thermal conductivity in Al2O3 dispersed p-type bismuth antimony telluride composites. Mater. Chem. Phys. 2019, 233, 9–15. [Google Scholar] [CrossRef]
- Snyder, G.J.; Toberer, E.S. Complex thermoelectric materials. Mater. Sustain. Energy Collect. Peer-Rev. Res. Rev. Artic. Nat. Publ. Gr. 2010, 7, 101–110. [Google Scholar]
- Madan, D.; Wang, Z.; Chen, A.; Wright, P.K.; Evans, J.W. High-Performance Dispenser Printed MA p-Type Bi0.5Sb1.5Te3 Flexible Thermoelectric Generators for Powering Wireless Sensor Networks. Appl. Mater. Interfaces 2013, 5, 11872–11876. [Google Scholar] [CrossRef]
- Kim, S.J.; Lee, H.E.; Choi, H.; Kim, Y.; We, J.H.; Shin, J.S.; Lee, K.J.; Cho, B.J. High-Performance Flexible Thermoelectric Power Generator Using Laser Multiscanning Lift-Off Process. ACS Nano 2016, 10, 10851–10857. [Google Scholar] [CrossRef]
- Madan, D.; Wang, Z.; Wright, P.K.; Evans, J.W. Printed flexible thermoelectric generators for use on low levels of waste heat. Appl. Energy 2015, 156, 587–592. [Google Scholar] [CrossRef]
- Choi, H.; Kim, Y.J.; Kim, C.S.; Yang, H.M.; Oh, M.W.; Cho, B.J. Enhancement of reproducibility and reliability in a high-performance flexible thermoelectric generator using screen-printed materials. Nano Energy 2018, 46, 39–44. [Google Scholar] [CrossRef]
- Shin, S.; Kumar, R.; Roh, J.W.; Ko, D.S.; Kim, H.S.; Kim, S.I.; Yin, L.; Schlossberg, S.M.; Cui, S.; You, J.M.; et al. High-Performance Screen-Printed Thermoelectric Films on Fabrics. Sci. Rep. 2017, 7, 1–9. [Google Scholar] [CrossRef]
- Lu, Z.; Layani, M.; Zhao, X.; Tan, L.P.; Sun, T.; Fan, S.; Yan, Q.; Magdassi, S.; Hng, H.H. Fabrication of flexible thermoelectric thin film devices by inkjet printing. Small 2014, 10, 3551–3554. [Google Scholar] [CrossRef]
- Snyder, A.H.S.; Jeffrey, G. Figure of merit ZT of a thermoelectric device defined from materials properties. Energy Environ. Sci. 2017, 10, 2280–2283. [Google Scholar] [CrossRef]
- Rowe, D.M. Thermoelectrics Handbook; CRC Press: Boca Raton, FL, USA, 2006; pp. 1-3–1-6. [Google Scholar]
- Ioffe, A.F. Semiconductor Thermoelements and Thermoelectric Cooling; Infosearch Ltd.: London, UK, 1957. [Google Scholar]
- Glaudell, A.M.; Cochran, J.E.; Patel, S.N.; Chabinyc, M.L. Impact of the doping method on conductivity and thermopower in semiconducting polythiophenes. Adv. Energy Mater. 2015, 5, 4. [Google Scholar] [CrossRef]
- Jang, E.; Poosapati, A.; Madan, D. Enhanced Thermoelectric Properties of F4TCNQ Doped P3HT and Its Use as a Binder for Sb2Te3 Based Printed Thermoelectric Films. ACS Appl. Energy Mater. 2018, 1, 1455–1462. [Google Scholar] [CrossRef]
- Varghese, T.; Hollar, C.; Richardson, J.; Kempf, N.; Han, C.; Gamarachchi, P.; Estrada, D.; Mehta, R.J.; Zhang, Y. High-performance and flexible thermoelectric films by screen printing solution-processed nanoplate crystals. Sci. Rep. 2016, 6, 33135. [Google Scholar] [CrossRef] [PubMed]
- Bed Poudel, Z.R.; Hao, Q.; Ma, Y.; Lan, Y.; Minnich, A.; Yu, B.; Yan, X.; Wang, D.; Muto, A.; Vashaee, D.; et al. High-Thermoelectric Performance of Nanostructured Bismuth Antimony Telluride Bulk Alloys. Science 2008, 320, 634–638. [Google Scholar] [CrossRef] [PubMed]
- Madan, D.; Wang, Z.; Chen, A.; Juang, R.; Keist, J.; Wright, P.K.; Evans, J.W. Enhanced performance of dispenser printed MA n-type Bi2Te3composite thermoelectric generators. Acs Appl. Mater. Interfaces 2012, 4, 6117–6124. [Google Scholar] [CrossRef]
- Venkatasubramanian, R.; Siivola, E.; Colpitts, T.; O’Quinn, B. Thin-film thermoelectric devices with high room-temperature figures of merit. Nature 2001, 413, 597–602. [Google Scholar] [CrossRef]
- Pei, Y.; Lalonde, A.; Iwanaga, S.; Snyder, G.J. High thermoelectric figure of merit in heavy hole dominated PbTe. Energy Environ. Sci. 2011, 4, 2085–2089. [Google Scholar] [CrossRef]
- Pei, Y.; Shi, X.; Lalonde, A.; Wang, H.; Chen, L.; Snyder, G.J. Convergence of electronic bands for high performance bulk thermoelectrics. Nature 2011, 473, 66–69. [Google Scholar] [CrossRef]
- Lee, J.H.; Lee, K.H.; Kim, S.W.; Kim, S.I.; Choi, S.M.; Kim, J.Y.; Kim, S.Y.; Roh, J.W.; Park, H.J. Doping and band engineering by vanadium to enhance the thermoelectric performance in n-type Cu0.008Bi2Te2.7Se0.3. Phys. B Condens. Matter 2017, 517, 1–5. [Google Scholar] [CrossRef]
- Madan, D.; Chen, A.; Wright, P.K.; Evans, J.W. Printed Se-doped MA n-Type Bi2Te3thick-film thermoelectric generators. J. Electron. Mater. 2012, 41, 1481–1486. [Google Scholar] [CrossRef]
- Kim, C.S.; Yang, H.M.; Lee, J.; Lee, G.S.; Choi, H.; Kim, Y.J.; Lim, S.H.; Cho, S.H.; Cho, B.J. Self-Powered Wearable Electrocardiography Using a Wearable Thermoelectric Power Generator. Energy Lett. 2018, 3, 501–507. [Google Scholar] [CrossRef]
- Choi, H.; Kim, S.J.; Kim, Y.; We, J.H.; Oh, M.W.; Cho, B.J. Enhanced thermoelectric properties of screen-printed Bi0.5Sb1.5Te3 and Bi2Te2.7Se0.3 thick films using a post annealing process with mechanical pressure. J. Mater. Chem. C 2017, 5, 8559–8565. [Google Scholar] [CrossRef]
- Madan, D.; Wang, Z.; Chen, A.; Winslow, R.; Wright, P.K.; Evans, J.W. Dispenser printed circular thermoelectric devices using Bi and Bi0.5Sb1.5Te3. Appl. Phys. Lett. 2014, 104, 2012–2016. [Google Scholar] [CrossRef]
- Cao, Z.; Koukharenko, E.; Tudor, M.J.; Torah, R.N.; Beeby, S.P. Flexible screen printed thermoelectric generator with enhanced processes and materials. Sens. Actuators A Phys. 2016, 238, 196–206. [Google Scholar] [CrossRef]
- Cao, Z.; Koukharenko, E.; Torah, R.N.; Tudor, J.; Beeby, S.P. Flexible screen printed thick film thermoelectric generator with reduced material resistivity. J. Phys. Conf. Ser. 2014, 557, 1. [Google Scholar] [CrossRef]
- Jang, E.; Poosapati, A.; Jang, N.; Hu, L.; Duffy, M.; Zupan, M.; Madan, D. Thermoelectric properties enhancement of p-type composite films using wood-based binder and mechanical pressing. Sci. Rep. 2019, 9, 1–10. [Google Scholar] [CrossRef]
- Cao, Z.; Tudor, M.J.; Torah, R.N.; Beeby, S.P. Screen Printable Flexible BiTe—SbTe-Based Composite Thermoelectric Materials on Textiles for Wearable Applications. IEEE Trans. Electron Devices 2016, 63, 4024–4030. [Google Scholar] [CrossRef]
- Kim, S.J.; Choi, H.; Kim, Y.; We, J.H.; Shin, J.S.; Lee, H.E.; Oh, M.W.; Lee, K.J.; Cho, B.J. Post ionized defect engineering of the screen-printed Bi2Te2.7Se0.3 thick film for high performance flexible thermoelectric generator. Nano Energy 2017, 31, 258–263. [Google Scholar] [CrossRef]
- Choi, H.; Kim, Y.J.; Song, J.; Kim, C.S.; Lee, G.S.; Kim, S.; Park, J.; Yim, S.H.; Park, S.H.; Hwang, H.R.; et al. Post ionized defect engineering of the screen-printed Bi2Te2.7Se0.3 thick film for high performance flexible thermoelectric generator. Nano Energy 2016, 31, 258–263. [Google Scholar]
- Kishimoto, K.; Koyanagi, T. Preparation of sintered degenerate n-type PbTe with a small grain size and its thermoelectric properties. J. Appl. Phys. 2002, 92, 2544–2549. [Google Scholar] [CrossRef]
- Zhao, L.D.; Zhang, B.P.; Liu, W.S.; Li, J.F. Effect of mixed grain sizes on thermoelectric performance of Bi2Te3 compound. J. Appl. Phys. 2009, 105, 2. [Google Scholar] [CrossRef]
- Takashiri, M.; Miyazaki, K.; Tanaka, S.; Kurosaki, J.; Nagai, D.; Tsukamoto, H. Effect of grain size on thermoelectric properties of n-type nanocrystalline bismuth-telluride based thin films. J. Appl. Phys. 2008, 104, 084302. [Google Scholar] [CrossRef]
- Bismuth. Available online: https://en.wikipedia.org/wiki/Bismuth (accessed on 14 December 2019).
- Hostler, S.R.; Qu, Y.Q.; Demko, M.T.; Abramson, A.R.; Qiu, X.; Burda, C. Thermoelectric properties of pressed bismuth nanoparticles. Superlattices Microstruct. 2008, 43, 195–207. [Google Scholar] [CrossRef]
- Braun, J.L.; Olson, D.H.; Gaskins, J.T.; Hopkins, P.E. A steady-state thermoreflectance method to measure thermal conductivity. Rev. Sci. Instrum. 2019, 90, 024905. [Google Scholar] [CrossRef] [PubMed]
- Payot, F.; Furdin, G.; Celzard, A.; Mareche, J.F. Electrical conductivity of carbonaceous powders. Carbon 2002, 40, 2801–2815. [Google Scholar]
- Mamunya, Y.P.; Davydenko, V.V.; Pissis, P.; Lebedev, E.V. Electrical and thermal conductivity of polymers filled with metal powders. Eur. Polym. J. 2002, 38, 1887–1897. [Google Scholar] [CrossRef]
- Braun, J.L.; Szwejkowski, C.J.; Giri, A.; Hopkins, P.E. On the steady-state temperature rise during laser heating of multilayer thin films in optical pump-probe techniques. J. Heat Transf. 2018, 140, 1–10. [Google Scholar] [CrossRef]
- Braun, J.L.; Hopkins, P.E. Upper limit to the thermal penetration depth during modulated heating of multilayer thin films with pulsed and continuous wave lasers: A numerical study. J. Appl. Phys. 2017, 121, 175107. [Google Scholar] [CrossRef]
- Chen, A.; Madan, D.; Wright, P.K.; Evans, J.W. Dispenser-printed planar thick-film thermoelectric energy generators. J. Micromech. Microeng. 2011, 21, 104006. [Google Scholar] [CrossRef]
- Kim, W.; Zide, J.; Gossard, A.; Klenov, D.; Stemmer, S.; Shakouri, A.; Majumda, A. Thermal conductivity reduction and thermoelectric figure of merit increase by embedding nanoparticles in crystalline semiconductors. Phys. Rev. Lett. 2006, 96, 1–4. [Google Scholar] [CrossRef]
- Yu, B.; Zebarjadi, M.; Wang, H.; Lukas, K.; Wang, H.; Wang, D.; Opeil, C.; Dresselhaus, M.; Chen, G.; Ren, Z. Enhancement of thermoelectric properties by modulation-doping in silicon germanium alloy nanocomposites. Nano Lett. 2012, 12, 2077–2082. [Google Scholar] [CrossRef] [PubMed]


| Thermoelectric Material | Wt. Ratio (Binder: Particle) | Particle Mesh Size | Applied Pressure (MPa) | Electrical Conductivity (S/cm) | Seebeck Coefficient (μV/K) | Power Factor (μW/m·K2) | Thermal Conductivity (W/m·K) | Figure of Merit |
|---|---|---|---|---|---|---|---|---|
| Bismuth | 1:2000 | 100 | 300 | 7370 ± 520 | −73 ± 3 | 4010 ± 390 | 4.4 ± 0.8 | 0.27 |
| Bismuth | 1:2000 | 200 | 300 | 7200 ± 640 | −73 ± 4 | 3890 ± 450 | - | - |
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Jang, E.; Banerjee, P.; Huang, J.; Holley, R.; Gaskins, J.T.; Hoque, M.S.B.; Hopkins, P.E.; Madan, D. Thermoelectric Performance Enhancement of Naturally Occurring Bi and Chitosan Composite Films Using Energy Efficient Method. Electronics 2020, 9, 532. https://doi.org/10.3390/electronics9030532
Jang E, Banerjee P, Huang J, Holley R, Gaskins JT, Hoque MSB, Hopkins PE, Madan D. Thermoelectric Performance Enhancement of Naturally Occurring Bi and Chitosan Composite Films Using Energy Efficient Method. Electronics. 2020; 9(3):532. https://doi.org/10.3390/electronics9030532
Chicago/Turabian StyleJang, Eunhwa, Priyanshu Banerjee, Jiyuan Huang, Rudolph Holley, John T. Gaskins, Md Shafkat Bin Hoque, Patrick E. Hopkins, and Deepa Madan. 2020. "Thermoelectric Performance Enhancement of Naturally Occurring Bi and Chitosan Composite Films Using Energy Efficient Method" Electronics 9, no. 3: 532. https://doi.org/10.3390/electronics9030532
APA StyleJang, E., Banerjee, P., Huang, J., Holley, R., Gaskins, J. T., Hoque, M. S. B., Hopkins, P. E., & Madan, D. (2020). Thermoelectric Performance Enhancement of Naturally Occurring Bi and Chitosan Composite Films Using Energy Efficient Method. Electronics, 9(3), 532. https://doi.org/10.3390/electronics9030532






