A Fractional-Order Kinetic Battery Model of Lithium-Ion Batteries Considering a Nonlinear Capacity
Abstract
:1. Introduction
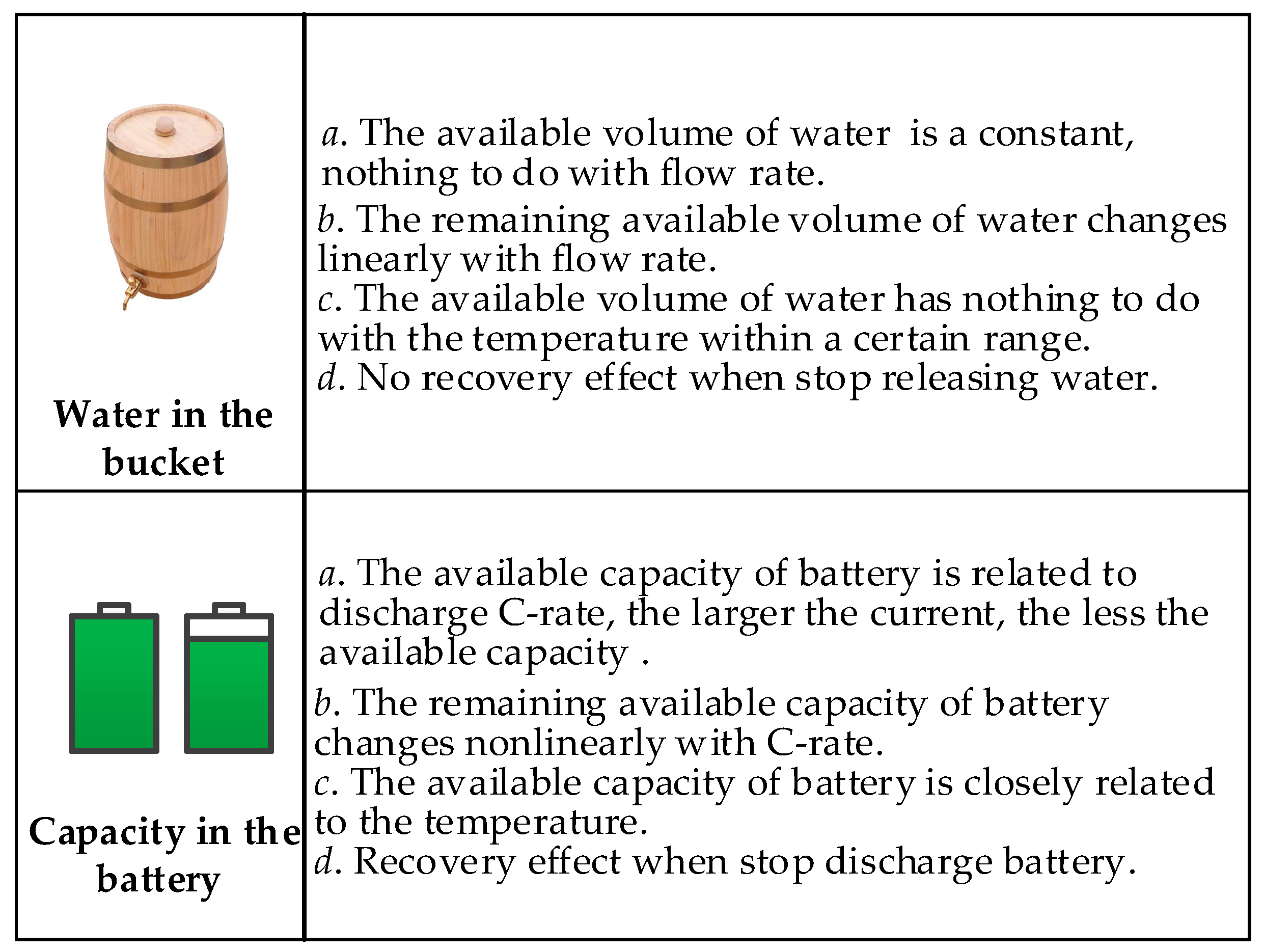
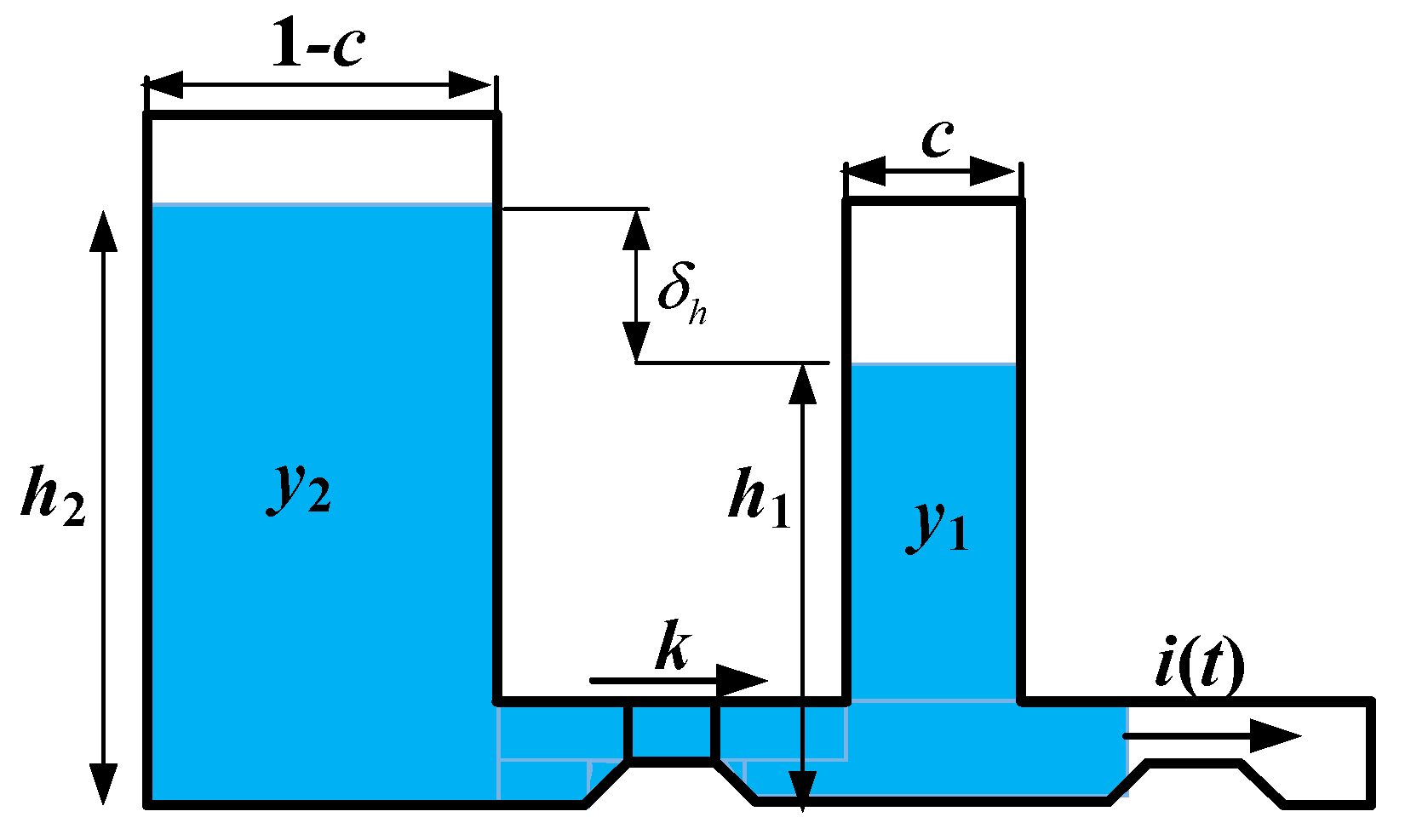
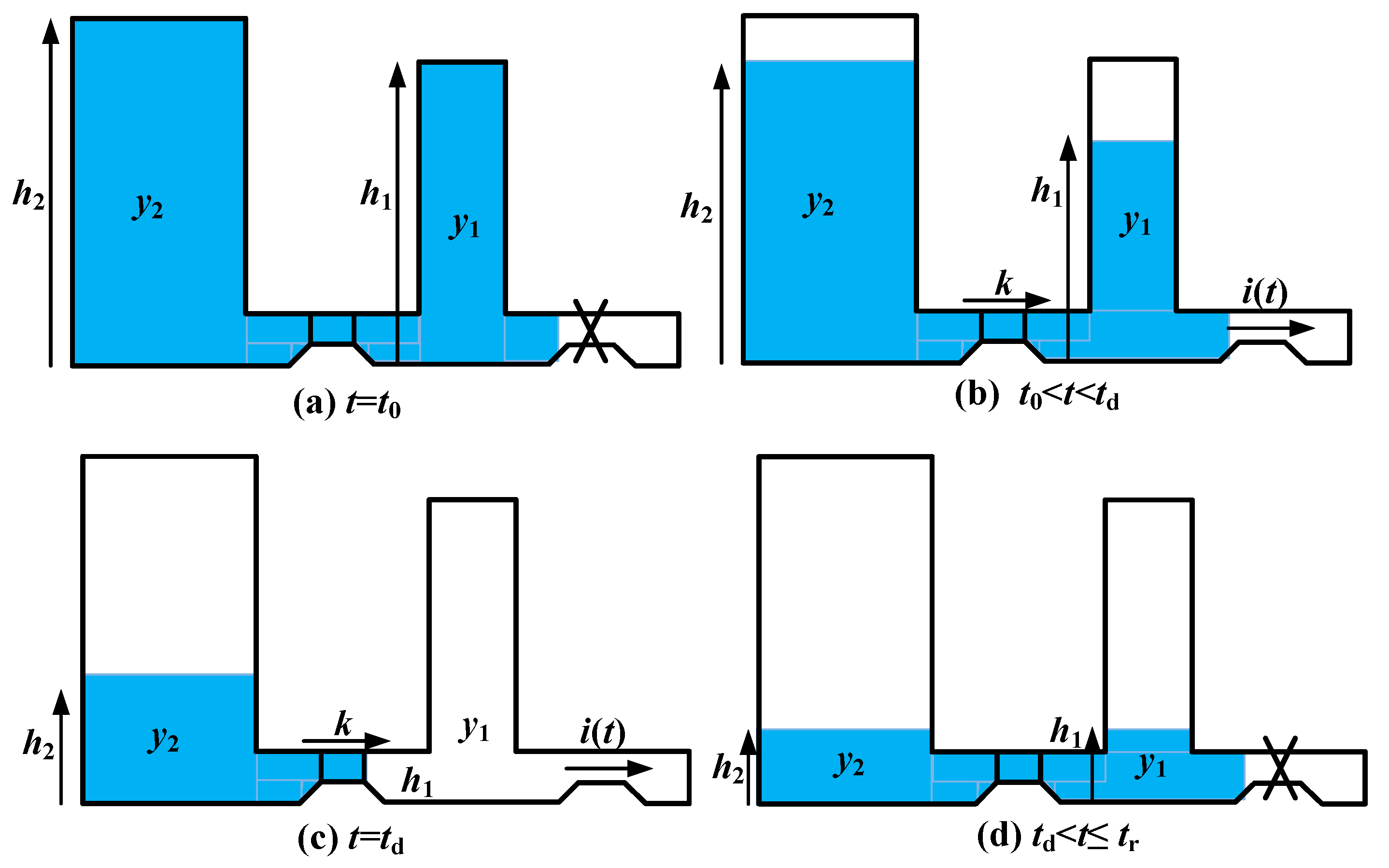
2. Kinetic Battery Model (KiBaM)
3. The Proposed Fractional-Order KiBaM (FO-KiBaM)
3.1. Fractional Calculus Theory
3.2. Fractional-Order KiBaM
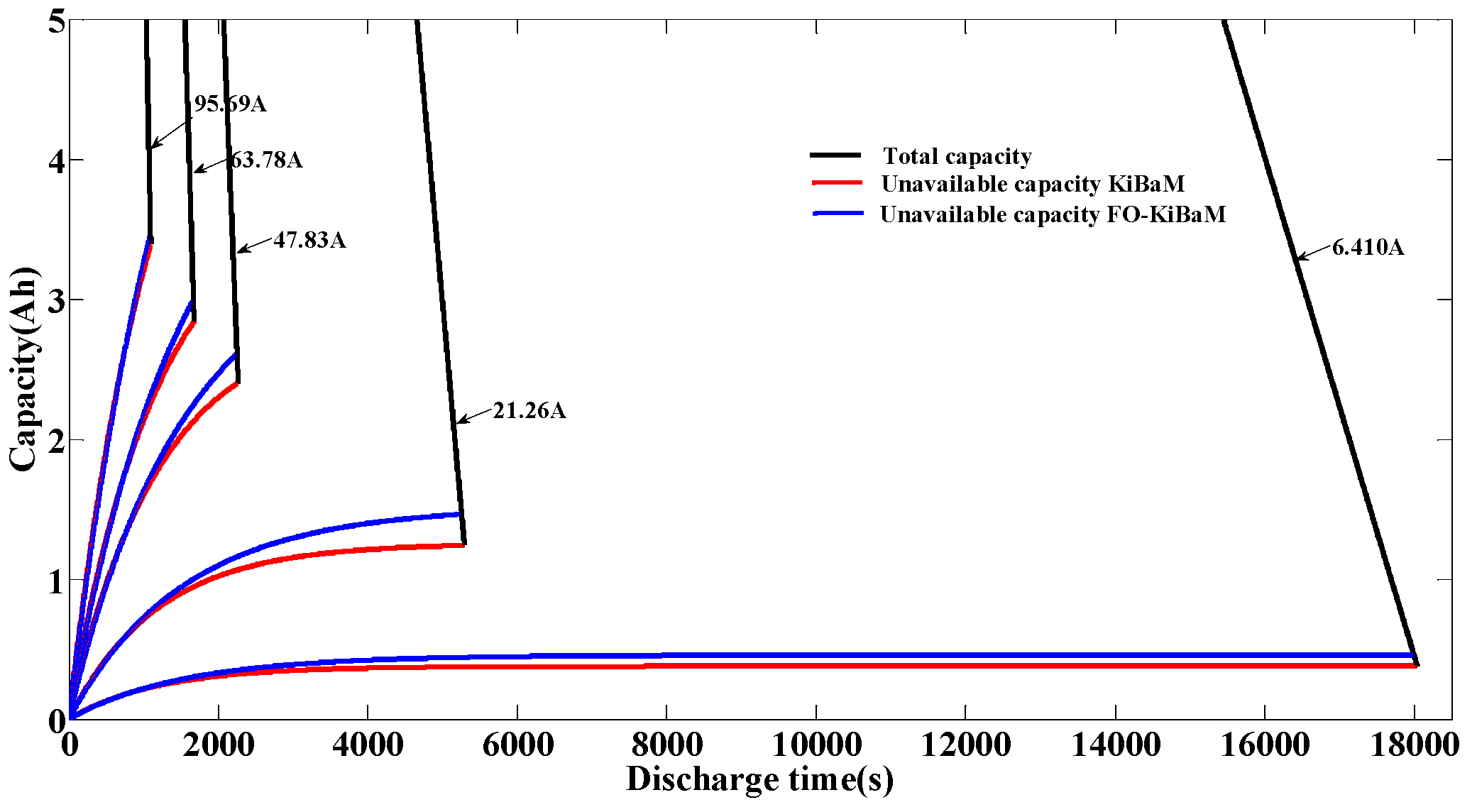
4. Parameter Identification and Experiment Verification
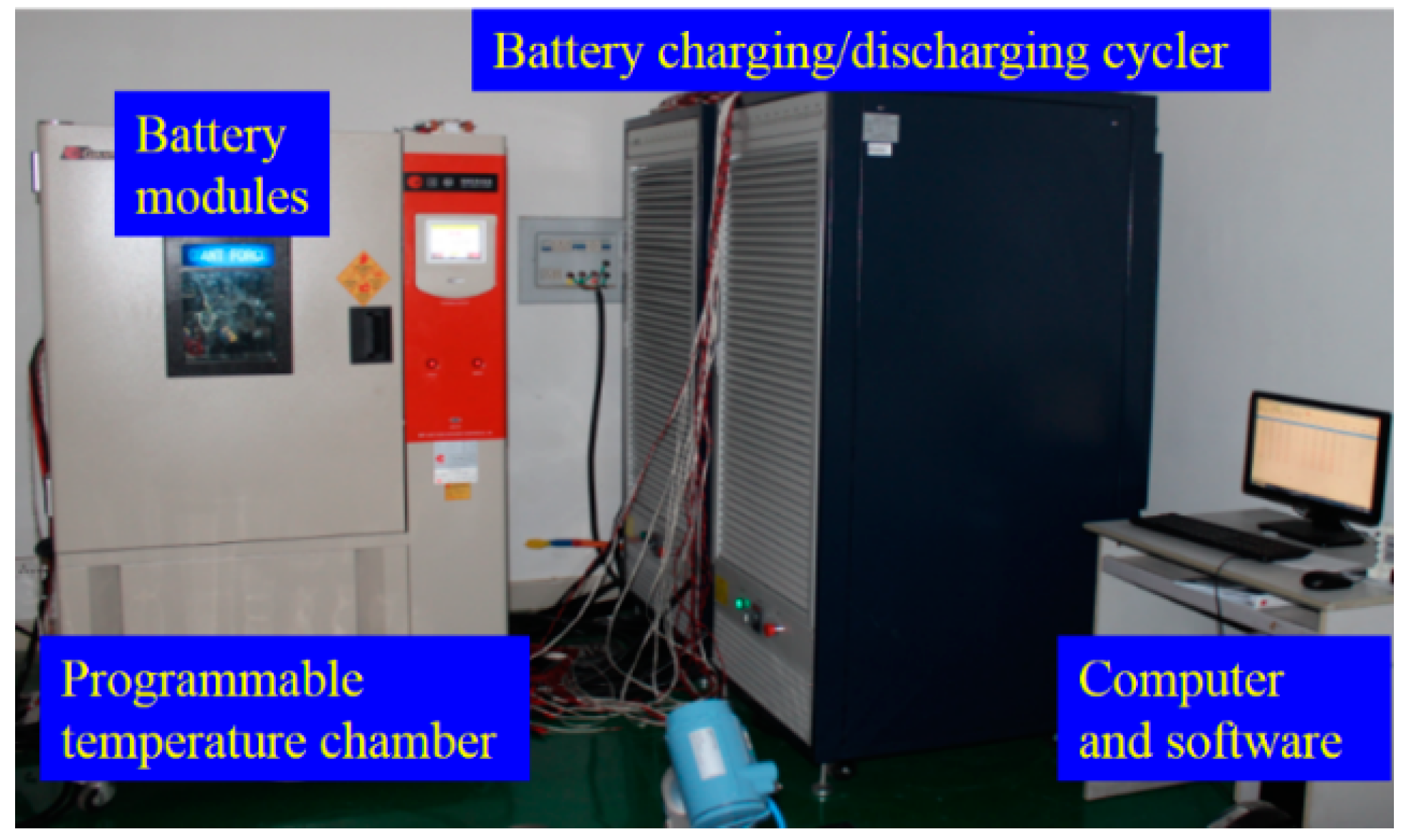
4.1. Experimental Platform and Test Results
4.2. Parameter Identification
4.3. Experiment Verification
5. Conclusions
6. Patents
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
Abbreviations
| LIB | lithium-ion battery |
| EchM | electrochemical model |
| AM | analytical model |
| SM | stochastic model |
| NNM | neural network model |
| ECM | equivalent circuit model |
| U-I | voltage-current |
| KiBaM | kinetic battery model |
| T-KiBaM | temperature-dependent kinetic battery model |
| FO-KiBaM | fractional-order kinetic battery model |
| BMS | battery management system |
| Ah | ampere hours |
| SOC | state of charge |
| SOH | state of health |
| SOP | state of peak power |
| SOF | state of function |
| SOE | state of energy |
| C-rate | current rate |
| CCCV | constant-current and constant-voltage |
| CC | constant current |
| CV | constant voltage |
| FDD | fractional derivative definition |
| GL-FDD | Grunwald-Letnikov fractional derivative definitions |
| RL-FDD | Riemann-Liouville fractional derivative definitions |
| MAE | mean absolute error |
| Nomenclature | |
| y1 | directly available capacity |
| h1 | height of directly available capacity |
| y2 | temporary capacity |
| h2 | height of temporary capacity well |
| the height difference of two wells | |
| k | rate that the charge flows from y2 into y1 |
| c | capacity proportion of two wells |
| i | discharge current |
| I | the constant discharge current |
| the initial time | |
| the discharge end time | |
| the recovery end time | |
| Cav | available capacity of battery |
| Cunav | unavailable capacity of battery |
| Crem | remaining available capacity of battery |
| Cmax | the maximum available capacity of battery |
| Ct0 | initial capacity of battery |
References
- Lievre, A.; Sari, A.; Venet, P.; Hijazi, A.; Ouattarabrigaudet, M.; Pelissier, S. Practical online estimation of lithium-ion battery apparent series resistance for mild hybrid vehicles. IEEE Trans. Veh. Technol. 2016, 65, 4505–4511. [Google Scholar] [CrossRef]
- Xia, B.; Nguyen, T.; Mi, C. A correlation-based fault detection method for short circuits in battery packs. J. Power Sources 2017, 337, 1–10. [Google Scholar] [CrossRef]
- Shang, Y.; Zhang, Q.; Cui, N.; Zhang, C. A cell-to-cell equalizer based on three-resonant-state switched-capacitor converters for series-connected battery strings. Energies 2017, 10, 206. [Google Scholar] [CrossRef]
- Hannan, M.A.; Lipu, M.S.H.; Hussain, A.; Mohamed, A. A review of lithium-ion battery state of charge estimation and management system in electric vehicle applications: Challenges and recommendations. Renew. Sustain. Energy Rev. 2017, 78, 834–854. [Google Scholar] [CrossRef]
- Rivera-Barrera, J.P.; Muñoz-Galeano, N.; Sarmiento-Maldonado, H.O. SoC Estimation for Lithium-ion Batteries: Review and Future Challenges. Electronics 2017, 6, 102. [Google Scholar] [CrossRef]
- Lai, X.; Yi, W.; Zheng, Y.; Zhou, L. An All-Region State-of-Charge Estimator Based on Global Particle Swarm Optimization and Improved Extended Kalman Filter for Lithium-Ion Batteries. Electronics 2018, 7, 321. [Google Scholar] [CrossRef]
- Jongerden, M.R.; Haverkort, B.R. Which battery model to use? IET Softw. 2009, 3, 445–457. [Google Scholar] [CrossRef]
- Ko, S.T.; Ahn, J.H.; Lee, B.K. Enhanced Equivalent Circuit Modeling for Li-ion Battery Using Recursive Parameter Correction. J. Electr. Eng. Technol. 2018, 13, 1147–1155. [Google Scholar]
- He, H.; Xiong, R.; Guo, H.; Li, S. Comparison study on the battery models used for the energy management of batteries in electric vehicles. Energy Convers. Manag. 2012, 64, 113–121. [Google Scholar] [CrossRef]
- Shang, Y.; Zhang, Q.; Zhang, C.; Cui, N. Research on variable-order RC equivalent circuit model for lithium-ion battery based on the AIC criterion. Trans. China Electrotech. Soc. 2015, 30, 55–62. [Google Scholar]
- Zhou, Y.; Huang, M. On-board Capacity Estimation of Lithium-ion Batteries Based on Charge Phase. J. Electr. Eng. Technol. 2018, 13, 733–741. [Google Scholar]
- Cloth, L.; Jongerden, M.R.; Haverkort, B.R. Computing battery lifetime distributions. In Proceedings of the 37th Annual IEEE/IFIP International Conference on Dependable Systems and Networks (DSN’07), Edinburgh, UK, 25–28 June 2007; pp. 780–789. [Google Scholar]
- Manwell, J.; McGowan, J. Lead acid battery storage model for hybrid energy systems. Sol. Energy 1993, 50, 399–405. [Google Scholar] [CrossRef]
- Kim, T.; Qiao, W. A hybrid battery model capable of capturing dynamic circuit characteristics and nonlinear capacity effects. IEEE Trans. Energy Convers. 2011, 26, 1172–1180. [Google Scholar] [CrossRef]
- Blanco, C.; Sánchez, L.; González, M.; Antón, J.C.; García, V.; Viera, J.C. An equivalent circuit model with variable effective capacity for lifepo4 batteries. IEEE Trans. Veh. Technol. 2014, 63, 3592–3599. [Google Scholar] [CrossRef]
- Sánchez, L.; Blanco, C.; Antón, J.C.; García, V.; González, M.; Viera, J.C. A variable effective capacity model for LiFePO4 traction batteries using computational intelligence techniques. IEEE Trans. Ind. Electron. 2015, 62, 555–563. [Google Scholar] [CrossRef]
- Baert, D.; Vervaet, A. Lead-acid battery model for the derivation of Peukert’s law. Electrochim. Acta 1999, 44, 3491–3504. [Google Scholar] [CrossRef]
- Rodrigues, L.M.; Montez, C.; Moraes, R.; Portugal, P.; Vasques, F. A Temperature-Dependent Battery Model for Wireless Sensor Networks. Sensors 2017, 17, 422. [Google Scholar] [CrossRef]
- Freeborn, T.J.; Maundy, B.; Elwakil, A.S. Fractional-order models of supercapacitors, batteries and fuel cells: A survey. Mater. Renew. Sustain. Energy 2015, 4, 9. [Google Scholar] [CrossRef]
- Wang, W.G.; Li, Y.; Chen, Y.Q. Ubiquitous fractional order capacitors. In Proceedings of the International Conference on Fractional Dierentiation and Its Applications, Novi Sad, Serbia, 18–20 July 2016. [Google Scholar]
- Malek, H.; Dadras, S.; Chen, Y. Fractional order equivalent series resistance modelling of electrolytic capacitor and fractional order failure prediction with application to predictive maintenance. IET Power Electron. 2016, 9, 1608–1613. [Google Scholar] [CrossRef]
- Sabatier, J.; Merveillaut, M.; Francisco, J.; Guillemard, F.; Porcelatto, D. Fractional models for lithium-ion batteries. In Proceedings of the 2013 European Control Conference, Zürich, Switzerland, 17–19 July 2013; pp. 3458–3463. [Google Scholar]
- Sabatier, J.; Cugnet, M.; Laruelle, S.; Grugeon, S.; Sahut, B.; Oustaloupa, A.; Tarasconb, J.M. A fractional order model for lead-acid battery crankability estimation. Commun. Nonlinear Sci. Numer. Simul. 2010, 15, 1308–1317. [Google Scholar] [CrossRef]
- Sun, Y.; Wu, X.; Cao, J.; Wei, Z.; Sun, G. Fractional extended Kalman filtering for nonlinear fractional system with Lévy noises. IET Control Theory Appl. 2017, 11, 349–358. [Google Scholar] [CrossRef]
- Sun, H.; Zhang, Y.; Wei, S.; Zhu, J.; Chen, W. A space fractional constitutive equation model for non-Newtonian fluid flow. Commun. Nonlinear Sci. Numer. Simul. 2018, 62, 409–417. [Google Scholar] [CrossRef]
- Zhang, Q.; Shang, Y.; Cui, N.; Li, Y.; Zhang, C. A fractional-order KiBaM of lithium-ion batteries with capacity nonlinearity. In Proceedings of the 2017 Chinese Automation Congress, Jinan, China, 20–22 October 2017; pp. 4995–5000. [Google Scholar]
- Li, Y.; Chen, Y.Q.; Podlubny, I. Mittag-Leffler stability of fractional order nonlinear dynamic systems. Automatica 2009, 45, 1965–1969. [Google Scholar] [CrossRef]
- Li, Y.; Chen, Y.Q.; Podlubny, I. Stability of fractional-order nonlinear dynamic systems: Lyapunov direct method and generalized Mittag-Leffler stability. Comput. Math. Appl. 2010, 59, 1810–1821. [Google Scholar] [CrossRef]
- Wang, Q.; Zhang, J.; Ding, D.; Qi, D. Adaptive Mittag-Leffler stabilization of a class of fractional order uncertain nonlinear systems. Asian J. Control 2016, 18, 2343–2351. [Google Scholar] [CrossRef]
- Khurram, A.; Rehman, H.; Mukhopadhyay, S.; Ali, D. Comparative Analysis of Integer-order and Fractional-order Proportional Integral Speed Controllers for Induction Motor Drive Systems. J. Power Electron. 2018, 18, 723–735. [Google Scholar]
- Yang, J.; Xia, B.; Shang, Y.L.; Huang, W.; Mi, C. Improved battery parameter estimation method considering operating scenarios for HEV/EV applications. Energies 2016, 10, 5. [Google Scholar] [CrossRef]
- Feng, F.; Lu, R.; Wei, G.; Zhu, C. Identification and analysis of model parameters used for LiFePO4 cells series battery pack at various ambient temperature. IET Electr. Syst. Transp. 2016, 6, 50–55. [Google Scholar] [CrossRef]
- Hua, C.C.; Fang, Y.H.; Chen, Y.L. Modified rectifications for improving the charge equalisation performance of series-connected battery stack. IET Power Electron. 2016, 6, 1924–1932. [Google Scholar] [CrossRef]

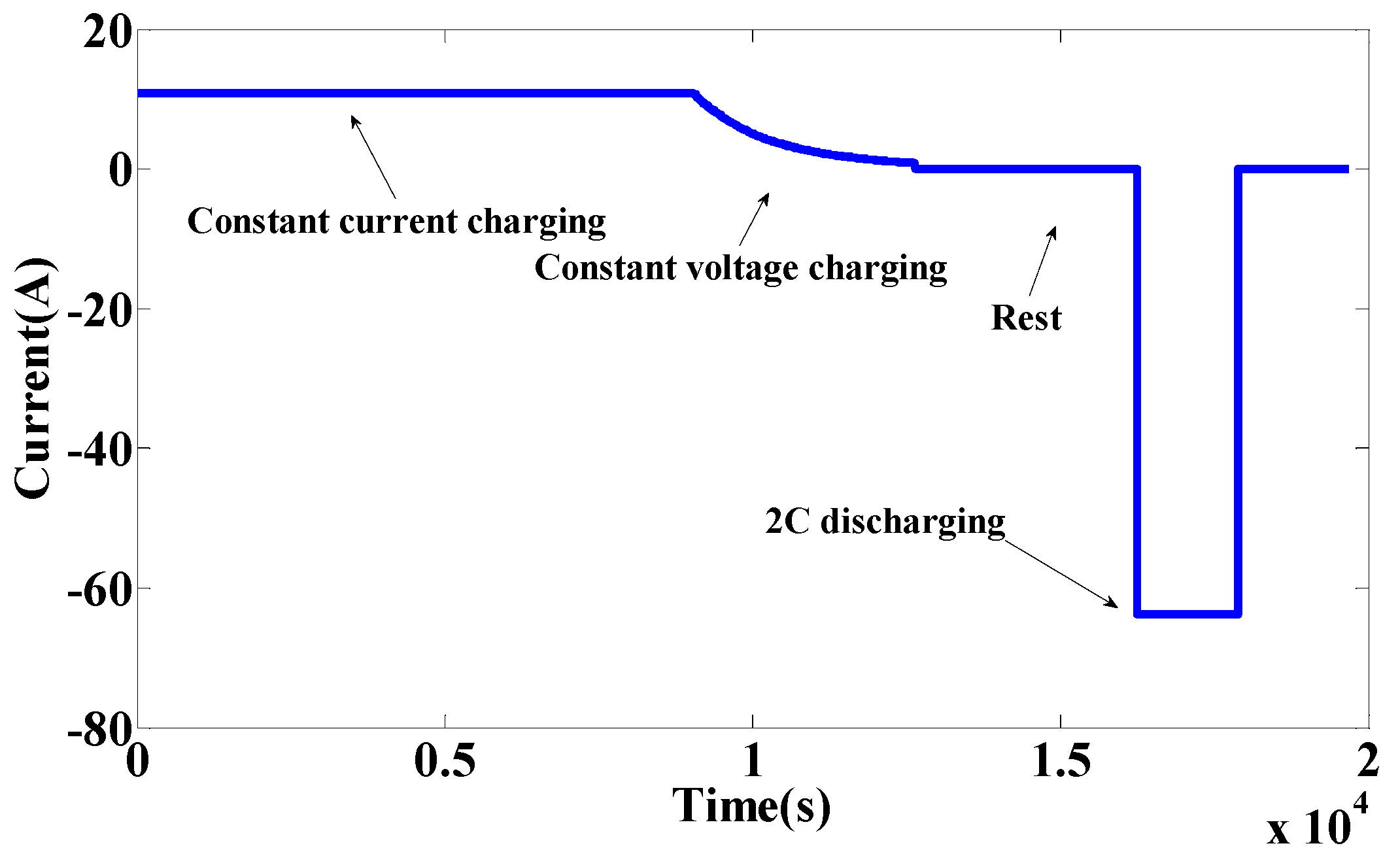

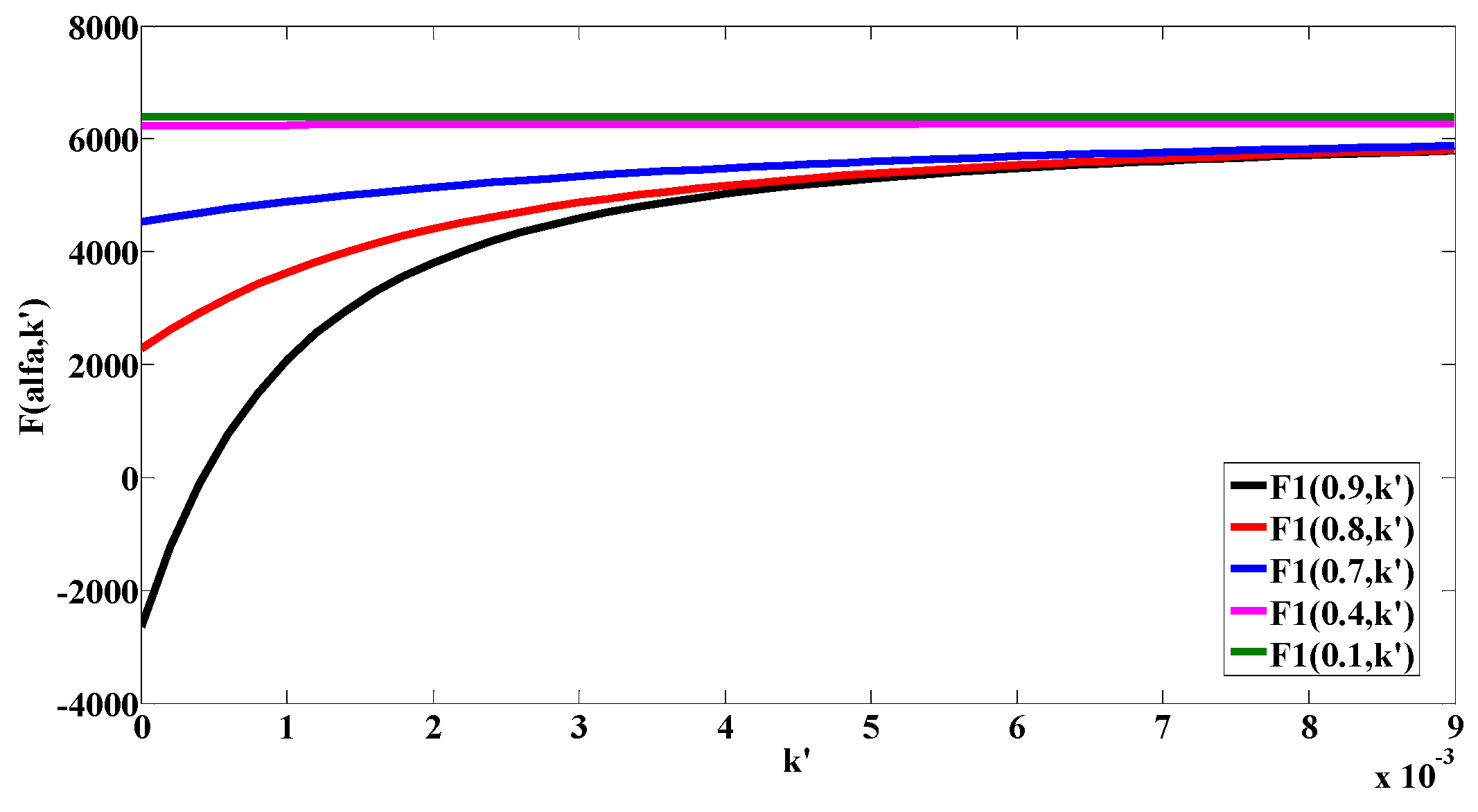
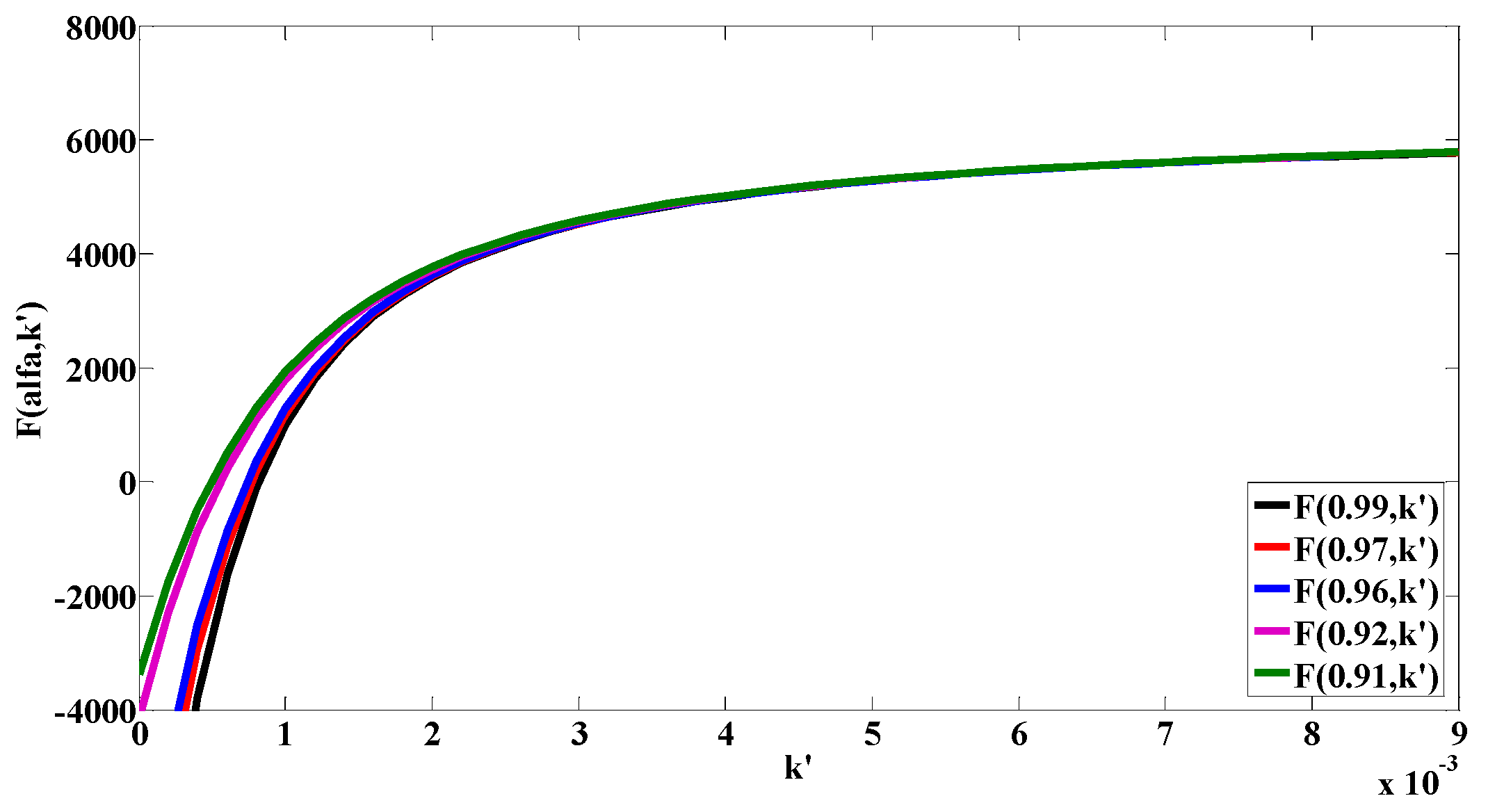
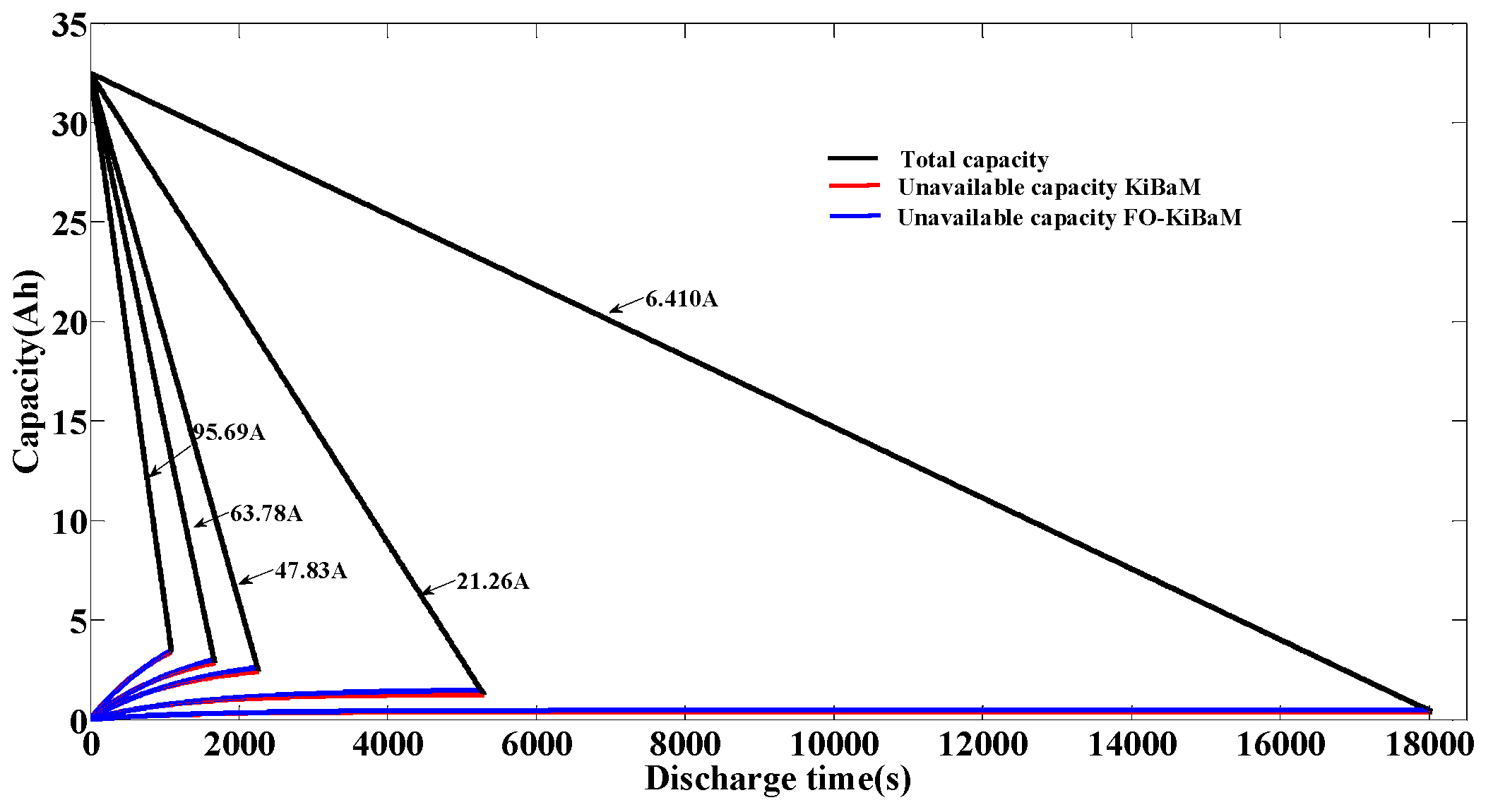
| Parameter | Value | Parameter | Value |
|---|---|---|---|
| Type | LR1865SZ | Series-parallel | 1S-13P |
| Nominal voltage | 3.6 V | Rated capacity | 2.5 Ah |
| End-of-charge voltage | 4.2 V | End-of-discharge voltage | 3.0 V |
| Discharge Rate (C) | Discharge Current (A) | Discharge Time (min) | Released Capacity (Ah) | Proportion (%) |
|---|---|---|---|---|
| 0.2 | 6.410 | 292.5 | 31.24 | 96.12 |
| 0.67 | 21.26 | 87.34 | 30.95 | 95.23 |
| 1 | 31.88 | 57.81 | 30.72 | 94.52 |
| 1.5 | 47.83 | 37.56 | 29.94 | 92.12 |
| 2 | 63.78 | 27.39 | 29.11 | 89.57 |
| 3 | 95.69 | 17.30 | 27.59 | 84.89 |
| Discharge Current (A) | Available Capacity (Ah) | Improve Accuracy (%) | ||
|---|---|---|---|---|
| Experimental Data | KiBaM | FO-KiBaM | ||
| 6.410 | 31.24 | 32.12 | 32.04 | 0.26 |
| 21.26 | 30.95 | 31.27 | 31.03 | 0.78 |
| 47.83 | 29.94 | 30.10 | 29.90 | 0.67 |
| 63.78 | 29.11 | 29.66 | 29.50 | 0.55 |
| 95.69 | 27.59 | 29.11 | 29.04 | 0.25 |
© 2019 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Zhang, Q.; Li, Y.; Shang, Y.; Duan, B.; Cui, N.; Zhang, C. A Fractional-Order Kinetic Battery Model of Lithium-Ion Batteries Considering a Nonlinear Capacity. Electronics 2019, 8, 394. https://doi.org/10.3390/electronics8040394
Zhang Q, Li Y, Shang Y, Duan B, Cui N, Zhang C. A Fractional-Order Kinetic Battery Model of Lithium-Ion Batteries Considering a Nonlinear Capacity. Electronics. 2019; 8(4):394. https://doi.org/10.3390/electronics8040394
Chicago/Turabian StyleZhang, Qi, Yan Li, Yunlong Shang, Bin Duan, Naxin Cui, and Chenghui Zhang. 2019. "A Fractional-Order Kinetic Battery Model of Lithium-Ion Batteries Considering a Nonlinear Capacity" Electronics 8, no. 4: 394. https://doi.org/10.3390/electronics8040394
APA StyleZhang, Q., Li, Y., Shang, Y., Duan, B., Cui, N., & Zhang, C. (2019). A Fractional-Order Kinetic Battery Model of Lithium-Ion Batteries Considering a Nonlinear Capacity. Electronics, 8(4), 394. https://doi.org/10.3390/electronics8040394







