Sufficient Condition-Based Stability Analysis of a Power Converter Applied Switching Transient Waveform Modification Using Kharitonov’s Theorem
Abstract
:1. Introduction
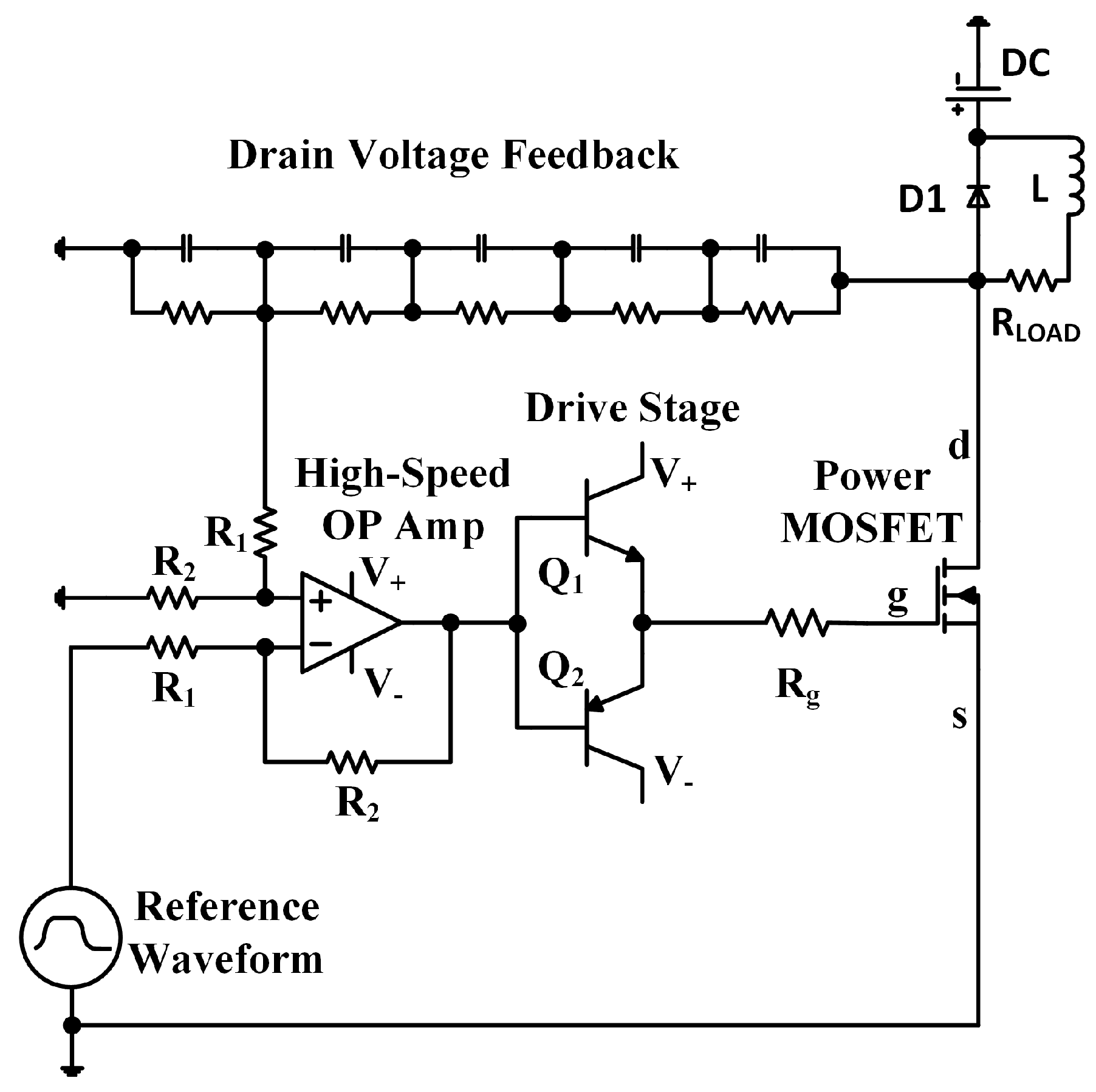
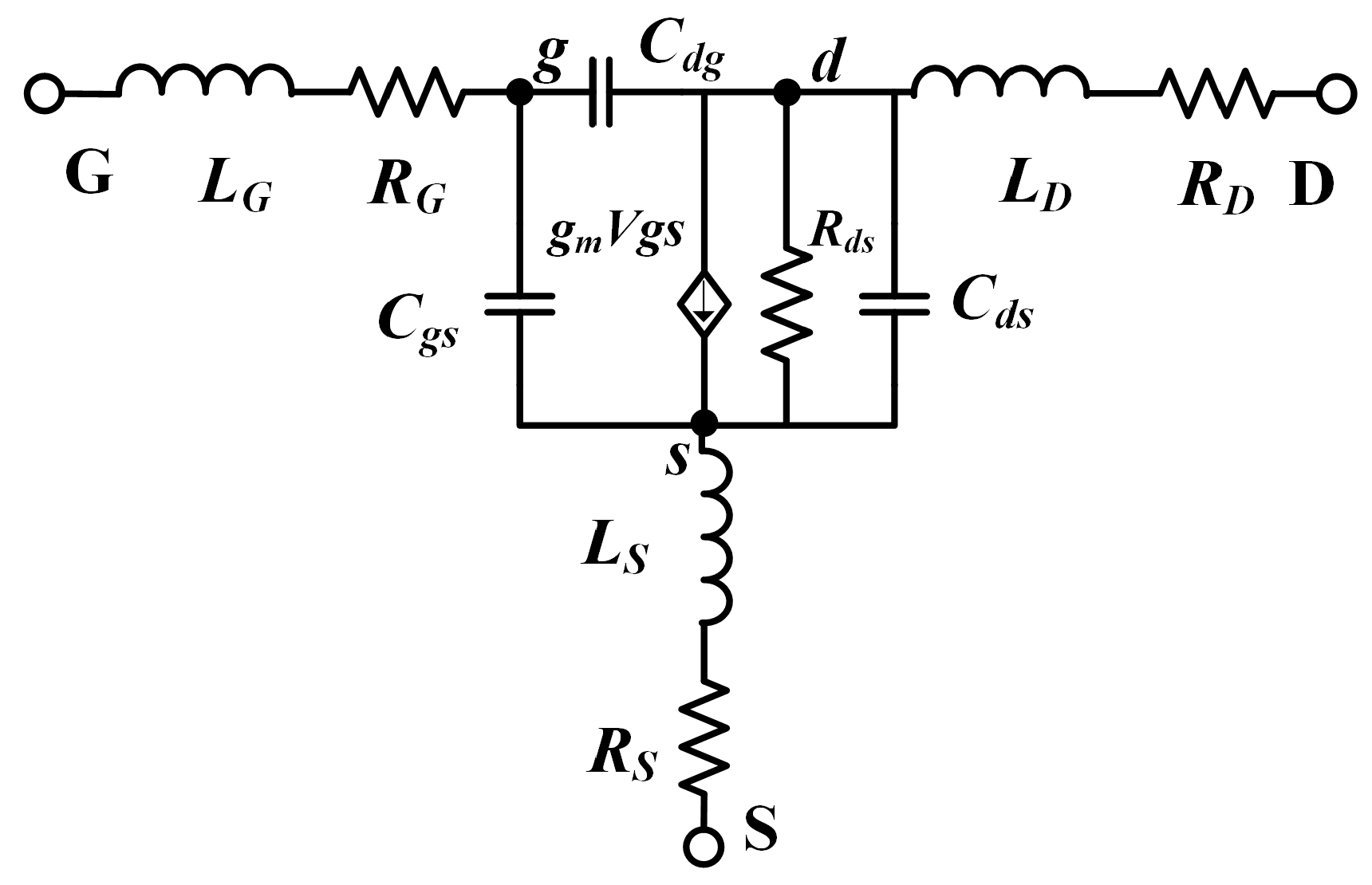
2. Modeling of Closed-Loop Gate Drive
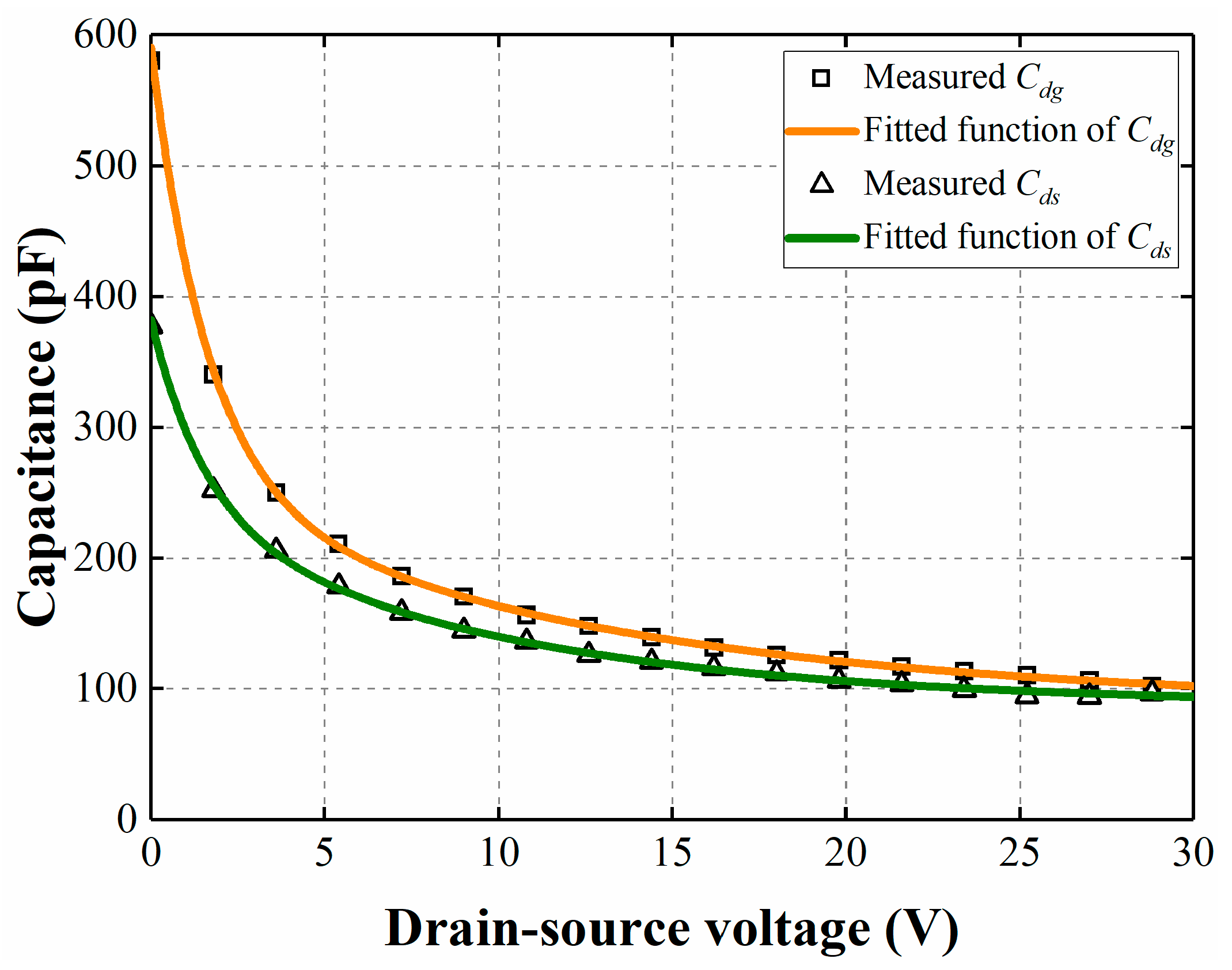
3. Determination of the Parameters
4. Stability Analysis
4.1. Kharitonov’s Theorem
4.2. Sufficient Condition-Based Stability Analysis
5. Experimental Results
6. Conclusions
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
References
- Zhu, H.; Liu, D.; Zhang, X.; Qu, F. Reliability of Boost PFC Converters with Improved EMI Filters. Electronics 2018, 7, 413. [Google Scholar] [CrossRef]
- Varajão, D.; Esteves Araújo, R.; Miranda, L.M.; Peças Lopes, J.A. EMI Filter Design for a Single-stage Bidirectional and Isolated AC–DC Matrix Converter. Electronics 2018, 7, 318. [Google Scholar] [CrossRef]
- Giglia, G.; Ala, G.; Di Piazza, M.C.; Giaconia, G.C.; Luna, M.; Vitale, G.; Zanchetta, P. Automatic EMI Filter Design for Power Electronic Converters Oriented to High Power Density. Electronics 2018, 7, 9. [Google Scholar] [CrossRef]
- John, V.; Suh, B.; Lipo, T.A. High-performance Active Gate Drive for high-power IGBT’s. IEEE Trans. Ind. Appl. 1999, 35, 1108–1117. [Google Scholar] [CrossRef]
- Palmer, P.R.; Rajamani, H.S. Active Voltage Control of IGBTs for High Power Applications. IEEE Trans. Power Electron. 2004, 19, 894–901. [Google Scholar] [CrossRef]
- Chen, L.; Peng, F.Z. Closed-loop Gate Drive for High Power IGBTs. In Proceedings of the 24th Annual IEEE Applied Power Electronics Conference and Exposition, Washington, DC, USA, 15–19 February 2009; pp. 1331–1337. [Google Scholar]
- Patin, N.; Vinals, M.L. Toward an Optimal Heisenberg’s Closed-loop Gate Drive for Power MOSFETs. In Proceedings of the 38th Annual Conference on IEEE Industrial Electronics Society, Montreal, QC, Canada, 25–28 October 2012; pp. 828–833. [Google Scholar]
- Yang, X.; Yuan, Y.; Zhang, X.; Palmer, P.R. Shaping High-power IGBT Switching Transitions by Active Voltage Control for Reduced EMI Generation. IEEE Trans. Ind. Appl. 2015, 51, 1669–1677. [Google Scholar] [CrossRef]
- Palmer, P.R.; Wang, Y.; Abu-khaizaran, M. Design of the Active Voltage Controller for Series IGBTs. In Proceedings of the 35th Annual Power Electronics Specialists Conference, Aachen, Germany, 20–25 June 2004; pp. 3248–3254. [Google Scholar]
- Wang, Y.; Palmer, P.R.; Bryant, A.T.; Finney, S.J.; Abu-Khaizaran, M.S.; Li, G. An Analysis of High-power IGBT Switching Under Cascade Active Voltage Control. IEEE Trans. Ind. Appl. 2009, 45, 861–870. [Google Scholar] [CrossRef]
- Yang, X.; Yuan, Y.; Long, Z.; Goncalves, J.; Palmer, P.R. Robust Stability Analysis of Active Voltage Control for High-power IGBT Switching by Kharitonov’s Theorem. IEEE Trans. Power Electron. 2016, 31, 2584–2595. [Google Scholar] [CrossRef]
- Groeger, J.; Wicht, B.; Norling, K. Dynamic Stability of a Closed-loop Gate Driver Enabling Digitally Controlled Slope Shaping. In Proceedings of the 13th Conference on Ph.D. Research in Microelectronics and Electronics, Giardini Naxos, Italy, 12–15 June 2017; pp. 61–64. [Google Scholar]
- Costa, F.; Magnon, D. Graphical Analysis of the Spectra of EMI Sources in Power Electronics. IEEE Trans. Power Electron. 2005, 20, 1491–1498. [Google Scholar] [CrossRef]
- Kharitonov, V.L. Asymptotic Stability of an Equilibrium Position of a Family of Systems of Differential Equations. Differ. Uravn. 1978, 14, 1483–1485. [Google Scholar]


| Circuit Designs | Drain Voltage Feedback | Op-Amp | Gate Resistance | ||
|---|---|---|---|---|---|
| Device | RC | THS3091 | R | ||
| α | fFB (MHz) | AOP | fOP (MHz) | Rg (Ω) | |
| Design I | 1/20 | 20 | 8 | 200 | 10 |
| Design II | 1/20 | 20 | 10 | 160 | 10 |
| Design III | 1/20 | 20 | 8 | 200 | 5 |
| Design IV | 1/25 | 20 | 10 | 160 | 10 |
| Subinterval | 0–100 ns | 100–200 ns | 200–300 ns | 300–400 ns | 400–500 ns | |||||
|---|---|---|---|---|---|---|---|---|---|---|
| Min | Max | Min | Max | Min | Max | Min | Max | Min | Max | |
| VDS (V) | 0 | 2.18 | 2.18 | 9.59 | 9.59 | 20.29 | 20.29 | 27.76 | 27.76 | 30 |
| Cdg (pF) | 316.36 | 590.59 | 166.12 | 316.36 | 119.75 | 166.12 | 104.99 | 119.75 | 102.07 | 104.99 |
| Cds (pF) | 241.37 | 381.97 | 142.39 | 241.37 | 105.23 | 142.39 | 95.57 | 105.23 | 93.88 | 95.57 |
| Subinterval | 0–100 ns | |
|---|---|---|
| Min | Max | |
| c0 | −8.2 × 1021 | −8.2 × 1021 |
| c1 | −1.8 × 1014 | −9.6 × 1013 |
| c2 | −1.8 × 1016 | −9.8 × 105 |
| c3 | −0.0037 | −0.002 |
| c4 | −2 × 10−12 | −1.1 × 10−12 |
| c5 | −1.3 × 10−22 | −6.1 × 10−23 |
© 2019 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Cui, T.; Ma, Q.; Xu, P. Sufficient Condition-Based Stability Analysis of a Power Converter Applied Switching Transient Waveform Modification Using Kharitonov’s Theorem. Electronics 2019, 8, 245. https://doi.org/10.3390/electronics8020245
Cui T, Ma Q, Xu P. Sufficient Condition-Based Stability Analysis of a Power Converter Applied Switching Transient Waveform Modification Using Kharitonov’s Theorem. Electronics. 2019; 8(2):245. https://doi.org/10.3390/electronics8020245
Chicago/Turabian StyleCui, Tongkai, Qishuang Ma, and Ping Xu. 2019. "Sufficient Condition-Based Stability Analysis of a Power Converter Applied Switching Transient Waveform Modification Using Kharitonov’s Theorem" Electronics 8, no. 2: 245. https://doi.org/10.3390/electronics8020245
APA StyleCui, T., Ma, Q., & Xu, P. (2019). Sufficient Condition-Based Stability Analysis of a Power Converter Applied Switching Transient Waveform Modification Using Kharitonov’s Theorem. Electronics, 8(2), 245. https://doi.org/10.3390/electronics8020245





