Design and Analysis of CCII-Based Oscillator with Amplitude Stabilization Employing Optocouplers for Linear Voltage Control of the Output Frequency
Abstract
1. Introduction
- (a)
- (b)
- (c)
- the majority of already known solutions provide only inversely proportional square root dependence of the FO on the driving force (value of the single resistor, FO~R−1/2), which limits the tunability to be quite narrow,
- (d)
- solutions combining control by value of passive element (or its replacement) and active parameter (for the CO control, for example) are not studied (only Reference [14] discusses the adjustment of the CO by an active parameter; however, the FO is also tuned by an active parameter (RX)),
- (e)
- solutions implementing two parameters for FO tuning (completely uncoupled from the CO) in order to extend tunability are not proposed (with the exception of Reference [14], where this operation was not verified),
- (f)
- the simple implementation of necessary systems for amplitude gain control circuit (AGC) for amplitude stabilization utilizing electronically (voltage) controlled CO by a specific active parameter is not considered in the majority of solutions summarized in Table 1. Therefore, many topologies have very uncomfortable CO (with a typical form of C1 = C2 or R1 = R2, and their presence is also found in the FO as product of R1R2 and C1C2, see for example solutions in [17,18]). An additional active or passive parameter suitable for the adjustment of CO (not included in the FO relation) should be included, but it cannot be revealed (in these simple solutions having 2 R, 2 C) without the analysis of general (not equal to unity) terminal transfer relations of the active elements (current conveyors).
2. Detailed Qualitative Comparison of the Most Similar Solutions and the New Proposal
3. Implementation of Optocouplers for Control of VCO
4. Topology Suitable for Selected Method of FO Control
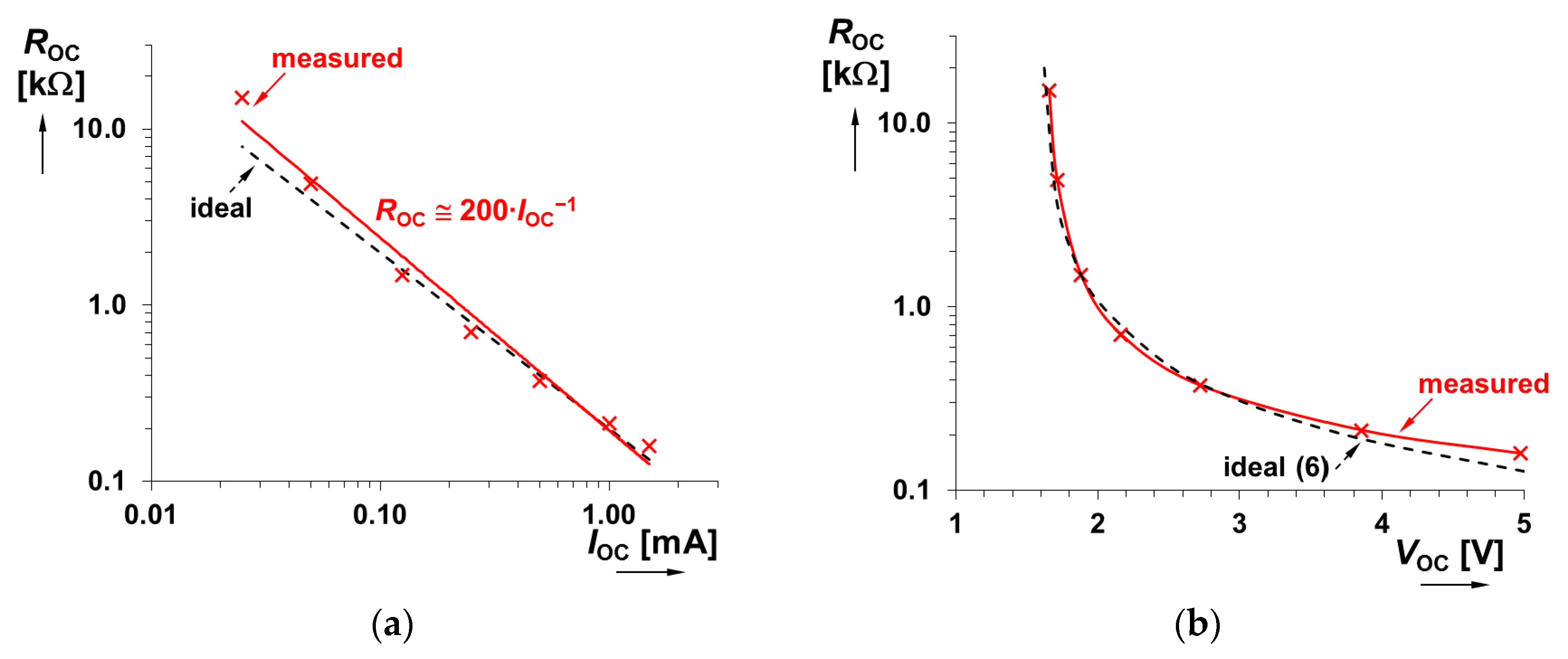
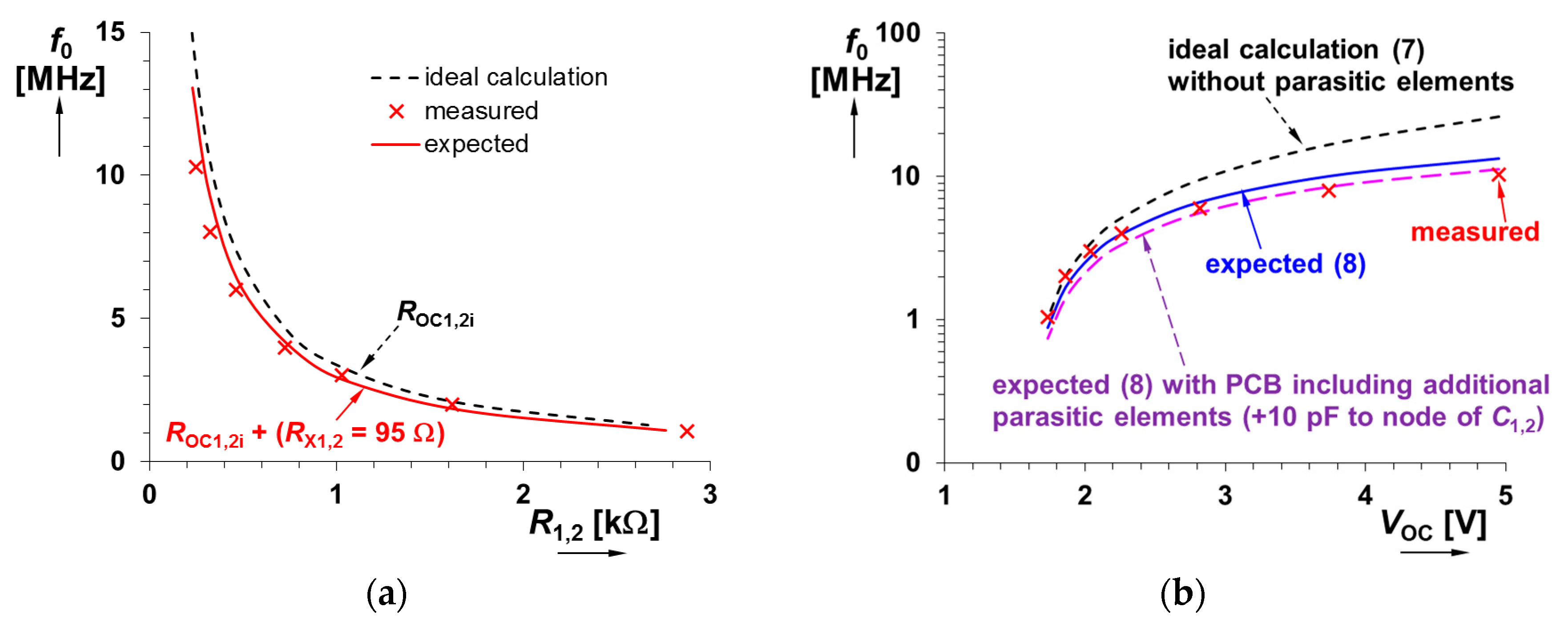
5. Design of Oscillator and Results of Experiments
6. Discussion of Other Methods of Indirect Electronic Control of FO
7. Conclusions
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
References
- Senani, R.; Bhaskar, D.R.; Singh, V.K.; Sharma, R.K. Sinusoidal Oscillators and Waveform Generators Using Modern Electronic Circuit Building Blocks; Springer International Publishing AG: Cham, Switzerland, 2016; pp. 1–622. ISBN 978-3-319-23712-1. [Google Scholar] [CrossRef]
- Senani, R.; Bhaskar, D.R.; Singh, A.K. Current Conveyors: Variants, Applications and Hardware Implementations, 1st ed.; Springer: Berlin/Heidelberg, Germany, 2015; ISBN 978-3-319-08684-2. [Google Scholar] [CrossRef]
- Liu, S.-I. Single-resistance-controlled/voltage-controlled oscillator using current conveyors and grounded capacitors. Electron. Lett. 1995, 31, 337–338. [Google Scholar] [CrossRef]
- Abuelmaatti, M.T.; Ghumaiz, A.-A. Novel CCI-based Single-Element-Controlled Oscillators Employing Grounded Resistors and Capacitors. IEEE Trans. Circuits Syst. I Fundam. Theory Appl. 1996, 43, 153–155. [Google Scholar] [CrossRef]
- Senani, R.; Singh, V.K. Novel single-resistance-controlled-oscillator configuration using current-feedback-amplifiers. IEEE Trans. Circuits Syst. I Fundam. Theory Appl. 1996, 43, 698–700. [Google Scholar] [CrossRef]
- Martinez, P.A.; Sabadell, J.; Aldea, C. Grounded resistor controlled sinusoidal oscillator using CFOAs. Electron. Lett. 1997, 33, 346–348. [Google Scholar] [CrossRef]
- Gupta, S.S.; Senani, R. State variable synthesis of single resistance controlled grounded capacitor oscillators using only two CFOAs. IEE Proc. Circuits Devices Syst. 1998, 145, 135–138. [Google Scholar] [CrossRef]
- Gupta, S.S.; Senani, R. State variable synthesis of single resistance controlled grounded capacitor oscillators using only two CFOAs: Additional new realisations. IEE Proc. Circuits Devices Syst. 1998, 145, 415–418. [Google Scholar] [CrossRef]
- Soliman, A.M. Synthesis of grounded capacitor and grounded resistor oscillators. J. Frankl. Inst. 1999, 336, 735–746. [Google Scholar] [CrossRef]
- Khan, A.A.; Bimal, S.; Dey, K.K.; Roy, S.S. Novel RC Sinusoidal Oscillator Using Second-Generation Current Conveyor. IEEE Trans. Inst. Meas. 2005, 54, 2402–2406. [Google Scholar] [CrossRef]
- Bhaskar, D.R.; Senani, R. New CFOA-Based Single-Element-Controlled Sinusoidal Oscillators. IEEE Trans. Inst. Meas. 2006, 55, 2014–2021. [Google Scholar] [CrossRef]
- Gupta, S.S.; Bhaskar, D.R.; Senani, R. New voltage controlled oscillators using CFOAs. AEU—Int. J. Electron. Commun. 2009, 63, 209–217. [Google Scholar] [CrossRef]
- Abuelmaatti, M.T. Identification of a class of two CFOA-based sinusoidal RC oscillators. Analog Integr. Circuits Signal Proc. 2010, 65, 419–428. [Google Scholar] [CrossRef]
- Kumngern, M.; Junnapiya, S. A sinusoidal oscillator using translinear current conveyors. In Proceedings of the IEEE International Asia Pacific Conference on Circuits and Systems (APCCAS), Kuala Lumpur, Malaysia, 6–9 December 2010; pp. 740–743. [Google Scholar] [CrossRef]
- Lahiri, A.; Jaikla, W.; Siripruchyanun, M. Explicit-current-output second-order sinusoidal oscillator using two CFOAs and grounded capacitors. AEU—Int. J. Electron. Commun. 2011, 65, 669–672. [Google Scholar] [CrossRef]
- Lahiri, A.; Gupta, M. Realizations of Grounded Negative Capacitance Using CFOAs. Circuits Syst. Signal Proc. 2011, 30, 143–155. [Google Scholar] [CrossRef]
- Lahiri, A. Current-mode variable frequency quadrature sinusoidal oscillators using two CCs and four passive components including grounded capacitors. Analog Integr. Circuits Signal Proc. 2012, 71, 303–311. [Google Scholar] [CrossRef]
- Chen, H.-P.; Hsien, M.-Y.; Lin, C.-C.; Huang, W.-Y. CFOA-based quadrature oscillator employing grounded capacitors. In Proceedings of the IEEE International Conference on Information Science, Electronics, and Electrical Engineering (ISEEE), Sapporo, Japan, 26–28 April 2014; pp. 470–473. [Google Scholar] [CrossRef]
- Bajer, J.; Lahiri, A.; Biolek, D. Current-mode CCII+ Based Oscillator Circuits using a Conventional and a Modified Wien-Bridge with All Capacitors Grounded. Radioengineering 2011, 20, 245–250. [Google Scholar]
- Sotner, R.; Jerabek, J.; Prokop, R.; Kledrowetz, V.; Polak, J.; Fujcik, L.; Dostal, T. Practically Implemented Electronically Controlled CMOS Voltage Differencing Current Conveyor. In Proceedings of the IEEE 59th International Midwest Symposium on Circuits and Systems (MWSCAS), Abu Dhabi, UAE, 16–19 October 2016; pp. 667–670. [Google Scholar] [CrossRef]
- Biolkova, V.; Bajer, J.; Biolek, D. Four-Phase Oscillators Employing Two Active Elements. Radioengineering 2011, 20, 334–339. [Google Scholar]
- Biolek, D.; Senani, R.; Biolkova, V.; Kolka, Z. Active elements for analog signal processing: Classification, Review and New Proposals. Radioengineering 2008, 17, 15–32. [Google Scholar]
- Surakampontorn, W.; Thitimajshima, W. Integrable electronically tunable current conveyors. IEE Proc. G 1988, 135, 71–77. [Google Scholar] [CrossRef]
- Fabre, A.; Mimeche, N. Class A/AB second-generation current conveyor with controlled current gain. Electron. Lett. 1994, 30, 1267–1268. [Google Scholar] [CrossRef]
- Minaei, S.; Sayin, O.K.; Kuntman, H. A new CMOS electronically tunable current conveyor and its application to current-mode filters. IEEE Trans. Circuits Syst. I 2006, 53, 1448–1457. [Google Scholar] [CrossRef]
- Sotner, R.; Jerabek, J.; Herencsar, N.; Hrubos, Z.; Dostal, T.; Vrba, K. Study of Adjustable Gains for Control of Oscillation Frequency and Oscillation Condition in 3R-2C Oscillator. Radioengineering 2012, 21, 392–402. [Google Scholar]
- Intersil (Elantec). EL2082 CN Current-Mode Multiplier (Datasheet); 1996; 14p. Available online: http://www.intersil.com/data/fn/fn7152.pdf (accessed on 18 August 2018).
- Texas Instruments. OPA615 Wide Bandwidth, DC Restoration Circuit (Datasheet); 2009; 33p. Available online: http://www.ti.com/lit/ds/symlink/opa615.pdf (accessed on 18 August 2018).
- Texas Instruments. OPA2652 SpeedPlus Dual, 700 MHz, Voltage-Feedback Operational Amplifier (Datasheet); 2006; 23p. Available online: https://www.ti.com/lit/ds/symlink/opa2652.pdf (accessed on 18 August 2018).
- Sanchez-Sinencio, E.; Silva-Martinez, J. CMOS transconductance amplifiers, architectures and active filters: A tutorial. IEE Proc. Circuits Devices Syst. 2000, 147, 3–12. [Google Scholar] [CrossRef]
- Geiger, R.L.; Sanchez-Sinencio, E. Active filter design using operational transconductance amplifiers: A tutorial. IEEE Circuits Devices Mag. 1985, 1, 20–32. [Google Scholar] [CrossRef]
- Luna Optoelectronics. NSL-32SR3 Optocoupler (Datasheet); 2016; 2p. Available online: http://lunainc.com/wp-content/uploads/2016/06/NSL-32SR3.pdf (accessed on 18 August 2018).
- Nay, K.; Budak, A. A Voltage-Controlled Resistance with Wide Dynamic Range and Low Distortion. IEEE Trans. Circuits Syst. 1983, CAS-30, 770–772. [Google Scholar] [CrossRef]
- Senani, R. Realisation of linear voltage-controlled resistance in floating form. Electron. Lett. 1994, 30, 1909–1910. [Google Scholar] [CrossRef]
- Senani, R.; Bhaskar, D.R.; Gupta, S.S.; Singh, V.K. A configuration for realising floating, linear, voltage-controlled resistance, inductance and FDNC elements. Int. J. Circuit Theory Appl. 2009, 37, 709–719. [Google Scholar] [CrossRef]
- ON Semiconductor. BF245A/B JFET VHF/UHF Amplifiers N-Channel–Depletion (Datasheet); 2001; 8p. Available online: https://www.onsemi.com/pub/Collateral/BF245A-D.PDF (accessed on 18 August 2018).
- Analog Devices. Choosing the Correct digiPOT for Your Application (Application Note); 2014; 6p. Available online: http://www.analog.com/media/en/news-marketing-collateral/product-selection-guide/Choosing_the_Correct_Digipot.pdf (accessed on 18 August 2018).
- Sevcik, B. Modeling and Signal Integrity Testing of Digital Potentiometers. In Proceedings of the IEEE 17th International Conference Mixed Design of Integrated Circuits and Systems (MIXDES), Wroclaw, Poland, 24–26 June 2010; pp. 570–575. [Google Scholar]
- Lunca, E.; Damian, C.; Petrisor, D.; Postolache, O. Programmable Active Filters Based on Digital Potentiometers. In Proceedings of the IEEE International Conference and Exposition on Electrical and Power Engineering (EPE), Iasi, Romania, 25–27 October 2012; pp. 787–791. [Google Scholar] [CrossRef]
- Pandiev, I.M. Behavioral Modeling of CMOS Digital Potentiometers Using VHDL-AMS. In Proceedings of the IEEE International Power Electronics and Motion Control Conference (PEMC), Varna, Bulgaria, 25–28 September 2016; pp. 940–945. [Google Scholar] [CrossRef]
- Maxim Integrated. DACs vs. Digital Potentiometers: Which Is Right for My Application? 2007; 1p. Available online: https://www.maximintegrated.com/en/app-notes/index.mvp/id/4025 (accessed on 18 August 2018).
- Razavi, B. Design of Analog CMOS Integrated Circuits; McGraw-Hill: New York, NY, USA, 2001; ISBN 978-0072380323. [Google Scholar]
- Gray, P.R.; Hurst, P.J.; Lewis, S.H.; Meyer, R.G. Analysis and Design of Analog Integrated Circuits, 5th ed.; Wiley: Hoboken, NJ, USA, 2009; ISBN 978-0470245996. [Google Scholar]
- Rogers, J.W.M.; Plett, C.; Marsland, I. Radio Frequency System Architecture and Design; Artech House: London, UK, 2013; ISBN 978-1608075379. [Google Scholar]
- Soliman, A.M. Two integrator loop quadrature oscillators: A review. J. Adv. Res. 2013, 4, 1–11. [Google Scholar] [CrossRef] [PubMed]
- Abuelmaatti, M.T. Recent Developments in Current-Mode Sinusoidal Oscillators: Circuits and Active Elements. Arab. J. Sci. Eng. 2017, 42, 2583–2614. [Google Scholar] [CrossRef]

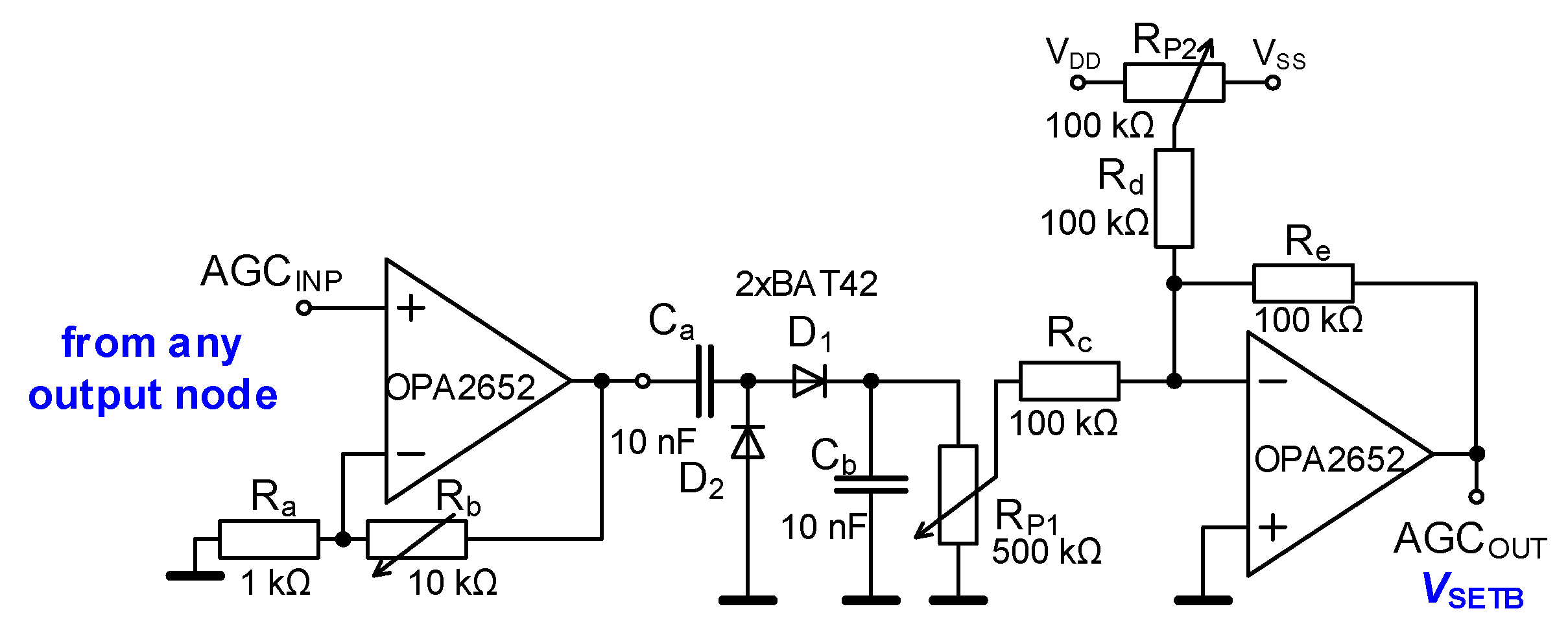
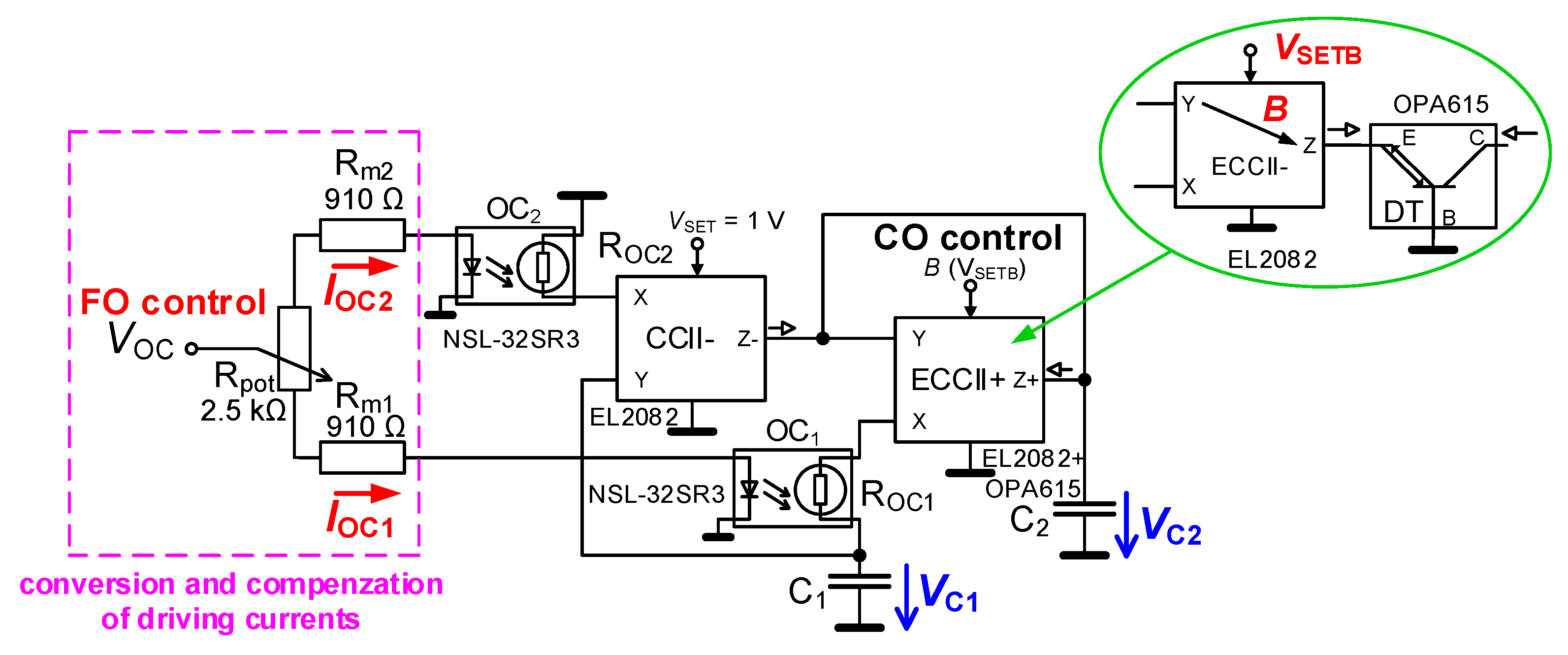


| Reference (Year) | Number of Active/Passive Elements | Type of Active Element(s) | Number and Type of Elements Suitable for Frequency of Oscillation (FO) Control | Allowed Character of FO Dependence | Tested Character of FO Dependence | Maximal Value of Tested FO (MHz) | Total Harmonic Distortion (THD) (%) | Power Consumption (mW) | Phase Noise (dBc/Hz) | Number and Type of Elements Suitable for Condition of Oscillation (CO) Control | Active Parameter for CO Control | Generated Levels (Amplitudes) Independent of Tuning Process | Produced Phase Shift (Degrees) | Verification | Amplitude Stabilization |
|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|
| [3] (1995) | 2/5 | CCI+, CCII− | 1 R grounded | ~R−1/2 | ~R−1/2 | 0.042 | N/A | N/A | N/A | 1 R grounded | - | N/A | N/A | M | N/A |
| [4] (1996) | 2/5 | CCI−, CCI− | 1 R grounded | ~R−1/2 | N/A | N/A | N/A | N/A | N/A | 1 R grounded | - | N/A | N/A | S | Yes a |
| [5] (1996) | 2/5 | CFOA | 1 R grounded | ~R−1/2 | ~R−1/2 | 0.460 (9.85) I | N/A | N/A | N/A | 1 R grounded | - | N/A | N/A | M | N/A |
| [6] (1997) | 2/5 | CFOA | 1 R grounded | ~R−1/2 | ~R−1/2 | 6.00 | <1 | N/A | N/A | 1 R grounded | - | N/A | N/A | M | N/A |
| [7] (1998) | 2/5 | CFOA | 1 R grounded | ~R−1/2 | ~R−1/2 | N/A | N/A | N/A | N/A | 1 R grounded | - | N/A | N/A | N/A | N/A |
| [8] (1998) | 2/5 | CFOA | 1 R grounded | ~ R−1/2 | N/A | 0.260 | N/A | N/A | N/A | 1 R grounded | - | N/A | N/A | M | N/A |
| [9] (1999) | 2/6 (5) | CCII+, CCII− | 2 R grounded | ~R−1 | N/A | 0.153 | N/A | N/A | N/A | 2 R grounded | - | N/A | 90 | S | N/A |
| [10] (2005) | 2/6 | CCII+ (CFOA) | 1 R grounded | b | b | 0.189 | 1.5 | N/A | N/A | 1 R floating | - | N/A | N/A | S | N/A |
| [11] (2006) | 2/5 | CFOA | 1 R floating or grounded c | ~R−1/2 | ~R−1/2 | 0.037 | <3.1 | N/A | N/A | c | - | N/A | N/A | M | N/A |
| [12] (2009) | 2/5 | CFOA | 1 R grounded | ~R−1/2 | ~R−1/2 | 290 (609) II | 1.6 | N/A | N/A | 1 R grounded | - | N/A | N/A | M | N/A |
| [13] (2010) | 2/4 | CFOA | d | ~R−1/2 | N/A | N/A | N/A | N/A | N/A | d | - | N/A | N/A | M | N/A |
| [14] (2010) | 2/2 | CCCII+/- | 2 RX | ~R−1 | ~R−1/2 | 1.80 | <7 | 3.5 | N/A | - | B | N/A | N/A | S | N/A |
| [15] (2011) | 2/5 | CFOA | 1 R grounded | ~R−1/2 | N/A | 1.320 | <3 | N/A | N/A | 1 R floating | - | N/A | N/A | M | N/A |
| [16] (2011) | 2/4 | CFOA | 1 Ceq grounded | ~Ceq−1/2 | N/A | 0.146 | <2 | N/A | N/A | 2 R floating * | - | N/A | 90 | S | N/A |
| [17] (2012) | 2/4 | DO-(I)CCII, CCI | 2 R floating and grounded | ~R−1 | ~R−1/2 | 0.182 | <1.7 | N/A | N/A | e* | - | No | 90 | S | N/A |
| [18] (2014) | 2/4 | CFOA | 2 R floating | ~R−1 | N/A | 0.065 (1.33) III | <0.8 | 0.86 | N/A | * | - | Yes | 90 | B | N/A |
| [19] (2011) | 2/6 | CCII+ | 2 R floating and grounded | ~R−1 | N/A | 1.43 | <0.3 | N/A | N/A | ** | - | N/A | N/A | M | Yes |
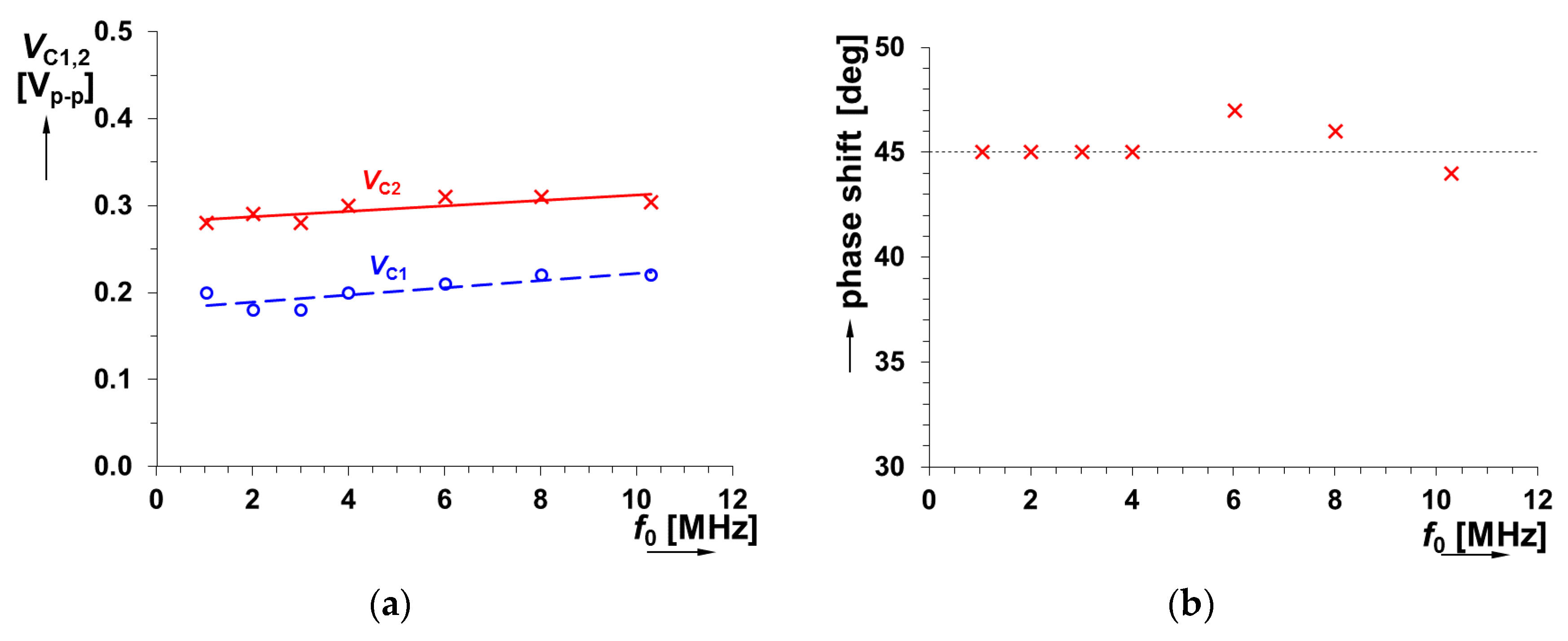
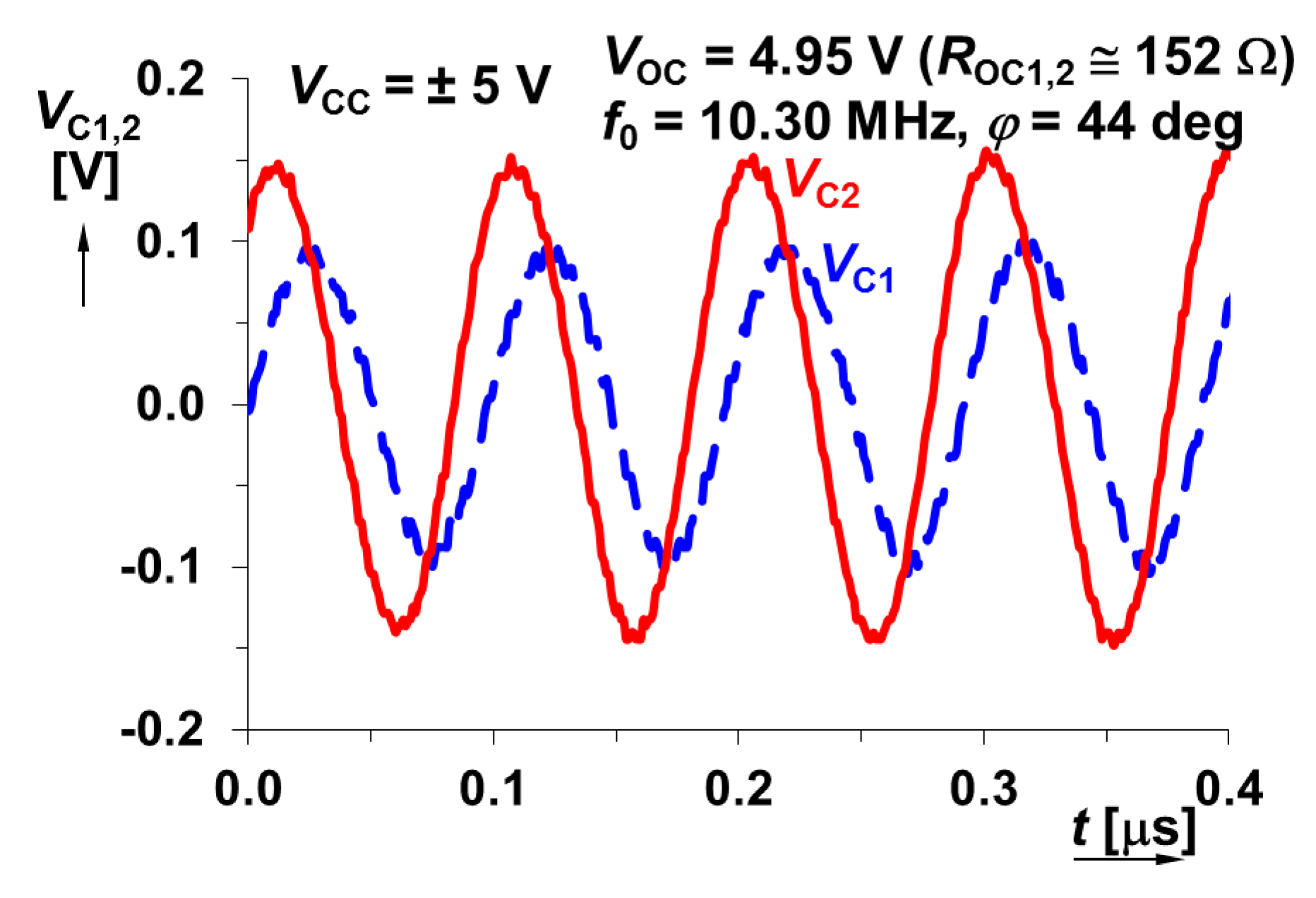
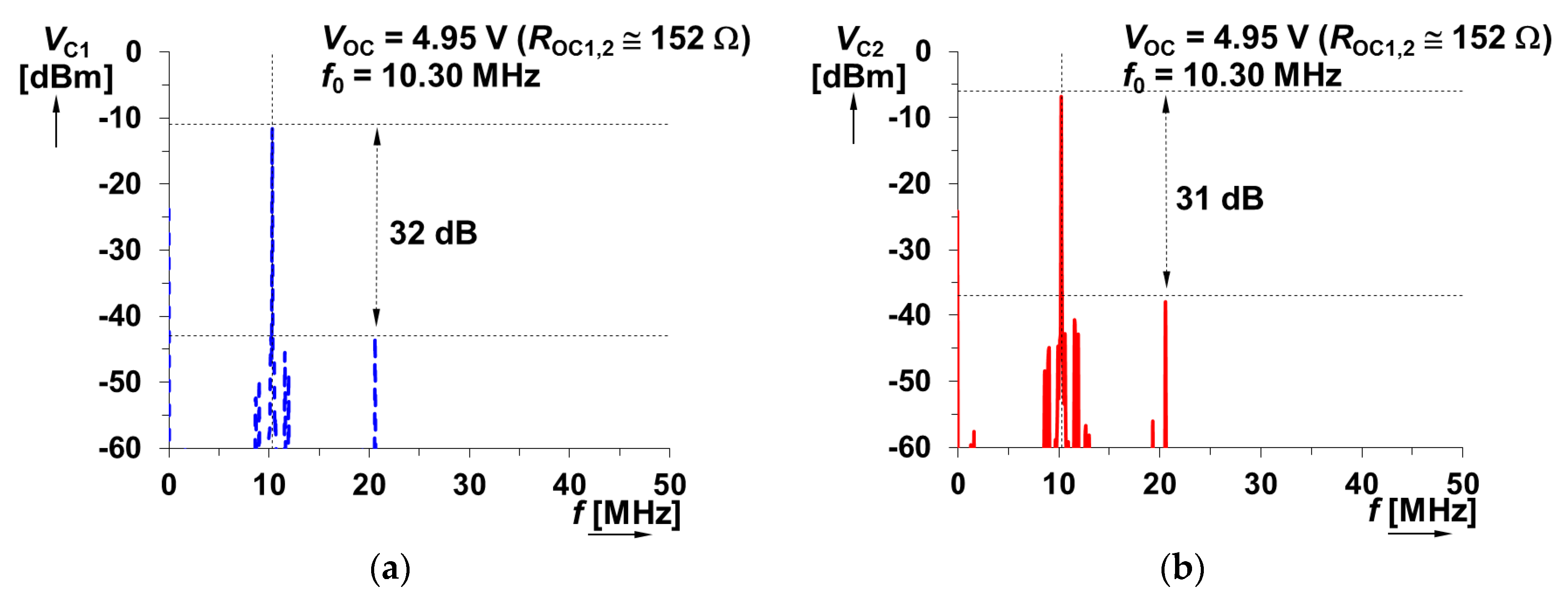
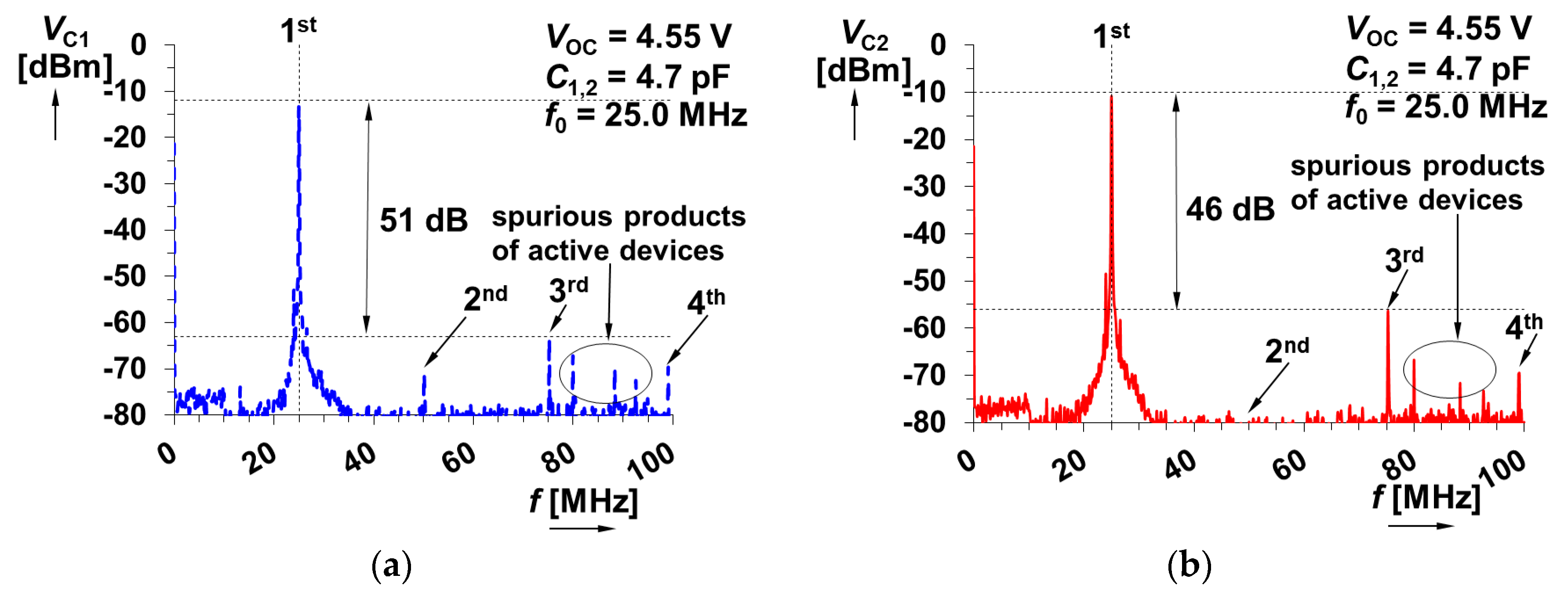
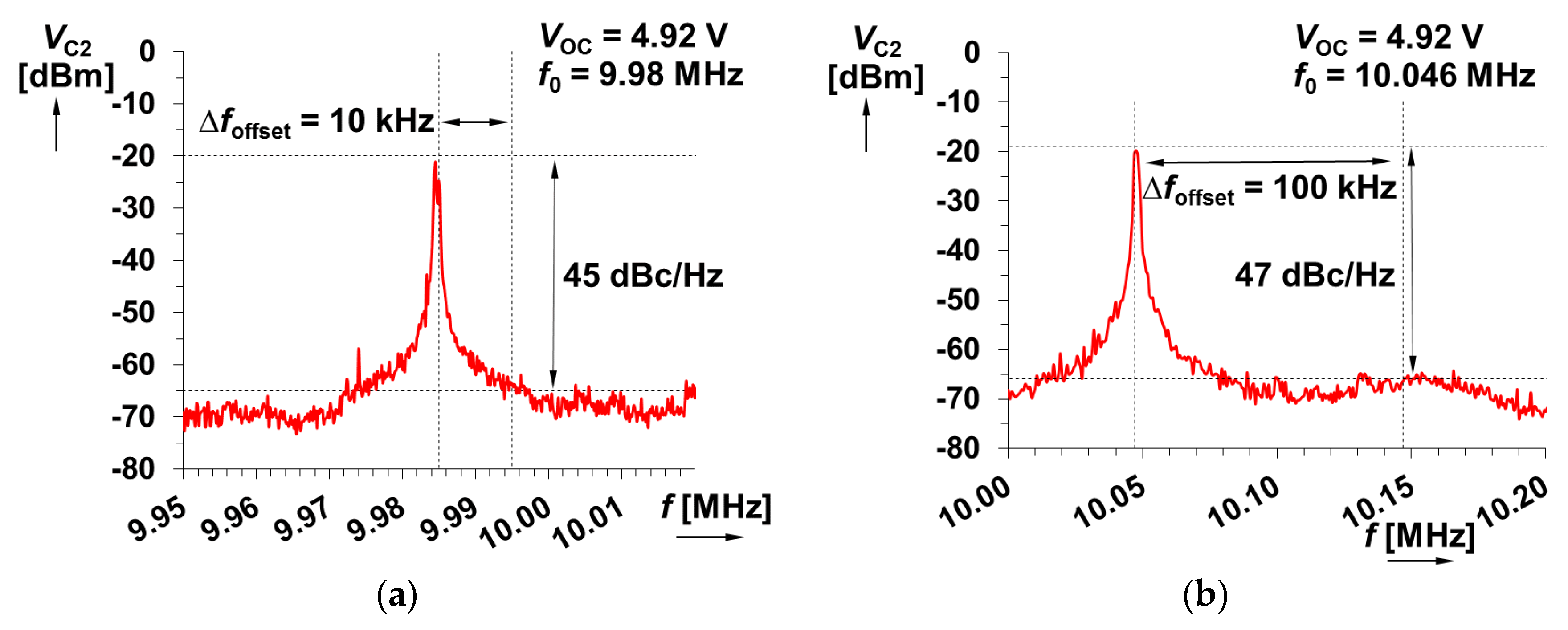
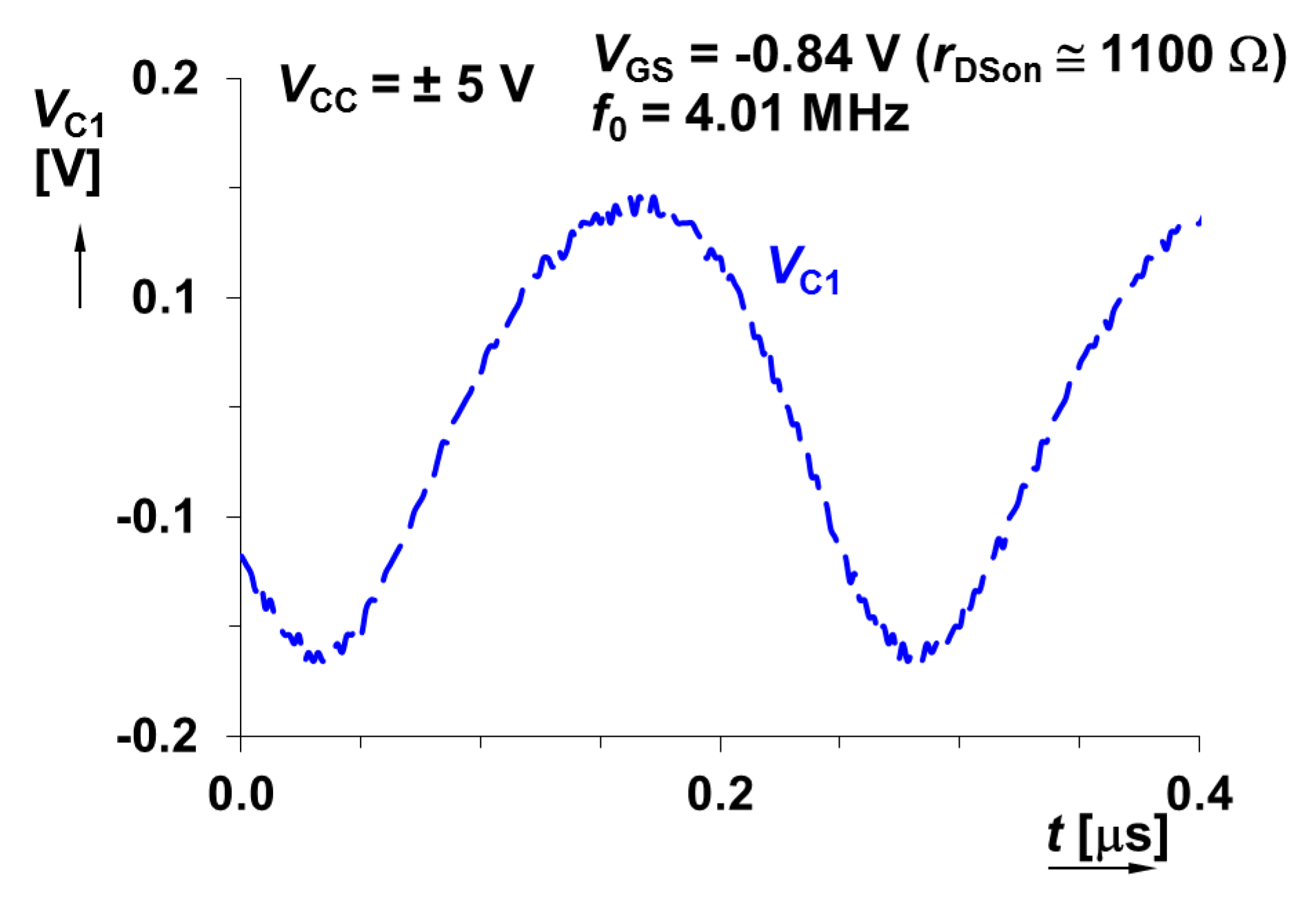
| Figure 1 | 2/4 | CCII−, ECCII+ | 2 R floating and grounded | ~R−1 | ~R−1 | 10.3 (25.0) IV | <3.3 | 570 V | >45 | - | B | Yes | 45 | M | Yes |
| Reference | [14] | [17] (Figure 1a) | Proposed (Figure 1) |
|---|---|---|---|
| No. of passive elements | 2 | 4 | 4 |
| No. of elements (parameters) suitable for FO control | 2 | 2 | 2 |
| No. of elements (parameters) used for FO control | 1 | 1 | 2 |
| Solution of FO control | resistance (RX) of X terminal | external resistance value (in X terminal of conveyor) * | resistances of optocouplers |
| Allowed character of FO dependence | ~R−1 | ~R−1 | ~R−1 |
| Tested character of FO dependence | ~R−1/2 | ~R−1/2 | ~R−1 |
| Range of driving force | 1 μA→500 μA | 8 kΩ→15 kΩ * | 1.73→4.95 V |
| Ratio of driving force | 500:1 (bias current) | 1.9:1 * | 3:1 (control voltage) |
| Obtained FO range (MHz) | 0.2→1.8 | 0.120→0.165 | 1.05→10.30 |
| Ratio of FO | 9:1 | 1.4:1 | 10:1 |
| Active parameter for CO control | Yes | No | Yes |
| Type of active parameter suitable for CO control | current gain | N/A | current gain |
| Outputs (nodes) used | 1 | 2 | 2 |
| Produced phase shift (°) | N/A | 90 | 45 |
| Amplitude stabilization | N/A | N/A | Yes |
| Generated levels (amplitudes) independent on tuning process | N/A | No | Yes |
| Output amplitude | 25 mV | 80→125 μA, 95→110 μA | 100 mV, 150 mV |
| THD (%) | 1→7 | 0.7→1.4 | 0.7→3.3 |
| Verification | simulated | simulated | measured |
| VOC (V) | IOC (μA) | ROCi (Ω) | ROCmeas (Ω) | ROCi + RX1,2 (Ω) | ROCmeas + RX1,2 (Ω) | f0i (MHz) | f0e (MHz) | f0e + 10pF (MHz) | f0meas (MHz) |
|---|---|---|---|---|---|---|---|---|---|
| 4.95 | 1500 | 133 | 152 | 228 | 247 | 25.27 | 13.32 | 11.21 | 10.30 |
| 3.74 | 884 | 226 | 227 | 321 | 322 | 16.78 | 10.05 | 8.46 | 8.01 |
| 2.82 | 500 | 400 | 366 | 495 | 461 | 9.57 | 6.64 | 5.59 | 6.02 |
| 2.23 | 280 | 714 | 629 | 809 | 724 | 5.18 | 3.98 | 3.35 | 4.00 |
| 2.04 | 193 | 1039 | 936 | 1134 | 1030 | 3.45 | 2.77 | 2.33 | 3.01 |
| 1.86 | 123 | 1633 | 1524 | 1727 | 1620 | 2.04 | 1.70 | 1.43 | 2.01 |
| 1.73 | 75 | 2667 | 2780 | 2762 | 2880 | 1.04 | 0.89 | 0.75 | 1.05 |
| Method | Frequency Features | Dynamical Features | Linearity | Response on Control | Value Range | Significant Additional Power Consumption | Notes |
|---|---|---|---|---|---|---|---|
| Resistor (potentiometer) | good * | good | good | fast | large (several decades) | No | mechanical features |
| Optocoupler with resistive output | good * | good (hundreds of mV) | good (nonlinear deviation up to units of %) | average (units of ms) | large | No | - |
| J-FET (or unipolar transistor) | good | bad (tens of mV) | bad (nonlinear deviation tens of %) | fast | large | No | maintain in linear regime |
| Digital potentiometer | ** | good | good | ** | limited (number of switched segments/bits) | Yes | discontinuous adjusting |
| D/A converter | *** | good | good | *** | limited (number of bits) | Yes | discontinuous adjusting |
| Active analog solution (OTA for example) | good | average/bad | average/bad | fast | limited (can be even less than a decade for MOS solution) | Yes | - |
© 2018 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Sotner, R.; Jerabek, J.; Langhammer, L.; Dvorak, J. Design and Analysis of CCII-Based Oscillator with Amplitude Stabilization Employing Optocouplers for Linear Voltage Control of the Output Frequency. Electronics 2018, 7, 157. https://doi.org/10.3390/electronics7090157
Sotner R, Jerabek J, Langhammer L, Dvorak J. Design and Analysis of CCII-Based Oscillator with Amplitude Stabilization Employing Optocouplers for Linear Voltage Control of the Output Frequency. Electronics. 2018; 7(9):157. https://doi.org/10.3390/electronics7090157
Chicago/Turabian StyleSotner, Roman, Jan Jerabek, Lukas Langhammer, and Jan Dvorak. 2018. "Design and Analysis of CCII-Based Oscillator with Amplitude Stabilization Employing Optocouplers for Linear Voltage Control of the Output Frequency" Electronics 7, no. 9: 157. https://doi.org/10.3390/electronics7090157
APA StyleSotner, R., Jerabek, J., Langhammer, L., & Dvorak, J. (2018). Design and Analysis of CCII-Based Oscillator with Amplitude Stabilization Employing Optocouplers for Linear Voltage Control of the Output Frequency. Electronics, 7(9), 157. https://doi.org/10.3390/electronics7090157





