Transient Stability Analysis and Enhancement of Grid-Forming Converters: A Comprehensive Review
Abstract
1. Introduction
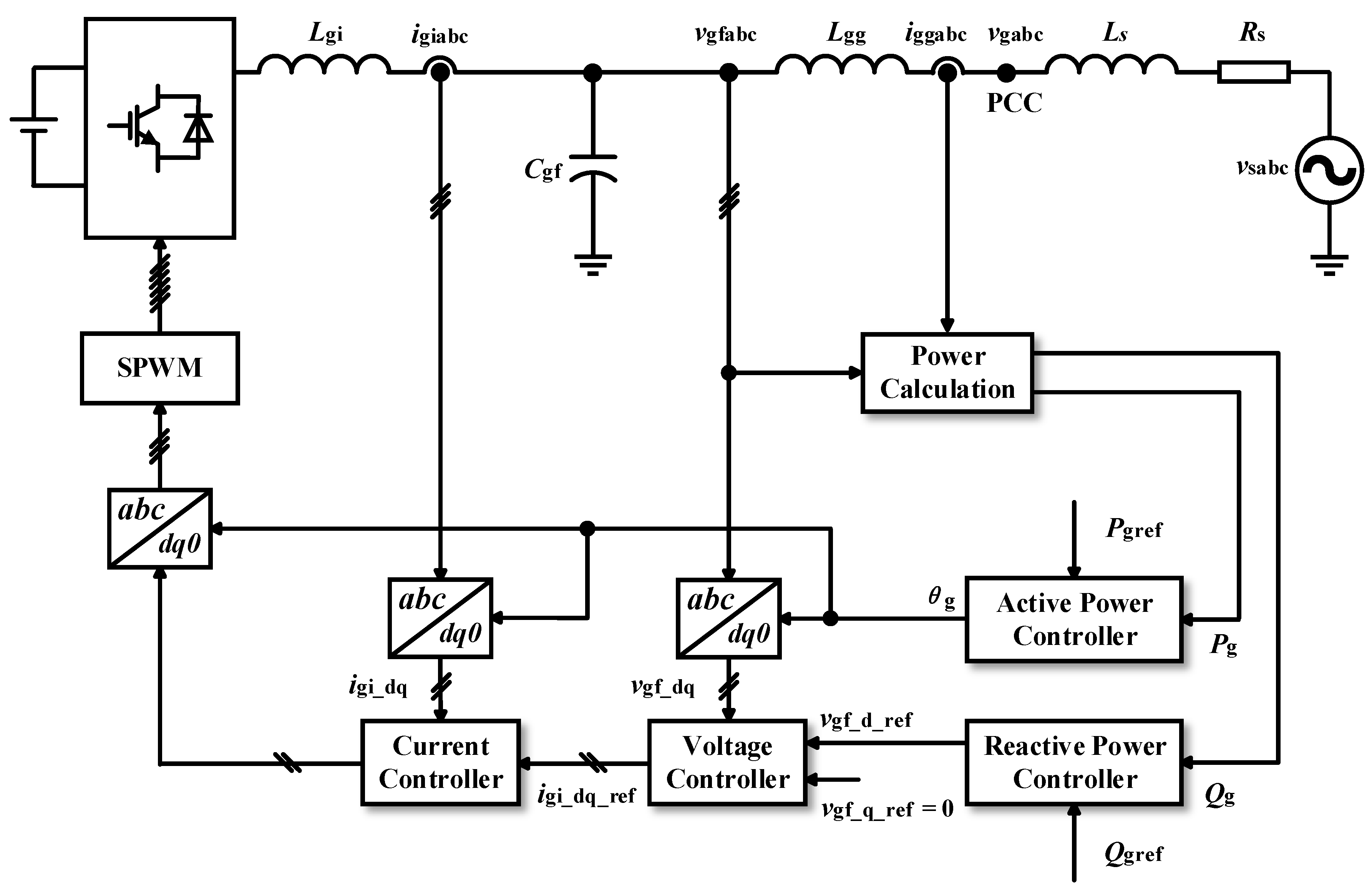
2. System Configuration and Control Structure of GFMCs
2.1. Schematics and Control Structure of GFMCs
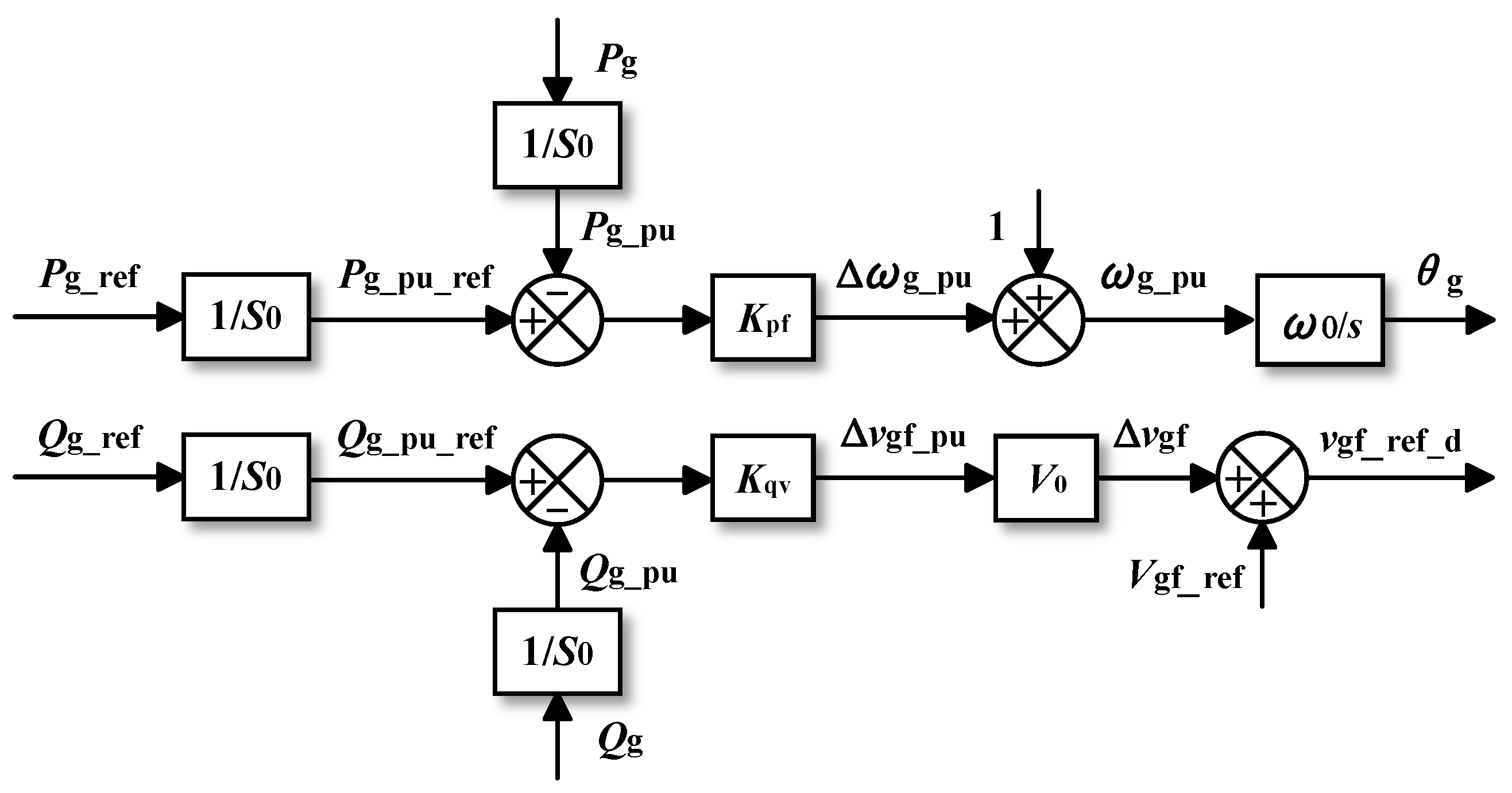
2.2. First-Order Control Scheme: Droop Control
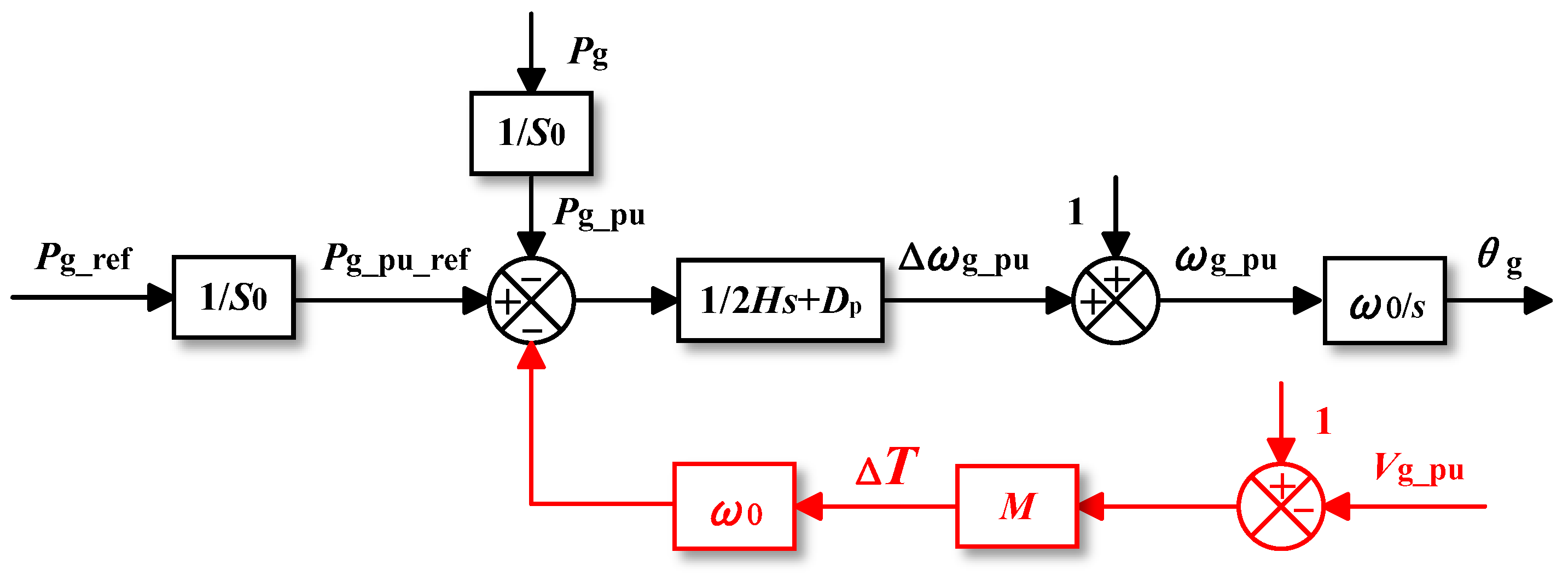
2.3. Second-Order Control Scheme: VSM Control
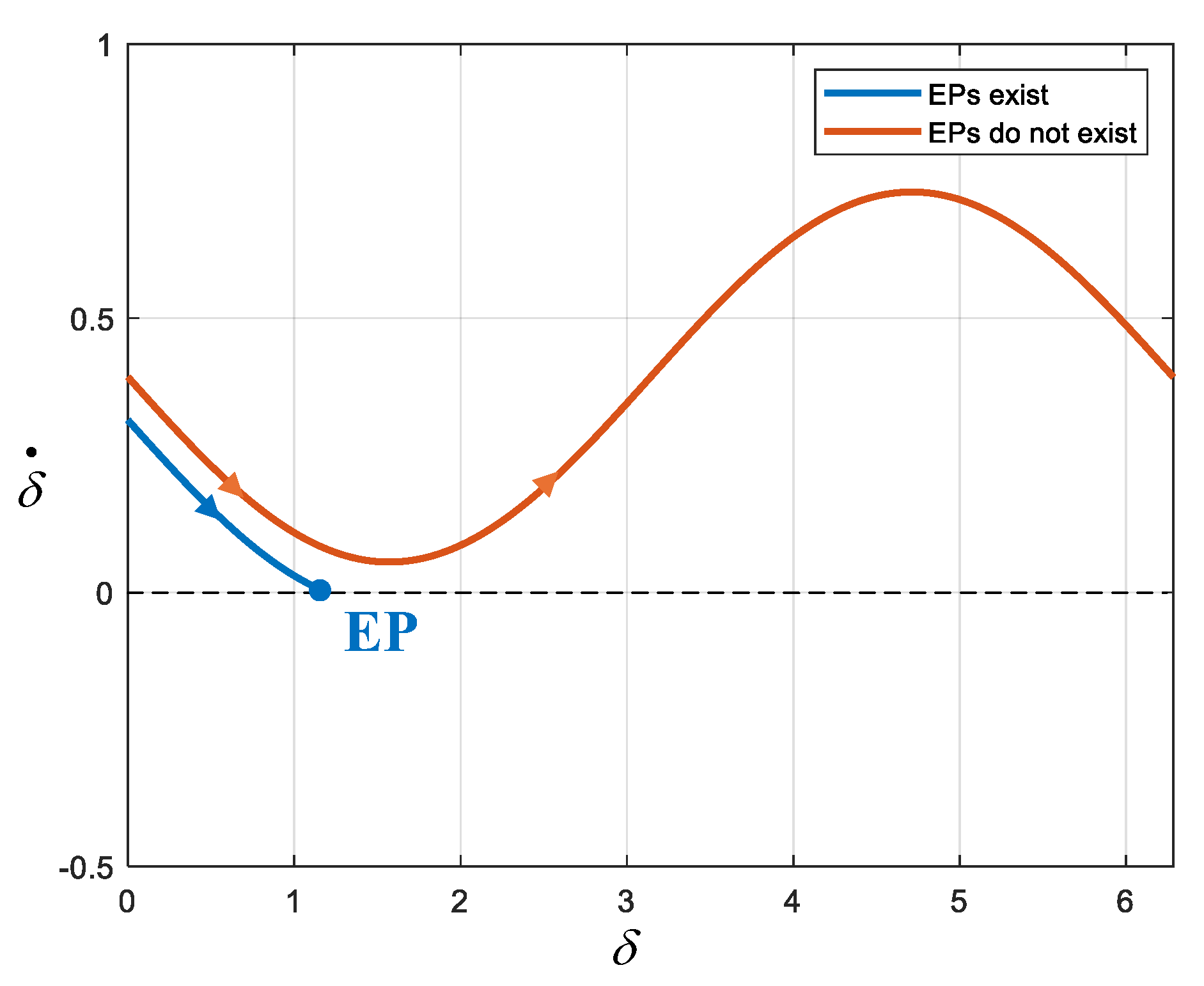
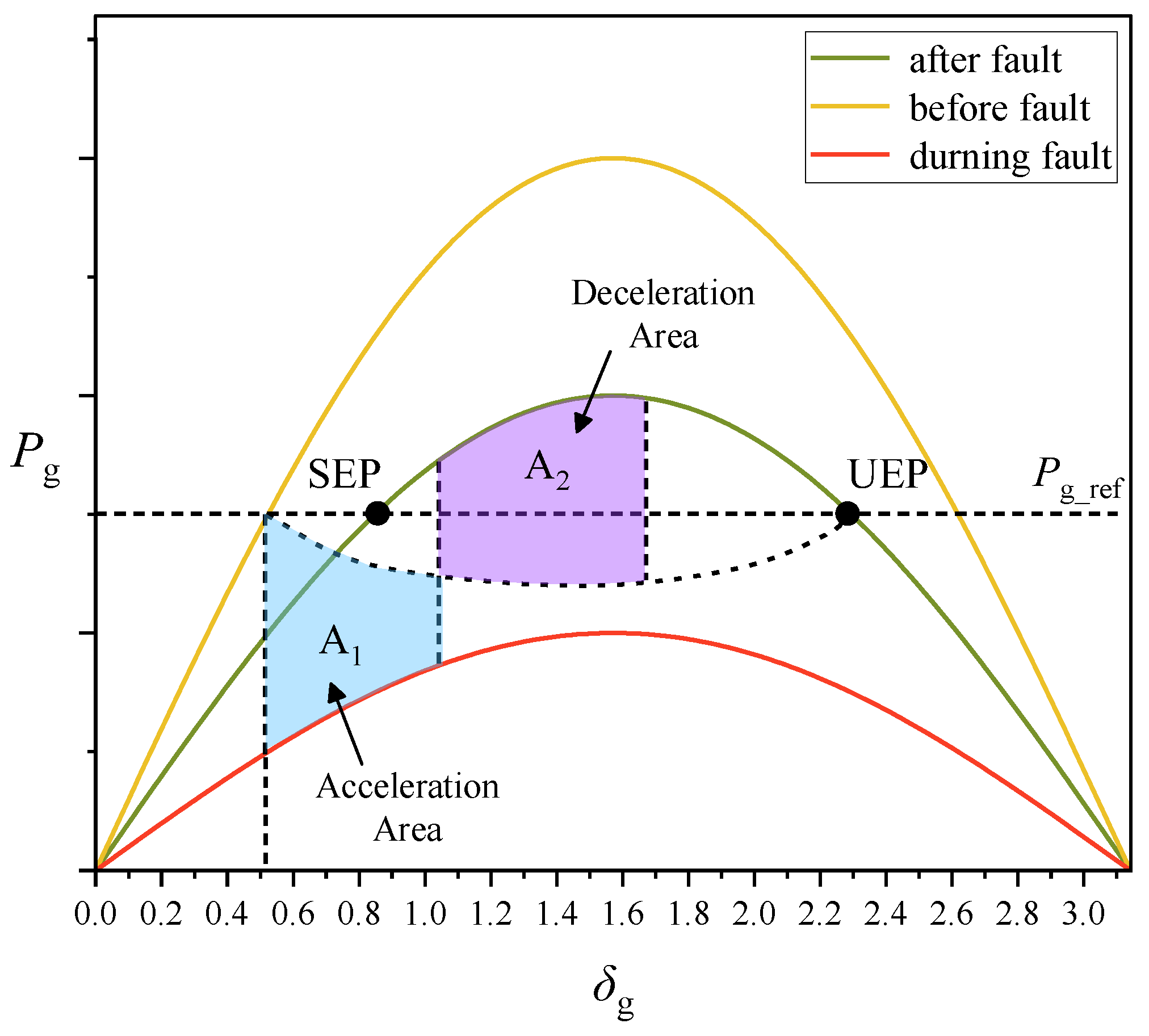
3. Transient Instability Mechanism of GFMC
3.1. Transient Instability Mechanism of Droop Control
3.2. Transient Instability Mechanism of VSM Control
4. Transient Stability Enhancement Methods of GFMCs
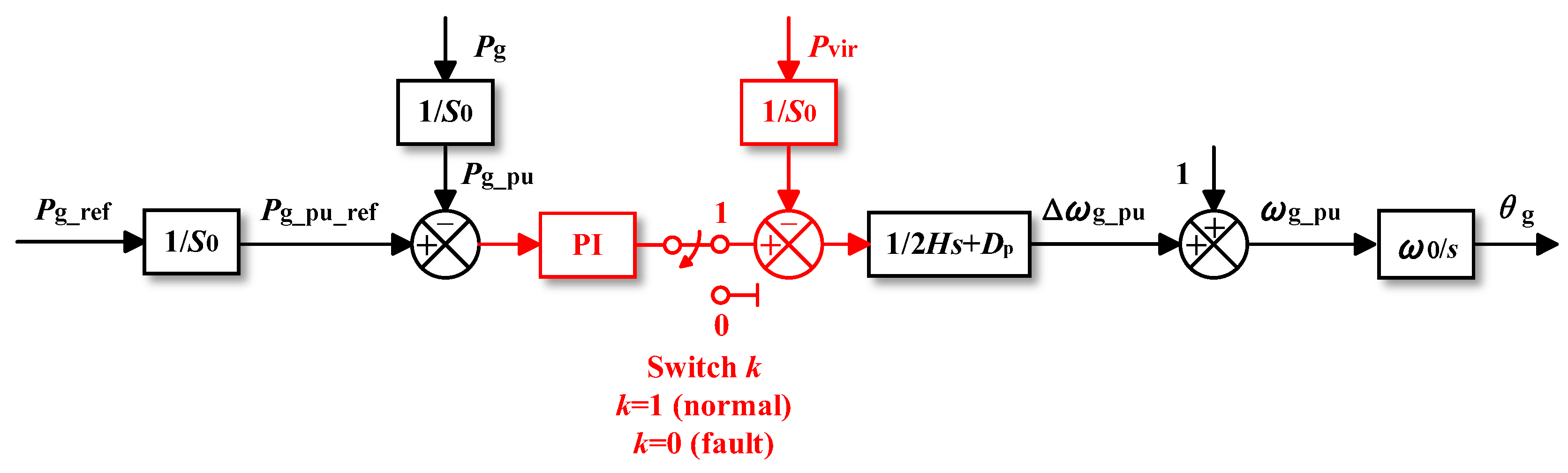
4.1. Active Power Regulation
4.2. Voltage Regulation
4.3. Reactive Power Regulation
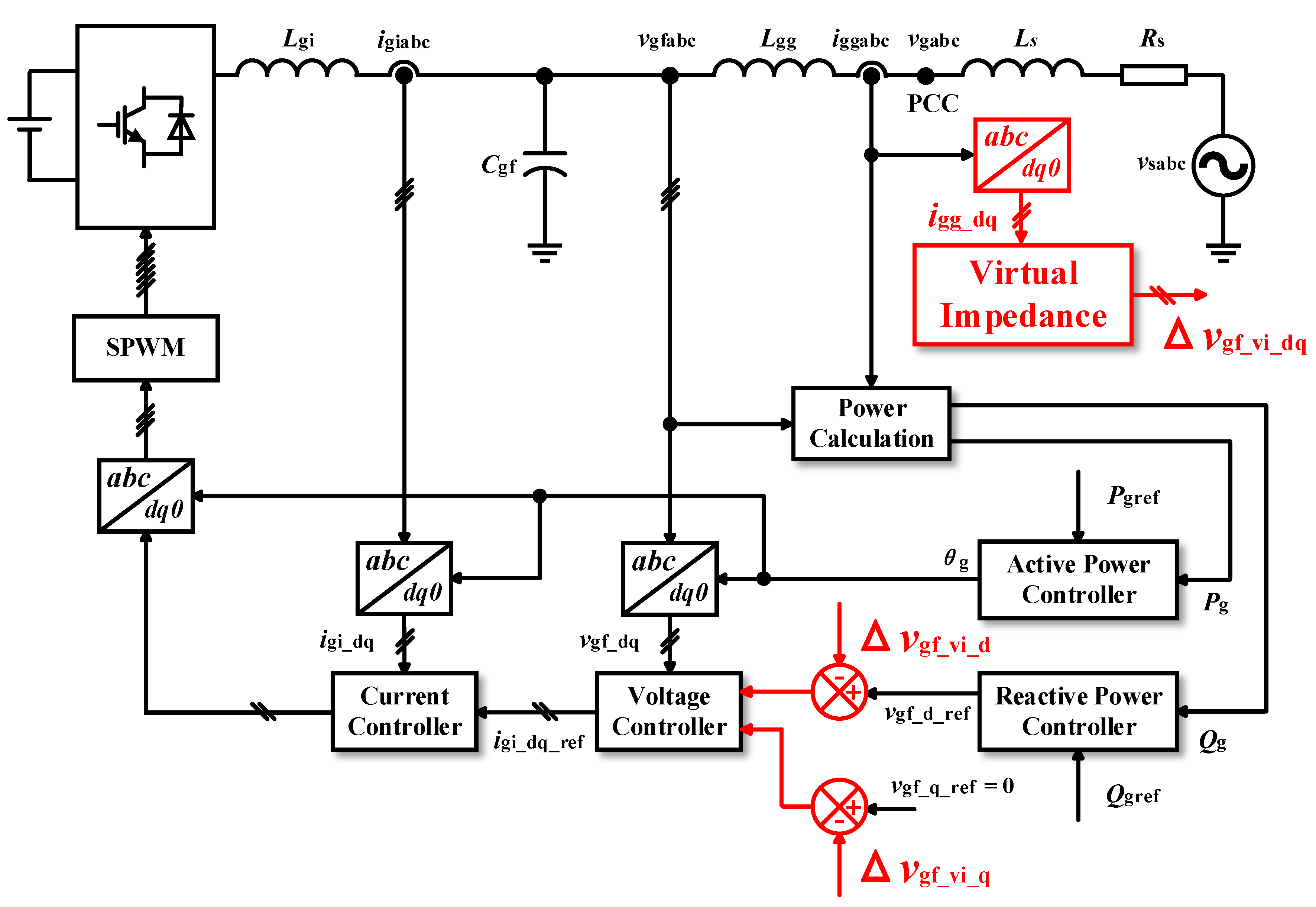
4.4. Virtual Impedance Regulation
4.5. Inertia Regulation
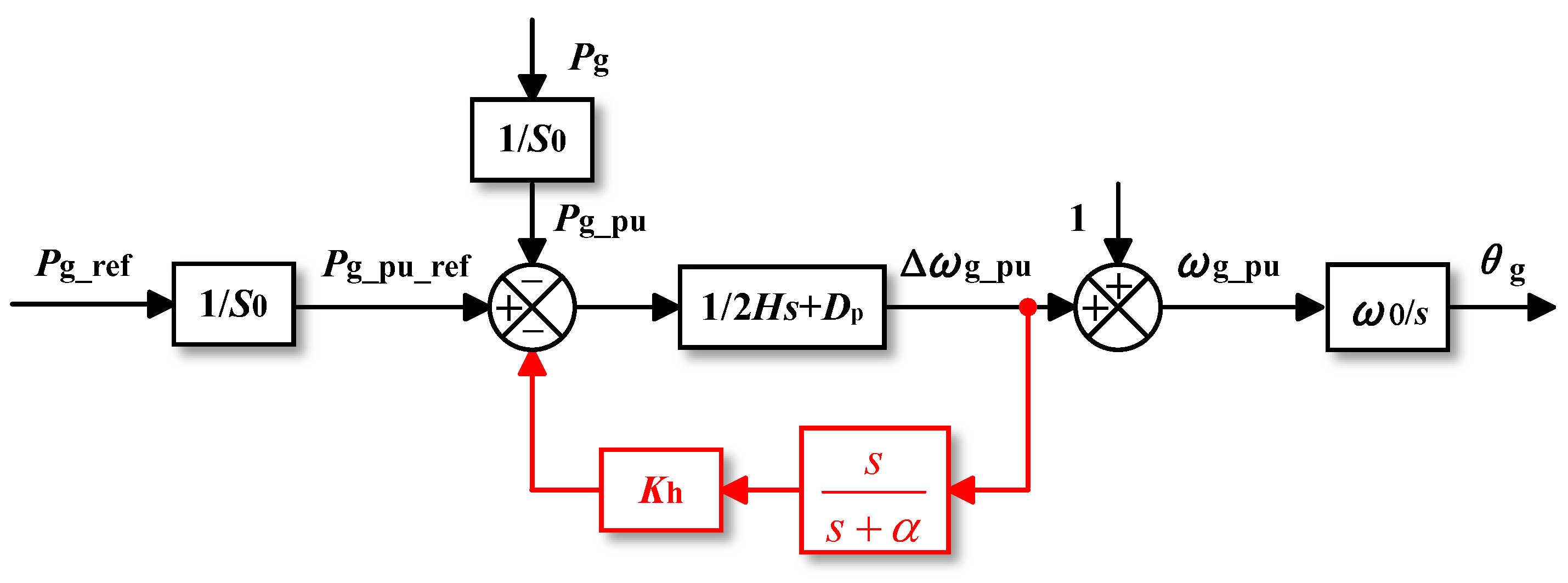
4.6. Damping Regulation
4.7. Comparison of Transient Stability Enhancement Methods
5. Future Trends
5.1. Comprehensive Stability Enhancement
5.2. Transient Stability Enhancement Method for Multi-Machine Systems
5.3. Utilizing Advanced Control Theory
6. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Wang, X.; Taul, M.G.; Wu, H.; Liao, Y.; Blaabjerg, F.; Harnefors, L. Grid-Synchronization Stability of Converter-Based Resources-An Overview. IEEE Open J. Ind. Appl. 2020, 1, 115–134. [Google Scholar] [CrossRef]
- Rocabert, J.; Luna, A.; Blaabjerg, F.; Rodríguez, P. Control of Power Converters in AC Microgrids. IEEE Trans. Power Electron. 2012, 27, 4734–4749. [Google Scholar] [CrossRef]
- Babu, V.V.; Roselyn, J.P.; Nithya, C.; Sundaravadivel, P. Development of Grid-Forming and Grid-Following Inverter Control in Microgrid Network Ensuring Grid Stability and Frequency Response. Electronics 2024, 13, 1958. [Google Scholar] [CrossRef]
- Ndreko, M.; Rüberg, S.; Winter, W. Grid forming control scheme for power systems with up to 100% power electronic interfaced generation: A case study on Great Britain test system. IET Renew. Power Gener. 2020, 14, 1268–1281. [Google Scholar] [CrossRef]
- Yu, C.; Xu, H.; Chen, C.; Sun, M.; Fu, X.; Zhang, X. A unified approach to modeling and stability equivalence analysis for grid-forming and grid-following converters. Electr. Power Syst. Res. 2024, 235, 110861. [Google Scholar] [CrossRef]
- Li, C.; Huang, Y.; Deng, H.; Zhang, X.; Zhao, H. A novel grid-forming technology for transient stability enhancement of power system with high penetration of renewable energy. Int. J. Electr. Power Energy Syst. 2022, 143, 108402. [Google Scholar] [CrossRef]
- Rosso, R.; Wang, X.; Liserre, M.; Lu, X.; Engelken, S. Grid-Forming Converters: Control Approaches, Grid-Synchronization, and Future Trends-A Review. IEEE Open J. Ind. Appl. 2021, 2, 93–109. [Google Scholar] [CrossRef]
- Zhang, H.; Xiang, W.; Lin, W.; Wen, J. Grid Forming Converters in Renewable Energy Sources Dominated Power Grid: Control Strategy, Stability, Application, and Challenges. J. Mod. Power Syst. Clean Energy 2021, 9, 1239–1256. [Google Scholar] [CrossRef]
- Yang, Z.; Zhan, M.; Liu, D.; Ye, C.; Cao, K.; Cheng, S. Small-Signal Synchronous Stability of a New-Generation Power System With 100% Renewable Energy. IEEE Trans. Power Syst. 2023, 38, 4269–4280. [Google Scholar] [CrossRef]
- Si, W.; Fang, J. Transient Stability Improvement of Grid-Forming Converters through Voltage Amplitude Regulation and Reactive Power Injection. IEEE Trans. Power Electron. 2023, 38, 12116–12125. [Google Scholar] [CrossRef]
- Li, M.; Shu, S.; Wang, Y.; Yu, P.; Liu, Y.; Zhang, Z.; Hu, W.; Blaabjerg, F. Analysis and Improvement of Large-Disturbance Stability for Grid-Connected VSG Based on Output Impedance Optimization. IEEE Trans. Power Electron. 2022, 37, 9807–9826. [Google Scholar] [CrossRef]
- Kothari, D.P.; Nagrath, I.J. Modern Power System Analysis; Tata McGraw-Hill Publishing Company: New Delhi, India, 2011; pp. 426–459. [Google Scholar]
- Pan, D.; Wang, X.; Liu, F.; Shi, R. Transient Stability of Voltage-Source Converters With Grid-Forming Control: A Design-Oriented Study. IEEE J. Emerg. Sel. Top. Power Electron. 2020, 8, 1019–1033. [Google Scholar] [CrossRef]
- Wu, H.; Wang, X. Design-Oriented Transient Stability Analysis of Grid-Connected Converters With Power Synchronization Control. IEEE Trans. Ind. Electron. 2019, 66, 6473–6482. [Google Scholar] [CrossRef]
- Gao, Z.; Du, W.; Wang, H.F. Transient stability analysis of a grid-connected type-4 wind turbine with grid-forming control during the fault. Int. J. Electr. Power Energy Syst. 2024, 155, 109514. [Google Scholar] [CrossRef]
- He, X.; Dörfler, F. Passivity and Decentralized Stability Conditions for Grid-Forming Converters. IEEE Trans. Power Syst. 2024, 39, 5447–5450. [Google Scholar] [CrossRef]
- He, X.; Huang, L.; Häberle, V.; Dörfler, F. Quantitative Stability Conditions for Grid-Forming Converters With Complex Droop Control. IEEE Trans. Power Electron. 2024, 39, 10834–10851. [Google Scholar] [CrossRef]
- Liu, C.C.; Tse, C.K.; Huang, M.; Wu, Z.; Han, H.; Yang, J. Design-Oriented Transient Stability Analysis of Grid-Connected Converters: A Comparative Study of Analysis Methods. IEEE Trans. Power Electron. 2025, 40, 749–763. [Google Scholar] [CrossRef]
- Sun, Z.; Zhang, S.; Zhang, C.; Tao, X.; Dai, T. A robust synchronous stability control for virtual synchronous generator of renewable energy adapted to large power grid voltage drop. Electr. Power Syst. Res. 2024, 232, 110388. [Google Scholar] [CrossRef]
- Xu, H.; Zhang, D.; Chen, L.; Li, Y.; Wang, Y.; Hu, T.; Qiao, L.; Wei, C. Coordination of Resistive SFCL and Additional Power Controller for Transient Stability Enhancement of Virtual Synchronous Generator. IEEE Trans. Appl. Supercond. 2021, 31, 3091093. [Google Scholar] [CrossRef]
- Shuai, Z.; Shen, C.; Liu, X.; Li, Z.; Shen, Z.J. Transient Angle Stability of Virtual Synchronous Generators Using Lyapunov’s Direct Method. IEEE Trans. Smart Grid 2019, 10, 4648–4661. [Google Scholar] [CrossRef]
- Xiang, Z.; Ni, Q.; Li, Z.; Xu, J.; Li, S.; Zhang, Z.; Xu, Z. Transient Stability Analysis of Grid-forming Converter Based on Virtual Synchronous Generator. In Proceedings of the 2022 Asian Conference on Frontiers of Power and Energy (ACFPE), Virtual, 21–23 October 2022; pp. 118–124. [Google Scholar]
- Wu, H.; Wang, X. A Mode-Adaptive Power-Angle Control Method for Transient Stability Enhancement of Virtual Synchronous Generators. IEEE J. Emerg. Sel. Top. Power Electron. 2020, 8, 1034–1049. [Google Scholar] [CrossRef]
- Shi, H.; Shi, P.; Zhou, B.; Wang, X.; Zeng, X.; Ma, J. Transient Synchronization Stability Analysis and Enhancement Control for Power Self-Synchronization Control Converters. Electronics 2024, 13, 3416. [Google Scholar] [CrossRef]
- Sun, R.; Ma, J.; Yang, W.; Wang, S.; Liu, T. Transient Synchronization Stability Control for LVRT With Power Angle Estimation. IEEE Trans. Power Electron. 2021, 36, 10981–10985. [Google Scholar] [CrossRef]
- Xiong, X.; Wu, C.; Blaabjerg, F. An Improved Synchronization Stability Method of Virtual Synchronous Generators Based on Frequency Feedforward on Reactive Power Control Loop. IEEE Trans. Power Electron. 2021, 36, 9136–9148. [Google Scholar] [CrossRef]
- Chen, M.; Zhou, D.; Blaabjerg, F. Enhanced Transient Angle Stability Control of Grid-Forming Converter Based on Virtual Synchronous Generator. IEEE Trans. Ind. Electron. 2022, 69, 9133–9144. [Google Scholar] [CrossRef]
- Avila-Martinez, R.E.; Renedo, J.; Rouco, L.; Garcia-Cerrada, A.; Sigrist, L.; Qoria, T.; Guillaud, X. Fast Voltage Boosters to Improve Transient Stability of Power Systems With 100% of Grid-Forming VSC-Based Generation. IEEE Trans. Energy Convers. 2022, 37, 2777–2789. [Google Scholar] [CrossRef]
- ávila-Martínez, R.E.; Renedo, J.; Rouco, L.; Garcia-Cerrada, A.; Sigrist, L.; Guillaud, X.; Qoria, T. Impact of current limiters and fast voltage boosters in grid-forming VSC-based generators on transient stability. Electr. Power Syst. Res. 2024, 235, 110753. [Google Scholar] [CrossRef]
- Pan, D.; Wang, X.; Liu, F.; Shi, R. Transient Stability Impact of Reactive Power Control on Grid-Connected Converters. In Proceedings of the 2019 IEEE Energy Conversion Congress and Exposition (ECCE), Baltimore, MD, USA, 29 September–3 October 2019; pp. 4311–4316. [Google Scholar]
- Jin, Z.; Wang, X. A DQ-Frame Asymmetrical Virtual Impedance Control for Enhancing Transient Stability of Grid-Forming Inverters. IEEE Trans. Power Electron. 2022, 37, 4535–4544. [Google Scholar] [CrossRef]
- Chen, S.; Sun, Y.; Han, H.; Fu, S.; Luo, S.; Shi, G. A Modified VSG Control Scheme With Virtual Resistance to Enhance Both Small-Signal Stability and Transient Synchronization Stability. IEEE Trans. Power Electron. 2023, 38, 6005–6014. [Google Scholar] [CrossRef]
- Hu, X.; Li, Z.; Pan, C.; Li, H.; Liang, Y. An Adaptive Virtual-Impedance-Based Current-Limiting Method with the Functionality of Transient Stability Enhancement for Grid-Forming Converter. Electronics 2024, 13, 2750. [Google Scholar] [CrossRef]
- Qoria, T.; Gruson, F.; Colas, F.; Kestelyn, X.; Guillaud, X. Current limiting algorithms and transient stability analysis of grid-forming VSCs. Electr. Power Syst. Res. 2020, 189, 106726. [Google Scholar] [CrossRef]
- Winkens, A.; Klein-Helmkamp, F.; Ulbig, A. Experimental Validation of Virtual Inductance for Synchronization of Grid-Forming Converters. IFAC-PapersOnLine 2024, 58, 56–61. [Google Scholar] [CrossRef]
- Xiaoxiao, M.; Yuchen, W.; Xun, M. Transient Synchronization Stability Analysis of Grid-Forming Converters Considering Virtual Negative Impedance Decoupling. In Proceedings of the 2024 IEEE 10th International Power Electronics and Motion Control Conference (IPEMC2024-ECCE Asia), Chengdu, China, 17–20 May 2024; pp. 3909–3914. [Google Scholar]
- Qoria, T.; Wu, H.; Wang, X.; Colak, I. Variable Virtual Impedance-Based Overcurrent Protection for Grid-Forming Inverters: Small-Signal, Large-Signal Analysis and Improvement. IEEE Trans. Smart Grid 2023, 14, 3324–3336. [Google Scholar] [CrossRef]
- Xiong, X.; Wu, C.; Cheng, P.; Blaabjerg, F. An Optimal Damping Design of Virtual Synchronous Generators for Transient Stability Enhancement. IEEE Trans. Power Electron. 2021, 36, 11026–11030. [Google Scholar] [CrossRef]
- Goh, H.H.; Ma, Y.; Lim, C.S.; Zhang, D.; Dai, W.; Liu, J.; Li, G.; Kurniawan, T.A. Comparative assessment of single-loop droop controlled grid-forming converter and its damping enhancement. Energy Rep. 2023, 9, 1048–1056. [Google Scholar] [CrossRef]
- Ge, P.; Tu, C.; Xiao, F.; Guo, Q.; Gao, J. Design-Oriented Analysis and Transient Stability Enhancement Control for a Virtual Synchronous Generator. IEEE Trans. Ind. Electron. 2023, 70, 2675–2684. [Google Scholar] [CrossRef]
- Liu, A.; Liu, J. Improved transient stability of virtual synchronous generators for grid voltage cascading faults. Electr. Power Syst. Res. 2022, 213, 108661. [Google Scholar] [CrossRef]
- Hou, X.; Han, H.; Zhong, C.; Yuan, W.; Yi, M.; Chen, Y. Improvement of transient stability in inverter-based AC microgrid via adaptive virtual inertia. In Proceedings of the 2016 IEEE Energy Conversion Congress and Exposition (ECCE), Milwaukee, WI, USA, 18–22 September 2016; pp. 1–6. [Google Scholar]
- Dolado, J.; Rodríguez Amenedo, J.L.; Arnaltes, S.; Eloy-Garcia, J. Improving the Inertial Response of a Grid-Forming Voltage Source Converter. Electronics 2022, 11, 2303. [Google Scholar] [CrossRef]
- Alipoor, J.; Miura, Y.; Ise, T. Power System Stabilization Using Virtual Synchronous Generator With Alternating Moment of Inertia. IEEE J. Emerg. Sel. Top. Power Electron. 2015, 3, 451–458. [Google Scholar] [CrossRef]
- Xiong, X.; Wu, C.; Hu, B.; Pan, D.; Blaabjerg, F. Transient Damping Method for Improving the Synchronization Stability of Virtual Synchronous Generators. IEEE Trans. Power Electron. 2021, 36, 7820–7831. [Google Scholar] [CrossRef]
- Yu, J.; Lu, X.; Xiong, S.; Xing, Y.; Liu, W.; Yu, J. Transient Stability Enhancement of Virtual Synchronous Generators Based on Additional Frequency Control. IEEE J. Emerg. Sel. Top. Power Electron. 2024, 12, 1129–1139. [Google Scholar] [CrossRef]
- Du, W.; Nguyen, Q.H.; Wang, S.; Kim, J.; Liu, Y.; Zhu, S.; Tuffner, F.K.; Huang, Z. Positive-Sequence Modeling of Droop-Controlled Grid-Forming Inverters for Transient Stability Simulation of Transmission Systems. IEEE Trans. Power Deliv. 2024, 39, 1736–1748. [Google Scholar] [CrossRef]
- Li, Z.; Xie, Z.; Xu, S.; Zhang, X. Improving Transient Stability of Grid-Forming DFIG Based on Enhanced Hybrid Synchronization Control. IEEE Trans. Ind. Electron. 2024, 71, 15881–15894. [Google Scholar] [CrossRef]
- Li, X.; Zhang, J.; Tian, Z.; Zha, X.; Wang, W.; Huang, M.; Shao, C. Transient stability analysis of converter-based islanded microgrids based on iterative equal area criterion considering reactive power loop dynamics and varying damping. J. Mod. Power Syst. Clean Energy 2023, 12, 1170–1182. [Google Scholar]
- Yuan, Y.; Ma, J.; Wang, S.; Wang, P.; Liu, T. Transient Stability Analysis of Grid-Forming Converters During Presynchronization Process in Islanded Mode. IEEE Trans. Power Electron. 2024, 39, 11007–11019. [Google Scholar] [CrossRef]
- D’Arco, S.; Suul, J.A. Equivalence of Virtual Synchronous Machines and Frequency-Droops for Converter-Based MicroGrids. IEEE Trans. Smart Grid 2014, 5, 394–395. [Google Scholar] [CrossRef]
- Kundur, P. Power System Stability and Control; McGrawHill: New York, NY, USA, 1994; pp. 17–36. [Google Scholar]
- Lei, J.; Xiang, X.; Liu, B.; Li, W.; He, X. Transient Stability Analysis of Grid forming Converters based on Damping Energy Visualization and Geometry Approximation. IEEE Trans. Ind. Electron. 2024, 71, 2510–2521. [Google Scholar] [CrossRef]
- Xin, H.; Huang, L.; Zhang, L.; Wang, Z.; Hu, J. Synchronous Instability Mechanism of P-f Droop-Controlled Voltage Source Converter Caused by Current Saturation. IEEE Trans. Power Syst. 2016, 31, 5206–5207. [Google Scholar] [CrossRef]
- Wang, J.; Zhang, X. Active Power and Voltage Cooperative Control for Improving Fault Ride-Through Capability of Grid-Forming Converters. IEEE Trans. Ind. Electron. 2024, 71, 12301–12311. [Google Scholar] [CrossRef]
- Lei, J.; Xiang, X.; Qu, Q.; Li, W.; He, X. On the Destabilizing Mechanism of Nonuniform Damping in Grid-Forming Converters Penetrated Multi-Machine System. IEEE Trans. Power Syst. 2024, 39, 5435–5438. [Google Scholar] [CrossRef]
- Luo, C.; Liao, S.; Chen, Y.; Huang, M. Quantitative Transient Stability Analysis for Parallel Grid-Tied Grid-Forming Inverters Considering Reactive Power Control. IEEE Trans. Power Electron. 2024, 40, 4780–4786. [Google Scholar] [CrossRef]
- Tian, Z.; Li, X.; Zha, X.; Tang, Y.; Sun, P.; Huang, M.; Yu, P. Transient Synchronization Stability of an Islanded AC Microgrid Considering Interactions Between Grid-Forming and Grid-Following Converters. IEEE J. Emerg. Sel. Top. Power Electron. 2023, 11, 4463–4476. [Google Scholar] [CrossRef]
- Cheng, H.; Shuai, Z.; Shen, C.; Liu, X.; Li, Z.; Shen, Z.J. Transient Angle Stability of Paralleled Synchronous and Virtual Synchronous Generators in Islanded Microgrids. IEEE Trans. Power Electron. 2020, 35, 8751–8765. [Google Scholar] [CrossRef]
- Choopani, M.; Hosseinian, S.H.; Vahidi, B. New Transient Stability and LVRT Improvement of Multi-VSG Grids Using the Frequency of the Center of Inertia. IEEE Trans. Power Syst. 2020, 35, 527–538. [Google Scholar] [CrossRef]
- Zhao, X.; Flynn, D. Grid-Forming Converter Angular Speed Freezing to Enhance Transient Stability in 100% Grid-Forming and Mixed Power Systems. IFAC-PapersOnLine 2022, 55, 425–430. [Google Scholar] [CrossRef]
- Zhao, X.; Flynn, D. Stability enhancement strategies for a 100% grid-forming and grid-following converter-based Irish power system. IET Renew. Power Gener. 2022, 16, 125–138. [Google Scholar] [CrossRef]
- Xu, C.; Zou, Z.; Yang, J.; Wang, Z.; Chen, W.; Buticchi, G. Transient Stability Analysis and Enhancement of Grid-Forming and Grid-Following Converters. IEEE J. Emerg. Sel. Top. Ind. Electron. 2024, 5, 1396–1408. [Google Scholar] [CrossRef]
- Ding, Y.; Gao, F.; Hou, K.; Jiang, Y.; Gao, Q. Transient Stability Enhancement of Grid-Forming Converter in an Islanded AC Microgrid. In Proceedings of the 2024 IEEE 10th International Power Electronics and Motion Control Conference (IPEMC2024-ECCE Asia), Chengdu, China, 17–20 May 2024; pp. 4865–4869. [Google Scholar]
- Chen, L.; Tang, J.; Qiao, X.; Chen, H.; Zhu, J.; Jiang, Y.; Zhao, Z.; Hu, R.; Deng, X. Investigation on Transient Stability Enhancement of Multi-VSG System Incorporating Resistive SFCLs Based on Deep Reinforcement Learning. IEEE Trans. Ind. Appl. 2024, 60, 1780–1793. [Google Scholar] [CrossRef]
- Shen, Y.; Zhou, Q.; Li, Y.; Shuai, Z. Data-driven Predictive Control for Grid-forming Converter Stability Enhancement in Island Microgrids. In Proceedings of the 2022 IEEE/IAS Industrial and Commercial Power System Asia (I&CPS Asia), Shanghai, China, 8–11 July 2022; pp. 1292–1297. [Google Scholar]




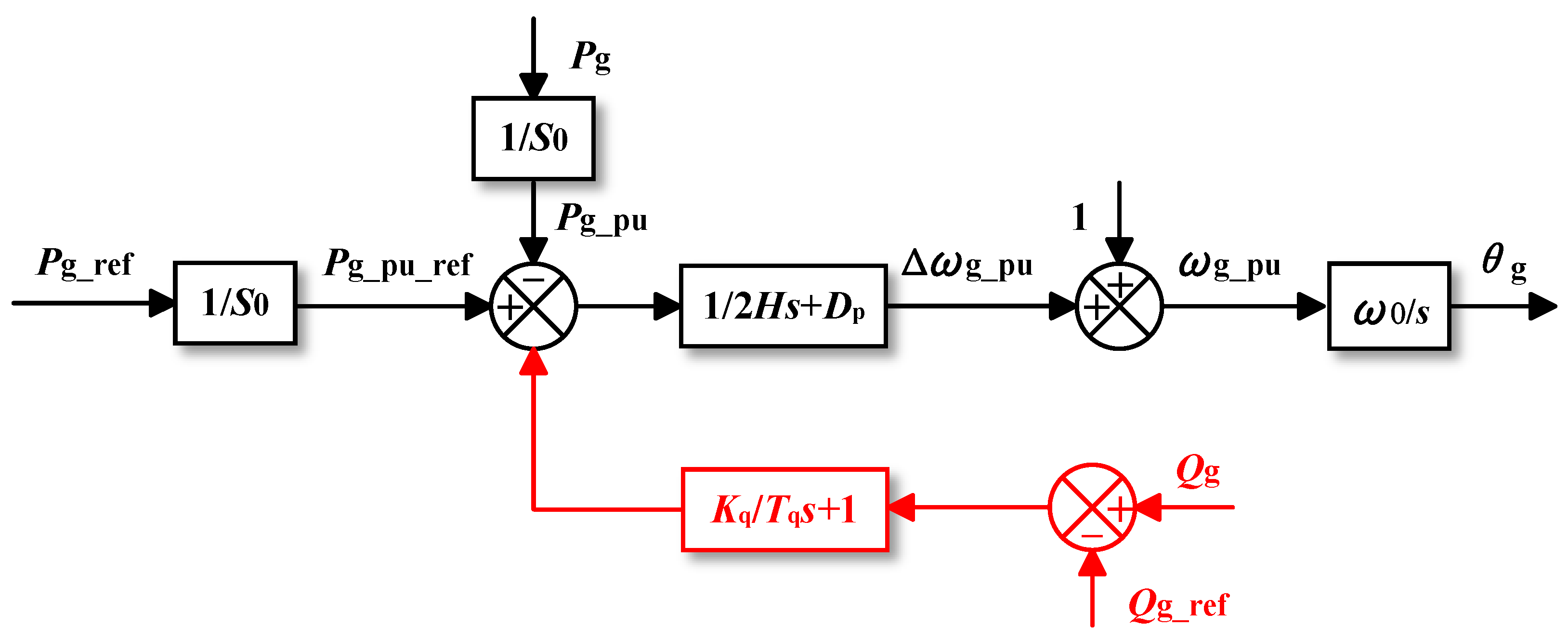

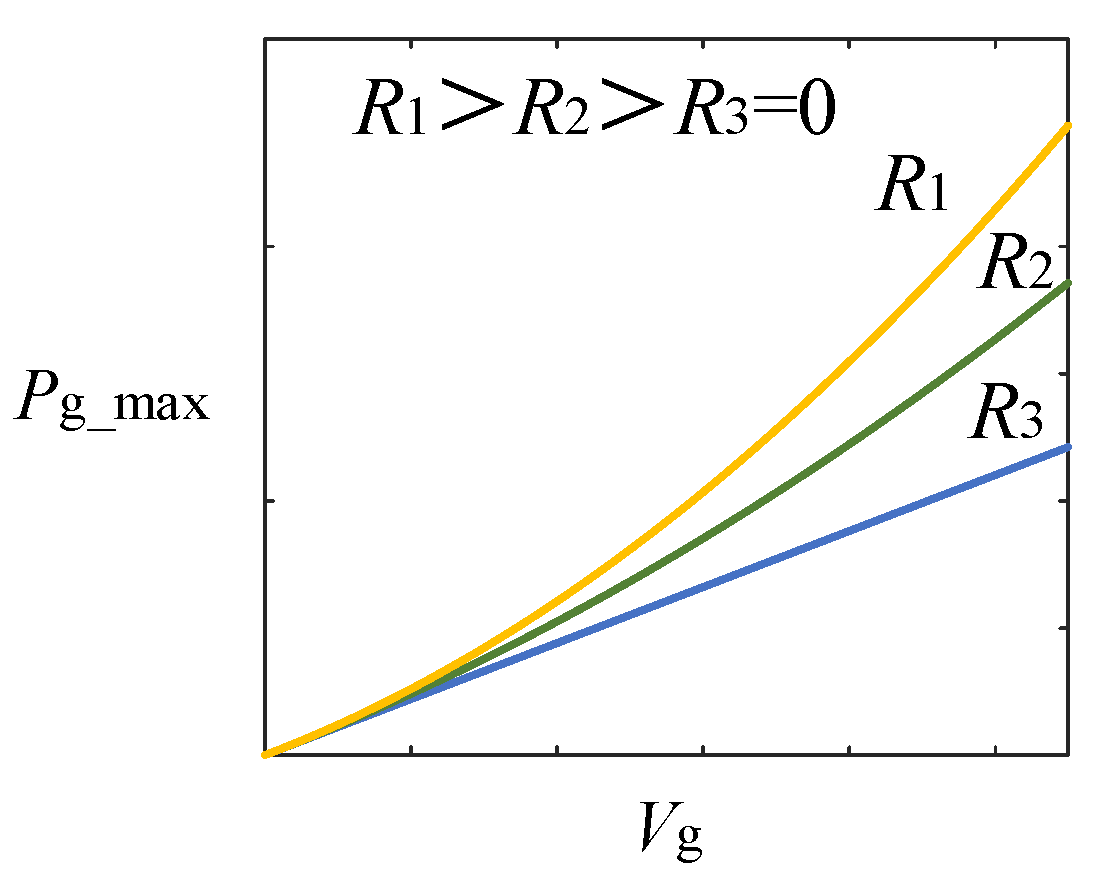
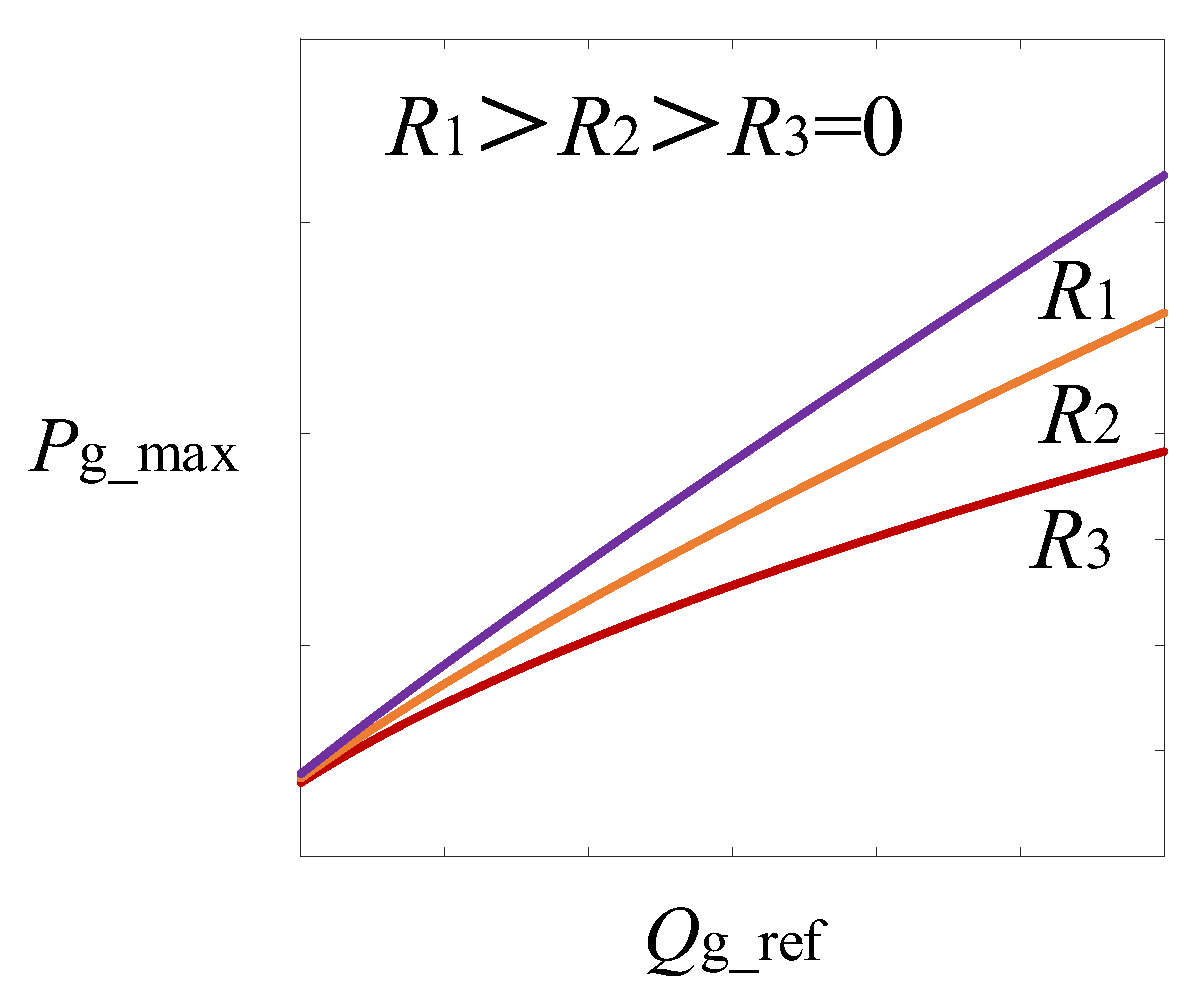

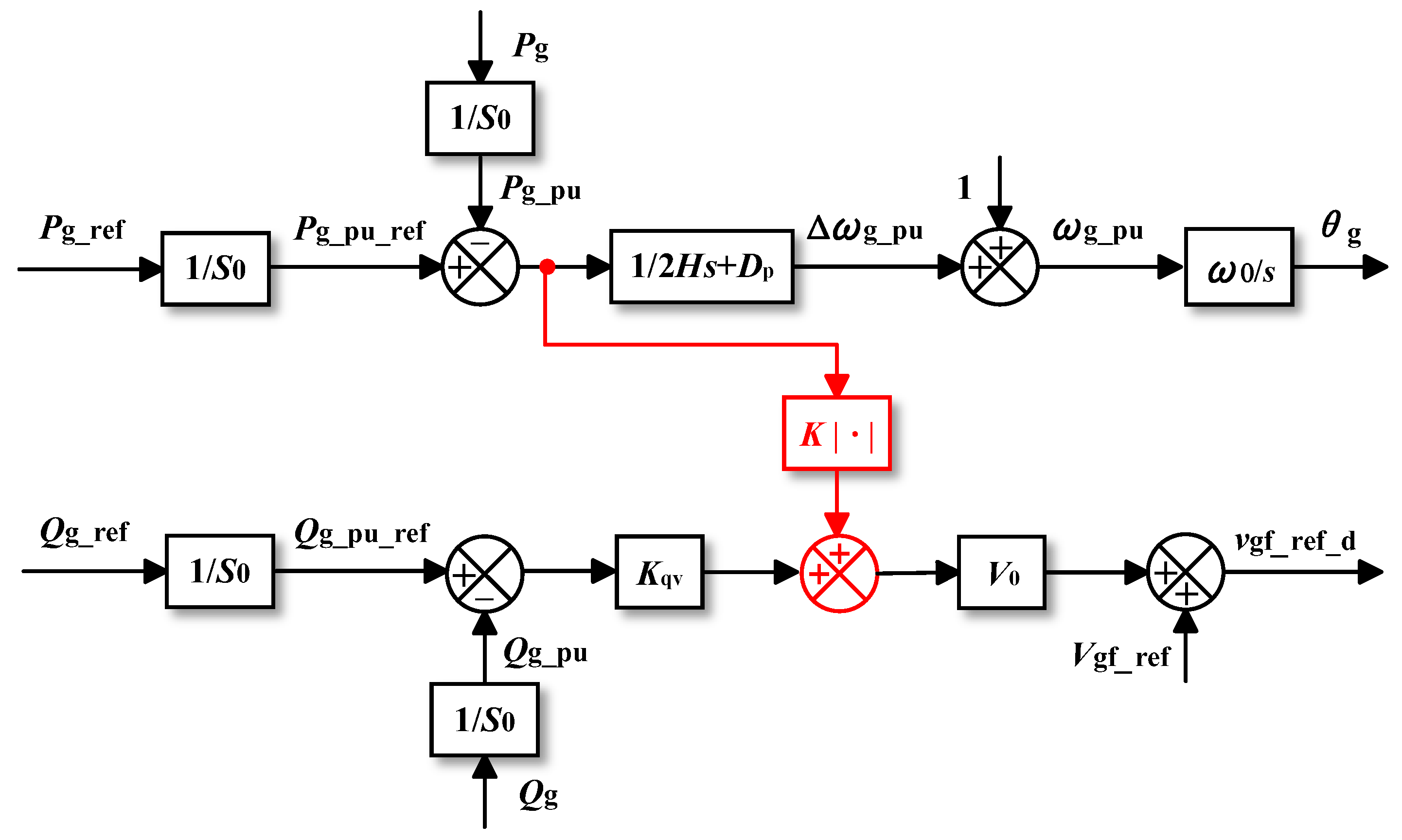



| Method | Reference | Year | Description |
|---|---|---|---|
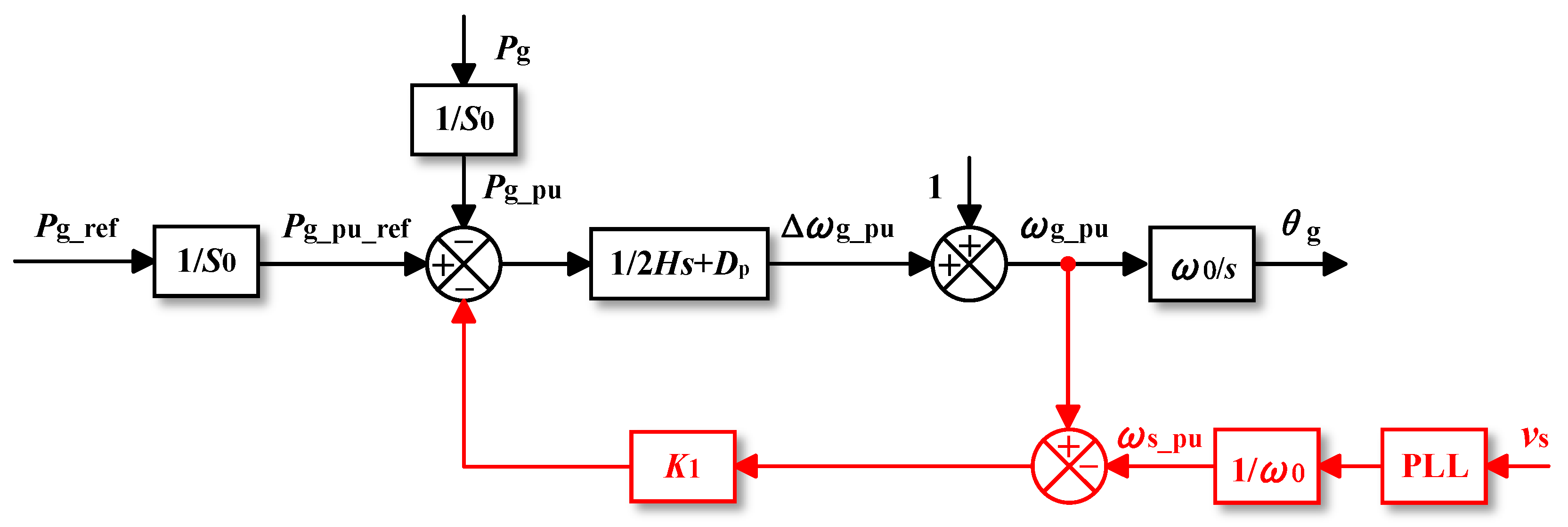
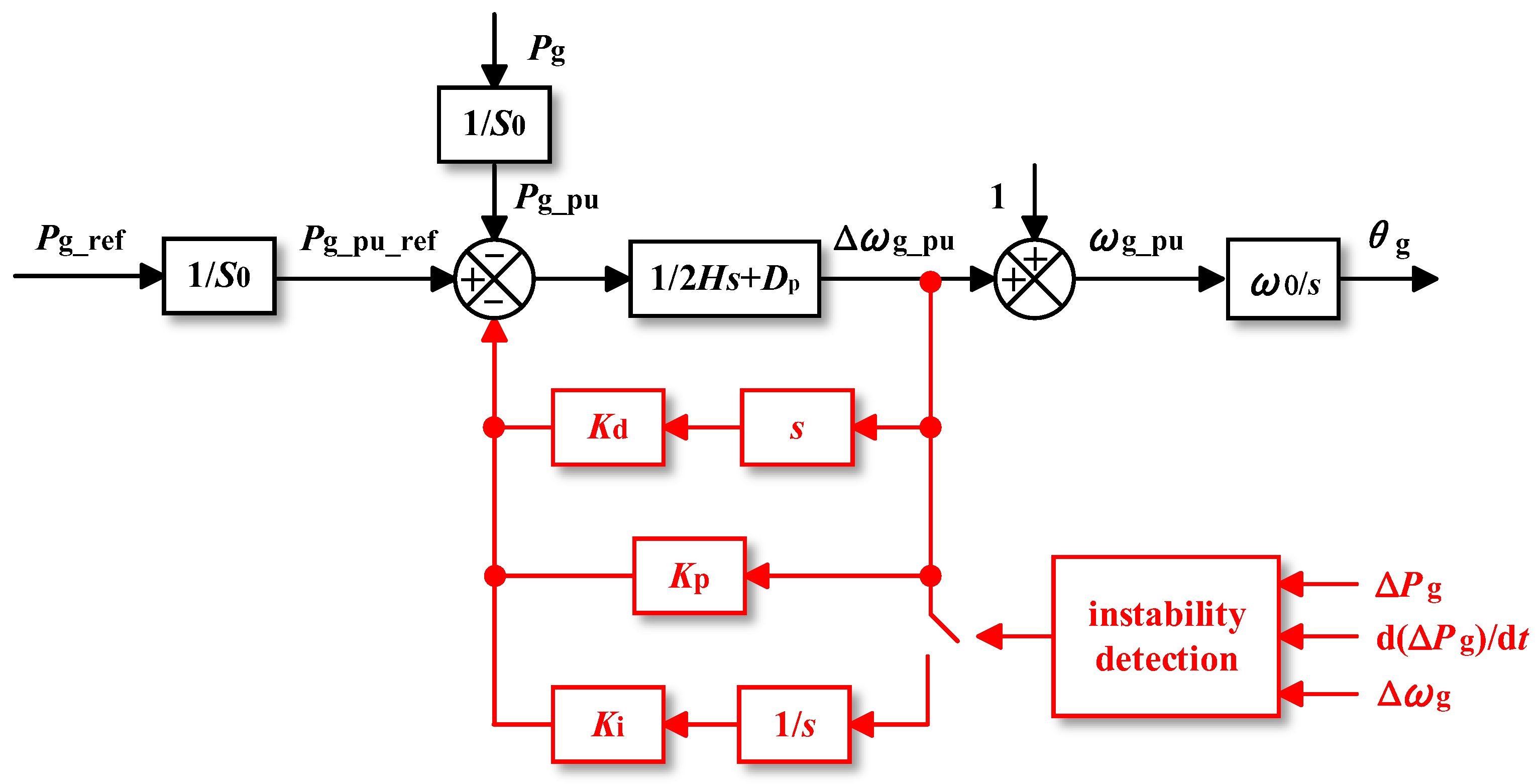
| Active power regulation | [21] | 2019 | Additional active power control (AAPC), decreases the active power reference |
| [23] | 2020 | Mode-adaptive power-angle control, changes the feedback mode of the active power control loop | |
| [20] | 2021 | Additional active power control (AAPC), decreases the active power reference | |
| [25] | 2021 | Power angle estimation, makes the power angle oscillate around π/2 | |
| [22] | 2022 | Additional active power control (AAPC), decreases the active power reference | |
| [24] | 2024 | Power angle estimation, makes the power angle oscillate around π/2 | |
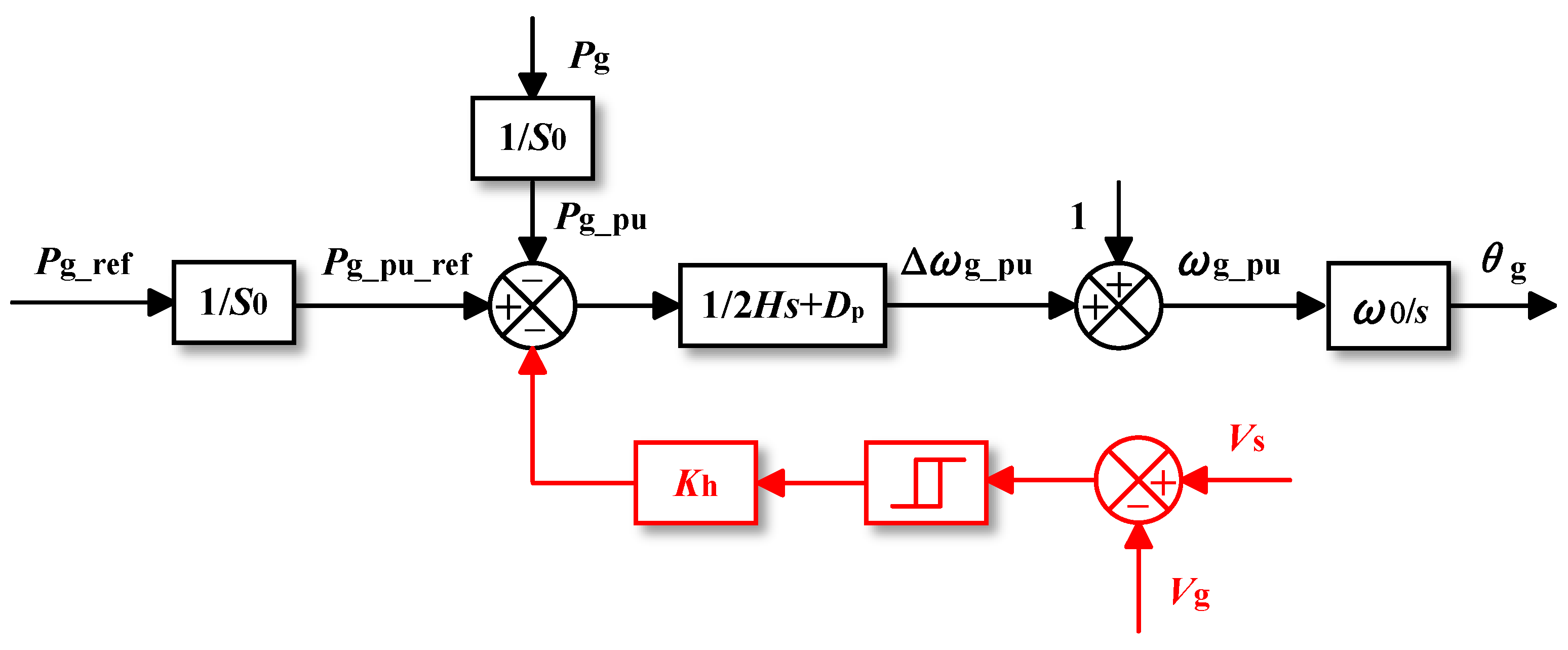
| Voltage regulation | [28] | 2022 | Fast voltage booster, increase the voltage after detecting faults |
| [10] | 2023 | Voltage amplitude regulation, calculates the voltage increase needed | |
| [29] | 2024 | Fast voltage booster, increases the voltage after detecting faults | |
| Reactive power regulation | [26] | 2021 | Frequency feedforward on reactive power control loop |
| [27] | 2022 | Active power feedforward on reactive power control loop, changes the power angle curve, increases the acceleration area and decreases the deceleration area | |
| [10] | 2023 | Reactive power injection, calculates the reactive power increase needed | |
| Virtual Impedance Regulation | [31] | 2022 | Increases the d-axis negative reactance and q-axis positive reactance simultaneously |
| [32] | 2023 | Increases the grid resistance | |
| [37] | 2023 | Changes the impedance ratio | |
| [11] | 2024 | Changes the impedance ratio | |
| [33] | 2024 | Changes the impedance ratio | |
| [35] | 2024 | Increases the grid inductance | |
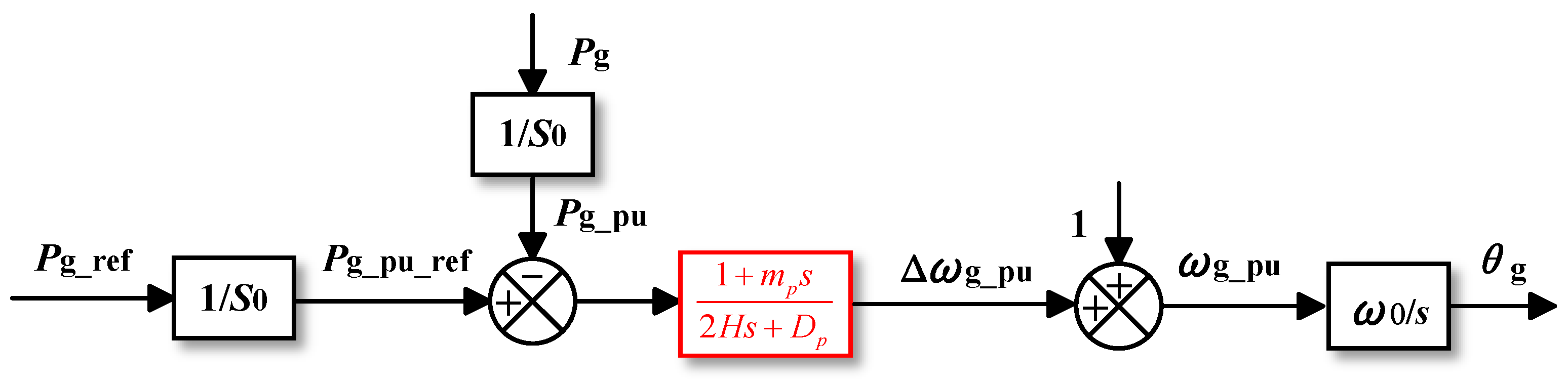
| Inertia regulation | [44] | 2015 | Sets different inertias for different periods |
| [42] | 2016 | Adaptive inertia | |
| [40] | 2023 | Sets different inertias for different periods | |
| Damping regulation | [38] | 2021 | Transient damping method (TDM), uses angular frequency feedback as transient damping |
| [45] | 2021 | Transient damping method (TDM), uses angular frequency feedback as transient damping | |
| [41] | 2022 | Dynamic damping control (DDC), introduces the detection of voltage sag | |
| [39] | 2023 | Differential compensation links | |
| [46] | 2024 | Transient damping method (TDM), uses angular frequency feedback as transient damping |
| Methods | Effects | Disadvantages |
|---|---|---|
| Active power regulation | Reducing the active power reference to restore EPs | Deteriorating frequency stability |
| Voltage regulation | Raising the power angle curve to restore EPs | Increasing current and leading to overload |
| Reactive power regulation | Raising the power angle curve to restore EPs | Increasing current and leading to overload |
| Virtual impedance regulation | Raising the power angle curve to restore EPs | Deteriorating voltage adjustment |
| Inertia regulation | Slowing down the dynamic process | Diminishing the effect of damping |
| Damping regulation | Slowing down the dynamic process and reducing overshoot | Affecting the power distribution results |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Chen, X.; Si, W.; Yu, Q.; Fang, J. Transient Stability Analysis and Enhancement of Grid-Forming Converters: A Comprehensive Review. Electronics 2025, 14, 645. https://doi.org/10.3390/electronics14040645
Chen X, Si W, Yu Q, Fang J. Transient Stability Analysis and Enhancement of Grid-Forming Converters: A Comprehensive Review. Electronics. 2025; 14(4):645. https://doi.org/10.3390/electronics14040645
Chicago/Turabian StyleChen, Xingyou, Wenjia Si, Qun Yu, and Jingyang Fang. 2025. "Transient Stability Analysis and Enhancement of Grid-Forming Converters: A Comprehensive Review" Electronics 14, no. 4: 645. https://doi.org/10.3390/electronics14040645
APA StyleChen, X., Si, W., Yu, Q., & Fang, J. (2025). Transient Stability Analysis and Enhancement of Grid-Forming Converters: A Comprehensive Review. Electronics, 14(4), 645. https://doi.org/10.3390/electronics14040645











