Application of Voltage Optimization Strategy for Rotary Power Flow Controllers in Loop Closing of Distribution Networks
Abstract
1. Introduction
- A two-stage optimal path-based RPFC loop-closing control strategy is proposed, focusing on adjusting voltage phase and magnitude at the loop-closing point to prevent the voltage fluctuations commonly seen in traditional methods.
- A phase angle difference calculation and rotation angle coordination control method are proposed, dynamically adjusting the RPFC rotation angle setpoint, significantly improving the stability and response speed of the loop-closing operation.
- Comparative simulations confirm the practicality and reliability of the proposed method, demonstrating the considerable potential of RPFC in enabling flexible loop-closing operations within distribution networks.
2. Basic Principle of RPFC
3. RPFC Loop-Closing Control Strategy
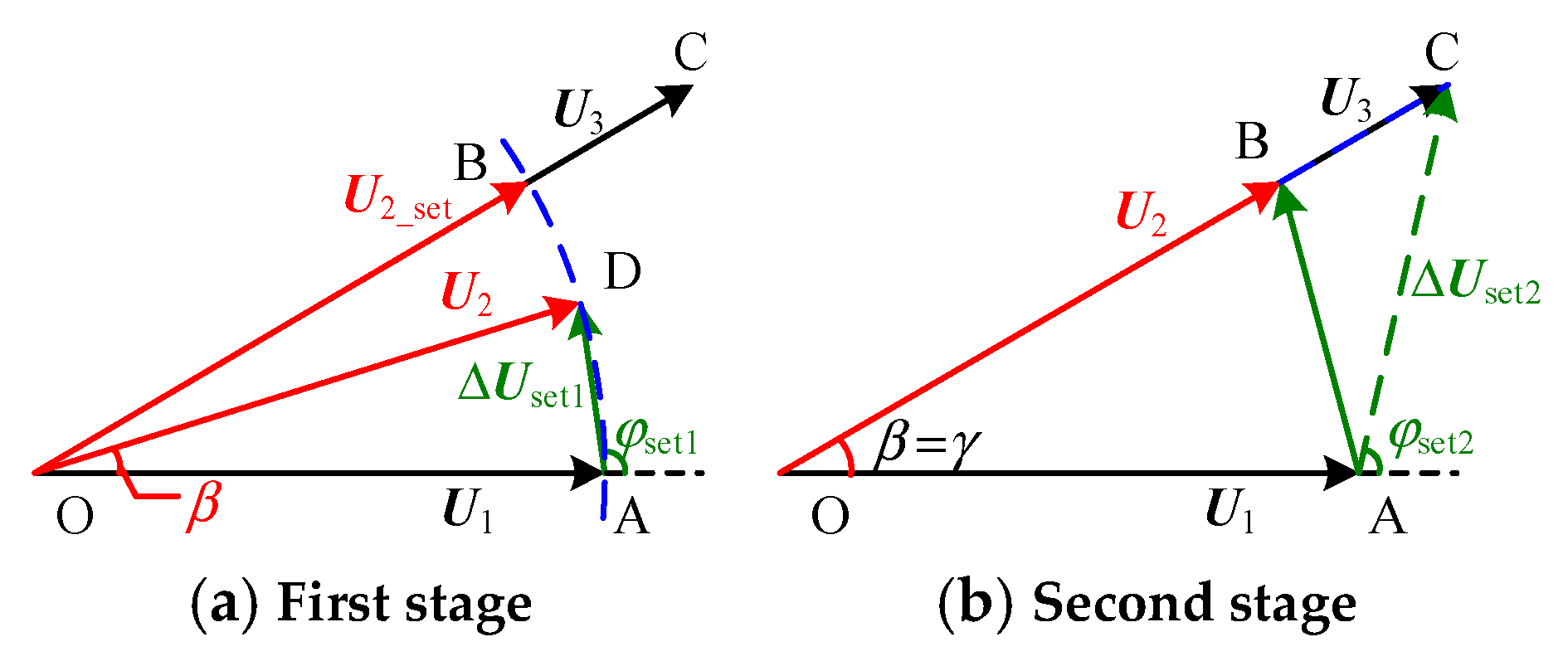
3.1. Two-Stage Operation Path for Output Voltage
3.2. Rotation Angle Coordination Control Method
- First-stage path planning. The RPFC aims to align the voltage phases at the loop-closing point, ensuring equal voltage magnitudes throughout the process.
- Second-stage path planning: The RPFC focuses on equalizing voltage magnitudes at the loop-closing point, maintaining consistent phases during the operation.
- Phase angle difference calculation: Determine the set angle δset between the stator and output voltages using Equation (1).
- Rotation angle coordination control: Compare the initial positions of the two stator voltages with their setpoints, and select the closest stator voltage setpoint as the target value.
4. RPFC Flexible Loop-Closing Simulation
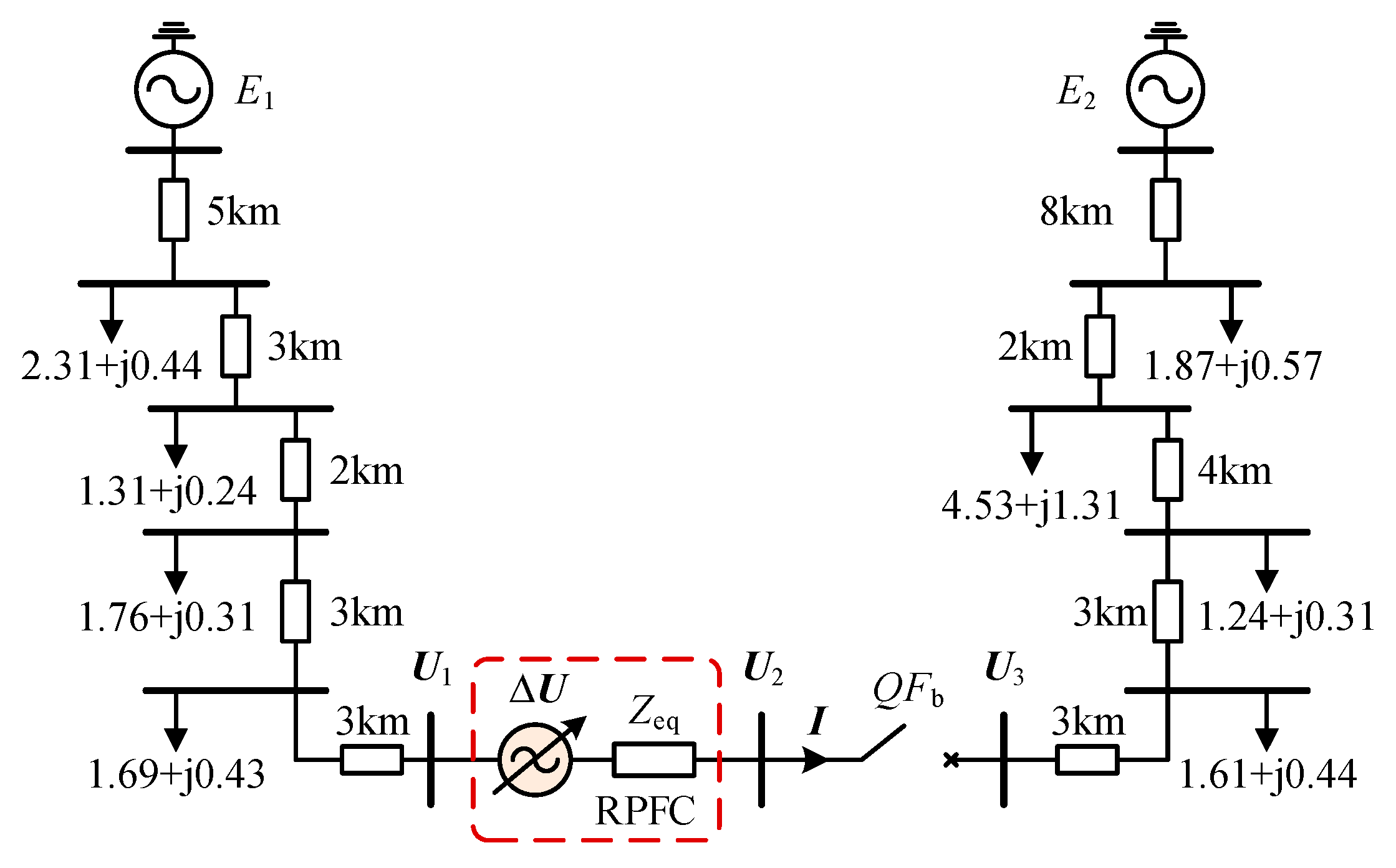
4.1. Loop-Closing Simulation Scenario
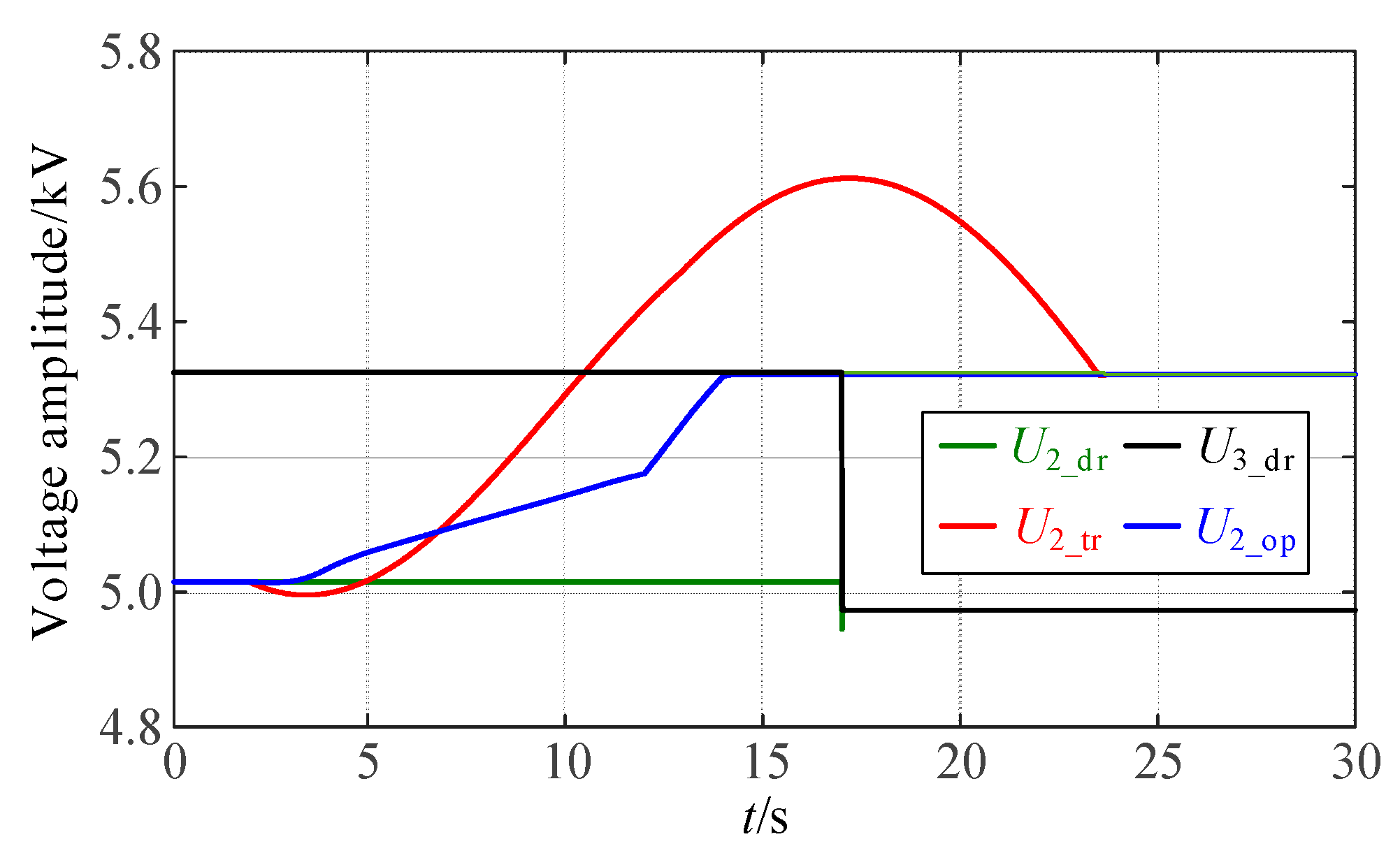
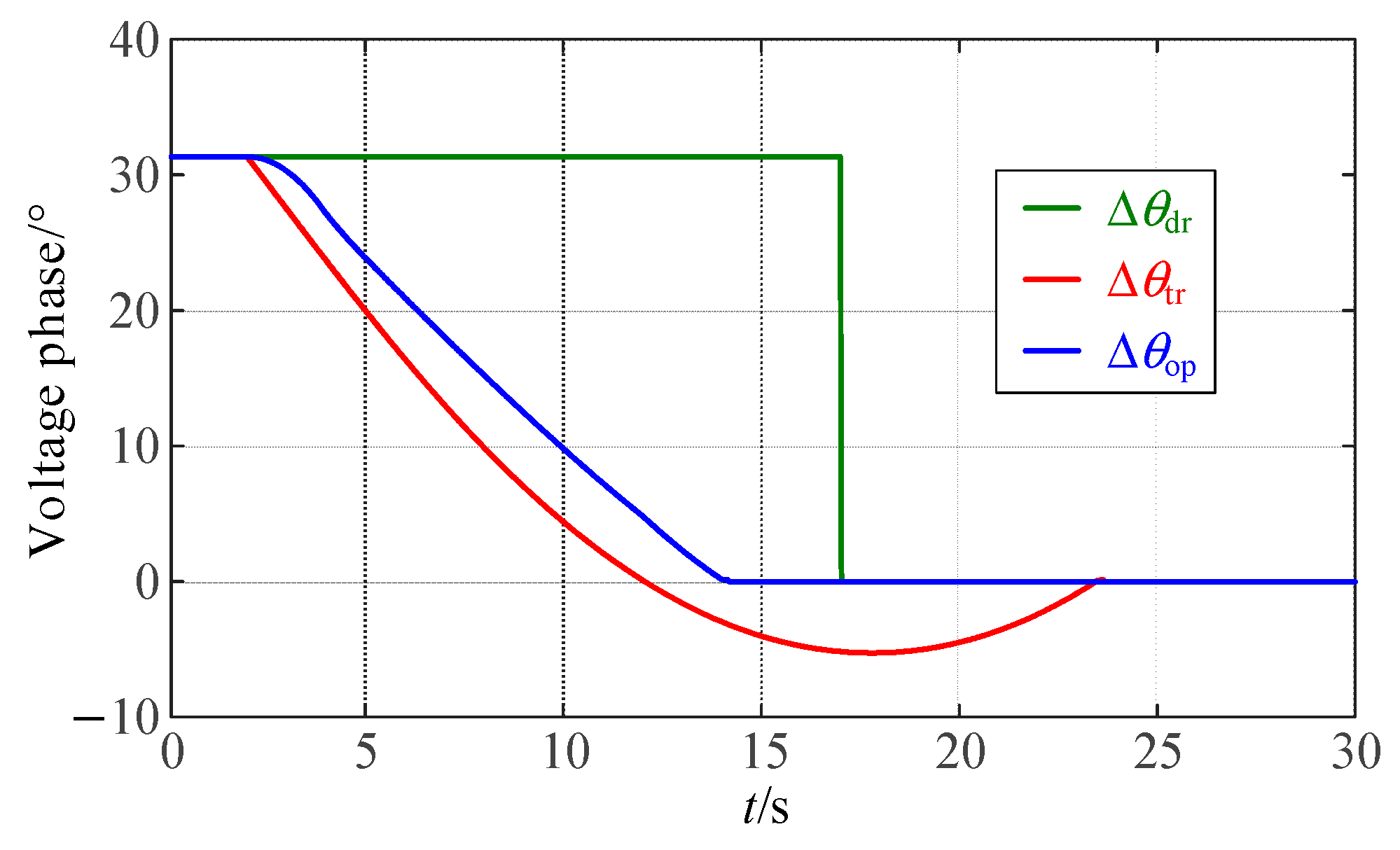
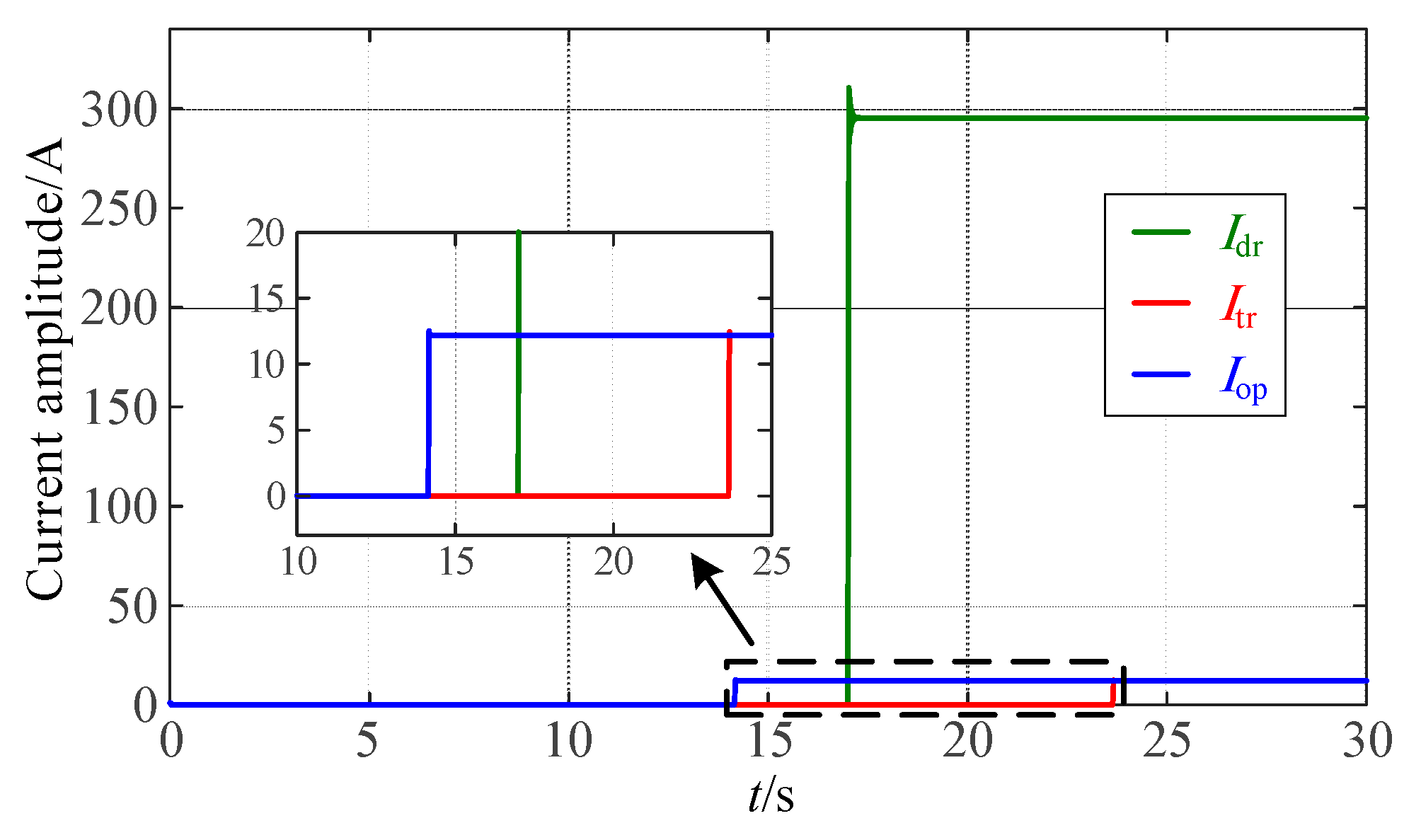
4.2. Loop-Closing Performance Analysis
5. Conclusions
- The proposed strategy effectively minimizes voltage fluctuations and current surges during loop-closing by optimizing the transitions of voltage magnitude and phase. This approach helps to prevent voltage limit violations, ensuring stable operation during the loop-closing process and avoiding system instability caused by voltage fluctuations.
- As an independent device, the RPFC can perform loop-closing control without relying on other system equipment (such as auxiliary voltage sources or distributed energy sources) for coordination. This characteristic makes RPFC more flexible and convenient for practical applications, reducing dependency on other devices and enhancing system operational efficiency and stability.
- Compared to traditional methods, the proposed control strategy significantly shortens the loop-closing time. Through rotational angle coordination control, the adjustment path of RPFC output voltage is optimized, effectively improving the response speed of loop-closing operations and ensuring quick restoration of the power grid.
- As an electromagnetic device, the RPFC offers excellent tolerance and low maintenance costs, but its operational efficiency may be slightly slower compared to power electronic devices. This limitation makes RPFC more suitable for long-term stable operation in scenarios where extremely fast response times are not critical, though it remains a reliable solution for many applications requiring flexible loop-closing control.
- Future research could focus on strengthening the application of RPFC after loop-closing, especially in its role in power flow distribution and scheduling within the entire power system. Further research could also explore how to integrate RPFC with energy storage systems, distributed energy sources, and other power system technologies to optimize configuration and collaborative scheduling, thereby enhancing the flexibility and stability of the grid.
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
Nomenclature
| Terms | optimal value |
| RPFC | Rotary power flow controller |
| B1-B5 | Breaker1-breaker5 |
| U1 | Delivery voltage |
| U2 | Left-side loop voltage |
| U3 | Right-side loop voltage |
| ΔU | RPFC output voltage |
| Ust1, Ust2 | Stator voltage of RPFC |
| Ust10, Ust20 | Initial stator voltage |
| Usta_set, Ustb_set | Setpoints of the stator voltage |
| α1, α2 | Rotation angles of RPST |
| QFb | Loop closing switch |
| Zeq | Equivalent impedance of RPFC |
| φ | Output voltage phase of RPFC |
| δ | Angle between output voltage and stator voltage |
| β | Phase at the left end of the loop closing point |
| γ | Phase at the right end of the loop closing point |
| ΔUset | Output voltage magnitude setpoint |
| φset | Output voltage phase setpoint |
| βset | Phase setpoint at the left end of the point |
| δset | Angle setpoint of d |
| αa_set, αb_set | Rotation angle setpoint |
| t1, t2 | Time of the first and second stages |
| kr | Variation rate of bset |
| θ1a | Angle with Ust10 and Usta_set |
| θ2b | Angle with Ust20 and Ustb_set |
| α1_set | Rotation angle a1 setpoint |
| α2_set | Rotation angle a2 setpoint |
| E1, E2 | Line electromotive force |
| ZL1, ZL2 | Line impedance |
| I | Loop-closing current |
| Dq | Phase difference at both ends of the loop-closing point |
| dr | Direct loop-closing method |
| tr | Method from Reference [22] |
| op | Proposed method from this paper |
References
- Ruan, H.; Gao, H.; Liu, Y.; Wang, L.; Liu, J. Distributed voltage control in active distribution network considering renewable energy: A novel network partitioning method. IEEE Trans. Power Syst. 2020, 35, 4220–4231. [Google Scholar] [CrossRef]
- Li, P.; Wu, Q.; Yang, M.; Li, Z.; Hatziargyriou, N.D. Distributed distributionally robust dispatch for integrated transmission-distribution systems. IEEE Trans. Power Syst. 2021, 36, 1193–1205. [Google Scholar] [CrossRef]
- Hayes, B.P.; Gruber, J.K.; Prodanovic, M. A closed-loop state estimation tool for MV network monitoring and operation. IEEE Trans. Smart Grid 2015, 6, 2116–2125. [Google Scholar] [CrossRef]
- Vardani, B.; Tummuru, N.R. A single-stage bidirectional inductive power transfer system with closed-loop current control strategy. IEEE Trans. Transp. Electrific. 2020, 6, 948–957. [Google Scholar] [CrossRef]
- Wang, Z.; Xie, J.; Wang, Y. Analytical of loop closing operation based on multi-dimensional index system. In Proceedings of the 2020 International Symposium on Computer Engineering and Intelligent Communications (ISCEIC), Guangzhou, China, 7–9 August 2020; pp. 76–81. [Google Scholar]
- Wang, H.; Yu, X.; Zhang, Z.; Yao, Y. Calculation, measurement and engineering application of loop closing current in power grid. In Proceedings of the 2021 IEEE 5th Conference on Energy Internet and Energy System Integration, Taiyuan, China, 22–25 October 2021; pp. 2237–2241. [Google Scholar]
- Wang, H.; Zhang, Z.; Dong, J.; Zhang, Y.; Wu, H.; Zhang, W. Application of incentive-type variable weight in decision of 500/220kV received electromagnetic looped grid decomposing operation. IEEE Access 2020, 8, 185169–185176. [Google Scholar] [CrossRef]
- Vargas, R.; Macedo, L.H.; Home-Ortiz, J.M.; Romero, R. Optimal restoration of distribution systems considering temporary closed-loop operation. IEEE Syst. J. 2021, 15, 5483–5494. [Google Scholar] [CrossRef]
- de Groot, R.J.W.; Morren, J.; Slootweg, J.G. Reliable and efficient operation of closed-ring distribution grids supported by distribution automation. Sustain. Energy Grids Netw. 2018, 15, 53–62. [Google Scholar] [CrossRef]
- Hafez, A.A.; Omran, W.A.; Hegazy, Y.G. A decentralized technique for autonomous service restoration in active radial distribution networks. IEEE Trans. Smart Grid 2018, 9, 1911–1919. [Google Scholar] [CrossRef]
- Marques, L.T.; Delbem, A.C.B.; London, J.B.A. Service restoration with prioritization of customers and switches and determination of switching sequence. IEEE Trans. Smart Grid 2018, 9, 2359–2370. [Google Scholar] [CrossRef]
- Singh, P.; Tiwari, R.; Sangwan, V.; Gupta, A.K. Optimal allocation of thyristor-controlled series capacitor (TCSC) and thyristor-controlled phase-shifting transformer (TCPST). In Proceedings of the 2020 International Conference on Power Electronics & IoT Applications in Renewable Energy and Its Control, Mathura, Uttar Pradesh, India, 28–29 February 2020; pp. 491–496. [Google Scholar]
- Xu, Z.; Qin, R.; Ma, H.; Lu, J.; Tang, J.; Yang, Y. Research on the optimal regulation strategy of loop closing device based on phase shifting transformer. In Proceedings of the 2021 IEEE 5th Conference on Energy Internet and Energy System Integration, Taiyuan, China, 22–25 October 2021; pp. 1203–1208. [Google Scholar]
- Xu, Z.; Qin, R.; Ma, H.; Lu, J.; Niu, C.; Yang, Y. Comparative study of AC/DC side loop-closing strategy based on back-to-back VSC-HVDC flexible loop-closing device in distribution network. In Proceedings of the 2021 5th International Conference on Power and Energy Engineering (ICPEE), Wuhan, China, 2–4 December 2021; pp. 53–60. [Google Scholar]
- Zhenping, Z.; Chaoqun, N.; Yuanyuan, M.; Jianxiong, T.; Yongchun, Y. Research on SVG participating in voltage regulation and non-power outage closing loop transfer control strategy of distribution network. In Proceedings of the 2022 6th International Conference on Power and Energy Engineering (ICPEE), Shenzhen, China, 25–27 November 2022; pp. 72–78. [Google Scholar]
- Haddadi, A.; Kazemi, A. Optimal power flow control by rotary power flow controller. Adv. Electr. Comput. Eng. 2011, 11, 79–86. [Google Scholar] [CrossRef]
- Larsen, E.V. A classical approach to constructing a power flow controller. In Proceedings of the 1999 IEEE Power Engineering Society Summer Meeting, Edmonton, AB, Canada, 18–22 July 1999; Volume 2, pp. 1192–1195. [Google Scholar]
- Tan, Z.; Zhang, C.; Jiang, Q. Research on characteristics and power flow control strategy of rotary power flow controller. In Proceedings of the International Youth Conference on Energy (IYCE), Pisa, Italy, 27–30 May 2015; pp. 1–8. [Google Scholar]
- Ba, A.O.; Peng, T.; Lefebvre, S. Rotary power flow controller for dynamic performance evaluation—Part I: RPFC modeling. IEEE Trans. Power Deliv. 2009, 24, 1406–1416. [Google Scholar] [CrossRef]
- Ba, A.O.; Peng, T.; Lefebvre, S. Rotary power-flow controller for dynamic performance evaluation—Part II: RPFC application in a transmission corridor. IEEE Trans. Power Deliv. 2009, 24, 1417–1425. [Google Scholar] [CrossRef]
- Jia, J.; Zhou, J.; Gao, Y.; Shao, C.; Lu, J.; Jia, J. A rotating tidal current controller and energy router siting and capacitation method considering spatio-temporal distribution. Energies 2024, 17, 5919. [Google Scholar] [CrossRef]
- Yan, X.; Shao, C.; Jia, J.; Aslam, W.; Peng, W.; Yang, R. An Accurate Power Control Strategy for Electromagnetic Rotary Power Controllers. IET Gener. Transm. Distrib. 2023, 17, 3524–3540. [Google Scholar] [CrossRef]
- Yan, X.; Deng, W.; Wang, G.; Rasool, A.; Qi, S. Research on novel var compensator based on dual-rotary phase-shifting transformers and its control strategy. Int. J. Electr. Power Energy Syst. 2023, 144, 108632–108642. [Google Scholar] [CrossRef]
- Yan, X.; Wu, W.; Shao, C.; Peng, W. Comprehensive compensation method for co-phase power supply in electrified railways based on V/v transformer and electromagnetic single-phase var compensator. IET Gener. Transm. Distrib. 2024, 18, 3170–3186. [Google Scholar] [CrossRef]
- Shao, C.; Yan, X.; Yang, Y.; Aslam, W.; Jia, J.; Li, J. Multiple-zone synchronous voltage regulation and loss reduction optimization of distribution networks based on a dual rotary phase-shifting transformer. Sustainability 2024, 16, 1029. [Google Scholar] [CrossRef]
- Yan, X.; Peng, W.; Wang, Y.; Aslam, W.; Shao, C.; Li, T. Flexible loop closing control method for an active distribution network based on dual rotary phase shifting transformers. IET Gener. Transm. Distrib. 2022, 16, 4204–4214. [Google Scholar] [CrossRef]
- Shao, C.; Yan, X.; Siddique, A.; Aslam, W.; Jia, J.; Xie, W.; Alqahtani, S.A.; Alamri, A.M. Two-stage power control method of rotary power flow controller for flexible interconnection of distribution network. IET Renew. Power Gener. 2024, early view, 1–14. [Google Scholar] [CrossRef]
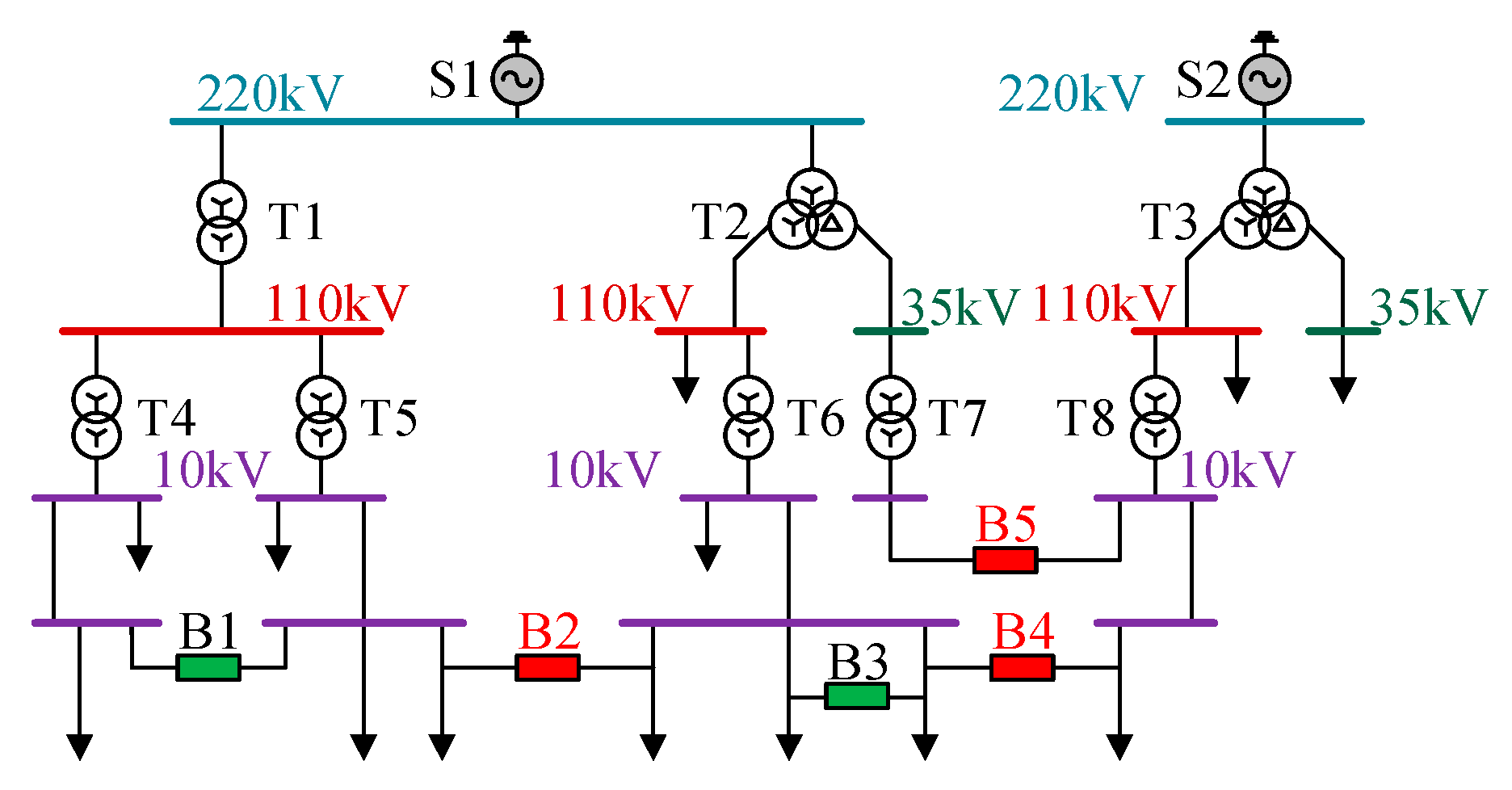





| Capacity /MVA | Voltage Level/kV | Turn Ratio | Rated Speed °/s | ||
|---|---|---|---|---|---|
| Primary | Secondary | ||||
| RPST1 | 2.0 | 10 | 2.9 | 3.44 | 6 |
| RPST2 | 2.0 | 10 | 2.9 | 3.44 | 6 |
| RPFC | 4.0 | 10 | 5.8 | 1.72 | 6 |
| Parameters | Direct Loop-Closing | Method in Reference [26] | Proposed Method |
|---|---|---|---|
| U2/kV | 5.01 | 5.31 | 5.32 |
| U3/kV | 5.32 | 5.32 | 5.32 |
| Dq/° | 31.35 | 0.11 | 0.10 |
| I/A | 295.36 | 12.20 | 12.18 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Xie, W.; Yuan, Y.; Zheng, X.; Chen, H.; Liu, J.; Zhang, C. Application of Voltage Optimization Strategy for Rotary Power Flow Controllers in Loop Closing of Distribution Networks. Electronics 2025, 14, 630. https://doi.org/10.3390/electronics14030630
Xie W, Yuan Y, Zheng X, Chen H, Liu J, Zhang C. Application of Voltage Optimization Strategy for Rotary Power Flow Controllers in Loop Closing of Distribution Networks. Electronics. 2025; 14(3):630. https://doi.org/10.3390/electronics14030630
Chicago/Turabian StyleXie, Wenqiang, Yubo Yuan, Xian Zheng, Hui Chen, Jian Liu, and Chenyu Zhang. 2025. "Application of Voltage Optimization Strategy for Rotary Power Flow Controllers in Loop Closing of Distribution Networks" Electronics 14, no. 3: 630. https://doi.org/10.3390/electronics14030630
APA StyleXie, W., Yuan, Y., Zheng, X., Chen, H., Liu, J., & Zhang, C. (2025). Application of Voltage Optimization Strategy for Rotary Power Flow Controllers in Loop Closing of Distribution Networks. Electronics, 14(3), 630. https://doi.org/10.3390/electronics14030630





