Load Rejection Overvoltage Suppression and Parameter Design Method of UHV AC Transmission Line
Abstract
1. Introduction
2. Mechanism of Load-Shedding Overvoltage
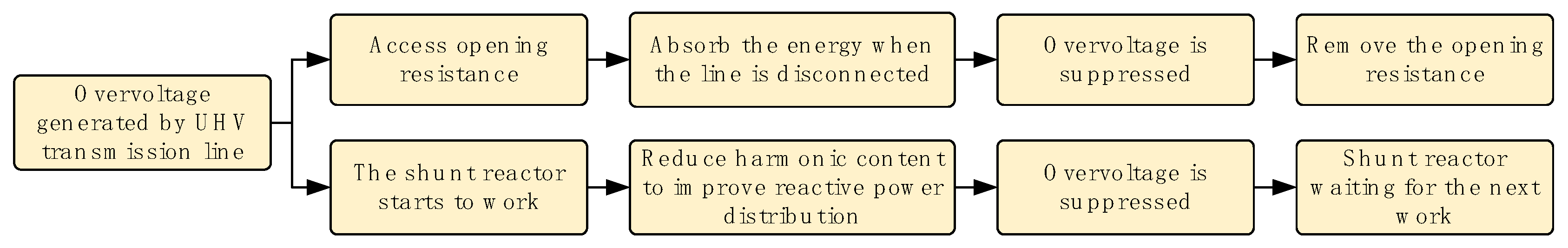
3. Load Rejection Overvoltage Suppression Method
3.1. Analysis of the Influence of Opening Resistance on Overvoltage
3.2. Analysis of the Influence of Reactor Compensation Degree on Overvoltage
4. Simulation Analysis
4.1. Establishment of Simulation Model
4.2. Simulation Results Under Different Influencing Factors
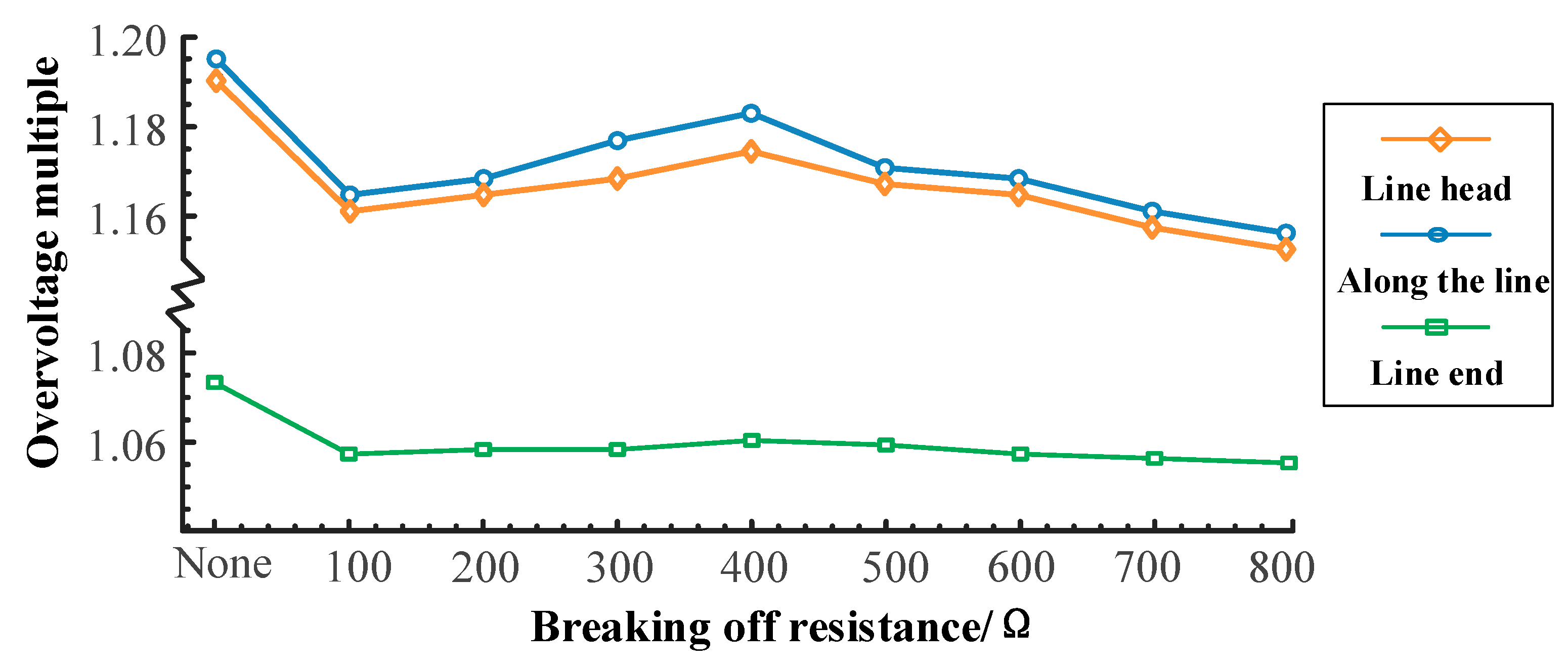
4.2.1. Subsubsection Simulation of the Influence of Opening Resistance on Overvoltage
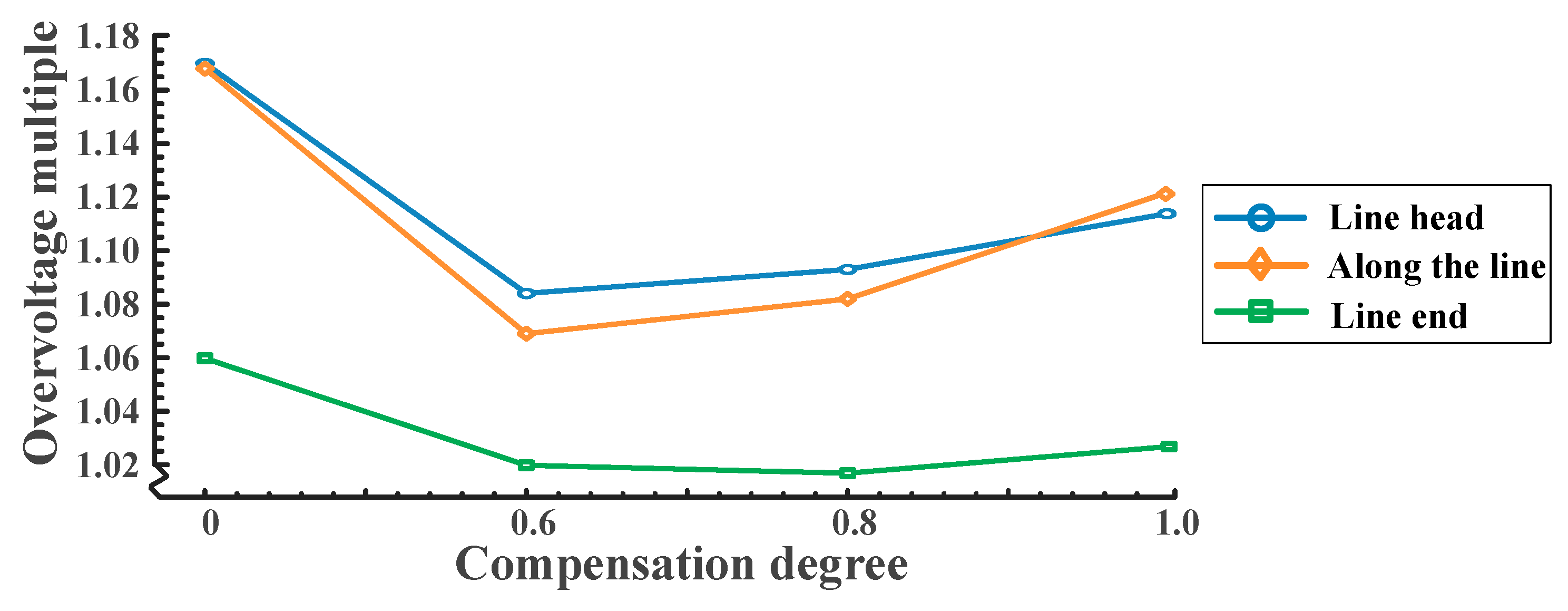
4.2.2. The Influence of Reactor Compensation Degree on Overvoltage
4.2.3. Combination of Two Suppression Methods
5. Conclusions
- (1)
- UHV AC transmission system is accompanied by huge reactive power. When the load drops sharply, it is easy to produce serious power frequency overvoltage. Setting appropriate opening resistance value and reactor compensation degree can suppress overvoltage. The two methods can be used at the same time to obtain a better suppression effect.
- (2)
- The setting value of the opening resistance of the circuit breaker has a significant effect on the overvoltage suppression level. When the opening resistance is 100 Ω, the load rejection overvoltage level is at the minimum value. When the opening resistance is greater than 400 Ω, the load rejection overvoltage level decreases slowly with the increase in resistance. In the project, the use of 100 Ω or 600 Ω~800 Ω opening resistor can effectively reduce the overvoltage phenomenon.
- (3)
- The compensation degree of the reactor has a significant effect on the overvoltage suppression level. When the compensation degree is in the range of 0.6~0.8, the line load rejection overvoltage suppression effect is the best.
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Sun, C.W.; Min, J.L. Dynamic trends and regional differences of economic effects of ultra-high-voltage transmission projects. Energy Econ. 2024, 138, 107871. [Google Scholar] [CrossRef]
- Fan, X.; Zhang, Y.H.; Su, L.N.; Liu, H.Y.; Cui, R.; Wei, Y.Z. Research on Short Circuit Current Control in Multi-infeed DC Receiving Power Grid. AC/DC Oper. 2018, 46, 61–67. [Google Scholar]
- Gan, F.; Wu, J.; Wang, K.; Zhao, Y.; Zhu, X.D. Research on the social benefit evaluation method of UHV power grid. Power Syst. Prot. Control 2016, 44, 12–17. [Google Scholar]
- Sun, K.; Yi, Q.; Dong, C.W.; Zhou, H. Research on Power Frequency Over-Voltage in UHV AC Transmission Lines. Power Syst. Technol. 2010, 34, 30–35. [Google Scholar]
- Xie, D.; Lin, C.X.; Deng, Q.L.; Lin, H.J.; Cai, C.S.; Thomas, B.; Ge, X.L. Simple Vector Calculation and Constraint-Based Fault-Tolerant Control for a Single-Phase CHBMC. IEEE Trans. Power Electron. 2025, 40, 2028–2041. [Google Scholar] [CrossRef]
- Huang, J.; Wang, G.; Li, H.F.; Han, F.X. Study on simulation of fundamental frequency overvoltage for UHV AC transmission lines. Relay 2007, 35, 32–39. [Google Scholar]
- Chen, S.M.; Xu, W.; He, J.L. Research on Power Frequency Transient Overvoltage in 1000kV UHV AC Transmission Line. Power Syst. Technol. 2005, 29, 1–5. [Google Scholar]
- Chai, X.Z.; Zeng, R.; Liang, X.D.; Sun, J.H.; Hao, J.J. Design and Planning Method for Series-compensated Power Transmission System Considering Power-frequency Overvoltage and Transient Stability. High Volt. Eng. 2015, 41, 3407–3413. [Google Scholar]
- Liu, Q.H.; Yu, J.W.; Chen, H.; Qin, Y. Studies on series compensation of UHV transmission line. In Proceedings of the 2010 International Conference on Power System Technology, Hangzhou, China, 24–28 October 2010. [Google Scholar]
- Amreiz, H.; Janbey, A.; Darwish, M. Emulation of Series and Shunt Reactor Compensation. In Proceedings of the 2020 55th International Universities Power Engineering Conference (UPEC), Turin, Italy, 1–4 September 2020. [Google Scholar]
- Su, X.L. Analysis of power frequency overvoltage of UHV AC transmission line and its suppression. Electron. Test 2016, 23, 140. [Google Scholar]
- Wang, X.T.; Xiang, Z.T.; Ban, L.G.; Zhang, Y.Y.; Han, B. Secondary Arc Current in 1000 kV Double-Circuit Long-Distance AC Transmission Lines on the Same Tower Under Heavy Power Flow and Its Restrictive Measures. Power Syst. Technol. 2013, 37, 3001–3006. [Google Scholar]
- Zhang, W. Analysis of Tripping Position Anomaly of Ultra-high Voltage Line Overvoltage Protection Circuit Breaker. Electr. Eng. 2024, 9, 168–169+173. [Google Scholar]
- Du, B.X.; Zhang, Y.P.; Tian, M.X.; Dong, H.Y.; Zhao, Y.K. Research on Compensation Degree of UHV Controllable Shunt Reactor. High Volt. Appar. 2010, 46, 5–8+11. [Google Scholar]
- Rui, Z.K. Study on the Mechanism of Generating Frequency Overvoltage of UHV Transmission Line and Its Suppression Measures. Master’s Thesis, Shandong University of Science and Technology, Qingdao, China, 2017. Volume 6. [Google Scholar]
- Chen, L.; Liu, H.J.; Wu, M.; Wei, D.L.; Niu, M. Study on Compensation for Secondary Arc Current on UHV Double-Circuit Lines. In Proceedings of the 2011 Asia-Pacific Power and Energy Engineering Conference, Wuhan, China, 25–28 March 2011. [Google Scholar]
- Xu, L.Y.; Zhang, Y.X.; Xue, S.M. Overvoltage superposition and protection caused by load shedding of UHV transmission lines. Electr. Power 2011, 44, 6–10. [Google Scholar]
- Yin, Z.F. Power Frequency Overvoltage Suppression of UHV AC Transmission Line. Shandong Electr. Power 2014, 41, 24–28. [Google Scholar]
- Meng, F.T.; He, B.N.; Liu, H.; Wu, S.; Liu, Y.; Dai, W.H.; Wang, S.; Dong, Y.C. TRV Suppression Measures of Controllable Hybrid Reactive Power Compensation Lines. South. Power Syst. Technol. 2024, 18, 1–11. [Google Scholar]
- Sun, S. Summary of Research on Overvoltage Characteristics and Suppression Technology of Power Cable Operation. J. New Ind. 2019, 9, 28–31. [Google Scholar]
- Li, Y.; Zhou, H.; Shen, Y.; Sun, K. Comparative Study on Measures to Limit Load-Rejection Opening Overvoltage of Long-Distance and Large-Capacity UHVAC Transmission Lines. Grid Technol. 2013, 37, 3564–3570. [Google Scholar]
- Kong, C.R.; Zhan, X.W.; Wen, H.P.; Zhou, H.; Li, W.J.; Peng, C.Z. Resonance Analysis and Counter measure Research of 10kV Shunt Capacitor. Mach. Electron. 2024, 42, 3–7. [Google Scholar]
- Zhao, Y.S. The Research on Over-Voltage and Restrain Strategy for UHV Transmission Line. Master’s Thesis, Southwest Jiaotong University, Chengdu, China, 2009. Volume 6. [Google Scholar]
- Zhang, B.H.; Xi, Z.Y.; Hu, W.H.; Cui, D.W.; Chen, C.W. Application and Significance of 500 kV Dry-Type Shunt Reactor Technologyin Brazil. Int. Stand. 2024, z1, 49–55. [Google Scholar] [CrossRef]
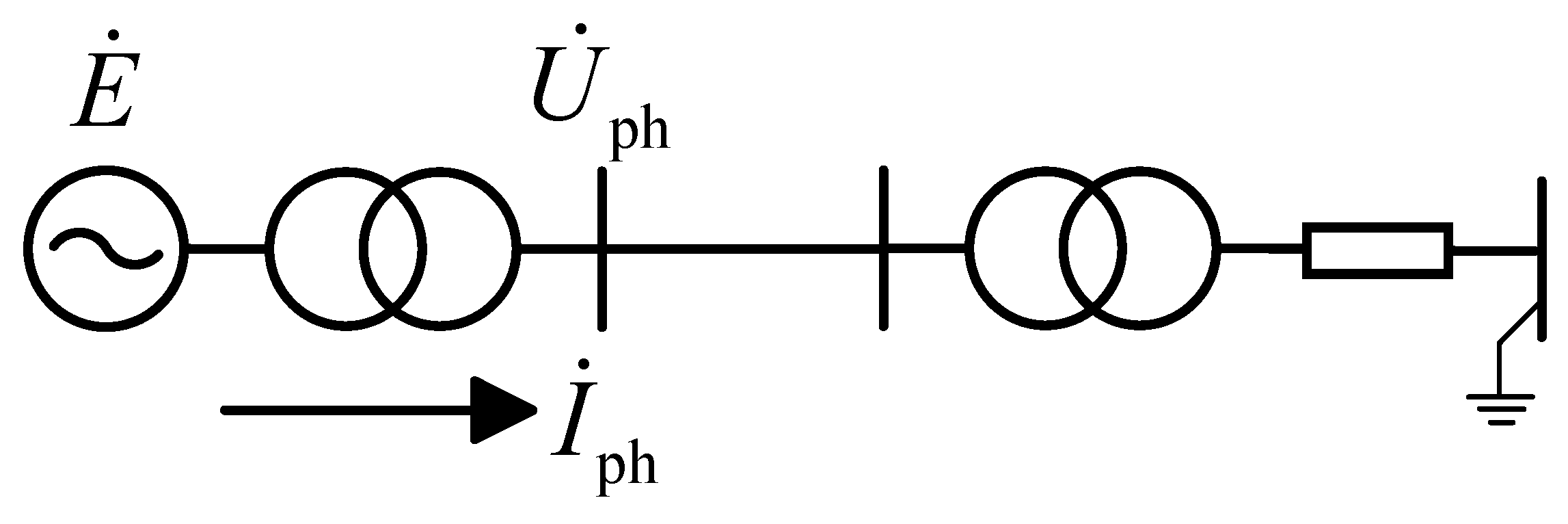




| Line Name | Length (km) | Per Unit Quantity (Un = 1050 kV, S = 100 MVA) | |||||
|---|---|---|---|---|---|---|---|
| R1 | X1 | B1 | R0 | X0 | B0 | ||
| XJ1 Line I | 340.84 | 0.000272 | 0.008053 | 17.117584 | 0.004662 | 0.022732 | 10.152498 |
| XJ1 Line II | 341.17 | 0.000272 | 0.008043 | 17.134358 | 0.004688 | 0.022714 | 10.162446 |
| Transformer Name | Capacity (MVA) | Per Unit Quantity (Ub = 1050 kV, Sb = 3000 MVA) | ||||
|---|---|---|---|---|---|---|
| X1-2 | X1-3 | X2-3 | PFe | PCu | ||
| XX1# | 3000 | 0.1799 | 0.6134 | 0.3995 | 0.000148 | 0.0012 |
| XX2# | 3000 | 0.1795 | 0.6207 | 0.3999 | 0.000148 | 0.0012 |
| GJ1# | 3000 | 0.1799 | 0.6192 | 0.4001 | 0.000165 | 0.0012 |
| GJ2# | 3000 | 0.1800 | 0.6207 | 0.3996 | 0.000165 | 0.0012 |
| Nodal Point | Resistance (Ω) | Reactance (H) |
|---|---|---|
| 500 kV DG | 0.594 | 0.045 |
| 500 kV LY | 0.316 | 0.026 |
| 500 kV XC | 0.386 | 0.027 |
| 500 kV LC | 0.658 | 0.041 |
| 500 kV JX | 0.778 | 0.038 |
| 500 kV YF | 0.903 | 0.053 |
| 1000 kV JM | 0.394 | 0.035 |
| Three-Phase Measuring Point | E1F | E2F | E3F | E4F | E5F | E6F | E7F | E8F |
|---|---|---|---|---|---|---|---|---|
| Single-phase Measuring point | E1A E1B E1C | E2A E2B E2C | E3A E3B E3C | E4A E4B E4C | E5A E5B E5C | E6A E6B E6C | E7A E7B E7C | E8A E8B E8C |
| Location | 0 | 63.917 | 152.002 | 170.585 | 202.058 | 258.691 | 296.613 | 341.005 |
| Type | Head | Commutation | Commutation | Midpoint | Split place | Merge place | Commutation | End |
| High Reactance L1 (H) | Low Reactance L2 (H) | Compensation Degree | Compensation Capacity (Mvar) |
|---|---|---|---|
| 0 | 0 | 0 | 0 |
| 3.994 | 1.2804 | 0.6 | 540 |
| 5.325 | 1.7713 | 0.8 | 720 |
| 6.656 | 2.1413 | 1.0 | 900 |
| Opening Resistance | None | 100 Ω | 200 Ω | 300 Ω | 400 Ω | 500 Ω | 600 Ω | 700 Ω | 800 Ω |
|---|---|---|---|---|---|---|---|---|---|
| Head | 1.193 | 1.168 | 1.171 | 1.178 | 1.183 | 1.173 | 1.171 | 1.165 | 1.161 |
| Along the line | 1.189 | 1.166 | 1.163 | 1.171 | 1.176 | 1.170 | 1.168 | 1.162 | 1.158 |
| End | 1.068 | 1.052 | 1.053 | 1.053 | 1.055 | 1.054 | 1.052 | 1.051 | 1.050 |
| Reactor | Line Head | Along the Line | Line End |
|---|---|---|---|
| No compensation | 1.170 | 1.168 | 1.058 |
| Compensation degree 0.6 | 1.084 | 1.069 | 1.018 |
| Compensation degree 0.8 | 1.093 | 1.082 | 1.015 |
| Compensation degree 1.0 | 1.114 | 1.122 | 1.025 |
| Type | Opening Resistance (600 Ω) and Reactor (Compensation Degree 0.6) | Opening Resistance (600 Ω) | Reactor (Compensation Degree 0.6) | |
|---|---|---|---|---|
| Position | ||||
| Line head | 1.047 | 1.171 | 1.084 | |
| Along the line | 1.057 | 1.168 | 1.069 | |
| Line end | 1.014 | 1.052 | 1.018 | |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Sun, G.; Ma, W.; Wang, Y.; Xu, D.; Liu, H.; Chen, R.; Ding, Y. Load Rejection Overvoltage Suppression and Parameter Design Method of UHV AC Transmission Line. Electronics 2025, 14, 619. https://doi.org/10.3390/electronics14030619
Sun G, Ma W, Wang Y, Xu D, Liu H, Chen R, Ding Y. Load Rejection Overvoltage Suppression and Parameter Design Method of UHV AC Transmission Line. Electronics. 2025; 14(3):619. https://doi.org/10.3390/electronics14030619
Chicago/Turabian StyleSun, Guanqun, Wang Ma, Yingge Wang, Dian Xu, Haiguang Liu, Rusi Chen, and Yixing Ding. 2025. "Load Rejection Overvoltage Suppression and Parameter Design Method of UHV AC Transmission Line" Electronics 14, no. 3: 619. https://doi.org/10.3390/electronics14030619
APA StyleSun, G., Ma, W., Wang, Y., Xu, D., Liu, H., Chen, R., & Ding, Y. (2025). Load Rejection Overvoltage Suppression and Parameter Design Method of UHV AC Transmission Line. Electronics, 14(3), 619. https://doi.org/10.3390/electronics14030619





