Investigation of a Physical Model for the Reverse Recovery Characteristics of PT-PIN FRD with a Buffer Layer
Abstract
1. Introduction
2. Analytical Model of Carrier Distribution in FRDs Under the High-Level Injection Conditions
2.1. Analytical Model of Carrier Distribution in the Conventional PIN-FRD Suspended Wire Structure
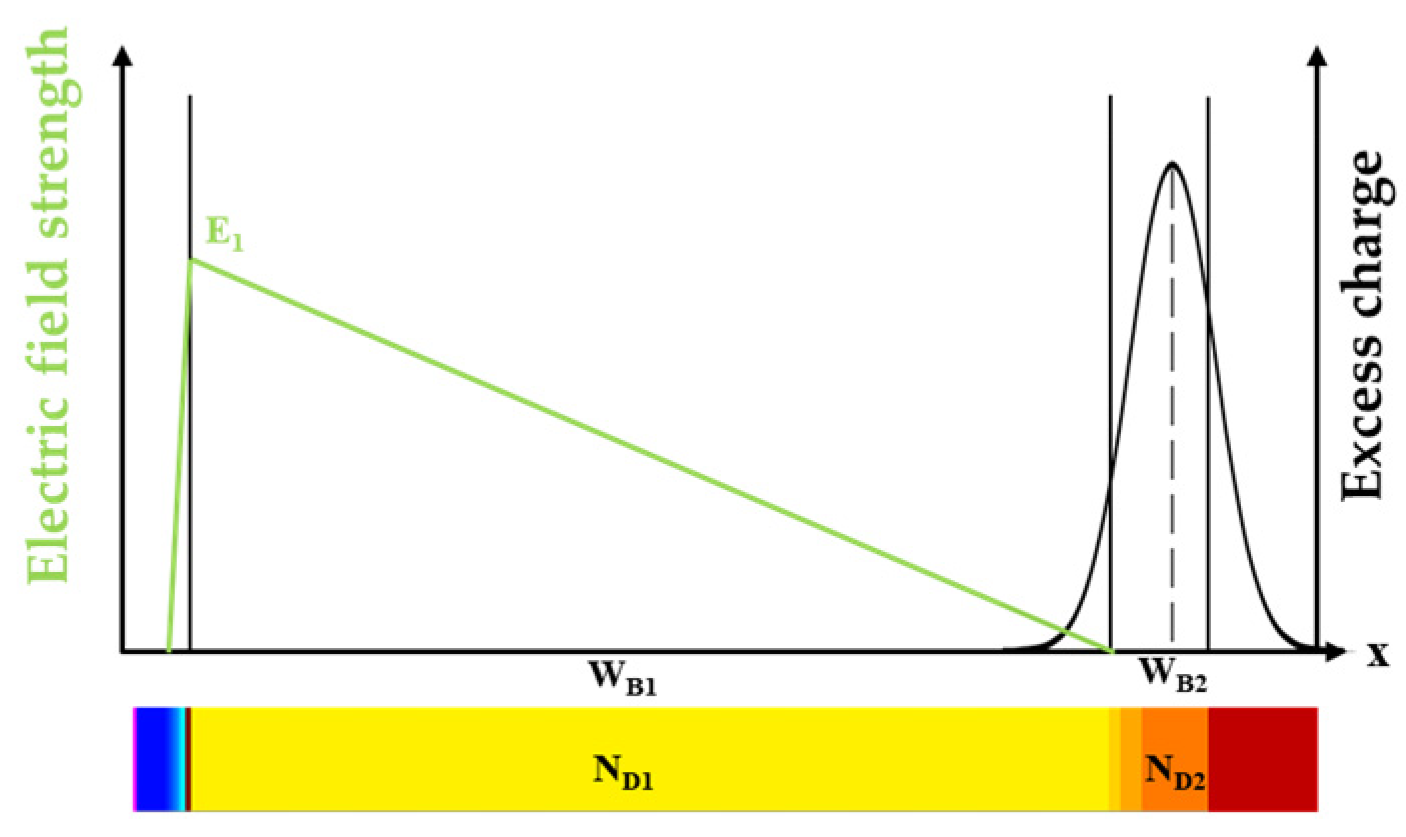
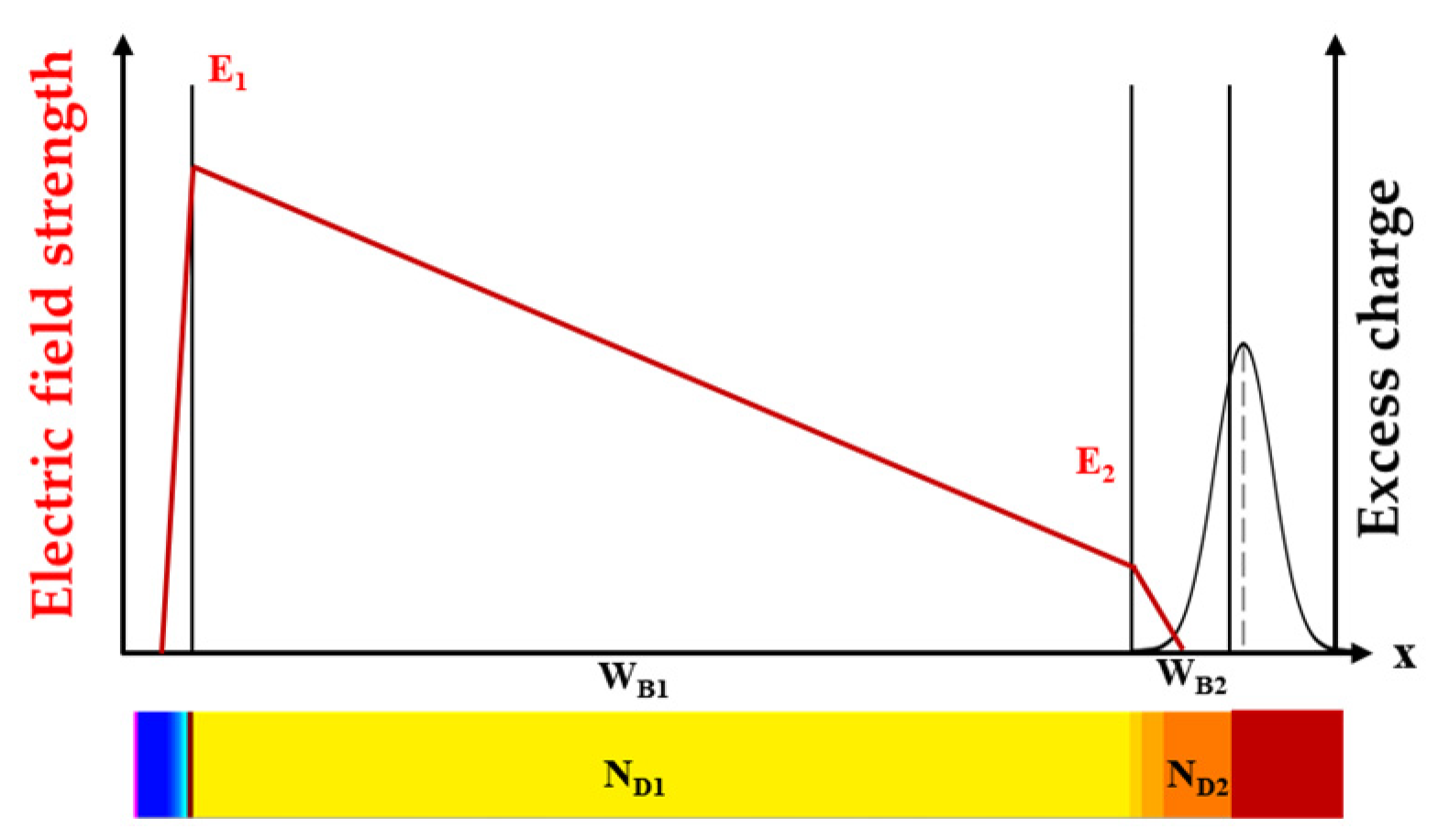
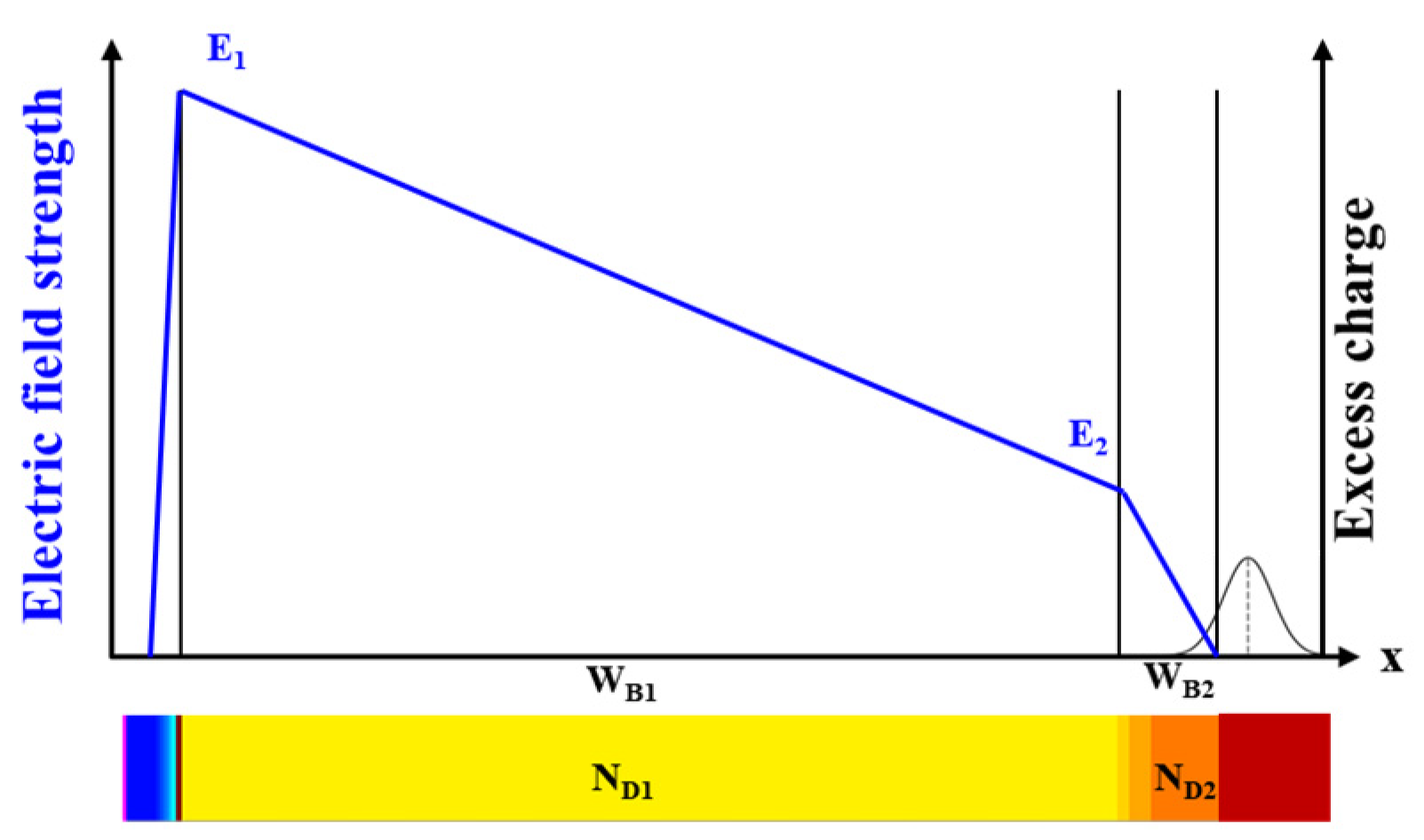
2.2. Analytical Model of Carrier Distribution in the Punchthrough P-I-N (PT-PIN) FRD with a Buffer Layer with a Suspended Wire Structure
2.3. Establishment of an Analytical Model for the Reverse Recovery Characteristics of PT-PIN FRD with a Buffer Layer
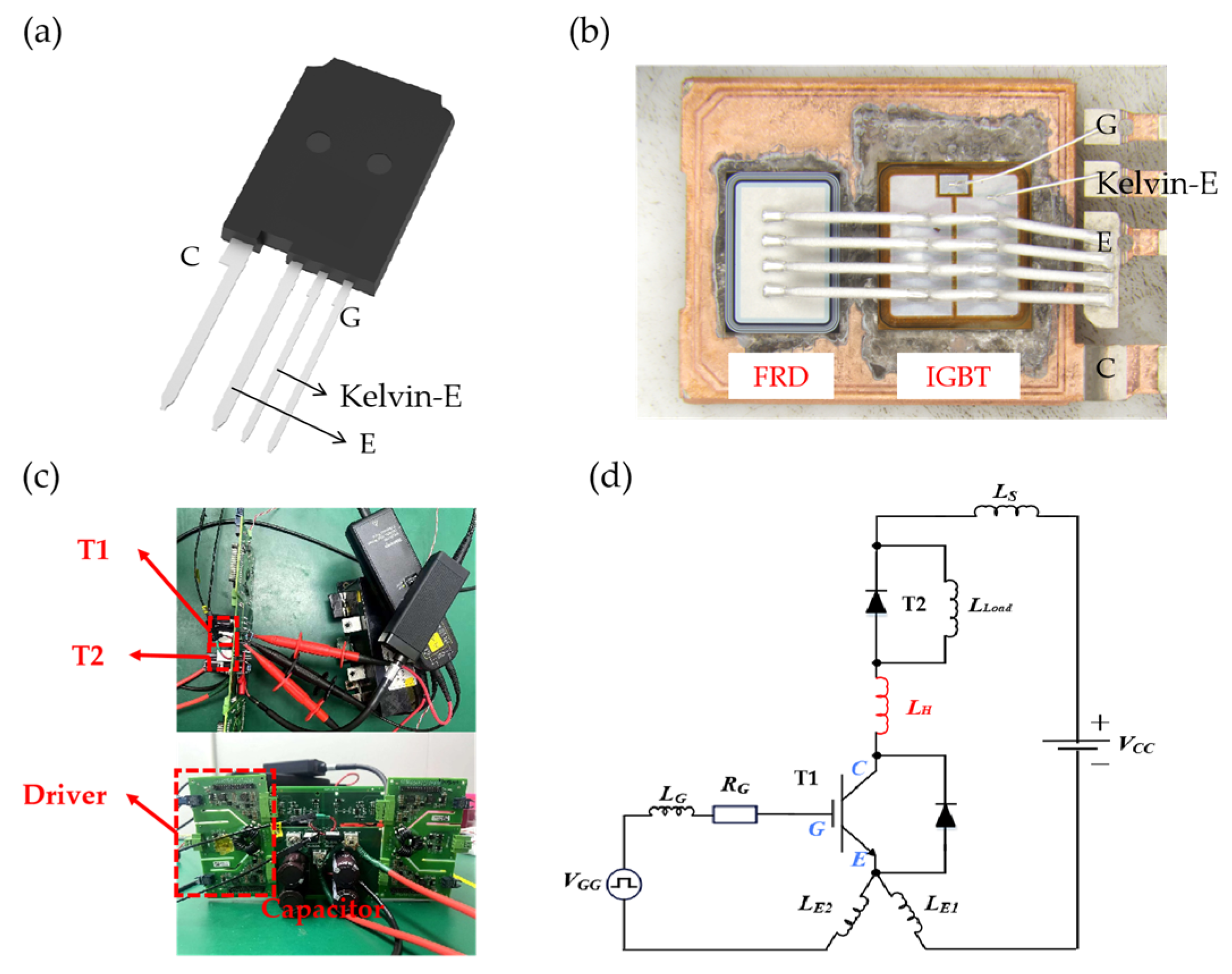
3. Device Physical Parameters and Experimental Setup
3.1. Physical Parameters of the Device
3.2. Construction of Experimental Platform
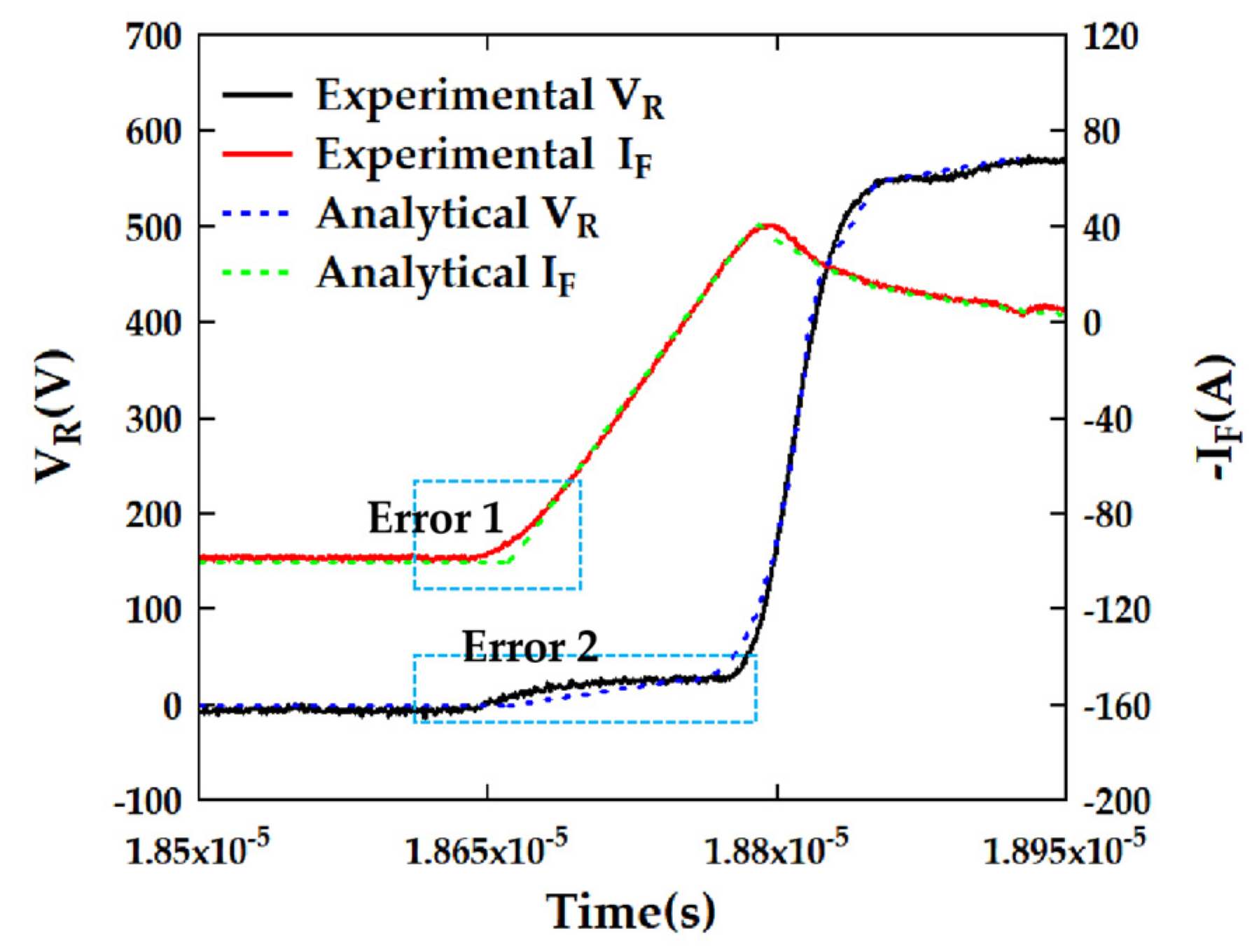
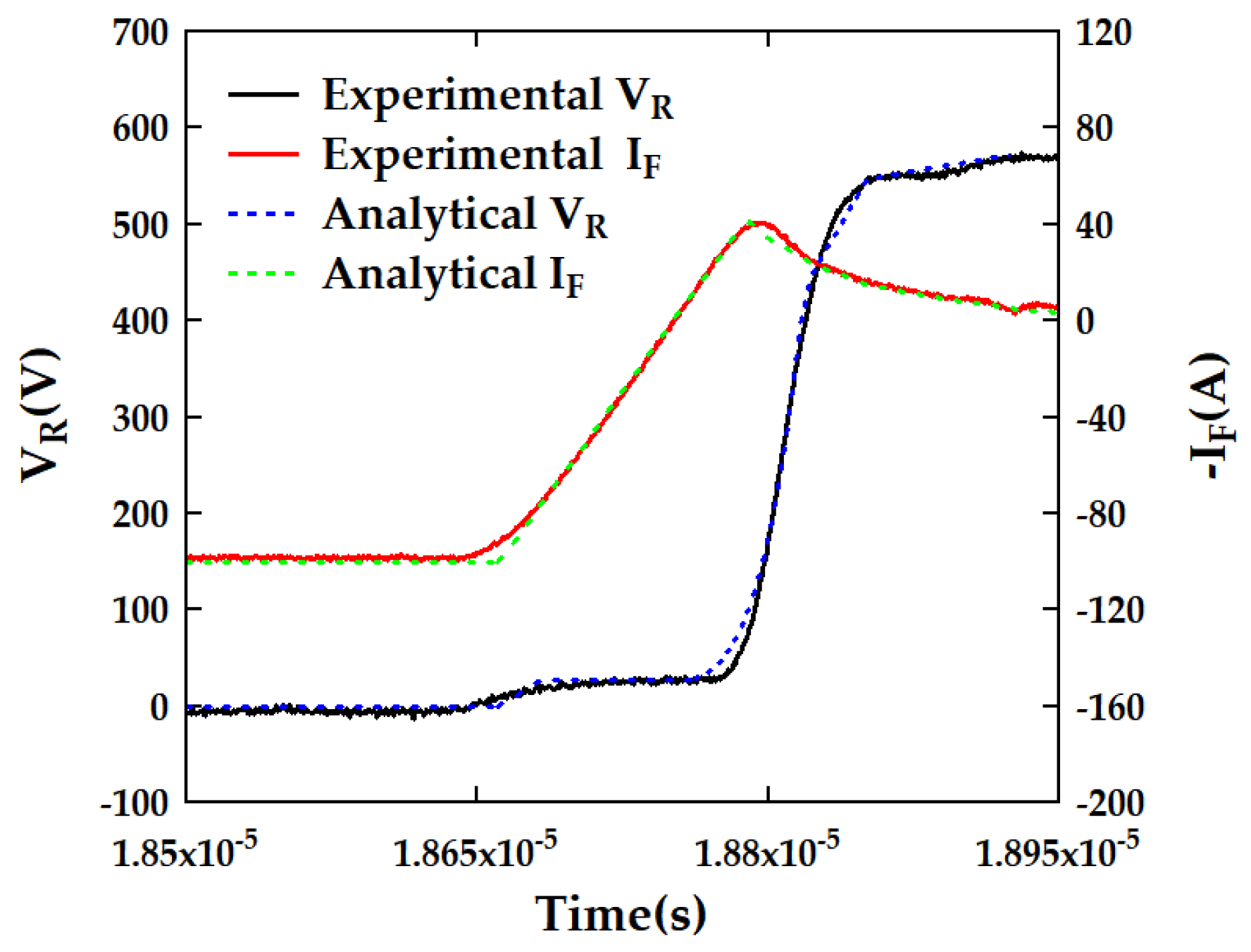
4. Experimental Certification and Discussion
4.1. Analytical Model Verification
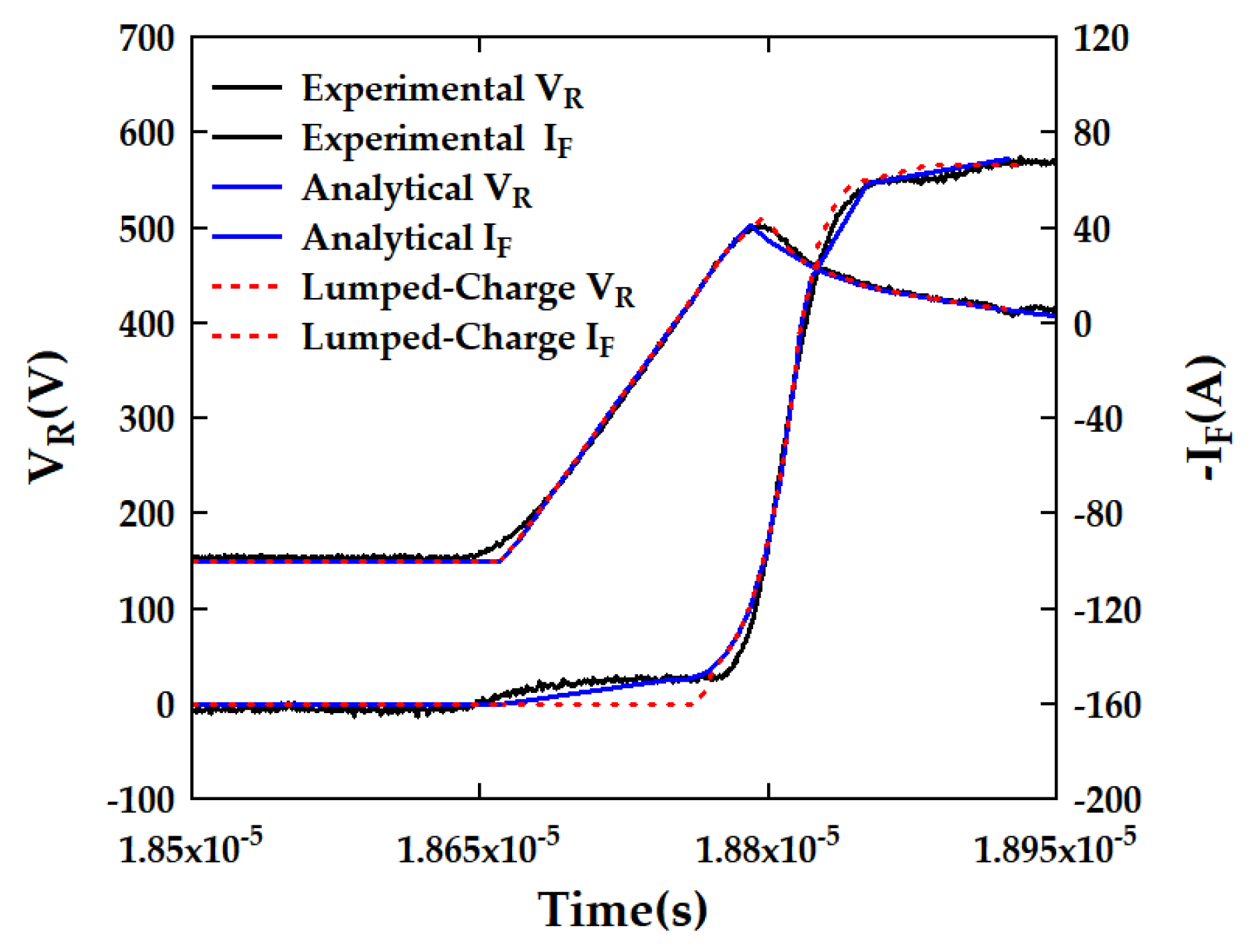
4.2. Comparison Between Analytical Model and the Lumped-Charge Model
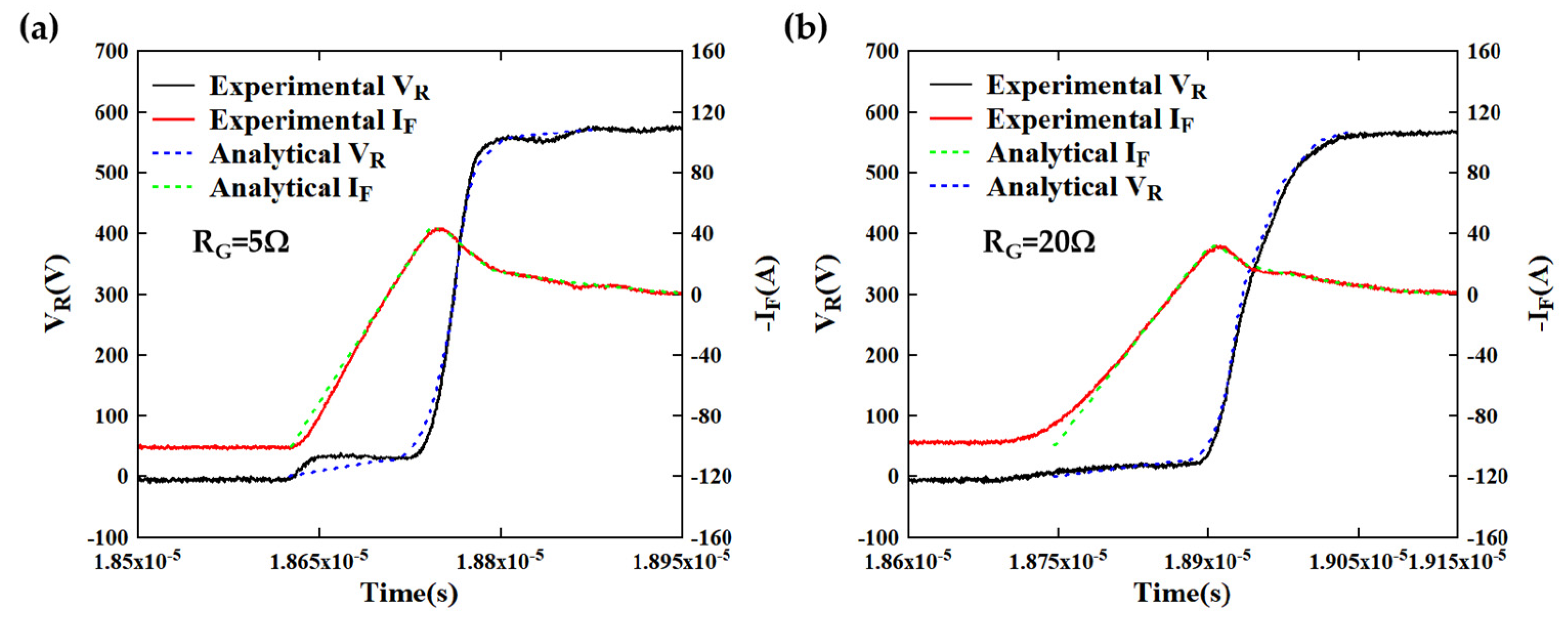
4.3. Analytical Model Certification Under Different Test RG
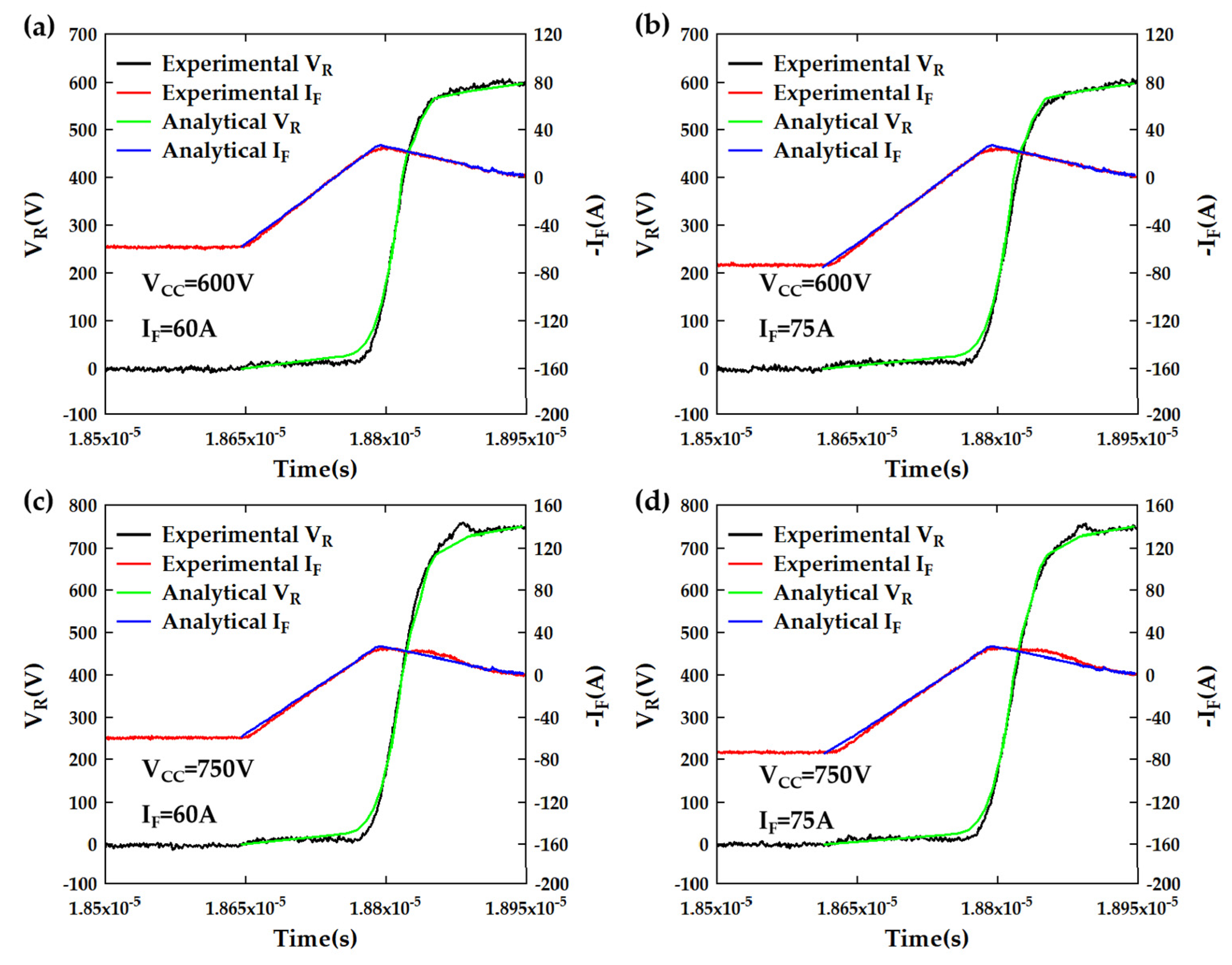
4.4. Analytical Model Certification Under Different VCC and IF
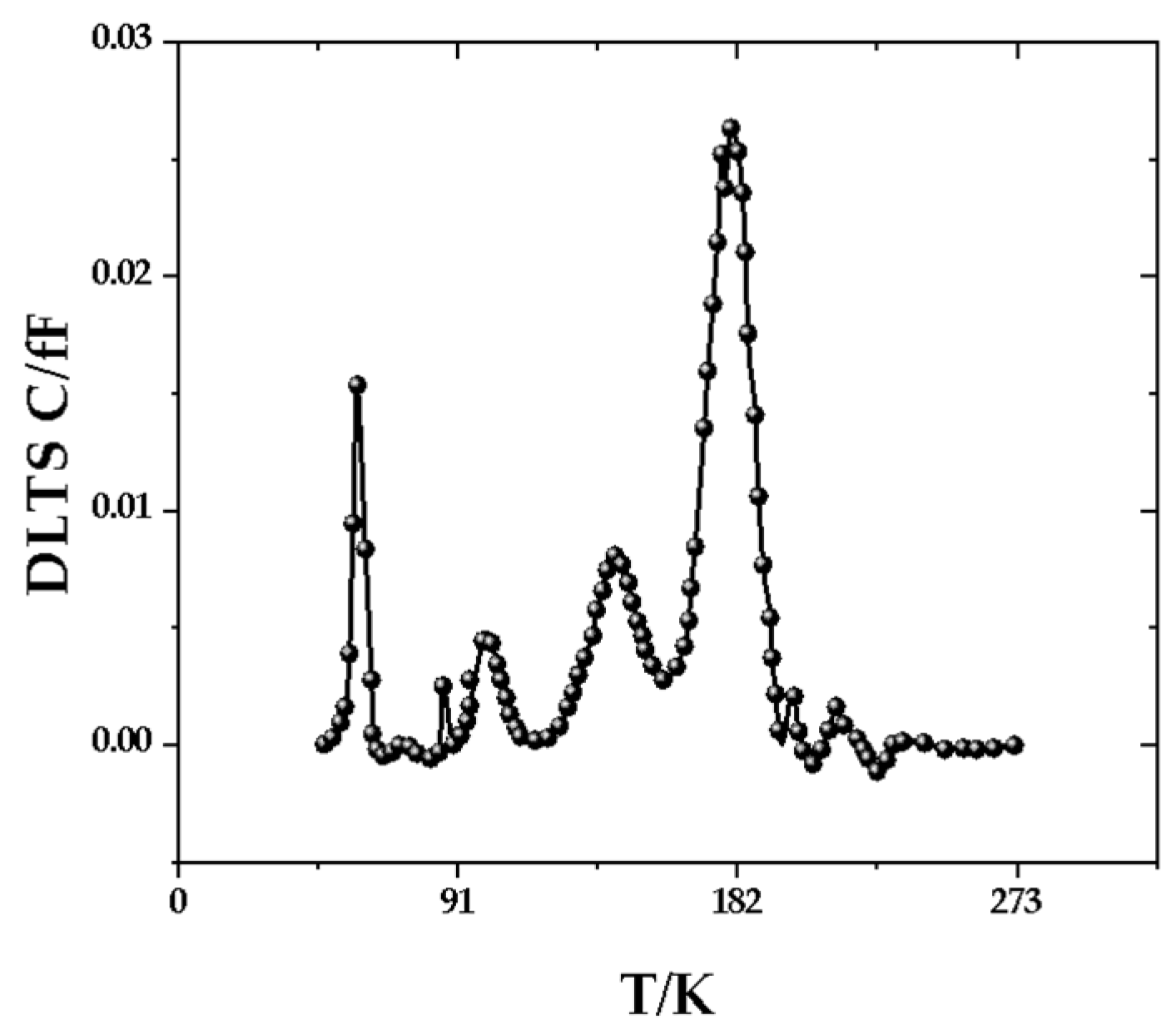
4.5. Analytical Model Certification Under Different Temperature
5. Conclusions
Supplementary Materials
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Wang, C.; Yin, L.; Wang, C. A physics-based model for fastrecovery diodes with lifetime control and emitter efficiency reduction. World Acad. Sci. Eng. Technol. 2012, 61, 7–11. [Google Scholar] [CrossRef]
- Bryant, A.; Lu, L.; Santi, E.; Hudgins, J.; Palmer, P. Modeling of IGBT resistive and inductive turn-on behavior. IEEE Trans. Ind. Appl. 2008, 44, 904–914. [Google Scholar] [CrossRef]
- Luo, Y.; Xiao, F.; Wang, B.; Liu, B.; Xia, Y. A voltage model of PIN diodes at reverse recovery under short-time freewheeling. IEEE Trans. Power Electron. 2017, 32, 142–149. [Google Scholar] [CrossRef]
- Mijlad, N.; Elwarraki, E.; Elbacha, A. SIMSCAPE electro-thermal modelling of the PIN diode for power circuits simulation. IET Power Electron. 2016, 9, 1521–1526. [Google Scholar] [CrossRef]
- Yamashita, Y.; Tadano, H. Numerical modeling of reverse recovery characteristic in silicon pin diodes. Solid-State Electron. 2018, 145, 8–18. [Google Scholar] [CrossRef]
- Karadžinov, Ĺ.; Jefferies, D.; Arsov, G.; Deane, J. Simple piecewise-linear diode model for transient behaviour. Int. J. Electron. 1995, 78, 143–160. [Google Scholar] [CrossRef]
- Jahdi, S.; Alatise, O.; Ran, L.; Mawby, P. Accurate analytical modeling for switching energy of PiN diodes reverse recovery. IEEE Trans. Ind. Electron. 2015, 62, 1461–1470. [Google Scholar] [CrossRef]
- Strollo, A. A new SPICE model of power PIN diode based onasymptotic waveform evaluation. IEEE Trans. Power Electron. 1997, 12, 12–20. [Google Scholar] [CrossRef]
- Shaker, A.; Abouelatta, M.; El-Banna, M.; Ossaimee, M.; Zekry, A. Full electrothermal physically-based modeling of the powerdiode using PSPICE. Solid-State Electron. 2016, 116, 70–79. [Google Scholar] [CrossRef]
- Li, X.; Luo, Y.; Duan, Y.; Huang, Y.; Liu, B. A lumped-charge model for high-power PT-p-i-n Diode with a buffer layer. IEEE J. Emerg. Sel. Top. Power Electron. 2019, 7, 52–61. [Google Scholar] [CrossRef]
- Li, X.; Xiao, F.; Luo, Y.; Wang, R.; Duan, Y. Modeling of high-voltage nonpunch-through PIN diode snappy reverse recovery and its optimal suppression method based on RC snubber circuit. IEEE Trans. Ind. Electron. 2021, 69, 5700–5712. [Google Scholar] [CrossRef]
- Jankovic, N.; Pesic, T.; Igic, P. All injection level power PIN diode model including temperature dependence. Solid-State Electron. 2007, 51, 719–725. [Google Scholar] [CrossRef]
- Benda, H.; Spenke, E. Reverse recovery processes in silicon power rectifiers. Proc. IEEE 1967, 55, 1331–1354. [Google Scholar] [CrossRef]
- Baliga, B.; Sun, E. Comparison of gold, platinum, and electron irradiation for controlling lifetime in power rectifiers. IEEE Trans. Electron Device 1977, 24, 685–688. [Google Scholar] [CrossRef]
- Takata, I.; Bessho, M.; Koyanagi, K.; Akamatsu, M.; Satoh, K.; Kurachi, K. Snubberless turn-off capability of four-inch 4.5 kV GCT thyristor. In Proceedings of the 10th International Symposium on Power Semiconductor Devices and ICs, Kyoto, Japan, 3–6 June 1998; pp. 177–180. [Google Scholar] [CrossRef]
- Xue, P.; Fu, G.; Zhang, D. Comprehensive physics-based compact model for fast pin diode using MATLAB and simulink. Solid-State Electron. 2016, 121, 1–11. [Google Scholar] [CrossRef]
- Ghandhi, S. Semiconductor Power Devices; Wiley: New York, NY, USA, 1977; pp. 112–128. [Google Scholar]
- Hall, R. Power rectifiers and transistors. Proc. IRE 1952, 40, 1512–1518. [Google Scholar] [CrossRef]
- Khaleqi Qaleh Jooq, M.; Miralaei, M.; Ramezani, A. Post-layout simulation of an ultra-low-power OTA using DTMOS input differential pair. Int. J. Electron. Lett. 2017, 6, 168–180. [Google Scholar] [CrossRef]
- Khaleqi Qaleh Jooq, M.; Mir, A.; Mirzakuchaki, S.; Farmani, A. A robust and energy-efficient near-threshold SRAM cell utilizing ballistic carbon nanotube wrap-gate transistors. AEU—Int. J. Electron. Commun. 2019, 110, 152874. [Google Scholar] [CrossRef]
- Ballga, J. Fundamentals of Power Semiconductor Devices; Springer: Berlin/Heidelberg, Germany, 2008; Volume 95. [Google Scholar]
- Carlson, R.; Sun, Y.; Assalit, H. Lifetime control in silicon power devices by electron or gamma irradiation. IEEE Trans. Electron Devices 1977, 24, 1103–1108. [Google Scholar] [CrossRef]
- Siemieniec, R.; Sudkamp, W.; Lutz, J. Determination of parameters of radiation induced traps in silicon. Solid-State Electron. 2002, 46, 891–901. [Google Scholar] [CrossRef]
- Siemieniec, R.; Lutz, J. Possibilities and limits of axial lifetime control by radiation induced centers in fast recovery diodes. Microelectron. J. 2004, 35, 259–267. [Google Scholar] [CrossRef]
- Onishi, H.; Shirai, H. Silicon ultrafast recovery diode with leakage current reduced via the combined lifetime process of gold diffusion and electron-beam irradiation. Semicond. Sci. Technol. 2024, 39, 015011. [Google Scholar] [CrossRef]







| Parameters | Physical Meaning |
|---|---|
| PN | Concentration of majority carriers in the P-region |
| P0 | Equilibrium concentration of majority carriers in the P-region |
| q | Electronic charge |
| Va | Diode junction voltage |
| k | Boltzmann constant |
| T | Absolute temperature |
| n(x) | Concentration of electrons |
| p(x) | Concentration of holes |
| ND | Doping concentration |
| τHL | Carrier lifetime |
| D | Diffusion coefficient |
| μ | Mobility |
| La | Diffusion length |
| J | Current density |
| JT/JF | Initial current density |
| W(2d) | Width of the N− drift |
| τi | Initial carrier lifetime |
| ϕ | Total number of electrons per unit area |
| K | Energy of the electrons that induce lattice damage |
| na | Average carrier concentration |
| Q | Charge extracted during the reverse recovery process |
| b | Location of the average carrier concentration |
| t1 | Moment at which IF first returns to 0 |
| a | dI/dt |
| t2 | Moment at which VR begins to increase from zero |
| t3 | Moment at which depletion region reaches the left boundary of the buffer layer |
| t4 | Moment at which depletion region extends within the buffer layer |
| E | Electric field strength |
| ε | Dielectric constant |
| Vsat,p | Holes drift at a saturation velocity |
| WSC | Depletion region width |
| IRR | Peak current |
| L | Distance from center of the undepleted region to edge |
| Physical Parameters | Value |
|---|---|
| P+ implant element | Boron |
| P+ implant energy | 80 (Kev) |
| P+ implant dose | 1 × 1013 (ion/cm−3) |
| P+ drive in temperature | 1150 (°C) |
| P+ drive in time | 450 (min) |
| N− drift doping concentration | 6.14 × 1013 (cm−3) |
| N− Drift thickness | 80 (μm) |
| Buffer layer doping concentration | 2 × 1014 (cm−3) |
| Buffer layer thickness | 25 (μm) |
| FRD area | 0.35 (cm2) |
| Conduction current | 100 (A) |
| Electron irradiation energy | 10 (Mev) |
| Electron irradiation dose | 120 (kGy) |
| Anneal temperature | 350 (°C) |
| Anneal time | 30 (min) |
| τHL [21,22,23,24,25] | 0.1 (μs) |
| Circuit Parameters | Value |
|---|---|
| Lload | 100 (uH) |
| Ls | 100 (nH) |
| RG (on) | 10 (Ω) |
| RG (off) | 15 (Ω) |
| VGE | −8~15 (V) |
| VCC | 600 (V) |
| Equipment | Model Number |
|---|---|
| Oscilloscope | MSO56 5-BW-500 |
| Pulse generator | QTJ15610A |
| High-voltage DC power supply | TDK Z+ 650-1 |
| Rothschild coil | IWATSU SS-286A |
| Driver plate | FZ1200R33KF2C |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Sun, Y.; Ma, K.; Yuan, X.; Chen, A.; Liu, X.; Song, Y.; Li, X.; Zi, T.; Zhou, Y.; Liu, S. Investigation of a Physical Model for the Reverse Recovery Characteristics of PT-PIN FRD with a Buffer Layer. Electronics 2025, 14, 570. https://doi.org/10.3390/electronics14030570
Sun Y, Ma K, Yuan X, Chen A, Liu X, Song Y, Li X, Zi T, Zhou Y, Liu S. Investigation of a Physical Model for the Reverse Recovery Characteristics of PT-PIN FRD with a Buffer Layer. Electronics. 2025; 14(3):570. https://doi.org/10.3390/electronics14030570
Chicago/Turabian StyleSun, Yameng, Kun Ma, Xiong Yuan, Anning Chen, Xun Liu, Yifan Song, Xuehan Li, Tongtong Zi, Yang Zhou, and Sheng Liu. 2025. "Investigation of a Physical Model for the Reverse Recovery Characteristics of PT-PIN FRD with a Buffer Layer" Electronics 14, no. 3: 570. https://doi.org/10.3390/electronics14030570
APA StyleSun, Y., Ma, K., Yuan, X., Chen, A., Liu, X., Song, Y., Li, X., Zi, T., Zhou, Y., & Liu, S. (2025). Investigation of a Physical Model for the Reverse Recovery Characteristics of PT-PIN FRD with a Buffer Layer. Electronics, 14(3), 570. https://doi.org/10.3390/electronics14030570






