Reactive Power Equalization Strategy for Islanded Microgrids Based on Improved Adaptive Droop Factor
Abstract
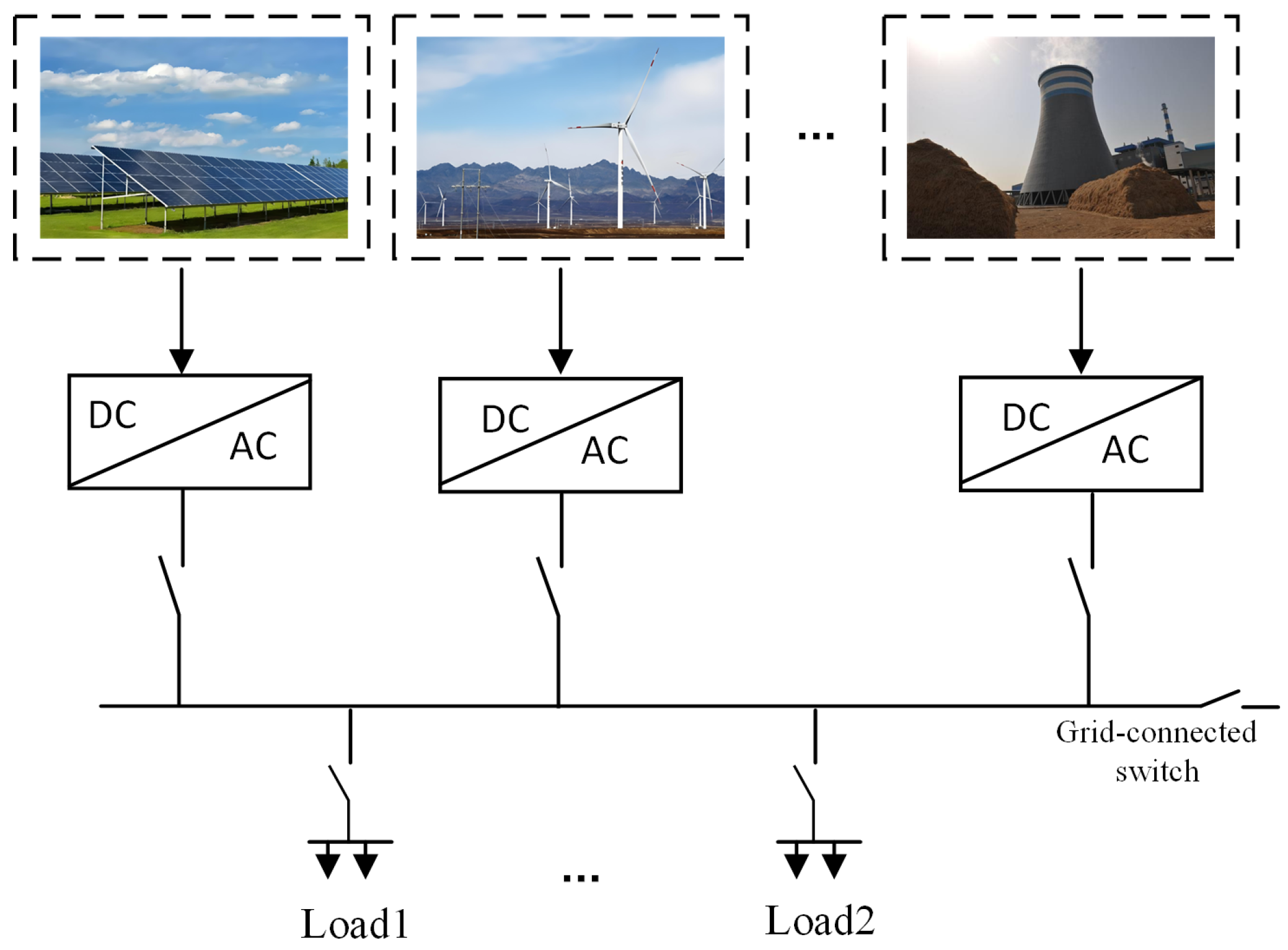
1. Introduction
2. Analysis of Traditional Droop Control Strategy
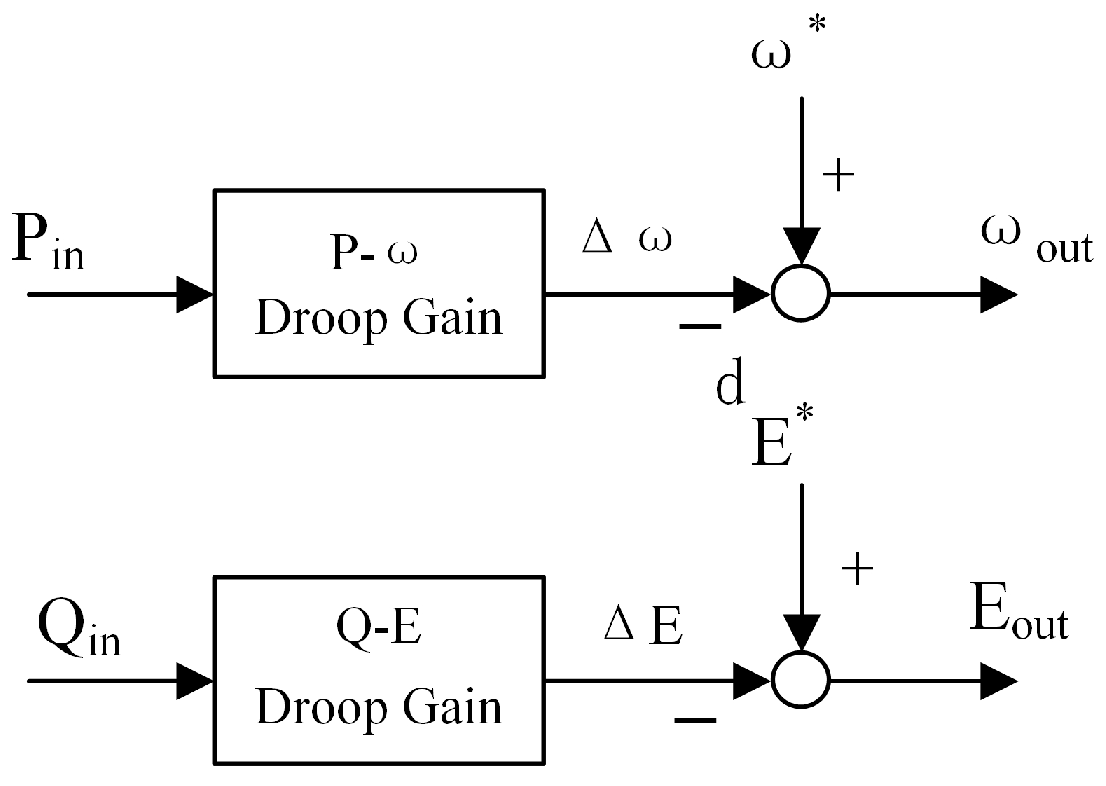
2.1. Traditional Droop Control Approach
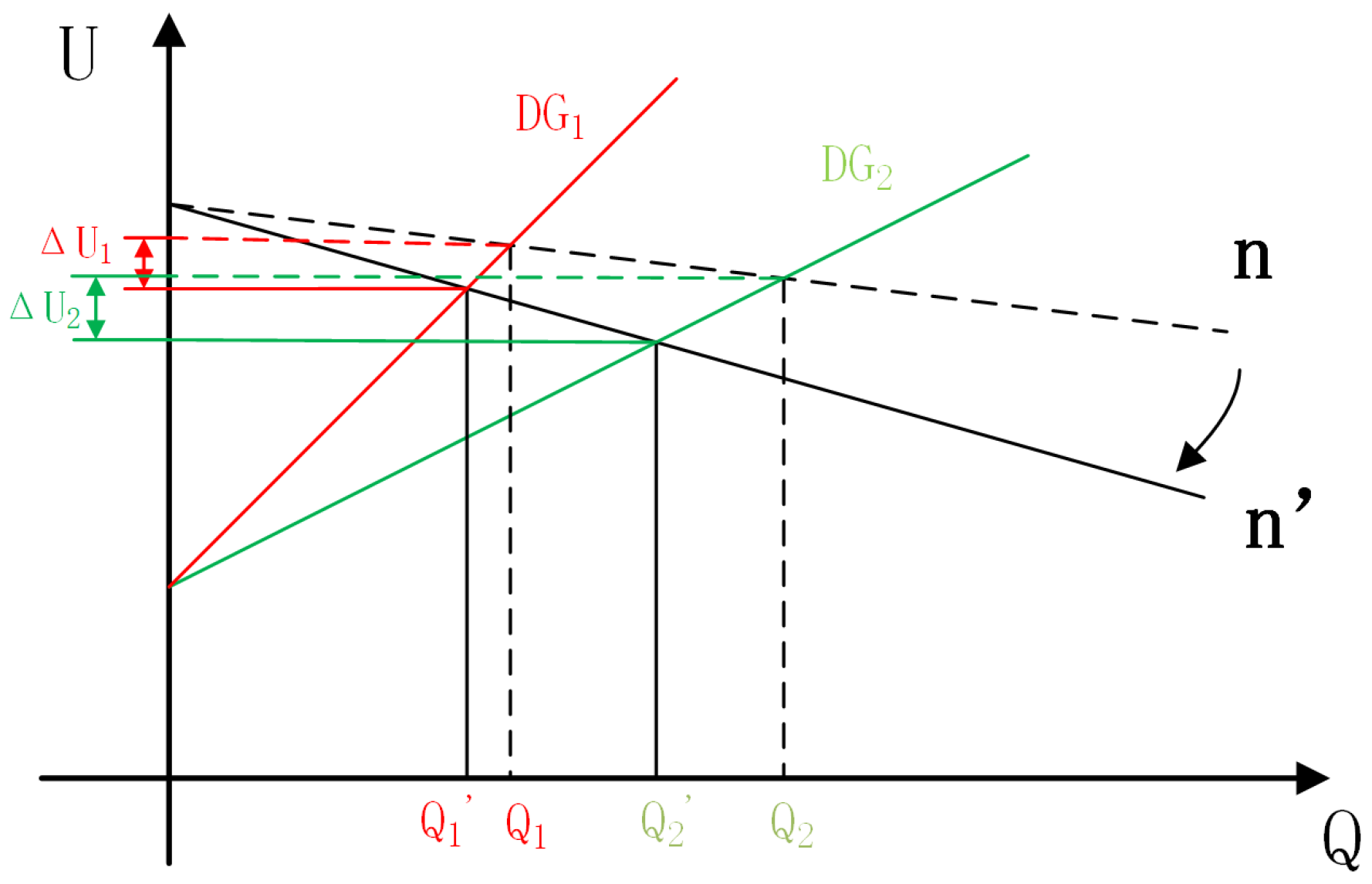
2.2. Power Sharing Analysis
3. Control Strategies for Adaptive Droop Coefficients
3.1. Adaptive Droop Coefficients Based on Improved Consistency Algorithms
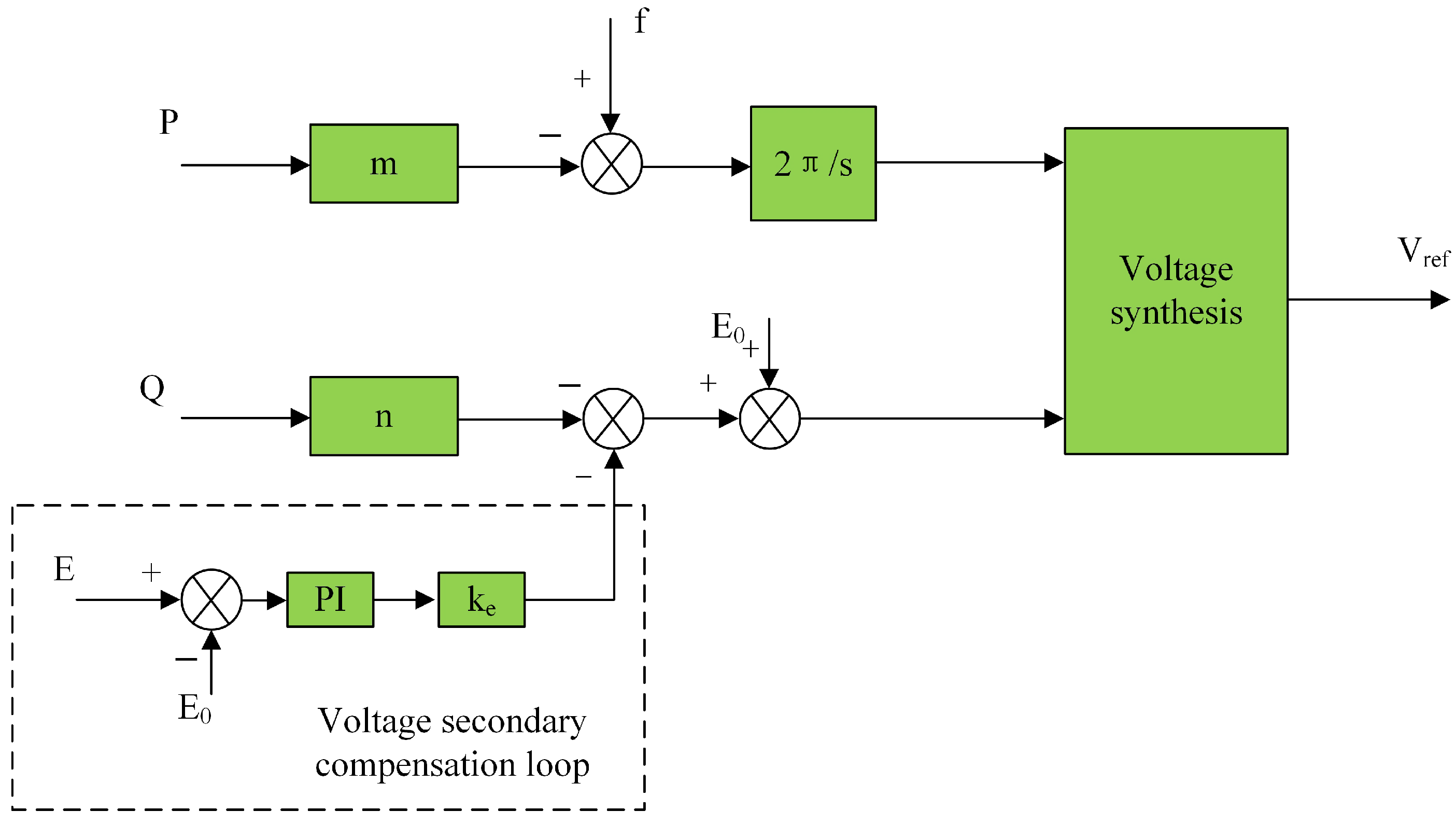
3.2. Voltage Secondary Compensation Strategy
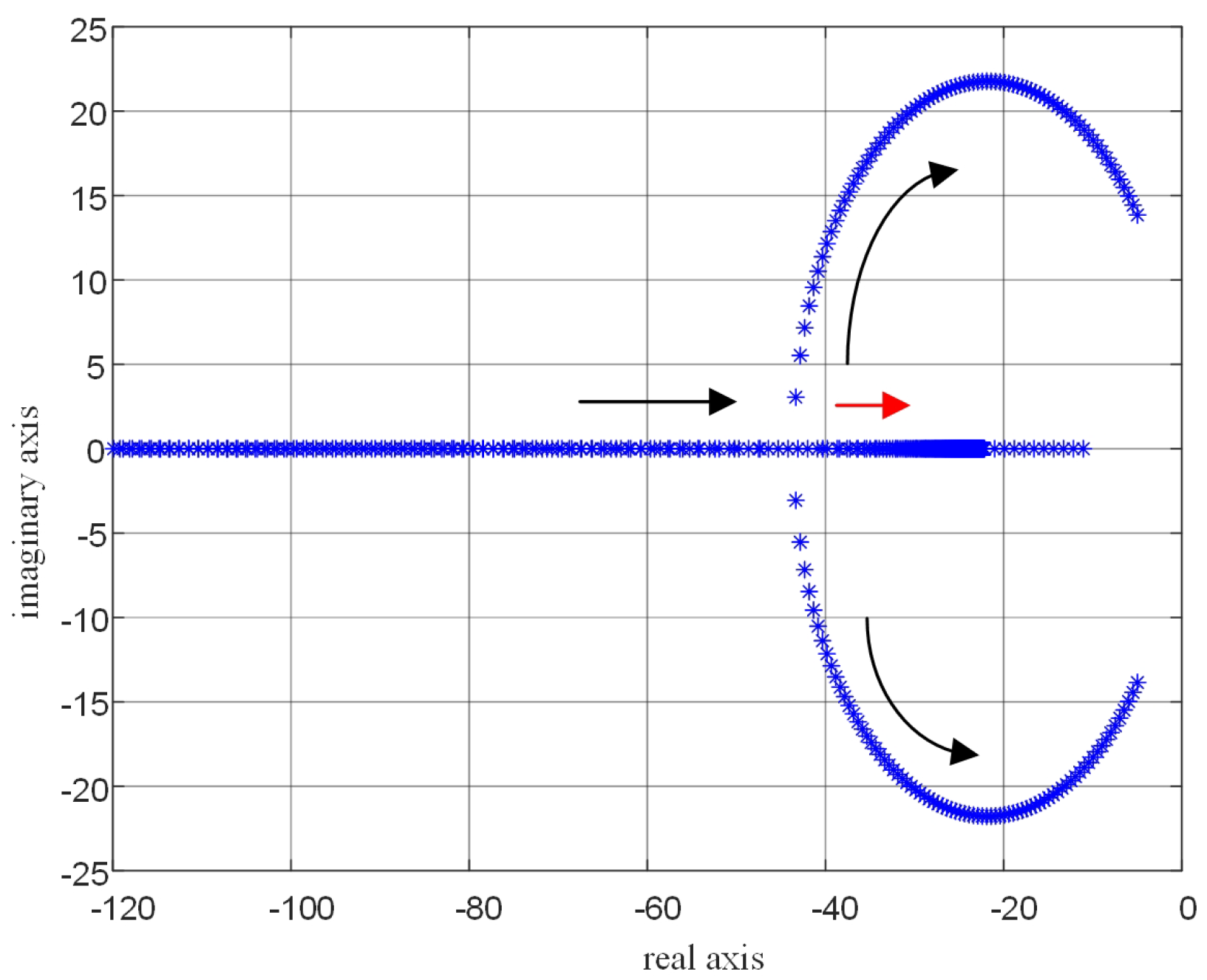
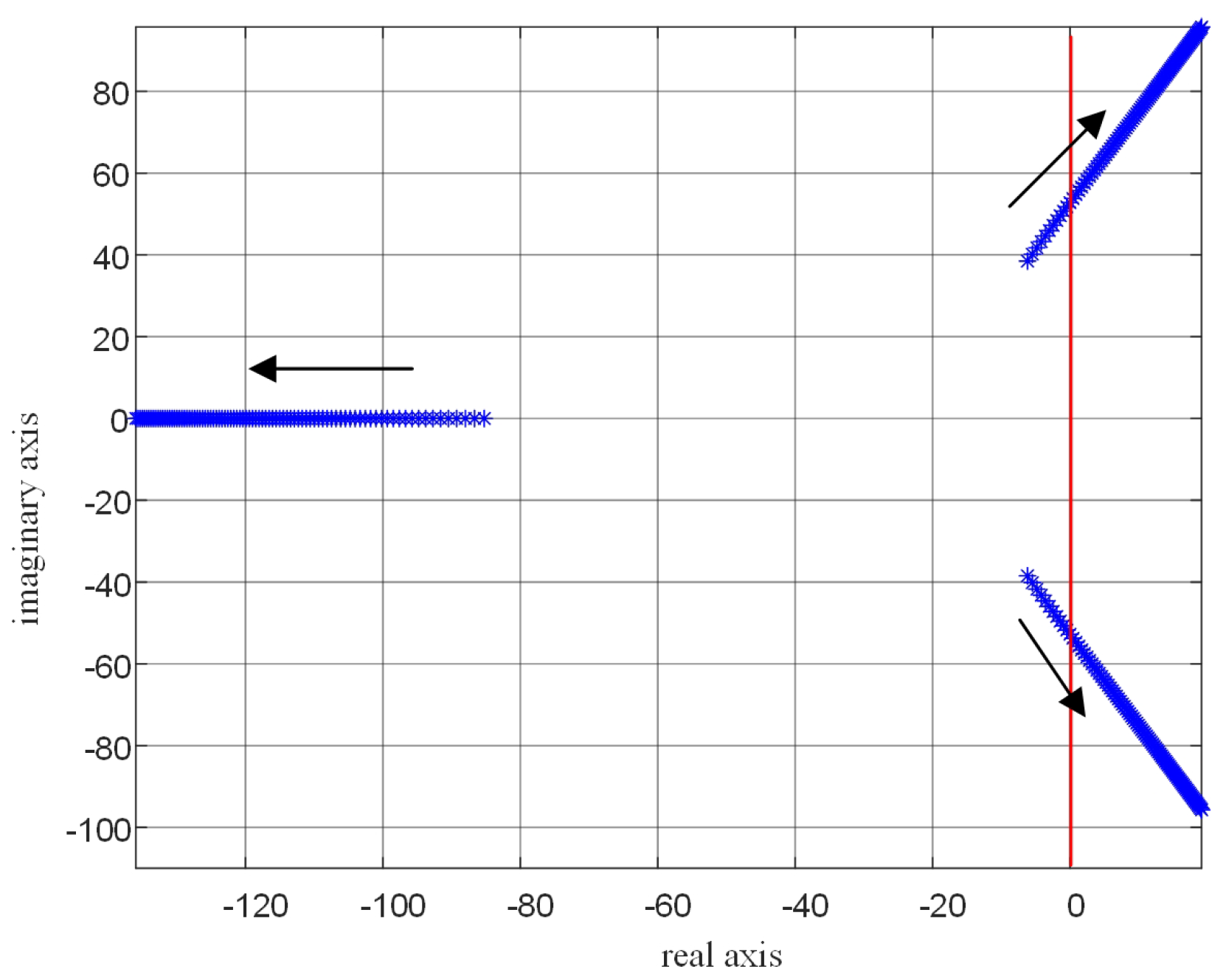
3.3. System Stability Analysis
4. Verification via Simulation
| Parameters | Values | Parameters | Values |
|---|---|---|---|
| DC link voltage | 800 V | Load 2 (W,) | 5000 + j5000 |
| Switching frequency | 20 kHZ | Active droop coefficient | 0.000314 |
| Filter inductance | 3 mH | Reactive droop coefficient | 0.000622 |
| Filter capacitor | 20 μF | Control gain c | 0.1 |
| Filter resistance | 0.2 | Voltage compensation coefficient | 0.14 |
| Nominal frequency | 50 Hz | Low-Pass filter | 50 Hz |
| Noninai line to line RMS voltage | 380 V | 0.1 | |
| ’s Line impedance | 0.7 + j0.007 | 10 | |
| ’s Line impedance | 0.5 + j0.0065 | 0.8 | |
| Load 1 (W,) | 10,000 + j10,000 | 20 |
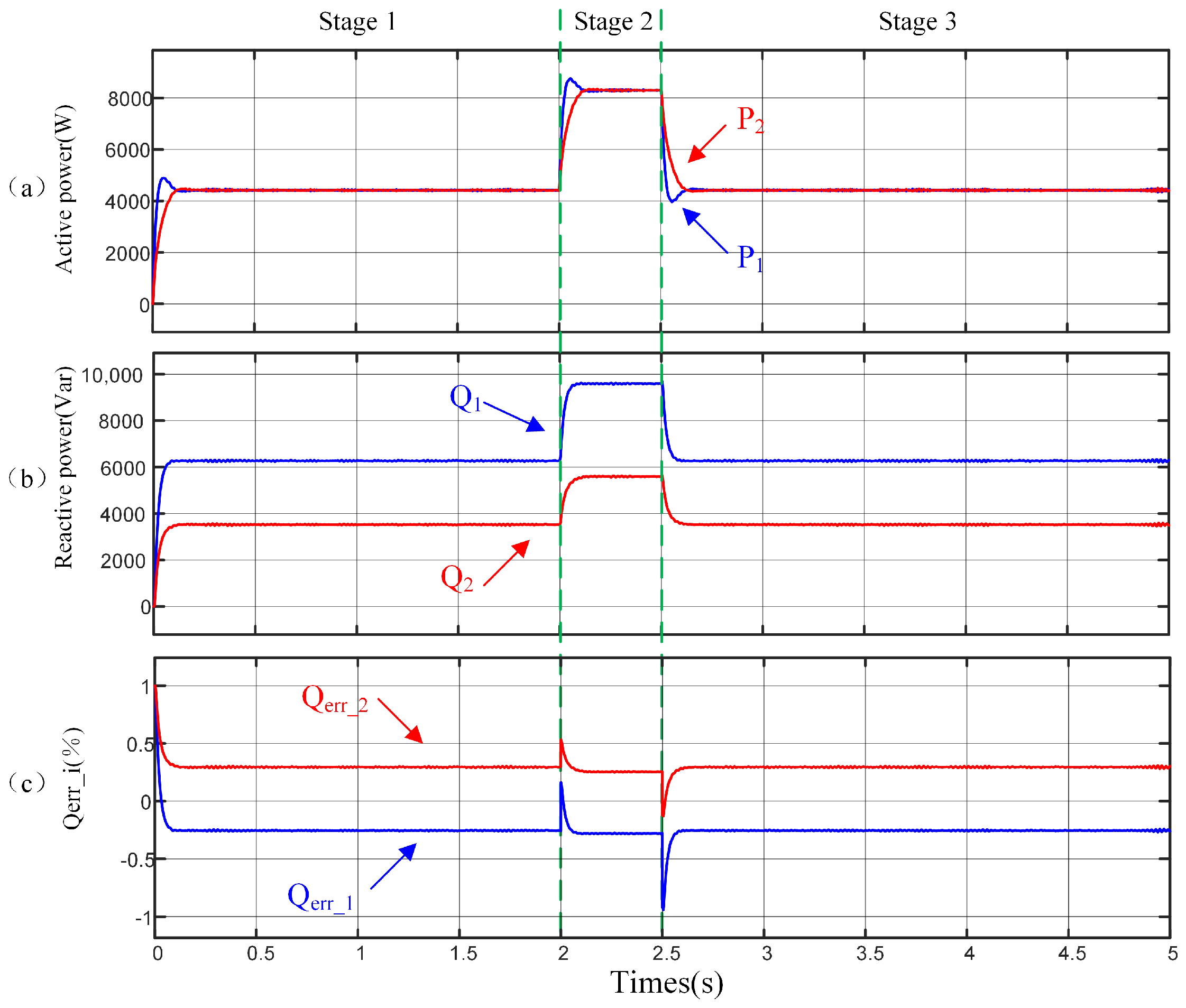
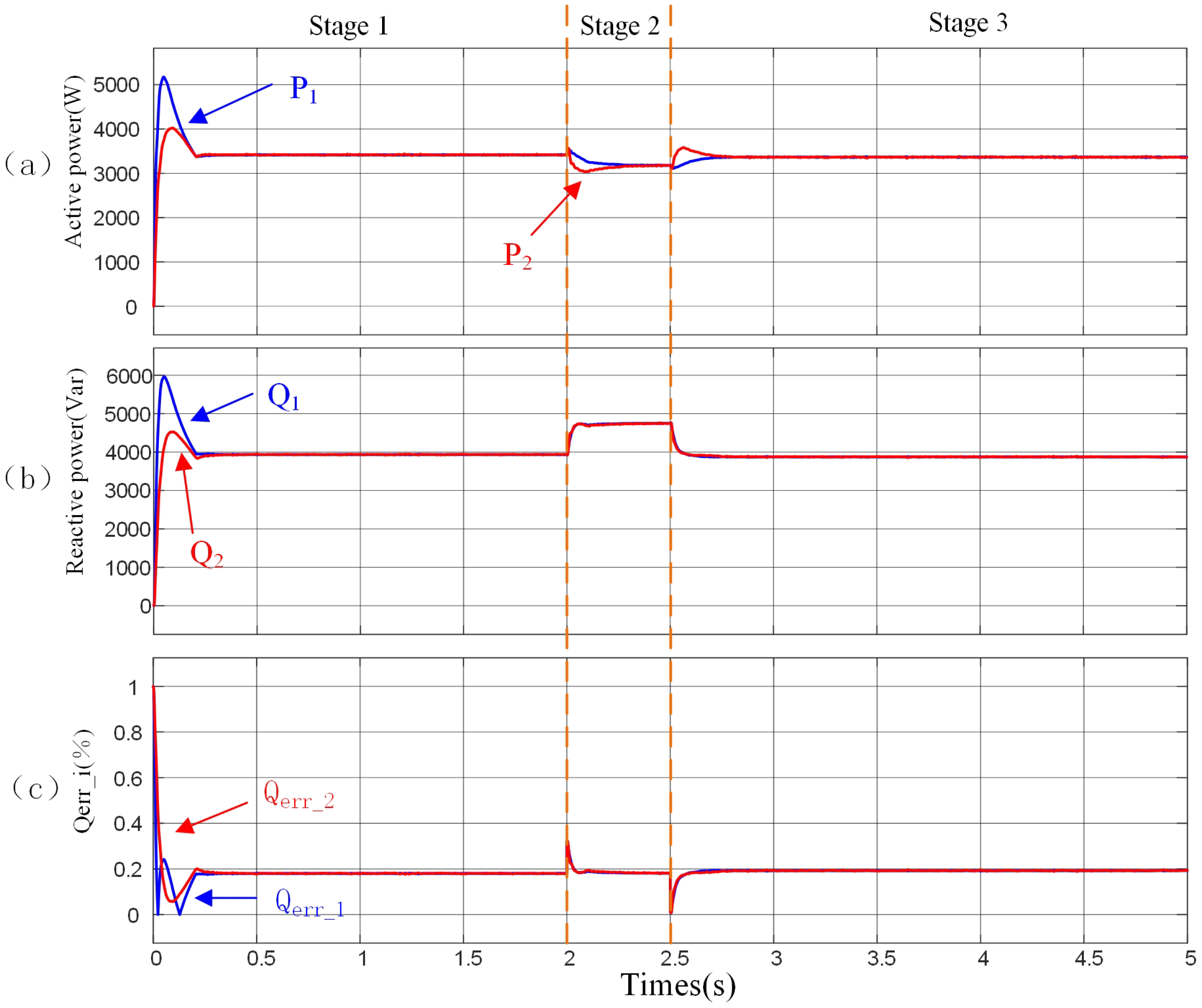
4.1. Performance with Conventional Controller
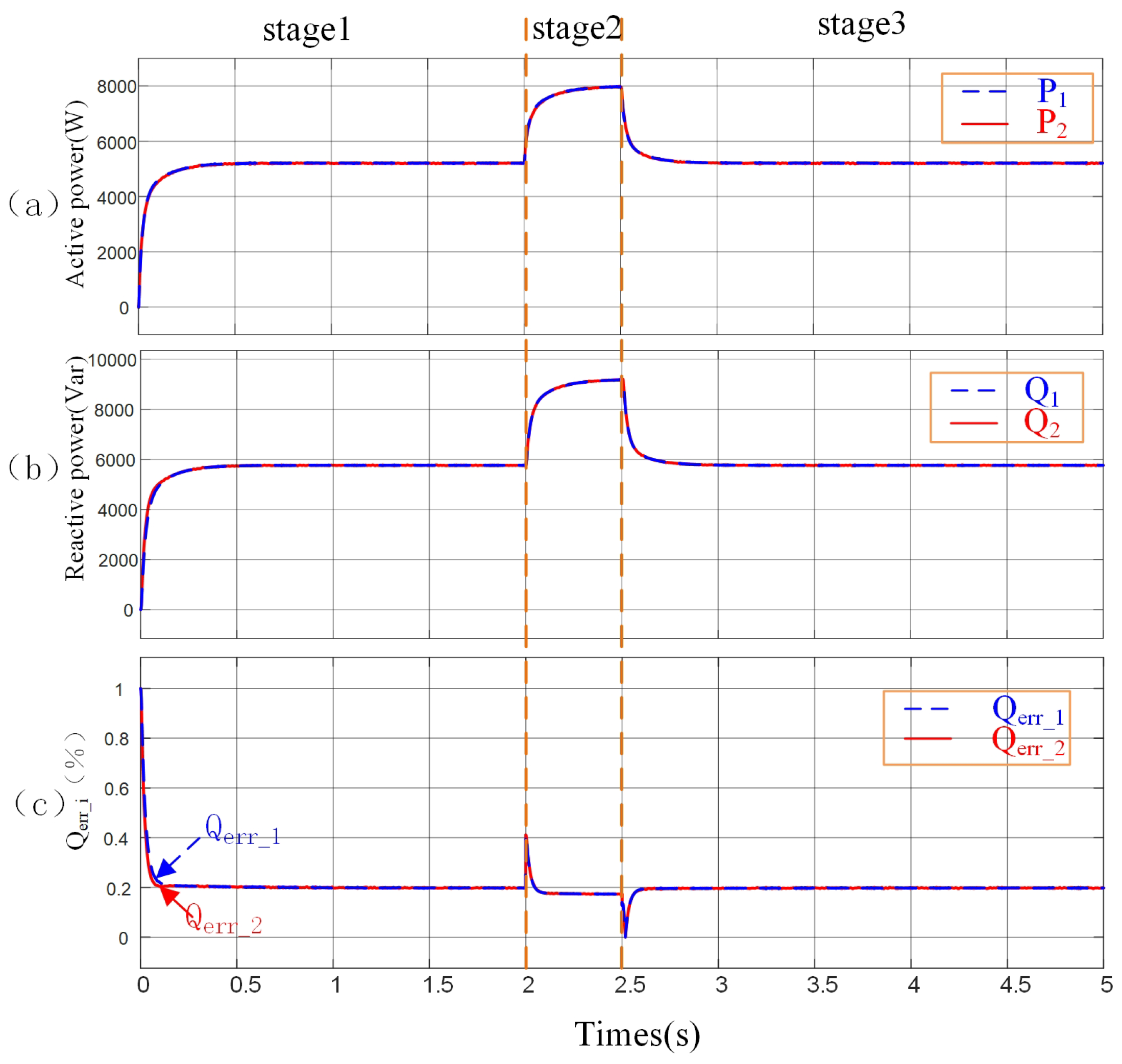
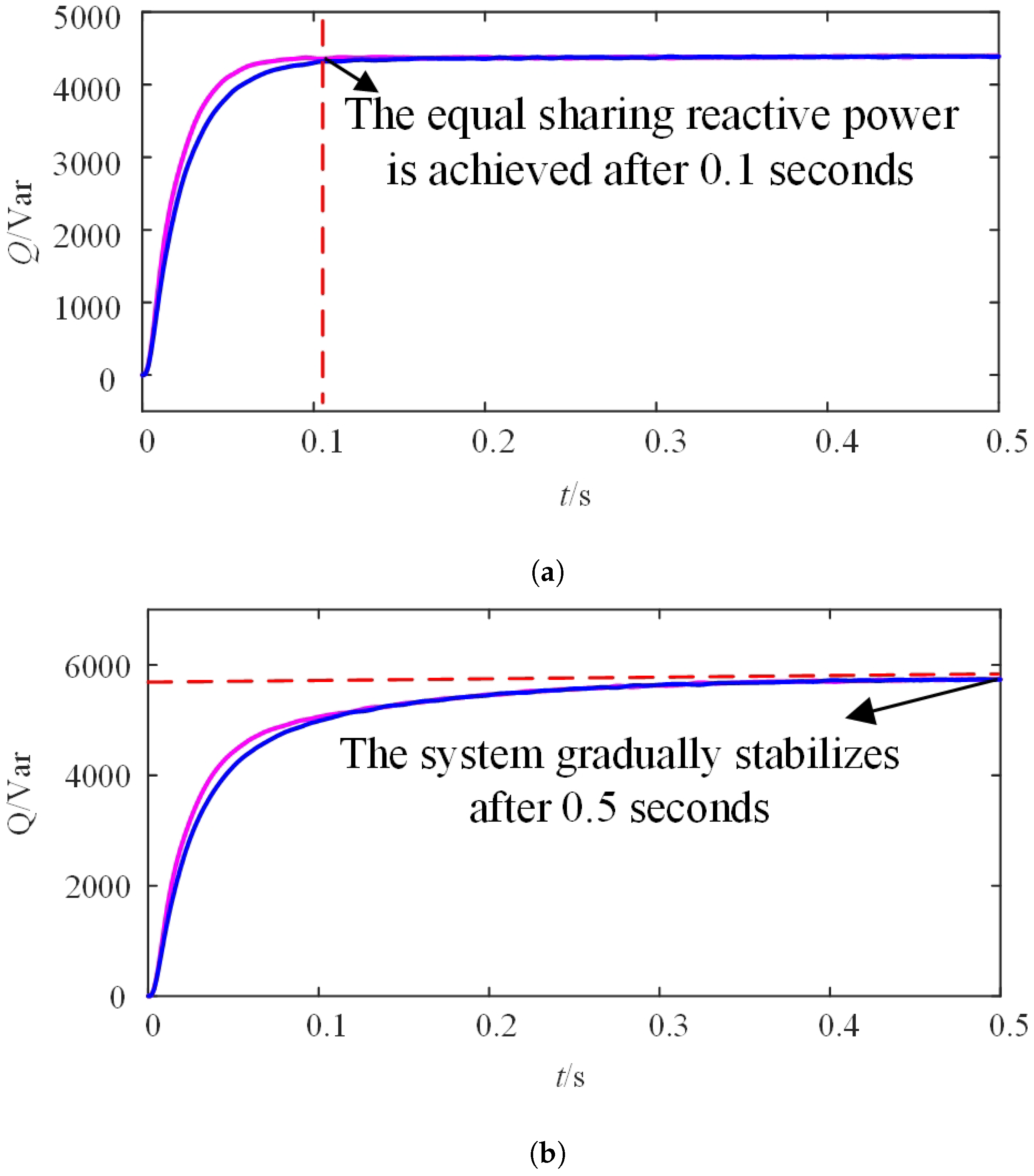
4.2. Performance When Using the Proposed Controller
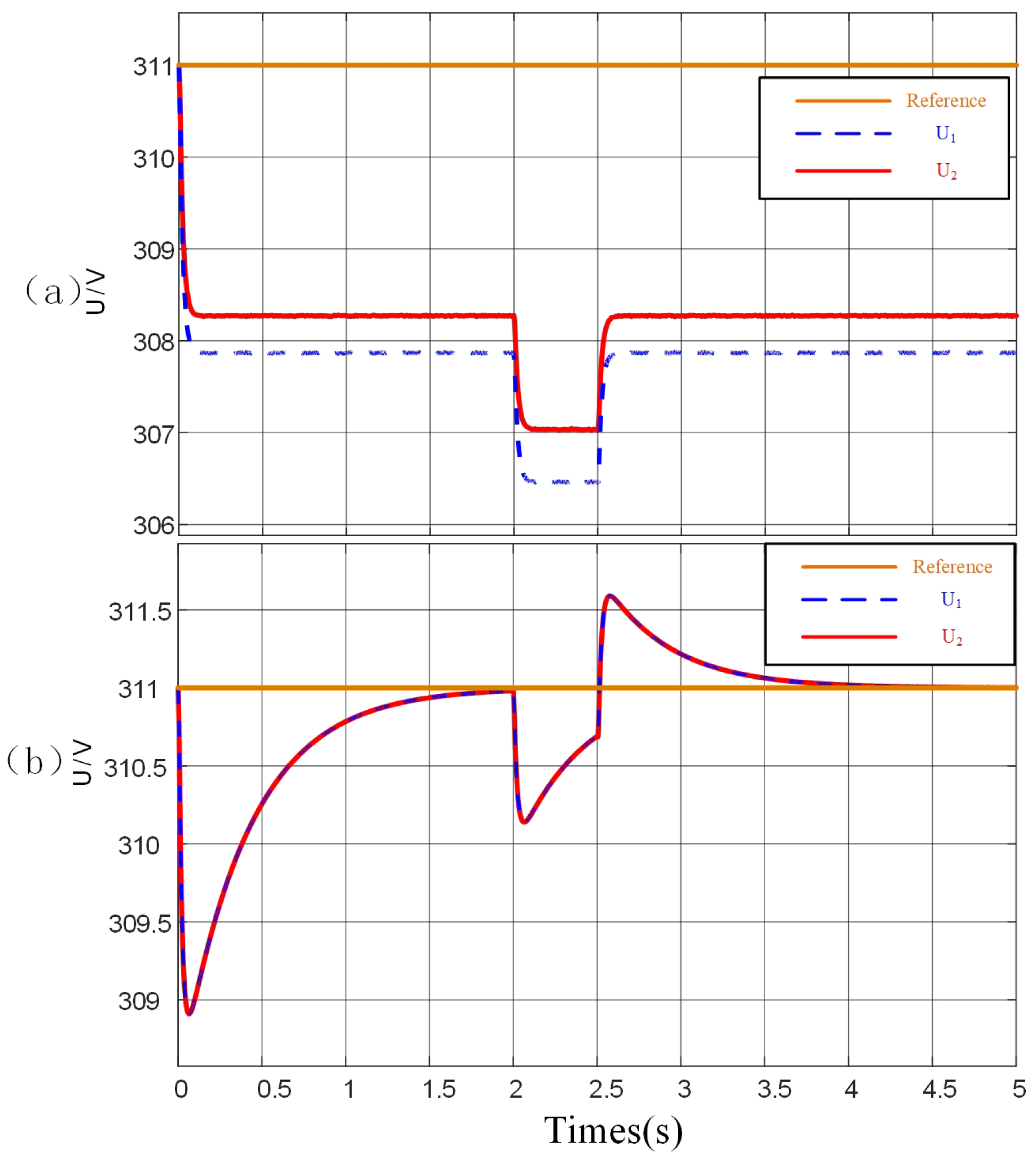
4.3. The Voltage Compensation Performance of the Proposed Method
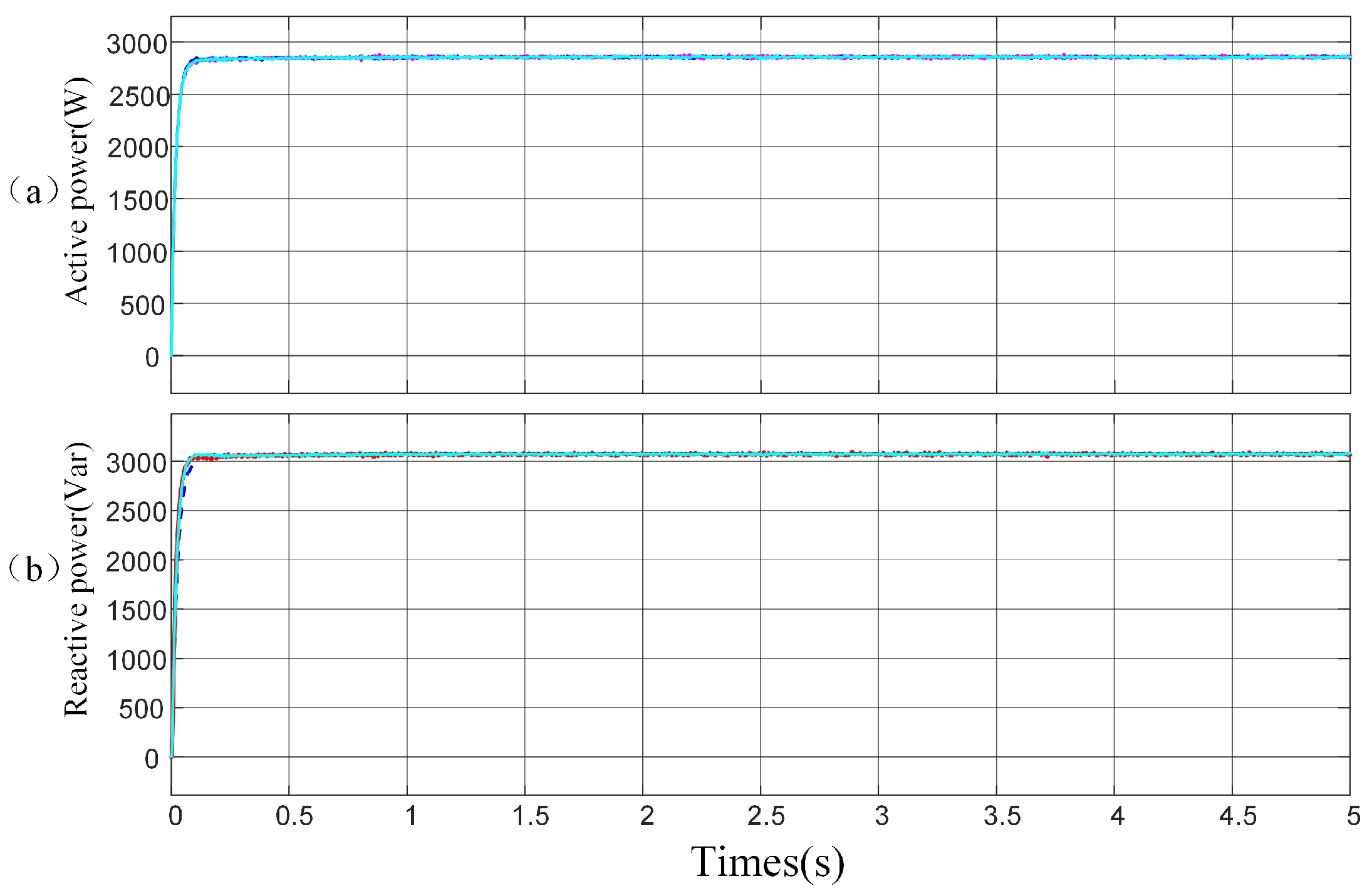
4.4. Power Sharing of Three Distributed Generators
5. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Meng, X.; Liu, J.; Liu, Z. A generalized droop control for grid supporting inverter based on comparison between traditional droop control and virtual synchronous generator control. IEEE Trans. Power Electron. 2019, 34, 5416–5438. [Google Scholar] [CrossRef]
- Tan, K.H.; Tseng, T.Y. Seamless switching and grid reconnection of microgrid using Petri recurrent wavelet fuzzy neural network. IEEE Trans. Power Electron. 2021, 36, 11847–11861. [Google Scholar] [CrossRef]
- Lin, F.-J.; Tan, K.-H.; Chang, C.-F.; Li, M.-Y.; Tseng, T.-Y. Development of intelligent controlled microgrid for power sharing and load shedding. IEEE Trans. Power Electron. 2022, 37, 7928–7940. [Google Scholar] [CrossRef]
- Liu, B.; Wu, T.; Liu, Z.; Liu, J. A small-AC-signal injection based decentralized secondary frequency control for droop-controlled islanded microgrids. IEEE Trans. Power Electron. 2020, 35, 11634–11651. [Google Scholar] [CrossRef]
- Tan, K.-H.; Li, M.-Y.; Weng, X.-Y. Droop controlled microgrid with DSTATCOM for reactive power compensation and power quality improvement. IEEE Access 2022, 10, 121602–121614. [Google Scholar] [CrossRef]
- Guerrero, J.M.; Chandorkar, M.; Lee, T.-L.; Loh, P.C. Advanced control architectures for intelligent microgrids—Part I: Decentralized and hierarchical control. IEEE Trans. Smart Grid 2016, 7, 695–705. [Google Scholar] [CrossRef]
- Fan, B.; Li, Q.; Wang, W.; Yao, G.; Ma, H.; Zeng, X.; Guerrero, J.M. A novel droop control strategy of reactive power sharing based on adaptive virtual impedance in microgrids. EEE Trans. Ind. Electron. 2022, 69, 11335–11347. [Google Scholar] [CrossRef]
- Shi, Z.; Li, J.; Nurdin, H.I.; Fletcher, J.E. Comparison of virtual oscillator and droop controlled islanded three-phase microgrids. IEEE Trans. Energy Convers. 2019, 34, 1769–1780. [Google Scholar] [CrossRef]
- Li, Z.; Chan, K.W.; Hu, J.; Guerrero, J.M. Adaptive droop control using adaptive virtual impedance for microgrids with variable PV outputs and load demands. IEEE Trans. Ind. Electron. 2021, 68, 9630–9640. [Google Scholar] [CrossRef]
- Alghamdi, B.; Cañizares, C.A. Frequency regulation in isolated microgrids through optimal droop gain and voltage control. IEEE Trans. Smart Grid 2021, 12, 988–998. [Google Scholar] [CrossRef]
- Zhang, W.; Wang, W.; Liu, H.; Xu, D. A disturbance rejection control strategy for droop-controlled inverter based on super-twisting algorithm. IEEE Access 2019, 7, 27037–27046. [Google Scholar] [CrossRef]
- Ling, Y.; Li, Y.; Xiang, J. Load support by droop-controlled distributed generations. IEEE Trans. Ind. Electron. 2021, 68, 8345–8355. [Google Scholar] [CrossRef]
- Ling, Y.; Li, Y.; Yang, Z.; Xiang, J. A dispatchable droop control method for distributed generators in islanded AC microgrids. IEEE Trans. Ind. Electron. 2021, 68, 8356–8366. [Google Scholar] [CrossRef]
- Han, H.; Liu, Y.; Sun, Y.; Su, M.; Guerrero, J.M. An improved droop control strategy for reactive power sharing in islanded microgrid. IEEE Trans. Power Electron. 2015, 30, 3133–3141. [Google Scholar] [CrossRef]
- Prakash, S.; Nougain, V.; Mishra, S. Adaptive droop-based control for active power sharing in autonomous microgrid for improved transient performance. IEEE J. Emerg. Sel. Top. Power Electron. 2020, 9, 3010–3018. [Google Scholar] [CrossRef]
- Minetti, M.; Rosini, A.; Denegri, G.B.; Bonfiglio, A.; Procopio, R. An advanced droop control strategy for reactive power assessment in islanded microgrids. IEEE Trans. Power Syst. 2021, 37, 3014–3025. [Google Scholar] [CrossRef]
- Xu, H.; Zhang, X.; Liu, F.; Shi, R.; Yu, C.; Cao, R. A reactive power sharing strategy of VSG based on virtual capacitor algorithm. IEEE Trans. Ind. Electron. 2017, 64, 7520–7531. [Google Scholar] [CrossRef]
- D’Arco, S.; Suul, J.A. Equivalence of virtual synchronous machines and frequency-droops for converter-based microgrids. IEEE Trans. Smart Grid 2014, 5, 394–395. [Google Scholar] [CrossRef]
- Liu, J.; Miura, Y.; Ise, T. Comparison of dynamic characteristics between virtual synchronous generator and droop control in inverter-based distributed generators. IEEE Trans. Power Electron. 2016, 31, 3600–3611. [Google Scholar] [CrossRef]
- Xiong, X.; Wu, C.; Hu, B.; Pan, D.; Blaabjerg, F. Transient damping method for improving the synchronization stability of virtual synchronous generators. IEEE Trans. Power Electron. 2021, 36, 7820–7831. [Google Scholar] [CrossRef]
- Wong, Y.C.C.; Lim, C.S.; Cruden, A.; Rotaru, M.D.; Ray, P.K. A consensus-based adaptive virtual output impedance control scheme for reactive power sharing in radial microgrids. IEEE Trans. Ind. Appl. 2020, 57, 784–794. [Google Scholar] [CrossRef]
- Chen, G.; Guo, Z. Distributed secondary and optimal active power sharing control for islanded microgrids with communication delays. IEEE Trans. Smart Grid 2017, 10, 2002–2014. [Google Scholar] [CrossRef]
- Bidram, A.; Davoudi, A.; Lewis, F.L.; Guerrero, J.M. Distributed cooperative secondary control of microgrids using feedback linearization. IEEE Trans. Power Syst. 2013, 28, 3462–3470. [Google Scholar] [CrossRef]
- Wu, X.; Shen, C.; Iravani, R. A distributed, cooperative frequency and voltage control for microgrids. IEEE Trans. Smart Grid 2016, 9, 2764–2776. [Google Scholar] [CrossRef]
- Schiffer, J.; Seel, T.; Raisch, J.; Sezi, T. Voltage stability and reactive power sharing in inverter-based microgrids with consensus-based distributed voltage control. IEEE Trans. Control Syst. Technol. 2015, 24, 96–109. [Google Scholar] [CrossRef]
- Guo, F.; Wen, C.; Mao, J.; Song, Y.-D. Distributed secondary voltage and frequency restoration control of droop-controlled inverter-based microgrids. IEEE Trans. Ind. Electron. 2014, 62, 4355–4364. [Google Scholar] [CrossRef]
- Simpson-Porco, J.W.; Shafiee, Q.; Dörfler, F.; Vasquez, J.C.; Guerrero, J.M.; Bullo, F. Secondary frequency and voltage control of islanded microgrids via distributed averaging. IEEE Trans. Ind. Electron. 2015, 62, 7025–7038. [Google Scholar] [CrossRef]
- Lin, F.-J.; Tan, K.-H.; Weng, X.-Y.; Li, Y.-R. An improved droop controlled microgrid using intelligent variable droop coefficient estimation. IEEE J. Emerg. Sel. Top. Power Electron. 2024, 12, 4117–4132. [Google Scholar] [CrossRef]









| Method | Complexity | Power Accuracy | Voltage Deviation | Calculation Requirements | Response Speed |
|---|---|---|---|---|---|
| Traditional droop control | Simple structure and easy to implement | Low accuracy of reactive power sharing | The voltage deviation is relatively large | Simple calculation | Fast response speed |
| Virtual inductor method | Need to calculate virtual inductor parameters | Slightly improve reactive power sharing accuracy | Relatively large voltage deviation | The virtual inductance needs to be calculated | The response speed is relatively fast |
| Adaptive virtual impedance method | The structure of the controller is complex | High accuracy of reactive power sharing | The voltage drop is severe | Dynamic calculation of virtual impedance values demands high computational capabilities | The system responds slowly |
| The method proposed in this paper | Simpler structure than adaptive virtual impedance | It can equally share reactive power | It can fully compensate voltage deviation | Dynamically adjusting the droop coefficient demands high-level calculations | It features a fast response speed, 80% faster than the adaptive virtual impedance method |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Wu, M.; Wu, C.; Wang, L.; Yuan, Y. Reactive Power Equalization Strategy for Islanded Microgrids Based on Improved Adaptive Droop Factor. Electronics 2025, 14, 1981. https://doi.org/10.3390/electronics14101981
Wu M, Wu C, Wang L, Yuan Y. Reactive Power Equalization Strategy for Islanded Microgrids Based on Improved Adaptive Droop Factor. Electronics. 2025; 14(10):1981. https://doi.org/10.3390/electronics14101981
Chicago/Turabian StyleWu, Minghu, Chenliang Wu, Lujun Wang, and Yichen Yuan. 2025. "Reactive Power Equalization Strategy for Islanded Microgrids Based on Improved Adaptive Droop Factor" Electronics 14, no. 10: 1981. https://doi.org/10.3390/electronics14101981
APA StyleWu, M., Wu, C., Wang, L., & Yuan, Y. (2025). Reactive Power Equalization Strategy for Islanded Microgrids Based on Improved Adaptive Droop Factor. Electronics, 14(10), 1981. https://doi.org/10.3390/electronics14101981






