Sequentially Coordinated and Cooperative Volt/Var Control of PV Inverters in Distribution Networks
Abstract
1. Introduction
- It does not require measuring/communicating the active power data. Therefore, a low bandwidth communication channel would suffice;
- It uses as little reactive power as possible, therefore reducing the chances for active power curtailment due to reactive power requirement, which increases the profitability of the PV system. Active power curtailment occurs if the inverter-rated apparent power does not allow both active power and reactive power flow;
- There is no need for droop settings, unlike the local droop controllers;
- The coordination between the inverters is satisfied at different load and PV operating conditions.
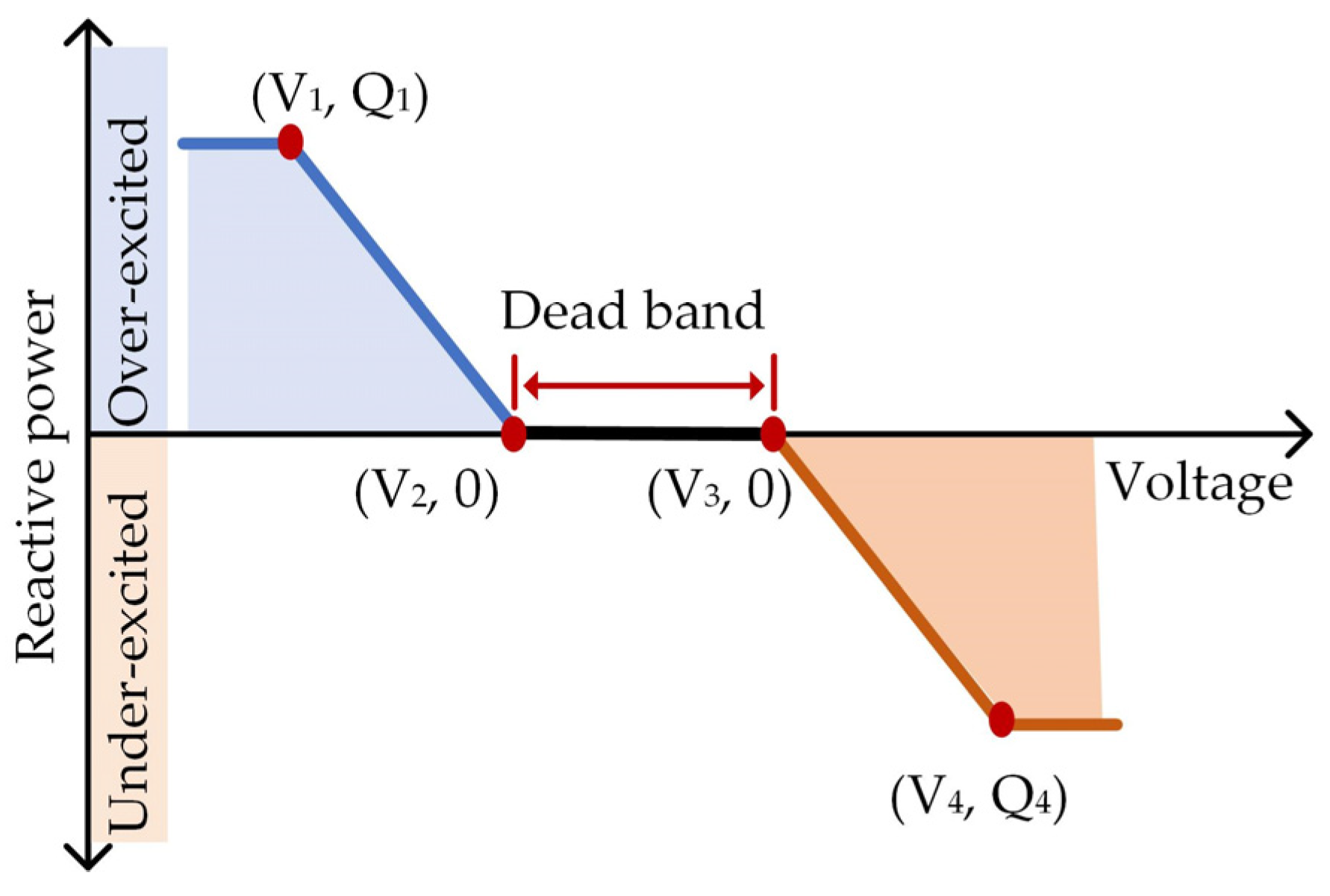
2. State of the Art Volt/Var Droop Control
3. Proposed Controller
- The voltage violation case is solved;
- The inverter is absorbing its maximum reactive power (Qmax).
- Unlike [20], the proposed controller does not require measuring/communicating power data. Therefore, a low bandwidth communication channel would suffice;
- It uses as little reactive power as possible, therefore reducing the chances for active power curtailment due to reactive power requirement, which increases the profitability of the PV system. Active power curtailment occurs if the inverter-rated apparent power does not allow both active power and reactive power flow;
- There is no need for droop settings, unlike the local droop controllers;
- The coordination between the inverters is satisfied at different operating conditions.
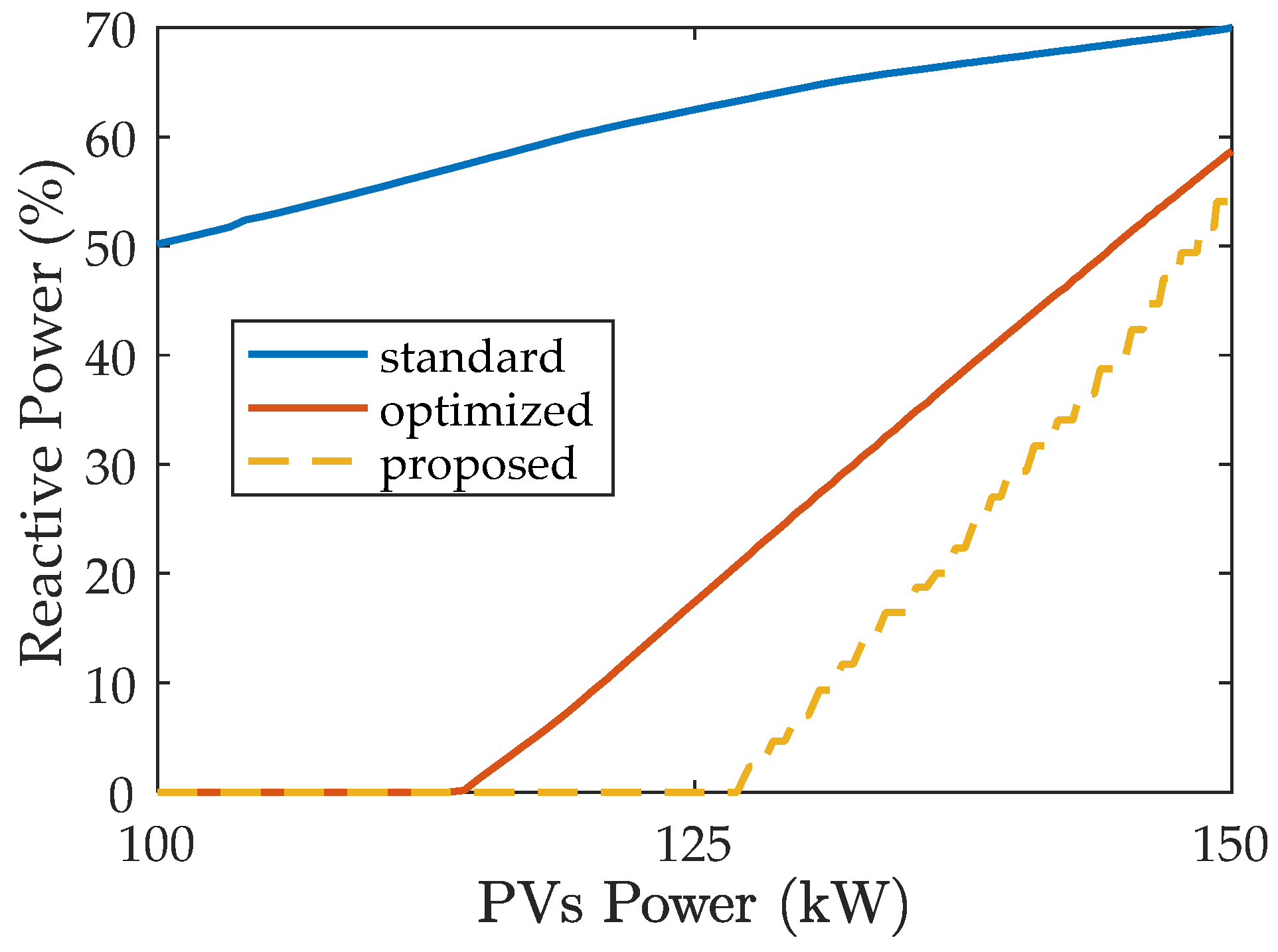
4. Comparative Study
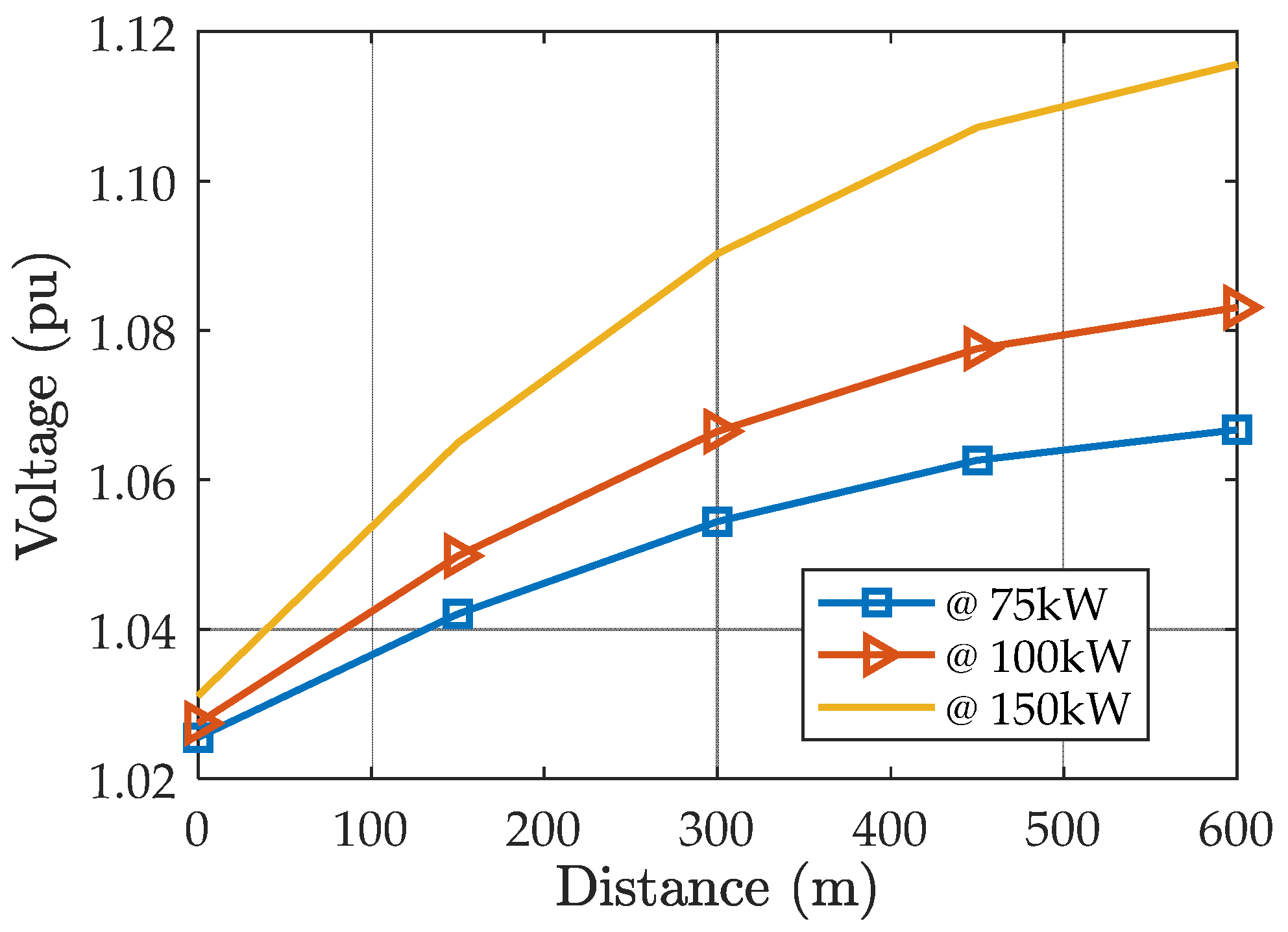
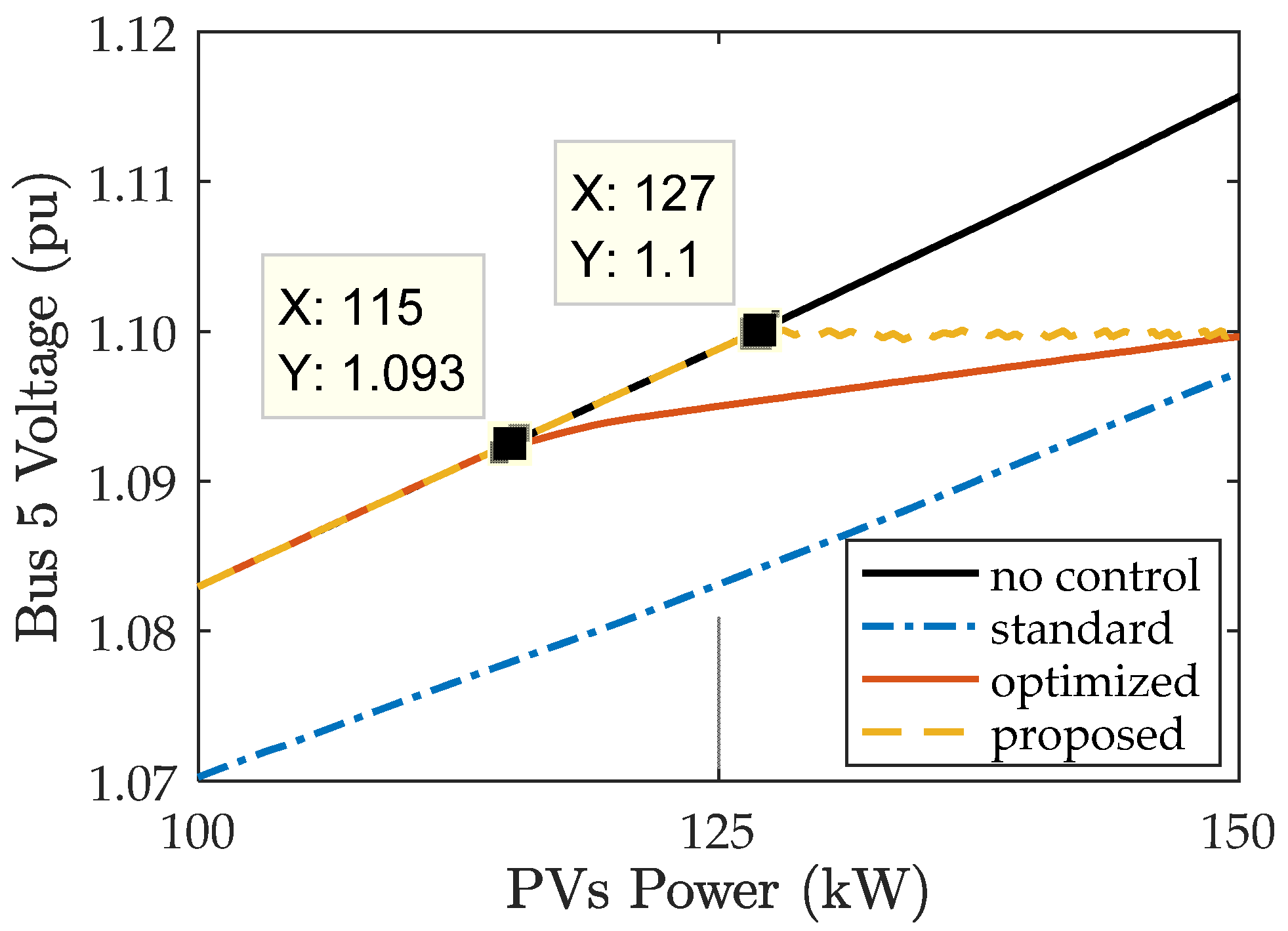
- The system was unloaded such that the generated PV active power flows to the main substation, which results in an increase in the system voltage;
- An upper voltage limit of 1.1 pu, following EN 50160 [28], was used;
- The total generated power was increased up to 150 kW.
- Reduce the active power loss associated to the reactive power flow;
- Avoid the need for large active power curtailment if the inverter cannot provide both active power and reactive power, which increases the profitability of the PV system.
5. Results and Discussion
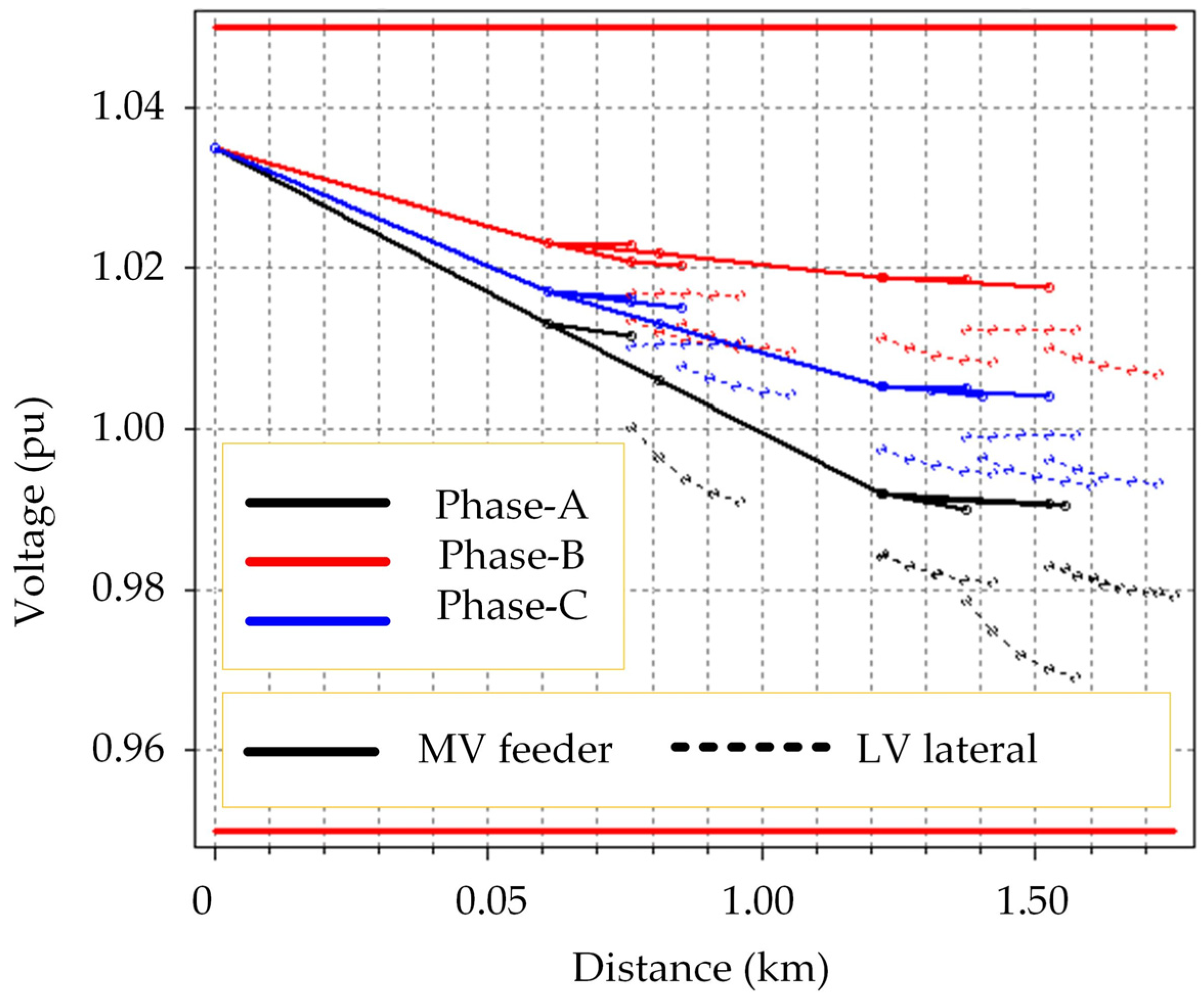
5.1. System Description
- Fixing the regulator tap position at the main substation;
- Disconnecting the capacitor banks at buses 675 and 611;
- Adding eleven low voltage laterals.
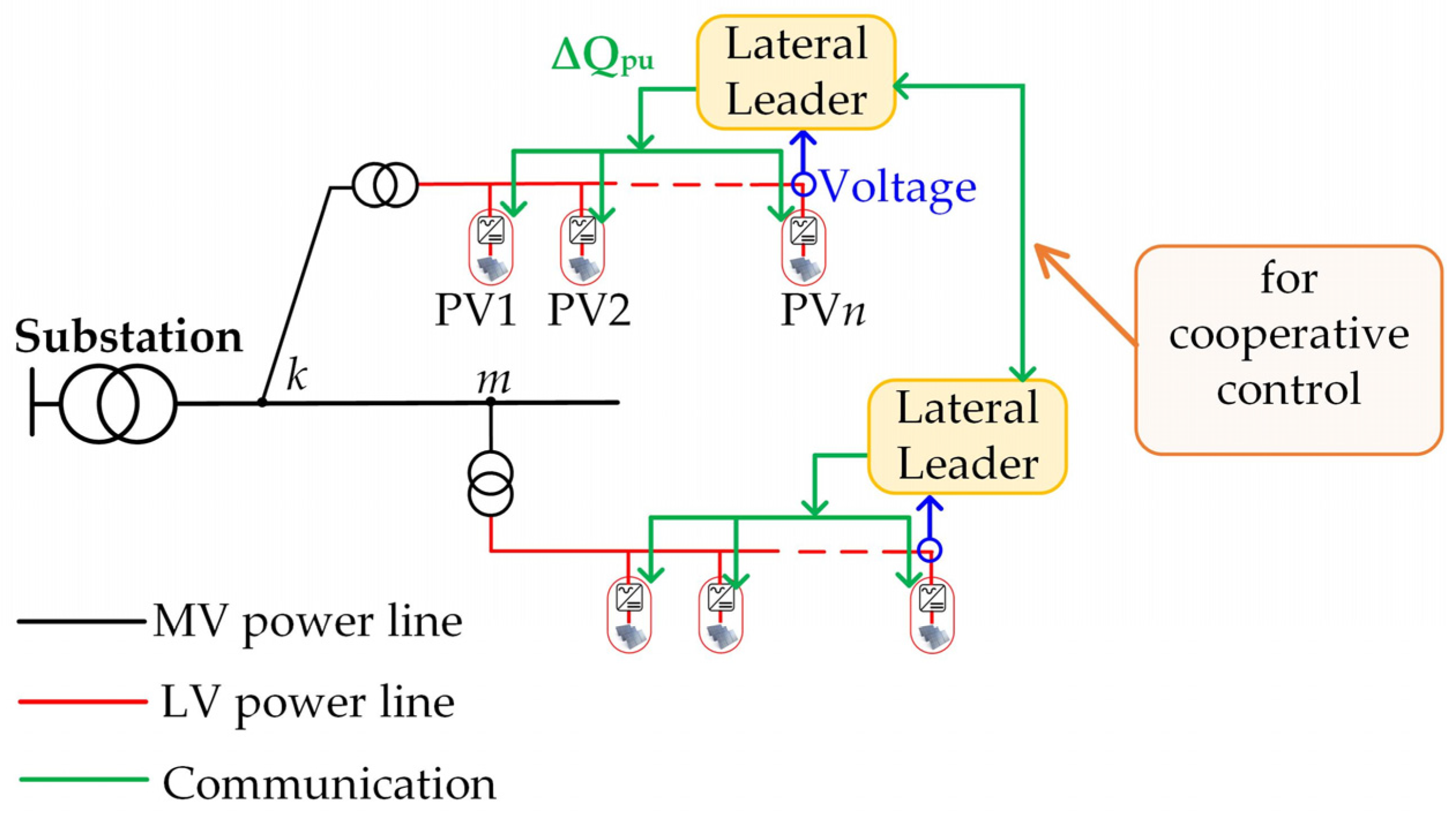
5.2. Voltage Sensitivity Analysis for Cooperative Control
- For once, all the PV systems were running at unity power factor;
- A single PV system on lateral 1 has been modified to absorb reactive power (under-excited mode), while the rest of the PV systems were operating at unity power factor;
- The previous step for different laterals instead of lateral 1 is repeated;
- Voltage at different leaders was recorded for the previous steps.
- As expected, a lateral self-effect is the maximum. This emphasizes the role of the individual lateral-based control part;
- From Table 4, absorbing Q by single-phase laterals (6 to 11) may have a negative impact on the three-phase laterals due to the unbalance caused by this single-phase reactive power flow. Therefore, using single-phase leaders to support three-phase leaders will be avoided;
- Different leaders may have the same impact on another leader. Looking at the row corresponding to lateral 4 in Table 3, the effect of laterals 2, 3, and 5 is the same on lateral 4. In this case, the leaders are ordered according to their electrical distance from the main substation (i.e., lower loss first);
- Single-phase leaders of the same phase (e.g., 6 and 7) have a larger impact on each other but may have a negative impact on the other phases due to system unbalance.
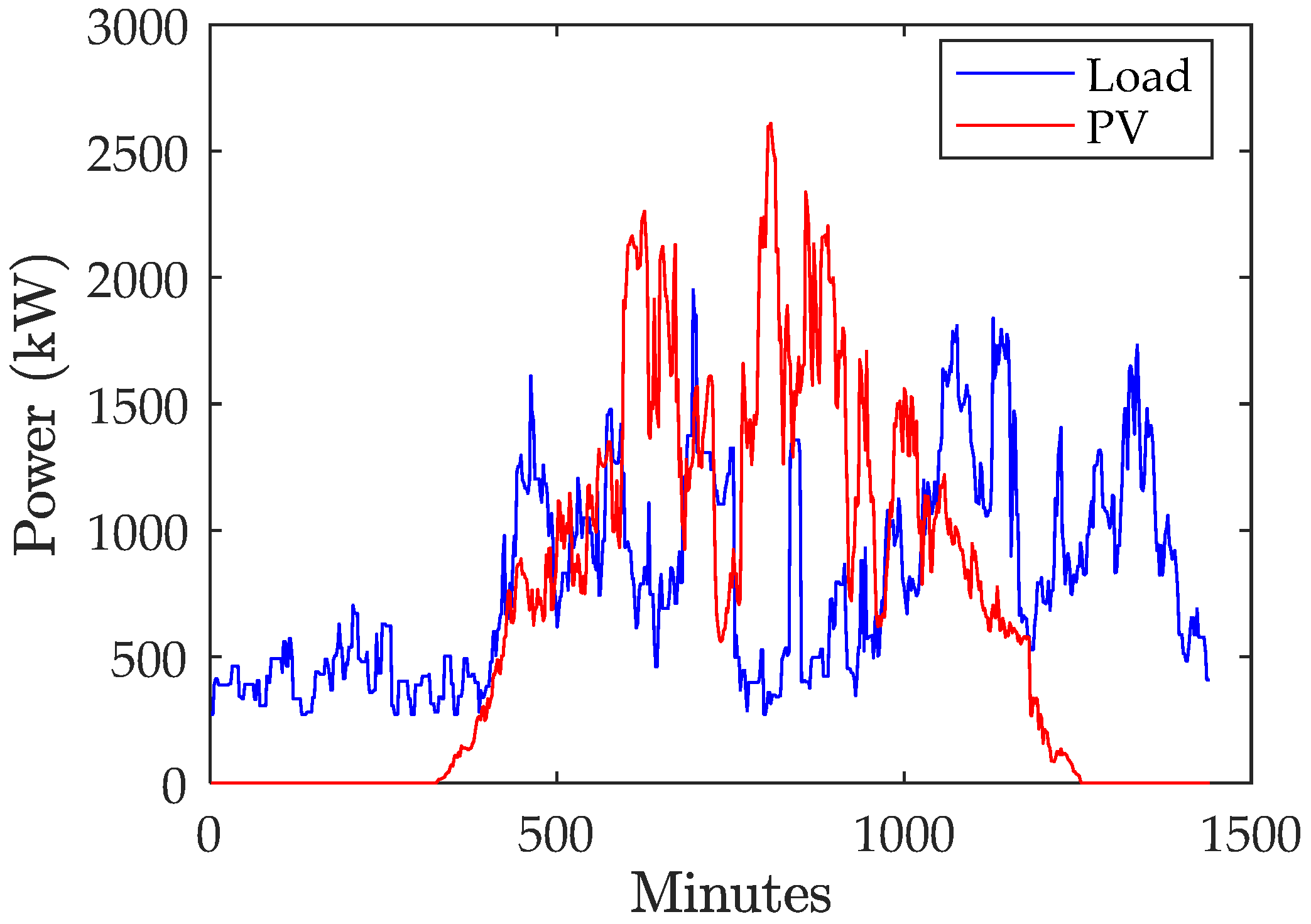
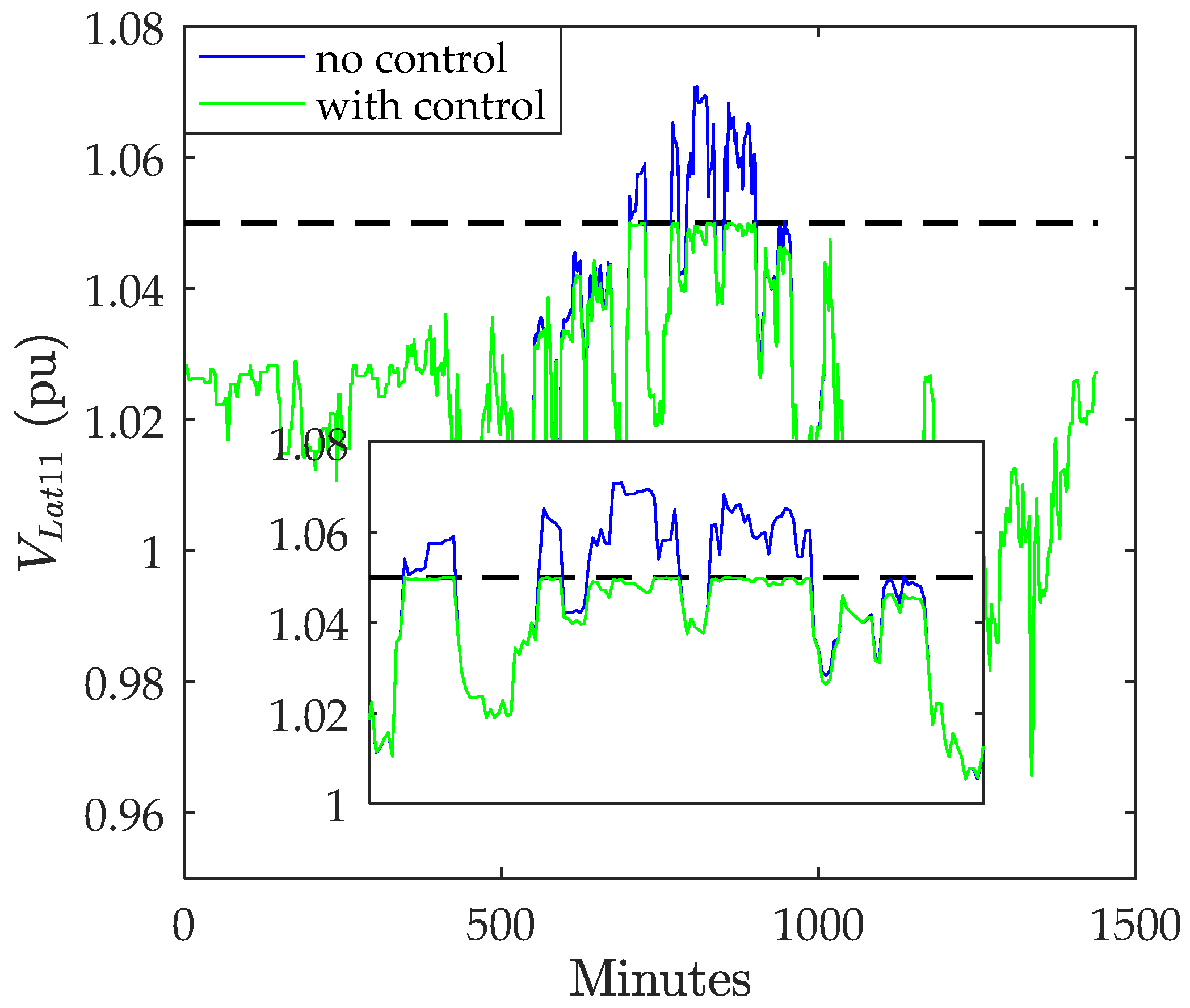
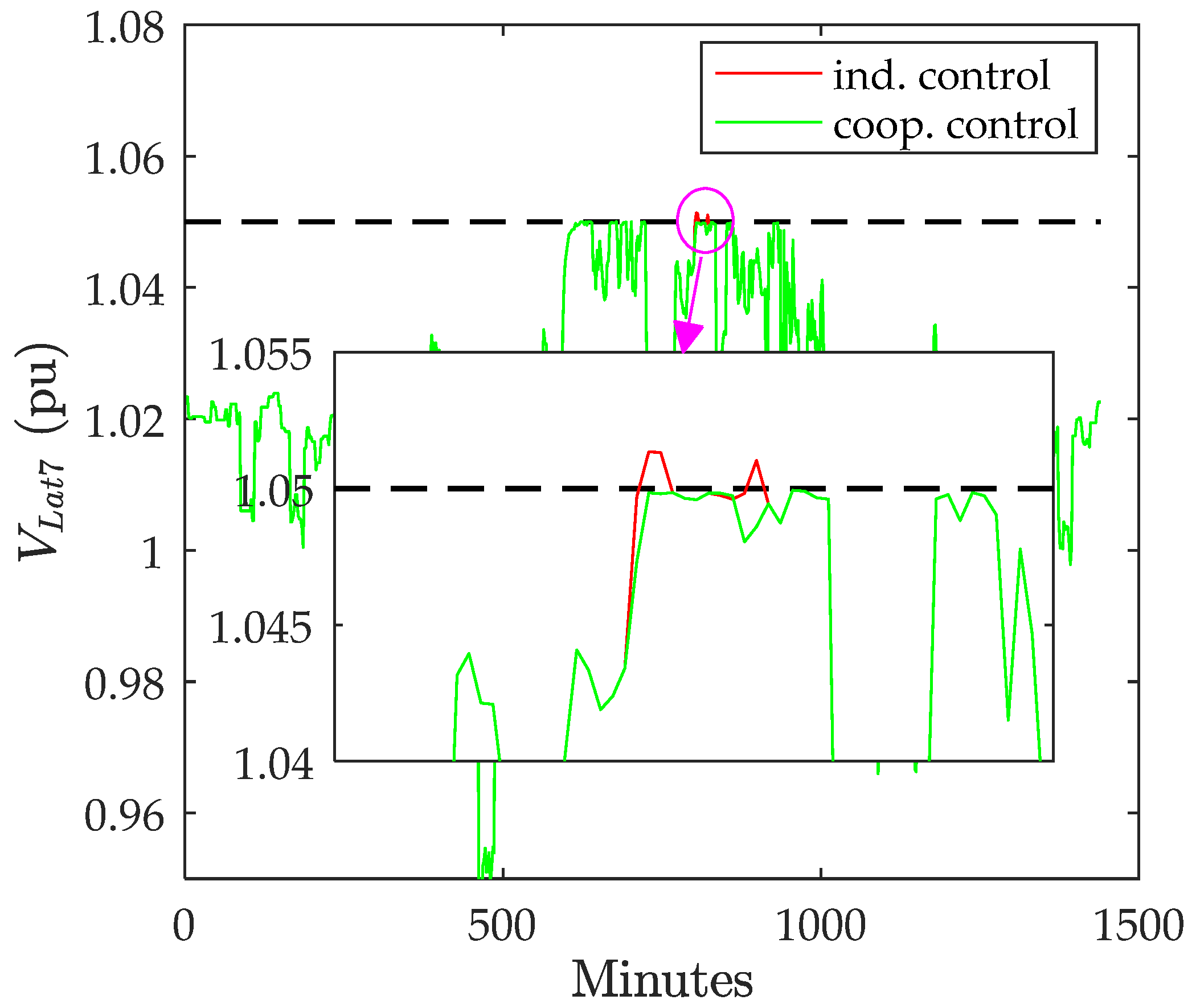
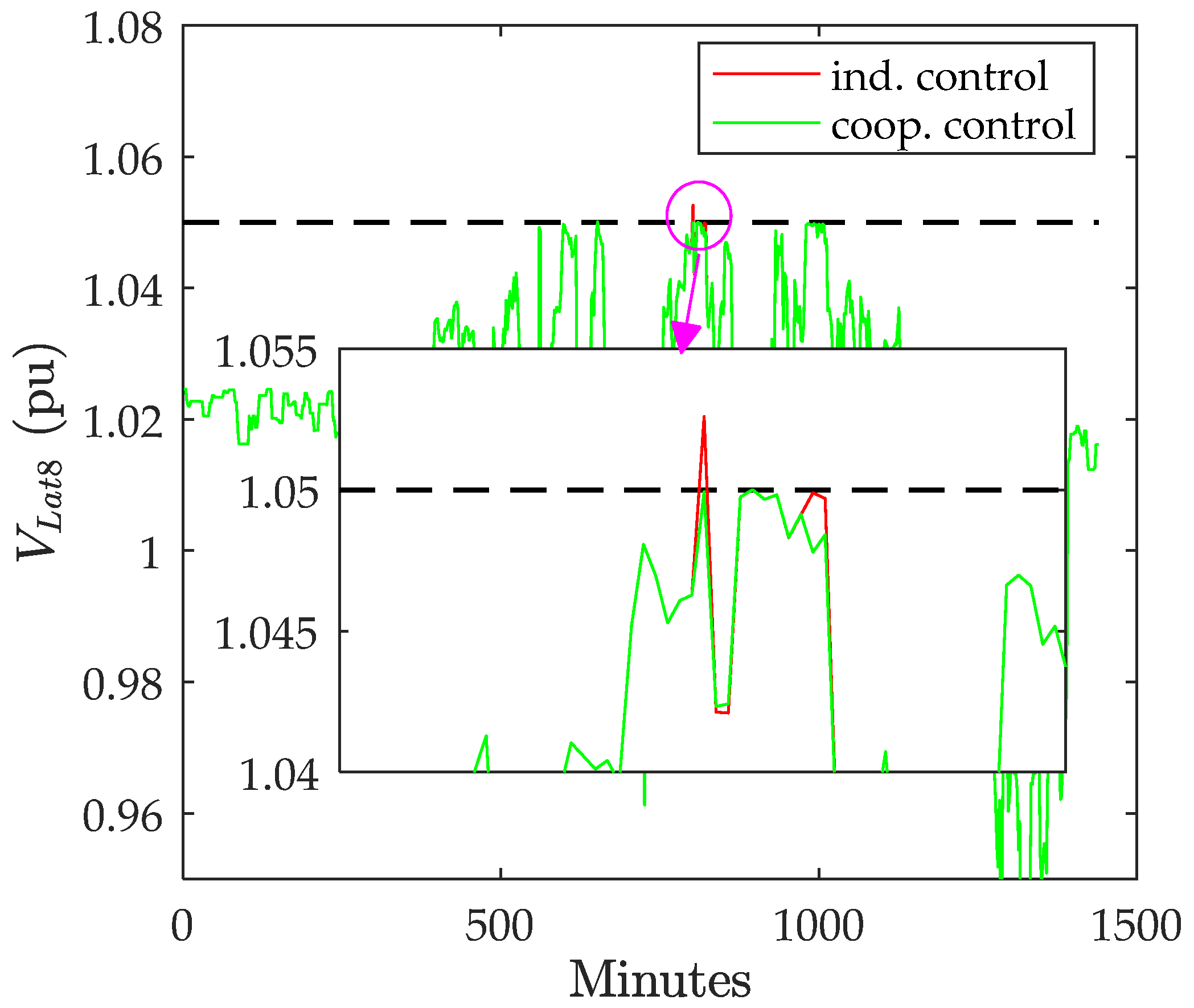
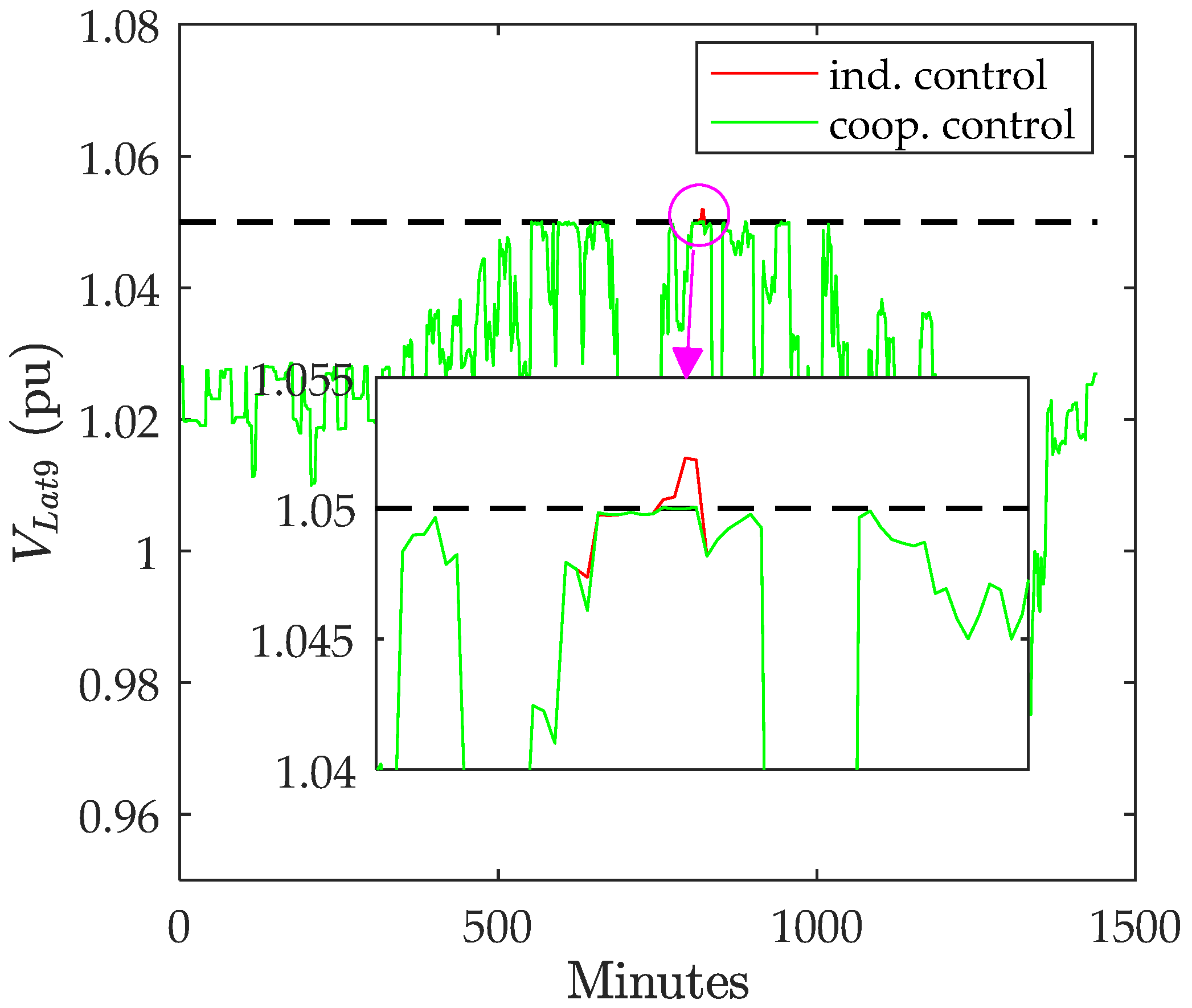
5.3. Time Series Daily Simulation
6. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
Appendix A
- All single-phase loads are rated at 30 kW;
- Laterals number 1, 2, and 3 have unbalanced loads for each loading point and are rated at (phase-A is 40 kW, phase-B is 30 kW, and phase-C is 30 kW);
- Laterals 4 and 5 are balanced and rated at 100 kW at each loading point.
| Element | Parameters |
|---|---|
| Single-phase transformer | 2.4/0.277 kV, 150 kVA, X = 2%, R = 1.1% |
| Three-phase transformer | 4.16/0.48 kV, 500 kVA, X = 2%, R = 1.1% |
| Single-phase cable section | 50 m, Z = 0.14 + j0.0357 Ω/km |
| Three-phase cable section | 50 m, Zs = 0.114 + j0.0359 Ω/km, Zm = 0.0228 + j0.0072 Ω/km |
References
- HM Government. Climate change Act 2008 (2050 Target Amendment) (No. SI 2019 No. 1056), UK Public General Acts. 2019. Available online: https://www.legislation.gov.uk/ukpga/2008/27/contents (accessed on 15 February 2023).
- Sambhi, S.; Sharma, H.; Bhadoria, V.; Kumar, P.; Chaurasia, R.; Fotis, G.; Vita, V. Technical and economic analysis of solar PV/diesel generator smart hybrid power plant using different battery storage technologies for SRM IST, Delhi-NCR Campus. Sustainability 2023, 15, 3666. [Google Scholar] [CrossRef]
- SolarPower Europe. Global Market Outlook for Solar Power 2022–2026. Available online: https://www.solarpowereurope.org/insights/market-outlooks/global-market-outlook-for-solar-power-2022 (accessed on 15 December 2022).
- Ismael, S.M.; Abdel Aleem, S.H.E.; Abdelaziz, A.Y.; Zobaa, A.F. State-of-the-art of hosting capacity in modern power systems with distributed generation. Renew. Energy 2019, 130, 1002–1020. [Google Scholar] [CrossRef]
- Uzum, B.; Onen, A.; Hasanien, H.M.; Muyeen, S.M. Rooftop solar PV penetration impacts on distribution network and further growth factors—A comprehensive review. Electronics 2021, 10, 55. [Google Scholar] [CrossRef]
- Vita, V.; Alimardan, T.; Ekonomou, L. The impact of distributed generation in the distribution networks’ voltage profile and energy losses. In Proceedings of the 2015 IEEE European Modelling Symposium (EMS), Madrid, Spain, 6–8 October 2015; pp. 260–265. [Google Scholar]
- Shayani, R.A.; de Oliveira, M.A.G. Photovoltaic generation penetration limits in radial distribution systems. IEEE Trans. Power Syst. 2011, 26, 1625–1631. [Google Scholar] [CrossRef]
- ANSI C84.1-2020; American National Standard for Electric Power Systems and Equipment Voltage Ratings (60 Hz). American National Standards Institute (ANSI): Washington, DC, USA, 2016.
- Sun, H.; Guo, Q.; Qi, J.; Ajjarapu, V.; Bravo, R.; Chow, J.; Li, Z.; Moghe, R.; Nasr-Azadani, E.; Tamrakar, U.; et al. Review of challenges and research opportunities for voltage control in smart grids. IEEE Trans. Power Syst. 2019, 34, 2790–2801. [Google Scholar] [CrossRef]
- Aboshady, F.M.; Pisica, I.; Ceylan, O.; Taylor, G.A.; Zobaa, A.F.; Ozdemir, A. Sequential volt/var controller for PV smart inverters in distribution systems. In Proceedings of the 2022 57th International Universities Power Engineering Conference (UPEC), Istanbul, Turkey, 30 August–2 September 2022; pp. 1–6. [Google Scholar]
- IEEE Std 1547-2018 (Revision of IEEE Std 1547-2003); IEEE Standards Coordinating Committee 21. IEEE Standard for Interconnection and Interoperability of Distributed Energy Resources with Associated Electric Power Systems Interfaces. IEEE: Piscataway, NZ, USA, 2018; pp. 1–138. [CrossRef]
- Almeida, D.; Pasupuleti, J.; Raveendran, S.K.; Basir Khan, M.R. Performance evaluation of solar PV inverter controls for overvoltage mitigation in MV distribution networks. Electronics 2021, 10, 1456. [Google Scholar] [CrossRef]
- Ghosh, S.; Rahman, S.; Pipattanasomporn, M. Distribution voltage regulation through active power curtailment with PV inverters and solar generation forecasts. IEEE Trans. Sustain. Energy 2017, 8, 13–22. [Google Scholar] [CrossRef]
- Rylander, M.; Li, H.; Smith, J.; Sunderman, W. Default volt-var inverter settings to improve distribution system performance. In Proceedings of the 2016 IEEE Power and Energy Society General Meeting (PESGM), Boston, MA, USA, 17–21 July 2016. [Google Scholar]
- Procopiou, A.T.; Ochoa, L.F. On the limitations of volt-var control in PV-rich residential LV networks: A UK case study. In Proceedings of the 2019 IEEE Milan PowerTech, Milan, Italy, 23–27 June 2019; pp. 1–6. [Google Scholar]
- Samadi, A.; Shayesteh, E.; Eriksson, R.; Rawn, B.; Söder, L. Multi-objective coordinated droop-based voltage regulation in distribution grids with PV systems. Renew. Energy 2014, 71, 315–323. [Google Scholar] [CrossRef]
- Samadi, A.; Eriksson, R.; Söder, L.; Rawn, B.G.; Boemer, J.C. Coordinated active power-dependent voltage regulation in distribution grids with PV systems. IEEE Trans. Power Deliv. 2014, 29, 1454–1464. [Google Scholar] [CrossRef]
- Kim, S.-B.; Song, S.-H. A hybrid reactive power control method of distributed generation to mitigate voltage rise in low-voltage grid. Energies 2020, 13, 2078. [Google Scholar] [CrossRef]
- Momeneh, A.; Castilla, M.; Miret, J.; Martí, P.; Velasco, M. Comparative study of reactive power control methods for photovoltaic inverters in low-voltage grids. IET Renew. Power Gener. 2016, 10, 310–318. [Google Scholar] [CrossRef]
- Ceylan, O.; Paudyal, S.; Pisica, I. Nodal sensitivity-based smart inverter control for voltage regulation in distribution feeder. IEEE J. Photovolt. 2021, 11, 1105–1113. [Google Scholar] [CrossRef]
- Wang, Y.; Tan, K.T.; Peng, X.Y.; So, P.L. Coordinated control of distributed energy-storage systems for voltage regulation in distribution networks. IEEE Trans. Power Deliv. 2016, 31, 1132–1141. [Google Scholar] [CrossRef]
- Mahdavi, S.; Panamtash, H.; Dimitrovski, A.; Zhou, Q. Predictive coordinated and cooperative voltage control for systems with high penetration of PV. IEEE Trans. Ind. Appl. 2021, 57, 2212–2222. [Google Scholar] [CrossRef]
- Zhang, C.; Xu, Y. Hierarchically-coordinated voltage/VAR control of distribution networks using PV inverters. IEEE Trans. Smart Grid 2020, 11, 2942–2953. [Google Scholar] [CrossRef]
- Xu, S.; Xue, Y.; Chang, L. Review of power system support functions for inverter-based distributed energy resources- standards, control algorithms, and trends. IEEE Open J. Power Electron. 2021, 2, 88–105. [Google Scholar] [CrossRef]
- Smith, J.W.; Sunderman, W.; Dugan, R.; Seal, B. Smart inverter volt/var control functions for high penetration of PV on distribution systems. In Proceedings of the 2011 IEEE/PES Power Systems Conference and Exposition, Phoenix, AZ, USA, 20–23 March 2011; pp. 1–6. [Google Scholar]
- Conti, S.; Gerco, A.M.; Raiti, S. Voltage sensitivity analysis in MV distribution networks. In Proceedings of the 6th WSEAS/IASME International Conference on Electric Power Systems, High Voltages, Electric Machines, Tenerife, Spain, 16–18 December 2006. [Google Scholar]
- EPRI. Open Distribution System Simulator, Sourceforge.Net. Available online: http://sourceforge.net/projects/electricdss/files/ (accessed on 1 December 2022).
- European Standard No. EN 50160; Power Quality Application Guide, Voltage Disturbances. European Committee for Electrotechnical Standardization (CENELEC): Brussels, Belgium, 2004.
- Test Feeder Working Group of the Distribution System Analysis Subcommittee. IEEE PES Test Feeder. Available online: https://cmte.ieee.org/pes-testfeeders/resources/ (accessed on 1 December 2022).
- Richardson, I.; Thomson, M. Integrated Domestic Electricity Demand and PV Micro-Generation Model, Loughborough University. Available online: https://hdl.handle.net/2134/7773 (accessed on 1 December 2022).








| Element | Parameters |
|---|---|
| Medium voltage grid | 20 kV, 100 MVA, X/R = 1 |
| Transformer | 20/0.4 kV, 250 kVA, Z = 4% |
| Cable impedance | 0.346 + j0.0754 Ω/km |
| Parameter | PV1 | PV2 | PV3 | PV4 | PV5 |
|---|---|---|---|---|---|
| V3 | 1.023 | 1.054 | 1.073 | 1.087 | 1.094 |
| (V4, Q4) | (1.026, 0) | (1.056, −0.207) | (1.078, −0.484) | (1.093, −0.484) | (1.1, −0.484) |
| Lateral Absorbing Q (j) | ||||||||||||
|---|---|---|---|---|---|---|---|---|---|---|---|---|
| 1 | 2 | 3 | 4 | 5 | 6 | 7 | 8 | 9 | 10 | 11 | ||
| %∆V on lateral (i) | 1 | 0.2619 | 0.0582 | 0.0582 | 0.0582 | 0.0582 | 0.0873 | 0.0873 | 0.0970 | 0.0970 | 0.0970 | 0.0970 |
| 2 | 0.0582 | 0.3104 | 0.1261 | 0.1164 | 0.1164 | 0.1843 | 0.1843 | 0.1843 | 0.0970 | 0.0970 | 0.0970 | |
| 3 | 0.0582 | 0.1261 | 0.3104 | 0.1164 | 0.1164 | 0.1843 | 0.1843 | 0.1843 | 0.0970 | 0.0970 | 0.0970 | |
| 4 | 0.0582 | 0.1164 | 0.1164 | 0.3298 | 0.1164 | 0.1843 | 0.1843 | 0.1843 | 0.0970 | 0.0970 | 0.0970 | |
| 5 | 0.0582 | 0.1164 | 0.1164 | 0.1164 | 0.3007 | 0.1843 | 0.1843 | 0.1843 | 0.0970 | 0.0970 | 0.0970 | |
| 6 | 0.0485 | 0.0970 | 0.0970 | 0.0970 | 0.0970 | 0.3976 | 0.1842 | −0.0097 | −0.0388 | −0.0097 | −0.0388 | |
| 7 | 0.0485 | 0.0970 | 0.0970 | 0.0970 | 0.0970 | 0.1842 | 0.4363 | −0.0194 | −0.0388 | −0.0097 | −0.0388 | |
| 8 | 0.0582 | 0.1164 | 0.1164 | 0.1164 | 0.1164 | −0.0582 | −0.0679 | 0.4363 | 0.0000 | 0.0970 | 0.0000 | |
| 9 | 0.0582 | 0.0582 | 0.0582 | 0.0582 | 0.0582 | −0.0097 | −0.0097 | −0.0291 | 0.3588 | −0.0388 | 0.1261 | |
| 10 | 0.0582 | 0.0582 | 0.0582 | 0.0582 | 0.0582 | −0.0291 | −0.0291 | 0.0970 | −0.0097 | 0.3588 | −0.0097 | |
| 11 | 0.0582 | 0.0582 | 0.0582 | 0.0582 | 0.0582 | −0.0097 | −0.0097 | −0.0291 | 0.1261 | −0.0388 | 0.3394 | |
| Lateral Absorbing Q (j) | ||||||||||||
|---|---|---|---|---|---|---|---|---|---|---|---|---|
| 1 | 2 | 3 | 4 | 5 | 6 | 7 | 8 | 9 | 10 | 11 | ||
| %∆V on lateral (i) | 1 | 0.2522 | 0.0485 | 0.0485 | 0.0485 | 0.0485 | −0.0291 | −0.0291 | −0.0291 | −0.0388 | −0.0291 | −0.0388 |
| 2 | 0.0485 | 0.3007 | 0.1067 | 0.0970 | 0.0970 | −0.0582 | −0.0582 | −0.0582 | −0.0388 | −0.0291 | −0.0388 | |
| 3 | 0.0485 | 0.1067 | 0.3007 | 0.0970 | 0.0970 | −0.0679 | −0.0679 | −0.0582 | −0.0388 | −0.0291 | −0.0388 | |
| 4 | 0.0485 | 0.0970 | 0.0970 | 0.3104 | 0.0970 | −0.0582 | −0.0582 | −0.0582 | −0.0388 | −0.0291 | −0.0388 | |
| 5 | 0.0485 | 0.0970 | 0.0970 | 0.0970 | 0.2910 | −0.0582 | −0.0582 | −0.0582 | −0.0388 | −0.0291 | −0.0388 | |
| Leader That Needs Support | ||||||||||||
|---|---|---|---|---|---|---|---|---|---|---|---|---|
| 1 | 2 | 3 | 4 | 5 | 6 | 7 | 8 | 9 | 10 | 11 | ||
| Supporters | 1st | 5 | 3 | 2 | 5 | 2 | 7 | 6 | 5 | 11 | 8 | 9 |
| 2nd | 2 | 5 | 5 | 2 | 3 | 5 | 5 | 2 | 1 | 1 | 1 | |
| 3rd | 3 | 4 | 4 | 3 | 4 | 2 | 2 | 3 | 5 | 5 | 5 | |
| 4th | 4 | 1 | 1 | 1 | 1 | 3 | 3 | 4 | 2 or 3 | 2 or 3 | 2 or 3 | |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Aboshady, F.; Ceylan, O.; Zobaa, A.F.; Ozdemir, A.; Taylor, G.; Pisica, I. Sequentially Coordinated and Cooperative Volt/Var Control of PV Inverters in Distribution Networks. Electronics 2023, 12, 1765. https://doi.org/10.3390/electronics12081765
Aboshady F, Ceylan O, Zobaa AF, Ozdemir A, Taylor G, Pisica I. Sequentially Coordinated and Cooperative Volt/Var Control of PV Inverters in Distribution Networks. Electronics. 2023; 12(8):1765. https://doi.org/10.3390/electronics12081765
Chicago/Turabian StyleAboshady, Fathy, Oguzhan Ceylan, Ahmed F. Zobaa, Aydogan Ozdemir, Gareth Taylor, and Ioana Pisica. 2023. "Sequentially Coordinated and Cooperative Volt/Var Control of PV Inverters in Distribution Networks" Electronics 12, no. 8: 1765. https://doi.org/10.3390/electronics12081765
APA StyleAboshady, F., Ceylan, O., Zobaa, A. F., Ozdemir, A., Taylor, G., & Pisica, I. (2023). Sequentially Coordinated and Cooperative Volt/Var Control of PV Inverters in Distribution Networks. Electronics, 12(8), 1765. https://doi.org/10.3390/electronics12081765









