Electromagnetic Effective-Degree-of-Freedom Limit of a MIMO System in 2-D Inhomogeneous Environment
Abstract
1. Introduction
2. Methodology
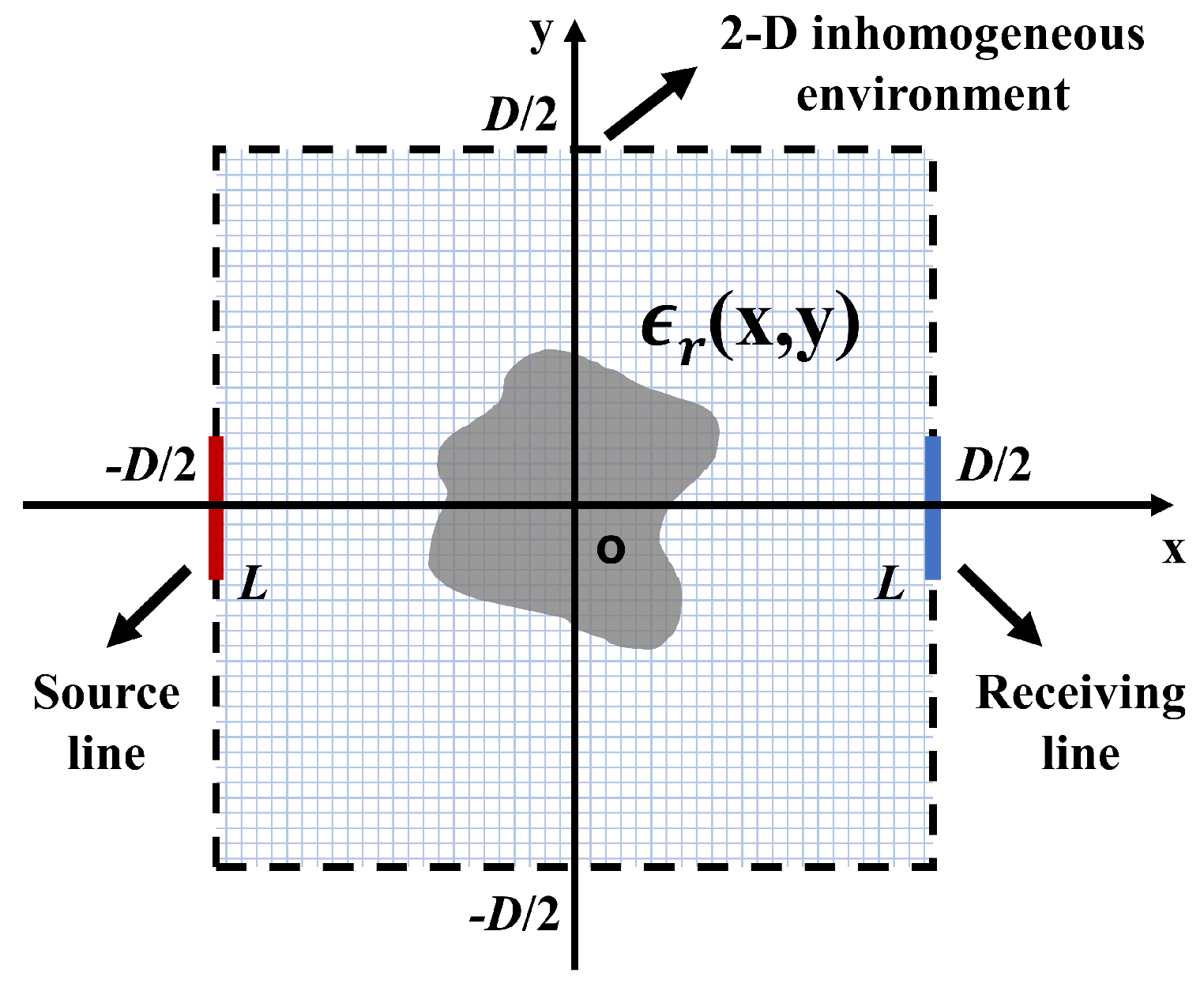
2.1. EM Model for Analyzing EDOF Limit
2.2. Numerical Method for Inhomogeneous Green’s Function
3. Numerical Examples
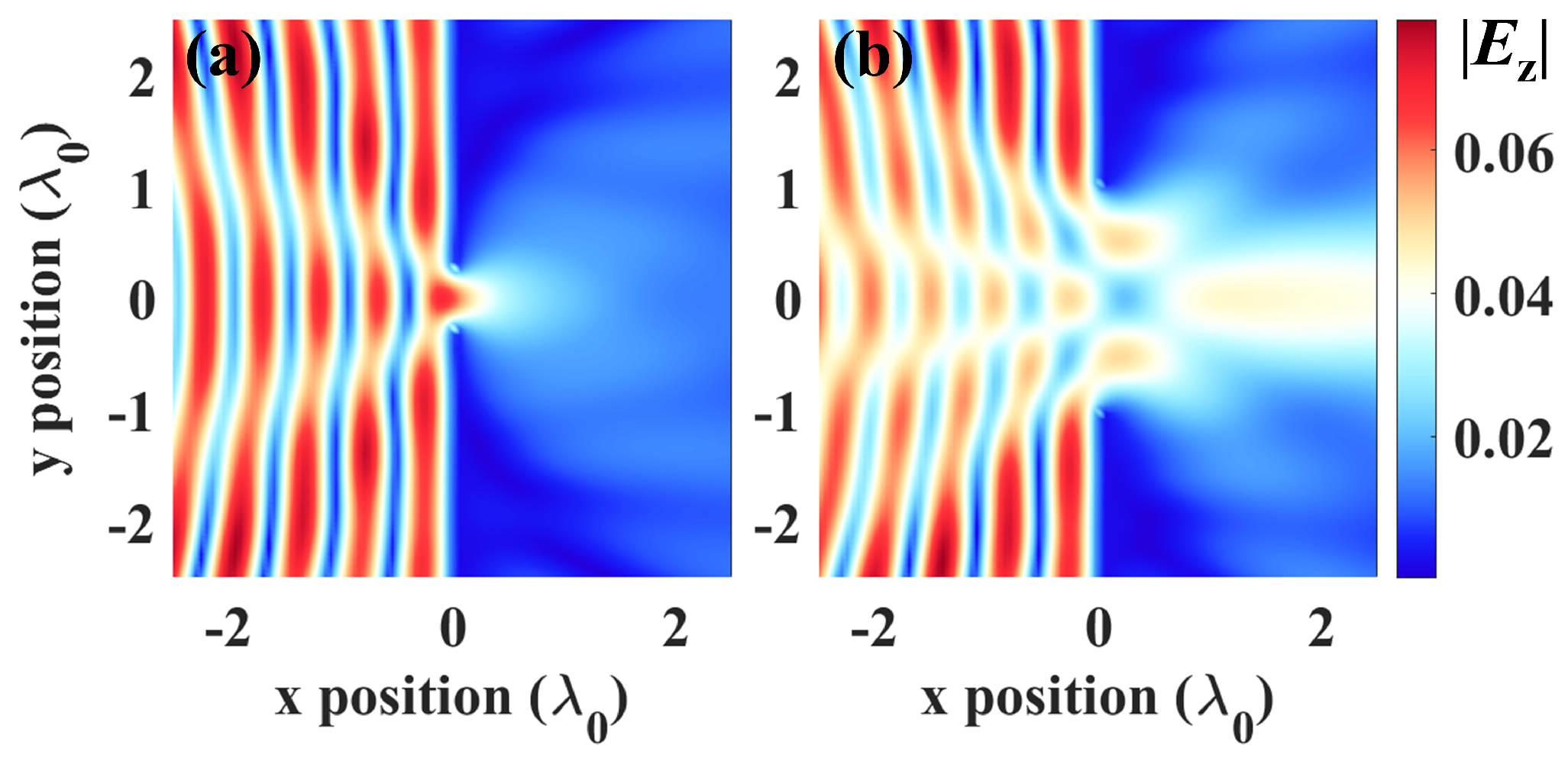
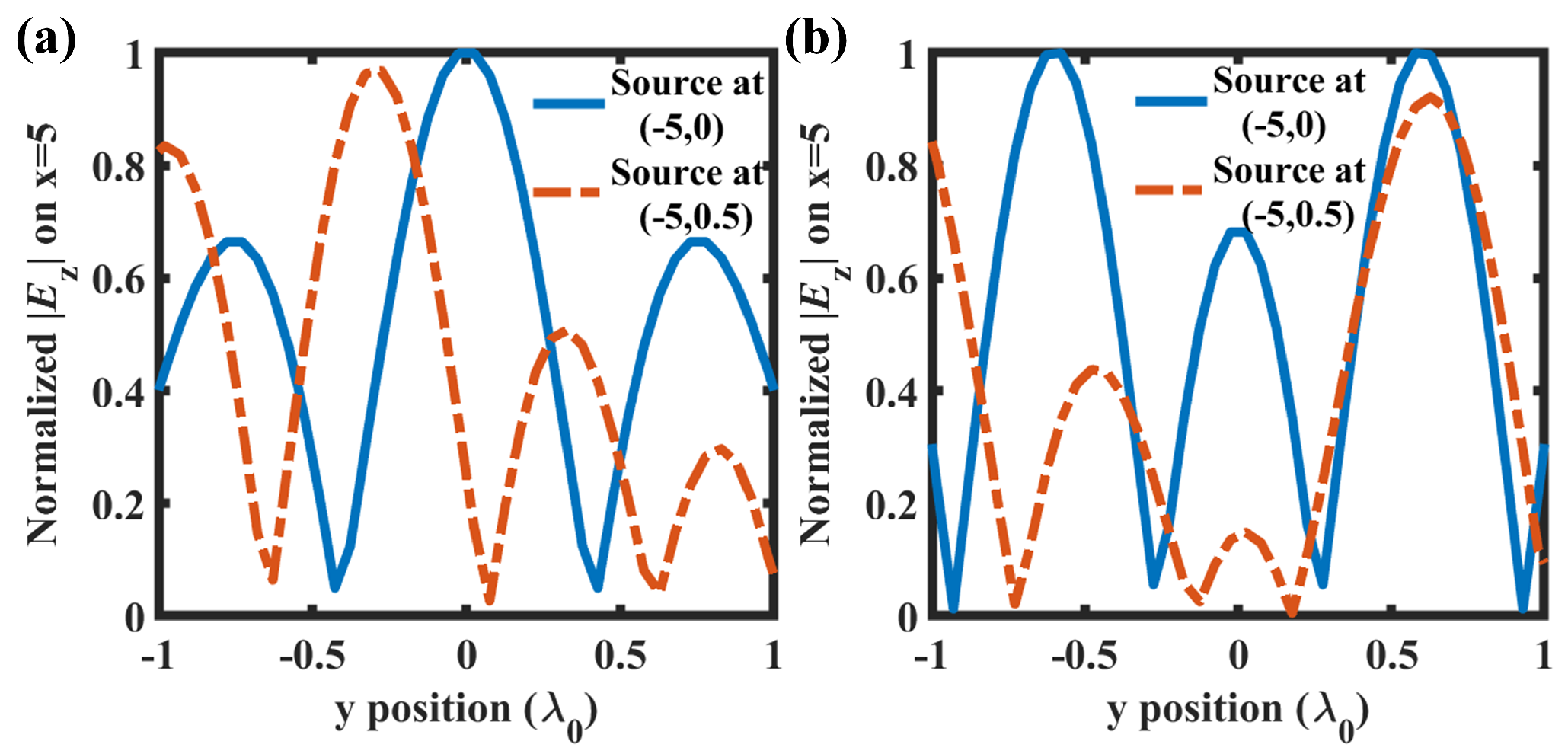
3.1. Key-Hole Scenario
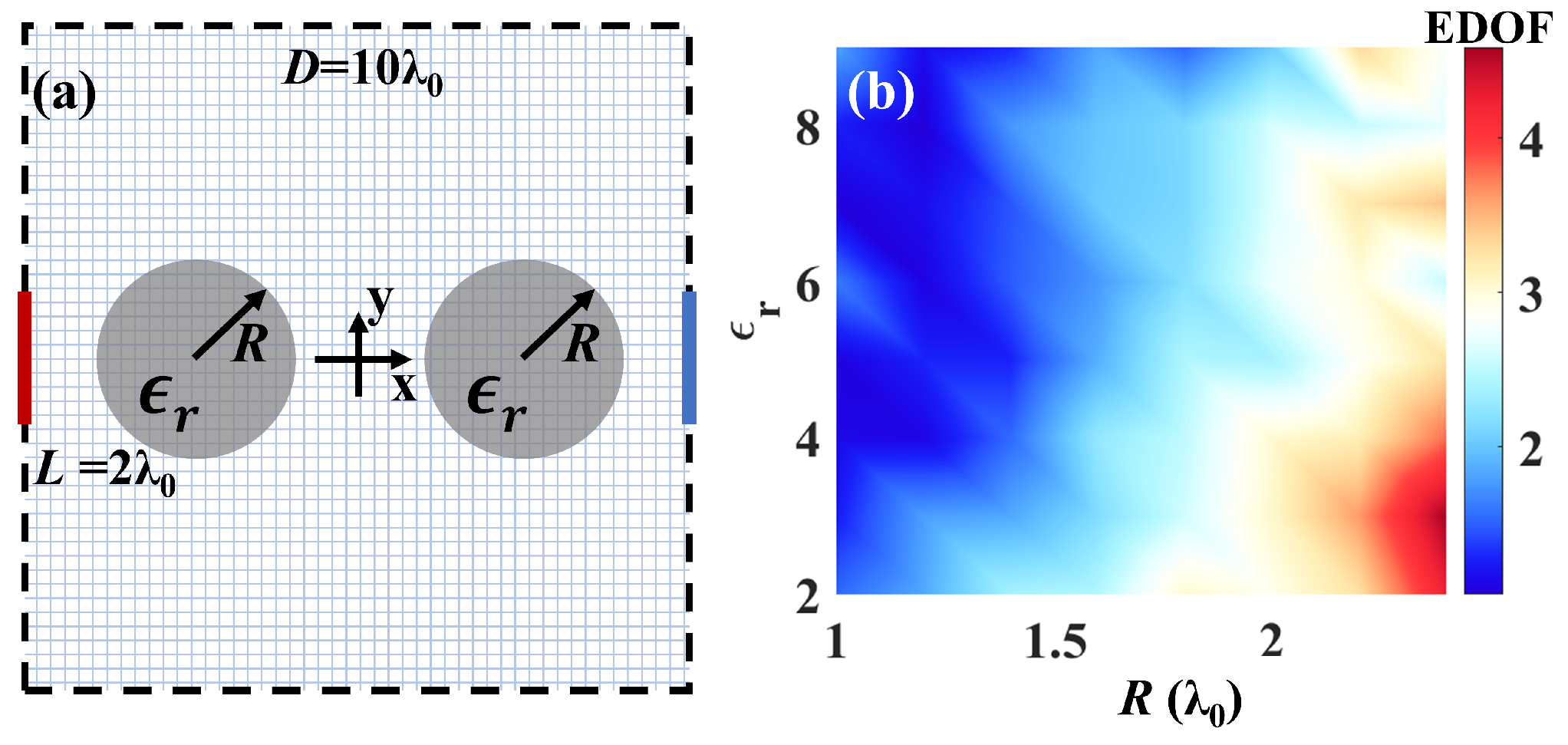
3.2. Cylindrical Scatterers
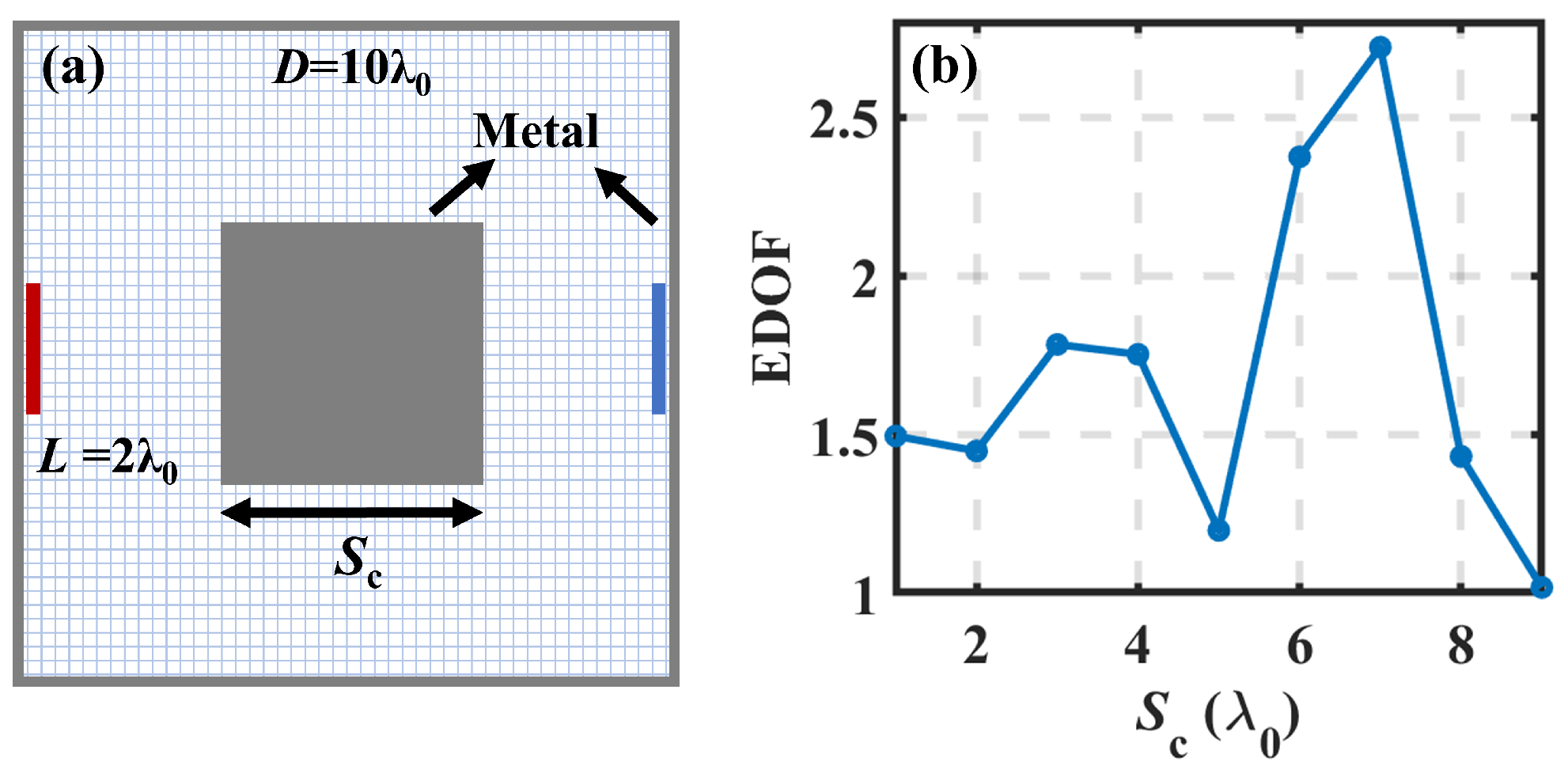
3.3. Cavity Structure
4. Relation between MIMO Performance and Scattering Characteristics
5. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Shannon, C.E. A mathematical theory of communication. Bell Syst. Tech. J. 1948, 27, 379–423. [Google Scholar] [CrossRef]
- Telatar, E. Capacity of multi-antenna Gaussian channels. Eur. Trans. Telecomm. 1999, 10, 585–595. [Google Scholar] [CrossRef]
- Larsson, E.G.; Edfors, O.; Tufvesson, F.; Marzetta, T.L. Massive MIMO for next generation wireless systems. IEEE Commun. Mag. 2014, 52, 186–195. [Google Scholar] [CrossRef]
- Tang, W.; Dai, J.Y.; Chen, M.Z.; Wong, K.K.; Li, X.; Zhao, X.; Jin, S.; Cheng, Q.; Cui, T.J. MIMO Transmission Through Reconfigurable Intelligent Surface: System Design, Analysis, and Implementation. IEEE J. Sel. Areas Commun. 2020, 38, 2683–2699. [Google Scholar] [CrossRef]
- Huang, C.; Zappone, A.; Alexandropoulos, G.C.; Debbah, M.; Yuen, C. Reconfigurable Intelligent Surfaces for Energy Efficiency in Wireless Communication. IEEE Trans. Wirel. Commun. 2019, 18, 4157–4170. [Google Scholar] [CrossRef]
- Pizzo, A.; Marzetta, T.L.; Sanguinetti, L. Spatially-Stationary Model for Holographic MIMO Small-Scale Fading. IEEE J. Sel. Areas Commun. 2020, 38, 1964–1979. [Google Scholar] [CrossRef]
- Huang, C.; Hu, S.; Alexandropoulos, G.C.; Zappone, A.; Yuen, C.; Zhang, R.; Renzo, M.D.; Debbah, M. Holographic MIMO Surfaces for 6G Wireless Networks: Opportunities, Challenges, and Trends. IEEE Wirel. Commun. 2020, 27, 118–125. [Google Scholar] [CrossRef]
- Tse, D.; Viswanath, P. Fundamentals of Wireless Communication; Cambridge University Press: Cambridge, UK, 2005. [Google Scholar]
- Bucci, O.; Franceschetti, G. On the degrees of freedom of scattered fields. IEEE Trans. Antennas Propag. 1989, 37, 918–926. [Google Scholar] [CrossRef]
- Miller, D.A. Waves, modes, communications, and optics: A tutorial. Adv. Opt. Photonics 2019, 11, 679–825. [Google Scholar] [CrossRef]
- Muharemovic, T.; Sabharwal, A.; Aazhang, B. Antenna packing in low-power systems: Communication limits and array design. IEEE Trans. Inf. Theory 2008, 54, 429–440. [Google Scholar] [CrossRef]
- Migliore, M. On the role of the number of degrees of freedom of the field in MIMO channels. IEEE Trans. Antennas Propag. 2006, 54, 620–628. [Google Scholar] [CrossRef]
- Yuan, S.S.A.; He, Z.; Chen, X.; Huang, C.; Sha, W.E.I. Electromagnetic Effective Degree of Freedom of a MIMO System in Free Space. IEEE Antennas Wirel. Propag. Lett. 2022, 21, 446–450. [Google Scholar] [CrossRef]
- Miller, D.A. Communicating with waves between volumes: Evaluating orthogonal spatial channels and limits on coupling strengths. Appl. Optics 2000, 39, 1681–1699. [Google Scholar] [CrossRef]
- Yuan, S.S.A.; Wu, J.; Chen, M.L.; Lan, Z.; Zhang, L.; Sun, S.; Huang, Z.; Chen, X.; Zheng, S.; Jiang, L.J.; et al. Approaching the Fundamental Limit of Orbital-Angular-Momentum Multiplexing Through a Hologram Metasurface. Phys. Rev. Appl. 2021, 16, 064042. [Google Scholar] [CrossRef]
- Loyka, S. Information theory and electromagnetism: Are they related? In Proceedings of the 2004 10th International Symposium on Antenna Technology and Applied Electromagnetics and URSI Conference, Ottawa, ON, Canada, 20–23 July 2004; pp. 1–5. [Google Scholar]
- Dardari, D. Communicating With Large Intelligent Surfaces: Fundamental Limits and Models. IEEE J. Sel. Areas Commun. 2020, 38, 2526–2537. [Google Scholar] [CrossRef]
- Oestges, C. Validity of the Kronecker Model for MIMO Correlated Channels. In Proceedings of the 2006 IEEE 63rd Vehicular Technology Conference, Melbourne, VIC, Australia, 7–10 May 2006; Volume 6, pp. 2818–2822. [Google Scholar]
- Clarke, R.H. A statistical theory of mobile-radio reception. Bell Syst. Tech. J. 1968, 47, 957–1000. [Google Scholar] [CrossRef]
- Piestun, R.; Miller, D.A. Electromagnetic degrees of freedom of an optical system. J. Opt. Soc. Am. A—Opt. Image Sci. Vis. 2000, 17, 892–902. [Google Scholar] [CrossRef]
- Migliore, M.D. Horse (Electromagnetics) is More Important Than Horseman (Information) for Wireless Transmission. IEEE Trans. Antennas Propag. 2019, 67, 2046–2055. [Google Scholar] [CrossRef]
- Jensen, M.A.; Wallace, J.W. Capacity of the Continuous-Space Electromagnetic Channel. IEEE Trans. Antennas Propag. 2008, 56, 524–531. [Google Scholar] [CrossRef]
- Wen, G. Multi-antenna information theory. Prog. Electromagn. Res. 2007, 75, 11–50. [Google Scholar] [CrossRef][Green Version]
- Xu, J.; Janaswamy, R. Electromagnetic Degrees of Freedom in 2-D Scattering Environments. IEEE Trans. Antennas Propag. 2006, 54, 3882–3894. [Google Scholar] [CrossRef]
- Ehrenborg, C.; Gustafsson, M.; Capek, M. Capacity Bounds and Degrees of Freedom for MIMO Antennas Constrained by Q-Factor. IEEE Trans. Antennas Propag. 2021, 69, 5388–5400. [Google Scholar] [CrossRef]
- Zhang, X.; Sood, N.; Siu, J.K.; Sarris, C.D. A Hybrid Ray-Tracing/Vector Parabolic Equation Method for Propagation Modeling in Train Communication Channels. IEEE Trans. Antennas Propag. 2016, 64, 1840–1849. [Google Scholar] [CrossRef]
- Lin, S.; Peng, Z.; Antonsen, T.M. A Stochastic Green’s Function for Solution of Wave Propagation in Wave-Chaotic Environments. IEEE Trans. Antennas Propag. 2020, 68, 3919–3933. [Google Scholar] [CrossRef]
- Biswas, S.; Masouros, C.; Ratnarajah, T. Performance Analysis of Large Multiuser MIMO Systems With Space-Constrained 2-D Antenna Arrays. IEEE Trans. Wirel. Commun. 2016, 15, 3492–3505. [Google Scholar] [CrossRef]
- Burr, A. Capacity bounds and estimates for the finite scatterers MIMO wireless channel. IEEE J. Sel. Areas Commun. 2003, 21, 812–818. [Google Scholar] [CrossRef]
- Bentosela, F.; Marchetti, N.; Cornean, H.D. Influence of Environment Richness on the Increase of MIMO Capacity With Number of Antennas. IEEE Trans. Antennas Propag. 2014, 62, 3786–3796. [Google Scholar] [CrossRef]
- Poon, A.; Tse, D.; Brodersen, R. Impact of scattering on the capacity, diversity, and propagation range of multiple-antenna channels. IEEE Trans. Inf. Theory 2006, 52, 1087–1100. [Google Scholar] [CrossRef][Green Version]
- Hanpinitsak, P.; Saito, K.; Takada, J.I.; Kim, M.; Materum, L. Multipath Clustering and Cluster Tracking for Geometry-Based Stochastic Channel Modeling. IEEE Trans. Antennas Propag. 2017, 65, 6015–6028. [Google Scholar] [CrossRef]
- Chew, W. Waves and Fields in Inhomogeneous Media; Inst of Electrical: New York, NY, USA, 1995. [Google Scholar]
- Peterson, A.F.; Ray, S.L.; Mittra, R. Computational Methods for Electromagnetics; IEEE Press: New York, NY, USA, 1998; Volume 351. [Google Scholar]
- Sarkar, T.; Arvas, E.; Rao, S. Application of FFT and the conjugate gradient method for the solution of electromagnetic radiation from electrically large and small conducting bodies. IEEE Trans. Antennas Propag. 1986, 34, 635–640. [Google Scholar] [CrossRef]
- Zhang, Z.; Liu, Q. Three-dimensional weak-form conjugate-and biconjugate-gradient FFT methods for volume integral equations. Microw. Opt. Technol. Lett. 2001, 29, 350–356. [Google Scholar] [CrossRef]
- Chizhik, D.; Foschini, G.J.; Gans, M.J.; Valenzuela, R.A. Keyholes, correlations, and capacities of multielement transmit and receive antennas. IEEE Trans. Wirel. Commun. 2002, 1, 361–368. [Google Scholar] [CrossRef]
- Almers, P.; Tufvesson, F.; Molisch, A.F. Keyhole effect in MIMO wireless channels: Measurements and theory. IEEE Trans. Wirel. Commun. 2006, 5, 3596–3604. [Google Scholar] [CrossRef]
- Sarkar, T.K.; Salazar-Palma, M. MIMO: Does It Make Sense From an Electromagnetic Perspective and Illustrated Using Computational Electromagnetics? IEEE J. Multiscale Multiphys. Comput. Techn. 2019, 4, 269–281. [Google Scholar] [CrossRef]
- Born, M.; Wolf, E. Principles of Optics: Electromagnetic Theory of Propagation, Interference and Diffraction of Light; Elsevier: Cambridge, UK, 2013. [Google Scholar]
- Bruns, C.; Vahldieck, R. A closer look at reverberation Chambers - 3-D Simulation and experimental verification. IEEE Trans. Electromagn. Compat. 2005, 47, 612–626. [Google Scholar] [CrossRef]
- Kildal, P.S.; Chen, X.; Orlenius, C.; Franzen, M.; Patane, C.S.L. Characterization of Reverberation Chambers for OTA Measurements of Wireless Devices: Physical Formulations of Channel Matrix and New Uncertainty Formula. IEEE Trans. Antennas Propag. 2012, 60, 3875–3891. [Google Scholar] [CrossRef]




Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Yuan, S.S.A.; He, Z.; Sun, S.; Chen, X.; Huang, C.; Sha, W.E.I. Electromagnetic Effective-Degree-of-Freedom Limit of a MIMO System in 2-D Inhomogeneous Environment. Electronics 2022, 11, 3232. https://doi.org/10.3390/electronics11193232
Yuan SSA, He Z, Sun S, Chen X, Huang C, Sha WEI. Electromagnetic Effective-Degree-of-Freedom Limit of a MIMO System in 2-D Inhomogeneous Environment. Electronics. 2022; 11(19):3232. https://doi.org/10.3390/electronics11193232
Chicago/Turabian StyleYuan, Shuai S. A., Zi He, Sheng Sun, Xiaoming Chen, Chongwen Huang, and Wei E. I. Sha. 2022. "Electromagnetic Effective-Degree-of-Freedom Limit of a MIMO System in 2-D Inhomogeneous Environment" Electronics 11, no. 19: 3232. https://doi.org/10.3390/electronics11193232
APA StyleYuan, S. S. A., He, Z., Sun, S., Chen, X., Huang, C., & Sha, W. E. I. (2022). Electromagnetic Effective-Degree-of-Freedom Limit of a MIMO System in 2-D Inhomogeneous Environment. Electronics, 11(19), 3232. https://doi.org/10.3390/electronics11193232






