A Simplified Gm − C Filter Technique for Reference Spur Reduction in Phase-Locked Loop
Abstract
1. Introduction
1.1. Overview of IRNSS PLL
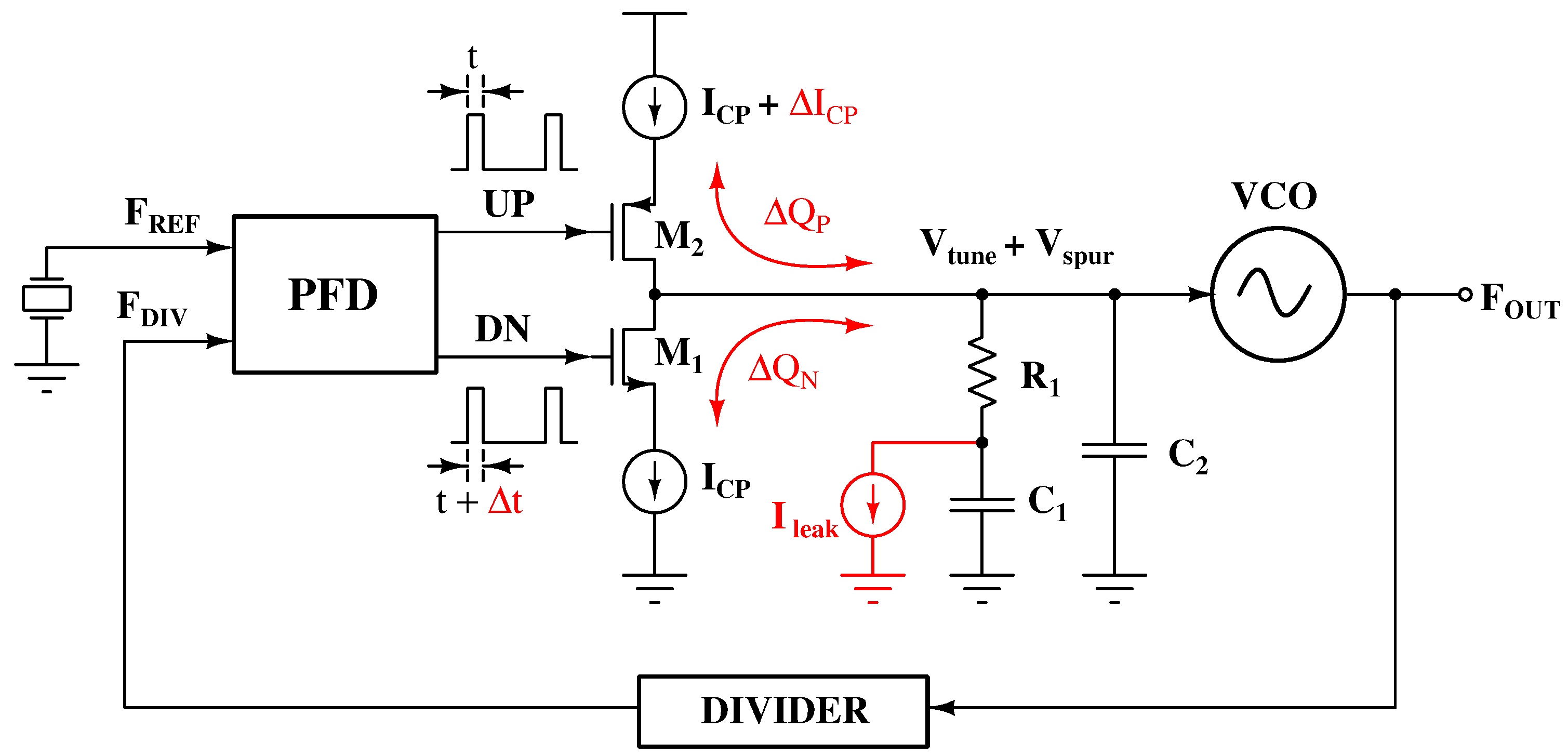
1.2. Reference Spur in PLL
1.3. Spur Reduction Techniques
1.4. Filter Technique for Reference Spur Reduction
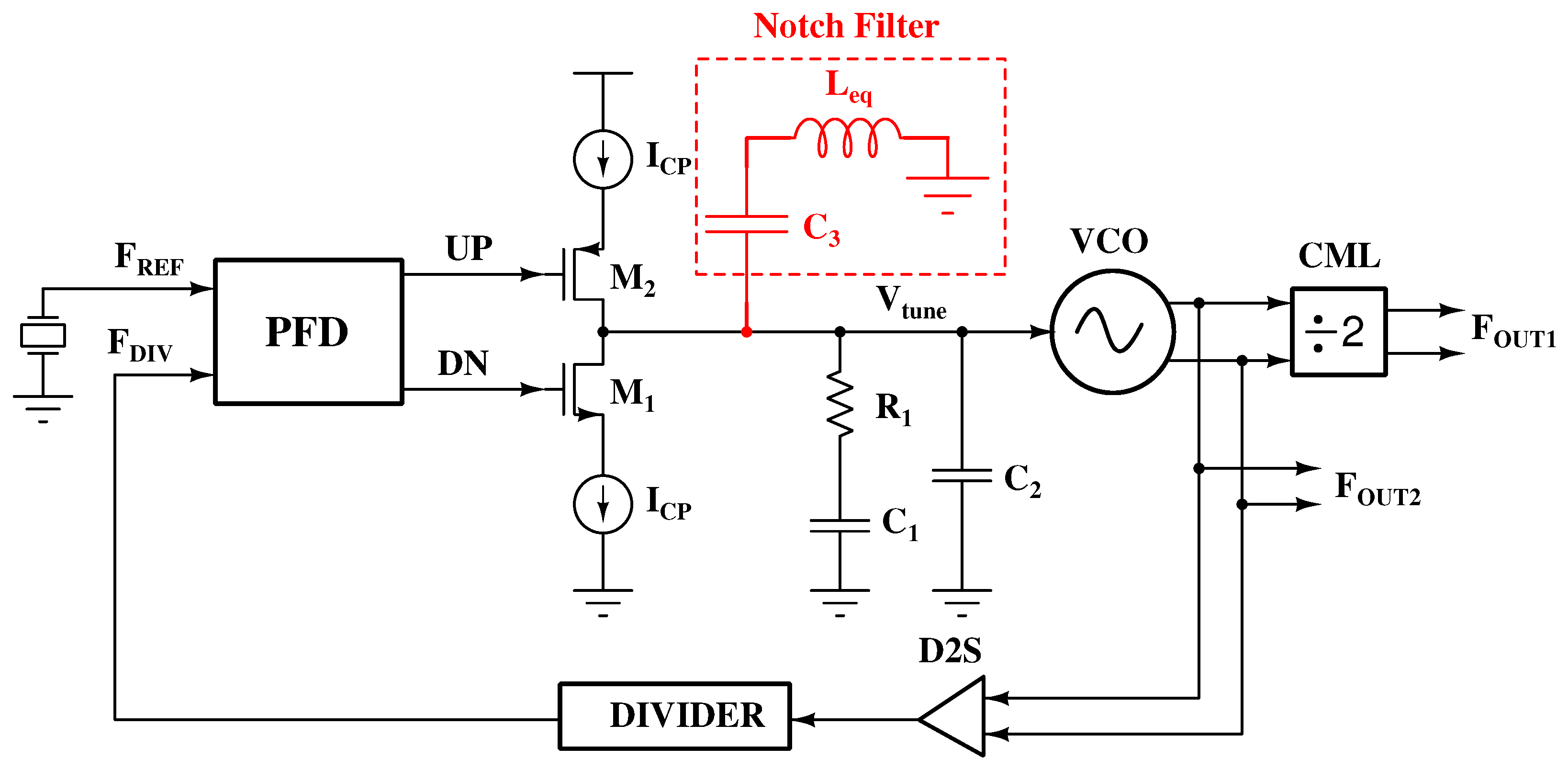
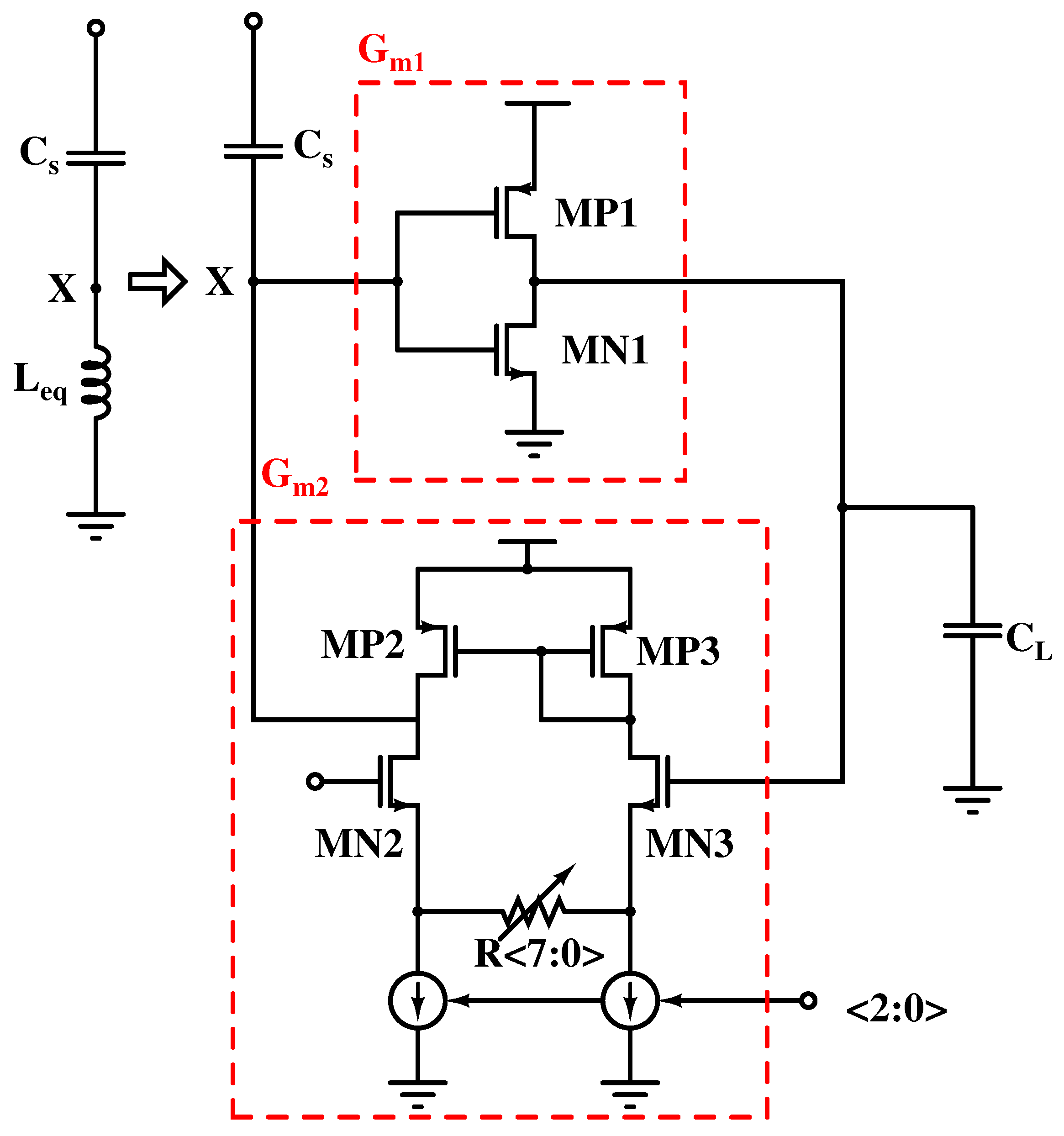
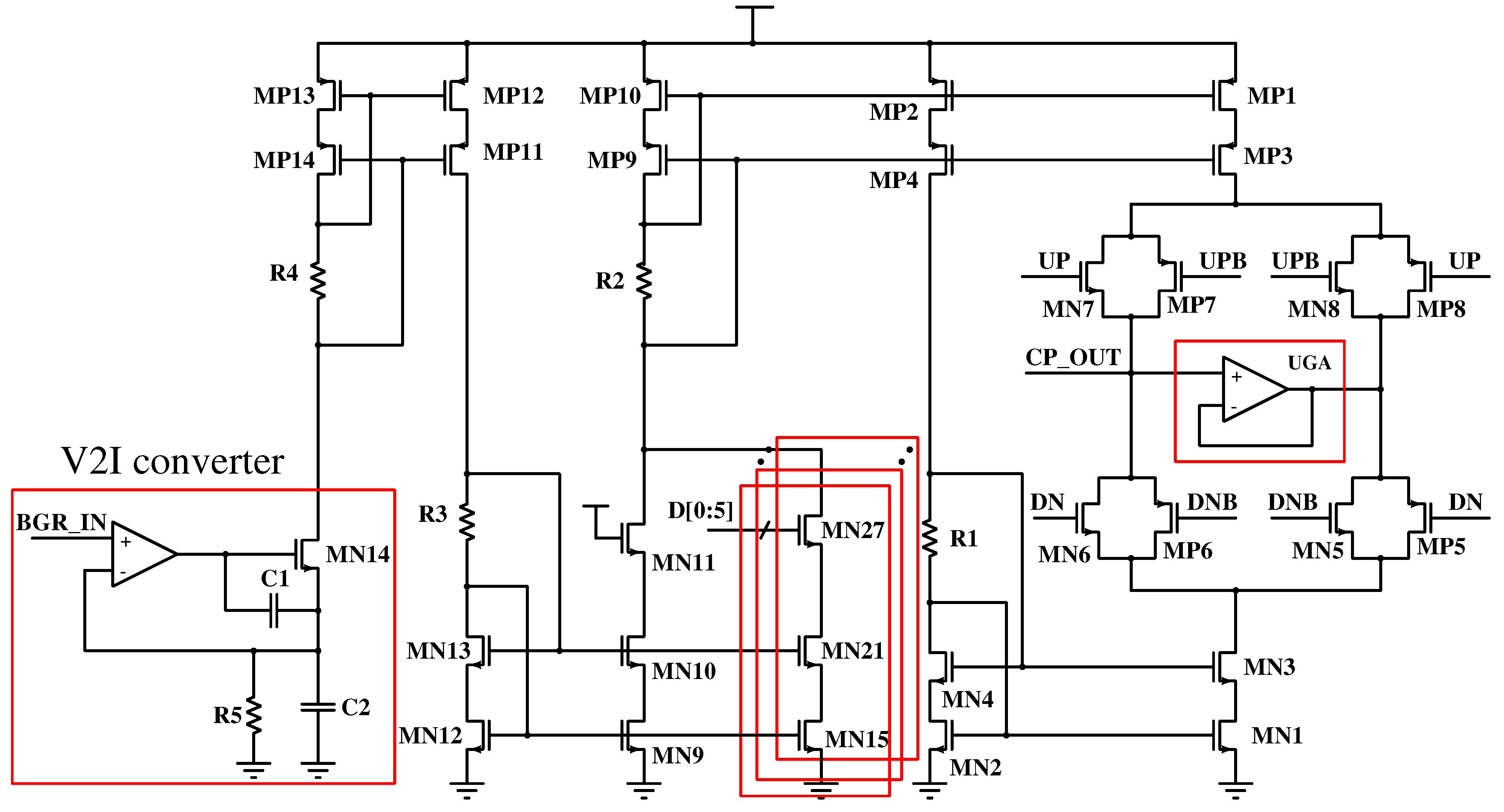
2. Proposed Filter Technique
2.1. Phase-Locked Loop Design
2.1.1. PFD and Charge Pump
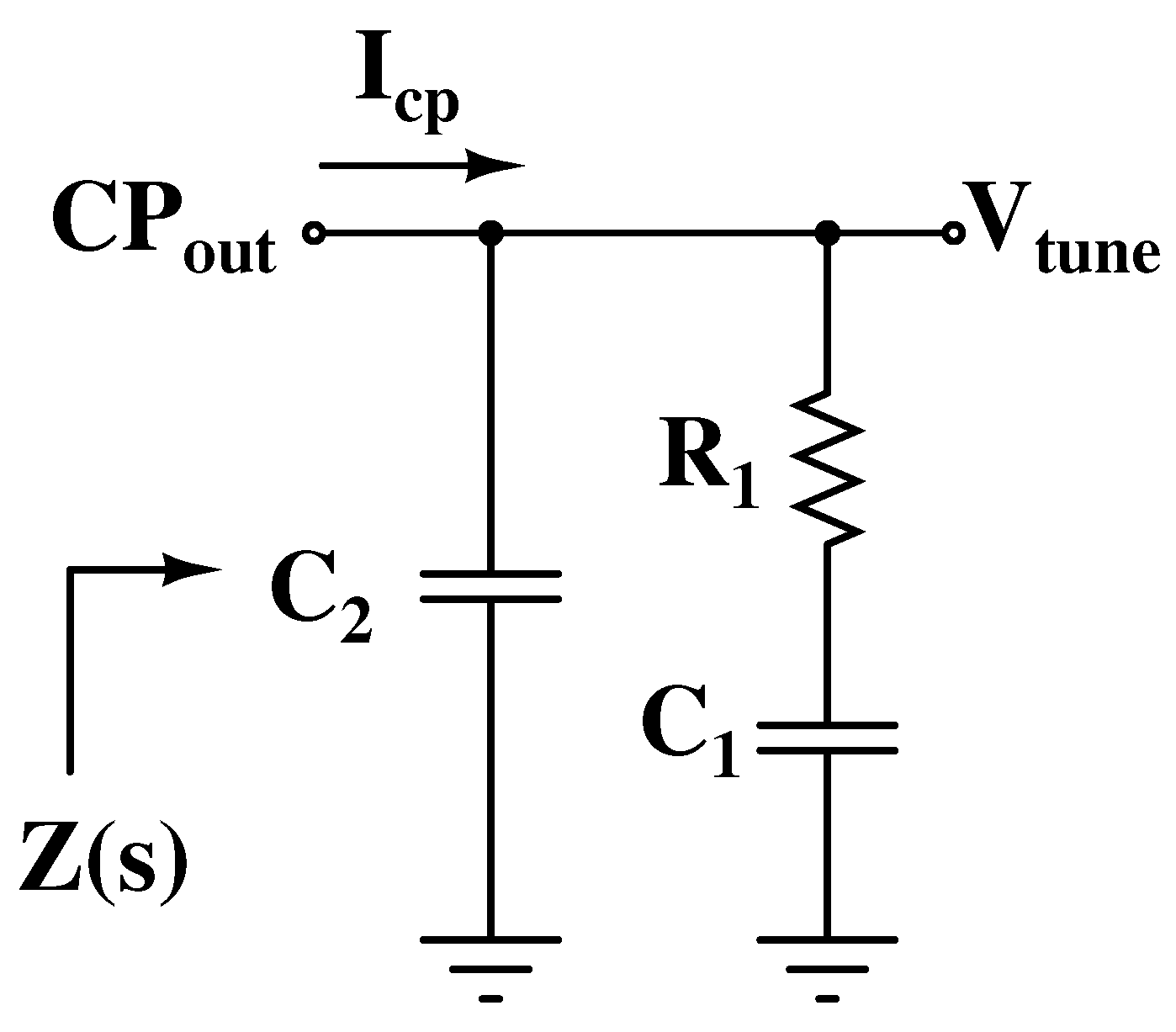
2.1.2. Second-Order Loop Filter
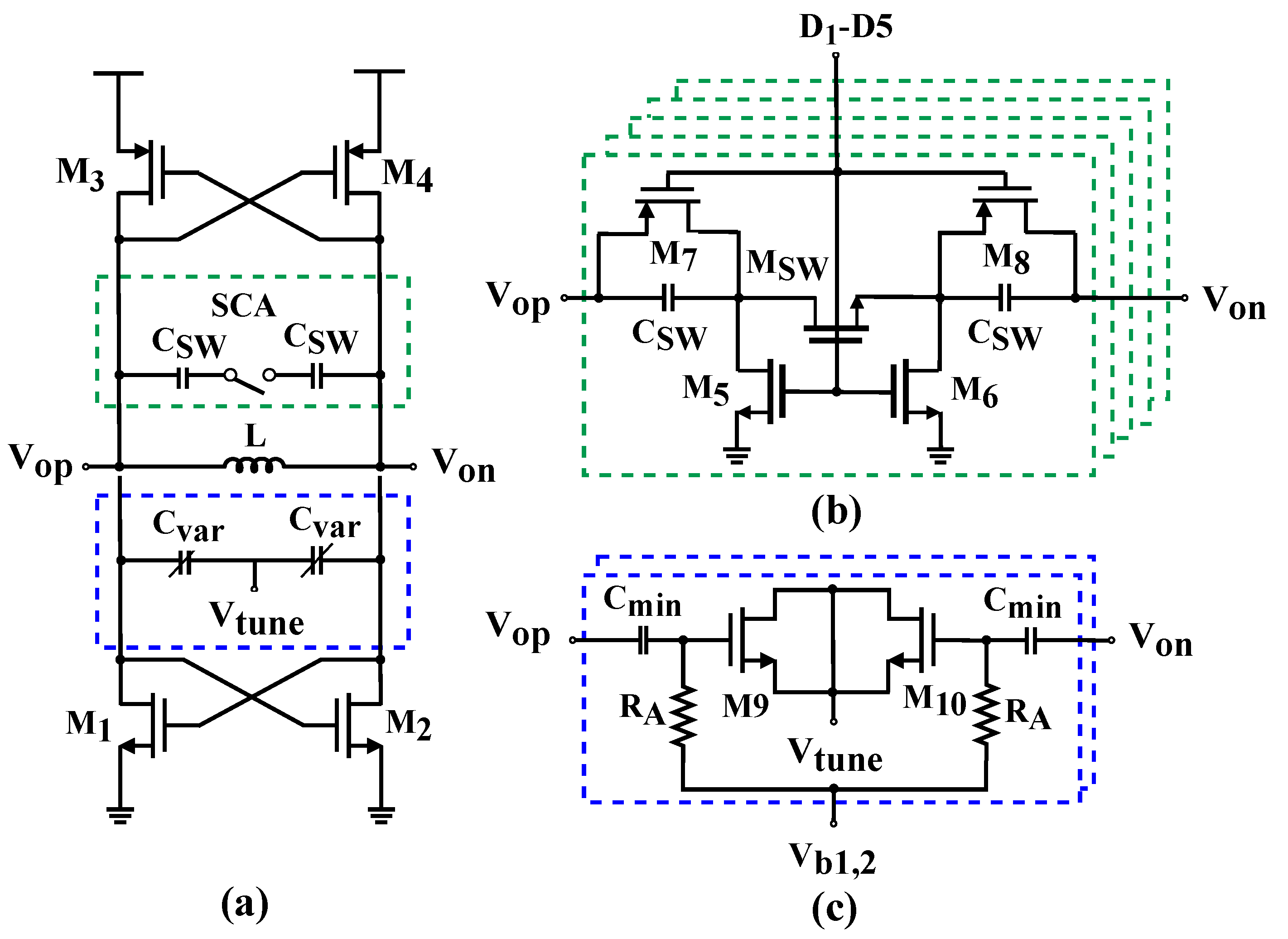
2.1.3. VCO and CML Divider
2.1.4. Feedback Divider
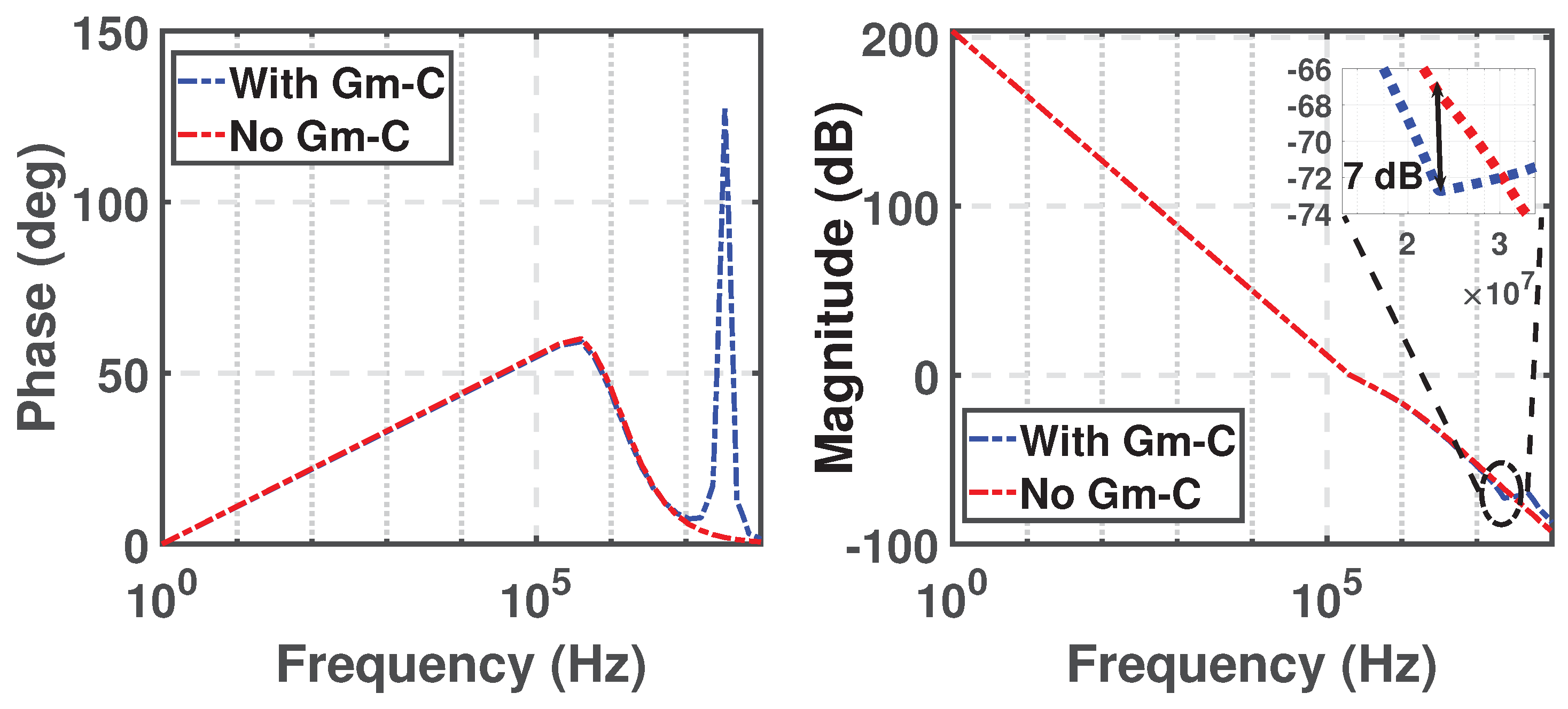
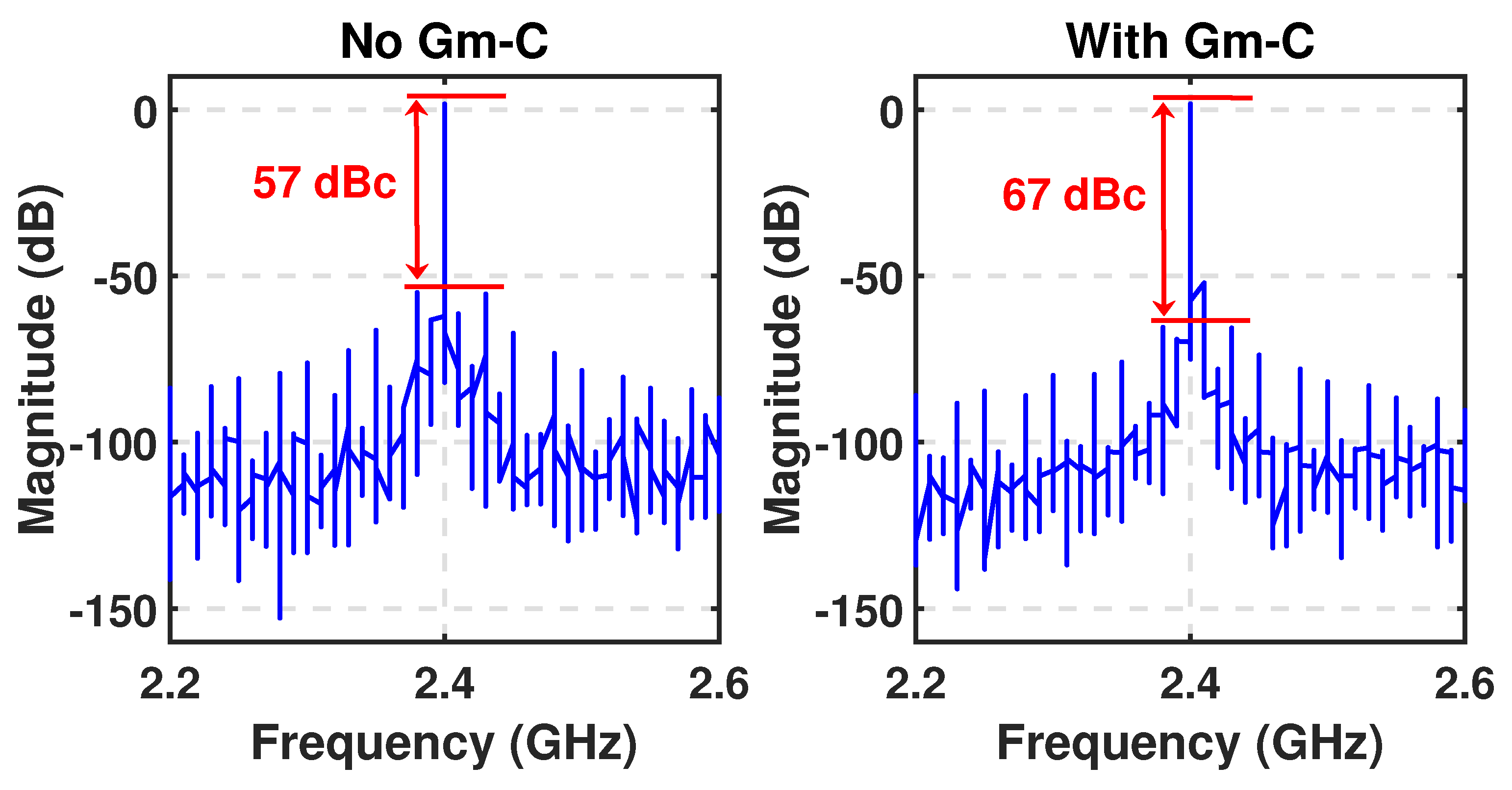
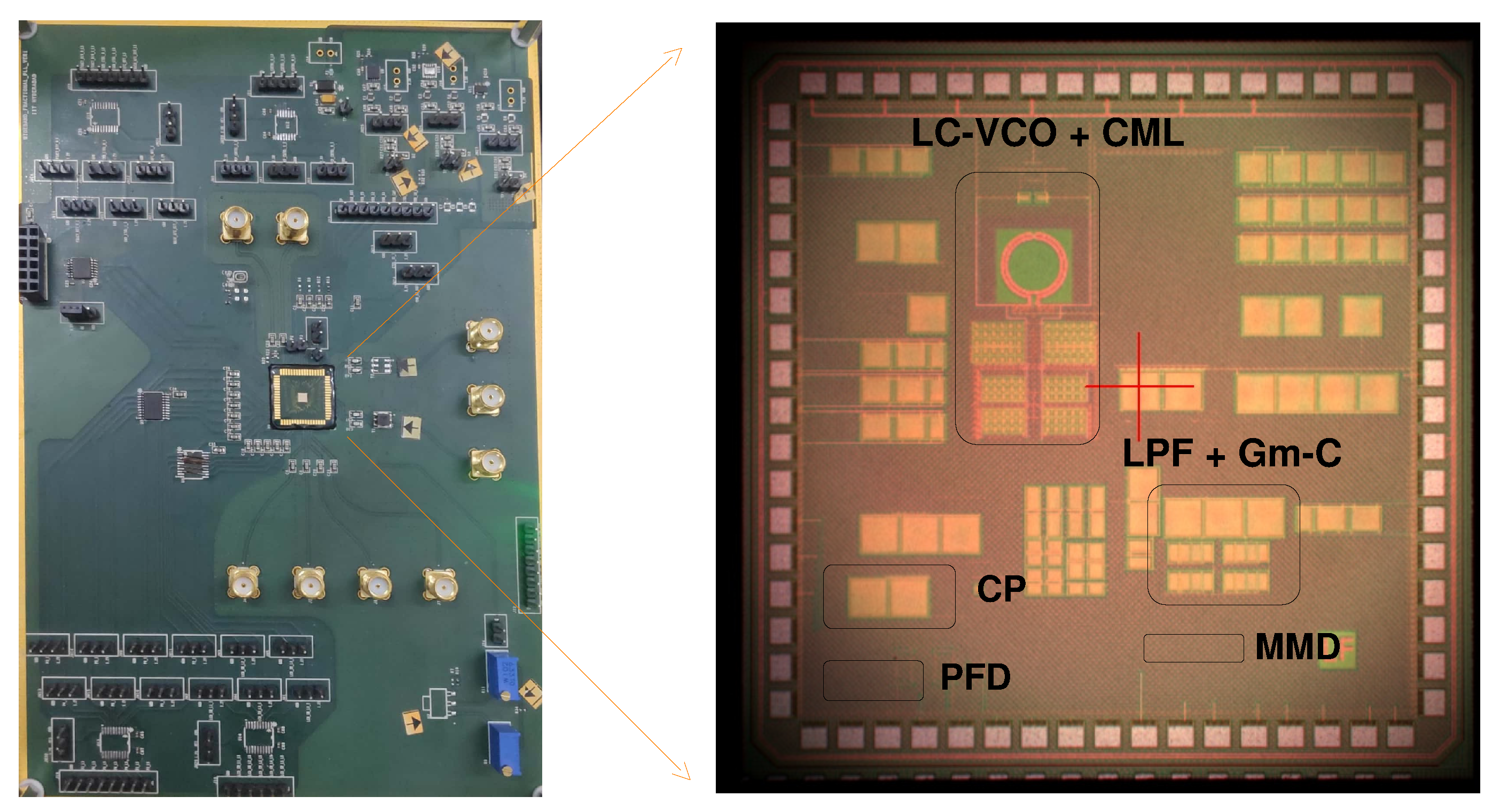
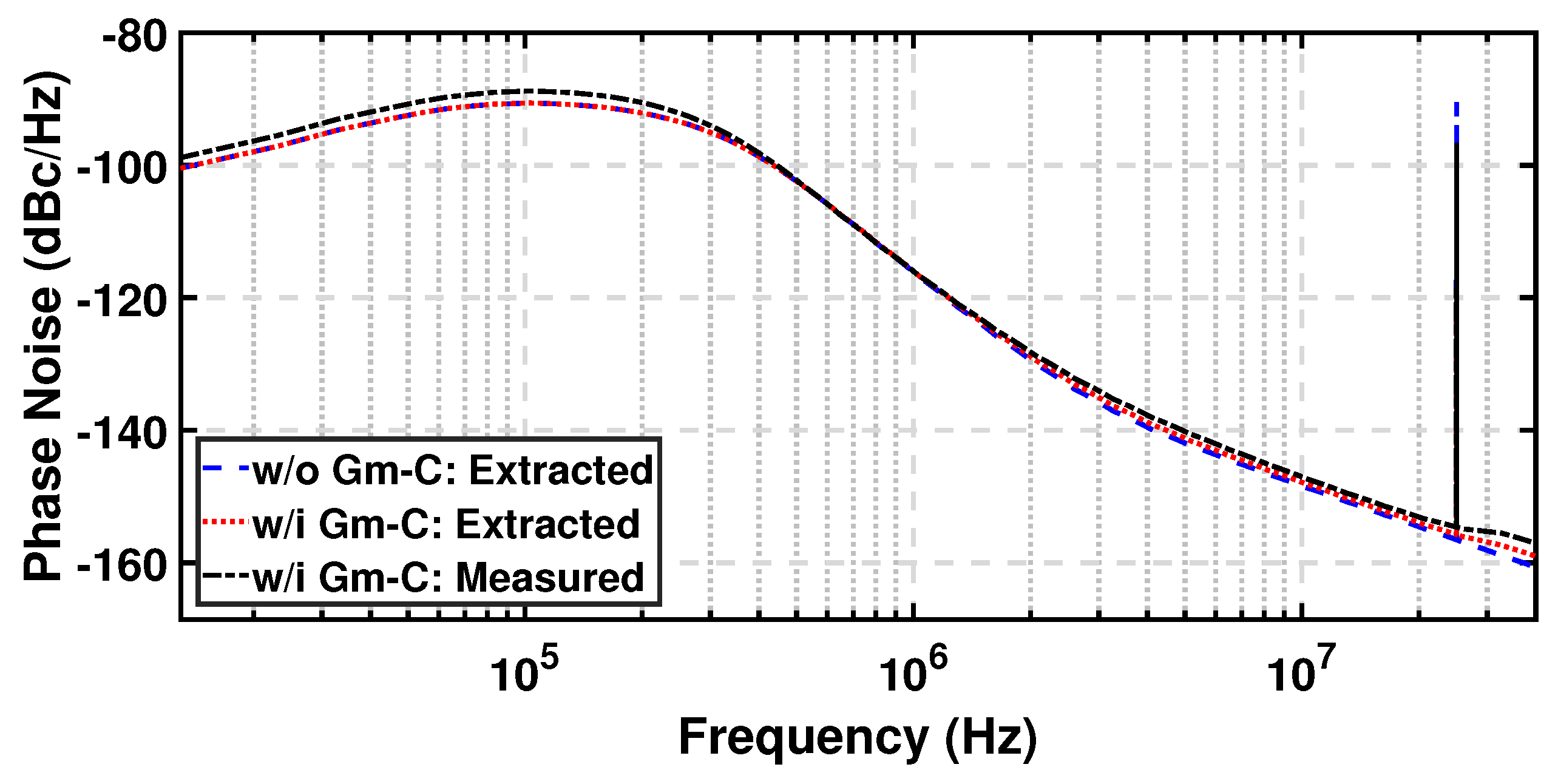
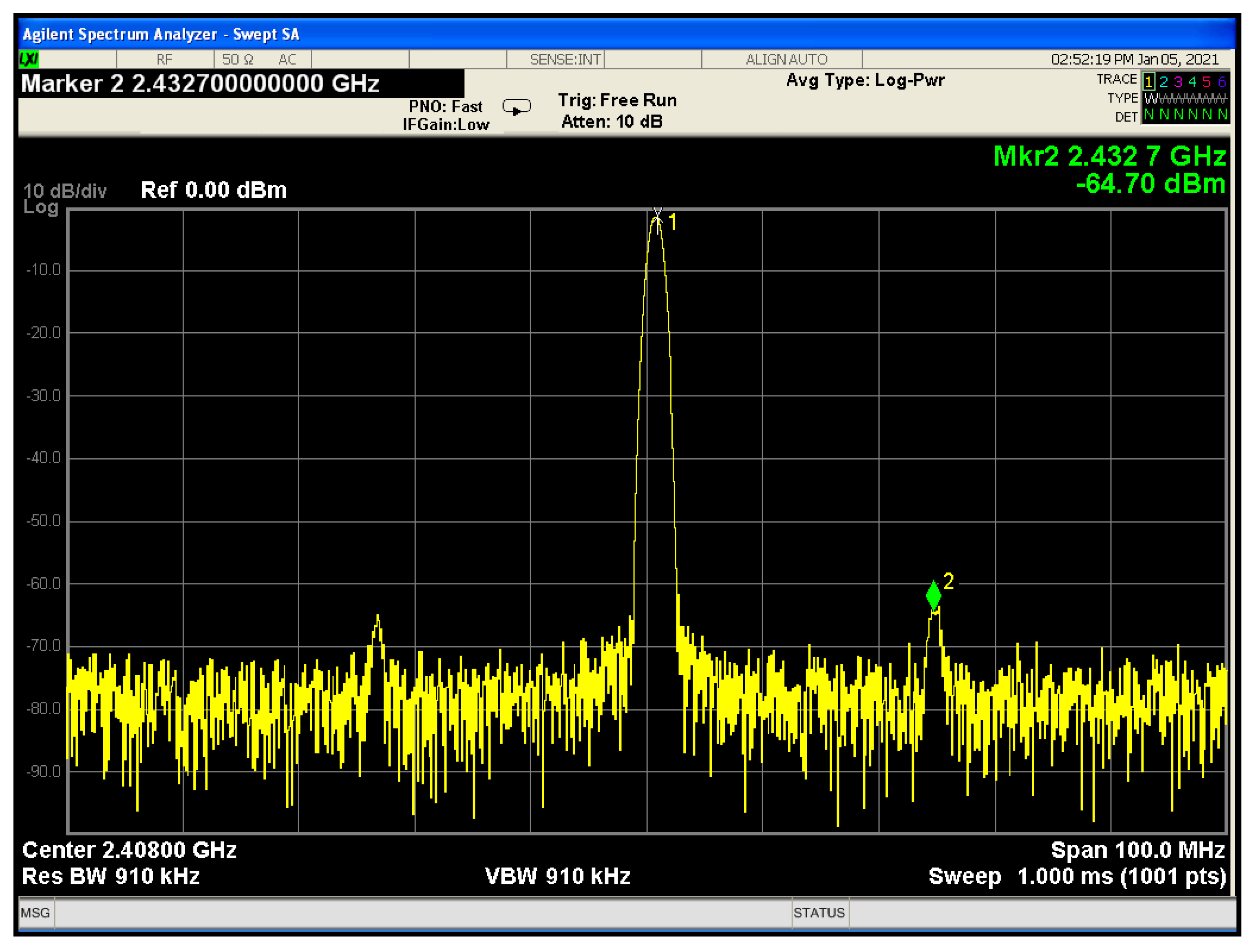
3. Measurement Results
4. Conclusions
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Ko, J.; Kim, J.; Cho, S.; Lee, K. A 19-mW 2.6-mm/sup 2/ L1/L2 dual-band CMOS GPS receiver. IEEE J. Solid-State Circuits 2005, 40, 1414–1425. [Google Scholar]
- Li, S.; Li, J.; Gu, X.; Wang, H.; Li, C.; Wu, J.; Tang, M. Reconfigurable All-Band RF CMOS Transceiver for GPS/GLONASS/Galileo/Beidou with Digitally Assisted Calibration. IEEE Trans. Very Large Scale Integr. Syst. 2015, 23, 1814–1827. [Google Scholar] [CrossRef]
- Hung, C.-M.; Kenneth, K.O. A fully integrated 1.5-V 5.5-GHz CMOS phase-locked loop. IEEE J. Solid-State Circuits 2002, 37, 521–525. [Google Scholar] [CrossRef]
- Li, A.; Chao, Y.; Chen, X.; Wu, L.; Luong, H.C. A Spur-and-Phase-Noise-Filtering Technique for Inductor-Less Fractional-N Injection-Locked PLLs. IEEE J. Solid-State Circuits 2017, 52, 2128–2140. [Google Scholar] [CrossRef]
- Lee, Y.; Seong, T.; Yoo, S.; Choi, J. A Low-Jitter and Low-Reference-Spur Ring-VCO-Based Switched-Loop Filter PLL Using a Fast Phase-Error Correction Technique. IEEE J. Solid-State Circuits 2018, 53, 1192–1202. [Google Scholar] [CrossRef]
- Seong, T.; Lee, Y.; Yoo, S.; Choi, J. A 320-fs RMS Jitter and −75-dBc Reference-Spur Ring-DCO-Based Digital PLL Using an Optimal-Threshold TDC. IEEE J. Solid-State Circuits 2019, 54, 2501–2512. [Google Scholar] [CrossRef]
- Yang, Z.; Chen, Y.; Mak, P.-I.; Martins, R.P. A Calibration-Free, Reference-Buffer-Free, Type-I Narrow-Pulse-Sampling PLL with −78.7-dBc REF Spur, −128.1-dBc/Hz Absolute In-Band PN and −254-dB FOM. IEEE-Solid-State Circuits Lett. 2020, 3, 494–497. [Google Scholar] [CrossRef]
- Yang, Z.; Chen, Y.; Mak, P.-I.; Martins, R.P. A 0.003-mm2 440fsRMS-Jitter and −64dBc-Reference-Spur Ring-VCO-Based Type-I PLL Using a Current-Reuse Sampling Phase Detector in 28-nm CMOS. IEEE Trans. Circuits Syst. I Regul. Pap. 2021, 68, 2307–2316. [Google Scholar] [CrossRef]
- Seol, J.-H.; Choo, K.; Blaauw, D.; Sylvester, D.; Jang, T. Reference Oversampling PLL Achieving −256-dB FoM and −78-dBc Reference Spur. IEEE J. Solid-State Circuits 2021, 56, 2993–3007. [Google Scholar] [CrossRef]
- Huang, Y.; Chen, Y.; Jiao, H.; Mak, P.-I.; Martins, R.P. A 3.36-GHz Locking-Tuned Type-I Sampling PLL with −78.6-dBc Reference Spur Merging Single-Path Reference-Feedthrough-Suppression and Narrow-Pulse-Shielding Techniques. IEEE Trans. Circuits Syst. II Express Briefs 2021, 68, 3093–3097. [Google Scholar] [CrossRef]
- Liang, Y.; Boon, C.C. A 40 GHz CMOS PLL with −75-dBc Reference Spur and 121.9-fsrms Jitter Featuring a Quadrature Sampling Phase-Frequency Detector. IEEE Trans. Microw. Theory Tech. 2022, 70, 2299–2314. [Google Scholar] [CrossRef]
- Liang, Y.; Boon, C.C.; Chen, Q. A 23.4 mW −72-dBc Reference Spur 40 GHz CMOS PLL Featuring a Spur-Compensation Phase Detector. IEEE Microw. Wirel. Components Lett. 2022, 32, 1091–1094. [Google Scholar] [CrossRef]
- Huang, Y.; Chen, Y.; Zhao, B.; Mak, P.-I.; Martins, R.P. A 3.6-GHz Type-II Sampling PLL with a Differential Parallel-Series Double-Edge S-PD Scoring 43.1-fsRMSJitter, −258.7-dB FOM, and −75.17-dBc Reference Spur. IEEE Trans. Very Large Scale Integr. (VLSI) Syst. 2023, 31, 188–198. [Google Scholar] [CrossRef]
- Verma, A.; Bhagavatula, V.; Singh, A.; Wu, W.; Nagarajan, H.; Lau, P.; Yu, X.; Elsayed, O.; Jain, A.; Sarkar, A.; et al. A 16-Channel, 28/39GHz Dual-Polarized 5G FR2 Phased-Array Transceiver IC with a Quad-Stream IF Transceiver Supporting Non-Contiguous Carrier Aggregation up to 1.6GHz BW. In Proceedings of the IEEE International Solid-State Circuits Conference (ISSCC), San Francisco, CA, USA, 18–22 February 2024; pp. 1–3. [Google Scholar]
- Chary, P.P.; Peerla, R.S.; Pula, B.T.; Dutta, A.; Sahoo, B.D. Package Aware LC-VCO with Self-Biased Switched Capacitor Structure for Better Supply Noise Rejection. IEEE TRans. Components Packag. Manuf. Tech. 2022, 12, 1653–1660. [Google Scholar] [CrossRef]
- Shi, C.; Wang, C.; Ye, L.; Liao, H. 99 dBc/Hz @ 10 KHz 1 MHz-step dual-loop integer-N PLL with anti-mislocking frequency calibration for global navigation satellite system receiver. In Proceedings of the 2011 IEEE International Symposium of Circuits and Systems (ISCAS), Rio de Janeiro, Brazil, 15–18 May 2011; pp. 1876–1879. [Google Scholar]
- Bai, Z.; Zhou, X.; Mason, R.D.; Allan, G. A 2-GHz Pulse Injection-Locked Rotary Traveling-Wave Oscillator. IEEE Trans. Microw. Theory Tech. 2016, 64, 1854–1866. [Google Scholar] [CrossRef]
- Kharalkar, A.; Pancholi, M.; Kanchetla, V.K.; Khade, A.; Khyalia, S.; Hameed, S.; Zele, R. A Compact, Low-Phase Noise Fractional-N PLL for Global Navigation Receiver. In Proceedings of the IEEE 19th New Circuits and Systems Conference (NEWCAS), Toulon, France, 13–16 June 2021; pp. 1–4. [Google Scholar]
- Peerla, R.S.; Chary, P.; Dutta, A.; Sahoo, B.D. A Dual VCO Based L5/S Band PLL with Extended Range Divider for IRNSS Application. In Proceedings of the IEEE International Symposium on Circuits and Systems (ISCAS), Austin, TX, USA, 27 May–1 June 2022; pp. 1699–1703. [Google Scholar]
- Shaik Peerla, R.; Dutta, A.; Sahoo, B.D. An Extended Range Divider Technique for Multi-Band PLL. J. Low Power Electron. Appl. 2023, 13, 43. [Google Scholar] [CrossRef]
- Elsayed, M.M.; Abdul-Latif, M.; Sanchez-Sinencio, E. A Spur-Frequency-Boosting PLL with a −74 dBc Reference-Spur Suppression in 90 nm Digital CMOS. IEEE J. Solid-State Circuits 2013, 48, 2104–2117. [Google Scholar] [CrossRef]
- Rhee, W. Design of high-performance CMOS charge pumps in phase-locked loops. In Proceedings of the IEEE International Symposium on Circuits and Systems (ISCAS), Orlando, FL, USA, 30 May–2 June 1999; Volume 2, pp. 545–548. [Google Scholar]
- Lin, T.H.; Kaiser, W.J. A 900-MHz 2.5-mA CMOS frequency synthesizer with an automatic SC tuning loop. IEEE J. Solid-State Circuits 2001, 36, 424–431. [Google Scholar]
- Gao, X.; Klumperink, E.A.; Socci, G.; Bohsali, M.; Nauta, B. Spur Reduction Techniques for Phase-Locked Loops Exploiting A Sub-Sampling Phase Detector. IEEE J. Solid-State Circuits 2010, 45, 1809–1821. [Google Scholar] [CrossRef]
- Lee, T.; Lee, W. A Spur Suppression Technique for Phase-Locked Frequency Synthesizers. In Proceedings of the IEEE International Solid-State Circuits Conference (ISSCC), San Francisco, CA, USA, 6–9 February 2006; pp. 2432–2441. [Google Scholar]
- Ko, H.G.; Bae, W.; Jeong, G.S.; Jeong, D.K. Reference Spur Reduction Techniques for a Phase-Locked Loop. IEEE Access 2019, 7, 38035–38043. [Google Scholar] [CrossRef]
- Sharma, J.; Krishnaswamy, H. A 2.4-GHz Reference-Sampling Phase-Locked Loop That Simultaneously Achieves Low-Noise and Low-Spur Performance. IEEE J. Solid-State Circuits 2019, 54, 1407–1424. [Google Scholar] [CrossRef]
- Kong, L.; Razavi, B. A 2.4 GHz 4 mW Integer-N Inductorless RF Synthesizer. IEEE J. Solid-State Circuits 2016, 51, 626–635. [Google Scholar] [CrossRef]
- Cheng, X.; Chen, F.J.; Zhang, L.; Gao, H.; Han, J.A.; Han, J.Y.; Yu, Y.; Deng, X.J. A Closed-Loop Reconfigurable Analog Baseband Circuitry with Open-Loop Tunable Notch Filters to Improve Receiver Tx Leakage and Close-in Blocker Tolerance. IEEE Trans. Circuits Syst. II Express Briefs 2022, 69, 839–843. [Google Scholar] [CrossRef]
- Razavi, B. Design of CMOS Phase-Locked Loops: From Circuit Level to Architecture Level; Cambridge University Press: Cambridge, UK, 2020. [Google Scholar]
- Lavrič, A.; Batagelj, B.; Vidmar, M. Calibration of an RF/Microwave Phase Noise Meter with a Photonic Delay Line. Photonics 2022, 9, 533. [Google Scholar] [CrossRef]
- Wu, W.; Yao, C.W.; Guo, C.; Chiang, P.Y.; Chen, L.; Lau, P.K.; Bai, Z.; Son, S.W.; Cho, T.B. A 14-nm Ultra-Low Jitter Fractional-N PLL Using a DTC Range Reduction Technique and a Reconfigurable Dual-Core VCO. IEEE J. Solid-State Circuits 2021, 56, 3756–3767. [Google Scholar] [CrossRef]





| Parameter | Value |
|---|---|
| Crystal frequency (MHz) | 23 to 27 |
| VCO range (GHz) | 1.6 to 3.2 |
| VCO gain (MHz/V) | 80 to 120 |
| (mA) | 0.1 to 1 |
| Division ratio | 64 to 127 |
| Loop bandwidth (kHz) | 160 to 240 |
| Phase margin (°) | 54 to 59 |
| PN @ 100 kHz (dBc/Hz) | −90 to −95 |
| PN @ 1 MHz (dBc/Hz) | −115 to −120 |
| X | 0 | ||
| 0 | 1 | ||
| 1 | 1 |
| This Work | [28] | [29] | |
|---|---|---|---|
| Supply (V) | 2.5 | 1.3 | 1.8 |
| Architecture (V) | Single ended | Differential | Differential |
| Operational amplifier in implementation | No | Yes (Single ended) | Yes (Fully differential) |
| Circuit Complexity | Low | Moderate | High |
| Current (mA) | 0.2 | 0.4 | 1.5 |
| Compatible with lower supply | Yes | No | No |
| Programmability | No † | Yes | Yes |
| This Work | [18] | [26] | [32] | |
|---|---|---|---|---|
| Technology (nm) | 65 | 65 | 65 | 14 |
| Supply (V) | 2.5/1.2 | 1.2 | 1.3 | 1/0.8 |
| Frequency (GHz) | 1.6–3.2 | 1.17–2.5 | 0.8 | 5–7 |
| Reference (MHz) | 23–27 | 23–27 | 50 | 76.8 |
| PN (dBc/Hz) @ 1 MHz | −116/−122 | −116 | −110 | −122 |
| Area () | 0.8 | 0.25 | 0.935 | 0.31 |
| Power (mW) | 30 | 15.7 | 4.8 | 14.2 |
| Ref. spur (dBc) | −64 (−57 ‡) | NA | −68.57 | −69.6 |
| FoM † | 171.3 | 165.4 | 161.25 | 186.6 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Chary, P.P.; Shaik Peerla, R.; Dutta, A. A Simplified Gm − C Filter Technique for Reference Spur Reduction in Phase-Locked Loop. J. Low Power Electron. Appl. 2024, 14, 17. https://doi.org/10.3390/jlpea14010017
Chary PP, Shaik Peerla R, Dutta A. A Simplified Gm − C Filter Technique for Reference Spur Reduction in Phase-Locked Loop. Journal of Low Power Electronics and Applications. 2024; 14(1):17. https://doi.org/10.3390/jlpea14010017
Chicago/Turabian StyleChary, P. Purushothama, Rizwan Shaik Peerla, and Ashudeb Dutta. 2024. "A Simplified Gm − C Filter Technique for Reference Spur Reduction in Phase-Locked Loop" Journal of Low Power Electronics and Applications 14, no. 1: 17. https://doi.org/10.3390/jlpea14010017
APA StyleChary, P. P., Shaik Peerla, R., & Dutta, A. (2024). A Simplified Gm − C Filter Technique for Reference Spur Reduction in Phase-Locked Loop. Journal of Low Power Electronics and Applications, 14(1), 17. https://doi.org/10.3390/jlpea14010017






