Implementation of Power-Efficient Class AB Miller Amplifiers Using Resistive Local Common-Mode Feedback
Abstract
:1. Introduction
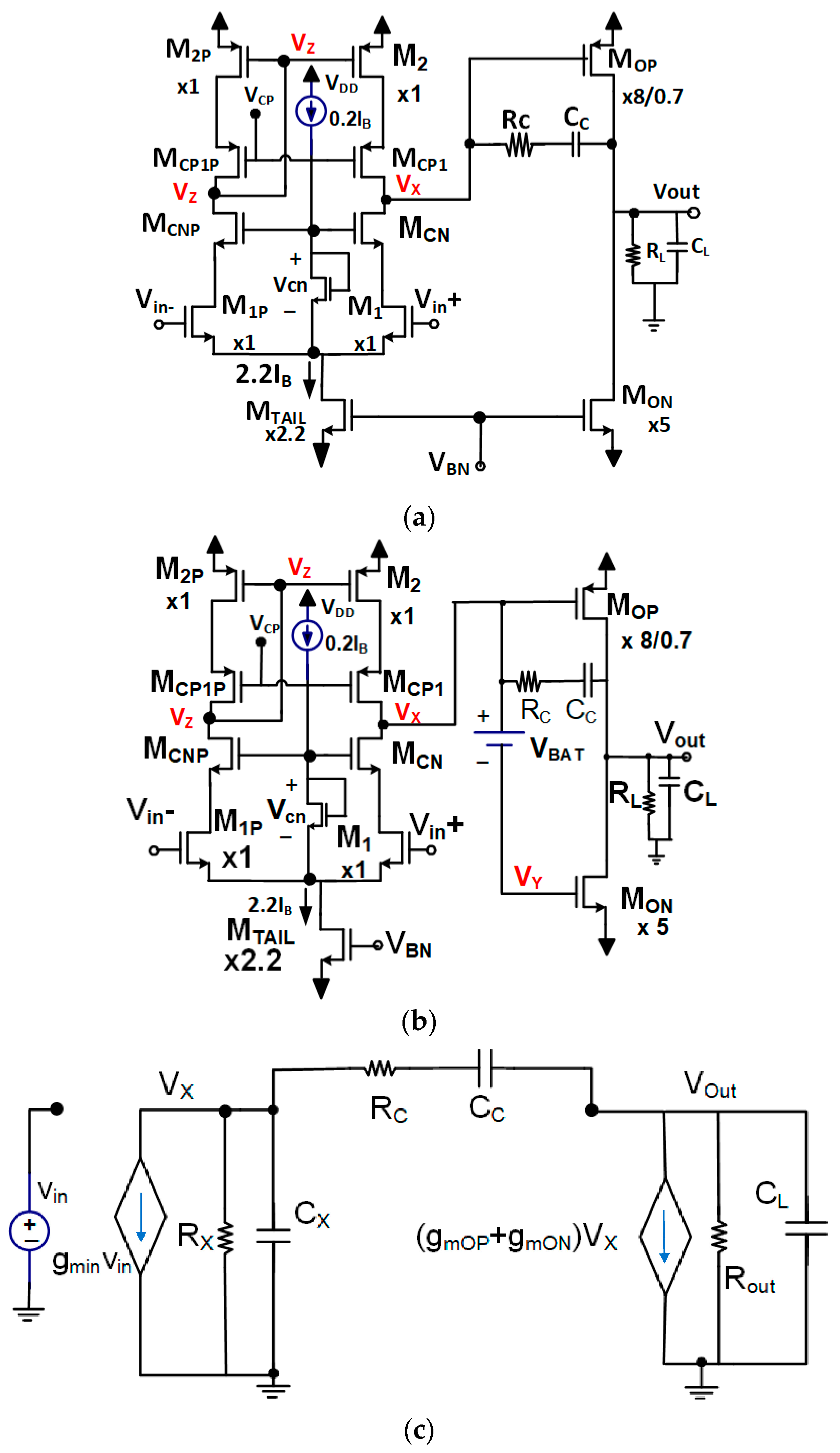
- (a)
- Upon application of a positive step at Vin, the output of the op-amp provides a positive output current to the load. When the output slews in the positive direction, the voltage at node Vx is subject to a large negative variation, which is followed by the voltage at node VY until it reaches the bottom rail. This increases the current in MOP and decreases the current in MON, bringing it to zero for large negative variations in VY that lead to VGSON < VTN, where VTN is the threshold voltage of MON. Since VX can have large negative variations, the peak positive output current IOutpk+ provided by MOP can be much larger than its quiescent current IOutQ, and a large positive slew rate can be achieved. The situation is quite different for negative steps in Vin. In this case, VX has a relatively limited excursion in the positive direction, which is transferred (in practice with some attenuation) to VY. This causes the current in MOP to decrease (and eventually go to zero) and MON to increase. However, given that the variation in the positive direction of Vx and VY is much smaller than the variation in the negative direction, the op-amp’s peak negative output current IOutpk− generated by MON is in general much smaller than IOutpk+. This leads to a negative slew rate, which can be much smaller than the positive slew rate. In practice, the smaller of the two slew rates determines the nominal op-amp’s slew rate SR ≡ MIN{SR+, SR−}.
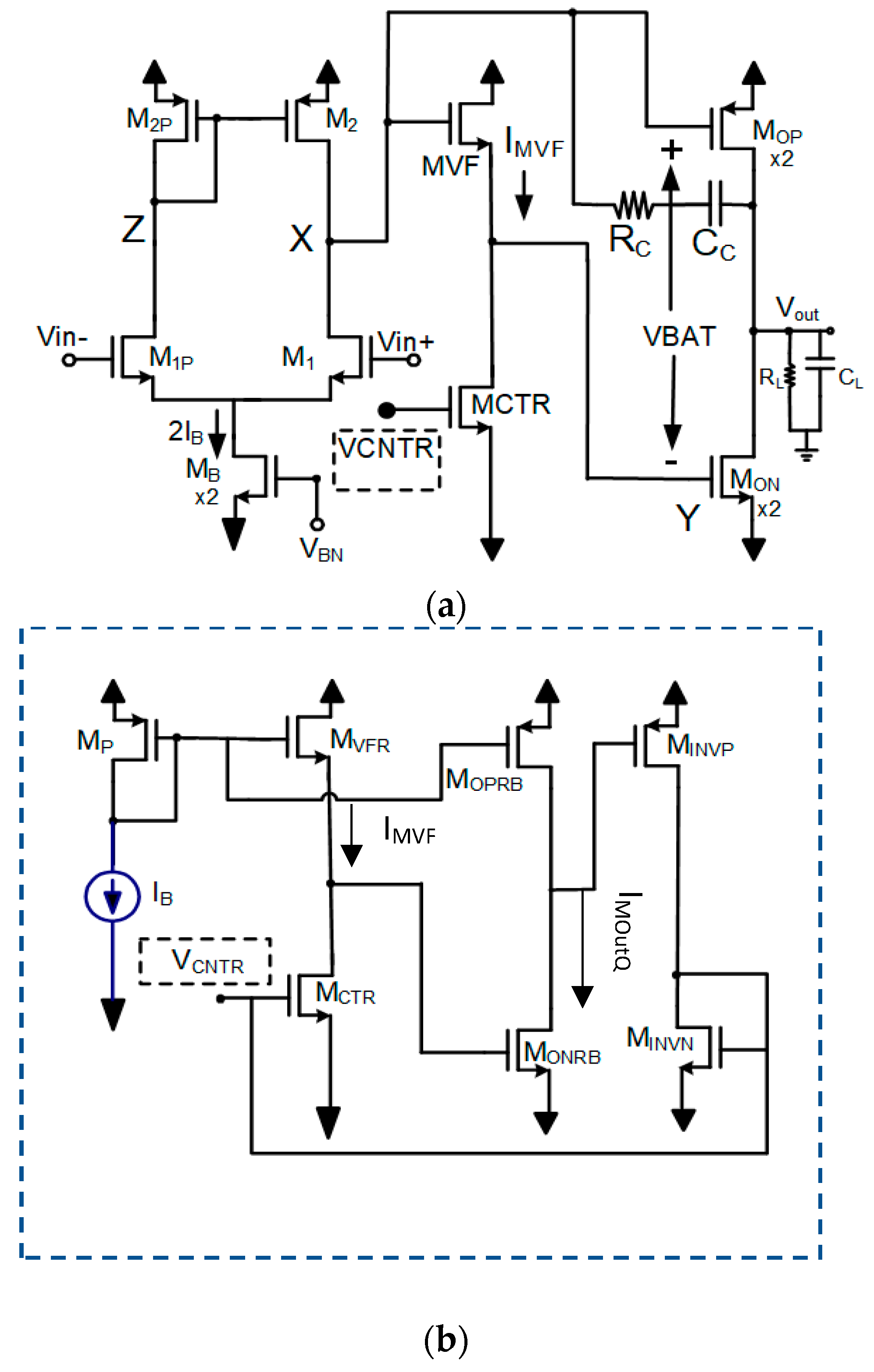
- (b)
- The practical implementation of the floating battery scheme requires a control circuit to achieve the desired nominal output quiescent current IOutQ, which adjusts the value of the floating battery VBAT so that its value is well defined and remains constant, independent of temperature, process parameters, and supply voltage variations. It can be shown that, in saturation, IOutQ is approximately given by, where βN, βP, VTN, and VTP are the NMOS and PMOS output transistors’ gain factors and threshold voltages, respectively. A control circuit to adjust the value of VBAT is required since a fixed VBAT value would lead to large variations in IOutQ with changes in Vsupply, VTN, VTP, βN, or βP. An example of the class-AB output stage using a foating battery and a control circuit is shown in Figure 2. In this example, the floating battery is implemented using a voltage follower. The circuit implementation of both VBAT and the control circuit [7,8] can be relatively complex (as shown in Figure 2b and in the circuit of [8]) and increases the total op-amp’s quiescent current. This can significantly lower the current efficiency of the class-AB op-amp. In some cases, it can also increase the supply requirements beyond the nominal supply voltage of the implementation technology, which has been reduced to sub-volt values (Vsupply < 1 V) in modern CMOS technologies.
2. Circuit Description
2.1. Operation
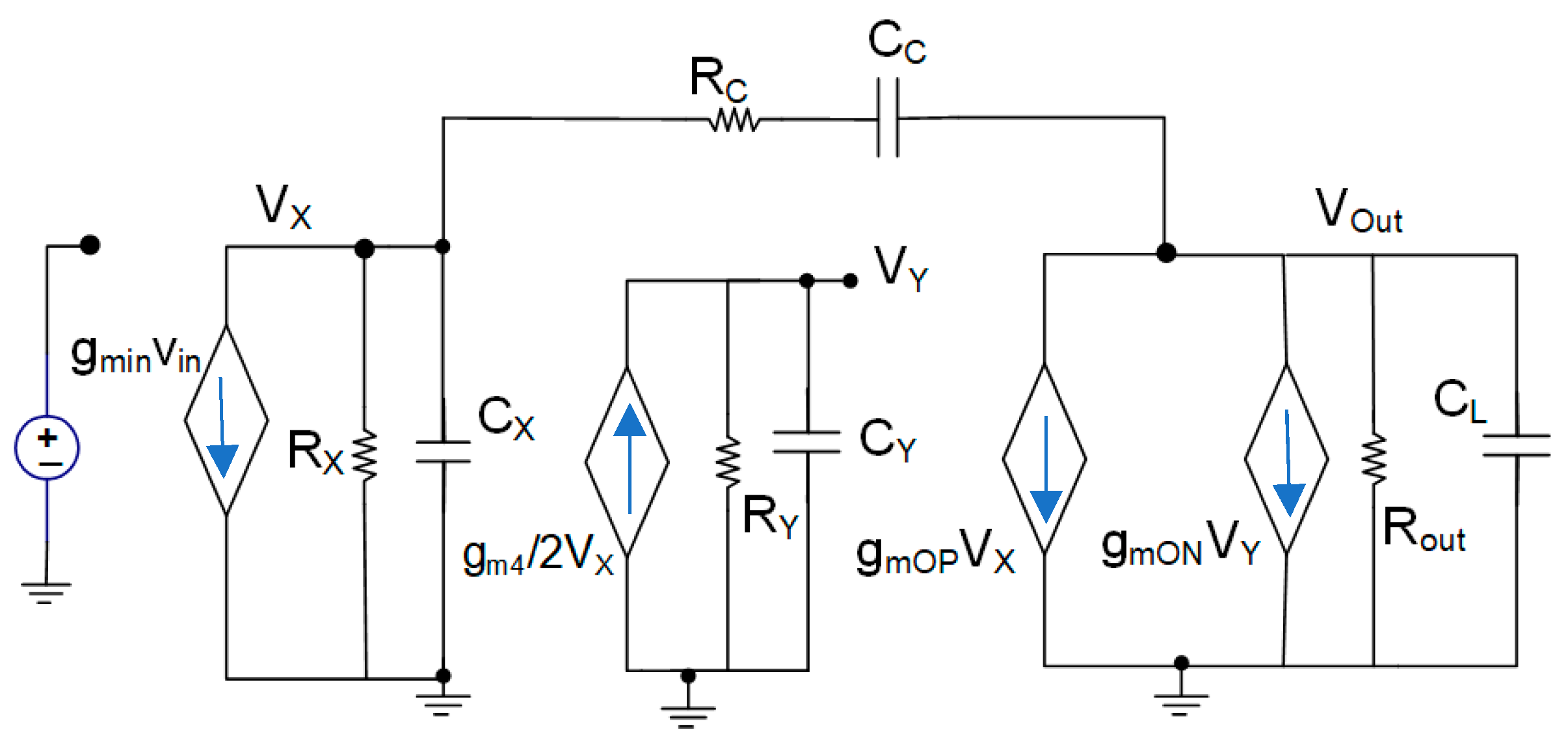
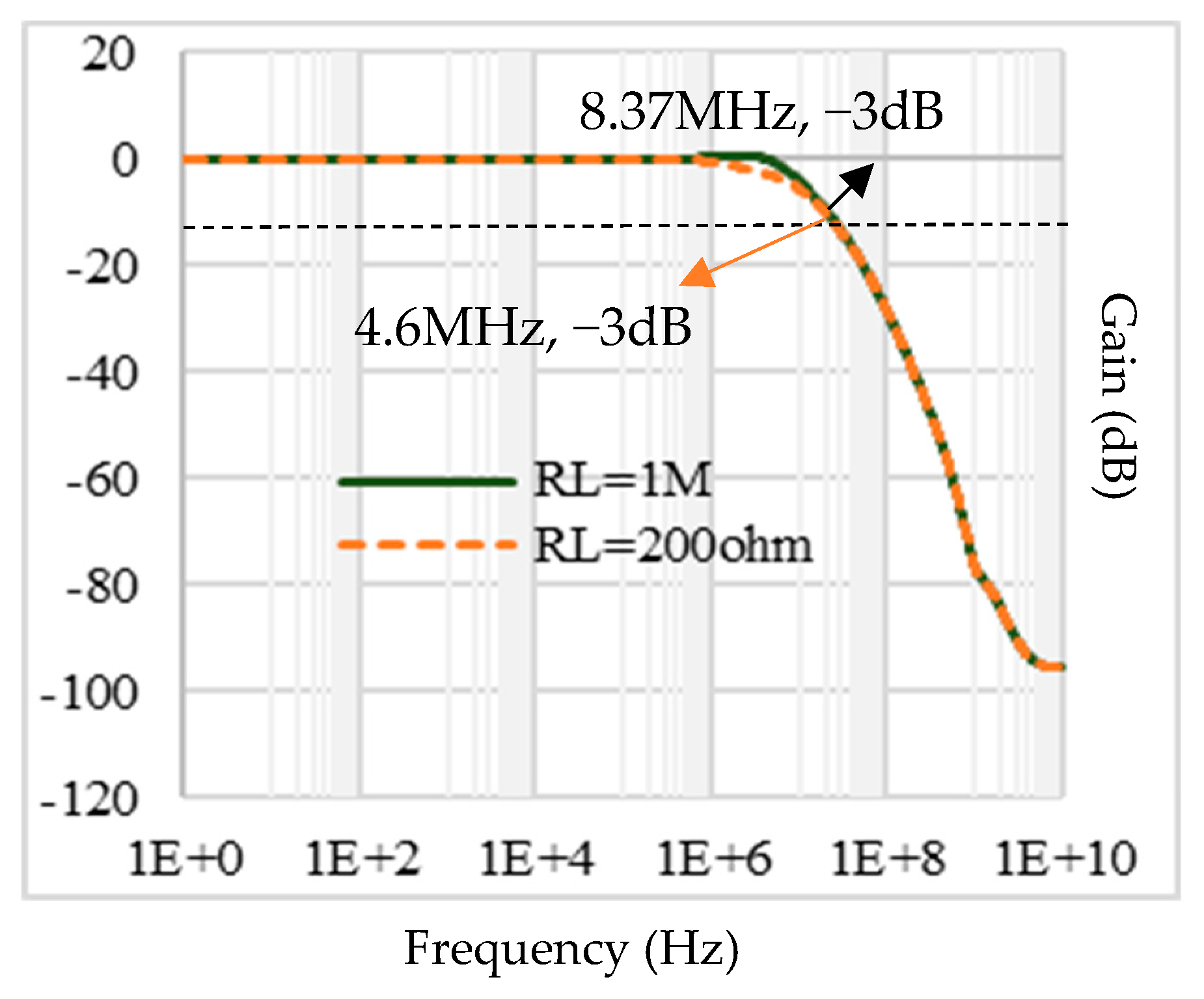
2.2. Frequency Response
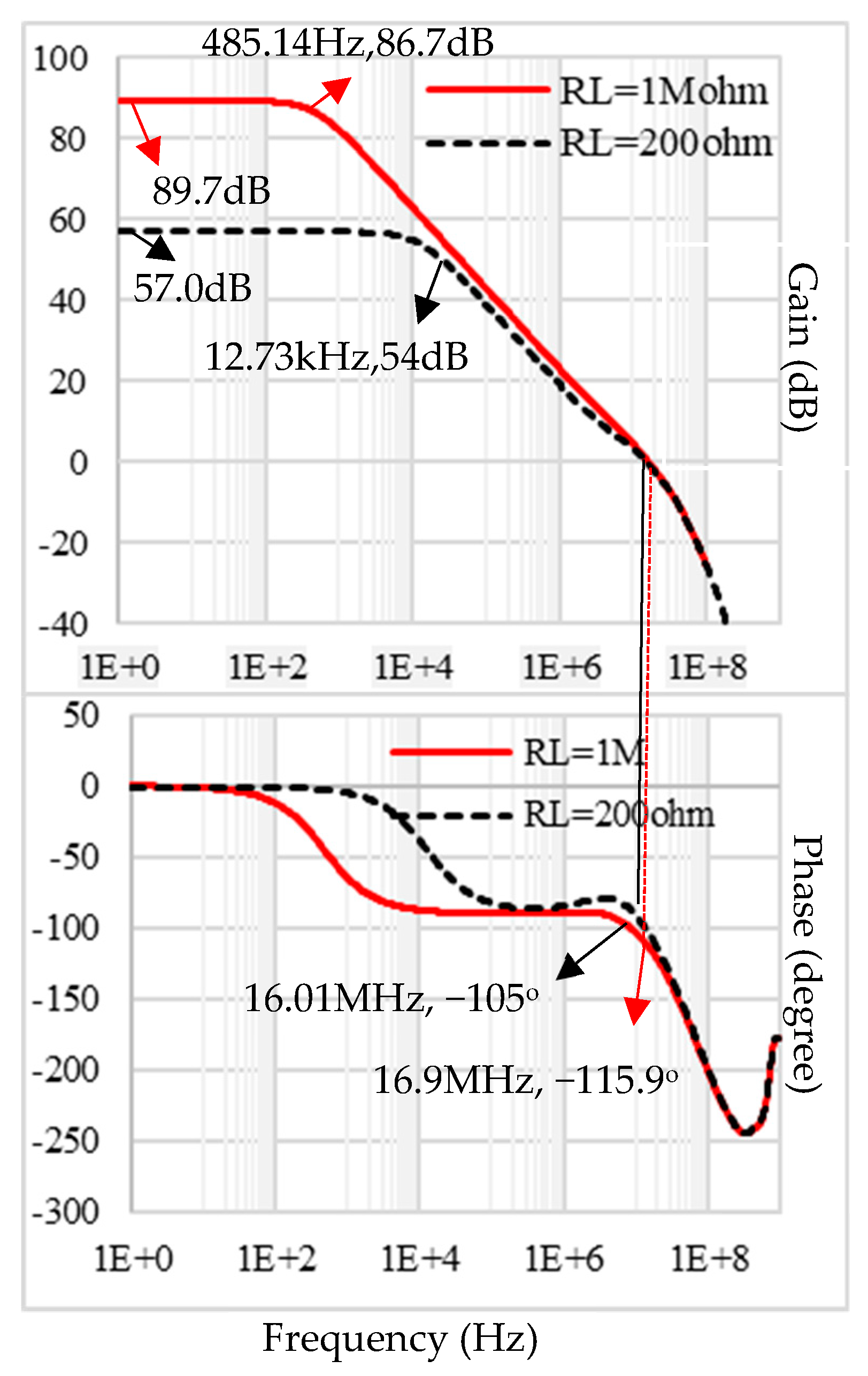
3. Results
4. Conclusions
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Cruz, S.C.D.; Reyes, M.G.T.D.; Gaffud, T.C.; Abaya, T.V.F.; Gusad, M.T.A.; Rosales, M.D. Design and implementation of operational amplifiers with programmable characteristics in a 90 nm CMOS process. In Proceedings of the 2009 European Conference on Circuit Theory and Design, Antalya, Turkey, 23–27 August 2009; pp. 209–212. [Google Scholar]
- Razavi, B. Design of Analog CMOS Integrated Circuits; McGraw-Hill: New York, NY, USA, 2001. [Google Scholar]
- William, S.M.C. Analog Design Essentials; Springer: New York, NY, USA; Berlin, Germany, 2006. [Google Scholar]
- Monticelli, D.M. A quad CMOS single-supply op amp with rail-to-rail output swing. IEEE J. Solid State Circuits 1986, 21, 1026–1034. [Google Scholar] [CrossRef] [Green Version]
- De Langen, K.-J.; Huijsing, J. Compact low-voltage power-efficient operational amplifier cells for VLSI. IEEE J. Solid State Circuits 1998, 33, 1482–1496. [Google Scholar] [CrossRef] [Green Version]
- Gregorian, R.; Temes, G.C. Analog MOS Integrated Circuits for Signal Processing; Wiley Series on Filters: Design Manufacturing and Applications; Wiley-Interscience: New York, NY, USA, 1986. [Google Scholar]
- Torralba, A.; Carvajal, R.G.; Ramirez-Angulo, J.; Tombs, J.; Galán, T. Class AB output stages for low voltage CMOS opamps with accurate quiescent current control by means of dynamic biasing. In Proceedings of the ICECS 2001 8th IEEE International Conference on Electronics, Circuits and Systems (Cat. No.01EX483), Malta City, Malta, 2–5 September 2001; Volume 2, pp. 967–970. [Google Scholar] [CrossRef]
- Padilla-Cantoya, I.; Molinar-Solis, J.E.; Medina-Vazquez, A.S.; Gurrola-Navarro, M.A.; Rizo-Dominguez, L.; Gutierrez-Frias, E.F. Class AB Op-Amp with Accurate Static Current Control for Low and High Supply Voltages. IEEE Trans. Circuits Syst. II Express Briefs 2021, 1. [Google Scholar] [CrossRef]
- Sutula, S.; Dei, M.; Terés, L.; Serra-Graells, F. Variable-Mirror Amplifier: A New Family of Process-Independent Class-AB Single-Stage OTAs for Low-Power SC Circuits. IEEE Trans. Circuits Syst. I Regul. Pap. 2016, 63, 1101–1110. [Google Scholar] [CrossRef] [Green Version]
- Cabrera-Bernal, E.; Pennisi, S.; Grasso, A.D.; Torralba, A.; Carvajal, R.G. 0.7-V Three-Stage Class-AB CMOS Operational Transconductance Amplifier. IEEE Trans. Circuits Syst. I Regul. Pap. 2016, 63, 1807–1815. [Google Scholar] [CrossRef]
- Anisheh, S.M.; Abbasizadeh, H.; Shamsi, H.; Dadkhah, C.; Lee, K.-Y. 98-dB Gain Class-AB OTA with 100 pF Load Capacitor in 180-nm Digital CMOS Process. IEEE Access 2019, 7, 17772–17779. [Google Scholar] [CrossRef]
- Grasso, A.D.; Pennisi, S.; Scotti, G.; Trifiletti, A. 0.9-V Class-AB Miller OTA in 0.35μm CMOS with Threshold-Lowered Non-Tailed Differential Pair. IEEE Trans. Circuits Syst. I Regul. Pap. 2017, 64, 1740–1747. [Google Scholar] [CrossRef]
- Kuo, P.-Y.; Tsai, S.-D. An Enhanced Scheme of Multi-Stage Amplifier with High-Speed High-Gain Blocks and Recycling Frequency Cascode Circuitry to Improve Gain-Bandwidth and Slew Rate. IEEE Access 2019, 7, 130820–130829. [Google Scholar] [CrossRef]
- Renteria-Pinon, M.; Ramirez-Angulo, J.; Diaz-Sanchez, A. Simple Scheme for the Implementation of Low Voltage Fully Differential Amplifiers without Output Common-Mode Feedback Network. J. Low Power Electron. Appl. 2020, 10, 34. [Google Scholar] [CrossRef]


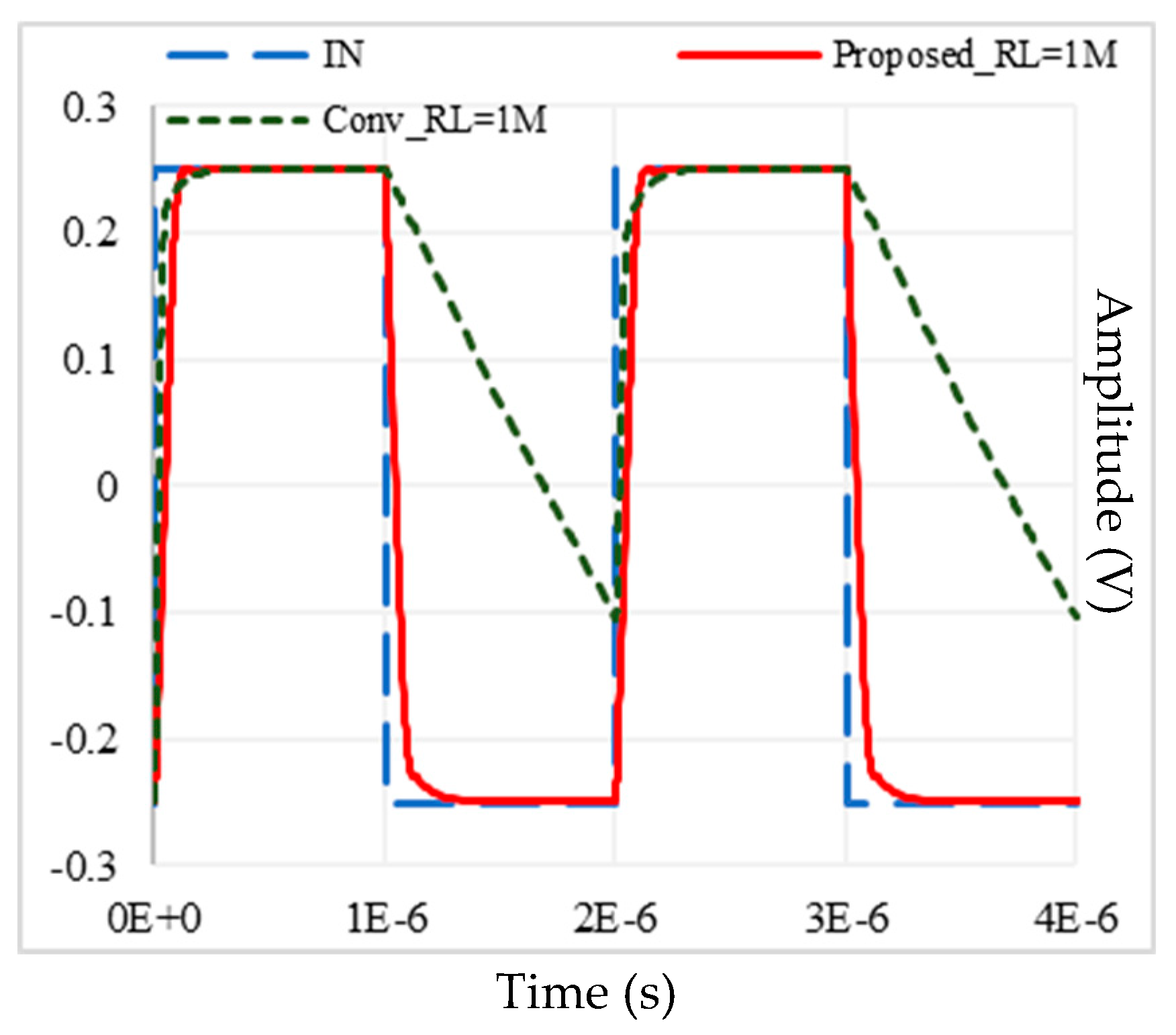

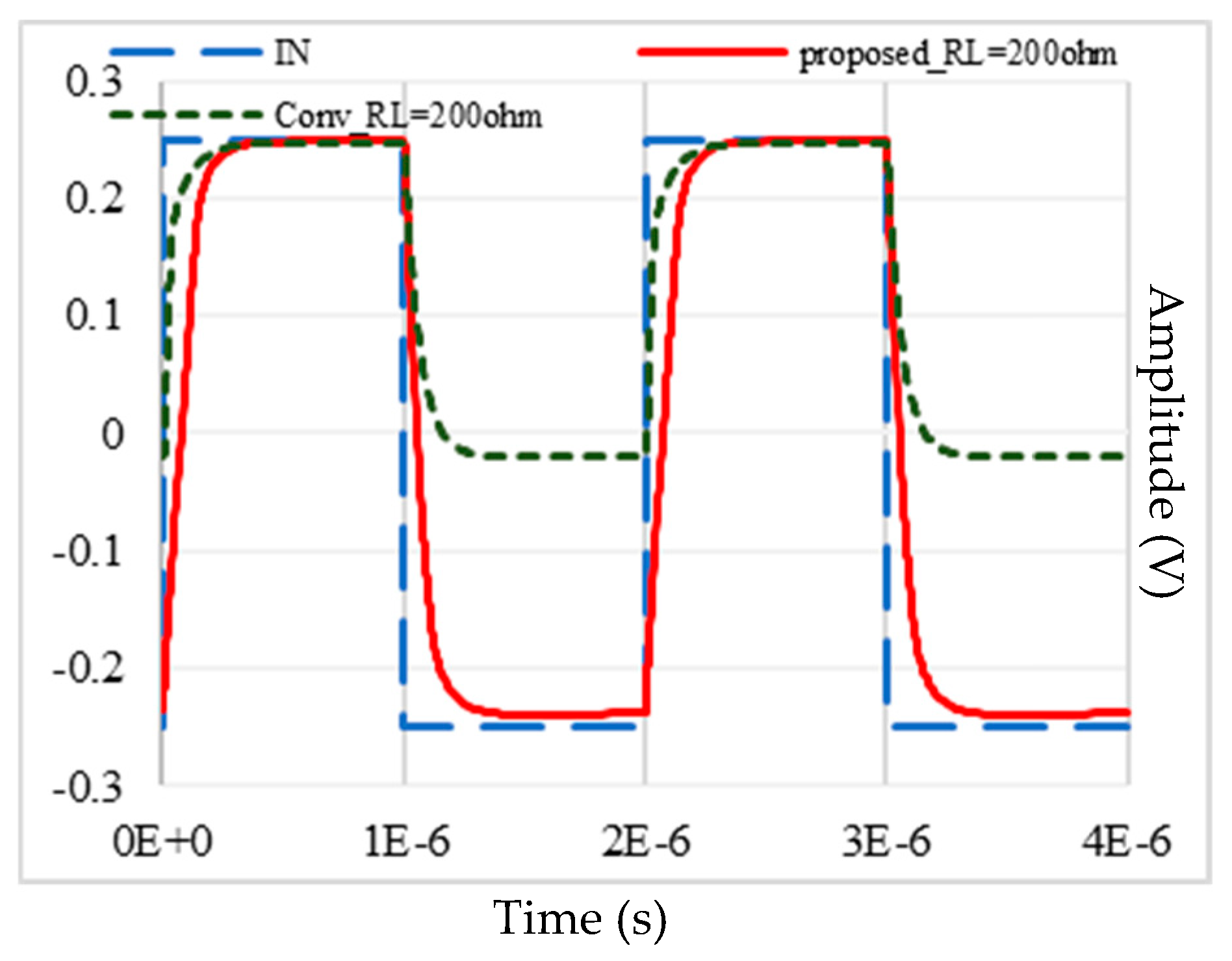
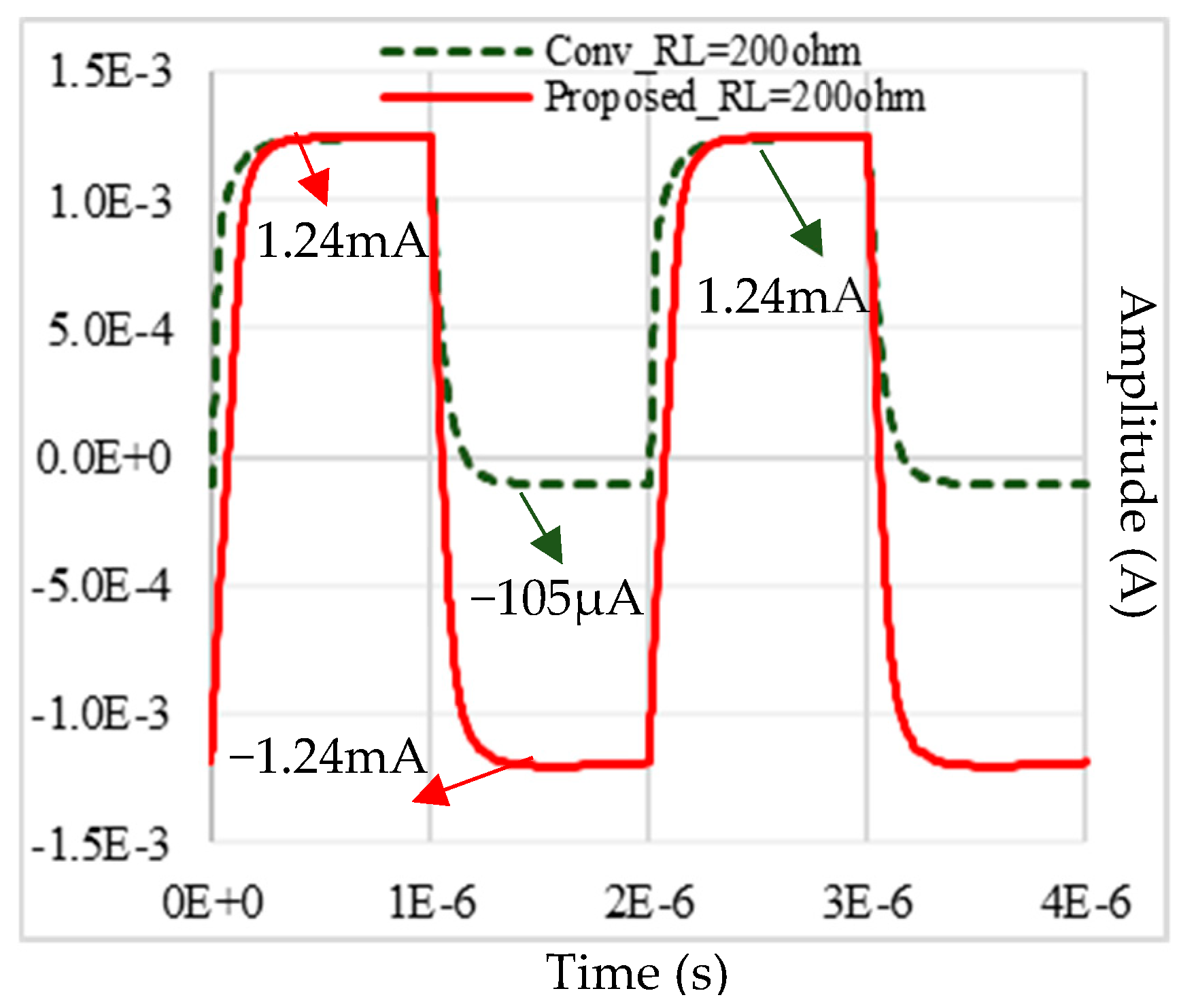
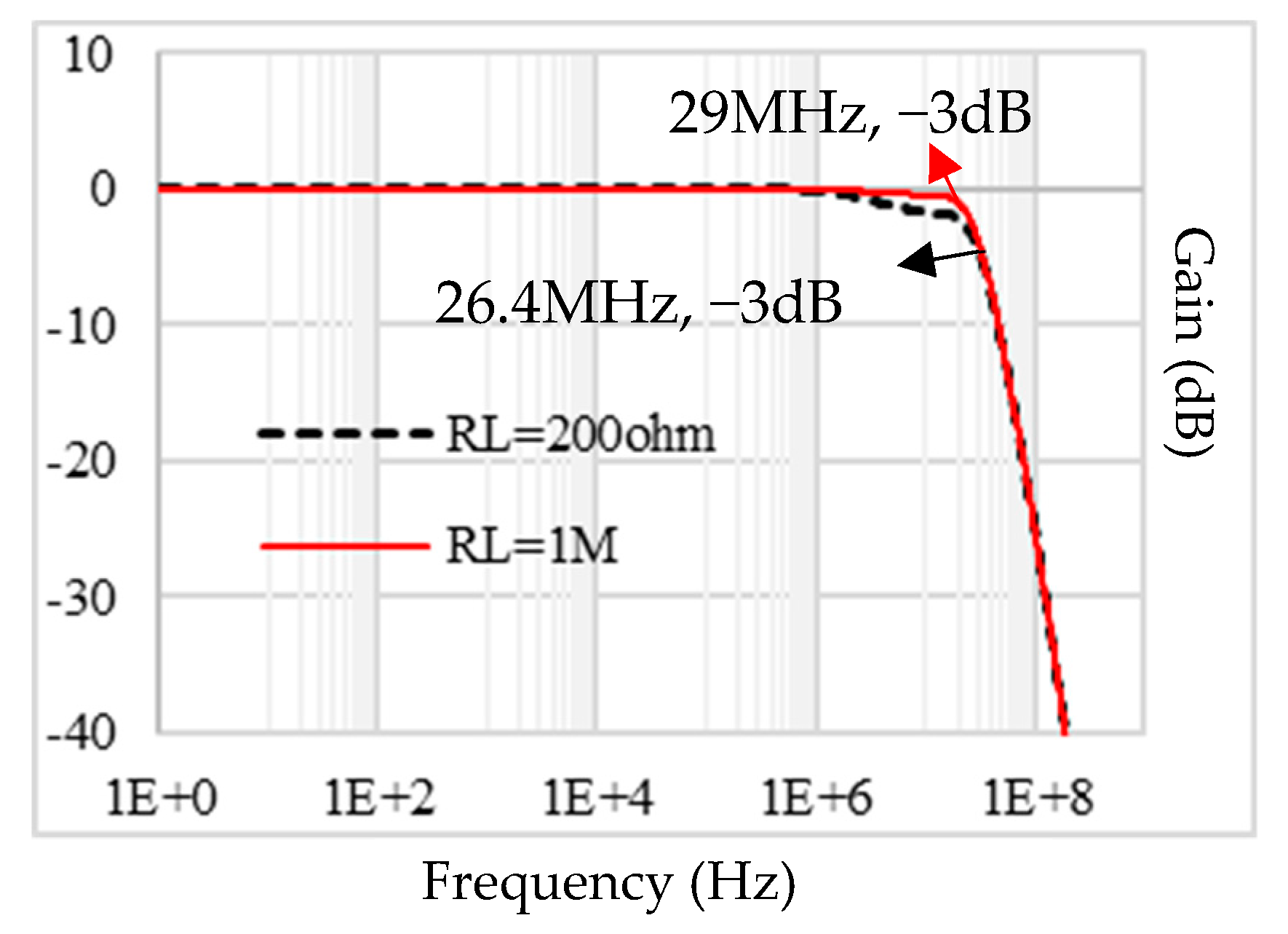
| At T = 27 °C | At T = 120 °C | At T = −20 °C | ||||||||||||||||
|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|
| Corner | tt | ff | fs | sf | ss | SD | tt | ff | fs | sf | ss | SD | tt | ff | fs | sf | ss | SD |
| ITotalQ (µA) | 147 | 148 | 146 | 146 | 146 | 0.8 | 163 | 167 | 163 | 164 | 160 | 2.2 | 135 | 135 | 133 | 134 | 134 | 0.75 |
| THD (dB) at RL = 200 Ω | −66 | −65 | −66 | −62 | −60 | 2.4 | −55 | −50 | −50 | −49 | −52 | 2.13 | −65 | −60 | −61 | −60 | −62 | 1.9 |
| fu (MHz) at RL = 200 Ω | 16.9 | 17 | 16.7 | 16.7 | 16 | 0.3 | 11.62 | 11.12 | 11.36 | 11.59 | 11.83 | 0.24 | 19.14 | 19.4 | 19 | 18.9 | 18.4 | 0.33 |
| PM (°) at RL = 1 MΩ | 64.1 | 63 | 65 | 65 | 67 | 1.31 | 70 | 68 | 72 | 69 | 68 | 1.5 | 62 | 62 | 60 | 62 | 64 | 1.26 |
| Gain | 90 | 87.7 | 89.4 | 85.7 | 90 | 1.65 | 74 | 70 | 78 | 71 | 79 | 3.6 | 91.3 | 90.7 | 91.5 | 90.3 | 90 | 0.57 |
| SR (V/µs) | 7.4 | 7 | 7.1 | 7.7 | 7.1 | 0.25 | 7 | 7.09 | 7.6 | 7.3 | 7.9 | 0.3 | 7.3 | 7.7 | 7.4 | 7.2 | 6.9 | 0.26 |
| Ioutpk−RL = 200 Ω (mA) | 1.24 | 1.23 | 1.24 | 1.23 | 1.24 | 0.004 | 1.19 | 1.2 | 1.21 | 1.15 | 1.20 | 0.02 | 1.24 | 1.23 | 1.1 | 1.25 | 1.24 | 0.06 |
| Parameter (Units) | Proposed | Conv-A | [8] 2020 | [9] 2016 | [10] 2016 | [11] 2019 | [12] 2017 | [13] 2019 | [14] 2020 |
|---|---|---|---|---|---|---|---|---|---|
| Inversion level | SI | SI | SI | SI | SBT | SI | SBT | SI | SBT |
| CMOS process (µm) | 0.13 | 0.13 | 0.5 | 0.18 | 0.18 | 0.18 | 0.35 | 0.18 | 0.18 |
| Supply voltage (V) | ±0.6 | ±0.6 | 1.2/2/2.5 | 1.8 | 0.7 | 1.8 | 0.9 | 1.8 | ±0.3 |
| Capacitive load (pF) | 300 | 300 | 16 | 200 | 20 | 100 | 10 | 5 | 10 |
| Resistive Load (Ω) | 1 M/200 | 1 M/200 | - | - | - | - | - | 1 k | - |
| SR (V/μs) | 7.4 | 0.36 | 3.9/4.3/4.3 | 74.1 | 2.8 | 51 | 0.25 | 13.25 | 8.4 |
| DC gain (dB) | 89.7/57 | 75.8/43.44 | 69/70/70 | 72 | 57.5 | 98 | 65 | 105.5 | 42.2 |
| PM (°) | 64.1/76 | 75/97 | 58/58/58.5 | 50 | 60 | 71 | 60 | 53 | 54 |
| fu (MHz) | 16.9/16.0 | 6.6/5.72 | 8.3/8.4/8.5 | 86.5 | 3 | 21 | 1 | 231.7 | 16.1 |
| CMRR at DC (dB) | 71 at RL = 1 M | 52 at RL = 1 M | 69/70/64 | NA | 19 | - | 45 | - | 85.12 |
| PSRR + at DC (dB) | 109 at RL = 1 M | 69 at RL = 1 M | 73/73/73 | NA | 52.1 | - | - | - | 53.25 |
| PSRR − at DC (dB) | 99 at RL = 1 M | 57 at RL = 1 M | 73/73/72 | NA | 66.4 | - | - | - | 56.89 |
| IOutpk+RL = 200 Ω (µA) | 1241 | 1241 | - | - | - | - | - | 1200 | - |
| IOutpk−RL = 200 Ω (µA) | 1241 | 105 | - | - | - | - | - | - | - |
| ItotQ (µA) | 147 | 133 | 162/166/168 | 6611 | 36.3 | 1666.7 | 27 | 472 | 41.33 |
| Power (μW) | 176 | 159.6 | 244/332/420 | 11900 | 25.4 | 3000 | 24.3 | 850 | 24.8 |
| FOMCEDyn (V.pF/µs.µW) | 12.6 | 0.8 | 0.25/0.21/0.16 | 1.25 | 2.2 | 1.7 | 0.1 | 0.078 | 3.3 |
| FOMSS (MHz.pF/µW) | 28.8/27.2 | 12.4/10.8 | 0.54/0.4/0.3 | 1.45 | 2.36 | 0.7 | 0.4 | 1.4 | 6.49 |
| FOMCEStat (µA/µW) | 7 | 0.66 | - | - | - | - | - | 1.4 | - |
| FOMGlobal | 19 | 3 | 0.4/0.29/0.22 | 1.34 | 2.27 | 1.09 | 0.2 | 1.4 | 4.6 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Paul, A.; Renteria-Pinon, M.; Ramirez-Angulo, J.; Bolaños-Pérez, R.; Vázquez-Leal, H.; Huerta-Chua, J.; Diaz-Sánchez, A. Implementation of Power-Efficient Class AB Miller Amplifiers Using Resistive Local Common-Mode Feedback. J. Low Power Electron. Appl. 2021, 11, 31. https://doi.org/10.3390/jlpea11030031
Paul A, Renteria-Pinon M, Ramirez-Angulo J, Bolaños-Pérez R, Vázquez-Leal H, Huerta-Chua J, Diaz-Sánchez A. Implementation of Power-Efficient Class AB Miller Amplifiers Using Resistive Local Common-Mode Feedback. Journal of Low Power Electronics and Applications. 2021; 11(3):31. https://doi.org/10.3390/jlpea11030031
Chicago/Turabian StylePaul, Anindita, Mario Renteria-Pinon, Jaime Ramirez-Angulo, Ricardo Bolaños-Pérez, Héctor Vázquez-Leal, Jesús Huerta-Chua, and Alejandro Diaz-Sánchez. 2021. "Implementation of Power-Efficient Class AB Miller Amplifiers Using Resistive Local Common-Mode Feedback" Journal of Low Power Electronics and Applications 11, no. 3: 31. https://doi.org/10.3390/jlpea11030031
APA StylePaul, A., Renteria-Pinon, M., Ramirez-Angulo, J., Bolaños-Pérez, R., Vázquez-Leal, H., Huerta-Chua, J., & Diaz-Sánchez, A. (2021). Implementation of Power-Efficient Class AB Miller Amplifiers Using Resistive Local Common-Mode Feedback. Journal of Low Power Electronics and Applications, 11(3), 31. https://doi.org/10.3390/jlpea11030031







