How Mitochondrial Signaling Games May Shape and Stabilize the Nuclear-Mitochondrial Symbiosis
Abstract
Simple Summary
Abstract
1. Introduction
2. Methods
Limitations
3. Results
Signaling Game Equilibria
4. Discussion
- Aging—The adversarial chase dynamics involving mitophagy, senescence, stem cells, apoptosis, necrosis and heteroplasmy reveal their effects via aging in multicellular organisms. Even if apoptosis and stem-cell replacement could in principle promise unbounded longevity, in reality, stem cells in the niche could act as deceptive mutants unwilling to participate in costly signaling (cowardly Casanova conjecture (Private communication, M.Wigler)) and lead to cancer and death. Telomere shortening and double-stranded breaks (in the lagging strand) could lead to complex genome repair, but there remains a hard-limit to lifespan that could not escape the constraints imposed by mitochondrial surveillance. In tissues where apoptosis is not feasible (e.g., neurons, muscles and heart), mitophagy may be necessary, leading to other manifestations of aging;
- Genetics and genomics—To understand the aging phenotypes and their connections to the genotypes, Crick’s Central Dogma and the information flow model postulated by it will need to be further extended. This may require novel algorithms and analyses involving copy numbers of mitochondria. So far, mitochondria have been somewhat overlooked. The modern synthesis (uniting Darwin and Mendel) and Crick’s dogma address the context set by the conventions and constraints agreed between mitochondrion and nucleus. While nuclear genomics data have been growing exponentially and ubiquitously, there is a dearth of data available to model heteroplasmic mitochondrial genomics;
- Technologies—Thus, for a proper translational impact, we would need to perform noninvasive measurements of mitochondrial heteroplasmy by developing systems for (long or medium-range reads) sequencing and mapping. Atomic force microscopy (AFM)-based nanomapping [20] with the imputation of cells of origin of heteroplasmy could offer the breakthrough required;
- Therapies—As we approach new therapies involving modified nuclear genomes, which generally go by the name “cellular therapies”, we will need to pay special attention to the cells’ mitochondria. These therapies will have a significant impact on IVF (in vitro fertilization), stem cell therapies, CRISPR editing and CAR T-based immuno-therapies;
- Diseases—The approaches suggested would change our understanding of a whole range of diseases, such as NF1 (a rare disease involving young adults [21]), neurodegeneration (Parkinson’s, Huntington’s and Alzheimer’s Diseases, and ALS), and cancer. An interesting application could involve a combination therapy synergizing with DDW (Deuterium Depleted Water,) which could affect mitochondrial respiratory chains [22].
5. Conclusions
Supplementary Materials
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Brandenburger, A. The Relationship between Quantum and Classical Correlation in Games. Games Econ. Behav. 2010, 69, 175–183. [Google Scholar] [CrossRef]
- Casey, W.; Massey, S.E.; Mishra, B. How Signalling Games Explain Mimicry at Many Levels: From Viral Epidemiology to Human Sociology. J. R. Soc. Interface 2021, 18, 20200689. [Google Scholar] [CrossRef]
- Cosmides, L.M.; Tooby, J. Cytoplasmic Inheritance and Intragenomic Conflict. J. Theor. Biol. 1981, 89, 83–129. [Google Scholar] [CrossRef]
- Fogel, D.B. Historic Perspective—Nils Barricelli-Artificial Life, Coevolution, Self-Adaptation. IEEE Comput. Intell. Mag. 2006, 1, 41–45. [Google Scholar] [CrossRef]
- Gupta, R.; Kanai, M.; Durham, T.J.; Tsuo, K.; McCoy, J.G.; Kotrys, A.V.; Zhou, W.; Chinnery, P.F.; Karczewski, K.J.; Calvo, S.E.; et al. Nuclear Genetic Control of mtDNA Copy Number and Heteroplasmy in Humans. Nature 2023, 620, 839–848. [Google Scholar] [CrossRef] [PubMed]
- Gutmann, D.H.; Ferner, R.E.; Listernick, R.H.; Korf, B.R.; Wolters, P.L.; Johnson, K.J. Neurofibromatosis Type 1. Nat. Rev. Dis. Primers 2017, 3, 17004. [Google Scholar] [CrossRef] [PubMed]
- Hayashi, T.; Stuchebrukhov, A.A. Electron Tunneling in Respiratory Complex I. Proc. Natl. Acad. Sci. USA 2010, 107, 19157–19162. [Google Scholar] [CrossRef] [PubMed]
- Hoang, D.M.; Pham, P.T.; Bach, T.Q.; Ngo, A.T.; Nguyen, Q.T.; Phan, T.T.; Nguyen, G.H.; Le, P.T.; Hoang, V.T.; Forsyth, N.R.; et al. Stem Cell-Based Therapy for Human Diseases. Signal Transduct. Target. Ther. 2022, 7, 272. [Google Scholar] [CrossRef] [PubMed]
- Hoffmeister, M.; Martin, W. Interspecific Evolution: Microbial Symbiosis, Endosymbiosis and Gene Transfer. Environ. Microbiol. 2003, 5, 641–649. [Google Scholar] [CrossRef] [PubMed]
- Imbrie, A.; Daniels, O.J.; Toner, H. Decoding Intentions. Cent. Secur. Emerg. Technol. 2023. Available online: https://cset.georgetown.edu/publication/decoding-intentions/ (accessed on 11 March 2024).
- Javadov, S.; Kozlov, A.V.; Camara, A.K.S. Mitochondria in Health and Diseases. Cells 2020, 9, 1177. [Google Scholar] [CrossRef]
- Jee, J.; Sundstrom, A.; Massey, S.E.; Mishra, B. What Can Information-Asymmetric Games Tell Us about the Context of Crick’s ‘frozen Accident’? J. R. Soc. Interface/R. Soc. 2013, 10, 20130614. [Google Scholar] [CrossRef]
- Keogh, M.J.; Chinnery, P.F. Mitochondrial DNA Mutations in Neurodegeneration. Biochim. Biophys. Acta 2015, 1847, 1401–1411. [Google Scholar] [CrossRef]
- Massey, S.E.; Mishra, B. Origin of Biomolecular Games: Deception and Molecular Evolution. J. R. Soc. Interface 2018, 15, 20180429. [Google Scholar] [CrossRef]
- Mikheikin, A.; Olsen, A.; Leslie, K.; Russell-Pavier, F.; Yacoot, A.; Picco, L.; Payton, O.; Toor, A.; Chesney, A.; Gimzewski, J.K.; et al. DNA Nanomapping Using CRISPR-Cas9 as a Programmable Nanoparticle. Nat. Commun. 2017, 8, 1665. [Google Scholar] [CrossRef] [PubMed]
- Mitra, A.; Barua, A.; Huang, L.; Ganguly, S.; Feng, Q.; He, B. From Bench to Bedside: The History and Progress of CAR T Cell Therapy. Front. Immunol. 2023, 14, 1188049. [Google Scholar] [CrossRef] [PubMed]
- Picard, M.; Shirihai, O.S. Mitochondrial Signal Transduction. Cell Metab. 2022, 34, 1620–1653. [Google Scholar] [CrossRef] [PubMed]
- Sagan, L. On the Origin of Mitosing Cells. J. Theor. Biol. 1967, 14, 255–274. [Google Scholar] [CrossRef] [PubMed]
- Sharma, P.; Sampath, H. Mitochondrial DNA Integrity: Role in Health and Disease. Cells 2019, 8, 100. [Google Scholar] [CrossRef]
- Smith, A.L.M.; Whitehall, J.C.; Greaves, L.C. Mitochondrial DNA Mutations in Ageing and Cancer. Mol. Oncol. 2022, 16, 3276–3294. [Google Scholar] [CrossRef] [PubMed]
- Sun, E.D.; Michaels, T.C.; Mahadevan, L. Optimal Control of Aging in Complex Networks. Proc. Natl. Acad. Sci. USA 2020, 117, 20404–20410. [Google Scholar] [CrossRef] [PubMed]
- Uddin, F.; Rudin, C.M.; Sen, T. CRISPR Gene Therapy: Applications, Limitations, and Implications for the Future. Front. Oncol. 2020, 10, 1387. [Google Scholar] [CrossRef] [PubMed]
- Zakirova, E.G.; Muzyka, V.V.; Mazunin, I.O.; Orishchenko, K.E. Natural and Artificial Mechanisms of Mitochondrial Genome Elimination. Life 2021, 11, 76. [Google Scholar] [CrossRef] [PubMed]
- Zhang, X.; Gaetani, M.; Chernobrovkin, A.; Zubarev, R.A. Anticancer Effect of Deuterium Depleted Water—Redox Disbalance Leads to Oxidative Stress. Mol. Cell. Proteom. 2019, 18, 2373–2387. [Google Scholar] [CrossRef]
- Zhao, J.; Wang, X.; Huo, Z.; Chen, Y.; Liu, J.; Zhao, Z.; Meng, F.; Su, Q.; Bao, W.; Zhang, L.; et al. The Impact of Mitochondrial Dysfunction in Amyotrophic Lateral Sclerosis. Cells 2022, 11, 2049. [Google Scholar] [CrossRef]
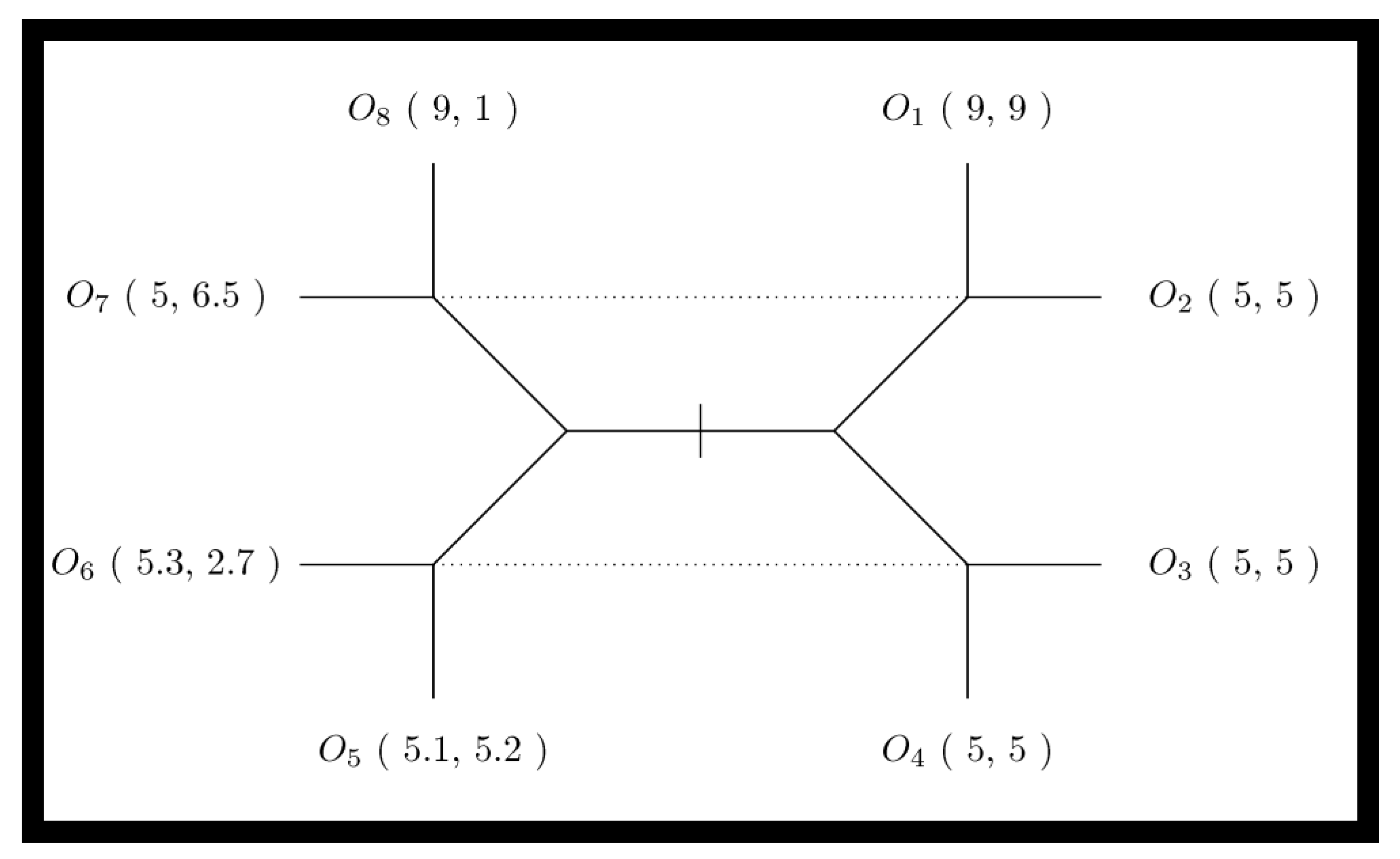
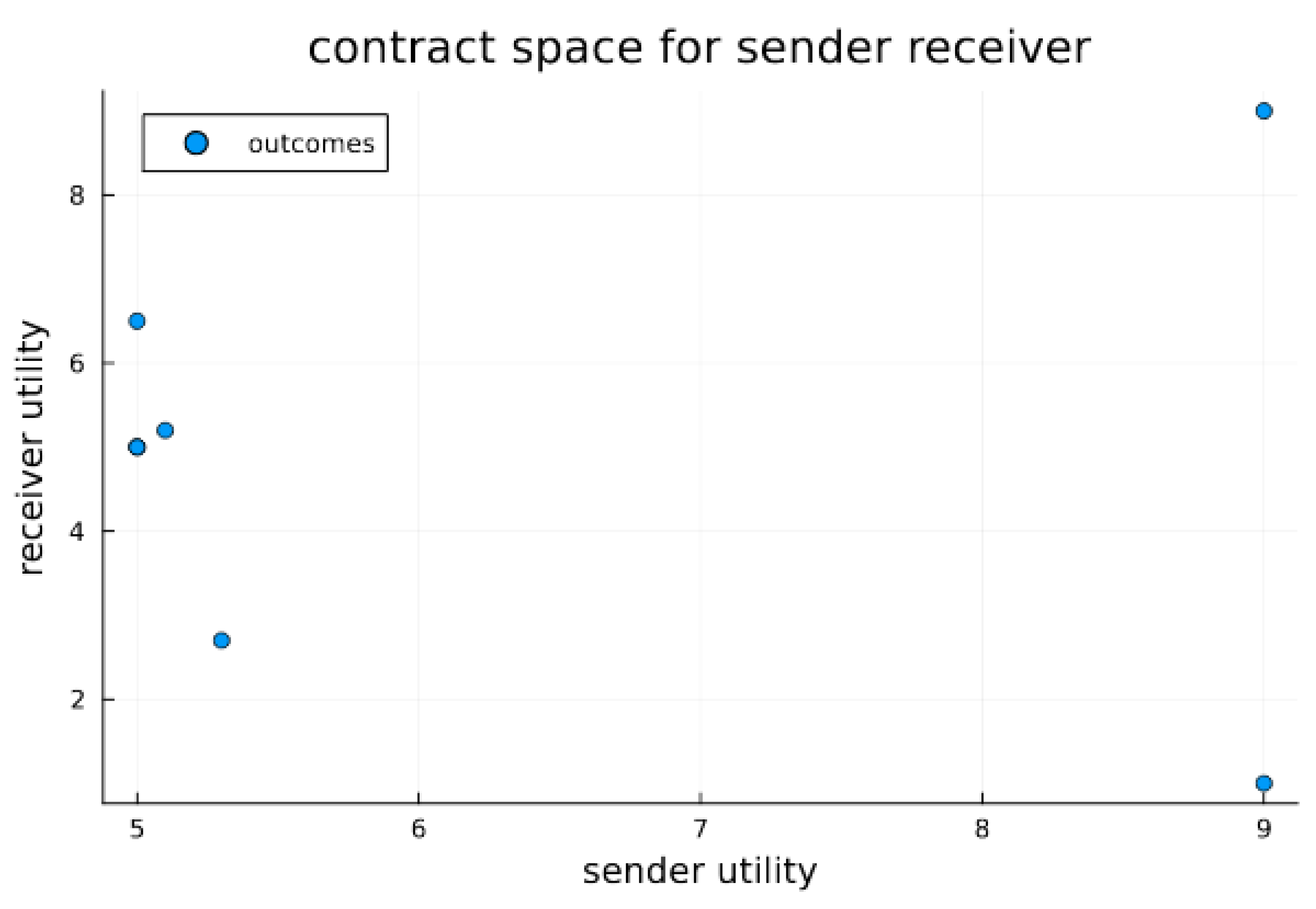


| Parameter | Value | Significance |
|---|---|---|
| Total agents | 10,000 | Fixed population size |
| Generations | 3000 | Time duration of the simulation |
| Signaling Game | Component specified in Figure 1 | |
| Encounters | Component specified in methodology | |
| Domain size | (1, 1) | Singular domain (a single location) for all interactions |
| Cellularization rate: α | 0.0001 | Bernoulli trial for transition to endo-pair, applied to encounters |
| Decellularization rate: β | 0.01 | Bernoulli trial for transition to independent sender/receiver, applied to encounters |
| Mutation rate: μ | 0.01 | Bernoulli trial for uniform random reselection of strategic type |
| Dispersion rate: γ | 0.01 | Dispersion rate, for the Bernoulli trial triggering a random movement to adjacent domain location |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Casey, W.; Kumaran, T.; Massey, S.E.; Mishra, B. How Mitochondrial Signaling Games May Shape and Stabilize the Nuclear-Mitochondrial Symbiosis. Biology 2024, 13, 187. https://doi.org/10.3390/biology13030187
Casey W, Kumaran T, Massey SE, Mishra B. How Mitochondrial Signaling Games May Shape and Stabilize the Nuclear-Mitochondrial Symbiosis. Biology. 2024; 13(3):187. https://doi.org/10.3390/biology13030187
Chicago/Turabian StyleCasey, Will, Thiviya Kumaran, Steven E. Massey, and Bud Mishra. 2024. "How Mitochondrial Signaling Games May Shape and Stabilize the Nuclear-Mitochondrial Symbiosis" Biology 13, no. 3: 187. https://doi.org/10.3390/biology13030187
APA StyleCasey, W., Kumaran, T., Massey, S. E., & Mishra, B. (2024). How Mitochondrial Signaling Games May Shape and Stabilize the Nuclear-Mitochondrial Symbiosis. Biology, 13(3), 187. https://doi.org/10.3390/biology13030187




