Thermomechanical Characterization of CFRPs under Elevated Temperatures for Strengthening Existing Structures
Abstract
1. Introduction
2. Relevant Experiments from the Literature
3. Materials and Methods
4. Results
4.1. At Room Temperature
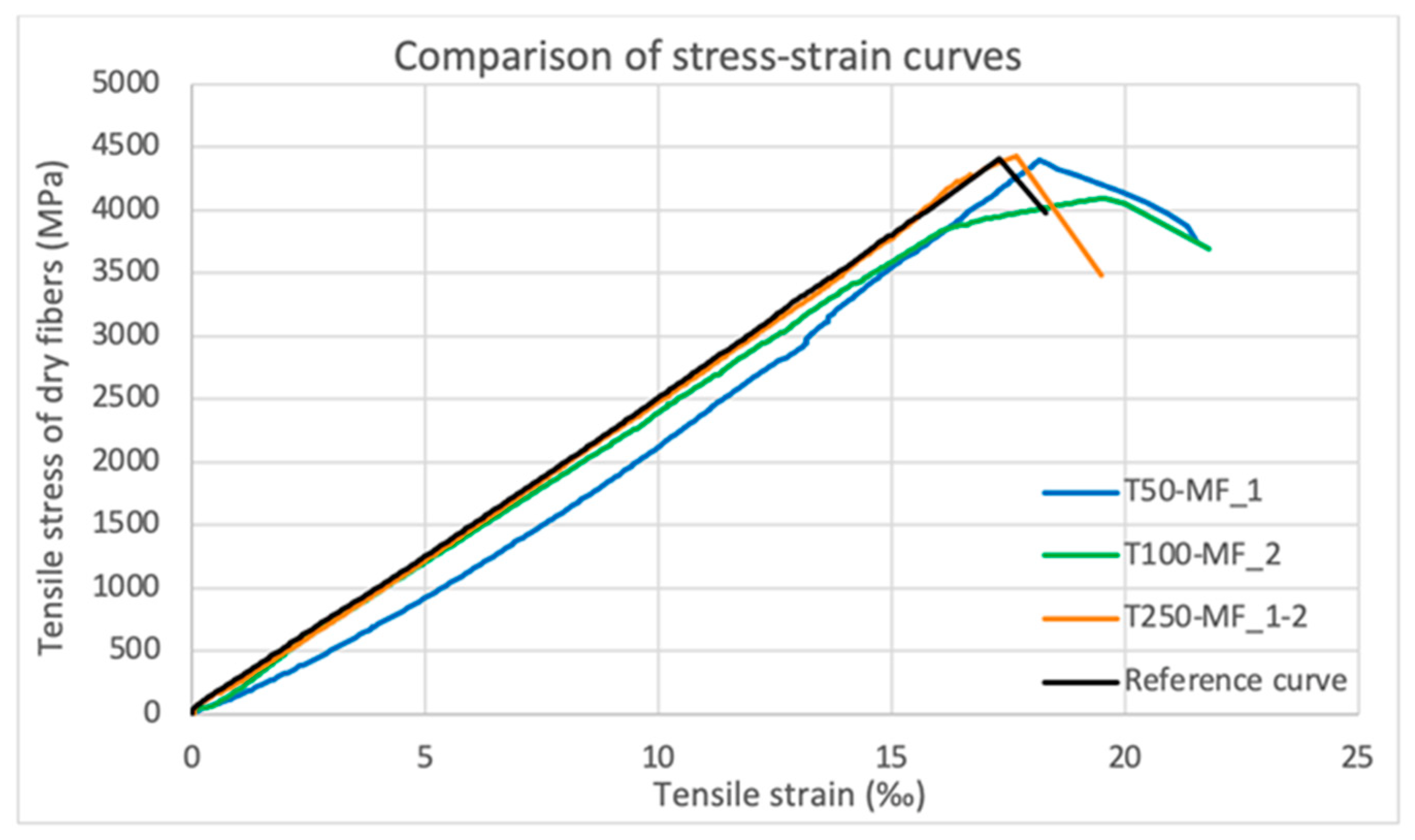
4.2. At Elevated Temperatures
5. Discussion of the Results of the Present Study
5.1. At Room Temperature
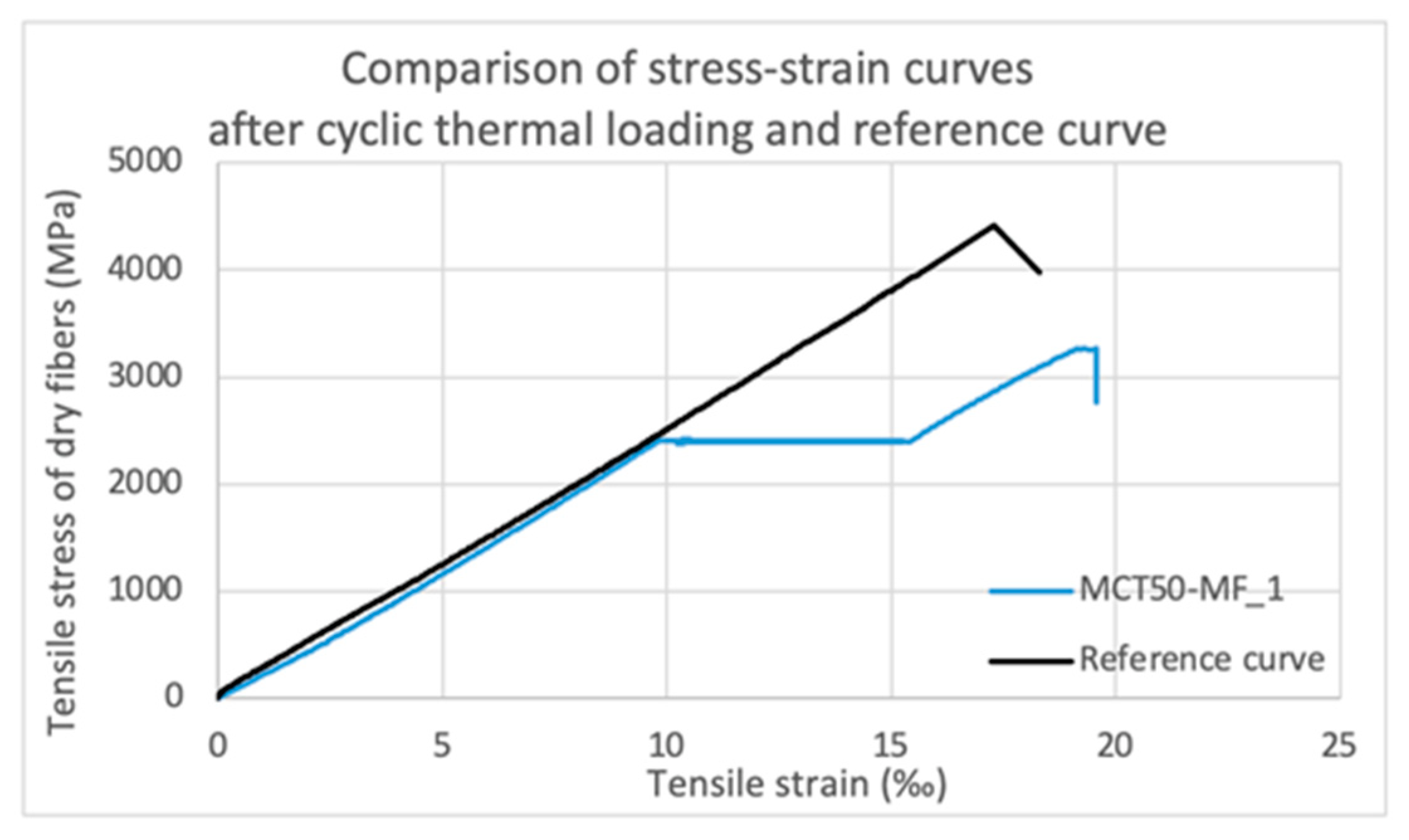
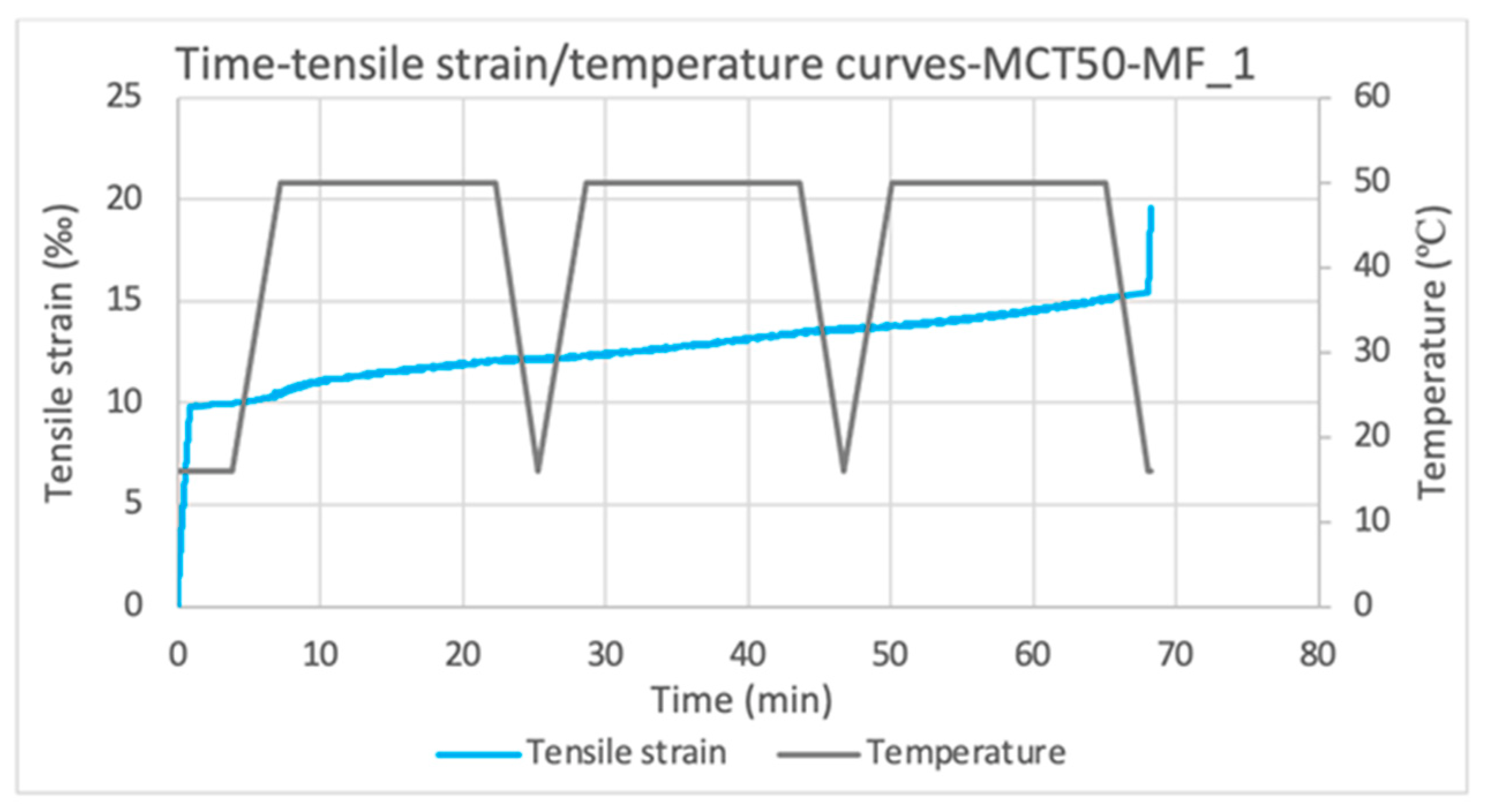
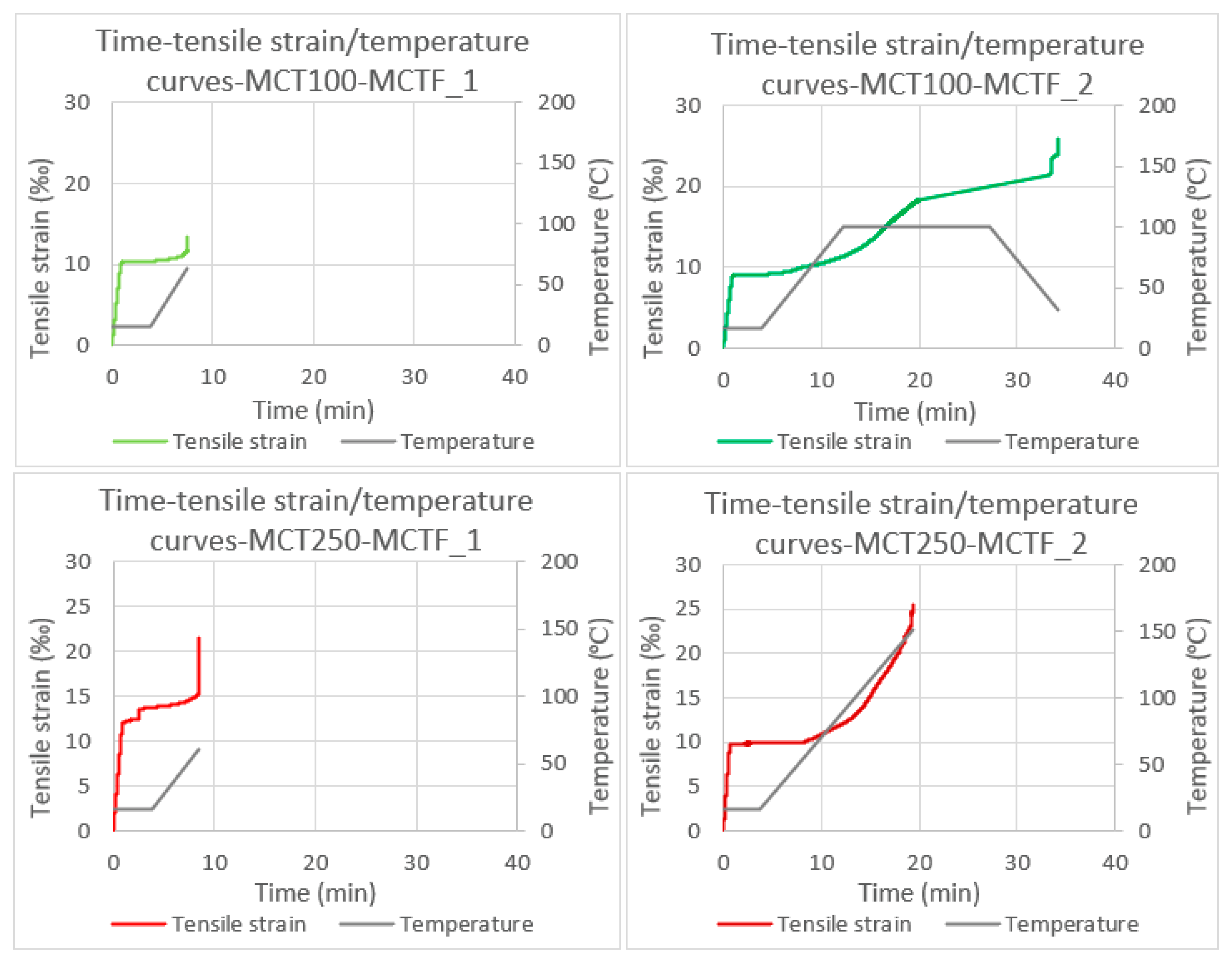
5.2. At Elevated Temperatures
6. Discussion with Results from the Literature
7. Conclusions
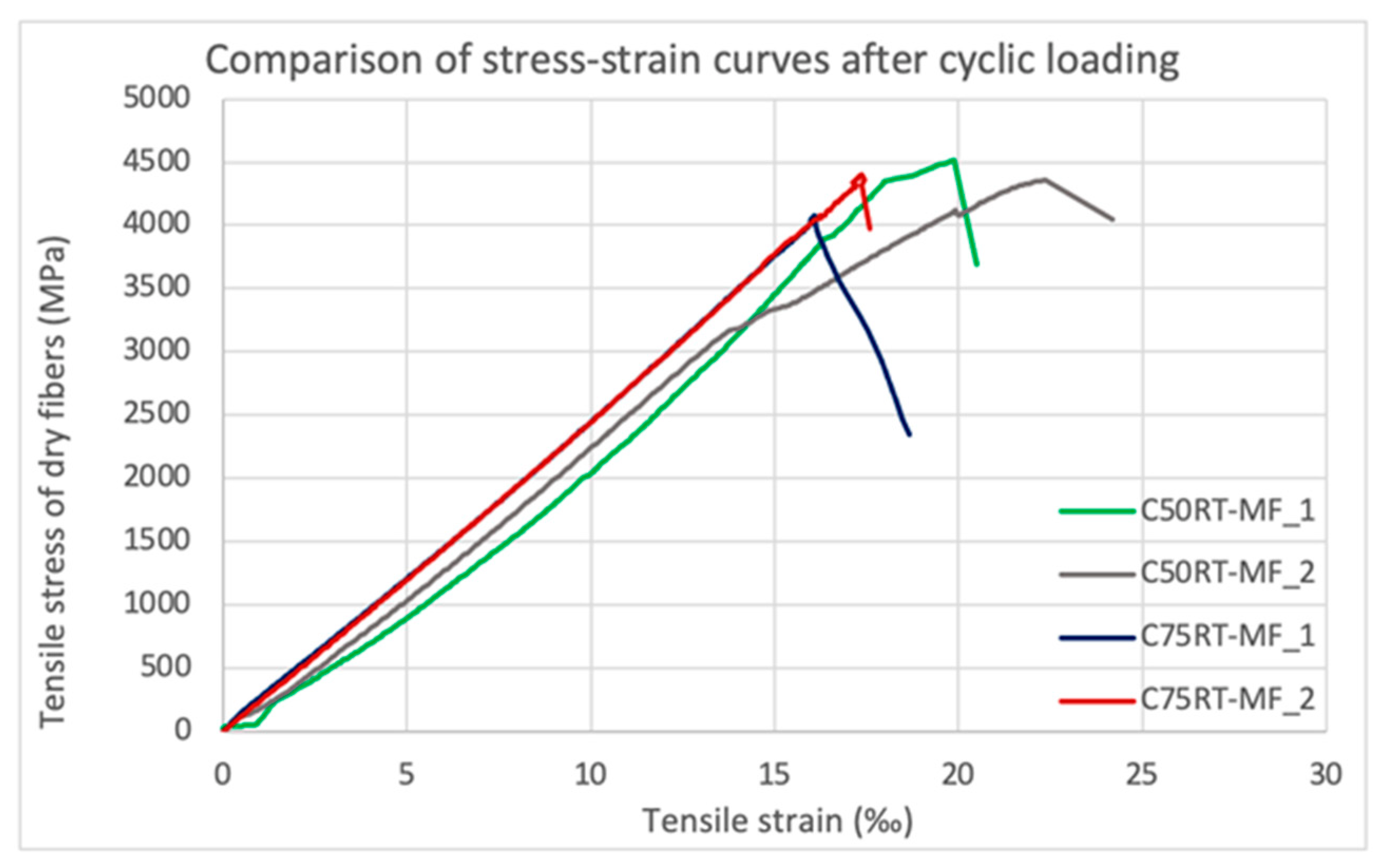
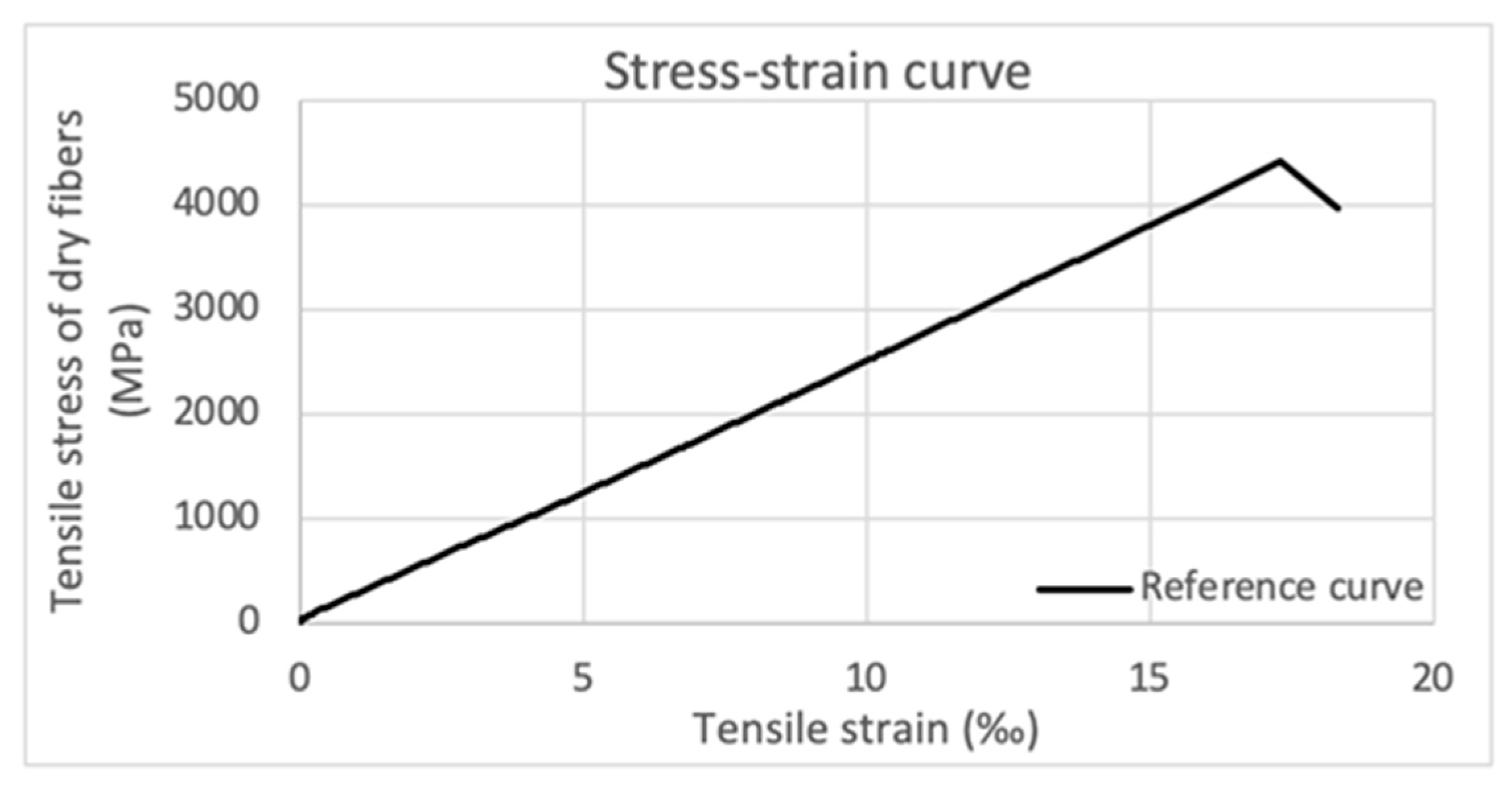
- At room temperature, CFRP laminates show high tensile strength and elastic modulus.
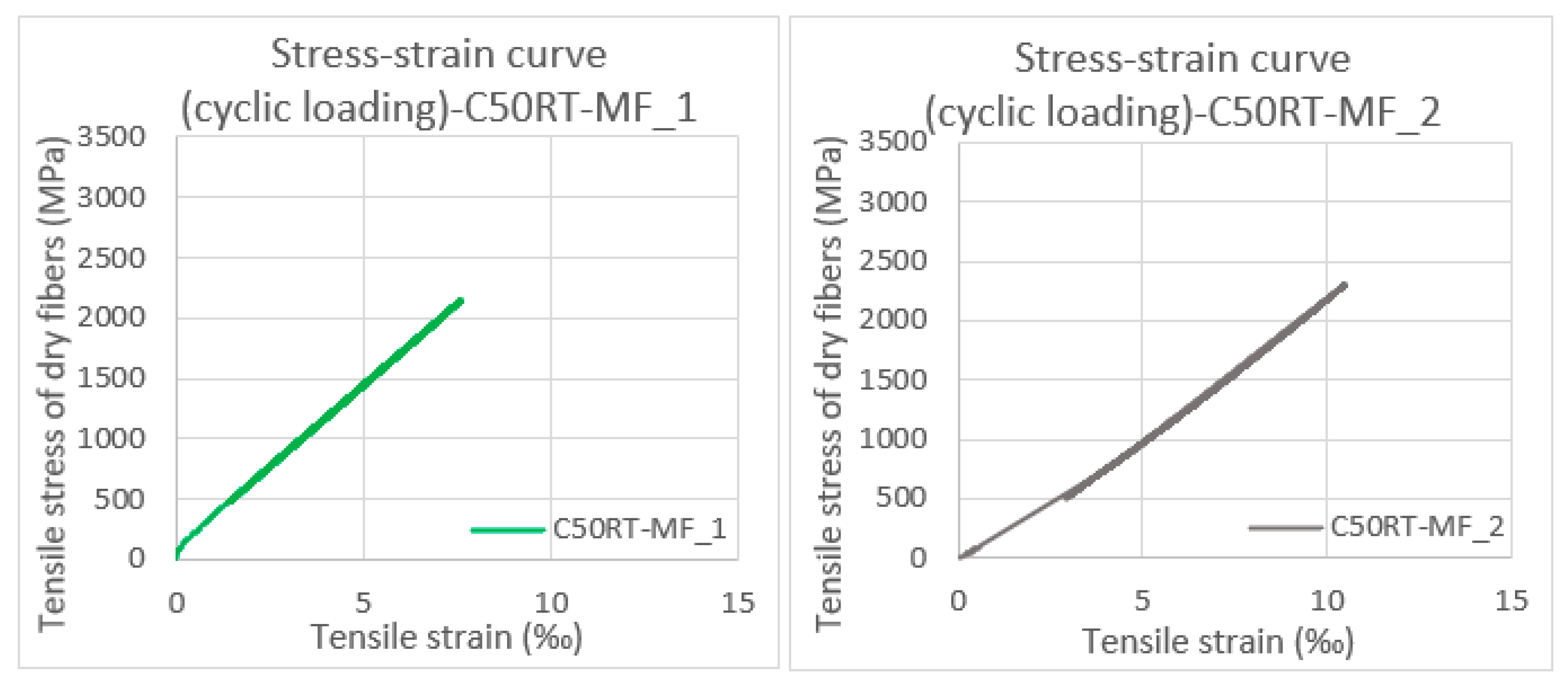
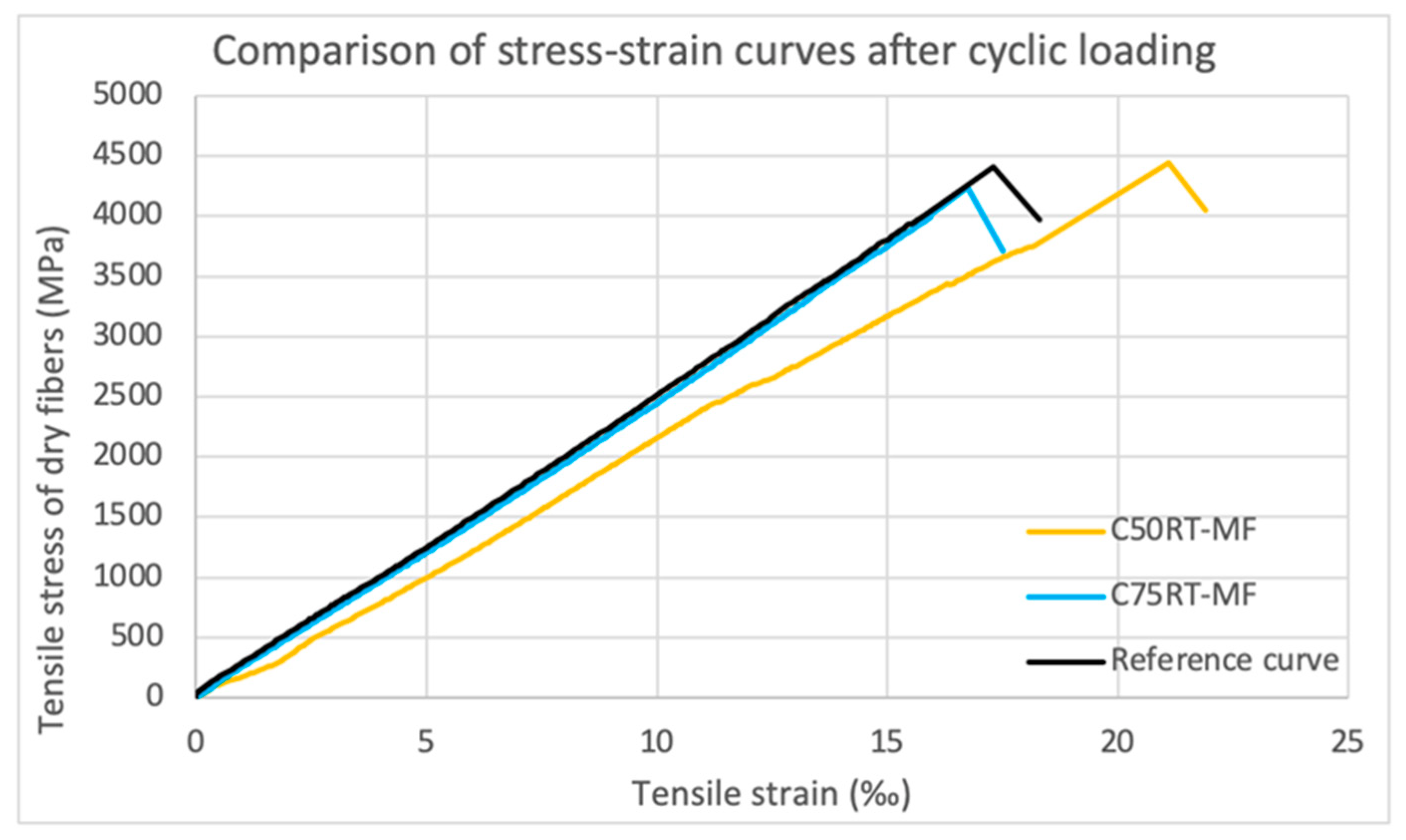
- Low-cycle fatigue at room temperature does not affect the tensile strength of CFRP laminates.
- Low-cycle fatigue at room temperature does not affect the stiffness of the CFRPs, as their elastic moduli remain at the same level.
- When CFRP laminates are exposed to elevated temperatures (100, 250 °C), the viscosity of the resin decreases, and it decomposes easily with the application of loading. In addition, FRPs acquire a dark, black color at 250 °C, depicting the change of matrix composition.
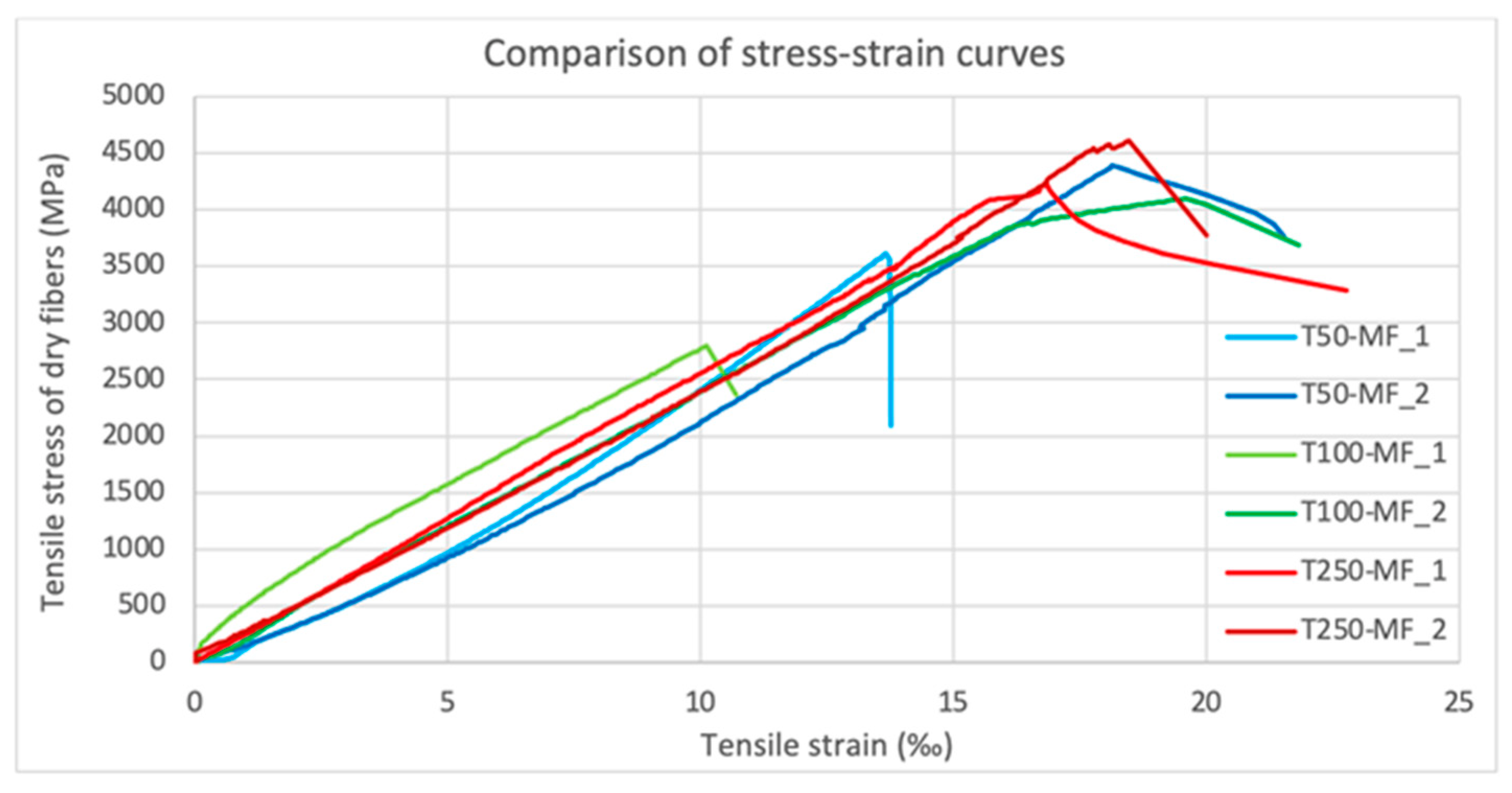
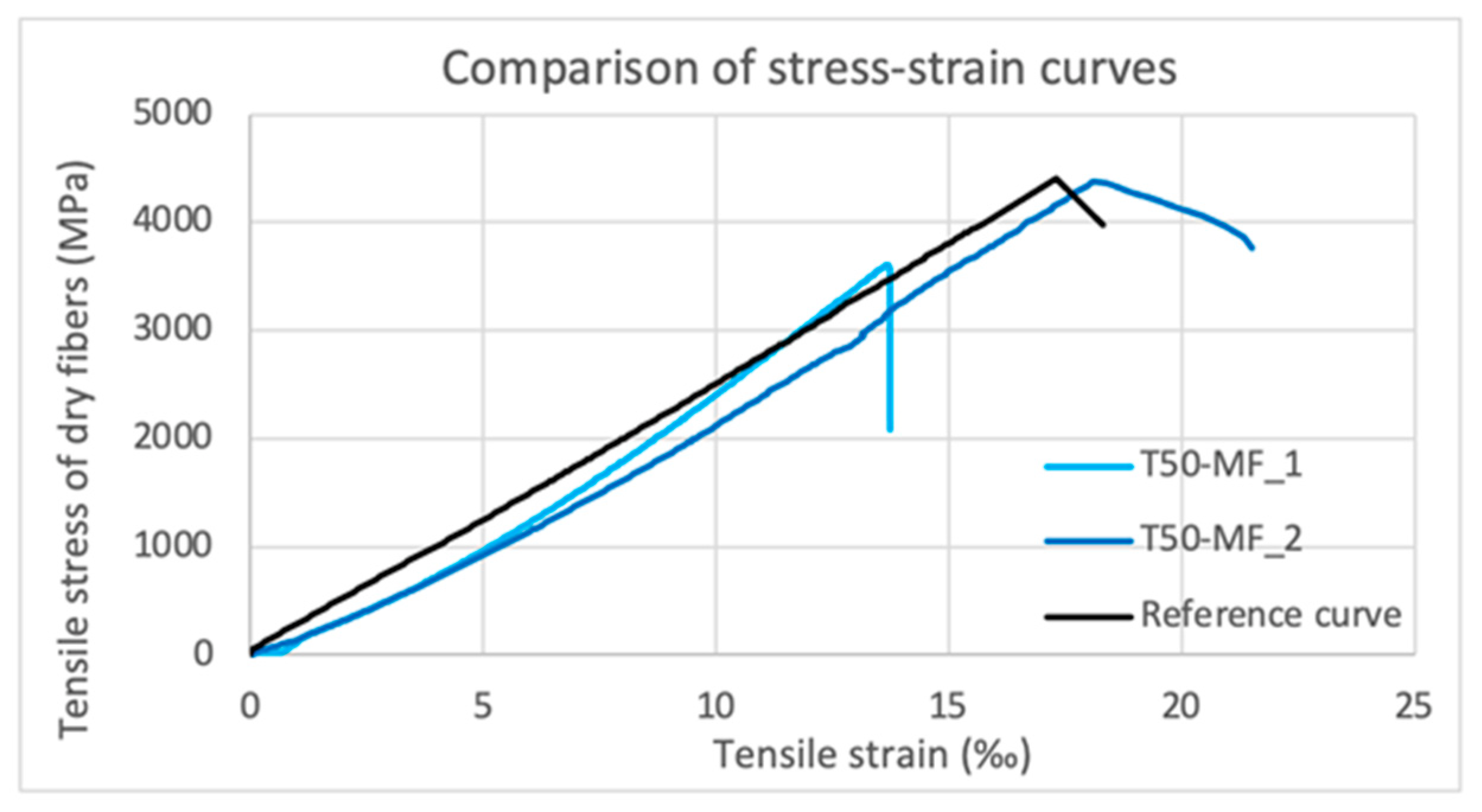
- When CFRP laminates are exposed to elevated temperatures, they show a reduction in their tensile strength. The rate of this reduction was 18.04% after exposure to the temperature of 50 °C.
- CFRPs which were exposed to high temperatures regain their hardness after cooling and the resin is no longer frail and brittle. In addition, their tensile strength does not decrease when they return to normal temperature.
- The cyclic thermal loading when combined with constant tensile load causes reduction in the tensile strength of CFRP laminates after the heating–cooling cycles. The tensile strength of the CFRP laminate that was under cyclic thermal loading of a maximum temperature of 50 °C was reduced by 25.87%.
- The cyclic thermal loading under constant tensile load reduces the elastic modulus of CFRP laminates. The elastic modulus of the CFRP laminate that was under cyclic thermal loading of a maximum temperature of 50 °C was reduced by 33.65%. The higher temperatures the CFRPs are exposed to, the greater the reduction in the elastic modulus.
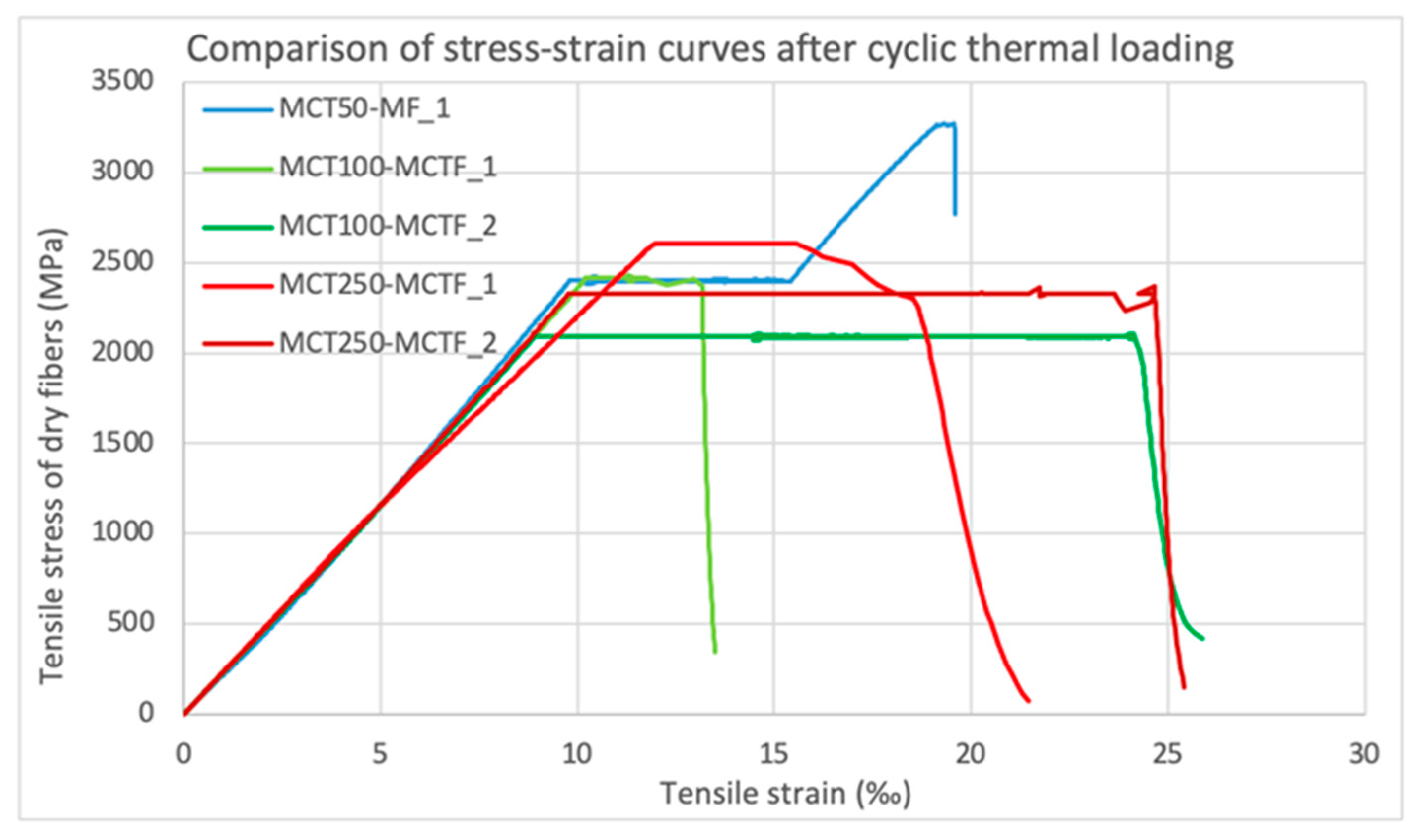
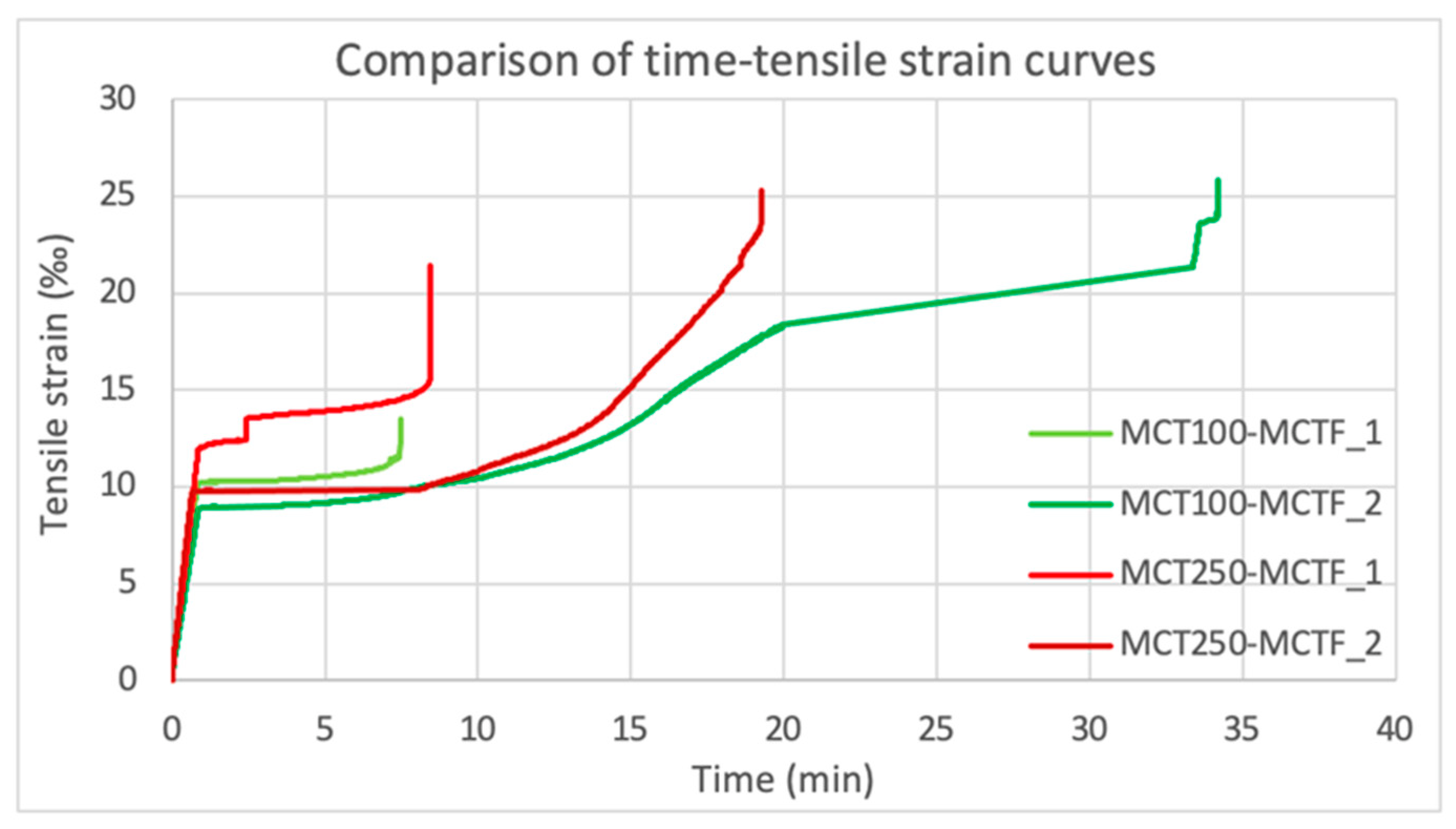
- The longer time the CFRP laminates remain at high temperatures under constant tensile load, the greater the deflections and reductions in their elastic modulus. The ultimate strain of the CFRP laminate that was under cyclic thermal loading of a maximum temperature of 100 °C increased by 39.63%, and the elastic modulus decreased by 65.81%. The ultimate strain of the CFRP laminate that was under cyclic thermal loading of a maximum temperature of 250 °C increased by 36.69% and the elastic modulus decreased by 60.66%.
- CFRPs fail due to fracture of fibers. When they are exposed to higher temperatures of 250 °C, separation of the fibers is also observed during their failure. This happens because of the change in the viscosity of the resin, which is the bonding together of the fibers.
- The measured ultimate stress from the present study is in good agreement with the equivalent results from the literature up to the imposed temperature of 100 °C.
- The measured modulus of elasticity reached a bigger decrease than the equivalent in the literature for the temperature of 100 °C. This reduction factor is 0.82.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Acknowledgments

Conflicts of Interest
Symbolisms
| Afrp | cross-sectional area of the fiber-reinforced polymer (FRP) (mm2) |
| Afibers | cross-sectional area of the FRP’s dry fibers (mm2) |
| Efibers | elastic modulus of the FRP’s dry fibers (GPa) |
| Eave,fibers | average elastic modulus of the FRP’s dry fibers (GPa) |
| Lo | initial length of the FRP (mm) |
| T | thickness of the FRP (mm) |
| tfiber | thickness of the FRP’s dry fiber layer (mm) |
| n | number of the FRP’s dry fiber layers |
| b | width of the FRP (mm) |
| fu,fibers | tensile strength of the FRP’s dry fibers (MPa) |
| fu,ave,fibers | average tensile strength of the FRP’s dry fibers (MPa) |
| εfibers | ultimate strain of the FRP’s dry fibers at the maximum tensile stress of the FRP (‰) |
| εave,fibers | average ultimate strain of the FRP’s dry fibers at the maximum tensile stress of the FRP (‰) |
| rf | tensile strength reduction factor of the FRP’s dry fibers (-) |
| rE | elastic modulus reduction factor of the FRP’s dry fibers (-) |
| Tg | glass transition temperature (°C) |
References
- Naser, M.Z.; Hawileh, R.A.; Abdalla, J.A. Fiber-reinforced polymer composites in strengthening reinforced concrete structures: A critical review. Eng. Struct. 2019, 198, 109542. [Google Scholar] [CrossRef]
- Katakalos, K. Experimental and Numerical Investigation of R/C Beams Strengthened Externally with Open Hoop Strips of Either CFRP or SFRP with or without Anchorage under Monotonic or Cyclic Loadings. Ph.D. Thesis, Aristotle University of Thessaloniki, Thessaloniki, Greece, 2013; pp. 3, 8–9. [Google Scholar]
- Dritsos, S.I. Composite materials in structures. In Proceedings of the 15th Concrete Conference, Technical Chamber of Greece, Technical Chamber of Cyprus, Alexandroupoli, Greece, 25–27 October 2006. [Google Scholar]
- Manos, G.; Katakalos, K. Reinforced Concrete Beams Retrofitted with External CFRP Strips towards Enhancing the Shear Capacity. Appl. Sci. 2021, 11, 7952. [Google Scholar] [CrossRef]
- Talikoti, R.S.; Kandekar, S.B. Strength and Durability Study of Concrete Structures Using Aramid-Fiber-Reinforced Polymer. Fibers 2019, 7, 11. [Google Scholar] [CrossRef]
- Thermou, G.; Katakalos, K.; Manos, G. Influence of the loading rate on the axial compressive behavior of concrete specimens confined with SRG jackets. In Proceedings of the COMPDYN 2013: 4th International Conference on Computational Methods in Structural Dynamics and Earthquake Engineering, Kos Island, Greece, 12–14 June 2013; National Technical University of Athens: Athens, Greece; pp. 1107–1122. [Google Scholar] [CrossRef][Green Version]
- Singh, S.B.; Sethi, A. A review of performance of fibre reinforced polymer strengthened structures under fire exposure. Proc. Indian Natl. Sci. Acad. 2017, 83, 521–532. [Google Scholar]
- Ashrafi, H.; Bazli, M.; Najifabadi, E.P.; Oskouei, A.V. The effect of mechanical and thermal properties of FRP bars on their tensile performance under elevated temperatures. Constr. Build. Mater. 2017, 157, 1001–1010. [Google Scholar] [CrossRef]
- Manos, G.; Katakalos, K.; Papakonstantinou, C. Shear behavior of rectangular beams strengthened with either carbon or steel fiber rein-forced polymers. Appl. Mech. Mater. 2011, 82, 571–576. [Google Scholar] [CrossRef]
- Manos, G.C.; Theofanous, M.; Katakalos, K. Numerical simulation of the shear behaviour of reinforced concrete rectangular beam specimens with or without FRP-strip shear reinforcement. Adv. Eng. Softw. 2014, 67, 47–56. [Google Scholar] [CrossRef]
- Karabinis, A.I.; Pantazopoulou, S. The Use of Composite Materials in the Design and Reinforcement of RC members. Tech. Chron. Sci. Publ. Tech. Chamb. Greece I 2000, 20, 13. [Google Scholar]
- Krzywoń, R. Behavior of EBR FRP strengthened beams exposed to elevated. Procedia Eng. 2017, 193, 297–304. [Google Scholar] [CrossRef]
- Juon, P.H.; Saeedipour, H.; Goh, K.L. Thermal damage in carbon-fiber-reinforced polymer composites: A critical review. Compos. Mater. Manuf. Prop. Appl. 2021, 285–305. [Google Scholar] [CrossRef]
- Najafabadi, E.P.; Oskouei, A.V.; Khaneghahi, M.H.; Shoaei, P.; Ozbakkaloglu, T. The tensile performance of FRP bars embedded in concrete under elevated temperatures. Constr. Build. Mater. 2019, 211, 1138–1152. [Google Scholar] [CrossRef]
- Ray, B.C.; Rathore, D. Durability and integrity studies of environmentally conditioned interfaces in fibrous polymeric composites: Critical concepts and comments. Adv. Colloid Interface Sci. 2014, 209, 68–83. [Google Scholar] [CrossRef]
- Li, Y.; Liu, X.; Wu, M. Mechanical properties of FRP-strengthened concrete at elevated temperature. Constr. Build. Mater. 2017, 134, 424–432. [Google Scholar] [CrossRef]
- Hawileh, R.A.; Abu-Obeidah, A.; Abdalla, J.A.; Al-Tamimi, A. Temperature effect on the mechanical properties of carbon, glass and carbon–glass FRP laminates. Constr. Build. Mater. 2015, 75, 342–348. [Google Scholar] [CrossRef]
- Li, G.; Zhao, J.; Wang, Z. Fatigue Behavior of Glass Fiber-Reinforced Polymer Bars after Elevated Temperatures Exposure. Materials 2018, 11, 1028. [Google Scholar] [CrossRef] [PubMed]
- Gawil, B.; Wu, H.; Elarbi, A. Modeling the Behavior of CFRP Strengthened Concrete Beams and Columns at Different Temperatures. Fibers 2020, 8, 10. [Google Scholar] [CrossRef]
- Spirakos, K. Reinforcement of Structures for Seismic Loads; Technical Chamber of Greece: Athens, Greece, 2004; pp. 170, 175–181. [Google Scholar]
- Cerniauskas, G.; Tetta, Z.; Bournas, D.A.; Bisby, L.A. Concrete confinement with TRM versus FRP jackets at elevated temperatures. Mater. Struct. 2020, 53, 58. [Google Scholar] [CrossRef]
- Bazli, M.; Abolfazli, M. Mechanical Properties of Fibre Reinforced Polymers under Elevated Temperatures: An Overview. Polymers 2020, 12, 2600. [Google Scholar] [CrossRef] [PubMed]
- Hamad, R.J.A.; Johari, M.A.M.; Haddad, R.H. Mechanical properties and bond characteristics of different fiber reinforced polymer rebars at elevated temperatures. Constr. Build. Mater. 2017, 142, 521–535. [Google Scholar] [CrossRef]
- Bai, Y.; Vallée, T.; Keller, T. Modeling of thermal responses for FRP composites under elevated and high temperatures. Compos. Sci. Technol. 2008, 68, 47–56. [Google Scholar] [CrossRef]
- Wang, Y.; Wong, P.; Kodur, V. An experimental study of the mechanical properties of fibre reinforced polymer (FRP) and steel reinforcing bars at elevated temperatures. Compos. Struct. 2007, 80, 131–140. [Google Scholar] [CrossRef]
- Bisby, L.A.; Green, M.F.; Kodur, V.K.R. Response to fire of concrete structures that incorporate FRP. Prog. Struct. Eng. Mater. 2005, 7, 136–149. [Google Scholar] [CrossRef]
- Bisby, L.A. Fire Behaviour of Fibre-Reinforced Polymer (FRP) Reinforced or Confined Concrete. Ph.D. Thesis, Queen’s University, Kingston, ON, Canada, 2003; pp. 34–52. [Google Scholar]
- Al-Abdwais, A.; Al-Mahaidi, R.; Al-Tamimi, A. Performance of NSM CFRP strengthened concrete using modified cement-based adhesive at elevated temperature. Constr. Build. Mater. 2017, 132, 296–302. [Google Scholar] [CrossRef]
- Papakonstantinou, C.; Katakalos, K. Mechanical Behavior of High Temperature Hybrid Carbon Fiber/Titanium Laminates. J. Eng. Mater. Technol. 2009, 131, 021008. [Google Scholar] [CrossRef]
- Saafi, M. Effect of fire on FRP reinforced concrete members. Compos. Struct. 2002, 58, 11–20. [Google Scholar] [CrossRef]
- Sen, R.; Mariscal, D.; Shahawy, M. Durability of fiberglass pretensioned beams. ACI Struct. J. 1993, 90, 525–533. [Google Scholar]
- Gates, T.S. Effects of Elevated Temperature on the Viscoelastic Modeling of Graphite/Polymeric Composites; NASA Technical Memorandum 104160, National Aeronautics and Space Administration: Langley, VA, USA, 1991; p. 29. [Google Scholar]
- Bank, L.C. Properties of FRP Reinforcements for Concrete. In Fibre-Reinforced-Plastic (FRP) Reinforcements for Concrete Structures: Properties and Applications; Nanni, A., Ed.; Elsevier: Amsterdam, The Netherlands, 1993; pp. 59–86. [Google Scholar]
- Kumahara, S.; Masuda, Y.; Tanano, H.; Shimizu, A. Tensile Strength of Continuous Fibre Bar under High Temperature. In Proceedings of the Fibre-Reinforced-Plastic Reinforcement for Concrete Structures: An International Symposium, Vancouver, BC, Canada, 28–31 March 1993; Nanni, A., Dolan, C.W., Eds.; American Concrete Institute: Detroit, MI, USA, 1993; pp. 731–742. [Google Scholar]
- Fujisaki, T.; Nakatsuji, T.; Sugita, M. Research and Development of Grid Shaped FRP Reinforcement. In Proceedings of the Fibre-Reinforced-Plastic Reinforcement for Concrete Structures: An International Symposium, Vancouver, BC, Canada, 28–31 March 1993; Nanni, A., Dolan, C.W., Eds.; American Concrete Institute: Detroit, MI, USA, 1993; pp. 177–192. [Google Scholar]
- Kodur, V.K.R.; Baingo, D. Fire Resistance of FRP Reinforced Concrete Slabs. Natl. Res. Counc. Can. Inst. Res. Constr. 1998, 39. [Google Scholar] [CrossRef]
- Mallick, P.K. Fibre-Reinforced Composites: Materials, Manufacturing and Design; Marcel Dekker Inc.: New York, NY, USA, 2007; pp. 355–361. [Google Scholar]
- Piliafa, S. Investigation of the Thermomechanical for Smart Materials to Monotonic and Low-Cycle Fatigue Conditions. Master’s Thesis, Aristotle University of Thessaloniki, Thessaloniki, Greece, 2020; p. 72. [Google Scholar]

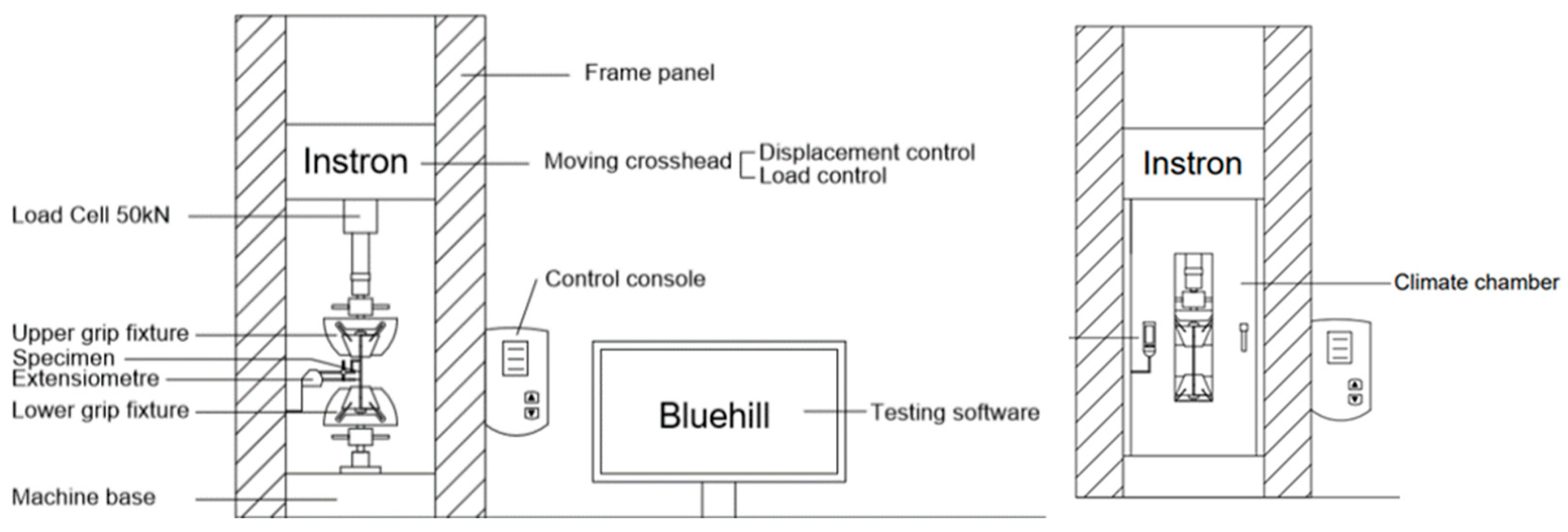



| Name | Loading | T (°C) | Comments |
|---|---|---|---|
| MRT-MF_1 | MUT 1 | RT (=25) | MUT up to failure |
| MRT-MF_2 | MUT | RT | MUT up to failure |
| MRT-MF_3 | MUT | RT | MUT up to failure |
| MRT-MF_4 | MUT | RT | MUT up to failure |
| MRT-MF_5 | MUT | RT | MUT up to failure |
| C50RT-MF_1 | LCF 2, MUT | RT | LCF (50 cycles) with max load 50% of the tensile strength and MUT up to failure |
| C50RT-MF_2 | LCF, MUT | RT | LCF (50 cycles) with max load 50% of the tensile strength and MUT up to failure |
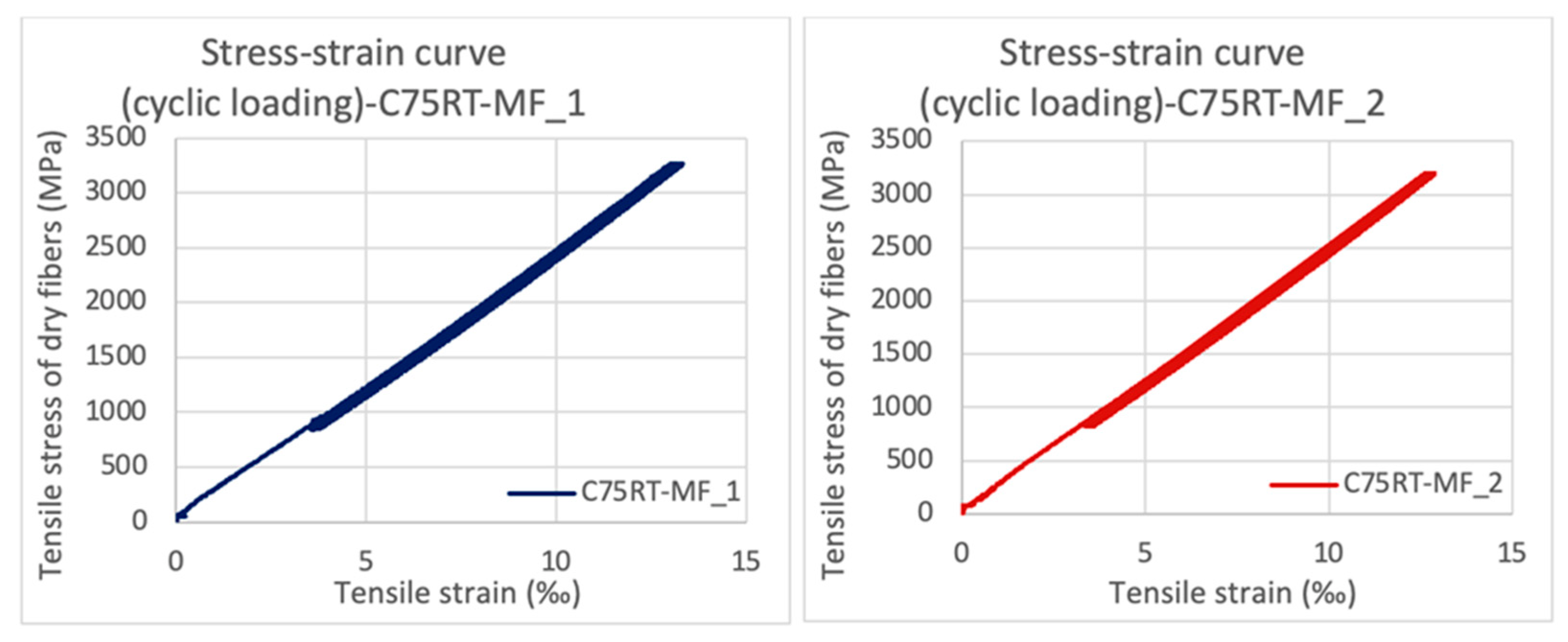
| C75RT-MF_1 | LCF, MUT | RT | LCF (50 cycles) with max load 75% of the tensile strength and MUT up to failure |
| C75RT-MF_2 | LCF, MUT | RT | LCF (50 cycles) with max load 75% of the tensile strength and MUT up to failure |
| Name | Dimensions (mm) | Afrp (mm2) | tfiber (mm) | n | Afibers (mm2) | |
|---|---|---|---|---|---|---|
| t | b | |||||
| MRT-MF_1 | 1.63 | 15.74 | 25.656 | 0.129 | 2 | 4.061 |
| MRT-MF_2 | 1.74 | 17.26 | 30.032 | 0.129 | 2 | 4.453 |
| MRT-MF_3 | 1.71 | 14.68 | 25.103 | 0.129 | 2 | 3.787 |
| MRT-MF_4 | 1.65 | 16.56 | 27.324 | 0.129 | 2 | 4.272 |
| MRT-MF_5 | 1.78 | 15.95 | 28.391 | 0.129 | 2 | 4.115 |
| C50RT-MF_1 | 2.06 | 16.26 | 33.496 | 0.129 | 2 | 4.195 |
| C50RT-MF_2 | 1.74 | 15.15 | 26.361 | 0.129 | 2 | 3.909 |
| C75RT-MF_1 | 1.98 | 16.04 | 31.759 | 0.129 | 2 | 4.138 |
| C75RT-MF_2 | 1.85 | 16.34 | 30.229 | 0.129 | 2 | 4.216 |
| Name | Loading | T (°C) | Comments |
|---|---|---|---|
| T50-MF_1 | TL 1, MUT | 50 | TL (1 cycle) and MUT up to failure |
| T50-MF_2 | TL, MUT | 50 | TL (1 cycle) and MUT up to failure |
| T100-MF_1 | TL, MUT | 100 | TL (1 cycle) and MUT up to failure |
| T100-MF_2 | TL, MUT | 100 | TL (1 cycle) and MUT up to failure |
| T250-MF_1 | TL, MUT | 250 | TL (1 cycle) and MUT up to failure |
| T250-MF_2 | TL, MUT | 250 | TL (1 cycle) and MUT up to failure |
| MCT50-MF_1 | MUT and LCTL 2 | 50 | MUT with constant load 50% of the tensile strength and concurrently LCTL up to failure (max 3 cycles) |
| MCT100-MCTF_1 | MUT and LCTL | 100 | MUT with constant load 50% of the tensile strength and concurrently LCTL up to failure (max 3 cycles) |
| MCT100-MCTF_2 | MUT and LCTL | 100 | MUT with constant load 50% of the tensile strength and concurrently LCTL up to failure (max 3 cycles) |
| MCT250-MCTF_1 | MUT and LCTL | 250 | MUT with constant load 50% of the tensile strength and concurrently LCTL up to failure (max 3 cycles) |
| MCT250-MCTF_2 | MUT and LCTL | 250 | MUT with constant load 50% of the tensile strength and concurrently LCTL up to failure (max 3 cycles) |
| Name | Dimensions (mm) | Afrp (mm2) | tfiber (mm) | n | Afibers (mm2) | |
|---|---|---|---|---|---|---|
| t | b | |||||
| T50-MF_1 | 1.90 | 16.25 | 30.875 | 0.129 | 2 | 4.193 |
| T50-MF_2 | 1.99 | 15.96 | 31.760 | 0.129 | 2 | 4.118 |
| T100-MF_1 | 1.84 | 16.05 | 29.532 | 0.129 | 2 | 4.141 |
| T100-MF_2 | 2.04 | 15.76 | 32.150 | 0.129 | 2 | 4.066 |
| T250-MF_1 | 1.89 | 15.96 | 30.164 | 0.129 | 2 | 4.118 |
| T250-MF_2 | 1.78 | 13.98 | 24.884 | 0.129 | 2 | 3.607 |
| MCT50-MF_1 | 1.73 | 14.52 | 25.120 | 0.129 | 2 | 3.746 |
| MCT100-MCTF_1 | 1.80 | 14.45 | 26.010 | 0.129 | 2 | 3.728 |
| MCT100-MCTF_2 | 1.71 | 16.68 | 28.523 | 0.129 | 2 | 4.303 |
| MCT250-MCTF_1 | 1.73 | 13.40 | 23.182 | 0.129 | 2 | 3.457 |
| MCT250-MCTF_2 | 1.87 | 11.05 | 20.664 | 0.129 | 2 | 2.851 |
| Experiment | 1st Treatment Level | 2nd Treatment Level | No. of Specimens |
|---|---|---|---|
| MRT-MF | Monotonic uniaxial tension (M) at room temperature (RT) up to failure (MF) | - | 5 |
| C50RT-MF | Cycle fatigue of 50 cycles with max load 50% of the tensile strength (C50) at room temperature (RT) | Monotonic uniaxial tension at room temperature up to failure (MF) | 2 |
| C75RT-MF | Cycle fatigue of 50 cycles with max load 75% of the tensile strength (C75) at room temperature (RT) | Monotonic uniaxial tension at room temperature up to failure (MF) | 2 |
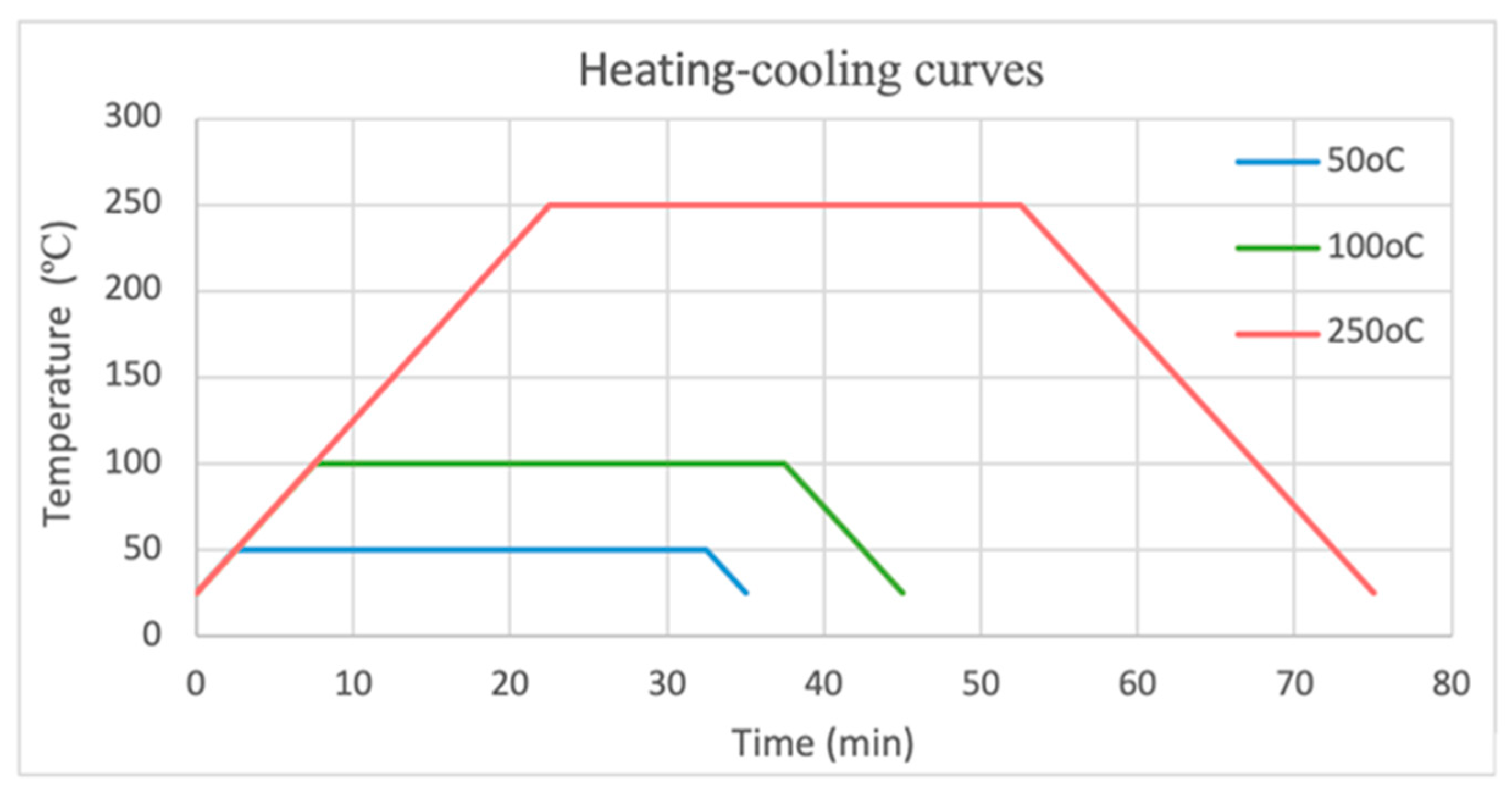
| T50-MF_1 | Thermal loading of 50 °C (T50) for 30 min | Monotonic uniaxial tension at 50 °C up to failure (MF) | 1 |
| T50-MF_2 | Thermal loading of 50 °C (T50) for 30 min and cooling process | Monotonic uniaxial tension at room temperature up to failure (MF) | 1 |
| T100-MF | Thermal loading of 100 °C (T100) for 30 min and cooling process | Monotonic uniaxial tension at room temperature up to failure (MF) | 2 |
| T250-MF | Thermal loading of 250 °C (T250) for 30 min and cooling process | Monotonic uniaxial tension at room temperature up to failure (MF) | 2 |
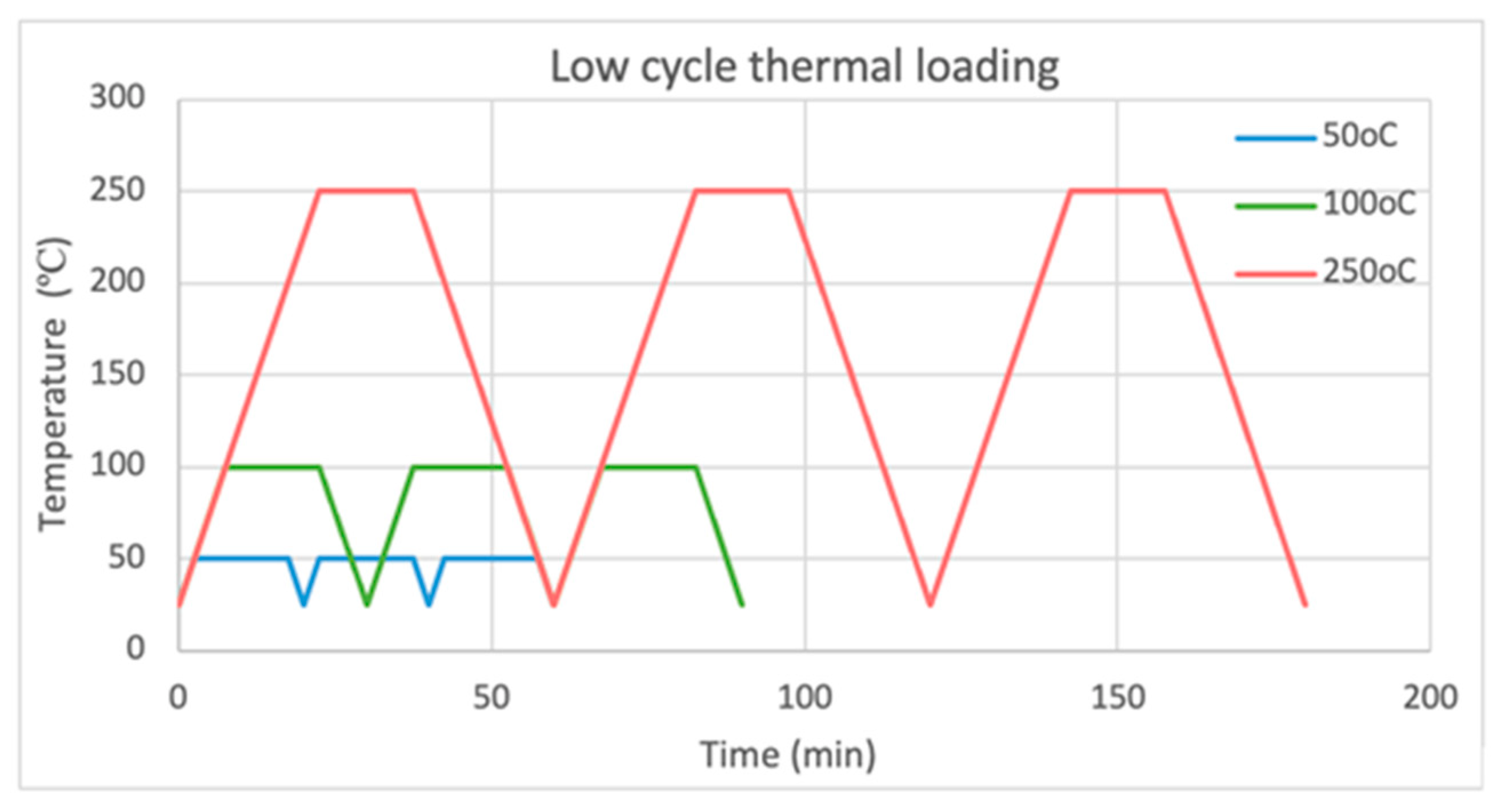
| MCT50-MCTF | Monotonic uniaxial tension until 50% of the tensile strength. 3 min remaining at this level. | Thermal loading of max 3 cycles (heating process, holding time 15 min at 50 °C, cooling process) under constant tensile load 50% of the tensile strength (MCT50) up to failure (MCTF) | 1 |
| MCT100-MCTF | Monotonic uniaxial tension until 50% of the tensile strength. 3 min remaining at this level. | Thermal loading of max 3 cycles (heating process, holding time 15 min at 100 °C, cooling process) under constant tensile load 50% of the tensile strength (MCT100) up to failure (MCTF) | 2 |
| MCT250-MCTF | Monotonic uniaxial tension until 50% of the tensile strength. 3 min remaining at this level. | Thermal loading of max 3 cycles (heating process, holding time 15 min at 250 °C, cooling process) under constant tensile load 50% of the tensile strength (MCT250) up to failure (MCTF) | 2 |
| Name | fu,fibers (MPa) | εfibers (‰) | Efibers (GPa) | Comments/Mode of Failure |
|---|---|---|---|---|
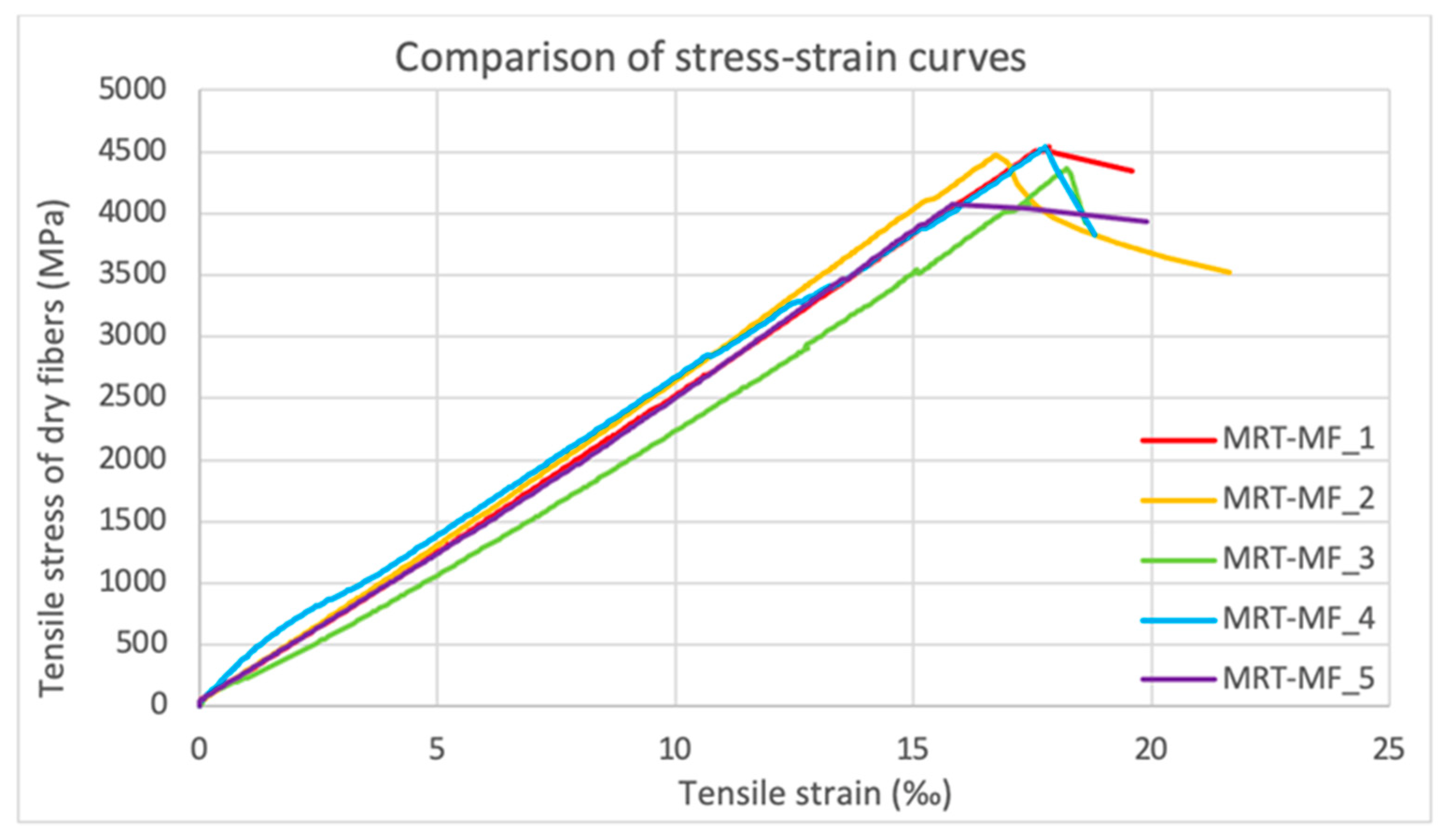
| MRT-MF_1 | 4546.28 | 17.86 | 254.61 | Fracture of fibers |
| MRT-MF_2 | 4482.52 | 16.75 | 267.61 | Fracture of fibers |
| MRT-MF_3 | 4361.10 | 18.22 | 239.36 | Fracture of fibers |
| MRT-MF_4 | 4548.91 | 17.81 | 255.41 | Fracture of fibers |
| MRT-MF_5 | 4115.30 | 15.81 | 260.30 | Fracture of fibers |
| MRT-MF (average) | 4410.82 | 17.29 | 255.12 | Fracture of fibers |
| C50RT-MF_1 | 4519.17 | 19.86 | 227.54 | Fracture of fibers |
| C50RT-MF_2 | 4358.59 | 22.34 | 195.13 | Fracture of fibers |
| C50RT-MF (average) | 4438.88 | 21.10 | 211.34 | Fracture of fibers |
| C75RT-MF_1 | 4079.09 | 16.12 | 253.08 | Fracture of fibers |
| C75RT-MF_2 | 4395.18 | 17.36 | 253.13 | Fracture of fibers |
| C75RT-MF (average) | 4237.14 | 16.74 | 253.11 | Fracture of fibers |
| T50-MF_1 | 3615.14 | 13.67 | 264.52 | Fracture of fibers |
| T50-MF_2 | 4396.47 | 18.15 | 242.23 | Fracture of fibers |
| T50-MF (average) | 4005.81 | 15.91 | 251.81 | Fracture of fibers |
| T100-MF_1 | 2807.13 | 10.14 | 276.95 | Fracture of fibers |
| T100-MF_2 | 4097.00 | 19.57 | 269.32 | Fracture of fibers |
| T100-MF (average) | 3452.07 | 14.85 | 232.39 | Fracture of fibers |
| T250-MF_1 | 4234.06 | 16.83 | 251.58 | Fracture of fibers |
| T250-MF_2 | 4621.66 | 18.48 | 250.09 | Fracture of fibers |
| T250-MF (average) | 4427.86 | 17.66 | 250.80 | Fracture of fibers |
| MCT50-MF_1 | 2393.71 3269.66 | 15.21 19.32 | 169.27 | Three successful thermal loading cycles. The values of the first line refer to the LCTL under constant tension and the values of the second line refer to the MUT up to failure. |
| MCT100-MCTF_1 | 2428.37 | 12.95 | 187.46 | Failure at the heating process of the first cycle at 64 °C. The values refer to the LCTL under constant tension. |
| MCT100-MCTF_2 | 2105.78 | 24.14 | 87.23 | Failure at the cooling process of the first cycle at 31 °C. The values refer to the LCTL under constant tension. |
| MCT250-MCTF_1 | 2604.95 | 15.55 | 167.53 | Failure at the heating process of the first cycle at 61 °C. The values refer to the LCTL under constant tension. |
| MCT250-MCTF_2 | 2371.75 | 23.63 | 100.36 | Failure at the heating process of the first cycle at 151 °C. The values refer to the LCTL under constant tension. |
| T (°C) | rf (-) | rE (-) |
|---|---|---|
| 25 | 1.00 | 1.00 |
| 50 | 1.00 | 0.95 |
| 100 | 0.93 | 0.82 |
| 250 | 1.00 | 0.98 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Papadimitriou, C.; Melidis, L.; Kotoulas, L.; Makris, N.; Katakalos, K. Thermomechanical Characterization of CFRPs under Elevated Temperatures for Strengthening Existing Structures. Fibers 2021, 9, 80. https://doi.org/10.3390/fib9120080
Papadimitriou C, Melidis L, Kotoulas L, Makris N, Katakalos K. Thermomechanical Characterization of CFRPs under Elevated Temperatures for Strengthening Existing Structures. Fibers. 2021; 9(12):80. https://doi.org/10.3390/fib9120080
Chicago/Turabian StylePapadimitriou, Christina, Lazaros Melidis, Lambros Kotoulas, Nikolaos Makris, and Konstantinos Katakalos. 2021. "Thermomechanical Characterization of CFRPs under Elevated Temperatures for Strengthening Existing Structures" Fibers 9, no. 12: 80. https://doi.org/10.3390/fib9120080
APA StylePapadimitriou, C., Melidis, L., Kotoulas, L., Makris, N., & Katakalos, K. (2021). Thermomechanical Characterization of CFRPs under Elevated Temperatures for Strengthening Existing Structures. Fibers, 9(12), 80. https://doi.org/10.3390/fib9120080









