Analytical Formulas for Dispersion and Effective Area in Hollow-Core Tube Lattice Fibers
Abstract
:1. Introduction
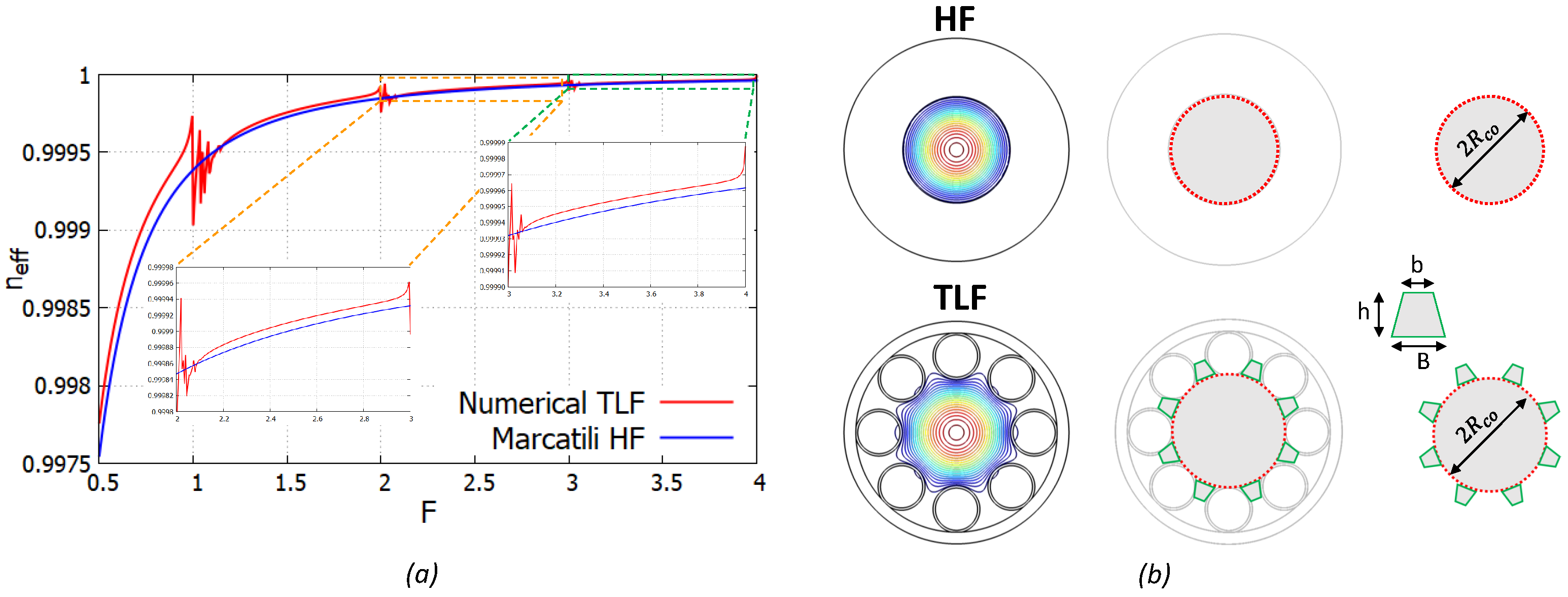
2. Model Description
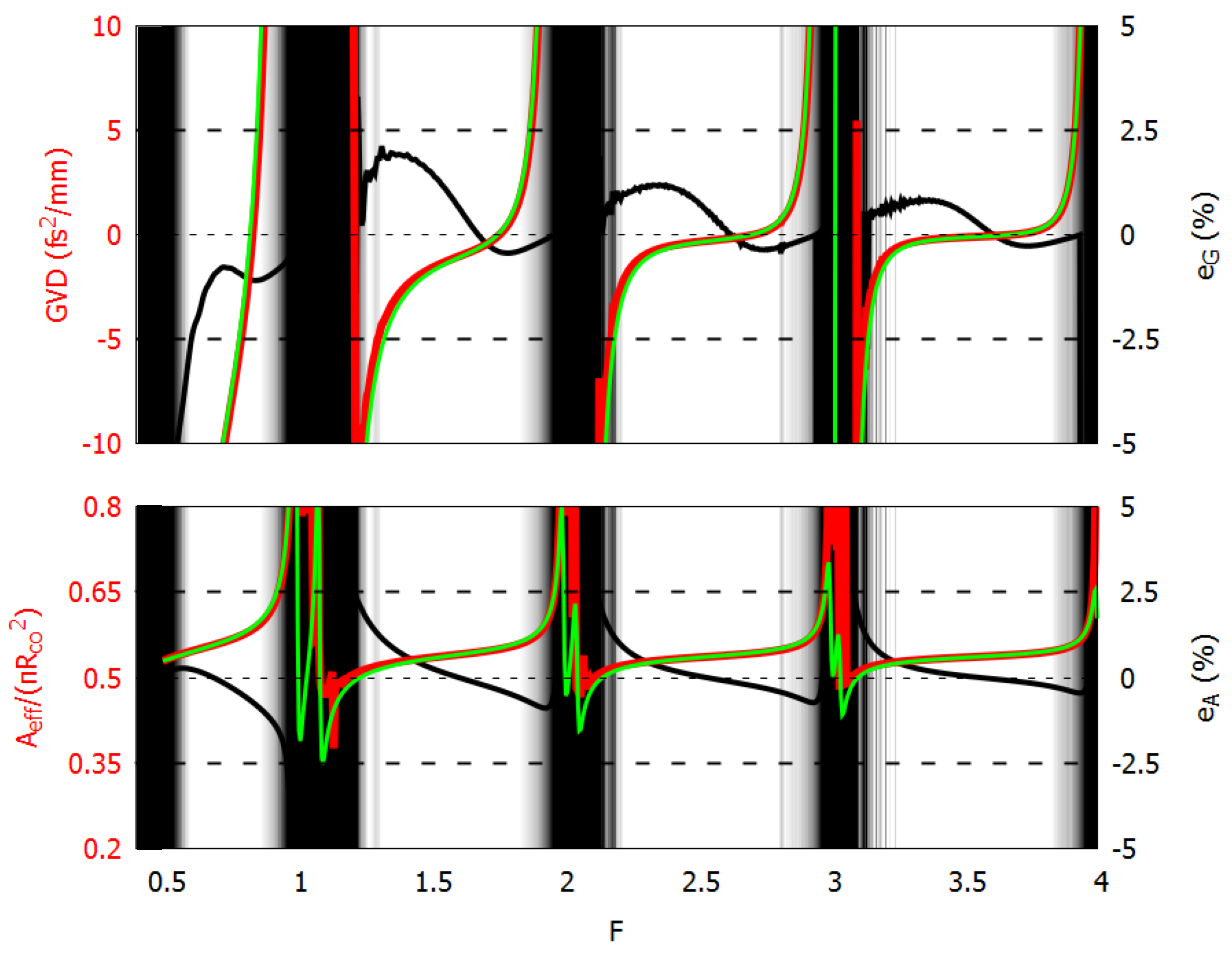
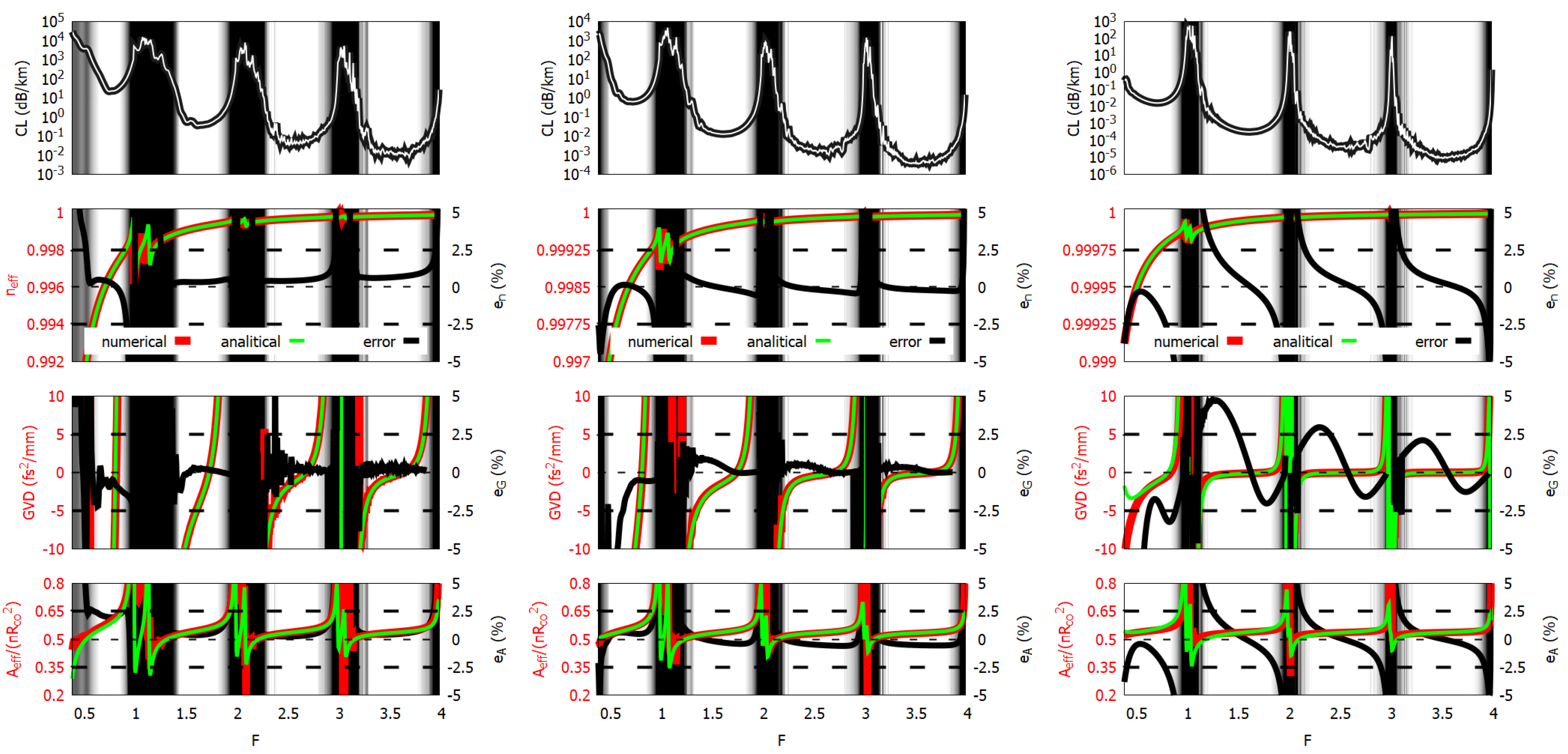
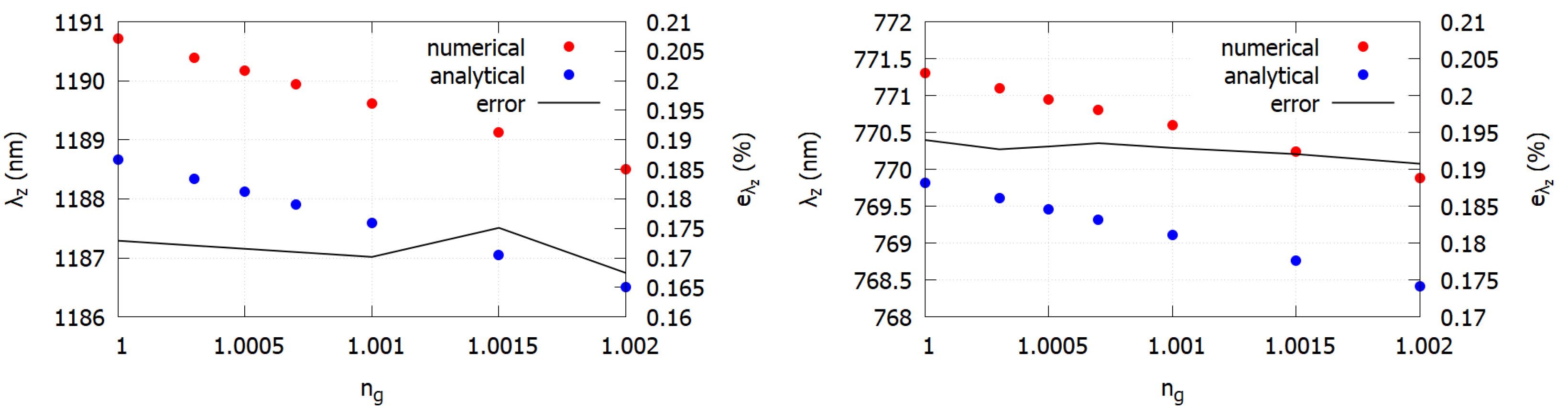
3. Validation
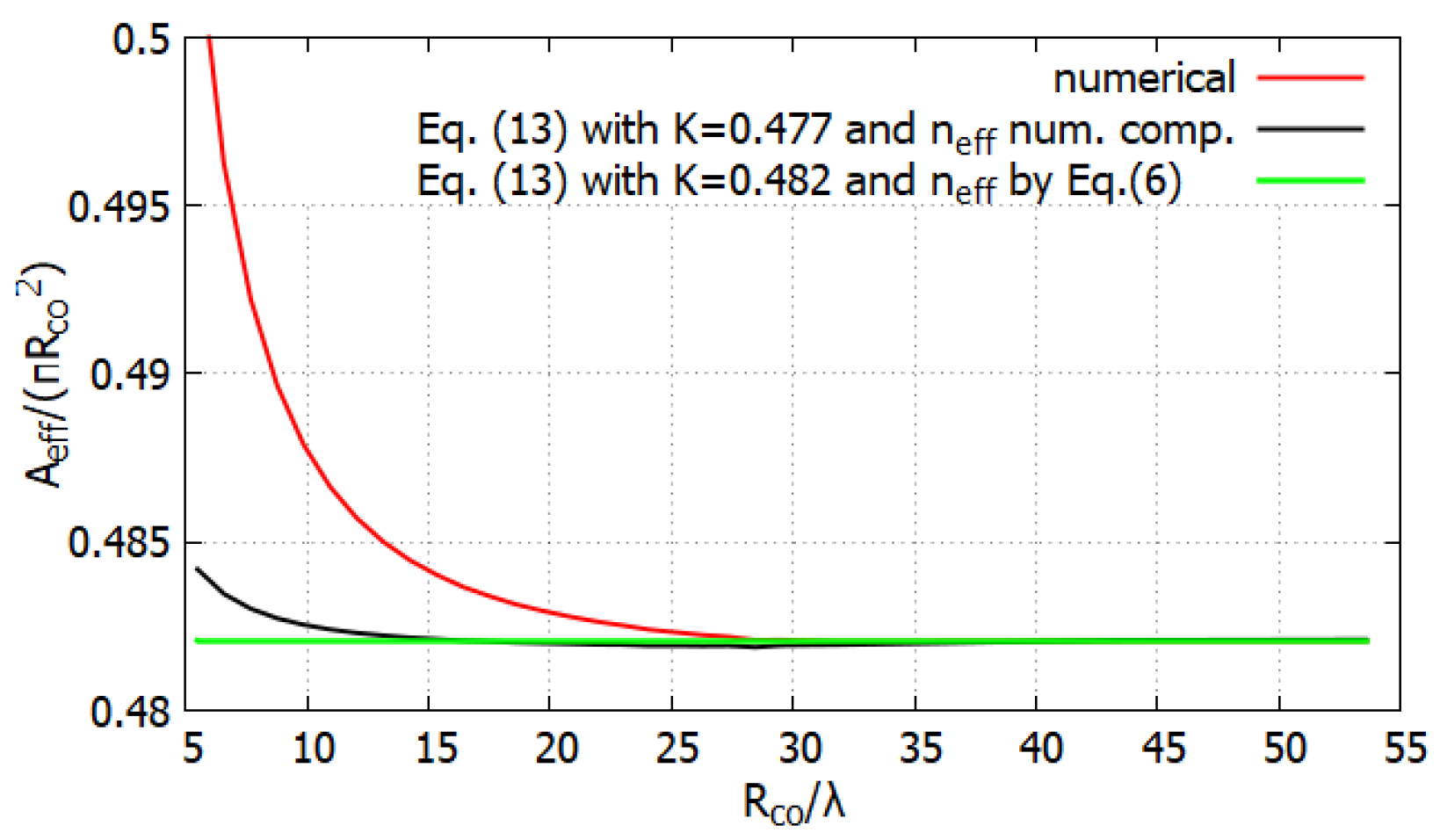
4. Scaling Law of Effective Area
5. Extension to Filled TLFs
6. Other HC-ICFs
7. Conclusions
Author Contributions
Funding
Conflicts of Interest
References
- Cregan, R.F.; Mangan, B.J.; Knight, J.C.; Birks, T.A.; Russell, P.S.J.; Roberts, P.J.; Allan, D.C. Single-Mode Photonic Band Gap Guidance of Light in Air. Science 1999, 285, 1537–1539. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Setti, V.; Vincetti, L.; Argyros, A. Flexible tube lattice fibers for terahertz applications. Opt. Express 2013, 21, 3388–3399. [Google Scholar] [CrossRef]
- Kolyadin, A.N.; Kosolapov, A.F.; Pryamikov, A.D.; Biriukov, A.S.; Plotnichenko, V.G.; Dianov, E.M. Light transmission in negative curvature hollow core fiber in extremely high material loss region. Opt. Express 2013, 21, 9514–9519. [Google Scholar] [CrossRef]
- Wei, C.; Young, J.T.; Menyuk, C.R.; Hu, J. Temperature Sensor Using Fluid-Filled Negative Curvature Fibers. In Proceedings of the Conference on Lasers and Electro-Optics, San Jose, CA, USA, 5–10 May 2018; Optical Society of America: Washington, DC, USA, 2018; p. JW2A.179. [Google Scholar] [CrossRef]
- Gattass, R.R.; Rhonehouse, D.; Gibson, D.; McClain, C.C.; Thapa, R.; Nguyen, V.Q.; Bayya, S.S.; Weiblen, R.J.; Menyuk, C.R.; Shaw, L.B.; et al. Infrared glass-based negative-curvature anti-resonant fibers fabricated through extrusion. Opt. Express 2016, 24, 25697–25703. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Debord, B.; Amsanpally, A.; Chafer, M.; Baz, A.; Maurel, M.; Blondy, J.M.; Hugonnot, E.; Scol, F.; Vincetti, L.; Gérôme, F.; et al. Ultralow transmission loss in inhibited-coupling guiding hollow fibers. Optica 2017, 4, 209–217. [Google Scholar] [CrossRef] [Green Version]
- Couny, F.; Benabid, F.; Roberts, P.J.; Light, P.S.; Raymer, M.G. Generation and Photonic Guidance of Multi-Octave Optical-Frequency Combs. Science 2007, 318, 1118–1121. [Google Scholar] [CrossRef]
- Cassataro, M.; Novoa, D.; Günendi, M.C.; Edavalath, N.N.; Frosz, M.H.; Travers, J.C.; Russell, P.S. Generation of broadband mid-IR and UV light in gas-filled single-ring hollow-core PCF. Opt. Express 2017, 25, 7637–7644. [Google Scholar] [CrossRef] [Green Version]
- Yu, F.; Knight, J.C. Negative Curvature Hollow-Core Optical Fiber. IEEE J. Sel. Top. Quantum Electron. 2016, 22, 146–155. [Google Scholar] [CrossRef] [Green Version]
- Liu, X.L.; Ding, W.; Wang, Y.Y.; Gao, S.F.; Cao, L.; Feng, X.; Wang, P. Characterization of a liquid-filled nodeless anti-resonant fiber for biochemical sensing. Opt. Lett. 2017, 42, 863–866. [Google Scholar] [CrossRef]
- Giovanardi, F.; Cucinotta, A.; Vincetti, L. Inhibited coupling guiding hollow fibers for label-free DNA detection. Opt. Express 2017, 25, 26215–26220. [Google Scholar] [CrossRef]
- Debord, B.; Amrani, F.; Vincetti, L.; Gérôme, F.; Benabid, F. Hollow-Core Fiber Technology: The Rising of “Gas Photonics”. Fibers 2019, 7, 16. [Google Scholar] [CrossRef] [Green Version]
- Vincetti, L.; Setti, V. Waveguiding mechanism in tube lattice fibers. Opt. Express 2010, 18, 23133–23146. [Google Scholar] [CrossRef] [PubMed]
- Litchinitser, N.M.; Abeeluck, A.K.; Headley, C.; Eggleton, B.J. Antiresonant reflecting photonic crystal optical waveguides. Opt. Lett. 2002, 27, 1592–1594. [Google Scholar] [CrossRef] [PubMed]
- Poletti, F. Nested antiresonant nodeless hollow core fiber. Opt. Express 2014, 22, 23807–23828. [Google Scholar] [CrossRef] [PubMed]
- Wang, Y.Y.; Wheeler, N.V.; Couny, F.; Roberts, P.J.; Benabid, F. Low loss broadband transmission in hypocycloid-core Kagome hollow-core photonic crystal fiber. Opt. Lett. 2011, 36, 669–671. [Google Scholar] [CrossRef]
- Debord, B.; Alharbi, M.; Bradley, T.; Fourcade-Dutin, C.; Wang, Y.; Vincetti, L.; Gérôme, F.; Benabid, F. Hypocycloid-shaped hollow-core photonic crystal fiber Part I: Arc curvature effect on confinement loss. Opt. Express 2013, 21, 28597–28608. [Google Scholar] [CrossRef] [PubMed]
- Pryamikov, A.; Alagashev, G.; Falkovich, G.; Turitsyn, S. Light transport and vortex-supported wave-guiding in micro-structured optical fibres. Sci. Rep. 2020, 10, 2045–2322. [Google Scholar] [CrossRef]
- Osório, J.; Foued, A.; Frederic, D.; Ali, D.; Kostiantyn, V.; Gilles, T.; Fabio, G.; Luca, V.; Benoit, D.; Frederic, G.; et al. Sub-thermodynamic equilibrium surface roughness in hollow-core fibers for the ultraviolet range. In Proceedings of the European Optical Society Annual Meeting, Rome, Italy, 13–17 September 2021. [Google Scholar]
- Gao, S.f.; Wang, Y.y.; Ding, W.; Hong, Y.f.; Wang, P. Conquering the Rayleigh Scattering Limit of Silica Glass Fiber at Visible Wavelengths with a Hollow-Core Fiber Approach. Laser Photonics Rev. 2020, 14, 1900241. [Google Scholar] [CrossRef] [Green Version]
- Gladyshev, A.V.; Kosolapov, A.F.; Khudyakov, M.M.; Yatsenko, Y.P.; Kolyadin, A.N.; Krylov, A.A.; Pryamikov, A.D.; Biriukov, A.S.; Likhachev, M.E.; Bufetov, I.A.; et al. 2.9, 3.3, and 3.5 μm Raman Lasers Based on Revolver Hollow-Core Silica Fiber Filled by 1H2/D2 Gas Mixture. IEEE J. Sel. Top. Quantum Electron. 2018, 24, 1–8. [Google Scholar] [CrossRef]
- Yu, F.; Knight, J.C. Spectral attenuation limits of silica hollow core negative curvature fiber. Opt. Express 2013, 21, 21466–21471. [Google Scholar] [CrossRef] [Green Version]
- Vincetti, L. Empirical formulas for calculating loss in hollow core tube lattice fibers. Opt. Express 2016, 24, 10313–10325. [Google Scholar] [CrossRef]
- Vincetti, L.; Rosa, L. A simple analytical model for confinement loss estimation in hollow-core Tube Lattice Fibers. Opt. Express 2019, 27, 5230–5237. [Google Scholar] [CrossRef] [PubMed]
- Bird, D. Attenuation of model hollow-core, anti-resonant fibres. Opt. Express 2017, 25, 23215–23237. [Google Scholar] [CrossRef]
- Zeisberger, M.; Schmidt, M.A. Analytic model for the complex effective index of the leaky modes of tube-type anti-resonant hollow core fibers. Sci. Rep. 2017, 7, 11761. [Google Scholar] [CrossRef] [Green Version]
- Hasan, M.I.; Akhmediev, N.; Chang, W. Empirical Formulae for Dispersion and Effective Mode Area in Hollow-Core Antiresonant Fibers. IEEE/OSA J. Light. Technol. 2018, 36, 4060–4065. [Google Scholar] [CrossRef]
- Provino, L. Effect of Nested Elements on Avoided Crossing between the Higher-Order Core Modes and the Air-Capillary Modes in Hollow-Core Antiresonant Optical Fibers. Fibers 2018, 6, 42. [Google Scholar] [CrossRef] [Green Version]
- Bache, M.; Habib, M.S.; Markos, C.; Lægsgaard, J. Poor-man’s model of hollow-core anti-resonant fibers. J. Opt. Soc. Am. B 2019, 36, 69–80. [Google Scholar] [CrossRef]
- Deng, A.; Hasan, I.; Wang, Y.; Chang, W. Analyzing mode index mismatch and field overlap for light guidance in negative-curvature fibers. Opt. Express 2020, 28, 27974–27988. [Google Scholar] [CrossRef] [PubMed]
- Song, P.; Phoong, K.Y.; Bird, D. Quantitative analysis of anti-resonance in single-ring, hollow-core fibres. Opt. Express 2019, 27, 27745–27760. [Google Scholar] [CrossRef]
- Agrawal, G.P. Nonlinear Fiber Optics, 6th ed.; Academic Press: Boston, MA, USA, 2019. [Google Scholar]
- Heckl, O.H.; Saraceno, C.J.; Baer, C.R.E.; Südmeyer, T.; Wang, Y.Y.; Cheng, Y.; Benabid, F.; Keller, U. Temporal pulse compression in a xenon-filled Kagome-type hollow-core photonic crystal fiber at high average power. Opt. Express 2011, 19, 19142–19149. [Google Scholar] [CrossRef] [PubMed]
- Debord, B.; Alharbi, M.; Vincetti, L.; Husakou, A.; Fourcade-Dutin, C.; Hoenninger, C.; Mottay, E.; Gérôme, F.; Benabid, F. Multi-meter fiber-delivery and pulse self-compression of milli-Joule femtosecond laser and fiber-aided laser-micromachining. Opt. Express 2014, 22, 10735–10746. [Google Scholar] [CrossRef] [PubMed]
- Belli, F.; Abdolvand, A.; Chang, W.; Travers, J.C.; Russell, P.S. Vacuum-ultraviolet to infrared supercontinuum in hydrogen-filled photonic crystal fiber. Optica 2015, 2, 292–300. [Google Scholar] [CrossRef]
- Debord, B.; Gérôme, F.; Honninger, C.; Mottay, E.; Husakou, A.; Benabid, F. Milli-Joule energy-level comb and supercontinuum generation in atmospheric air-filled inhibited coupling Kagome fiber. In Proceedings of the 2015 Conference on Lasers and Electro-Optics (CLEO), San Jose, CA, USA, 10–15 May 2015; pp. 1–2. [Google Scholar] [CrossRef]
- Belli, F.; Abdolvand, A.; Travers, J.C.; Russell, P.S.J. Highly efficient deep UV generation by four-wave mixing in gas-filled hollow-core photonic crystal fiber. Opt. Lett. 2019, 44, 5509–5512. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Marcatili, E.A.J.; Schmeltzer, R.A. Hollow metallic and dielectric waveguides for long distance optical transmission and lasers. Bell Syst. Tech. J. 1964, 43, 1783–1809. [Google Scholar] [CrossRef]

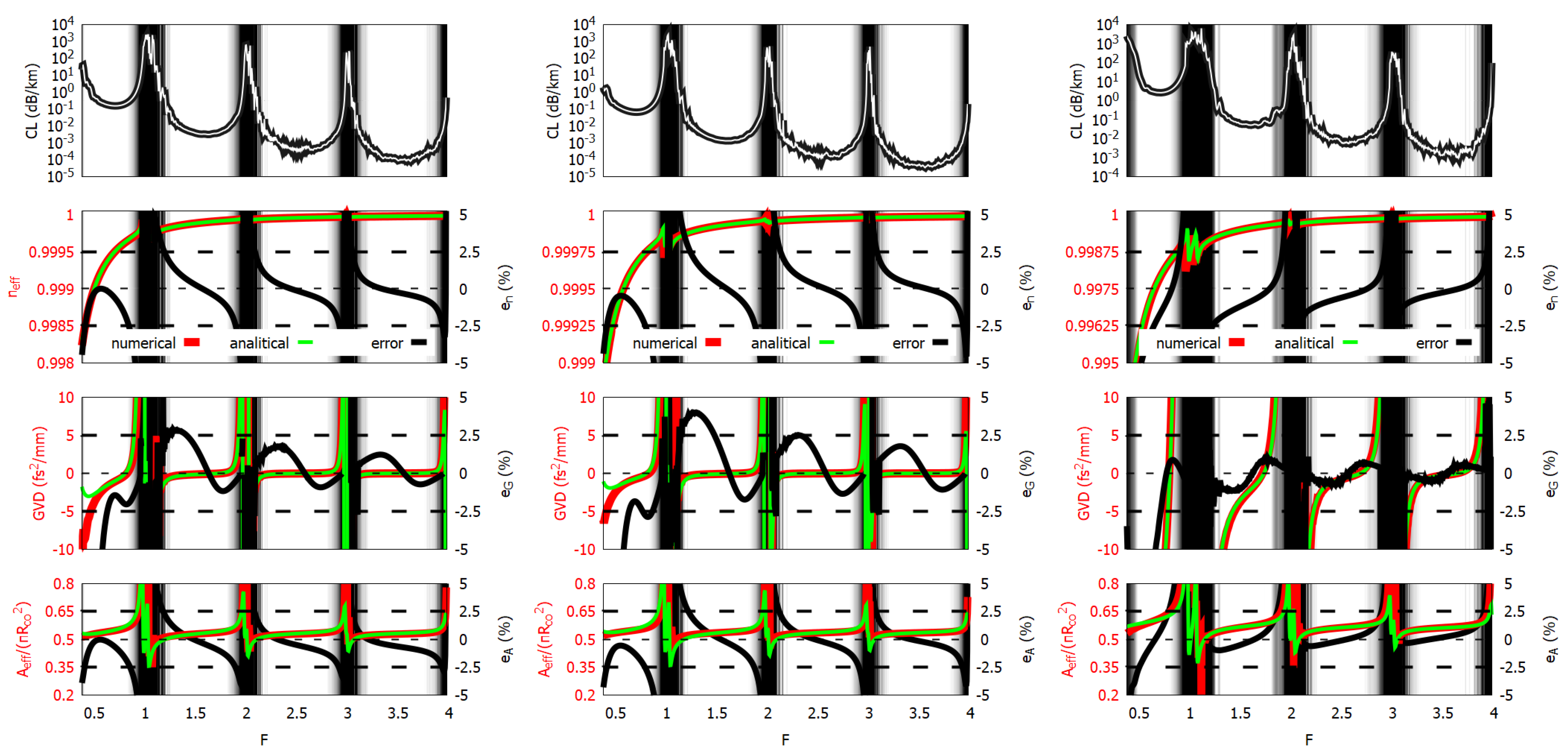
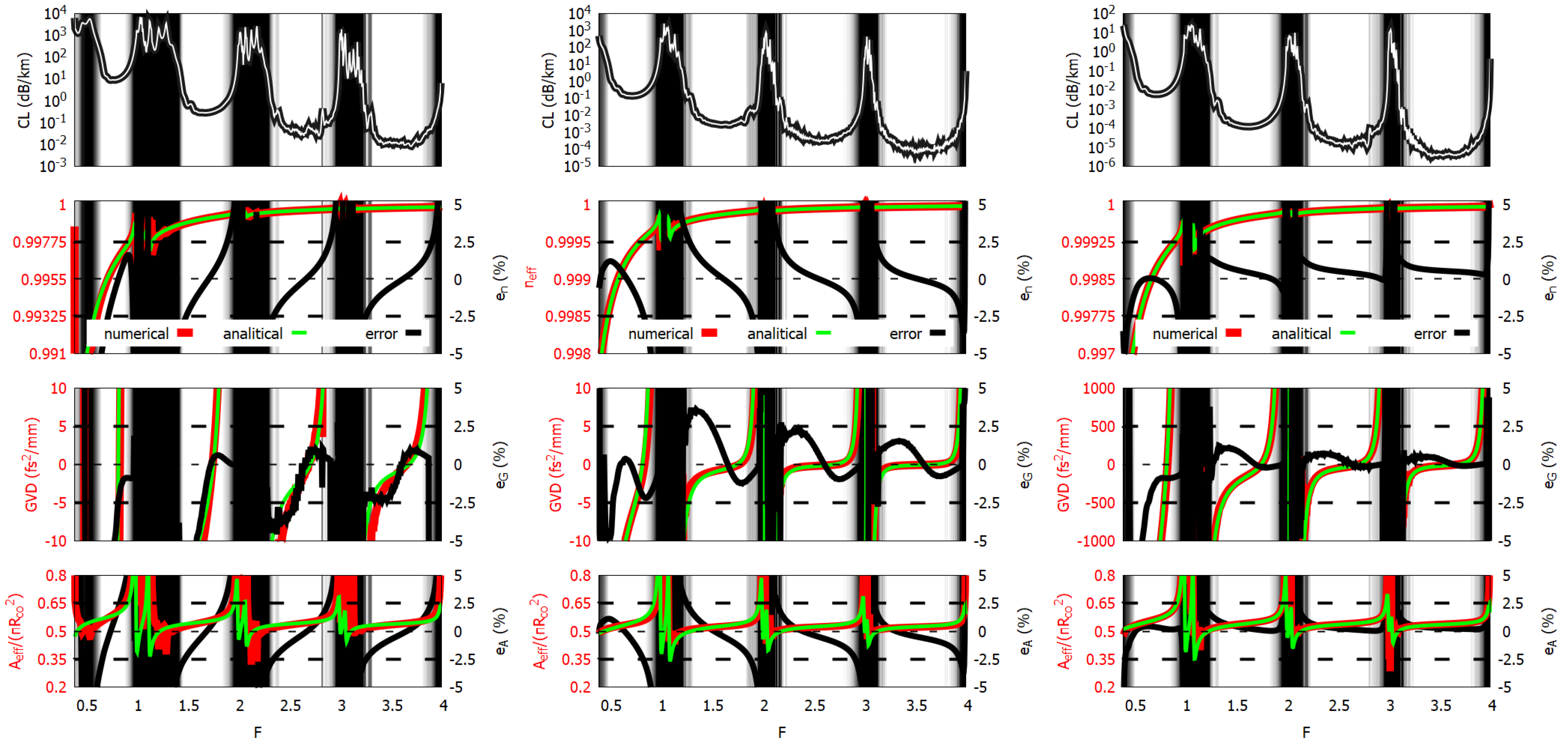
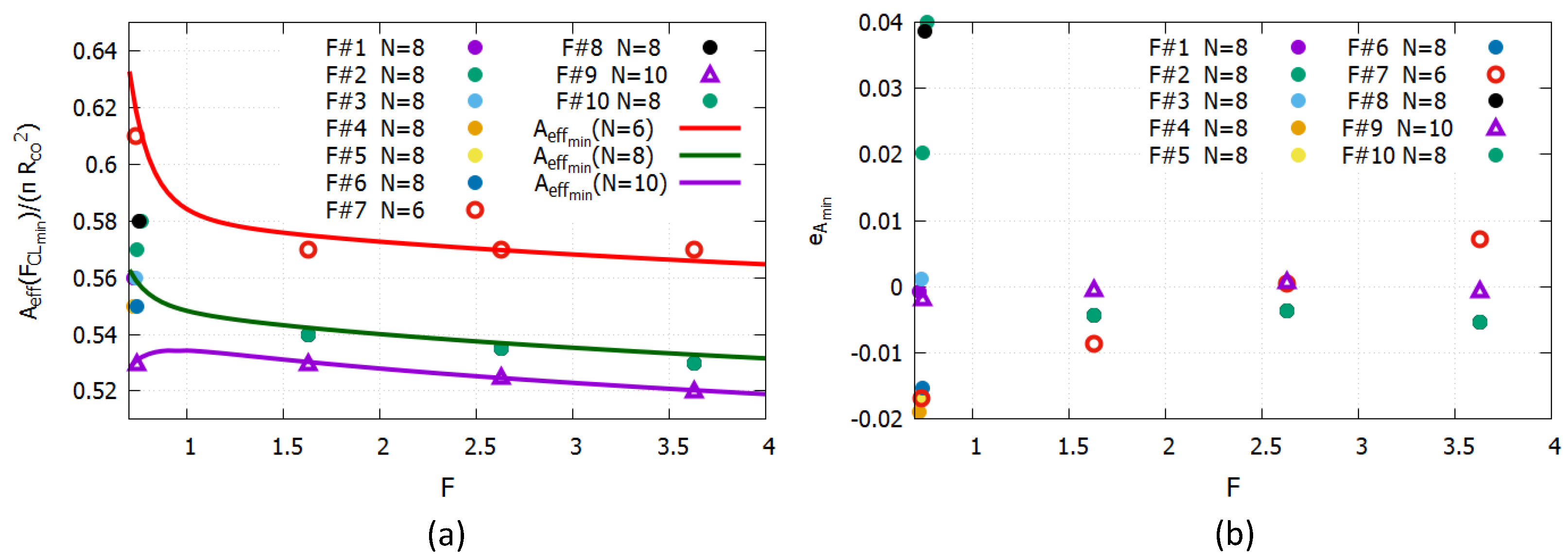
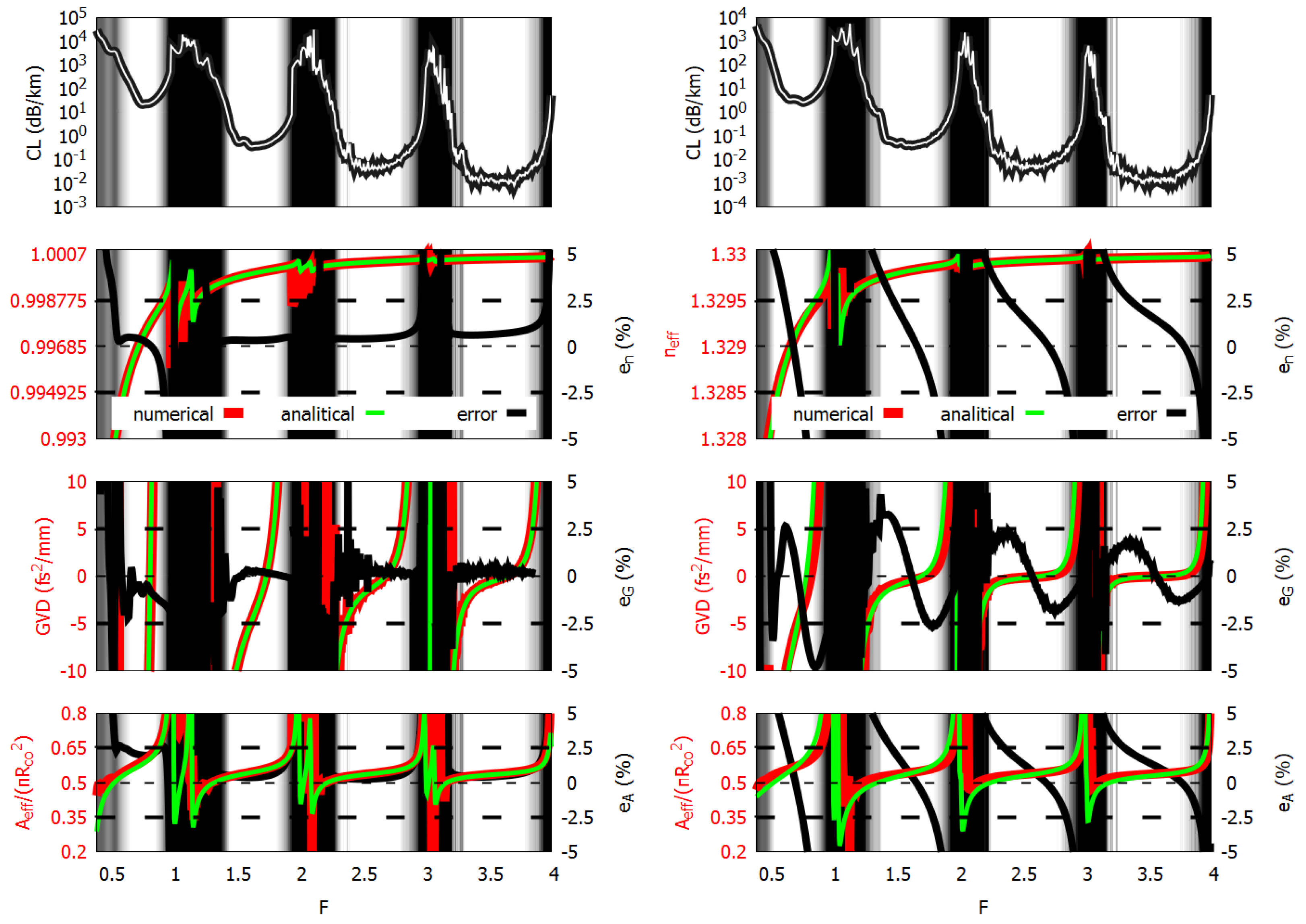
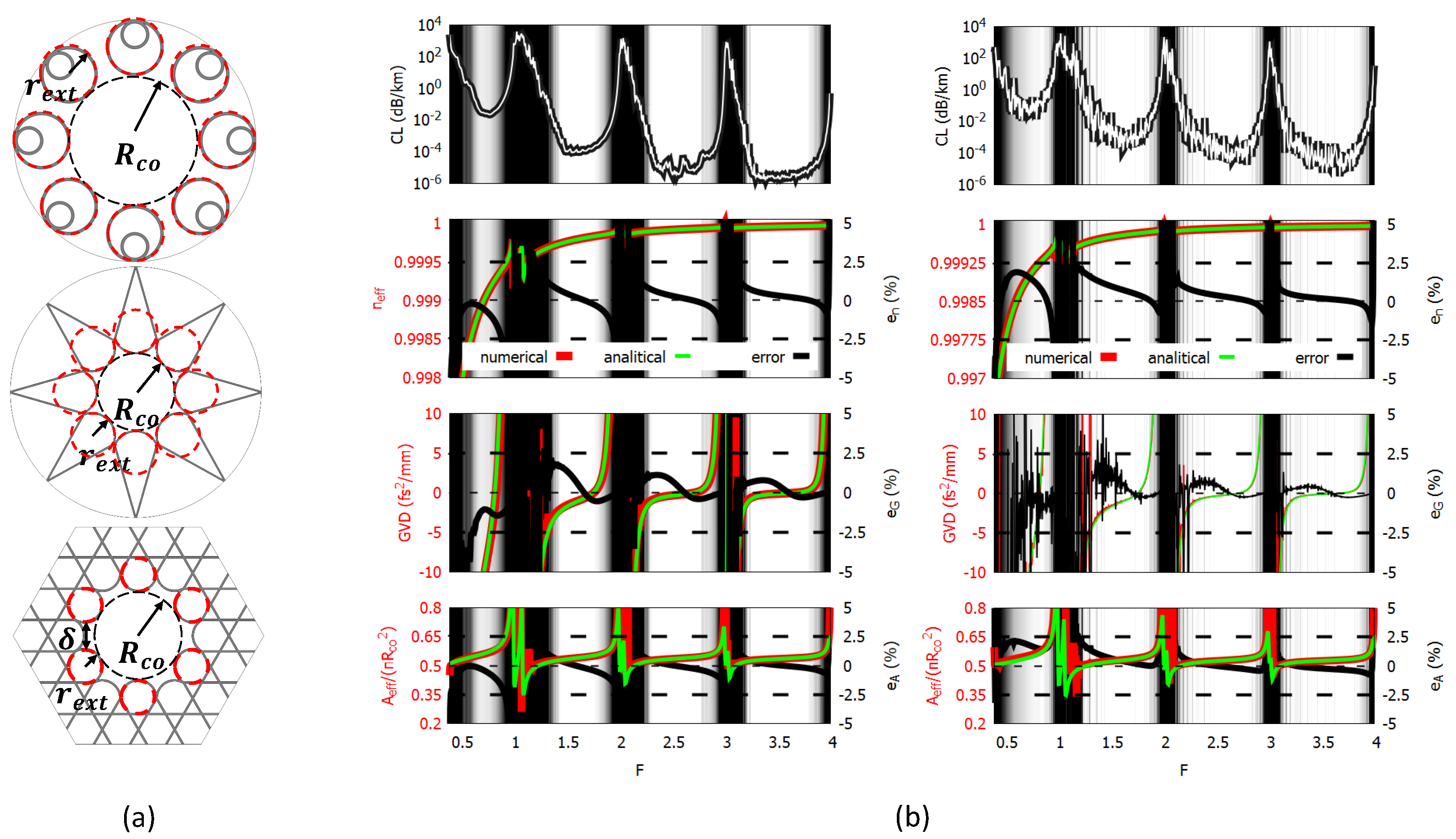
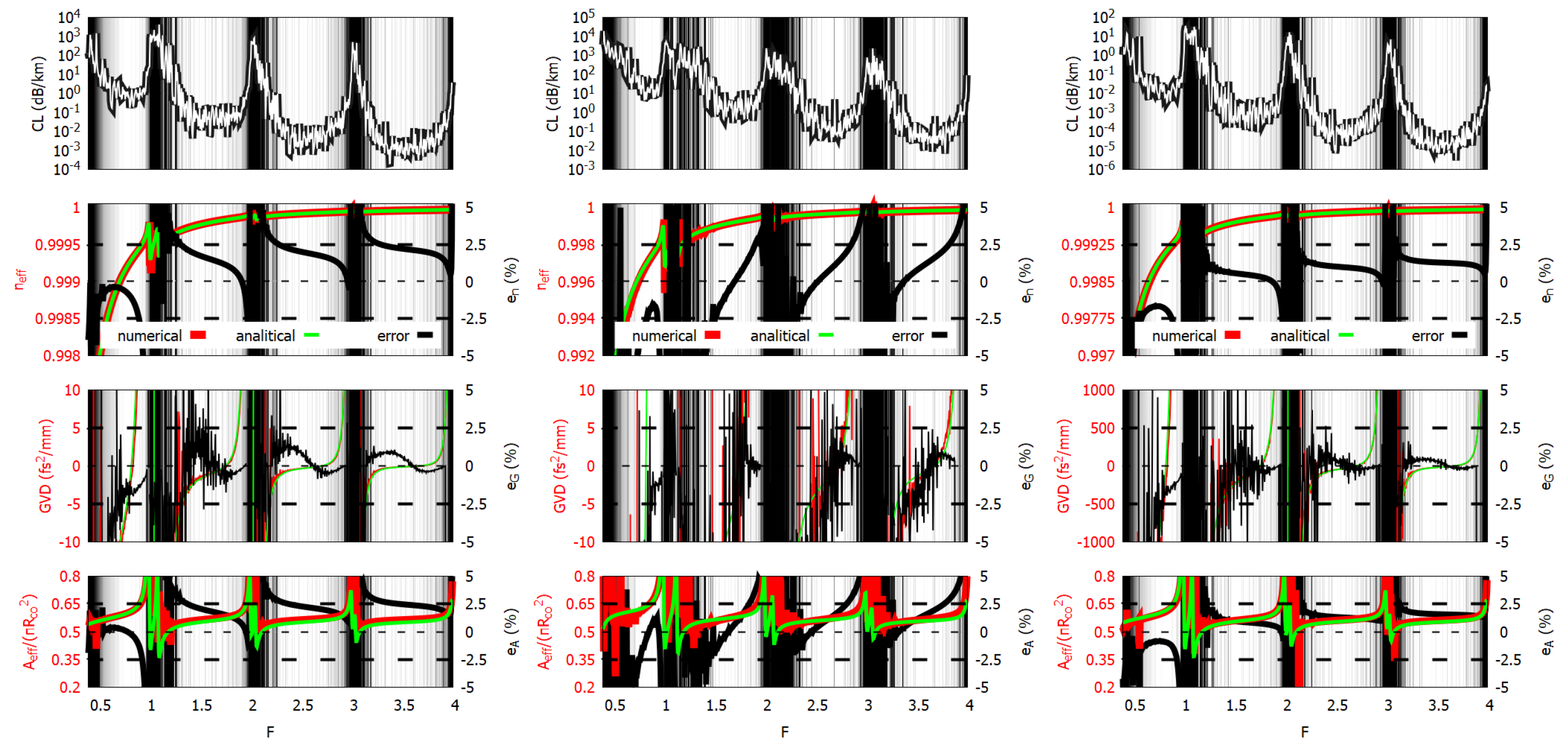
| Fiber | t (m) | (m) | (m) | n | N | Spectral Range |
|---|---|---|---|---|---|---|
| F#1 | 1.0 | 10 | 5.0 | 1.44 | 8 | NIR |
| F#2 | 1.0 | 5.0 | 2.5 | 1.44 | 8 | NIR |
| F#3 | 1.0 | 10 | 2.5 | 1.44 | 8 | NIR |
| F#4 | 0.5 | 10 | 5.0 | 1.44 | 8 | VIS |
| F#5 | 0.2 | 4.0 | 0.419 | 1.50 | 8 | UV |
| F#6 | 0.2 | 4.0 | 1.6 | 1.50 | 8 | UV |
| F#7 | 1.0 | 10 | 5.0 | 1.44 | 6 | NIR |
| F#8 | 1.0 | 10 | 5.0 | 2.42 | 8 | MIR |
| F#9 | 1.0 | 10 | 4.0 | 1.44 | 10 | NIR |
| F#10 | 100 | 1000 | 445 | 1.521 | 8 | THz |
| Fiber | t (m) | (m) | (m) | n | Spectral Range |
|---|---|---|---|---|---|
| NF#1 | 1.0 | 10 | 22.7 | 1.44 | NIR |
| IF#1 | 1.0 | 14.05 | 0 | 1.44 | NIR |
| KF#1 | 1.0 | 9.75 | 22.7 | 1.44 | NIR |
| KF#2 | 1.0 | 9.75 | 22.7 | 2.42 | MIR |
| KF#3 | 100 | 1000 | 2205 | 1.521 | THz |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Rosa, L.; Melli, F.; Vincetti, L. Analytical Formulas for Dispersion and Effective Area in Hollow-Core Tube Lattice Fibers. Fibers 2021, 9, 58. https://doi.org/10.3390/fib9100058
Rosa L, Melli F, Vincetti L. Analytical Formulas for Dispersion and Effective Area in Hollow-Core Tube Lattice Fibers. Fibers. 2021; 9(10):58. https://doi.org/10.3390/fib9100058
Chicago/Turabian StyleRosa, Lorenzo, Federico Melli, and Luca Vincetti. 2021. "Analytical Formulas for Dispersion and Effective Area in Hollow-Core Tube Lattice Fibers" Fibers 9, no. 10: 58. https://doi.org/10.3390/fib9100058
APA StyleRosa, L., Melli, F., & Vincetti, L. (2021). Analytical Formulas for Dispersion and Effective Area in Hollow-Core Tube Lattice Fibers. Fibers, 9(10), 58. https://doi.org/10.3390/fib9100058








