Advanced Study of Columns Confined by Ultra-High-Performance Concrete and Ultra-High-Performance Fiber-Reinforced Concrete Confinements
Abstract
1. Introduction
2. Materials and Methods
2.1. Materials
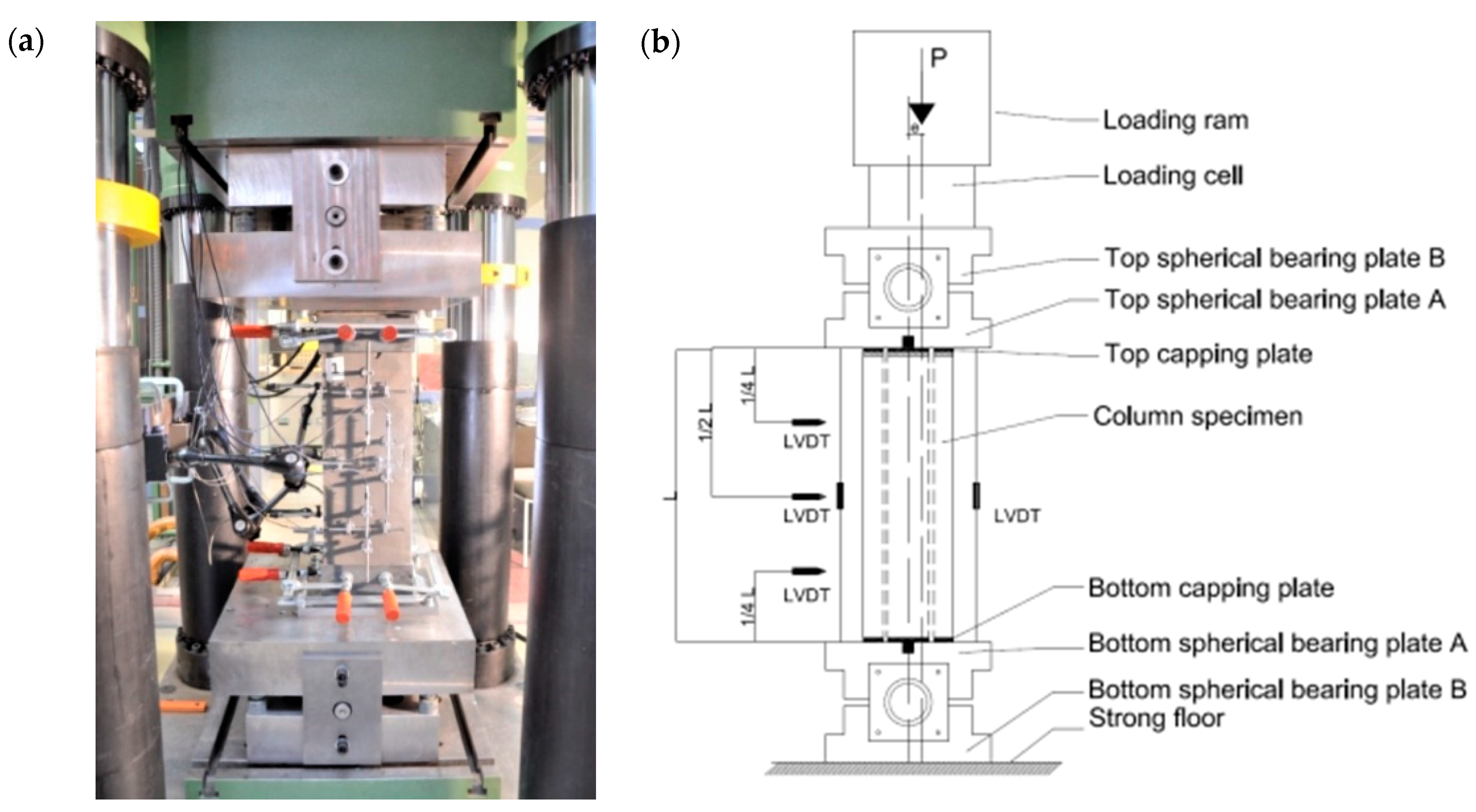
2.2. Experiment Setup
2.3. Methods
2.3.1. Modeling of UHPC/UHPFRC Compressive Strength
2.3.2. Stress–Strain Relationship of UHPC/UHPFRC
3. Results
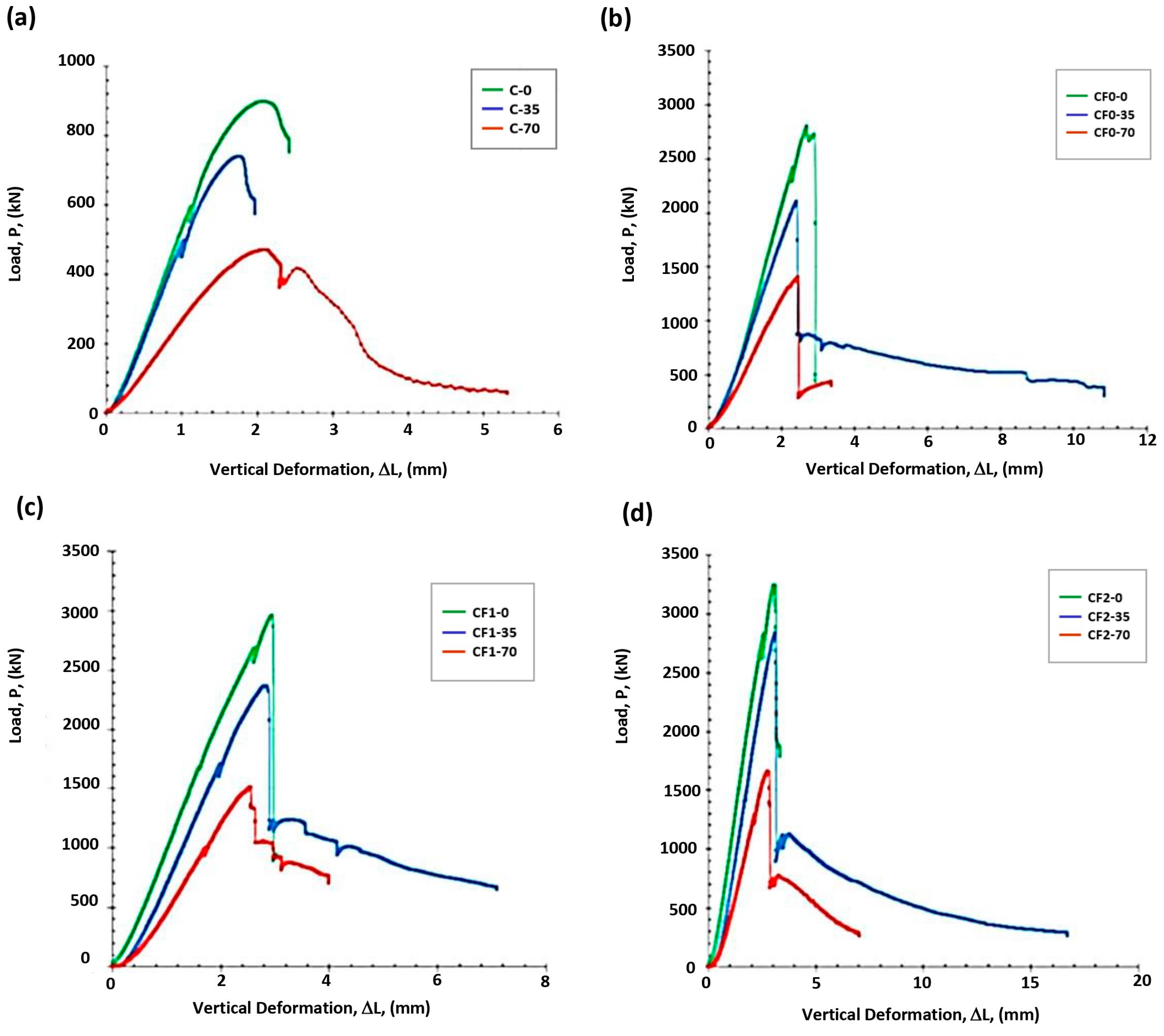
3.1. Load–Deformation
3.2. Stress–Strain Behavior
3.3. Crack Pattern
4. Discussion
5. Conclusions
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Aziz, O.Q.; Ahmed, G.H. Mechanical Properties of Ultra High Performance Concrete (UHPC); ACI Special Publicatio; American Concrete Institute: Farmington Hills, MI, USA, 2012; pp. 331–346. [Google Scholar]
- Akeed, M.H.; Qaidi, S.; Ahmed, H.U.; Faraj, R.H.; Mohammed, A.S.; Emad, W.; Tayeh, B.A.; Azevedo, A.R.G. Ultra-High-Performance Fiber-Reinforced Concrete. Part II: Hydration and Microstructure. Case Stud. Constr. Mater. 2022, 17, e01289. [Google Scholar] [CrossRef]
- El-khoriby, R.S.; Taher, S.E.; Ghazy, M.F.; Abd-elaty, M.A. How Practical Is Ultra High Performance Concrete for Construction Projects How Practical Is Ultra High Performance Concrete For. In Proceedings of the International Conference on Advances in Structural and Geotechnical Engineering (ICASGE’19), Tanta, Egypt, 25–28 March 2019; Volume 3, pp. 93–137. [Google Scholar]
- Abbas, S.; Nehdi, M.L.; Saleem, M.A. Ultra-High Performance Concrete: Mechanical Performance, Durability, Sustainability and Implementation Challenges. Int. J. Concr. Struct. Mater. 2016, 10, 271–295. [Google Scholar] [CrossRef]
- Roy, D.M.; Gouda, G.R.; Bobrowsky, A. Very High Strength Cement Pastes Prepared by Hot Pressing and Other High Pressure Techniques. Cem. Concr. Res. 1972, 2, 349–366. [Google Scholar] [CrossRef]
- Yudenfreund, M.; Odler, I.; Brunauer, S. Hardened Portland Cement Pastes of Low Porosity I. Materials and Experimental Methods. Cem. Concr. Res. 1972, 2, 313–330. [Google Scholar] [CrossRef]
- Biswas, R.K.; Bin Ahmed, F.; Haque, M.E.; Provasha, A.A.; Hasan, Z.; Hayat, F.; Sen, D. Effects of Steel Fiber Percentage and Aspect Ratios on Fresh and Harden Properties of Ultra-High Performance Fiber Reinforced Concrete. Appl. Mech. 2021, 2, 501–515. [Google Scholar] [CrossRef]
- Alford, N.M.; Birchall, J.D. The Properties and Potential Applications of Macro-Defect-Free Cement. MRS Proc. 1984, 42, 265–276. [Google Scholar] [CrossRef]
- Bache, H.H. Introduction to Compact Reinforced Composite; Nordic Concrete Federation: Aalborg Portland, Denmark, 1987; pp. 19–33. [Google Scholar]
- de Larrard, F.; Sedran, T. Optimization of Ultra-High-Performance Concrete by the Use of a Packing Model. Cem. Concr. Res. 1994, 24, 997–1009. [Google Scholar] [CrossRef]
- Richard, P.; Cheyrezy, M. Composition of Reactive Powder Concretes. Cem. Concr. Res. 1995, 25, 1501–1511. [Google Scholar] [CrossRef]
- Hoang, A.L.; Fehling, E.; Lai, B.; Thai, D.-K.; Van Chau, N. Experimental Study on Structural Performance of UHPC and UHPFRC Columns Confined with Steel Tube. Eng. Struct. 2019, 187, 457–477. [Google Scholar] [CrossRef]
- Ding, Y.; Zeng, B.; Zhou, Z.; Wei, Y.; Huang, Y. Behavior of UHPC Columns Confined by High-Strength Transverse Reinforcement under Eccentric Compression. J. Build. Eng. 2023, 70, 106352. [Google Scholar] [CrossRef]
- Koo, I.-Y.; Hong, S.-G. Strengthening RC Columns with Ultra High Performance Concrete. In Proceedings of the 2016 Structures Congress, Jeju Island, Korea, 28 August–1 September 2016. [Google Scholar]
- Ashteyat, A.M.; Obaidat, A.T.; Obaidat, Y.T.; Abdel-Jaber, M.; Al-Tarawneh, D. The Behavior of Strengthened and Repaired RC Columns with (CFRP) Rope under Different Preloading Levels. Eur. J. Environ. Civ. Eng. 2023, 1–25. [Google Scholar] [CrossRef]
- Mhuder, W.J.; Chassib, S.M. Strengthening of RC Circular Short Columns with Fibrous Jacket. IOP Conf. Ser. Mater. Sci. Eng. 2020, 928, 022075. [Google Scholar] [CrossRef]
- Wu, X.; Kang, T.H.K.; Mpalla, I.B.; Kim, C.S. Axial Load Testing of Hybrid Concrete Columns Consisting of UHPFRC Tube and Normal-Strength Concrete Core. Int. J. Concr. Struct. Mater. 2018, 12, 43. [Google Scholar] [CrossRef]
- Chauhan, M.A.; Agha, S.; Rattan, I. Strengthening of Reinforced Concrete Beam Using FRP. Int. J. Eng. Res. Technol. 2021, 10, 416–436. [Google Scholar]
- Luu, X.-B.; Kim, S.-K. Finite Element Modeling of Interface Behavior between Normal Concrete and Ultra-High Performance Fiber-Reinforced Concrete. Buildings 2023, 13, 950. [Google Scholar] [CrossRef]
- Kusumawardaningsih, Y.; Fehling, E.; Ismail, M.; Aboubakr, A.A.M. Tensile Strength Behavior of UHPC and UHPFRC. Procedia Eng. 2015, 125, 1081–1086. [Google Scholar] [CrossRef]
- Kusumawardaningsih, Y.; Fehling, E. Behavior of Ultra High Perfoemance Concrete (UHPC) Confinement on Normal Strength Concrete (NSC) Column. In Proceedings of the Deutscher Ausschuss fur Stahlbeton- 53 Dafstb Forschungskolloqium, Kassel, Germany, 9–10 October 2012; Intitut fur Konstruktiven Ingenieurbau der Universitat Kassel: Kassel, Germany, 2012; pp. 165–170. [Google Scholar]
- Kusumawardaningsih, Y.; Susilorini, R.M.I.R.; Aboubakr, A. Properties of Ultra High Performance Concrete. In Proceedings of the International Conference on Concrete and Infrastructure 2015, Semarang, Indonesia, 28–30 October 2015; pp. 44–49. [Google Scholar]
- Kusumawardaningsih, Y.; Fehling, E. Behavior of RC Columns Confined with UHPC. In Proceedings of the 5th International Symposium on Ultra-high Performance Concrete and High Performance Construction Materials, Kassel, Germany, 11–13 March 2020; Middendorf, B., Fehling, E., Wetzel, A., Eds.; Kassel University Press: Kassel, Germany, 2020; pp. 13–16. [Google Scholar]
- Kusumawardaningsih, Y.; Fehling, E.; Hardjasaputra, H.; Al-Ani, Y.; Aboubakr, A.A.M. Axial Tensile Strengths of UHPC and UHPFRC. IOP Conf. Ser. Mater. Sci. Eng. 2019, 615, 3–12. [Google Scholar] [CrossRef]
- Kusumawardaningsih, Y.; Fehling, E.; Ismail, M. UHPC Compressive Strength Test Specimens: Cylinder or Cube? Procedia Eng. 2015, 125, 1076–1080. [Google Scholar] [CrossRef]
- Gowripalan, N.; Gilbert, I.R. Design Guidelines for Ductal Prestressed Design Guidelines for Ductal Prestressed Concrete Beams N Gowripalan and R I Gilbert the University of New South Wales; VSL (Aust) Pty Ltd.: Sidney, Australia, 2000. [Google Scholar]
- Janvier. Bétons Fibrés à Ultra-Hautes Performances-Recommandations Provisoires; AFGC: Griffith, Australia, 2002; ISBN 9780870316418. [Google Scholar]
- Niwa, J.; Gakkai, D. Recommendations for Design and Construction of Ultra High Strength Fiber Reinforced Concrete Structures (Draft); JSCE Guidelines for Concrete; Subcommittee on Research of Ultra High Strength Fiber Reinforced Concrete, Japan Society of Civil Engineers (JSCE): Tokyo, Japan, 2006; ISBN 9784810605570. [Google Scholar]
- Schmidt, P.M.; Bunje, D.K.; Dehn, J.F.; Greiner, S.; Horvath, J.; Kleen, D.E.; Müller, C.; Teutsch, M.; Tue, N.V. Sachstandsbericht-Ultrahochfester Beton; Deutscher Ausschuss für Stahlbeton DAfStb: Berlin, Germany; Wien, Austria; Zürich, Switzerland, 2008. [Google Scholar]
- European Committee for Standardization. Eurocode 2: Design of Concrete Structures—Part 1-1: General Rules and Rules for Buildings—EN 1992-1-1; European Committee for Standardization: Brussel, Belgium, 2004. [Google Scholar]
- Leutbecher, T.; Fehling, E. A Simple Design Approach for UHPFRC in Bending. In Proceedings of the RILEM-fib-AFGC International Symposium on Ultra-High Performance Fibre-Reinforced Concrete, Marseille, France, 1–3 October 2013; pp. 509–518. [Google Scholar]




| No. | Specimen Code | Description | Fiber Percentage | Loading Type | Eccentricity |
|---|---|---|---|---|---|
| 1 | C-0 | CONTROL 1 | 0% | concentric | e = 0 mm |
| 2 | CF0-0 | NSC + UHPC 0% | 0% | concentric | e = 0 mm |
| 3 | CFl-0 | NSC + UHPFRC 1% | 1% | concentric | e = 0 mm |
| 4 | CF2-0 | NSC + UHPFRC 2% | 2% | concentric | e = 0 mm |
| 5 | C-35 | CONTROL 2 | 0% | eccentric | e = 35 mm |
| 6 | CF0-35 | NSC + UHPC 0% | 0% | eccentric | e = 35 mm |
| 7 | CFl-35 | NSC + UHPFRC 1% | 1% | eccentric | e = 35 mm |
| 8 | CF2-35 | NSC + UHPFRC 2% | 2% | eccentric | e = 35 mm |
| 9 | C-70 | CONTROL 3 | 0% | eccentric | e = 70 mm |
| 10 | CF0-70 | NSC + UHPC 0% | 0% | eccentric | e = 70 mm |
| 11 | CFl-70 | NSC + UHPFRC 1% | 1% | eccentric | e = 70 mm |
| 12 | CF2-70 | NSC + UHPFRC 2% | 2% | eccentric | e = 70 mm |
| Description | Unit | NSC | UHPC 0% | UHPFRC 1% | UHPFRC 2% | Fiber | Steel Bars | |
|---|---|---|---|---|---|---|---|---|
| Ø 12 mm | Ø 8 mm | |||||||
| Slump | mm | 20.19 | 67.28 | 77.44 | 77.56 | |||
| Compressive strength | ||||||||
| Mean | MPa | 35.81 | 18.02 | 188.72 | 189.97 | |||
| Characteristic | MPa | 33.74 | 178.85 | 181.25 | 182.59 | |||
| Modulus rupture (flexural) strength | MPa | 3.42 | ||||||
| Density | kg/m3 | 2197.20 | 2295.67 | 2370.33 | 2425.50 | 7850 | 7850 | 7850 |
| Modulus elasticity | MPa | 33,768.87 | 50,307.63 | 50,501.98 | 50,619.86 | 200,000 | 200,000 | 200,000 |
| Length | mm | 10 | ||||||
| Diameter | mm | 0.2 | ||||||
| Tensile strength, | ||||||||
| Splitting/indirect Flexural | MPa | 2.85 | ||||||
| MPa | 8.04 | 15.57 | 16.02 | |||||
| Axial | MPa | |||||||
| Ultimate | MPa | 0.85 | 1.08 | 7.30 | 1250 | 678.53 | 641.8 | |
| Yield | MPa | 582.08 | 550.53 | |||||
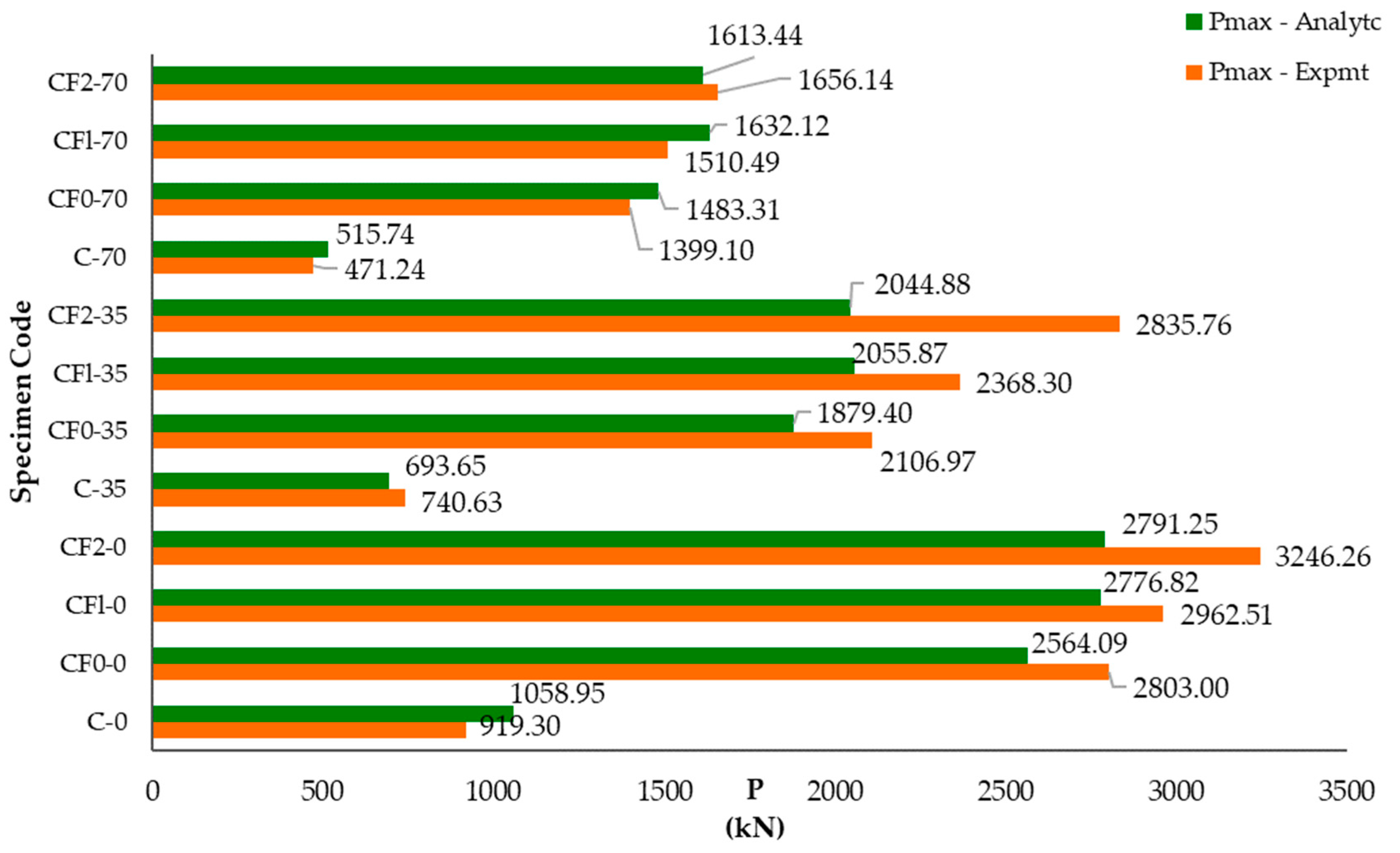
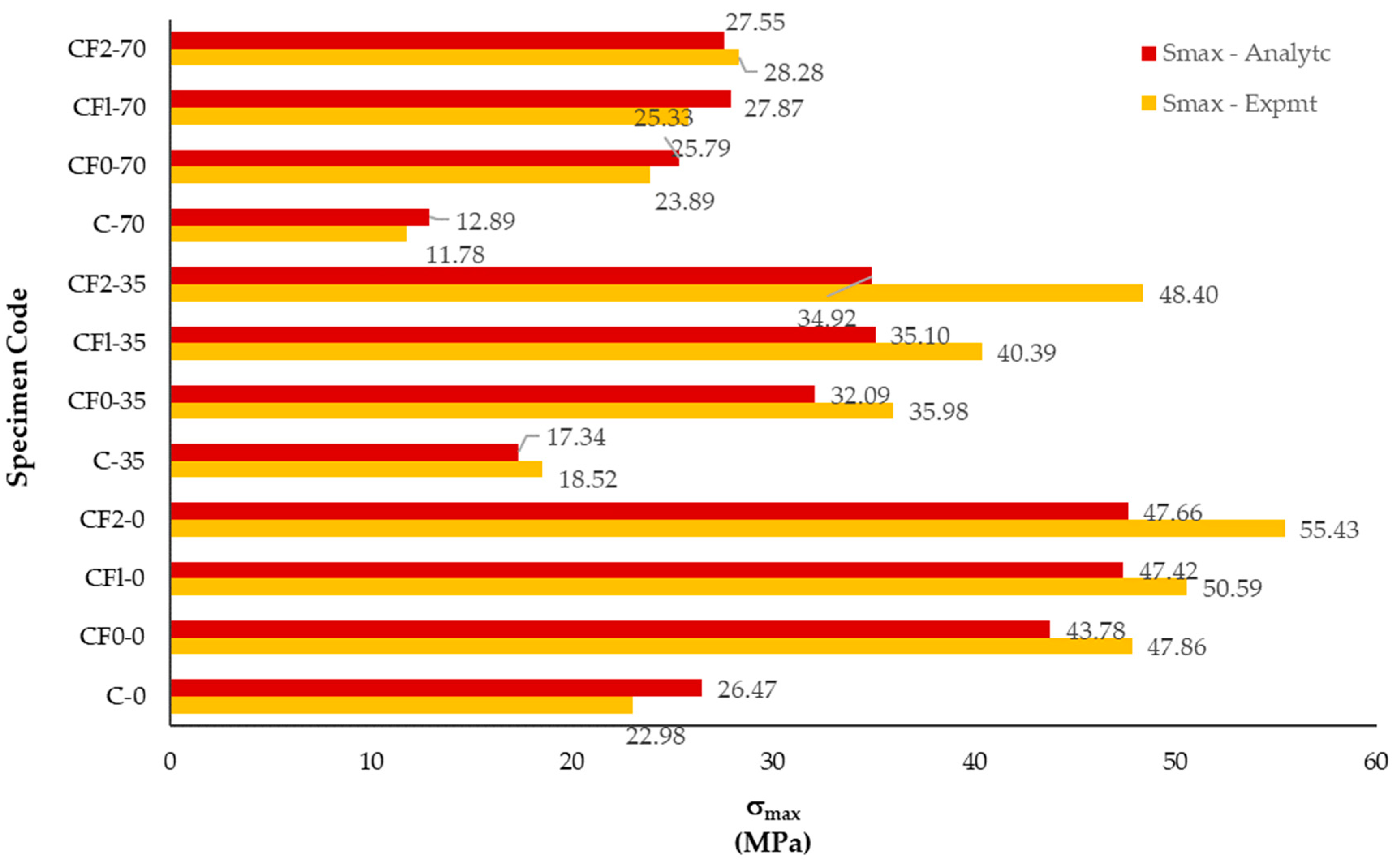
| No | Specimen Code | Experimental Result | Analytical Result | ||
|---|---|---|---|---|---|
| Pmax | σmax | Pmax | σmax | ||
| (kN) | (MPa) | (kN) | (MPa) | ||
| 1 | C-0 | 919.30 | 22.98 | 1058.95 | 26.47 |
| 2 | CF0-0 | 2803.00 | 47.86 | 2564.09 | 43.78 |
| 3 | CFl-0 | 2962.51 | 50.59 | 2776.82 | 47.42 |
| 4 | CF2-0 | 3246.26 | 55.43 | 2791.25 | 47.66 |
| 5 | C-35 | 740.63 | 18.52 | 693.65 | 17.34 |
| 6 | CF0-35 | 2106.97 | 35.98 | 1879.40 | 32.09 |
| 7 | CFl-35 | 2368.30 | 40.39 | 2055.87 | 35.10 |
| 8 | CF2-35 | 2835.76 | 48.40 | 2044.88 | 34.92 |
| 9 | C-70 | 471.24 | 11.78 | 515.74 | 12.89 |
| 10 | CF0-70 | 1399.10 | 23.89 | 1483.31 | 25.33 |
| 11 | CFl-70 | 1510.49 | 25.79 | 1632.12 | 27.87 |
| 12 | CF2-70 | 1656.14 | 28.28 | 1613.44 | 27.55 |
| Description | Unit | Current Study | Wu et al. |
|---|---|---|---|
| Column dimension | |||
| width | mm | 242 | 400 |
| height | mm | 750 | 1200 |
| UHPFRC confinement | |||
| materials | |||
| thickness | mm | 21 | 20 |
| fiber volume | % | 2 | 2 |
| No | Specimen Code | Ratio | |||
|---|---|---|---|---|---|
| Specimen/Control | |||||
| Pmax | LV | σmax | εmax | ||
| 1 | C-0 | 1.00 | 1.00 | 1.00 | 1.00 |
| 2 | CF0-0 | 3.05 | 1.41 | 2.08 | 1.30 |
| 3 | CFl-0 | 3.22 | 1.42 | 2.20 | 1.40 |
| 4 | CF2-0 | 3.53 | 1.49 | 2.41 | 1.49 |
| 5 | C-35 | 1.00 | 1.00 | 1.00 | 1.00 |
| 6 | CF0-35 | 2.84 | 1.24 | 1.94 | 1.23 |
| 7 | CFl-35 | 3.20 | 1.46 | 2.18 | 1.45 |
| 8 | CF2-35 | 3.83 | 1.61 | 2.61 | 1.60 |
| 9 | C-70 | 1.00 | 1.00 | 1.00 | 1.00 |
| 10 | CF0-70 | 2.97 | 1.03 | 2.03 | 1.43 |
| 11 | CFl-70 | 3.21 | 1.14 | 2.19 | 1.14 |
| 12 | CF2-70 | 3.51 | 1.16 | 2.40 | 1.16 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Susilorini, R.M.I.R.; Kusumawardaningsih, Y. Advanced Study of Columns Confined by Ultra-High-Performance Concrete and Ultra-High-Performance Fiber-Reinforced Concrete Confinements. Fibers 2023, 11, 44. https://doi.org/10.3390/fib11050044
Susilorini RMIR, Kusumawardaningsih Y. Advanced Study of Columns Confined by Ultra-High-Performance Concrete and Ultra-High-Performance Fiber-Reinforced Concrete Confinements. Fibers. 2023; 11(5):44. https://doi.org/10.3390/fib11050044
Chicago/Turabian StyleSusilorini, Rr. M. I. Retno, and Yuliarti Kusumawardaningsih. 2023. "Advanced Study of Columns Confined by Ultra-High-Performance Concrete and Ultra-High-Performance Fiber-Reinforced Concrete Confinements" Fibers 11, no. 5: 44. https://doi.org/10.3390/fib11050044
APA StyleSusilorini, R. M. I. R., & Kusumawardaningsih, Y. (2023). Advanced Study of Columns Confined by Ultra-High-Performance Concrete and Ultra-High-Performance Fiber-Reinforced Concrete Confinements. Fibers, 11(5), 44. https://doi.org/10.3390/fib11050044









