Bending Behavior Analysis of Box Beams with the Reinforcement of Composite Materials for Wind Turbine Blades
Abstract
:1. Introduction
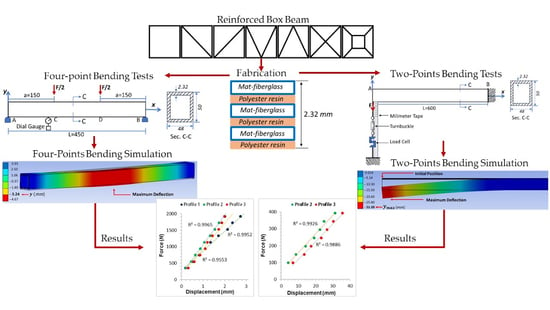
2. Materials and Methods
2.1. Materials
2.2. Methods
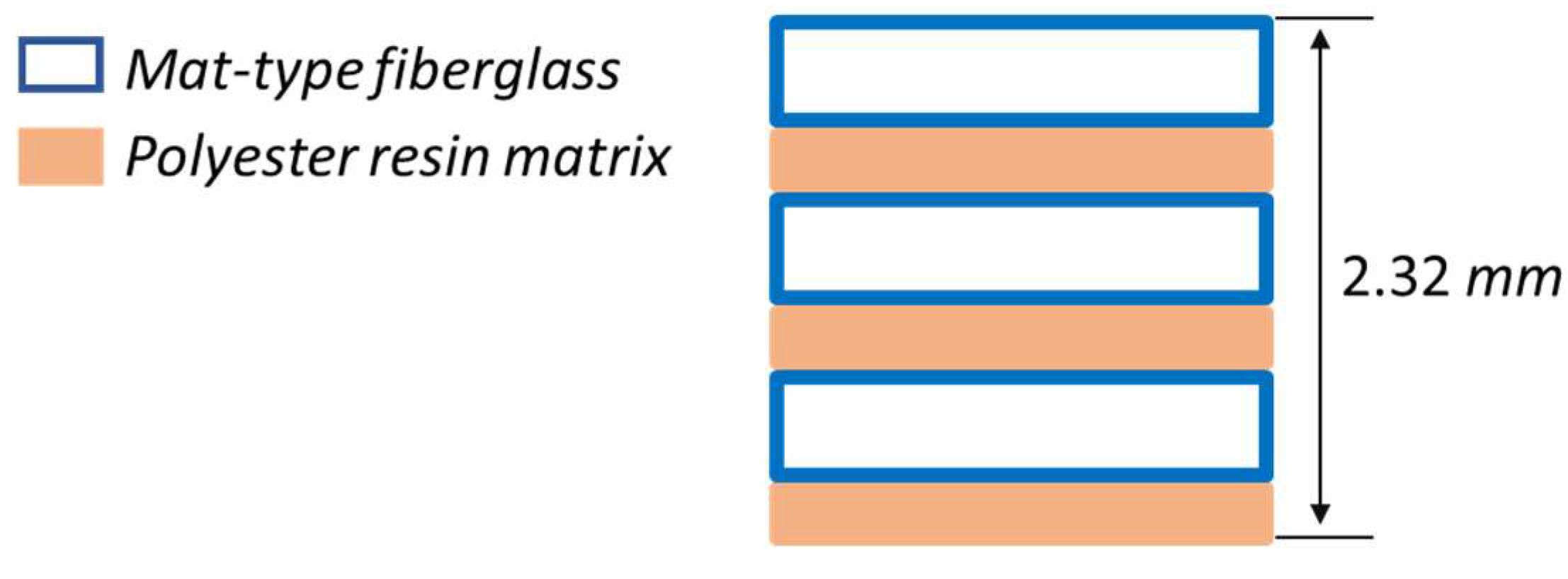
2.3. Beam Manufacturing
2.4. Experimental Setup and Instrumentation
2.4.1. Four-Point Bending Tests
2.4.2. Two-Points Bending Tests
2.5. Finite Element Simulation
3. Results
3.1. Design of Experiments
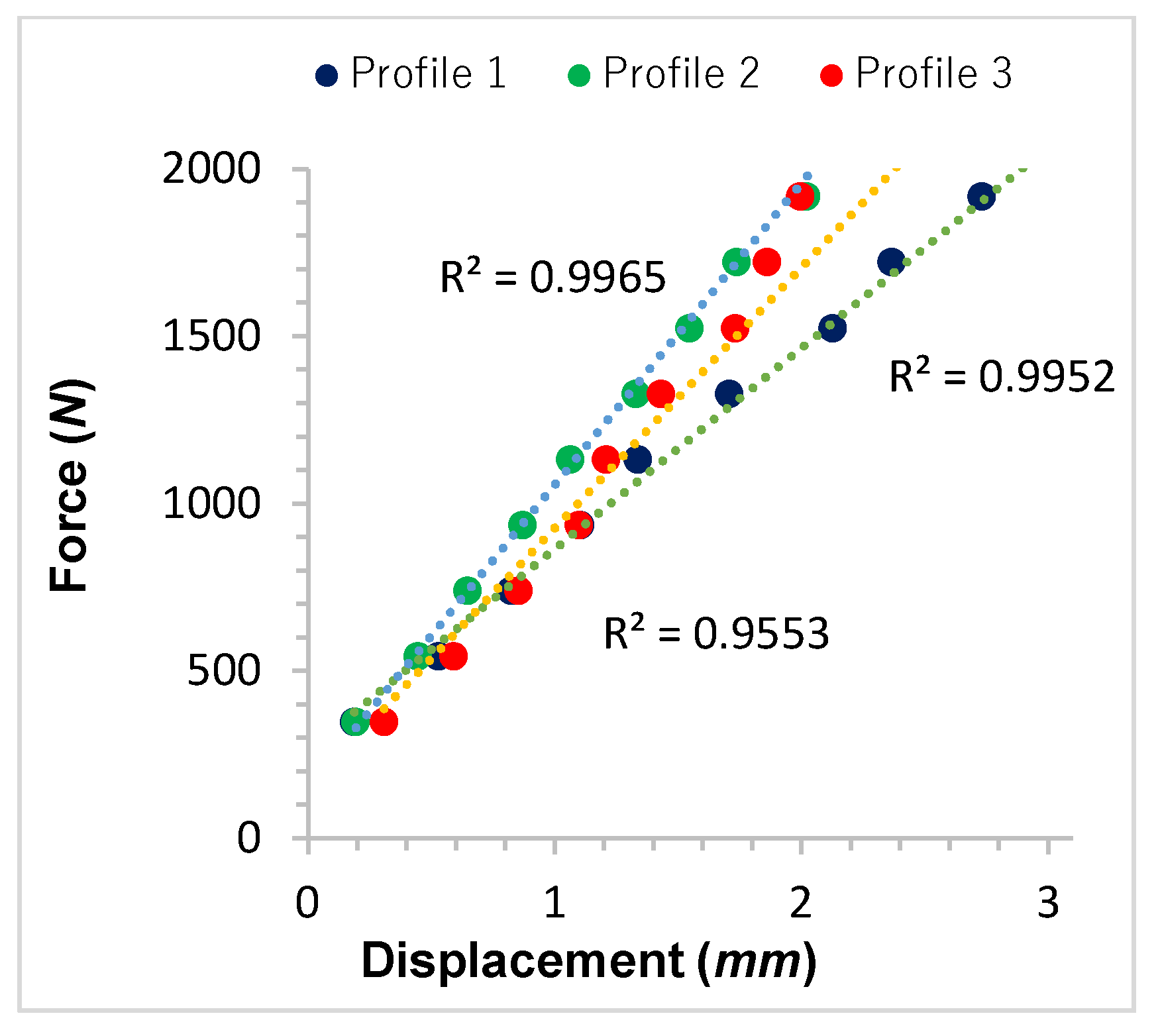
3.2. Four-Point Bending Tests
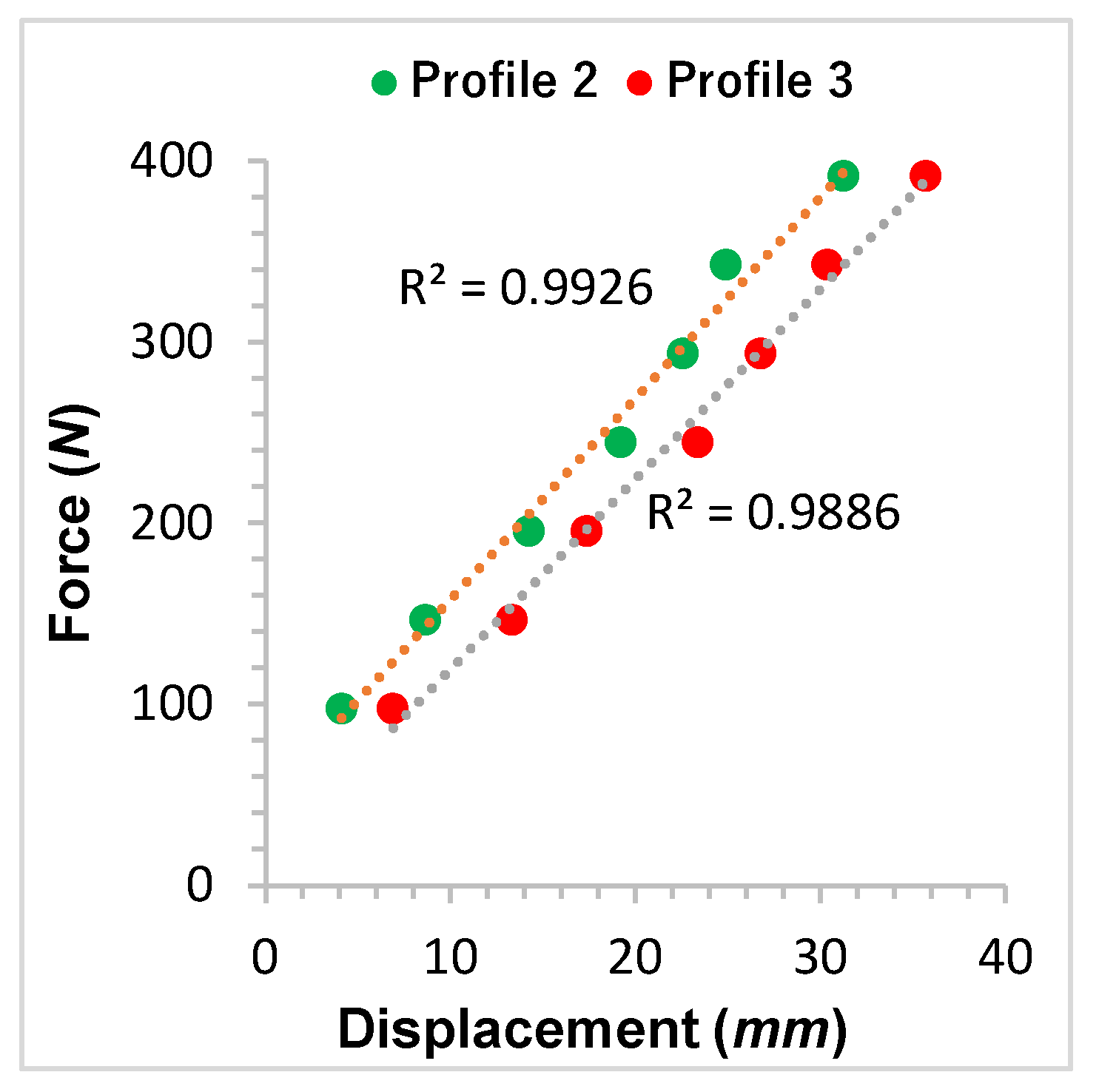
3.3. Bending Tests at Two-Points
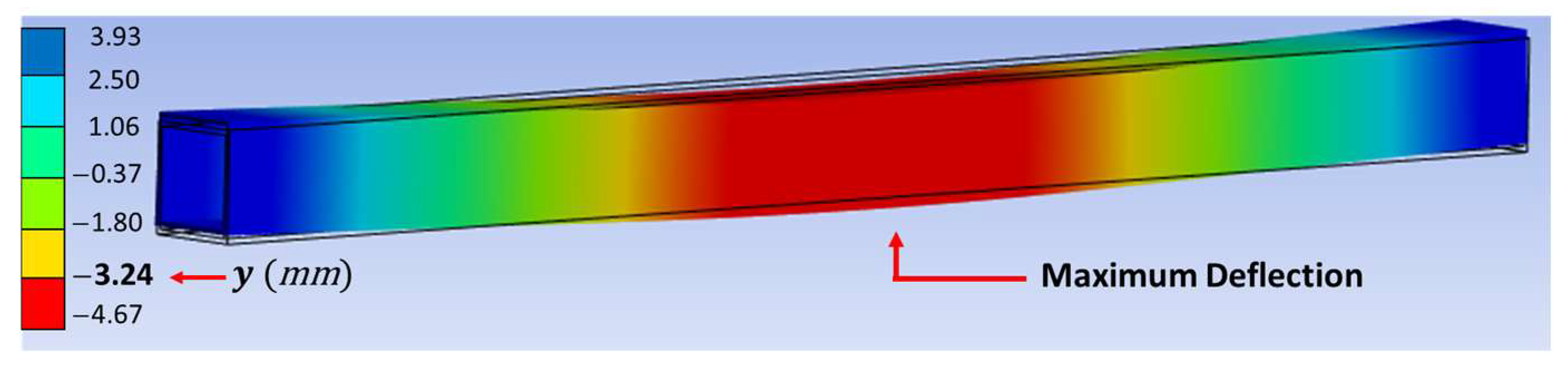
3.4. Four-Point Bending Simulation
3.5. Two-Point Bending Simulation
4. Discussion
5. Conclusions
- In the bending analysis at four points, it was determined that profile 2, with an inverted V shape, and profile 3, with an X shape, decrease their bending by 30.09% and 19.41%, respectively, compared to profile 1.
- Comparisons between simulations and experiments were made regarding the bending response, and the most excellent convergence in the results was obtained with the bending tests at two points, with an error of less than 10%.
- The box beam reinforced with the same composite material has a higher structural resistance of 43.41% (profile 2) and 24.33% (profile 3) in four-point bending tests. In addition, by using the same material for structural reinforcements, properties such as lightness and flexibility are preserved.
- The elastic modulus of the material was determined theoretically and experimentally by bending tests at two points and bending tests at four points. The values range from 3.66 GPa to 6.57 GPa, respectively.
- Using inverted V-shaped fiberglass and polyester resin laminates as a structural reinforcement improved the flexural strength of the box beam by 43.41%. In this way, the reinforcement of profile 2 with an inverted V shape improves the resistance of the box beams when they are subjected to loads in the flag direction. For this reason, applying WTB is a viable option.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Cooperman, A.; Eberle, A.; Lantz, E. Wind Turbine Blade Material in the United States: Quantities, Cost, and end-of-life Options. Resour. Conserv. Recycl. 2021, 168, 105439. [Google Scholar] [CrossRef]
- Santiuste, C. Análisis y Modelización de Vigas de Tipo Laminado Sometidas a Cargas Impulsivas. Ph.D. Thesis, Universidad Carlos III de Madrid, Madrid, Spain, 2007. [Google Scholar]
- Hernandez-Estrada, E.; Lastres-Danguillecourt, O.; Robles-Ocampo, J.B.; Lopez-Lopez, A.; Sevilla-Camacho, P.Y.; Perez-Sariñana, B.Y.; Dorrego-Portela, J.R. Considerations for the structural analysis and design of wind turbine towers: A review. Renew. Sustain. Energy Rev. 2021, 137, 110447. [Google Scholar] [CrossRef]
- Manwell, J.F.; McGowan, J.G.; Rogers, A.L. Wind Energy Explained, Theory, Design and Application, 2nd ed.; Wiley: Hoboken, NJ, USA, 2009. [Google Scholar]
- Maheri, A. Multiobjective Optimisation and Integrated Design of Wind Turbine Blades using WTBM-ANSYS for High Fidelity Structural Analysis. Renew. Energy 2019, 145, 814–834. [Google Scholar] [CrossRef]
- Veledurthi, A.; Bolleddu, V. Experimental Buckling Analysis of NACA 63415 Aerofoil Wind Turbine Blade. Mater. Today Proc. 2021, 46, 205–211. [Google Scholar] [CrossRef]
- Kam, T.Y.; Su, H.M.; Huang, C.Y. Quasi-Static Buckling and First-Ply Failure Loads of Shear Web Reinforced Glass-Fabric Composite Wind Blades. Compos. Struct. 2017, 160, 1225–1235. [Google Scholar] [CrossRef]
- Zhang, L.; Guo, Y.; Yu, L.; Wei, X.; Liu, W.; Huang, X. Structural Collapse Characteristics of a 48.8 m Wind Turbine Blade under Ultimate Bending Loading. Eng. Fail. Anal. 2019, 106, 104150. [Google Scholar] [CrossRef]
- Zuo, Y.; Montesano, J.; Veer Singh, C. Assessing Progressive Failure in Long Wind Turbine Blades Under Quasi-Static and Cyclic Loads. Renew. Energy 2018, 119, 754–766. [Google Scholar] [CrossRef]
- Jensen, F.M.; Puri, A.S.; Dear, J.P.; Branner, K.; Morris, A. Investigating the Impact of Non-Linear Geometrical Effects on Wind Turbine Blades—Part 1 Current Status of Design and Test Methods and Future Challenges in Design Optimization. Wind Energy 2011, 14, 239–254. [Google Scholar] [CrossRef]
- Clausen, P.; Reynal, F.; Wood, D. Advances in Wind Turbine Blade Design and Materials; Elsevier Science: Exeter Devon, UK, 2013. [Google Scholar]
- Jensen, F.M.; Weaver, P.M.; Cecchini, L.S.; Stang, H.; Nielsen, R.F. The Brazier Effect in Wind Turbine Blades and its Influence on Design. Wind Energy 2012, 15, 319–333. [Google Scholar] [CrossRef]
- Eker, B.; Akdogan, A.; Vardar, A. Using of Composite Material in Wind Turbine Blades. Appl. Sci. 2006, 6, 2917–2921. [Google Scholar] [CrossRef]
- Ghasemi, A.R.; Mohandes, M. Composite Blades of Wind Turbine: Design, Stress Analysis, Aeroelasticity, and Fatigue. In Wind Turbines—Design, Control and Applications; IntechOpen: London, UK, 2016. [Google Scholar]
- Tang, J.; Chen, X. Experimental Investigation on Ultimate Strength and Failure Response of Composite Box Beams Used in Wind Turbine Blades. Compos. Struct. 2018, 198, 19–34. [Google Scholar] [CrossRef]
- Doeva, O.; Masjedi, P.K.; Weaver, P.M. Static Deflection of Fully Coupled Composite Timoshenko Beams: An Exact Analytical Solution. Eur. J. Mech. A Solids 2020, 81, 103975. [Google Scholar] [CrossRef]
- Masjedi, P.K.; Doeva, O.; Weaver, P.M. Closed-Form Solutions for the Coupled Deflection of anisotropic Euler–Bernoulli composite beams with arbitrary boundary conditions. Thin Walled Struct. 2021, 161, 107479. [Google Scholar] [CrossRef]
- Chen, H.; Yu, W.; Capellaro, M. A Critical Assessment of Computer Tools for Calculating Composite Wind Turbine Blade Properties. Wind Energy 2010, 13, 497–516. [Google Scholar] [CrossRef]
- Li, J.; Wang, J.; Yu, L.; Liu, W.; Zhang, L. Study on Failure Mechanisms of Composite Box Beam on Wind Turbine Blades under Extreme Combined Loading. Mech. Adv. Mater. Struct. 2020, 29, 2176–2185. [Google Scholar] [CrossRef]
- Cestino, E.; Frulla, G.; Piana, P.; Duella, R. Numerical/Experimental Validation of Thin-Walled Composite Box Beam Optimal Design. Aerospace 2020, 7, 111. [Google Scholar] [CrossRef]
- Du, Y.; Zhou, S.; Jing, X.; Peng, Y.; Wu, H.; Kwok, N. Damage Detection Techniques for Wind Turbine Blades A Review. Mech. Syst. Signal Process. 2020, 141, 106445. [Google Scholar] [CrossRef]
- Chen, X.; Tang, J.; Yang, K. Modeling Multiple Failures of Composite Box Beams used in Wind Turbine Blades. Compos. Struct. 2019, 217, 130–142. [Google Scholar] [CrossRef]
- Emara, M.; EI-Zohairy, A.; Fekry, M.; Husain, M. Effect of using ECC Layer on the Flexural Performance of RC Beams Previously Strengthened with EB CFRP Laminates. Sustainability 2022, 14, 16990. [Google Scholar] [CrossRef]
- Bilston, D.; Ruan, D.; Candido, A.; Durandet, Y. Parametric Study of the Cross-Section Shape of Aluminium tubes in Dynamic three-point bending. Thin Walled Struct. 2019, 136, 315–322. [Google Scholar] [CrossRef]
- Lopez-Lopez, A.; Robles-Ocampo, J.B.; Sevilla-Camacho, P.Y.; Lastres-Danguillecourt, O.; Muniz, J.; Perez-Sariñana, B.Y.; de la Cruz, S. Dynamic Instability of a Wind Turbine Blade Due to Large Deflections: An Experimental Validation. J. Mech. Eng. 2020, 66, 523–533. [Google Scholar] [CrossRef]
- Chen, X.; Haselbach, P.U.; Branner, K.; Madsen, S.H. Effects of Different Material Failures and Surface Contact on Structural Response of Trailing Edge Sections in Composite Wind Turbine Blades. Compos. Struct. 2019, 226, 111306. [Google Scholar] [CrossRef]
- Gliszczyński, A.; Kubiak, T. Load-Carrying Capacity of Thin-Walled Composite Beams Subjected to Pure Bending. Thin Walled Struct. 2017, 115, 76–85. [Google Scholar] [CrossRef]
- Obst, M.; Kurpisz, D.; Paczos, P. The Experimental and Analytical Investigations of Torsion Phenomenon of Thin-Walled Cold Formed Channel Beams Subjected to Four-Point Bending. Thin Walled Struct. 2016, 106, 179–186. [Google Scholar] [CrossRef]
- Yeou-Fong, L.; Tseng-Hsing, H.; Fu-Chr, H. Study on Improving the Mechanical Behaviors of the Pultruded GFRP Composite Material Members. Sustainability 2019, 11, 577. [Google Scholar]
- ASTM D7264; Standard Test Method for Flexural Properties of Polymer Matrix Composite Materials. American Society for Testing and Materials: West Conshohocken, PA, USA, 2021.
- NMX-C-191-ONNCCE-2004; Industria de la Construcción-Concreto Determinación de la Resistencia a la Flexión del Concreto Usando una viga Simple con Carga en los Tercios del Claro. Organismo Nacional de Normalización y Certificación de la Construcción y Edificación, S.C.: Juárez, México, 2004.
- Barbero, E.J. Introduction to Composite Materials Desing, 2nd ed.; CRC Press: Boca Raton, FL, USA, 2011. [Google Scholar]
- Vcelka, M.; Dunn, M.; Durandet, Y.; Berndt, C.C.; Ruan, D. Behavior of CFRC/Al Foam Composite Sandwich Beams Under Three-Point Bending. Adv. Eng. Mater. 2014, 16, 9–14. [Google Scholar] [CrossRef]
- Vcelka, M.; Durandet, Y.; Berndt, C.C.; Ruan, D. Deformation and Energy Absorption of Composite Sandwich Beams. Key Eng. Mater. 2014, 626, 468–473. [Google Scholar] [CrossRef]
- Abbass, A.; Abid, S.; Özakça, M. Experimental Investigation on the Effect of Steel Fibers on the Flexural Behavior and Ductility of High-Strength Concrete Hollow Beams. Adv. Civ. Eng. 2019, 2019, 8390345. [Google Scholar] [CrossRef]
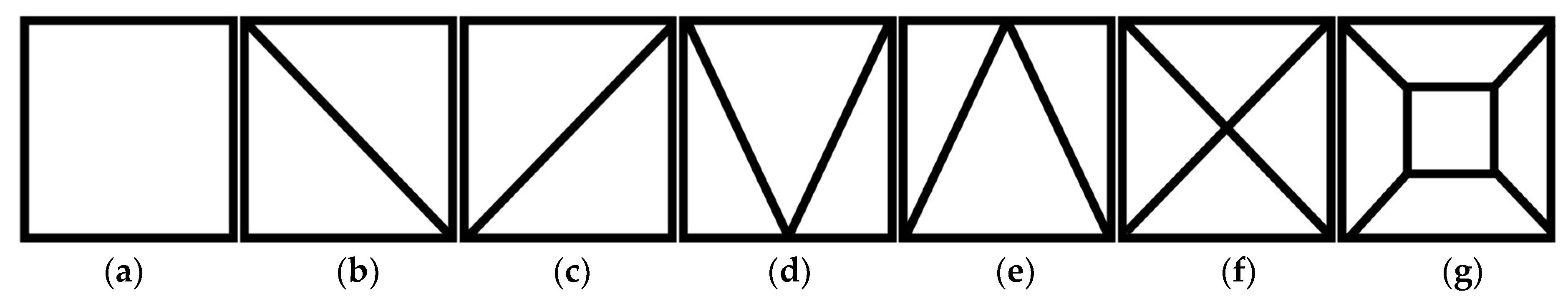
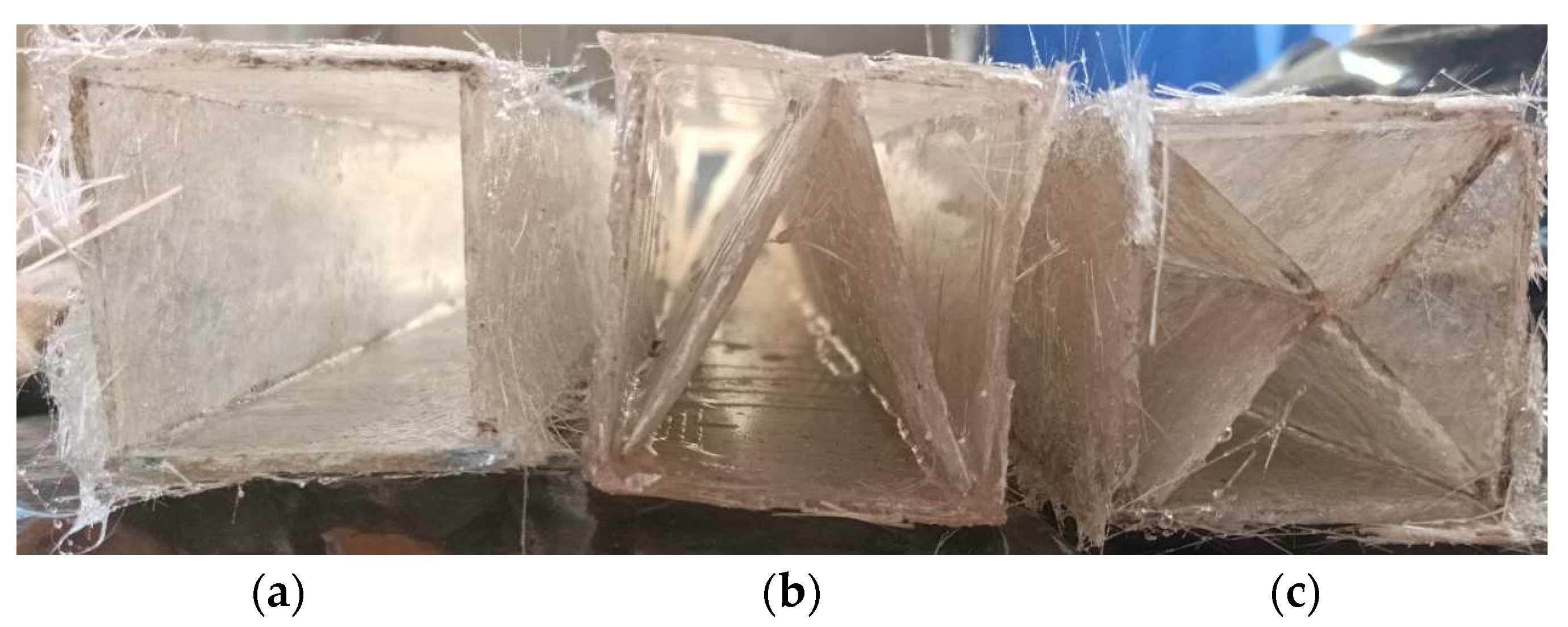

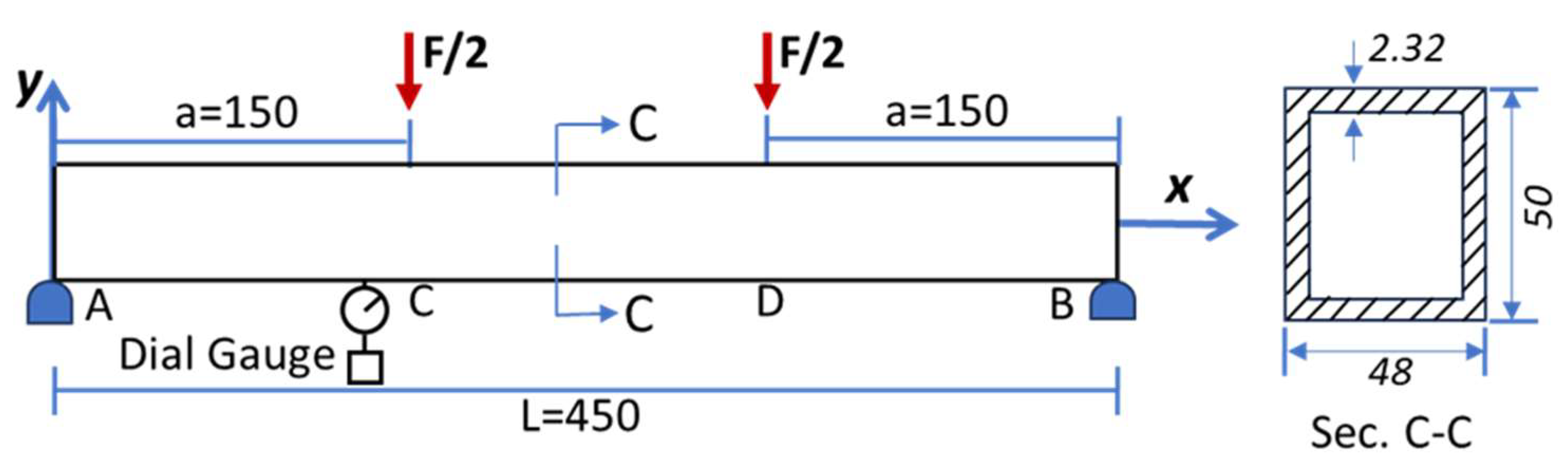




| Cross-Sectional Area (mm2) | Inertia Moment (mm4) | |
|---|---|---|
| Profiles | ||
| 1 | 433.19 | 162,769.25 |
| 2 | 661.30 | 201,114.18 |
| 3 | 708.10 | 250,022.23 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Maldonado-Santiago, O.; Robles-Ocampo, J.B.; Gálvez, E.; Sevilla-Camacho, P.Y.; de la Cruz, S.; Rodríguez-Reséndiz, J.; Hernández, E. Bending Behavior Analysis of Box Beams with the Reinforcement of Composite Materials for Wind Turbine Blades. Fibers 2023, 11, 99. https://doi.org/10.3390/fib11120099
Maldonado-Santiago O, Robles-Ocampo JB, Gálvez E, Sevilla-Camacho PY, de la Cruz S, Rodríguez-Reséndiz J, Hernández E. Bending Behavior Analysis of Box Beams with the Reinforcement of Composite Materials for Wind Turbine Blades. Fibers. 2023; 11(12):99. https://doi.org/10.3390/fib11120099
Chicago/Turabian StyleMaldonado-Santiago, Ofelia, Jose Billerman Robles-Ocampo, Eduardo Gálvez, Perla Yazmin Sevilla-Camacho, Sergio de la Cruz, Juvenal Rodríguez-Reséndiz, and Edwin Hernández. 2023. "Bending Behavior Analysis of Box Beams with the Reinforcement of Composite Materials for Wind Turbine Blades" Fibers 11, no. 12: 99. https://doi.org/10.3390/fib11120099
APA StyleMaldonado-Santiago, O., Robles-Ocampo, J. B., Gálvez, E., Sevilla-Camacho, P. Y., de la Cruz, S., Rodríguez-Reséndiz, J., & Hernández, E. (2023). Bending Behavior Analysis of Box Beams with the Reinforcement of Composite Materials for Wind Turbine Blades. Fibers, 11(12), 99. https://doi.org/10.3390/fib11120099