Effect of Microstructure on the Thermal Conductivity of Plasma Sprayed Y2O3 Stabilized Zirconia (8% YSZ)
Abstract
:1. Introduction
2. Materials and Methods
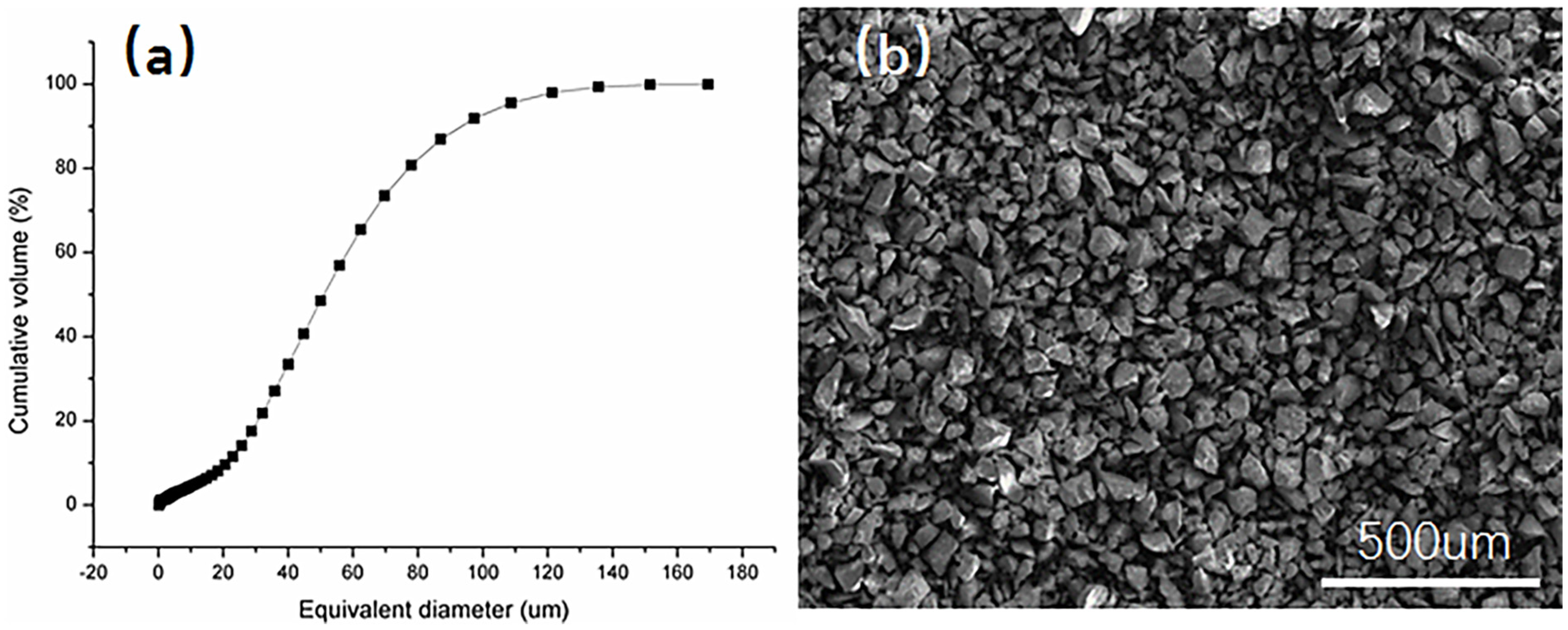
2.1. Materials and Preparation
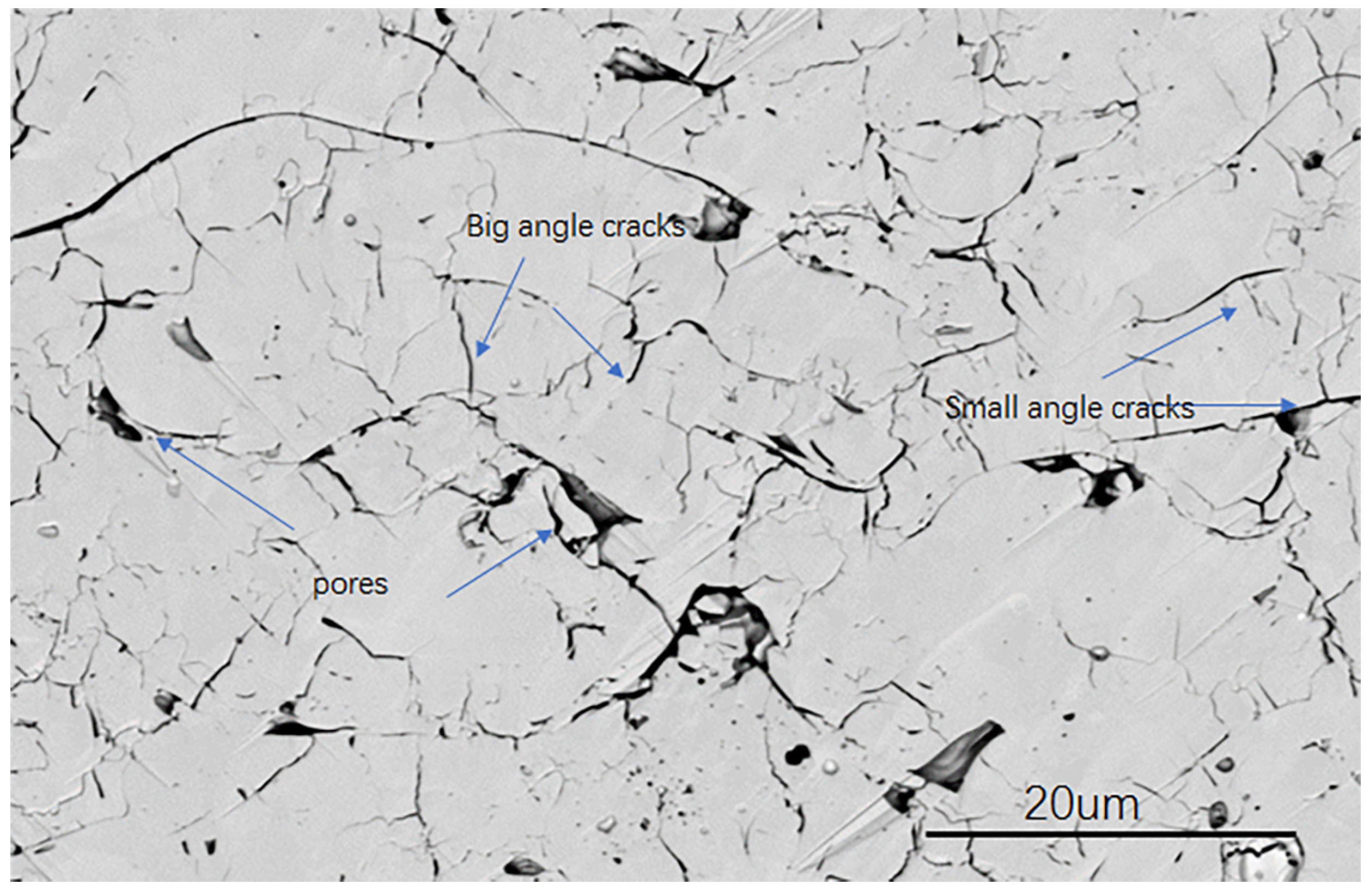
2.2. Microstructure Characterization
2.3. Thermal Conductivity Measurements
3. Results and Discussion
3.1. Spray Parameters and Phase Content
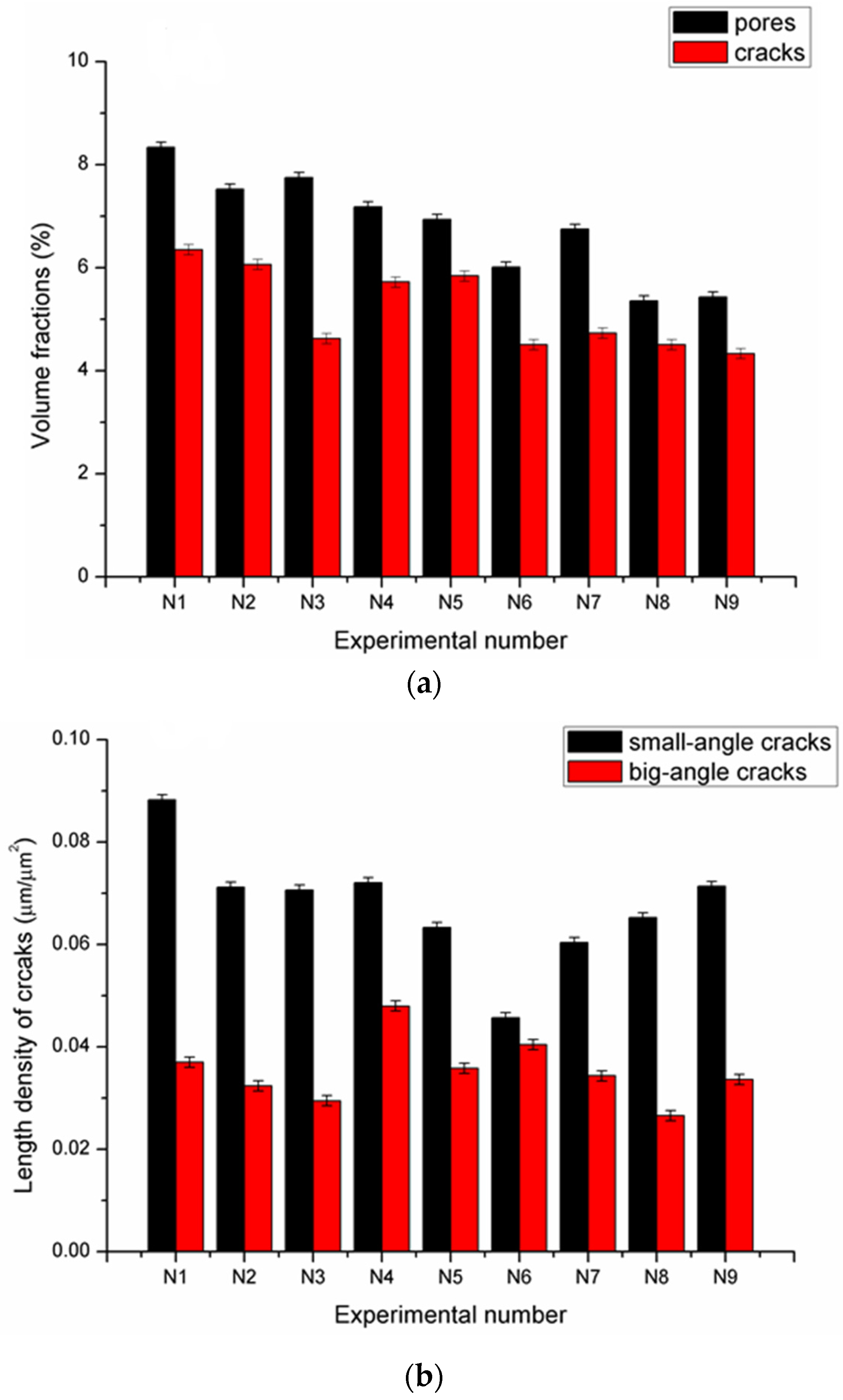
3.2. Microstructure of TBCs
| Porosity (%) = 10.825 − 0.00014X1 − 0.00042X2 − 1.499X3 | R2 = 0.90 | (1) |
| Crack-porosity (%) = 14.599 − 0.015816965X1 − 0.001496453X2 − 0.878686315X3 | R2 = 0.79 | (2) |
| Length Density of Small-angle Cracks (μm/μm2) = 0.215790122 − 0.000371226X1 − 0.00000980847X2 − 0.026043962X3 | R2 = 0.91 | (3) |
| Length Density of Big-angle Cracks (μm/μm2) = 0.049720041 + 0.000355754X1 − 2.94618 × 10−5X2 − 0.001130176X3 | R2 = 0.27 | (4) |
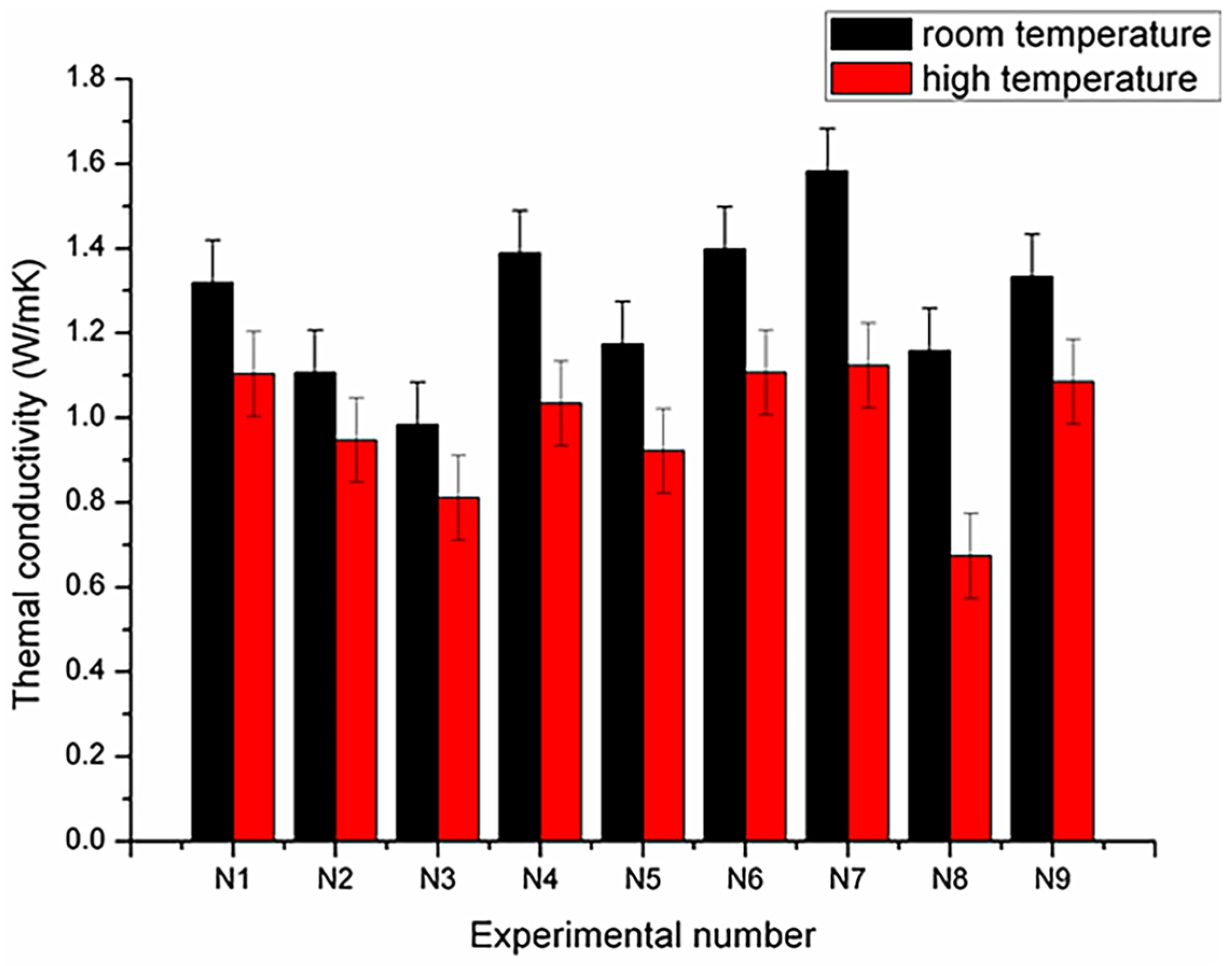
3.3. Relationship between Microstructure and Thermal Conductivity
| Thermal Conductivity (room temperature) = 1.79 − 0.104864402P − 0.038644461C − 0.009469272S + 23.24351385B − 0.170350528G | R2 = 0.82 | (5) |
| Thermal conductivity (1200 °C) = 0.476267554 + 21.50434266B + 0.092513106M − 0.168259582G | R2 = 0.888 | (6) |
4. Conclusions
- Spraying processes affect the m-phase contents of the coating, and increasing the current and the plasma gas flow rates will lead to an increase in the m-phase content.
- The volume change associated with the phase transition during the spraying process affects the microstructure of the coating. M-phase content and the in-flight particle state play significant roles in the formation of micro-morphology. The formation of big-angle cracks results from stress release, and their length density generally had very small and random variations with the process parameters.
- At low temperature (25 °C), pores, cracks (particularly the small-angle-cracks) and grain boundary density decrease the thermal conductivity, but the big-angle cracks increase the heat flow into the coating. At high temperatures (1200 °C), the effect of pores and cracks is negligible. The grain boundary density reduces the thermal conductivity. The effect of big-angle cracks on heat diffusion at both low and high temperatures is significant.
Acknowledgments
Author Contributions
Conflicts of Interest
References
- Feuerstein, A.; Knapp, J.; Taylor, T. Technical and economical aspects of current thermal barrier coating systems for gas turbine engines by thermal spray and EBPVD: A Review. J. Therm. Spray Technol. 2008, 17, 199–213. [Google Scholar] [CrossRef]
- Liebert, C.H.; Stepka, F.S. Ceramic thermal barrier coatings for cooled turbines. J. Aircr. 2015, 14, 487–493. [Google Scholar] [CrossRef]
- Osorio, J.D. Correlations between microstructure and mechanical properties of air plasma-sprayed thermal barrier coatings exposed to a high temperature. J. Am. Ceram. Soc. 2013, 96, 3901–3907. [Google Scholar] [CrossRef]
- Lee, S.Y.; Kwon, J.Y.; Kang, T.W. Effects of thickness on thermal and mechanical properties of air-plasma sprayed thermal barrier coatings. Mater. Sci. Forum 2010, 658, 372–375. [Google Scholar] [CrossRef]
- Liu, H.; Jazi, H.R.S.; Bussmann, M. Experiments and modeling of rapid solidification of plasma-sprayed yttria-stabilized zirconia. Acta Mater. 2009, 57, 6013–6021. [Google Scholar] [CrossRef]
- Chi, W.; Sanjay, S.; Wang, H. Microstructure-thermal conductivity relationships for plasma-sprayed yttria-stabilized zirconia coatings. J. Am. Ceram. Soc. 2008, 91, 2636–2645. [Google Scholar] [CrossRef]
- Zhao, L.; Bai, Y.; Tang, J.J. Effect of particle in-flight behavior on the composition of thermal barrier coatings. Appl. Surf. Sci. 2013, 286, 184–191. [Google Scholar] [CrossRef]
- Cheng, D.; Xu, Q.; Lavernia, E.J. The effect of particle size and morphology on the in-flight behavior of particles during high-velocity oxyfuel thermal spraying. Metall. Mater. Trans. B 2001, 32, 525–535. [Google Scholar] [CrossRef]
- Vaßen, R.; Czech, N.; Malléner, W. Influence of impurity content and porosity of plasma-sprayed yttria-stabilized zirconia layers on the sintering behaviour. Surf. Coat. Technol. 2001, 141, 135–140. [Google Scholar] [CrossRef]
- Arai, M.; Ochiai, H.; Suidzu, T. A novel low-thermal-conductivity plasma-sprayed thermal barrier coating controlled by large-pores. Surf. Coat. Technol. 2016, 285, 120–127. [Google Scholar] [CrossRef]
- Song, X.; Liu, Z.; Suhonen, T. Effect of melting state on the thermal shock resistance and thermal conductivity of APS ZrO2–7.5 wt % Y2O3, coatings. Surf. Coat. Technol. 2015, 270, 132–138. [Google Scholar] [CrossRef]
- Wei, S.; Fu-chi, W.; Qun-Bo, F.; Zhuang, M. Effects of defects on the effective thermal conductivity of thermal barrier coatings. Appl. Math. Model. 2012, 36, 1995–2002. [Google Scholar] [CrossRef]
- Raghavan, S.; Wang, H.; Dinwiddie, R.B. The effect of grain size, porosity and yttria content on the thermal conductivity of nanocrystalline zirconia. Scr. Mater. 1998, 39, 1119–1125. [Google Scholar] [CrossRef]
- Dwivedi, G.; Wentz, T.; Sampath, S.; Nakamura, T. Assessing process and coating reliability through monitoring of process and design relevant coating properties. J. Therm. Spray Technol. 2010, 19, 695–712. [Google Scholar] [CrossRef]
- Zhang, C. Effect of in-flight particle velocity on the performance of plasma-sprayed YSZ electrolyte coating for solid oxide fuel cells. Surf. Coat. Technol. 2008, 202, 2654–2660. [Google Scholar] [CrossRef]
- Soyez, G.; Eastman, J.A.; Thompson, L.J. Grain-size-dependent thermal conductivity of nanocrystalline yttria-stabilized zirconia films grown by metal-organic chemical vapor deposition. Appl. Phys. Lett. 2000, 77, 1155–1157. [Google Scholar] [CrossRef]
- Limarga, A.M.; Clarke, D.R. The grain size and temperature dependence of the thermal conductivity of polycrystalline, tetragonal yttria-stabilized zirconia. Appl. Phys. Lett. 2011, 98, 211906. [Google Scholar] [CrossRef]
- Becher, P.F.; Swain, M.V. Grain-size-dependent transformation behavior in polycrystalline tetragonal zirconia. J. Am. Ceram. Soc. 2010, 75, 493–502. [Google Scholar] [CrossRef]
- Ganvir, A.; Kumar, C.; Gupta, M.; Nylen, P. Thermal conductivity in suspension sprayed thermal barrier coatings: Modeling and experiments. Therm. Spray Technol. 2017, 26, 71–82. [Google Scholar] [CrossRef]
- Wang, L.; Li, D.C.; Yang, J.S.; Shao, F.; Zhon, X.H. Modeling of thermal properties and failure of thermal barrier coatings with the use of finite element methods: A review. J. Eur. Ceram. Soc. 2016, 36, 1313–1331. [Google Scholar] [CrossRef]
- Qiao, J.H.; Bolot, R.; Liao, H.; Bertrand, P. A 3D finite-difference model for the effective thermal conductivity of thermal barrier coatings produced by plasma spraying. Int. J. Therm. Sci. 2013, 65, 120–126. [Google Scholar] [CrossRef]
- Fox, E.B.; Visser, A.E.; Bridges, N.J. Nanoparticle Enhanced Ionic Liquid Heat Transfer Fluids. U.S. Patent 20130068994 A1, 12 August 2014. [Google Scholar]
- Touloukian, Y.S.; Bucco, E.H. Thermophysical Properties of Matter—the TPRC Data Series. Volume 5. Specific Heat—Nonmetallic Solids; Springer: New York, NY, USA, 1970. [Google Scholar]
- Levi, C.G.; Hutchinson, J.W. Environmental degradation of thermal-barrier coatings by molten deposits. MRS Bull. 2012, 37, 932–941. [Google Scholar] [CrossRef]
- Wang, Y.; Xu, F.; Gauvin, R. Growth modes for monoclinic yttria-stabilized zirconia during the martensitic transformation. J. Am. Ceram. Soc. 2017, 100, 4874–4883. [Google Scholar] [CrossRef]
- Guo, H.B.; Vaben, R.; Stover, D. Atmospheric plasma sprayed thick thermal barrier coatings with high segmentation crack density. Surf. Coat. Technol. 2004, 186, 353–363. [Google Scholar] [CrossRef]
- Chen, N.; Song, X.; Liu, Z. Quantitative analysis of the relationship between microstructures and thermal conductivity for YSZ coatings. J. Therm. Spray Technol. 2017, 26, 745–754. [Google Scholar] [CrossRef]

| Spraying Conditions | N1 | N2 | N3 | N4 | N5 | N6 | N7 | N8 | N9 |
|---|---|---|---|---|---|---|---|---|---|
| Current (A) | 550 | 550 | 550 | 600 | 600 | 600 | 650 | 650 | 650 |
| Ar (L/min) | 35 | 40 | 36 | 35 | 40 | 36 | 35 | 40 | 36 |
| H2 (L/min) | 7 | 10 | 12 | 7 | 10 | 12 | 7 | 10 | 12 |
| Velocity (m/s) | 197 | 217 | 201 | 206 | 213 | 228 | 214 | 205 | 237 |
| Temperature (°C) | 2750 | 2931 | 2893 | 2835 | 3007 | 3221 | 2903 | 3079 | 3294 |
| Coating Parameters | N1 | N2 | N3 | N4 | N5 | N6 | N7 | N8 | N9 |
|---|---|---|---|---|---|---|---|---|---|
| Y2O3 Content (wt %) | 7.85 | 7.78 | 7.71 | 7.68 | 7.73 | 7.65 | 7.46 | 7.38 | 7.31 |
| m-ZrO2 Content (%) | 1.03 | 1.37 | 1.51 | 1.55 | 1.47 | 1.95 | 1.90 | 2.74 | 2.85 |
| Grain Boundary Density(m/μm2) | 2.59 | 2.56 | 2.61 | 3.48 | 2.29 | 2.87 | 1.42 | 3.43 | 2.43 |
© 2017 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Hu, N.; Khan, M.; Wang, Y.; Song, X.; Lin, C.; Chang, C.; Zeng, Y. Effect of Microstructure on the Thermal Conductivity of Plasma Sprayed Y2O3 Stabilized Zirconia (8% YSZ). Coatings 2017, 7, 198. https://doi.org/10.3390/coatings7110198
Hu N, Khan M, Wang Y, Song X, Lin C, Chang C, Zeng Y. Effect of Microstructure on the Thermal Conductivity of Plasma Sprayed Y2O3 Stabilized Zirconia (8% YSZ). Coatings. 2017; 7(11):198. https://doi.org/10.3390/coatings7110198
Chicago/Turabian StyleHu, Ningning, Matiullah Khan, Yongzhe Wang, Xuemei Song, Chucheng Lin, Chengkang Chang, and Yi Zeng. 2017. "Effect of Microstructure on the Thermal Conductivity of Plasma Sprayed Y2O3 Stabilized Zirconia (8% YSZ)" Coatings 7, no. 11: 198. https://doi.org/10.3390/coatings7110198
APA StyleHu, N., Khan, M., Wang, Y., Song, X., Lin, C., Chang, C., & Zeng, Y. (2017). Effect of Microstructure on the Thermal Conductivity of Plasma Sprayed Y2O3 Stabilized Zirconia (8% YSZ). Coatings, 7(11), 198. https://doi.org/10.3390/coatings7110198






