Study on Mechanical Properties and Structural Deformation of [111] Oriented Mg-Li Alloy
Abstract
:1. Introduction
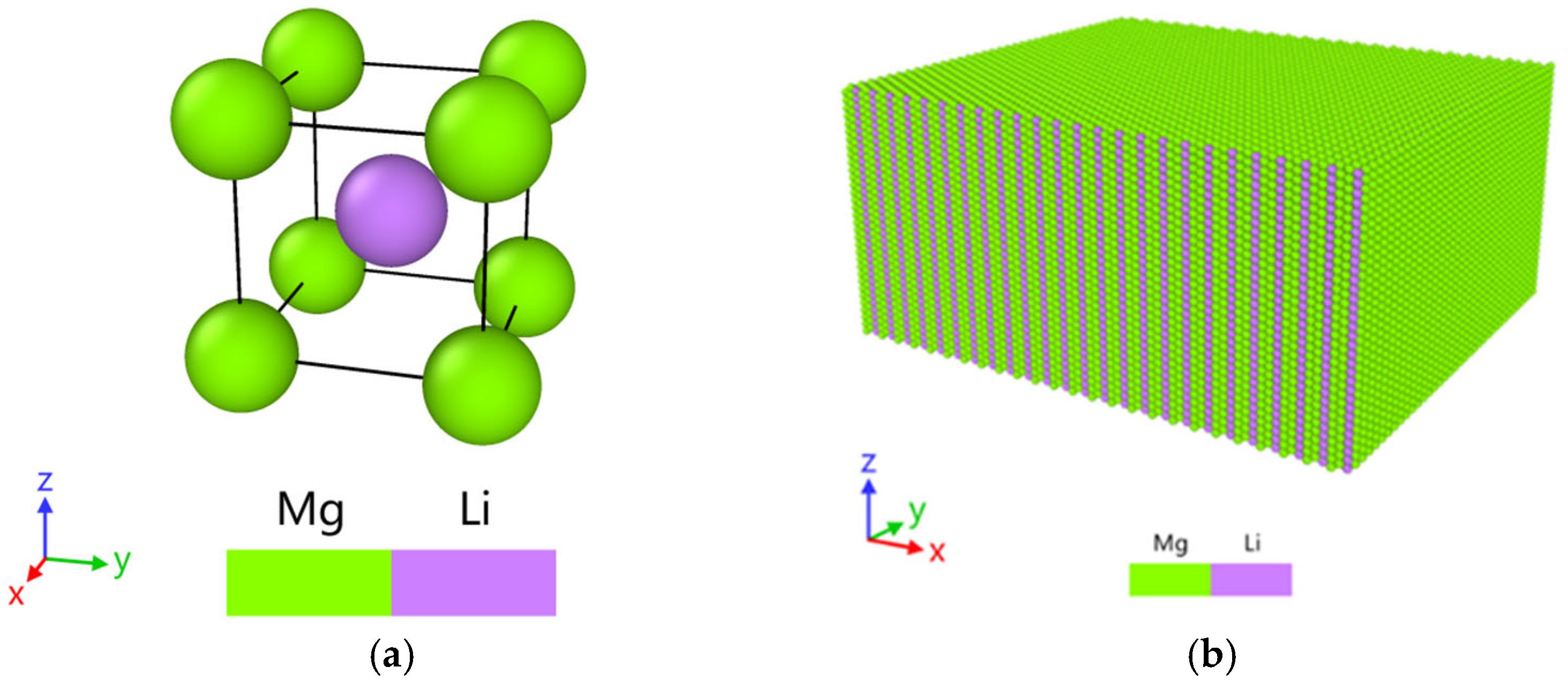
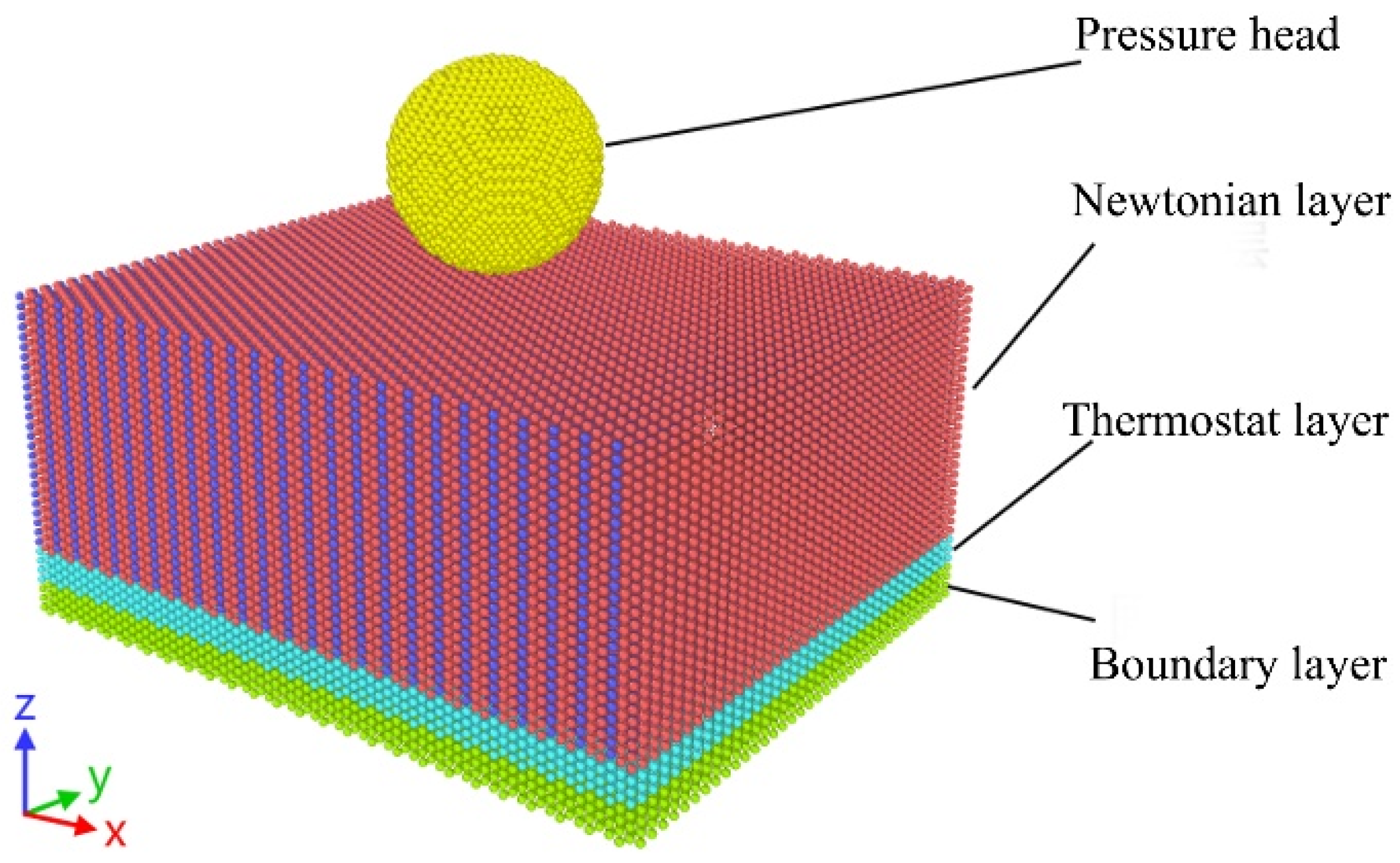
2. Numerical Model Setup and Boundary Conditions
3. Simulation Result and Analysis
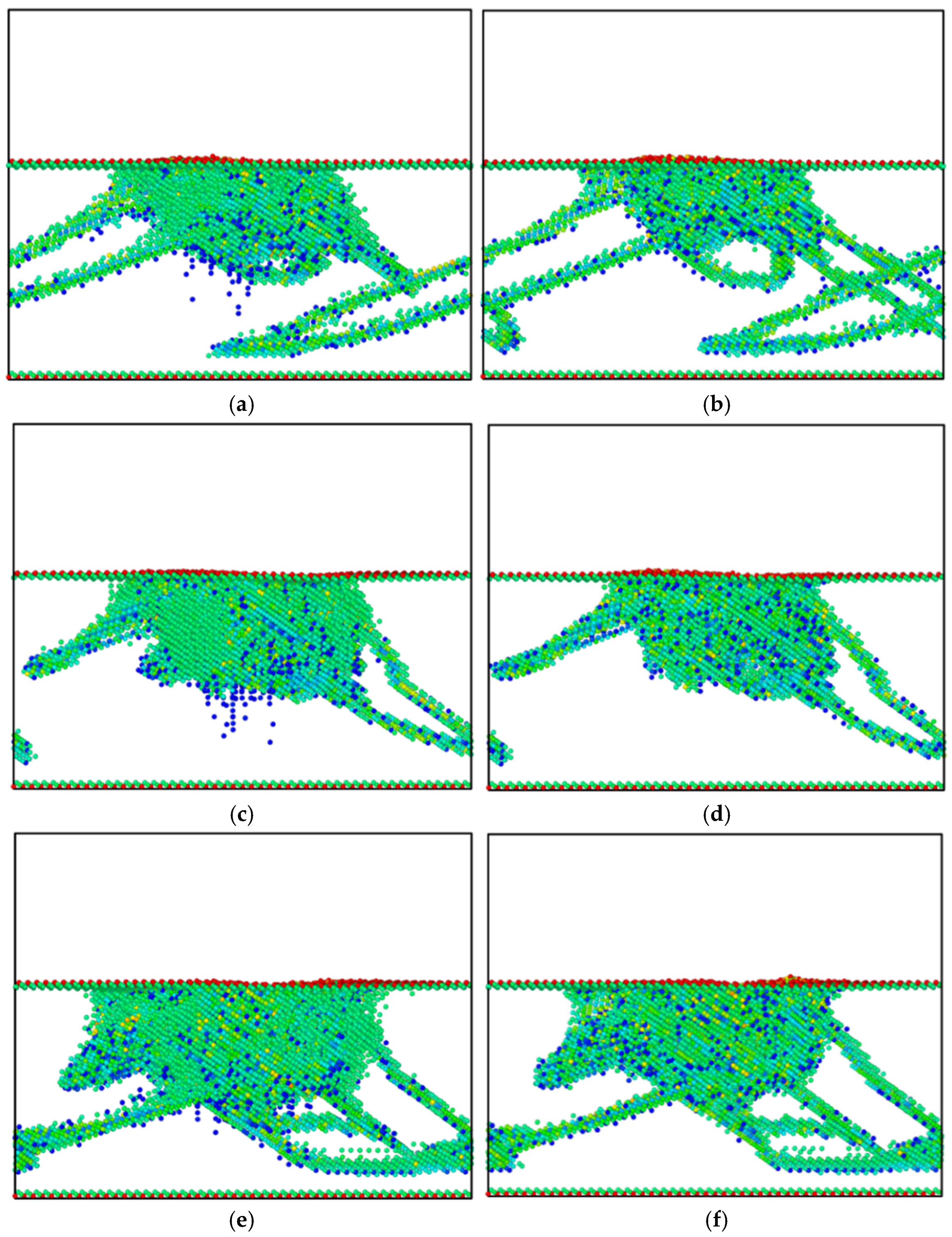
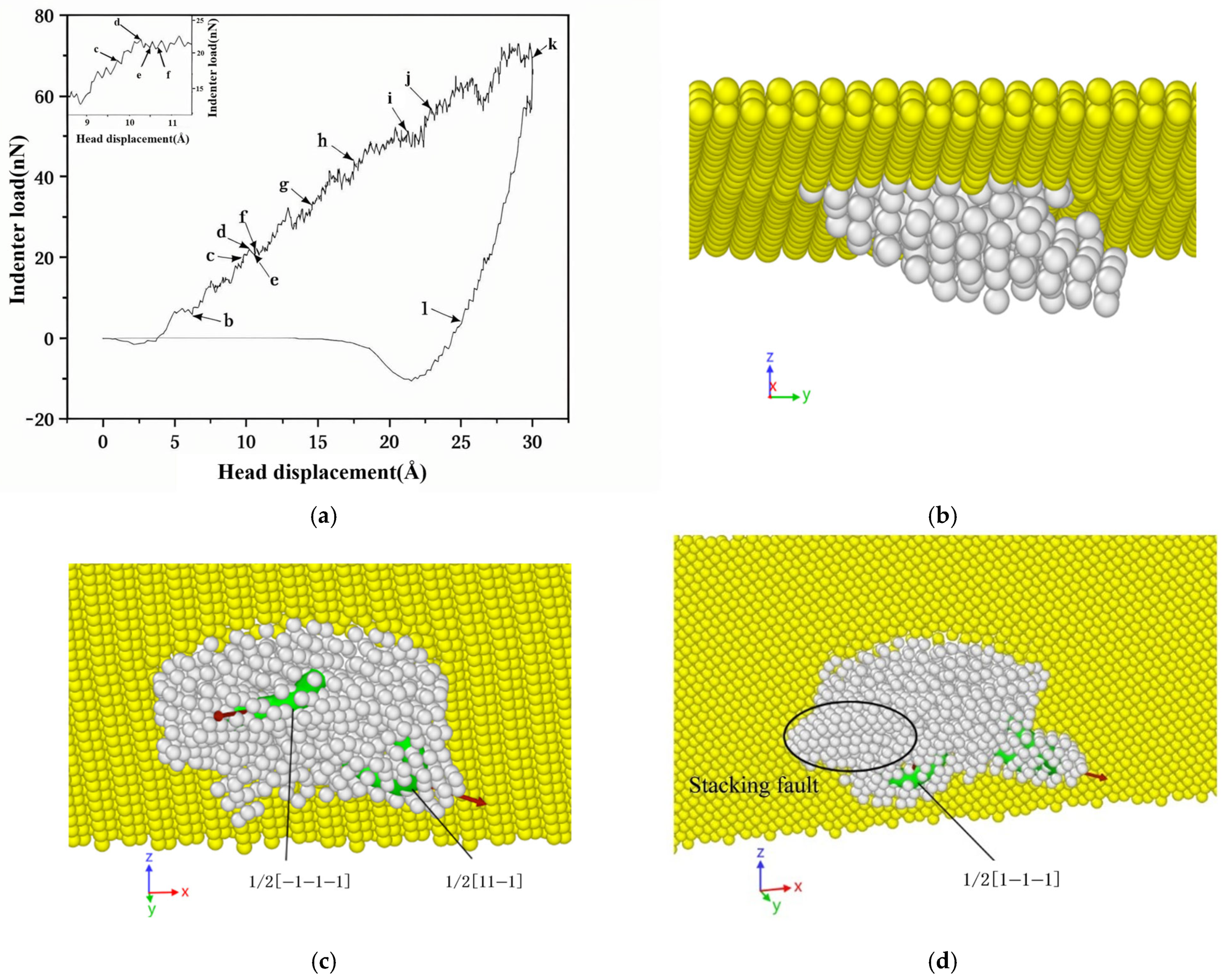
3.1. Surface Accumulation Analysis
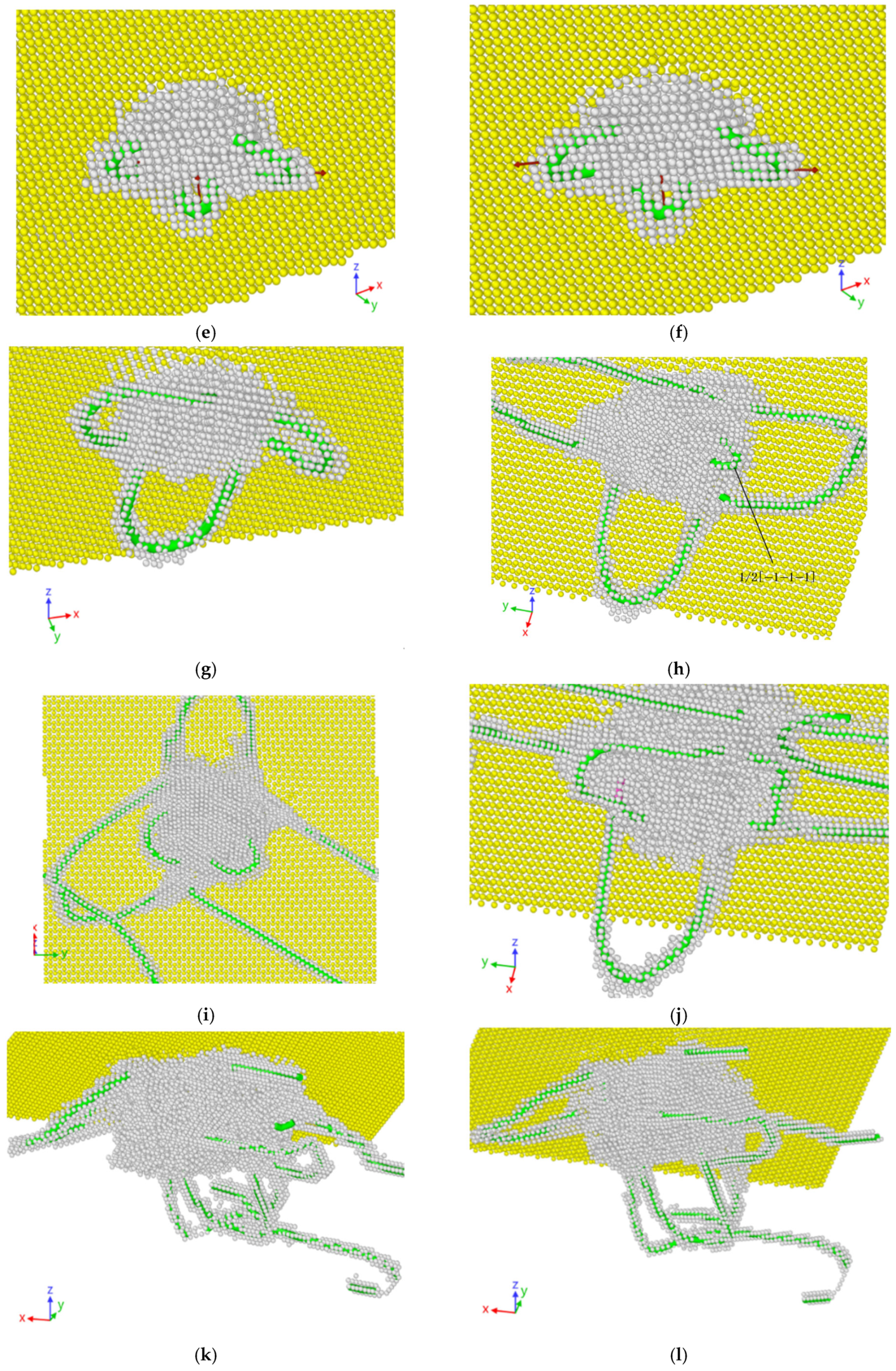
3.2. Atomic Displacement Vector Analysis
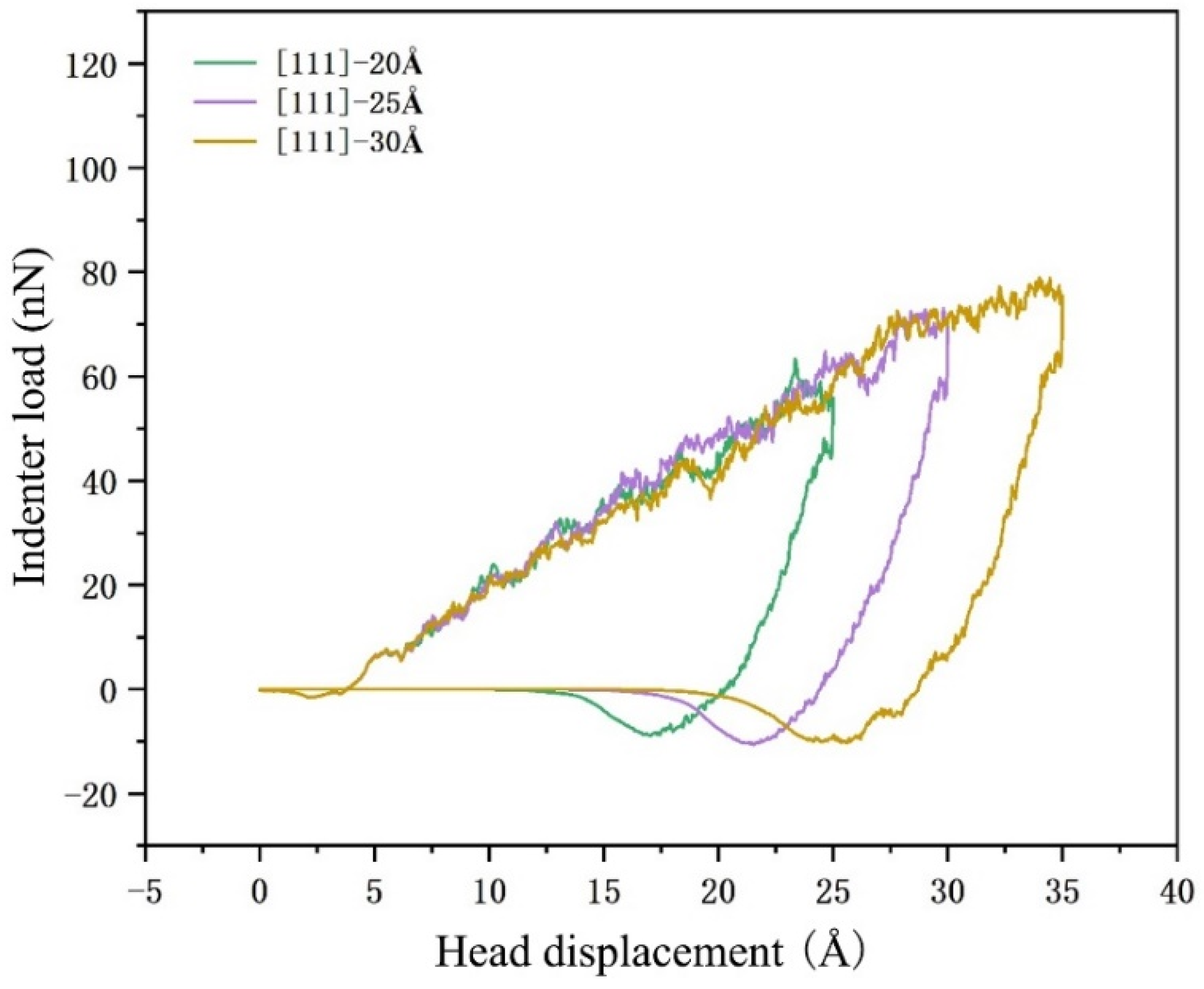
3.3. Load–Displacement Curve Analysis of Single Crystal Mg-Li Model
3.3.1. Load–Displacement Curve Analysis
3.3.2. CSP Defect Analysis
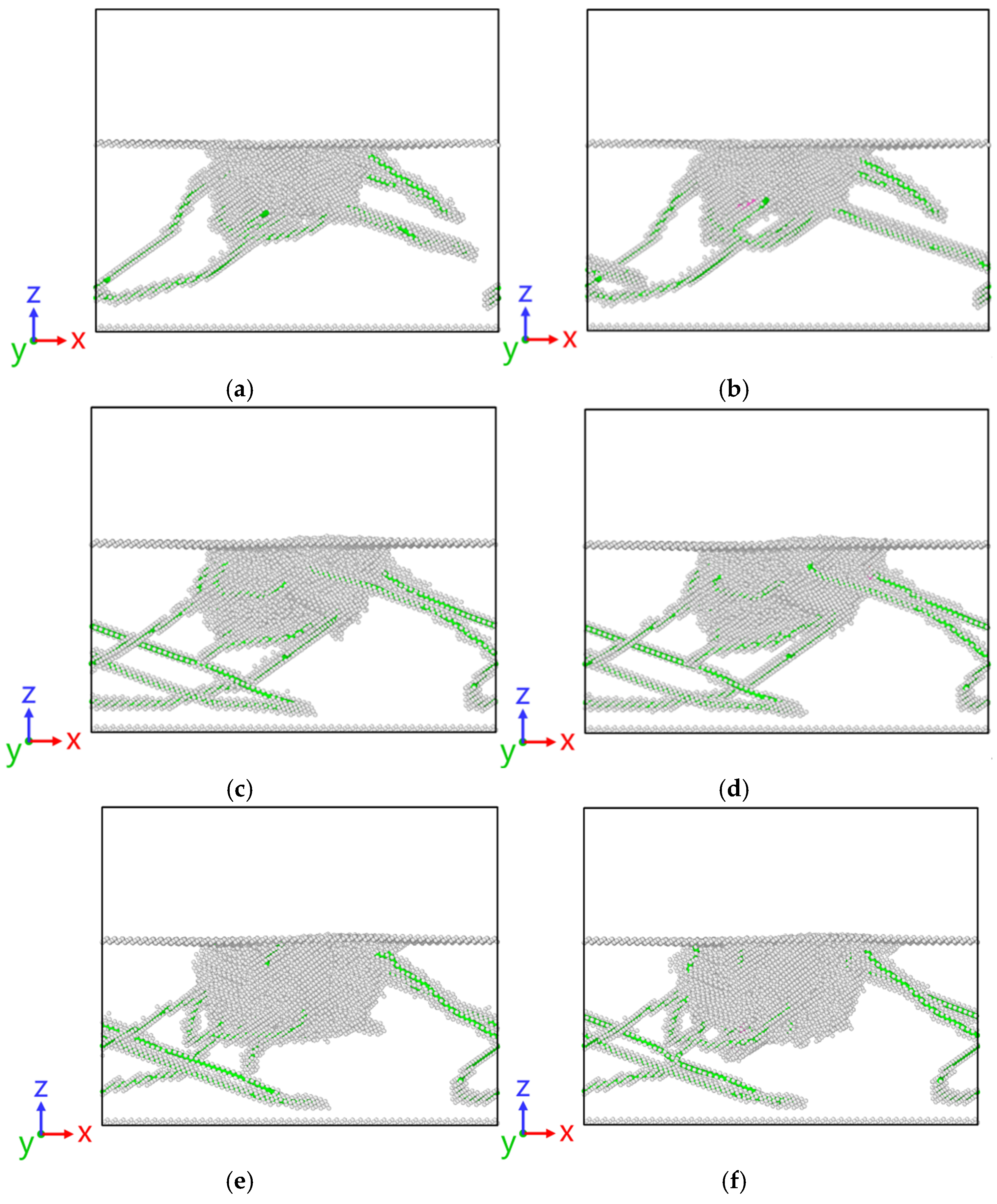
3.3.3. DXA Defect Analysis
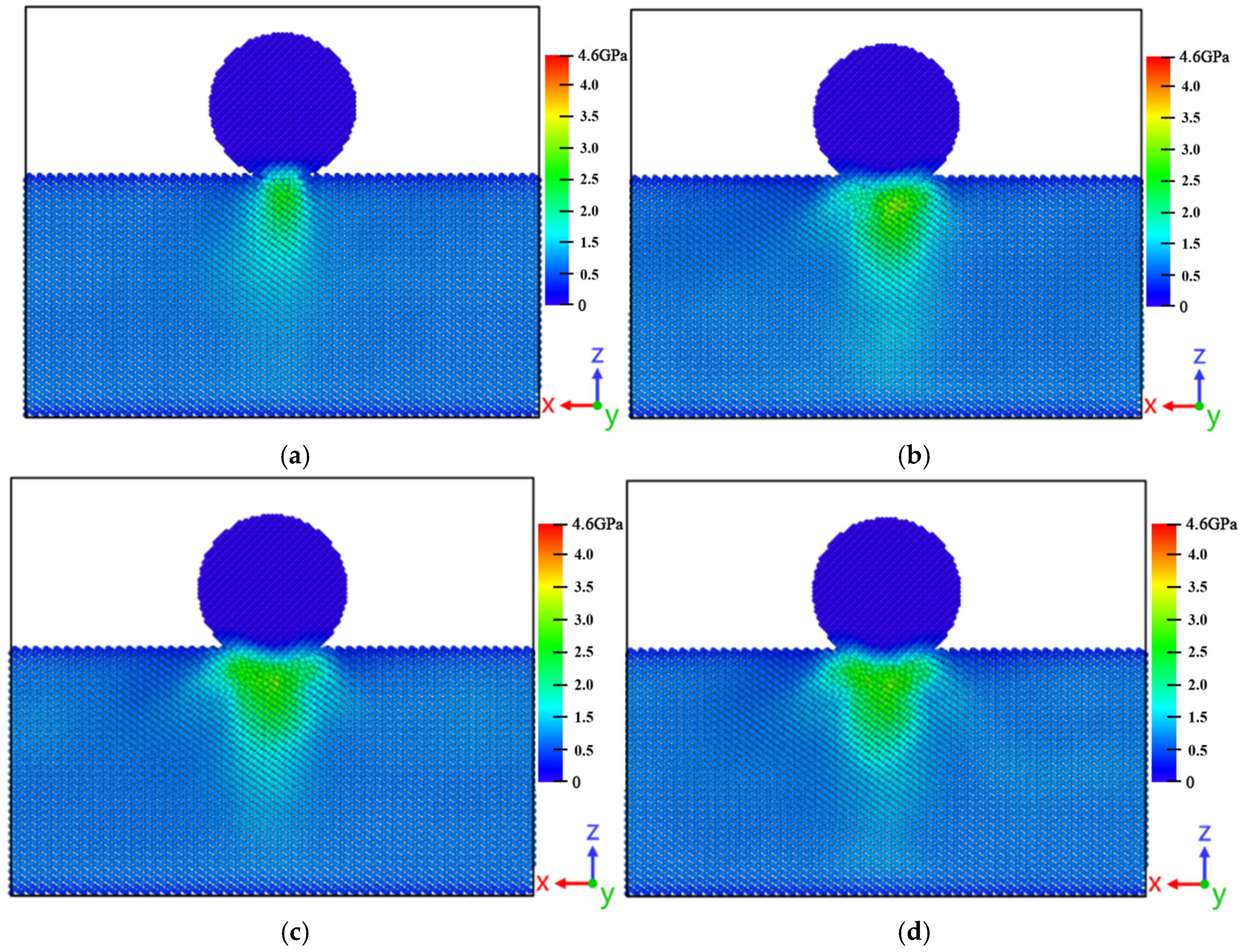
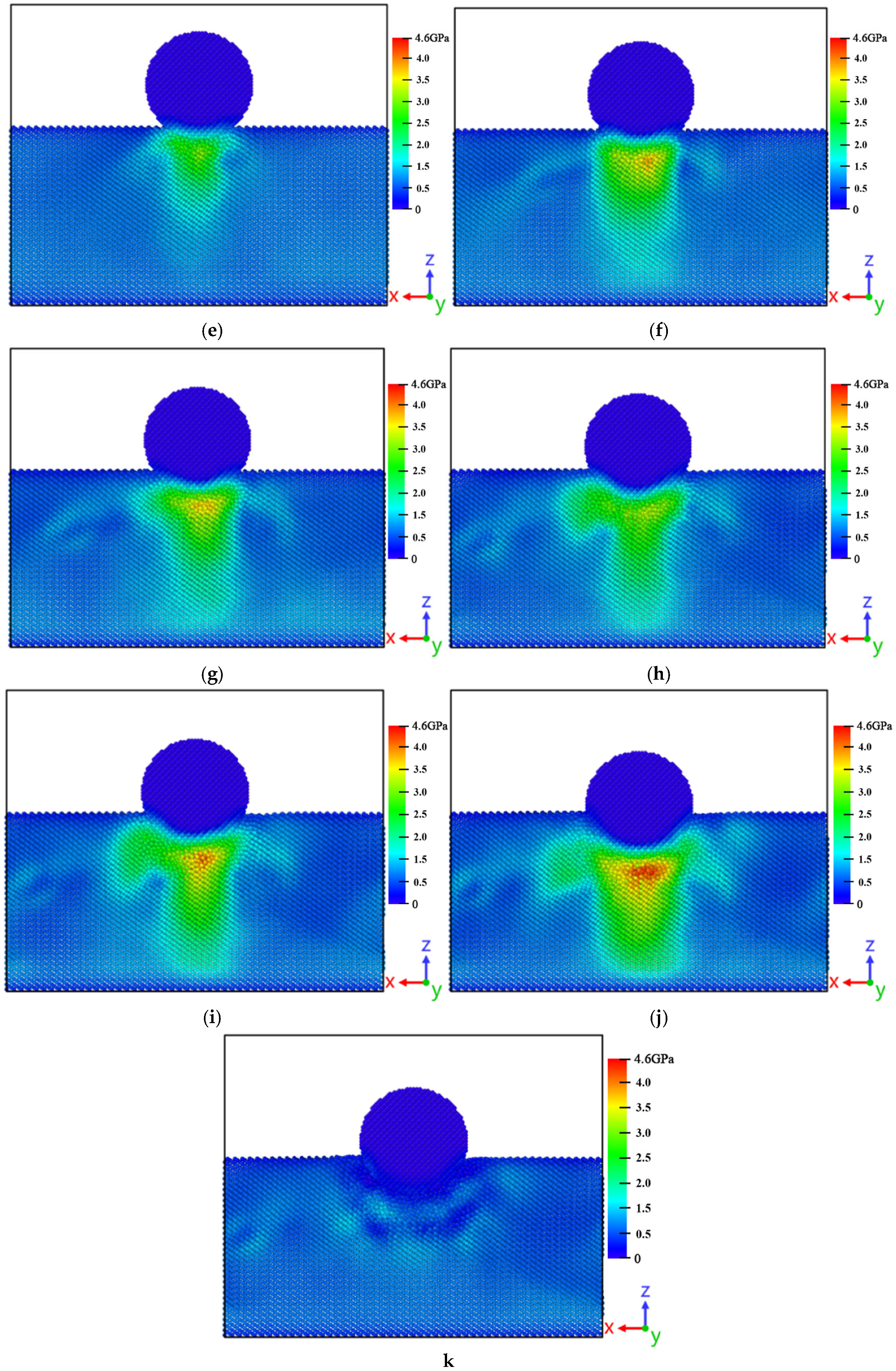
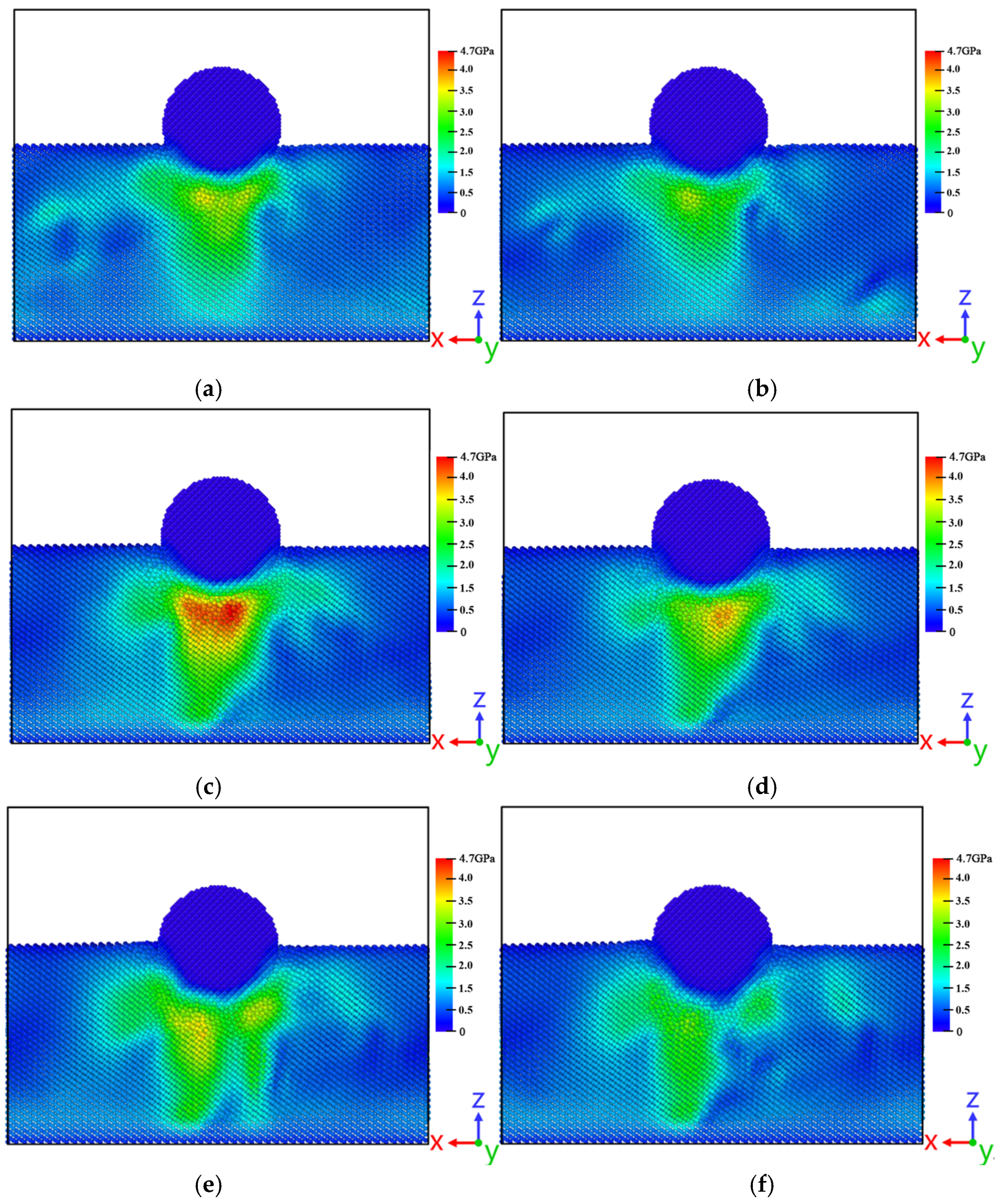
3.3.4. Stress Analysis
3.4. Stress-Relaxation-Simulation Analysis of Single Crystal Mg-Li Model
3.4.1. Load–Displacement Curve Analysis
3.4.2. DXA Defect Analysis
3.4.3. Stress Analysis
4. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Aval, H.J. Impressive effect of li microalloying and severe plastic deformation of Al-Cu-Mg Alloy on strength and corrosion resistance. Arab. J. Sci. Eng. 2023, 48, 12485–12497. [Google Scholar] [CrossRef]
- Bednarczyk, I. Characteristics of the microstructure and properties of mg-li magnesium alloy after deformation by the kobo method. Arch. Metall. Mater. 2022, 67, 1179–1184. [Google Scholar] [CrossRef]
- Bhat, K.; Schlotterose, L.; Hanke, L.; Helmholz, H.; Quandt, E.; Hattermann, K.; Willumeit-Römer, R. Magnesium-lithium thin films for neurological applications—An in vitro investigation of glial cytocoMPatibility and neuroinflammatory response. Acta Biomater. 2024, 265, 113754. [Google Scholar] [CrossRef]
- Eunji, S.; Taheri, A.M.; Amit, M. Investigation of grain size and geometrically necessary dislocation density dependence of flow stress in Mg-4Al by using nanoindentation. Acta Mater. 2024, 265, 119633. [Google Scholar]
- Custodio, A.G.; Lindquist, K.J.; Tolentino, M.; Aranas, C.; Saha, G.C. Investigating the nanoscale hardness/strength properties of high-entropy alloy particles using the nanoindentation technique. J. Alloys Metall. Syst. 2023, 4, 100043. [Google Scholar] [CrossRef]
- Wu, J.H.; Choi, J.; Uba, S.A.; Soper, S.A.; Park, S. Engineering inlet structures to enhance DNA capture into nanochannels in a polymer nanofluidic device produced via nanoimprint lithography. Micro Nano Eng. 2023, 21, 113256. [Google Scholar] [CrossRef]
- Wang, J.F.; Dziadkowiec, J.; Liu, Y.K.; Jiang, W.M.; Zheng, Y.J.; Xiong, Y.Q.; Peng, P.A.; Renard, F. Combining atomic force microscopy and nanoindentation helps characterizing in-situ mechanical properties of organic matter in shale. Int. J. Coal Geol. 2024, 281, 104406. [Google Scholar] [CrossRef]
- Li, Q.; Wang, J.S.; Kang, Y.L.; Li, Y.L.; Qin, Q.H.; Wang, Z.L.; Zhong, X.H. Multi-scale experimental studies on mechanical properties of three-dimensional porous graphene cementitious composite. Cem. Concr. Compos. 2024, 147, 105412. [Google Scholar]
- Cao, F.R.; Zhou, B.J.; Ding, X.; Zhang, J.; Xu, G.M. Mechanical properties and microstructural evolution in a superlight Mg-7.28Li-2.19Al-0.091Y alloy fabricated by rolling. J. Alloys Compd. 2018, 745, 436–445. [Google Scholar]
- Zheng, H.P.; Fei, P.F.; Wu, R.Z.; Hou, L.G.; Zhang, M.L. Microstructure and hardness of Mg–9Li–6Al– x La (x = 0, 2, 5) alloys during solid solution treatment. Mater. Sci. Eng. A 2015, 625, 169–176. [Google Scholar]
- Li, Z.J.; Kuang, Q.; Dong, X.L.; Yuan, T.W.; Ren, Q.H.; Wang, X.X.; Wang, J.; Jing, X.Y. Characteristics of high-performance anti-corrosion/anti-wear ceramic coatings on magnesium-lithium alloy by plasma electrolytic oxidation surface engineering. Surf. Coat. Technol. 2019, 375, 600–607. [Google Scholar] [CrossRef]
- Jiang, L.Y.; Jiang, W.; Guo, F.; Huang, W.J.; Dong, H.P.; Hu, H.J.; Dai, Q.W. Micro-nano structure characteristics and texture evolution of the friction stir processed dual-phase Mg-Li alloy. Mater. Charact. 2021, 173, 110979. [Google Scholar] [CrossRef]
- Mahata, A.; Sikdar, K. Molecular dynamics simulation of nanometer scale mechanical properties of hexagonal Mg-Li alloy. J. Magnes. Alloys 2016, 4, 36–43. [Google Scholar] [CrossRef]
- Liu, P.; Zhao, Y.; Baisheng, N.; Liu, X.; Deng, B.; He, H.; Cao, M. Probing nano-mechanical behavior of coal using nanoindentation tests. J. China Coal Society 2023, 936, 1–17. [Google Scholar]
- Li, L.; Wei, H.; Song, D.; Huang, S.Y.; Xie, L.Z.; Chen, Z.W. Mesoscale elastic-plastic constitutive relationship of Fe-32Ni super-invar alloy based on nanoindentation inverse analysis. J. Plast. Eng. 2024, 31, 129–136. [Google Scholar]
- Luo, J.; Li, N.; Wang, X.; Liu, C.K. Research Progress of Nanoindentation Methods for Measuring Residual Stress in Critical Materials of Aero-engine. Mater. Rev. 2023, 21, 1–33. [Google Scholar]
- Zhang, Q.S.; Liu, Z.B.; Tang, Y.S.; Deng, Y.F.; Luo, T.Y.; Wang, Y.T. Mechanical property characterization of mudstone based on nanoindentation technique combined with upscaling method. Environ. Earth Sci. 2023, 82, 485. [Google Scholar] [CrossRef]
- Huang, T.J.; Niverty, S.; Sundar, A.; Chawla, N. Microstructural characterization and mechanical behavior of Aba Panu meteorite by correlative microscopy and nanoindentation. Mater. Charact. 2023, 205, 132616. [Google Scholar] [CrossRef]
- Teixeira, E.D.; Rossignolo, J.A.; Ferreira, T.I.B.; Medeiros, C.M.D.; Barbosa, N.P. Study of the transition zone of concretes prepared with metakaolin using sem/eds-associated nanoindentation technique. Constr. Build. Mater. 2024, 412, 134717. [Google Scholar] [CrossRef]
- Wang, Y.Q.; Zhou, Z.Y.; Zhou, W.Z.; Xu, L.Q.; Guo, J.; Lan, Z.Q. Effects of in-situ formed Mg 2 Si phase on the hydrogen storage properties of MgLi solid solution alloys. Mater. Des. 2016, 111, 248–252. [Google Scholar] [CrossRef]
- Wu, T.; Blawert, C.; Lu, X.P.; Serdechnova, M.; Zheludkevich, M.L. Difference in formation of plasma electrolytic oxidation coatings on MgLi alloy in coMParison with pure Mg. J. Magnes. Alloys 2021, 9, 1725–1740. [Google Scholar] [CrossRef]
- Song, W.J.; Liu, J.; He, S.; Shen, J.H.; Yang, G.; Liu, Y.H.; Chen, Y.X.; Wei, Q.M. Microstructure and mechanical properties of as-cast ultralight and high strength Mg-10Li-3Al-3Zn-xY alloy with multi-precipitates. Mater. Charact. 2022, 189, 111972. [Google Scholar] [CrossRef]
- Man, J.L.; Wu, B.L.; Duan, G.S.; Zhang, L.; Wan, G.; Zhang, L.; Zou, N.F.; Liu, Y.D. The synergistic addition of Al, Ti, Mo and W to strengthen the equimolar CoCrFeNi high-entropy alloy via thermal-mechanical processing. J. Alloys Compd. 2022, 902, 163774. [Google Scholar] [CrossRef]
- Liu, H.R.; Jiang, B.L.; Li, H.T. Mechanical properties of TiN films prepared in glow-arc transition region. Surf. Eng. 2021, 37, 154–159. [Google Scholar] [CrossRef]
- News of Science. Nanoindentation of Zrh2 by Molecular Dynamics Simulation; Reports from University of Florida Advance Knowledge in Nanoindentation: Gainesville, FL, USA, 2020; p. 1654. [Google Scholar]
- Le, N.C.; Phong, T.D.; Tien, T.V.N. Optimal Design of a Dragonfly-Inspired Compliant Joint for Camera Positioning System of Nanoindentation Tester Based on a Hybrid Integration of Jaya-ANFIS. Math. Probl. Eng. 2018, 2018, 8546095. [Google Scholar]
- Lei, M.; Dang, F.N.; Xue, H.B.; Zhang, Y.; He, M.M. Study on Mechanical Properties of Granite Minerals Based on Nanoindentation Test Technology. Therm. Sci. 2021, 25, 4457–4463. [Google Scholar] [CrossRef]
- Li, Y.C.; Kang, Z.W.; Zhang, X.K.; Pan, J.S.; Ren, Y.; Zhou, G.S. Fabricating an anti-corrosion carbonate coating on Mg-Li alloy by low-temperature plasma. Surf. Coat. Technol. 2022, 439, 2111. [Google Scholar] [CrossRef]
- Rezaei, M.; Aval, H.J. Effect of Cu/Mg Ratio on Microstructure and Corrosion Resistance of Al-Cu-Mg-Li cast Alloy During Non-Isothermal Aging. Met. Mater. Int. 2023, 29, 1907–1922. [Google Scholar] [CrossRef]
- Moudir, D.; Souag, R.; Kamel, N.; Aouchiche, F.; Mouheb, Y.; Kamariz, S. Microwave chemical durability of an iron-rich glass-ceramic dedicated for high-level radioactive waste. Mater. Res. Express 2023, 10, 065503. [Google Scholar] [CrossRef]
- Jeong, H.T.; Kim, W.J. Critical review of superplastic magnesium alloys with emphasis on tensile elongation behavior and deformation mechanisms. J. Magnes. Alloys 2022, 10, 1133–1153. [Google Scholar] [CrossRef]
- Hanke, L.; Jessen, L.K.; Weisheit, F.; Bhat, K.; Westernströer, U.; Garbe-Schönberg, D.; Willumeit-Römer, R.; Quandt, E. Structural characterisation and degradation of Mg-Li thin films for biodegradable implants. Sci. Rep. 2023, 13, 52–56. [Google Scholar] [CrossRef] [PubMed]
- MacLeod, K.; Nash, D.H.; Bow, D.R.; Ma, L. Influence of Heat Treatments on the Microstructure and Mechanical Properties of Two Fine Mg-Li-Y Alloy Wires for Bioresorbable Applications. Adv. Eng. Mater. 2023, 25, 200143. [Google Scholar] [CrossRef]





| Simulation Parameters | Simulation Parameters |
|---|---|
| Workpiece material | Crystal Mg-Li |
| Indenter material | Diamond |
| Workpiece size | 213.35 Å × 177.37 Å × 99.57 Å |
| Indenter size | Radius 30 Å |
| Time step | 1 fs |
| Penetration speed | 50 m/s |
| Indentation depth | 20 Å, 25 Å, 30 Å |
| Holding time | 10 ps |
| Unloading speed | 50 m/s |
| Pressing into the crystal orientation | [111] |
| Potential function | MEAM, Lennard-Jones |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Xu, C.; Liu, Y.; Li, J.; Yan, F.; Li, A.; Li, D.; Zheng, Z. Study on Mechanical Properties and Structural Deformation of [111] Oriented Mg-Li Alloy. Coatings 2025, 15, 540. https://doi.org/10.3390/coatings15050540
Xu C, Liu Y, Li J, Yan F, Li A, Li D, Zheng Z. Study on Mechanical Properties and Structural Deformation of [111] Oriented Mg-Li Alloy. Coatings. 2025; 15(5):540. https://doi.org/10.3390/coatings15050540
Chicago/Turabian StyleXu, Chengyu, Yankai Liu, Junye Li, Feng Yan, Aozhi Li, Danni Li, and Zixian Zheng. 2025. "Study on Mechanical Properties and Structural Deformation of [111] Oriented Mg-Li Alloy" Coatings 15, no. 5: 540. https://doi.org/10.3390/coatings15050540
APA StyleXu, C., Liu, Y., Li, J., Yan, F., Li, A., Li, D., & Zheng, Z. (2025). Study on Mechanical Properties and Structural Deformation of [111] Oriented Mg-Li Alloy. Coatings, 15(5), 540. https://doi.org/10.3390/coatings15050540






