Research on the Formation Mechanism of Hot-Water Heat Damage in High-Temperature Roadway and the Cooling Effect of Spraying a Thermal Insulation Layer
Abstract
1. Introduction
2. Engineering Background
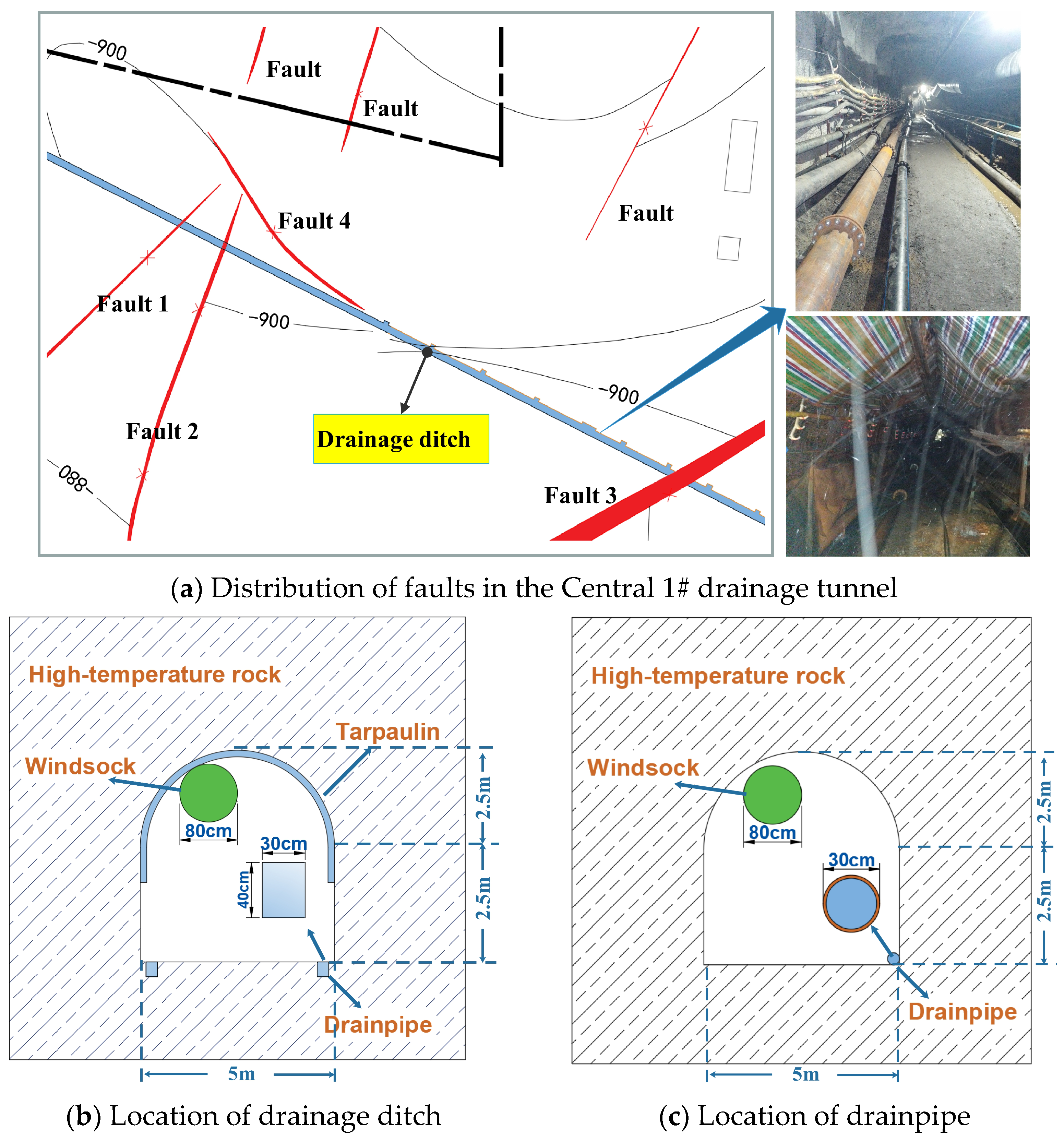
2.1. Status of Works
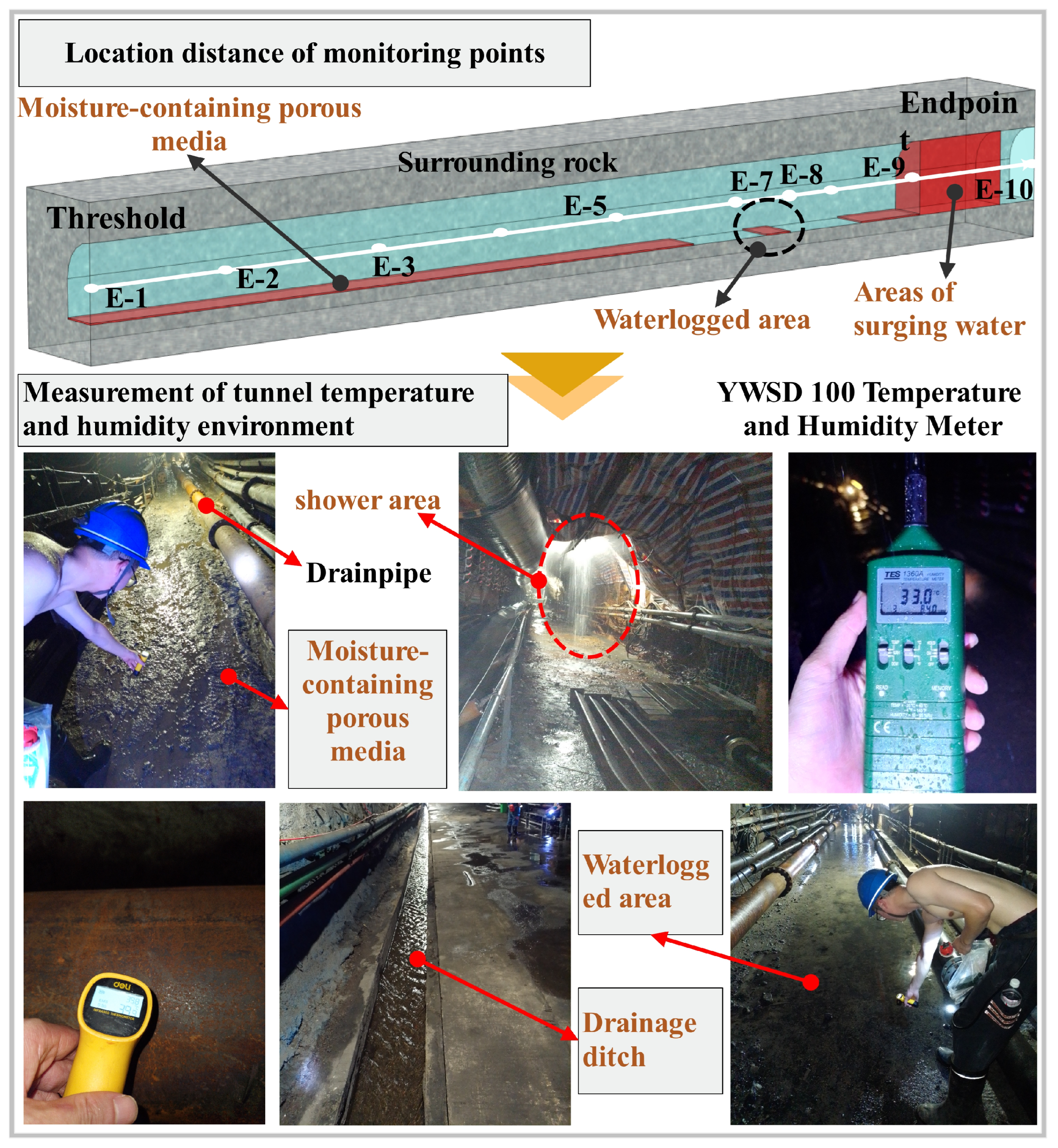
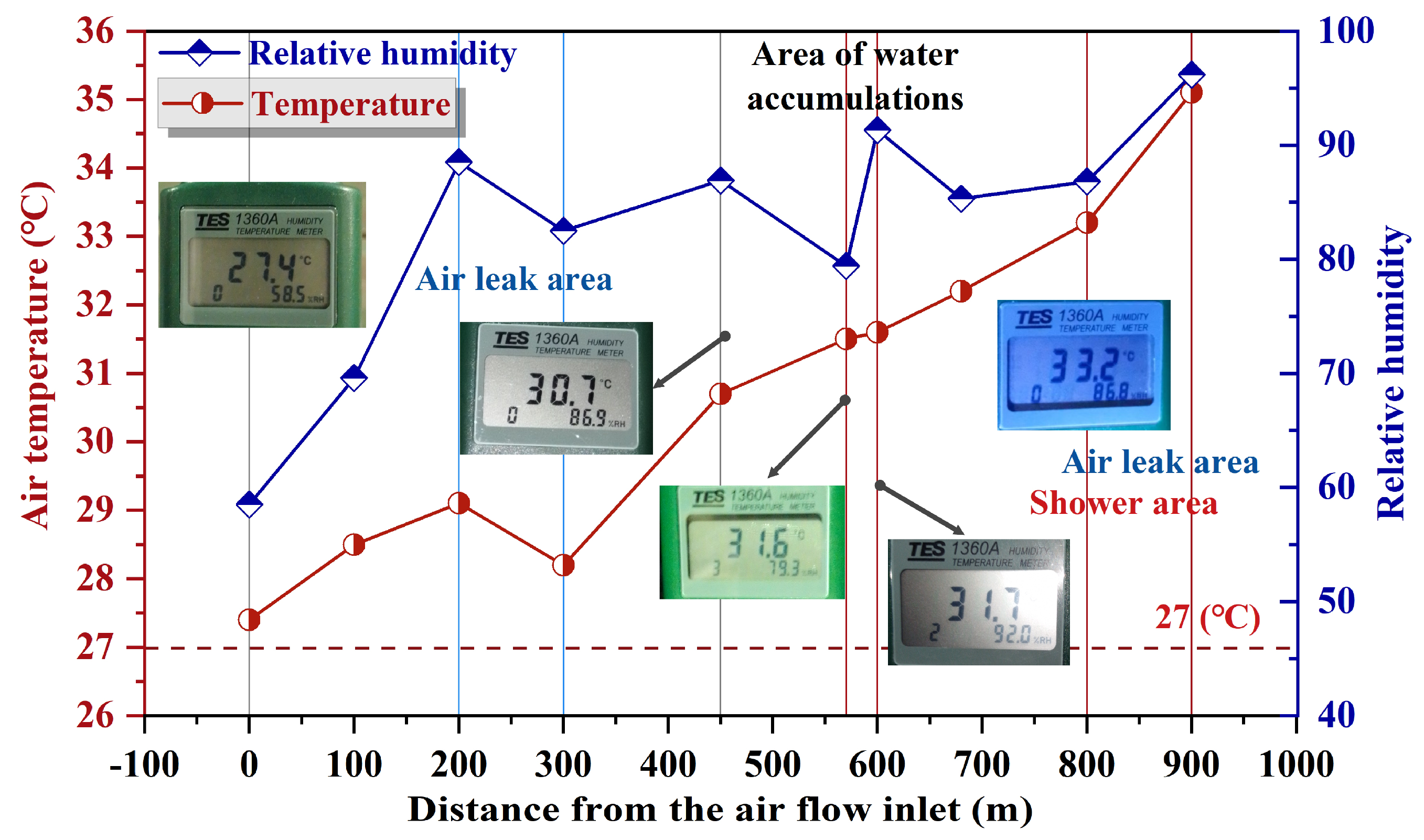
2.2. Analysis of the Measured Results of Ambient Temperature and Humidity in the Tunnel
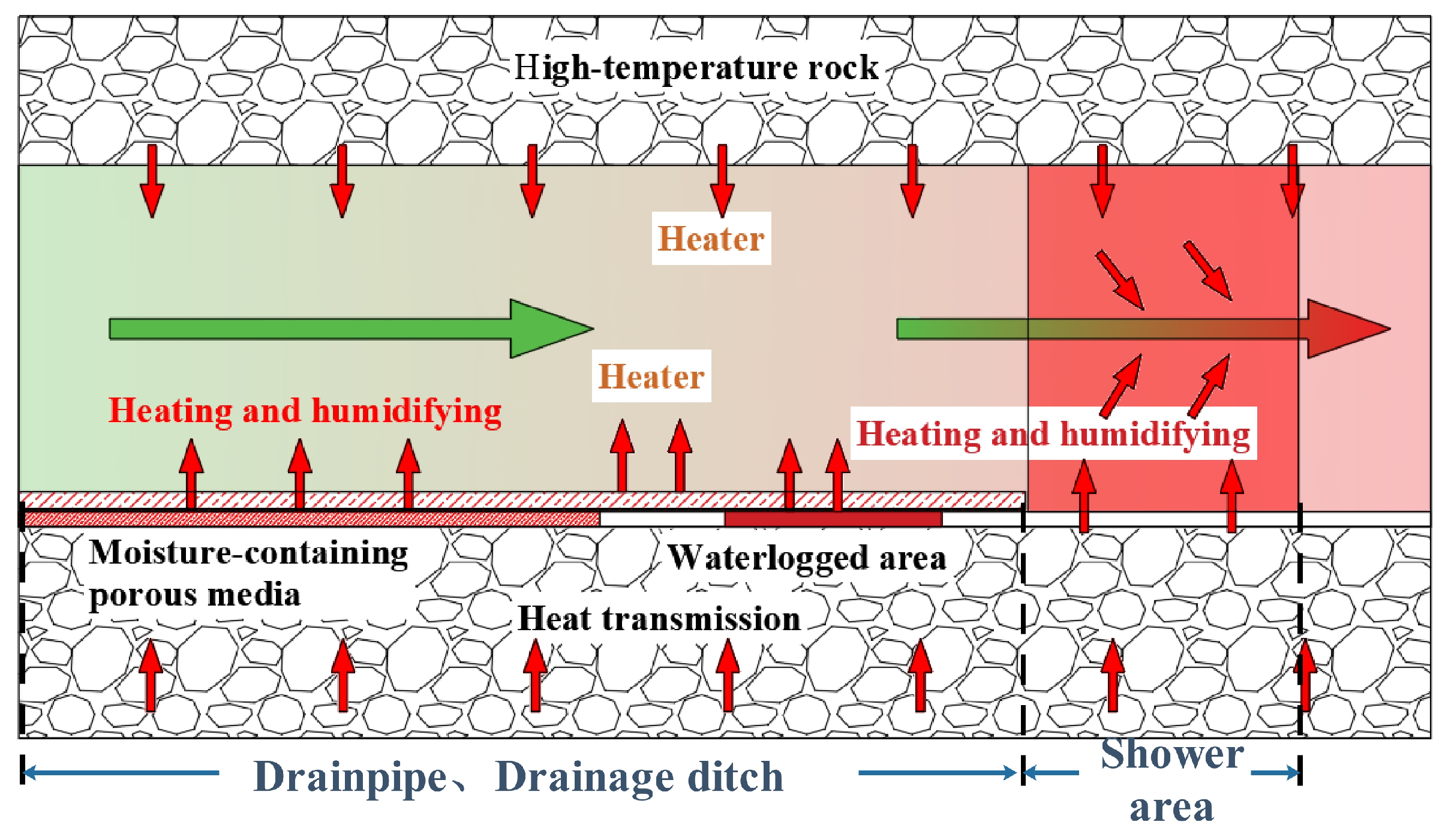
- (1)
- In the region of moisture-containing porous media, heat and moisture exchange occurs between the gas flow and wet porous media, and RH rises continuously. Due to the small amount of fracture water in this area, the heat carried has little effect on the temperature of the moisture-containing porous media, and the temperature of the moisture-containing porous media is mainly affected by the heating of the surrounding rock. The vertical distribution trend of Ta is primarily controlled by the high-temperature surrounding rock and the drainpipe, and the moisture-containing porous medium mainly plays the role of humidifying the airflow.
- (2)
- In the water accumulation area, the continuous evaporation of standing water leads to an increase in the temperature and humidity of the airflow. However, due to the relatively small amount of water being added, the heat carried is also minimal, and the standing water primarily serves to humidify the airflow.
- (3)
- In regions where large quantities of fracture water enter the tunnel, both Ta and RH increase rapidly. The airflow is heated and humidified by the high-temperature shower water and hot water in the drainage ditch. Simultaneously, the hot water transfers heat to the surrounding rock, initiating convection between the hot water and surrounding rock, followed by heat transfer from the surrounding rock to the airflow. This process leads to a significant increase in both the temperature and humidity of the airflow.
3. Numerical Simulations
3.1. Basic Assumptions
- (1)
- Homogeneous medium assumption: the surrounding rock and insulation layer are treated as homogeneous, continuous media with constant physical properties.
- (2)
- Incompressible fluid assumption: since the tunnel environment is at normal temperature and pressure, it is assumed that both air and water are incompressible fluids, and the effects of internal thermal radiation are neglected.
- (3)
- Smooth surface assumption: the inner surfaces of the tunnel, drainage ditch, and drainage pipe are assumed to be smooth.
- (4)
- Impermeable rock mass assumption: The surrounding rock mass is considered impermeable, restricting the movement of hot water to drains and pipes. The water flow is assumed to be uniform and unidirectional.
3.2. Mathematical Modeling
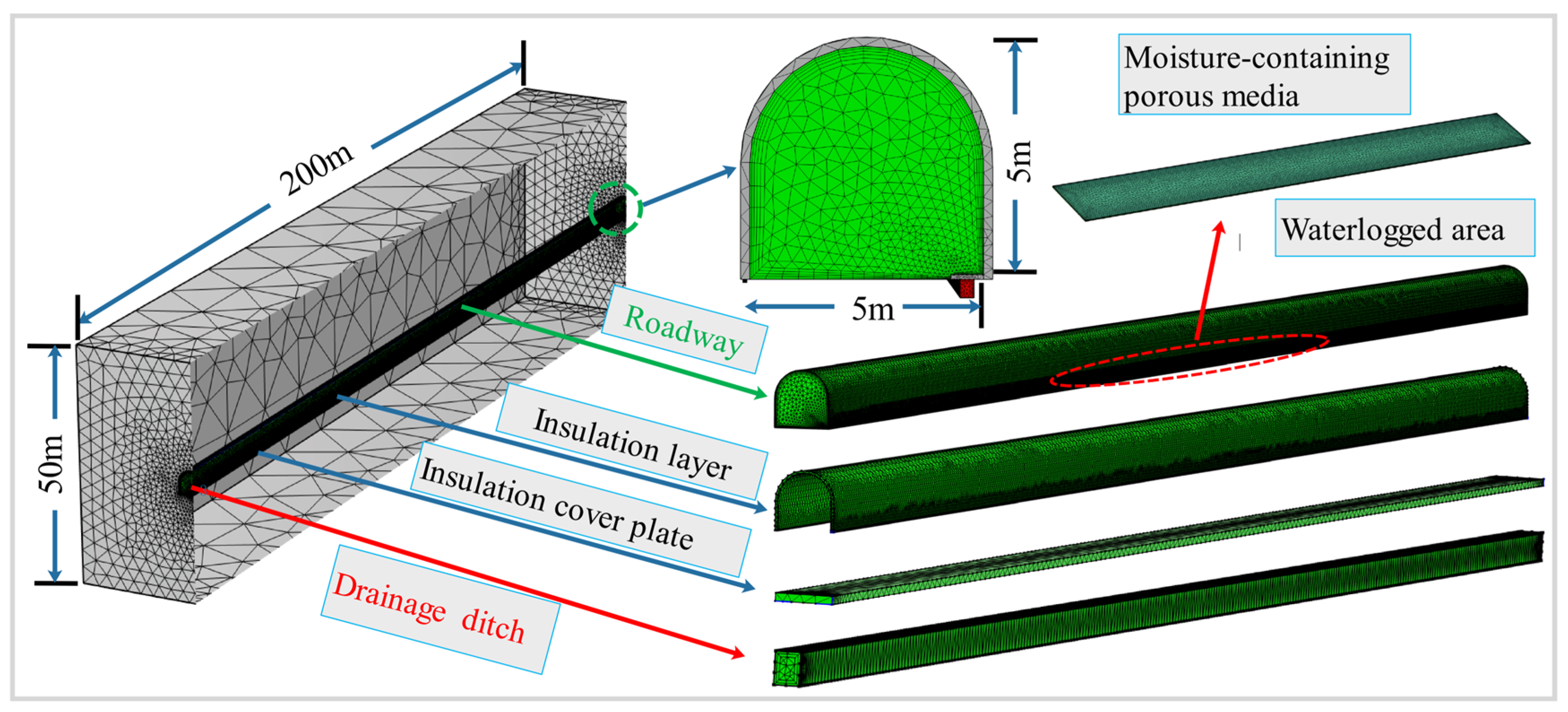
3.3. Model Geometry and Material Parameters
3.4. Boundary Conditions and Solution Settings
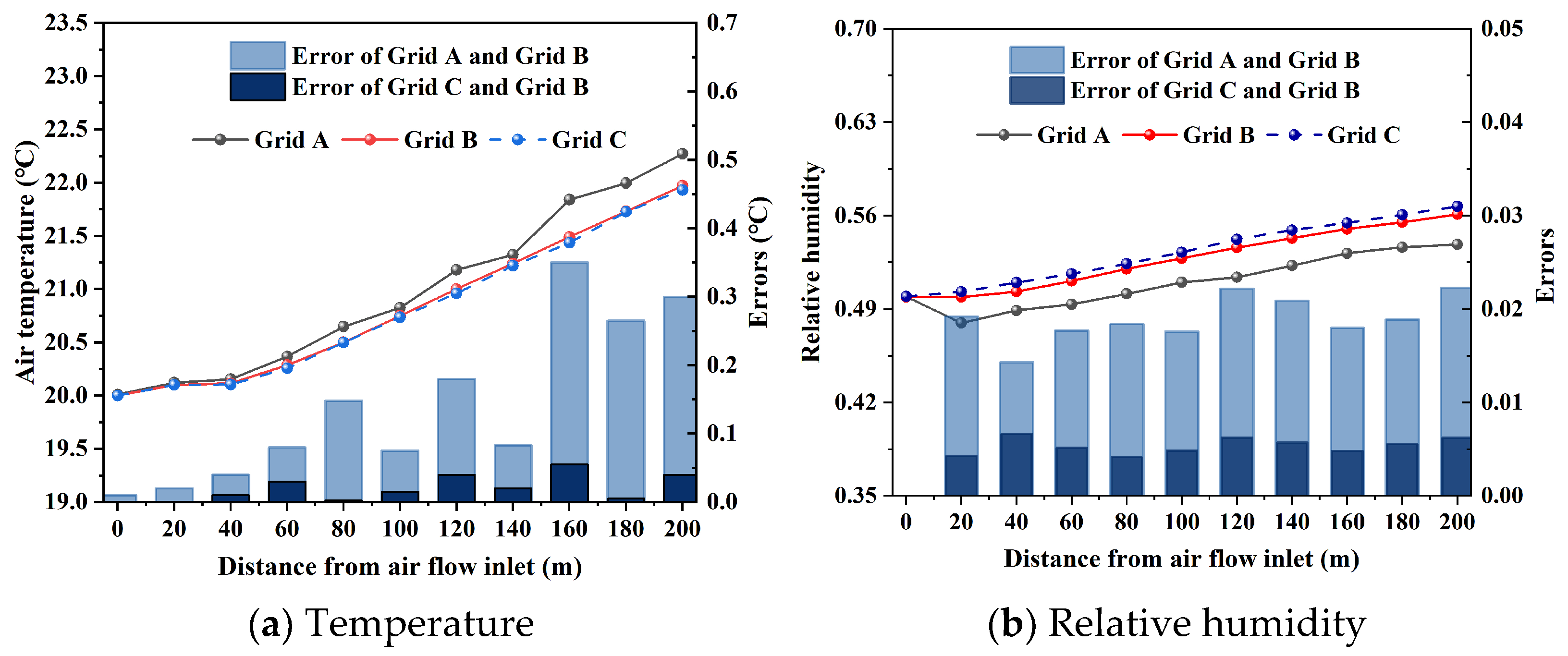
3.5. Grid Independence Test
4. Distribution Characteristics of Ambient Temperature and Humidity in the Tunnel Under Different Factors
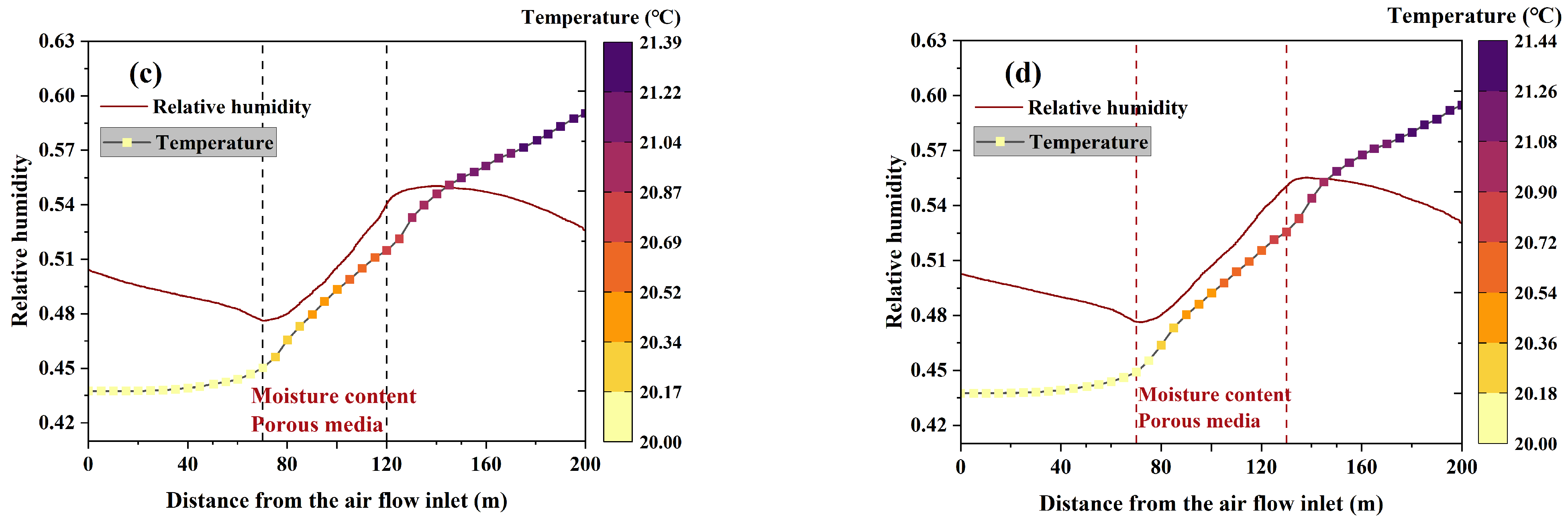
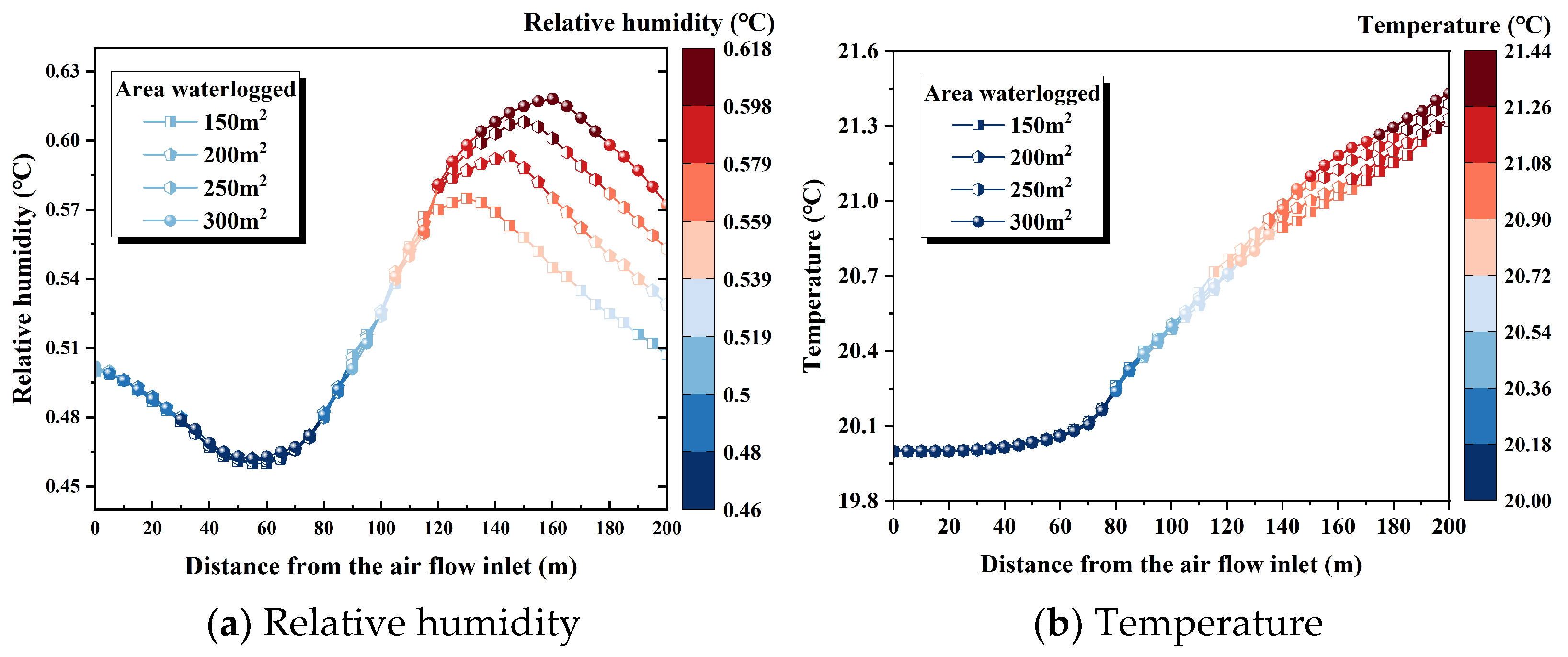
4.1. Area of Moisture-Containing Porous Media
4.2. Area of Water Accumulations
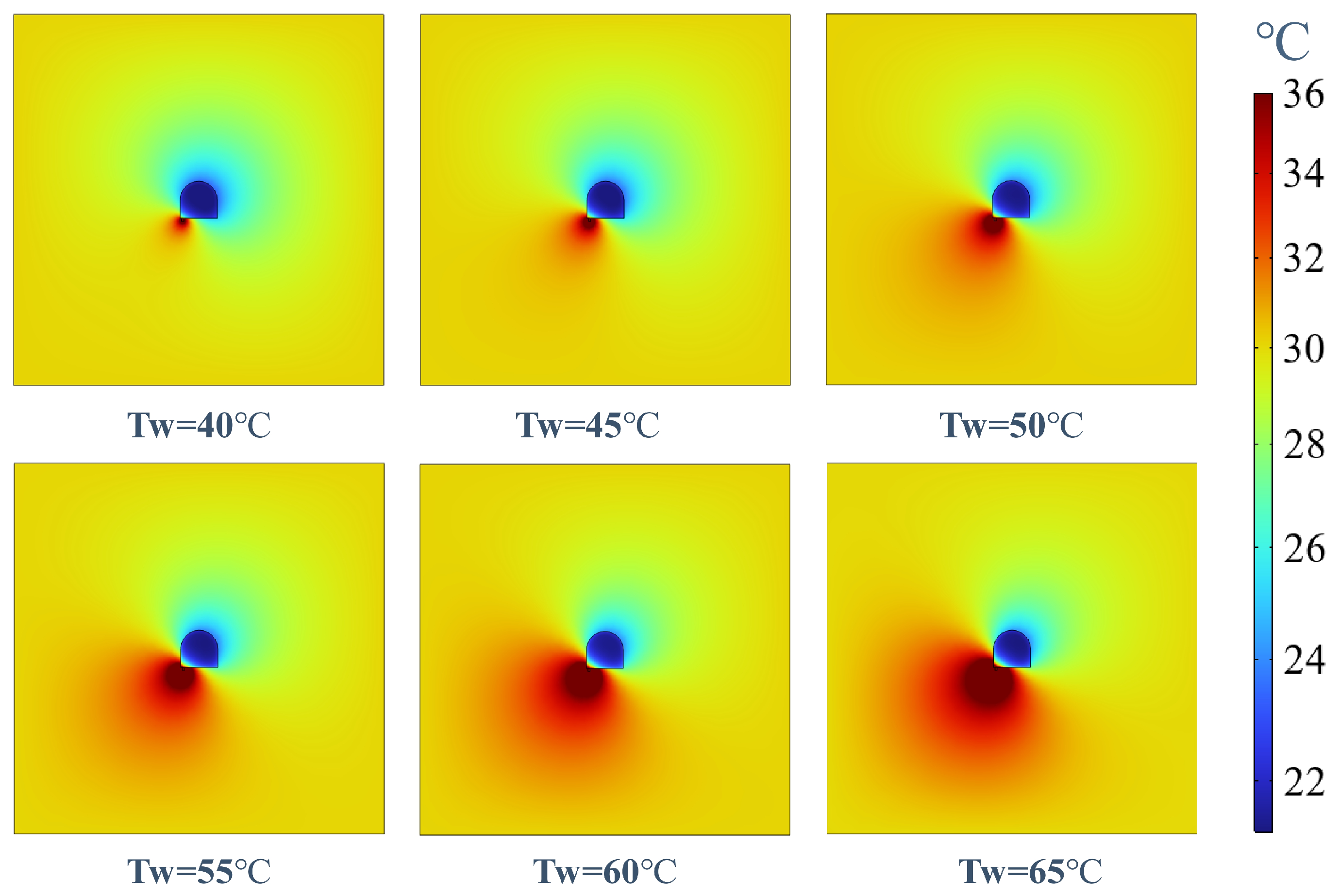
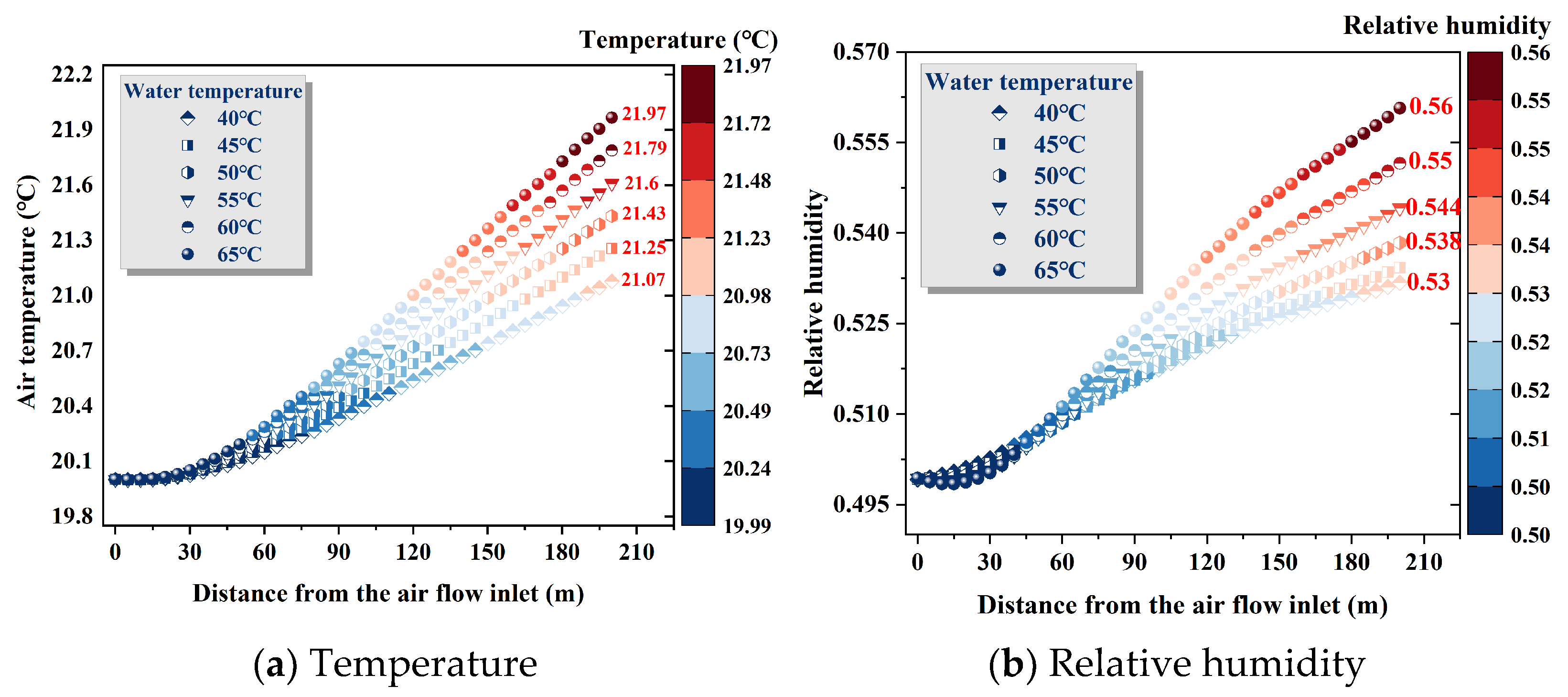
4.3. Water Temperature
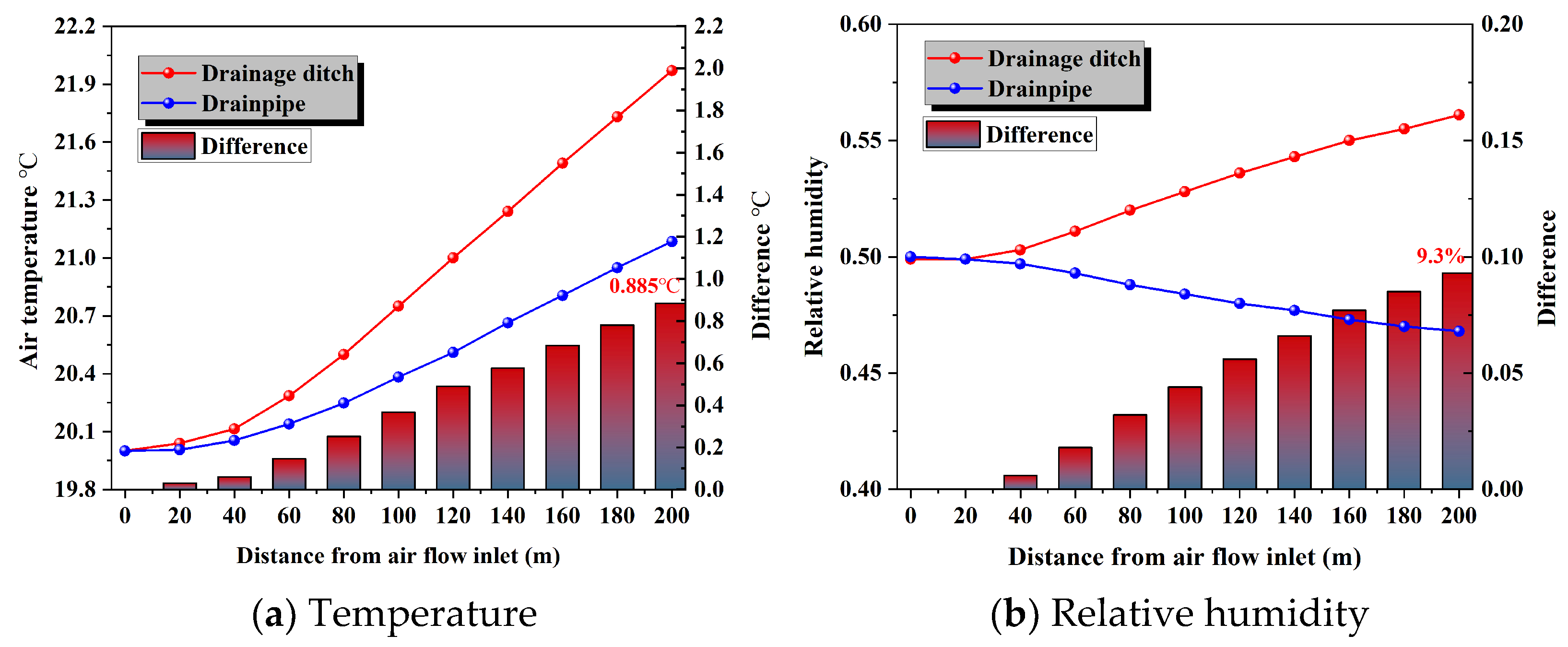
4.4. Comparison of the Effect of Drainage Ditch and Drainpipe on the Hot and Humid Environment
4.5. Optimization of Drainage Methods for Drains
5. Conclusions
- (1)
- Field measurements indicated that fissure water entering the tunnel forms moisture-containing porous media and water accumulation areas. For an area of 150 m2, the moisture-containing porous media increased the RH of the airflow by 5.1%, while the water accumulation area raised the RH by 9.8%. The degree of influence was positively correlated with the area covered. Due to the small amount of water inflow, the longitudinal distribution of Ta is mainly controlled by the high-temperature surrounding rock and drainage facilities.
- (2)
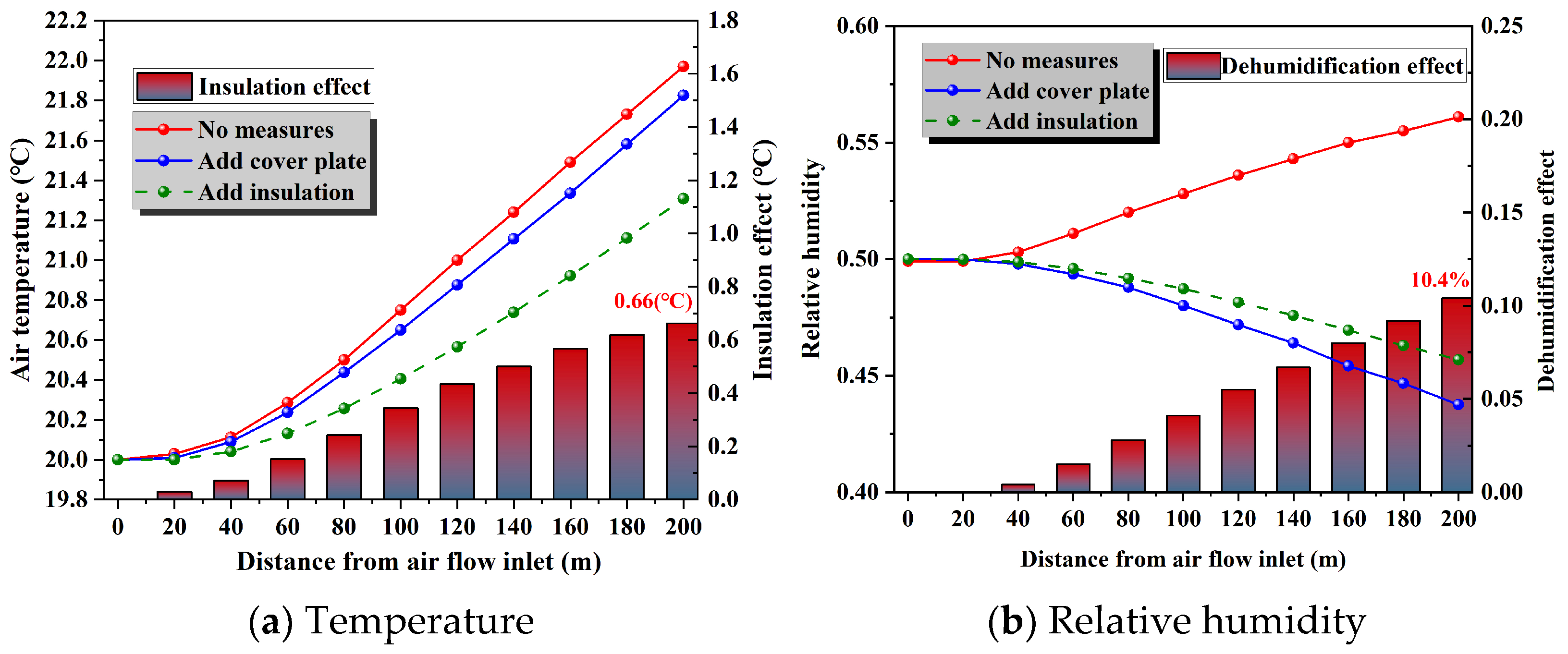
- The hot water in the drainage ditch heats and humidifies the airflow. At the same time, the hot water also transfers heat to the surrounding rock, experiencing convection between the hot water and the surrounding rock→heat conduction in the surrounding rock→convection between the airflow and the surrounding rock, leading to a substantial increase in Ta and RH, with heat transfer through the surrounding rock being the dominant mechanism. Under the same fissure water conditions, the air temperature (Ta) values at the ventilation outlets of the drainage ditch and drainage pipe tunnels are 21.97 °C and 20.08 °C, respectively. In comparison, the use of the drainage pipe results in a decrease in Ta by 0.89 °C (a reduction of 4.2%), with heat transfer reduced by 44.9% and relative humidity (RH) decreased by 9.3%.
- (3)
- When a large amount of fissure water enters the tunnel space, both Ta and RH increase rapidly. The water temperature directly influences the distribution of Ta and RH. Specifically, the Ta value at the ventilation outlet exhibited a linear growth trend with increasing Tw. When the fissure water volume was 90 m3/h, the Ta value at the ventilation outlet rose by 0.15 °C for every 5 °C increase in water temperature, and the RH growth rate accelerated as Tw increased.
- (4)
- The addition of a cover plate above the drainage ditch resulted in a 12.3% decrease in RH, with minimal impact on Ta. Spraying an insulation layer on the inner wall of the tunnel caused a significant reduction in Ta, with a 0.66 °C decrease observed when the insulation layer thickness reached 10 cm.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Han, Q.; Zhang, Y.; Li, K.; Zou, S. Computational evaluation of cooling system under deep hot and humid coal mine in China: A thermal comfort study. Tunn. Undergr. Space Technol. 2019, 90, 394–403. [Google Scholar]
- Chen, X.; Zhang, Y.; Ji, J.; Miao, D. Ventilation and cooling of coal mining face based on CFD model optimization. Process Saf. Environ. Protect. 2023, 172, 764–777. [Google Scholar]
- Chen, L.; Li, J.; Zhang, Y.; Han, F.; Ji, C.; Zhang, J. Study on coupled heat transfer and seepage in large sparsely fractured surrounding rocks in deep underground spaces. Appl. Therm. Eng. 2019, 162, 114277. [Google Scholar]
- Xie, H.; Gao, G.; Ju, Y. Research and exploration of deep rock mechanics. J. Rock Mech. Eng. 2015, 11, 2161–2178. [Google Scholar]
- Xu, C.; Nie, W.; Peng, H.; Li, H.; Wan, J. Enhancing Dust Concentration Monitoring in High Particulate Matter Environments: A Dual-Light Source Particulate Matter Sensor Approach Based on Mie Scattering. Sens. Actuators A Phys. 2025, 387, 116348. [Google Scholar]
- Chen, Z.; Huang, L.; Li, X.; Chen, J.; Liu, Z. Comprehensive heat extraction performance and fractured reservoir cooling effect analysis of a novel mine enhanced geothermal system. Appl. Therm. Eng. 2024, 245, 122790. [Google Scholar]
- Guo, L.; Nie, W.; Liu, Q.; Hua, Y.; Xue, Q.; Sun, N. Study on the coupling pollution law of dust and gas and determination of the optimal purification position of air duct during tunnel excavation. Powder Technol. 2022, 411, 117843. [Google Scholar]
- Wang, W.P.; Wang, S.; Dai, M.M.; Shao, K.; Cui, Z.; Liu, Y.; Cheng, L. Experimental analysis on dynamic performance of refrigeration systems in a deep gold mine. Appl. Therm. Eng. 2022, 217, 119259. [Google Scholar]
- Du, C.; Wei, D.; Zhang, H.; Zhang, L. Research on factors influencing the deep gushing water cooling process in constant temperature zone. Case Stud. Therm. Eng. 2019, 14, 100438. [Google Scholar]
- Wang, H. Numerical study of surrounding rock heat dissipation and wind temperature prediction in high geotemperature coal face. Energy Explor. Exploit. 2024, 42, 17–39. [Google Scholar]
- Xin, S.; Wang, W.; Zhang, N.; Zhang, C.; Yuan, S.; Li, H.; Yang, W. Comparative studies on control of thermal environment in development headings using force/exhaust overlap ventilation systems. J. Build. Eng. 2021, 38, 102227. [Google Scholar]
- Qu, Z.Q.; Zhang, W.; Guo, T.K. Influence of different fracture morphology on heat mining performance of enhanced geothermal systems based on COMSOL. Int. J. Hydrogen Energy 2017, 42, 18263–18278. [Google Scholar]
- Gong, F.; Guo, T.; Sun, W.; Li, Z.; Yang, B.; Chen, Y.; Qu, Z. Evaluation of geothermal energy extraction in Enhanced Geothermal System (EGS) with multiple fracturing horizontal wells (MFHW). Renew. Energy 2020, 151, 1339–1351. [Google Scholar]
- Chen, Y.; Zhao, Z.; Peng, H. Convective heat transfer of water flow in intersected rock fractures for enhanced geothermal extraction. J. Rock Mech. Geotech. Eng. 2022, 14, 108–122. [Google Scholar]
- Zeng, Y.; Tao, L.; Ye, X.; Zhou, X.; Fang, Y.; Fan, L.; Yang, Z. Temperature reduction for extra-long railway tunnel with high geotemperature by longitudinal ventilation. Tunn. Undergr. Space Technol. 2020, 99, 103381. [Google Scholar]
- Li, Z.X.; Wang, T.M.; Zhang, M.; Lin, L. Construction of heat transfer coefficients for drench evaporation and calculation of wind flow temperature in a mine tunnel. J. Coal 2017, 42, 3176–3181. [Google Scholar]
- Wei, Y.X.; Hu, H.H.; He, F.L.; Li, J. Numerical simulation of heat and humidity exchange law between tunnel wall and air. Chin. J. Saf. Sci. 2012, 22, 42–47. [Google Scholar]
- Chen, G.Y. Numerical Simulation of Temperature Field of Surrounding Rock and Wind Flow in Deep Mine Tunnel. Ph.D. Thesis, Hunan University of Science and Technology, Xiangtan, China, 2014. [Google Scholar]
- Li, Z.; Xu, Y.; Li, R.; Jia, M.; Wang, Q.; Chen, Y.; Cai, R.; Han, Z. Impact of the water evaporation on the heat and moisture transfer in a high-temperature underground tunnel. Case Stud. Therm. Eng. 2021, 28, 101551. [Google Scholar]
- Wang, J.C. Experimental Study on the Changing Law of Temperature and Humidity Field in Tunnel Wind Flow Under the Action of High Temperature Fissure Water. Ph.D. Thesis, China University of Mining and Technology, Xuzhou, China, 2019. [Google Scholar]
- Xin, S.; Wang, W.; Zhang, C.; Li, C.; Li, H.; Yang, W. Effects of rock-airflow conjugated heat transfer in development headings: A numerical study. Int. J. Therm. Sci. 2022, 172, 107301. [Google Scholar]
- Xiao, Y.; Zheng, J.; He, Y.; Wang, L. Percolation transitions of spontaneous imbibition in fractional-wet porous media. Colloid Surf. A-Physicochem. Eng. Asp. 2023, 673, 131826. [Google Scholar]
- Paustian, R.; Andersson, L.; Helland, J.O.; Wildenschild, D. On the relationship between capillary pressure, saturation, and interfacial area for three-phase flow in water-wet porous media. Adv. Water Resour. 2021, 151, 103905. [Google Scholar]
- Yu, H.; Zahidi, I.; Liang, D. Sustainable porous-insulation concrete (SPIC) material: Recycling aggregates from mine solid waste, white waste and construction waste. J. Mater. Res. Technol. 2023, 23, 5733–5745. [Google Scholar]
- Zhang, Y.; Yin, S.; Qu, M.; Liu, Y.; Zhu, Z.; Zhou, Y.; Xing, Y. Feasibility of natural bamboo branches aggregate applied to the thermal insulation layer of rock walls in roadways. Constr. Build. Mater. 2024, 435, 136870. [Google Scholar]
- Yang, L.; Li, X.; Pan, M.; Fu, J.; Wang, A.; Kong, Y.; Ma, C. Light-weight high strength porous thermal insulation materials based on dolomite-granite waste. Ceram. Int. 2024, 50, 55498–55507. [Google Scholar]
- Cheng, J.; Ran, D.; Shu, C.M.; Zhu, S.; Zhang, Y. Resource utilisation of waste TPU: Reform and modification for improving a bentonite-based thermal insulation material’s performance. Inorg. Chem. Commun. 2023, 154, 110866. [Google Scholar]
- Qin, Y.; Hou, H.; Guo, M.; Liu, Q.; Tang, F. Simulated and experimental study on effect of thermal insulation layer on temperature field and heat dissipation of tunnel surrounding rock. Case Stud. Therm. Eng. 2024, 53, 103960. [Google Scholar]
- Yao, W.; Lyimo, H.; Pang, J. Evolution regularity of temperature field of active heat insulation tunnel considering thermal insulation spraying and grouting: A case study of Zhujidong Coal Mine, China. High Temp. Mater. Process. 2021, 40, 151–170. [Google Scholar]
- Li, W.; Feng, Z.; Su, H.; Zou, S.; Zeng, L. Analysis of thermal insulation effect of off-wall insulation lining in high-temperature tunnel. Case Stud. Therm. Eng. 2024, 61, 104957. [Google Scholar]
- Wang, C.; Du, Y.; Deng, Y.; Zhang, Y.; Deng, J.; Zhao, X.; Duan, X. Study on spontaneous combustion characteristics and early warning of coal in a deep mine. Fire 2023, 6, 396. [Google Scholar] [CrossRef]
- Kang, F.; Li, Y. Numerical study on airflow temperature field in a high-temperature tunnel with insulation layer. Appl. Therm. Eng. 2020, 179, 115654. [Google Scholar]
- Lu, T.; Zhang, G.; Liu, S.; Zheng, B.; Zhang, X. Numerical investigation of the temperature field and thermal insulation design of cold-region tunnels considering airflow effect. Appl. Appl. Therm. Eng. 2021, 191, 116923. [Google Scholar]
- Chen, X.; Zhou, X.; Wang, Y.; Liang, N.; Xu, B. Study on ambient temperature and humidity in the construction tunnel under the influence of ventilation and hot water gushing. Tunn. Undergr. Space Technol. 2024, 143, 105501. [Google Scholar]
- Ta, X.P. Supercritical CO2 Foam Concrete Foaming Mechanism and Its Application to Tunnel Surrounding Rock Insulation. Ph.D. Thesis, China University of Mining and Technology, Xuzhou, China, 2023. [Google Scholar]


| Previous Research and Shortcomings | Highlights of This Article |
|---|---|
| Qu et al. [12], Gong et al. [13], Chen et al. [14], and Zeng et al. [15] studied the temperature variation patterns in tunnels under the influence of fracture water, but did not consider changes in humidity. Li et al. [16] derived a model for the impact of water evaporation on airflow and thermal exchange between the tunnel surrounding rock. Wei et al. [17] and Wang et al. [20] studied the variation trends of temperature and humidity fields in tunnels under the influence of fissure water, without considering the presence of moisture-containing porous media. Qin et al. [28], Yao et al. [29], and Li et al. [30] conducted numerical simulations to study the effect of the method of using sprayed thermal insulation materials on the temperature field inside tunnels. | The impact of humid porous media on the thermal and humidity environment in tunnels is considered for the first time, quantitatively revealing the coupling mechanisms of surrounding rock thermal conduction, hot water evaporation, and airflow convection. The effects of drainage methods and insulation layers on the thermal and humidity environment in tunnels are also quantified. |
| Materials | Density kg/m3 | Thermal Conductivity Coefficient W/(m⋅K) | Specific Heat Capacity J/(kg⋅K) | Permeability (m2) | Porosity |
|---|---|---|---|---|---|
| Rock | 2400 | 2.3 | 880 | / | / |
| insulation layer | 750 | 0.08 | 2 | / | / |
| Cover plate | 2300 | 2.9 | 850 | / | / |
| Moisture-containing porous media | 1300 | 2 | 900 | 1 × 10−9 m2 | 0.45 |
| Parameters | Water Volume (m3/h) | Water Temperature Tw (°C) | Humidity-Containing Porous Media Areas (m2) | Area of Water Accumulations (m) |
|---|---|---|---|---|
| Values | 2.5 m3/h, 90 m3/h | 40 °C, 45 °C, 50 °C, 55 °C, 60 °C, 65 °C | 150 m2, 200 m2, 250 m2, 300 m2 | 150 m2, 200 m2, 250 m2, 300 m2 |
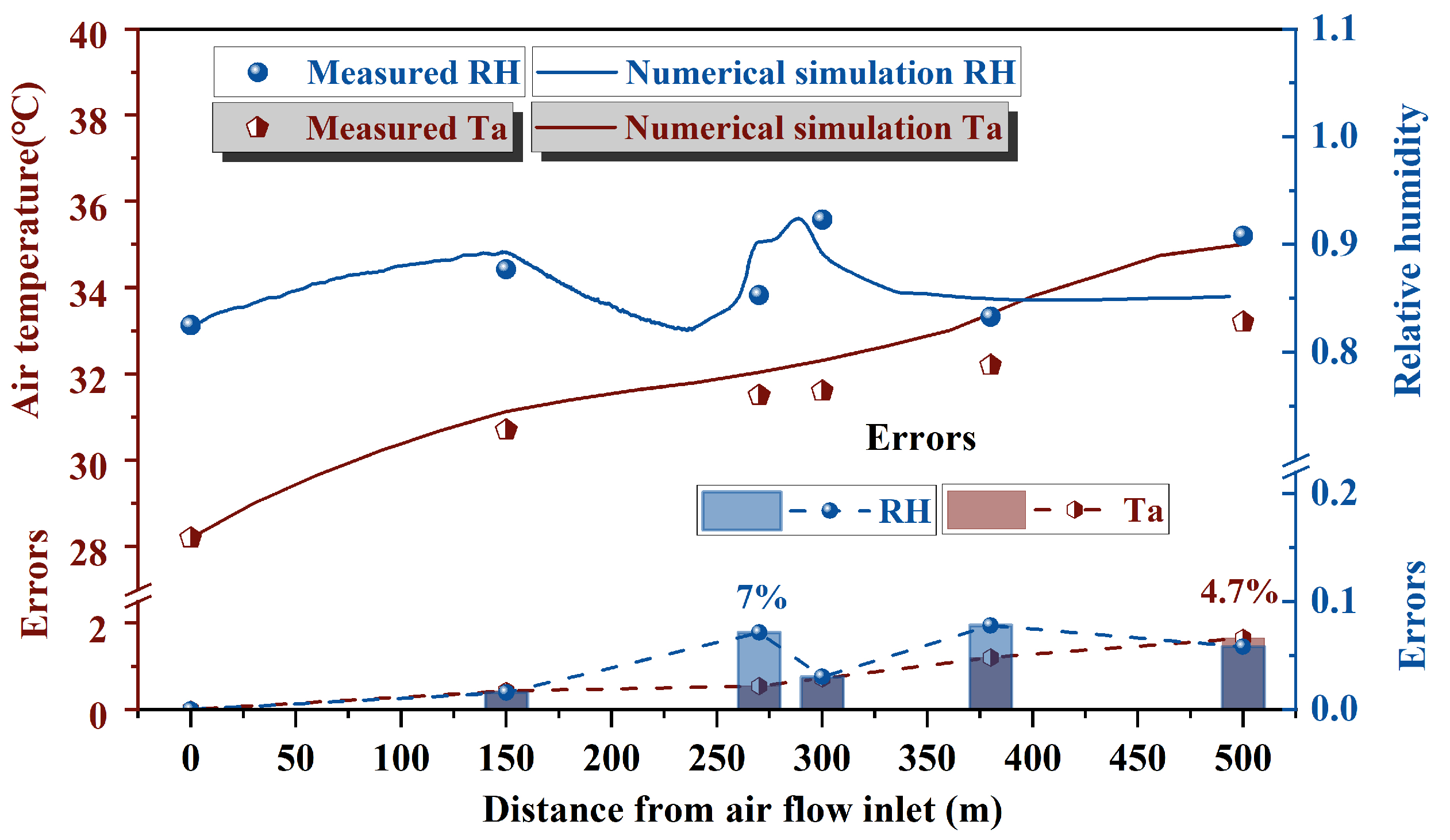
| Point 1 | Point 2 | Point 3 | Point 4 | Point 5 | Point 6 | |
|---|---|---|---|---|---|---|
| Ta (%) | 0 | 1.4 | 1.68 | 2.2 | 3.5 | 4.7 |
| RH (%) | 0 | 1.6 | 7 | 3.1 | 7 | 5.9 |
| Simulation Group | Water Temperature (°C) | Surge Volume (m3/s) | Cover Thickness (cm) | Insulation Thickness (cm) |
|---|---|---|---|---|
| Working Condition ① | 65 | 90 | 0 | 0 |
| Working Condition ② | 5 | 0 | ||
| Working condition ③ | 5 | 10 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Zhang, X.; Xin, S.; Liu, S.; Wang, W.; Shi, C. Research on the Formation Mechanism of Hot-Water Heat Damage in High-Temperature Roadway and the Cooling Effect of Spraying a Thermal Insulation Layer. Coatings 2025, 15, 382. https://doi.org/10.3390/coatings15040382
Zhang X, Xin S, Liu S, Wang W, Shi C. Research on the Formation Mechanism of Hot-Water Heat Damage in High-Temperature Roadway and the Cooling Effect of Spraying a Thermal Insulation Layer. Coatings. 2025; 15(4):382. https://doi.org/10.3390/coatings15040382
Chicago/Turabian StyleZhang, Xuan, Song Xin, Shangxiao Liu, Wei Wang, and Caihua Shi. 2025. "Research on the Formation Mechanism of Hot-Water Heat Damage in High-Temperature Roadway and the Cooling Effect of Spraying a Thermal Insulation Layer" Coatings 15, no. 4: 382. https://doi.org/10.3390/coatings15040382
APA StyleZhang, X., Xin, S., Liu, S., Wang, W., & Shi, C. (2025). Research on the Formation Mechanism of Hot-Water Heat Damage in High-Temperature Roadway and the Cooling Effect of Spraying a Thermal Insulation Layer. Coatings, 15(4), 382. https://doi.org/10.3390/coatings15040382





