Voltage Tunable Spoof Surface Plasmon Polariton Waveguide Loaded with Ferroelectric Resonators
Abstract
1. Introduction
2. Experiments
3. Results and Discussion
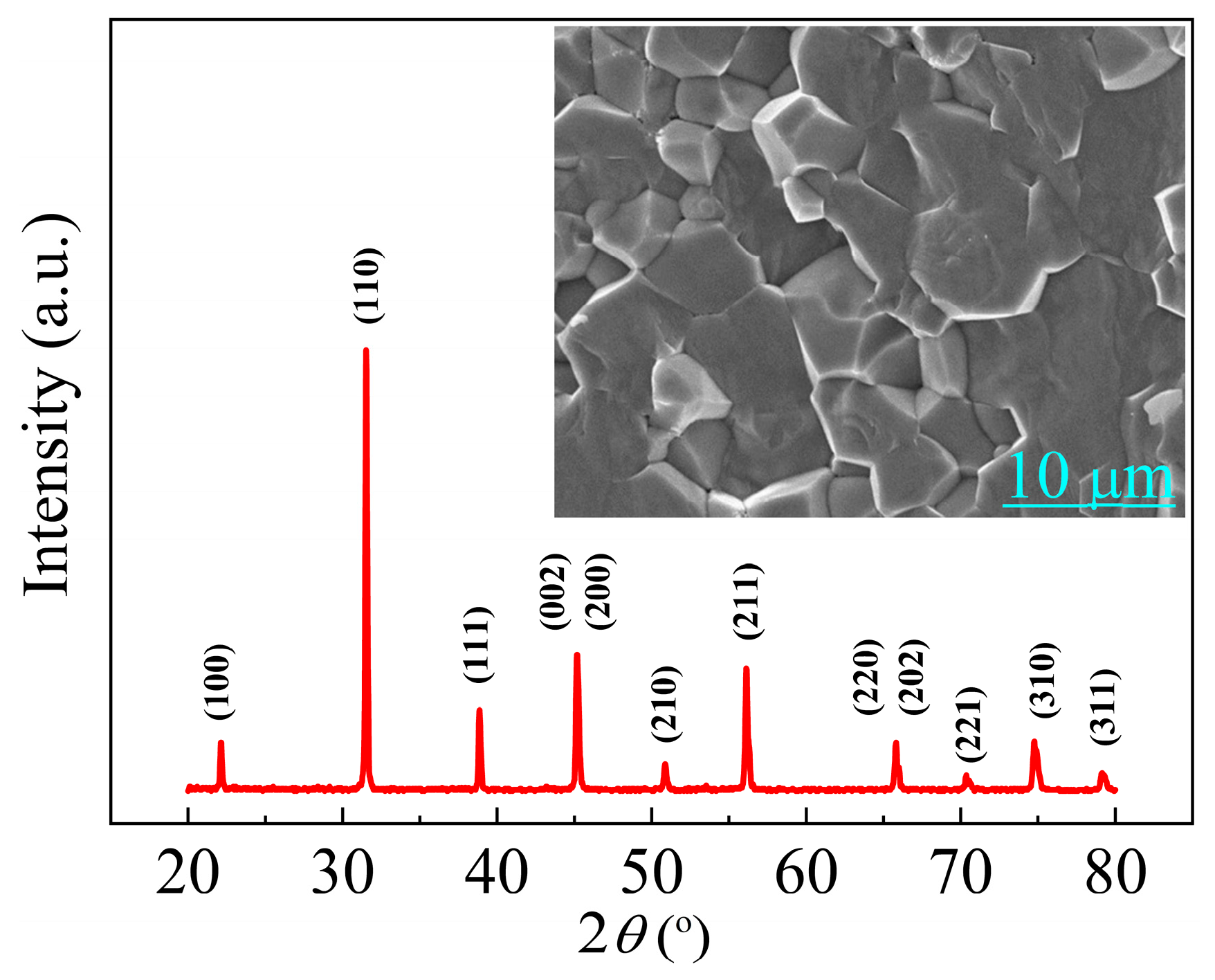
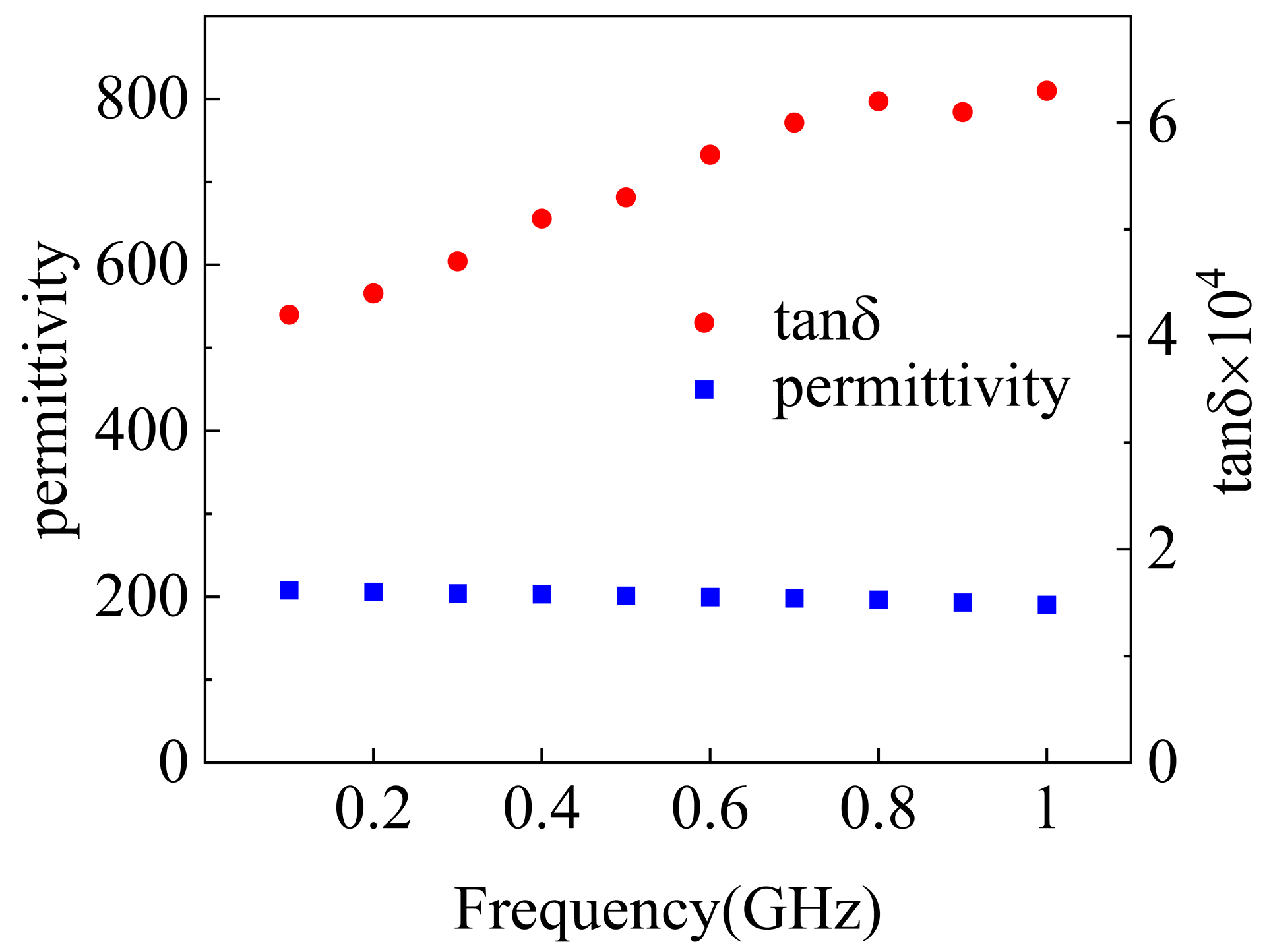
3.1. Crystal Structure and Dielectric Properties of BCZT Ferroelectric
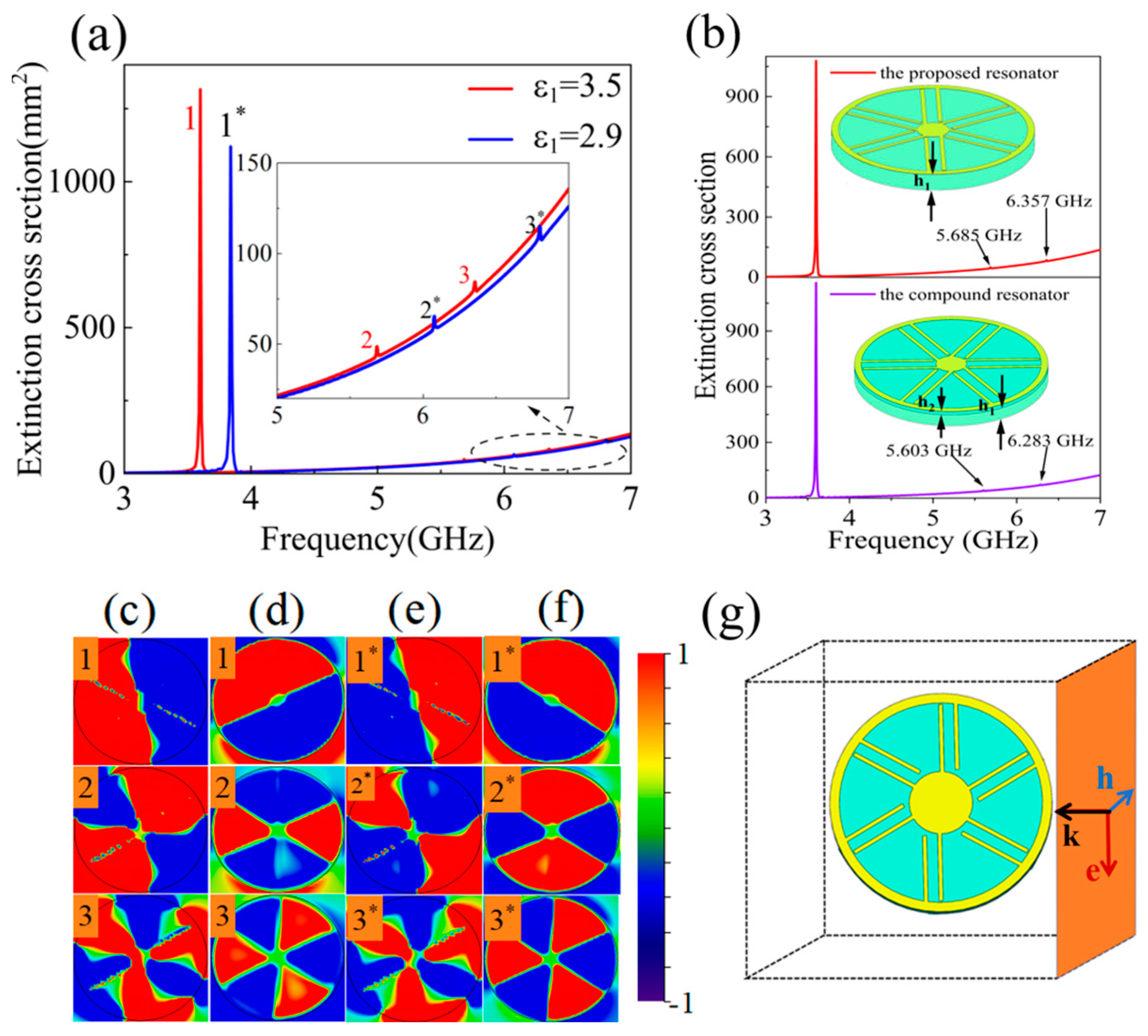
3.2. Design of LSSP Resonator with BCZT Ferroelectric Compound Substrate
3.3. Tunability of SSPP Waveguide Loaded with BCZT LSSP Resonator
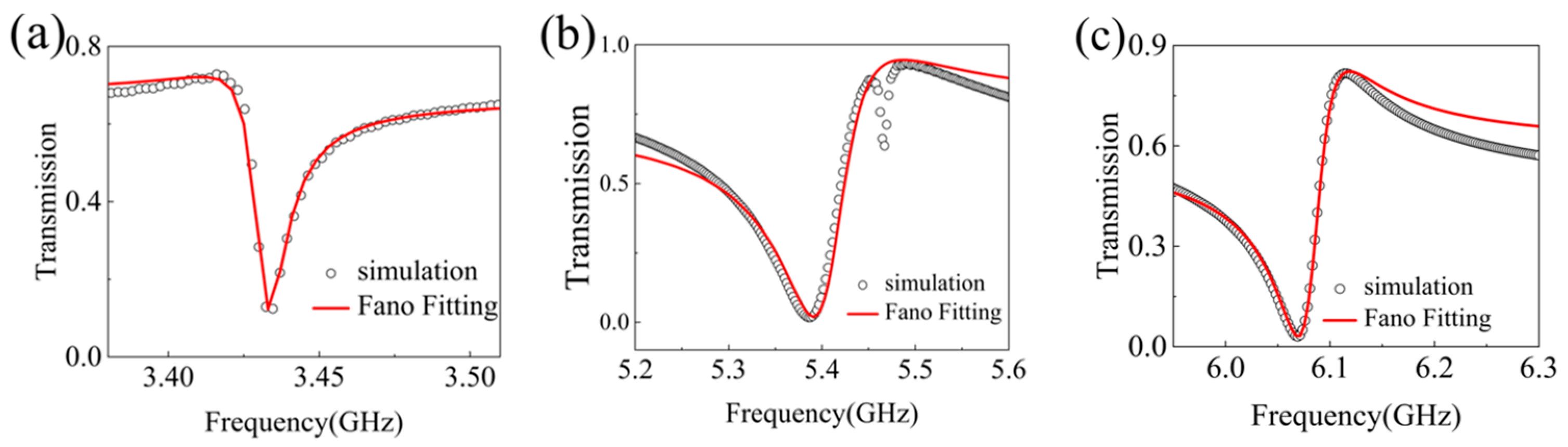
3.4. Coupling Mechanism Between BCZT LSSP Resonator and SSPP Waveguide
4. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Pendry, J.B.; Holden, A.J.; Stewart, W.J.; Youngs, I. Extremely low frequency plasmons in metallic mesostructures. Phys. Rev. Lett. 1996, 76, 4773. [Google Scholar] [PubMed]
- Zayats, A.V.; Smolyaninov, I.I.; Maradudin, A.A. Nano-optics of surface plasmon polaritons. Phys. Rep. 2005, 408, 131–314. [Google Scholar]
- Garcia-Vidal, F.J.; Martin-Moreno, L.; Pendry, J.B. Surfaces with holes in them: New plasmonic metamaterials. J. Opt. A Pure Appl. Opt. 2005, 7, S97. [Google Scholar]
- Pendry, J.B.; Martin-Moreno, L.; Garcia-Vidal, F.J. Mimicking surface plasmons with structured surfaces. Science 2004, 305, 847–848. [Google Scholar]
- Ritchie, R.H. Plasma losses by fast electrons in thin films. Phys. Rev. 1957, 106, 874. [Google Scholar]
- Liebsch, A. Electronic Excitations at Metal Surfaces; Springer Science & Business Media: Berlin/Heidelberg, Germany, 2013. [Google Scholar]
- Zhang, X.; Cui, T.J. Deep-subwavelength and high-Q trapped mode induced by symmetry-broken in toroidal plasmonic resonator. IEEE Trans. Antennas Propag. 2020, 69, 2122–2129. [Google Scholar]
- Maier, S.A. Plasmonics: Fundamentals and Applications; Springer: New York, NY, USA, 2007. [Google Scholar]
- Baimuratov, A.S.; Tepliakov, N.V.; Gun’ko, Y.K.; Baranov, A.V.; Fedorov, A.V. Mixing of quantum states: A new route to creating optical activity. Sci. Rep. 2016, 6, 5. [Google Scholar]
- Homola, J. Present and future of surface plasmon resonance biosensors. Anal. Bioanal. Chem. 2003, 377, 528–539. [Google Scholar]
- Pan, L.; Wu, Y.; Wang, W.; Wei, Y.; Yang, Y. A flexible high-selectivity single-layer coplanar waveguide bandpass filter using interdigital spoof surface plasmon polaritons of bow-tie cells. IEEE Trans. Plasma Sci. 2020, 48, 3582–3588. [Google Scholar]
- Jaiswal, R.K.; Pandit, N.; Pathak, N.P. Spoof plasmonic-based band-pass filter with high selectivity and wide rejection bandwidth. IEEE Photonics Technol. Lett. 2019, 31, 1293–1296. [Google Scholar]
- Wang, J.; Zhao, L.; Hao, Z.C. A band-pass filter based on the spoof surface plasmon polaritons and CPW-based coupling structure. IEEE Access 2019, 7, 35089–35096. [Google Scholar] [CrossRef]
- Guo, Y.J.; Xu, K.D.; Deng, X.; Cheng, X.; Chen, Q. Millimeter-wave on-chip bandpass filter based on spoof surface plasmon polaritons. IEEE Electron Device Lett. 2020, 41, 1165–1168. [Google Scholar] [CrossRef]
- Zhang, D.; Sun, Y.; Zhang, K.; Wu, Q.; Jiang, T. Short-circuited stub-loaded spoof surface plasmon polariton transmission lines with flexibly controllable lower out-of-band rejections. Opt. Lett. 2021, 46, 4354–4357. [Google Scholar] [CrossRef] [PubMed]
- Yang, Z.; Zhang, B.; Chen, W.; Yang, T. Rejection of spoof SPPs using the second resonant mode of vertical split-ring resonator. IEEE Microw. Wirel. Compon. Lett. 2018, 29, 23–25. [Google Scholar] [CrossRef]
- Li, W.; Qin, Z.; Wang, Y.; Ye, L.; Liu, Y. Spoof surface plasmonic waveguide and its band-rejection filter based on H-shaped slot units. J. Phys. D Appl. Phys. 2019, 52, 365303. [Google Scholar] [CrossRef]
- Yan, D.; Li, X.; Ma, C. Terahertz refractive index sensing based on gradient metasurface coupled confined spoof surface plasmon polaritons mode. IEEE Sens. J. 2021, 22, 324–329. [Google Scholar] [CrossRef]
- Kumari, A.; Singh, S.P.; Tiwari, N.K. Design of a differential spoof surface plasmon sensor for dielectric sensing and defect detection. IEEE Sens. J. 2022, 22, 3188–3195. [Google Scholar] [CrossRef]
- Fu, J.H.; Wu, W.J.; Wang, D.W. High-sensitivity microfluidic sensor based on quarter-mode interdigitated spoof plasmons. IEEE Sens. J. 2022, 22, 23888–23895. [Google Scholar] [CrossRef]
- Dai, L.H.; Zhao, H.Z.; Zhao, X. Flexible and printed microwave plasmonic sensor for noninvasive measurement. IEEE Access. 2020, 8, 163238–163243. [Google Scholar]
- Xu, K.D.; Lu, S.; Guo, Y.J.; Chen, Q. High-order mode of spoof surface plasmon polaritons and its application in bandpass filters. IEEE Trans. Plasma Sci. 2020, 49, 269–275. [Google Scholar] [CrossRef]
- Mira, F.; Mateu, J.; Collado, C. Mechanical tuning of substrate integrated waveguide filters. IEEE Trans. Microw. Theory Tech. 2015, 63, 3939–3946. [Google Scholar] [CrossRef]
- Zhang, C.; Zhou, Y.J. Electronically controlled spoof localized surface plasmons on the corrugated ring with a shorting pin. J. Mod. Opt. 2018, 65, 1535–1541. [Google Scholar]
- Ding, J.; Zhao, P.; Chen, H.; Fu, H. Ultraviolet photodetectors based on wide bandgap semiconductor: A review. J. Appl. Phys. A 2024, 130, 350. [Google Scholar]
- Pande, S.; Patil, D.; Kumar, A.; Tyavlovsky, A.K.; Muthanna, A. Design of a metasurface loaded with RF varactor and PIN diode integration dual-band reconfigurable antenna for bluetooth, Wi-Fi, WLAN and wireless 5G applications. Phys. Scr. 2025, 100, 025537. [Google Scholar]
- Xiong, Y.Z.; Christy, A.; Dong, Y. Combinatorial split-ring and spiral metaresonator for efficient magnon-photon coupling. Phys. Rev. Appl. 2024, 21, 034034. [Google Scholar] [CrossRef]
- Ma, J.; Hu, J.M.; Li, Z.; Nan, C.W. Recent progress in multiferroic magnetoelectric composites: From bulk to thin films. Adv. Mater. 2011, 42, 1062–1087. [Google Scholar]
- Li, S.Q.; Du, C.H. Efficient magnetic-coupling excitation of LSSPs on high-Q multilayer planar-circular-grating resonators. Opt. Express 2021, 29, 25189–25201. [Google Scholar] [CrossRef] [PubMed]
- Zhu, B.; Hu, M.Z. Plasmonic dual-band waveguide with independently controllable band-notched characteristics. Appl. Phys. Express 2023, 16, 086001. [Google Scholar]
- Ramesh, R.; Spaldin, N.A. Multiferroics: Progress and prospects in thin films. Nat. Mater. 2007, 6, 21–29. [Google Scholar]
- Zhang, G.; Dong, S.; Yan, Z.; Guo, Y.; Zhang, Q.; Yunoki, S.; Dagotto, E.; Liu, J.M. Multiferroic properties of CaMn7O12. Phys. Rev. B 2011, 84, 174413. [Google Scholar] [CrossRef]
- Lou, J.; Wang, J.; Ma, H. Tunable spoof surface plasmon polariton transmission line based on ferroelectric thick film. Appl. Phys. A 2019, 125, 737. [Google Scholar] [CrossRef]
- Miranda, F.A.; Subramanyam, G.; Vankeuls, F.W.; Romanofsky, R.R. Design and development of ferroelectric tunable microwave components for Ku- and K-band satellite communication systems. ZEEE Trans. Microw. Theory Tech. 2000, 48, 1181–1189. [Google Scholar]
- Schileo, G.; Pascual-Gonzalez, C.; Alguero, M.; Reaney, I.M.; Postolache, P.; Mitoseriu, L.; Reichmann, K.; Venet, M.; Feteira, A. Multiferroic and magnetoelectric properties of Pb0.99[Zr0.45Ti0.47(Ni1/3Sb2/3)0.08] O3-CoFe2O4 multilayer composites fabricated by tape casting. J. Eur. Ceram. Soc. 2018, 38, 1473–1478. [Google Scholar] [CrossRef]
- Liu, W.; Ren, X. Large piezoelectric effect in Pb-free ceramics. Phys. Rev. Lett. 2009, 103, 257602. [Google Scholar]
- Nan, C.W.; Bichurin, M.I.; Dong, S.; Viehland, D.; Srinivasan, G. Multiferroic magnetoelectric composites: Historical perspective, status, and future directions. J. Appl. Phys. 2008, 103, 03110. [Google Scholar]
- Li, T.; Zhang, F.; Fang, H.; Li, K.; Yu, F. The magnetoelectric properties of La0.7Sr0.3MnO3/BaTiO3 bilayers with various orientations. J. Alloy. Compd. 2013, 560, 167–170. [Google Scholar] [CrossRef]
- Li, T.; Wang, H.; Ma, D.; Li, K.; Hu, Z. Influence of clamping effect in BaTiO3 film on the magnetoelectric behavior of layered multiferroic heterostructures. Mater. Res. Bull. 2019, 115, 116–120. [Google Scholar] [CrossRef]
- Martin, L.W.; Ramesh, R. Multiferroic and magnetoelectric heterostructures. Acta Mater. 2012, 60, 2449–2470. [Google Scholar] [CrossRef]
- Fetisov, Y.K.; Srinivasana, G. Electric field tuning characteristics of a ferrite-piezoelectric microwave resonator. Appl. Phys. Lett. 2006, 88, 143503. [Google Scholar] [CrossRef]
- Ustinov, A.; Srinivasan, G.; Kalinikos, B.A. Ferrite-ferroelectric hybrid wave phase shifters. Appl. Phys. Lett. 2007, 90, 031913. [Google Scholar] [CrossRef]
- Pettiford, C.; Dasgupta, S.; Lou, J.; Yoon, S.D.; Sun, N.X. Bias field effects on microwave frequency behavior of PZT/YIG magnetoelectric bilayer. IEEE Trans. Magn. 2007, 43, 3343. [Google Scholar]
- Zine-El-Abidine, I.; Okoniewski, M. A tunable radio frequency MEMS inductor using MetalMUMPs. J. Micromech. Microeng. 2007, 17, 2280. [Google Scholar]
- Hummel, G.; Hui, Y.M.; Rinaldi, Y. Reconfigurable piezoelectric MEMS resonator using phase change material programmable vias. J. Microelectromech. Syst. 2015, 24, 2145–2151. [Google Scholar] [CrossRef]
- Wang, J.; Li, Z.; Wang, J.; He, H.; Nan, C. Effect of thickness on the stress and magnetoelectric coupling in bilayered Pb (Zr0.52Ti0.48) O3-CoFe2O4 films. J. Appl. Phys. 2015, 117, 759. [Google Scholar]
- Fan, K.B.; Averitt, R.D.; Padilla, W.J. Active and tunable nanophotonic metamaterials. Nanophotonics 2022, 11, 3769–3803. [Google Scholar]
- Dai, Y.Q.; Dai, J.M.; Tang, X.W.; Zhang, K.J.; Zhu, X.B.; Yang, J.; Sun, Y.P. Thickness effect on the properties of BaTiO3-CoFe2O4 multilayer thin films prepared by chemical solution deposition. J. Alloy. Compd. 2014, 587, 681–687. [Google Scholar] [CrossRef]
- Pertsev, N.A.; Zembilgotov, A.G.; Tagantsev, A.K. Effect of mechanical boundary conditions on phase diagrams of epitaxial ferroelectric thin films. Phys. Rev. Lett. 1998, 80, 1988–1991. [Google Scholar]
- Ion, V.; Craciun, F.; Scarisoreanu, N.D.; Moldovan, A.; Andrei, A.; Birjega, R.; Ghica, C. Impact of thickness variation on structural, dielectric and piezoelectric properties of (Ba, Ca) (Ti, Zr) O3 epitaxial thin films. Sci. Rep. 2018, 8, 2056. [Google Scholar]
- Pei, H.; Zhang, Y.; Guo, S.; Ren, L.; Yan, H.; Luo, B. Orientation-dependent optical magnetoelectric effect in patterned BaTiO3/La0. 67Sr0. 33MnO3 heterostructures. ACS Appl. Mater. Interfaces 2018, 10, 30895–30900. [Google Scholar]
- Li, S.B.; Wang, C.B.; Shen, Q.; Zhang, L.M. Enhanced dielectric properties in Ba0.85Ca0.15Zr0.10Ti0.90O3/La0.67Ca0.33MnO3 laminated composite. Scr. Mater. 2018, 144, 40–43. [Google Scholar]
- Hu, M.Z.; Li, S.; Wang, C.B. Orientation-dependent multiferroic properties in BCZT/LCMO thin films. Ceram. Int. 2021, 103, 385–991. [Google Scholar]
- Mercier, D.; Niembro-Martin, A.; Sibuet, H.; Baret, C.; Chautagnat, J. X Band Distributed Phase Shifter Based on Sol-Gel BCTZ Varactors. In Proceedings of the 2017 European Radar Conference (EURAD), Nuremberg, Germany, 11–13 October 2017; IEEE: New York, NY, USA; pp. 382–385. [Google Scholar]
- Mercier, D.; Sibuet, H.; Dieppedale, C.; Chautagnat, J.; Baret, C.; Bonnard, C.; Guillaume, J.; Gardes, P.; Poveda, P.; Le Rhun, G.; et al. 8 to 13 GHz tunable filter based on sol-gel BCTZ varactors. In Proceedings of the 2017 IEEE Asia Pacific Microwave Conference (APMC), Kuala Lumpur, Malaysia, 13–16 November 2017; IEEE: New York, NY, USA; pp. 686–689. [Google Scholar]
- Zhang, X.R.; Yan, R.T.; Cui, T.J. High-FoM Resonance in Single Hybrid Plasmonic Resonator via Electro-Magnetic Modal Interference. IEEE Trans. Antennas Propag. 2020, 68, 6447–6451. [Google Scholar]
- Hu, M.Z.; Gu, H.S.; Chu, X.C.; Qian, J.; Xia, Z. Crystal structure and dielectric properties of (1−x) Ca0. 61Nd0. 26TiO3+ xNd (Mg1/2Ti1/2) O3 complex perovskite at microwave frequencies. J. Appl. Phys. 2008, 104, 124104. [Google Scholar]
- Lei, S.; Hu, M.Z.; Xu, J.; Zhou, C.; Zhao, Q.; Zhang, L.; Zhang, H. Real-time tunable notched waveguide based on voltage controllable ferroelectric resonator. Opt. Express 2024, 32, 10587–10598. [Google Scholar] [PubMed]
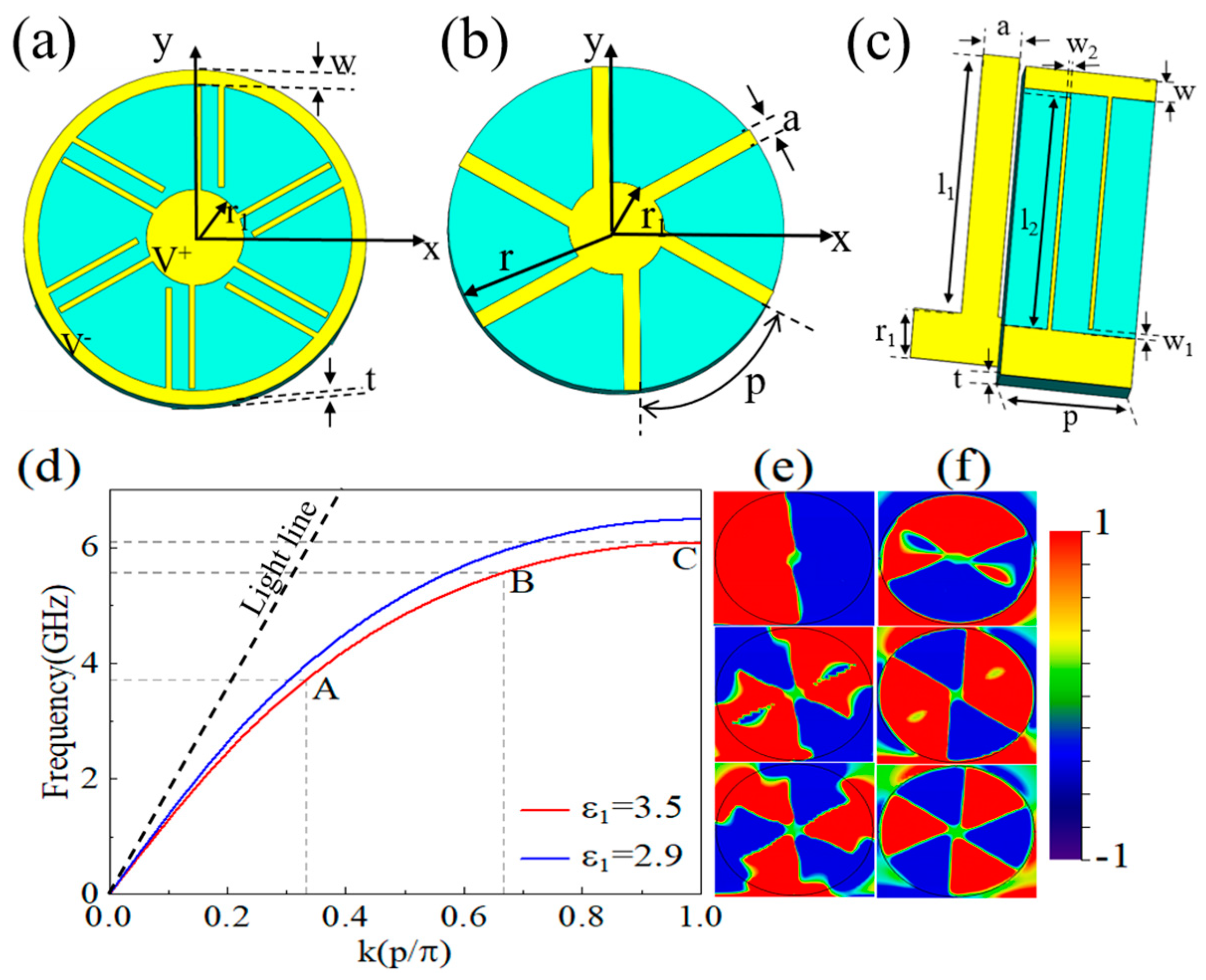
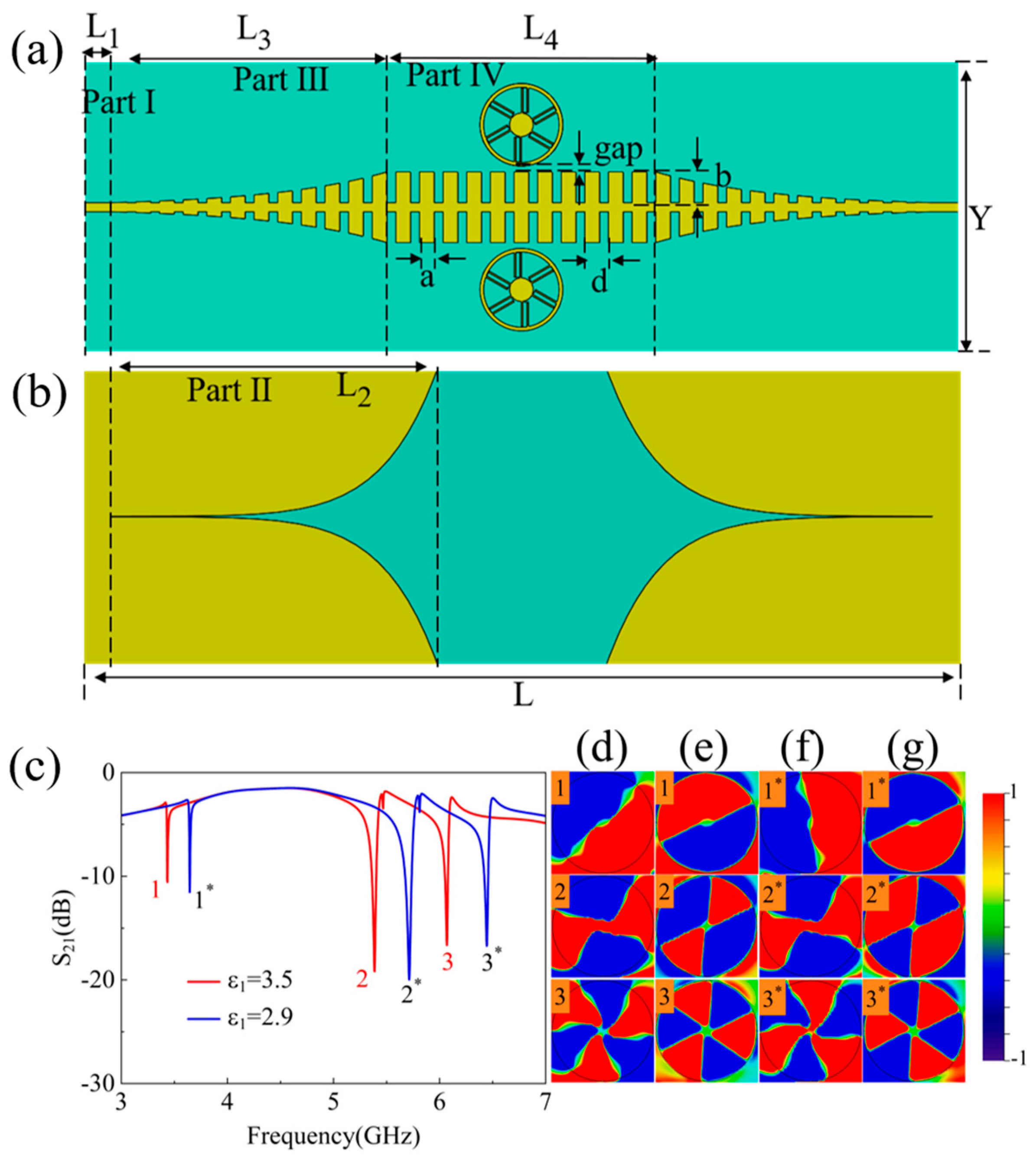

| Structure | Symbol | Size (mm) |
|---|---|---|
| the lower surface metal strip length | l1 | 5.25 |
| the upper metal strip length | l2 | 4.71 |
| the gap between upper center circle and metal strip | w1 | 0.1 |
| the upper surface metal strip width | w2 | 0.08 |
| the upper and lower center metal circle radius | r1 | 1 |
| the upper metal ring width | w | 0.44 |
| the lower surface metal strip width | a | 0.7 |
| the periodic of the circle slots | p | 6.545 |
| the thickness of the dielectric substrate | t | 0.65 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Shen, J.; Lei, S.; Hu, M.; Zhou, C.; Luo, S.; Wang, C. Voltage Tunable Spoof Surface Plasmon Polariton Waveguide Loaded with Ferroelectric Resonators. Coatings 2025, 15, 378. https://doi.org/10.3390/coatings15040378
Shen J, Lei S, Hu M, Zhou C, Luo S, Wang C. Voltage Tunable Spoof Surface Plasmon Polariton Waveguide Loaded with Ferroelectric Resonators. Coatings. 2025; 15(4):378. https://doi.org/10.3390/coatings15040378
Chicago/Turabian StyleShen, Jiaxiong, Shun Lei, Mingzhe Hu, Chaobiao Zhou, Shengyun Luo, and Chuanbin Wang. 2025. "Voltage Tunable Spoof Surface Plasmon Polariton Waveguide Loaded with Ferroelectric Resonators" Coatings 15, no. 4: 378. https://doi.org/10.3390/coatings15040378
APA StyleShen, J., Lei, S., Hu, M., Zhou, C., Luo, S., & Wang, C. (2025). Voltage Tunable Spoof Surface Plasmon Polariton Waveguide Loaded with Ferroelectric Resonators. Coatings, 15(4), 378. https://doi.org/10.3390/coatings15040378






