1. Introduction
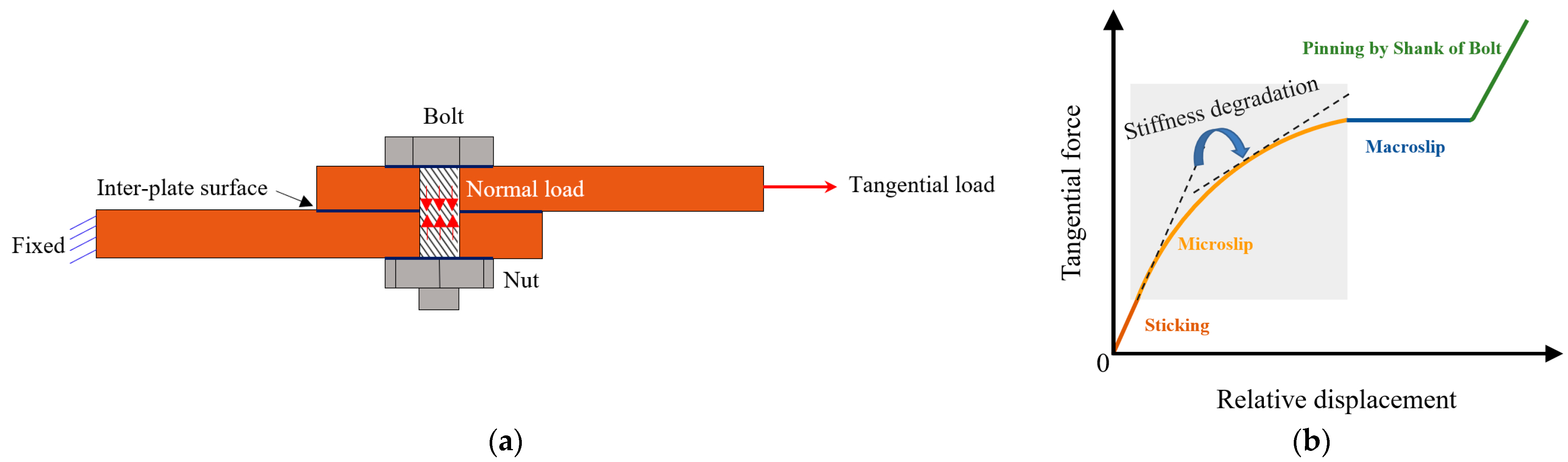
Bolted joints are indispensable elements in mechanical systems, as shown in
Figure 1a. The stiffness of bolted joints depends on the amplitude of oscillatory displacements, and the energy dissipation caused by the friction mechanism dominates the structural damping, reaching 90% of the total damping [
1,
2]. Due to the presence of friction at joint interfaces, the dynamic behavior of joint structures is nonlinear and shows stiffness softening and energy dissipation [
3]. The tangential stiffness softening is accompanied by the evolution of contact conditions. The evolution of contact states can be divided into four stages [
4]: sticking, microslip, macroslip, and pinning by bolt shank. The relationship between stiffness softening and evolution is shown in
Figure 1b, and the stiffness degradation occurs in the microslip. In addition, the evolution of the contact conditions may result in the instability of mechanical systems [
5,
6,
7]. Therefore, a constitutive model is required to describe and predict the softening process to reduce the impact of the stiffness degradation of bolted joints in the design.
In the past decades, scholars have conducted a great deal of research on joints under different loadings [
8,
9,
10,
11], and several dynamic friction models have been developed to represent the nonlinear degradation of the bolted joint. The Iwan model was first proposed by S. P. Timoshenko to characterize the elastic–plastic behavior of materials and was later extended by Iwan to describe the nonlinear degradation behavior of bolted joints [
12]. The Dahl model [
13] is designed based on the dynamic friction experiments of the servo systems to simulate a symmetrical hysteresis loop. The Bristle model [
14] assumes contact as two rigid bodies contacted by elastic bristles, namely elastic bristles and rigid bristles. Haessig and Friedland developed a bristle model to characterize random behavior and a reset-integral model to describe bristle aggregation behavior [
15]. Based on the Dahl and the Bristle models, the LuGre model [
16,
17] was developed to characterize the static friction force and the Stribeck phenomenon. The Valanis model [
18] can describe the evolution of the micro- and macro-slip and reproduce the response under mixed-mode loadings, which is widely used in plasticity.
The Iwan model is widely used in joint dynamics for its ability to reproduce the elastoplastic features in the stiffness softening and has been developed continuously. The core of the Iwan model is the elastic–plastic elements consisting of springs and sliders. The theoretical degradation curve of the joint can be derived by solving the density function of the critical yield force of the elements. Therefore, the core of the Iwan model is the density function of the yield force, and its parameters are identified by fitting the experimental results in the classical Iwan model. In recent years, the Iwan model and its density function (DF) have been revised. Song [
19] proposed a modified Iwan model with an individual linear spring to characterize the residual stiffness in microslip. Segalman [
20] designed the BMD experimental device to study the power–law relationship between energy dissipation and load amplitude and proposed the truncated power law density function to describe the power–law feature in energy dissipation. Li et al. [
21] improved the truncated DF of the Segalman model and proposed a six-parameter DF that can reproduce the energy dissipation and residual stiffness characteristic. The earlier studies have advanced the development of the Iwan model, but in general, they all lack predictive ability and physical mechanisms.
Among the latest research, a new method for establishing the Iwan model is proposed, which improves the prediction ability and physical mechanism. Li et al. first proposed a technique of identifying the DF by Coulomb friction theory [
3,
22,
23]. Zhao et al. [
24] applied this method and performed a verification experiment.
However, the basic assumption of this method is that the pressure distribution remains constant under mixed-mode loading. Wang et al. [
25] found that the assumption was not tenable and proposed a new approach to solve the DF by discretizing the dynamic contact region. This method provides a technique to solve the DF under mixed-mode loading without the assumption of constant pressure. However, one of the prerequisites is to establish a function to describe the dynamic pressure distribution under mixed-mode loading. The key point of the new model is the function describing the dynamic pressure distribution.
Many scholars have studied the pressure distribution on the inter-plate surface of bolted joints. Ito et al. [
26] applied the ultrasonic to the pressure distribution of the flange and reached two conclusions: the pressure distribution is related to the surface roughness, and the contact area is larger than the theoretical analysis results. Mantelli et al. [
27] applied a pressure-sensitive film to measure the pressure distribution of bolted joints and analyzed the applicability of various theoretical models. The non-invasive feature of the ultrasonic method has attracted many researchers. Many scholars have also conducted research using this method. Many studies based on ultrasonic methods have been carried out [
28,
29,
30]. However, the ultrasonic method also has certain limitations, and the pressure measurement will be seriously interfered with by the nut-plate surface. Thus, the bolted joints are usually redesigned before measurement. In addition, there is currently no experimental protocol for measuring the pressure distribution of bolted joints under mixed-mode loading conditions. Therefore, the finite element method is widely used in the pressure distribution of bolted joints [
19,
31,
32,
33].
This paper aims to study the law of contact pressure under mixed-mode loading and establish the dynamic pressure distribution function. We analyzed the variation law of the contact boundary and the pressure amplitude with the load, respectively. Two functions are proposed to describe the contact boundary and pressure distribution, and the correction methods unifying the two functions are proposed and analyzed. The functions and methods in this paper can provide a basis for the DF solution of the dynamic Iwan model.
2. Dynamic Density Function Modeling Technique
The classic Iwan model is composed of
Jenkins elements, as shown in
Figure 2a [
25]. The Jenkins element is composed of a spring with stiffness
and a friction resistor with critical sliding force
in series. The Jenkins element is an ideal piecewise unit that can reproduce either slip or stick [
3]. In the dynamic DF modelling technique, the yield force of each Jenkins element is related to the contact pressure by Coulomb friction law, as shown in
Figure 2b.
The function of contact boundary in microslip is [
25]:
The ellipticity of the
ellipse is
linearly increasing from 1 to with the increase of
from 0 to
. Therefore, the
can be expressed as:
The semi-minor axis
and semi-major axis
of the
ellipse is:
Then, the area of the
sliding area is:
The elliptic discrete method sacrifices part of the preload, and the pressure needs to be corrected based on the bolt preload. The corrected pressure distribution is:
where
is the angle with the
x-axis, and is the pressure distribution before correction at angle
.
The normal load of the sliding area is:
Thus, the friction shear stress
of the
sliding area is:
When
tends to infinite, the DF of friction shear stress
can be deduced by normalization and derivation. Additionally, the DF of the yield force in Iwan model can be obtained through the mapping relationship as:
where
is the mapping parameter.
In the dynamic DF solution, it is necessary to determine the dynamic function expressions of the contact pressure. In this paper, the relationship between the contact pressure and the tangential force is studied based on the modeling method in the Ref. [
25].
4. Function Consistency and Preload Compensation
Through the above analysis, the pressure distribution presents different rules at different angles. The pressure and boundary functions have increased the difficulty of solving the DF of the Iwan model. Essentially, the boundary function is an expression for the location at zero pressure. Therefore, the pressure function needs to be reconciled with the boundary function. In addition, since the boundary function is assumed to be elliptical, the preload loss needs to be compensated by modifying the dynamic pressure function.
In conclusion, there are two points that need to be corrected:
- (1)
Correction of the preload: enforce total normal force equal to bolt preload .
- (2)
Correction of the initial pressure distribution function at : make the pressure function boundary equal to .
The valid interval of is . There are two correction methods: translation method and constraint method.
4.1. Translation Method
In the translation method, the consistency of the boundary function and pressure function is satisfied by shifting the initial function
. The first corrected function
is:
According to Equation (17), the second corrected dynamic function
is:
Based on Equation (18), the pressure in the
sliding area is:
The normal force
of the
area is
, and the total normal force
is
. Then, the compensation parameter
is:
The final dynamic pressure function and normal load of
area is:
The parameters of pressure distribution are identified by the finite element calculation, as shown in
Table 1.
The influence of different discrete orders
on the correction results of translation method was analyzed. According to Equation (17) and
Table 1, the contact pressure distribution function
was obtained. Based on
Table 1, the parameters of the discrete regions can be derived, as shown in
Table 2.
Based on
Table 2 and
,
can be deduced. When tangential force
, the curves of
and
coincide.
can be obtained by correcting the normal force. The normal forces of discrete areas before and after correction are shown in
Table 3.
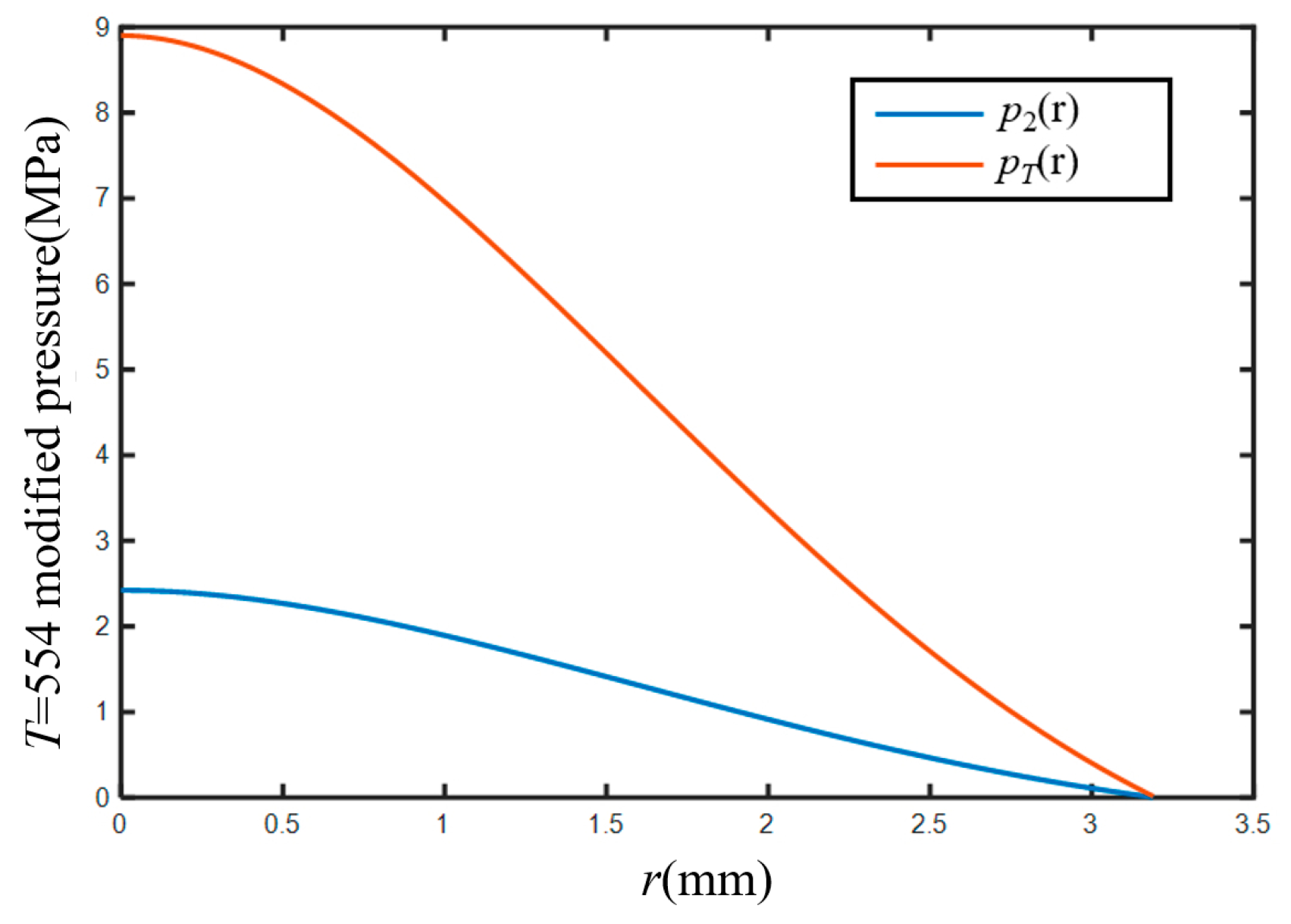
Figure 7 shows the pre- and post-corrected distribution curves. The final corrected curve
under the dispersion of the third order is very different from the curve of
, which means that the compensated pre-tightening force is large.
Through finite element calculation, microslip occurs when
. Therefore,
is the solution interval of the dynamic Iwan model. Taking
as an example, the semi-major axis, the semi-minor axis and the corresponding area of the discrete area can be deduced, as shown in
Table 4.
When
, the correction results of normal forces are as shown in
Table 5.
The corrected curves are shown in
Figure 8. The amplitude of the correction is large in the 3rd discrete, and the maximum pressure is raised by about four times.
The influence of discrete order on pressure correction was calculated in two cases of
and
. The contact pressure curves of higher discrete orders such as 5th, 8th and 100th were calculated, respectively, as shown in
Figure 9. It can be seen that for different tangential loads, the higher the discrete order, the smaller the correction amplitude. The pressure curves of the 100th discrete and 1000th discrete were basically the same. That is, the pressure correction curve
is stable when the discrete order is high.
4.2. Constraint Method
In the constraint method, consistency can be achieved by the constraint of parameter identification as
. Under the constraint, the initial pressure distribution function is consistent with
. The corrected pressure distribution
with constraint method is:
Then, the pressure in the discrete region is:
The contact pressure in the
region is
, and the total contact pressure is
. The compensation parameter
is:
The dynamic contact pressure distribution and discrete area contact pressure of constraint method are:
The parameters of the constraint method are identified based on FEM results, as shown in
Table 6.
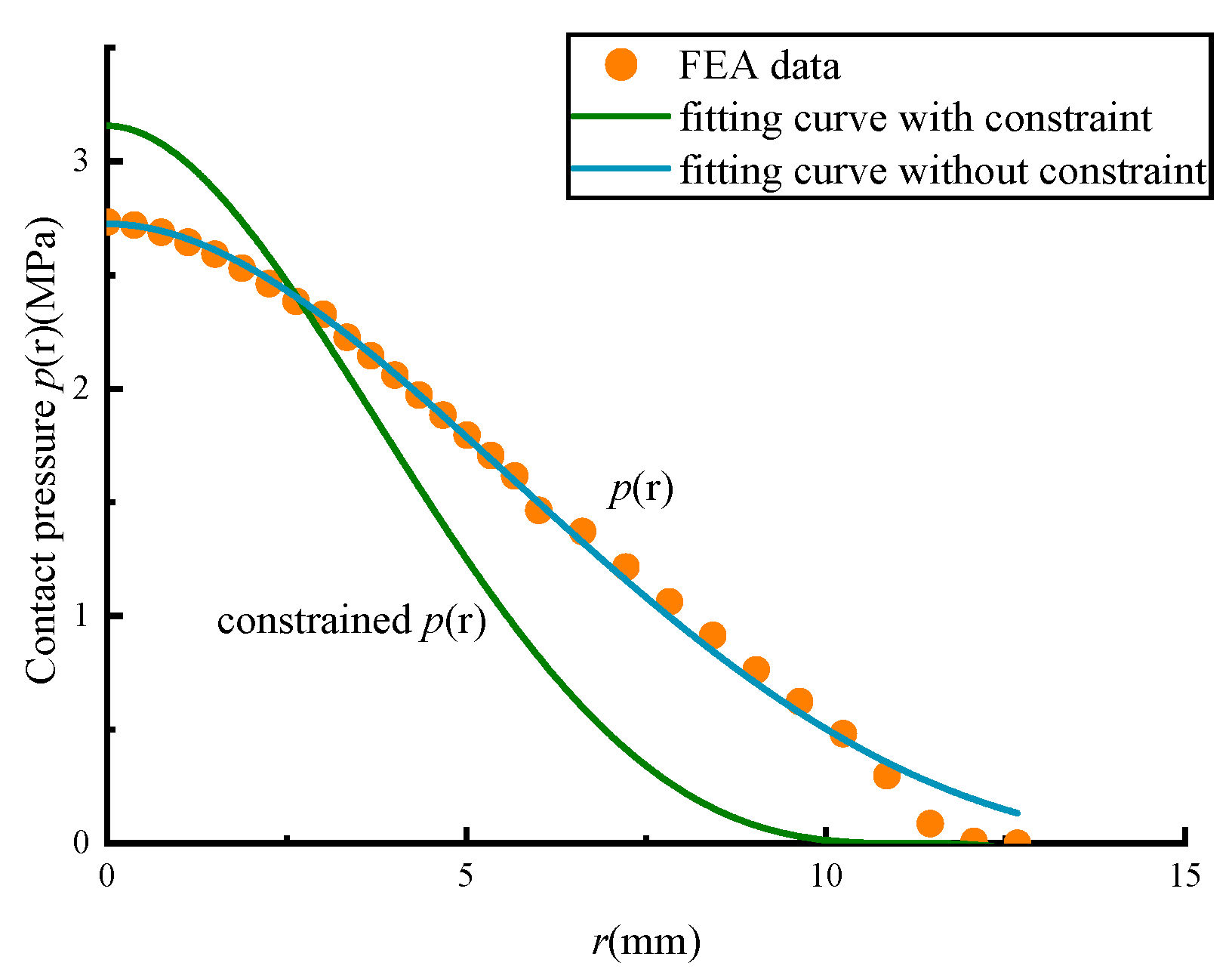
According to
Table 6, the curves of the pre- and post-constrained
are plotted in
Figure 10. The amplitude of the correction is small, and the nonlinearity of the contact pressure is preserved. Because the quadratic gradient of the Fernlund distribution is equal to 0 at the boundary, the reduction rate of pressure at the end is slow, which is an important source of deviation.
After the preload correction, the total normal force is modified to satisfy the preload, as shown in
Table 7. The compensation parameter can be obtained.
The distribution curve before and after the preload correction is shown in
Figure 11, where
is 2.610.
When
, the normal force in the discrete area before and after preload correction can be obtained, as shown in
Table 8, where
is 4.813.
The corrected pressure curves are shown in
Figure 12. The correction amplitude is large, and there is obvious deviation.
For the constraint method, the 3rd discretization requires more compensation of contact pressure. The discrete contact pressure curves of higher orders such as 5th, 8th, 100th and 500th order, under a tangential load of
and
, were calculated. With the increase of
, the amplitude of the second correction decreases obviously, and the correction amplitude is basically fixed when the order increases to 100, as shown in
Figure 13.
5. Discussion
In this paper, dynamic functions of the contact boundary and pressure changing with tangential force have been proposed and verified. The finite element calculation results show that the contact boundary function and the pressure function can well characterize the dynamic characteristics of pressure distribution. However, the elliptical boundary causes the loss of preload which needs to be compensated. Besides, the consistency of the two functions can not be reached due to the different parameter identification, which blocks the solution of the DF of the Iwan model. Therefore, two correction methods are proposed to solve the inconsistency and preload loss. The translation method is to modify the functions obtained by the independent parameter identification. The constraint method is to add the parameter constraints of the boundary function in the parameter identification of the pressure distribution function. Both can solve the inconsistency, and with the increase of the discrete order, the correction results of the two methods tend to be stable. For example, the corrected distribution function of the 100th-order discrete and the 1000th-order discrete are similar, which means that the discrete solution method is a good way to reduce the complexity of solving the dynamic Iwan model.
Through the analysis of the two correction methods, the nonlinear pressure distribution of the constraint method is constrained by the gradient of 0 at both ends, causing the partial preload around the contact boundary to be very low. Therefore, more preload needs to be compensated in the constraint method. The constraint method is more suitable for solving the complex dynamic Iwan model, considering the more concise correction process and the retained nonlinear distribution characteristics of the constraint method.