Numerical Simulation of Heat Mass Transfer Effects on MHD Flow of Williamson Nanofluid by a Stretching Surface with Thermal Conductivity and Variable Thickness
Abstract
1. Introduction
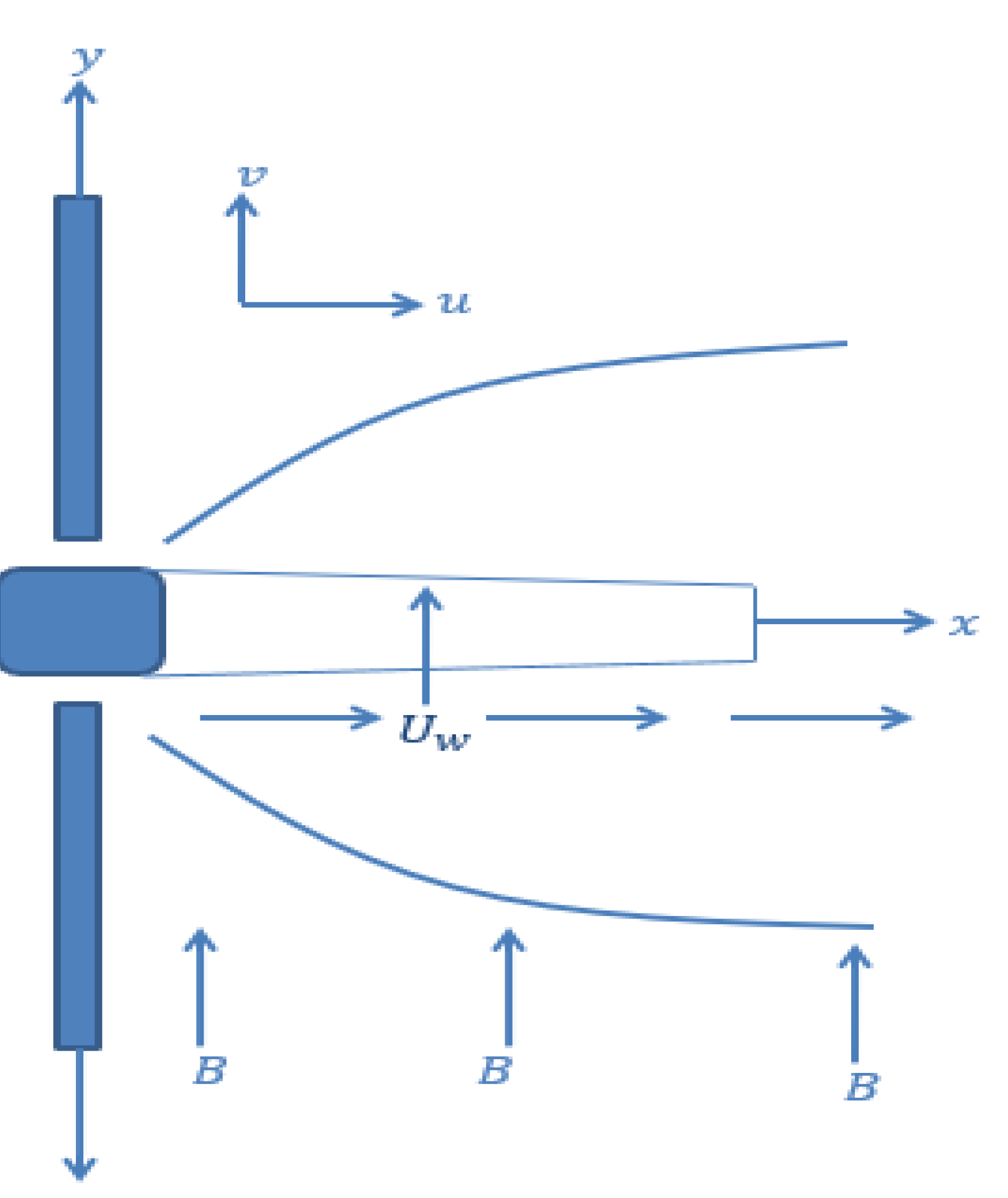
2. Mathematical formulation
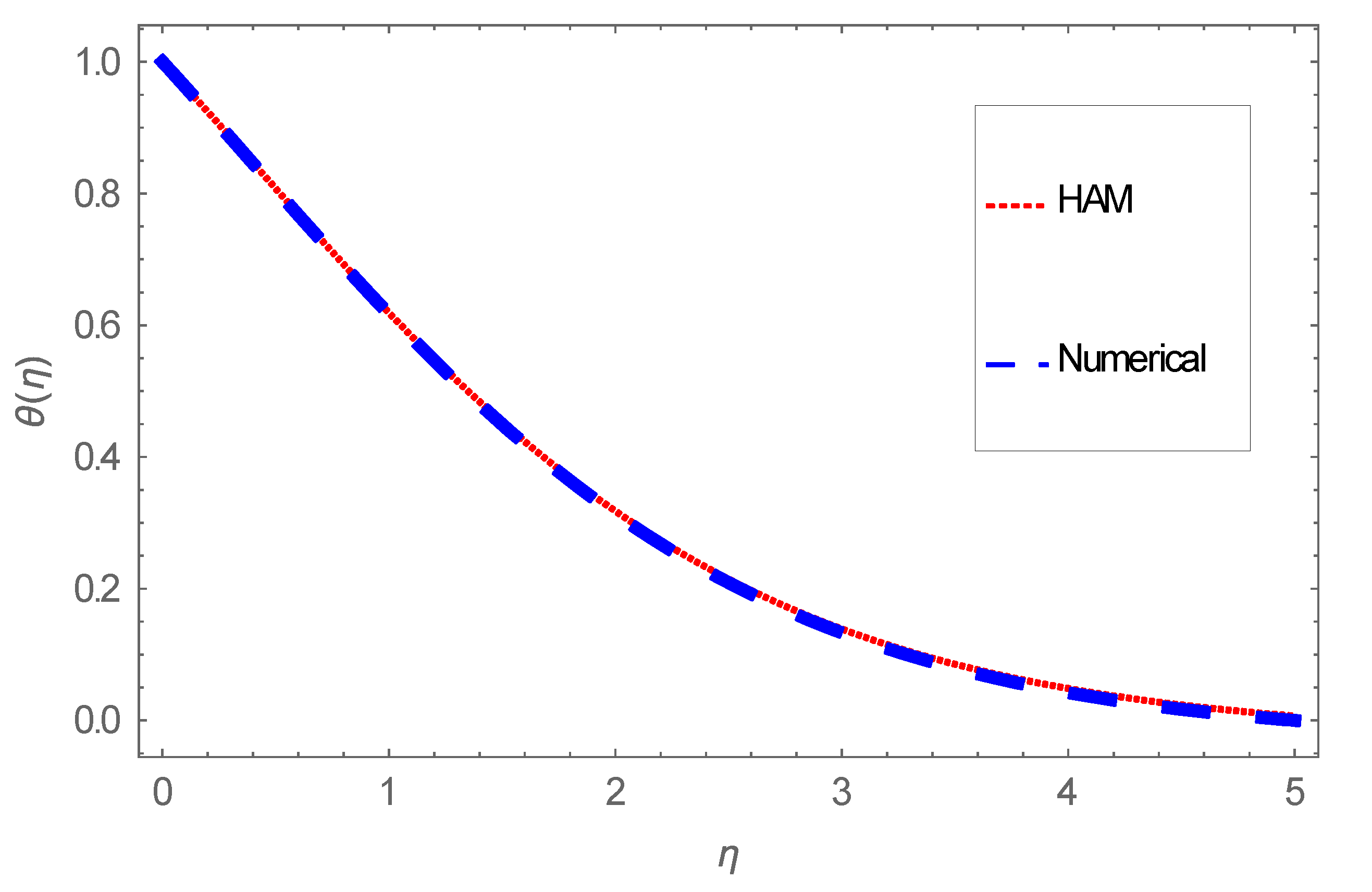
3. Numerical Scheme
4. Discussion
5. Closing Remarks
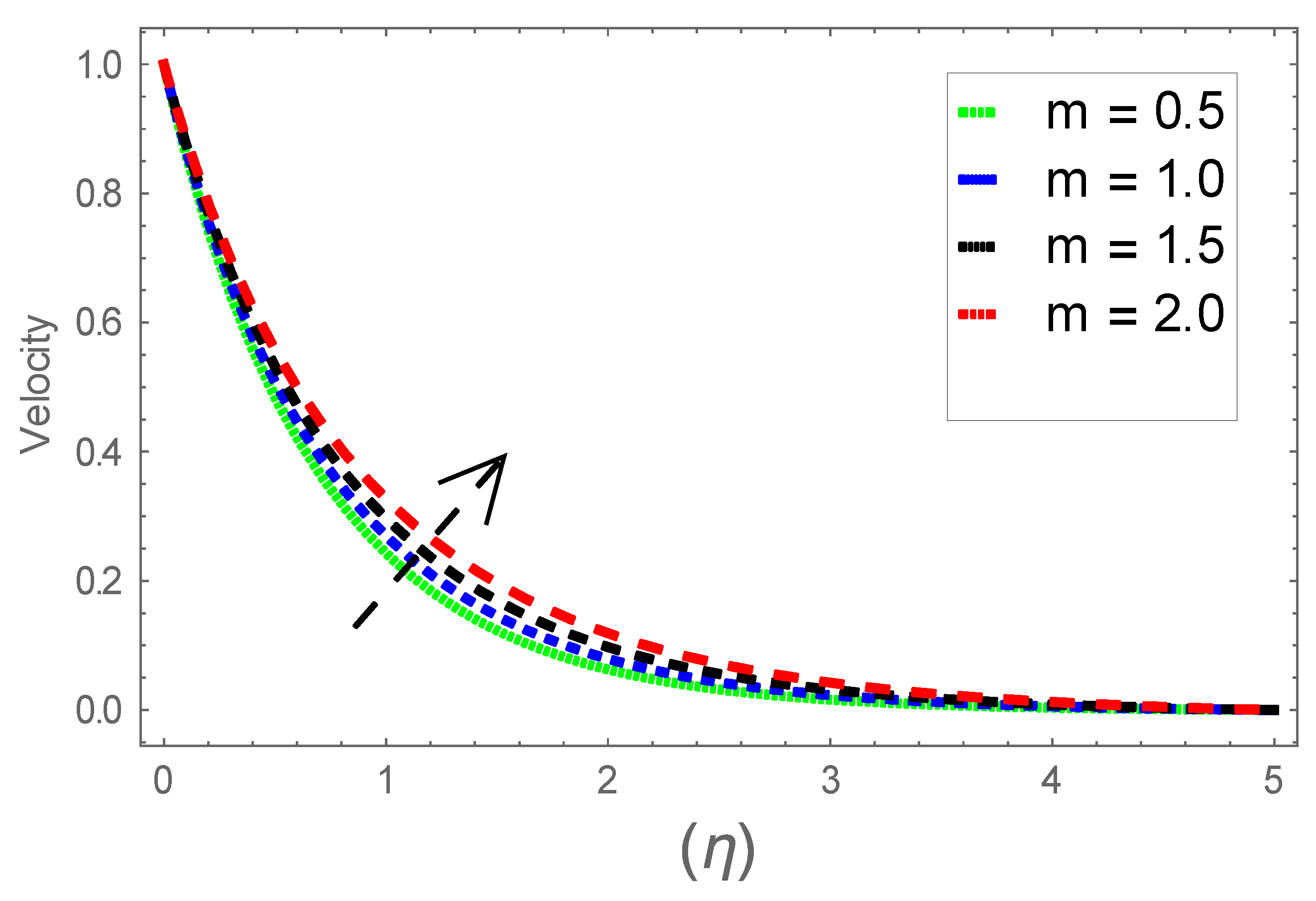
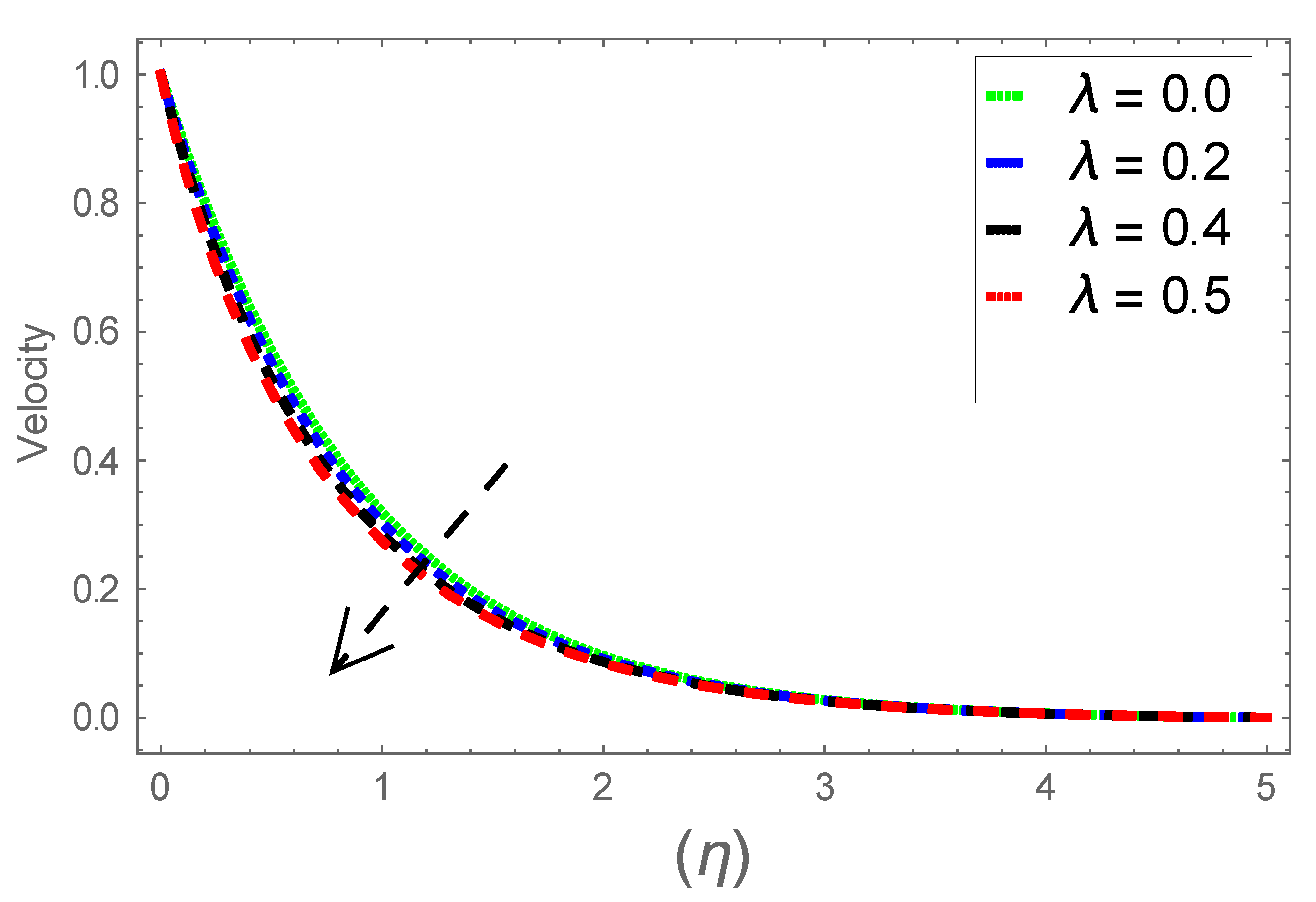
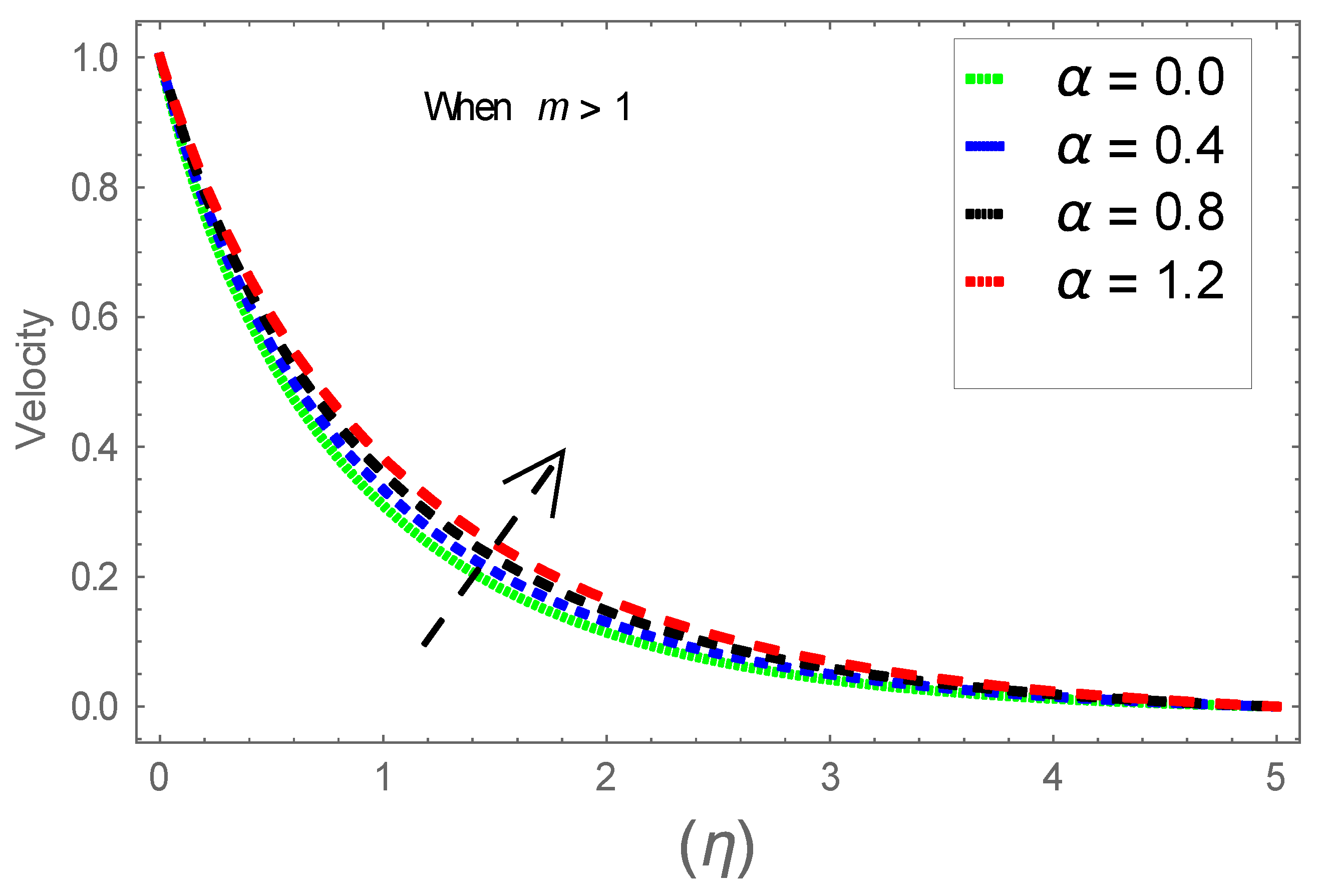
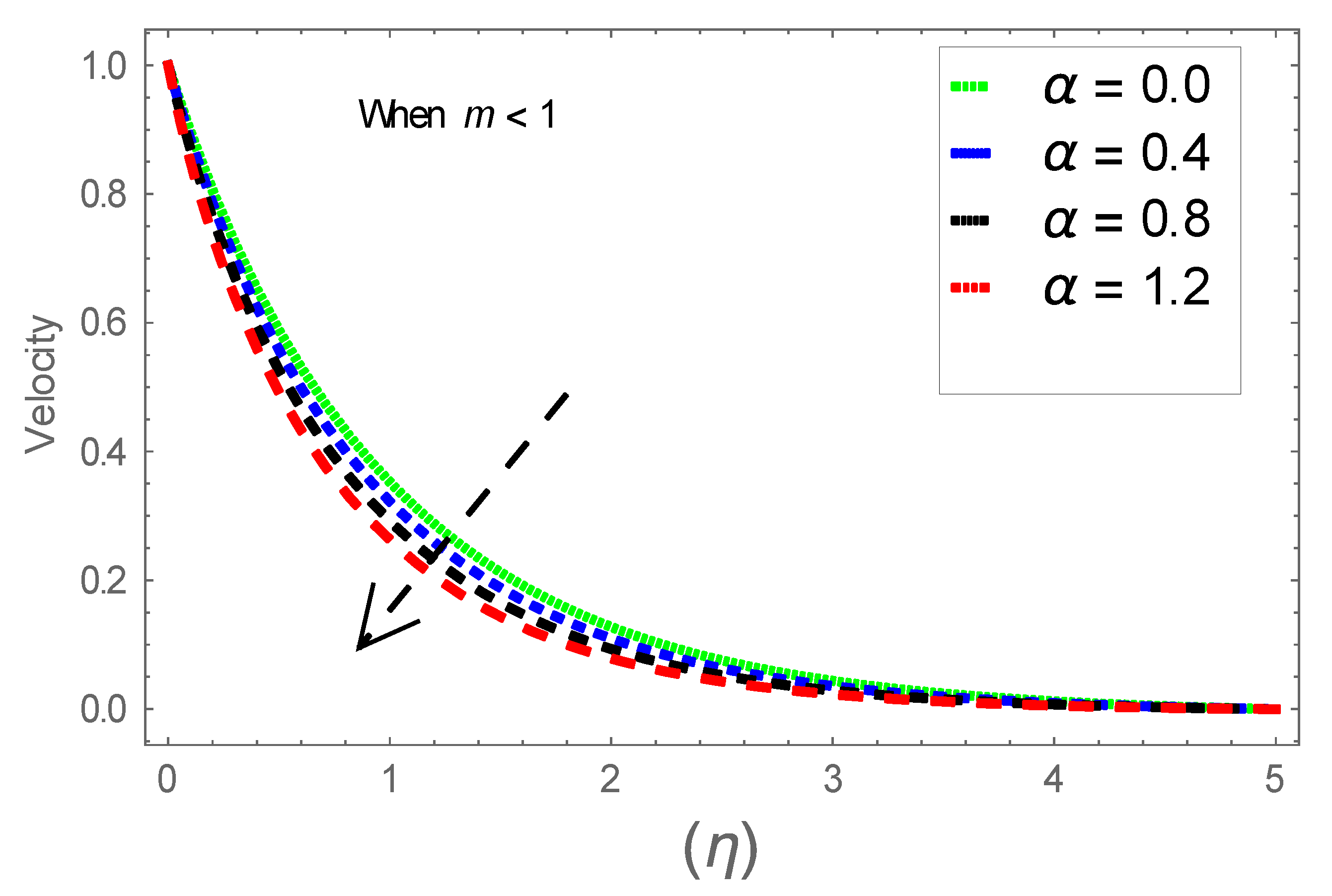
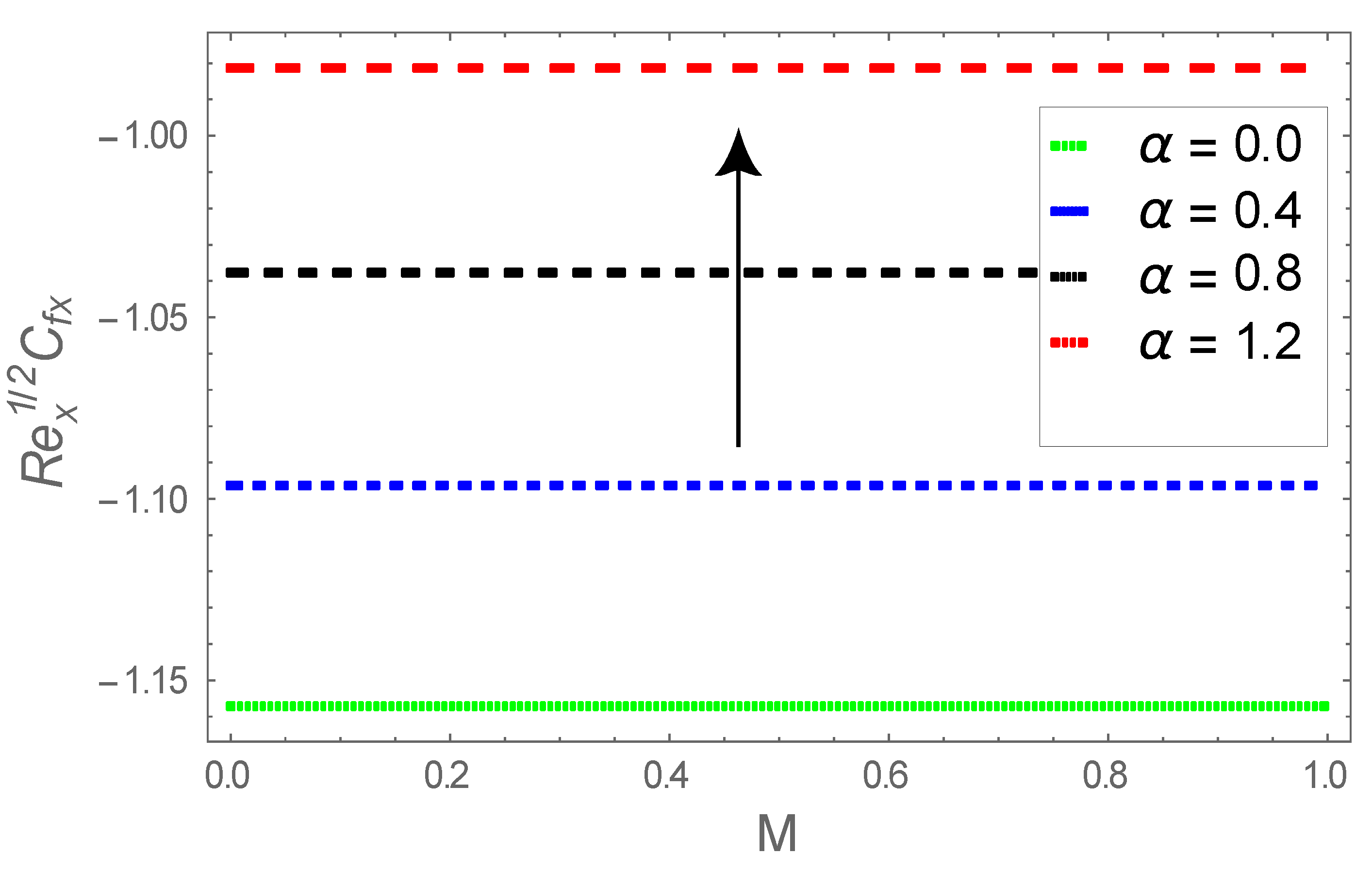
- It is perceived that velocity field diminishes when wall thickness factor augments for and reverse trends noticed in when .
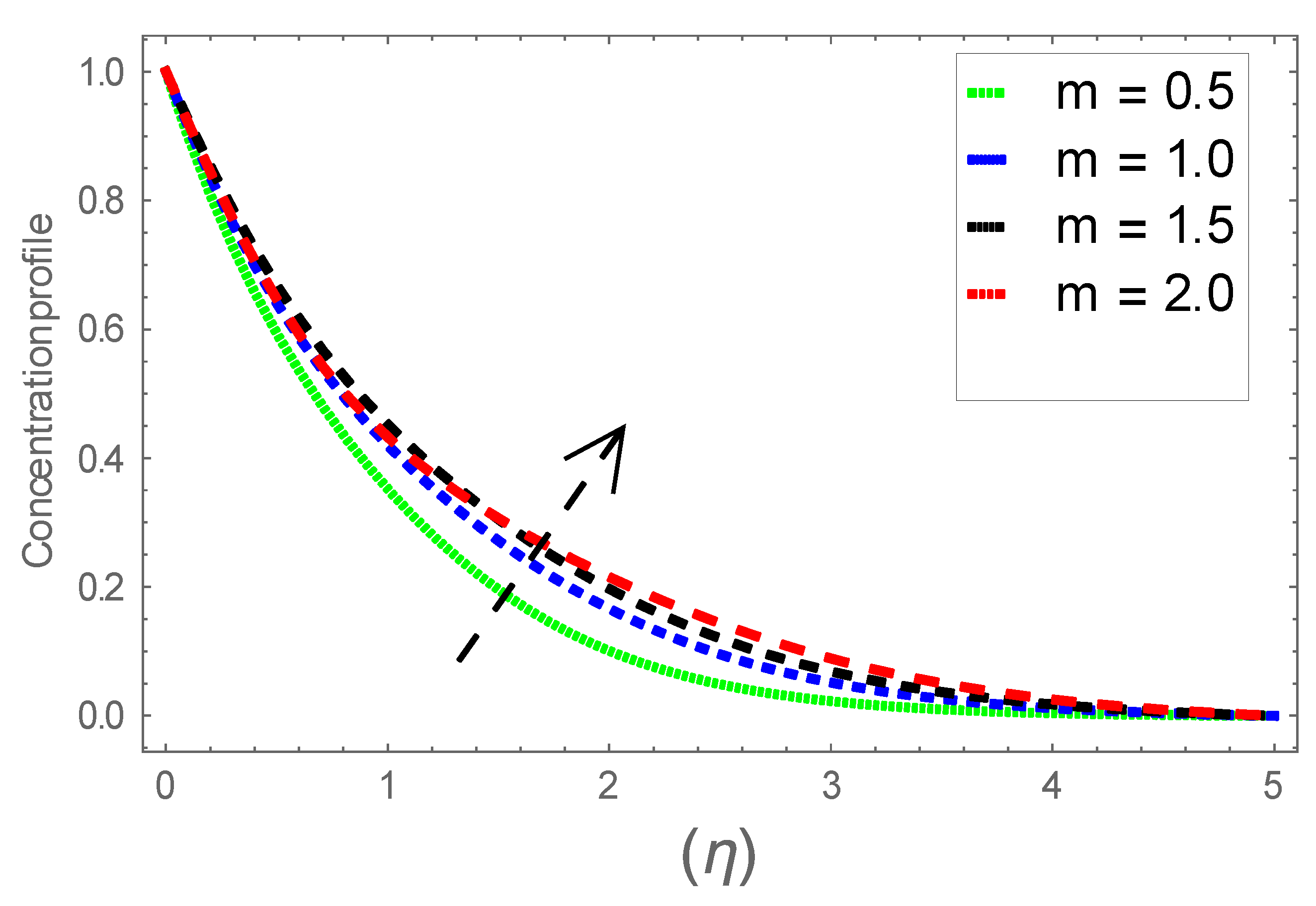
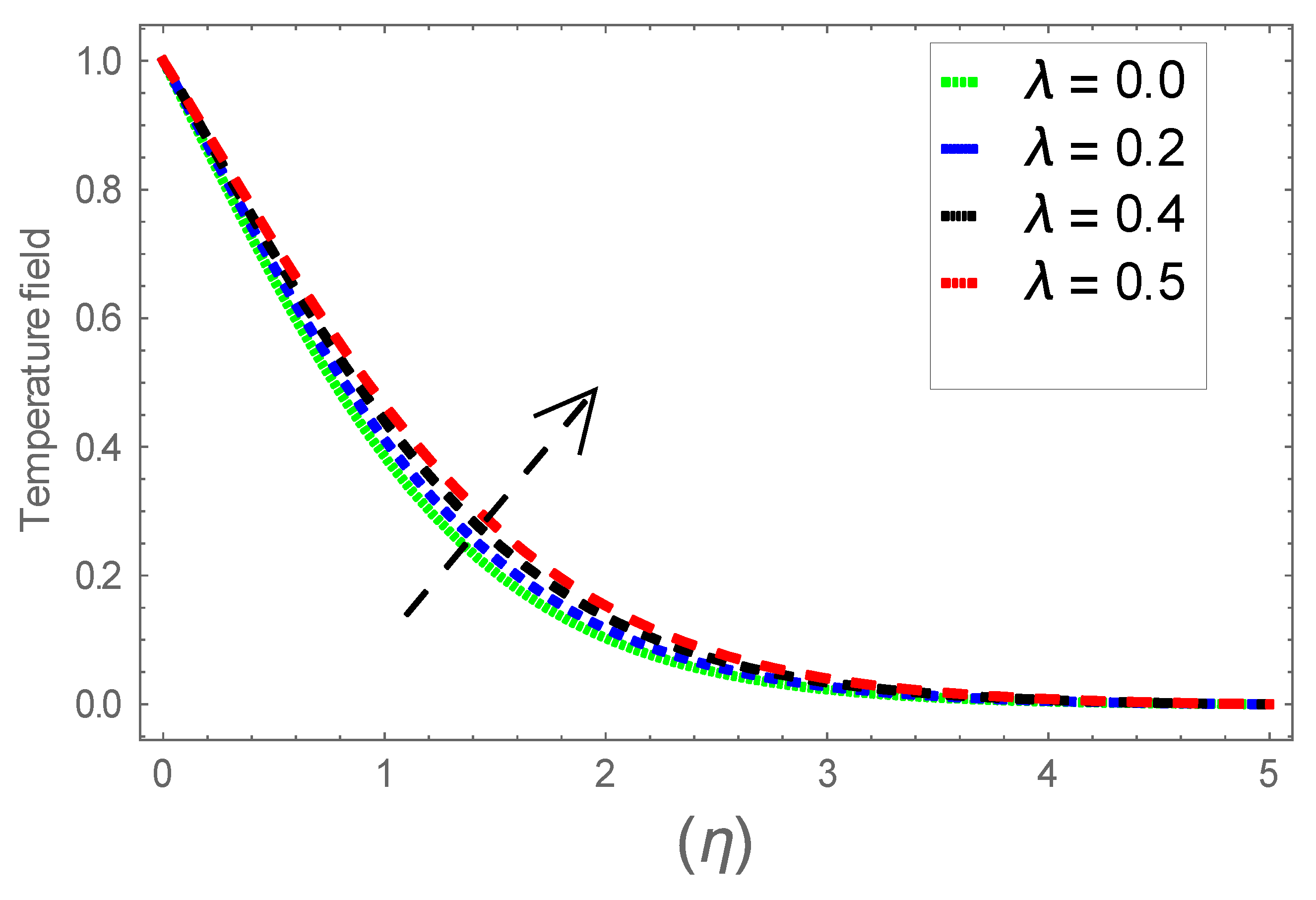
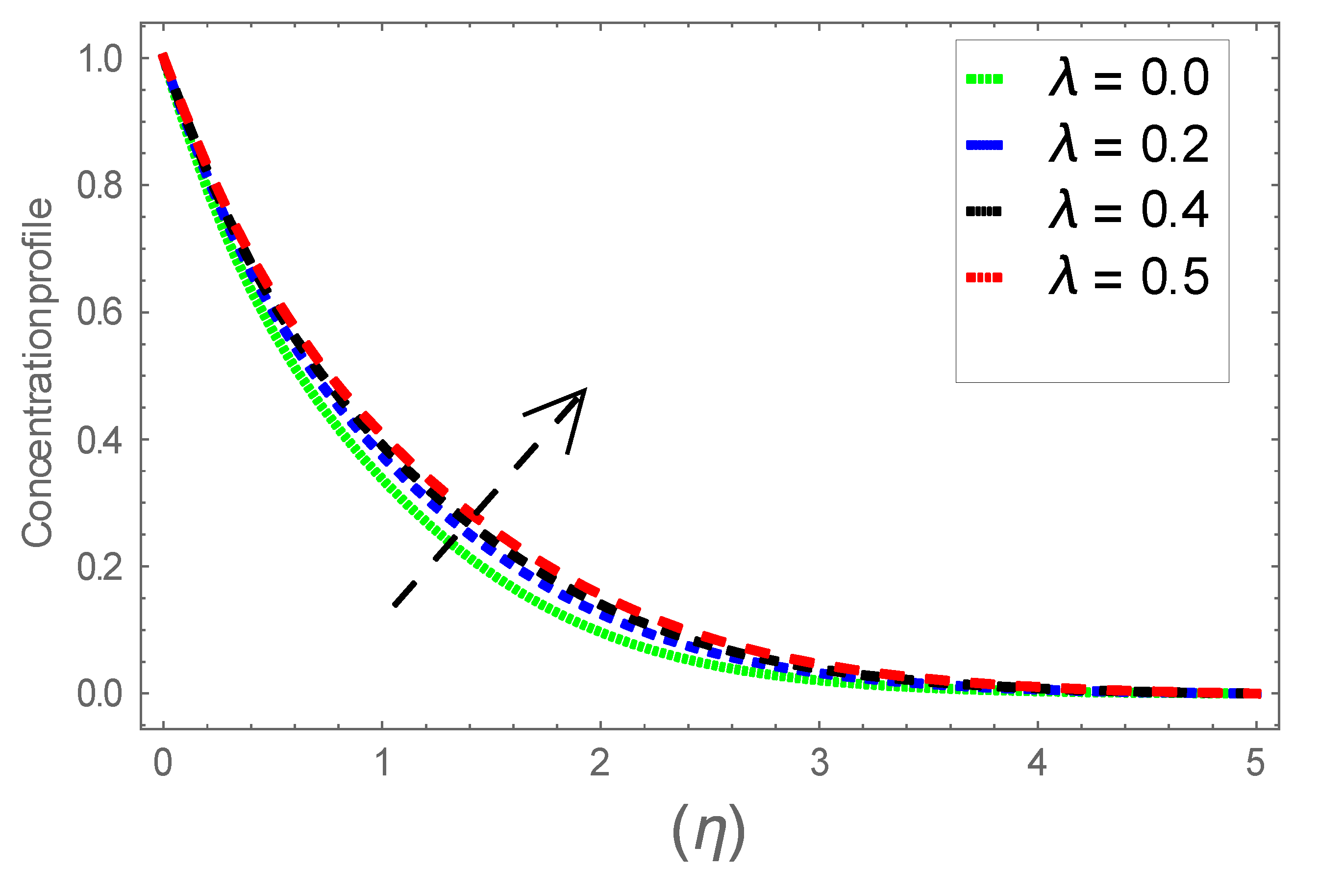
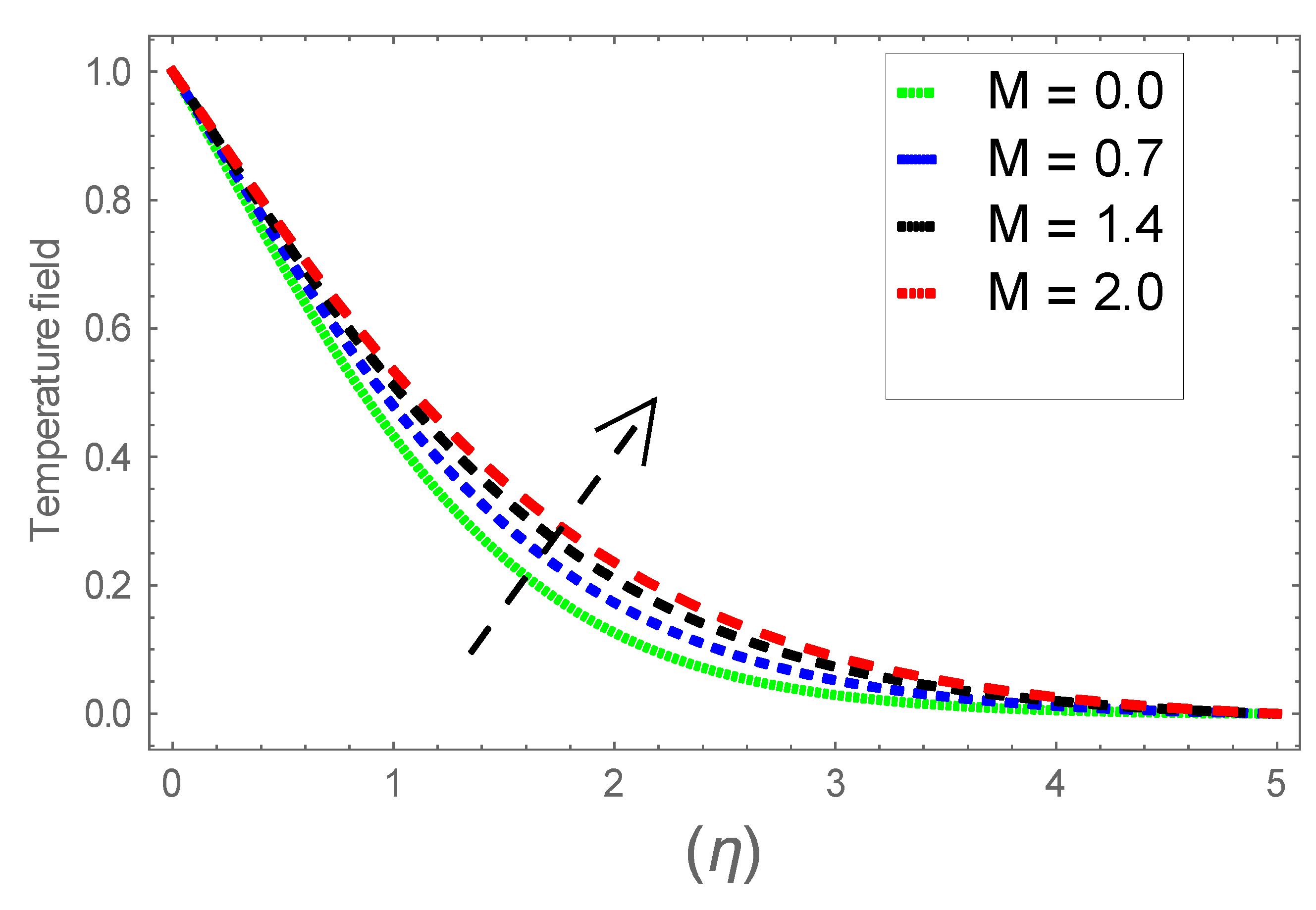
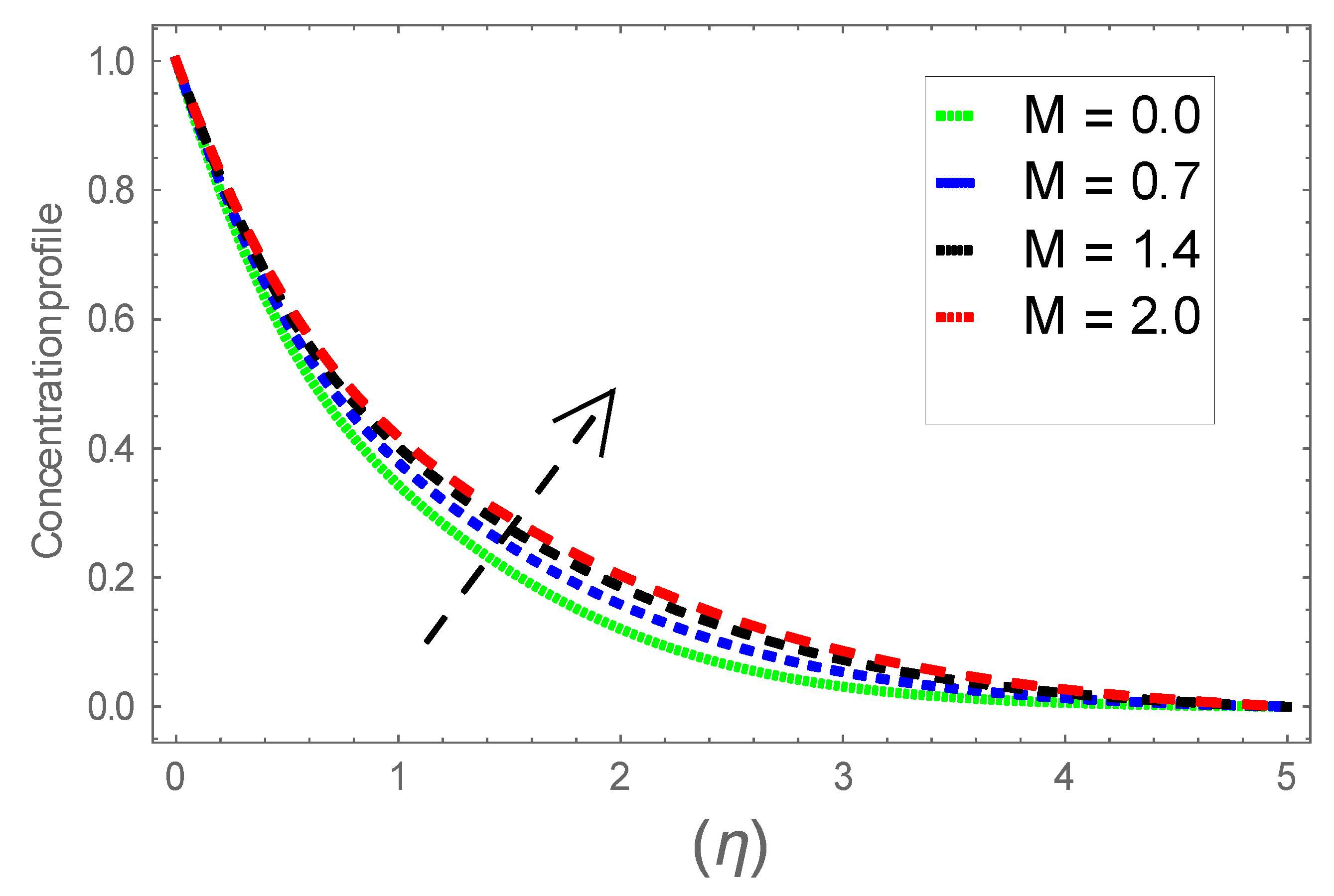
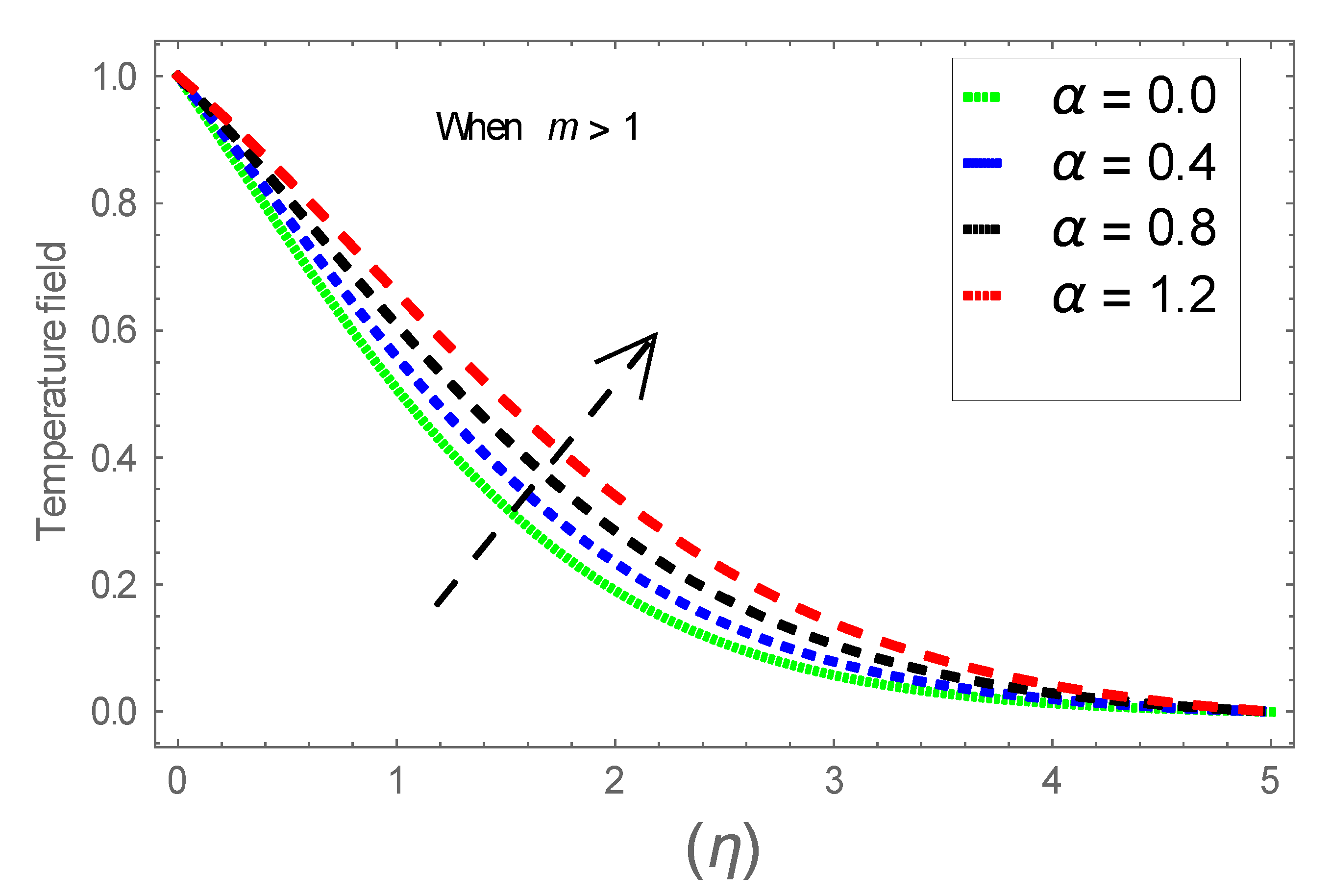
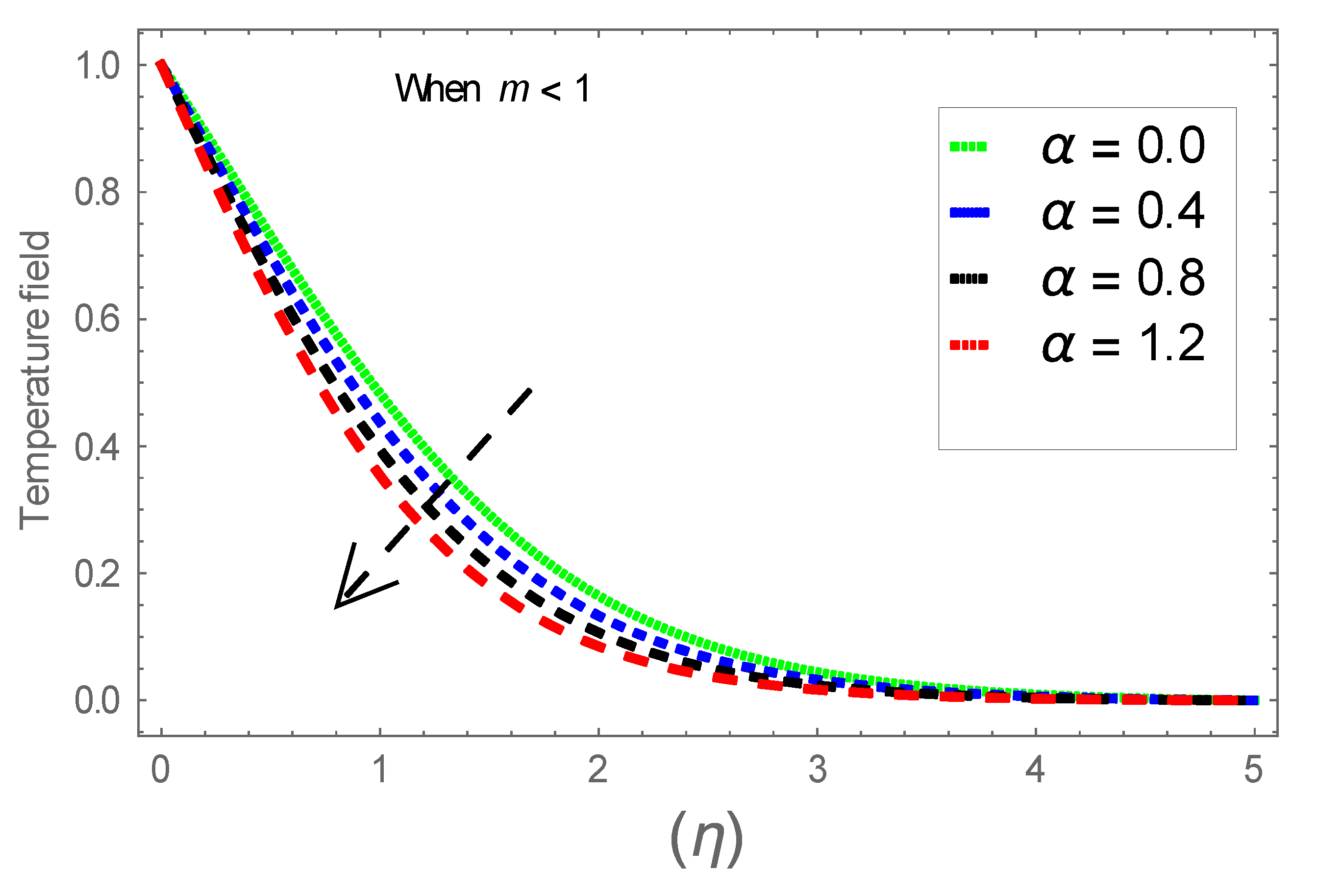
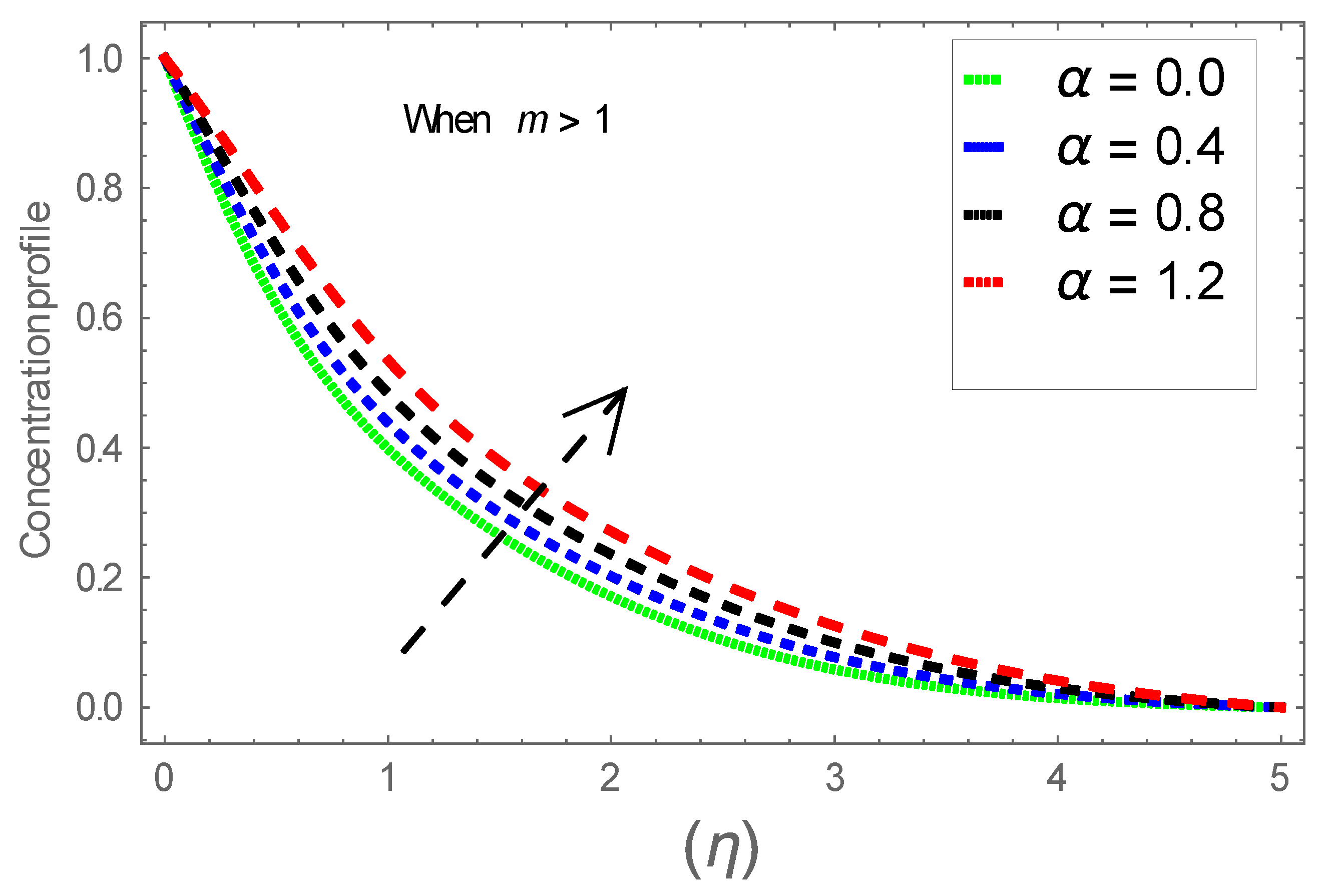
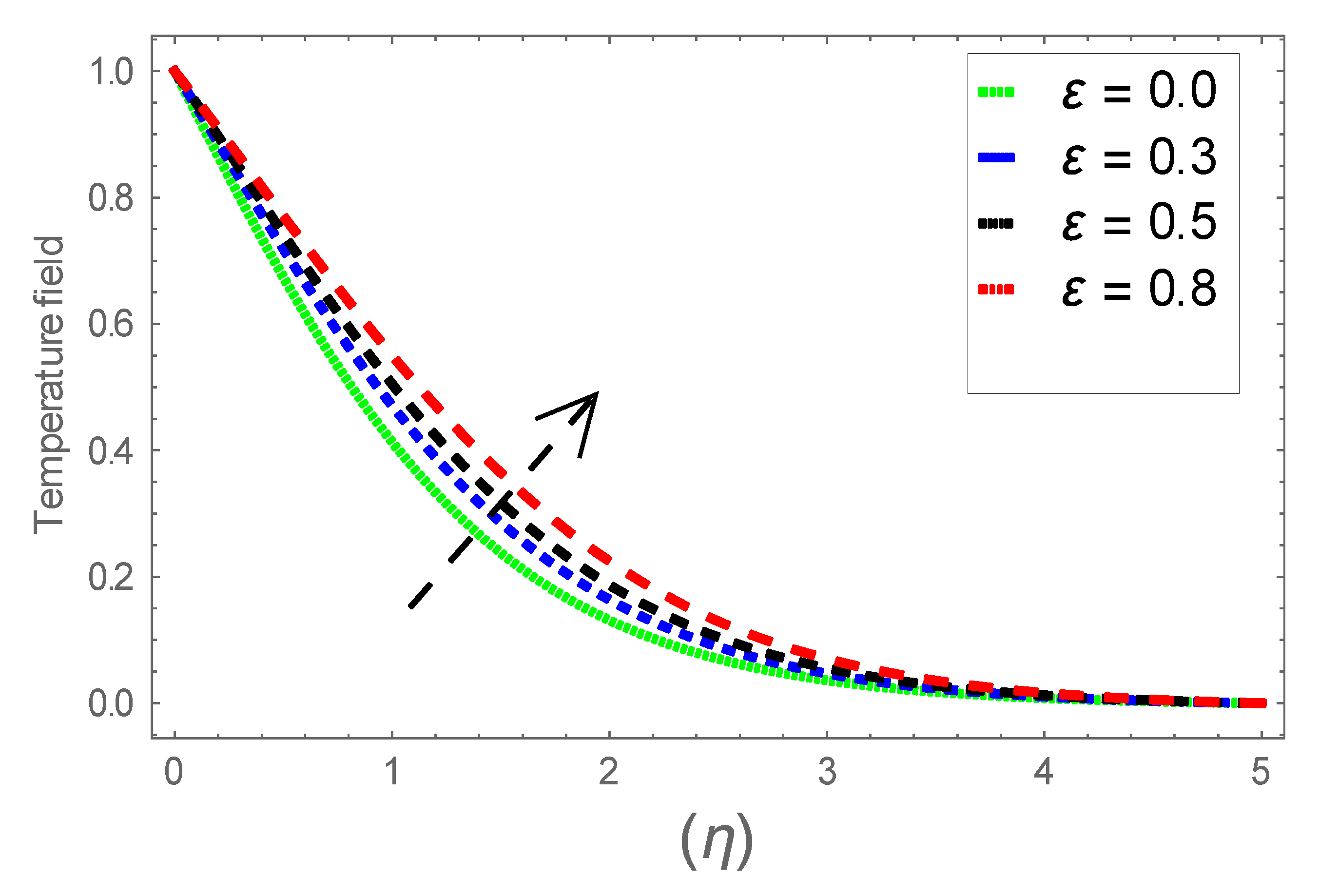
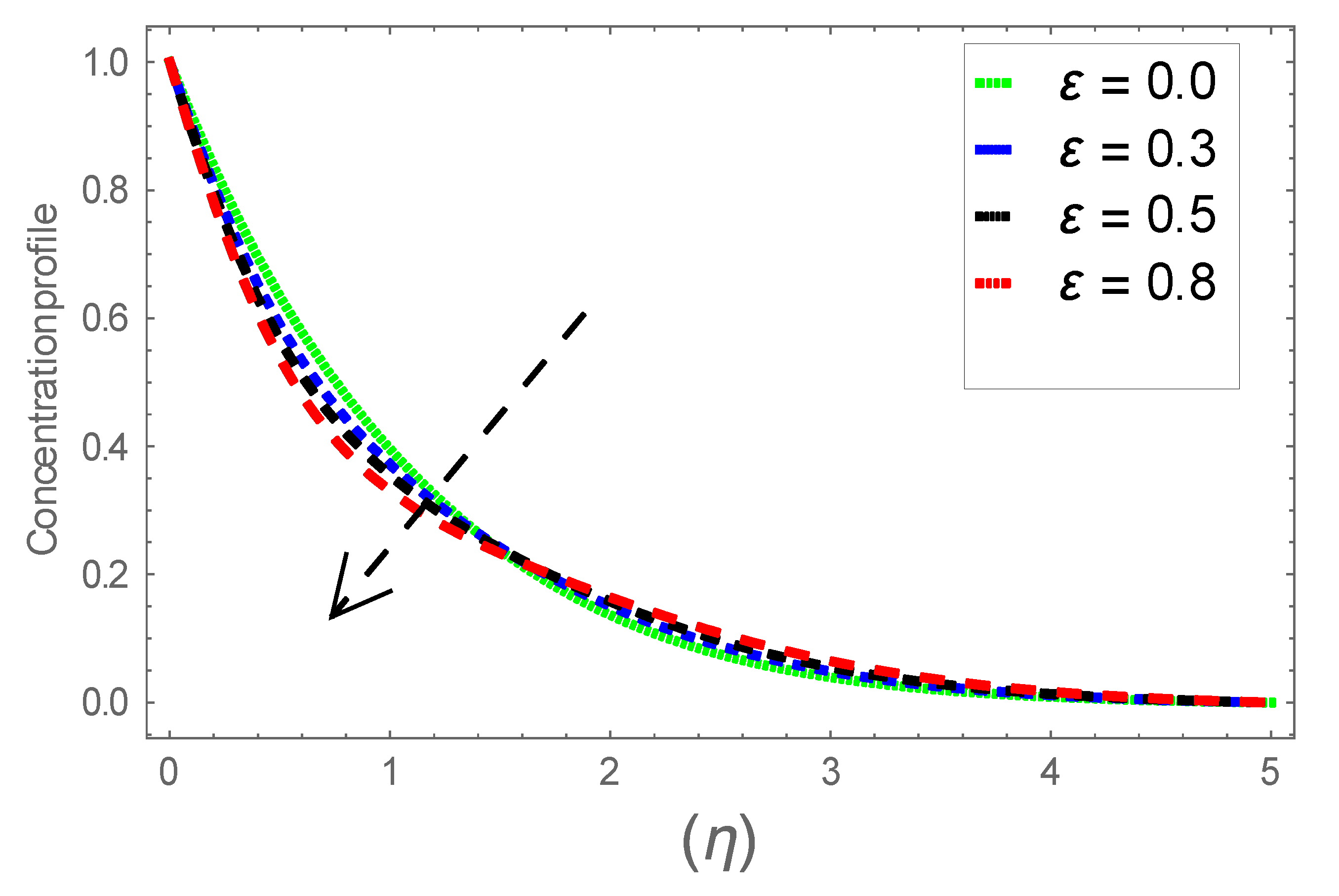
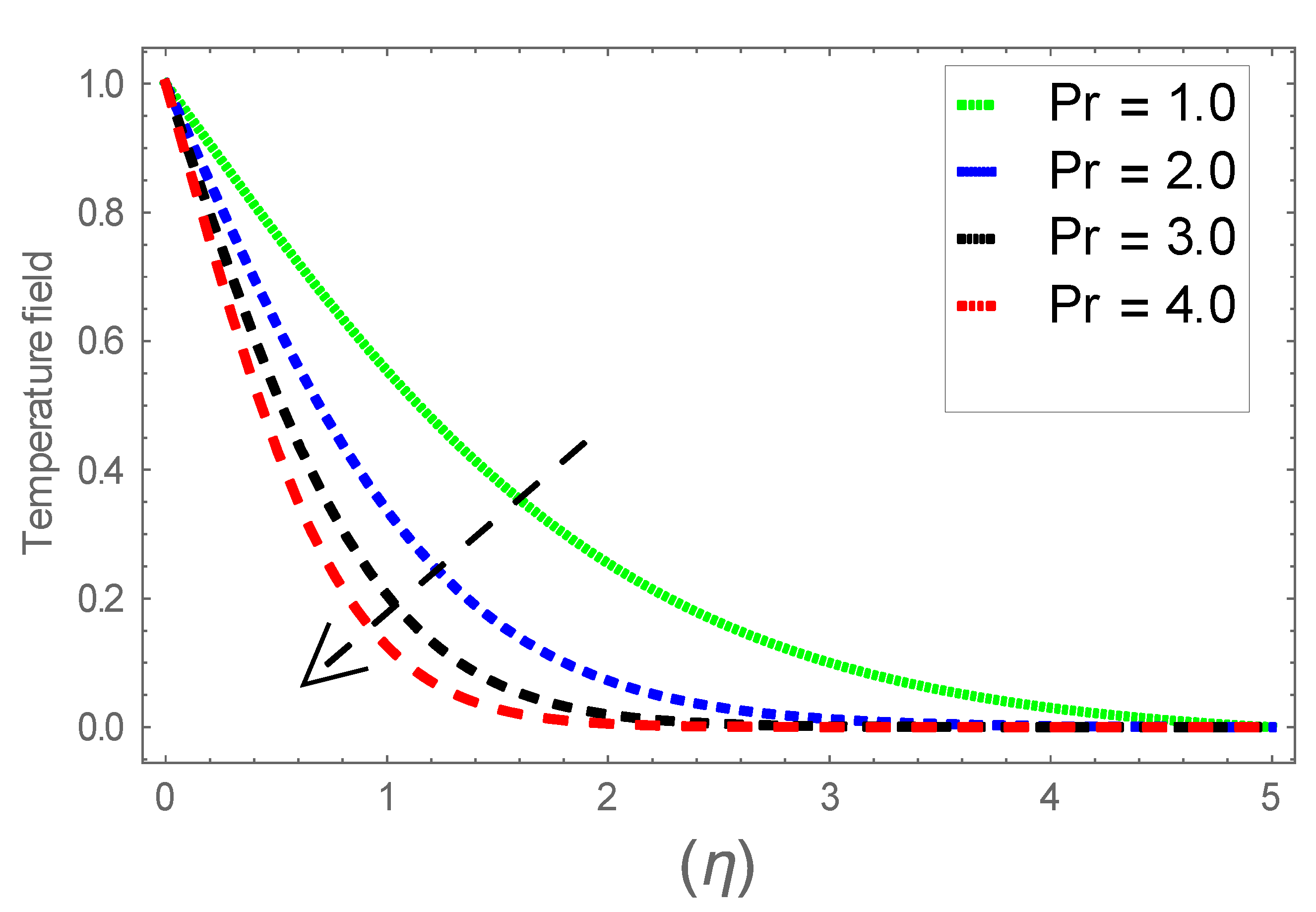
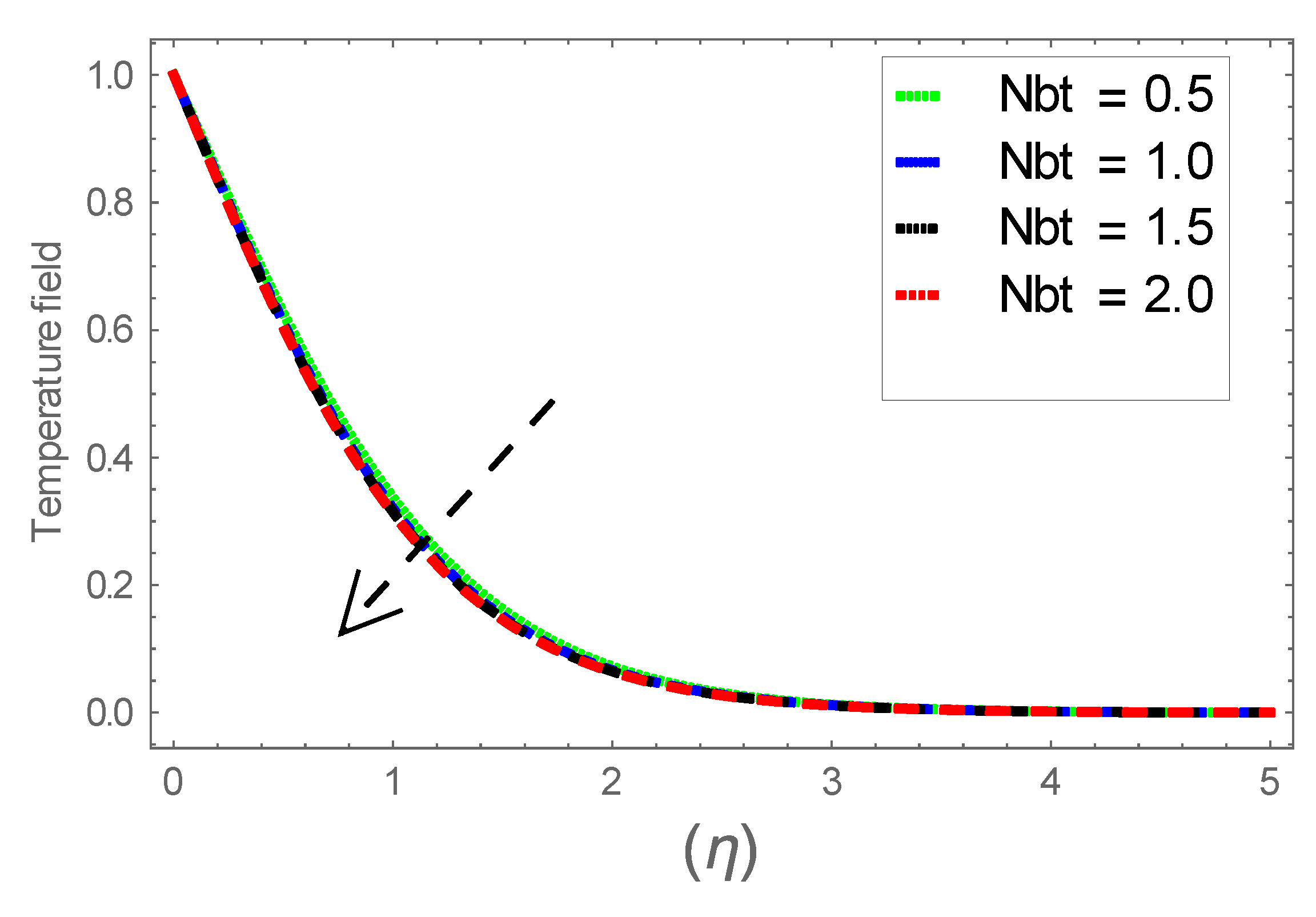
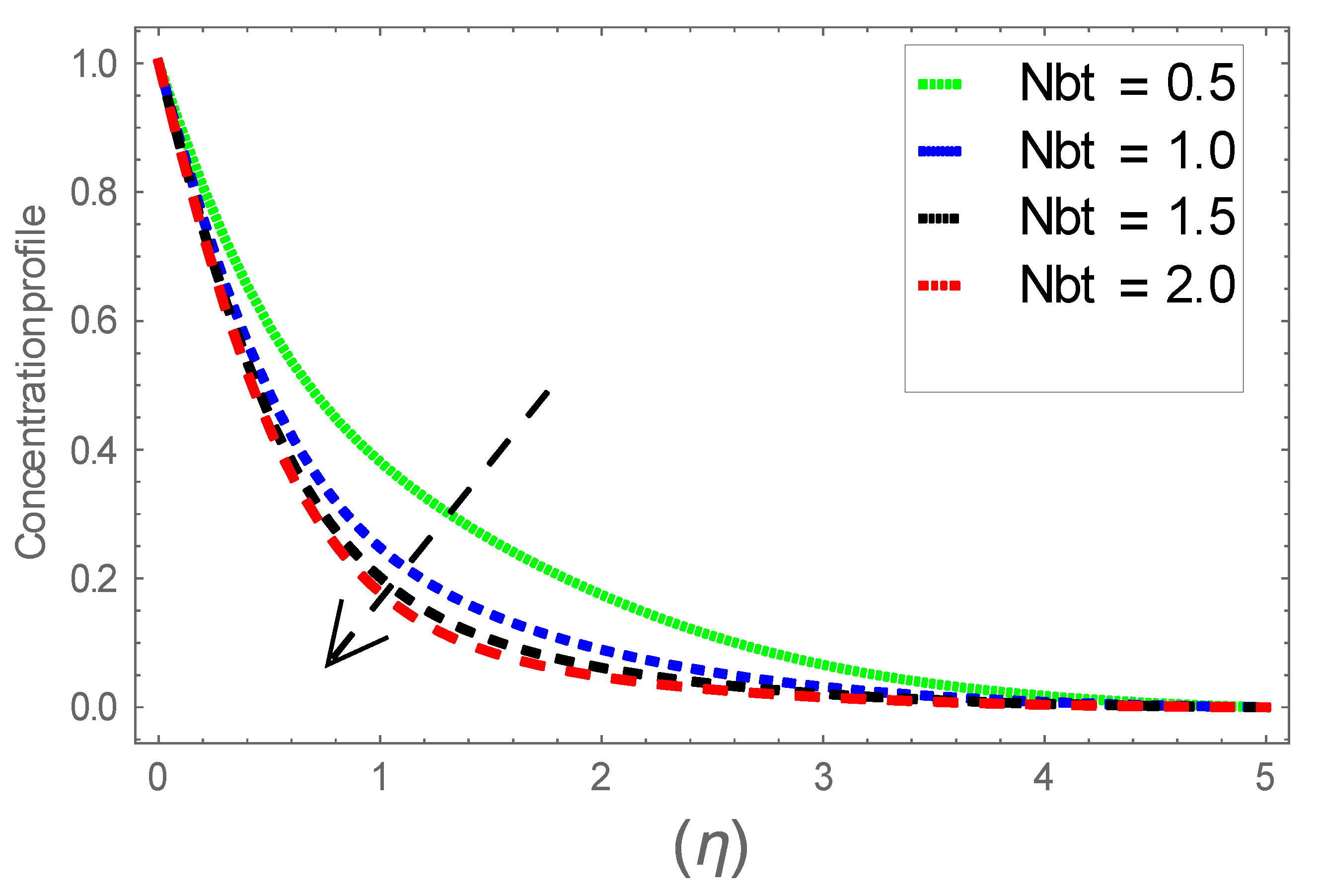
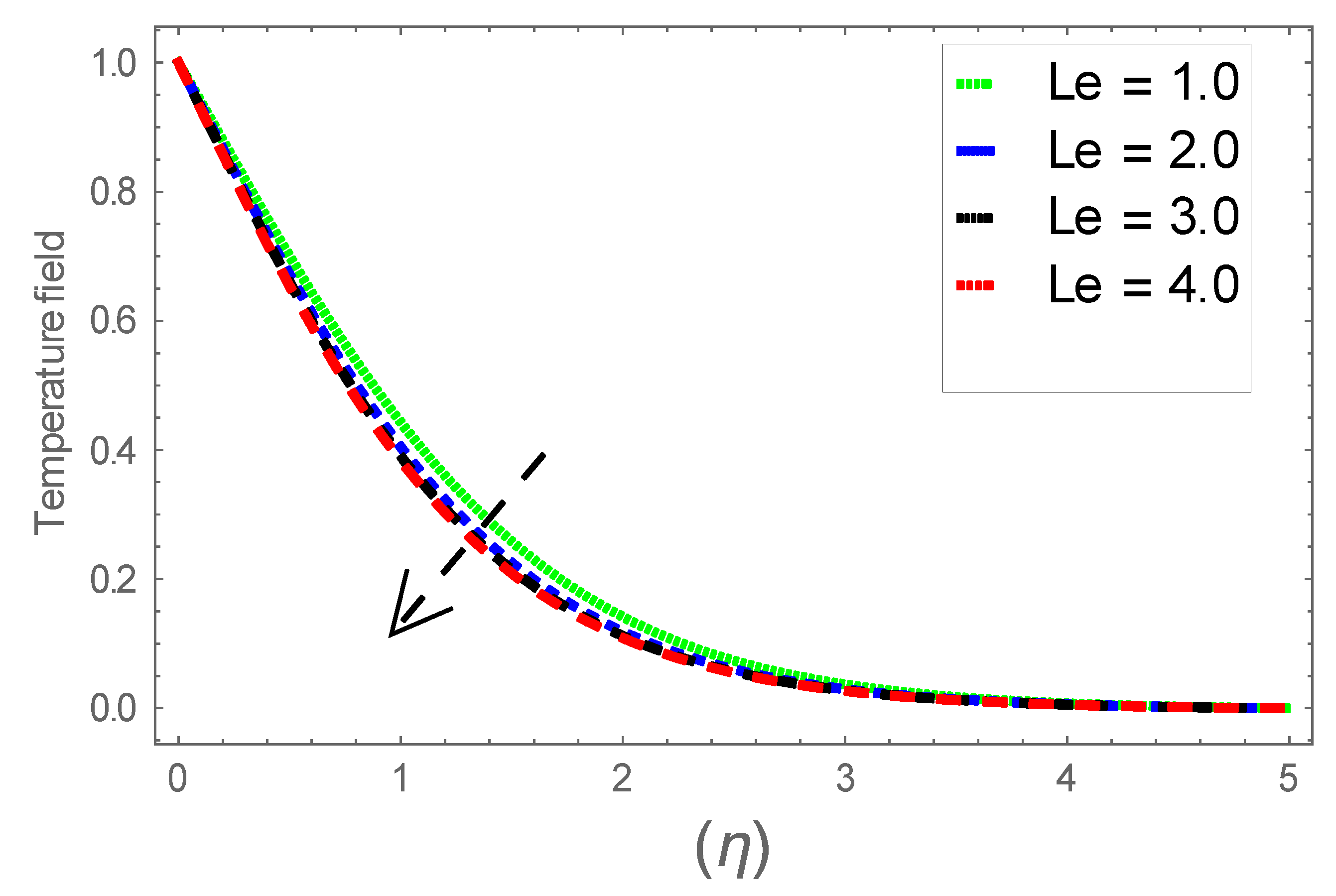
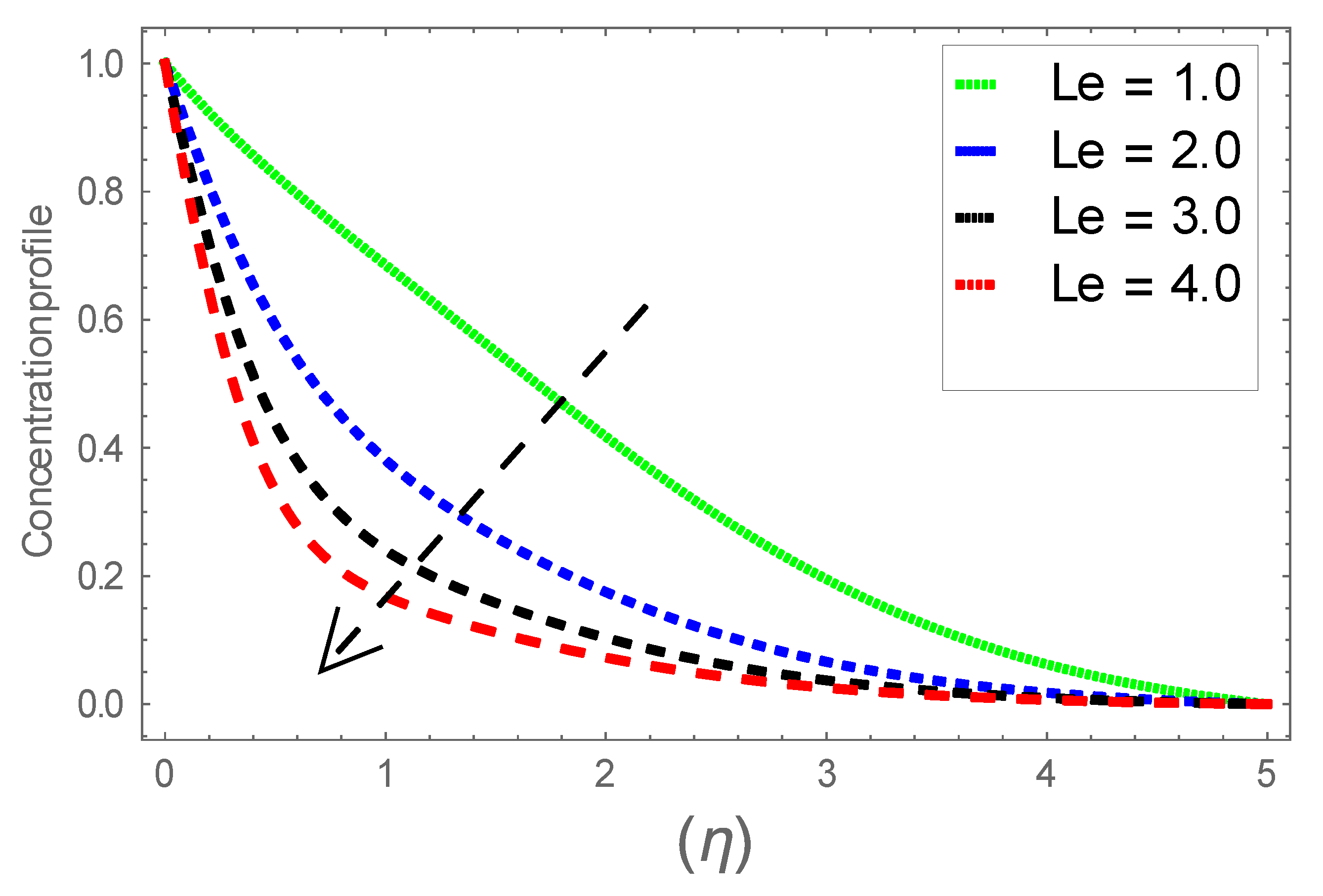
- Thermal and solutal fields upsurge subject to increment in and diminishes with higher .
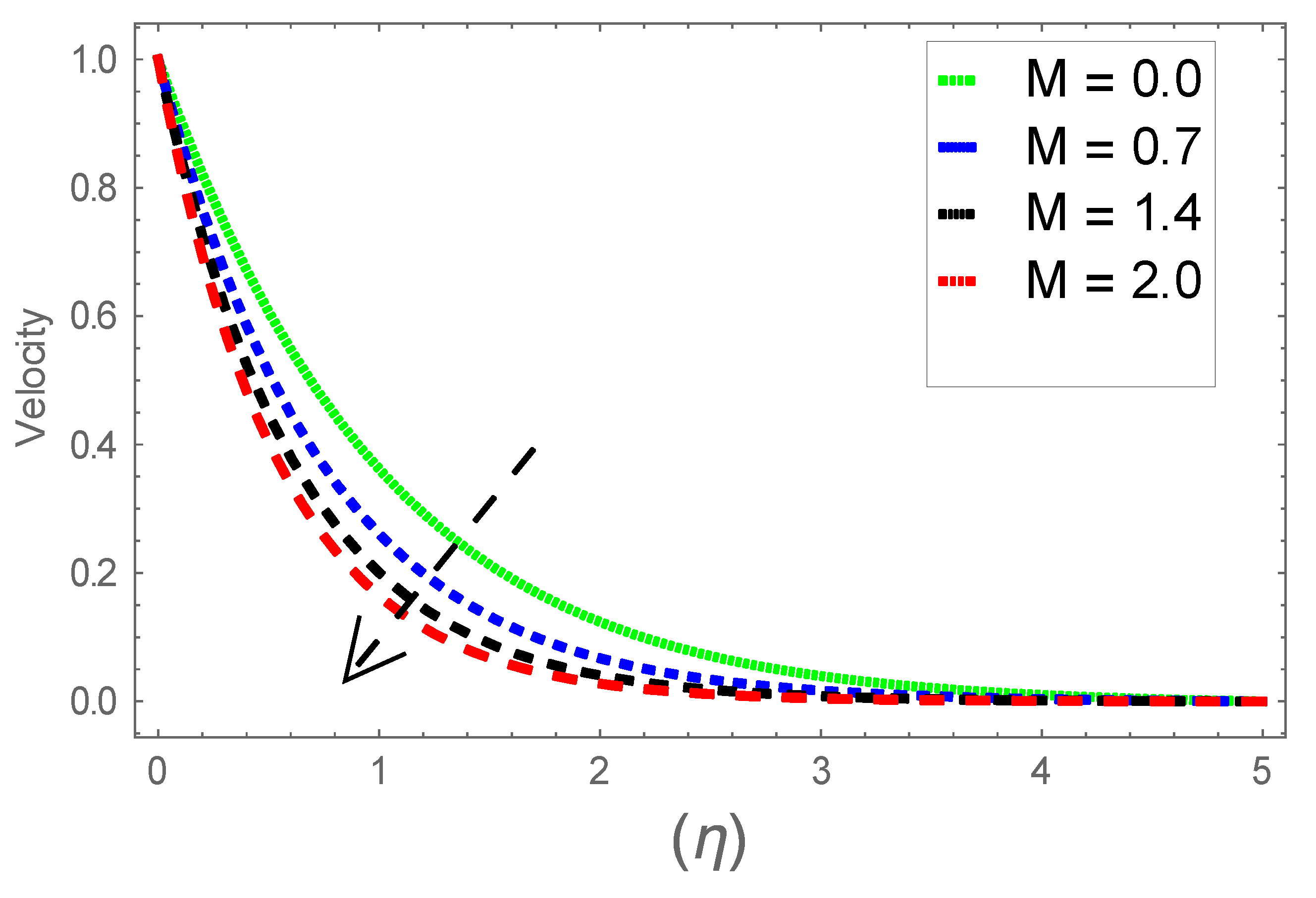
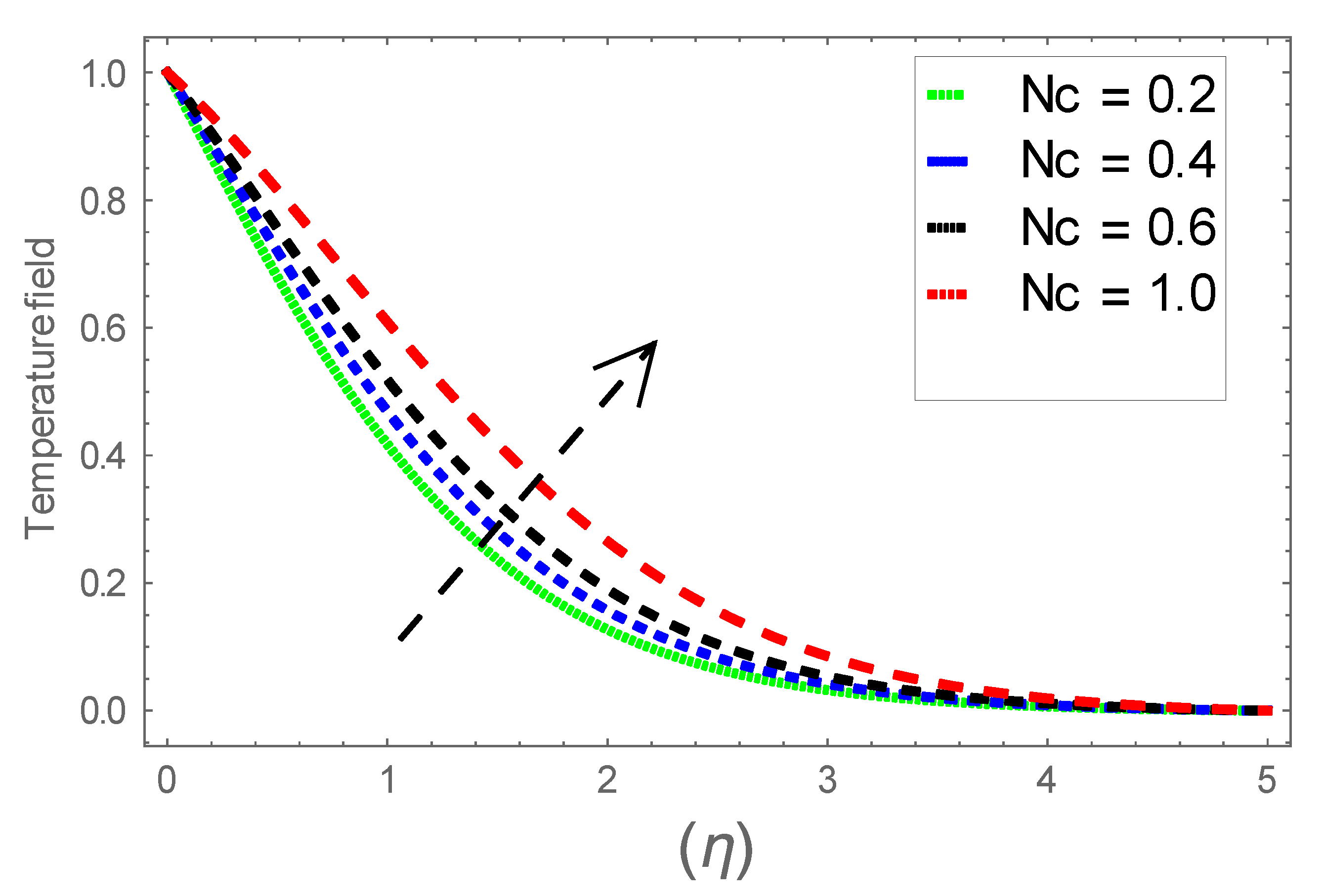
- An increment in parameter yields decays in and escalates thermal and concentration fields.
- Variable thermal conductivity parameter diminishes heat transfer coefficient .
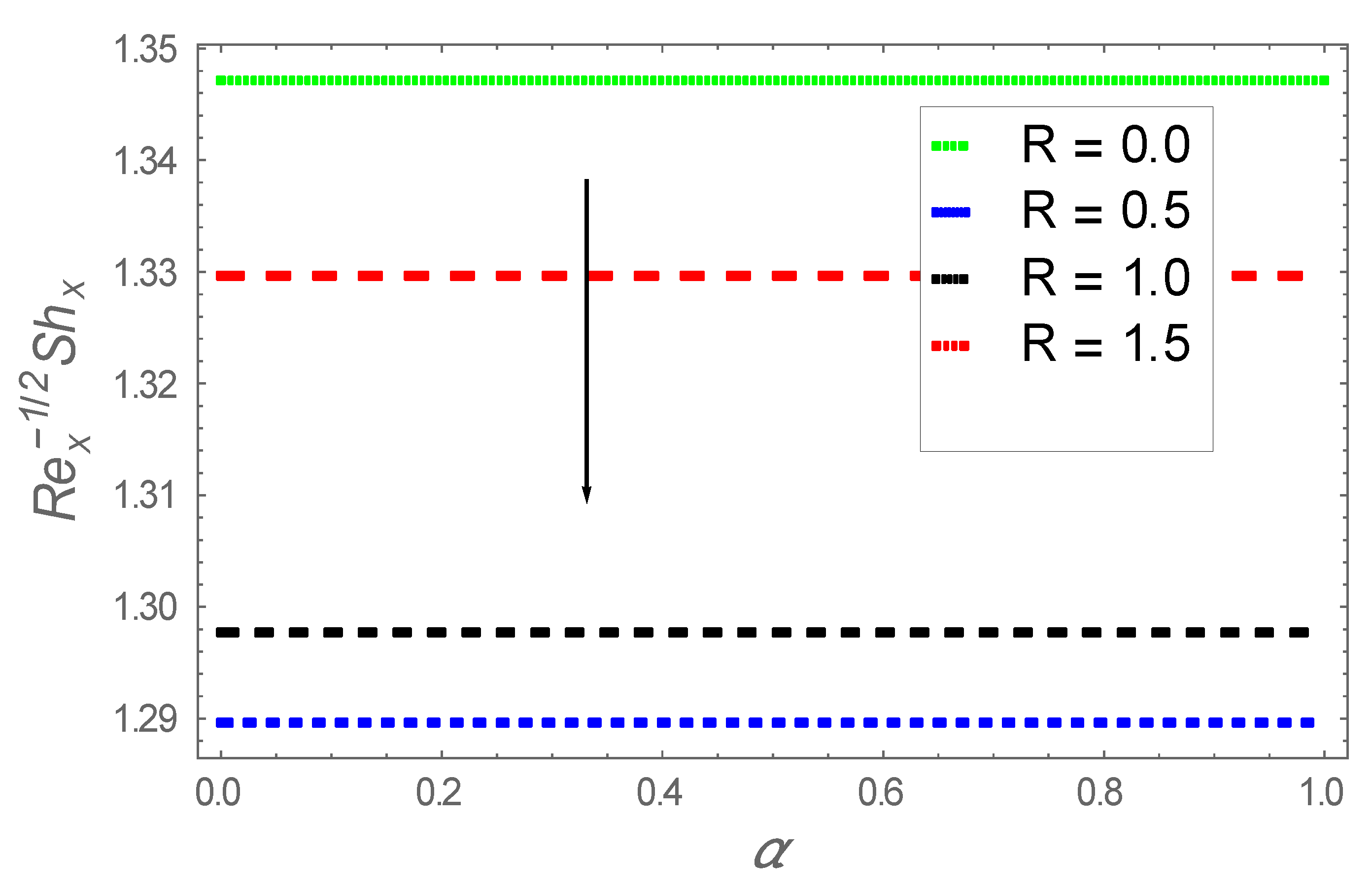
- Sherwood number diminishes subject to increment in .
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Choi, S.U.S.; Eastman, J. Enhancing thermal conductivity of fluids with nanoparticle. Master Sci. 1995, 231, 99–105. [Google Scholar]
- Buongiorno, J. Convective transport in nanofluids. ASME J. Heat Transf. 2006, 128, 240–250. [Google Scholar] [CrossRef]
- Daungthongsuk, W.; Wongwises, S.A. Critical review of convective heat transfer nanofluids. Renew. Sustain. Eng. Rev. 2007, 11, 797–817. [Google Scholar] [CrossRef]
- Wang, X.Q.; Mujumdar, A.S. A review on nanofluids part I: Theoretical and numerical investigations. Braz. J. Chem. Eng. 2008, 25, 613–630. [Google Scholar] [CrossRef]
- Wang, X.Q.; Mujumdar, A.S. A review on nanofluids part II: Experiments and applications. Braz. J. Chem. Eng. 2008, 25, 631–648. [Google Scholar] [CrossRef]
- Kakac, S.; Pramuanjaroenkij, A. Review of convective heat transfer enhancement with nanofluids. Int. J. Heat Mass Transf. 2009, 52, 3187–3196. [Google Scholar] [CrossRef]
- Ibrahim, W.; Gamachu, D. Nonlinear convection flow of Williamson nanofluid past a radially stretching surface. AIP Adv. 2019, 9. [Google Scholar] [CrossRef]
- Ibrahim, W.; Negera, M. Viscous dissipation effect on Williamson nanofluid over stretching/shrinking wedge with thermal radiation and chemical reaction. J. Phys. Commun. 2020, 4, 045015. [Google Scholar] [CrossRef]
- Ibrahim, W.; Negera, M. The investigation of MHD Williamson nanofluid over stretching cylinder with the effect of activation energy. Adv. Math. Phys. 2020, 2020, 9523630. [Google Scholar] [CrossRef]
- Yusuf, T.A.; Adesanya, S.O.; Gbadeyan, J.A. Entropy generation in MHD Williamson nanofluid over a convectively heated stretching plate with chemical reaction. Heat Transfer 2020, 49, 1982–1999. [Google Scholar] [CrossRef]
- Aldabesh, A.; Hussain, M.; Khan, N.; Riahi, A.; Khan, S.; Tlili, I. Thermal variable conductivity features in Buongiorno nanofluid model between parallel stretching disks: Improving energy system efficiency. Case Stud. Therm. Eng. 2021, 23. [Google Scholar] [CrossRef]
- Alhamaly, A.S.; Khan, M.; Shuja, S.Z.; Yilbas, B.S.; Al-Qahtani, H. Axisymmetric stagnation point flow on linearly stretching surfaces and heat transfer: Nanofluid with variable physical properties. Case Stud. Therm. Eng. 2021, 24. [Google Scholar] [CrossRef]
- Ramesh, G.K.; Gireesha, B.J.; Kumara, B.C.; Gorla, R.S. MHD stagnation point flow of nanofluid towards a stretching surface with variable thickness and thermal radiation. J. Nanofluids 2015, 4, 247–253. [Google Scholar] [CrossRef]
- Srinivasulu, T.; Goud, B.S. Effect of inclined magnetic field on flow, heat and mass transfer of Williamson nanofluid over a stretching sheet. Case Stud. Therm. Eng. 2021, 23, 100819. [Google Scholar] [CrossRef]
- Reddy, Y.R.; Rao, V.S.; Kumar, M.A. Effect of heat generation/absorption on MHD copper-water nanofluid flow over a non-linear stretching/shrinking sheet. AIP Conf. Proc. 2020, 2246. [Google Scholar] [CrossRef]
- Nayak, M.K.; Shaw, S.; Chamkha, S.J. 3D MHD free convective stretched flow of a radiative nanofluid inspired by variable magnetic field. Arab. J. Sci. Eng. 2019, 44, 1269–1282. [Google Scholar] [CrossRef]
- Williamson, R.V. The flow of pseudo plastic materials. Ind. Eng. Chem. 1929, 21, 1108–1111. [Google Scholar] [CrossRef]
- Rasheed, H.; AL-Zubaidi, A.; Islam, S.; Saleem, S.; Khan, Z.; Khan, W. Effects of joule heating and viscous dissipation on magnetohydrodynamic boundary layer flow of Jeffrey nanofluid over a vertically stretching cylinder. Coatings 2021, 11, 353. [Google Scholar] [CrossRef]
- Ibrahim, W.; Negera, M. Melting and viscous dissipation effect on upper-convected Maxwell and Williamson nanofluid. Eng. Rep. 2020, 2. [Google Scholar] [CrossRef]
- Vasudev, C.; Rao, U.R.; Reddy, M.V.S.; Rao, G.P. Peristaltic Pumping of Williamson fluid through a porous medium in a horizontal channel with heat transfer. Am. J. Sci. Ind. Res. 2010, 1, 656–666. [Google Scholar] [CrossRef]
- Nadeem, S.; Hussain, S.T. Flow and heat transfer analysis of Williamson nanofluid. Appl. Nanosci. 2014, 4, 1005–1012. [Google Scholar] [CrossRef]
- Gorla, R.S.R.; Gireesha, B.J. Dual solutions for stagnation-point flow and convective heat transfer of a Williamson nanofluid past a stretching/shrinking sheet. Heat Mass Transf. 2016, 52, 1153–1162. [Google Scholar] [CrossRef]
- Abbas, Z.; Rafiq, M.Y.; Alshomrani, A.S.; Ullah, M.Z. Analysis of entropy generation on peristaltic phenomena of MHD slip flow of viscous fluid in a diverging tube. Case Stud. Therm. Eng. 2021, 23. [Google Scholar] [CrossRef]
- Fang, T.; Zhang, J.; Zhong, Y. Boundary layer flow over a stretching sheet with variable thickness. Appl. Math. Comput. 2012, 218, 7241–7252. [Google Scholar] [CrossRef]
- Subhashini, S.V.; Sumathi, R.; Pop, I. Dual solutions in a thermal diffusive flow over a stretching sheet with variable thickness. Int. Comput. Heat Mass Transf. 2013, 48, 61–66. [Google Scholar] [CrossRef]
- Khader, M.M.; Meghad, M.A. Differential transformation method for studying flow and heat transfer due to stretching sheet embedded in porous medium with variable thickness, variable thermal conductivity, and thermal radiation. Appl. Math. Mech. Engl. Ed. 2014, 35, 1387–1400. [Google Scholar] [CrossRef]
- Nayak, M.K.; Shaw, S.; Makinde, O.D. Chemically reacting and radiating nanofluid flow past an exponentially stretching sheet in a porous medium. Indian J. Pure Appl. Phys. 2018, 56, 773–786. [Google Scholar]
- Sachin, S.; Motsa, S.S.; Sibanda, P. Magnetic field and viscous dissipation effect on bioconvection in a permeable sphere embedded in a porous medium with a nanofluid containing gyrotactic micro-organisms. Heat Transfer Asian Res. 2018, 47, 718–734. [Google Scholar]
- Sachin, S.; Motsa, S.S.; Sibanda, P. Nanofluid flow over three different geometries under viscous dissipation and thermal radiation using the local linearization method. Heat Transfer Asian Res. 2019, 48, 2370–2386. [Google Scholar]
- Rasheed, H.; Khan, Z.; Islam, S.; Khan, I.; Guirao, J.L.G.; Khan, W. Investigation of two-dimensional viscoelastic fluid with nonuniform heat generation over permeable stretching sheet with slip condition. Complexity 2019, 2019, 3121896. [Google Scholar] [CrossRef]
- Rasheed, H.; Khan, Z.; Khan, I.; Ching, D.L.C.; Nisar, K.S. Numerical and analytical investigation of an unsteady thin film nanofluid flow over an angular surface. Processes 2019, 7, 486. [Google Scholar] [CrossRef]
- Chaim, T.C. Heat transfer in a fluid with variable thermal conductivity over a linearly stretching sheet. Acta Mech. 1998, 129, 63–72. [Google Scholar] [CrossRef]









Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Islam, S.; Ur Rasheed, H.; Nisar, K.S.; Alshehri, N.A.; Zakarya, M. Numerical Simulation of Heat Mass Transfer Effects on MHD Flow of Williamson Nanofluid by a Stretching Surface with Thermal Conductivity and Variable Thickness. Coatings 2021, 11, 684. https://doi.org/10.3390/coatings11060684
Islam S, Ur Rasheed H, Nisar KS, Alshehri NA, Zakarya M. Numerical Simulation of Heat Mass Transfer Effects on MHD Flow of Williamson Nanofluid by a Stretching Surface with Thermal Conductivity and Variable Thickness. Coatings. 2021; 11(6):684. https://doi.org/10.3390/coatings11060684
Chicago/Turabian StyleIslam, Saeed, Haroon Ur Rasheed, Kottakkaran Sooppy Nisar, Nawal A. Alshehri, and Mohammed Zakarya. 2021. "Numerical Simulation of Heat Mass Transfer Effects on MHD Flow of Williamson Nanofluid by a Stretching Surface with Thermal Conductivity and Variable Thickness" Coatings 11, no. 6: 684. https://doi.org/10.3390/coatings11060684
APA StyleIslam, S., Ur Rasheed, H., Nisar, K. S., Alshehri, N. A., & Zakarya, M. (2021). Numerical Simulation of Heat Mass Transfer Effects on MHD Flow of Williamson Nanofluid by a Stretching Surface with Thermal Conductivity and Variable Thickness. Coatings, 11(6), 684. https://doi.org/10.3390/coatings11060684







