Prediction of Dissolved Impurities and Movement of Oxide Particles in the Primary Circuit of LBE Fast Reactor
Abstract
:1. Introduction
2. Service Condition of Structure Materials in LFR
2.1. Operation Condition of Typical LFR
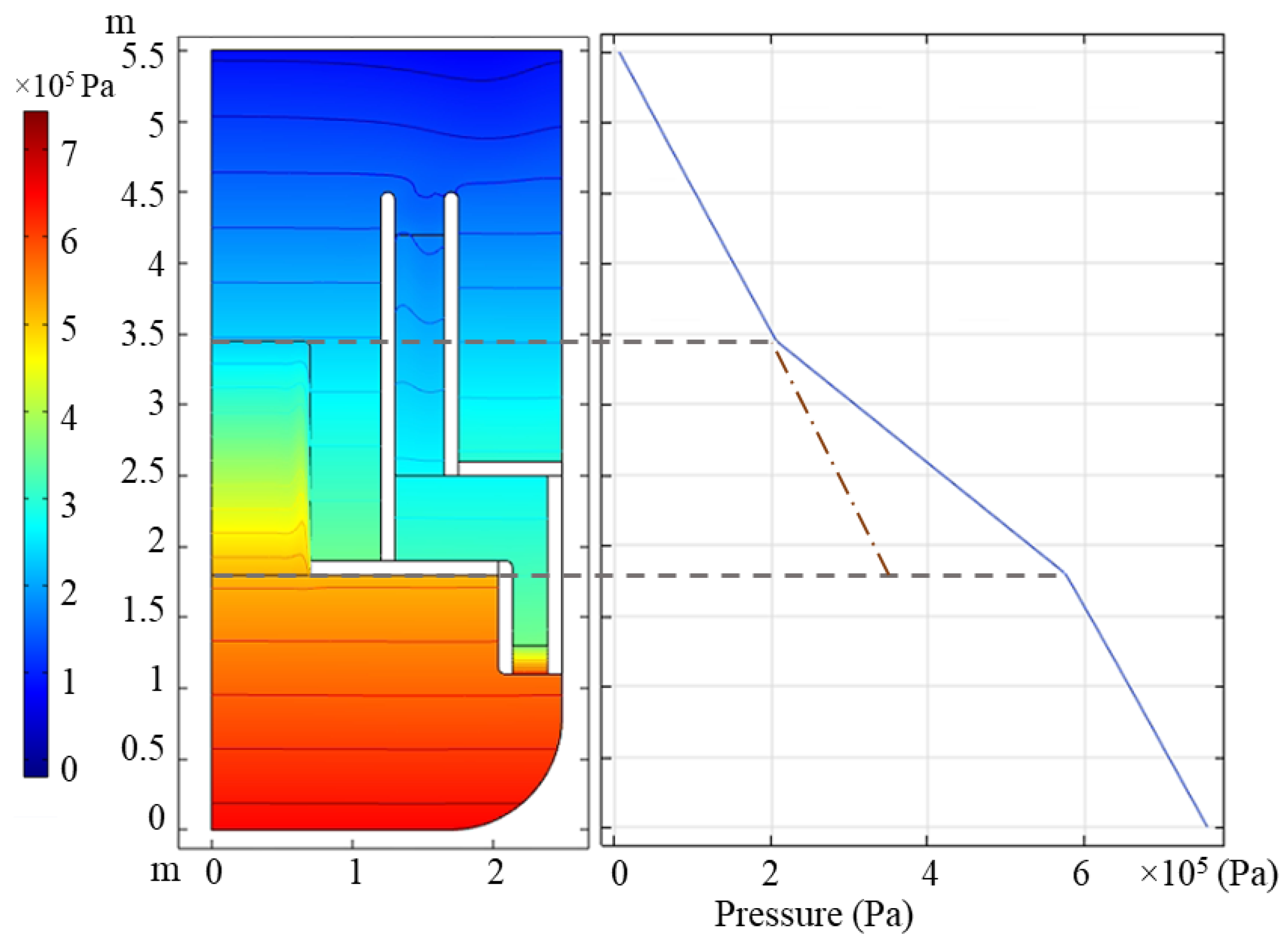
2.2. Flow Field and Temperature Field
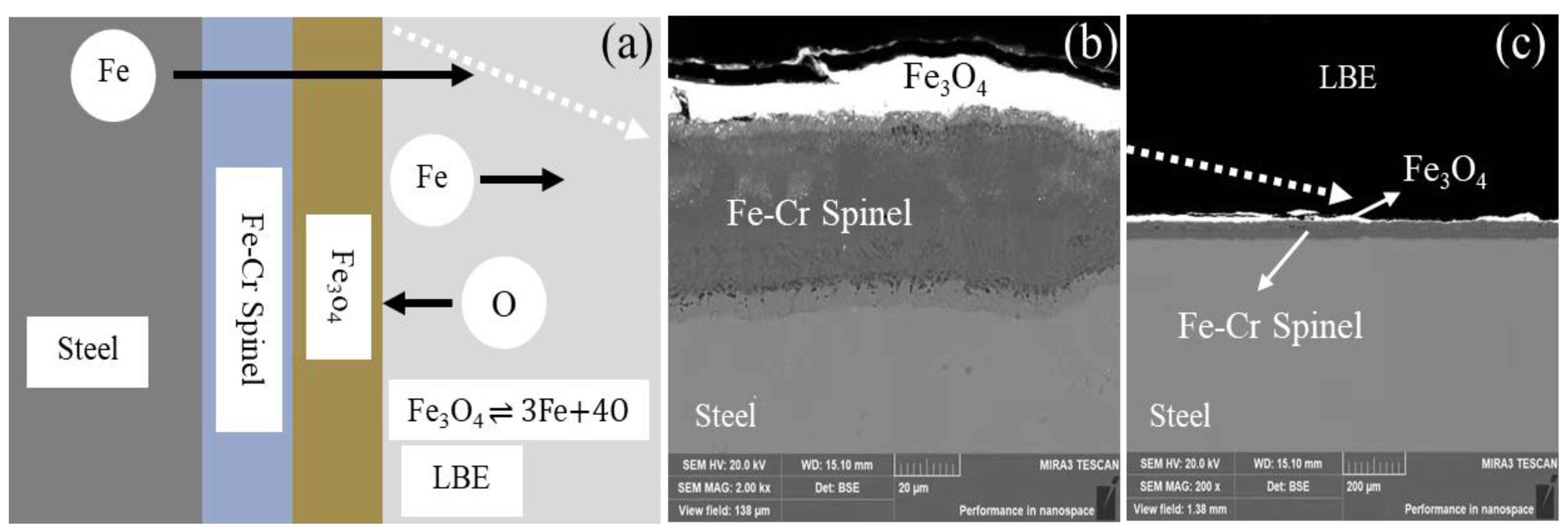
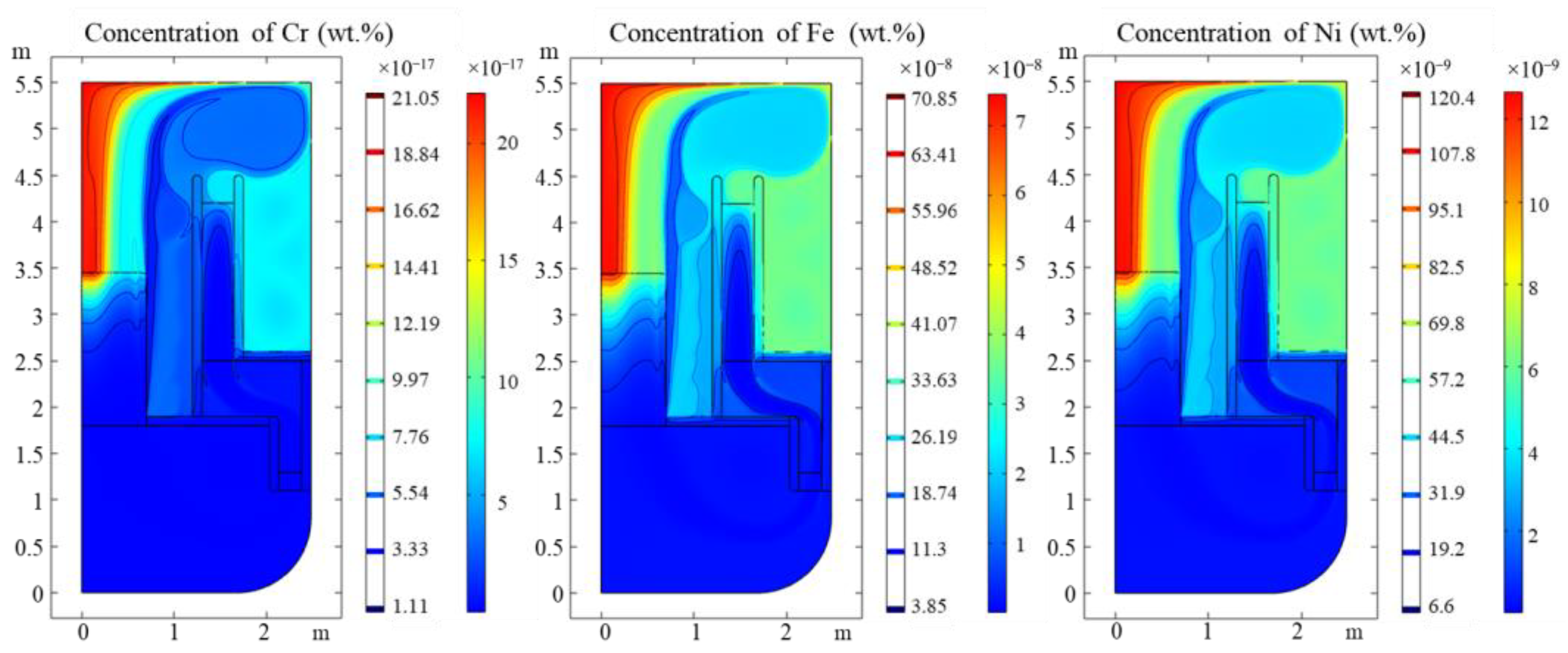
3. Corrosion Products Concentration Distribution
4. Tracing of CPs
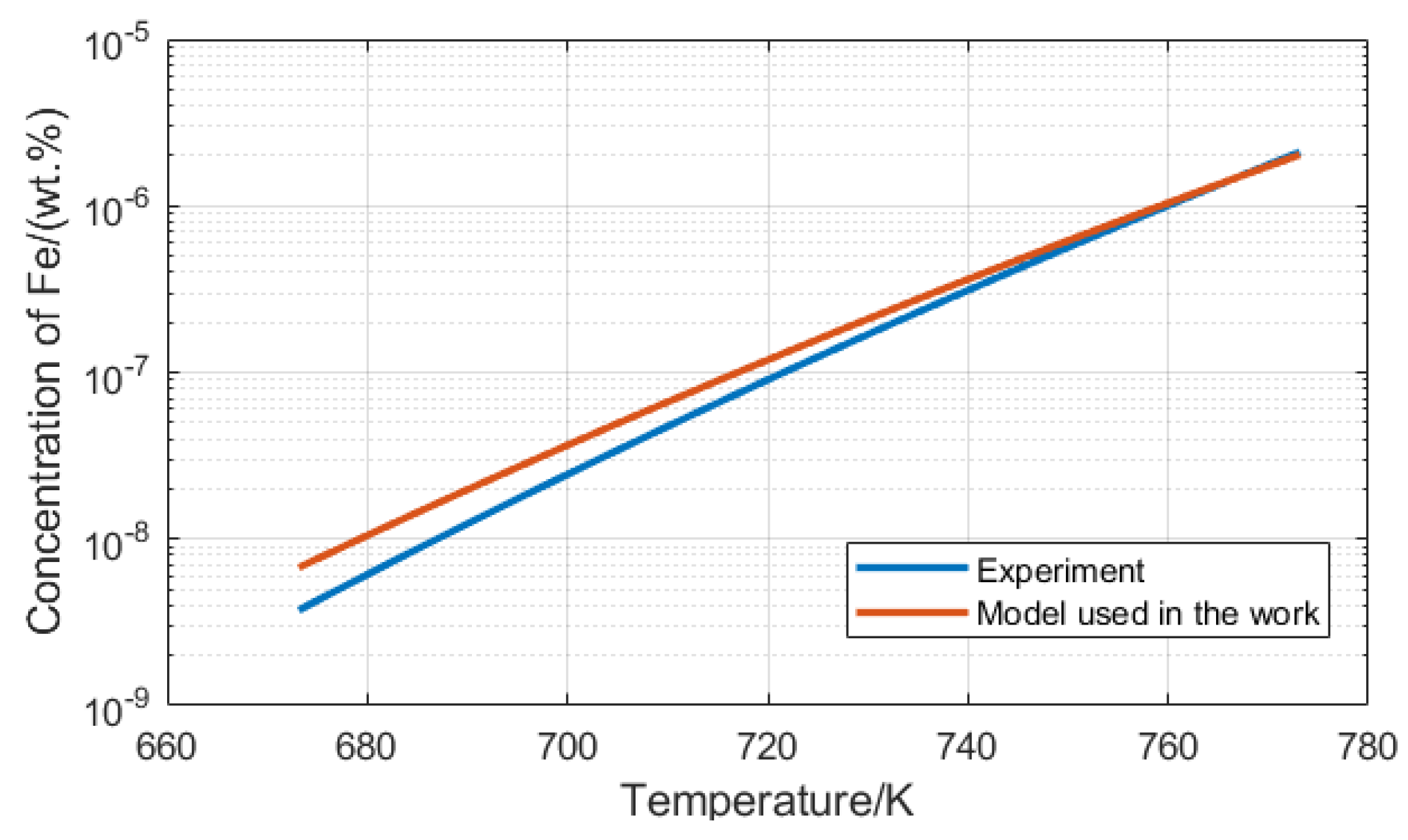
4.1. Numerical Model and Basic Assumptions
4.2. Particles Behavior in the Primary Circuit
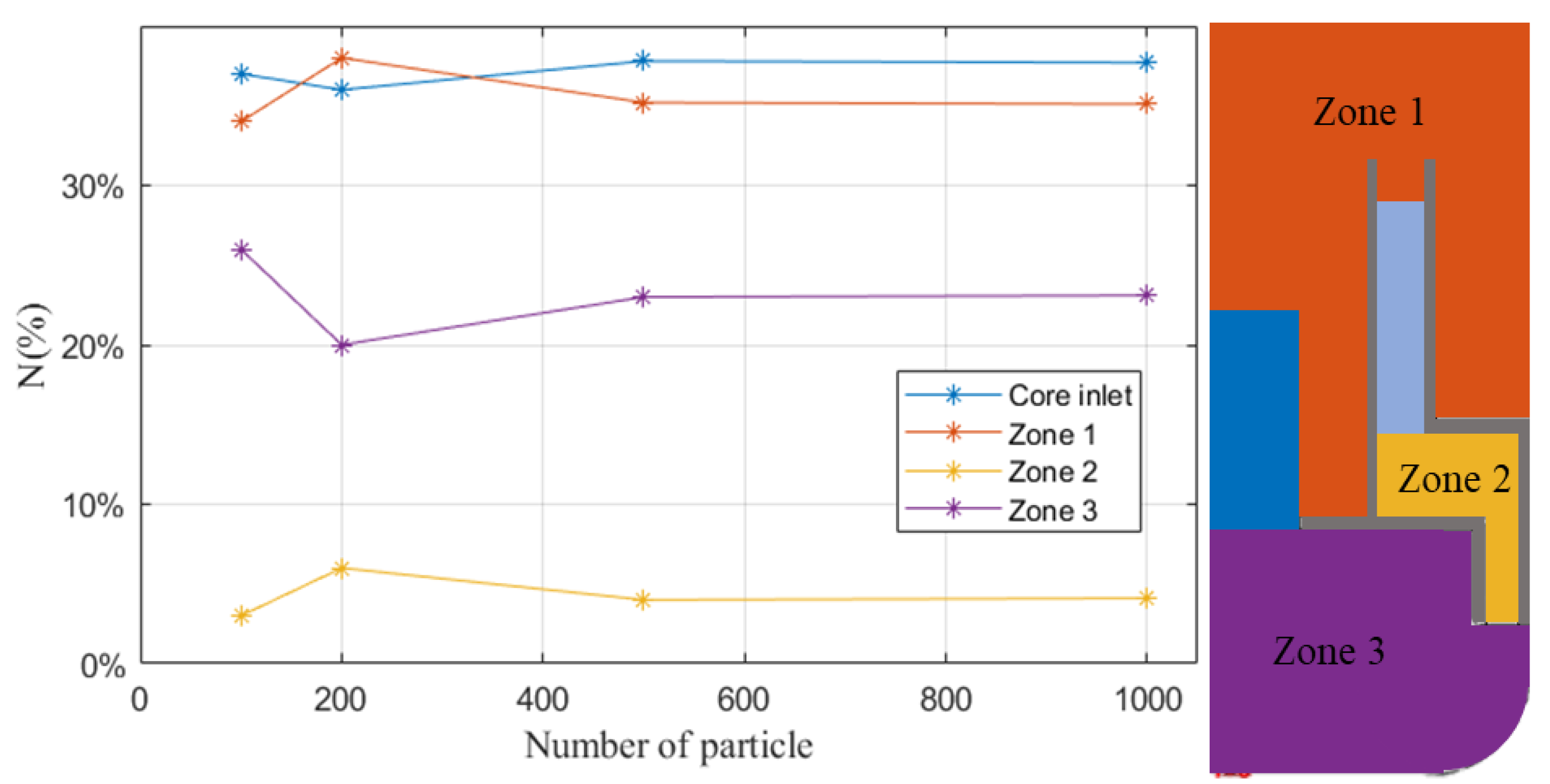
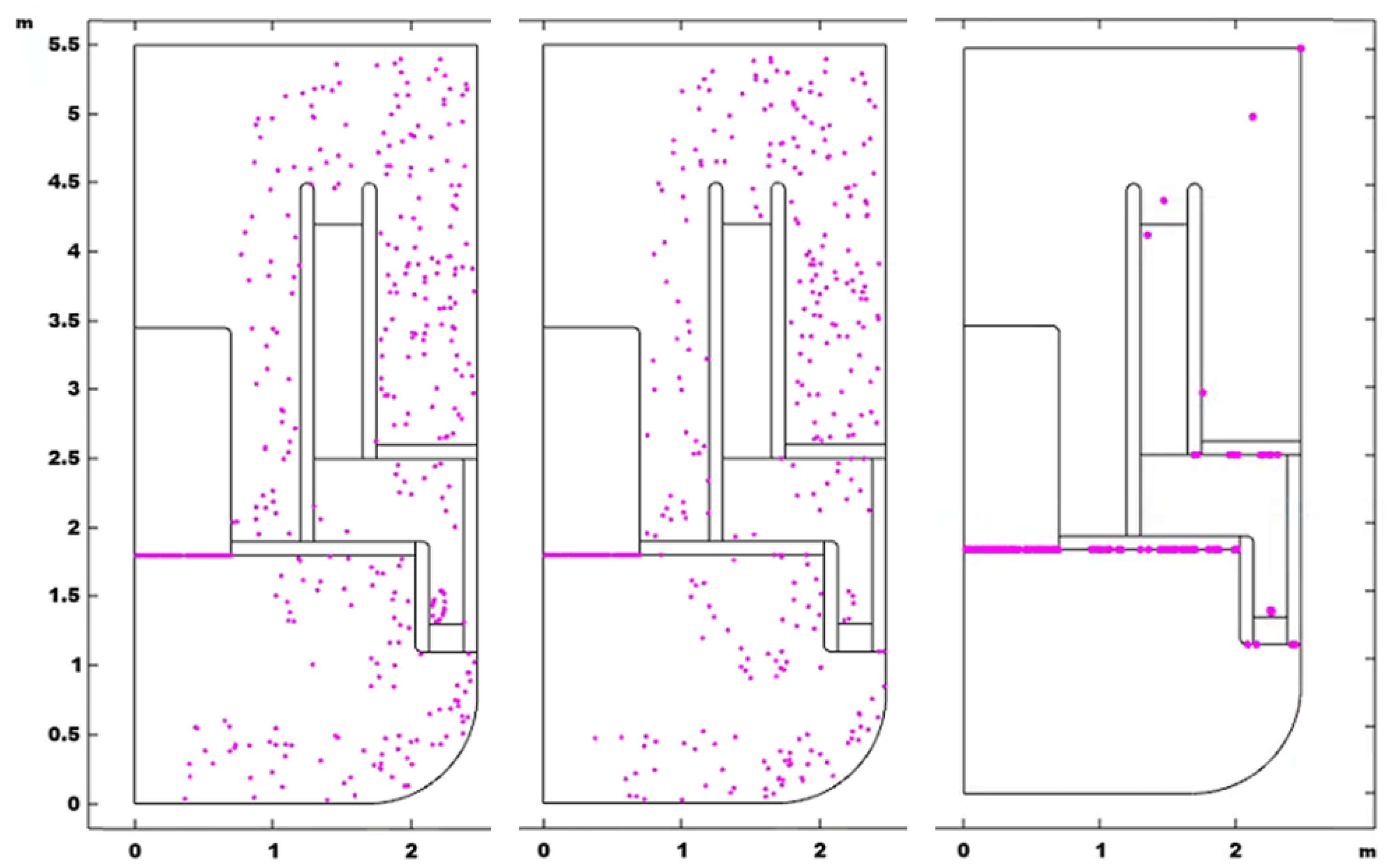
4.2.1. Random Particle Release
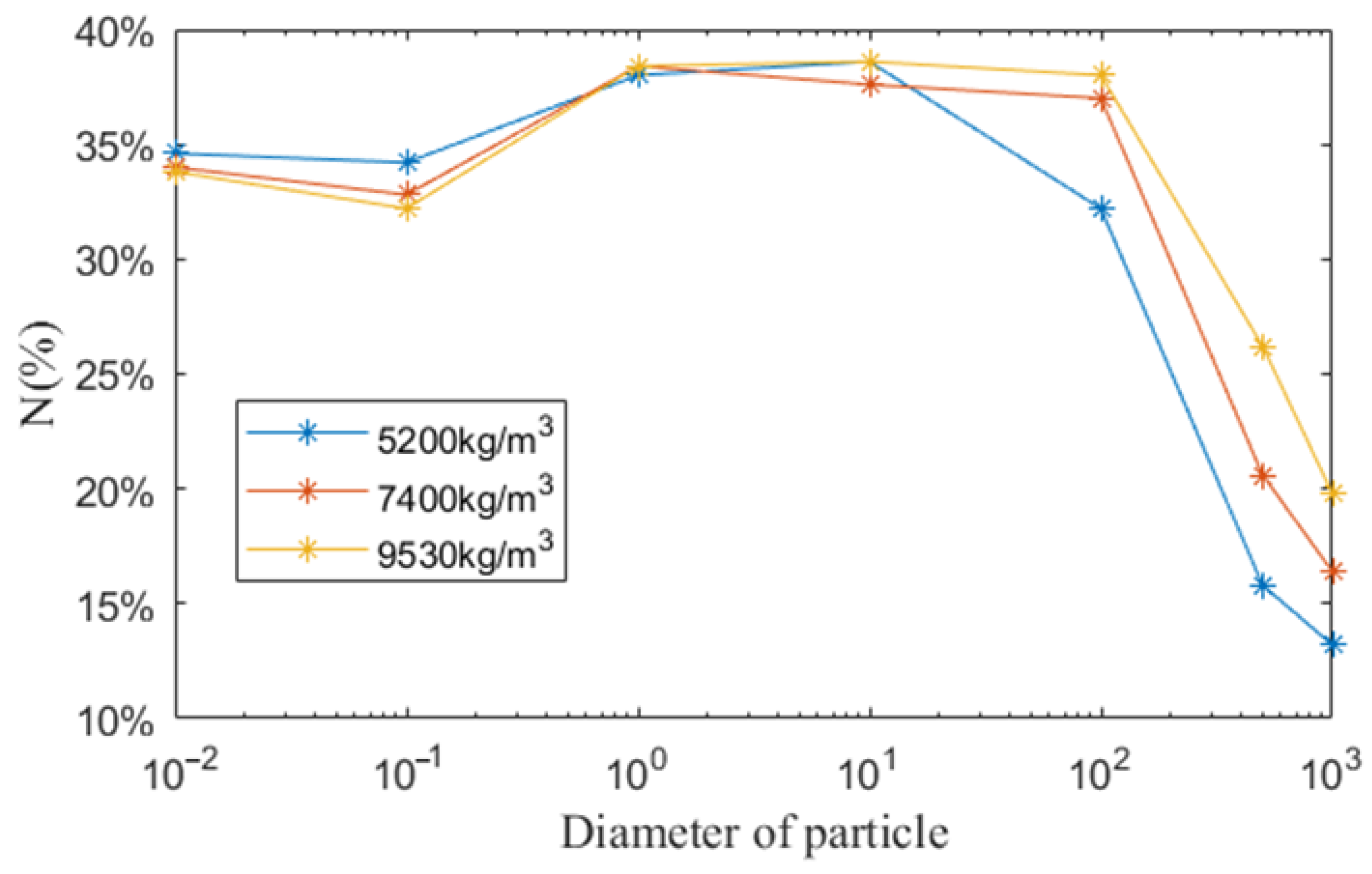
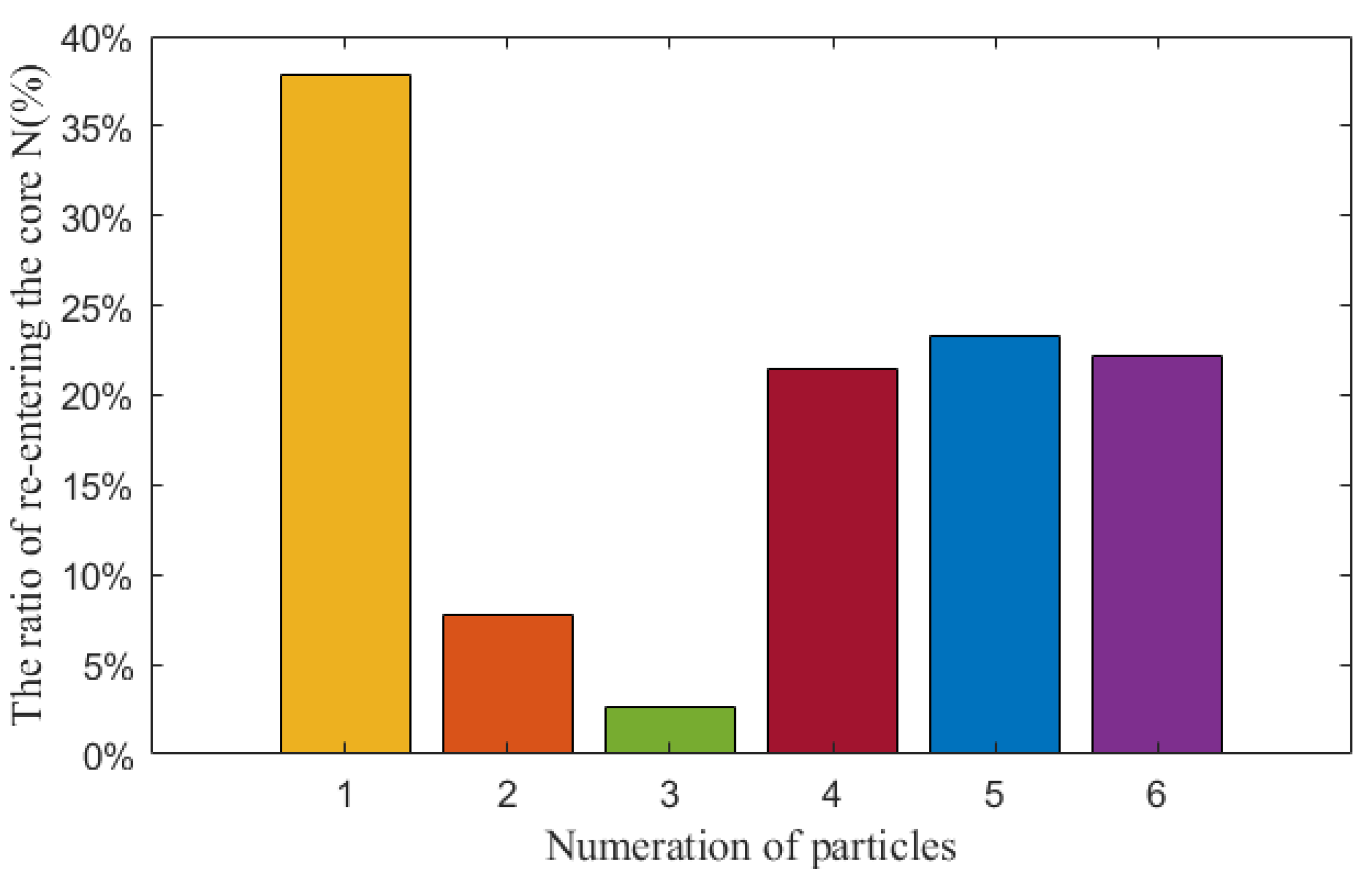
4.2.2. The Influence of Initial Position
5. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Zhang, J.; Li, N. Review of the studies on fundamental issues in LBE corrosion. J. Nucl. Mater. 2008, 373, 351–377. [Google Scholar] [CrossRef]
- Ballinger, R.G.; Lim, J. An overview of corrosion issues for the design and operation of high-temperature lead-and lead-bismuth-cooled reactor systems. Nucl. Technol. 2004, 147, 418–435. [Google Scholar] [CrossRef]
- Gromov, B.; Orlov, Y.; Martynov, P.; Gulevsky, V. The problems of technology of the heavy liquid metal coolants. In Proceedings of the Heavy Liquid Metal Coolants in Nuclear Technology Conference, Obninsk, Russia, 5–9 October 1998; pp. 87–100. [Google Scholar]
- Schroer, C.; Voß, Z.; Wedemeyer, O.; Novotny, J.; Konys, J. Oxidation of steel T91 in flowing lead–bismuth eutectic (LBE) at 550° C. J. Nucl. Mater. 2006, 356, 189–197. [Google Scholar] [CrossRef]
- Zhang, J.; Li, N. Corrosion/precipitation in non-isothermal and multi-modular LBE loop systems. J. Nucl. Mater. 2004, 326, 201–210. [Google Scholar] [CrossRef]
- Orlov, Y.I.; Efanov, A.D.; Martynov, P.N.; Gulevsky, V.A.; Papovyants, A.K.; Levchenko, Y.D.; Ulyanov, V.V. Hydrodynamic problems of heavy liquid metal coolants technology in loop-type and mono-block-type reactor installations. Nucl. Eng. Des. 2007, 237, 1829–1837. [Google Scholar] [CrossRef]
- Marino, A.; Buckingham, S.; Gladinez, K.; Keijers, S.; Planquart, P.; Van Tichelen, K.; Aerts, A. Numerical modeling of iron-based corrosion product oxides mass transport in the MYRRHA reactor during normal operation. Nucl. Eng. Des. 2018, 338, 199–208. [Google Scholar] [CrossRef]
- Schroer, C.; Konys, J.; Furukawa, T.; Aoto, K. Oxidation behaviour of P122 and a 9Cr–2W ODS steel at 550 C in oxygen-containing flowing lead–bismuth eutectic. J. Nucl. Mater. 2010, 398, 109–115. [Google Scholar] [CrossRef]
- Müller, G.; Schumacher, G.; Zimmermann, F. Investigation on oxygen controlled liquid lead corrosion of surface treated steels. J. Nucl. Mater. 2000, 278, 85–95. [Google Scholar] [CrossRef]
- Li, N. Active control of oxygen in molten lead–bismuth eutectic systems to prevent steel corrosion and coolant contamination. J. Nucl. Mater. 2002, 300, 73–81. [Google Scholar] [CrossRef]
- Gorynin, I.; Karzov, G.; Markov, V.; Lavrukhin, V.; Yakovlev, V.Y. Structural materials for power plants with heavy liquid metals as coolants. JOM 1999, 1, 372. [Google Scholar]
- Martynov, P.; Askhadullin, R.S.; Simakov, A.; Chaban’, A.Y.; Legkikh, A.Y. Designing mass exchangers for control of oxygen content in Pb-Bi (Pb) coolants in various research facilities. In Proceedings of the International Conference on Nuclear Engineering, Brussels, Belgium, 12–16 July 2009; pp. 555–561. [Google Scholar]
- Aerts, A.; Gavrilov, S.; Manfredi, G.; Marino, A.; Rosseel, K.; Lim, J. Oxygen–iron interaction in liquid lead–bismuth eutectic alloy. Phys. Chem. Chem. Phys. 2016, 18, 19526–19530. [Google Scholar] [CrossRef] [PubMed]
- Zhang, J.; Hosemann, P.; Maloy, S. Models of liquid metal corrosion. J. Nucl. Mater. 2010, 404, 82–96. [Google Scholar] [CrossRef]
- Lyutyi, E. Problems of high-temperature liquid-metal corrosion of refractory metals and alloys. Sov. Mater. Sci. A Transl. Fiz.-Khimicheskaya Mekhanika Mater. Acad. Sci. Ukr. SSR 1989, 24, 441–445. [Google Scholar] [CrossRef]
- Zrodnikov, A.; Efanov, A.; Orlov, Y.I.; Martynov, P.; Troyanov, V.; Rusanov, A. Heavy liquid metal coolant–lead–bismuth and lead–technology. At. Energy 2004, 97, 534–537. [Google Scholar] [CrossRef]
- Koloszar, L. CFD simulation of the thermohydraulics of the MYRRHA reactor. In Proceedings of the THINS 2014 International Workshop, Modena, Italy, 20–22 January 2014. [Google Scholar]
- Saeidi, S. Study of MHD Corrosion and Transport of Corrosion Products of Ferritic/Martensitic Steels in the Flowing PbLi and Its Application to Fusion Blanket; University of California: Los Angeles, CA, USA, 2014. [Google Scholar]
- Deng, L.; Wang, Y.; Zhai, Z.; Huang, B.; He, J.; Wu, J.; Ni, M. Multi-physics model development for Polonium transport behavior in Lead-cooled Fast Reactor. Front. Energy Res. 2021, 9, 365. [Google Scholar]
- Koloszar, L.; Buckingham, S.; Planquart, P.; Keijers, S. MyrrhaFoam: A CFD model for the study of the thermal hydraulic behavior of MYRRHA. Nucl. Eng. Des. 2017, 312, 256–265. [Google Scholar] [CrossRef]
- Fazio, C.; Sobolev, V.; Aerts, A.; Gavrilov, S.; Lambrinou, K.; Schuurmans, P.; Gessi, A.; Agostini, P.; Ciampichetti, A.; Martinelli, L. Handbook on Lead-Bismuth Eutectic Alloy and Lead Properties, Materials Compatibility, Thermal-Hydraulics and Technologies, 2015th ed.; Organisation for Economic Co-Operation and Development: Paris, France, 2015. [Google Scholar]
- Crespo, L. Compatibility of structural materials with LBE and Pb: Standardization of data, corrosion mechanism and rate. In Handbook on Lead–Bismuth Eutectic Alloy and Lead Properties, Material Compatibility, Thermal-Hydraulics and Technologies; Nuclear Energy Agency, Nuclear Science Committee: Paris, France, 2007; pp. 231–274. [Google Scholar]
- Martynov, P.N.; Askhadullin, R.S. Filtration purification of heavy liquid metal coolants from impurities. Probl. At. Sci. Technol. Ser. Nucl. React. Constants 2015, 1, 129–213. [Google Scholar]
- Morsi, S.; Alexander, A. An investigation of particle trajectories in two-phase flow systems. J. Fluid Mech. 1972, 55, 193–208. [Google Scholar] [CrossRef]



| Design Parameters | CLEAR-I | SVBR-100 | MYRRHA | This Case |
|---|---|---|---|---|
| Country | China | Russia | Belgium | |
| Thermal capacity (MWth) | 10 | 280 | 100 | 100 |
| System pressure (MPa) | 0.05 | 6.7 | 0.17 | 3 |
| inlet/outlet (°C) | 260/390 | 340/490 | 270/410 | 250/350 |
| Primary circulation | Natural | Forced | Forced | Forced |
| Reactor vessel D/H (m) | 4.53/6.92 | 8.0/12 | 5/7 | |
| Flow rate of LBE coolant (kg/s) | 529.5 | 11,760 | 9400 | 529.5 |
| Core: D/H (m) | 1.64/0.9 | 1.83/1.75 | 1.4/1.75 | |
| LBE inventory (ton) | 700 | 18.4 | 4500 | 110 |
| Average coolant flow rate (m/s) | 0.55 | 0.27 | ||
| The high coolant flow rate (m/s) | 2.37 | 1.88 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Wu, J.; Wu, R.; Wang, Y.; He, J.; Hu, C.; Zeng, X.; Ni, M. Prediction of Dissolved Impurities and Movement of Oxide Particles in the Primary Circuit of LBE Fast Reactor. Coatings 2021, 11, 1263. https://doi.org/10.3390/coatings11101263
Wu J, Wu R, Wang Y, He J, Hu C, Zeng X, Ni M. Prediction of Dissolved Impurities and Movement of Oxide Particles in the Primary Circuit of LBE Fast Reactor. Coatings. 2021; 11(10):1263. https://doi.org/10.3390/coatings11101263
Chicago/Turabian StyleWu, Jiewei, Rongjun Wu, Yuqing Wang, Jianbo He, Chen Hu, Xian Zeng, and Muyi Ni. 2021. "Prediction of Dissolved Impurities and Movement of Oxide Particles in the Primary Circuit of LBE Fast Reactor" Coatings 11, no. 10: 1263. https://doi.org/10.3390/coatings11101263
APA StyleWu, J., Wu, R., Wang, Y., He, J., Hu, C., Zeng, X., & Ni, M. (2021). Prediction of Dissolved Impurities and Movement of Oxide Particles in the Primary Circuit of LBE Fast Reactor. Coatings, 11(10), 1263. https://doi.org/10.3390/coatings11101263






