Darcy-Forchheimer MHD Hybrid Nanofluid Flow and Heat Transfer Analysis over a Porous Stretching Cylinder
Abstract
:1. Introduction
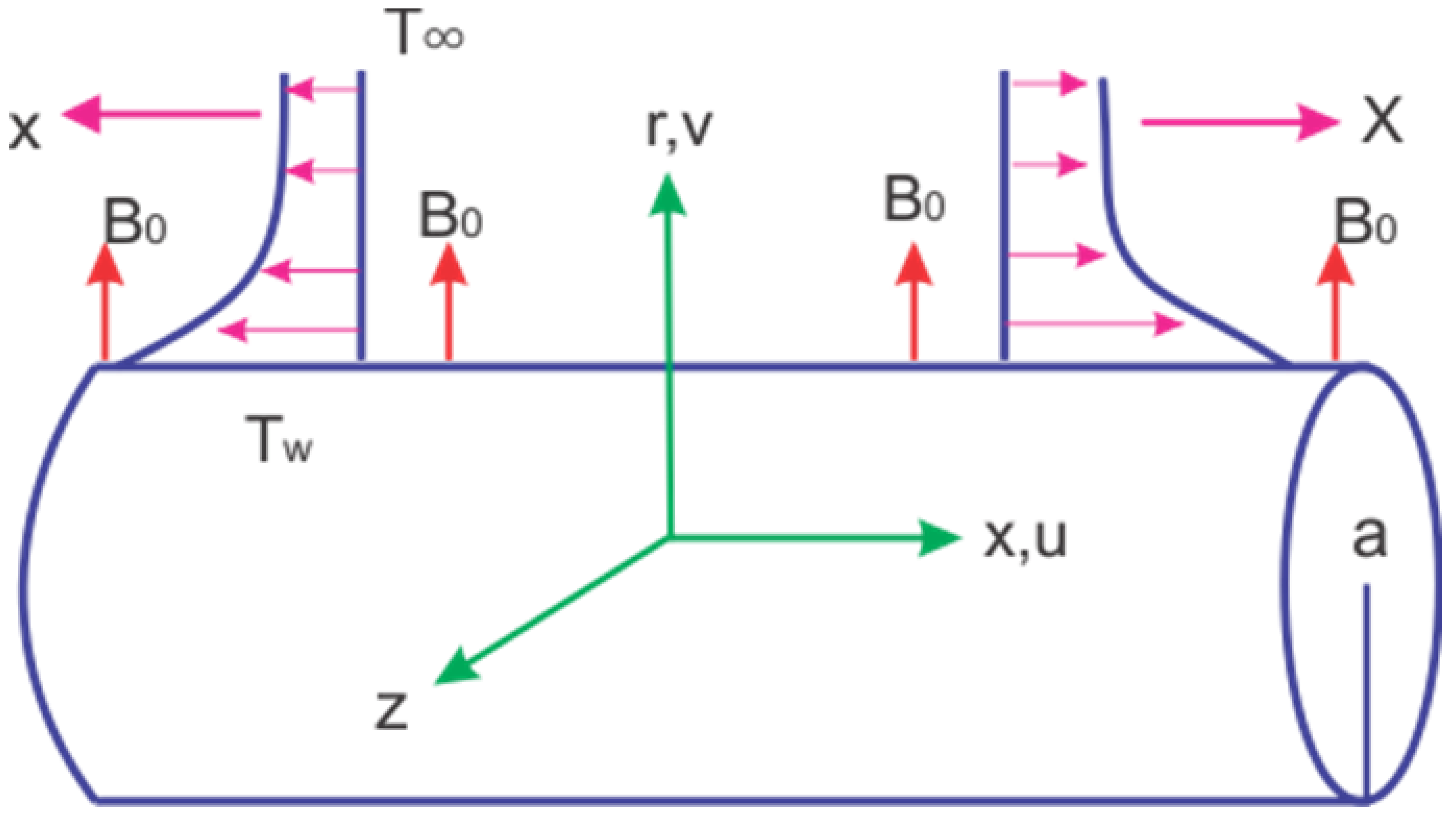
2. Mathematical Formulation
2.1. Flow Modelling
2.2. Important Physical Quantities
2.3. Hybrid Nanofluid Modelling
2.4. Solution by HAM
3. Result and Discussion
3.1. Velocity
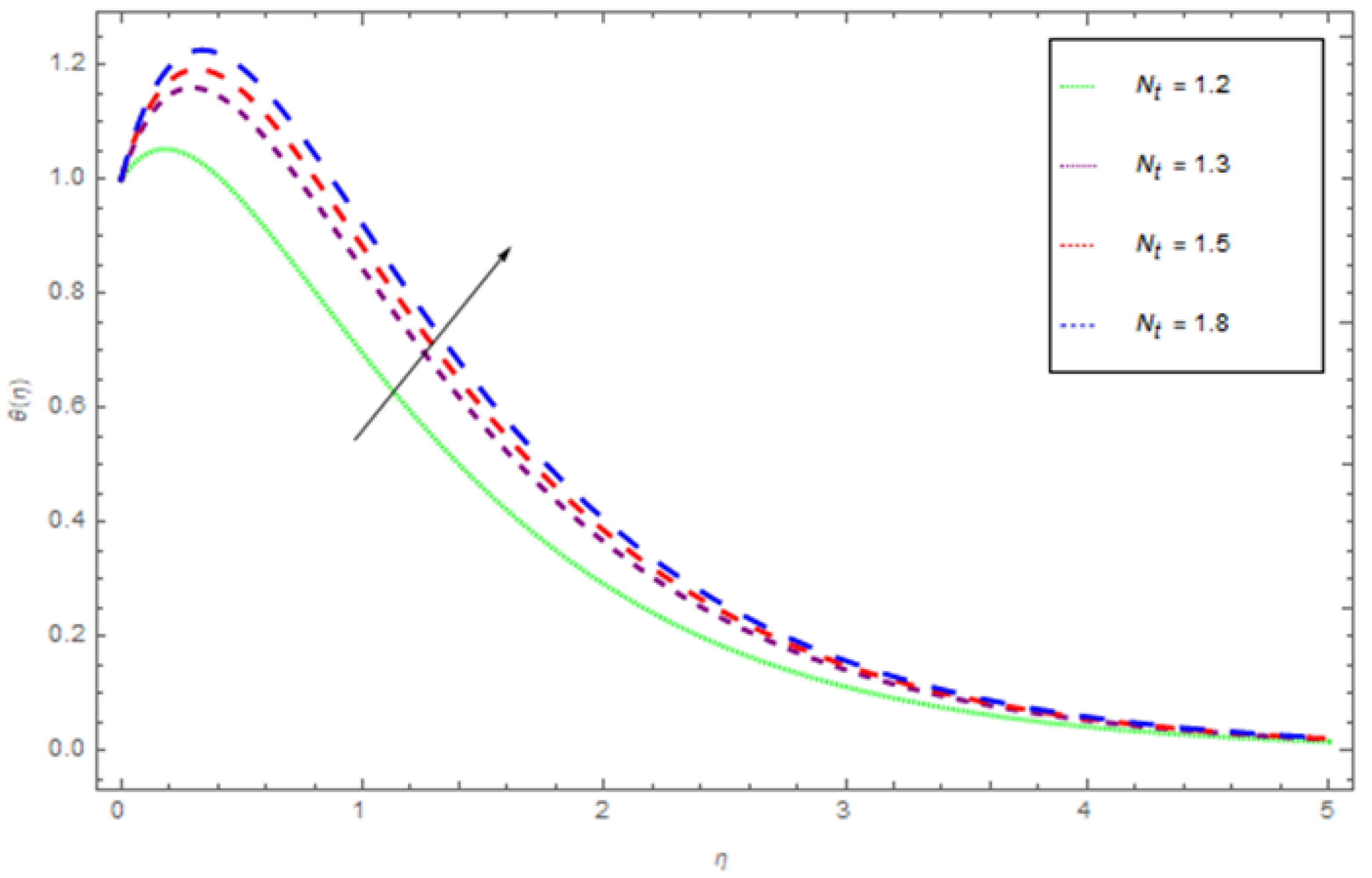
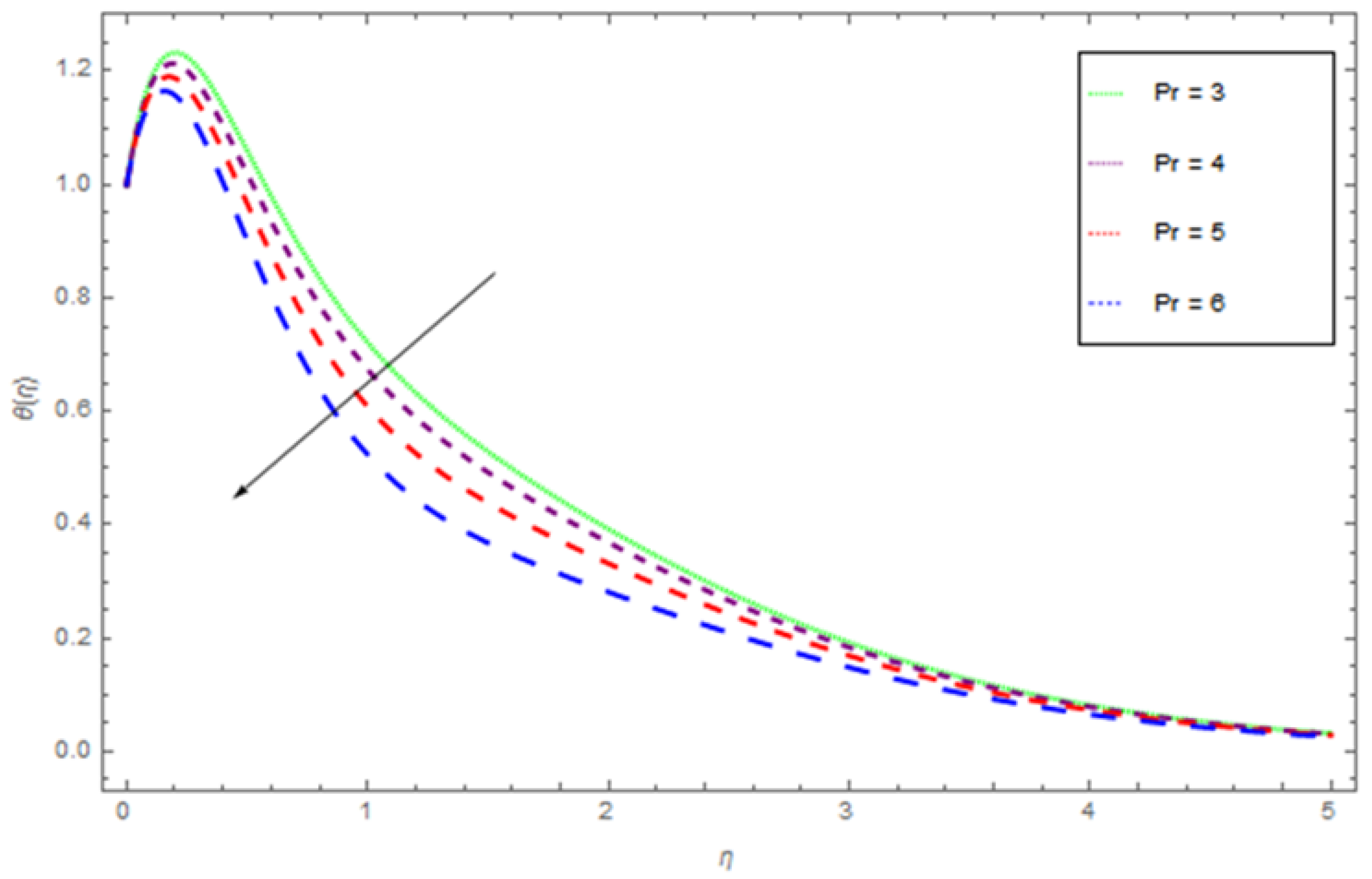
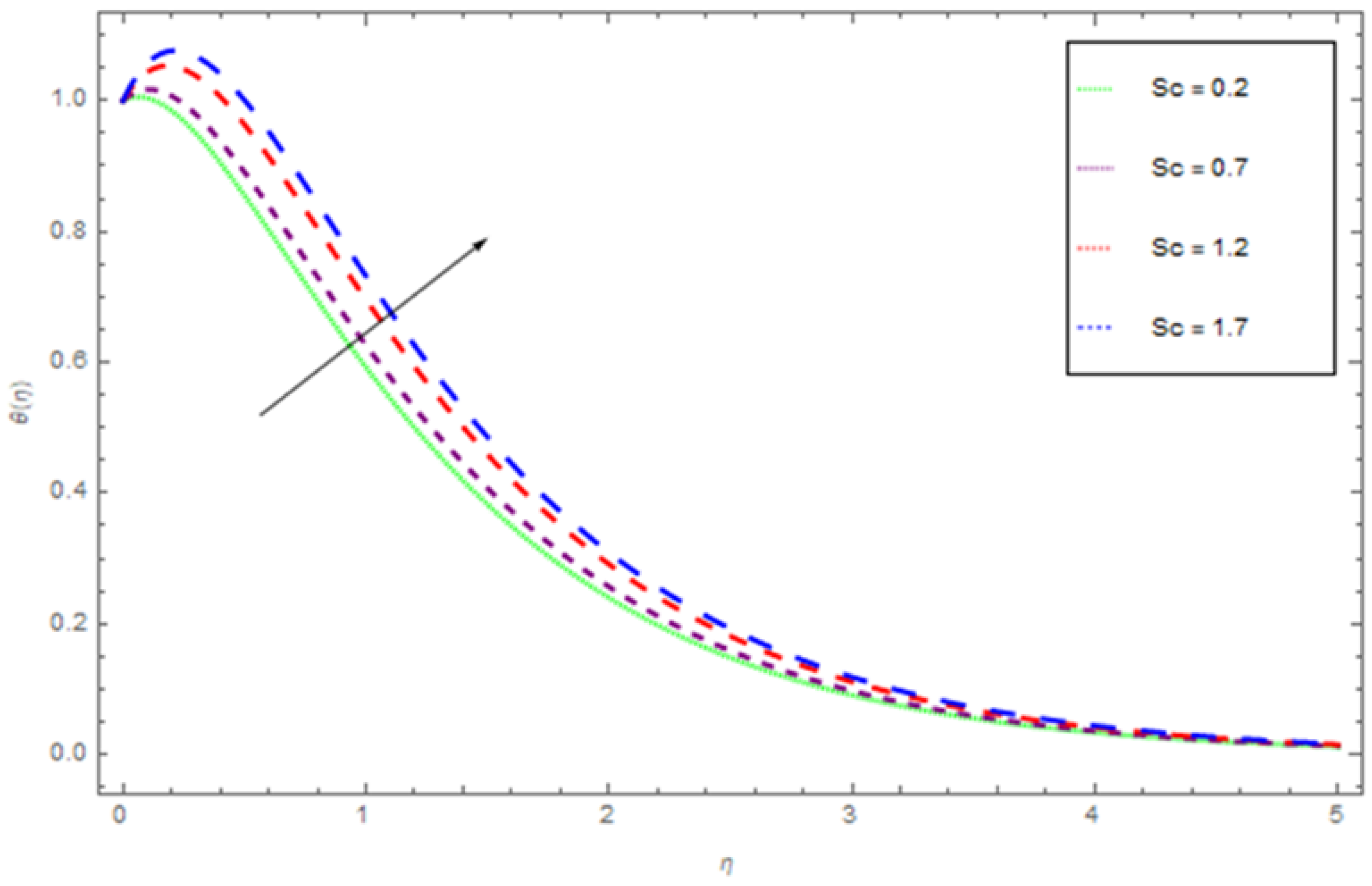
3.2. Temperature
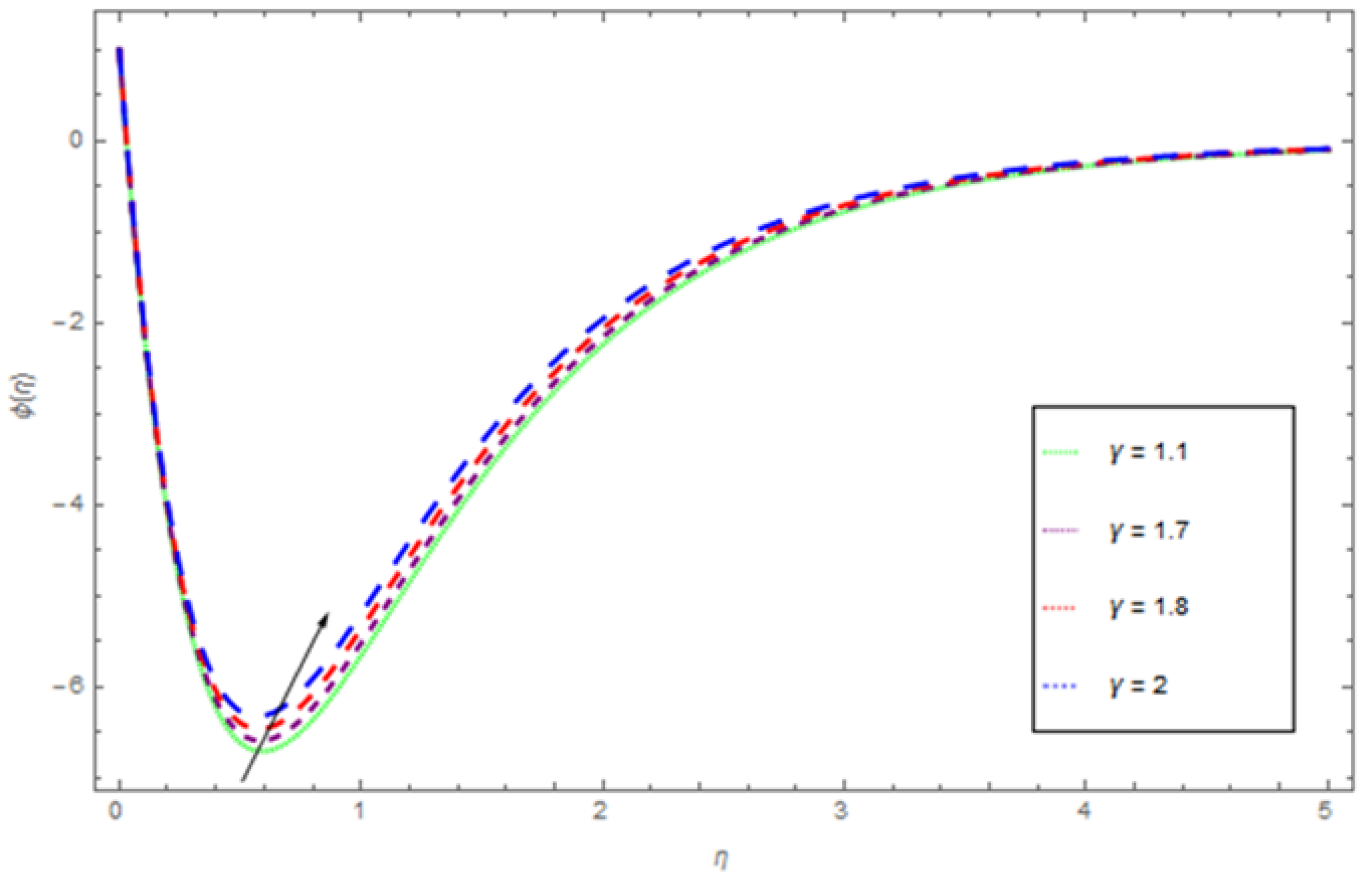
3.3. Concentration
3.4. Table Discussion
4. Conclusions
- The higher value of permeability parameter decreases .
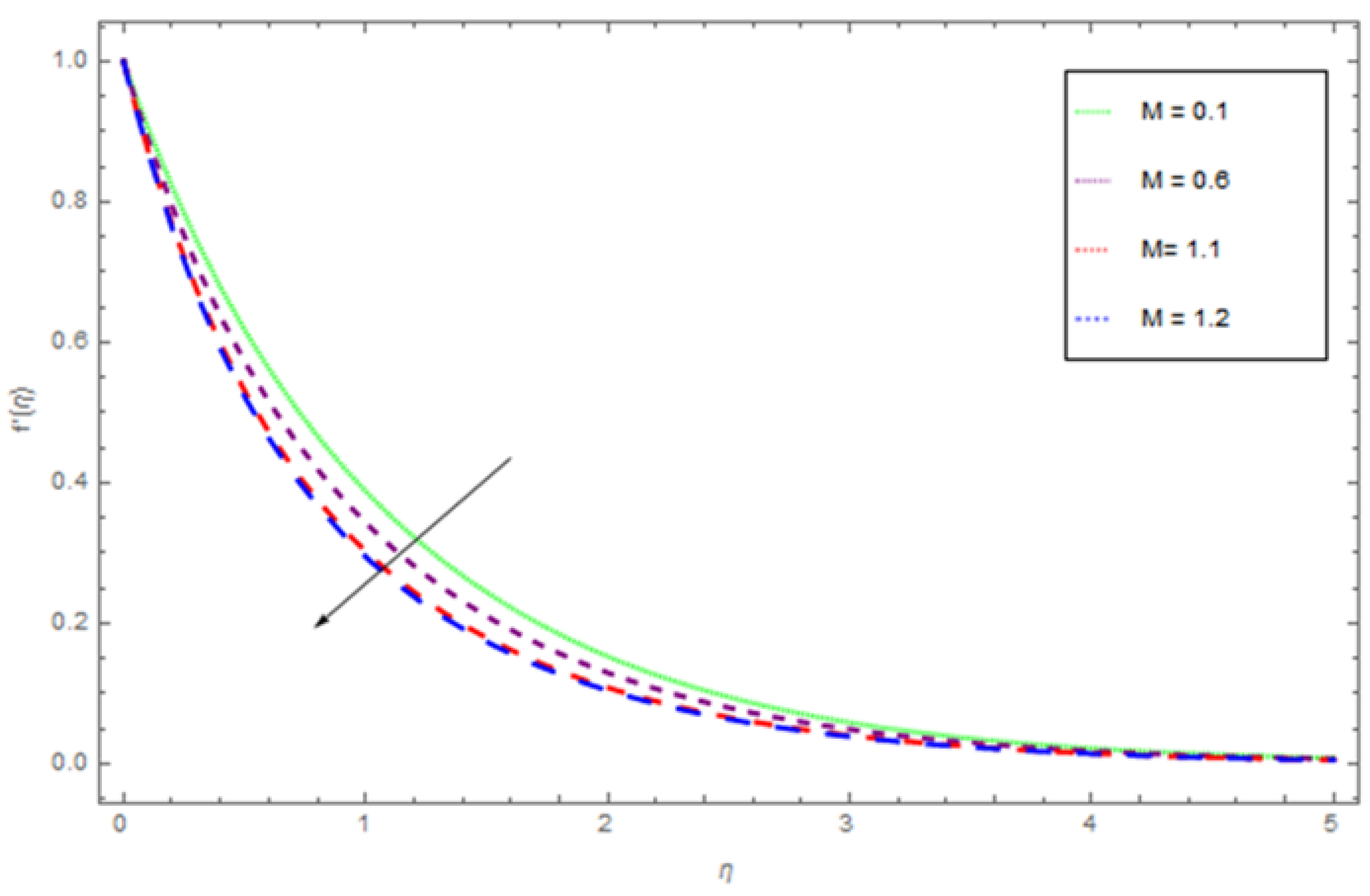
- Increase in causes a better flow resistance, so velocity of fluid is reduced.
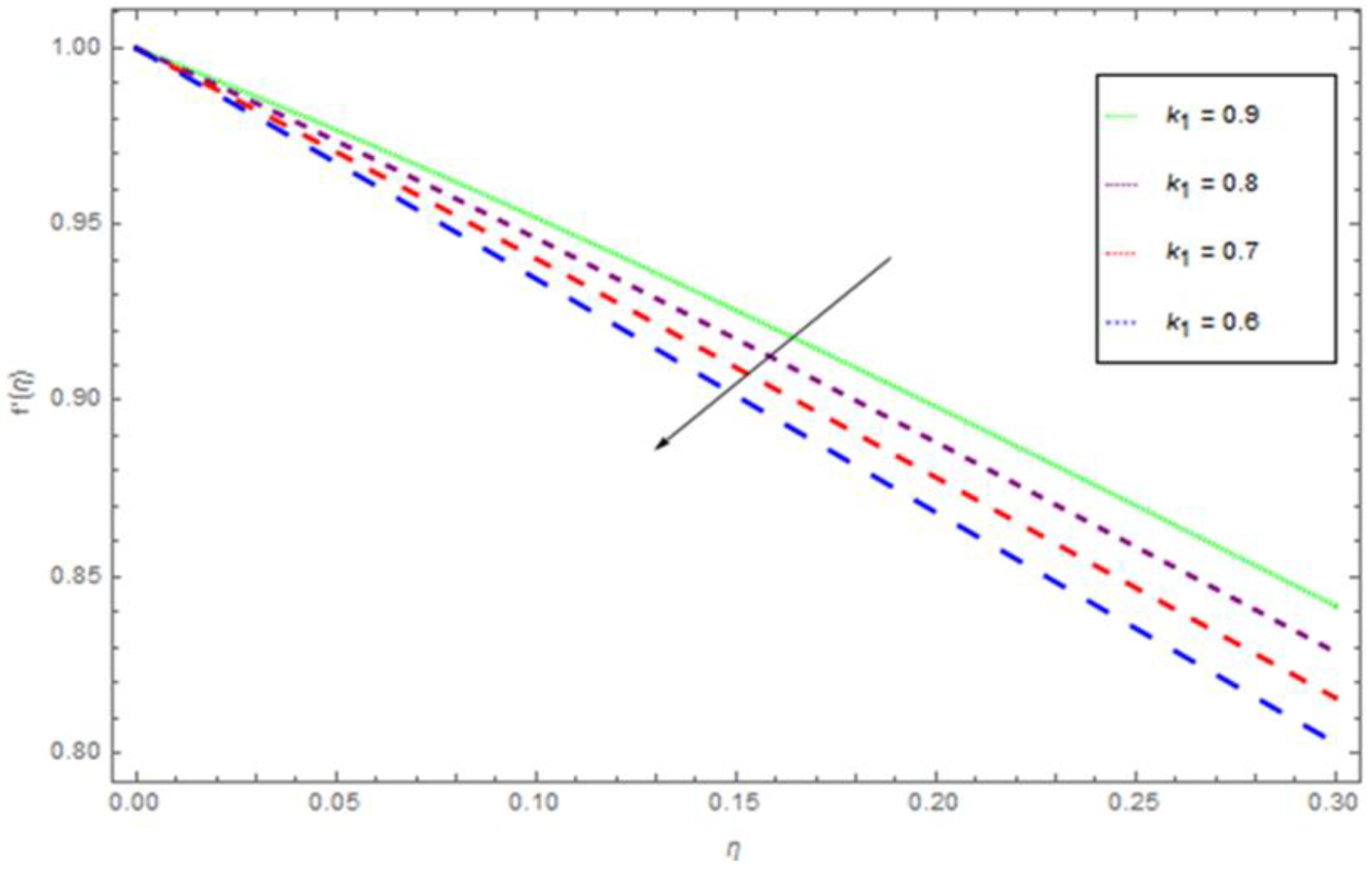
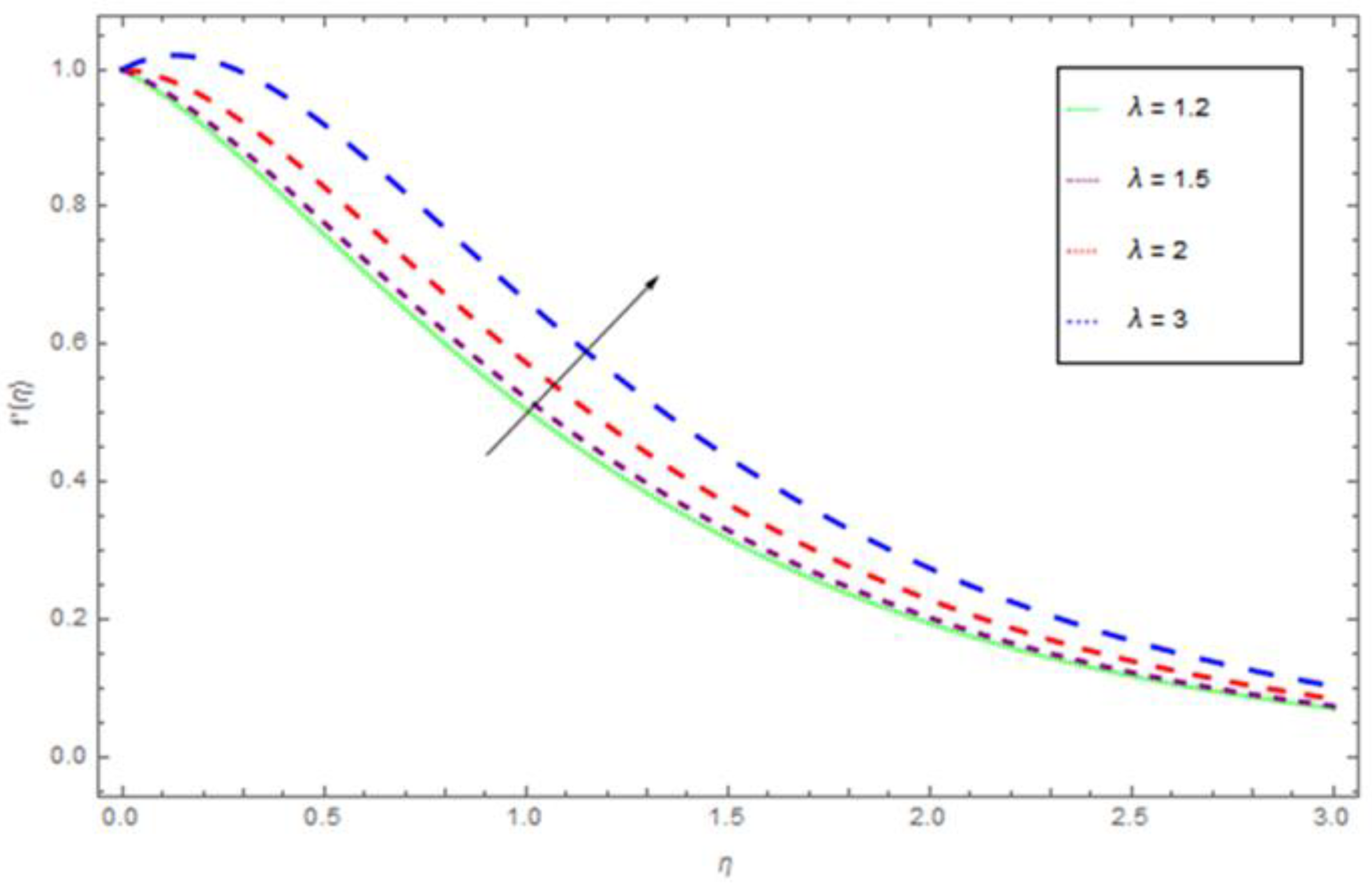
- The velocity profile decreases exponentially with the increasing of , while it rises with the rising values of the permeability, inertial, curvature, and convection parameters.
- The augmented value of shows stouter thermophoretic force due to the temperature gradient, which transfers the nanoparticles from the warm surface to the quiescent fluid. Thermophoresis force is generated by temperature gradient, which fashions a degenerate flow away from the surface.
- The temperature profile of the hybrid nanofluid increases almost in the same manner with the increasing values of , Reynold number and convection parameter. The variation is more prominent for the intermediate values of the independent variable η.
- The temperature profile decreases with the higher values of the Prandtl number. The drop in is more significant from η = 0.2 to η = 3.6. Furthermore, the temperature function drops at a maximum rate for the highest value of , i.e., .
- The increases with the higher values of from η = 0.3 to η = 2.6, while it reduces with the rising values of Brownian motion parameter from η = 0.4 to η = 3.2.
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
Nomenclature
| Components of Velocity | |
| Axial and radial Coordinates | |
| Strength of Magnetic field | |
| Characteristic radius | |
| Temperature | |
| Dimensional velocity | |
| Dimensional temperature | |
| Eckert number | |
| Hear source/sink | |
| Thermal diffusivity | |
| Specific heat | |
| Density | |
| Local inertial parameter | |
| Stretching velocity | |
| Stephan-Boltzmann constant | |
| Mean absorption coefficient | |
| Volume fraction of the nanoparticles | |
| similarity variable | |
| Prandtl Number | |
| Skin friction coefficient | |
| Nusselt number | |
| Local Reynolds number | |
| Coefficients of mean absorption | |
| Radiative heat flux | |
| Starching rate | |
| Thermal conductivity | |
| Schimid number | |
| Temperature difference | |
| Kinematic viscosity | |
| Shear stress | |
| Curvature parameter | |
| Magnetic parameter | |
| Thermal radiation parameter | |
| Dynamic viscosity | |
| Electrical conductivity | |
| Convection parameter | |
| Subscripts | |
| Base fluid | |
| Nanofluid | |
| Ambient | |
| Hybrid nanofluid | |
| Wall | |
| particles | |
References
- Davis, G.d.V. Natural convection of air in a square cavity, a bench mark numerical solution. Int. J. Numer. Methods Fluids 1983, 3, 249–264. [Google Scholar] [CrossRef]
- Fusegi, T.; Hyun, J.M.; Kuwahara, K.; Farouk, B. A numerical study of three dimensional natural convection in a differentially heated cubical enclosure. Int. J. Heat Mass Transf. 1991, 34, 1543–1557. [Google Scholar] [CrossRef]
- Barakos, G.; Mistoulis, E. Natural convection flow in a square cavity revisited: Laminar and turbulent models with wall functions. Int. J. Numer. Method Heat Fluid Flow 1994, 18, 695–719. [Google Scholar] [CrossRef]
- Skok, H.; Ramadhyani, S.; Schoenhals, R.J. Natural convection in a side-facing open cavity. Int. J. Heat Fluid Flow 1991, 12, 36–45. [Google Scholar] [CrossRef]
- Cha, S.S.; Choi, K.J. An interferometric investigation of open-cavity natural convection heat transfer. Exp. Heat Transf. 1989, 2, 27–40. [Google Scholar] [CrossRef]
- Sheikholeslami, M.; Shah, Z.; Shafee, A.; Khan, I.; Tlili, I. Uniform magnetic force impact on water based nanofluid thermal behavior in a porous enclosure with ellipse shaped obstacle. Sci. Rep. 2019, 9, 1196. [Google Scholar] [CrossRef] [Green Version]
- Chan, Y.L.; Tien, C.L. A numerical study of two-dimensional laminar natural convection in shallow open cavities. Int. J. Heat Mass Transf. 1985, 28, 603–612. [Google Scholar] [CrossRef]
- Choi, S.U.S.; Eastman, J.A. Enhancing thermal conductivity of fluids with nanoparticles. In Proceedings of the 1995 International Mechanical Engineering Congress and Exhibition, San Francisco, CA, USA, 12–17 November 1995. [Google Scholar]
- Mahmoudi, A.H.; Shahi, M.; Shahedin, A.M.; Hemati, N. Numerical mod-eling of natural convection in an open enclosure with two vertical thin heat sources subjected to a nanofluid. Int. Commun. Heat Mass Transf. 2011, 38, 110–118. [Google Scholar] [CrossRef]
- Sheremet, M.A.; Pop, A.S.I. Unsteady free convection in a porous open wavy cavity filled with a nanofluid using Buongiorno’s mathematical model. Int. Commun. Heat Mass Transf. 2015, 67, 66–72. [Google Scholar] [CrossRef]
- Tassaddiq, A.; Amin, I.; Shutaywi, M.; Shah, Z.; Ali, F.; Islam, S.; Ullah, A. Thin Film Flow of Couple Stress Magneto-Hydrodynamics Nanofluid with Convective Heat over an Inclined Exponentially Rotating Stretched Surface. Coatings 2020, 10, 338. [Google Scholar] [CrossRef] [Green Version]
- Tayebi, T.; Chamkha, A.J. Free convection enhancement in an annulus between horizontal confocal elliptical cylinders using hybrid nanofluids. Numer. Heat Transf. Part A 2016, 70, 1141–1156. [Google Scholar] [CrossRef]
- Abbas, T.; Bhatti, M.M.; Ayub, M. Aiding and opposing of mixed convection Casson nanofluid flow with chemical reactions through a porous Riga plate. Proc. Inst. Mech. Eng. Part E J. Process Mech. Eng. 2018, 232, 519–527. [Google Scholar] [CrossRef]
- Shah, Z.; Kumam, P.; Deebani, W. Radiative MHD Casson Nanofluid Flow with Activation energy and chemical reaction over past nonlinearly stretching surface through Entropy generation. Sci. Rep. 2020, 10, 4402. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Rashidi, S.; Akar, S.; Bovand, M.; Ellahi, R. Volume of fluid model to simulate the nanofluid flow and entropy generation in a single slope solar still. Renew. Energy 2018, 115, 400–410. [Google Scholar] [CrossRef]
- Akbarzadeh, M.; Rashidi, S.; Karimi, N.; Ellahi, R. Convection of heat and thermodynamic irreversibilities in two-phase, turbulent nanofluid flows in solar heaters by corrugated absorber plates. Adv. Powder Technol. 2018, 29, 2243–2254. [Google Scholar] [CrossRef] [Green Version]
- Shah, Z.; Alzahrani, E.O.; Alghamdi, W.; Ullah, M.Z. Influences of electrical MHD and Hall current on squeezing nanofluid flow inside rotating porous plates with viscous and joule dissipation effects. J. Therm. Anal. Calorim. 2020, 140, 1215–1227. [Google Scholar] [CrossRef]
- Takabi, B.; Shokouhmand, H. Effects of Al2O3–Cu/water hybrid nanofluid on heat transfer and flow characteristics in turbulent regime. Int. J. Mod. Phys. C 2015, 26, 1550047. [Google Scholar] [CrossRef]
- Shah, Z.; Babazadeh, H.; Kumam, P.; Shafee, A.; Thounthong, P. Numerical simulation of magnetohydrodynamic nanofluids under the influence of shape factor and thermal transport in a porous media using CVFEM. Front. Phys. 2019, 7, 164. [Google Scholar] [CrossRef] [Green Version]
- Minea, A.A. Hybrid nanofluids based on Al2O3, TiO2 and SiO2: Numerical evaluation of different approaches. Int. J. Heat Mass Transf. 2017, 104, 852–860. [Google Scholar] [CrossRef]
- Taylor, P.K.M.; Della, R.J.; Huxford, R.C.; Lin, W. Hybrid nanomaterials for biomedical applications. Chem. Commun. 2010, 46, 5832–5849. [Google Scholar] [CrossRef]
- Farahani, M.M.; Movassagh, J.; Taghavi, F.; Eghbali, P.; Salimi, F. Magnetite–polyoxometalate hybrid nanomaterials: Synthesis and characterization. Chem. Eng. J. 2012, 184, 342–346. [Google Scholar] [CrossRef]
- Mehryan, S.A.M.; Izadpanahi, E.; Ghalambaz, M.; Chamkha, A.J. Mixed convection flow caused by an oscillating cylinder in a square cavity filled with Cu-Al2O3/water hybrid nanofluid. J. Therm. Anal. Calorim. 2019. [Google Scholar] [CrossRef]
- Suresh, S.; Venkitaraj, K.P.; Selvakumar, P.; Chandrasekar, M. Synthesis of Al2O3–Cu/water hybrid nanofluids using two step method and its thermo physical properties. Colloids Surf. A 2011, 388, 41–48. [Google Scholar] [CrossRef]
- Suresh, S.; Venkitaraj, K.P.; Selvakumar, P.; Chandrasekar, M. Effect of Al2O3–Cu/water hybrid nanofluid in heat transfer. Exp. Fluid Sci. 2012, 38, 54–60. [Google Scholar] [CrossRef]
- Rokni, H.B.; Alsaad, D.M.; Valipour, P. Electro hydrodynamic nanofluid flow and heat transfer between two plates. J. Mol. Liq. 2016, 216, 583–589. [Google Scholar] [CrossRef]
- Shehzad, S.A.; Hayat, A.; Alsaedi, A. MHD flow of Jeffrey nanofluid with convective boundary conditions. Braz. Soc. Mech. Sci. Eng. 2014, 3, 873–883. [Google Scholar] [CrossRef]
- Mahmoodi, M.; Kandelousi, S.H. Kerosene∓alumina nanofluid flow and heat transfer for cooling application. J. Cent. South Univ. 2016, 23, 983–990. [Google Scholar] [CrossRef]
- Fakour, M.; Ganji, D.D.; Abbasi, M. Scrutiny of underdeveloped nanofluid MHD flow and heat conduction in a channel with porous walls. Int. J. Case Stud. Therm. Eng. 2014, 4, 202–214. [Google Scholar] [CrossRef] [Green Version]
- Fakour, M.; Rahbari, A.; Khodabandeh, E. Nanofluid thin film flow and heat transfer over an unsteady stretching elastic sheet by LMS. J. Mech. Sci. Technol. 2018, 32, 177–183. [Google Scholar] [CrossRef]
- Hatami, M.; Sheikholeslami, M.; Ganji, D.D. Laminar flow and heat transfer of nanofluid between contracting and rotating disks by least square method. Power Technol. 2014, 253, 769–779. [Google Scholar] [CrossRef]
- Nadeem, S.; Mehmood, R.; Akbar, N.S. Nonorthogonal stagnation point flow of a nano non-Newtonian fluid towards a stretching surface with heat transfer. Int. J. Heat Mass Transf. 2013, 57, 679–689. [Google Scholar] [CrossRef]
- Sheikholeslami, M.; Hatami, M.; Ganji, D.D. Analytical investigation of MHD nanofluid flow in a semi-porous channel. Powder Technol. 2013, 246, 327–336. [Google Scholar] [CrossRef]
- Akbar, N.S.; Tripathi, D.; Khan, Z.H.; Beg, O.A. A numerical study of magneto hydrodynamic transport of nanofluids over a vertical stretching sheet with exponential temperature-dependent viscosity and buoyancy effects. Chem. Phys. Lett. 2016, 661, 20–30. [Google Scholar] [CrossRef]
- Fakour, M.; Vahabzadeh, A.; Ganji, D.D. Scrutiny of mixed convection flow of a nanofluid in a vertical channel. Int. J. Case Stud. Therm. Eng. 2014, 4, 15–23. [Google Scholar] [CrossRef] [Green Version]
- Maskeen, M.M.; Zeeshan, A.; Mehmood, O.U.; Hassan, M. Heat transfer enhancement in hydromagnetic alumina–copper/water hybrid nanofluid flow over a stretching cylinder. J. Anal. Calorim. 2019, 138, 1127–1136. [Google Scholar] [CrossRef]
- Akilu, S.; Sharma, K.V.; Baheta, A.T.; Mamat, R. A review of thermophysical properties of water based composite nanofluids. Renew. Sustain. Energy Rev. 2016, 66, 654–678. [Google Scholar] [CrossRef] [Green Version]
- Hayat, T.; Khan, M.I.; Waqas, M.; Alsaedi, A. Newtonian heating effect in nanofluid flow by a permeable cylinder. Result Phys. 2017, 7, 256–262. [Google Scholar] [CrossRef] [Green Version]
- Jamaludin, A.; Naganthran, K.; Nazar, R.; Pop, I. Thermal radiation and MHD effects in the mixed convection flow of Fe3O4–water ferrofluid towards a nonlinearly moving surface. Processes 2020, 8, 95. [Google Scholar] [CrossRef] [Green Version]
- Zaib, A.; Khan, U.; Khan, I.; Seikh, A.H.; Sherif, E.-S.M. Entropy generation and dual solutions in mixed convection stagnation point flow of micropolar Ti6Al4V nanoparticle along a riga surface. Processes 2020, 8, 14. [Google Scholar] [CrossRef] [Green Version]
- Saeed, A.; Islam, S.; Dawar, A.; Shah, Z.; Kumam, P.; Khan, W. Influence of Cattaneo–Christov heat flux on MHD Jeffrey, Maxwell, and Oldroyd-B nanofluids with homogeneous-heterogeneous reaction. Symmetry 2019, 11, 439. [Google Scholar] [CrossRef] [Green Version]
- Ahmad Farooq, A.; Shah, Z.; Alzahrani, E.O. Heat transfer analysis of a magneto-bio-fluid transport with variable thermal viscosity through a vertical ciliated channel. Symmetry 2019, 11, 1240. [Google Scholar] [CrossRef] [Green Version]
- Jena, P.K.; Brocchi, E.A.; Motta, M.S. In-situ formation of Cu–Al2O3 nano-scale composites by chemical routes and studies on their microstructures. Mater. Sci. Eng. A 2001, 313, 180–186. [Google Scholar] [CrossRef]
- Niihara, K. New design concept of structural ceramics–ceramic nanocomposites. J. Ceram. Soc. Jpn. 1991, 99, 974–982. [Google Scholar] [CrossRef] [Green Version]
- Oh, S.-T.; Sekino, T.; Niihara, K. Effect of particle size distribution and mixing homogeneity on microstructure and strength of alumina/copper composites. Nanostruct. Mater. 1998, 10, 327–332. [Google Scholar] [CrossRef]
- Oh, S.-T.; Sekino, T.; Niihara, K. Fabrication and mechanical properties of 5 vol% copper dispersed alumina nanocomposite. J. Eur. Ceram. Soc. 1998, 18, 31–37. [Google Scholar] [CrossRef]
- Forchheimer, P. Wasserbewegung Durch Boden; Zeitschrift des Vereins Deutscher Ingenieure: Düsseldorf, Germany, 1901; Volume 45, pp. 1782–1788. [Google Scholar]
- Muskat, M. The Flow of Homogeneous Fluids through Porous Media; JW Edwards: Edwards, MI, USA, 1946. [Google Scholar]
- Pal, D.; Mondal, H. Hydromagnetic convective diffusion of species in Darcy-Forchheimer porous medium with non-uniform heat source/sink and variable viscosity. Int. Commun. Heat Mass Transf. 2012, 39, 913–917. [Google Scholar] [CrossRef]
- Ganesh, N.V.; Hakeem, A.K.A.; Ganga, B. Darcy-Forchheimer flow of hydromagnetic nanofluid over a stretching/shrinking sheet in a thermally stratified porous medium with second order slip, viscous and Ohmic dissipations effects. Ain Shams Eng. J. 2016, 9, 939–951. [Google Scholar] [CrossRef] [Green Version]
- Hayat, T.; Muhammad, T.; Al-Mezal, S.; Liao, S.J. Darcy-Forchheimer flow with variable thermal conductivity and Cattaneo-Christov heat flux. Int. J. Numer. Methods Heat Fluid Flow 2016, 26, 2355–2369. [Google Scholar] [CrossRef]
- Muhammad, T.; Alsaedi, A.; Shahzad, S.A.; Hayat, T. A revised model for Darcy-Forchheimer flow of Maxwell nanofluid subject to convective boundary condition. Chin. J. Phys. 2017, 55, 963–976. [Google Scholar] [CrossRef]
- Jawad, M.; Shah, Z.; Islam, S.; Bonyah, E.; Khan, A.Z. Darcy-Forchheimer Flow of MHD Nanofluid thin Film Flow with Joule dissipation and Navier’s partial slip. J. Phys. Commun. 2018, 2, 115014. [Google Scholar] [CrossRef]
- Uddin, I.; Akhtar, R.; Zhiyu, Z.; Islam, S.; Shoaib, M.; Raja, M.A.Z. Numerical treatment for Darcy-Forchheimer flow of Sisko nanomaterial with nonlinear thermal radiation by lobatto IIIA technique. Math. Probl. Eng. 2019, 2019. [Google Scholar] [CrossRef]
- Mohamed, R.E.; Mahny, K.L.; Dar, A.; Muhammad, T. Numerical study for Carreau nanofluid flow over a convectively heated nonlinear stretching surface with chemically reactive species. Physica A 2020, 540, 123063. [Google Scholar]
- Lahmar, S.; Kezzar, M.; Eid, M.R.; Sari, M.R. Heat transfer of squeezing unsteady nanofluid flow under the effects of an inclined magnetic field and variable thermal conductivity. Physica A 2020, 540, 123138. [Google Scholar] [CrossRef]
- Eid, M.R.; Al-Hossainy, A.F.; Zoromba, M.S. FEM for blood-based SWCNTs flow through a circular cylinder in a porous medium with electromagnetic radiation. Commun. Theor. Phys. 2019, 71, 1425–1434. [Google Scholar] [CrossRef]
- Al-Hossainy, A.F.; Eid, M.R.; Zoromba, M.S. SQLM for external yield stress effect on 3D MHD nanofluid flow in a porous medium. Phys. Scr. 2019, 94, 105208. [Google Scholar] [CrossRef]
- Mohamed, R.E. Chemical reaction effect on MHD boundary-layer flow of two-phase nanofluid model over an exponentially stretching sheet with a heat generation. J. Mol. Liq. 2016, 220, 718–725. [Google Scholar]
- Mohamed, R.E. Time-dependent flow of water-NPs over a stretching sheet in a saturated porous medium in the stagnation-point region in the presence of chemical reaction. J. Nanofluids 2017, 6, 550–557. [Google Scholar]
- Sheikholeslami, M. New computational approach for exergy and entropy analysis of nanofluid under the impact of Lorentz force through a porous media. Comput. Methods Appl. Mech. Eng. 2019, 344, 319–333. [Google Scholar] [CrossRef]
- Awais, M.; Shah, Z.; Parveen, N.; Ali, A.; Kumam, P.; Rehman, H.; Thounthong, P. MHD effects on ciliary-induced peristaltic flow coatings with rheological hybrid nanofluid. Coatings 2020, 10, 186. [Google Scholar] [CrossRef] [Green Version]
- Alsagri, A.S.; Nasir, S.; Gul, T.; Islam, S.; Nisar, K.; Shah, Z.; Khan, I. MHD thin film flow and thermal analysis of blood with CNTs nanofluid. Coatings 2019, 9, 175. [Google Scholar] [CrossRef] [Green Version]






| Present Results | Results [36] | Present Results | Results [36] | Present Results | Results [36] | ||
|---|---|---|---|---|---|---|---|
| 0.0 | 0.5 | 0.8987 | 0.8976 | 1.0227 | 0.9557 | 1.1439 | 1.0110 |
| 0.2 | - | 0.9052 | 0.9756 | 1.0292 | 1.0346 | 1.15049 | 1.0908 |
| 0.3 | - | 0.9085 | 1.0157 | 1.0325 | 1.0748 | 1.1537 | 1.1311 |
| 0.4 | - | 0.9118 | 1.0562 | 1.0358 | 1.1152 | 1.1570 | 1.1716 |
| 0.2 | 0.0 | 1.0222 | 1.0296 | 1.1450 | 1.0897 | 1.2650 | 1.1468 |
| 0.5 | 0.9052 | 0.9756 | 1.0292 | 1.0346 | 1.1504 | 1.0908 | |
| 0.8 | 0.8350 | 0.9441 | 0.9597 | 1.0023 | 1.0817 | 1.0580 | |
| 1.0 | 0.7862 | 0.9233 | 0.9134 | 0.9811 | 1.0359 | 1.0364 |
| Results [36] | Results [36] | Results [36] | ||||||
|---|---|---|---|---|---|---|---|---|
| 0.7 | 0.5 | 0.5 | 0.1630 | 0.1653 | 0.1636 | 0.0897 | 0.1642 | 0.0198 |
| 0. 9 | - | - | 0.1582 | 0.2296 | 0.1584 | 0.1609 | 0.1586 | 0.0964 |
| 1.2 | - | - | 0.1512 | 0.2703 | 0.1508 | 0.2060 | 0.1505 | 0.1448 |
| 1.5 | - | - | 0.1444 | 0.3287 | 0.1435 | 0.2704 | 0.4227 | 0.2139 |
| 0.7 | 0.1 | 0.5 | 0.1751 | 0.1312 | 0.1764 | 0.0592 | 0.1776 | 0.0225 |
| 0.5 | - | 0. 1630 | 0.2086 | 0.1636 | 0.1377 | 0.1642 | 0.1036 | |
| 0.6 | - | 0.1600 | 0.2387 | 0.1604 | 0.1692 | 0.1609 | 0.1372 | |
| 0.7 | - | 0.1570 | 0.2544 | 0.1573 | 0.1861 | 0.1577 | 0.1555 | |
| 0.7 | 0.5 | 0.0 | 0.1769 | 1.9523 | 0.1782 | 1.9867 | 0.1795 | 2.0244 |
| - | 0.5 | 0. 1630 | 1.5141 | 0.1636 | 1.5219 | 0.1642 | 1.5334 | |
| - | 0.6 | 0.1603 | 1.0774 | 0.1608 | 1.0588 | 0.1613 | 1.0442 | |
| - | 0.7 | 0.15767 | 0.6423 | 0.15804 | 0.5974 | 0.15844 | 0.5569 |
| Water, Alumina and Copper Particles | Physical Property | ||||
|---|---|---|---|---|---|
| Alumina (Al2O3) | 0.85 | 765 | 3970 | 40 | - |
| Copper (Cu) | 1.65 | 385 | 8933 | 400 | - |
| Water (H2O) | 21 | 4179 | 997.1 | 0.613 | 0.894 |
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Saeed, A.; Tassaddiq, A.; Khan, A.; Jawad, M.; Deebani, W.; Shah, Z.; Islam, S. Darcy-Forchheimer MHD Hybrid Nanofluid Flow and Heat Transfer Analysis over a Porous Stretching Cylinder. Coatings 2020, 10, 391. https://doi.org/10.3390/coatings10040391
Saeed A, Tassaddiq A, Khan A, Jawad M, Deebani W, Shah Z, Islam S. Darcy-Forchheimer MHD Hybrid Nanofluid Flow and Heat Transfer Analysis over a Porous Stretching Cylinder. Coatings. 2020; 10(4):391. https://doi.org/10.3390/coatings10040391
Chicago/Turabian StyleSaeed, Anwar, Asifa Tassaddiq, Arshad Khan, Muhammad Jawad, Wejdan Deebani, Zahir Shah, and Saeed Islam. 2020. "Darcy-Forchheimer MHD Hybrid Nanofluid Flow and Heat Transfer Analysis over a Porous Stretching Cylinder" Coatings 10, no. 4: 391. https://doi.org/10.3390/coatings10040391
APA StyleSaeed, A., Tassaddiq, A., Khan, A., Jawad, M., Deebani, W., Shah, Z., & Islam, S. (2020). Darcy-Forchheimer MHD Hybrid Nanofluid Flow and Heat Transfer Analysis over a Porous Stretching Cylinder. Coatings, 10(4), 391. https://doi.org/10.3390/coatings10040391





